Brightness Perception in World-Centered Coordinates Assessed by Pupillometry
Abstract
1. Introduction
2. Materials and Methods
2.1. Participants
2.2. Stimuli and Apparatus
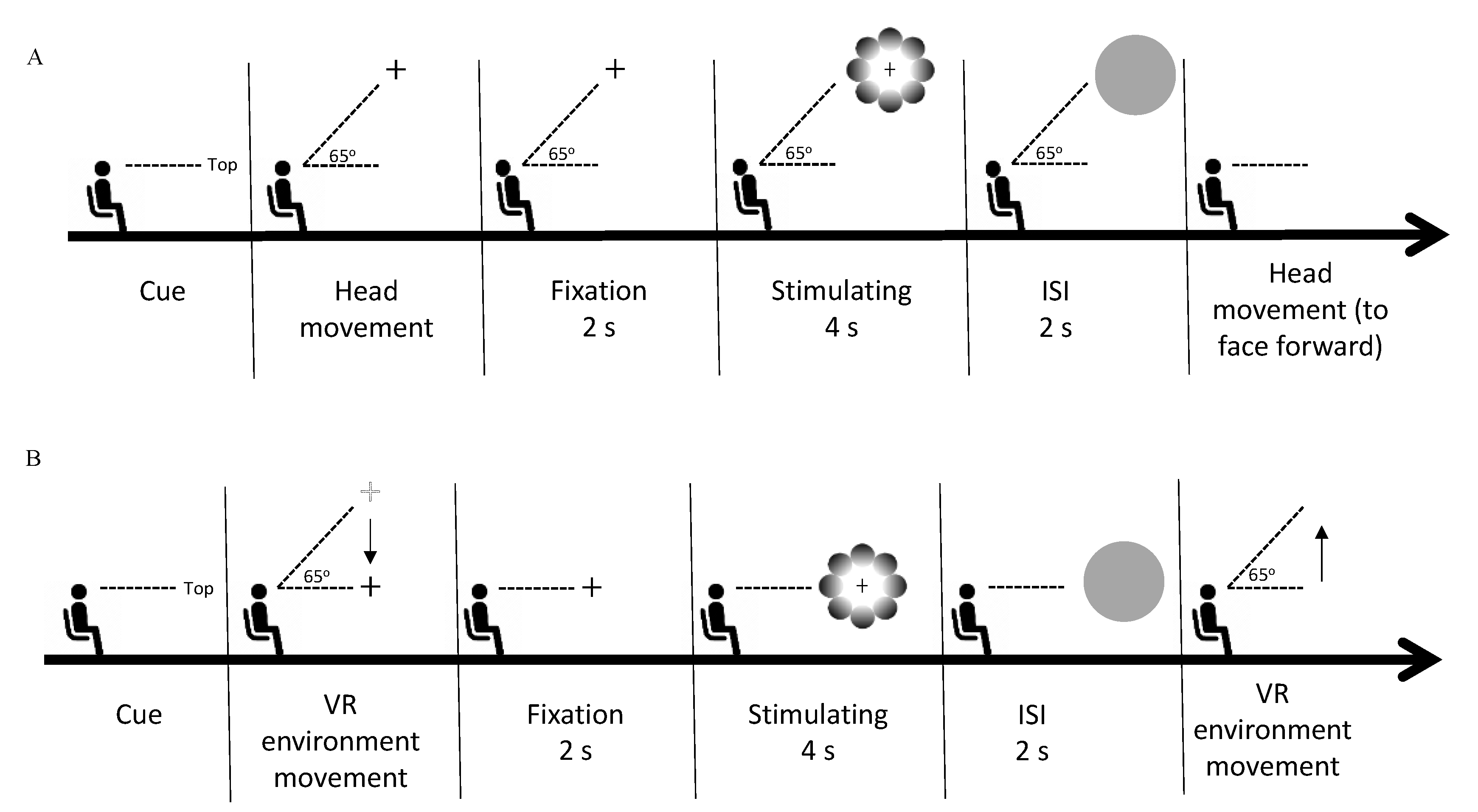
2.3. Procedure
2.4. Pupil and Eye Gaze Analyses
2.5. Statistical Analyses
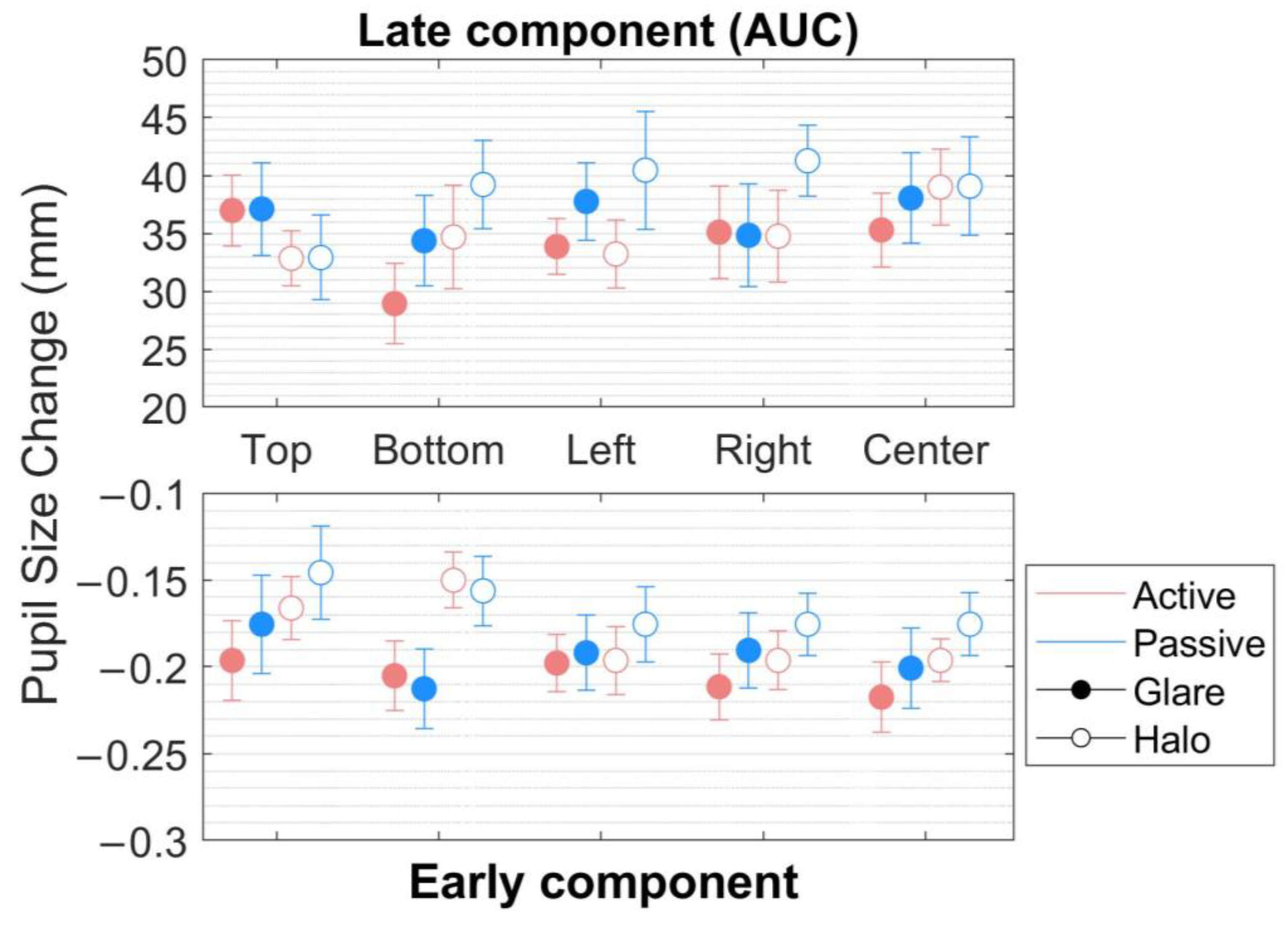
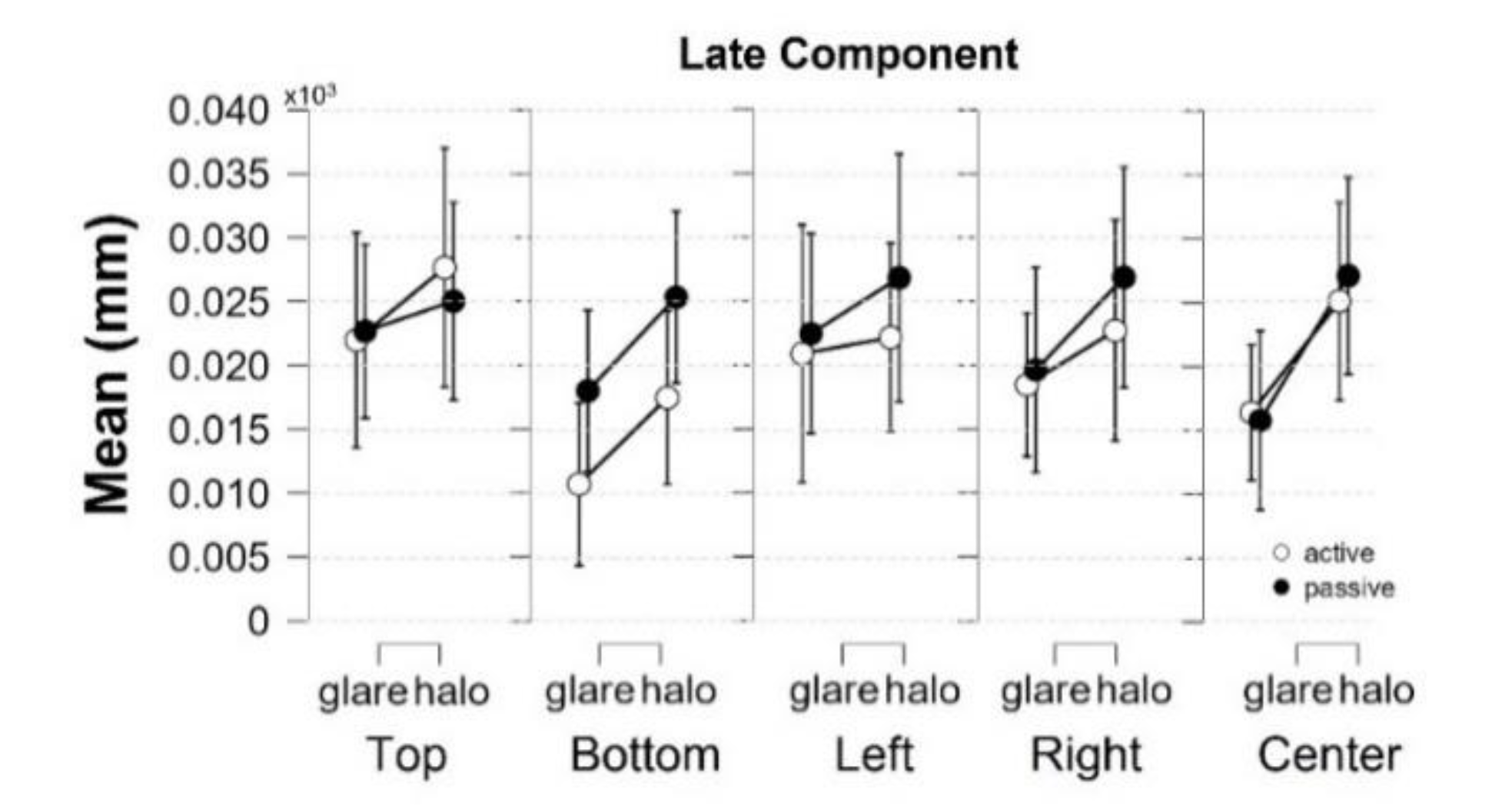
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Andersen, R.A.; Snyder, L.H.; Li, C.-S.; Stricanne, B. Coordinate transformations in the representation of spatial information. Curr. Opin. Neurobiol. 1993, 3, 171–176. [Google Scholar] [CrossRef] [PubMed]
- Qian, K.; Mitsudo, H. Spatial dynamics of the eggs illusion: Visual field anisotropy and peripheral vision. Vision Res. 2020, 177, 12–19. [Google Scholar] [CrossRef]
- Sakaguchi, Y. Visual field anisotropy revealed by perceptual filling-in. Vision Res. 2003, 43, 2029–2038. [Google Scholar] [CrossRef]
- Bertulis, A.; Bulatov, A. Distortions in length perception: Visual field anisotropy and geometrical illusions. Neurosci. Behav. Physiol. 2005, 35, 423–434. [Google Scholar] [CrossRef] [PubMed]
- Wada, Y.; Saijo, M.; Kato, T. Visual field anisotropy for perceiving shape from shading and shape from edges. Interdiscip. Inf. Sci. 1998, 4, 157–164. [Google Scholar] [CrossRef]
- Schwartz, S.; Kirsner, K. Laterality effects in visual information processing: Hemispheric specialisation or the orienting of attention? Q. J. Exp. Psychol. Sect. A 1982, 34, 61–77. [Google Scholar] [CrossRef]
- Portengen, B.L.; Roelofzen, C.; Porro, G.L.; Imhof, S.M.; Fracasso, A.; Naber, M. Blind spot and visual field anisotropy detection with flicker pupil perimetry across brightness and task variations. Vision Res. 2021, 178, 79–85. [Google Scholar] [CrossRef]
- Hong, S.; Narkiewicz, J.; Kardon, R.H. Comparison of Pupil Perimetry and Visual Perimetry in Normal Eyes: Decibel Sensitivity and Variability. Invest. Ophthalmol. Vis. Sci. 2001, 42, 957–965. [Google Scholar]
- Sabeti, F.; James, A.C.; Maddess, T. Spatial and temporal stimulus variants for multifocal pupillography of the central visual field. Vision Res. 2011, 51, 303–310. [Google Scholar] [CrossRef]
- Carrasco, M.; Talgar, C.P.; Cameron, E.L. Characterizing visual performance fields: Effects of transient covert attention, spatial frequency, eccentricity, task and set size. Spat. Vis. 2001, 15, 61–75. [Google Scholar] [CrossRef]
- Talgar, C.P.; Carrasco, M. Vertical meridian asymmetry in spatial resolution: Visual and attentional factors. Psychon. Bull. Rev. 2002, 9, 714–722. [Google Scholar] [CrossRef] [PubMed]
- Levine, M.W.; McAnany, J.J. The relative capabilities of the upper and lower visual hemifields. Vision Res. 2005, 45, 2820–2830. [Google Scholar] [CrossRef] [PubMed]
- Amenedo, E.; Pazo-Alvarez, P.; Cadaveira, F. Vertical asymmetries in pre-attentive detection of changes in motion direction. Int. J. Psychophysiol. 2007, 64, 184–189. [Google Scholar] [CrossRef] [PubMed]
- Intriligator, J.; Cavanagh, P. The Spatial Resolution of Visual Attention. Cogn. Psychol. 2001, 43, 171–216. [Google Scholar] [CrossRef]
- Curcio, C.A.; Allen, K.A. Topography of ganglion cells in human retina. J. Comp. Neurol. 1990, 300, 5–25. [Google Scholar] [CrossRef]
- Binda, P.; Pereverzeva, M.; Murray, S.O. Pupil constrictions to photographs of the sun. J. Vis. 2013, 13, 8. [Google Scholar] [CrossRef] [PubMed]
- Castellotti, S.; Conti, M.; Feitosa-Santana, C.; del Viva, M.M. Pupillary response to representations of light in paintings. J. Vis. 2020, 20, 14. [Google Scholar] [CrossRef] [PubMed]
- Istiqomah, N.; Takeshita, T.; Kinzuka, Y.; Minami, T.; Nakauchi, S. The Effect of Ambiguous Image on Pupil Response of Sun and Moon Perception. In Proceedings of the 8th International Symposium on Affective Science and Engineering, Online Academic Symposium, 27 March 2022; pp. 1–4. [Google Scholar] [CrossRef]
- Tortelli, C.; Pomè, A.; Turi, M.; Igliozzi, R.; Burr, D.C.; Binda, P. Contextual Information Modulates Pupil Size in Autistic Children. Front. Neurosci. 2022, 16, 1–10. [Google Scholar] [CrossRef]
- Laeng, B.; Endestad, T. Bright illusions reduce the eye’s pupil. Proc. Natl. Acad. Sci. USA 2012, 109, 2162–2167. [Google Scholar] [CrossRef] [PubMed]
- Mamassian, P.; de Montalembert, M. A simple model of the vertical-horizontal illusion. Vision Res. 2010, 50, 956–962. [Google Scholar] [CrossRef]
- Suzuki, Y.; Minami, T.; Laeng, B.; Nakauchi, S. Colorful glares: Effects of colors on brightness illusions measured with pupillometry. Acta Psychol. (Amst) 2019, 198, 102882. [Google Scholar] [CrossRef]
- Laeng, B.; Sulutvedt, U. The Eye Pupil Adjusts to Imaginary Light. Psychol. Sci. 2014, 25, 188–197. [Google Scholar] [CrossRef] [PubMed]
- Mathôt, S.; Grainger, J.; Strijkers, K. Pupillary Responses to Words That Convey a Sense of Brightness or Darkness. Psychol. Sci. 2017, 28, 1116–1124. [Google Scholar] [CrossRef] [PubMed]
- Istiqomah, N.; Suzuki, Y.; Kinzuka, Y.; Minami, T.; Nakauchi, S. Anisotropy in the peripheral visual field based on pupil response to the glare illusion. Heliyon 2022, 8, e09772. [Google Scholar] [CrossRef]
- Chun, M.M. Contextual cueing of visual attention. Trends Cogn. Sci. 2000, 4, 170–178. [Google Scholar] [CrossRef] [PubMed]
- Jackson, I.; Sirois, S. Infant cognition: Going full factorial with pupil dilation. Dev. Sci. 2009, 12, 670–679. [Google Scholar] [CrossRef] [PubMed]
- Kinner, V.L.; Kuchinke, L.; Dierolf, A.M.; Merz, C.J.; Otto, T.; Wolf, O.T. What our eyes tell us about feelings: Tracking pupillary responses during emotion regulation processes. Psychophysiology 2017, 54, 508–518. [Google Scholar] [CrossRef]
- Bradley, M.M.; Miccoli, L.; Escrig, M.A.; Lang, P.J. The pupil as a measure of emotional arousal and autonomic activation. Psychophysiology 2008, 45, 602–607. [Google Scholar] [CrossRef]
- Cohen, J. Statistical Power Analysis for the Behavioral Sciences, 2nd ed.; Routledge: New York, NY, USA, 1988. [Google Scholar]
- van den Bergh, D.; Wagenmakers, E.-J.; Aust, F. Bayesian Repeated-Measures ANOVA: An Updated Methodology Implemented in JASP. PsyArXiv 2022. [Google Scholar] [CrossRef]
- Jeffreys, H. The Theory of Probability, 3rd ed.; OUP: Oxford, UK, 1961; ISBN 9780198503682. [Google Scholar]
- Wilhelm, H.; Neitzel, J.; Wilhelm, B.; Beuel, S.; Lüdtke, H.; Kretschmann, U.; Zrenner, E. Pupil perimetry using M-sequence stimulation technique. Invest. Ophthalmol. Vis. Sci. 2000, 41, 1229–1238. [Google Scholar]
- Mathôt, S. Pupillometry: Psychology, physiology, and function. J. Cogn. 2018, 1, 1–23. [Google Scholar] [CrossRef] [PubMed]
- Mathôt, S.; van der Linden, L.; Grainger, J.; Vitu, F. The pupillary light response reveals the focus of covert visual attention. PLoS One 2013, 8, e78168. [Google Scholar] [CrossRef] [PubMed]
- Keysers, C.; Gazzola, V.; Wagenmakers, E.J. Using Bayes factor hypothesis testing in neuroscience to establish evidence of absence. Nat. Neurosci. 2020, 23, 788–799. [Google Scholar] [CrossRef]
- Previc, F.H. The neuropsychology of 3-D space. Psychol. Bull. 1998, 124, 123–164. [Google Scholar] [CrossRef] [PubMed]
- Lee, J.; Cheon, M.; Moon, S.E.; Lee, J.S. Peripersonal space in virtual reality: Navigating 3D space with different perspectives. In Proceedings of the UIST 2016 Adjunct—29th Annual Symposium on User Interface Software and Technology, Tokyo, Japan, 16–19 October 2016; pp. 207–208. [Google Scholar] [CrossRef]


| Effects | df | F | p | η2p |
|---|---|---|---|---|
| scene | 1.000 | 0.034 | 0.855 | 0.002 |
| pattern | 1.000 | 58.899 | <0.001 | 0.776 |
| location | 2.430 | 2.103 | 0.126 | 0.110 |
| scene × pattern | 1.000 | 1.368 | 0.258 | 0.074 |
| scene × location | 2.931 | 2.003 | 0.127 | 0.105 |
| pattern × location | 2.677 | 0.810 | 0.483 | 0.045 |
| scene × pattern × location | 3.064 | 0.483 | 0.700 | 0.028 |
| Models | P(M) | P(M|Data) | BFM | BF10 | Error % |
|---|---|---|---|---|---|
| pattern | 0.053 | 0.834 | 90.205 | 1.000 | |
| Scene + pattern | 0.053 | 0.121 | 2.483 | 0.145 | 2.028 |
| Scene + pattern + Scene × pattern | 0.053 | 0.027 | 0.493 | 0.032 | 2.595 |
| pattern + location | 0.053 | 0.015 | 0.279 | 0.018 | 1.753 |
| Scene + pattern + location | 0.053 | 0.002 | 0.041 | 0.003 | 1.875 |
| Scene + pattern + location + Scene × pattern | 0.053 | 4.728 × 10−4 | 0.009 | 5.672 × 10−4 | 2.286 |
| pattern + location + pattern × location | 0.053 | 3.294 × 10−4 | 0.006 | 3.951 × 10−4 | 2.715 |
| Scene + pattern + location + Scene × location | 0.053 | 7.812 × 10−5 | 0.001 | 9.371 × 10−5 | 2.651 |
| Scene + pattern + location + pattern × location | 0.053 | 4.981 × 10−5 | 8.966 × 10−4 | 5.975 × 10−5 | 4.499 |
| Scene + pattern + location + Scene × pattern + Scene × location | 0.053 | 1.853 × 10−5 | 3.335 × 10−4 | 2.223 × 10−5 | 9.561 |
| Scene + pattern + location + Scene × pattern + pattern × location | 0.053 | 1.152 × 10−5 | 2.074 × 10−4 | 1.382 × 10−5 | 8.862 |
| Scene + pattern + location + Scene × location + pattern × location | 0.053 | 1.628 × 10−6 | 2.930 × 10−5 | 1.953 × 10−6 | 2.833 |
| Scene + pattern + location + Scene × pattern + Scene × location + pattern × location | 0.053 | 3.526 × 10−7 | 6.346 × 10−6 | 4.229 × 10−7 | 3.111 |
| Scene + pattern + location + Scene × pattern + Scene × location + pattern × location + Scene × pattern × location | 0.053 | 1.671 × 10−8 | 3.008 × 10−7 | 2.004 × 10−8 | 3.190 |
| Null model (incl. subject) | 0.053 | 1.534 × 10−8 | 2.761 × 10−7 | 1.840 × 10−8 | 1.172 |
| Scene | 0.053 | 2.205 × 10−9 | 3.969 × 10−8 | 2.645 × 10−9 | 2.138 |
| location | 0.053 | 2.526 × 10−10 | 4.547 × 10−9 | 3.030 × 10−10 | 1.397 |
| Scene + location | 0.053 | 3.690 × 10−11 | 6.643 × 10−10 | 4.427 × 10−11 | 1.888 |
| Scene + location + Scene × location | 0.053 | 1.153 × 10−12 | 2.076 × 10−11 | 1.384 × 10−12 | 2.079 |
| df | F | p | η2p | |
|---|---|---|---|---|
| scene | 1.000 | 0.268 | 0.612 | 0.016 |
| pattern | 1.000 | 12.437 | 0.003 | 0.423 |
| location | 2.944 | 3.469 | 0.023 | 0.169 |
| scene × pattern | 1.000 | 0.194 | 0.665 | 0.011 |
| scene × location | 2.509 | 1.183 | 0.323 | 0.065 |
| pattern × location | 2.370 | 1.551 | 0.222 | 0.084 |
| scene × pattern × location | 3,476 | 0.381 | 0.795 | 0.022 |
| Models | P(M) | P(M|Data) | BFM | BF10 | Error % |
|---|---|---|---|---|---|
| pattern | 0.053 | 0.591 | 26.005 | 1.000 | |
| Scene + pattern | 0.053 | 0.243 | 5.767 | 0.411 | 3.596 |
| pattern + location | 0.053 | 0.072 | 1.390 | 0.121 | 2.012 |
| Scene + pattern + Scene × pattern | 0.053 | 0.039 | 0.729 | 0.066 | 2.610 |
| Scene + pattern + location | 0.053 | 0.029 | 0.534 | 0.049 | 2.257 |
| Null model (incl. subject) | 0.053 | 0.010 | 0.174 | 0.016 | 1.546 |
| Scene + pattern + location + Scene × pattern | 0.053 | 0.005 | 0.093 | 0.009 | 3.534 |
| Scene | 0.053 | 0.004 | 0.065 | 0.006 | 1.794 |
| pattern + location + pattern × location | 0.053 | 0.003 | 0.053 | 0.005 | 2.218 |
| Scene + pattern + location + Scene × location | 0.053 | 0.002 | 0.043 | 0.004 | 30.011 |
| Scene + pattern + location + pattern × location | 0.053 | 0.001 | 0.022 | 0.002 | 2.722 |
| location | 0.053 | 0.001 | 0.019 | 0.002 | 1.607 |
| Scene + location | 0.053 | 4.200 × 10−4 | 0.008 | 7.107 × 10−4 | 2.787 |
| Scene + pattern + location + Scene × pattern + Scene × location | 0.053 | 3.108 × 10−4 | 0.006 | 5.260 × 10−4 | 6.270 |
| Scene + pattern + location + Scene × pattern + pattern × location | 0.053 | 2.234 × 10−4 | 0.004 | 3.781 × 10−4 | 6.914 |
| Scene + pattern + location + Scene × location + pattern × location | 0.053 | 6.962 × 10−5 | 0.001 | 1.178 × 10−4 | 3.157 |
| Scene + location + Scene × location | 0.053 | 2.189 × 10−5 | 3.940 × 10−4 | 3.704 × 10−5 | 1.888 |
| Scene + pattern + location + Scene × pattern + Scene × location + pattern × location | 0.053 | 1.308 × 10−5 | 2.354 × 10−4 | 2.213 × 10−5 | 9.265 |
| Scene + pattern + location + Scene × pattern + Scene × location + pattern × location + Scene × pattern × location | 0.053 | 6.316 × 10−7 | 1.137 × 10−5 | 1.069 × 10−6 | 3.348 |
| df | F | p | η2p | |
|---|---|---|---|---|
| scene | 1.000 | 0.157 | 0.697 | 0.009 |
| pattern | 1.000 | 0.480 | 0.498 | 0.027 |
| location | 2.705 | 2.749 | 0.059 | 0.139 |
| scene × pattern | 1.000 | 0.406 | 0.533 | 0.023 |
| scene × location | 2.854 | 2.088 | 0.117 | 0.109 |
| pattern × location | 3.087 | 0.842 | 0.480 | 0.047 |
| scene × pattern × location | 3.106 | 0.369 | 0.783 | 0.021 |
| Model Comparison | |||||
|---|---|---|---|---|---|
| Models | P(M) | P(M|Data) | BFM | BF10 | Error % |
| Null model (incl. subject and random slopes) | 0.053 | 0.566 | 23.507 | 1.000 | |
| pattern | 0.053 | 0.276 | 6.845 | 0.486 | 8.861 |
| location | 0.053 | 0.075 | 1.467 | 0.133 | 3.666 |
| pattern + location | 0.053 | 0.032 | 0.593 | 0.056 | 2.686 |
| Scene | 0.053 | 0.026 | 0.489 | 0.047 | 98.912 |
| Scene + location + Scene × location | 0.053 | 0.016 | 0.293 | 0.028 | 93.708 |
| Scene + pattern + location + Scene × location | 0.053 | 0.003 | 0.056 | 0.005 | 68.789 |
| pattern + location + pattern × location | 0.053 | 0.002 | 0.037 | 0.004 | 3.033 |
| Scene + pattern + location | 0.053 | 0.002 | 0.031 | 0.003 | 99.897 |
| Scene + pattern + location + Scene × pattern + Scene × location | 0.053 | 6.867 × 10−4 | 0.012 | 0.001 | 76.290 |
| Scene + pattern + Scene × pattern | 0.053 | 5.223 × 10−4 | 0.009 | 9.222 × 10−4 | 70.330 |
| Scene + pattern + location + Scene × location + pattern × location | 0.053 | 2.177 × 10−4 | 0.004 | 3.845 × 10−4 | 87.934 |
| Scene + pattern | 0.053 | 1.094 × 10−4 | 0.002 | 1.931 × 10−4 | 54.002 |
| Scene + pattern + location + pattern × location | 0.053 | 7.478 × 10−6 | 1.346 × 10−4 | 1.320 × 10−5 | 92.223 |
| Scene + location | 0.053 | 5.183 × 10−6 | 9.330 × 10−5 | 9.152 × 10−6 | 41.615 |
| Scene + pattern + location + Scene × pattern + Scene × location + pattern × location | 0.053 | 3.021 × 10−6 | 5.438 × 10−5 | 5.335 × 10−6 | 71.873 |
| Scene + pattern + location + Scene × pattern | 0.053 | 1.987 × 10−6 | 3.576 × 10−5 | 3.508 × 10−6 | 57.978 |
| Scene + pattern + location + Scene × pattern + Scene × location + pattern × location + Scene × pattern × location | 0.053 | 1.462 × 10−7 | 2.632 × 10−6 | 2.581 × 10−7 | 97.918 |
| Scene + pattern + location + Scene × pattern + pattern × location | 0.053 | 1.983 × 10−8 | 3.569 × 10−7 | 3.501 × 10−8 | 49.837 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Istiqomah, N.; Kinzuka, Y.; Minami, T.; Nakauchi, S. Brightness Perception in World-Centered Coordinates Assessed by Pupillometry. Behav. Sci. 2023, 13, 60. https://doi.org/10.3390/bs13010060
Istiqomah N, Kinzuka Y, Minami T, Nakauchi S. Brightness Perception in World-Centered Coordinates Assessed by Pupillometry. Behavioral Sciences. 2023; 13(1):60. https://doi.org/10.3390/bs13010060
Chicago/Turabian StyleIstiqomah, Novera, Yuya Kinzuka, Tetsuto Minami, and Shigeki Nakauchi. 2023. "Brightness Perception in World-Centered Coordinates Assessed by Pupillometry" Behavioral Sciences 13, no. 1: 60. https://doi.org/10.3390/bs13010060
APA StyleIstiqomah, N., Kinzuka, Y., Minami, T., & Nakauchi, S. (2023). Brightness Perception in World-Centered Coordinates Assessed by Pupillometry. Behavioral Sciences, 13(1), 60. https://doi.org/10.3390/bs13010060





