Use of Paleoflood Deposits to Determine the Contribution of Anthropogenic Trace Metals to Alluvial Sediments in the Hyperarid Rio Loa Basin, Chile
Abstract
:1. Introduction
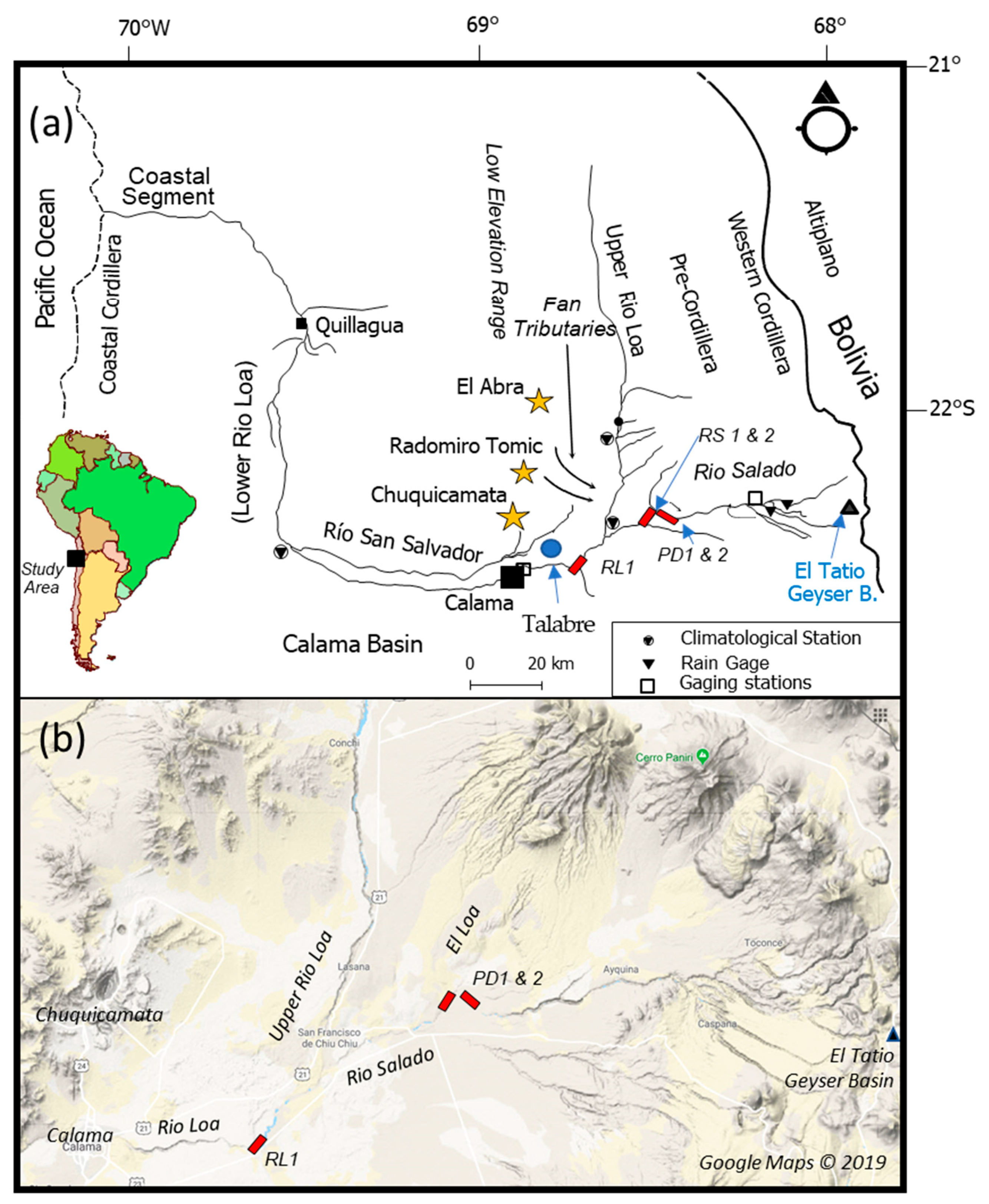
2. Geographic and Climatic Setting
3. Methods
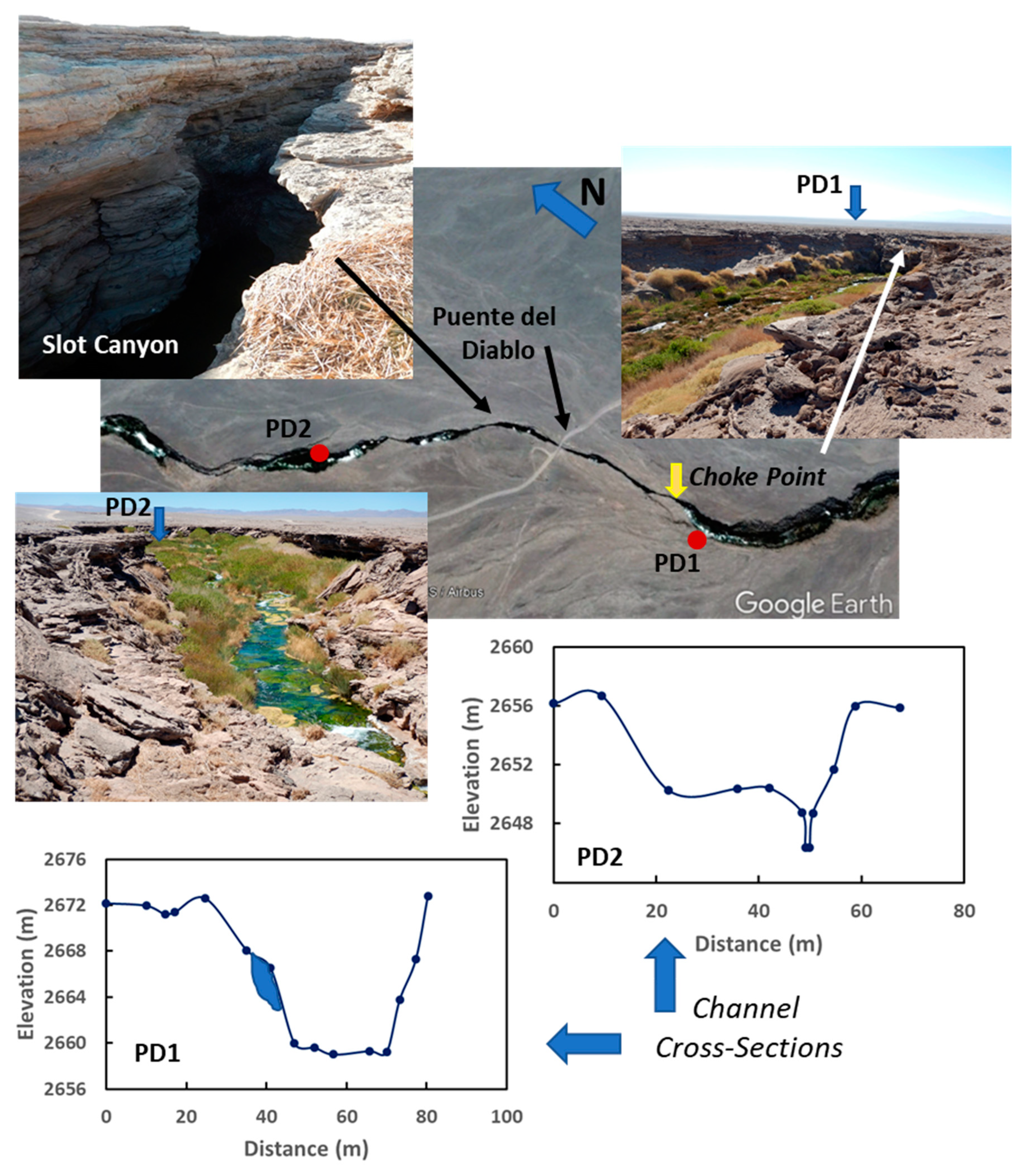
3.1. Characterization of Paleoflood Deposits
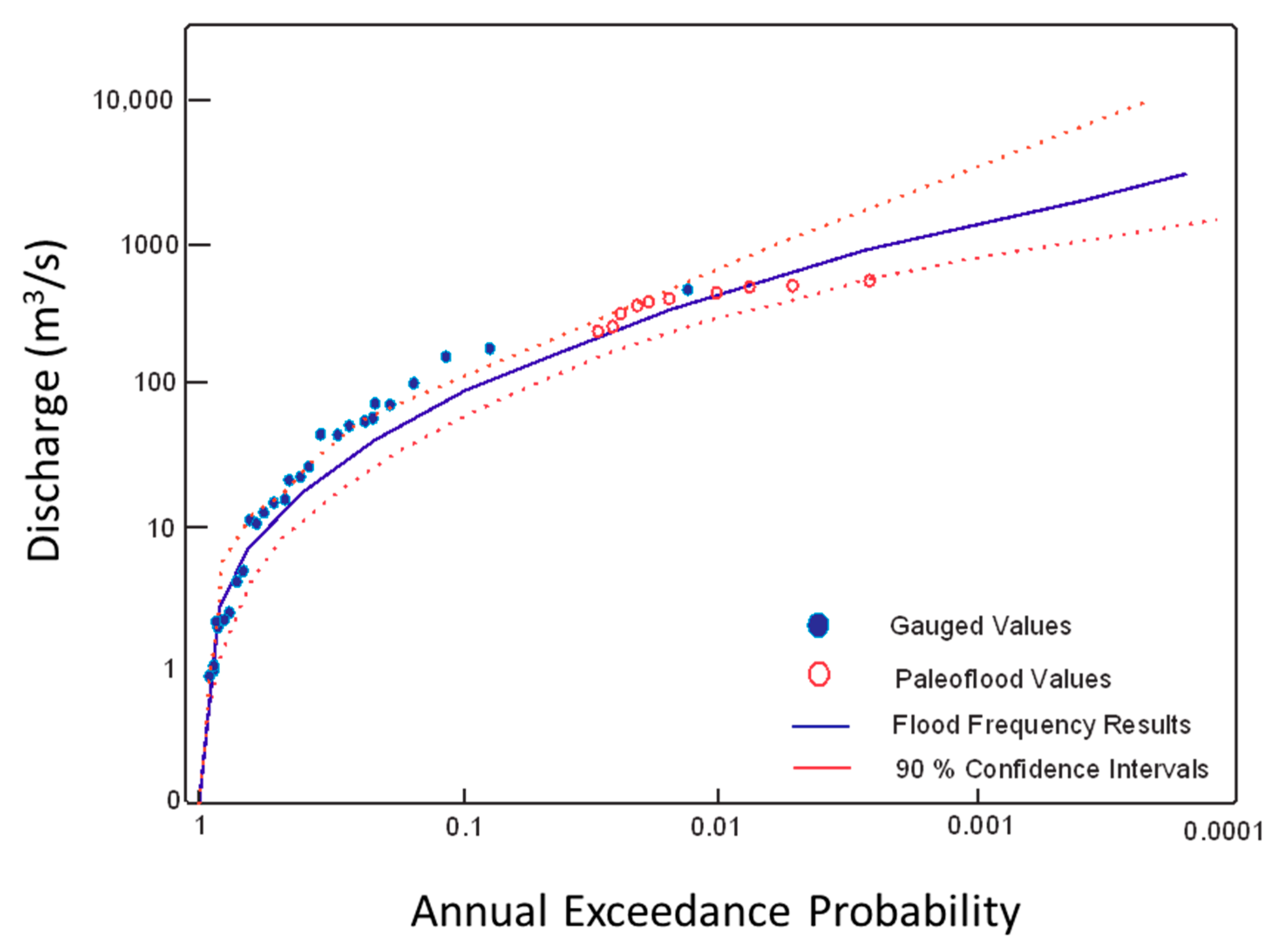
3.2. Determination of Flood Magnitude and Frequency
3.3. Analytical Methods
3.4. Statistical Analysis
4. Results
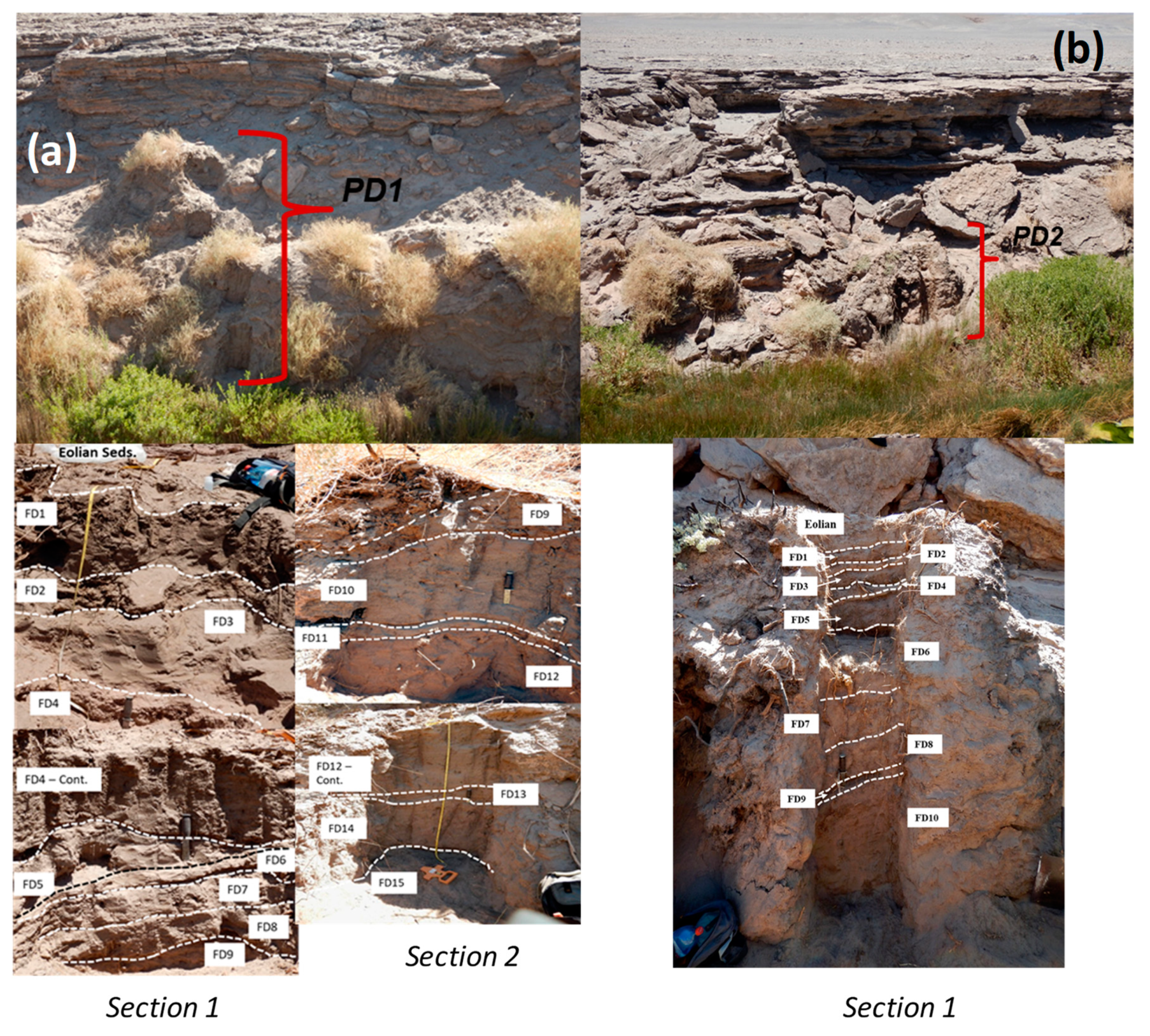
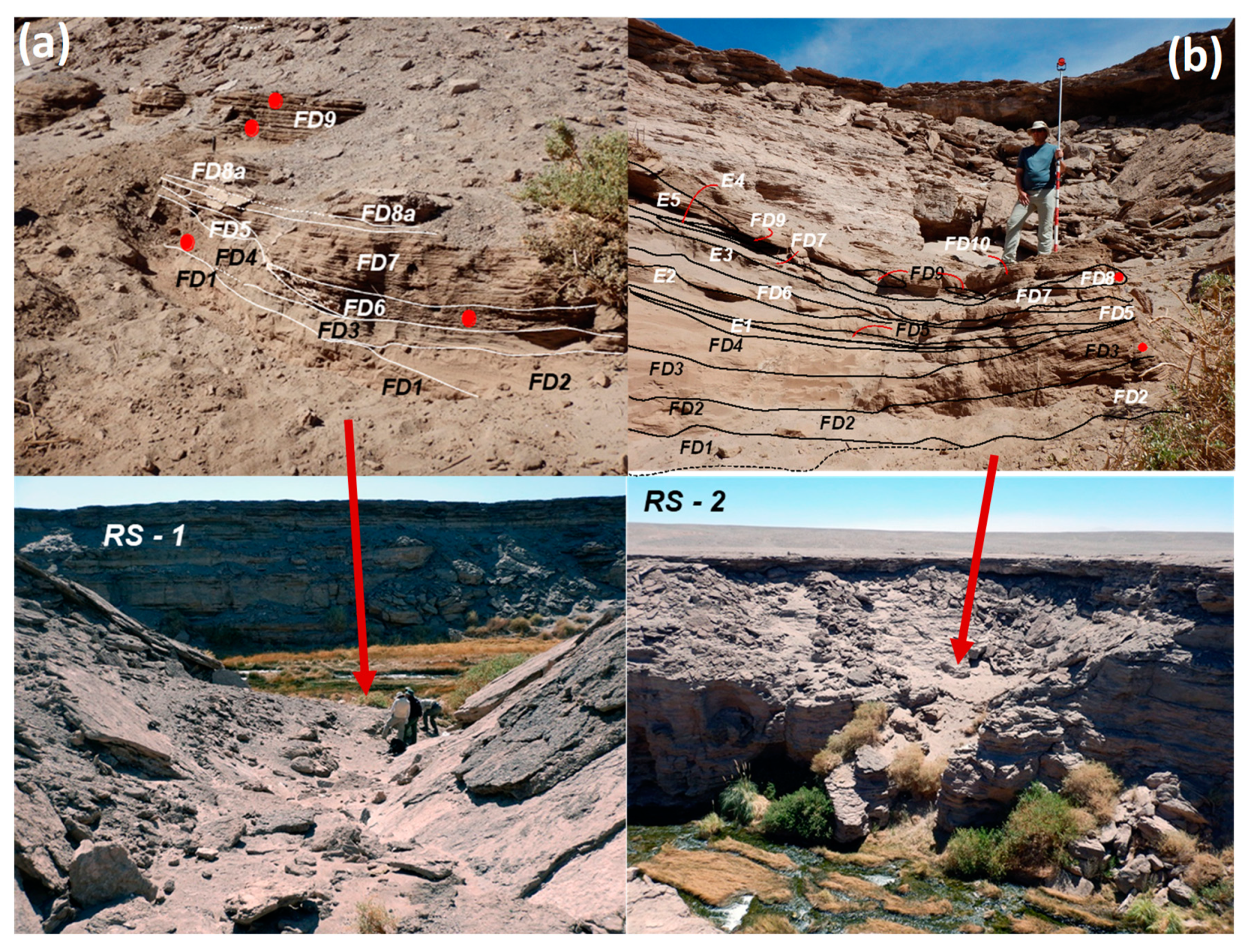
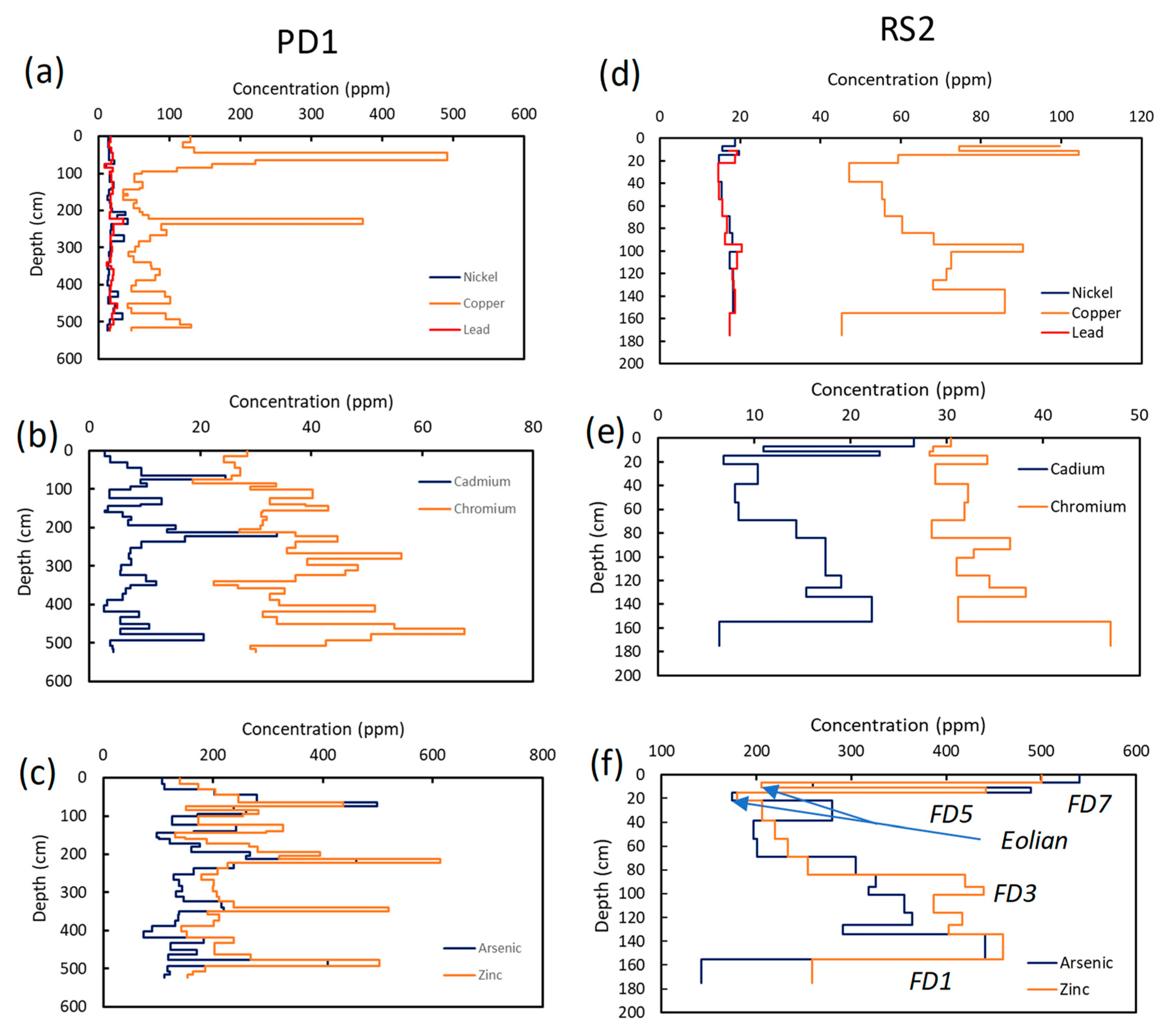
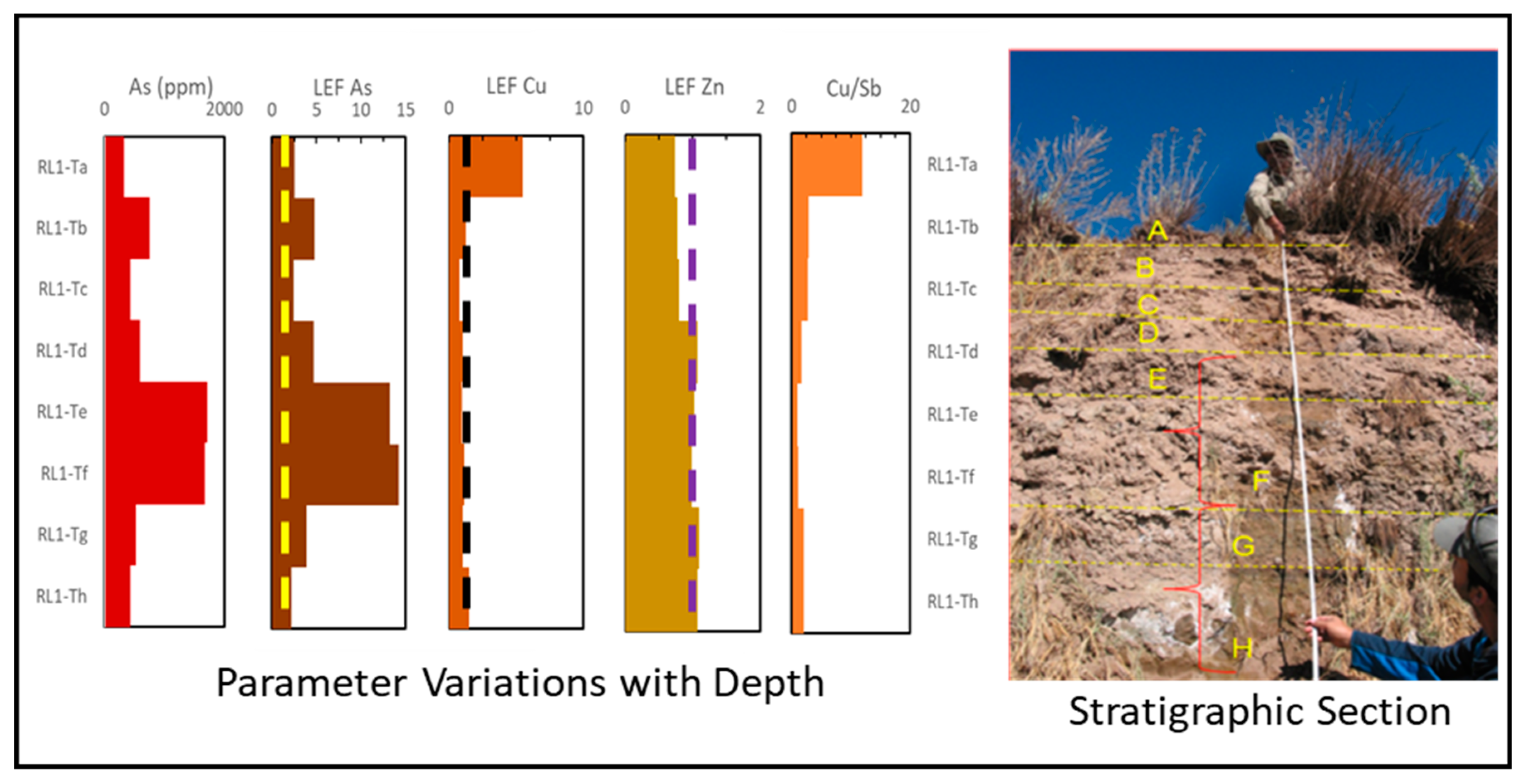
4.1. Identification and Characterization of Flood Deposits
4.2. Paleohydrologic Analyses
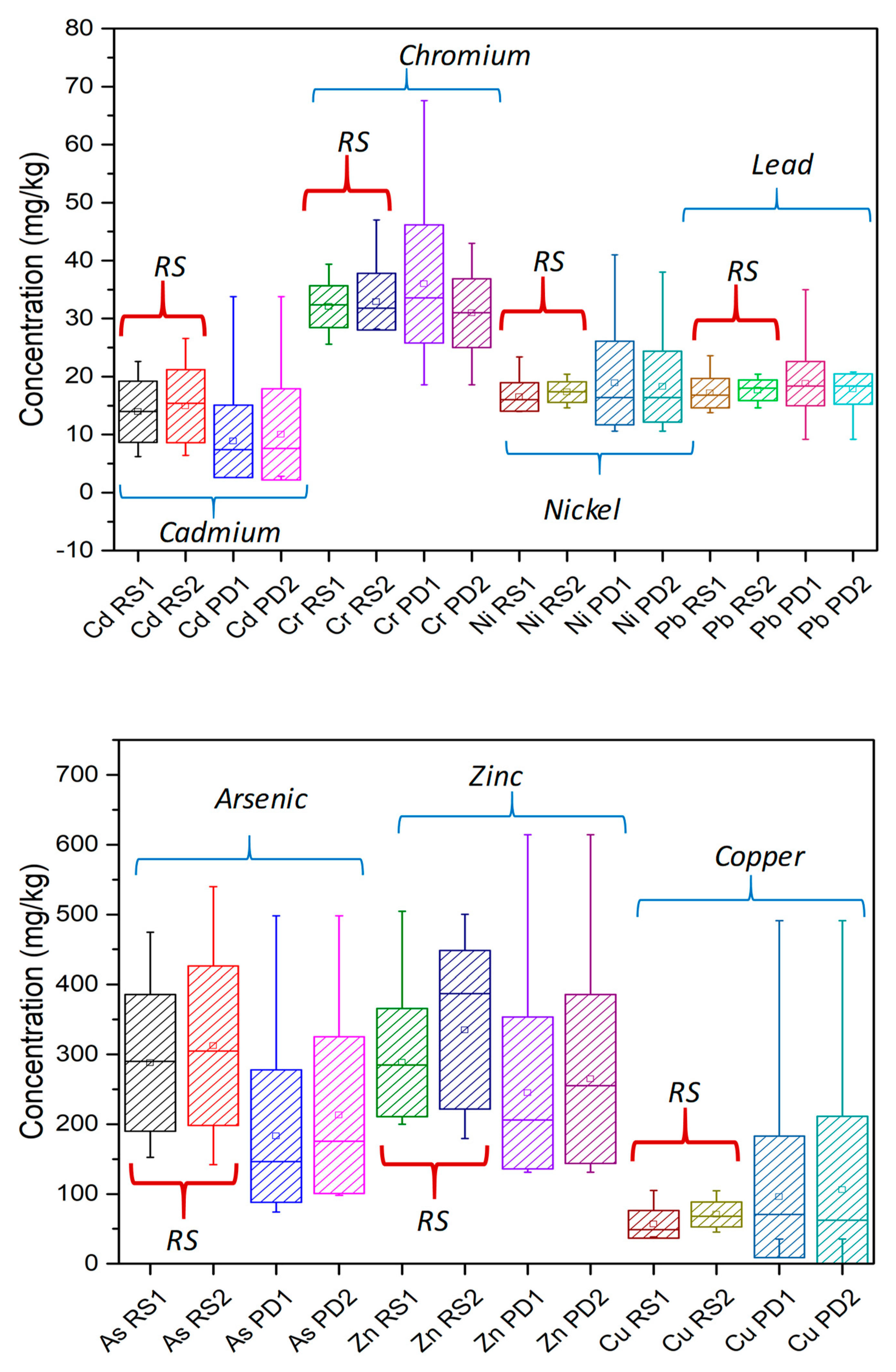
4.3. Deposit Geochemistry
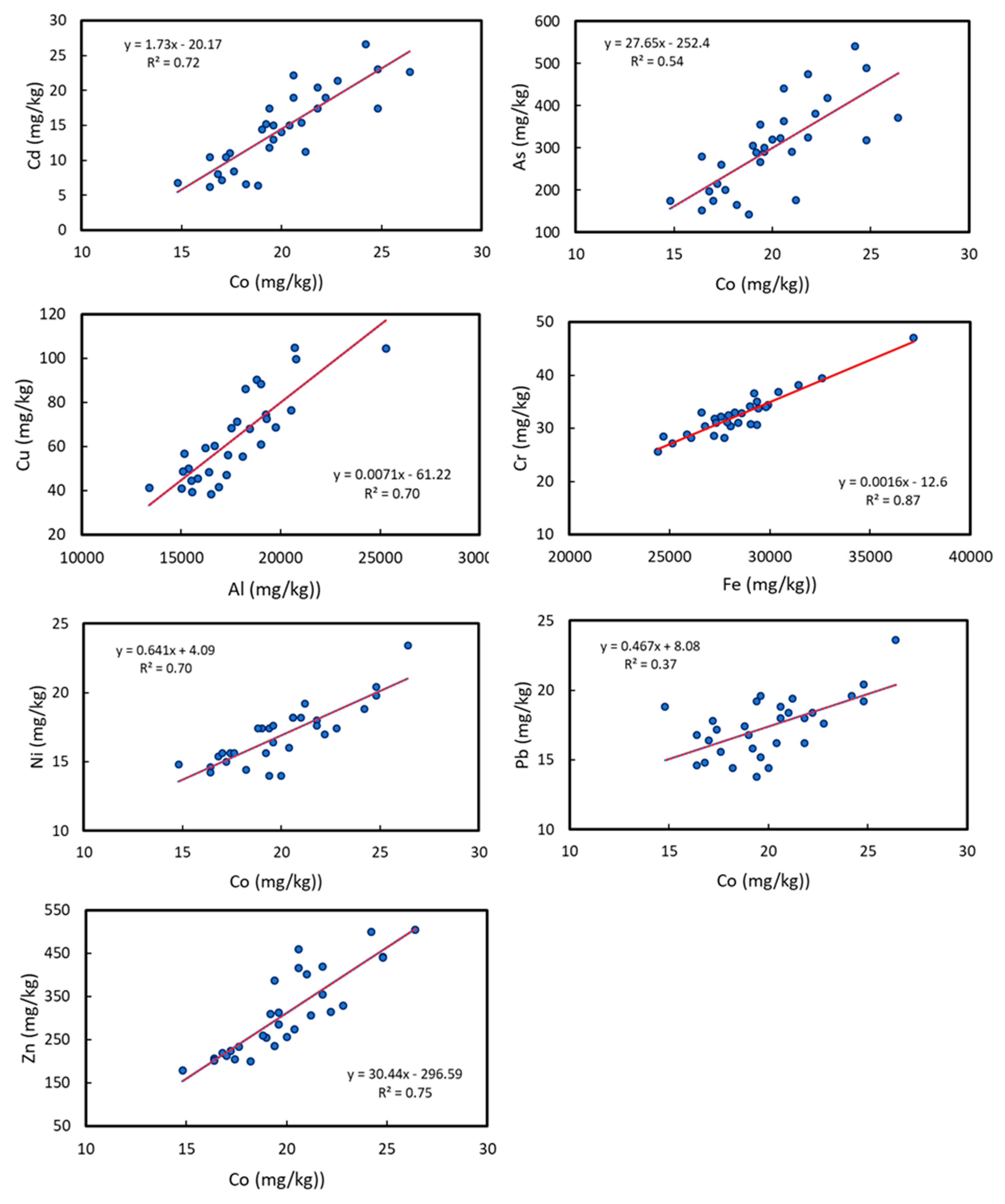
4.4. Calculation and Selection of Background Functions
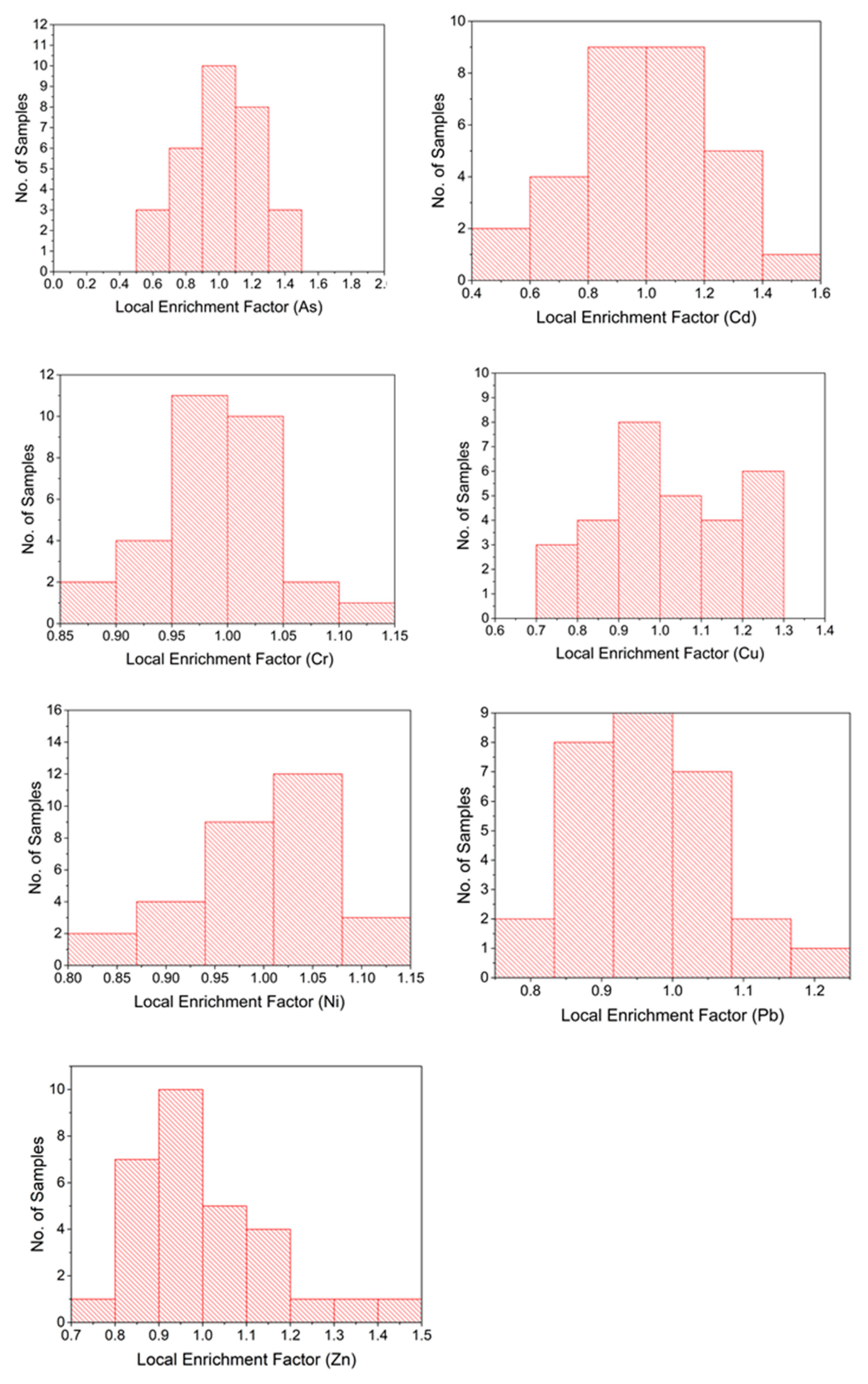
4.5. Calculation and Analysis of Local Enrichment Factors
4.6. Downstream Alluvial Deposits
5. Discussion
5.1. Sedimentologic, Hydrologic and Source Controls on Metal Concentrations
5.2. Use of Paleoflood Deposits to Calculate Background Functions
5.3. Calculated Local Enrichment Factors
5.4. Application of LEF Thresholds to Downstream Alluvial Deposits
6. Summary and Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- USEPA. The Incidence and Severity of Sediment Contamination in Surface Waters of the United States; National Sediment Quality Survey, 2nd ed.; EPA-823-R-04-007; US Environmental Protection Agency, Office of Science and Technology: Washington, DC, USA, 2004.
- Davutluoglu, O.I.; Seckin, G.; Ersu, C.B.; Yilmaz, T.; Sari, B. Heavy Metal Content and Distribution in Surface Sediments of the Seyhan River, Turkey. J. Environ. Manag. 2011, 92, 2250–2259. [Google Scholar] [CrossRef]
- Relic, D.; Dordevic, D.; Sakan, S.; Andelkovic, I.; Pantelic, A.; Stankovic, R.; Popovic, A. Conventional, Microwave, and Ultrasound Sequential Extractions for the Fractionation of Metals in Sediments within the Petrochemical Industry, Serbia. Environ. Monit. Assess. 2013, 85, 7627–7645. [Google Scholar] [CrossRef] [PubMed]
- Sakan, S.; Dordevic, D. Distinguishing between Natural and Anthropogenic Sources of Trace Elements in the Sediments Using the Methods of Geochemical Normalization and Statistical Analysis. In Trace Elements: Environmental Sources, Geochemistry and Human Health; Nova Science Publishers, Inc.: Hauppauge, NY, USA, 2012; pp. 117–134. [Google Scholar]
- Sakan, S.M.; ĐorĐević, D.S.; Lazić, M.M.; Tadić, M.M. Assessment of Arsenic and Mercury Contamination in the Tisa River Sediments and Industrial Canal Sediments (Danube Alluvial Formation), Serbia. J. Environ. Sci. Heal. Part A 2012, 47, 109–116. [Google Scholar] [CrossRef] [PubMed]
- Sakan, S.; Dordevic, D. Assessment of Trace Element Contamination in the River and Alluvial Sediments Using a Sequential Extraction Technique and Statistical Analysis. In Metal Contamination: Sources, Detection and Environmental Impact; Hong-Bo, S., Ed.; Nova Science Publishers, Inc.: Hauppauge, NY, USA, 2013; pp. 119–155. [Google Scholar]
- Gibbs, R. Transport Phases of Transition Metals in the Amazon and Yukon Rivers. Geol. Soc. Am. Bull. 1977, 88, 829–843. [Google Scholar] [CrossRef]
- Martin, J.M.; Meybeck, M. Elemental Mass-Balance of Material Carried by Major World Rivers. Mar. Chem. 1979, 7, 173–206. [Google Scholar] [CrossRef]
- Miller, J.R.; Miller, S.M.O. Contaminated Rivers: A Geomorphological-Geochemical Approach to Site Assessment and Remediation; Springer Science & Business Media: Berlin, Germany, 2007. [Google Scholar]
- Comoro, S.; Vaccaro, G.; De Dapitani, E.; Bawlik, B.W. Characterization of the Danube River Sediments Using PMF Multivariate Approach. Chemosphere 2014, 95, 329–335. [Google Scholar] [CrossRef]
- Reimann, C.; Garrett, R.G. Geochemical Background—Concept and Reality. Sci. Total. Environ. 2005, 350, 12–27. [Google Scholar] [CrossRef]
- Grygar, T.M.; Popelka, J. Revisiting Geochemical Methods of Distinguishing Natural Concentrations and Pollution by Risk Elements in Fluvial Sediments. J. Geochem. Explor. 2016, 170, 39–57. [Google Scholar] [CrossRef]
- Horowitz, A.J. Primer on Sediment-Trace Element Chemistry; Lewis Publishers: Boca Raton, FL, USA, 1991. [Google Scholar]
- Sakan, S.; Sakan, N.M.; Đorđević, D. Evaluation of the Possibility of Using Normalization with Cobalt in Detection of Anthropogenic Heavy Metals in Sediments. Adv. Chem. Res. 2015, 167–183. [Google Scholar]
- Ho, H.H.; Swennen, R.; Cappuyns, V.; Vassilieva, E.; Van Tran, T. Necessity of Normalization to Aluminum to Assess the Contamination by Heavy Metals and Arsenic in Sediments near Haiphong Harbor, Vietnam. J. Asian Earth Sci. 2012, 56, 229–239. [Google Scholar] [CrossRef]
- Borovec, Z. Elements in Size-Fractionated Bottom Sediments of the Elbe River in Its Czech Part. Aquat. Sci. 2000, 62, 232–251. [Google Scholar] [CrossRef]
- Reimann, C.; de Caritat, P. Distinguishing between Natural and Anthropogenic Sources for Elements in the Environment: Regional Geochemical Surveys versus Enrichment Factors. Sci. Total. Environ. 2005, 337, 91–107. [Google Scholar] [CrossRef]
- Covelli, S.; Fontolan, G. Application of a Normalization Procedure in Determining Regional Geochemical Baselines. Environ. Geol. 1997, 30, 34–45. [Google Scholar] [CrossRef]
- Vijver, M.G.; Spijker, J.; Vink, J.P.M.; Posthuma, L. Determining Metal Origins and Availability in Fluvial Deposits by Analysis of Geochemical Baselines and Solid–Solution Partitioning Measurements and Modelling. Environ. Pollut. 2008, 156, 832–839. [Google Scholar] [CrossRef] [PubMed]
- Turekian, K.K.; Wedepohl, K.H. Distribution of the Elements in Some Major Units of the Earth’s Crust. Geol. Soc. Am. Bull. 1961, 72, 175–192. [Google Scholar] [CrossRef]
- Beck, M.L.; Hopkins, W.A.; Jackson, B.P. Spatial and Temporal Variation in the Diet of Tree Swallows: Implications for Trace Element Exposure Following Habitat Remediation. Arch. Environ. Contam. Toxicol. 2013, 65, 575–587. [Google Scholar] [CrossRef]
- Grygar, T.M.; Elznicová, J.; Bábek, O.; Hošek, M.; Engel, Z.; Kiss, T. Obtaining Isochrones from Pollution Signals in a Fluvial Sediment Record: A Case Study in a Uranium-Polluted Floodplain of the Ploučnice River, Czech Republic. Appl. Geochem. 2014, 48, 1–15. [Google Scholar] [CrossRef]
- Novakova, T.; Kotková, K.; Elznicova, J.; Strnad, L.; Engel, Z.; Grygar, T.M. Pollutant Dispersal and Stability in a Severely Polluted Floodplain: A Case Study in the Litavka River, Czech Republic. J. Geochem. Explor. 2015, 156, 131–144. [Google Scholar] [CrossRef]
- Sims, D.B.; Hooda, P.S.; Gillmore, G.K. Mining Activities and Associated Environmental Impacts in Arid Climates: A Literature Review. Environ. Pollut. 2013, 2, 22. [Google Scholar] [CrossRef]
- Rech, J.A.; Pigati, J.S.; Quade, J.; Betancourt, J.L. Re-Evaluation of Mid-Holocene Deposits at Quebrada Puripica, Northern Chile. Palaeogeogr. Palaeoclimatol. Palaeoecol. 2003, 194, 207–222. [Google Scholar] [CrossRef]
- Houston, J. The Great Atacama Flood of 2001 and Its Implications for Andean Hydrology. Hydrol. Process. 2006, 20, 591–610. [Google Scholar] [CrossRef]
- Magilligan, F.J.; Goldstein, P.S.; Fisher, G.B.; Bostick, B.C.; Manners, R.B. Late Quaternary Hydroclimatology of a Hyper-Arid Andean Watershed: Climate Change, Floods, and Hydrologic Responses to the El Niño-Southern Oscillation in the Atacama Desert. Geomorphology 2008, 101, 14–32. [Google Scholar] [CrossRef]
- Rech, J.A.; Quade, J.; Betancourt, J.L. Late Quaternary Paleohydrology of the Central Atacama Desert (Lat 22°–24°S), Chile. GSA Bull. 2002, 114, 334–348. [Google Scholar] [CrossRef]
- MacDonald, D.D.; Ingersoll, C.G.; Berger, T.A. Development and Evaluation of Consensus-Based Sediment Quality Guidelines for Freshwater Ecosystems. Arch. Environ. Contam. Toxicol. 2000, 39, 20–31. [Google Scholar] [CrossRef] [PubMed]
- Romero, L.; Alonso, H.; Campano, P.; Fanfani, L.; Cidu, R.; Dadea, C.; Keegan, T.; Thornton, I.; Farago, M. Arsenic Enrichment in Waters and Sediments of the Rio Loa (Second Region, Chile). Applie Geochem. 2003, 18, 399–1416. [Google Scholar] [CrossRef]
- Landrum, J.T.; Bennett, P.C.; Engel, A.S.; Alsina, M.A.; Pasten, P.A.; Milliken, K. Partitioning Geochemistry of Arsenic and Antimony, El Tatio Geyser Field, Chile. Appl. Geochem. 2009, 24, 664–676. [Google Scholar] [CrossRef]
- Wilson, L.R. Determination of Trace Element Provenance, Rio Loa Basin, Northern Chile. Master’s Thesis, Western Carolina University, Cullowhee, NC, USA, 2011. [Google Scholar]
- Kottlowski, F.E. Measuring Stratigraphic Sections; Holt, Rinehart, and Winston: New York, NY, USA, 1965. [Google Scholar]
- Bridge, J.S. Rivers and Floodplains; Wiley-Blackwell: Hoboken, NJ, USA, 2003. [Google Scholar]
- Kochel, R.C.; Baker, V.R. Paleoflood Hydrology. Science 1982, 215, 353–361. [Google Scholar] [CrossRef] [PubMed]
- Baker, V.R. Palaeoflood Hydrology in a Global Context. CATENA 2006, 66, 161–168. [Google Scholar] [CrossRef]
- Baker, V.R.; Kochel, R.C.; Patton, P.C.; Pickup, G. Palaeohydrologic Analysis of Holocene Flood Slack-Water Sediments. In Modern and Ancient Fluvial Systems; John Wiley & Sons: Hoboken, NJ, USA, 2009; pp. 229–239. [Google Scholar]
- Baker, V.R. Paleoflood Hydrology: Origin, Progress, Prospects. Geomorphology 2008, 101, 1–13. [Google Scholar] [CrossRef]
- O’Connor, J.E.; Webb, R.W. Hydraulic Modeling for Paleoflood Analysis. In Flood Geomorphology; Baker, V.R., Kochel, R.C., Patton, P., Eds.; John Wiley & Sons: Hoboken, NJ, USA, 1988; pp. 393–402, 503. [Google Scholar]
- Webb, R.H.; Jarrett, R.D. One-Dimensional Estimation Techniques for Discharges of Paleofloods and Historical Floods: Ancient Floods, Modern Hazards: Principles and Applications of Paleoflood Hydrology. In Ancient Floods, Modern Hazards: Principles and Applications of Paleoflood Hydrology; House, P.K., Webb, R.H., Baker, V.R., Levish, D.R., Eds.; Water Science and Applications; American Geophysical Union: Washington, DC, USA, 2002; Volume 5, pp. 111–125. [Google Scholar]
- Cudworth, A.G., Jr. Flood Hydrology Manual; Surface Water Branch, U.S Department of Interior, Bureau of Reclamation: Denver, CO, USA, 1989.
- Cohn, T. User Manual for Program PeakfqSA Flood-Frequency Analysis with the Expected Moments Algorithm; United States Geological Survey: Reston, VA, USA, 2012.
- England, J.F., Jr.; Salas, J.D.; Jarrett, R.D. Comparison of Two Moment-Based Estimators That Utilize Historical and Paleoflood Data for the Log-Pearson Type III Distribution. Water Resour. Res. 2003, 39, 1243. [Google Scholar] [CrossRef]
- Walsh, D. Chemostratigraphy of Slackwater Deposits and Paleoflood Hydrology of the Rio Salado, Antofagasta Region, Chile. Master’s Thesis, Western Carolina University, Cullowhee, NC, USA, 2016. [Google Scholar]
- Miller, J.R.; Lechler, P.J.; Mackin, G.; Germanoski, D.; Villarroel, L.F. Evaluation of Particle Dispersal from Mining and Milling Operations Using Lead Isotopic Fingerprinting Techniques, Rio Pilcomayo Basin, Bolivia. Sci. Total. Environ. 2007, 384, 355–373. [Google Scholar] [CrossRef]
- Falkowska, E.; Falkowski, T. Trace Metals Distribution Pattern in Floodplain Sediments of a Lowland River in Relation to Contemporary Valley Bottom Morphodynamics. Earth Surf. Process. Landforms 2015, 40, 876–887. [Google Scholar] [CrossRef]
- Greenbaum, N.; Harden, T.M.; Baker, V.R.; Weisheit, J.; Cline, M.L.; Porat, N.; Halevi, R.; Dohrenwend, J. A 2000 Year Natural Record of Magnitudes and Frequencies for the Largest Upper Colorado River Floods near Moab, Utah. Water Resour. Res. 2014, 50, 5249–5269. [Google Scholar] [CrossRef]
- Webb, R.H.; O’Connor, J.E.; Baker, V.R. Paleohydrologic Reconstruction of Flood Frequency on the Escalante River, South-Central Utah. In Flood Geomorphology; Baker, V.R., Kochel, R.C., Patton, P.C., Eds.; John Wiley & Sons: Hoboken, NJ, USA, 1988; pp. 403–418. [Google Scholar]
- Hussain, Z. Application of the Regional Flood Frequency Analysis to the Upper and Lower Basins of the Indus River, Pakistan. Water Resour. Manag. 2011, 25, 2797–2822. [Google Scholar] [CrossRef]
- Grygar, T.; Světlík, I.; Lisá, L.; Koptíková, L.; Bajer, A.; Wray, D.S.; Ettler, V.; Mihaljevič, M.; Nováková, T.; Koubová, M.; et al. Geochemical Tools for the Stratigraphic Correlation of Floodplain Deposits of the Morava River in Strážnické Pomoraví, Czech Republic from the Last Millennium. CATENA 2010, 80, 106–121. [Google Scholar] [CrossRef]
- Majerová, L.; Grygar, T.M.; Elznicova, J.; Strnad, L. The Differentiation between Point and Diffuse Industrial Pollution of the Floodplain of the Ploučnice River, Czech Republic. Water Air Soil Pollut. 2013, 224, 1688. [Google Scholar] [CrossRef]
- Horowitz, A.J.; Elrick, K.A. The Relation of Stream Sediment Surface Area, Grain Size and Composition to Trace Element Chemistry. Appl. Geochem. 1987, 2, 437–451. [Google Scholar] [CrossRef]
- Filgueiras, A.V.; Lavilla, I.; Bendicho, C. Evaluation of Distribution, Mobility and Binding Behaviour of Heavy Metals in Surficial Sediments of Louro River (Galicia, Spain) Using Chemometric Analysis: A Case Study. Sci. Total. Environ. 2004, 330, 115–129. [Google Scholar] [CrossRef] [PubMed]
- Benson, N.U.; Asuquo, F.E.; Williams, A.B.; Essien, J.P.; Ekong, C.I.; Akpabio, O.; Olajire, A.A. Source Evaluation and Trace Metal Contamination in Benthic Sediments from Equatorial Ecosystems Using Multivariate Statistical Techniques. PLoS ONE 2016, 11, e0156485. [Google Scholar] [CrossRef]
- Keefer, D.K.; Moseley, M.E.; deFrance, S.D. A 38 000-Year Record of Floods and Debris Flows in the Ilo Region of Southern Peru and Its Relation to El Niño Events and Great Earthquakes. Palaeogeogr. Palaeoclimatol. Palaeoecol. 2003, 194, 41–77. [Google Scholar] [CrossRef]
- Manners, R.B.; Magilligan, F.J.; Goldstein, P.S. Floodplain Development, El Niño, and Cultural Consequences in a Hyperarid Andean Environment. Ann. Assoc. Am. Geogr. 2007, 97, 229–249. [Google Scholar] [CrossRef]
- Wells, L.E. Holocene History of the El Niño Phenomenon as Recorded in Flood Sediments of Northern Coastal Peru. Geology 1990, 18, 1134–1137. [Google Scholar] [CrossRef]
- Wang, Z.; Darilek, J.L.; Zhao, Y.; Huang, B.; Sun, W. Defining Soil Geochemical Baselines at Small Scales Using Geochemical Common Factors and Soil Organic Matter as Normalizers. J. Soils Sediments 2011, 11, 3–14. [Google Scholar] [CrossRef]
- Loring, D.H. Lithium—A New Approach for the Granulometric Normalization of Trace Metal Data. Mar. Chem. 1990, 29, 155–168. [Google Scholar] [CrossRef]
- Soto-Jemenez, M.; Paez-Osuma, F. Cd, Cu, Pb, and Zn in Lagoonal Sediments from Mazatlán Harbor (SE Gulf of California): Bioavailability and Geochemical Fractioning. Bulliten Environ. Contam. Toxicol. 2001, 66, 350–356. [Google Scholar]
- Schiff, K.C.; Weisberg, S.B. Iron as a Reference Element for Determining Trace Metal Enrichment in Southern California Coastal Shelf Sediments. Mar. Environ. Res. 1999, 48, 161–176. [Google Scholar] [CrossRef]
- Dung, T.T.T.; Cappuyns, V.; Swennen, R.; Phung, N.K. From Geochemical Background Determination to Pollution Assessment of Heavy Metals in Sediments and Soils. Rev. Environ. Sci. Biotechnol. 2013, 12, 335–353. [Google Scholar] [CrossRef]
- Föstner, U.; Wittman, G.T.W. Metal Pollution in the Aquatic Environment; Springer: New York, NY, USA, 1981. [Google Scholar]
- Baeyens, W.; Panutrakul, S.; Elsken, M.; Leermakers, M.; Navez, J.; Monteny, F. Geochemical Processes in Muddy Adn Sandy Tidal Flat Sediments. Geo-Mar. Lett. 1991, 11, 188. [Google Scholar] [CrossRef]
- Herut, B.; Sandler, A. Normalization Methods for Pollutants in Marine Sediments: Review and Recommendations for the Mediterranean Basin; Oil Report H18/2006; UNEP/MAP: Nairobi, Kenya, 2006. [Google Scholar]
- The Intergovernmental Océanographie Commission (IOC); The International Atomic Energy Agency; Marine Environment Laboratory (IAEAMEL); The United Nations Environment Programme (UNEP). Manual for the Geochemical Analyses of Marine Sediments and Suspended Particulate Matter; Reference Methods for Marine Pollution Studies, NO. 63; UNEP: New York, NY, USA, 1995. [Google Scholar]
- WHO. Guidelines for Drinking-Water Quality, 2nd ed.; World Health Organization: Geneva, Switzerland, 1993; Volume 1. [Google Scholar]



| Element | Mean Chanel Bed Sediment Concentrations (mg/kg) | ||||||
|---|---|---|---|---|---|---|---|
| TEC | PEC | El Tatio Geyser Basin | Upper Rio Loa | Rio Loa | Upper Rio Loa Mine Tribs * | Rio San Salvador * | |
| As | 9.79 | 33.0 | 2960 | 34.4 | 248 | 162 | 544 |
| Cd | 0.99 | 4.98 | 0.69 | 2.17 | 1.03 | 2.30 | 6.64 |
| Cr | 43.4 | 111 | 21.3 | 48.4 | 15.2 | 42.1 | 55.9 |
| Cu | 31.6 | 149 | 55.8 | 63.7 | 16.9 | 726 | 1580 |
| Ni | 22.7 | 48.6 | 5.58 | 17.4 | 7.94 | 22.7 | 24.7 |
| Pb | 35.8 | 128 | 15.6 | 11.4 | 9.58 | 24.2 | 154 |
| Sb | - | - | 521 | 7.84 | 7.40 | 9.92 | 22.4 |
| Zn | 121 | 459 | 44.0 | 74.2 | 46.0 | 117 | 1040 |
| Reach RS | ||||
| Trace Metal | n | Mean | Range | Standard Deviation |
| As | 30 | 299.9 | 141.8–540.0 | 105.3 |
| Cd | 30 | 14.42 | 6.200–26.6 | 5.72 |
| Cr | 30 | 32.50 | 25.60–47.0 | 4.24 |
| Cu | 30 | 63.57 | 38.2–104.8 | 19.94 |
| Ni | 30 | 16.91 | 14.00–23.4 | 2.15 |
| Pb | 30 | 17.41 | 13.80–23.6 | 2.16 |
| Zn | 30 | 311.5 | 179.4–504.4 | 98.37 |
| % <63 µm | 30 | 15.1 | 2.0–55.8 | 13.7 |
| % Organics | 30 | 0.600 | 0.002–1.273 | 0.291 |
| Reach PD | ||||
| Trace Metal | n | Mean | Range | Standard Deviation |
| As | 60 | 192.4 | 74.20–498.2 | 100.8 |
| Cd | 60 | 9.21 | 2.60–33.80 | 6.76 |
| Cr | 60 | 34.39 | 18.60–67.60 | 9.30 |
| Cu | 60 | 99.04 | 35.40–491.2 | 92.53 |
| Ni | 60 | 18.70 | 10.60–41.0 | 6.82 |
| Pb | 60 | 18.49 | 9.20–35.0 | 3.49 |
| Zn | 60 | 251.0 | 131.0–614.2 | 112.3 |
| % <63 µm | 60 | 19.3 | 5.0–70.5 | 12.7 |
| % Organics | 60 | 0.864 | 0.218–4.224 | 0.735 |
| All Data (Both Reaches; n = 90) | ||||||||
| Parameter | As | Cd | Cr | Cu | Ni | Pb | Zn | Organics |
| As | 1.00 | - | - | - | - | - | - | - |
| Cd | 0.94 ** | 1.00 | - | - | - | - | - | - |
| Cr | −0.25 * | -0.13 | 1.00 | - | - | - | - | - |
| Cu | −0.21 * | 0.12 | −0.22 * | 1.00 | - | - | - | - |
| Ni | 0.32 * | 0.45 ** | 0.33 ** | 0.13 | 1.00 | - | - | - |
| Pb | −0.01 | 0.08 | 0.49 ** | 0.31 ** | 0.42 ** | 1.00 | - | - |
| Zn | 0.82 * | 0.90 * | −0.06 | 0.03 | 0.43 ** | 0.06 | 1.00 | - |
| Organics | 0.09 | 0.01 | −0.28 ** | 0.38 ** | 0.14 | −0.12 | 0.09 | 1.00 |
| Reach PD (n = 60) | ||||||||
| Parameter | As | Cd | Cr | Cu | Ni | Pb | Zn | Organics |
| As | 1.00 | - | - | - | - | - | - | - |
| Cd | 0.93 ** | 1.00 | - | - | - | - | - | - |
| Cr | −0.19 | -0.05 | 1.00 | - | - | - | - | - |
| Cu | 0.36 ** | 0.19 | −0.26 | 1.00 | - | - | - | - |
| Ni | 0.47 ** | 0.57 ** | 0.33 ** | 0.08 | 1.00 | - | - | - |
| Pb | −0.02 | 0.06 | 0.54 ** | 0.26 * | 0.37 ** | 1.00 | - | - |
| Zn | 0.84 ** | 0.90 ** | −0.03 | 0.03 | 0.48 ** | −0.05 | 1.00 | - |
| Organics | 0.13 | −0.01 | −0.30 * | 0.34 ** | −0.21 | −0.22 | -0.12 | 1.00 |
| Reach RS (n = 30) | ||||||||
| Parameter | As | Cd | Cr | Cu | Ni | Pb | Zn | Organics |
| As | 1.00 | - | - | - | - | - | - | - |
| Cd | 0.96 ** | 1.00 | - | - | - | - | - | - |
| Cr | −0.45 * | −0.36 * | 1.00 | - | - | - | - | - |
| Cu | 0.69 ** | 0.73 ** | −0.16 | 1.00 | - | - | - | - |
| Ni | 0.52 ** | 0.68 ** | 0.11 | 0.83 ** | 1.00 | - | - | - |
| Pb | 0.40 * | 0.53 ** | 0.11 | 0.80 ** | 0.84 ** | 1.00 | - | - |
| Zn | 0.77 ** | 088 ** | −0.08 | 0.80 ** | 0.85 * | 0.66 ** | 100 | - |
| Organics | 0.68 ** | 0.62 ** | 0.01 | 0.55 ** | 0.39 * | 0.31 | 0.44 * | 1.00 |
| Background Function | n | R2 | LEF (p = 95%) | LEF (p = 99%) |
|---|---|---|---|---|
| Reach RS | ||||
| As = 27.65Co − 252.44 | 30 | 0.54 | 1.079 | 1.104 |
| Cd = 1.73Co − 20.17 | 30 | 0.72 | 1.078 | 1.102 |
| Cr = 0.0016Fe − 12.60 | 30 | 0.87 | 1.006 | 1.012 |
| Cu = 0.007Al − 61.22 | 30 | 0.70 | 1.076 | 1.096 |
| Ni = 0.641Co + 4.09 | 30 | 0.70 | 1.025 | 1.083 |
| Pb = 0.467Co + 8.075 | 30 | 0.37 | 1.000 | 1.062 |
| Zn = 30.44Co − 296.59 | 30 | 0.75 | 1.011 | 1.079 |
| Reach PD | ||||
| As = 19.57Co − 175.38 | 60 | 0.74 | 1.116 | 1.270 |
| Cd = 1.29Co − 15.01 | 60 | 0.72 | 1.520 | 1.789 |
| Cr = None significant | 60 | - | - | - |
| Cu = None significant | 60 | - | - | - |
| Ni = 0.79Co + 3.89 | 60 | 0.26 | 1.075 | 1.142 |
| Pb = Not significant | 60 | - | - | - |
| Zn = 22.02Co − 162.69 | 60 | 9.76 | 1.052 | 1.094 |
| Trace Metal | Mean | Range | Standard Deviation |
|---|---|---|---|
| As | 806.6 | 330.7–1713 | 560.2 |
| Cd | 2.790 | 1.620–5.140 | 1.259 |
| Cr | 29.81 | 1.620–5.410 | 6.765 |
| Cu | 67.65 | 27.85–219.6 | 65.85 |
| Ni | 15.34 | 12.25–20.15 | 2.499 |
| Pb | 10.89 | 4.88–16.38 | 4.085 |
| Zn | 123.1 | 85.77–191.5 | 31.22 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Miller, J.R.; Walsh, D.; Villarroel, L.F. Use of Paleoflood Deposits to Determine the Contribution of Anthropogenic Trace Metals to Alluvial Sediments in the Hyperarid Rio Loa Basin, Chile. Geosciences 2019, 9, 244. https://doi.org/10.3390/geosciences9060244
Miller JR, Walsh D, Villarroel LF. Use of Paleoflood Deposits to Determine the Contribution of Anthropogenic Trace Metals to Alluvial Sediments in the Hyperarid Rio Loa Basin, Chile. Geosciences. 2019; 9(6):244. https://doi.org/10.3390/geosciences9060244
Chicago/Turabian StyleMiller, Jerry R., Danvey Walsh, and Lionel F. Villarroel. 2019. "Use of Paleoflood Deposits to Determine the Contribution of Anthropogenic Trace Metals to Alluvial Sediments in the Hyperarid Rio Loa Basin, Chile" Geosciences 9, no. 6: 244. https://doi.org/10.3390/geosciences9060244
APA StyleMiller, J. R., Walsh, D., & Villarroel, L. F. (2019). Use of Paleoflood Deposits to Determine the Contribution of Anthropogenic Trace Metals to Alluvial Sediments in the Hyperarid Rio Loa Basin, Chile. Geosciences, 9(6), 244. https://doi.org/10.3390/geosciences9060244




