Lithospheric Structure of a Transitional Magmatic to Amagmatic Continental Rift System—Insights from Magnetotelluric and Local Tomography Studies in the North Tanzanian Divergence, East African Rift
Abstract
1. Introduction
2. Geological Setting
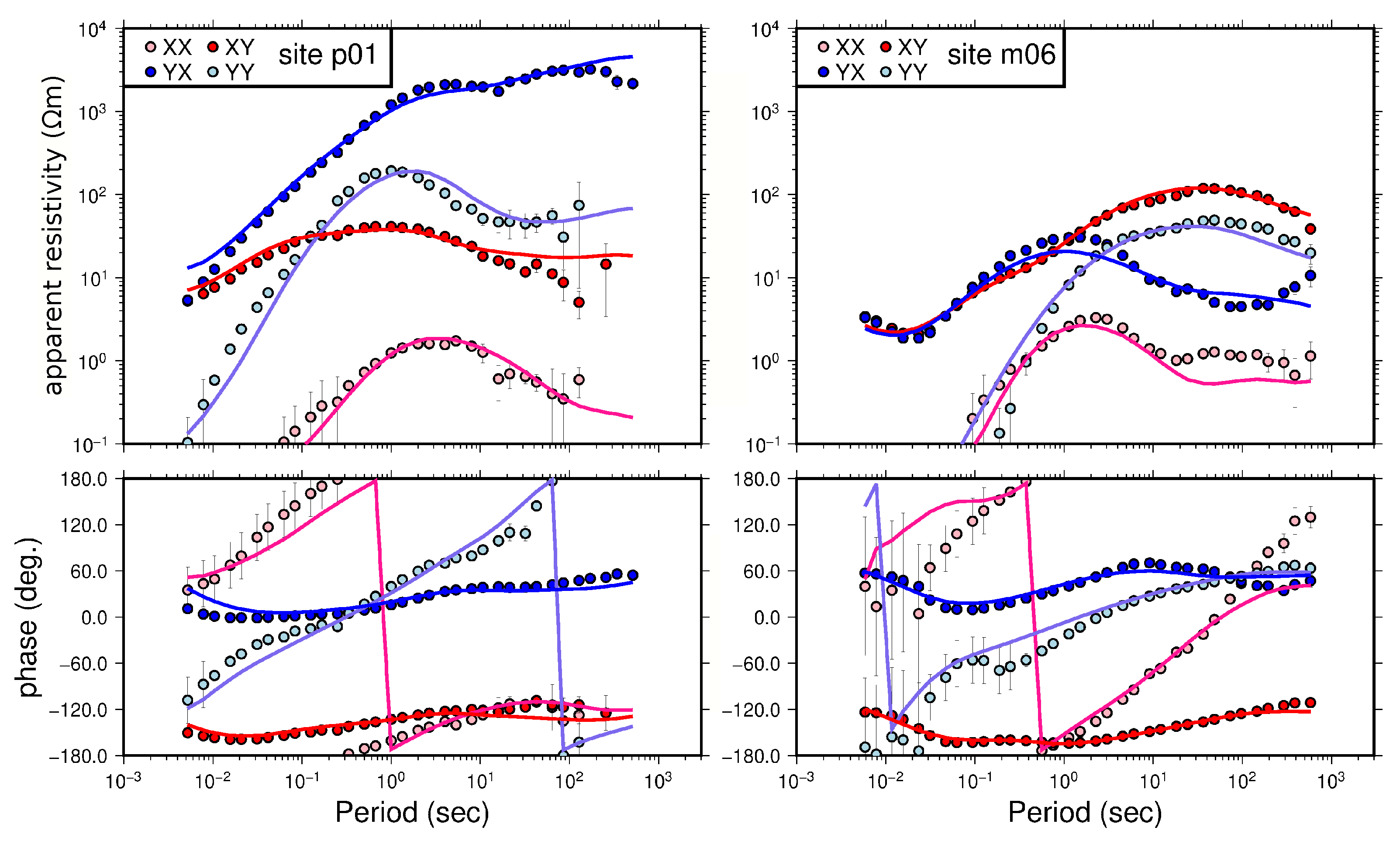
3. Magnetotelluric Data Acquisition and Processing
4. Data Analysis and 3-D Inversion
4.1. Geoelectrical Strike
4.2. Three-Dimensional Inversion
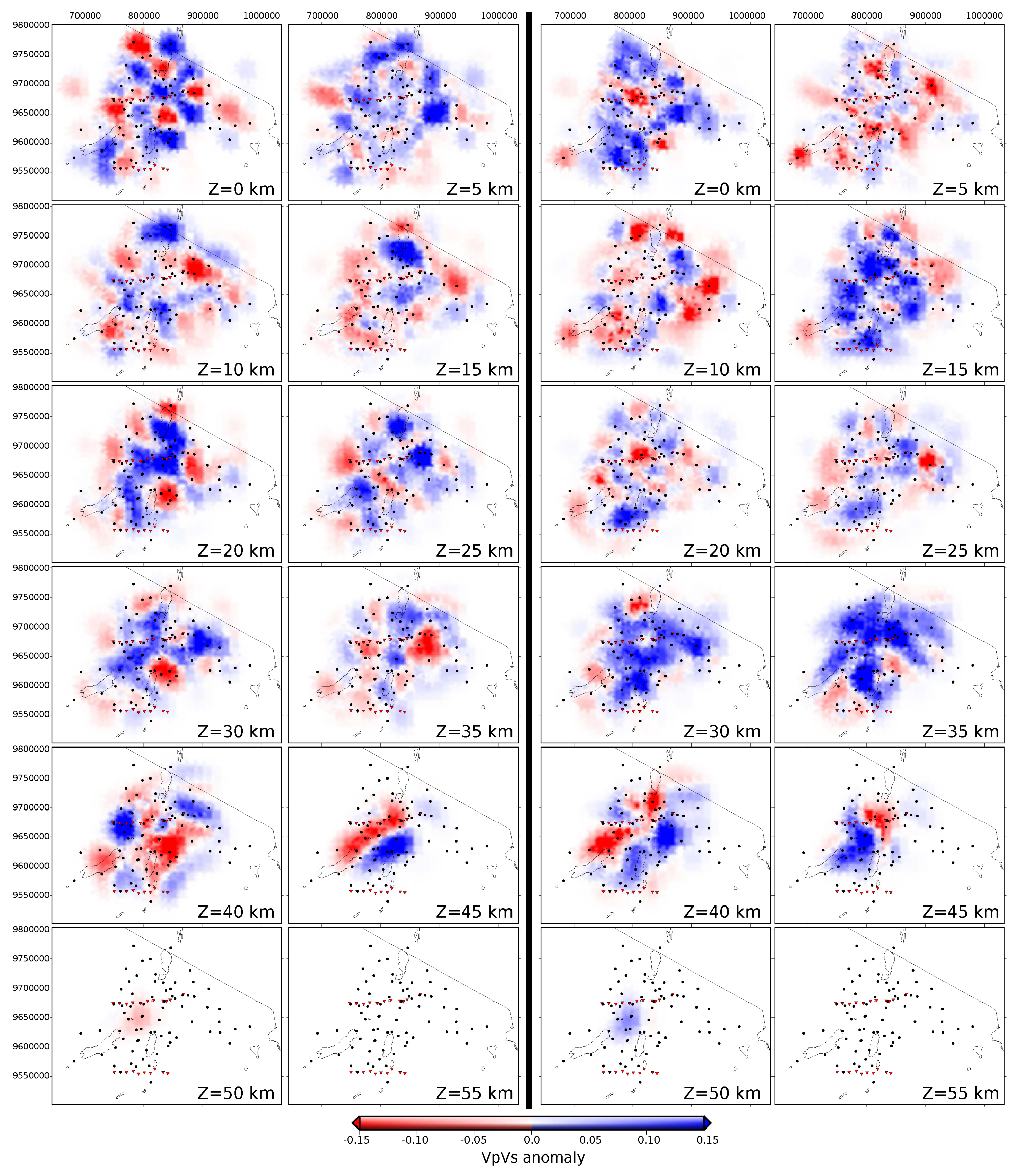
4.3. Sensitivity Analysis
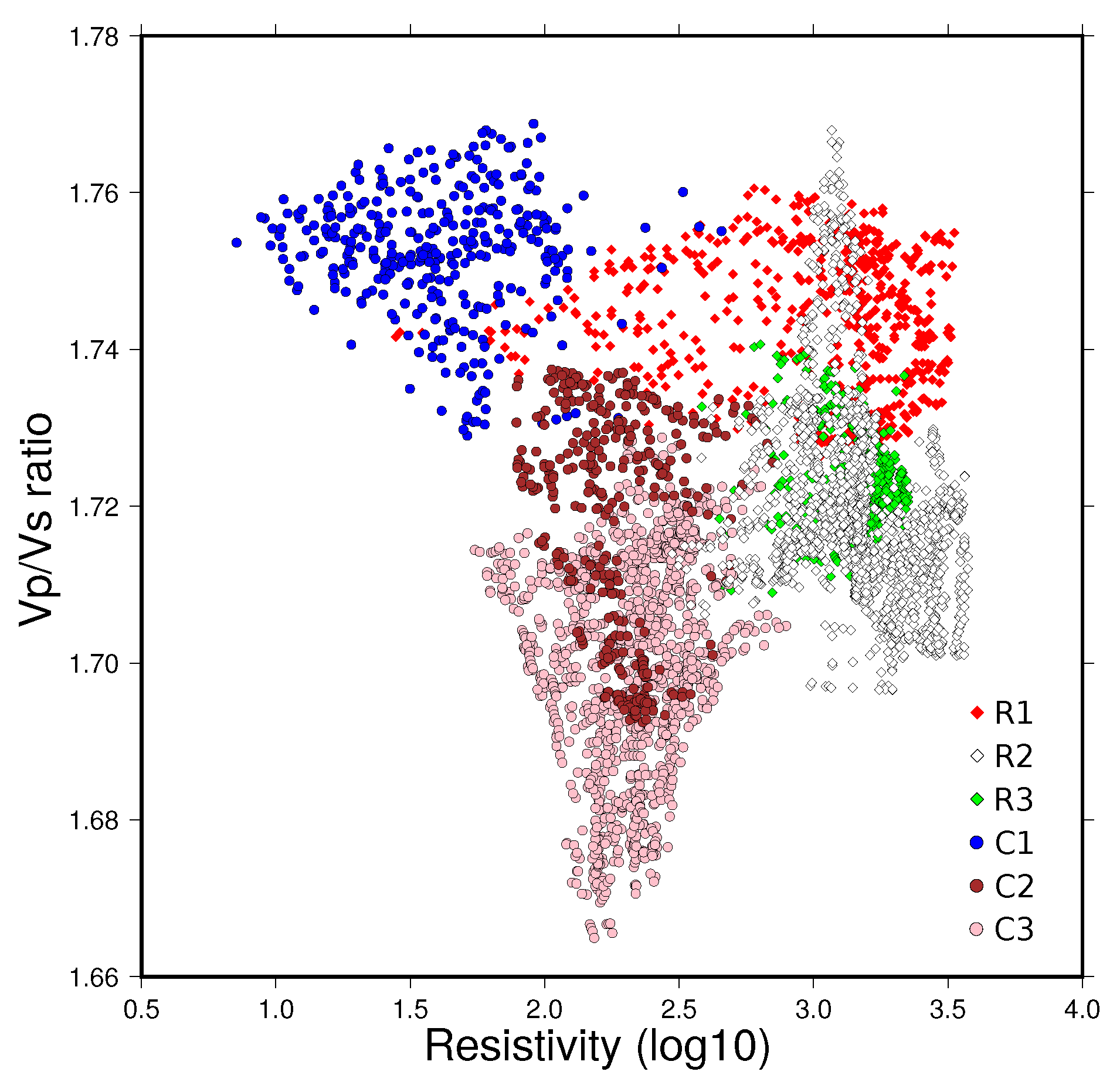
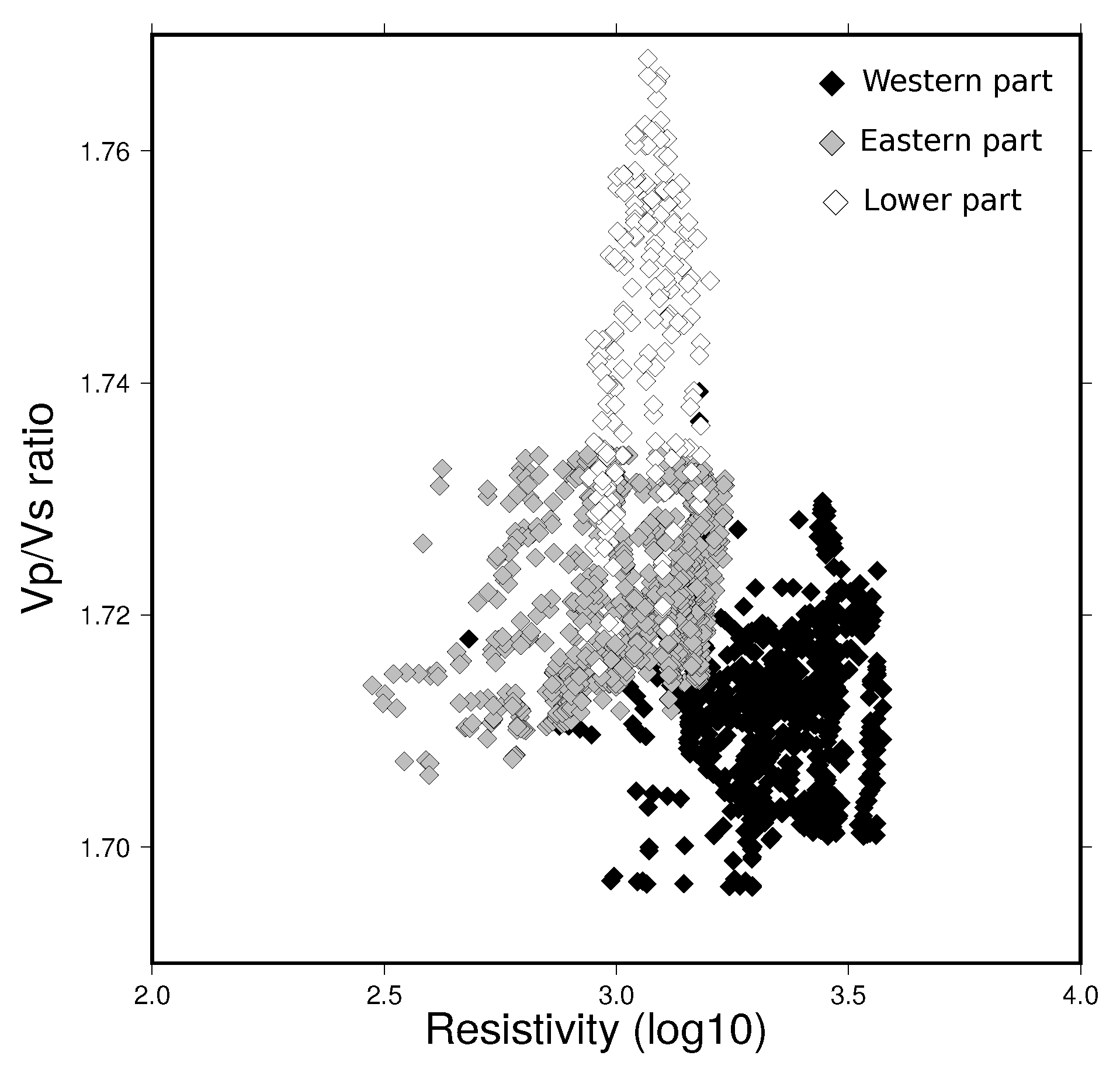
5. Results and Discussion
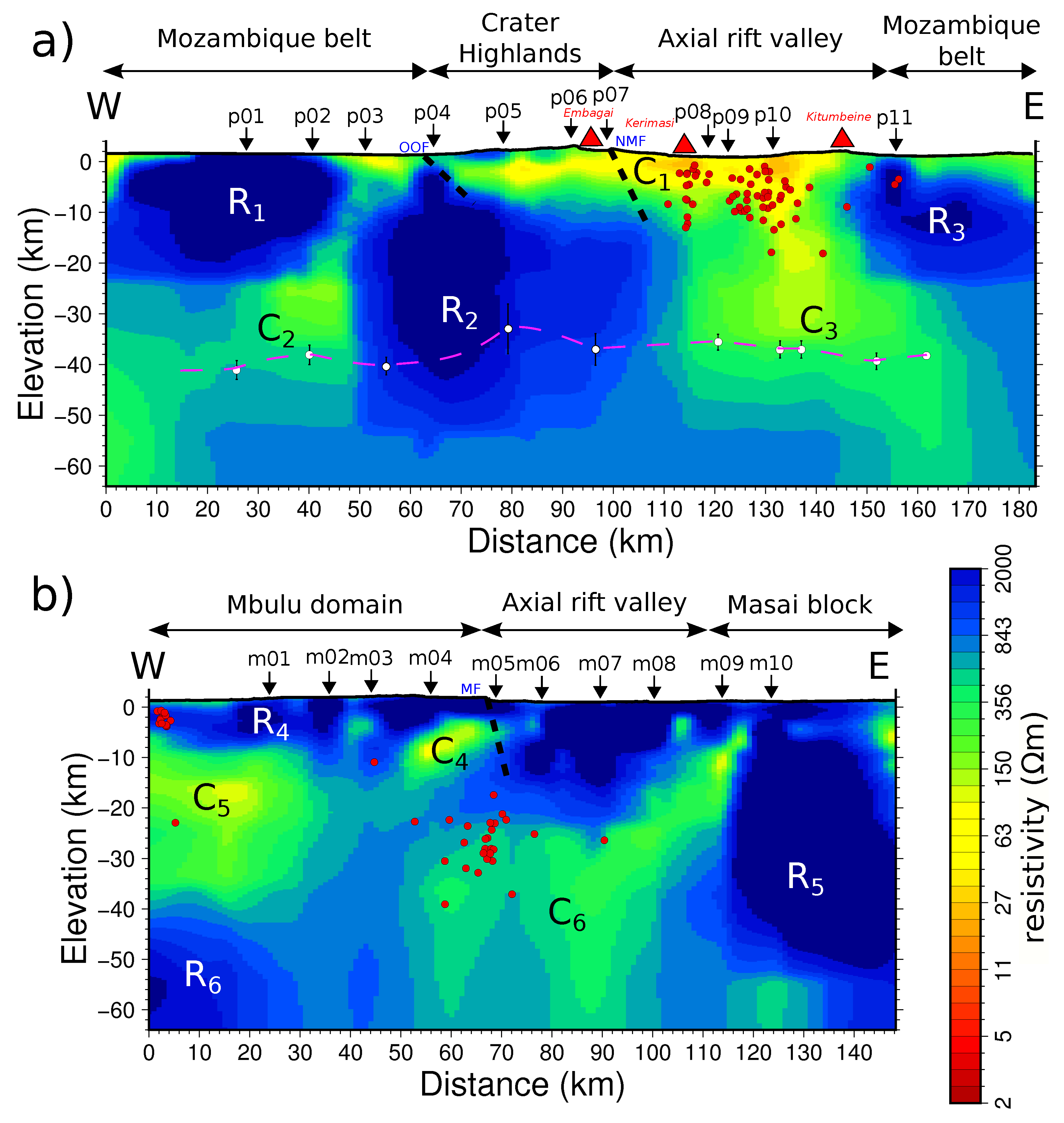
5.1. The Northern MT Profile
- -
- a well resolved shallow conductive layer (∼30 Ωm, Figure 3a and Figure A7) of about ∼5 km thick and localized between MT sites p05 and p11. We relate this structure to either a sediment layer representing axial infilling sequences [49,50] or a deep volcanic/sedimentary sequence beneath the Quaternary transverse volcanic belt (Figure 1A [7,31]). We favour the second interpretation in light of the extension of this conductive layer towards the Crater Highlands (through the Embagai and Kerimasi volcanoes, Figure 1 and Figure 3).
- -
- a large resistive structure located between p03 and p07 and observed from the surface down to ∼50 km depth. The SA (Figure A7) shows that only the lower bound of the resistivity is resolved which means that the structure can be more resistive than this value (∼2000 Ωm).
- -
- a shallow (0–∼20 km) resistor () located beneath the Mozambique belt (Figure 1) West of and with a resolved lower bound only for the resistivity value.
- -
- a narrow vertical conductive conduit which links the surface (p03) to a conductive structure located at 30 km depth beneath p02. The SA shows that this feature is resolved (Figure A7).
- -
- a large conductive body in the eastern part of the profile down to the Moho. Its lateral boundaries are well resolved. With depth (∼40 km) the eastern side becomes less resolved. The small variations in the central part of the structure are not well resolved either (∼25 km depth under p10, Figure A7). In contrast the transition between and this structure is well defined. Its width encompasses the whole axial rift valley (between p08–p11).
- -
- a shallow resistive structure located at the easternmost part of the profile (p11) which extends down to ∼25 km depth. Its resistivity value is only a lower bound (Figure A7).
5.2. The Southern MT Profile
- -
- a moderate conductive structure located between m04–m08, ranging from 25 to 60 km depth and encompassing the rift valley. Above this conductive feature, the resistivity considerably increases up to the surface. This transition is well resolved (Figure A8). The upper eastern part of is composed by a west-dipping geometry structure, well resolved, reaching ∼5 km depth under m09–m10 (Figure A8).
- -
- a 50 km-thick well resolved resistive unit () in the eastern side of the model, which correlates well with the surface expression of Masai block (Figure 1).
- -
- a 10 km thick resistive area () located on the top of the western part of the model (from m01 to m04) which corresponds to the surface expression of Mbulu domain.
- -
- the structure overlies a conductive body , which extends from 10 to 40 km depth beneath m01 and m02 sites.
- -
- a resistive structure is observed at about 50 km depth in the western corner of the profile. This feature is reasonably well resolved despite its location at the edge of the profile (m01, Figure A8).
- -
- the resistive central and shallow parts of the model (from the surface to 15 km depth) encloses a narrow conductive body between m04–m05 at ca. 10 km depth. From the SA results, a connection of this structure with the other conductive structures and seems possible.
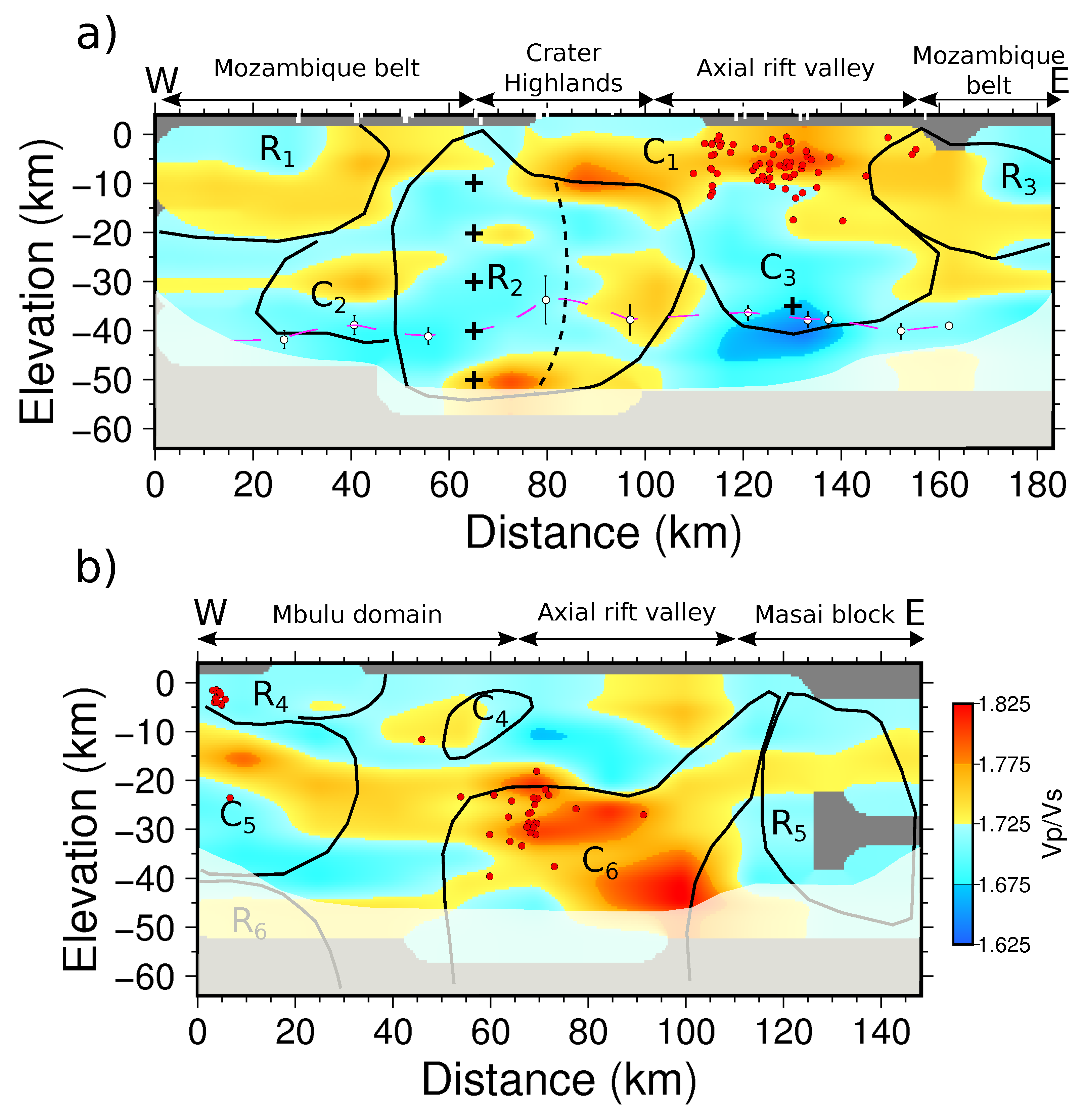
5.3. Comparison with Seismology
5.4. Presence of CO2?
5.5. Tectonic and Magmatism Interactions
5.6. Western Conductive Crustal Structure
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
Appendix A.1. Data
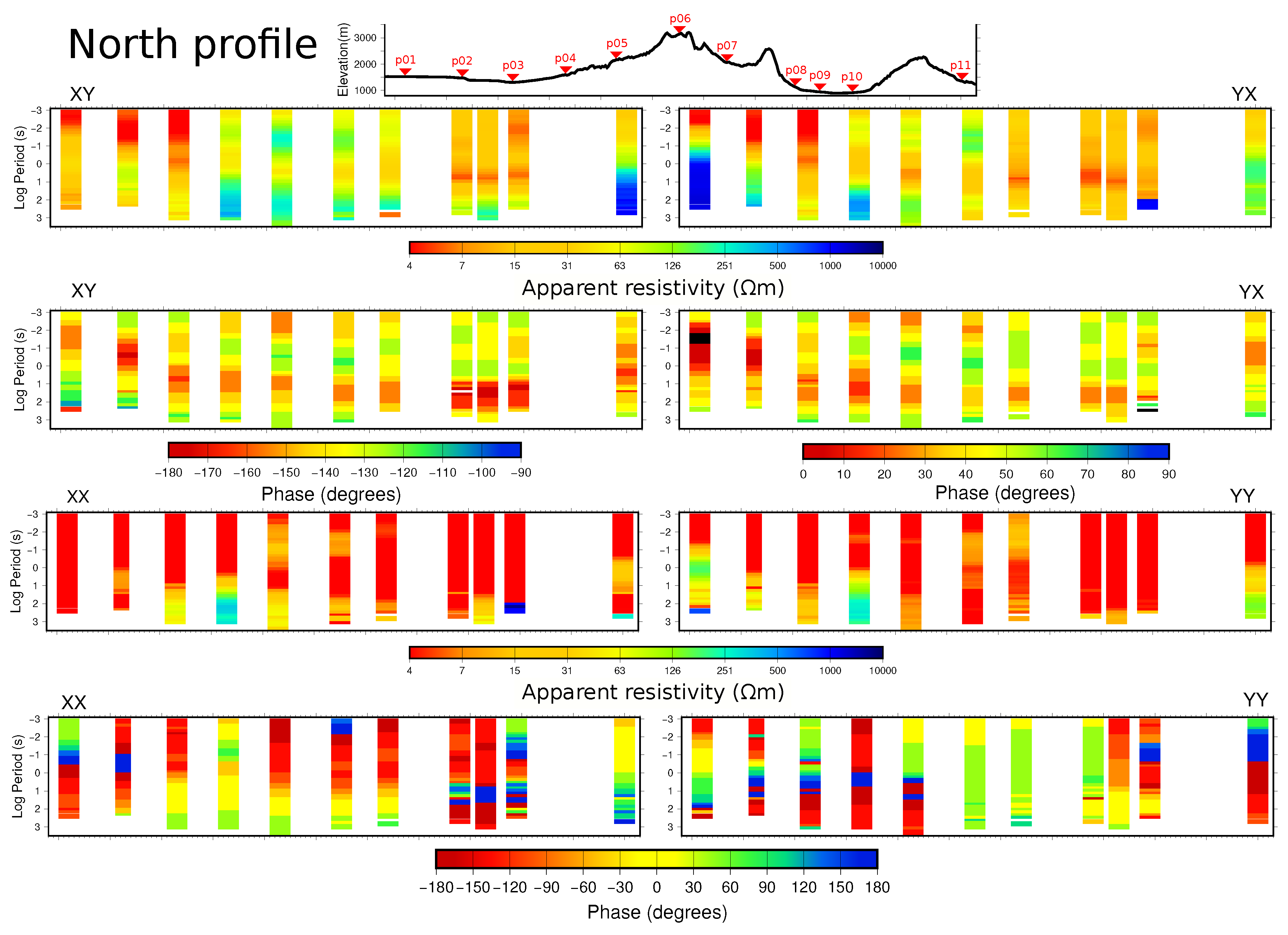

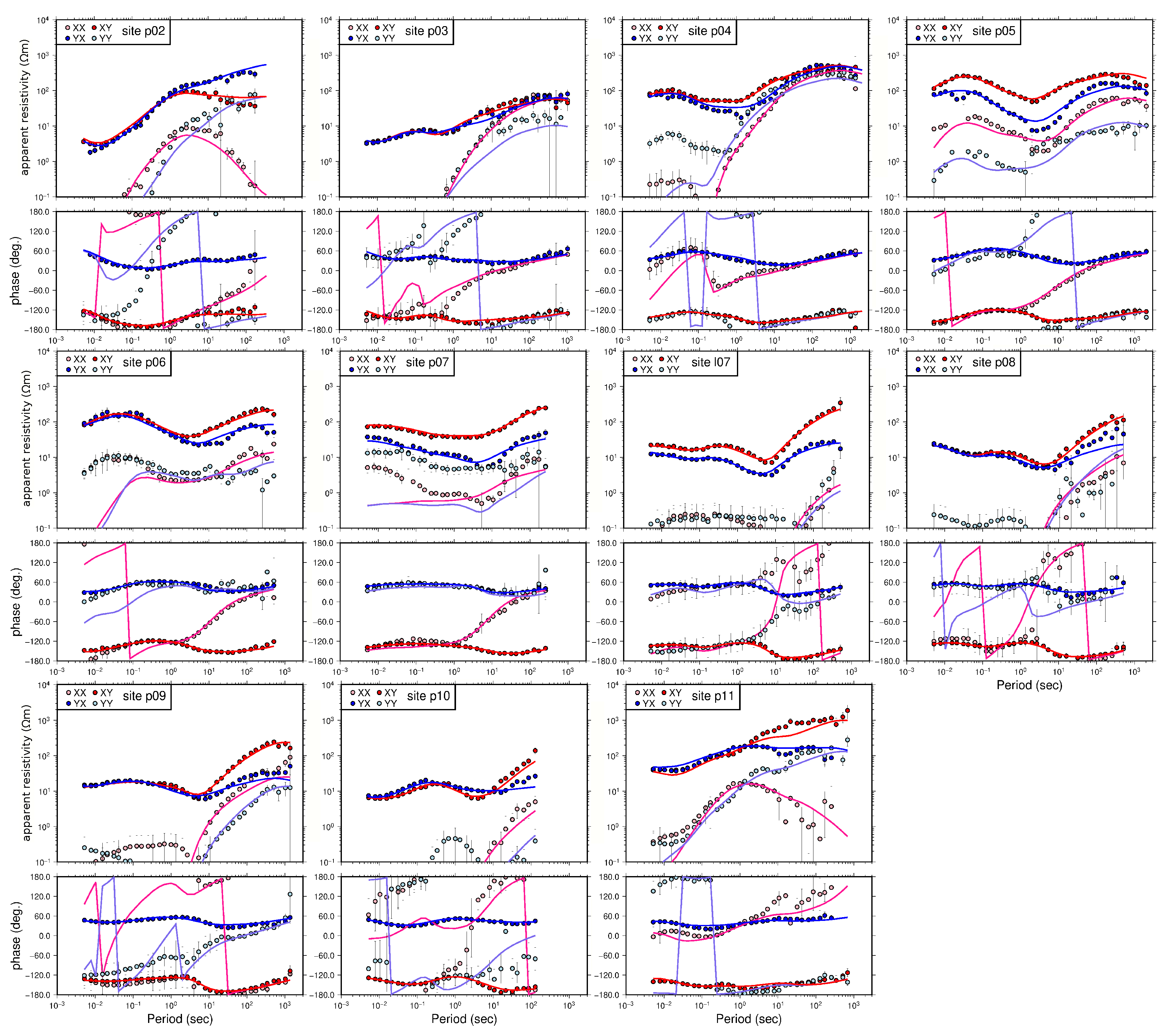
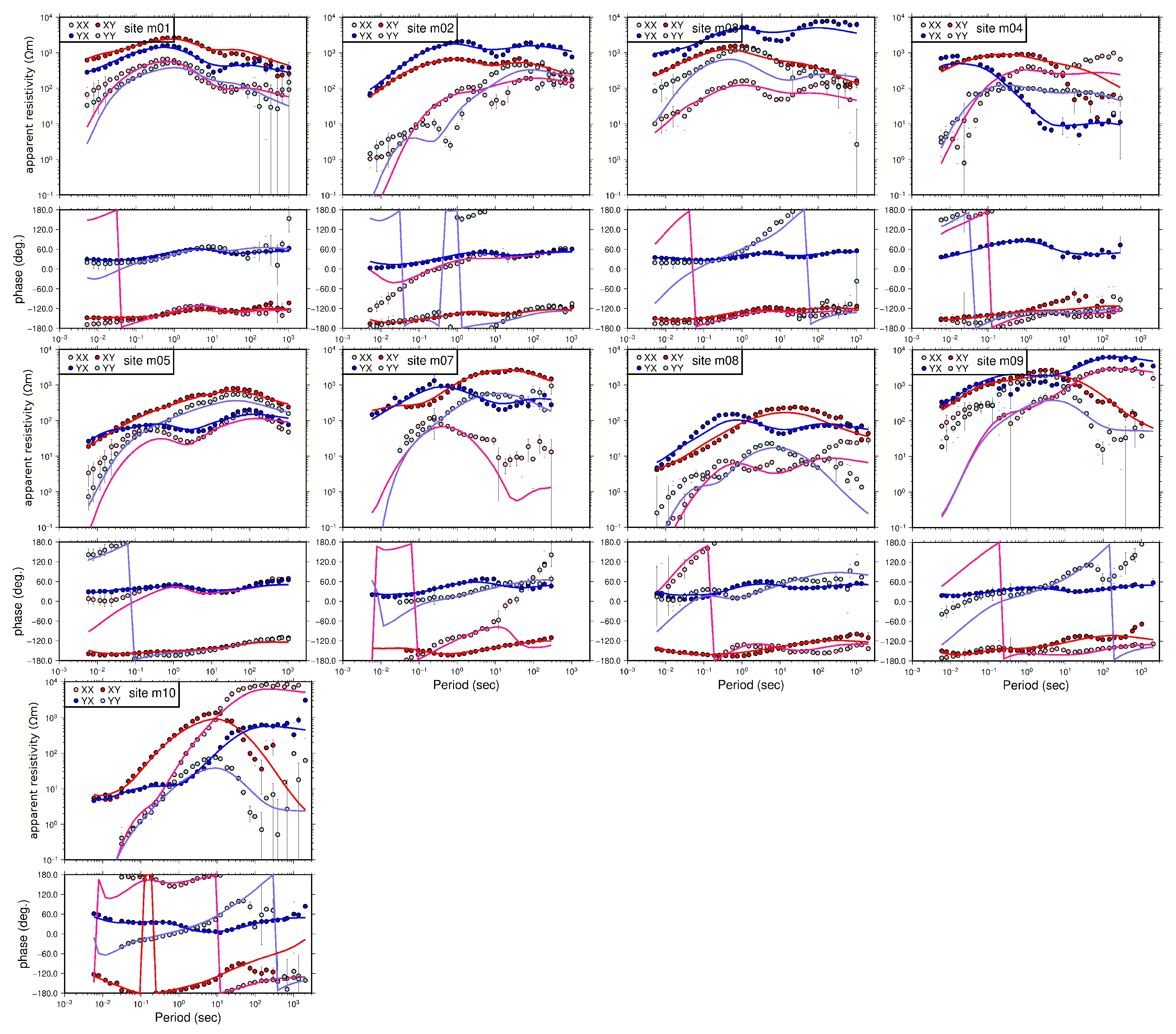
Appendix A.2. Maximum Electrical Direction
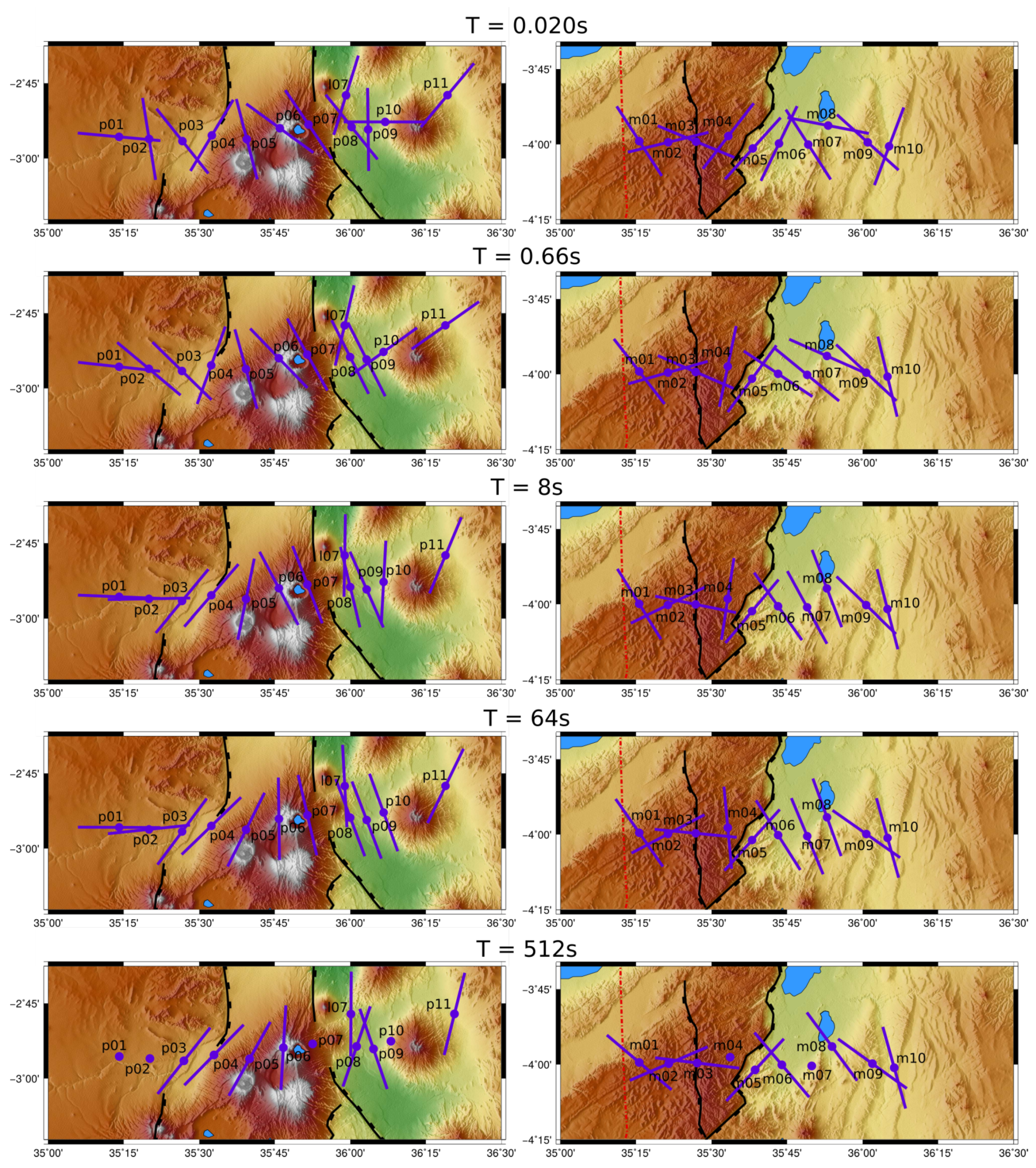
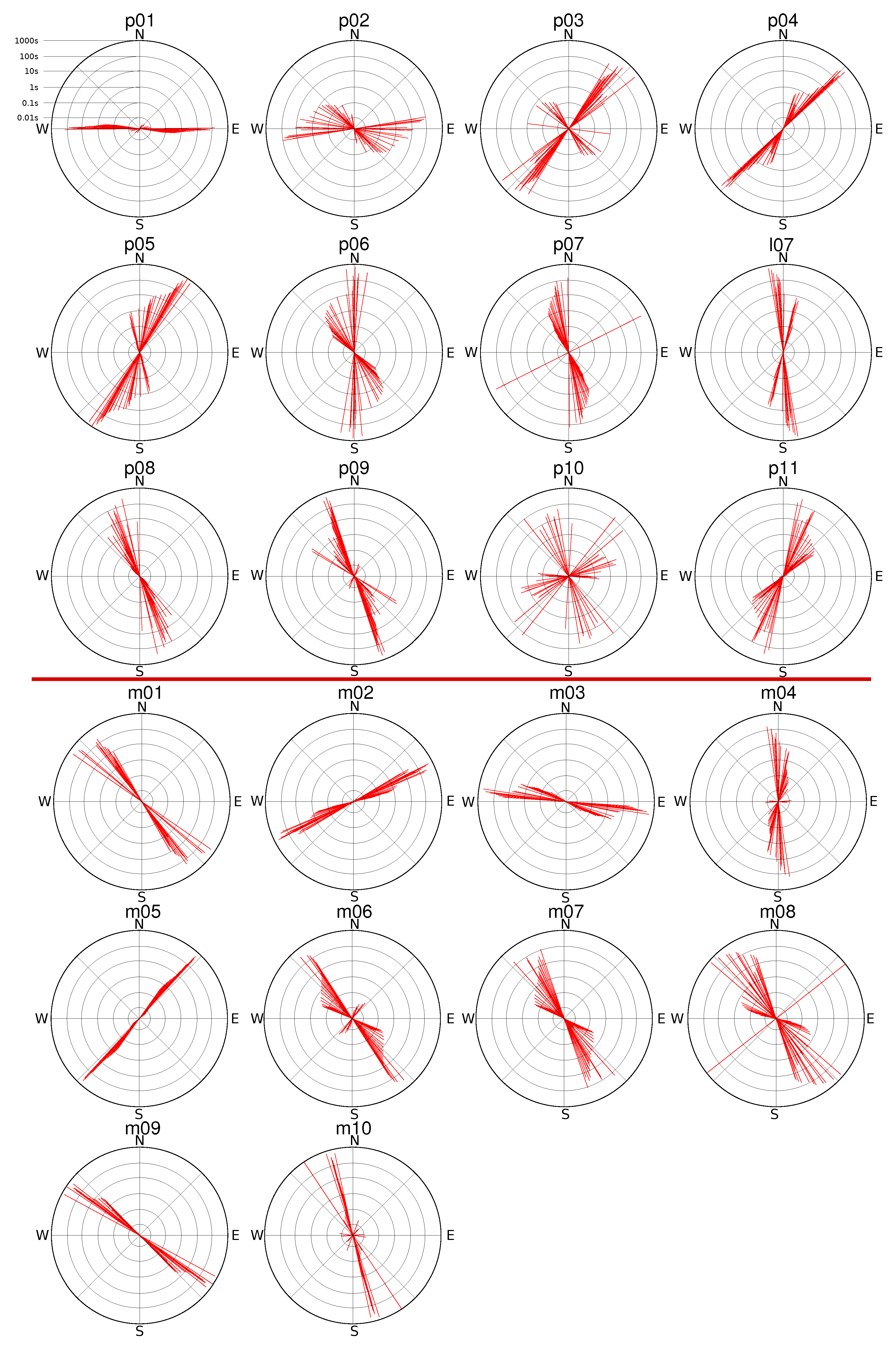
Appendix A.3. Sensitivity Analysis
Appendix A.3.1. Electrical Resistivity Model
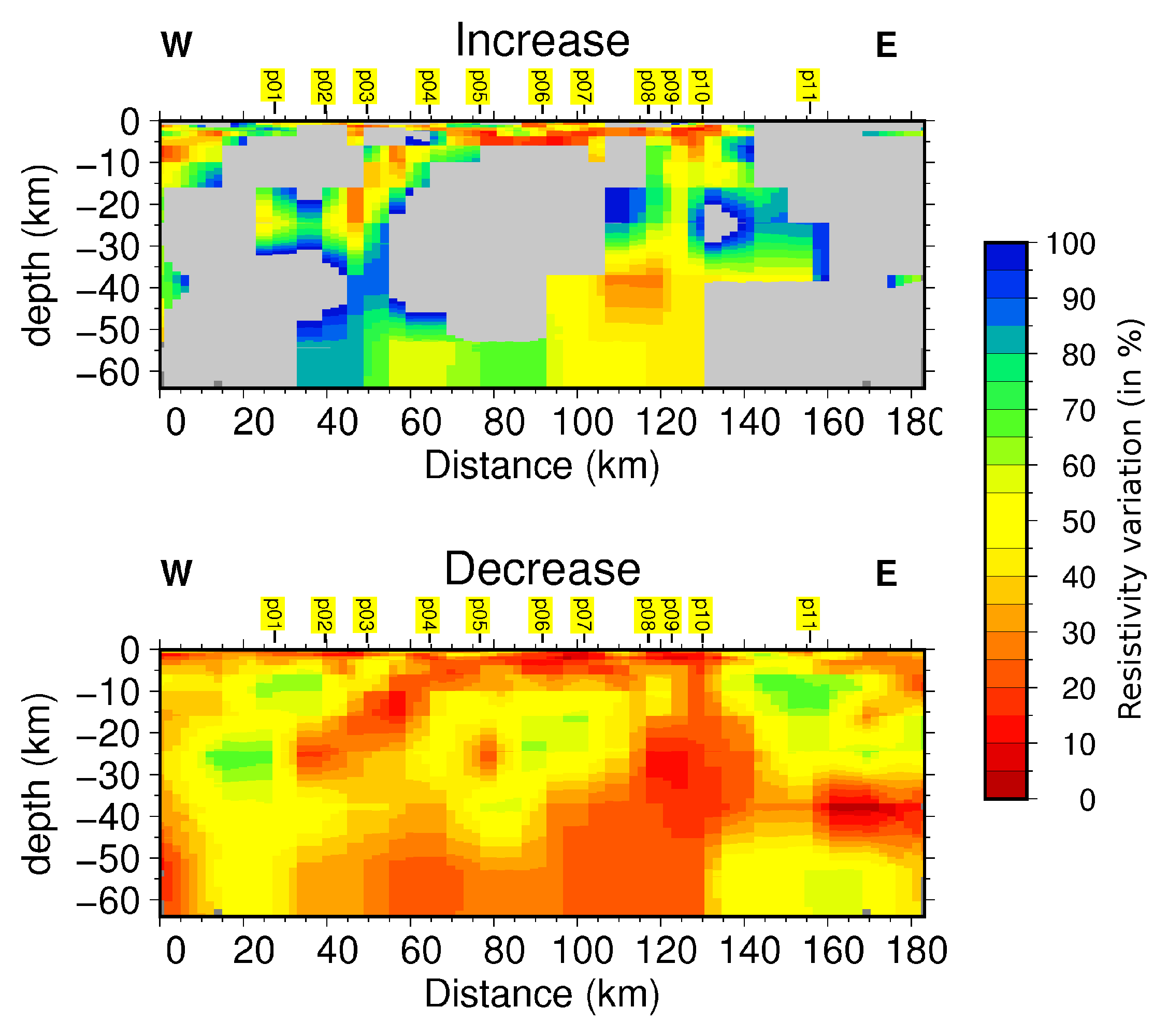
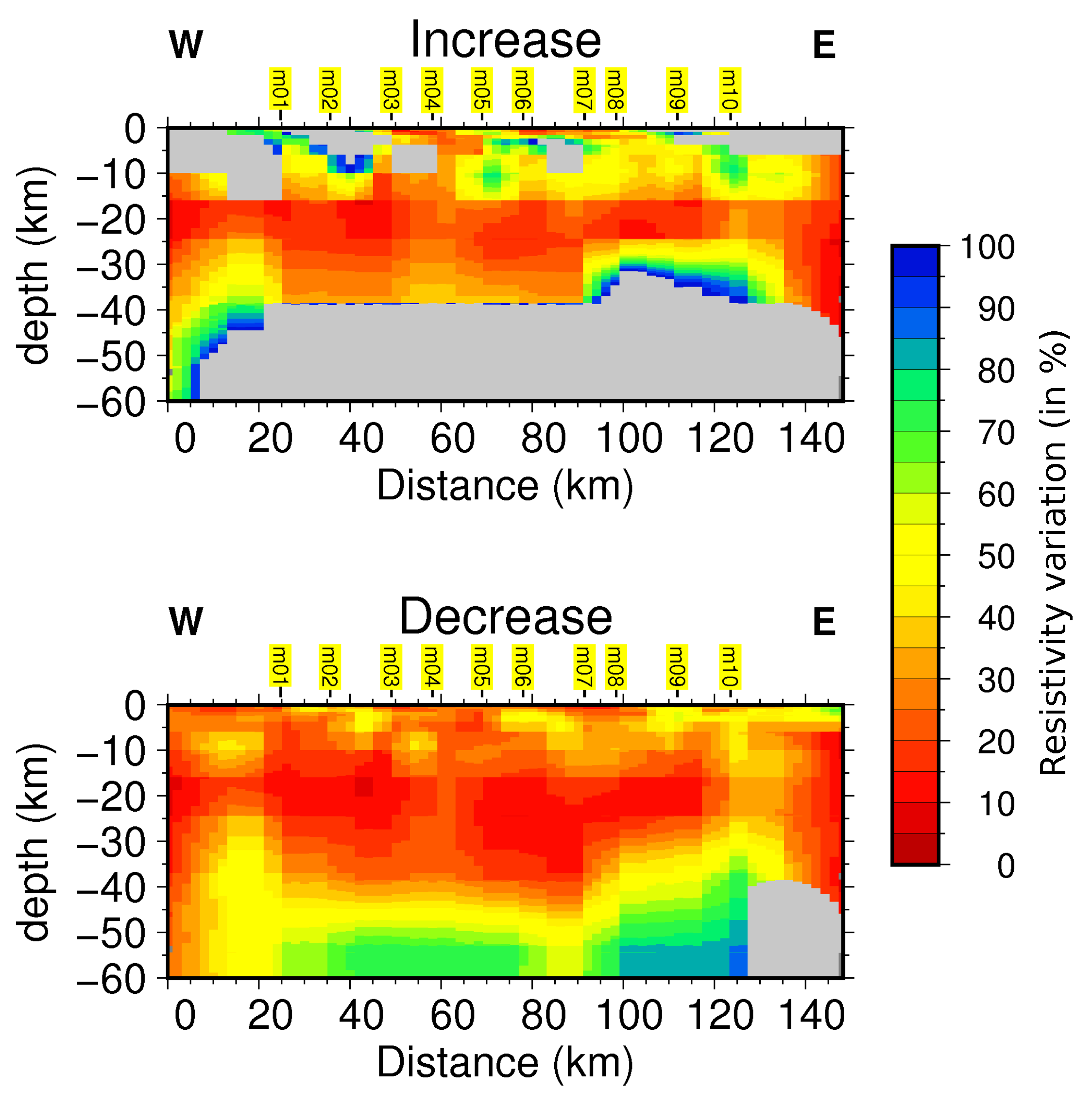
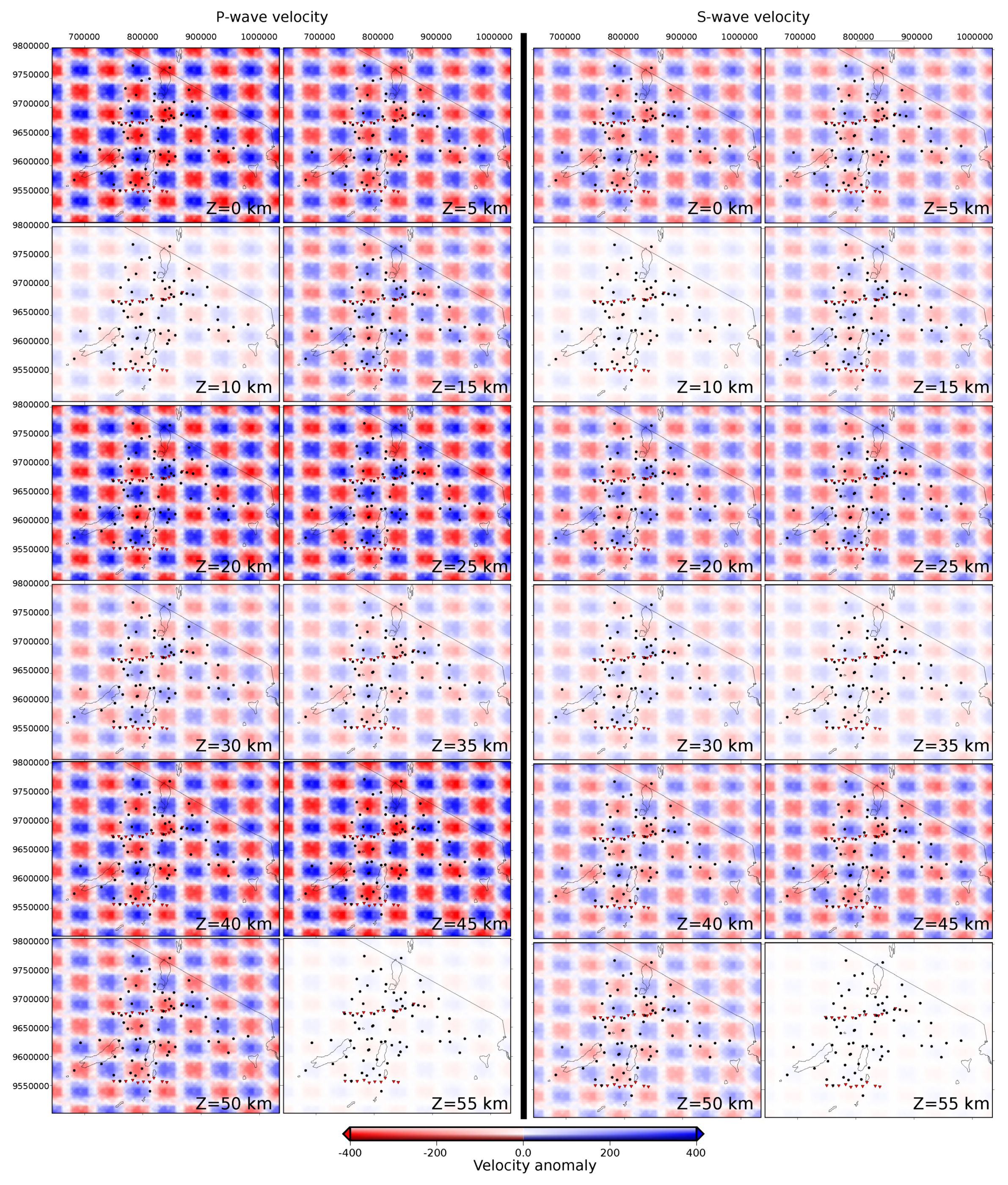
Appendix A.3.2. P- and S-Wave Velocity Models


References
- Prodehl, C.; Fuchs, K.; Mechie, J. Seismic-refraction studies of the Afro-Arabian rift system—A brief review. Tectonophysics 1997, 278, 1–13. [Google Scholar] [CrossRef]
- Corti, G. Continental rift evolution: From rift initiation to incipient break-up in the Main Ethiopian Rift, East Africa. Earth-Sci. Rev. 2009, 96, 1–53. [Google Scholar] [CrossRef]
- Koptev, A.; Calais, E.; Burov, E.; Leroy, S.; Gerya, T. Dual continental rift systems generated by plume-lithosphere interaction. Nat. Geosci. 2015, 8, 388–392. [Google Scholar] [CrossRef]
- Le Gall, B.; Nonnotte, P.; Rolet, J.; Benoit, M.; Guillou, H.; Mousseau-Nonnotte, M.; Albaric, J.; Deverchère, J. Rift propagation at craton margin: Distribution of faulting and volcanism in the North Tanzanian Divergence (East Africa) during Neogene times. Tectonophysics 2008, 448, 1–19. [Google Scholar] [CrossRef]
- Dawson, J. Neogene tectonics and volcanicity in the North Tanzania sector of the Gregory Rift Valley: Contrasts with the Kenya sector. Tectonophysics 1992, 204, 81–92. [Google Scholar] [CrossRef]
- Foster, A.; Ebinger, C.; Mbede, E.; Rex, D. Tectonic development of the northern Tanzanian sector of the East African Rift System. J. Geol. Soc. 1997, 154, 689–700. [Google Scholar] [CrossRef]
- Nonnotte, P.; Guillou, H.; Le Gall, B.; Benoit, M.; Cotten, J.; Scaillet, S. New K–Ar age determinations of Kilimanjaro volcano in the North Tanzanian diverging rift, East Africa. J. Volcanol. Geotherm. Res. 2008, 173, 99–112. [Google Scholar] [CrossRef]
- Ebinger, C.J.; van Wijk, J.; Keir, D. The time scales of continental rifting: Implications for global processes. Geol. Soc. Am. Spec. Pap. 2013, 500, 371–396. [Google Scholar]
- Muirhead, J.D.; Kattenhorn, S.A.; Le Corvec, N. Varying styles of magmatic strain accommodation across the East African Rift. Geochem. Geophys. Geosyst. 2015, 16, 2775–2795. [Google Scholar] [CrossRef]
- Lee, H.; Muirhead, J.D.; Fischer, T.P.; Ebinger, C.J.; Kattenhorn, S.A.; Sharp, Z.D.; Kianji, G. Massive and prolonged deep carbon emissions associated with continental rifting. Nat. Geosci. 2016, 9, 145. [Google Scholar] [CrossRef]
- Calais, E.; d’Oreye, N.; Albaric, J.; Deschamps, A.; Delvaux, D.; Déverchère, J.; Ebinger, C.; Ferdinand, R.W.; Kervyn, F.; Macheyeki, A.S.; et al. Strain accommodation by slow slip and dyking in a youthful continental rift, East Africa. Nature 2008, 456, 783–787. [Google Scholar] [CrossRef] [PubMed]
- Weinstein, A.; Oliva, S.; Ebinger, C.; Roecker, S.; Tiberi, C.; Aman, M.; Lambert, C.; Witkin, E.; Albaric, J.; Gautier, S.; et al. Fault-magma interactions during early continental rifting: Seismicity of the Magadi-Natron-Manyara basins, Africa. Geochem. Geophys. Geosyst. 2017, 18, 3662–3686. [Google Scholar] [CrossRef]
- Albaric, J.; Déverchère, J.; Perrot, J.; Jakovlev, A.; Deschamps, A. Deep crustal earthquakes in North Tanzania, East Africa: Interplay between tectonic and magmatic processes in an incipient rift. Geochem. Geophys. Geosyst. 2014, 15, 374–394. [Google Scholar] [CrossRef]
- Albaric, J.; Perrot, J.; Déverchère, J.; Deschamps, A.; Le Gall, B.; Ferdinand, R.; Petit, C.; Tiberi, C.; Sue, C.; Songo, M. Contrasted seismogenic and rheological behaviours from shallow and deep earthquake sequences in the North Tanzanian Divergence, East Africa. J. Afr. Earth Sci. 2010, 58, 799–811. [Google Scholar] [CrossRef]
- Gautier, S.; Plasman, M.; Tiberi, C.; Lopez, M.; Peyrat, S.; Perrot, J.; Albaric, J.; Déverchère, J.; Deschamps, A.; Ebinger, C.; et al. Interaction between an incipient rift and a cratonic lithosphere: The North Tanzania Rift seen from some seismic tools. In Proceedings of the EGU General Assembly Conference Abstracts, Vienna, Austria, 17–22 April 2016; Volume 18, p. 8935. [Google Scholar]
- Rooney, D.; Hutton, V. A magnetotelluric and magnetovariational study of the Gregory Rift Valley, Kenya. Geophys. J. Int. 1977, 51, 91–119. [Google Scholar] [CrossRef]
- Sakkas, V.; Meju, M.A.; Khan, M.A.; Haak, V.; Simpson, F. Magnetotelluric images of the crustal structure of Chyulu Hills volcanic field, Kenya. Tectonophysics 2002, 346, 169–185. [Google Scholar] [CrossRef]
- Meju, M.A.; Sakkas, V. Heterogeneous crust and upper mantle across southern Kenya and the relationship to surface deformation as inferred from magnetotelluric imaging. J. Geophys. Res. Solid Earth 2007, 112. [Google Scholar] [CrossRef]
- Samrock, F.; Grayver, A.V.; Eysteinsson, H.; Saar, M.O. Magnetotelluric image of transcrustal magmatic system beneath the Tulu Moye geothermal prospect in the Ethiopian Rift. Geophys. Res. Lett. 2018, 45, 12–847. [Google Scholar] [CrossRef]
- Simpson, F.; Bahr, K. Practical Magnetotellurics; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Calais, E.; Ebinger, C.; Hartnady, C.; Nocquet, J. Kinematics of the East African Rift from GPS and earthquake slip vector data. Geolog. Soc. Lond. Spec. Publ. 2006, 259, 9–22. [Google Scholar] [CrossRef]
- McConnell, R. Geological development of the rift system of eastern Africa. Geol. Soc. Am. Bull. 1972, 83, 2549–2572. [Google Scholar] [CrossRef]
- Smith, M.; Mosley, P. Crustal heterogeneity and basement influence on the development of the Kenya Rift, East Africa. Tectonics 1993, 12, 591–606. [Google Scholar] [CrossRef]
- Simiyu, S.M.; Keller, G.R. An integrated analysis of lithospheric structure across the East African plateau based on gravity anomalies and recent seismic studies. Tectonophysics 1997, 278, 291–313. [Google Scholar] [CrossRef]
- Bagley, B.; Nyblade, A.A. Seismic anisotropy in eastern Africa, mantle flow, and the African superplume. Geophys. Res. Lett. 2013, 40, 1500–1505. [Google Scholar] [CrossRef]
- Koptev, A.; Burov, E.; Calais, E.; Leroy, S.; Gerya, T.; Guillou-Frottier, L.; Cloetingh, S. Contrasted continental rifting via plume-craton interaction: Applications to Central East African Rift. Geosci. Front. 2016, 7, 221–236. [Google Scholar] [CrossRef]
- Koptev, A.; Burov, E.; Gerya, T.; Le Pourhiet, L.; Leroy, S.; Calais, E.; Jolivet, L. Plume-induced continental rifting and break-up in ultra-slow extension context: Insights from 3D numerical modeling. Tectonophysics 2018, 746, 121–137. [Google Scholar] [CrossRef]
- Baker, B.T.; Wohlenberg, J. Structure and evolution of the Kenya Rift Valley. Nature 1971, 229, 538–542. [Google Scholar] [CrossRef] [PubMed]
- Fairhead, J.; Mitchell, J.G.; Williams, L. New K/Ar determinations on rift volcanics of S. Kenya and their bearing on age of rift faulting. Nature 1972, 238, 66–69. [Google Scholar] [CrossRef]
- Evans, A.L.; Fairhead, J.; Mitchell, J. Potassium—Argon ages from the volcanic province of Northern Tanzania. Nat. Phys. Sci. 1971, 229, 19. [Google Scholar] [CrossRef]
- Mana, S.; Furman, T.; Turrin, B.D.; Feigenson, M.D.; Swisher, C.C. Magmatic activity across the East African North Tanzanian Divergence Zone. J. Geol. Soc. 2015, 172, 368–389. [Google Scholar] [CrossRef]
- Baer, G.; Hamiel, Y.; Shamir, G.; Nof, R. Evolution of a magma-driven earthquake swarm and triggering of the nearby Oldoinyo Lengai eruption, as resolved by InSAR, ground observations and elastic modeling, East African Rift, 2007. Earth Planet. Sci. Lett. 2008, 272, 339–352. [Google Scholar] [CrossRef]
- Last, R.J.; Nyblade, A.A.; Langston, C.A.; Owens, T.J. Crustal structure of the East African Plateau from receiver functions and Rayleigh wave phase velocities. J. Geophys. Res. Solid Earth 1997, 102, 24469–24483. [Google Scholar] [CrossRef]
- Dugda, M.T.; Nyblade, A.A.; Julia, J.; Langston, C.A.; Ammon, C.J.; Simiyu, S. Crustal structure in Ethiopia and Kenya from receiver function analysis: Implications for rift development in eastern Africa. J. Geophys. Res. Solid Earth 2005, 110. [Google Scholar] [CrossRef]
- Plasman, M.; Tiberi, C.; Ebinger, C.; Gautier, S.; Albaric, J.; Peyrat, S.; Déverchère, J.; Le Gall, B.; Tarits, P.; Roecker, S.; et al. Lithospheric low-velocity zones associated with a magmatic segment of the Tanzanian Rift, East Africa. Geophys. J. Int. 2017, 210, 465–481. [Google Scholar] [CrossRef]
- Roecker, S.; Ebinger, C.; Tiberi, C.; Mulibo, G.; Ferdinand-Wambura, R.; Mtelela, K.; Kianji, G.; Muzuka, A.; Gautier, S.; Albaric, J.; et al. Subsurface Images of the Eastern Rift, Africa, from the Joint Inversion of Body Waves, Surface Waves, and Gravity: Investigating the Role of Fluids in Early-Stage Continental Rifting. Geophys. J. Int. 2017, 210, 931–950. [Google Scholar] [CrossRef]
- Albaric, J.; Déverchère, J.; Petit, C.; Perrot, J.; Le Gall, B. Crustal rheology and depth distribution of earthquakes: Insights from the central and southern East African Rift System. Tectonophysics 2009, 468, 28–41. [Google Scholar] [CrossRef]
- Mulibo, G.; Nyblade, A. The 1994–1995 Manyara and Kwamtoro earthquake swarms: Variation in the depth extent of seismicity in Northern Tanzania. S. Afr. J.Geol. 2009, 112, 387–404. [Google Scholar] [CrossRef]
- Craig, T.; Jackson, J.; Priestley, K.; McKenzie, D. Earthquake distribution patterns in Africa: Their relationship to variations in lithospheric and geological structure, and their rheological implications. Geophys. J. Int. 2011, 185, 403–434. [Google Scholar] [CrossRef]
- Chave, A.D.; Thomson, D.J. Bounded influence magnetotelluric response function estimation. Geophys. J. Int. 2004, 157, 988–1006. [Google Scholar] [CrossRef]
- Counil, J.; Le Mouel, J.; Menvielle, M. Associate and conjugate directions concepts in magnetotellurics. Ann. Geophys. 1986, 4, 115–130. [Google Scholar]
- Siripunvaraporn, W.; Egbert, G.; Lenbury, Y.; Uyeshima, M. Three-dimensional magnetotelluric inversion: Data-space method. Phys. Earth Planet. Inter. 2005, 150, 3–14. [Google Scholar] [CrossRef]
- Hautot, S.; Tarits, P. 3-D magnetotelluric inversion of 2-D profiles: Application to land and Marine data for shallow crustal investigation. ASEG Ext. Abstr. 2010, 2010, 1–4. [Google Scholar] [CrossRef]
- Hautot, S.; Tarits, P.; Whaler, K.; Le Gall, B.; Tiercelin, J.J.; Le Turdu, C. Deep structure of the Baringo Rift Basin (central Kenya) from three-dimensional magnetotelluric imaging: Implications for rift evolution. J. Geophys. Res. Solid Earth 2000, 105, 23493–23518. [Google Scholar] [CrossRef]
- Hautot, S.; Single, R.; Watson, J.; Harrop, N.; Jerram, D.; Tarits, P.; Whaler, K.; Dawes, D. 3-D magnetotelluric inversion and model validation with gravity data for the investigation of flood basalts and associated volcanic rifted margins. Geophys. J. Int. 2007, 170, 1418–1430. [Google Scholar] [CrossRef]
- Rosenbrock, H. An automatic method for finding the greatest or least value of a function. Comput. J. 1960, 3, 175–184. [Google Scholar] [CrossRef]
- Patro, P.K.; Egbert, G.D. Application of 3D inversion to magnetotelluric profile data from the Deccan Volcanic Province of Western India. Phys. Earth Planet. Inter. 2011, 187, 33–46. [Google Scholar] [CrossRef]
- Gao, J.; Zhang, H.; Zhang, S.; Chen, X.; Cheng, Z.; Jia, X.; Li, S.; Fu, L.; Gao, L.; Xin, H. Three-dimensional magnetotelluric imaging of the geothermal system beneath the Gonghe Basin, Northeast Tibetan Plateau. Geothermics 2018, 76, 15–25. [Google Scholar] [CrossRef]
- Birt, C.; Maguire, P.; Khan, M.; Thybo, H.; Keller, G.R.; Patel, J. The influence of pre-existing structures on the evolution of the southern Kenya Rift Valley—Evidence from seismic and gravity studies. Tectonophysics 1997, 278, 211–242. [Google Scholar] [CrossRef]
- Ebinger, C.; Djomani, Y.P.; Mbede, E.; Foster, A.; Dawson, J. Rifting Archaean lithosphere: The Eyasi-Manyara- Natron rifts, East Africa. J. Geol. Soc. 1997, 154, 947–960. [Google Scholar] [CrossRef]
- Selway, K. Negligible effect of hydrogen content on plate strength in East Africa. Nat. Geosci. 2015, 8, 543–546. [Google Scholar] [CrossRef]
- Mulibo, G.D.; Nyblade, A.A. The P and S wave velocity structure of the mantle beneath eastern Africa and the African superplume anomaly. Geochem. Geophys. Geosyst. 2013, 14, 2696–2715. [Google Scholar] [CrossRef]
- Macheyeki, A.S.; Delvaux, D.; De Batist, M.; Mruma, A. Fault kinematics and tectonic stress in the seismically active Manyara–Dodoma Rift segment in Central Tanzania–Implications for the East African Rift. J. Afr. Earth Sci. 2008, 51, 163–188. [Google Scholar] [CrossRef]
- Gaillard, F.; Malki, M.; Iacono-Marziano, G.; Pichavant, M.; Scaillet, B. Carbonatite melts and electrical conductivity in the asthenosphere. Science 2008, 322, 1363–1365. [Google Scholar] [CrossRef] [PubMed]
- Sifré, D.; Gardés, E.; Massuyeau, M.; Hashim, L.; Hier-Majumder, S.; Gaillard, F. Electrical conductivity during incipient melting in the oceanic low-velocity zone. Nature 2014, 509, 81–85. [Google Scholar] [CrossRef]
- Tiberi, C.; Gautier, S.; Ebinger, C.; Roecker, S.; Plasman, M.; Albaric, J.; Déverchère, J.; Peyrat, S.; Perrot, J.; Wambura, R.F.; et al. Lithospheric modification by extension and magmatism at the craton-orogenic boundary: North Tanzania Divergence, East Africa. Geophys. J. Int. 2018, 216, 1693–1710. [Google Scholar] [CrossRef]
- Marquis, G.; Hyndman, R.D. Geophysical support for aqueous fluids in the deep crust: Seismic and electrical relationships. Geophys. J. Int. 1992, 110, 91–105. [Google Scholar] [CrossRef]
- Meju, M.A.; Gallardo, L.A.; Mohamed, A.K. Evidence for correlation of electrical resistivity and seismic velocity in heterogeneous near-surface materials. Geophys. Res. Lett. 2003, 30. [Google Scholar] [CrossRef]
- Podvin, P.; Lecomte, I. Finite difference computation of traveltimes in very contrasted velocity models: A massively parallel approach and its associated tools. Geophys. J. Int. 1991, 105, 271–284. [Google Scholar] [CrossRef]
- Tatham, R.H. V p/V s and lithology. Geophysics 1982, 47, 336–344. [Google Scholar] [CrossRef]
- Domenico, S.N. Rock lithology and porosity determination from shear and compressional wave velocity. Geophysics 1984, 49, 1188–1195. [Google Scholar] [CrossRef]
- Christensen, N.I. Poisson’s ratio and crustal seismology. J. Geophys. Res. Solid Earth 1996, 101, 3139–3156. [Google Scholar] [CrossRef]
- Watanabe, T. Effects of water and melt on seismic velocities and their application to characterization of seismic reflectors. Geophys. Res. Lett. 1993, 20, 2933–2936. [Google Scholar] [CrossRef]
- Rojas, E.; Davis, T.L.; Batzle, M.; Prasad, M.; Michelena, R.J. V p-V s ratio sensitivity to pressure, fluid, and lithology changes in tight gas sandstones. In SEG Technical Program Expanded Abstracts 2005; Society of Exploration Geophysicists: Tulsa, OK, USA, 2005; pp. 1401–1404. [Google Scholar]
- Kim, J.; Matsuoka, T.; Xue, Z. Monitoring and detecting CO2 injected into water-saturated sandstone with joint seismic and resistivity measurements. Explor. Geophys. 2011, 42, 58–68. [Google Scholar] [CrossRef]
- Riedel, M.; Collett, T.S.; Kim, H.S.; Bahk, J.J.; Kim, J.H.; Ryu, B.J.; Kim, G. Large-scale depositional characteristics of the Ulleung Basin and its impact on electrical resistivity and Archie-parameters for gas hydrate saturation estimates. Mar. Pet. Geol. 2013, 47, 222–235. [Google Scholar] [CrossRef]
- Wang, Z.; Nur, A.M. Effects of CO2 flooding on wave velocities in rocks with hydrocarbons. SPE Reserv. Eng. 1989, 4, 429–436. [Google Scholar] [CrossRef]
- Mavko, G.; Chan, C.; Mukerji, T. Fluid substitution: Estimating changes in Vp without knowing Vs. Geophysics 1995, 60, 1750–1755. [Google Scholar] [CrossRef]
- Harris, J.M.; Langan, R.T.; Fasnacht, T.; Melton, D.; Smith, B.; Sinton, J.; Tan, H. Experimental verification of seismic monitoring of CO2 injection in carbonate reservoirs. In SEG Technical Program Expanded Abstracts 1996; Society of Exploration Geophysicists: Tulsa, OK, USA, 1996; pp. 1870–1872. [Google Scholar]
- Krief, M.; Garat, J.; Stellingwerff, J.; Ventre, J. A petrophysical interpretation using the velocities of P and S waves (full-waveform sonic). Log Anal. 1990, 31. Available online: https://www.onepetro.org/journal-paper/SPWLA-1990-v31n6a2 (accessed on 8 September 2019).
- Foulger, G.; Julian, B.; Pitt, A.; Hill, D.; Malin, P.; Shalev, E. Three-dimensional crustal structure of Long Valley caldera, California, and evidence for the migration of CO2 under Mammoth Mountain. J. Geophys. Res. Solid Earth 2003, 108. [Google Scholar] [CrossRef]
- Fleury, M.; Deschamps, H. Electrical conductivity and viscosity of aqueous NaCl solutions with dissolved CO2. J. Chem. Eng. Data 2008, 53, 2505–2509. [Google Scholar] [CrossRef]
- Schmidt-Hattenberger, C.; Bergmann, P.; Kießling, D.; Krüger, K.; Rücker, C.; Schütt, H.; Group, K. Application of a Vertical Electrical Resistivity Array (VERA) for monitoring CO2 migration at the Ketzin site: First performance evaluation. Energy Procedia 2011, 4, 3363–3370. [Google Scholar] [CrossRef]
- Dawson, J.; Pinkerton, H.; Norton, G.; Pyle, D. Physicochemical properties of alkali carbonatite lavas: Data from the 1988 eruption of Oldoinyo Lengai, Tanzania. Geology 1990, 18, 260–263. [Google Scholar] [CrossRef]
- Julià, J.; Ammon, C.J.; Nyblade, A.A. Evidence for mafic lower crust in Tanzania, East Africa, from joint inversion of receiver functions and Rayleigh wave dispersion velocities. Geophys. J. Int. 2005, 162, 555–569. [Google Scholar] [CrossRef]
- Hammond, J.; Kendall, J.M.; Wookey, J.; Stuart, G.; Keir, D.; Ayele, A. Differentiating flow, melt, or fossil seismic anisotropy beneath Ethiopia. Geochem. Geophys. Geosyst. 2014, 15, 1878–1894. [Google Scholar] [CrossRef]
- MacIntyre, R. Age of fault movements in Tanzanian sector of East African Rift System. Nature 1974, 247, 354–356. [Google Scholar] [CrossRef]
- Baudouin, C.; Parat, F.; Michel, T. CO2-rich phonolitic melt and carbonatite immiscibility in early stage of rifting: Melt inclusions from Hanang volcano (Tanzania). J. Volcanol. Geotherm. Res. 2018, 358, 261–272. [Google Scholar] [CrossRef]
- Archie, G.E. The electrical resistivity log as an aid in determining some reservoir characteristics. Trans. AIME 1942, 146, 54–62. [Google Scholar] [CrossRef]
- Alemu, B.L.; Aker, E.; Soldal, M.; Johnsen, Ø.; Aagaard, P. Effect of sub-core scale heterogeneities on acoustic and electrical properties of a reservoir rock: A CO2 flooding experiment of brine saturated sandstone in a computed tomography scanner. Geophys. Prospect. 2013, 61, 235–250. [Google Scholar] [CrossRef]
- Baudoin, C. Volcanisme Alcalin à L’initiation de la Rupture Continentale. Rift Est Africain, Nord Tanzanie, Bassin de Manyara. Ph.D. Thesis, Université de Montpellier, Montpellier, France, 2016. [Google Scholar]
- Spratt, J.E.; Jones, A.G.; Jackson, V.A.; Collins, L.; Avdeeva, A. Lithospheric geometry of the Wopmay orogen from a Slave craton to Bear Province magnetotelluric transect. J. Geophys. Res. Solid Earth 2009, 114. [Google Scholar] [CrossRef]
- Miensopust, M.P.; Jones, A.G.; Muller, M.R.; Garcia, X.; Evans, R.L. Lithospheric structures and Precambrian terrane boundaries in northeastern Botswana revealed through magnetotelluric profiling as part of the Southern African Magnetotelluric Experiment. J. Geophys. Res. Solid Earth 2011, 116. [Google Scholar] [CrossRef]
- Sarafian, E.; Evans, R.L.; Abdelsalam, M.G.; Atekwana, E.; Elsenbeck, J.; Jones, A.G.; Chikambwe, E. Imaging Precambrian lithospheric structure in Zambia using electromagnetic methods. Gondwana Res. 2018, 54, 38–49. [Google Scholar] [CrossRef]
- Khoza, D.; Jones, A.; Muller, M.; Evans, R.; Webb, S.; Miensopust, M. Tectonic model of the Limpopo belt: Constraints from magnetotelluric data. Precambrian Res. 2013, 226, 143–156. [Google Scholar] [CrossRef]
- Ritter, O.; Weckmann, U.; Vietor, T.; Haak, V. A magnetotelluric study of the Damara Belt in Namibia: 1. Regional scale conductivity anomalies. Phys. Earth Planet. Inter. 2003, 138, 71–90. [Google Scholar] [CrossRef]
- Santos, F.A.M.; Mateus, A.; Almeida, E.P.; Pous, J.; Mendes-Victor, L.A. Are some of the deep crustal conductive features found in SW Iberia caused by graphite? Earth Planet. Sci. Lett. 2002, 201, 353–367. [Google Scholar] [CrossRef]
- Ogawa, Y.; Honkura, Y. Mid-crustal electrical conductors and their correlations to seismicity and deformation at Itoigawa-Shizuoka Tectonic Line, Central Japan. Earth Planets Space 2004, 56, 1285–1291. [Google Scholar] [CrossRef]
- Schmucker, U. Anomalies of geomagnetic variations in the southwestern United States. J. Geomagn. Geoelectr. 1964, 15, 193–221. [Google Scholar] [CrossRef][Green Version]
- Jiracek, G.R.; Ander, M.E.; Holcombe, H.T. Magnetotelluric Soundings of Crustal Conductive Zones in Major Continental Rifts. Rio Grande Rift Tecton. Magmat. 1979, 14, 209–222. [Google Scholar]
- Jiracek, G.R.; Gustafson, E.P.; Mitchell, P.S. Magnetotelluric results opposing magma origin of crustal conductors in the Rio Grande rift. Tectonophysics 1983, 94, 299–326. [Google Scholar] [CrossRef]
- Isola, I.; Mazzarini, F.; Bonini, M.; Corti, G. Spatial variability of volcanic features in early-stage rift settings: The case of the Tanzania Divergence, East African rift system. Terra Nova 2014, 26, 461–468. [Google Scholar] [CrossRef]
- Nyblade, A.A.; Langston, C.A.; Last, R.J.; Birt, C.; Owens, T.J. Seismic experiment reveals rifting of craton in Tanzania. EOS Trans. Am. Geophys. Union 1996, 77, 517–521. [Google Scholar] [CrossRef]
- Currie, C.A.; van Wijk, J. How craton margins are preserved: Insights from geodynamic models. J. Geodyn. 2016, 100, 144–158. [Google Scholar] [CrossRef]
- Fletcher, A.W.; Abdelsalam, M.G.; Emishaw, L.; Atekwana, E.A.; Laó-Dávila, D.A.; Ismail, A. Lithospheric Controls on the Rifting of the Tanzanian Craton at the Eyasi Basin, Eastern Branch of the East African Rift System. Tectonics 2018, 37, 2818–2832. [Google Scholar] [CrossRef]

© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Plasman, M.; Hautot, S.; Tarits, P.; Gautier, S.; Tiberi, C.; Le Gall, B.; Mtelela, K.; Gama, R. Lithospheric Structure of a Transitional Magmatic to Amagmatic Continental Rift System—Insights from Magnetotelluric and Local Tomography Studies in the North Tanzanian Divergence, East African Rift. Geosciences 2019, 9, 462. https://doi.org/10.3390/geosciences9110462
Plasman M, Hautot S, Tarits P, Gautier S, Tiberi C, Le Gall B, Mtelela K, Gama R. Lithospheric Structure of a Transitional Magmatic to Amagmatic Continental Rift System—Insights from Magnetotelluric and Local Tomography Studies in the North Tanzanian Divergence, East African Rift. Geosciences. 2019; 9(11):462. https://doi.org/10.3390/geosciences9110462
Chicago/Turabian StylePlasman, Matthieu, Sophie Hautot, Pascal Tarits, Stéphanie Gautier, Christel Tiberi, Bernard Le Gall, Khalfan Mtelela, and Remigius Gama. 2019. "Lithospheric Structure of a Transitional Magmatic to Amagmatic Continental Rift System—Insights from Magnetotelluric and Local Tomography Studies in the North Tanzanian Divergence, East African Rift" Geosciences 9, no. 11: 462. https://doi.org/10.3390/geosciences9110462
APA StylePlasman, M., Hautot, S., Tarits, P., Gautier, S., Tiberi, C., Le Gall, B., Mtelela, K., & Gama, R. (2019). Lithospheric Structure of a Transitional Magmatic to Amagmatic Continental Rift System—Insights from Magnetotelluric and Local Tomography Studies in the North Tanzanian Divergence, East African Rift. Geosciences, 9(11), 462. https://doi.org/10.3390/geosciences9110462




