The Internal Structural Evolution of Calderas: Results from 3D Discrete Element Simulations
Abstract
1. Introduction
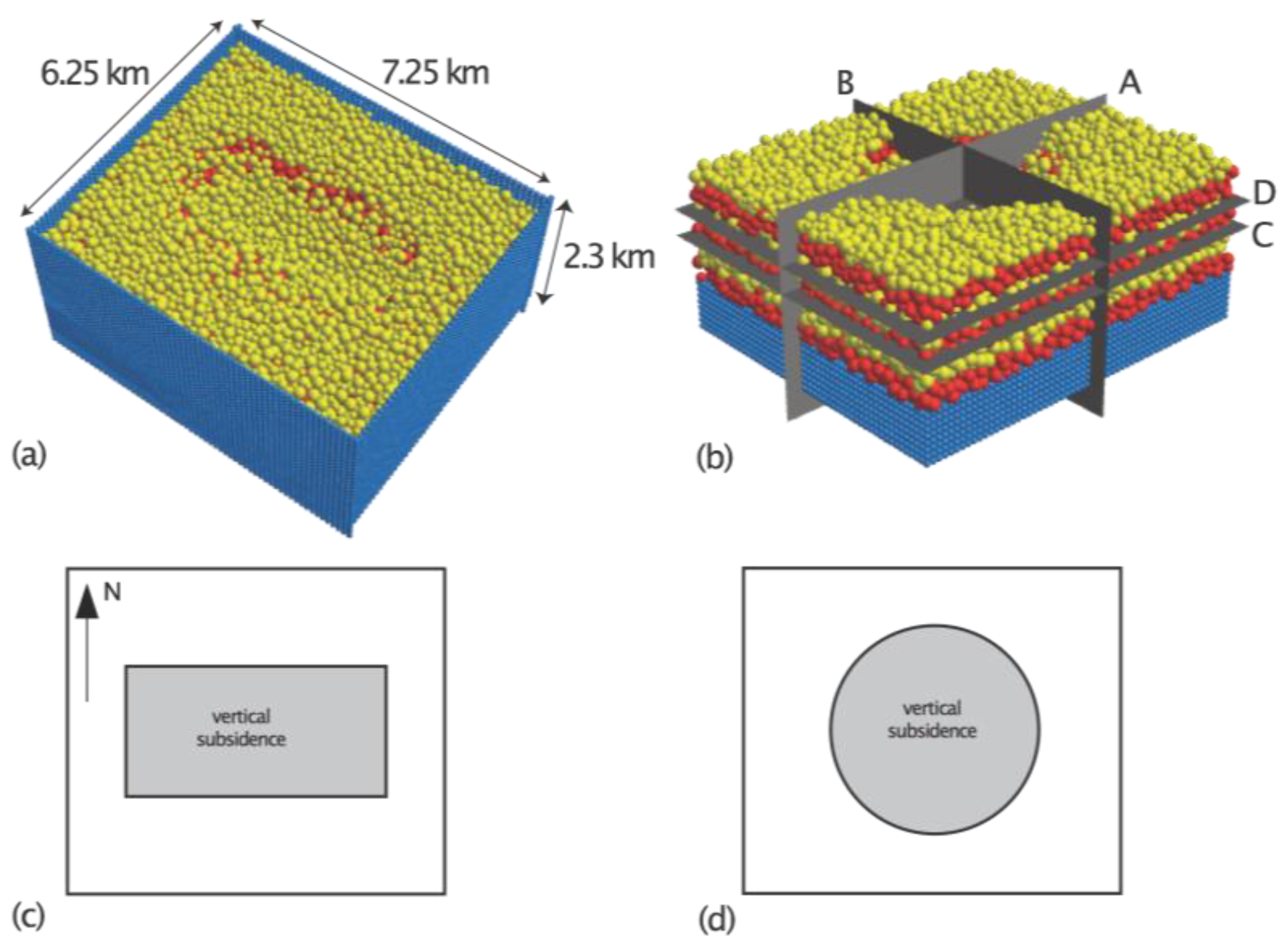
2. Modeling Methodology and Initial and Boundary Conditions
3. Simulation Results
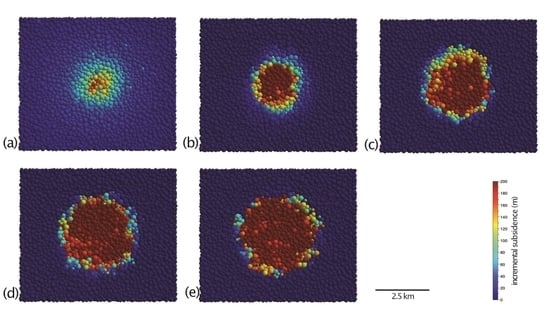
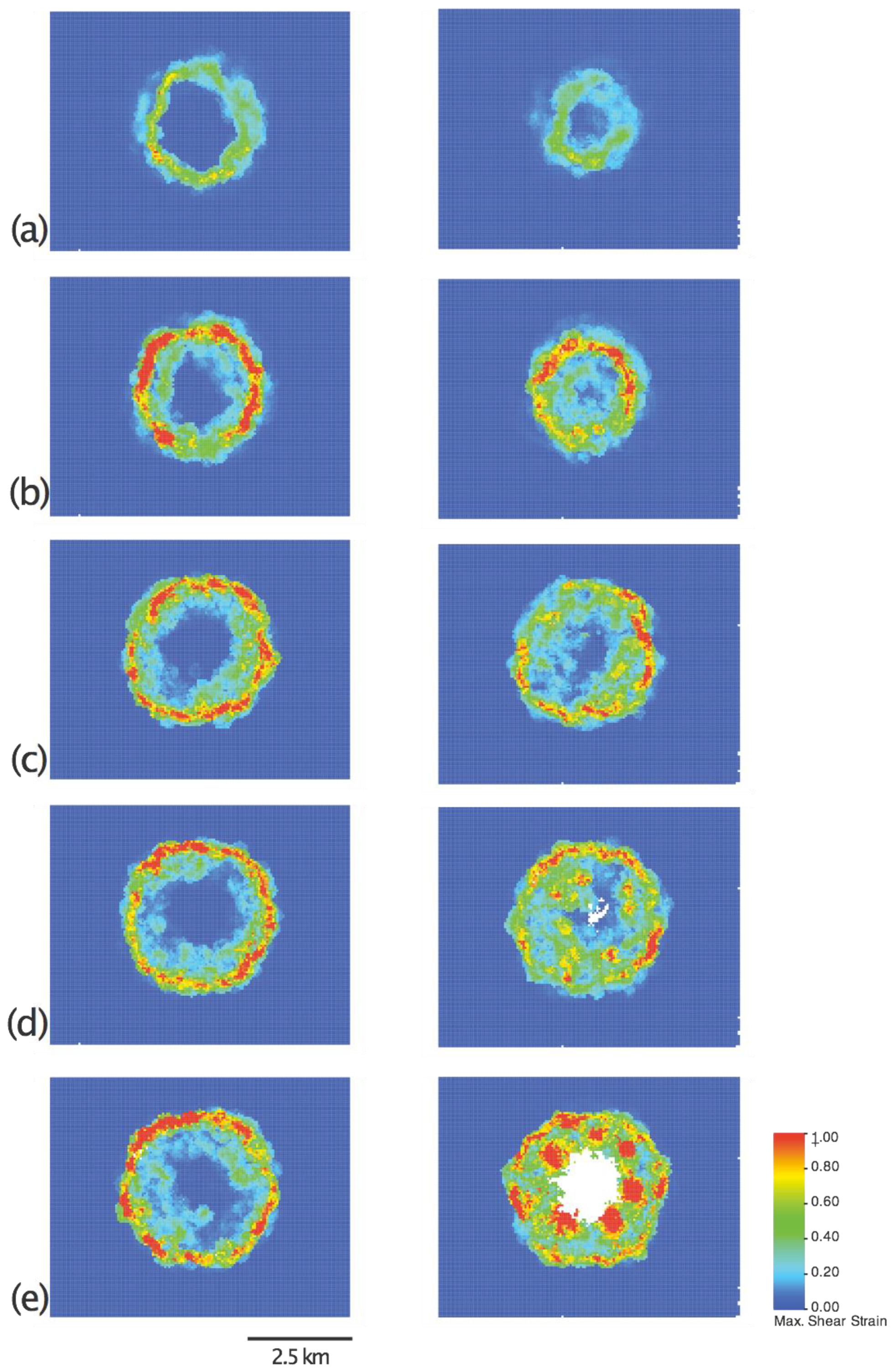
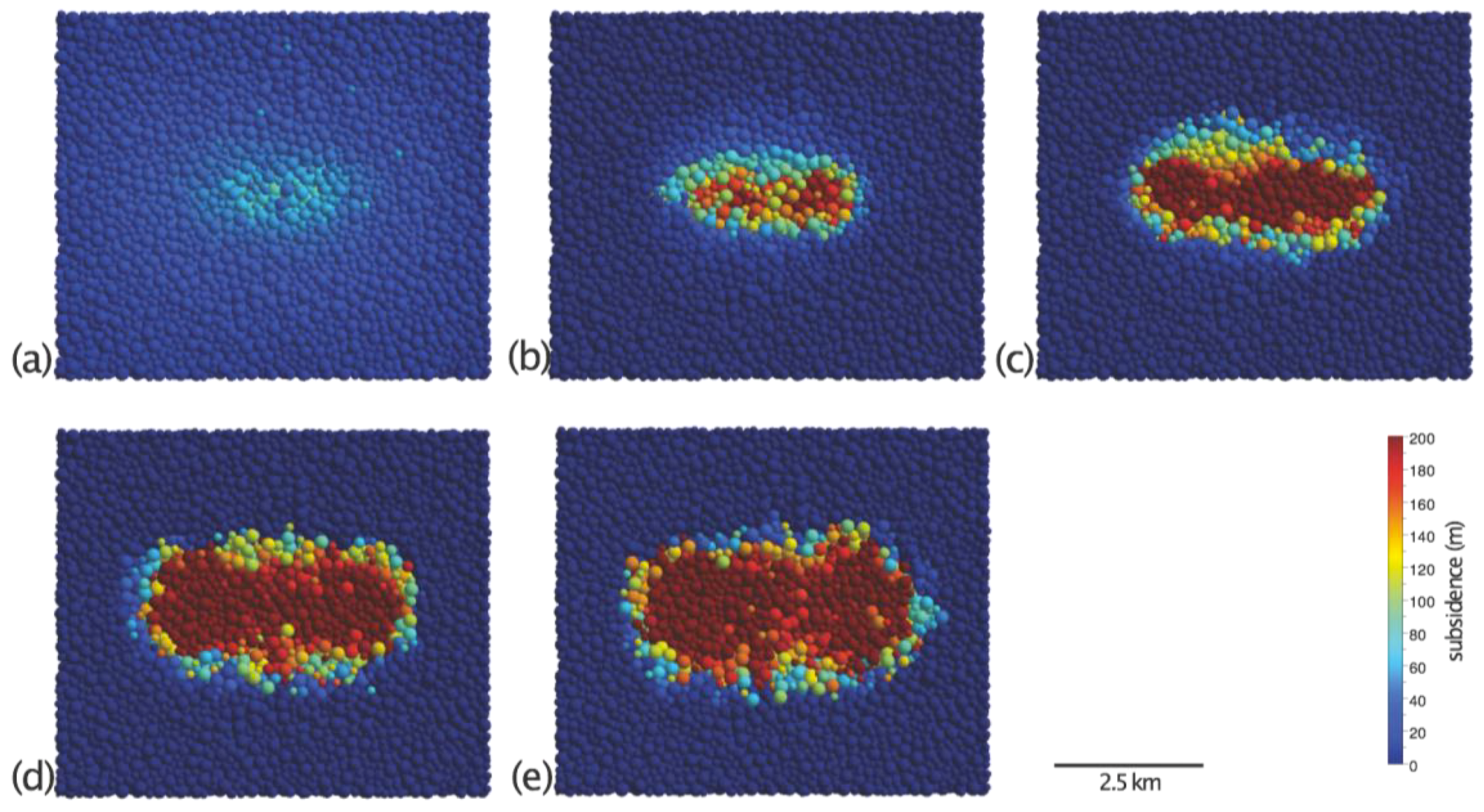
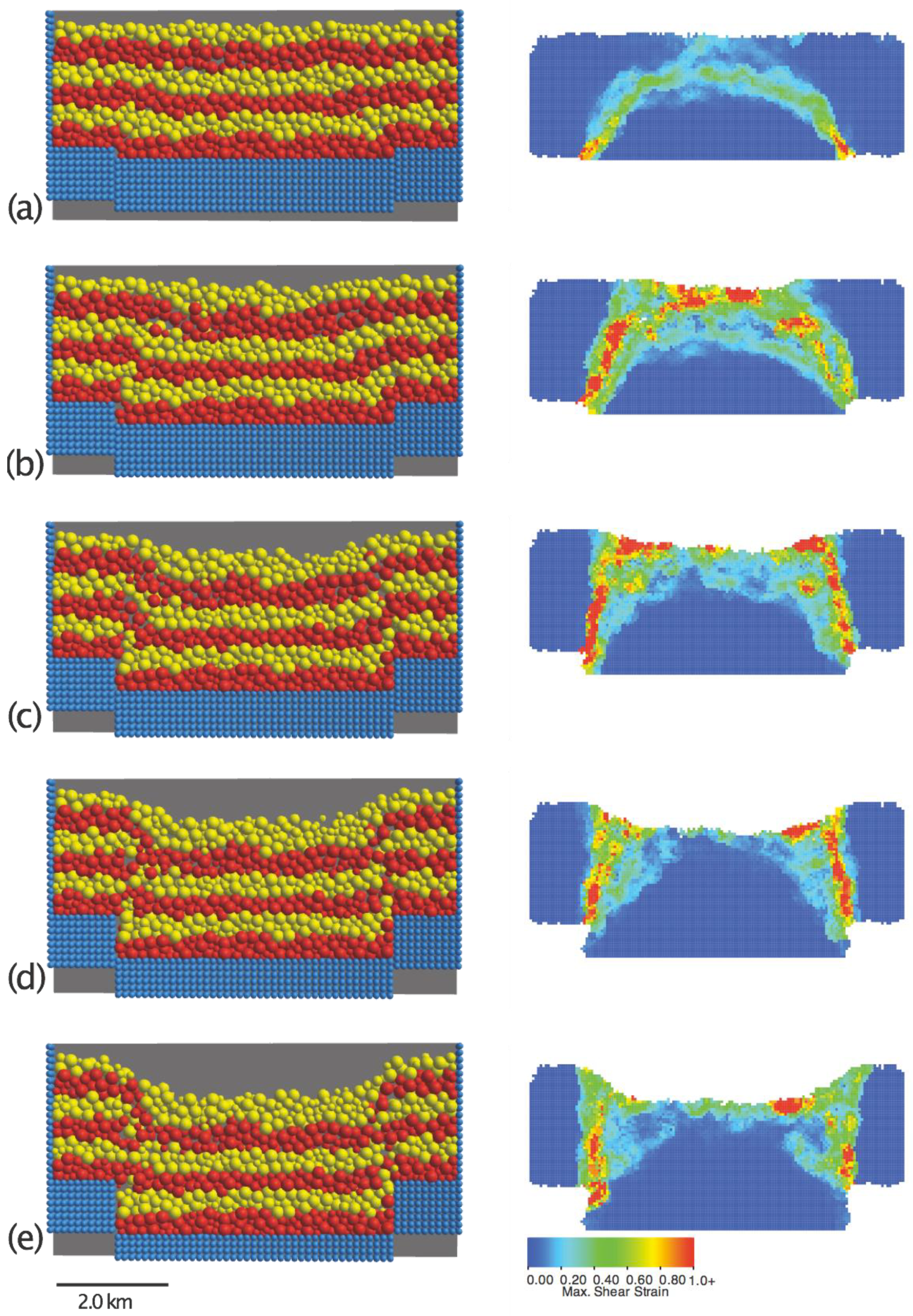
3.1. Experiment 1: Basal Piston with a Circular Top
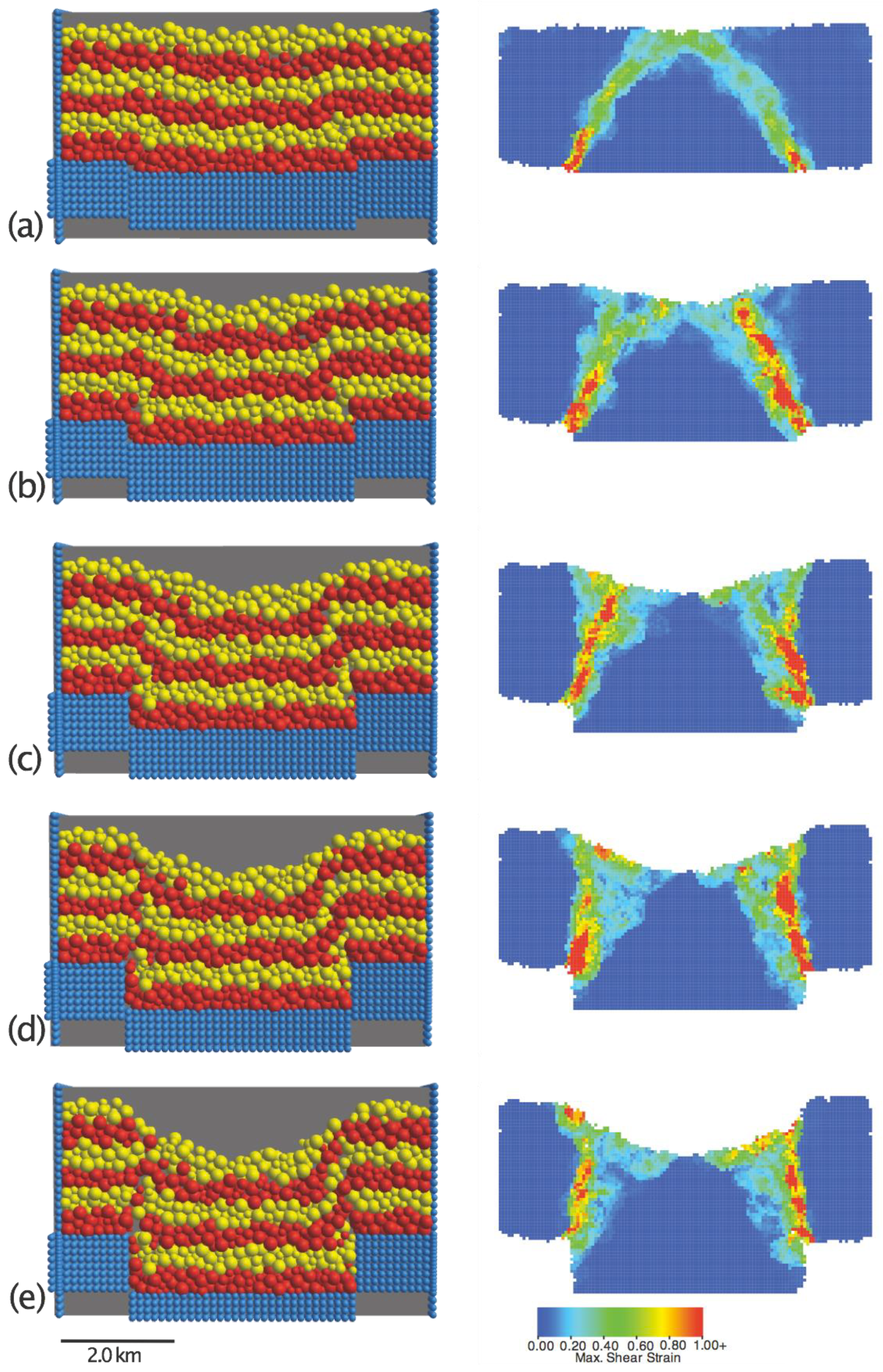
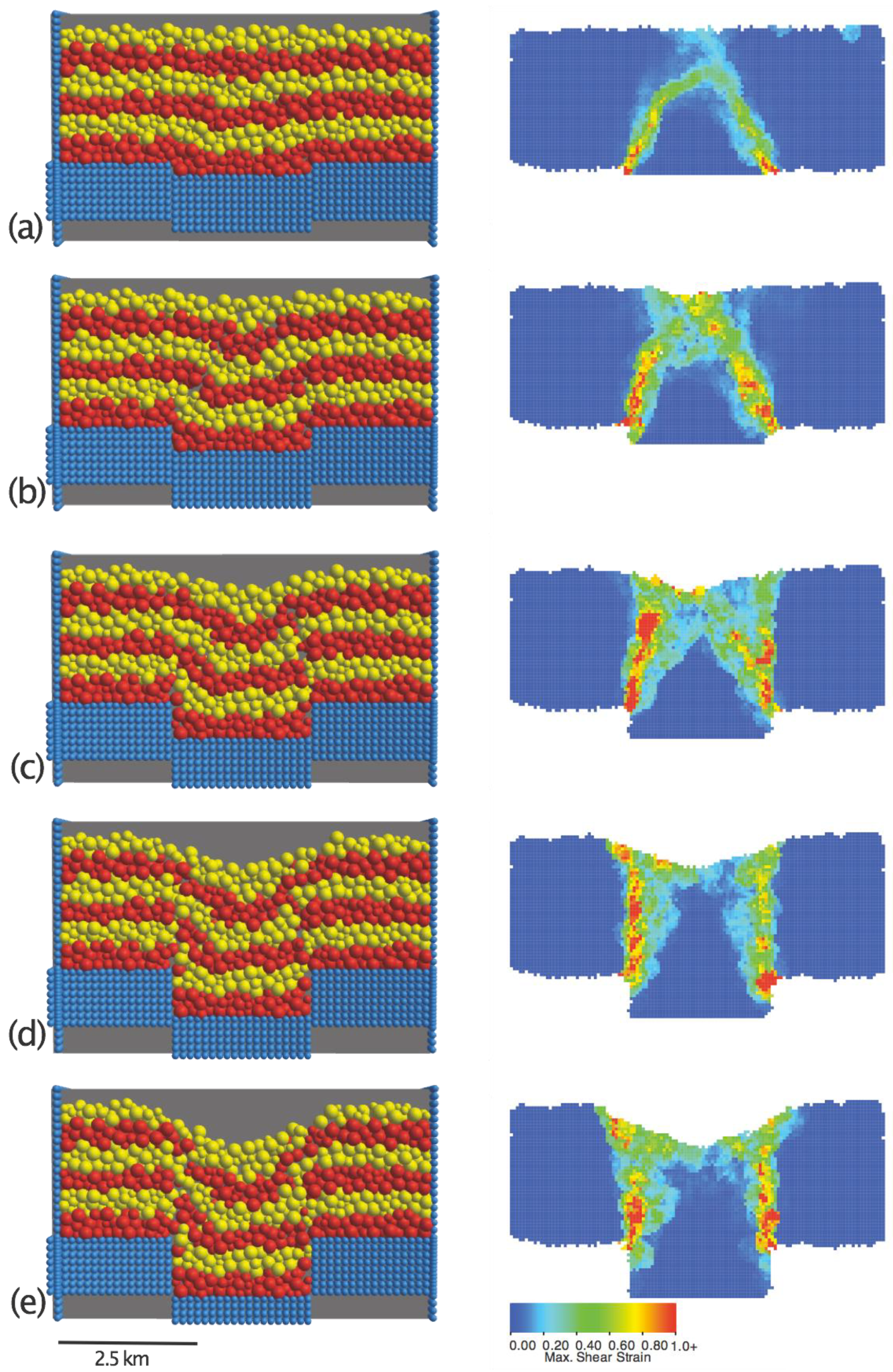
3.2. Experiment 2: Basal Piston with a Rectangular Top
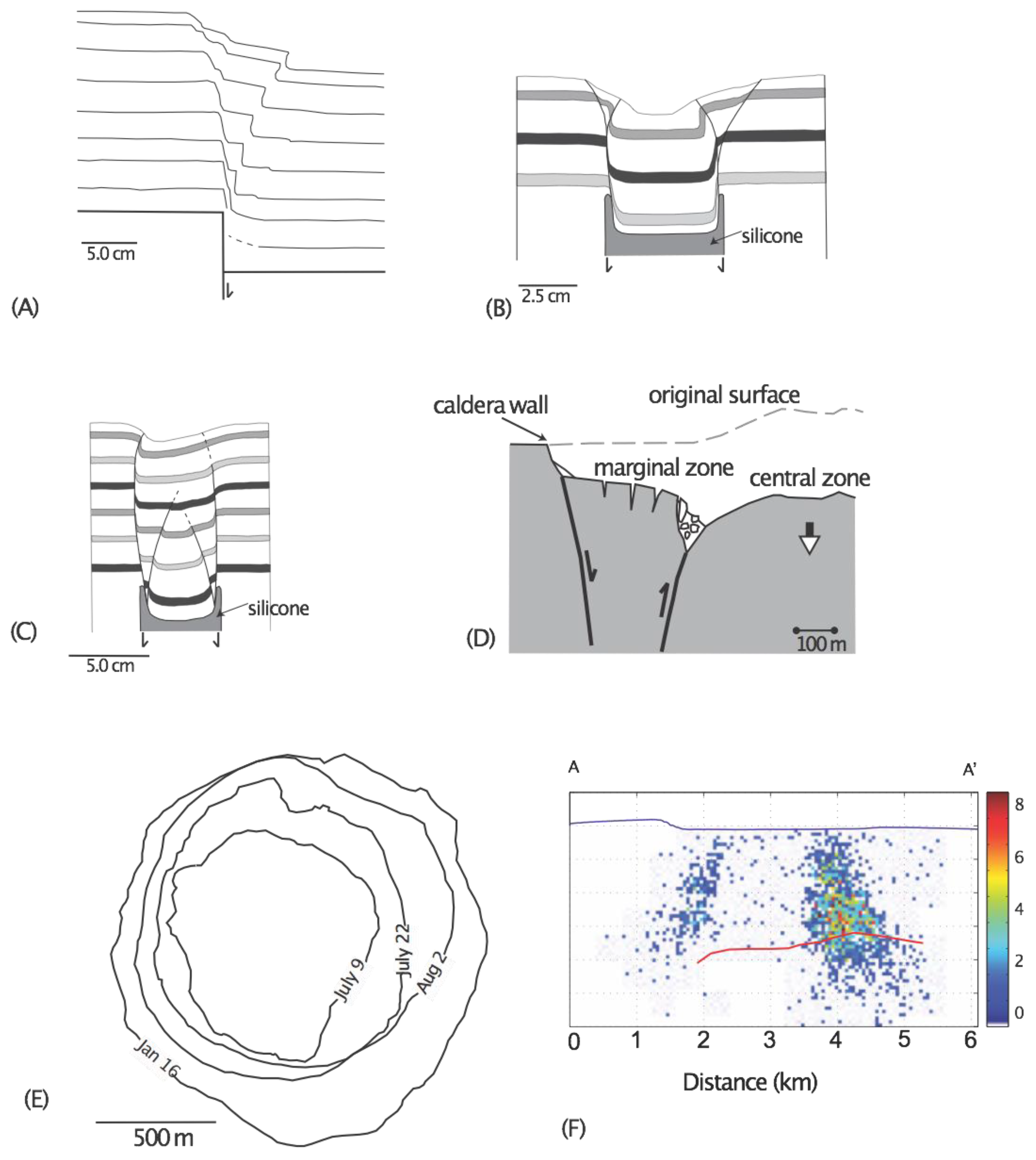
4. Discussion
5. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Martí, J.; Ablay, G.J.; Redshaw, L.T.; Sparks, R.S.J. Experimental studies of collapse calderas. J. Geol. Soc. 1994, 151, 919–929. [Google Scholar] [CrossRef]
- Merle, O.; Roche, O.; Druitt, T.H. Experimental study of caldera formation. J. Geophys. Res. Space Phys. 2000, 105, 395–416. [Google Scholar] [CrossRef]
- Geshi, N.; Shimano, T.; Chiba, T.; Nakada, S. Caldera collapse during the 2000 eruption of Miyakejima Volcano, Japan. Bull. Volcanol. 2002, 64, 55–68. [Google Scholar] [CrossRef]
- Kusumoto, S.; Takemura, K. Numerical simulation of caldera formation due to collapse of a magma chamber. Geophys. Res. Lett. 2003, 30, 2278. [Google Scholar] [CrossRef]
- Geyer, A.; Folch, A.; Martí, J. Relationship between caldera collapse and magma chamber withdrawal: An experimental approach. J. Volcanol. Geotherm. Res. 2006, 157, 375–386. [Google Scholar] [CrossRef]
- Acocella, V. Understanding caldera structure and development: An overview of analogue models compared to natural calderas. Earth-Sci. Rev. 2007, 85, 125–160. [Google Scholar] [CrossRef]
- Hardy, S. Structural evolution of calderas: Insights from two-dimensional discrete element simulations. Geology 2008, 36, 927–930. [Google Scholar] [CrossRef]
- Holohan, E.P.; Troll, V.R.; Vries, B.V.W.D.; Walsh, J.J.; Walter, T.R. Unzipping Long Valley: An explanation for vent migration patterns during an elliptical ring fracture eruption. Geol. 2008, 36, 323–326. [Google Scholar] [CrossRef]
- Holohan, E.; Schöpfer, M.; Walsh, J.; Holohan, E. Stress evolution during caldera collapse. Earth Planet. Sci. Lett. 2015, 421, 139–151. [Google Scholar] [CrossRef]
- Bathke, H.; Sudhaus, H.; Holohan, E.; Walter, T.R.; Shirzaei, M. An active ring fault detected at Tendürek volcano by using InSAR. J. Geophys. Res. 2013, 118, 4488–4502. [Google Scholar] [CrossRef]
- Wilcock, W.S.D.; Tolstoy, M.; Waldhauser, F.; Garcia, C.; Tan, Y.J.; Bohnenstiehl, D.R.; Caplan-Auerbach, J.; Dziak, R.P.; Arnulf, A.F.; Mann, M.E. Seismic constraints on caldera dynamics from the 2015 Axial Seamount eruption. Science 2016, 354, 1395–1399. [Google Scholar] [CrossRef] [PubMed]
- Wilcock, W.; Dziak, R.; Tolstoy, M.; Chadwick, W.; Nooner, S.; Bohnenstiel, D.; Caplan-Auerbach, J.; Waldhauser, F.; Arnulf, A.; Ballard, C.; et al. The Recent Volcanic History of Axial Seamount: Geophysical Insights into Past Eruption Dynamics with an Eye Toward Enhanced Observations of Future Eruptions. Oceanography 2018, 31, 114–123. [Google Scholar] [CrossRef]
- Nobile, A.; Acocella, V.; Ruch, J.; Aoki, Y.; Borgstrom, S.; Siniscalchi, V.; Geshi, N. Steady subsidence of a repeatedly erupting caldera through InSAR observations: Aso, Japan. Bull. Volcanol. 2017, 79. [Google Scholar] [CrossRef]
- Levy, S.; Bohnenstiehl, D.R.; Sprinkle, P.; Boettcher, M.S.; Wilcock, W.S.D.; Tolstoy, M.; Waldhauser, F. Mechanics of fault reactivation before, during, and after the 2015 eruption of Axial Seamount. Geology 2018, 46, 447–450. [Google Scholar] [CrossRef]
- Neal, C.A.; Brantley, S.R.; Antolik, L.; Babb, J.L.; Burgess, M.; Calles, K.; Cappos, M.; Chang, J.C.; Conway, S.; Desmither, L.; et al. The 2018 rift eruption and summit collapse of Kīlauea Volcano. Science 2019, 363, 367–374. [Google Scholar] [CrossRef]
- Cole, J.W.; Milner, D.M.; Spinks, K.D. Calderas and caldera structures: A review. Earth-Sci. Rev. 2005, 69, 1–26. [Google Scholar] [CrossRef]
- Walter, T.R.; Troll, V.R. Formation of caldera periphery faults: An experimental study. Bull. Volcanol. 2001, 63, 191–203. [Google Scholar] [CrossRef]
- Parks, M.M.; Heimisson, E.R.; Sigmundsson, F.; Hooper, A.; Vogfjörd, K.S.; Árnadóttir, T.; Ófeigsson, B.; Hreinsdóttir, S.; Hjartardóttir, Á.R.; Einarsson, P.; et al. Evolution of deformation and stress changes during the caldera collapse and dyking at Bárdarbunga, 2014–2015: Implication for triggering of seismicity at nearby Tungnafellsjökull volcano. Earth Planet. Sci. Lett. 2017, 462, 212–223. [Google Scholar] [CrossRef]
- Gudmundsson, A. Formation and development of normal-fault calderas and the initiation of large explosive eruptions. Bull. Volcanol. 1998, 60, 160–170. [Google Scholar] [CrossRef]
- Kusumoto, S.; Takemura, K. Caldera geometry determined by the depth of the magma chamber. Earth Planet. Space 2005, 57, 17–20. [Google Scholar] [CrossRef]
- Gray, J.; Monaghan, J. Numerical modelling of stress fields and fracture around magma chambers. J. Volcanol. Geotherm. Res. 2004, 135, 259–283. [Google Scholar] [CrossRef]
- Pinel, V.; Jaupart, C. Caldera formation by magma withdrawal from a reservoir beneath a volcanic edifice. Earth Planet. Sci. Lett. 2005, 230, 273–287. [Google Scholar] [CrossRef]
- Gudmundsson, A. Conceptual and numerical models of ring-fault formation. J. Volcanol. Geotherm. Res. 2007, 164, 142–160. [Google Scholar] [CrossRef]
- Holohan, E.P.; Schöpfer, M.P.J.; Walsh, J.J. Mechanical and geometric controls on the structural evolution of pit crater and caldera subsidence. J. Geophys. Res. Solid Earth 2011, 116. [Google Scholar] [CrossRef]
- Horsfield, W.T. An experimental approach to basement-controlled faulting. Geol. Mijnb. 1977, 56, 363–370. [Google Scholar]
- Saltzer, S.D.; Pollard, D.D. Distinct element modeling of structures formed in sedimentary overburden by extensional reactivation of basement normal faults. Tectonics 1992, 11, 165–174. [Google Scholar] [CrossRef]
- Morgan, J.K. Volcanotectonic interactions between Mauna Loa and Kilauea: Insights from 2-D discrete element simulations. J. Volcanol. Geotherm. Res. 2006, 151, 109–131. [Google Scholar] [CrossRef]
- Hardy, S.; McClay, K.; Muñoz, J.A. Deformation and fault activity in space and time in high-resolution numerical models of doubly vergent thrust wedges. Mar. Pet. Geol. 2009, 26, 232–248. [Google Scholar] [CrossRef]
- Cundall, P.A.; Strack, O.D.L. A discrete numerical model for granular assemblies. Géotechnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Mora, P.; Place, D. A lattice solid model for the non-linear dynamics of earthquakes. Int. J. Mod. Phys. C 1993, 4, 1059–1074. [Google Scholar] [CrossRef]
- Cardozo, N.; Allmendinger, R.W. SSPX: A program to compute strain from displacement/velocity data. Comput. Geosci. 2008, 35, 1343–1357. [Google Scholar] [CrossRef]
- Hardy, S. Does shallow dike intrusion and widening remain a possible mechanism for graben formation on Mars? Geology 2016, 44, 107–110. [Google Scholar] [CrossRef]
- Sanford, A.R. Analytical and experimental study of simple geological structures. Geol. Soc. Am. Bull. 1959, 70, 19–52. [Google Scholar] [CrossRef]
- Vendeville, B.C. Scale-models of basement induced-extension. C. R. Acad. Sci. 1988, 307, 1013–1019. [Google Scholar]




© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hardy, S. The Internal Structural Evolution of Calderas: Results from 3D Discrete Element Simulations. Geosciences 2019, 9, 419. https://doi.org/10.3390/geosciences9100419
Hardy S. The Internal Structural Evolution of Calderas: Results from 3D Discrete Element Simulations. Geosciences. 2019; 9(10):419. https://doi.org/10.3390/geosciences9100419
Chicago/Turabian StyleHardy, Stuart. 2019. "The Internal Structural Evolution of Calderas: Results from 3D Discrete Element Simulations" Geosciences 9, no. 10: 419. https://doi.org/10.3390/geosciences9100419
APA StyleHardy, S. (2019). The Internal Structural Evolution of Calderas: Results from 3D Discrete Element Simulations. Geosciences, 9(10), 419. https://doi.org/10.3390/geosciences9100419