Closed-System Magma Degassing and Disproportionation of SO₂ Revealed by Changes in the Concentration and δ³⁴S Value of H₂S_(g) in the Solfatara Fluids (Campi Flegrei, Italy)

Abstract

The use of a conceptual model of reference and modelling of relevant processes is mandatory to correctly interpret chemical and isotopic data. Adopting these basic guidelines, we have interpretated the unprecedented increase in the H₂S_(g) concentration and the concurrent unexpected decrease in the δ³⁴S value of H₂S_(g) recorded since 2018 in the fumarolic effluents of the Bocca Grande fumarolic vent at Solfatara, Campi Flegrei caldera, in the framework of our conceptual model of the Solfatara magmatic–hydrothermal system. Assuming that the magma chamber situated at depths ≥ 8 km was filled at the end of the 1982–1984 bradyseismic crisis and no refilling episodes took place afterwards, as suggested by gas geochemistry, the concentration and the δ³⁴S value of H₂S_(g) of the Bocca Grande fumarolic effluents are controlled by closed-system degassing of the melt at depths ≥ 8 km and disproportionation of SO₂ in the deep hydrothermal reservoir (6.5–7.5 km depth) hosted in carbonate rocks where H₂S equilibrates. These processes have been active during the last 40 years, but 41.1% (±6.4%) of the sulfur initially stored in the melt (2200 mg/kg) was lost in the 4-year period of April 2018–April 2022. This marked loss of S from the melt in 2018–2022 might be due to the high solubility of sulfur in the melt, which caused its preferential separation during the late degassing stages. These findings are of utmost importance for the surveillance of the Solfatara magmatic–hydrothermal system during the ongoing bradyseismic crisis.

1. Introduction

Sulfur isotope fractionation during magmatic degassing chiefly depends on redox conditions of both the melt and the gases separated from it, e.g., [1,2,3,4,5,6], due to the remarkable difference in the oxidation state of the main S species in silicate melts, SO₄²⁻_(m) and S²⁻_(m), and magmatic gases, SO_2(g) and H₂S_(g). Magmatic degassing under oxidizing conditions is controlled by SO₄²⁻_(m) in the melt and SO_2(g) in the gas phase, whereas magmatic degassing under reducing conditions is governed by S²⁻_(m) in the melt and H₂S_(g) in the gas phase, e.g., [7,8,9,10]. Consequently, the S isotopes in the melt will gradually become heavier due to equilibrium magma degassing under oxidizing conditions and become lighter owing to equilibrium magma degassing under reducing conditions [4,5,6,11,12]. In addition to magmatic degassing, another process that can cause a considerable fractionation of sulfur isotopes is SO₂ disproportionation, as recognized in numerous studies of both active magmatic–hydrothermal systems and their past analogues, which are represented by different types of ore deposits [6,13]. Thus, sulfur isotopes of volcanic rocks and magmatic and hydrothermal fluids are effective indicators of the extent of both magmatic degassing and SO₂ disproportionation. These processes can be modeled considering a conceptual model of reference and the temperature and pressure in the environments where they occur. This approach was used in this work to correctly interpret the sulfur isotope data of Solfatara fumaroles at Campi Flegrei.

Since 1950, the Campi Flegrei caldera has been affected by four positive bradyseismic episodes, the last of which began in 2005 and is still occurring. As reported in several studies, as that of Giudicepietro et al. [14] and references therein, ground movements are accompanied by both shallow seismic activity and increasing emission of fumarolic fluids from the Solfatara-Pisciarelli, the most important manifestations in the Campi Flegrei caldera. In particular, an unprecedented increase in the H₂S_(g) concentration and the concurrent unexpected decrease in the δ³⁴S value of H₂S_(g) have been recorded since 2018 in the fumarolic effluents of the Bocca Grande fumarolic vent at Solfatara, in the Campi Flegrei caldera. Incidentally, we recall that the δ³⁴S value is defined by the following relation:

$${\mathsf{\delta }}^{34}\mathrm{S}=\left[{\displaystyle \frac{{\left(\frac{{}^{34}\mathrm{S}}{{}^{32}\mathrm{S}}\right)}_{\mathrm{X}}-{\left(\frac{{}^{34}\mathrm{S}}{{}^{32}\mathrm{S}}\right)}_{\mathrm{S}\mathrm{t}\mathrm{d}}}{{\left(\frac{{}^{34}\mathrm{S}}{{}^{32}\mathrm{S}}\right)}_{\mathrm{S}\mathrm{t}\mathrm{d}}}}\right]·1000$$

(1)

in which subscripts X and Std identify the sample and the reference standard, respectively. The reference standard of sulfur isotopes is the Vienna Canyon Diablo Troilite (VCDT). The increase in the H₂S_(g) concentration and the concurrent decrease in the δ³⁴S value of H₂S_(g) recorded since 2018 in the Bocca Grande fumarolic effluents have been attributed to decompression-driven degassing of mafic magma at ≥6 km depth, along with some extent of sulfur remobilization from hydrothermal minerals by Caliro et al. [15].

However, taking as interpretation framework the conceptual model of the Solfatara magmatic–hydrothermal system proposed by Marini et al. [16], it is reasonable to attribute these changes to closed-system degassing of the magma positioned at depths ≥ 8 km and disproportionation of SO₂ in the deep hydrothermal reservoir (6.5–7.5 km depth) hosted in carbonate rocks. In this paper, we intend to explore this alternative interpretation.

2. Geological Background

The present knowledge of the Campi Flegrei geology is mainly founded on the surface studies by Rosi et al. [17], the subsurface information provided by the deep geothermal wells drilled by AGIP-ENEL in the 1970s–1980s [18], and the synthesis of geological information with inland and offshore gravimetric and aeromagnetic data analyses [19] performed by Barberi et al. [20]. The numerous scientific studies performed more recently have ameliorated some aspects of the geological knowledge of Campi Flegrei, providing further details, but some controversial points still remain (see below). The Campi Flegrei is situated inside the Campanian Plain (Figure 1a), a Neogene graben filled by clastic and volcanoclastic sediments and volcanic rocks overlying the Mesozoic carbonate basement, which is found at depths ≥ 4 km [19,21].

The Campi Flegrei evolution has been subdivided into different phases comprising several eruptive episodes, both explosive and effusive, including two large-scale, caldera-forming eruptions [17] (Figure 1b). The most significant event of this last type is known as the Campanian Ignimbrite (CI) eruption, which took place 39.85 ± 0.14 ka BP [24], and produced either a single caldera [17] or a nested caldera [20,25]. The second most important caldera-forming eruption, known as Neapolitan Yellow Tuff (NYT) eruption, occurred 14.9 ± 0.4 ka BP [26] from several vents, and it either generated a new caldera [27] or reactivated the inner CI caldera [20] or reactivated both compartments of the nested CI caldera [25]. In the interval of time between the CI and the NYT eruptions, submarine volcanic activity took place, whereas chiefly subaerial volcanic activity occurred after the NYT eruption [17]. Most volcanic products of the Campi Flegrei are pyroclastics, whereas lava flows and domes are small in volume and were chiefly erupted during the pre-caldera period [28]. Volcanic rocks range in composition from trachybasalts to peralkaline phonolitic trachytes, with intermediate compositions represented by latites, trachytes, and alkali trachytes [28,29]. Trachybasalts are very rare and are separated from latites by a compositional gap, whereas the series between latites and the trachytic varieties is complete [29]. Trachytic rocks are much more voluminous than latites [29]. Based on melt inclusion evidence, trachybasalts are the most mafic volcanic products at Campi Flegrei, and therefore, the most representative of the parental melts of mantle provenance, as pointed out by Caliro et al. [15] and references therein.

A controversial aspect concerns the Solfatara edifice. According to some authors [30,31,32], it was generated by a pyroclastic eruption that emitted a basal phreatomagmatic coarse breccia, above which there are few meters of stratified pyroclastic surges. In contrast, according to Principe [33], the basal breccia is a purely hydrothermal debris flow—without any magma involvement—and the overlying deposits come from the younger Astroni volcanic center. Irrespective of the nature of the event, it possibly occurred about 4200–4400 ka BP [34].

The last volcanic eruption in the Campi Flegrei began on September 29th, 1538, and had the duration of one week [35]. It consisted in a small phreatomagmatic event that involved a highly differentiated peralkaline phonolitic–trachytic magma and produced the ash and scoria edifice of Monte Nuovo [17]. The eruption was preceded by a fast and local uplift in that the vent site was affected by a sudden uprise of ca. 6 m in the 36 h before the eruption [35,36].

The fast and local uplift preceding the Monte Nuovo eruption is completely different from the slow vertical ground movements known as bradyseism, which have affected the whole Campi Flegrei caldera since Roman times or even before, as suggested by the presence of different orders of terraces known as Starza terraces, dating back to at least 12 ka ago [37]. The bradyseism comprises cycles of inflation or uplift and deflation or subsidence [36]. The Campi Flegrei bradyseism has had a wide echo in the scientific world since the 19th century, since it was described by Charles Lyell in his famous “Principles of Geology” [38]. The last uplift cycle started in 1950, brought about maximum uplifts of 1.77 m in 1969–1972 and further 1.79 m in 1982–1984, both followed by periods of limited subsidence, the last of which terminated in 2005, when the still ongoing uplift phase began [39,40,41] reaching 1.43 m in March 2025 [42]. In the scientific literature, there is a lack of consensus on the processes controlling the bradyseism, with some authors suggesting the magma ascent, e.g., D’Auria, L. [43] or the pressurization of the magma chamber, e.g., Barberi et al. [20], and other authors invoking the pressurization of the overlying hydrothermal system, e.g., Marini et al. [16], whereas a combination of both factors was proposed in other studies, e.g., Todesco [44].

3. Materials and Methods

Use of a conceptual model of reference and modeling of relevant processes are mandatory actions to correctly interpret the H₂S_(g) concentration data and the δ³⁴S values of H₂S_(g) of Bocca Grande fumarolic effluents. Therefore, the adopted conceptual model and the relevant process are discussed in the next section, before presenting the calculation methods used in this work.

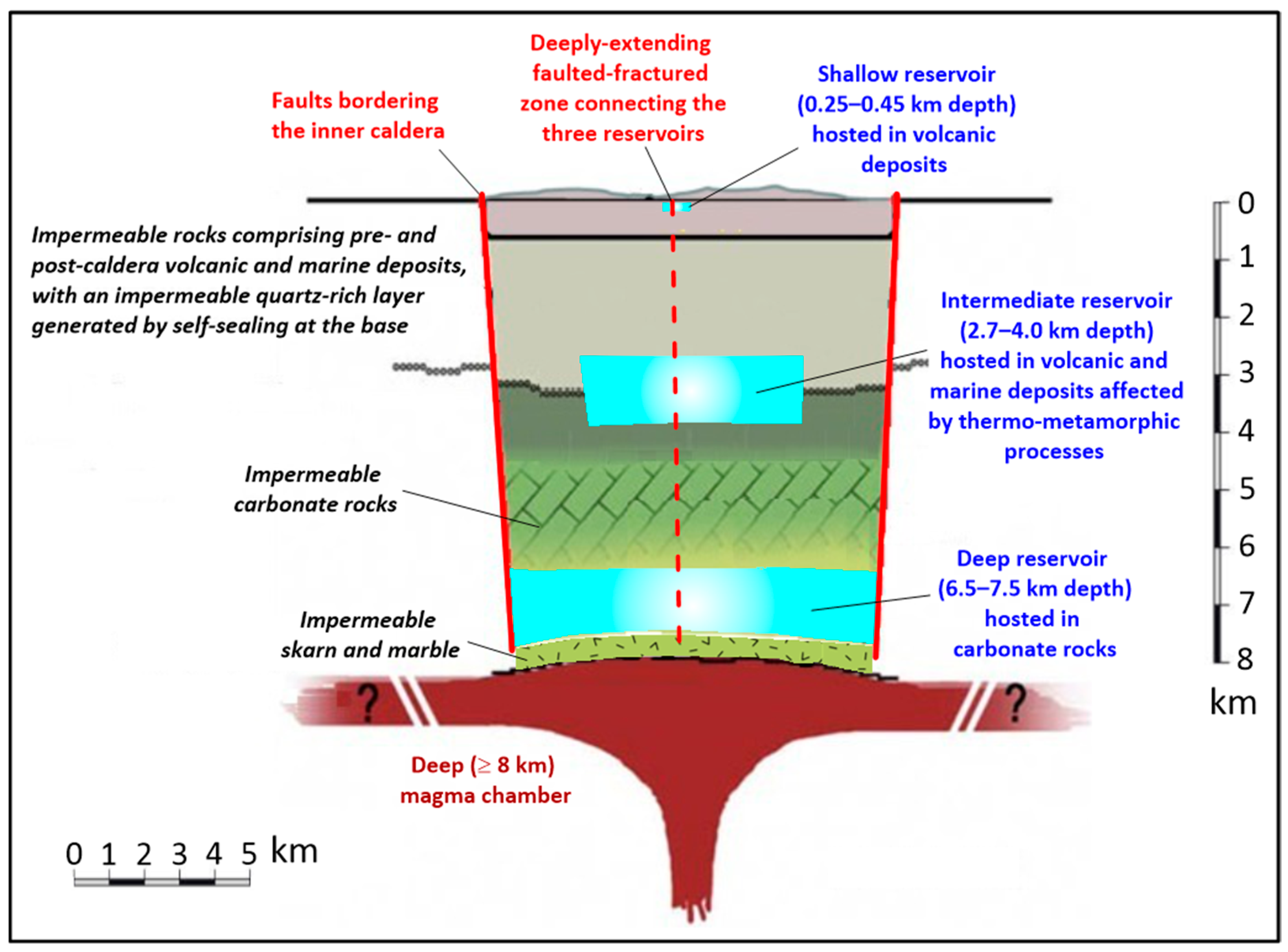
3.1. The Conceptual Model of the Solfatara Magmatic–Hydrothermal System

The conceptual model of the Solfatara magmatic–hydrothermal system proposed by Marini et al. [16] comprises the following units (Figure 2):

(1)

The cover of the shallow hydrothermal reservoir (0–0.25 km depth), made up of volcanic deposits influenced by advanced argillic hydrothermal alteration close to the surface and by argillic hydrothermal alteration away from it [18,45]. Its area is around 1 km², as indicated by the Solfatara diffuse degassing structure [46,47].

(2)

The shallow hydrothermal reservoir (0.25–0.45 km depth), a steam and gas pocket hosted in volcanic deposits. It has the same area as its cover and a small volume, ~0.2 km³.

(3)

An impermeable unit (0.45–2.7 km depth), including volcanic and marine deposits, influenced by phyllitic hydrothermal alteration in the upper portion and by propylitic hydrothermal alteration in the lower part, with an impermeable quartz-rich layer generated by self-sealing at the bottom [18,45]. The area of this impermeable unit corresponds to that of the inner caldera block of Barberi et al. [20].

(4)

The intermediate hydrothermal reservoir (2.7–4.0 km depth) hosted in volcanic and marine deposits modified by thermometamorphic hydrothermal alteration. According to several studies, e.g., [21,48,49,50,51,52,53], over-pressurized supercritical fluids are stored in the intermediate hydrothermal reservoir, which, consequently, is the engine of the ground uplift and the associated shallow seismicity. Again, the area of the intermediate hydrothermal reservoir matches that of the inner caldera block of Barberi et al. [20]. Nevertheless, the intermediate hydrothermal reservoir might be constituted by distinct compartments rather than a unique aquifer, as indicated by the piecemeal collapse of the inner caldera [54] and the distribution of seismic events during the last years of the ongoing unrest (https://terremoti.ov.ingv.it/gossip/flegrei/index.html, last accessed 14 February 2025).

(5)

A thick carbonate sequence (4.0–6.5 km depth), behaving as aquiclude evidently due to nil to negligible dissolution and fracturing, similar to what was found by the deep geothermal well Nisyros-1 [55], which intersected an 830 m thick pile of impermeable carbonate rocks overlying a diorite intrusion and the associated thermometamorphic rocks.

(6)

The deep hydrothermal reservoir (6.5–7.5 km depth) hosted in fractured carbonate rocks, also affected by dissolution–precipitation processes and/or gas–solid reactions governed by acidic magmatic fluids and/or gases. Its area is the same as that of the underlying units.

(7)

An impermeable unit made up of skarn and marble (7.5–8.0 km depth) generated by thermometamorphic and metasomatic processes and behaving as an aquiclude due to their nil porosity [56].

(8)

The melt zone (depths ≥ 8 km) in which a trachybasaltic magma is presumably stored [57]. Its area corresponds to that of the whole outer caldera of Barberi et al. [20].

3.2. Temperature and Pressure Conditions

Temperature and pressure conditions are based on the gas-geoindicators of Marini et al. [58] calibrated for the decompression path of a NaCl brine in equilibrium with a vapor phase and the geochemical data of Bocca Grande fumarolic fluids, from June 1983 to December 2023 [15,57,59,60,61,62,63,64,65,66,67,68,69,70,71,72]. The obtained indications are as follows:

(i)

CO equilibrated in the shallow hydrothermal reservoir at nearly constant temperature, 217 ± 9 °C, and total fluid pressure, 24.5 ± 3.9 bar.

(ii)

CH₄ attained thermochemical equilibrium in the intermediate hydrothermal reservoir at temperature and total fluid pressure increasing with time, from 235 °C and 31.1 bar in March 1984 to peak values of 609 °C and 1340 bar in September 2023.

(iii)

H₂S achieved the equilibrium condition in the deep hydrothermal reservoir at temperature and total fluid pressure incrementing with time, from 618 °C and 1120 bar in July 1984 to maximum values of 1040 °C and 3280 bar in October 2019. These temperatures are in satisfactory agreement with those of 880–1020 °C obtained by extrapolating the geothermal gradient of ca. 134 °C/km measured in the deepest levels of the San Vito 1 well [58].

3.3. Processes Controlling the Concentration and the δ³⁴S of H₂S_(g)

On the one hand, H₂S_(g) is the only S species present in relevant concentrations in the Solfatara fluids, 600–6000 μmol/mol, and is the third gaseous species, in order of decreasing importance, after H₂O_(g), 689,000–873,000 μmol/mol, and CO_2(g), 125,000–309,000 μmol/mol [15,57,59,60,61,62,63,64,65,66,67,68,69,70,71,72]. Other detectable gaseous S species, such as COS_(g), CS_2(g), and CH₃SH_(g), have low concentrations [73], whereas SO_2(g) is virtually absent in Solfatara fluids [58]. On the other hand, both H₂S_(g) and SO_2(g) are present in significant concentrations in the fluids separated from the melt at depths ≥ 8 km, according to Caliro et al. [15]. Therefore, to explain the presence of H₂S_(g) and the absence of SO_2(g) in fumarolic fluids, first, it must be taken into account that there is no process capable to quantitatively convert SO_2(g) into H₂S_(g), and second, it is necessary to invoke either SO_2(g) disproportionation, which is able to convert up to one-quarter of magma-sourced SO_2(g) into H₂S_(g) (see Appendix A, Appendix A.1) or magmatic gas scrubbing [74,75], which removes all SO_2(g) and some H₂S_(g) from magmatic gases. Between these two options, we consider SO_2(g) disproportionation more likely than magmatic gas scrubbing because fumarolic fluids are superheated (with discharge temperatures of 150–165 °C at Bocca Grande and 135–154 °C at Bocca Nuova [58]), which makes the inflow of external water and/or steam condensation, necessary conditions for magmatic gas scrubbing occurrence, unlikely.

Based on the adopted conceptual model and previous considerations, we have assumed that the processes controlling the concentration of H₂S_(g) and its δ³⁴S value in the Bocca Grande fumarolic gases are as follows:

(1)

The separation of SO_2(g)- and H₂S_(g)-bearing magmatic gases from the melt, occurring in the melt zone, at depths ≥ 8 km, under closed-system conditions;

(2)

The SO₂ disproportionation reaction occurring in the deep hydrothermal reservoir and the related production of anhydrite.

Conversely, the reactions involving pyrite do not control the H₂S concentration of Solfatara fluids, as discussed in Appendix A, Appendix A.3. It is not excluded that other processes may take place between the deep hydrothermal reservoir outlet and the surface, such as the formation or destruction of S-bearing solid phases identified at the surface in the Solfatara-Pisciarelli area and/or in the cores from the deep geothermal wells Mofete 1, Mofete 5, and San Vito 1 [45,76], e.g., elemental sulfur, sulfides and bisulfides (e.g., pyrrhotite, pyrite, galena, and sphalerite), and sulfates (e.g., anhydrite and alunite). Nevertheless, it is reasonable to assume that they have a nil to negligible impact on the concentration of H₂S_(g) and its δ³⁴S value, at least during the last years, due to the prolonged heating of the Solfatara magmatic–hydrothermal system and the considerable temperatures attained by both the intermediate and deep hydrothermal reservoirs (see above). In contrast, by analogy with what was suggested by Giggenbach [77], it is probable that elemental sulfur or sulfur stored in S-bearing minerals was remobilized and entered the magmatic–hydrothermal gases during the early heating phase of the Solfatara magmatic–hydrothermal system when temperatures were much lower, as already recognized by Allard et al. [78].

3.4. Modeling of Magma Degassing

Modeling of magma degassing is based on the hypothesis that the magma chamber positioned at depths ≥ 8 km was filled at the end of the 1982–1984 bradyseismic crisis, and no refilling episodes took place afterwards, as suggested by gas geochemistry [57]. On the other hand, there is no geophysical evidence indicating the occurrence of refilling episodes of the magma chamber situated at depths ≥ 8 km because magma transfer occurs in the absence of earthquakes in this portion of the lower crust with ductile behavior, as pointed out by Buono et al. [79].

The trachybasaltic melt stored in the magma chamber was assumed to be at constant temperature T = 1120 °C and constant pressure ${\mathrm{P}}_{\mathrm{t}\mathrm{o}\mathrm{t}}$ = 2879 bar [57,58], as well as at constant redox conditions defined by ${\log \mathrm{f}}_{{\mathrm{O}}_2}$ = −8.28, corresponding to ΔNNO = 0.254 and ΔQFM = 0.974, according to the following relations (T in K):

$${∆\mathrm{NNO}=\log \mathrm{f}}_{{\mathrm{O}}_2}-9.36+\mathrm{24,930}/\mathrm{T}$$

(2)

$${∆\mathrm{QFM}=\log \mathrm{f}}_{{\mathrm{O}}_2}-8.29+\mathrm{24,441.9}/\mathrm{T}.$$

(3)

Equations (2) and (3) are from Huebner and Sato [80] and Myers and Eugster [81], respectively. At these redox conditions, the melt is undersaturated with Fe sulfides [15], meaning that the decrease in the S concentration of the melt is controlled by degassing. Constant redox conditions result in a constant molar fraction of sulfate in the total ionic S dissolved in the melt, ${\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_4^{2-},\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}$, which was computed using the equation [9]:

$${\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_4^{2-},\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}={\mathrm{X}}_{\mathrm{S}{\mathrm{O}}_4^{2-},\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}/\left({\mathrm{X}}_{\mathrm{S}{\mathrm{O}}_4^{2-},\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}+{\mathrm{X}}_{{\mathrm{S}}^{2-},\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}\right)=1/\left[1+{10}^{(2.1-2·∆\mathrm{Q}\mathrm{F}\mathrm{M})}\right]$$

(4)

The gas phase coexisting at equilibrium with the melt was assumed to be under the same redox conditions as the melt, and the molar fraction ratio ${\mathrm{X}}_{{\mathrm{S}\mathrm{O}}_2}/{\mathrm{X}}_{{\mathrm{H}}_2\mathrm{S}}$ was calculated using the relation (T in K):

$$\log \left({\mathrm{X}}_{{\mathrm{S}\mathrm{O}}_2}/{\mathrm{X}}_{{\mathrm{H}}_2\mathrm{S}}\right)=27377/\mathrm{T}-3.986-{\log \mathrm{f}}_{{\mathrm{H}}_2\mathrm{O}}+{1.5·\log \mathrm{f}}_{{\mathrm{O}}_2}$$

(5)

Equation (5) was obtained by Marini et al. [5] from the log K of the equilibrium reaction:

H₂S_(g) + 1.5 O_2(g) = SO_2(g) + H₂O_(g),

(6)

considering the thermodynamic data of Stull et al. [82] and assuming that the fugacity coefficients of H₂S and SO₂ have the same value. This is a reasonable assumption in that the critical temperature and pressure of H₂S, 373.6 K and 88.9 bar, are relatively close to the critical properties of SO₂, 430.4 K and 77.7 bar. Indeed, there is a small difference between the fugacity coefficients of H₂S and SO₂, ${\mathsf{\phi }}_{{\mathrm{H}}_2\mathrm{S}}$ = 1.680 and ${\mathsf{\phi }}_{{\mathrm{S}\mathrm{O}}_2}$ = 1.795, at T = 1120 °C and ${\mathrm{P}}_{\mathrm{t}\mathrm{o}\mathrm{t}}$ = 2879 bar, computed by Caliro et al. [15] using the code Sulfur_X [83]. Knowing the ${\mathrm{X}}_{{\mathrm{S}\mathrm{O}}_2}/{\mathrm{X}}_{{\mathrm{H}}_2\mathrm{S}}$ ratio, the SO₂ molar fraction in total gaseous S is readily obtained utilizing the equation:

$${\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}}={\mathrm{X}}_{\mathrm{S}{\mathrm{O}}_2}/\left({\mathrm{X}}_{\mathrm{S}{\mathrm{O}}_2}+{\mathrm{X}}_{{\mathrm{H}}_2\mathrm{S}}\right)=1/\left[\left({\mathrm{X}}_{{\mathrm{H}}_2\mathrm{S}}/{\mathrm{X}}_{{\mathrm{S}\mathrm{O}}_2}\right)+1\right]$$

(7)

The fugacity of water to be plugged in Equation (5) was obtained from the relation:

$${\mathrm{f}}_{{\mathrm{H}}_2\mathrm{O}}={\mathrm{P}}_{\mathrm{t}\mathrm{o}\mathrm{t}}·{\mathrm{X}}_{{\mathrm{H}}_2\mathrm{O}}·{\mathsf{\phi }}_{{\mathrm{H}}_2\mathrm{O}}$$

(8)

using the average values of the molar fraction of water, ${\mathrm{X}}_{{\mathrm{H}}_2\mathrm{O}}$ = 0.433 (±0.002), and the fugacity coefficient of H₂O, ${\mathsf{\phi }}_{{\mathrm{H}}_2\mathrm{O}}$ = 1.008 (±0.000), at T = 1120 °C and ${\mathrm{P}}_{\mathrm{t}\mathrm{o}\mathrm{t}}$ = 2879 bar, in the three preferred degassing models by Caliro et al. [15] for CO₂ = 2 wt%.

Owing to the mantle provenance of the trachybasaltic melt, its initial δ³⁴S value was set equal to the pristine mantle value, 0 ‰, as observed in many hydrothermal–magmatic systems and ore deposits [6,84]. The initial sulfur concentration of the trachybasaltic melt is considered to be 2200 mg/kg [15]. The variations in the δ³⁴S value of the melt caused by isothermal degassing under closed-system conditions were computed utilizing the following equation [4,5,6]:

$${\mathsf{\delta }}^{34}{\mathrm{S}}_{\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t},\mathrm{f}}={\mathsf{\delta }}^{34}{\mathrm{S}}_{\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t},\mathrm{i}}+\left(\mathrm{F}-1\right)·{\mathsf{\epsilon }}_{\mathrm{g}\mathrm{a}\mathrm{s}-\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}$$

(9)

in which subscripts melt,f and melt,i identify the final and initial states of the melt, respectively; F refers to the fraction of S remaining in the melt; and ${\mathsf{\epsilon }}_{\mathrm{g}\mathrm{a}\mathrm{s}-\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}$ is the gas–melt equilibrium enrichment factor of S isotopes, which is related to the corresponding equilibrium fractionation factor, ${\mathsf{\alpha }}_{\mathrm{g}\mathrm{a}\mathrm{s}-\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}$, by the relation:

$${\mathsf{\epsilon }}_{\mathrm{g}\mathrm{a}\mathrm{s}-\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}=1000·\left({\mathsf{\alpha }}_{\mathrm{g}\mathrm{a}\mathrm{s}-\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}-1\right)$$

(10)

The gas–melt fractionation factor was calculated using the fractionation factors experimentally determined by Fiege et al. [85] because they filled a gap in knowledge since the only previous experimental study performed at magmatic T (800–1000 °C) on the fractionation pairs ${\mathrm{S}\mathrm{O}}_4^{2-}-{\mathrm{S}}^{2-}$ and ${\mathrm{S}\mathrm{O}}_4^{2-}-{\mathrm{H}}_2\mathrm{S}$ was carried out utilizing molten salts, i.e., Na₂SO₄ and Na₂S [86]. Fiege et al. [85] proposed the following relation:

$${\mathsf{\alpha }}_{\mathrm{g}\mathrm{a}\mathrm{s}-\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}=\mathrm{A}·{\mathsf{\alpha }}_{{\mathrm{S}\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}-{\mathrm{S}\mathrm{O}}_4^{2-}\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}+\mathrm{B}·{\mathsf{\alpha }}_{{\mathrm{H}}_2\mathrm{S},\mathrm{g}\mathrm{a}\mathrm{s}-{\mathrm{S}}^{2-},\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}+\mathrm{C}·{\mathsf{\alpha }}_{{\mathrm{S}\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}-{\mathrm{S}}^{2-},\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}+\mathrm{D}·{\mathsf{\alpha }}_{{\mathrm{H}}_2\mathrm{S},\mathrm{g}\mathrm{a}\mathrm{s}-{\mathrm{S}\mathrm{O}}_4^{2-}\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}$$

(11)

with:

$${\mathrm{A}=\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}} \mathrm{I}\mathrm{F} {\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}}\le {\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_4^{2-},\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}} \mathrm{E}\mathrm{L}\mathrm{S}\mathrm{E} \mathrm{A}={\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_4^{2-},\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}$$

(12)

$${\mathrm{B}=\mathrm{y}}_{{\mathrm{H}}_2\mathrm{S},\mathrm{g}\mathrm{a}\mathrm{s}} \mathrm{I}\mathrm{F} {\mathrm{y}}_{{\mathrm{H}}_2\mathrm{S},\mathrm{g}\mathrm{a}\mathrm{s}}\le {\mathrm{y}}_{{\mathrm{S},}^{2-}\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}} \mathrm{E}\mathrm{L}\mathrm{S}\mathrm{E} \mathrm{B}={\mathrm{y}}_{{\mathrm{S},}^{2-}\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}$$

(13)

$$\mathrm{C}=0 \mathrm{I}\mathrm{F} {\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}}\le {\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_4^{2-},\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}} \mathrm{E}\mathrm{L}\mathrm{S}\mathrm{E} \mathrm{C}={\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}}-{\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_4^{2-},\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}$$

(14)

$$\mathrm{D}=0 \mathrm{I}\mathrm{F} {\mathrm{y}}_{{\mathrm{H}}_2\mathrm{S},\mathrm{g}\mathrm{a}\mathrm{s}}\le {\mathrm{y}}_{{\mathrm{S},}^{2-}\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}} \mathrm{E}\mathrm{L}\mathrm{S}\mathrm{E} \mathrm{D}={\mathrm{y}}_{{\mathrm{H}}_2\mathrm{S},\mathrm{g}\mathrm{a}\mathrm{s}}-{\mathrm{y}}_{{\mathrm{S},}^{2-}\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}$$

(15)

The fluid–melt S isotope fractionation factors of Fiege et al. [85] are:

$${\mathsf{\alpha }}_{{\mathrm{S}\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}-{\mathrm{S}\mathrm{O}}_4^{2-}\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}=0.9985\pm 0.0007, {\mathsf{\alpha }}_{{\mathrm{H}}_2\mathrm{S},\mathrm{g}\mathrm{a}\mathrm{s}-{\mathrm{S}}^{2-},\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}=1.0067\pm 0.0023,$$

$${\mathsf{\alpha }}_{{\mathrm{S}\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}-{\mathrm{S}}^{2-},\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}=1.0090\pm 0.0025, \mathrm{and} {\mathsf{\alpha }}_{{\mathrm{H}}_2\mathrm{S},\mathrm{g}\mathrm{a}\mathrm{s}-{\mathrm{S}\mathrm{O}}_4^{2-}\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}=0.9962\pm 0.0002.$$

However, the experiments of Fiege et al. [85] were carried out on andesitic melts at 1030 °C and $f_{{\mathrm{O}}_2}$ varying from QFM + 0.8 to QFM + 4.2 log units, while the melt of interest is a trachybasalt at 1120 °C. Therefore, we used the fractionation factors of Fiege et al. [85] and the temperature dependence of the ${\mathrm{S}\mathrm{O}}_2-{\mathrm{H}}_2\mathrm{S}$, ${\mathrm{S}}^{2-}-{\mathrm{S}\mathrm{O}}_4^{2-}$, and ${\mathrm{H}}_2\mathrm{S}-{\mathrm{S}}^{2-}$ enrichment factors of Taylor [3] to compute the fluid–melt S isotope fractionation factors at 1120 °C. The obtained values, ${\mathsf{\alpha }}_{{\mathrm{S}\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}-{\mathrm{S}\mathrm{O}}_4^{2-}\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}=0.99759$, ${\mathsf{\alpha }}_{{\mathrm{H}}_2\mathrm{S},\mathrm{g}\mathrm{a}\mathrm{s}-{\mathrm{S}}^{2-},\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}=1.00662$, ${\mathsf{\alpha }}_{{\mathrm{S}\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}-{\mathrm{S}}^{2-},\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}=1.00864$, and ${\mathsf{\alpha }}_{{\mathrm{H}}_2\mathrm{S},\mathrm{g}\mathrm{a}\mathrm{s}-{\mathrm{S}\mathrm{O}}_4^{2-}\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}=0.99557$, were adopted in melt degassing modeling.

At each degassing step, the δ³⁴S value of the gas separated from the melt is obtained from the relation:

$${\mathsf{\delta }}^{34}{\mathrm{S}}_{\mathrm{g}\mathrm{a}\mathrm{s}}={\mathsf{\delta }}^{34}{\mathrm{S}}_{\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}+{\mathsf{\epsilon }}_{\mathrm{g}\mathrm{a}\mathrm{s}-\mathrm{m}\mathrm{e}\mathrm{l}\mathrm{t}}$$

(16)

Finally, the δ³⁴S values of SO_2(g) and H₂S_(g) are calculated using the equations:

$${\mathsf{\delta }}^{34}{\mathrm{S}}_{{\mathrm{H}}_2{\mathrm{S}}_{\left(\mathrm{g}\right)}}={\mathsf{\delta }}^{34}{\mathrm{S}}_{\mathrm{g}\mathrm{a}\mathrm{s}}-{\mathsf{\epsilon }}_{{\mathrm{S}\mathrm{O}}_2-{\mathrm{H}}_2\mathrm{S}}·{\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}}$$

(17)

$${\mathsf{\delta }}^{34}{\mathrm{S}}_{{\mathrm{S}\mathrm{O}}_{2\left(\mathrm{g}\right)}}={\mathsf{\epsilon }}_{{\mathrm{S}\mathrm{O}}_2-{\mathrm{H}}_2\mathrm{S}}+{\mathsf{\delta }}^{34}{\mathrm{S}}_{{\mathrm{H}}_2{\mathrm{S}}_{\left(\mathrm{g}\right)}}$$

(18)

The SO₂-H₂S enrichment factor is computed utilizing the relation ([87]; T in K):

$${\mathsf{\epsilon }}_{{\mathrm{S}\mathrm{O}}_2-{\mathrm{H}}_2\mathrm{S}}=4.7·{\left(1000/\mathrm{T}\right)}^2-0.5$$

(19)

Strictly speaking, Equation (19) is valid in the temperature range 350–1050 °C, but it was extrapolated to 1120 °C to obtain the ${\mathsf{\epsilon }}_{{\mathrm{S}\mathrm{O}}_2-{\mathrm{H}}_2\mathrm{S}}$ value with acceptable approximation.

3.5. Modeling the SO₂ Disproportionation Reaction in the Deep Hydrothermal Reservoir

The temperature of the deep hydrothermal reservoir was calculated using the H₂S-CO₂ gas-geothermometer of Marini et al. [58], calibrated for the saturation decompression path of Solfatara fluids involving a vapor phase and a brine containing 33.5 wt% NaCl. The obtained temperature of the deep hydrothermal reservoir was then used to compute the δ³⁴S values of SO_2(g) and H₂S_(g) in the gas mixture entering the deep hydrothermal reservoir using again Equations (17)–(19).

We assume that the chemical effects caused by the SO₂ disproportionation reaction (further details in Appendix A, Appendix A.1) consist of the conversion of one-quarter of the SO₂ into H₂S and the remaining three-quarters into sulfate ion or sulfuric acid, as dictated by the stoichiometry of reaction (A1). Thus, they are described by the relations:

$${\mathrm{y}}_{{\mathrm{H}}_2\mathrm{S},\mathrm{D}\mathrm{R}}={1-\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}}+{\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}}/4$$

(20)

$${\mathrm{y}}_{{\mathrm{S}\mathrm{O}}_4^{2-},\mathrm{D}\mathrm{R}}={3/4·\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}}$$

(21)

in which ${\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}}$ is the value given by Equation (7). Equations (20) and (21) were used to compute the molar fractions of H₂S and ${\mathrm{S}\mathrm{O}}_4^{2-}$ in the deep hydrothermal reservoir.

The isotopic effects brought about by SO₂ disproportionation are defined by the equation:

$${\mathsf{\delta }}^{34}{\mathrm{S}}_{{\mathrm{H}}_2\mathrm{S},\mathrm{D}\mathrm{R},\mathrm{o}\mathrm{u}\mathrm{t}}={\mathsf{\delta }}^{34}{\mathrm{S}}_{\mathrm{g}\mathrm{a}\mathrm{s}}-{\mathsf{\epsilon }}_{{\mathrm{S}\mathrm{O}}_4^{2-}-{\mathrm{H}}_2\mathrm{S}}·{\mathrm{y}}_{{\mathrm{S}\mathrm{O}}_4^{2-},\mathrm{D}\mathrm{R}}$$

(22)

The ${\mathrm{S}\mathrm{O}}_4^{2-}$-H₂S enrichment factor is calculated using the relation ([88]; T in K):

$${\mathsf{\epsilon }}_{{\mathrm{S}\mathrm{O}}_4^{2-}-{\mathrm{H}}_2\mathrm{S}}=2.119·{{10}^{-4}·\left(1000/\mathrm{T}\right)}^6+9.332·{{10}^{-3}·\left(1000/\mathrm{T}\right)}^4-0.8910{·\left(1000/\mathrm{T}\right)}^3+9.104·{\left(1000/\mathrm{T}\right)}^2-2.222·\left(1000/\mathrm{T}\right)+0.6131$$

(23)

which holds in the temperature interval 0–2000 °C.

Following Marini et al. [16,58], we assume that the gas mixture leaving the deep hydrothermal reservoir does not experience any significant change in the concentration and δ³⁴S value of H₂S_(g) along the ascent path towards the surface discharge (see Section 3.1). Therefore, ${\mathsf{\delta }}^{34}{\mathrm{S}}_{{\mathrm{H}}_2{\mathrm{S}}_{\mathrm{D}\mathrm{R},\mathrm{o}\mathrm{u}\mathrm{t}}}$ value is expected to be the same as the ${\mathsf{\delta }}^{34}{\mathrm{S}}_{{\mathrm{H}}_2\mathrm{S}}$ value measured in the Bocca Grande fumarolic effluents. This equality condition is considered in our calculation strategy (see Section 3.6).

Similarly, the assumption of S conservation along the transit from the deep hydrothermal reservoir to the surface was adopted to calculate the concentrations of H₂S, SO₂, and total gaseous S at the inlet of deep hydrothermal reservoir, based on the measured H₂S concentration, ${\mathrm{X}}_{{\mathrm{H}}_2\mathrm{S},\mathrm{B}\mathrm{G}}$, using the relations:

$${\mathrm{X}}_{{\mathrm{H}}_2\mathrm{S},\mathrm{D}\mathrm{R},\mathrm{i}\mathrm{n}}={\mathrm{X}}_{{\mathrm{H}}_2\mathrm{S},\mathrm{B}\mathrm{G}}/\left\{1+\mathrm{¼}·\left[{\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}}/\left(1-{\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}}\right)\right]\right\}$$

(24)

$${\mathrm{X}}_{{\mathrm{S}\mathrm{O}}_2,\mathrm{D}\mathrm{R},\mathrm{i}\mathrm{n}}={\mathrm{X}}_{{\mathrm{H}}_2\mathrm{S},\mathrm{D}\mathrm{R},\mathrm{i}\mathrm{n}}·\left[{\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}}/\left(1-{\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}}\right)\right]$$

(25)

$$X_{S_{tot},DR,in}=X_{H_{2}S,DR,in}+X_{{SO}_2,DR,in}$$

(26)

3.6. Calculation Strategy

As already recalled in Section 3.4, the melt at depths ≥ 8 km was assumed to be at constant temperature, T = 1120 °C; constant pressure, ${\mathrm{P}}_{\mathrm{t}\mathrm{o}\mathrm{t}}$ = 2879 bar; and constant ${\log \mathrm{f}}_{{\mathrm{O}}_2}$ = −8.28, at all degassing steps, while the initial δ³⁴S value of the melt was assumed to be 0 ‰, which is the pristine mantle value. Therefore, the only unknown, at each degassing step, is the fraction of S remaining in the melt, F. Thus, the data of Caliro et al. [15], apart from the 10 data of the period August 1998–March 2001, and the data of Allard et al. [78] were ordered by sampling date, from the oldest to the most recent, and the value of F, at each degassing step, was changed iteratively until the ${\mathsf{\delta }}^{34}{\mathrm{S}}_{{\mathrm{H}}_2\mathrm{S}}$ at the outlet of the deep hydrothermal reservoir given by Equation (22) resulted in being equal to the corresponding ${\mathsf{\delta }}^{34}{\mathrm{S}}_{{\mathrm{H}}_2\mathrm{S}}$ value measured in the Bocca Grande fumarolic effluents, within analytical uncertainty. For F ≤ 0.7, the error on F ranges between 0.129 and 0.038 units, with an average value of 0.064 units. For F > 0.7, the error is higher and difficult to define due to the considerable oscillations of the analytical ${\mathsf{\delta }}^{34}{\mathrm{S}}_{{\mathrm{H}}_2\mathrm{S}}$ values.

The ten ${\mathsf{\delta }}^{34}{\mathrm{S}}_{{\mathrm{H}}_2\mathrm{S}}$ data of the period August 1998–March 2001 (range +1.2 to +2.8‰) were disregarded, as was also done by Caliro et al. [15], because they were at variance with all the other available data (range −1.26 to +0.80‰), probably because they were affected by sample conservation and/or analytical issues. The chemical data of Allard et al. [78], for the period March 1984–January 1987, give H₂S equilibrium temperatures varying between 502 and 773 °C. These values contrast with the H₂S equilibrium temperatures of 714–761 °C, which are computed using the chemical data of Cioni et al. [59,60] and Caliro et al. [62]. Therefore, the H₂S equilibrium temperatures for the period March 1984–January 1987 were computed by interpolation between 714 and 761 °C. Input data and results are reported in the Supplementary Material File.

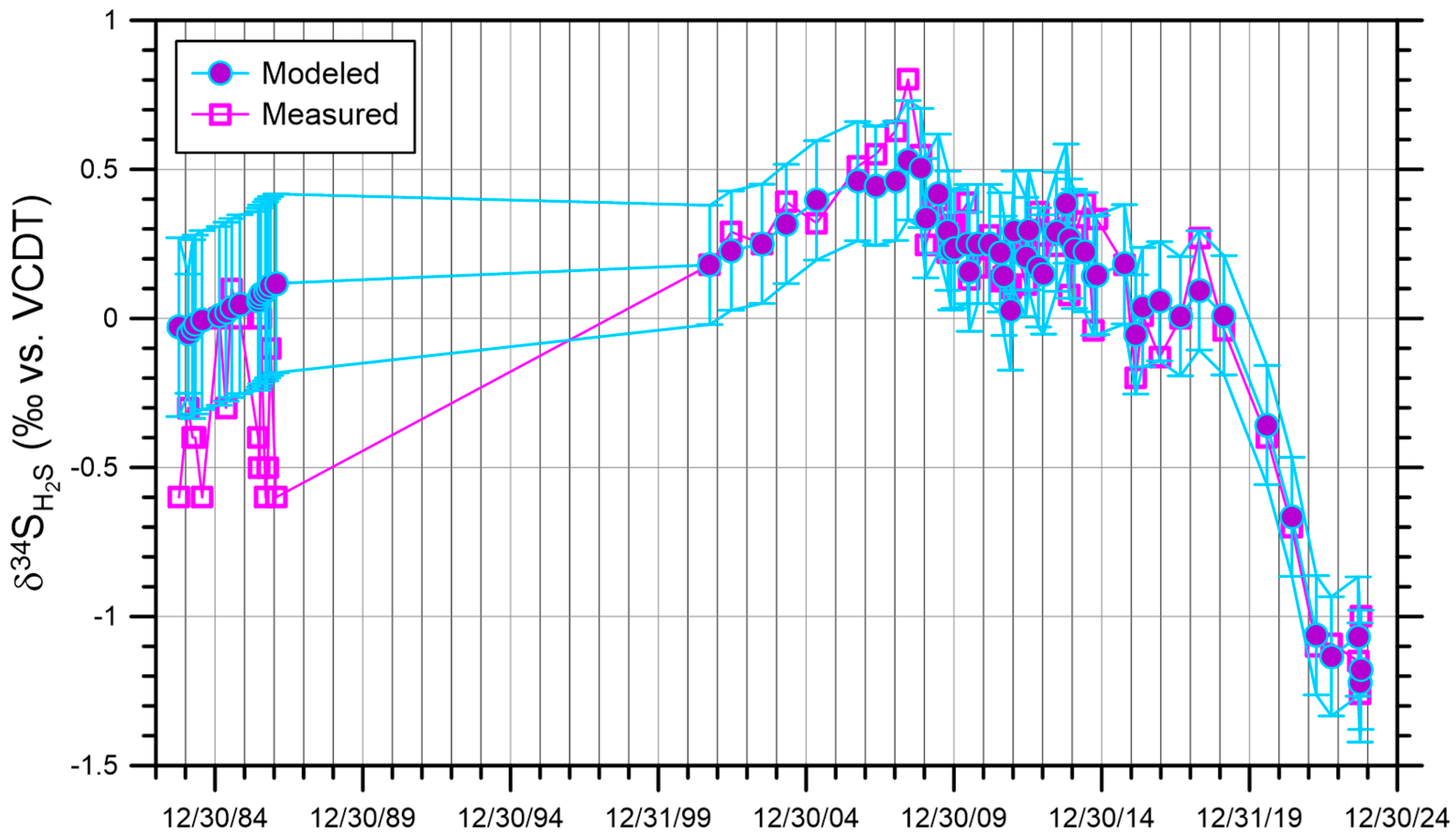
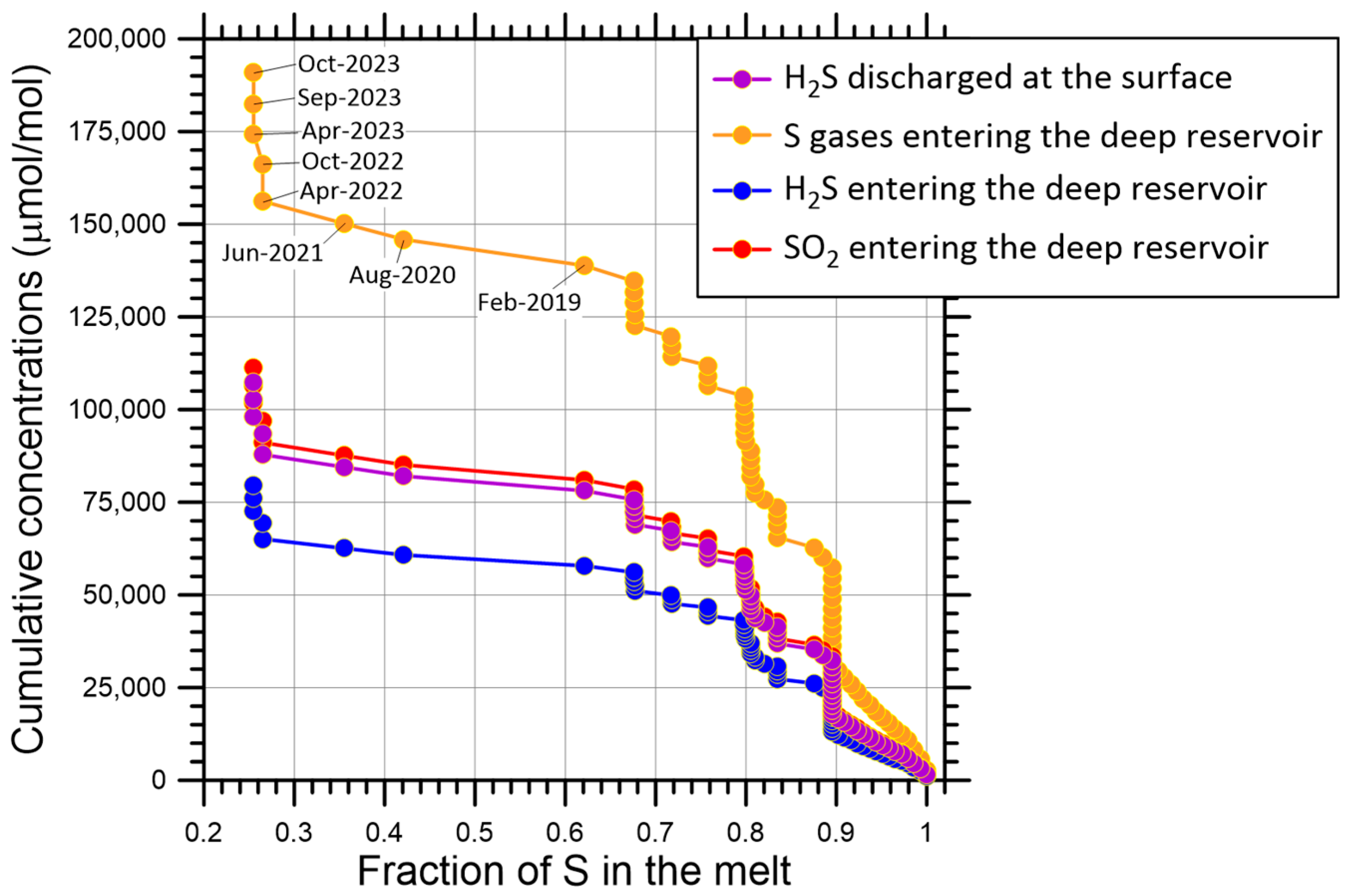
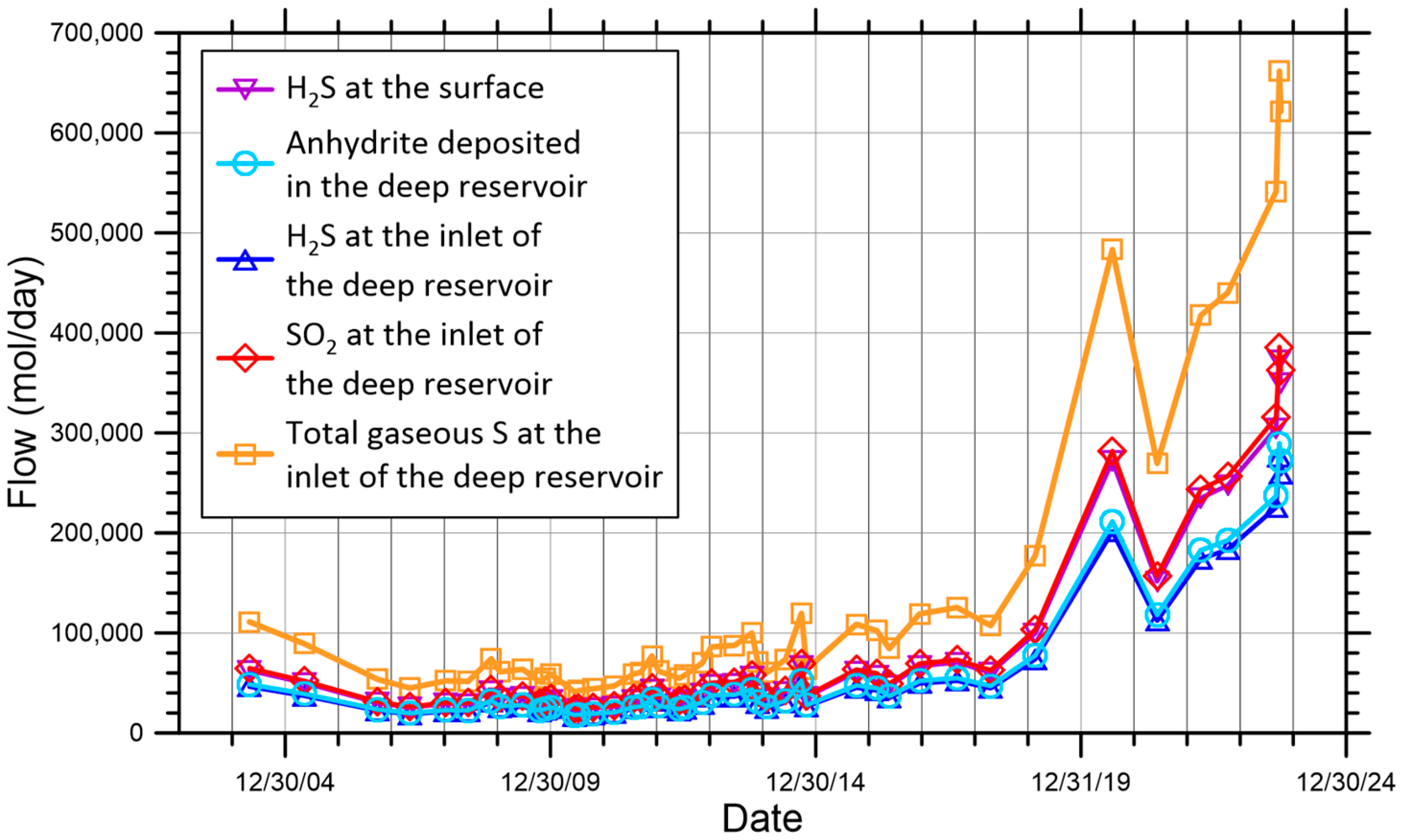
4. Results and Discussion

There is generally good agreement, within analytical uncertainty, between the ${\mathsf{\delta }}^{34}{\mathrm{S}}_{{\mathrm{H}}_2\mathrm{S}}$ values at the outlet of the deep hydrothermal reservoir given by Equation (22) and the corresponding ${\mathsf{\delta }}^{34}{\mathrm{S}}_{{\mathrm{H}}_2\mathrm{S}}$ values measured in the Bocca Grande fumarolic effluents (Figure 3).

Exceptions are mostly samples from the time interval 1983–1987, all but one collected by Allard et al. [78], which have ${\mathsf{\delta }}^{34}{\mathrm{S}}_{{\mathrm{H}}_2\mathrm{S}}$ values lower than predicted by the model, and the sample obtained on June 11th, 2008, which has a ${\mathsf{\delta }}^{34}{\mathrm{S}}_{{\mathrm{H}}_2\mathrm{S}}$ value slightly higher than that computed by the model. As already noted above, for the early samples, it is probable that elemental S or the S stored in sulfide/bisulfide and/or sulfate minerals was remobilized and entered the magmatic–hydrothermal gas flow due to heating of the Solfatara magmatic–hydrothermal system [77,78]. Accepting this explanation for the discrepancies between the modeled and measured ${\mathsf{\delta }}^{34}{\mathrm{S}}_{{\mathrm{H}}_2\mathrm{S}}$ values of early samples, it can be concluded that magma degassing and SO₂ disproportionation in the deep hydrothermal reservoir satisfactorily explain the sulfur isotope data of Bocca Grande fumarolic effluents.

Further details on the results of our model are shown in the diagram of Figure 4, in which the fraction of S remaining in the melt is used as a reference variable because it describes the extent of the magma degassing process.

The diagram shows the evolution of the sulfur isotope data predicted by the model for the melt and the gas mixture in different points of the Solfatara magmatic–hydrothermal system, namely, the δ³⁴S value of:

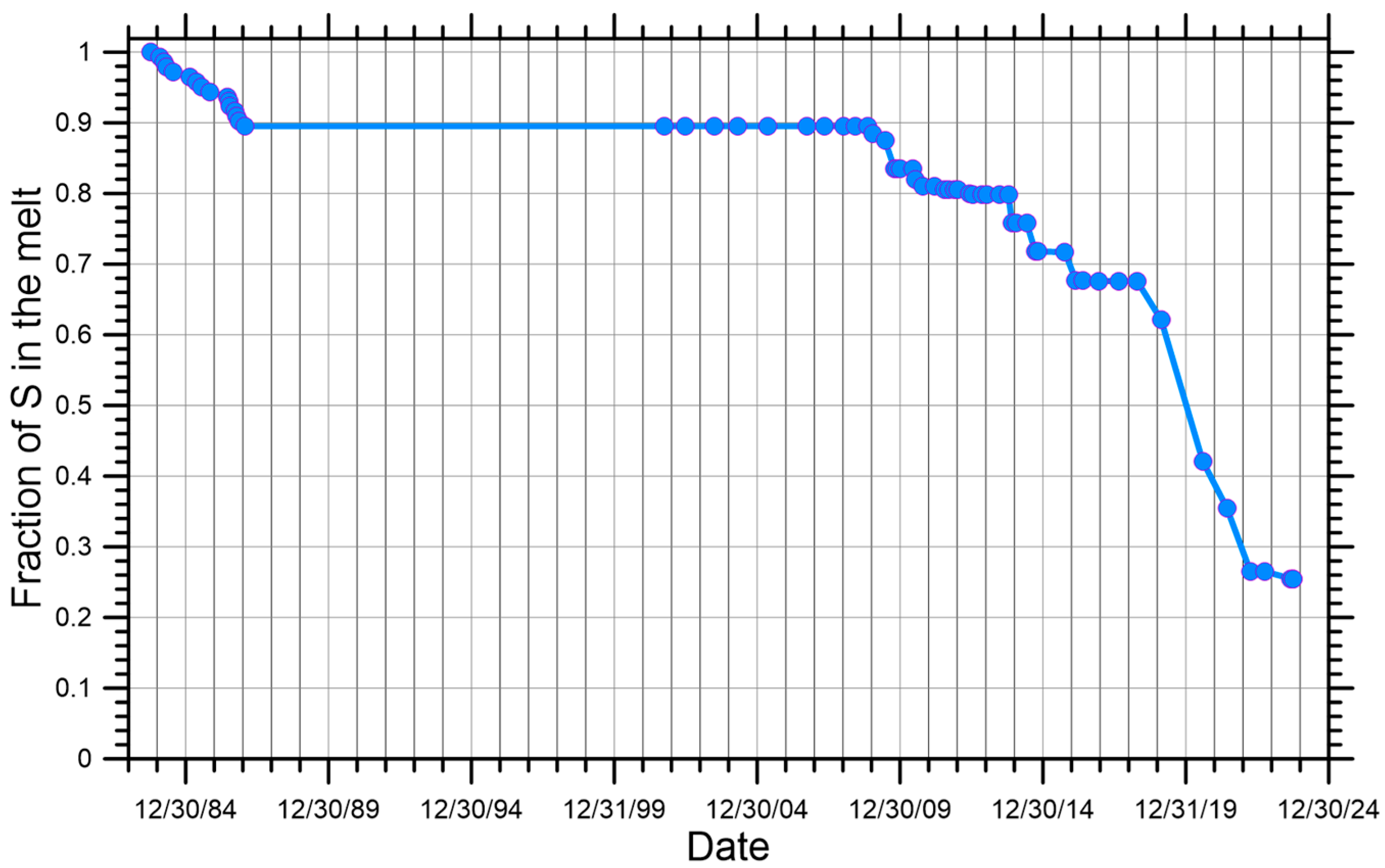
(i)

Total ionic S dissolved in the melt, which decreases from 0.00 to −2.40‰ and has a perfect linear relationship with F, as dictated by Equation (9).

(ii)

Total gaseous S in the gas mixture separated from the melt, which declines from +3.22 to +0.82‰ and, again, has an exact linear relationship with F, constrained by Equations (9) and (16).

(iii)

Sulfur dioxide in the gas mixture separated from the melt, which diminishes from +4.02 to +1.62‰ and, again, has a perfect linear relationship with F, defined by Equations (9) and (16)–(18).

(iv)

Hydrogen sulfide in the gas mixture separated from the melt, which decreases from +2.10 to −0.30‰ and has an exact linear relationship with F, constrained by Equations (9), (16) and (17).

(v)

Sulfur dioxide in the gas mixture at the inlet of the deep hydrothermal reservoir, which declines from +5.21 to +1.90‰, describing a non-linear trend with a weak upward curvature and several small oscillations. These are due to the joint effects of magma degassing and SO₂ disproportionation. It is worth noting that magma degassing, in the absence of other processes, would determine a linear trend, as in previous points. Moreover, SO₂ disproportionation is responsible for the deviations from linearity because of the temperature changes in the deep hydrothermal reservoir from 671 to 1022 °C.

(vi)

Hydrogen sulfide in the gas mixture at the inlet of the deep hydrothermal reservoir, which oscillates from +0.94 to −0.83‰, delineating a generally decreasing, non-linear trend with a weak downward curvature and numerous little fluctuations due to the combined influences of magma degassing and SO₂ disproportionation, similar to the previous point.

(vii)

Hydrogen sulfide in the gas mixture at the outlet of the deep hydrothermal reservoir, which varies from +0.53 to −1.22‰, outlining a trend similar to that of the previous point but shifted downward by 0.46–0.37‰ units, corresponding to the difference $\left({\mathsf{\epsilon }}_{{\mathrm{S}\mathrm{O}}_2-{\mathrm{H}}_2\mathrm{S}}·{\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}}\right)-\left({\mathsf{\epsilon }}_{{\mathrm{S}\mathrm{O}}_4^{2-}-{\mathrm{H}}_2\mathrm{S}}·{3/4·\mathrm{y}}_{\mathrm{S}{\mathrm{O}}_2,\mathrm{g}\mathrm{a}\mathrm{s}}\right)$.

It is worth noting that the analytical data, apart from the early ones, are distributed close to the trend of H₂S_(g) in the gas mixture at the outlet of the deep hydrothermal reservoir, as already observed in Figure 3. Last but not least, the major decrease in F, from 0.676 to 0.265, occurred in 4 years only, from April 2018 to April 2022, with a loss of 41.1% (±6.4%) of the sulfur initially stored in the melt, which amounts to 2200 mg/kg according to Caliro et al. [15]. Afterwards, from April 2022 to October 2023, F experienced a very small decrement, from 0.265 to 0.255, even if this evidence is based on a limited observation time.

Since the fraction of S remaining in the melt is a cumulative parameter, it is advisable to contrast it with the measured cumulative concentrations of H₂S discharged at the surface (rather than the individual values) and to also consider the cumulative concentrations of H₂S, SO₂, and total gaseous S entering the deep hydrothermal reservoir predicted by the model (Figure 5). The cumulative concentrations of H₂S measured at the surface are higher than those of H₂S entering the deep hydrothermal reservoir (i.e., released from the melt) by a factor 1.35; however, the cumulative concentrations of both total gaseous S and SO₂ entering the deep hydrothermal reservoir are higher than those of H₂S measured at the surface by a factor 1.78 and 1.04, respectively, indicating that most S released from the melt is converted into sulfate by the SO₂ disproportionation reaction and is sequestered as anhydrite in the deep hydrothermal reservoir (see Appendix A, Appendix A.1 and Appendix A.3).

Using the H₂S flow data at the surface for the time interval April 2004–October 2023 [15] as well as the results of our model, we have computed the flows of H₂S, SO₂, and total gaseous S entering the deep hydrothermal reservoir using simple proportions between flows and concentrations, as well as the flow of anhydrite deposited in the deep hydrothermal reservoir, by difference between the flow of total gaseous S entering the deep hydrothermal reservoir and the flow of H₂S leaving it (Figure 6).

It is evident that a marked increase in all flows occurred from April 2018 to October 2023, including the flow of anhydrite deposited in the deep hydrothermal reservoir, which varied from a minimum value of 46,999 mol/day in April 2018 to a maximum value of 289,448 mol/day in September 2023. The last two figures, corresponding to 6.4 and 39.4 metric tons/day, contrast with the assertion on the rarity of anhydrite in the Solfatara magmatic–hydrothermal system [15], which is based on the occurrence of anhydrite in the geothermal wells drilled in the Campi Flegrei, as reported in Piochi et al. [45]. This topic is further discussed in Appendix A and Appendix A.3.

Apart from the early years, whose data are not representative (see above), the S loss experienced by the melt was negligible before 2008, moderate from 2009 to April 2018 (with some fluctuations), very high from April 2018 to April 2022, and returned to be moderate afterwards, from April 2022 to October 2023 (Figure 7). Thus, magma degassing has caused a considerable decrease in the fraction of sulfur remaining in the melt, from the initial value of 1 in October 1983 to the final value of 0.255 in October 2023, which is the end of the considered 40-year time interval. Nevertheless, the major decrement in F, from 0.676 to 0.265, has occurred in 4 years only, from April 2018 to April 2022, with a loss of 41.1% (±6.4%) of the sulfur initially stored in the melt, as already noted above. This marked S loss in 2018–2022 might be explained by the high solubility of sulfur in the melt, causing its preferential separation during late degassing stages, as suggested by the simplified fluid–melt partitioning and solubility relations of Giggenbach [89,90], although degassing of real magmas is undoubtedly more complicated, and a rigorous thermodynamic model is needed to describe S behavior in silicate melts [91,92,93]. Interestingly, the marked S loss by magma degassing in the years 2018–2022 was accompanied by simultaneous peak values of temperature and fluid pressure in both the deep and intermediate hydrothermal reservoirs [16].

5. Conclusions

Closed-system degassing of the melt stored in the magma chamber, located at depths ≥ 8 km, and disproportionation of SO₂ in the deep hydrothermal reservoir, positioned at 6.5–7.5 km depth (and hosted in carbonate rocks where H₂S equilibrates), explain the concentration and the δ³⁴S value of H₂S_(g) of the Bocca Grande fumarolic effluents. In simple words, melt degassing transfers both SO₂ and H₂S to the separated gas phase. Then, due to SO₂ disproportionation in the deep hydrothermal reservoir, three-quarters of SO₂ is converted into H₂SO₄ and sequestered as anhydrite, while one-quarter of SO₂ is converted into H₂S. The production of anhydrite in the deep hydrothermal reservoir hosted in carbonate rocks, up to 6.4 and 39.4 metric tons/day in the period April 2018–October 2023, is supported by its abundance in the carbonate–evaporite geothermal systems of Central Italy and its stability under the temperature and pressure conditions in the deep hydrothermal reservoir.

We reached these conclusions referring to the conceptual model of the Solfatara magmatic–hydrothermal system of Marini et al. [16] and assuming that the magma chamber was filled at the end of the 1982–1984 crisis and there were no subsequent refilling episodes, as suggested by gas geochemistry [57]. This way, we contextualized magma degassing and SO₂ disproportionation, the two most important and common processes controlling S isotope fractionation in active magmatic–hydrothermal systems and ore deposits which are the past analogues of magmatic–hydrothermal systems [6,13]. Furthermore, the sulfur isotope data of the Bocca Grande fumarolic effluents suggest that 41.1% (±6.4%) of the S initially dissolved in the melt (2200 mg/kg, according to Caliro et al. [15]) was lost through degassing from April 2018 to April 2022. This marked S loss might be due to the high solubility of sulfur in the melt, which brings about its preferential separation during the late degassing stages. These findings improve forecasting and hazard assessment at Solfatara and similar magmatic–hydrothermal systems.