Global Rayleigh Wave Attenuation and Group Velocity from International Seismological Centre Data
Abstract
1. Introduction
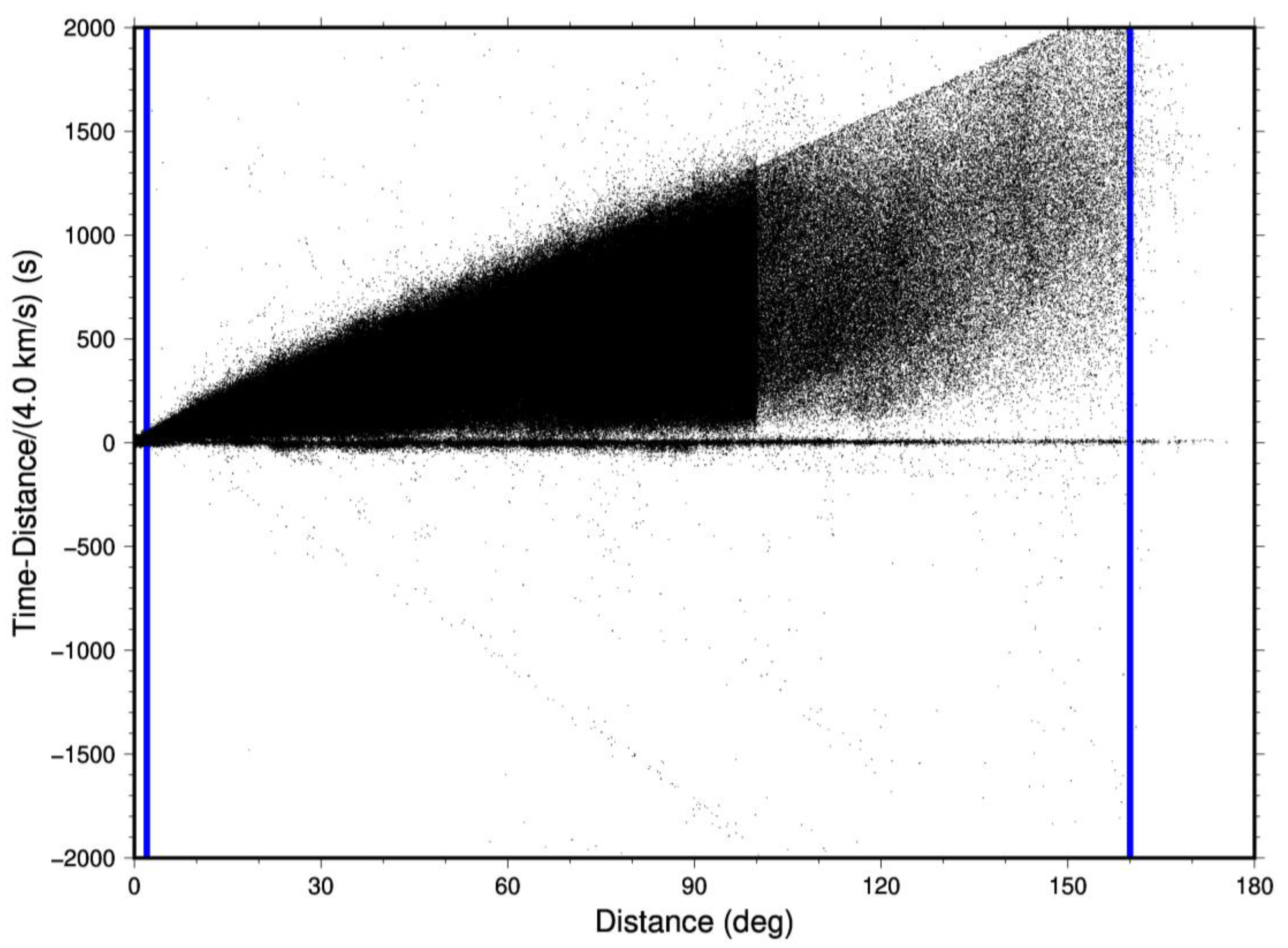
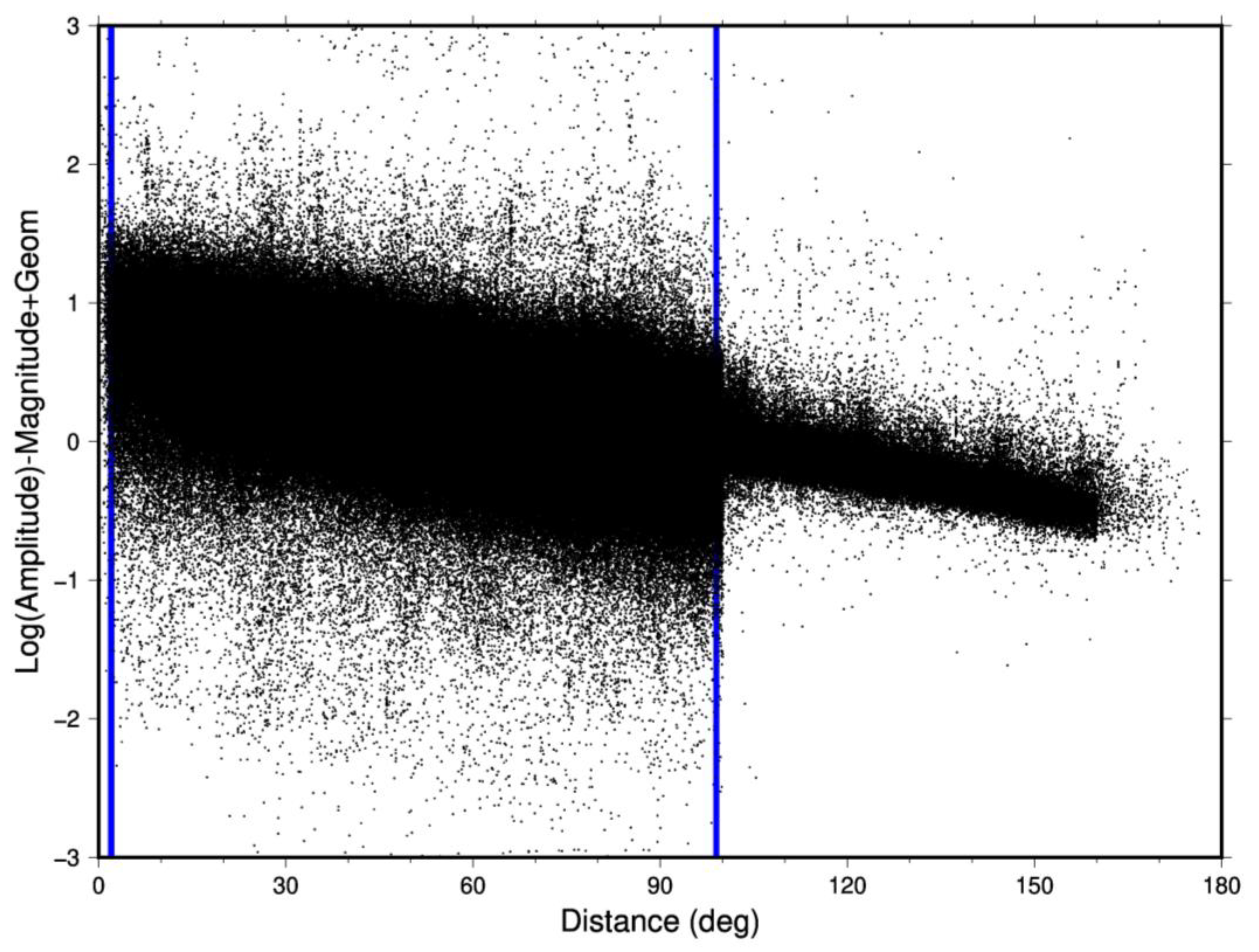
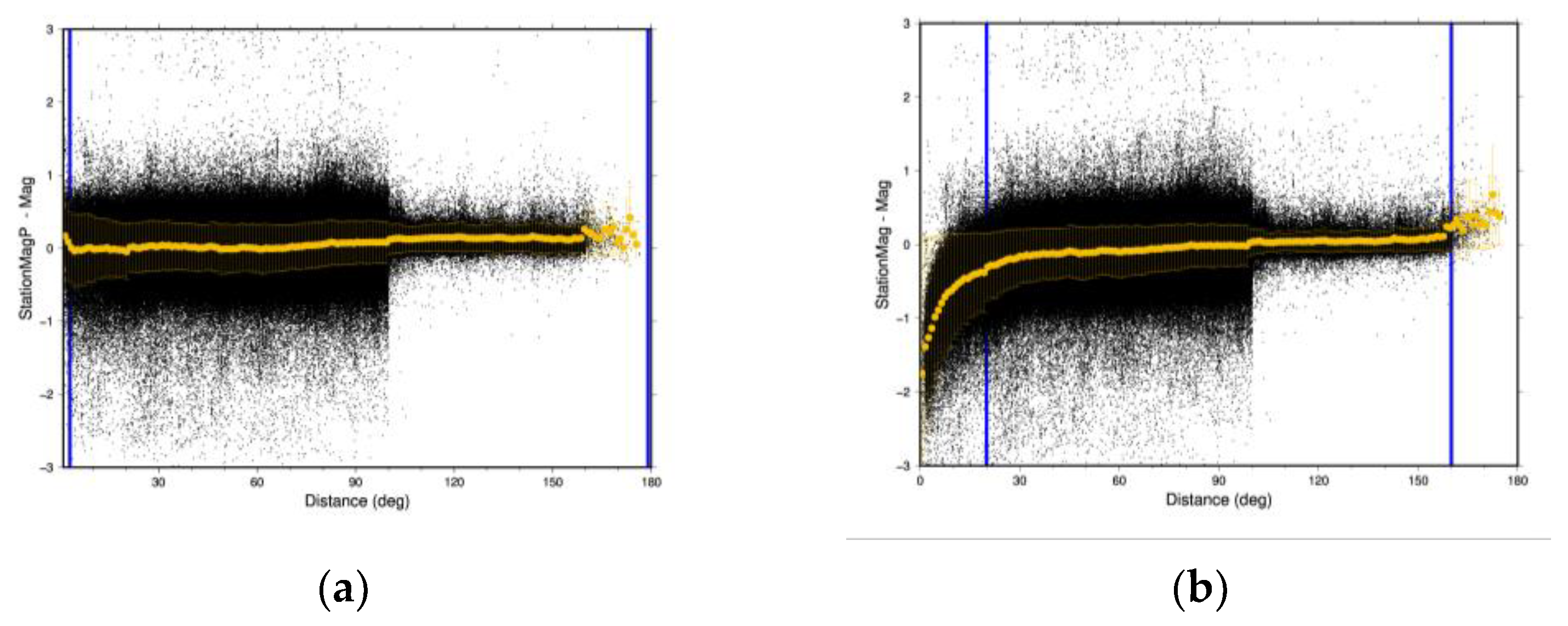
2. Methods
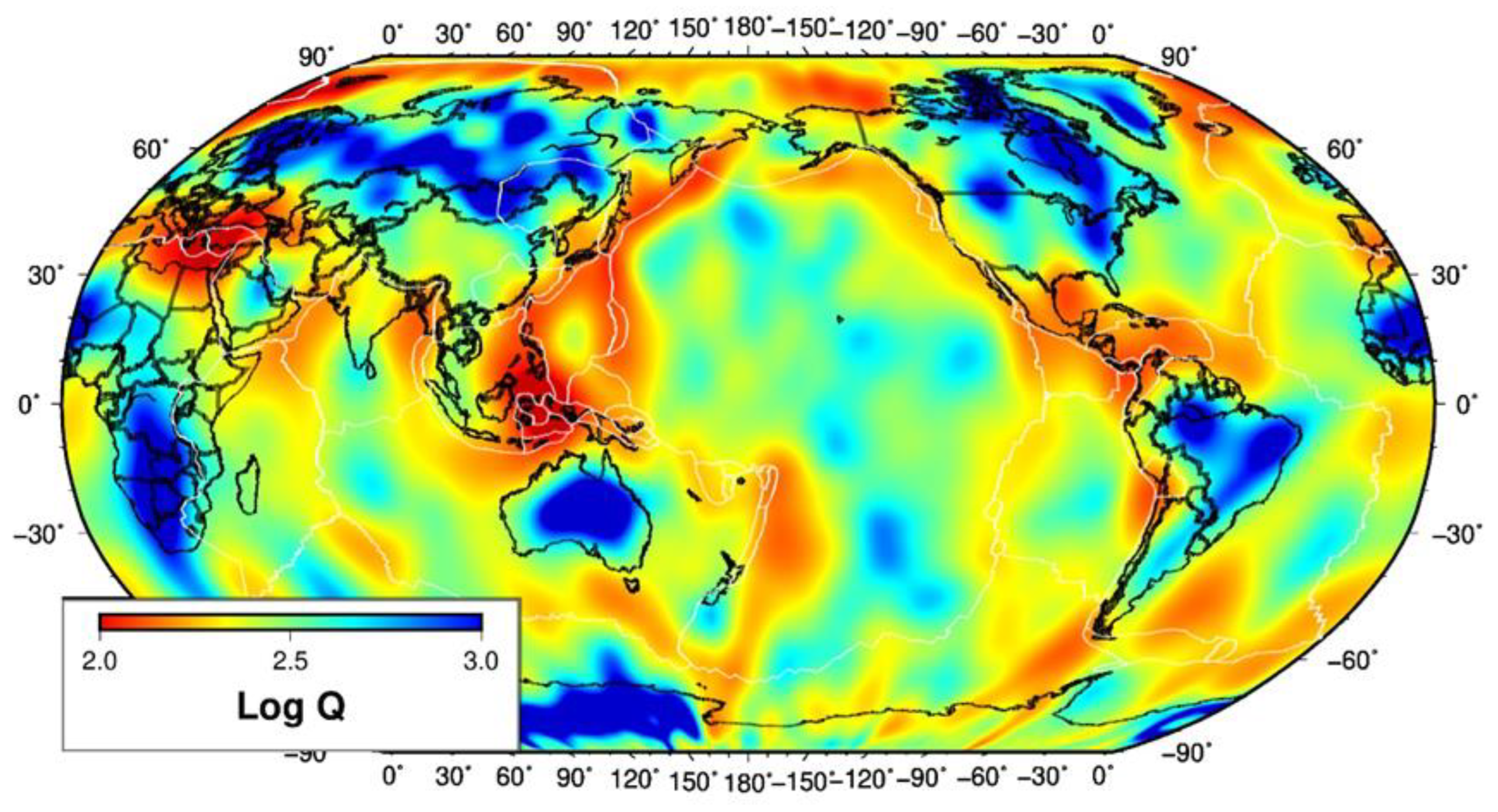
3. Results
4. Discussion
4.1. Other Studies
4.2. Interpretation
5. Conclusions
Supplementary Materials
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Adenis, A.; Debayle, E.; Ricard, Y. Seismic Evidence for Broad Attenuation Anomalies in the Asthenosphere beneath the Pacific Ocean. Geophys. J. Int. 2017, 209, 1677–1698. [Google Scholar] [CrossRef]
- Ma, Z.; Masters, G.; Mancinelli, N. Two-Dimensional Global Rayleigh Wave Attenuation Model by Accounting for Finite-Frequency Focusing and Defocusing Effect. Geophys. J. Int. 2016, 204, 631–649. [Google Scholar] [CrossRef]
- Billien, M.; Lévêque, J.; Trampert, J. Global Maps of Rayleigh Wave Attenuation for Periods between 40 and 150 Seconds. Geophys. Res. Lett. 2000, 27, 3619–3622. [Google Scholar] [CrossRef]
- Dalton, C.A.; Ekström, G. Constraints on Global Maps of Phase Velocity from Surface-Wave Amplitudes. Geophys. J. Int. 2006, 167, 820–826. [Google Scholar] [CrossRef]
- Dalton, C.A.; Ekström, G. Global Models of Surface Wave Attenuation. J. Geophys. Res. 2006, 111, 1–19. [Google Scholar] [CrossRef]
- Dalton, C.A.; Ekström, G.; Dziewoński, A.M. The Global Attenuation Structure of the Upper Mantle. J. Geophys. Res. 2008, 113, 1–24. [Google Scholar] [CrossRef]
- Magrini, F.; Boschi, L.; Gualtieri, L.; Lekić, V.; Cammarano, F. Rayleigh-Wave Attenuation across the Conterminous United States in the Microseism Frequency Band. Sci. Rep. 2021, 11, 10149. [Google Scholar] [CrossRef]
- Levshin, A.L.; Ritzwoller, M.H.; Barmin, M.P.; Villaseñor, A.; Padgett, C.A. New Constraints on the Arctic Crust and Uppermost Mantle: Surface Wave Group Velocities, Pn, and Sn. Phys. Earth Planet. Inter. 2001, 123, 185–204. [Google Scholar] [CrossRef]
- Ritzwoller, M.H. Global Surface Wave Diffraction Tomography. J. Geophys. Res. 2002, 107, 1–13. [Google Scholar] [CrossRef]
- Yanovskaya, T. 3D S-Wave Velocity Pattern in the Upper Mantle beneath the Continent of Asia from Rayleigh Wave Data. Phys. Earth Planet. Inter. 2003, 138, 263–278. [Google Scholar] [CrossRef]
- Yang, X.; Taylor, S.R.; Patton, H.J. The 20-s Rayleigh Wave Attenuation Tomography for Central and Southeastern Asia. J. Geophys. Res. 2004, 109, 2004JB003193. [Google Scholar] [CrossRef]
- Levshin, A.L.; Yang, X.; Barmin, M.P.; Ritzwoller, M.H. Midperiod Rayleigh Wave Attenuation Model for Asia: Rayleigh wave attenuation in Asia. Geochem. Geophys. Geosyst. 2010, 11. [Google Scholar] [CrossRef]
- Bao, X.; Song, X.; Li, J. High-Resolution Lithospheric Structure beneath Mainland China from Ambient Noise and Earthquake Surface-Wave Tomography. Earth Planet. Sci. Lett. 2015, 417, 132–141. [Google Scholar] [CrossRef]
- Babikoff, J.C.; Dalton, C.A. Long-Period Rayleigh Wave Phase Velocity Tomography Using USArray. Geochem. Geophys. Geosyst. 2019, 20, 1990–2006. [Google Scholar] [CrossRef]
- Zhou, Y.; Nolet, G.; Dahlen, F.A.; Laske, G. Global Upper-Mantle Structure from Finite-Frequency Surface-Wave Tomography. J. Geophys. Res. 2006, 111, B04304. [Google Scholar] [CrossRef]
- International Seismological Centre On-Line Bulletin 2023. Available online: https://www.isc.ac.uk (accessed on 2 February 2024).
- Hearn, T.M. Two-Dimensional Attenuation and Velocity Tomography of Iran. Geosciences 2022, 12, 397. [Google Scholar] [CrossRef]
- Vincenty, T. Direct and Inverse Solutions of Geodesics on the Ellipsoid with Application of Nested Equations. Surv. Rev. 1975, 22, 88–93. [Google Scholar] [CrossRef]
- Paige, C.C.; Saunders, M.A. Algorithm 583, LSQR: Sparse Linear Equations and Least-Squares Problems. ACM Trans. Math. Softw. 1982, 8, 43–71. [Google Scholar] [CrossRef]
- Paige, C.C.; Saunders, M.A. LSQR: An Algorithm for Sparse Linear Equations and Sparse Least Squares, Trans. ACM Trans. Math. Softw. 1982, 8, 195–209. [Google Scholar] [CrossRef]
- Ewing, W.M.; Jardetzky, W.S.; Press, F. Elastic Waves in Layered Media; McGraw-Hill Book Company: New York, NY, USA, 1957. [Google Scholar]
- Dahlen, F.A.; Tromp, J. Theoretical Global Seismology; Princeton University Press: Princeton, NJ, USA, 1998; ISBN 978-0-691-00116-6. [Google Scholar]
- Bao, X.; Dalton, C.A.; Ritsema, J. Effects of Elastic Focusing on Global Models of Rayleigh Wave Attenuation. Geophys. J. Int. 2016, 207, 1062–1079. [Google Scholar] [CrossRef]
- Chen, H.; Ni, S.; Chu, R.; Chong, J.; Liu, Z.; Zhu, L. Influence of the Off-Great-Circle Propagation of Rayleigh Waves on Event-Based Surface Wave Tomography in Northeast China. Geophys. J. Int. 2018, 214, 1105–1124. [Google Scholar] [CrossRef]
- Hearn, T.M.; Wang, S.; Ni, J.F.; Xu, Z.; Yu, Y.; Zhang, X. Uppermost Mantle Velocities beneath China and Surrounding Regions. J. Geophys. Res. 2004, 109, B11301. [Google Scholar] [CrossRef]
- Rezapour, M.; Pearce, R.G. Bias in Surface-Wave Magnitude Ms Due to Inadequate Distance Corrections. Bull. Seismol. Soc. Am. 1998, 88, 43–61. [Google Scholar] [CrossRef]
- Selby, N.D.; Bowers, D.; Marshall, P.D.; Douglas, A. Empirical Path and Station Corrections for Surface-Wave Magnitude, Ms, Using a Global Network. Geophys. J. Int. 2003, 155, 379–390. [Google Scholar] [CrossRef]
- Zhou, L.; Song, X.; Yang, X.; Zhao, C. Rayleigh Wave Attenuation Tomography in the Crust of the Chinese Mainland. Geochem. Geophys. Geosyst. 2020, 21, e2020GC008971. [Google Scholar] [CrossRef]
- Feng, M.; Assumpção, M.; Van Der Lee, S. Group-Velocity Tomography and Lithospheric S-Velocity Structure of the South American Continent. Phys. Earth Planet. Inter. 2004, 147, 315–331. [Google Scholar] [CrossRef]
- Nascimento, A.V.D.S.; França, G.S.; Chaves, C.A.M.; Marotta, G.S. Rayleigh Wave Group Velocity Maps at Periods of 10–150 s beneath South America. Geophys. J. Int. 2021, 228, 958–981. [Google Scholar] [CrossRef]
- Ekström, G.; Tromp, J.; Larson, E.W.F. Measurements and Global Models of Surface Wave Propagation. J. Geophys. Res. 1997, 102, 8137–8157. [Google Scholar] [CrossRef]
- Durand, S.; Debayle, E.; Ricard, Y. Rayleigh Wave Phase Velocity and Error Maps up to the Fifth Overtone. Geophys. Res. Lett. 2015, 42, 3266–3272. [Google Scholar] [CrossRef]
- Liu, K.; Zhou, Y. Global Rayleigh Wave Phase-Velocity Maps from Finite-Frequency Tomography. Geophys. J. Int. 2016, 205, 51–66. [Google Scholar] [CrossRef][Green Version]
- Zhou, Y.; Dahlen, F.A.; Nolet, G.; Laske, G. Finite-Frequency Effects in Global Surface-Wave Tomography. Geophys. J. Int. 2005, 163, 1087–1111. [Google Scholar] [CrossRef]
- Dalton, C.A.; Ekström, G.; Dziewonski, A.M. Global Seismological Shear Velocity and Attenuation: A Comparison with Experimental Observations. Earth Planet. Sci. Lett. 2009, 284, 65–75. [Google Scholar] [CrossRef]
- Selby, N.D.; Woodhouse, J.H. Controls on Rayleigh Wave Amplitudes: Attenuation and Focusing. Geophys. J. Int. 2000, 142, 933–940. [Google Scholar] [CrossRef]
- Selby, N.D. The Q Structure of the Upper Mantle: Constraints from Rayleigh Wave Amplitudes. J. Geophys. Res. 2002, 107, 2097. [Google Scholar] [CrossRef]
- Pilidou, S.; Priestley, K.; Gudmundsson, Ó.; Debayle, E. Upper Mantle S -Wave Speed Heterogeneity and Anisotropy beneath the North Atlantic from Regional Surface Wave Tomography: The Iceland and Azores Plumes. Geophys. J. Int. 2004, 159, 1057–1076. [Google Scholar] [CrossRef]
- Herrmann, R.B. Computer Programs in Seismology: An Evolving Tool for Instruction and Research. Seism. Res. Lettr. 2013, 84, 1081–1088. [Google Scholar] [CrossRef]
- Abbott, D.H.; Mooney, W.D.; VanTongeren, J.A. The Character of the Moho and Lower Crust within Archean Cratons and the Tectonic Implications. Tectonophysics 2013, 609, 690–705. [Google Scholar] [CrossRef]
- Bird, P. An Updated Digital Model of Plate Boundaries. Geochem. Geophys. Geosyst. 2003, 4, 2001GC000252. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hearn, T.M. Global Rayleigh Wave Attenuation and Group Velocity from International Seismological Centre Data. Geosciences 2024, 14, 50. https://doi.org/10.3390/geosciences14020050
Hearn TM. Global Rayleigh Wave Attenuation and Group Velocity from International Seismological Centre Data. Geosciences. 2024; 14(2):50. https://doi.org/10.3390/geosciences14020050
Chicago/Turabian StyleHearn, Thomas Martin. 2024. "Global Rayleigh Wave Attenuation and Group Velocity from International Seismological Centre Data" Geosciences 14, no. 2: 50. https://doi.org/10.3390/geosciences14020050
APA StyleHearn, T. M. (2024). Global Rayleigh Wave Attenuation and Group Velocity from International Seismological Centre Data. Geosciences, 14(2), 50. https://doi.org/10.3390/geosciences14020050






