Measurement of In-Situ Flow Rate in Borehole by Heat Pulse Flowmeter: Field-Case Study and Reflection
Abstract
1. Introduction
1.1. Project Engineering Background
1.2. Review of Multi-Scale Groundwater Theories
1.3. Review of Shortages of Laboratory Seepage Tests
1.4. Review of In-Situ Geological Investigation Methods
1.5. Review of In-Situ Flow Measuring Techniques
1.6. Review of Heat Pulse Flowmeter Development
1.7. Research Challenges Identification
1.8. Field Selection and Research Objective
2. Methodology and Field Study
2.1. HPFM Specification and Working Principle
- Initially, characterising and delimiting any individual geological units or formations (e.g., fractures and faults) along each borehole profile.
- Then, separating the flow zones based on the characterised geological formations.
- Further estimating the far-field hydraulic head of the aquifer having fractures and faults (H), hydraulic gradients (dH/dz) and transmissivity (T) for those flow zones.
- Subsequentially, identifying any potential flow conduits.
- Consequently, numerical modelling (e.g., forward and inverse modelling) and hydrogeological mapping.
2.2. HPFM In-Situ Installation
- (1)
- Lower the HPFM probe into each borehole by a cable connected to a winch (see Figure 2a).
- (2)
- Measure the water flow rate by pressing the trigger assembly button when the probe is centrally positioned at an investigating depth in a borehole.
- (3)
- The centre conductor of the probe will receive a pulse; then, this pulse will fire the heat grid wire, and the software will receive the signal through the surface monitoring equipment to start a flow measurement (see Figure 2b).
- (4)
- The heated water transports with the in-situ flow in each borehole from the heat gird wire to the upper or lower thermistor sensor.
- (5)
- An amplifier will detect the temperature difference between the upper and lower sensors.
- (6)
- The amplifier’s output will then be converted to a frequency, sent out through the data cable, and finally monitored by the surface equipment and plotted in software (see Figure 2b–d).
- (7)
- When surface equipment and software have received the information, the tool will charge the capacitors immediately.
- (8)
- After the capacitors are charged, they will produce the voltage for the heat gird wire to be prepared for the next round of flow rate measurement.
- (9)
- Finally, the flow rate measurement is completed after the period between raising a heat pulse and the flow-carried peak temperature variation accurately detected by the sensor located above or below the heat grid wire (see Figure 2c,d).
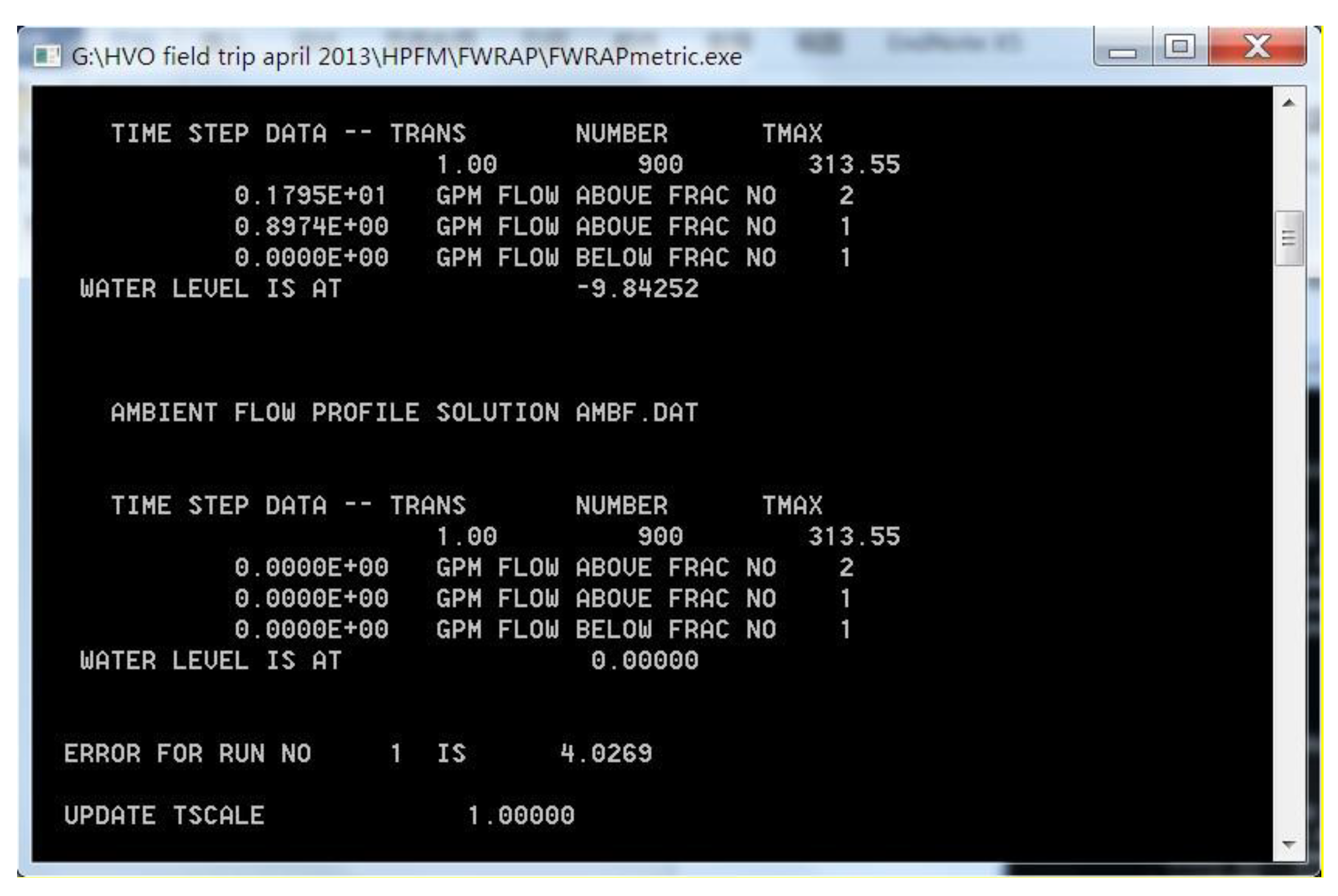
2.3. HPFM Data Analysis
2.3.1. Heat Pulse Signal Processing
2.3.2. Flow Rate Profile Analysis
- (1)
- The distribution of the flow rate scatters in standard form but should not be in a littery pattern (mussy, covered with littering and untidy data scatters) compared to successful instances of flow rate profiles in publications.
- (2)
- The scatter distribution is reasonable, meaning that most data points of the injection test should be on the left side of the datasets of the ambient tests, which is the case in this fieldwork. However, if any HPFM practitioner carried out pumping tests, most data points of the pumping test should be on the right side of the datasets of the ambient tests.
- (1)
- Calculate the following separately under ambient conditions and injection/pumping conditions:
- (a)
- Calculate the average water flow rates of each zone (delimited by fractures).
- (b)
- Calculate the in/outflows of each fracture, which is the difference between the average flow rate above the fracture and the average flow rate below the fracture.
- (c)
- Calculate the total in/outflows under each condition to verify the accuracy of the previous calculation. For example, the total in/outflows of the ambient test should be zero, and the total in/outflows of the injection/pumping test should be equal to the injection/pumping rate.
- (2)
- Calculate the difference in/outflows between the ambient and injection/pumping conditions; the result should be all negative numbers for the injection condition, and the result should be all positive numbers for the pumping condition.
- (3)
- Calculate the percentage of initially estimated transmissivity (T0) of each formation-contained aquifer (i.e., an initial guess of total T0 of aquifer having flow-producing geological formations), which results from the total in/outflows divided by this fracture’s different in/outflows; the total percentage of T0 should be 100%.
2.3.3. Groundwater Inversion Modelling
2.4. Field-Case Study
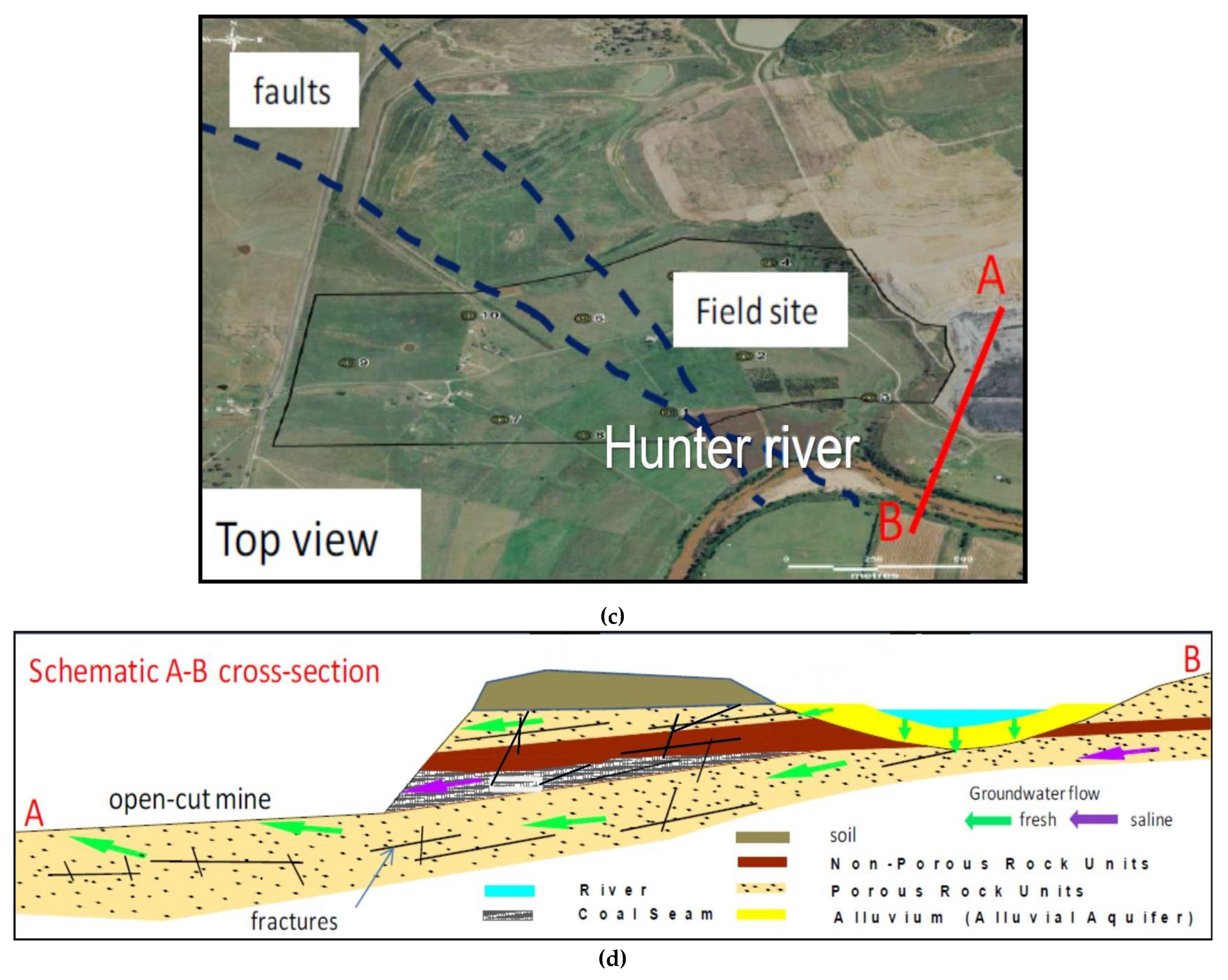
2.4.1. Site Brief
2.4.2. Challenges in In-Situ Setup of HPFM
2.4.3. In-Situ Calibration
3. Results and Discussion
3.1. In-Situ Borehole Selection
3.2. In-Situ Calibration for Bypass Ratio
3.3. Positive and Verified Outcomes and Fracture Analysis
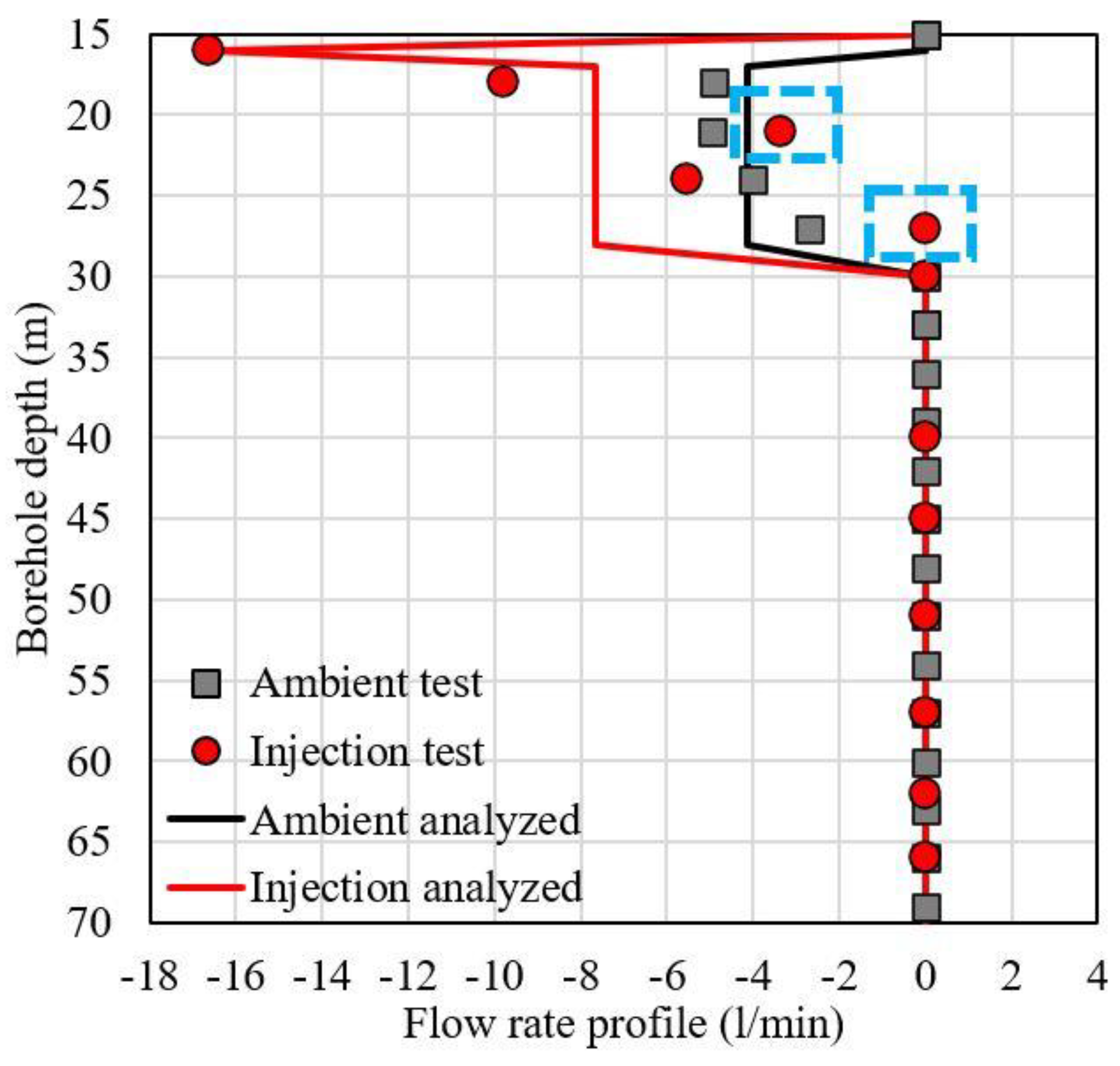
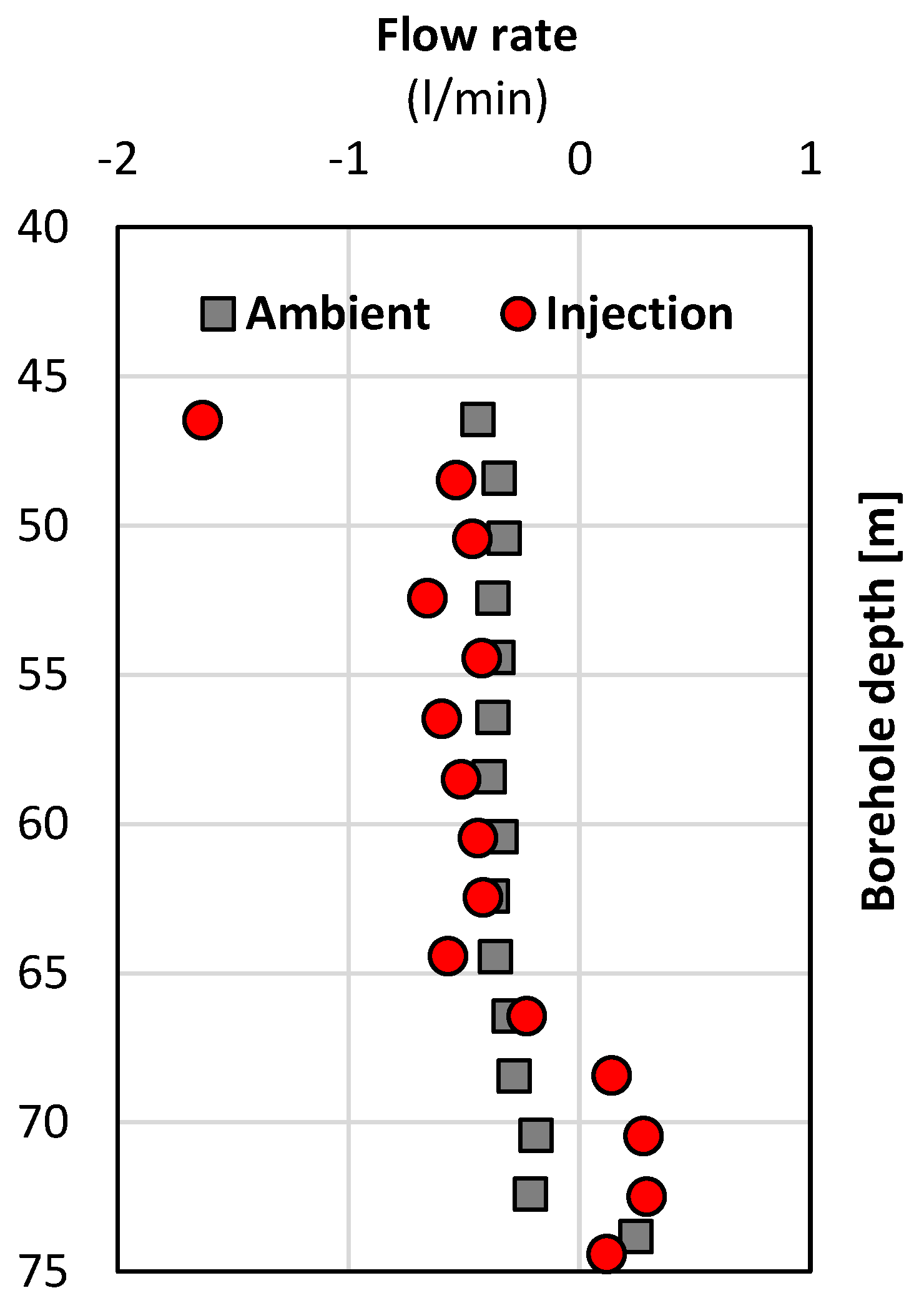
3.3.1. Borehole A435_R_045 (Trip 2)
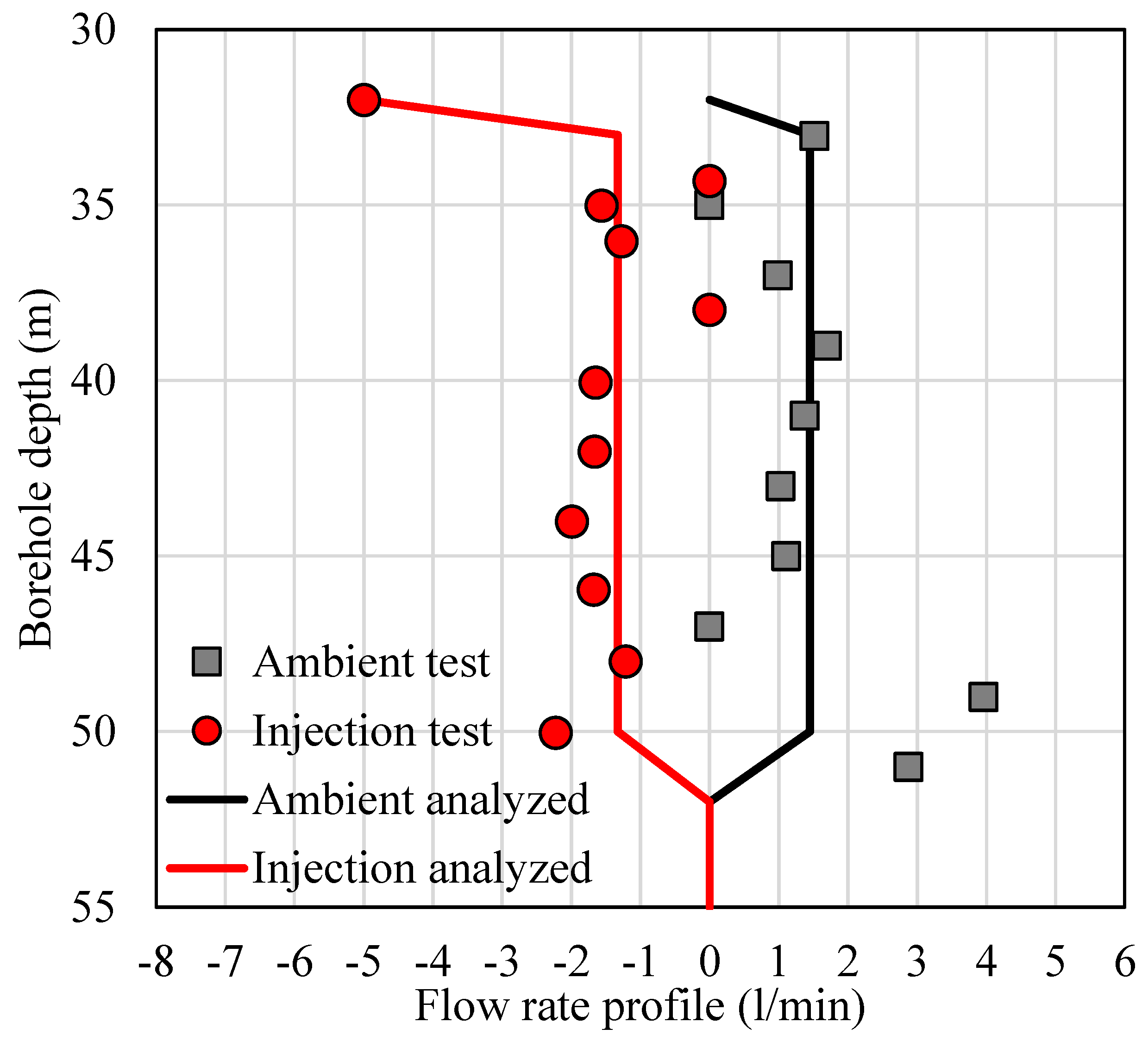
3.3.2. Borehole A435_R_056 (Trip 2)
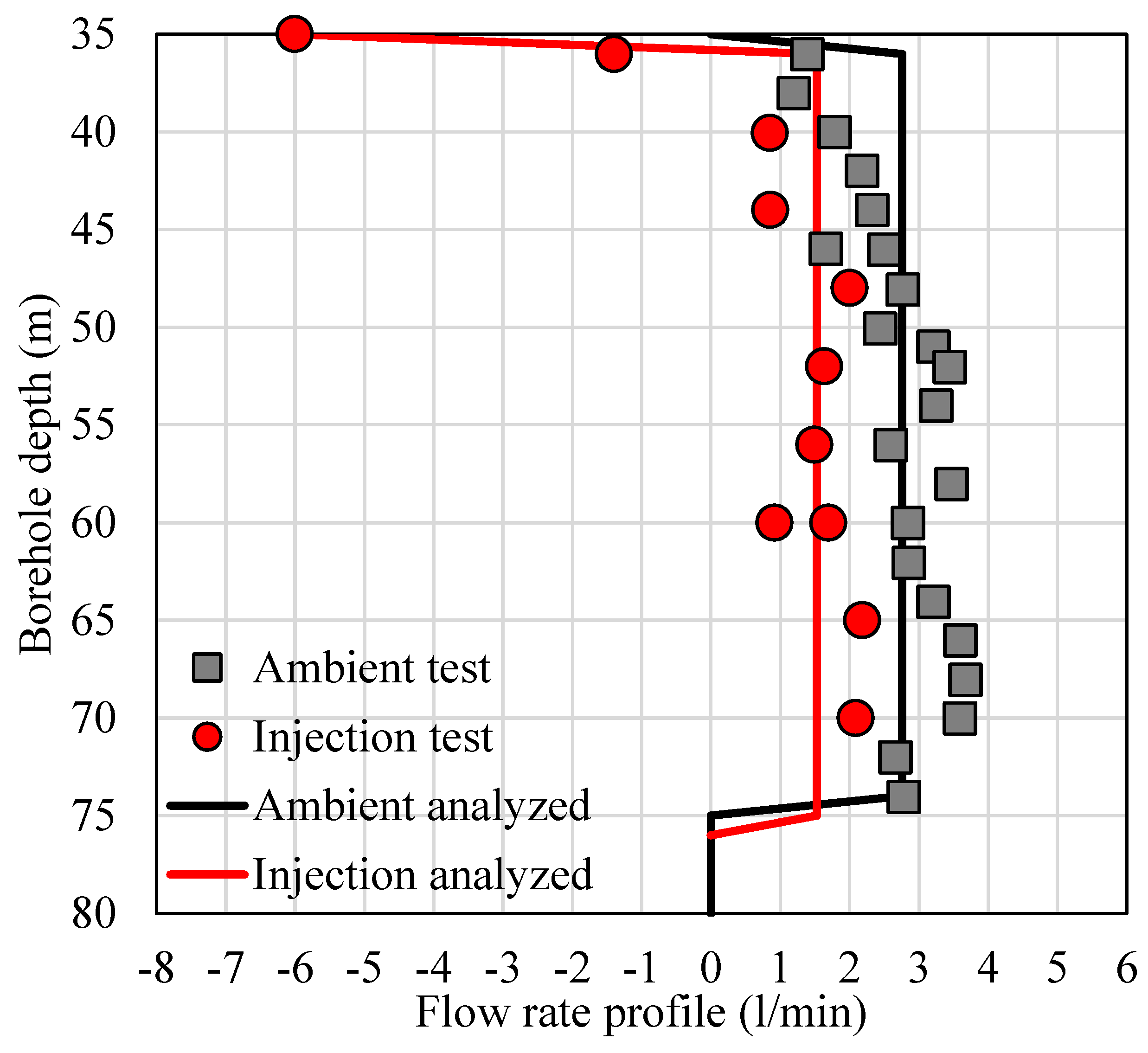
3.3.3. Borehole A435_R_059 (Trip 2)
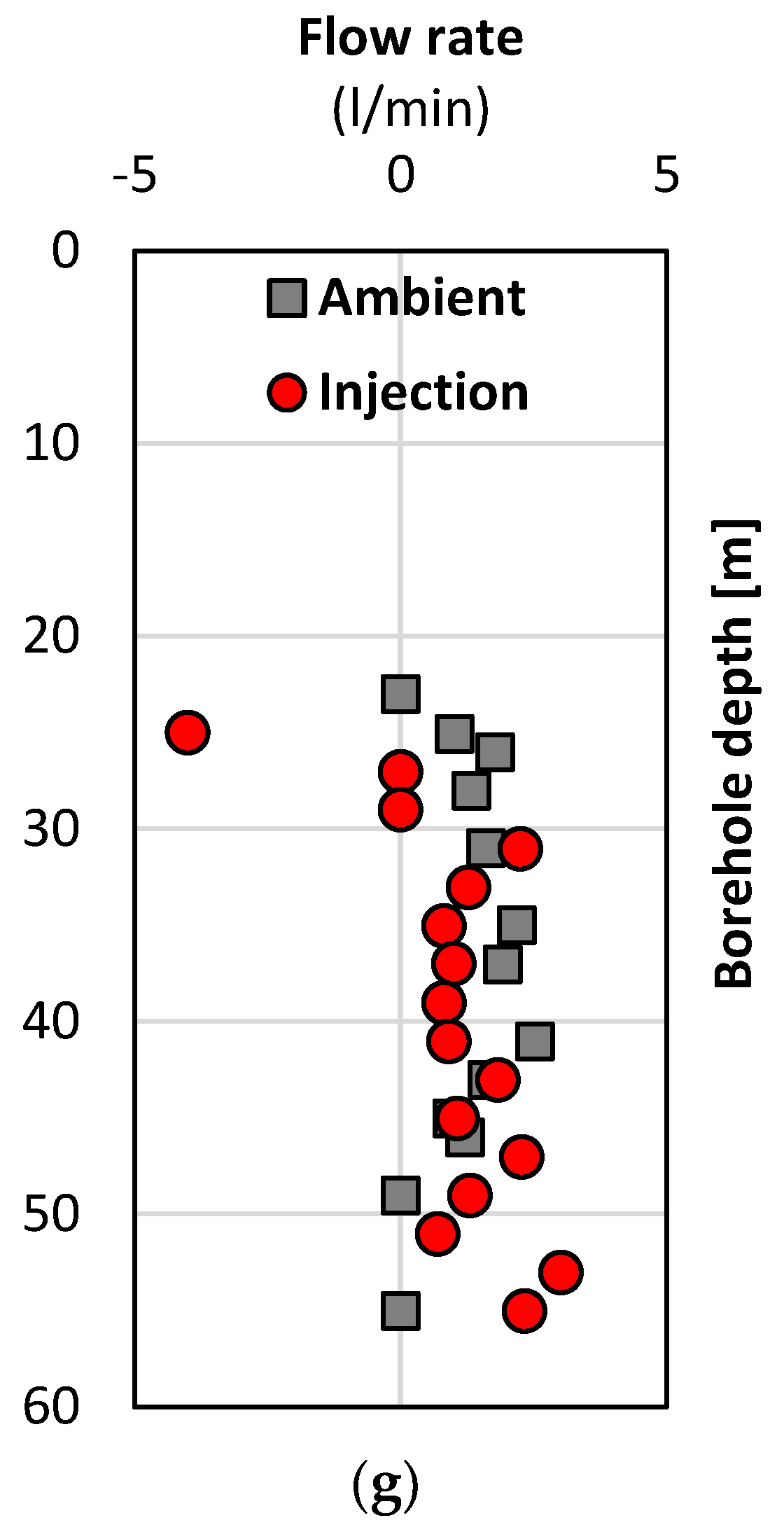
3.4. Negative Outcomes and Reflection
3.4.1. Comparison between Unanalysable and Analysable Datasets
3.4.2. Totally Unanalysable Datasets
3.4.3. Partially Unanalysable or Analysable Datasets
3.4.4. Fracture-Identifiable Datasets with Apparent in/Outflow Errors
4. Summary and Reflections
- (1)
- HPFM is a powerful flow measuring technique developed for detecting the lower flow rate range compared to the impeller flowmeter. Due to its high sensitivity to lower flow rates, it can be used to more accurately estimate the locations of geological formations (e.g., fracture and fault).
- (2)
- The HPFM measurement can be easily carried out in the field, but the installation of HPFM measurement is labour-intensive.
- (3)
- The fracture locations can be well-observed from the flow rate profile detected by HPFM, and the in/outflow around each identified water-producing zone can be analysed based on the principle of mass conservation.
- (4)
- With those analysed hydraulic parameters and variables inputted as initial guesses, the flow-producing zone’s transmissivity and far-field hydraulic head can be correctly back-calculated using groundwater inversion modelling.
- (5)
- When the flow diverter is not set up on the HPFM probe tip, the in-situ calibration tests must be carried out to determine the flow bypass ratio. Otherwise, HPFM will not provide the in-situ flow logging in a reasonable range.
- (6)
- Three positive outcomes of HPFM application and post-analysis have been demonstrated, and two of them could be verified by other GI logging profiles (e.g., density, calliper, etc.). Those positive and verified outcomes demonstrate the success of the HPFM technique integrated with groundwater inversion modelling.
- (7)
- Many failure cases and reasons have been reflected, including the unreasonable swap between ambient test and injection test datasets, the spurious flow-induced scattering datasets, regionally irrational flow directions, etc., which could be partially analysable or totally unanalysable.
- (8)
- Although fracture locations had been roughly found in the partially analysable datasets, the in/outflow analysis could not be processed due to the measuring error-induced violation of mass conservation.
- (9)
- The adverse outcomes from the field tests are mainly due to two issues: (a) no installation of the flow diverter and (b) the borehole casing with steel and PVC pipes was backfilled with gravel to prevent borehole collapse.
- (1)
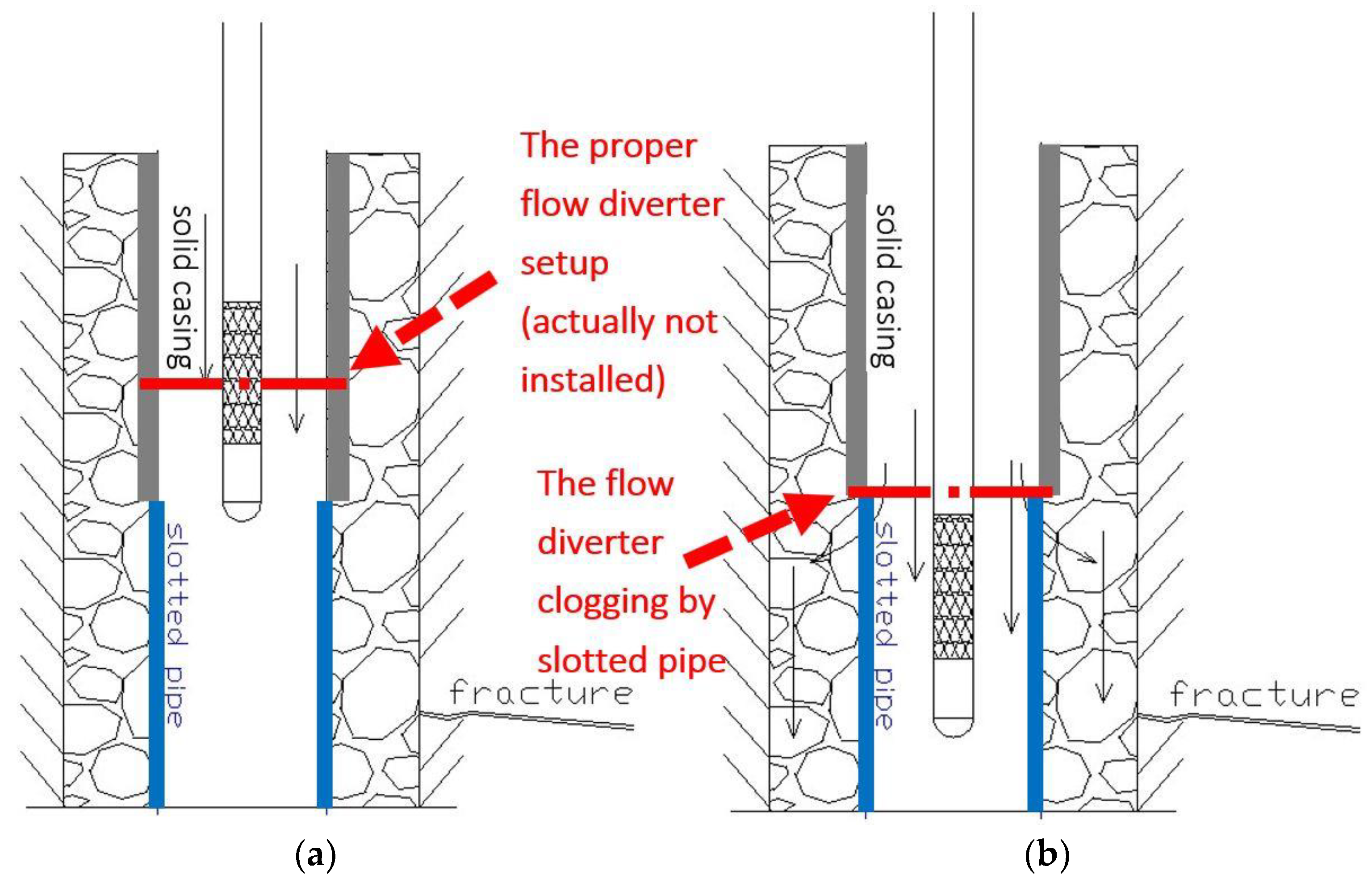
- Due to lacking experience in using HPFM, the predrilled borehole was solid-cased by steel and PVC pipes with backfilled gravel. Unfortunately, the diameter of the cased borehole was also smaller than that of the flow diverter (see Figure 6a,b), so the diverter could not be fitted into each borehole, and the in-situ calibration tests had to be done.
- (2)
- Again, the best testing condition should be with installing the HPFM diverter, as without it, the insufficient flow was diverted into the probe tip, where more water flow could bypass. Thus, the borehole diameter should be carefully prepared to fit the diverter diameter almost perfectly.
- (3)
- Otherwise, provided that the borehole diameter is much larger than the diverter diameter, not only does more water flow bypass the probe tip, but the borehole will also be more vulnerable to potential borehole collapsing.
- (4)
- The backfilled gravel tightly contacted with the borehole wall became a segregated section for generating vertical preferential flow paths that alleviate in/outflow around a fracture at its corresponding location but discharged more water downward to supply water circulating at the borehole bottom.
- (5)
- Based on the aforementioned reflections, no borehole casing seems to provide a perfect testing condition for HPFM; i.e., it is vital to mention the dilemma between the collapsing-induced mud-clogging of the HPFM probe tip (under no borehole casing condition) and the unexpected spurious flow and upward flow at the borehole bottom (under borehole casing condition).
- (6)
- Moreover, since many studies have reported the success of in-situ flow measurement under borehole casing conditions, there is no completely suitable recommendation regarding this dilemma. Still, other HPFM practitioners must find their best option based on the in-situ geological conditions.
5. Patents
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, L. Multi-Scale, Two-Phase Flow in Complex Coal Seam Systems; Australian Coal Association Research Program (ACARP): Brisbane, Australia, 2011; pp. 7–15. [Google Scholar]
- Hancock, P.J. Human impacts on the stream–groundwater exchange zone. Environ. Manag. 2002, 29, 763–781. [Google Scholar] [CrossRef]
- Ma, Y. Preferential Flow Paths in A Complex Coal Seam System. Ph.D. Thesis, University of Queensland, Brisbane, Australia, 2015. [Google Scholar]
- Ma, Y.; Kong, X.Z.; Zhang, C.; Scheuermann, A.; Bringemeier, D.; Li, L. Quantification of Natural CO2 Emission Through Faults and Fracture Zones in Coal Basins. Geophys. Res. Lett. 2021, 48, e2021GL092693. [Google Scholar] [CrossRef]
- Busse, J.; Scheuermann, A.; Bringemeier, D.; Hossack, A.; Li, L. In-Situ coal seam and overburden permeability characterization combining downhole flow meter and temperature logs. Contemp. Trends Geosci. 2016, 5, 1–17. [Google Scholar] [CrossRef]
- Busse, J.; Paillet, F.; Hossack, A.; Bringemeier, D.; Scheuermann, A.; Li, L. Field performance of the heat pulse flow meter: Experiences and recommendations. J. Appl. Geophys. 2016, 126, 158–171. [Google Scholar] [CrossRef]
- Bear, J. Dynamics of Fluids in Porous Media; American Elsevier Publishing Company, Inc.: New York, NY, USA, 1972. [Google Scholar]
- Lu, N.; Likos, W.J. Unsaturated Soil Mechanics; John Wiley & Sons: Hoboken, NJ, USA, 2004. [Google Scholar]
- Fredlund, D.G.; Rahardjo, H. Soil Mechanics for Unsaturated Soils; John Wiley & Sons: Hoboken, NJ, USA, 1993. [Google Scholar]
- Yan, G.; Li, Z.; Galindo Torres, S.A.; Scheuermann, A.; Li, L. Transient Two-Phase Flow in Porous Media: A Literature Review and Engineering Application in Geotechnics. Geotechnics 2022, 2, 32–90. [Google Scholar] [CrossRef]
- Freeze, R.A.; Cherry, J.A. Groundwater; Prentice-Hall Inc.: Eaglewood Cliffs, NJ, USA, 1979. [Google Scholar]
- Fetter, C. Applied Hydrogeology, 4th ed.; Prantice-Hall Inc.: Eaglewood Cliffs, NJ, USA, 2001; p. 621. [Google Scholar]
- Yan, G.; Ma, Y.; Scheuermann, A.; Li, L. The Hydraulic Properties of Aquabeads Considering Forchheimer Flow and Local Heterogeneity. Geotech. Test. J. 2022, 45, 891–900. [Google Scholar] [CrossRef]
- ASTM D2434-68; Standard Test Method for Permeability of Granular Soils (Constant Head). ASTM International: West Conshohocken, PA, USA, 2006.
- ASTM D7664-10; Standard Test Methods for Measurement of Hydraulic Conductivity of Unsaturated Soils. ASTM International: West Conshohocken, PA, USA, 2010.
- Yan, G.; Bore, T.; Schlaeger, S.; Scheuermann, A.; Li, L. Investigating scale effects in soil water retention curve via spatial time domain reflectometry. J. Hydrol. 2022, 612, 128238. [Google Scholar] [CrossRef]
- Yan, G.; Bore, T.; Bhuyan, H.; Schlaeger, S.; Scheuermann, A. The Technical Challenges for Applying Unsaturated Soil Sensors to Conduct Laboratory-Scale Seepage Experiments. Sensors 2022, 22, 3724. [Google Scholar] [CrossRef]
- Li, Z.; Galindo-Torres, S.; Yan, G.; Scheuermann, A.; Li, L. A lattice Boltzmann investigation of steady-state fluid distribution, capillary pressure and relative permeability of a porous medium: Effects of fluid and geometrical properties. Adv. Water Resour. 2018, 116, 153–166. [Google Scholar] [CrossRef]
- Li, Z.; Galindo-Torres, S.; Yan, G.; Scheuermann, A.; Li, L. Pore-scale simulations of simultaneous steady-state two-phase flow dynamics using a lattice Boltzmann model: Interfacial area, capillary pressure and relative permeability. Transp. Porous Media 2019, 129, 295–320. [Google Scholar] [CrossRef]
- Yan, G.; Li, Z.; Bore, T.; Torres, S.A.G.; Scheuermann, A.; Li, L. Discovery of Dynamic Two-Phase Flow in Porous Media Using Two-Dimensional Multiphase Lattice Boltzmann Simulation. Energies 2021, 14, 4044. [Google Scholar] [CrossRef]
- Yan, G.; Li, Z.; Bore, T.; Galindo Torres, S.A.; Scheuermann, A.; Li, L. A lattice Boltzmann exploration of two-phase displacement in 2D porous media under various pressure boundary conditions. J. Rock Mech. Geotech. Eng. 2022, 14, 1782–1798. [Google Scholar] [CrossRef]
- Darcy, H.; Bazin, H. Recherches Hydrauliques: Recherches Expérimentales sur l’écoulement de l’eau dans les Canaux Découverts. 1ère Partie; Dunod: Pairs, French, 1865; Volume 1. [Google Scholar]
- Yan, G.; Shi, H.; Ma, Y.; Scheuermann, A.; Li, L. Intrinsic permeabilities of transparent soil under various aqueous environmental conditions. Géotechnique Lett. 2022, 12, 225–231. [Google Scholar] [CrossRef]
- Singhal, B. Nature of hard rock aquifers: Hydrogeological uncertainties and ambiguities. In Groundwater Dynamics in Hard Rock Aquifers; Springer: Berlin/Heidelberg, Germany, 2008; pp. 20–39. [Google Scholar]
- Bonnet, E.; Bour, O.; Odling, N.E.; Davy, P.; Main, I.; Cowie, P.; Berkowitz, B. Scaling of fracture systems in geological media. Rev. Geophys. 2001, 39, 347–383. [Google Scholar] [CrossRef]
- Clarkson, C.R.; Bustin, R. Coalbed methane: Current field-based evaluation methods. SPE Reserv. Eval. Eng. 2011, 14, 60–75. [Google Scholar] [CrossRef]
- Dron, R. Notes on cleat in the Scottish coalfield. Trans. Inst. Min. Metall. 1925, 70, 115–117. [Google Scholar]
- Tsang, C.F.; Neretnieks, I. Flow channeling in heterogeneous fractured rocks. Rev. Geophys. 1998, 36, 275–298. [Google Scholar] [CrossRef]
- Massarotto, P.; Rudolph, V.; Golding, S.; Wang, F.; Iyer, R. Scaleup of laboratory measured coal permeability. In Proceedings of the 2008 Asia Pacific Coalbed Methane Symposium, Brisbane, Australia, 22–24 September 2008. [Google Scholar]
- DeAngelis, D.L.; Yeh, G.; Huff, D. Integrated Compartmental Model for Describing The Transport of Solute in a Fractured Porous Medium; Oak Ridge National Lab.: Oak Ridge, TN, USA, 1984.
- Legrand, H. Evaluation techniques of fractured-rock hydrology. In Developments in Water Science; Elsevier: Amsterdam, The Netherlands, 1979; Volume 12, pp. 333–346. [Google Scholar]
- Neuville, A.; Toussaint, R.; Schmittbuhl, J. Hydrothermal coupling in a self-affine rough fracture. Phys. Rev. E 2010, 82, 036317. [Google Scholar] [CrossRef]
- Kirsch, R. Groundwater Geophysics: A Tool for Hydrogeology; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Price, D.G. Engineering Geology: Principles and Practice; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Elci, A.; Molz, F.J., III; Waldrop, W.R. Implications of observed and simulated ambient flow in monitoring wells. Groundwater 2001, 39, 853–862. [Google Scholar] [CrossRef]
- Mayo, A.L. Ambient well-bore mixing, aquifer cross-contamination, pumping stress, and water quality from long-screened wells: What is sampled and what is not? Hydrogeol. J. 2010, 18, 823. [Google Scholar] [CrossRef]
- Izbicki, J.A.; Teague, N.F.; Hatzinger, P.B.; Böhlke, J.K.; Sturchio, N.C. Groundwater movement, recharge, and perchlorate occurrence in a faulted alluvial aquifer in California (USA). Hydrogeol. J. 2015, 23, 467. [Google Scholar] [CrossRef]
- Poulsen, D.L.; Cook, P.G.; Simmons, C.T.; McCallum, J.L.; Dogramaci, S. Effects of intraborehole flow on purging and sampling long-screened or open wells. Groundwater 2019, 57, 269–278. [Google Scholar] [CrossRef]
- Poulsen, D.L.; Cook, P.G.; Simmons, C.T.; Solomon, D.K.; Dogramaci, S. Depth-Resolved Groundwater Chemistry by Longitudinal Sampling of Ambient and Pumped Flows within Long-Screened and Open Borehole Wells. Water Resour. Res. 2019, 55, 9417–9435. [Google Scholar] [CrossRef]
- Huang, Y.; Li, Y.; Knappett, P.S.; Montiel, D.; Wang, J.; Aviles, M.; Hernandez, H.; Mendoza-Sanchez, I.; Loza-Aguirre, I. Water quality assessment bias associated with long-screened wells screened across aquifers with high nitrate and arsenic concentrations. Int. J. Environ. Res. Public Health 2022, 19, 9907. [Google Scholar] [CrossRef]
- Molz, F.; Boman, G.; Young, S.; Waldrop, W. Borehole flowmeters: Field application and data analysis. J. Hydrol. 1994, 163, 347–371. [Google Scholar] [CrossRef]
- Pitrak, M.; Mares, S.; Kobr, M. A simple borehole dilution technique in measuring horizontal ground water flow. Groundwater 2007, 45, 89–92. [Google Scholar] [CrossRef]
- Monier-Williams, M.; Davis, R.; Paillet, F.; Turpening, R.; Sol, S.; Schneider, G. Review of Borehole Based Geophysical Site Evaluation Tools and Techniques; Nuclear Waste Management Organization: Toronto, ON, Canada, 2009. [Google Scholar]
- Hess, A.E. Identifying hydraulically conductive fractures with a slow-velocity borehole flowmeter. Can. Geotech. J. 1986, 23, 69–78. [Google Scholar] [CrossRef]
- Paillet, F.L.; Hess, A.; Cheng, C.; Hardin, E. Characterization of fracture permeability with high-resolution vertical flow measurements during borehole pumping. Groundwater 1987, 25, 28–40. [Google Scholar] [CrossRef]
- Pehme, P.E.; Greenhouse, J.P.; Parker, B.L. The active line source temperature logging technique and its application in fractured rock hydrogeology. J. Environ. Eng. Geophys. 2007, 12, 307–322. [Google Scholar] [CrossRef]
- Paillet, F.L. Flow modeling and permeability estimation using borehole flow logs in heterogeneous fractured formations. Water Resour. Res. 1998, 34, 997–1010. [Google Scholar] [CrossRef]
- Paillet, F.; Hite, L.; Carlson, M. Integrating surface and borehole geophysics in ground water studies—An example using electromagnetic soundings in south Florida. J. Environ. Eng. Geophys. 1999, 4, 45–55. [Google Scholar] [CrossRef]
- Paillet, F.L. A field technique for estimating aquifer parameters using flow log data. Groundwater 2000, 38, 510–521. [Google Scholar] [CrossRef]
- Paillet, F.L. Borehole flowmeter applications in irregular and large-diameter boreholes. J. Appl. Geophys. 2004, 55, 39–59. [Google Scholar] [CrossRef]
- Mount Sopris Instruments, Co. Mannual of HFP-2293—Heat Pulse Flowmeter. Mount Sopris Instruments Co.: Denver, CO, USA, 2002. [Google Scholar]
- Molz, F.J.; Morin, R.H.; Hess, A.E.; Melville, J.G.; Güven, O. The impeller meter for measuring aquifer permeability variations: Evaluation and comparison with other tests. Water Resour. Res. 1989, 25, 1677–1683. [Google Scholar] [CrossRef]
- Paillet, F. FWRAP; U.S. Geological Survery: Denver, CO, USA, 2012.
- Cooper, H.H., Jr.; Bredehoeft, J.D.; Papadopulos, I.S. Response of a finite-diameter well to an instantaneous charge of water. Water Resour. Res. 1967, 3, 263–269. [Google Scholar] [CrossRef]
- Davis, S.N.; De Wiest, R.J. Hydrogeology; Wiley: New York, NY, USA, 1966; Volume 463. [Google Scholar]
- Coal & Allied Operations Pty Ltd. Hunter Valley Operations South Coal Project: Environmental Assessment Report Volume I; Coal & Allied Operations Pty Ltd.: Sydney, Australia, 2008; pp. 1–1224. [Google Scholar]
- Age Australasian Groundwater & Environmental Consultants Pty Ltd. 2011 Hunter Valley Operation (HVO) Carrington Groundwater Impacts Report; Australian Coal Association Research Program (ACARP): Brisbane, Australia, 2012. [Google Scholar]
- Williams, J.; Paillet, F.L. Using flowmeter pulse tests to define hydraulic connections in the subsurface: A fractured shale example. J. Hydrol. 2002, 265, 100–117. [Google Scholar] [CrossRef]









| Specification | Values |
|---|---|
| Power requirements | D.C. voltage at probe tip. Min. 30 VDC and Max. 68 VDC @ 200 mA |
| Tool output | Pulse type, positive going, 1.25 uS wide from 4.5 kHz to 37,000 kHz |
| Measuring range | 0.113 l/min to 3.785 l/min |
| Resolution | 5% (0.1836 l/min) |
| Accuracy | 5% (mid-range) to 15% (extremes) (0.1836–0.5508 l/min) |
| Dimensions | Length = 122 cm, Diameter = 4.1 cm, Weight = 15.5 kg |
| Ambient Test | Injection Test | ||||||
|---|---|---|---|---|---|---|---|
| Depth [m] | HPFM [l/min] | Adjusted [l/min] | Zone Average [l/min] | Depth [m] | HPFM [l/min] | Adjusted [l/min] | Zone Average [l/min] |
| 29 | NF * | 0.00 | 0.00 | 30 | 2.46 | 2.95 | 2.91 |
| 31 | 2.38 | 2.88 | |||||
| 34 | Fracture 2 | ||||||
| 40 | −1.14 | −1.36 | −1.25 | 35 | 1.14 | 1.36 | 1.44 |
| 40 | 1.21 | 1.44 | |||||
| 50 | −0.98 | −1.17 | 50 | 1.14 | 1.36 | ||
| 55 | 1.25 | 1.51 | |||||
| 60 | −0.98 | −1.17 | 60 | 1.21 | 1.44 | ||
| 70 | 1.32 | 1.59 | |||||
| 73 | Fracture 1 | ||||||
| 74 | NF * | 0.00 | 0.00 | 74 | NF * | 0.00 | 0.00 |
| Zone | Ambient | Injection | Difference between the Ambient and Injection Tests [l/min] | Percentage of T0 * (%) | ||||
|---|---|---|---|---|---|---|---|---|
| Depth [m] | Above [l/min] | Below [l/min] | Flow [l/min] | Above [l/min] | Below [l/min] | Flow [l/min] | ||
| 34 | 0.00 | −1.25 | 1.25 | 2.92 | 1.44 | 1.48 | 0.23 | 8 |
| 73 | −1.25 | 0.00 | −1.25 | 1.44 | 0.00 | 1.44 | 2.69 | 92 |
| Total | 0.00 | 2.92 | 2.92 | 100 | ||||
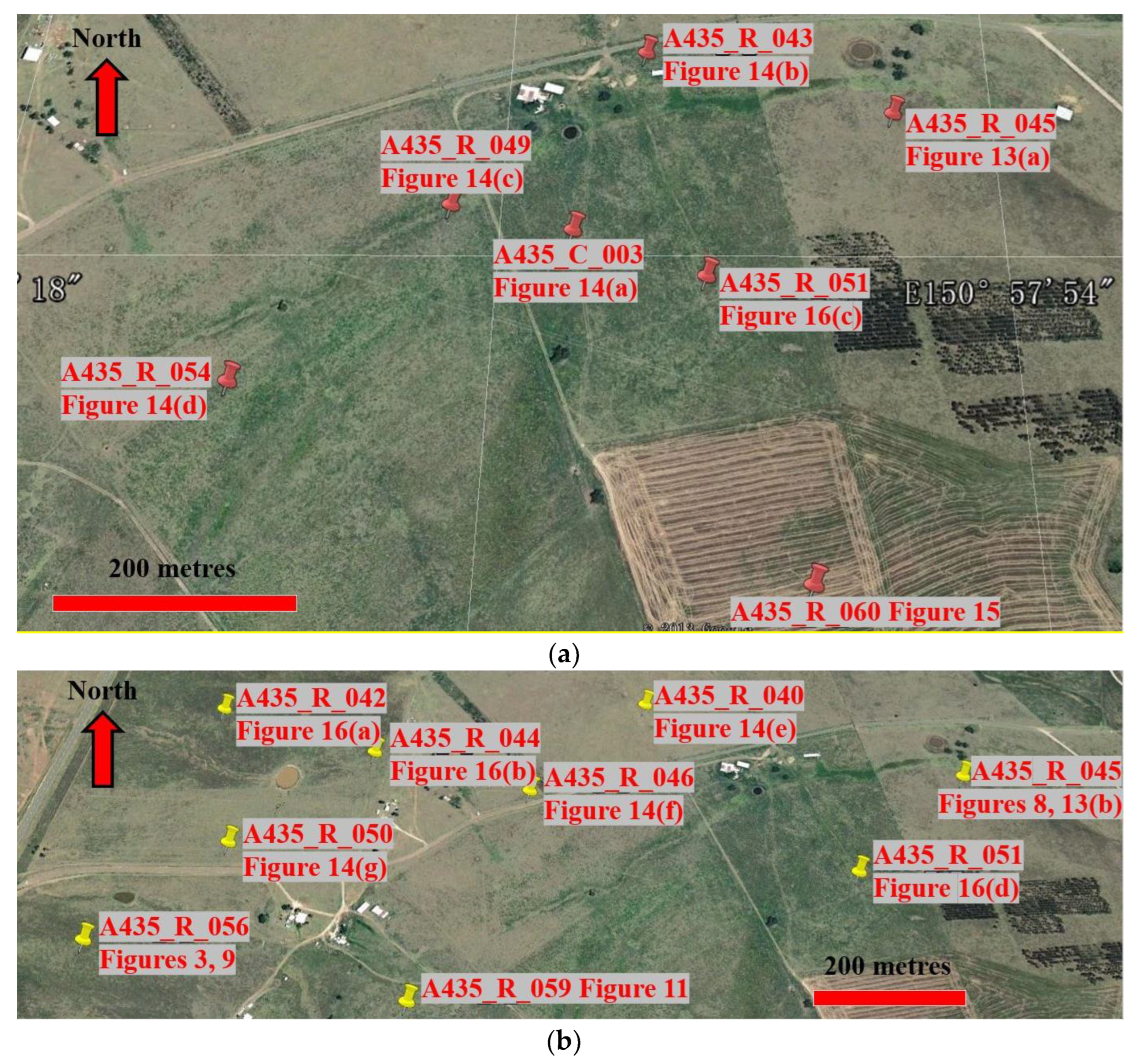
| Borehole No. | Location | |
|---|---|---|
| Latitude | Longitude | |
| A435_C_003 (Figure 14a) | 32°29′36.54″ S | 150°57′38.45″ E |
| A435_R_040 (Figure 14e) | 32°29′27.89″ S | 150°57′31.71″ E |
| A435_R_042 (Figure 16a) | 32°29′28.78″ S | 150°57′06.45″ E |
| A435_R_043 (Figure 14b) | 32°29′30.15″ S | 150°57′40.93″ E |
| A435_R_044 (Figure 16b) | 32°29′31.24″ S | 150°57′15.66″ E |
| A435_R_045 (Figures 8 and 13) | 32°29′32.41″ S | 150°57′50.15″ E |
| A435_R_046 (Figure 14f) | 32°29′33.43″ S | 150°57′24.84″ E |
| A435_R_049 (Figure 14c) | 32°29′35.69″ S | 150°57′34.03″ E |
| A435_R_050 (Figure 14g) | 32°29′36.83″ S | 150°57′08.22″ E |
| A435_R_051 (Figure 16c,d) | 32°29′38.05″ S | 150°57′43.21″ E |
| A435_R_054 (Figure 14d) | 32°29′41.36″ S | 150°57′27.08″ E |
| A435_R_056 (Figure 3 and Figure 9) | 32°29′42.31″ S | 150°57′01.85″ E |
| A435_R_059 (Figure 11) | 32°29′44.89″ S | 150°57′18.84″ E |
| A435_R_060 (Figure 15) | 32°29′47.03″ S | 150°57′46.57″ E |
| Boreholes No. | Depth (m) | Casing Part (Solid or Slotted) | Actual Flow Rate (l/min) | Measured Flow Rate (l/min) | Bypass Ratio (Actual/Detected) |
|---|---|---|---|---|---|
| A435_R_040 | 15 | Solid | 7.00 | 1.80 | 3.90 |
| A435_R_042 | 20 | Slotted | 3.00 | 0.76 | 3.95 |
| A435_R_042 | 20 | Slotted | 3.50 | 0.96 | 3.65 |
| A435_R_042 | 20 | Slotted | 4.00 | 1.09 | 3.67 |
| A435_R_044 | 18 | Solid | 3.00 | 0.89 | 3.37 |
| A435_R_044 | 18 | Solid | 4.00 | 1.26 | 3.17 |
| A435_R_044 | 18 | Solid | 5.00 | 1.43 | 3.50 |
| A435_R_044 | 20 | Slotted | 3.00 | 0.14 * | 21.43 * |
| A435_R_044 | 20 | Slotted | 4.00 | 0.24 * | 16.67 * |
| A435_R_044 | 20 | Slotted | 5.00 | 0.32 * | 15.63 * |
| A435_R_045 | 15 | Solid | 16.89 | 3.67 | 4.60 |
| A435_R_046 | 17 | Solid | 3.00 | 0.91 | 3.30 |
| A435_R_046 | 17 | Solid | 4.00 | 1.16 | 3.45 |
| A435_R_046 | 17 | Solid | 5.00 | 1.44 | 3.47 |
| A435_R_046 | 19 | Slotted | 3.00 | 0.17* | 17.65 * |
| A435_R_046 | 19 | Slotted | 4.00 | 0.16 * | 25.00 * |
| A435_R_046 | 19 | Slotted | 5.00 | 0.16 * | 31.25 * |
| A435_R_050 | 17.5 | Solid | 1.50 | 0.42 | 3.57 |
| A435_R_050 | 17.5 | Solid | 2.00 | 0.51 | 3.92 |
| A435_R_050 | 17.5 | Solid | 2.50 | 0.73 | 3.42 |
| A435_R_050 | 17.5 | Solid | 3.00 | 0.89 | 3.37 |
| A435_R_050 | 17.5 | Solid | 4.00 | 1.23 | 3.25 |
| A435_R_050 | 27 | Slotted | 1.50 | 0.05 * | 30.00 * |
| A435_R_050 | 27 | Slotted | 2.50 | 0.03 * | 83.33 * |
| A435_R_050 | 27 | Slotted | 4.00 | 0.23 * | 17.39 * |
| Mean | – | – | – | – | 3.60 |
| SD | – | – | – | – | ±0.35 |
| Zone | Ambient | Injection | Difference between an Ambient and an Injection [l/min] | Percentage of T0 * (%) | ||||
|---|---|---|---|---|---|---|---|---|
| Depth [m] | Above [l/min] | Below [l/min] | Flow [l/min] | Above [l/min] | Below [l/min] | Flow [l/min] | ||
| 17 | −4.14 | 0.00 | −4.14 | −7.65 | 0.00 | −7.65 | −3.50 | 87.71 |
| 28 | −0.00 | −4.14 | 4.14 | −4.00 | −7.65 | 3.65 | −0.49 | 12.29 |
| Total | 0.00 | −4.00 | −4.00 | 100.00 | ||||
| Detected Flow-Producing Zone in Depth (m) | Transmissivity (m2/day) | Water Level below Ground Surface in Depth (m) |
|---|---|---|
| 17 | 1.61 | 10.14 |
| 28 | 0.27 | 39.71 |
| Zone | Ambient | Injection | Difference between an Ambient and an Injection [l/min] | Percentage of T0 * (%) | ||||
|---|---|---|---|---|---|---|---|---|
| Depth [m] | Above [l/min] | Below [l/min] | Flow [l/min] | Above [l/min] | Below [l/min] | Flow [l/min] | ||
| 36 | 0.00 | 2.76 | −2.76 | −6.20 | 1.53 | −7.73 | −4.96 | 80.04 |
| 75 | 2.76 | 0.00 ** | 2.76 | 1.53 | 0.00 | 1.53 | −1.24 | 19.96 |
| Total | 0.00 | −6.20 | −6.20 | 100.00 | ||||
| Detected Flow-Producing Zone in Depth (m) | Transmissivity (m2/day) | Water Level below Ground Surface in Depth (m) |
|---|---|---|
| 36 | 7.78 | 36.09 |
| 75 | 0.07 | 30.79 |
| Zone | Ambient | Injection | Difference between an Ambient and an Injection [l/min] | Percentage of T0 * (%) | ||||
|---|---|---|---|---|---|---|---|---|
| Depth [m] | Above [l/min] | Below [l/min] | Flow [l/min] | Above [l/min] | Below [l/min] | Flow [l/min] | ||
| 33 | 0.00 | 1.45 | −1.45 | −5.00 | −1.33 | −3.67 | −2.23 | 44.52 |
| 52 | 1.45 | 0.00 ** | 1.45 | −1.33 | 0.00 | −1.33 | −2.77 | 55.48 |
| Total | 0.00 | −5.00 | −5.00 | 100.00 | ||||
| Detected Flow-Producing Zone in Depth (m) | Transmissivity (m2/day) | Water Level below Ground Surface in Depth (m) |
|---|---|---|
| 33 | 1.05 | 34.17 |
| 52 | 1.28 | 30.07 |
| Zone | Ambient | Injection | Difference between an Ambient and an Injection [l/min] | Percentage of T0 * (%) | ||||
|---|---|---|---|---|---|---|---|---|
| Depth [m] | Above [l/min] | Below [l/min] | Flow [l/min] | Above [l/min] | Below [l/min] | Flow [l/min] | ||
| Borehole A435_R_042 | ||||||||
| 21 | 0.00 | 0.21 | −0.21 | −4.08 | −0.25 | −3.83 | −3.62 | 88.79 |
| 35 | 0.21 | 2.89 | −2.68 | −0.25 | 0.30 | −0.55 | 2.13 | −52.05 |
| 60 | 2.89 | 0.00 | 2.89 | 0.30 | 0.00 | 0.30 | −2.58 | 63.27 |
| Total | 0.00 | −4.08 | −4.08 | 100.00 | ||||
| Borehole A435_R_044 | ||||||||
| 18 | 0.00 | 6.53 | −6.53 | −4.00 | −2.67 | −1.33 | 5.20 | −130.02 |
| 25 | 6.53 | 3.74 | 2.78 | −2.67 | −4.05 | 1.37 | −1.41 | 35.25 |
| 51 | 3.74 | 3.65 | 0.10 | −4.05 | 1.82 | −5.86 | −5.96 | 148.92 |
| 60 | 3.65 | 0.00 | 3.65 | 1.82 | 0.00 | 1.82 | −1.83 | 45.85 |
| Total | 0.00 | −4.00 | −4.00 | 100.00 | ||||
| Borehole A435_R_051 (Trip 1) | ||||||||
| 34 | 1.64 | −0.34 | −1.98 | 0.12 | −0.58 | 0.70 | 2.68 | 120.00 |
| 51 | −0.34 | −0.68 | 0.34 | −0.58 | −1.07 | 0.49 | 0.15 | 7.00 |
| 54 | −0.68 | −0.40 | −0.28 | −1.07 | −0.32 | −0.75 | −0.47 | −21.00 |
| 58 | −0.40 | −0.24 | −0.16 | −0.32 | −0.03 | −0.29 | −0.13 | −6.00 |
| Total | −2.09 | −0.26 | 2.24 | 100.00 | ||||
| Borehole A435_R_051 (Trip 2) | ||||||||
| 36 | 0.00 | −0.73 | 0.73 | −0.18 | −1.08 | 0.90 | 0.17 | −94.12 |
| 58 | −0.73 | −0.08 | −0.65 | −1.08 | −0.13 | −0.96 | −0.30 | 166.39 |
| 67 | −0.08 | 0.00 | −0.08 | −0.13 | 0.00 | −0.13 | −0.05 | 27.72 |
| Total | 0.00 | −0.18 | −0.18 | 100.00 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, B.; Yan, G.; Ma, Y.; Scheuermann, A. Measurement of In-Situ Flow Rate in Borehole by Heat Pulse Flowmeter: Field-Case Study and Reflection. Geosciences 2023, 13, 146. https://doi.org/10.3390/geosciences13050146
Liu B, Yan G, Ma Y, Scheuermann A. Measurement of In-Situ Flow Rate in Borehole by Heat Pulse Flowmeter: Field-Case Study and Reflection. Geosciences. 2023; 13(5):146. https://doi.org/10.3390/geosciences13050146
Chicago/Turabian StyleLiu, Bing, Guanxi Yan, Ye Ma, and Alexander Scheuermann. 2023. "Measurement of In-Situ Flow Rate in Borehole by Heat Pulse Flowmeter: Field-Case Study and Reflection" Geosciences 13, no. 5: 146. https://doi.org/10.3390/geosciences13050146
APA StyleLiu, B., Yan, G., Ma, Y., & Scheuermann, A. (2023). Measurement of In-Situ Flow Rate in Borehole by Heat Pulse Flowmeter: Field-Case Study and Reflection. Geosciences, 13(5), 146. https://doi.org/10.3390/geosciences13050146








