Modeling the Trajectories of Ballistics in the Summit Area of Mt. Etna (Italy) during the 2020–2022 Sequence of Lava Fountains
Abstract
:1. Introduction
2. Materials and Methods
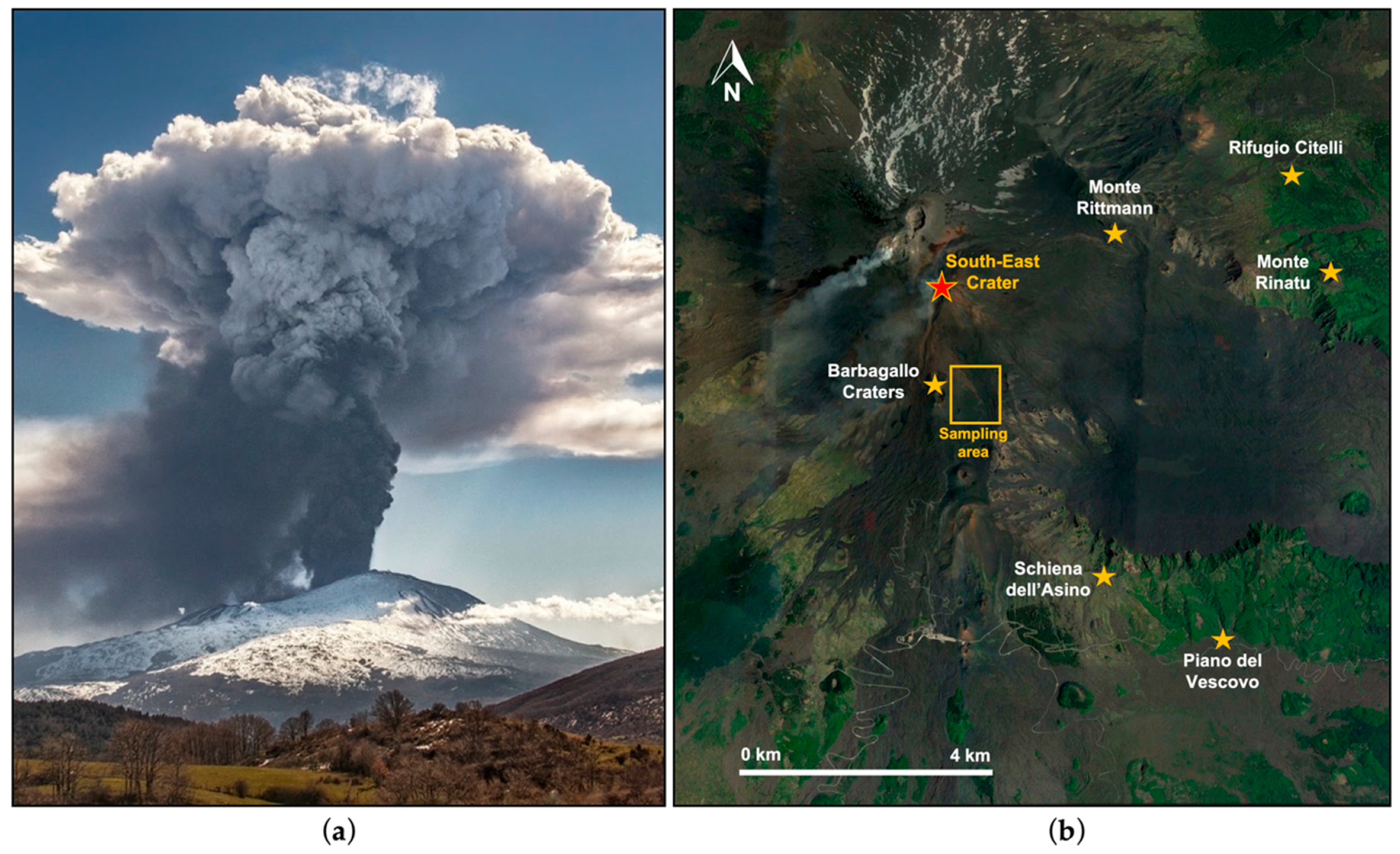
2.1. Field Data Collection
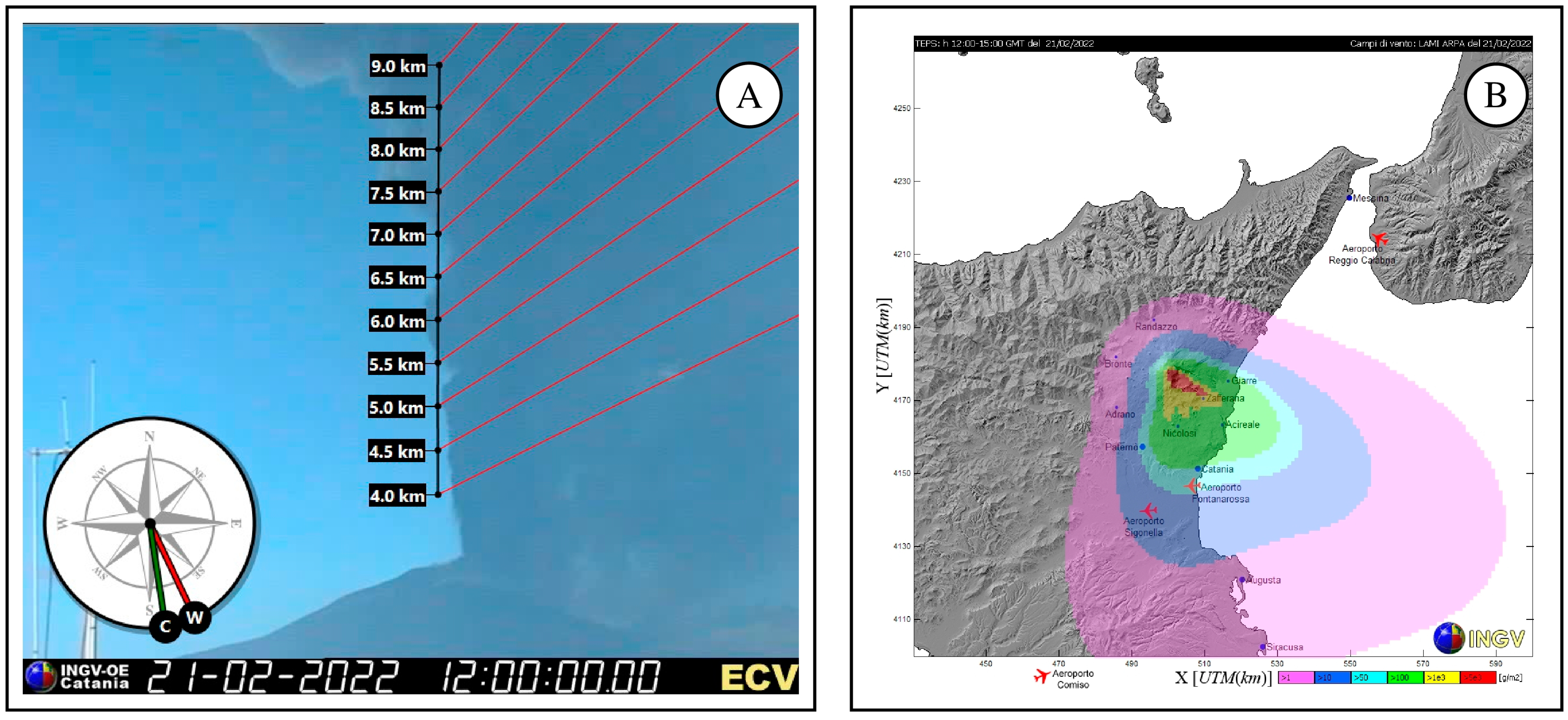
2.2. Analysis of Images from the INGV Thermal Camera
2.3. The Eject! Software
3. Results
3.1. Sampling Results
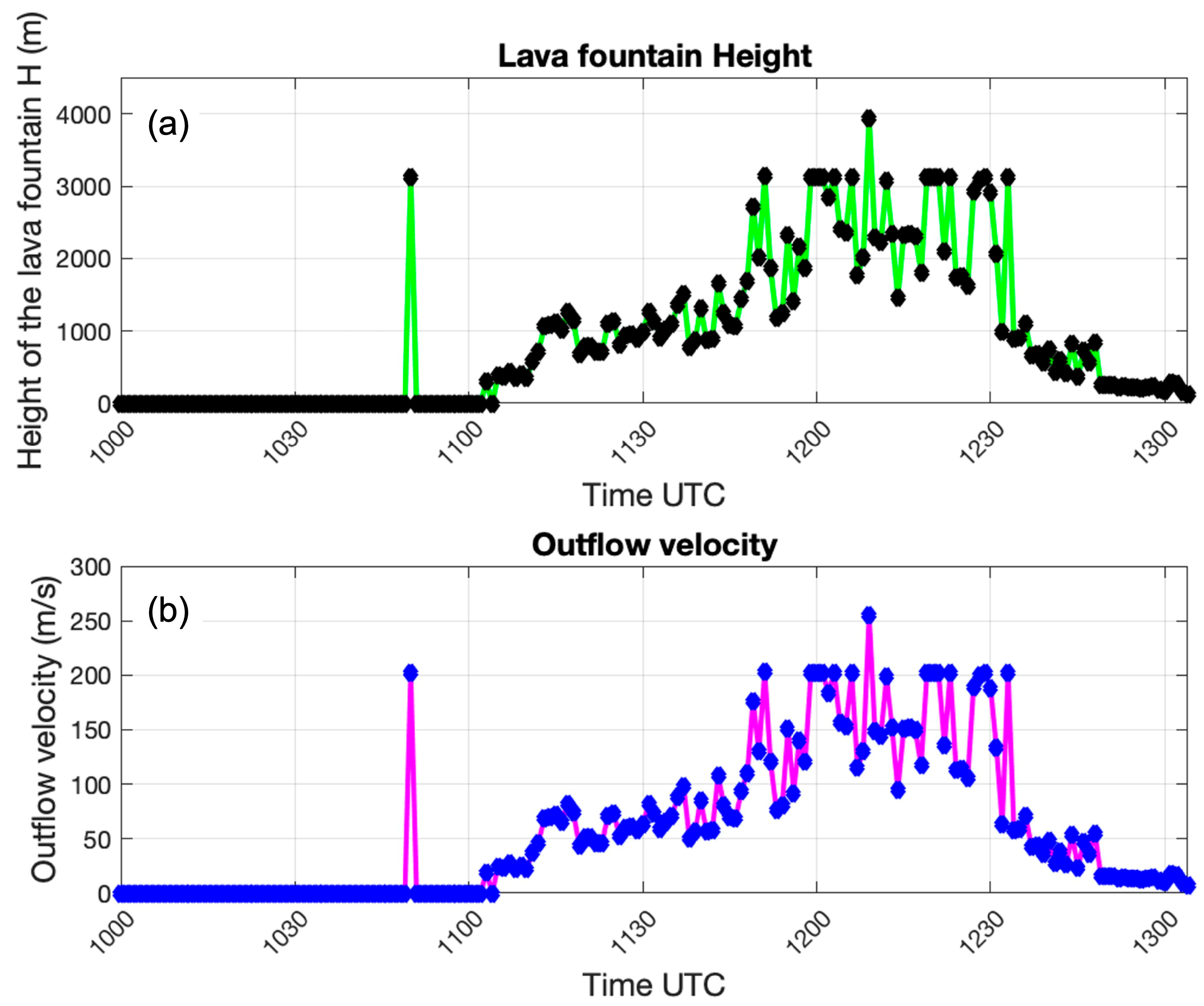
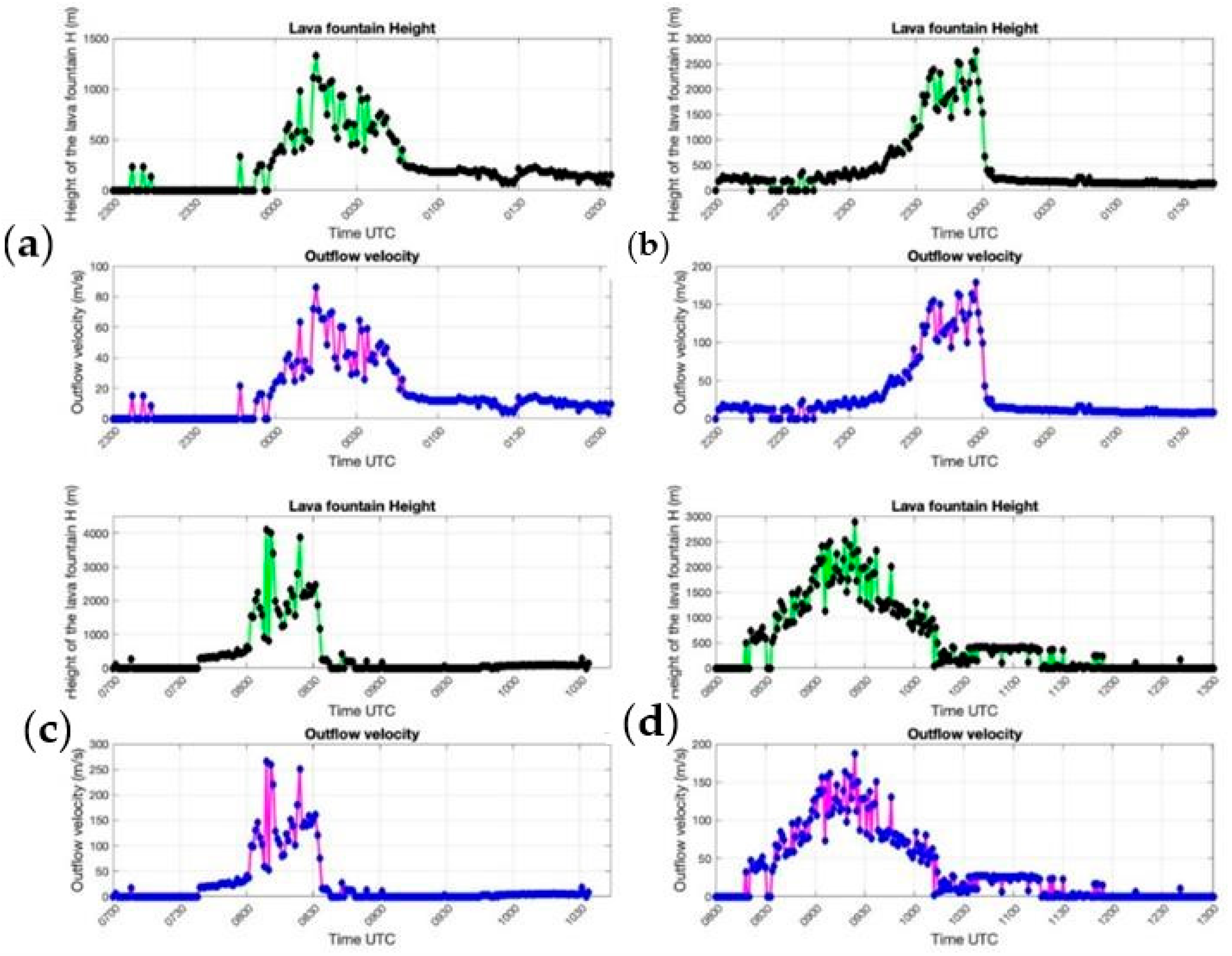
3.2. Analysis of the Lava Fountain Height Variation
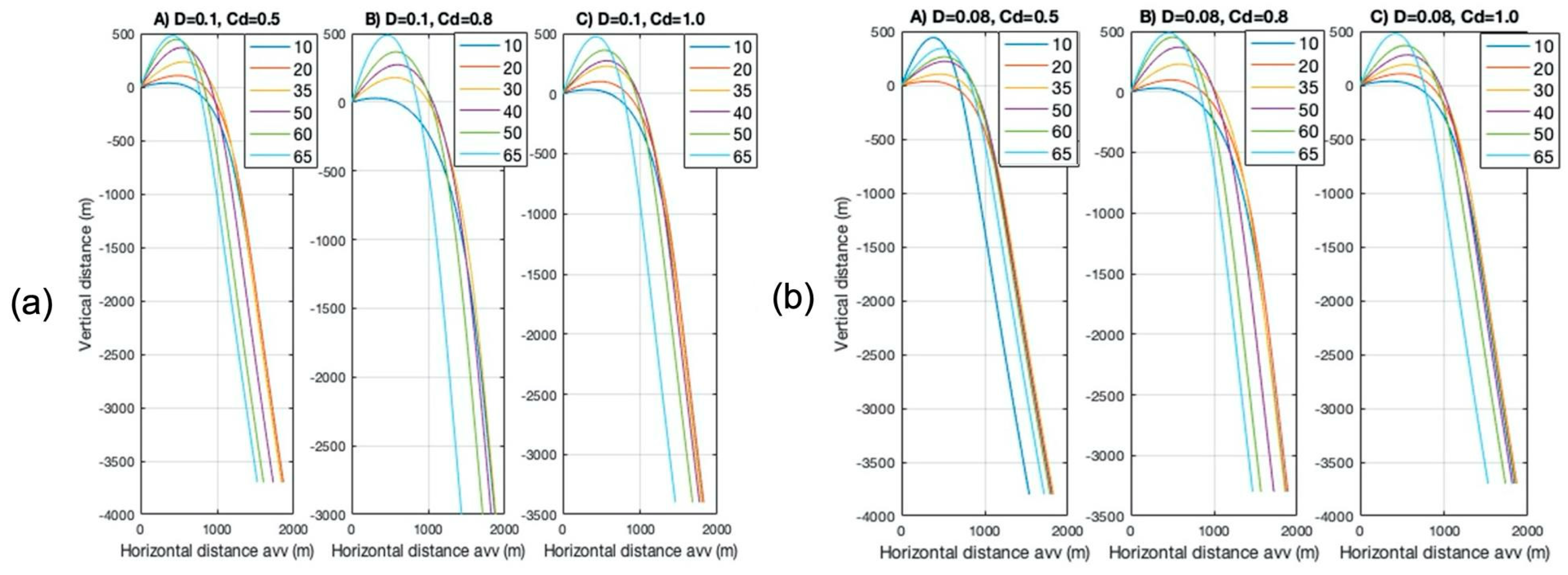
3.3. Eject! Results for the Paroxysmal Episode of 21 February 2022
3.4. Eject! Results for Other Paroxysmal Episodes
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Houghton, B.F.; Wilson, C.; Del Carlo, P.; Coltelli, M.; Sable, J.; Carey, R. The influence of conduit processes on changes in style of basaltic Plinian eruptions: Tarawera 1886 and Etna 122 BC. J. Volcanol. Geotherm. Res. 2004, 137, 1–14. [Google Scholar] [CrossRef]
- Giuffrida, M.; Viccaro, M. Three years (2011–2013) of eruptive activity at Mt. Etna: Working modes and timescales of the modern volcano plumbing system from micro-analytical studies of crystals. Earth-Sci. Rev. 2017, 171, 289–322. [Google Scholar] [CrossRef]
- Giuffrida, M.; Viccaro, M.; Ottolini, L. Ultrafast syn-eruptive degassing and ascent trigger high-energy basic eruptions. Sci. Rep. 2018, 8, 147. [Google Scholar] [CrossRef] [PubMed]
- Zuccarello, F.; Schiavi, F.; Viccaro, M. Magma dehydration controls the energy of recent eruptions at Mt. Etna volcano. Terra Nova 2021, 33, 423–429. [Google Scholar] [CrossRef]
- Zuccarello, F.; Schiavi, F.; Viccaro, M. The eruption run-up at Mt. Etna volcano: Constraining magma decompression rates and their relationships with the final eruptive energy. Earth Planet. Sci. Lett. 2022, 597, 117821. [Google Scholar] [CrossRef]
- Andronico, D.; Cannata, A.; Di Grazia, G.; Ferrari, F. The 1986–2021 paroxysmal episodes at the summit craters of Mt. Etna: Insights into volcano dynamics and hazard. Earth-Sci. Rev. 2021, 220, 103686. [Google Scholar] [CrossRef]
- Cannata, A.; Di Grazia, G.; Giuffrida, M.; Gresta, S.; Palano, M.; Sciotto, M.; Viccaro, M.; Zuccarello, F. Space-Time Evolution of Magma Storage and Transfer at Mt. Etna Volcano (Italy): The 2015–2016 Reawakening of Voragine Crater. Geochem. Geophys. Geosystems 2018, 19, 471–495. [Google Scholar] [CrossRef]
- Viccaro, M.; Giuffrida, M.; Zuccarello, F.; Scandura, M.; Palano, M.; Gresta, S. Violent paroxysmal activity drives self-feeding magma replenishment at Mt. Etna. Sci. Rep. 2019, 9, 6717. [Google Scholar] [CrossRef]
- Calvari, S.; Nunnari, G. Comparison between Automated and Manual Detection of Lava Fountains from Fixed Monitoring Thermal Cameras at Etna Volcano, Italy. Remote Sens. 2022, 14, 2392. [Google Scholar] [CrossRef]
- Mereu, L.; Scollo, S.; Bonadonna, C.; Freret-Lorgeril, V.; Marzano, F.S. Multisensor Characterization of the Incandescent Jet Region of Lava Fountain-Fed Tephra Plumes. Remote Sens. 2020, 12, 3629. [Google Scholar] [CrossRef]
- Scollo, S.; Prestifilippo, M.; Bonadonna, C.; Cioni, R.; Corradini, S.; Degruyter, W.; Rossi, E.; Silvestri, M.; Biale, E.; Carparelli, G.; et al. Near-Real-Time Tephra Fallout Assessment at Mt. Etna, Italy. Remote Sens. 2019, 11, 2987. [Google Scholar] [CrossRef]
- Andronico, D.; Scollo, S.; Cristaldi, A. Unexpected hazards from tephra fallouts at Mt Etna: The 23 November 2013 lava fountain. J. Volcanol. Geotherm. Res. 2015, 304, 118–125. [Google Scholar] [CrossRef]
- De Beni, E.; Behncke, B.; Branca, S.; Nicolosi, I.; Carluccio, R.; D’Ajello Caracciolo, F.; Chiappini, M. The continuing story of Etna’s New Southeast Crater (2012–2014): Evolution and volume calculations based on field surveys and aerophotogrammetry. J. Volcanol. Geotherm. Res. 2015, 303, 175–186. [Google Scholar] [CrossRef]
- Osman, S.; Rossi, E.; Bonadonna, C.; Frischknecht, C.; Andronico, D.; Cioni, R.; Scollo, S. Exposure-based risk assessment and emergency management associated with the fallout of large clasts at Mount Etna. Nat. Hazards Earth Syst. Sci. 2019, 19, 589–610. [Google Scholar] [CrossRef]
- Corradini, S.; Guerrieri, L.; Lombardo, V.; Merucci, L.; Musacchio, M.; Prestifilippo, M.; Scollo, S.; Silvestri, M.; Spata, G.; Stelitano, D. Proximal Monitoring of the 2011–2015 Etna Lava Fountains Using MSG-SEVIRI Data. Geosciences 2018, 8, 140. [Google Scholar] [CrossRef]
- Scollo, S.; Prestifilippo, M.; Spata, G.; D’Agostino, M.; Coltelli, M. Monitoring and forecasting Etna volcanic plumes. Nat. Hazards Earth Syst. Sci. 2009, 9, 1573–1585. [Google Scholar] [CrossRef]
- Coltelli, M.; Miraglia, L.; Scollo, S. Characterization of shape and terminal velocity of tephra particles erupted during the 2002 eruption of Etna volcano, Italy. Bull. Volcanol. 2008, 70, 1103–1112. [Google Scholar] [CrossRef]
- Bonaccorso, A.; Calvari, S.; Linde, A.; Sacks, S. Eruptive processes leading to the most explosive lava fountain at Etna volcano: The 23 November 2013 episode. Geophys. Res. Lett. 2014, 41, 4912–4919. [Google Scholar] [CrossRef]
- Calvari, S.; Salerno, G.G.; Spampinato, L.; Gouhier, M.; La Spina, A.; Pecora, E.; Harris, A.J.L.; Labazuy, P.; Biale, E.; Boschi, E. An unloading foam model to constrain Etna’s 11–13 January 2011 lava fountaining episode. J. Geophys. Res. Solid Earth 2011, 116, B11207. [Google Scholar] [CrossRef]
- Saunderson, H.C. Equations of motion and ballistic paths of volcanic ejecta. Comput. Geosci. 2008, 34, 802–814. [Google Scholar] [CrossRef]
- Sparks, R.S.J. The dimensions and dynamics of volcanic eruption columns. Bull. Volcanol. 1986, 48, 3–15. [Google Scholar] [CrossRef]
- Marzano, F.S.; Picciotti, E.; Montopoli, M.; Vulpiani, G. Inside Volcanic Clouds: Remote Sensing of Ash Plumes Using Microwave Weather Radars. Bull. Am. Meteorol. Soc. 2013, 94, 1567–1586. [Google Scholar] [CrossRef]
- Calvari, S.; Bonaccorso, A.; Ganci, G. Anatomy of a Paroxysmal Lava Fountain at Etna Volcano: The Case of the 12 March 2021, Episode. Remote Sens. 2021, 13, 3052. [Google Scholar] [CrossRef]
- Mereu, L.; Scollo, S.; Bonadonna, C.; Donnadieu, F.; Freret-Lorgeril, V.; Marzano, F.S. Ground-Based Remote Sensing and Uncertainty Analysis of the Mass Eruption Rate Associated With the 3–5 December 2015 Paroxysms of Mt. Etna. IEEE J. Sel. Top. Appl. Earth Obs. Remote. Sens. 2021, 15, 504–518. [Google Scholar] [CrossRef]
- Calvari, S.; Cannavò, F.; Bonaccorso, A.; Spampinato, L.; Pellegrino, A.G. Paroxysmal Explosions, Lava Fountains and Ash Plumes at Etna Volcano: Eruptive Processes and Hazard Implications. Front. Earth Sci. 2018, 6, 107. [Google Scholar] [CrossRef]
- Mastin, L.G. A Simple Calculator of Ballistic Trajectories for Blocks Ejected during Volcanic Eruptions; Technical Reports, 01–45; United States Geological Survey: Reston, VA, USA, 2001. Available online: http://pubs.usgs.gov/of/2001/0045/ (accessed on 28 December 2022). [CrossRef]
- Tsunematsu, K.; Chopard, B.; Falcone, J.-L.; Bonadonna, C. A numerical model of ballistic transport with collisions in a volcanic setting. Comput. Geosci. 2014, 63, 62–69. [Google Scholar] [CrossRef]
- Taddeucci, J.; Alatorre-Ibarguengoitia, M.A.; Palladino, D.M.; Scarlato, P.; Camaldo, C. High-speed imaging of Strombolian eruptions: Gas-pyroclast dynamics in initial volcanic jets. Geophys. Res. Lett. 2015, 42, 6253–6260. [Google Scholar] [CrossRef]
- Núñez-Corrales, S.; Brenes-André, J. BALISTICA: A software suite for ballistic motion with applications to geophysics research and education. Softw. Impacts 2023, 16, 100488. [Google Scholar] [CrossRef]
- Scollo, S.; Folch, A.; Costa, A. A parametric and comparative study of different tephra fallout models. J. Volcanol. Geotherm. Res. 2008, 176, 199–211, ISSN 0377-0273. [Google Scholar] [CrossRef]
- Taddeucci, J.; Alatorre-Ibargüengoitia, M.A.; Cruz-Vázquez, O.; Del Bello, E.; Scarlato, P.; Ricci, T. In-flight dynamics of volcanic ballistic projectiles. Rev. Geoph. 2017, 55, 675–718. [Google Scholar] [CrossRef]




| Ballistics | LAT | LON | ALT (m) | D (m) | a (m) | b (m) | c (m) | dg (m) | M (kg) | AR |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 37.7310 | 15.0078 | 2706 | 1809.0 | 0.090 | 0.065 | 0.060 | 0.071 | 0.119 | 0.667 |
| 2 | 37.7310 | 15.0079 | 2706 | 1806.8 | 0.085 | 0.075 | 0.060 | 0.073 | 0.104 | 0.706 |
| 3 | 37.7310 | 15.0081 | 2706 | 1811.6 | 0.105 | 0.075 | 0.065 | 0.080 | 0.170 | 0.619 |
| 4 | 37.7311 | 15.0083 | 2700 | 1812.3 | 0.110 | 0.080 | 0.070 | 0.085 | 0.174 | 0.636 |
| 5 | 37.7310 | 15.0083 | 2700 | 1824.4 | 0.085 | 0.075 | 0.050 | 0.068 | 0.710 | 0.588 |
| 6 | 37.7309 | 15.0083 | 2703 | 1832.9 | 0.075 | 0.050 | 0.045 | 0.055 | 0.058 | 0.600 |
| 7 | 37.7309 | 15.0083 | 2702 | 1836.2 | 0.080 | 0.055 | 0.045 | 0.058 | 0.124 | 0.563 |
| 8 | 37.7308 | 15.0083 | 2702 | 1843.7 | 0.090 | 0.065 | 0.050 | 0.066 | 0.110 | 0.556 |
| 9 | 37.7308 | 15.0082 | 2705 | 1843.1 | 0.073 | 0.070 | 0.055 | 0.066 | 0.102 | 0.753 |
| 10 | 37.7308 | 15.0082 | 2705 | 1843.1 | 0.065 | 0.055 | 0.055 | 0.058 | 0.057 | 0.846 |
| 11 | 37.7308 | 15.0082 | 2705 | 1843.1 | 0.070 | 0.055 | 0.040 | 0.054 | 0.047 | 0.571 |
| 12 | 37.7308 | 15.0082 | 2705 | 1843.1 | 0.060 | 0.043 | 0.045 | 0.049 | 0.050 | 0.750 |
| 13 | 37.7305 | 15.0080 | 2703 | 1864.2 | 0.166 | 0.110 | 0.100 | 0.122 | 0.420 | 0.602 |
| 14 | 37.7310 | 15.0081 | 2708 | 1815.8 | 0.055 | 0.045 | 0.030 | 0.042 | 0.025 | 0.545 |
| 15 | 37.7310 | 15.0081 | 2708 | 1815.8 | 0.065 | 0.045 | 0.042 | 0.050 | 0.310 | 0.646 |
| 16 | 37.7310 | 15.0081 | 2708 | 1815.8 | 0.055 | 0.040 | 0.035 | 0.043 | 0.021 | 0.636 |
| 17 | 37.7312 | 15.0081 | 2711 | 1792.6 | 0.135 | 0.110 | 0.100 | 0.114 | 0.437 | 0.741 |
| 18 | 37.7314 | 15.0080 | 2714 | 1769.9 | 0.155 | 0.115 | 0.095 | 0.119 | 0.497 | 0.613 |
| 19 | 37.7314 | 15.0080 | 2713 | 1773.0 | 0.140 | 0.120 | 0.110 | 0.123 | 0.516 | 0.786 |
| 20 | 37.7316 | 15.0072 | 2726 | 1727.1 | 0.090 | 0.075 | 0.065 | 0.076 | 0.108 | 0.722 |
| 21 | 37.7316 | 15.0072 | 2726 | 1727.1 | 0.045 | 0.040 | 0.030 | 0.038 | 0.030 | 0.667 |
| 22 | 37.7316 | 15.0072 | 2726 | 1727.1 | 0.085 | 0.070 | 0.070 | 0.075 | 0.102 | 0.24 |
| 23 | 37.7316 | 15.0072 | 2726 | 1727.1 | 0.080 | 0.050 | 0.045 | 0.056 | 0.088 | 0.563 |
| 24 | 37.7316 | 15.0072 | 2726 | 1727.1 | 0.055 | 0.045 | 0.035 | 0.044 | 0.043 | 0.636 |
| 25 | 37.7316 | 15.0072 | 2726 | 1727.1 | 0.100 | 0.075 | 0.065 | 0.079 | 0.142 | 0.650 |
| 26 | 37.7316 | 15.0072 | 2726 | 1727.1 | 0.043 | 0.035 | 0.025 | 0.034 | 0.021 | 0.581 |
| 27 | 37.7315 | 15.0069 | 2722 | 1733.7 | 0.065 | 0.055 | 0.040 | 0.052 | 0.066 | 0.615 |
| 28 | 37.7315 | 15.0069 | 2722 | 1733.7 | 0.090 | 0.050 | 0.045 | 0.059 | 0.095 | 0.500 |
| 29 | 37.7315 | 15.0069 | 2722 | 1733.7 | 0.060 | 0.045 | 0.040 | 0.048 | 0.055 | 0.667 |
| 30 | 37.7315 | 15.0069 | 2722 | 1733.7 | 0.055 | 0.045 | 0.035 | 0.044 | 0.043 | 0.636 |
| 31 | 37.7314 | 15.0068 | 2721 | 1735.8 | 0.130 | 0.070 | 0.085 | 0.092 | 0.427 | 0.654 |
| 32 | 37.7315 | 15.0068 | 2723 | 1727.9 | 0.095 | 0.070 | 0.055 | 0.072 | 0.128 | 0.579 |
| 33 | 37.7315 | 15.0068 | 2723 | 1727.9 | 0.070 | 0.05 | 0.040 | 0.052 | 0.068 | 0.571 |
| 34 | 37.7315 | 15.0068 | 2723 | 1727.9 | 0.090 | 0.07 | 0.055 | 0.070 | 0.144 | 0.611 |
| 35 | 37.7315 | 15.0068 | 2723 | 1727.9 | 0.060 | 0.05 | 0,03 | 0.045 | 0.038 | 0.500 |
| 36 | 37.7316 | 15.0072 | 2638 | 1725.5 | 0.050 | 0.04 | 0.025 | 0.037 | 0.029 | 0.500 |
| 37 | 37.7316 | 15.0072 | 2638 | 1725.5 | 0.075 | 0055 | 0.040 | 0.548 | 0.077 | 0.533 |
| 38 | 37.7316 | 15.0072 | 2638 | 1725.5 | 0.070 | 0.055 | 0.045 | 0.056 | 0.077 | 0.643 |
| 39 | 37.7316 | 15.0072 | 2638 | 1725.5 | 0.055 | 0.050 | 0.035 | 0.046 | 0.032 | 0.636 |
| 40 | 37.7316 | 15.0072 | 2638 | 1725.5 | 0.050 | 0.040 | 0.035 | 0.041 | 0.031 | 0.700 |
| INPUT | OUTPUT | |||||||
|---|---|---|---|---|---|---|---|---|
| Diameter Class (m) | Ejection Angle (Degree) | Initial Velocity (m/s) | Distance of Landing Point below the IJR (m) | Drag Coefficient | Distance (m) | Maximum Height (m) | Final Velocity (m/s) | Travel Time (s) |
| 0.08 | 45 | 155 | 3300 | 0.5 | 1762 | 318 | 70 | 60 |
| 0.08 | 45 | 180 | 3700 | 0.8 | 1768 | 319 | 57 | 75 |
| 0.08 | 45 | 200 | 3800 | 1.0 | 1747 | 297 | 51 | 83 |
| INPUT | OUTPUT | |||||||
| Diameter Class (m) | Ejection Angle (Degree) | Initial Velocity (m/s) | Distance of Landing Point below the IJR (m) | Drag Coefficient | Distance (m) | Maximum Height (m) | Final Velocity (m/s) | Travel Time (s) |
| 0.1 | 45 | 120 | 3000 | 0.5 | 1773 | 319 | 78 | 52 |
| 0.1 | 45 | 110 | 3400 | 0.8 | 1730 | 317 | 63 | 65 |
| 0.1 | 45 | 90 | 3700 | 1.0 | 1768 | 319 | 57 | 75 |
| INPUT | OUTPUT | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Lava Fountain | Diameter Class (m) | Ejection Angle (Degree) | Initial Velocity (m/s) | Distance of Landing Point below the IJR (m) | Drag Coefficient | Density (kg/m3) | Distance (m) | Maximum Height (m) | Final Velocity (m/s) | Travel Time (s) |
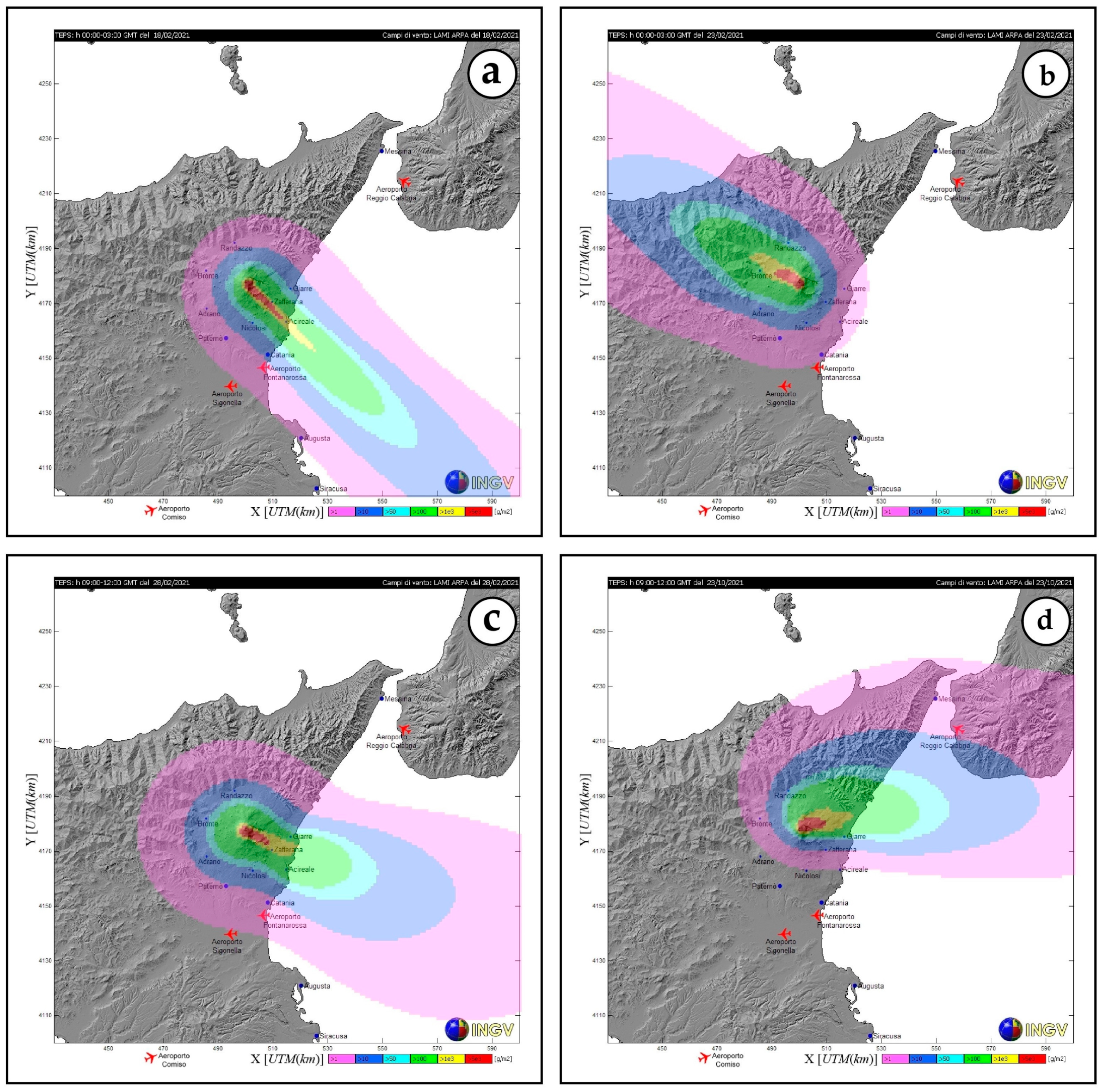
| Feb 18 | 0.1 | 45 | 200 | 4720 | 0.1 | 1300 | 7066 | 923 | 266 | 50 |
| Feb 23 | 0.1 | 70 | 180 | 2950 | 0.1 | 1500 | 2721 | 1272 | 229 | 47 |
| Feb 28 | 0.1 | 70 | 280 | 5700 | 0.1 | 1500 | 5936 | 2935 | 295 | 70 |
| Oct 23 | 0.1 | 50 | 230 | 4250 | 0.1 | 1500 | 5459 | 1173 | 195 | 54 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Costa, G.; Mereu, L.; Prestifilippo, M.; Scollo, S.; Viccaro, M. Modeling the Trajectories of Ballistics in the Summit Area of Mt. Etna (Italy) during the 2020–2022 Sequence of Lava Fountains. Geosciences 2023, 13, 145. https://doi.org/10.3390/geosciences13050145
Costa G, Mereu L, Prestifilippo M, Scollo S, Viccaro M. Modeling the Trajectories of Ballistics in the Summit Area of Mt. Etna (Italy) during the 2020–2022 Sequence of Lava Fountains. Geosciences. 2023; 13(5):145. https://doi.org/10.3390/geosciences13050145
Chicago/Turabian StyleCosta, Giorgio, Luigi Mereu, Michele Prestifilippo, Simona Scollo, and Marco Viccaro. 2023. "Modeling the Trajectories of Ballistics in the Summit Area of Mt. Etna (Italy) during the 2020–2022 Sequence of Lava Fountains" Geosciences 13, no. 5: 145. https://doi.org/10.3390/geosciences13050145
APA StyleCosta, G., Mereu, L., Prestifilippo, M., Scollo, S., & Viccaro, M. (2023). Modeling the Trajectories of Ballistics in the Summit Area of Mt. Etna (Italy) during the 2020–2022 Sequence of Lava Fountains. Geosciences, 13(5), 145. https://doi.org/10.3390/geosciences13050145












