Analytical and Numerical Solutions for Three-Dimensional Granular Collapses
Abstract
1. Introduction
2. Materials and Methods
2.1. Laboratory Experiments
2.1.1. Experimental Setup and Data Collection
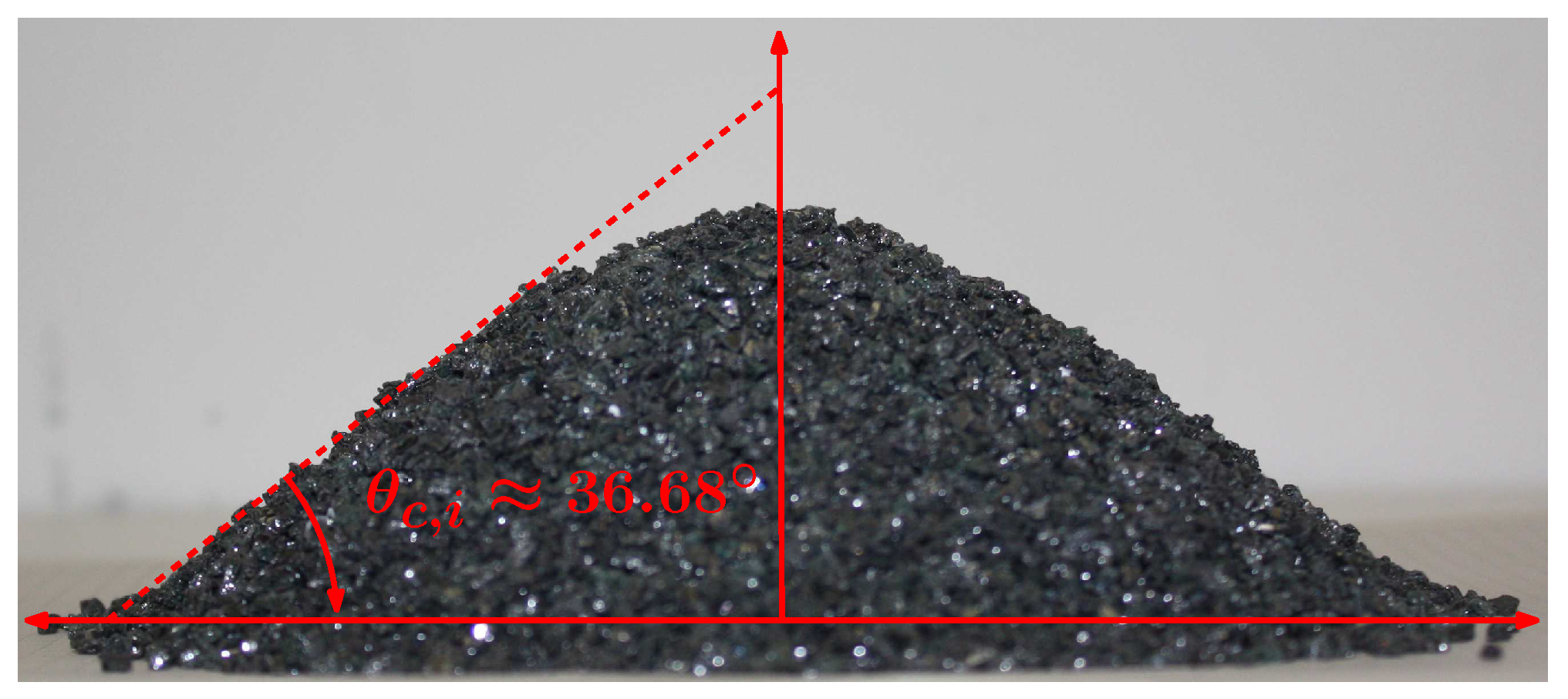
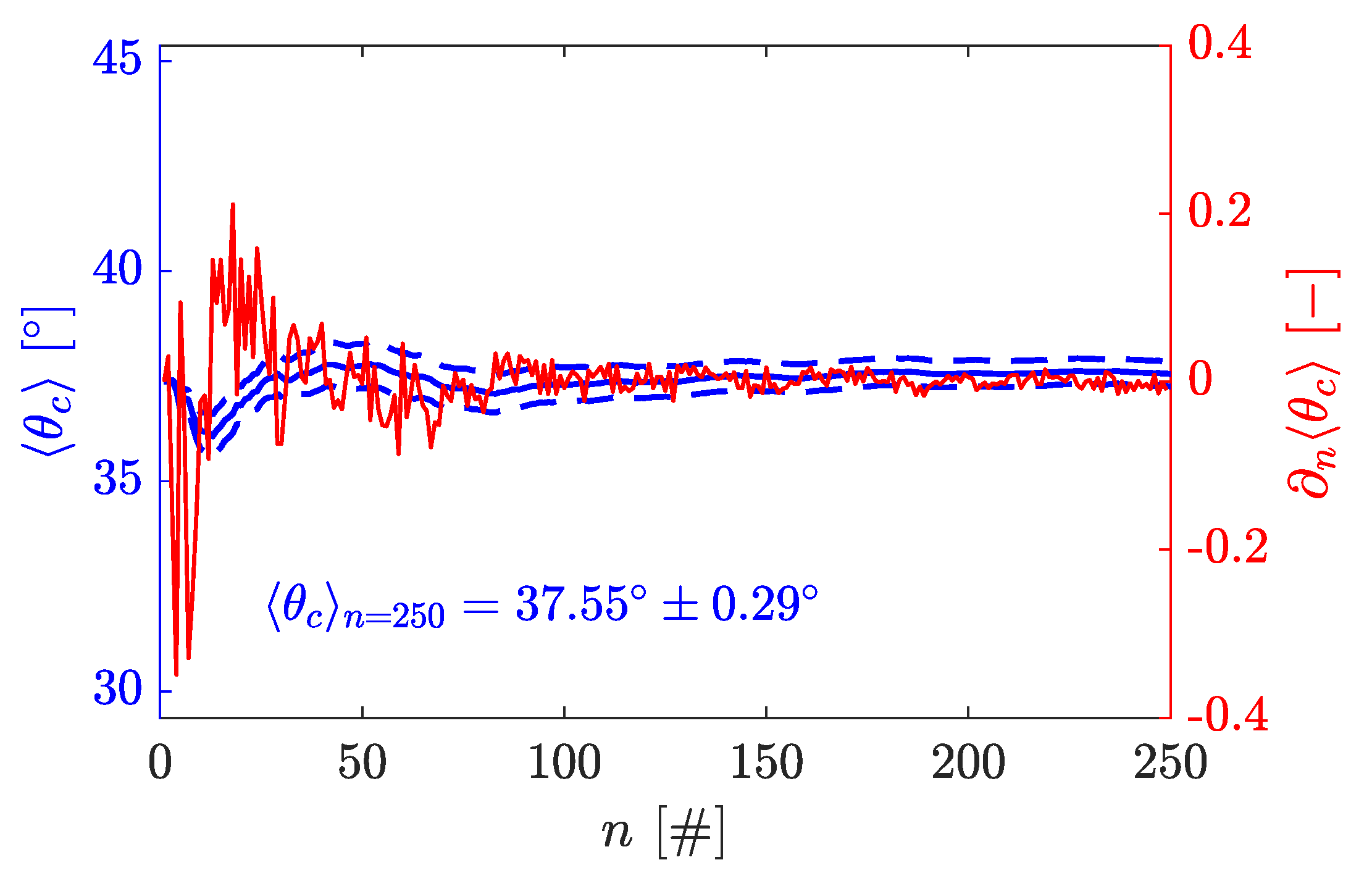
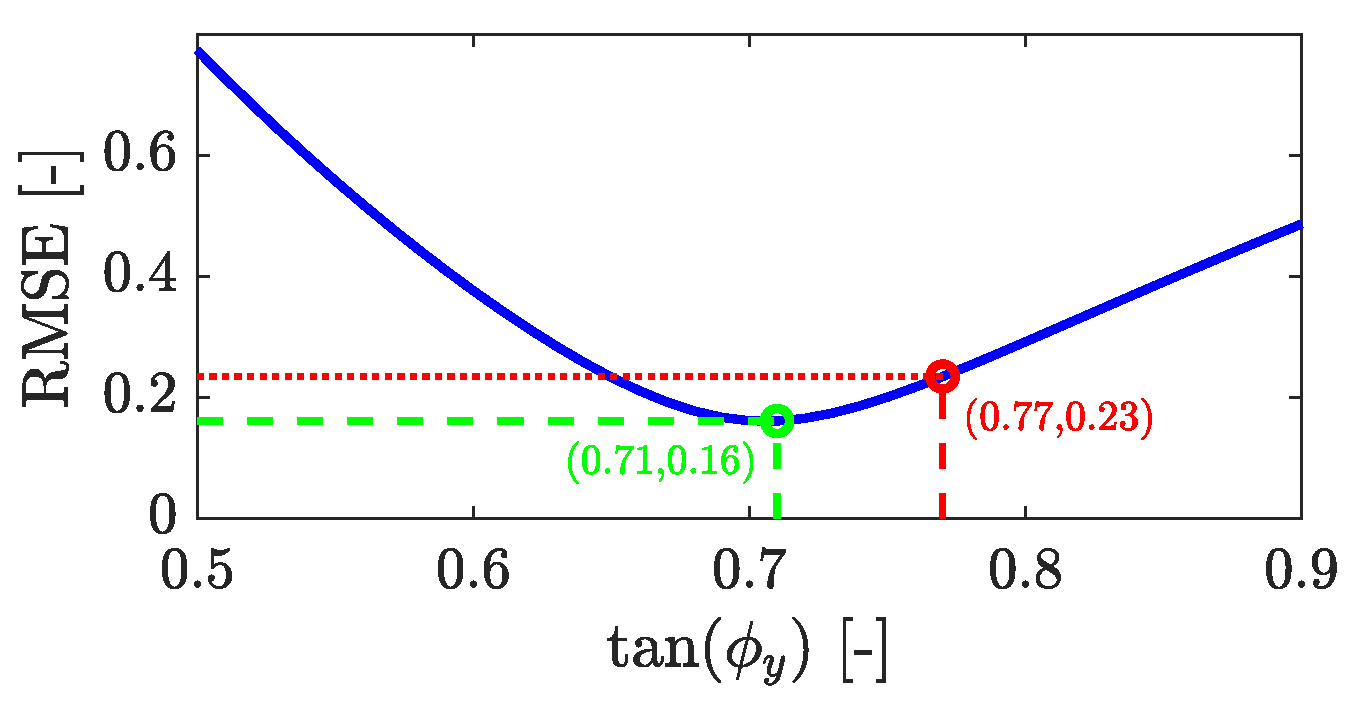
2.1.2. Experimental Estimation of the Angle of Repose
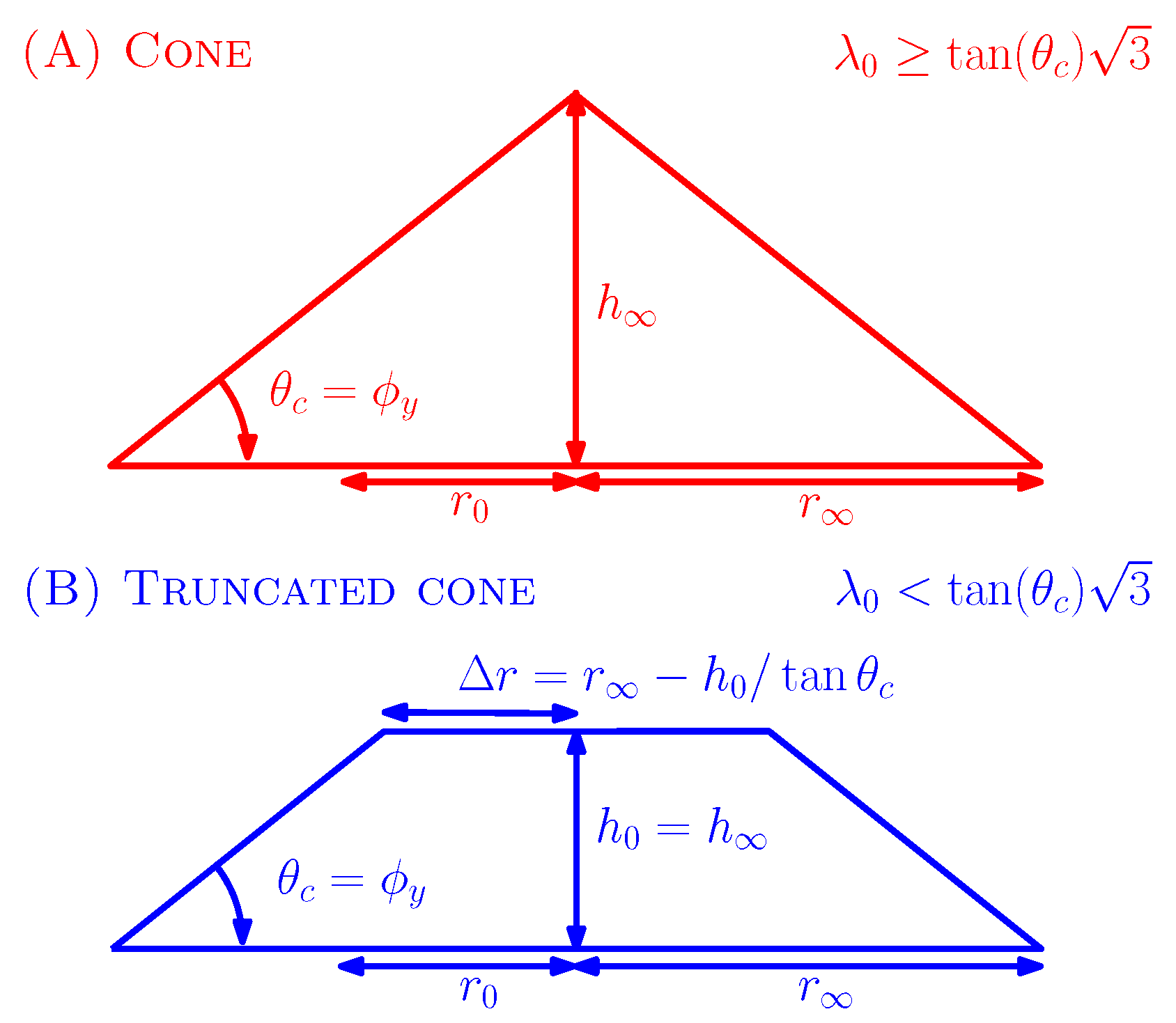
2.2. A Continuously Smooth Piecewise Analytical Solution
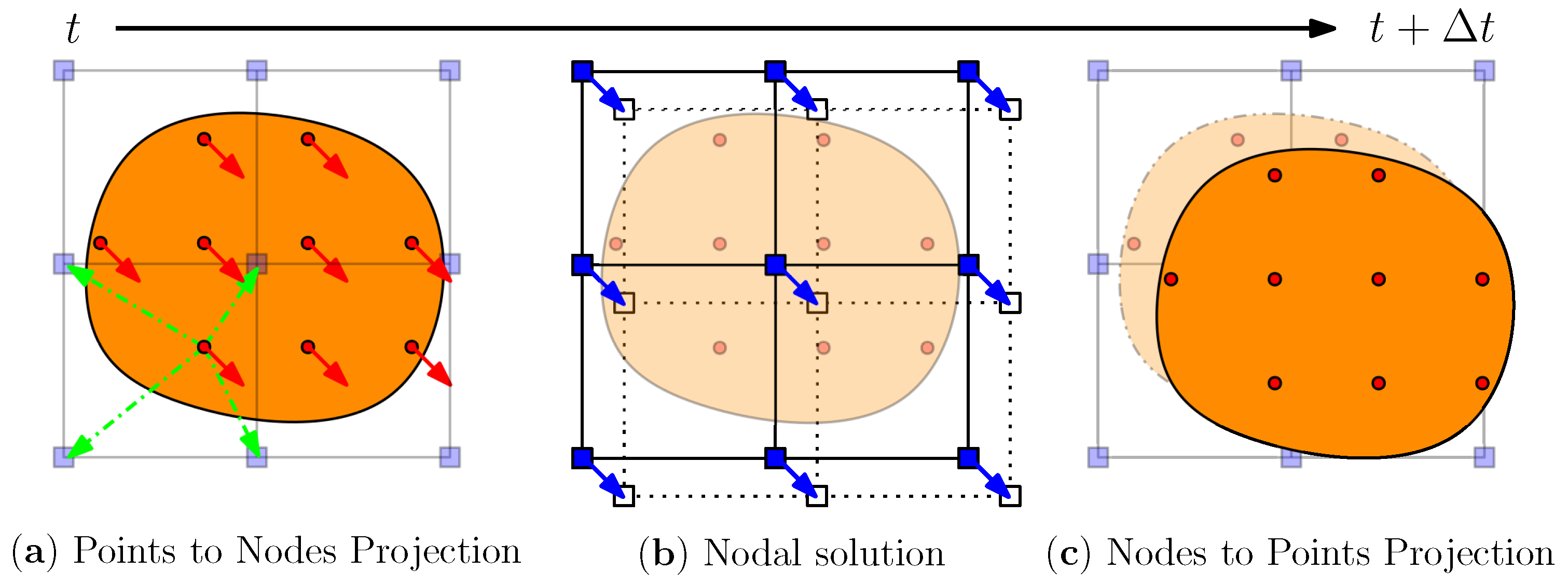
2.3. Numerical Simulation
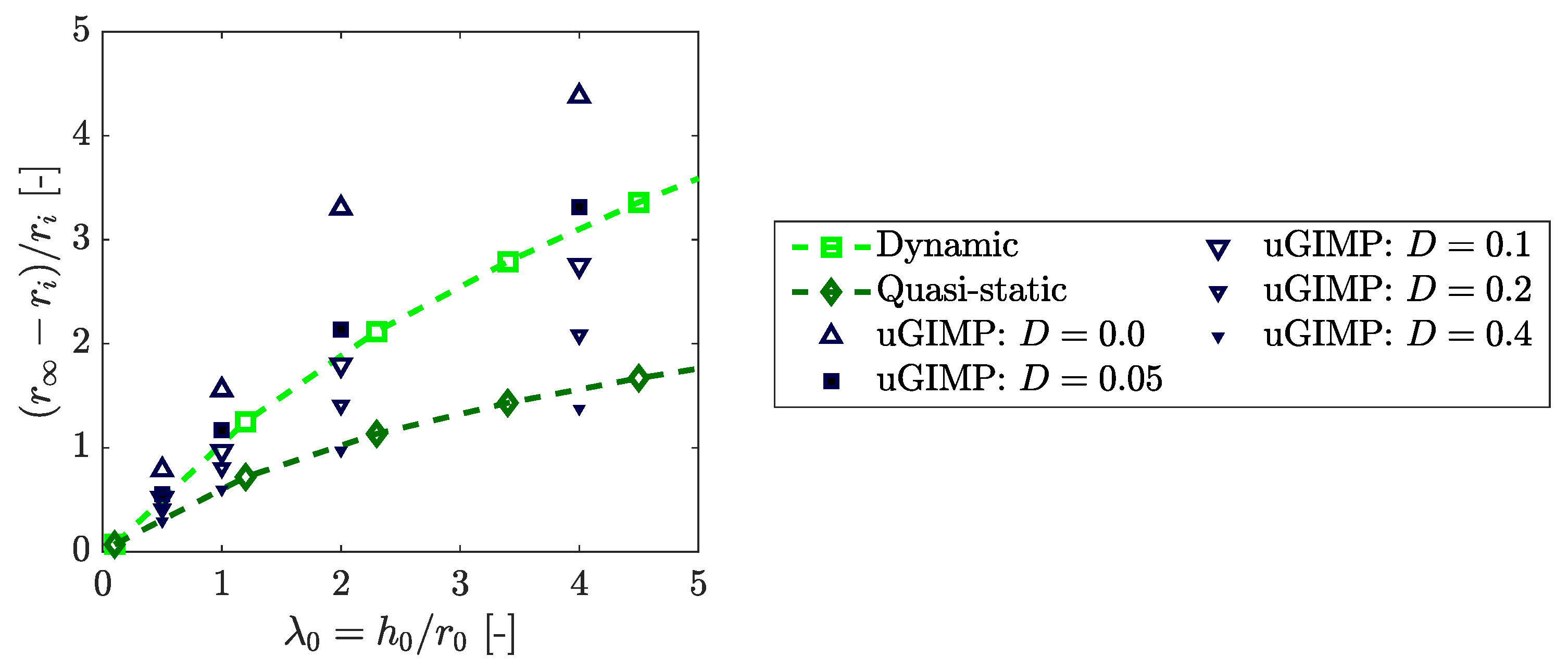
2.4. Numerical Parameters and Geometry
3. Results
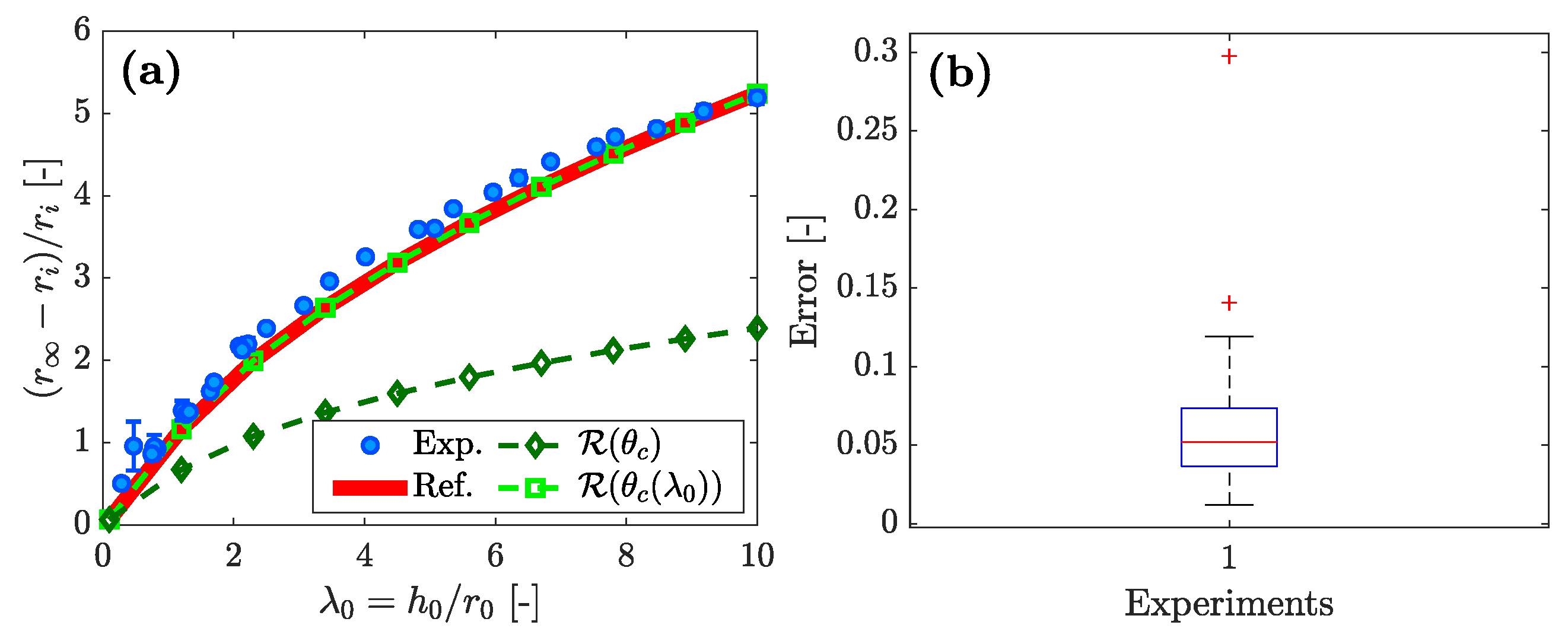
3.1. Analytical Solutions and Experimental Collapses
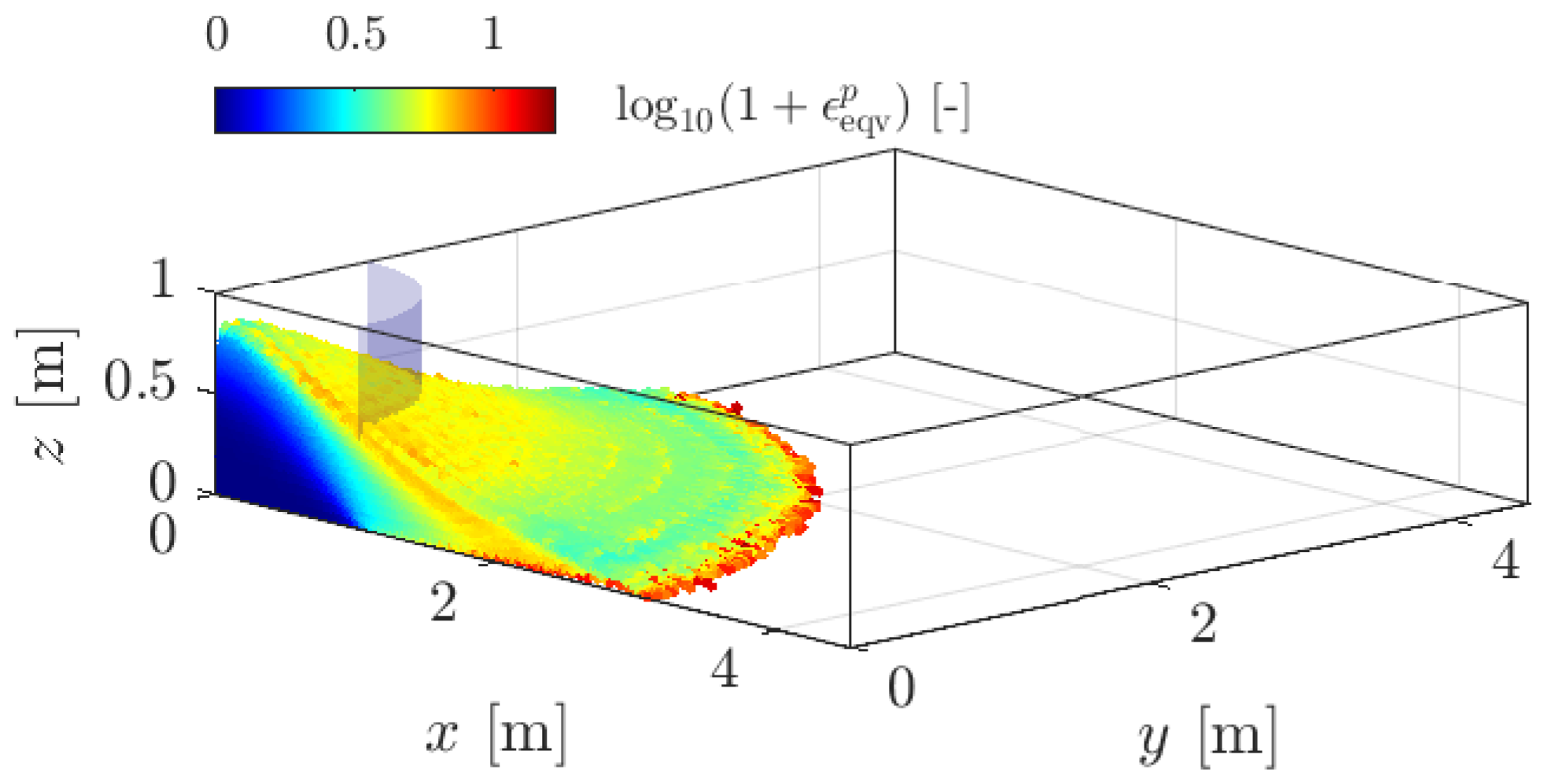
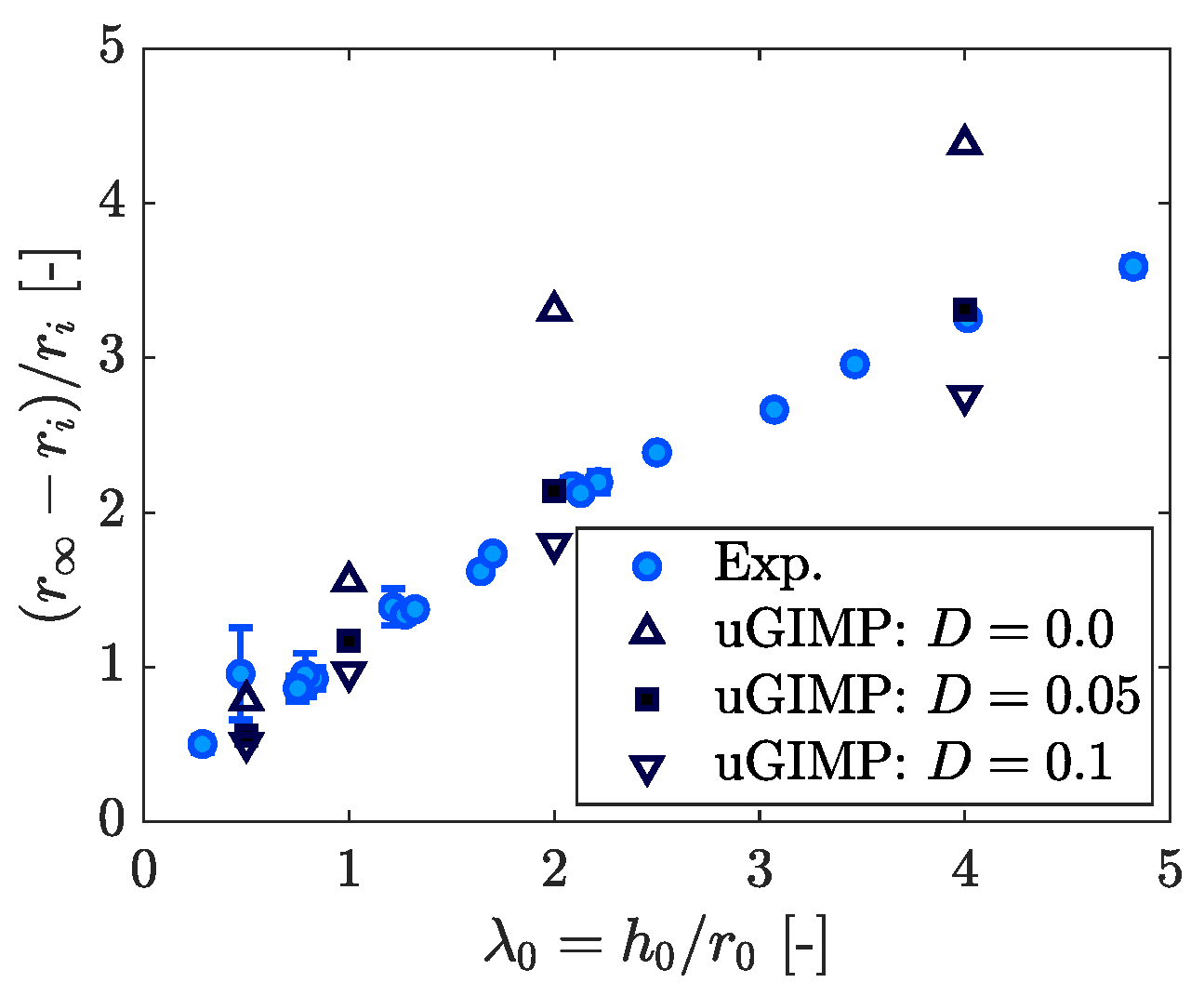
3.2. Experimental and Numerical Granular Collapses
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
Abbreviations
| MPM | Material point method |
| GIMP | Generalised interpolation material point method |
| GPU | Graphics processing unit |
| MPI | Message-passing interface |
Appendix A
References
- Lowe, D.R. Grain flow and grain flow deposits. J. Sediment. Res. 1976, 46, 188–199. [Google Scholar]
- Barabási, A.L.; Albert, R.; Schiffer, P. The physics of sand castles: Maximum angle of stability in wet and dry granular media. Phys. A Stat. Mech. Its Appl. 1999, 266, 366–371. [Google Scholar] [CrossRef]
- Tsuji, D.; Otsuki, M.; Katsuragi, H. Relaxation dynamics of a granular pile on a vertically vibrating plate. Phys. Rev. Lett. 2018, 120, 128001. [Google Scholar] [CrossRef]
- Carrigy, M.A. Experiments on the angles of repose of granular materials 1. Sedimentology 1970, 14, 147–158. [Google Scholar] [CrossRef]
- Balmforth, N.J.; Kerswell, R.R. Granular collapse in two dimensions. J. Fluid Mech. 2005, 538, 399–428. [Google Scholar] [CrossRef]
- Pohlman, N.A.; Severson, B.L.; Ottino, J.M.; Lueptow, R.M. Surface roughness effects in granular matter: Influence on angle of repose and the absence of segregation. Phys. Rev. E 2006, 73, 031304. [Google Scholar] [CrossRef]
- Mitarai, N.; Nori, F. Wet granular materials. Adv. Phys. 2006, 55, 1–45. [Google Scholar] [CrossRef]
- Lee, J.; Herrmann, H.J. Angle of repose and angle of marginal stability: Molecular dynamics of granular particles. J. Phys. A Math. Gen. 1993, 26, 373–383. [Google Scholar] [CrossRef]
- Miller, R.L.; Byrne, R.J. The angle of repose for a single grain on a fixed rough bed. Sedimentology 1966, 6, 303–314. [Google Scholar] [CrossRef]
- Buffington, J.M.; Dietrich, W.E.; Kirchner, J.W. Friction angle measurements on a naturally formed gravel streambed: Implications for critical boundary shear stress. Water Resour. Res. 1992, 28, 411–425. [Google Scholar] [CrossRef]
- Grasselli, Y.; Herrmann, H.J.; Oron, G.; Zapperi, S. Effect of impact energy on the shape of granular heaps. Granul. Matter 2000, 2, 97–100. [Google Scholar] [CrossRef]
- Kleinhans, M.G.; Markies, H.; de Vet, S.J.; in’t Veld, A.C.; Postema, F.N. Static and dynamic angles of repose in loose granular materials under reduced gravity. J. Geophys. Res. Planets 2011, 116. Available online: https://agupubs.onlinelibrary.wiley.com/doi/pdf/10.1029/2011JE003865 (accessed on 3 March 2018). [CrossRef]
- Al-Hashemi, H.M.B.; Al-Amoudi, O.S.B. A review on the angle of repose of granular materials. Powder Technol. 2018, 330, 397–417. [Google Scholar] [CrossRef]
- Mangeney, A.; Roche, O.; Hungr, O.; Mangold, N.; Faccanoni, G.; Lucas, A. Erosion and mobility in granular collapse over sloping beds. J. Geophys. Res. Earth Surf. 2010, 115, F03040. [Google Scholar] [CrossRef]
- Rondon, L.; Pouliquen, O.; Aussillous, P. Granular collapse in a fluid: Role of the initial volume fraction. Phys. Fluids 2011, 23, 073301. [Google Scholar] [CrossRef]
- Kumar, K.; Delenne, J.Y.; Soga, K. Mechanics of granular column collapse in fluid at varying slope angles. J. Hydrodyn. 2017, 29, 529–541. [Google Scholar] [CrossRef]
- Bougouin, A.; Lacaze, L. Granular collapse in a fluid: Different flow regimes for an initially dense-packing. Phys. Rev. Fluids 2018, 3, 064305. [Google Scholar] [CrossRef]
- Staron, L.; Hinch, E.J. Study of the collapse of granular columns using two-dimensional discrete-grain simulation. J. Fluid Mech. 2005, 545, 1–27. [Google Scholar] [CrossRef]
- Lube, G.; Huppert, H.E.; Sparks, R.S.J.; Freundt, A. Collapses of two-dimensional granular columns. Phys. Rev. E 2005, 72, 041301. [Google Scholar] [CrossRef]
- Lagrée, P.Y.; Staron, L.; Popinet, S. The granular column collapse as a continuum: Validity of a two-dimensional Navier-Stokes model with a [mu](I)-rheology. J. Fluid Mech. 2011, 686, 378. [Google Scholar] [CrossRef]
- Dunatunga, S.; Kamrin, K. Continuum modelling and simulation of granular flows through their many phases. J. Fluid Mech. 2015, 779, 483–513. [Google Scholar] [CrossRef]
- Thompson, E.L.; Huppert, H.E. Granular column collapses: Further experimental results. J. Fluid Mech. 2007, 575, 177. [Google Scholar] [CrossRef]
- Warnett, J.; Denissenko, P.; Thomas, P.; Kiraci, E.; Williams, M. Scalings of axisymmetric granular column collapse. Granul. Matter 2014, 16, 115–124. [Google Scholar] [CrossRef]
- Langlois, V.J.; Quiquerez, A.; Allemand, P. Collapse of a two-dimensional brittle granular column: Implications for understanding dynamic rock fragmentation in a landslide. J. Geophys. Res. Earth Surf. 2015, 120, 1866–1880. [Google Scholar] [CrossRef]
- Mériaux, C. Two dimensional fall of granular columns controlled by slow horizontal withdrawal of a retaining wall. Phys. Fluids 2006, 18, 093301. [Google Scholar] [CrossRef]
- Lo Giudice, A.; Giammanco, G.; Fransos, D.; Preziosi, L. Modeling sand slides by a mechanics-based degenerate parabolic equation. Math. Mech. Solids 2019, 24, 2558–2575. [Google Scholar] [CrossRef]
- Lajeunesse, E.; Mangeney-Castelnau, A.; Vilotte, J. Spreading of a granular mass on a horizontal plane. Phys. Fluids 2004, 16, 2371–2381. [Google Scholar] [CrossRef]
- Baumgarten, A.S.; Kamrin, K. A general fluid–sediment mixture model and constitutive theory validated in many flow regimes. J. Fluid Mech. 2019, 861, 721–764. [Google Scholar] [CrossRef]
- Fern, J.; Rohe, A.; Soga, K.; Alonso, E. The Material Point Method for Geotechnical Engineering: A Practical Guide; CRC Press: Boca Raton, FL, USA, 2019. [Google Scholar]
- Fern, E.J.; Soga, K. The role of constitutive models in MPM simulations of granular column collapses. Acta Geotech. 2016, 11, 659–678. [Google Scholar] [CrossRef]
- Soga, K.; Alonso, E.; Yerro, A.; Kumar, K.; Bandara, S. Trends in large-deformation analysis of landslide mass mouvements with particular exmphasis on the material point method. Géotechnique 2016, 66, 248–273. [Google Scholar] [CrossRef]
- Wyser, E.; Alkhimenkov, Y.; Jaboyedoff, M.; Podladchikov, Y.Y. A fast and efficient MATLAB-based MPM solver: fMPMM-solver v1.1. Geosci. Model Dev. 2020, 13, 6265–6284. [Google Scholar] [CrossRef]
- Bardenhagen, S.G.; Kober, E.M. The generalized interpolation material point method. Comput. Model. Eng. Sci. 2004, 5, 477–496. [Google Scholar]
- Wyser, E.; Alkhimenkov, Y.; Jaboyedoff, M.; Podladchikov, Y.Y. An explicit GPU-based material point method solver for elastoplastic problems (ep2-3De v1.0). Geosci. Model Dev. 2021, 14, 7749–7774. [Google Scholar] [CrossRef]
- Coombs, W.; Augarde, C.; Brennan, A.; Brown, M.; Charlton, T.; Knappett, J.; Motlagh, Y.; Wang, L. On Lagrangian mechanics and the implicit meterial point method for large deformation elasto-plasticity. Comput. Methods Appl. Mech. Eng. 2020, 358, 112622. [Google Scholar] [CrossRef]
- Huang, P.; Li, S.l.; Guo, H.; Hao, Z.m. Large deformation failure analysis of the soil slope based on the material point method. Comput. Geosci. 2015, 19, 951–963. [Google Scholar] [CrossRef]
- Nguyen, N.H.T.; Bui, H.H.; Nguyen, G.D. Effects of material properties on the mobility of granular flow. Granul. Matter 2020, 22, 59. [Google Scholar] [CrossRef]
- Wang, B.; Vardon, P.; Hicks, M. Investigation of retrogressive and progressive slope failure mechanisms using the material point method. Comput. Geotech. 2016, 78, 88–98. [Google Scholar] [CrossRef]
- Charlton, T.J.; Coombs, W.M.; Augarde, C.E. iGIMP: An implicit generalised interpolation material point method for large deformations. Comput. Struct. 2017, 190, 108–125. [Google Scholar] [CrossRef]
- Coombs, W.M.; Augarde, C.E. AMPLE: A Material Point Learning Environment. Adv. Eng. Softw. 2020, 139, 102748. [Google Scholar] [CrossRef]



| [m] | ||||
|---|---|---|---|---|
| 0.5 | 27,104 | 30,375 | 25,120 | 0.05 |
| 1.0 | 169,344 | 180,625 | 50,240 | 0.05 |
| 2.0 | 1,183,424 | 1,225,125 | 100,480 | 0.05 |
| 4.0 | 8,817,984 | 8,978,125 | 200,960 | 0.05 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wyser, E.; Alkhimenkov, Y.; Jaboyedoff, M.; Podladchikov, Y.Y. Analytical and Numerical Solutions for Three-Dimensional Granular Collapses. Geosciences 2023, 13, 119. https://doi.org/10.3390/geosciences13040119
Wyser E, Alkhimenkov Y, Jaboyedoff M, Podladchikov YY. Analytical and Numerical Solutions for Three-Dimensional Granular Collapses. Geosciences. 2023; 13(4):119. https://doi.org/10.3390/geosciences13040119
Chicago/Turabian StyleWyser, Emmanuel, Yury Alkhimenkov, Michel Jaboyedoff, and Yury Y. Podladchikov. 2023. "Analytical and Numerical Solutions for Three-Dimensional Granular Collapses" Geosciences 13, no. 4: 119. https://doi.org/10.3390/geosciences13040119
APA StyleWyser, E., Alkhimenkov, Y., Jaboyedoff, M., & Podladchikov, Y. Y. (2023). Analytical and Numerical Solutions for Three-Dimensional Granular Collapses. Geosciences, 13(4), 119. https://doi.org/10.3390/geosciences13040119







