GRACE Accelerometers Sensitive to Ionosphere Plasma Waves: Similarities between Twangs and Whistlers
Abstract
1. Introduction
2. Materials and Methods
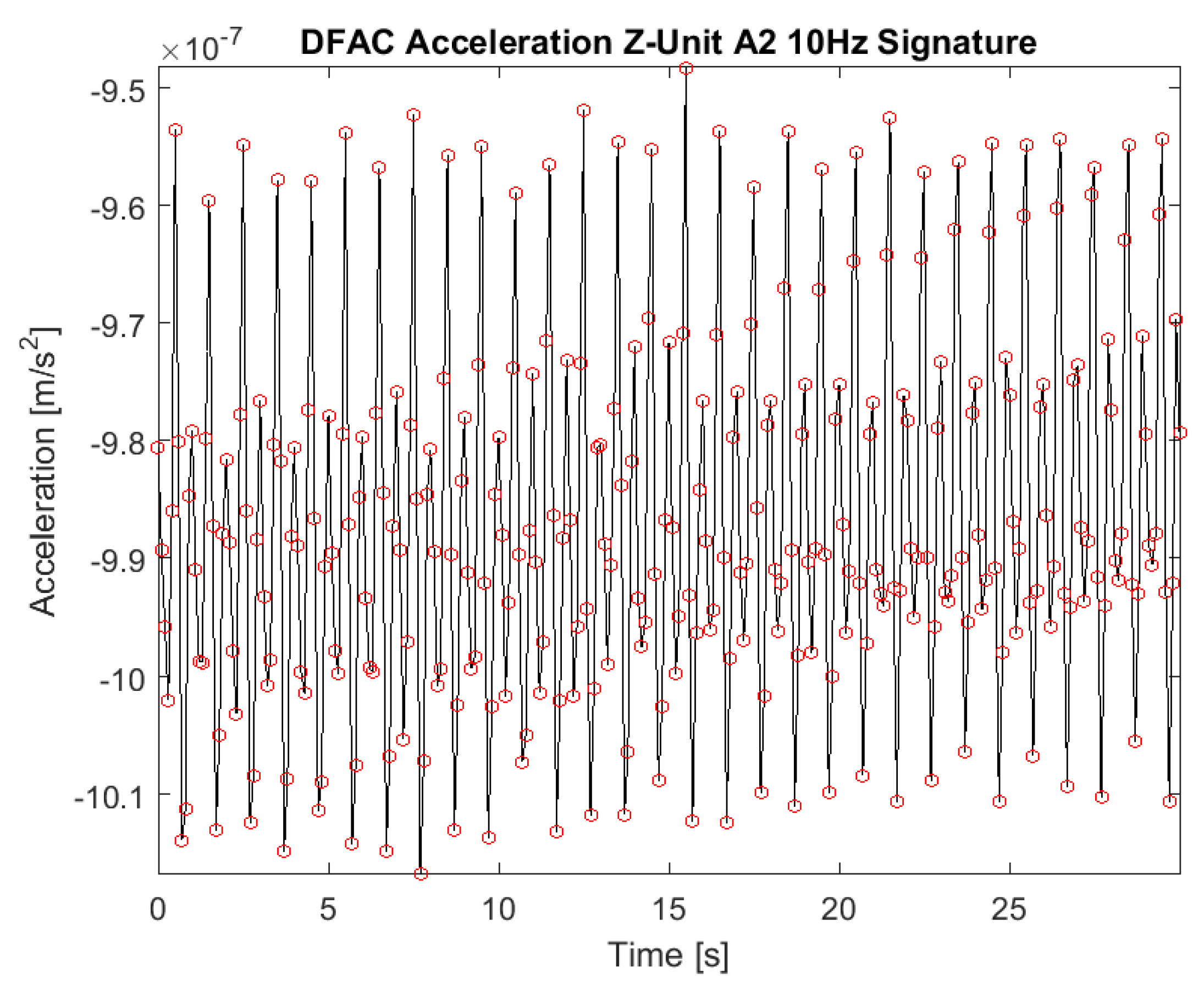
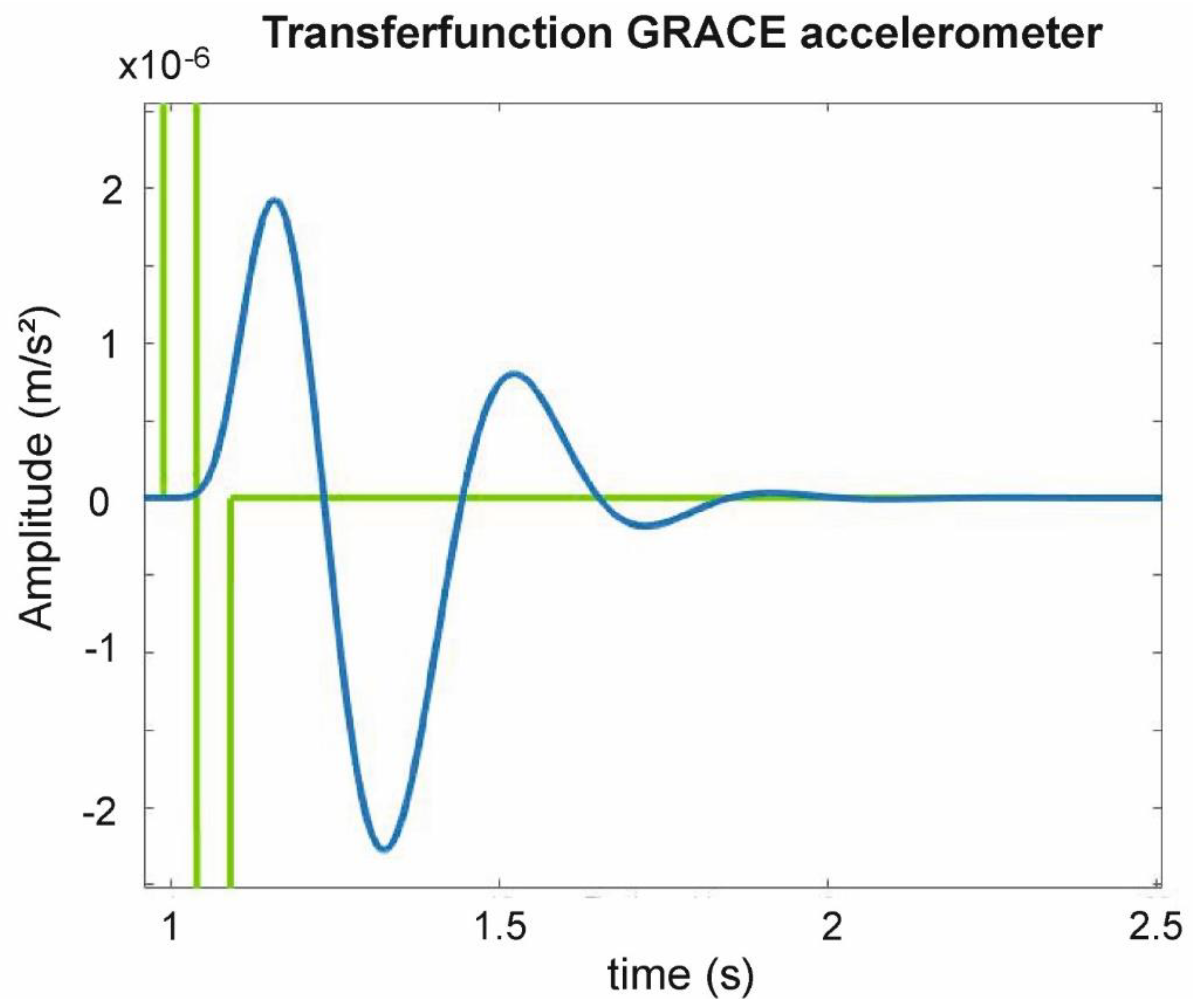
2.1. Electrostatic Space Accelerometers
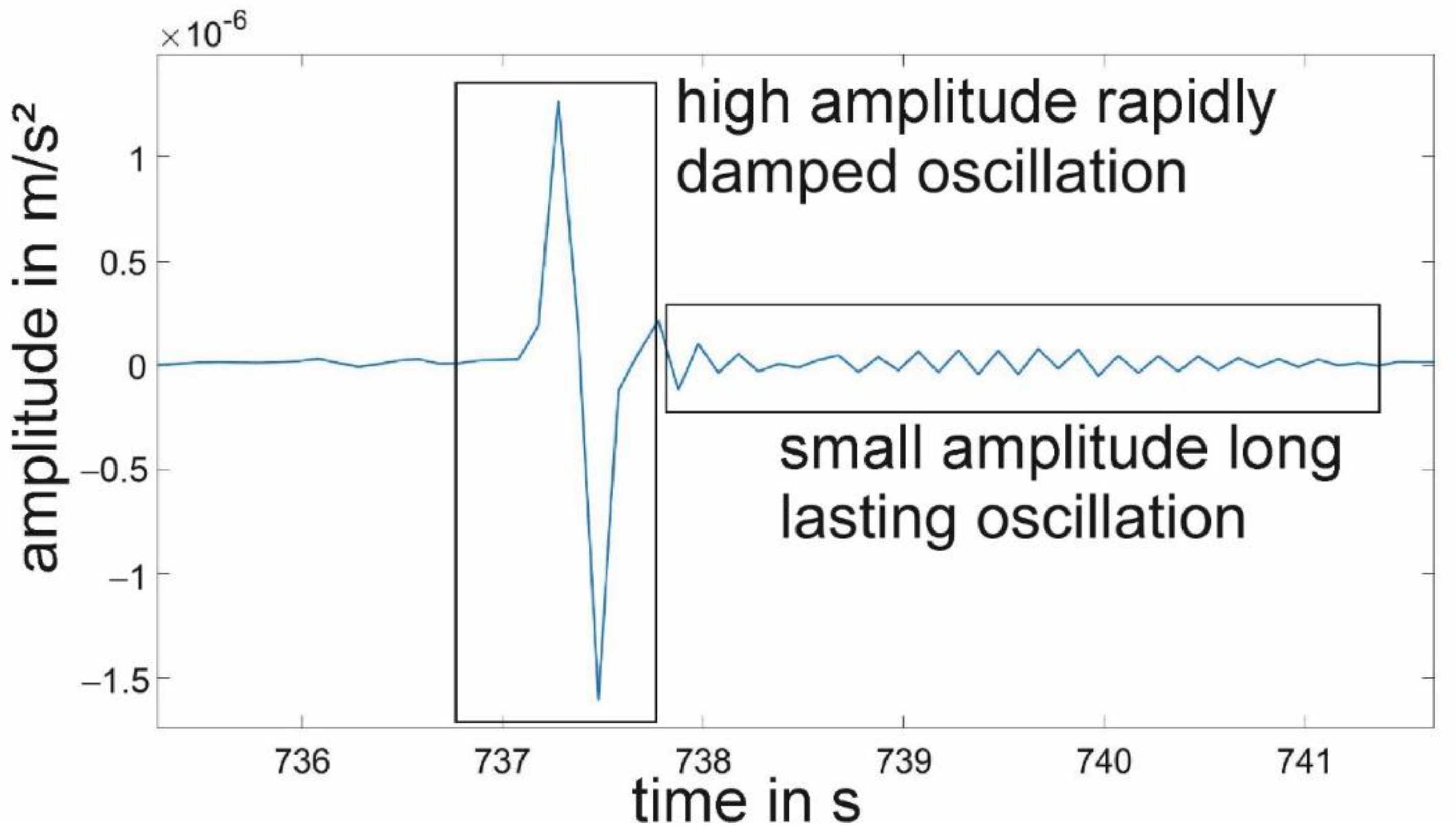
2.2. Twangs and Their Detection
3. Results
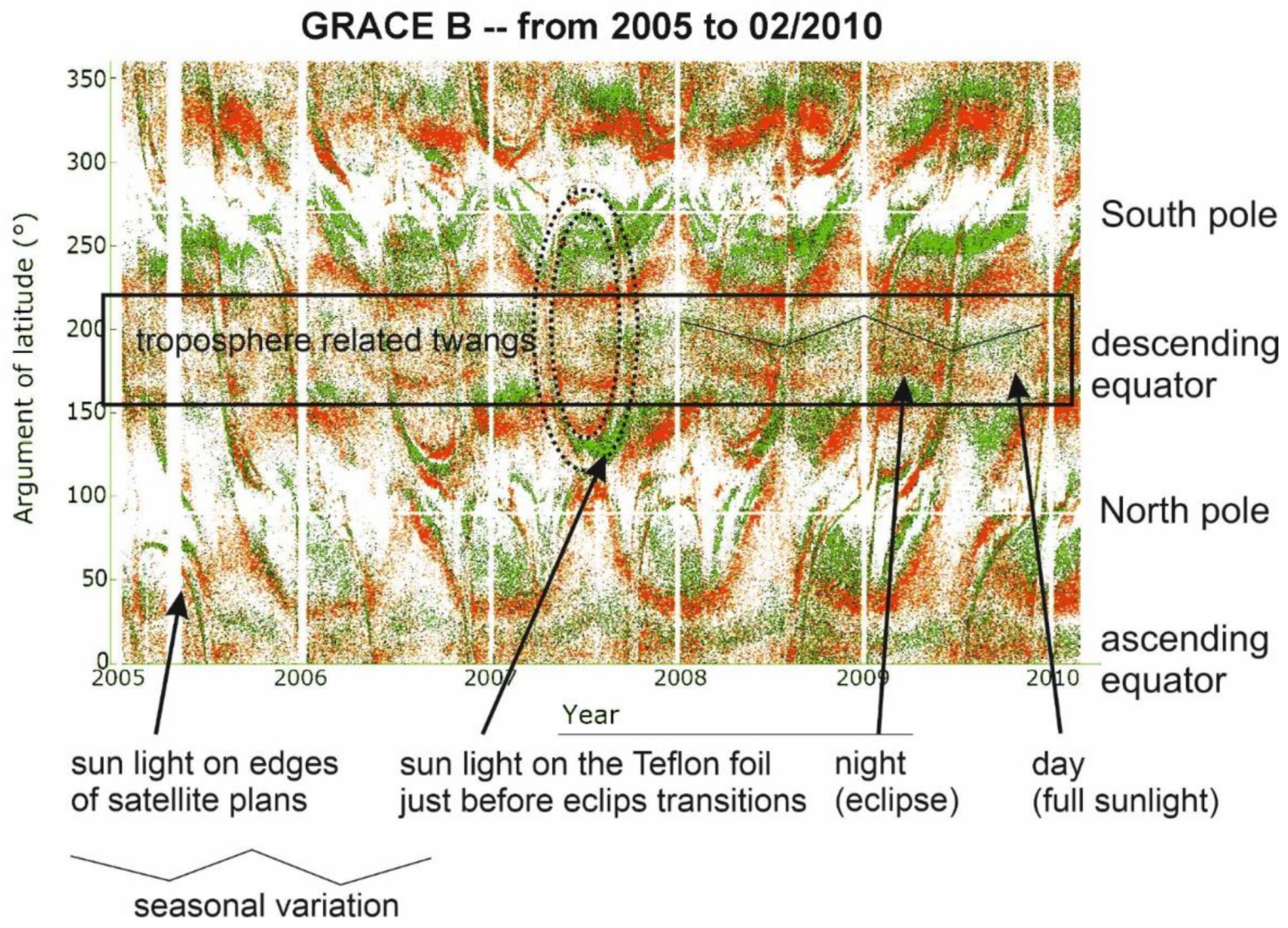
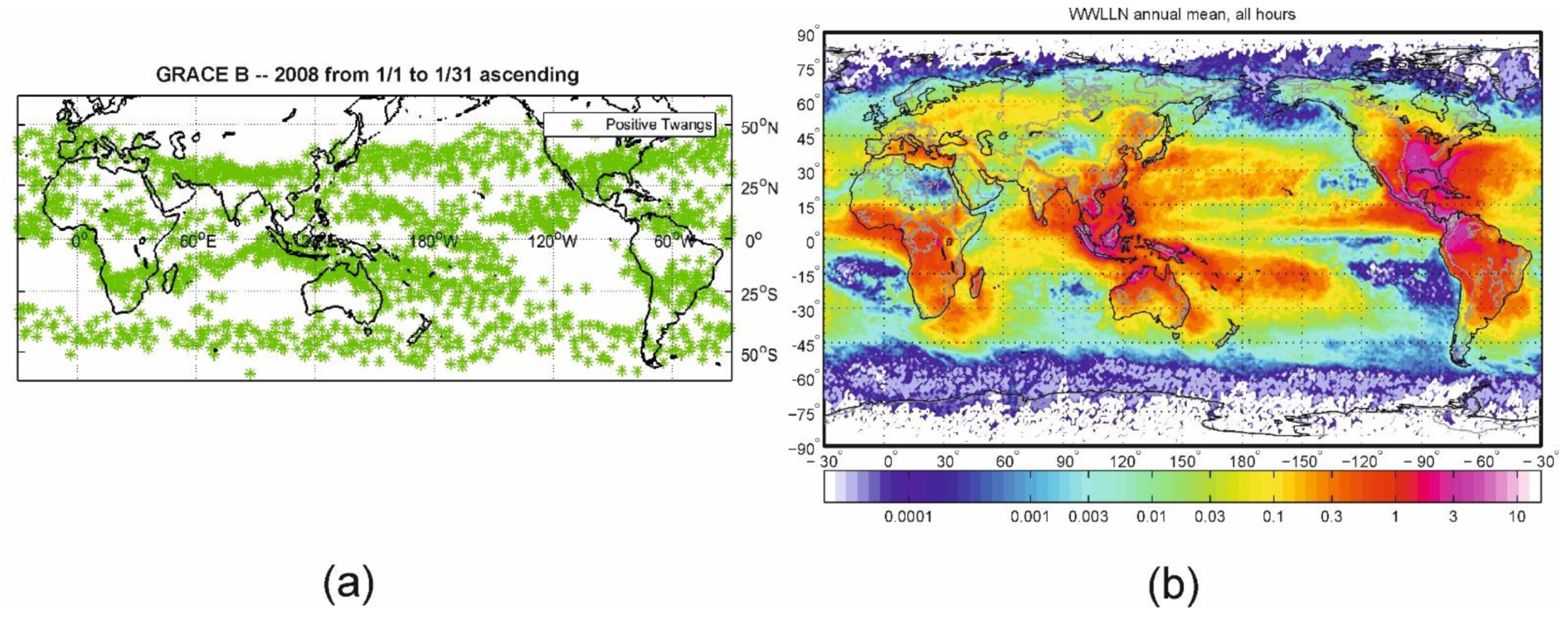
3.1. Troposphere and Ionosphere Coupling
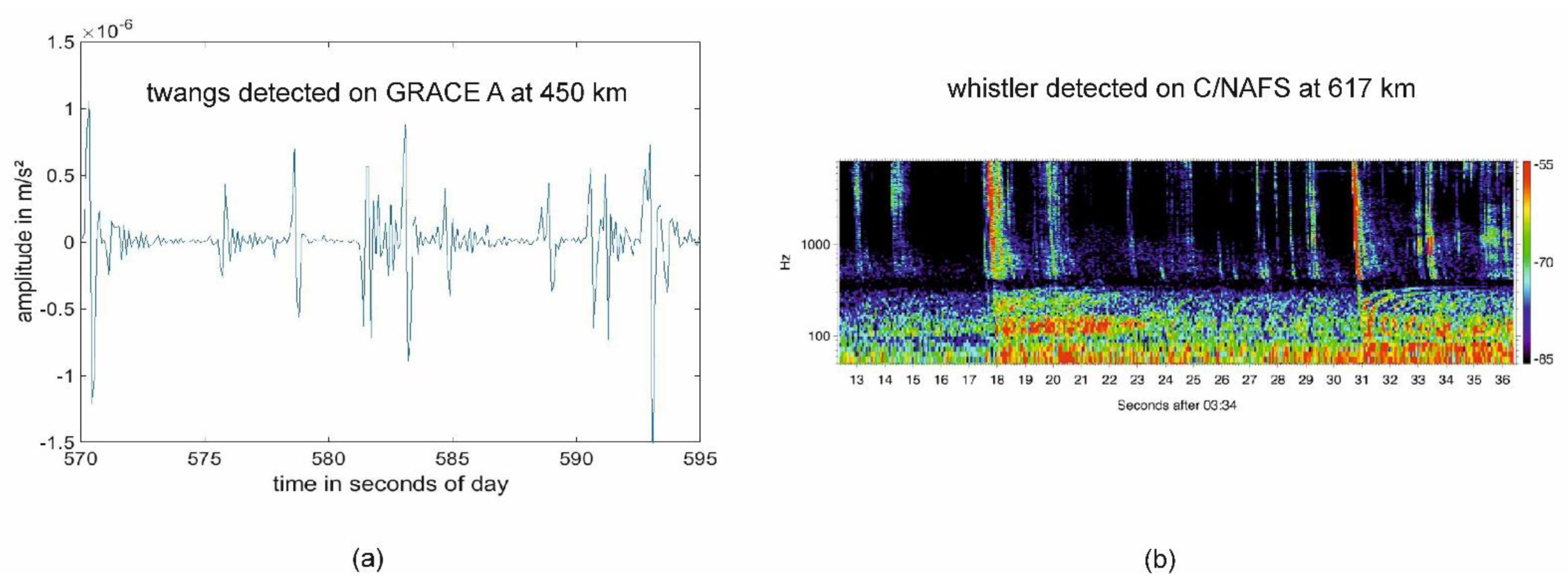
3.2. Comparison between Cloud-Related Twangs and Whistlers
3.3. Comparison between Cloud-Related Twangs and Lightning Strikes
4. Discussion
- Latitude variability: Whistlers that originate from lightning and low-latitude twangs occur between 60° north and south.
- Seasonal variability: The band shifts north in the northern summer.
- Bands predominant within 30–50° north and south: This is the region where the Earth magnetic field favors the coupling of the troposphere to the ionosphere. A structure related to the radial direction of the magnetic field was discussed in Peterseim et al [29].
- Clustering at the equator of low-latitude twangs in Indonesia, Africa, and Middle America with distinct gaps. At low latitude, where the magnetic field is not favorable for whistler formation, we expect a high geographic correlation with lightning.
- Day and night asymmetry: The damping of whistlers is less evident at nighttime, when the density of the ionosphere is lower, and more lightning strikes take place. We think that this is the reason for the local time variability of twangs.
- Repetition rate: the C/NOFS satellite detects whistlers with a comparable rate to twangs on GRACE B.
- The variability is the same.
- We know no other coupling mechanism between the troposphere and ionosphere, which is relevant.
- The accelerometers are sensitive in the VLF domain.
5. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bai, Y.; Li, Z.; Hu, M.; Liu, L.; Qu, S.; Tan, D.; Tu, H.; Wu, S.; Yin, H.; Li, H.; et al. Research and Development of Electrostatic Accelerometers for Space Science Missions at HUST. Sensors 2017, 17, 1943. [Google Scholar] [CrossRef] [PubMed]
- Touboul, P.; Foulon, B.; Christophe, B.; Marque, J.P. CHAMP, GRACE, GOCE instruments and beyond. In Geodesy for Planet Earth; Kenyon, S., Pacino, M., Marti, U., Eds.; Springer: Berlin, Germany, 2012; pp. 215–221. [Google Scholar]
- Friis-Christensen, E.; Lühr, H.; Hulot, G. Swarm: A constellation to study the Earth’s magnetic field. Earth Planets Space 2006, 58, 351–358. [Google Scholar] [CrossRef]
- Drinkwater, M.R.; Floberghagen, R.; Haagmans, R.; Muzi, D.; Popescu, A. GOCE: ESA’s first Earth Explorer Core mission. In Earth Gravity Field from Space—From Sensors to Earth Sciences; Space Sciences Series of ISSI; Beutler, G.B., Drinkwater, M.R., Rummel, R., von Steiger, R., Eds.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2003; Volume 18, pp. 419–432. ISBN 1-4020-1408-2. [Google Scholar]
- Hudson, D.; Chhun, R.; Touboul, P. Development status of the differential accelerometer for the MICROSCOPE mission. Adv. Space Res. 2007, 39, 307–314. [Google Scholar] [CrossRef]
- Dolesi, R.; Bortoluzzi, D.; Bosetti, P.; Carbone, L.; Cavalleri, A.; Cristofolini, I.; DaLio, M.; Fontana, G.; Fontanari, V.; Foulon, B.; et al. Gravitational sensor for LISA and its technology demonstration mission. Class. Quantum Gravity 2003, 20, 99–108. [Google Scholar] [CrossRef]
- Armano, M.; Audley, H.; Auger, G.; Baird, J.T.; Bassan, M.; Binetruy, P.; Born, M.; Bortoluzzi, D.; Brandt, N.; Caleno, M.; et al. Sub-Femto-g Free Fall for Space-Based Gravitational Wave Observatories: LISA Pathfinder Results. Phys. Rev. Lett. 2016, 116, 231101. [Google Scholar] [CrossRef]
- Gruenwaldt, L.; Meehan, T. CHAMP Orbit and Gravity Instrument Status. In First CHAMP Mission Results for Gravity, Magnetic and Atmospheric Studies; Reigber, C., Lühr, H., Schwintzer, P., Eds.; Springer: Berlin, Germany, 2003; pp. 3–10. [Google Scholar]
- Gruenwaldt, L.; Bock, R. Heater Switching Procedure and Test Results; Technical Report CH-GFZ-TR-2201; GFZ Potsdam: Potsdam, Germany, 2000. [Google Scholar]
- Flury, J.; Bettadpur, S.; Tapley, B. Precise accelerometry on board the GRACE gravity field satellite mission. Adv. Space Res. 2008, 42, 1414–1423. [Google Scholar] [CrossRef]
- Peterseim, N.; Flury, J.; Schlicht, A. Magnetic torquer induced disturbing signals within GRACE accelerometer data. Adv. Space Res. 2012, 49, 1388–1394. [Google Scholar] [CrossRef]
- Peterseim, N. TWANGS—High-Frequency Disturbing Signals in 10 Hz Accelerometer Data of the GRACE Satellites. Ph.D. Thesis, Verlag der Bayerischen Akademie der Wissenschaften, Munich, Germany, 2014. [Google Scholar]
- Bonin, G.R.; Orr, N.G.; Zee, R.E.; Cain, J. Solar Array Arcing Mitigation for Polar Low-Earth Orbit Spacecraft. In Proceedings of the 24th Annual AIAA/USU Conference on Small Satellites, Logah, UT, USA, 9–12 August 2010. [Google Scholar]
- Siemes, C.; de Teixeira da Encarnacuo, J.; Doornbos, E.; van den Ijssel, J.; Kraus, J.; Perestry, R.; Grunwaldt, L.; Apelbaum, G.; Flury, J.; Olsen, P.E.H. Swarm accelerometer data processing from raw accelerations to thermospheric neutral densities. Earth Planets Space 2016, 68, 92. [Google Scholar] [CrossRef]
- Behzadpour, S.; Mayer-Gürr, T.; Krauss, S. GRACE follow-on accelerometer data recovery. J. Geophys. Res. Solid Earth 2021, 126, e2020JB021297. [Google Scholar] [CrossRef]
- Tzamali, M.; Peidou, A.; Pagiatakis, S. GRACE-FO and Swarm integrated data analysis reveals ionospheric disturbances on the accelerometer meaurements. In Proceedings of the EGU General Assembly Conference, Online, 4–8 May 2020. EGU2020-12931. [Google Scholar]
- Frommknecht, B.; Oberndorfer, H.; Flechtner, F.; Schmidt, R. Integrated sensor analysis for GRACE – development and verfication. Adv. Geosci. 2003, 1, 57–63. [Google Scholar] [CrossRef]
- ECSS European Cooperation for Space Standardization, ECSS_E-ST-20-07C Space Engineering: Electromagnetic compatibility Rev 2, 3 January 2022. Available online: https://ecss.nl/standard/ecss-e-st-20-07c-rev-2-electromagnetic-compatibility-3-january-2022/ (accessed on 13 April 2022).
- Bettadpur, S. Brief Note on the ACC Transfer Function & Its Application to Simulated Drag Time Series; Note; Center for Space Research, University of Texas at Austin: Austin, TX, USA, 2000. [Google Scholar]
- Hudson, D. In-Flight Characterization and Calibration of the SuperSTAR Accelerometer. Master’s Thesis, The University of Texas at Austin, Austin, TX, USA, 2003. [Google Scholar]
- Simões, F.; Pfaff, R.; Berthelier, J.; Klenzing, J. A review of low frequency electromagnetic wave phenomena related to tropospheric-ionospheric coupling mechanisms. Space Sci. Rev. 2012, 168, 551–593. [Google Scholar] [CrossRef]
- Singh, A.K. Diagnostic features of VLF waves in terrestrial magnetosphere. Adv. Geosci. 2011, 27, 31–40. [Google Scholar]
- Barr, R.; Llanwayn Jones, D.; Roger, C.J. ELF and VLF radio waves. J. Atmos. Sol. Terr. Phys. 2000, 62, 1689–1718. [Google Scholar] [CrossRef]
- Dowden, R.L.; Brundell, J.B.; Rodger, C.J. VLF lightning location by time of group arrival (TOGA) at multiple sites. J. Atmos. Sol. Terr. Phys. 2002, 64, 817–830. [Google Scholar] [CrossRef]
- Hayakawa, M. Whistlers. In Handbook of Atmospheric Electrodynamics; Volland, H., Ed.; CRC Press: Boca Raton, FL, USA, 2002; ISBN 0-8493-2520-X (v. 2). [Google Scholar]
- Holzworth, R.H.; McCarthy, M.P.; Pfaff, R.F.; Jacobson, A.R.; Willcockson, W.L.; Rowland, D.E. Lightning-generated whistler waves observed by probes on the Communication/Navigation Outage Forecast System satellite at low latitudes. J. Geophys. Res. 2011, 116, A06306. [Google Scholar] [CrossRef][Green Version]
- Jacobson, A.R.; Holzworth, R.H.; Pfaff, R.F.; McCarthy, M.P. Study of oblique whistlers in the low-latitude ionosphere, jointly with the C/NOFS satellite and the World-Wide Lightning Location Network. Ann. Geophys. 2011, 29, 851–863. [Google Scholar] [CrossRef]
- Labelle, J.; Treumann, R.A. Auroral radio emissions, 1. Hiss, Roars, and Bursts. Space Sci. Rev. 2002, 101, 295–440. [Google Scholar] [CrossRef]
- Peterseim, N.; Schlicht, A.; Flury, J. Improved Acceleration Modelling and Level 1 Processing Alternative for GRACE. In GEOTECHNOLOGIEN Science Report; Münch, U., Dransch, W., Eds.; GEOTECHNOLOGIEN: Potsdam, Germany, 2010; Volume 17, pp. 31–34. [Google Scholar] [CrossRef]
- Virts, K.S.; Wallace, J.M.; Hutchius, M.L.; Holzworth, R.H. Highlights of a new ground-based, hourly global lightning climatology. Bull. Am. Meteo. Soc. 2013, 94, 1381. [Google Scholar] [CrossRef]
- Jacobson, A.R.; Holzworth, R.H.; Harlin, J.; Dowden, R.; Lay, E. Performance assessment of the World Wide Lightning Location Network (WWLLN), using the Los Alamos Sferic Array (LASA) array as ground-truth. J. Atmos. Ocean. Technol. 2006, 23, 1082–1092. [Google Scholar] [CrossRef]
- Lay, E.H.; Jacobson, A.R.; Holzworth, R.H.; Rodger, C.J.; Dowden, R.L. Local time variation in land/ocean lightning flash density as measured by the World Wide Lightning Location Network. J. Geophys. Res. 2007, 112, D13111. [Google Scholar] [CrossRef]
- Lay, E.H.; Holzworth, R.H.; Rodger, C.J.; Thomas, J.N.; Pinto, O.; Dowden, R.L. WWLLN global lightning detection system: Regional validation study in Brazil. Geophys. Res. Lett. 2004, 31, L03102. [Google Scholar] [CrossRef]
- Rodger, C.J.; Werner, S.; Brundell, J.B.; Lay, E.H.; Thomson, N.R.; Holzworth, R.H.; Dowden, R.L. Detection efficiency of the VLF World-Wide Lightning Location Network (WWLLN): Initial case study. Ann. Geophys. 2006, 24, 3197–3214. [Google Scholar] [CrossRef]
- Rodger, C.J.; Brundell, J.B.; Dowden, R.L. Location accuracy of VLF World-Wide Lightning Location (WWLL) network: Post-algorithm upgrade. Ann. Geophys. 2005, 23, 277–290. [Google Scholar] [CrossRef]
- Rodger, C.J.; Brundell, J.B.; Dowden, R.L.; Thomson, N.R. Location accuracy of long distance VLF lightning location network. Ann. Geophys. 2004, 22, 747–758. [Google Scholar] [CrossRef]
- Holzworth, R.H. (University of Washington, Washington, DC, USA). Personal communication, 2018.


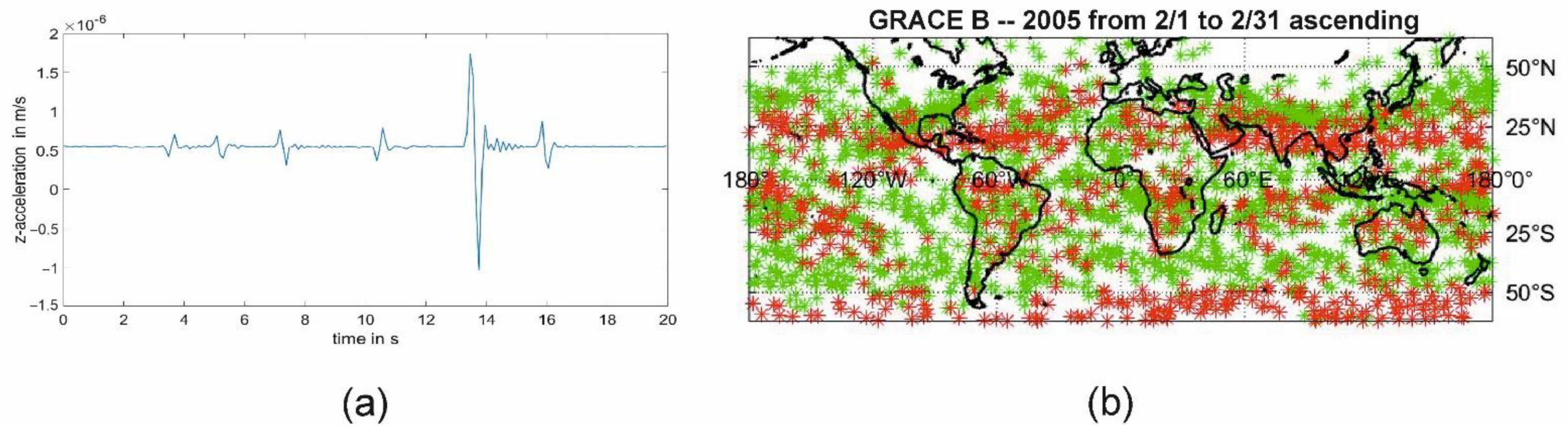

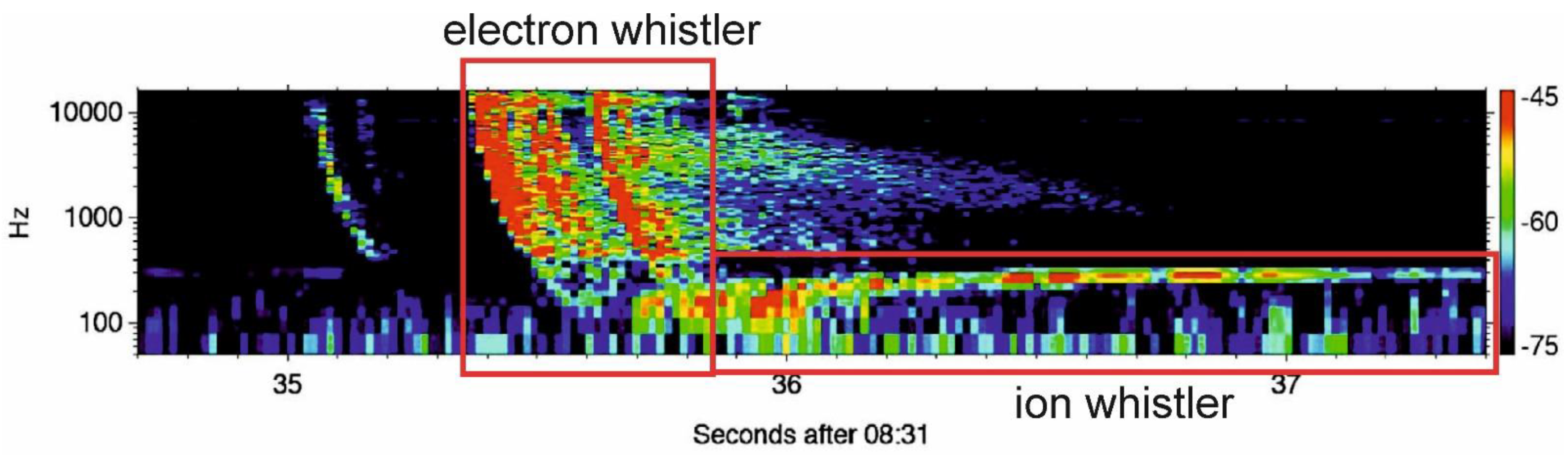
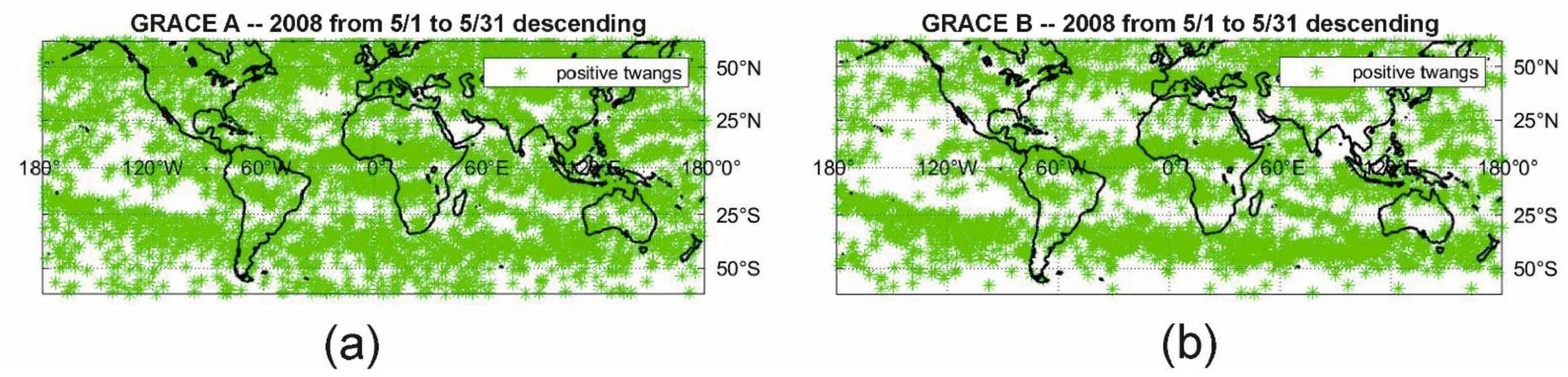
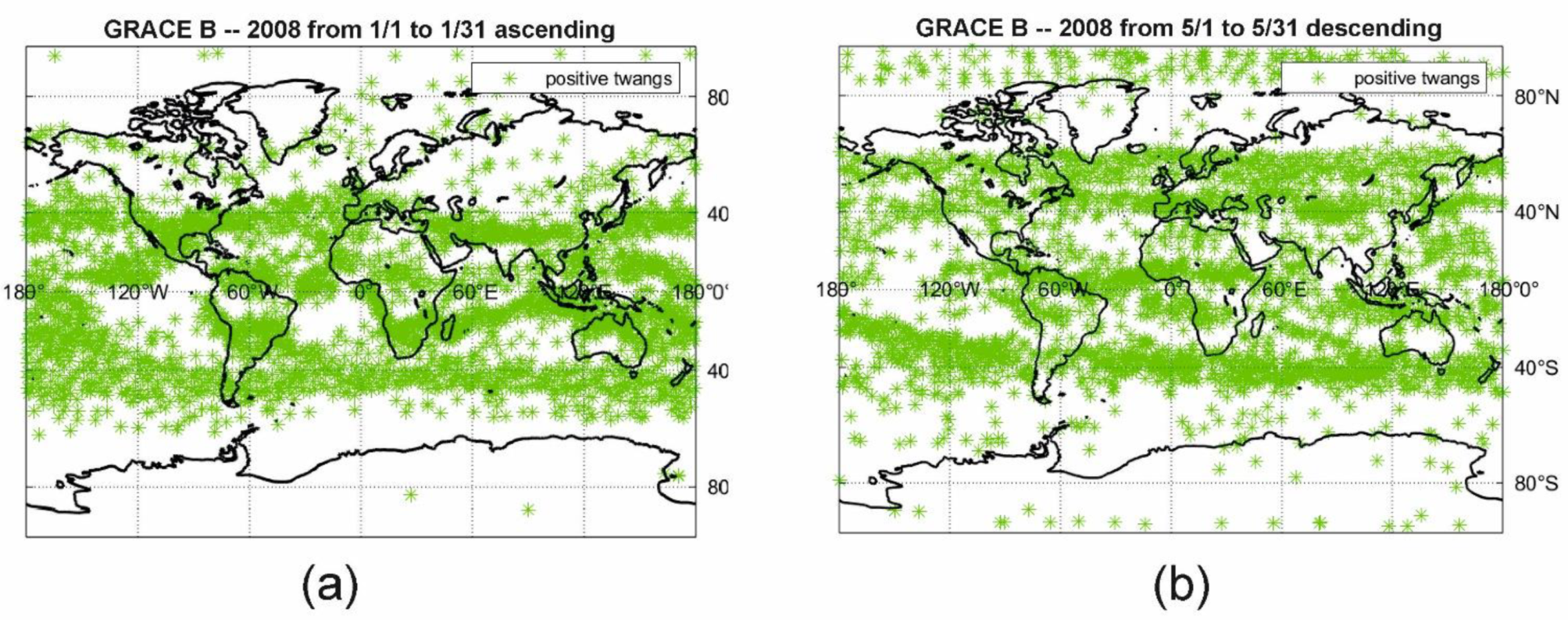


| Axis | Range in m/s2 | Accuracy in m/(s2√Hz) |
|---|---|---|
| x | ±5 × 10−5 | 1 × 10−10 |
| y | ±5 × 10−4 | 1 × 10−9 |
| z | ±5 × 10−5 | 1 × 10−10 |
| Category | Description |
|---|---|
| Shape | Two parts: Electron whistler Ion whistler |
| Day-night asymmetry | Absorption in E and D layers of ionosphere |
| Geography | Low and mid-latitudes, Shifting north or south with season |
| Correlations | Lightning Magnetic field |
| Time/Distance between Lightning and Twang | May 2008 (Total: 618 Positive Twangs) Number of Matches (Percentage) | June 2008 (Total: 922 Positive Twangs) Number of Matches (Percentage) | |||
|---|---|---|---|---|---|
| ±30 min | 30° | 616 | 100 | 912 | 99 |
| 20° | 612 | 99 | 898 | 97 | |
| 10° | 355 | 57 | 599 | 65 | |
| 5° | 337 | 55 | 558 | 61 | |
| 3° | 240 | 39 | 397 | 43 | |
| 1.5° | 125 | 20 | 201 | 22 | |
| −3 s | 30° | 276 | 61 | 512 | 56 |
| 20° | 246 | 40 | 360 | 39 | |
| 10° | 92 | 15 | 161 | 17 | |
| 5° | 38 | 6 | 62 | 7 | |
| 3° | 18 | 3 | 24 | 3 | |
| 1.5° | 8 | 1 | 7 | 0.8 | |
| −1 s | 30° | 207 | 33 | 296 | 32 |
| 20° | 118 | 19 | 184 | 20 | |
| 10° | 38 | 6 | 79 | 9 | |
| 5° | 13 | 2 | 31 | 3 | |
| 3° | 8 | 1 | 7 | 0.8 | |
| 1.5° | 4 | 0.6 | 3 | 0.3 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schlicht, A. GRACE Accelerometers Sensitive to Ionosphere Plasma Waves: Similarities between Twangs and Whistlers. Geosciences 2022, 12, 228. https://doi.org/10.3390/geosciences12060228
Schlicht A. GRACE Accelerometers Sensitive to Ionosphere Plasma Waves: Similarities between Twangs and Whistlers. Geosciences. 2022; 12(6):228. https://doi.org/10.3390/geosciences12060228
Chicago/Turabian StyleSchlicht, Anja. 2022. "GRACE Accelerometers Sensitive to Ionosphere Plasma Waves: Similarities between Twangs and Whistlers" Geosciences 12, no. 6: 228. https://doi.org/10.3390/geosciences12060228
APA StyleSchlicht, A. (2022). GRACE Accelerometers Sensitive to Ionosphere Plasma Waves: Similarities between Twangs and Whistlers. Geosciences, 12(6), 228. https://doi.org/10.3390/geosciences12060228





