On Two Formulations of Polar Motion and Identification of Its Sources
Abstract
1. Introduction
2. Two Formulations of the Liouville-Euler Equations
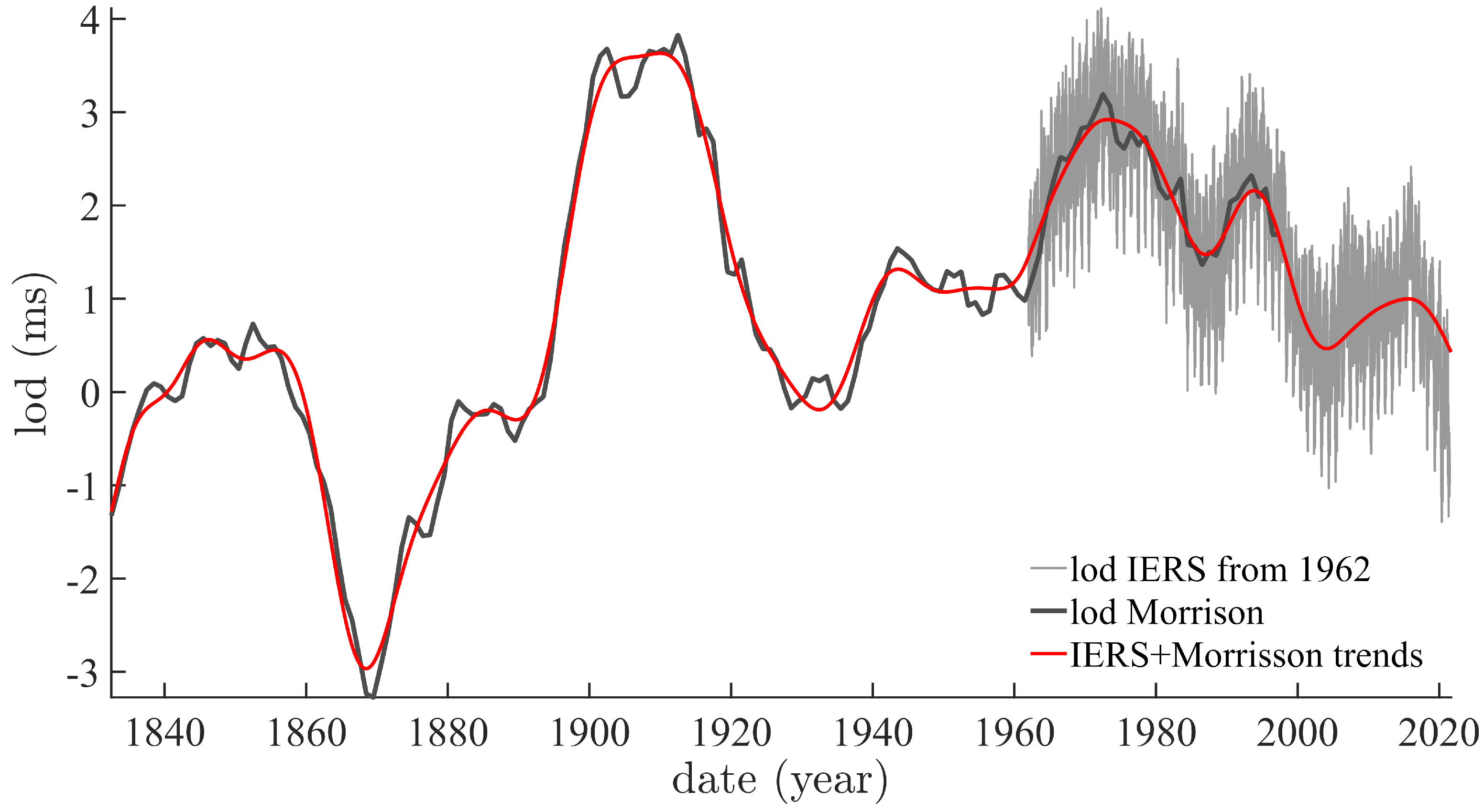
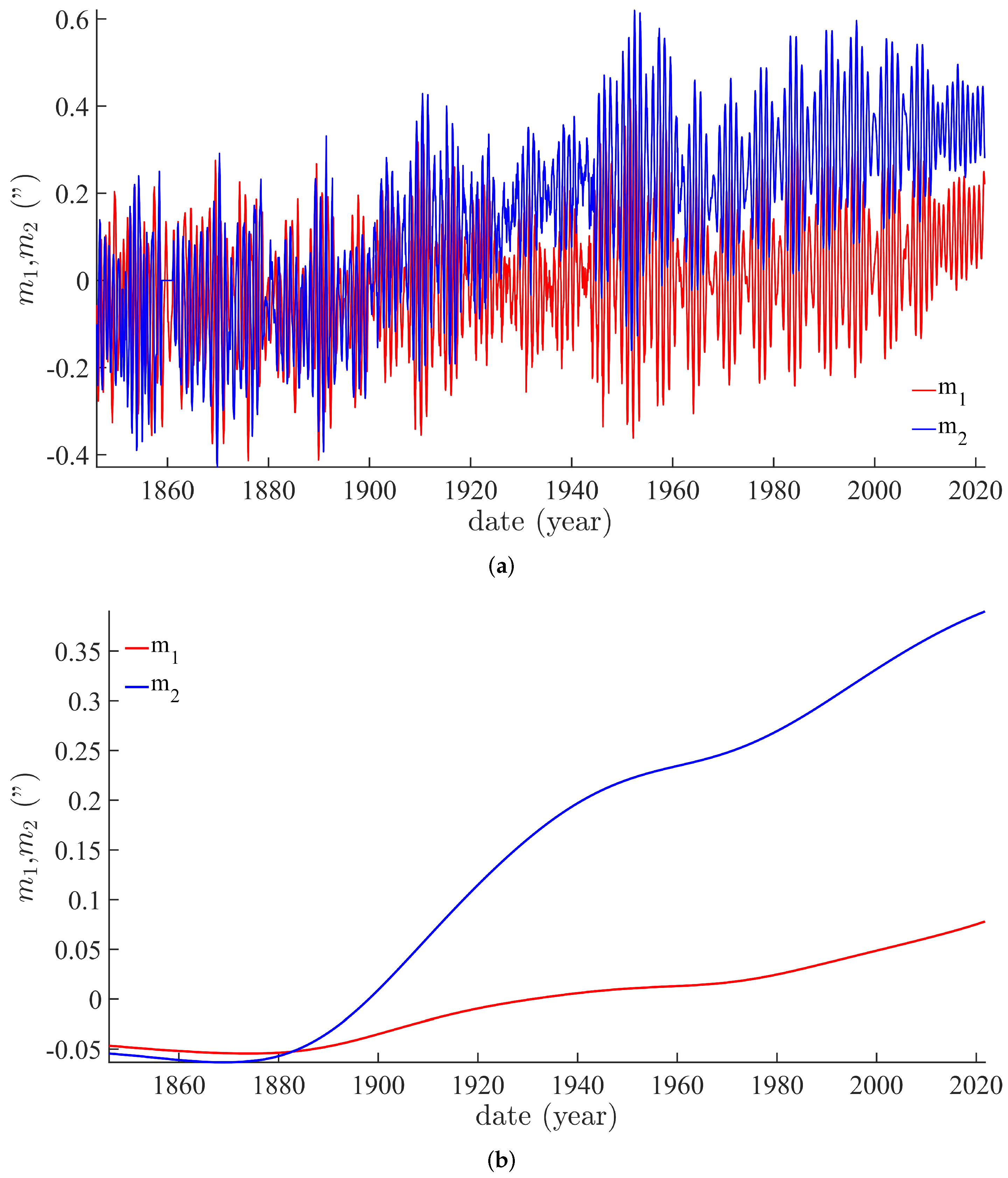
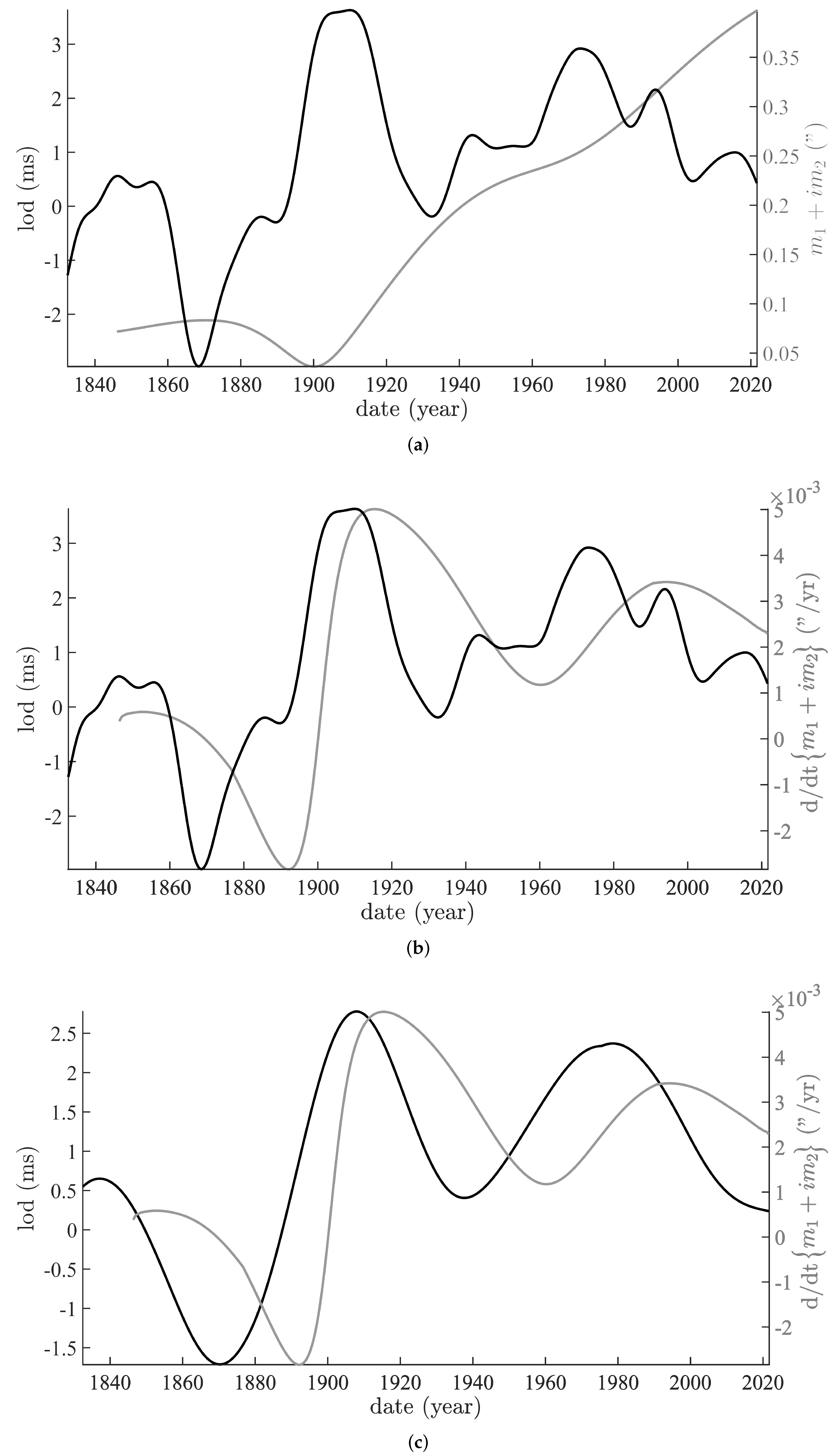
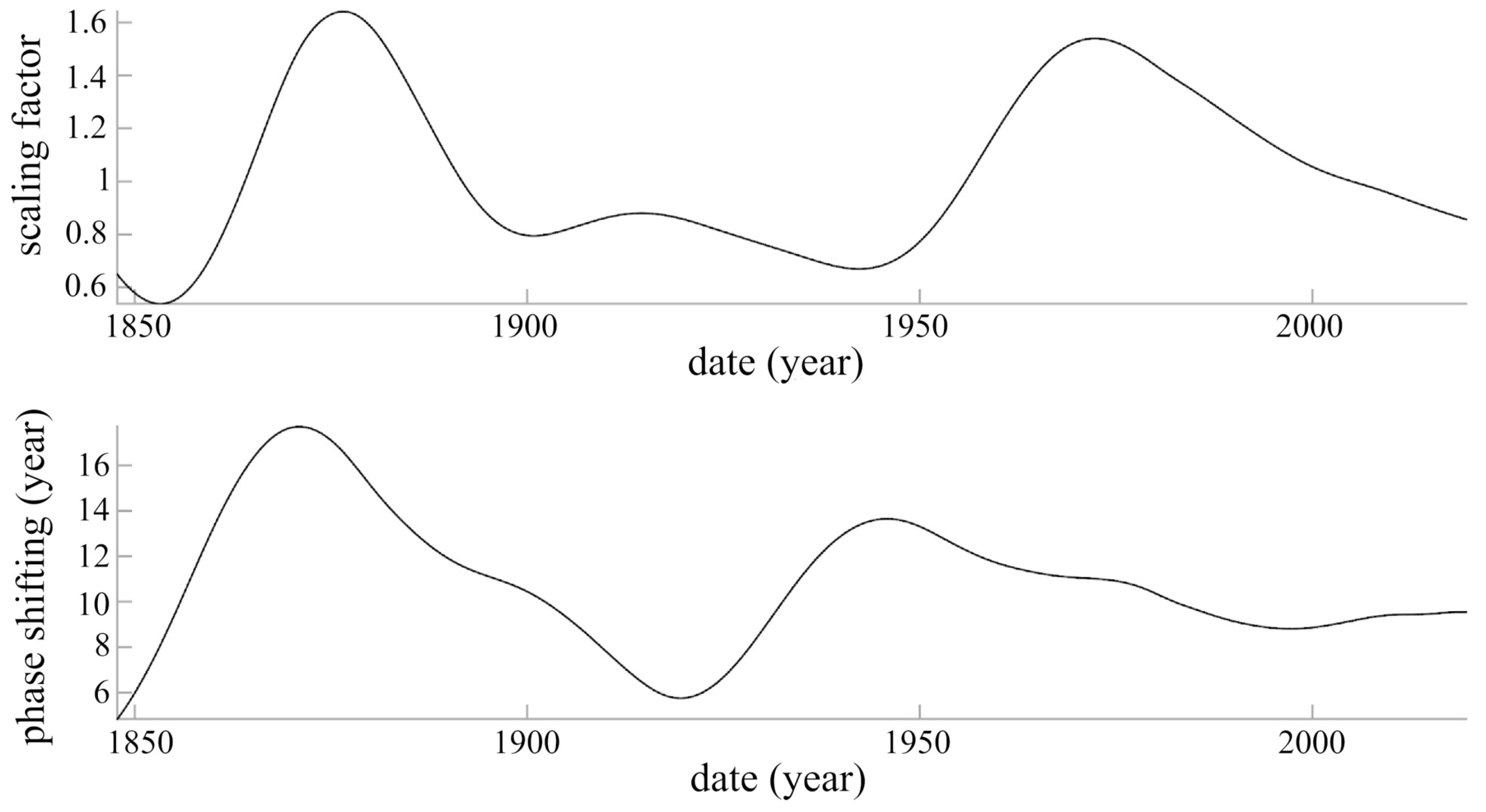
3. Confronting the Theory with the Observations
4. Discussion and Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Laplace, P.S. Traité de Mécanique Céleste; l’Imprimerie de Crapelet: Paris, France, 1799. [Google Scholar]
- Poincaré, H. Les Méthodes Nouvelles de la Mécanique Céleste; Gauthier-Villars: Paris, France, 1893. [Google Scholar]
- Lambeck, K. The Earth’s Variable Rotation: Geophysical Causes and Consequences; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Abreu, J.A.; Beer, J.; Ferriz-Mas, A.; McCracken, K.G.; Steinhilber, F. Is there a planetary influence on solar activity? Astron. Astrophys. 2012, 548, A88. [Google Scholar] [CrossRef]
- Scafetta, N. Solar oscillations and the orbital invariant inequalities of the solar system. Sol. Phys. 2020, 295, 33. [Google Scholar] [CrossRef]
- Courtillot, V.; Lopes, F.; Le Mouël, J.L. On the prediction of solar cycles. Sol. Phys. 2021, 296, 21. [Google Scholar] [CrossRef]
- Lopes, F.; Le Mouël, J.L.; Courtillot, V.; Gibert, D. On the shoulders of Laplace. Phys. Earth Planet. Inter. 2021, 316, 106693. [Google Scholar] [CrossRef]
- Le Mouël, J.L.; Lopes, F.; Courtillot, V. Identification of Gleissberg cycles and a rising trend in a 315-year-long series of sunspot numbers. Sol. Phys. 2017, 292, 43. [Google Scholar] [CrossRef]
- Le Mouël, J.L.; Lopes, F.; Courtillot, V. Sea-Level Change at the Brest (France) Tide Gauge and the Markowitz Component of Earth’s Rotation. J. Coast. Res. 2021, 37, 683–690. [Google Scholar] [CrossRef]
- Chen, W.; Shen, W. New estimates of the inertia tensor and rotation of the triaxial nonrigid Earth. J. Geophys. Res. Solid Earth 2010, 115, B12. [Google Scholar] [CrossRef]
- Peltier, W.R.; Andrews, J.T. Glacial-isostatic adjustment—I. The forward problem. Geophys. J. Int. 1976, 46, 605–646. [Google Scholar] [CrossRef]
- Nakiboglu, S.M.; Lambeck, K. Deglaciation effects on the rotation of the Earth. Geophys. J. Int. 1980, 62, 49–58. [Google Scholar] [CrossRef]
- Melchior, P. The Earth Tides; Pergamon Press: Oxford, UK, 1966; 458p. [Google Scholar]
- Ray, R.D.; Erofeeva, S.Y. Long-period tidal variations in the length of day. J. Geophys. Res. Solid Earth 2014, 119, 1498–1509. [Google Scholar] [CrossRef]
- Wahr, J.M.; Sasao, T.; Smith, M.L. Effect of the fluid core on changes in the length of day due to long period tides. Geophys. J. Int. 1981, 64, 635–650. [Google Scholar] [CrossRef]
- Le Mouël, J.L.; Lopes, F.; Courtillot, V.; Gibert, D. On forcings of length of day changes: From 9-day to 18.6-year oscillations. Phys. Earth Planet. Inter. 2019, 292, 1–11. [Google Scholar] [CrossRef]
- Lagrange, J.L. Mécanique Analytique; Mallet-Bachelier: Paris, France, 1853. [Google Scholar]
- Milanković, M. Théorie Mathématique des Phénomènes Thermiques Produits par la Radiation Solaire; Gauthier-Villars: Paris, France, 1920. [Google Scholar]
- Laskar, J.; Robutel, P.; Joutel, F.; Gastineau, M.; Correia, A.C.M.; Levrard, B. A long-term numerical solution for the insolation quantities of the Earth. Astron. Astrophys. 2004, 428, 261–285. [Google Scholar] [CrossRef]
- Laskar, J.; Fienga, A.; Gastineau, M.; Manche, H. La2010: A new orbital solution for the long-term motion of the Earth. Astron. Astrophys. 2011, 532, A89. [Google Scholar] [CrossRef]
- Guinot, B. Variation du pôle et de la vitesse de rotation de la Terre, ch. 19. In Traité de Géophysique Interne; Coulomb, J., Jobert, G., Eds.; Masson: Paris, France, 1976; Volume 1, pp. 529–564. [Google Scholar]
- Stephenson, F.R.; Morrison, L.V. Long-term changes in the rotation of the Earth: 700 BC to AD 1980. Philos. Trans. Royal Soc. A 1984, 313, 47–70. [Google Scholar] [CrossRef]
- Gross, R.S. A combined length-of-day series spanning 1832–1997: LUNAR97. Phys. Earth Planet. Inter. 2001, 123, 65–76. [Google Scholar] [CrossRef]
- Golyandina, N.; Zhigljavsky, A. Singular Spectrum Analysis for Time Series; Springer: Berlin/Heidelberg, Germany, 2013; Volume 120, ISBN 978-3642349126. [Google Scholar]
- Lemmerling, P.; Van Huffel, S. Analysis of the structured total least squares problem for hankel/toeplitz matrices. Numer. Algorithms 2001, 27, 89–114. [Google Scholar] [CrossRef]
- Golub, G.H.; Reinsch, C. Singular value decomposition and least squares solutions. In Linear Algebra; Springer: Berlin/Heidelberg, Germany, 1971; pp. 134–151. [Google Scholar]
- Lopes, F.; Courtillot, V.; Le Mouël, J.-L. Triskeles and Symmetries of Mean Global Sea-Level Pressure. Atmosphere 2022, 13, 1354. [Google Scholar] [CrossRef]
- Markowitz, W. Concurrent astronomical observations for studying continental drift, polar motion, and the rotation of the Earth. In Symposium-International Astronomical Union; Cambridge University Press: Cambridge, UK, 1968; Volume 32, pp. 25–32. [Google Scholar]
- Stoyko, A. Mouvement seculaire du pole et la variation des latitudes des stations du SIL. In Symposium-International Astronomical Union; Cambridge University Press: Cambridge, UK, 1968; Volume 32, pp. 52–56. [Google Scholar]
- Hulot, G.; Le Huy, M.; Le Mouël, J.L. Influence of core flows on the decade variations of the polar motion. Geophys. Astrophys. Fluid Dyn. 1996, 82, 35–67. [Google Scholar] [CrossRef]
- Gibert, D.; Holschneider, M.; Le Mouël, J.L. Wavelet analysis of the Chandler wobble. J. Geophys. Res. Solid Earth 1998, 103, 27069–27089. [Google Scholar] [CrossRef]
- Zotov, L.; Bizouard, C. On modulations of the Chandler wobble excitation. J. Geodyn. 2012, 62, 30–34. [Google Scholar] [CrossRef]
- Kirkpatrick, S.; Gelatt, C.D., Jr.; Vecchi, M.P. Optimization by simulated annealing. Science 1983, 220, 671–680. [Google Scholar] [CrossRef]
- Le Mouël, J.L.; Lopes, F.; Courtillot, V. Solar turbulence from sunspot records. Mon. Not. R. Astron. Soc. 2020, 492, 1416–1420. [Google Scholar] [CrossRef]
- Bank, M.J.; Scafetta, N. Scaling, mirror symmetries and musical consonances among the distances of the planets of the solar system. arXiv 2022, arXiv:2202.03939. [Google Scholar] [CrossRef]
- Dickman, S.R. A complete spherical harmonic approach to luni-solar tides. Geophys. J. Int. 1989, 99, 457–468. [Google Scholar] [CrossRef][Green Version]
- Chao, B.F.; Merriam, J.B.; Tamura, Y. Geophysical analysis of zonal tidal signals in length of day. Geophys. J. Int. 1995, 122, 765–775. [Google Scholar] [CrossRef]
- Varga, P.; Gambis, D.; Bizouard, C.; Bus, Z.; Kiszely, M. Tidal influence through LOD variations on the temporal distribution of earthquake occurrences. In Proceedings of the Journées 2005 “Systèmes de Référence Spatio-temporels”: Earth Dynamics and Reference Systems: Five Years after the Adoption of the IAU 2000 Resolutions, Held at Space Research Centre PAS, Warsaw, Poland, 19–21 September 2005. [Google Scholar]
- Chandler, S.C. On the variation of latitude, I. Astron. J. 1891, 11, 59–61. [Google Scholar] [CrossRef]
- Chandler, S.C. On the variation of latitude, II. Astron. J. 1891, 11, 65–70. [Google Scholar] [CrossRef]
- Lopes, F.; Le Mouël, J.L.; Gibert, D. The mantle rotation pole position. A solar component. Comptes Rendus Geosci. 2017, 349, 159–164. [Google Scholar] [CrossRef]
- Mansinha, L.; Smylie, D.E. Effect of earthquakes on the Chandler wobble and the secular polar shift. J. Geophys. Res. Atmos. 1967, 72, 4731–4743. [Google Scholar] [CrossRef]
- Dahlen, F.A. The excitation of the Chandler wobble by earthquakes. Geophys. J. Int. 1971, 25, 157–206. [Google Scholar] [CrossRef]
- O’Connell, R.J.; Dziewonski, A.M. Excitation of the Chandler wobble by large earthquakes. Nature 1976, 262, 259–262. [Google Scholar] [CrossRef]
- Gross, R.S. The influence of earthquakes on the Chandler wobble during 1977–1983. Geophys. J. Int. 1986, 85, 161–177. [Google Scholar] [CrossRef]
- Rochester, M.G. Causes of fluctuations in the rotation of the Earth. Philos. Trans. R. Soc. A 1984, 313, 95–105. [Google Scholar] [CrossRef]
- Gross, R.S. The excitation of the Chandler wobble. Geophys. Res. Lett. 2000, 27, 2329–2332. [Google Scholar] [CrossRef]
- Aoyama, Y.; Naito, I. Atmospheric excitation of the Chandler wobble, 1983–1998. J. Geophys. Res. Solid Earth 2001, 106, 8941–8954. [Google Scholar] [CrossRef]
- Brzeziński, A.; Nastula, J. Oceanic excitation of the Chandler wobble. Adv. Space Res. 2002, 30, 195–200. [Google Scholar] [CrossRef]
- Desai, S.D. Observing the pole tide with satellite altimetry. J. Geophys. Res. Oceans 2002, 107, 7-1–7-13. [Google Scholar] [CrossRef]
- Gross, R.S.; Fukumori, I.; Menemenlis, D.; Gegout, P. Atmospheric and oceanic excitation of length-of-day variations during 1980–2000. J. Geophys. Res. Solid Earth 2004, 109, B1. [Google Scholar] [CrossRef]
- Landerer, F.W.; Jungclaus, J.H.; Marotzke, J. Ocean bottom pressure changes lead to a decreasing length-of-day in a warming climate. Geophys. Res. Lett. 2007, 34. [Google Scholar] [CrossRef]
- Chen, J.; Wilson, C.R.; Kuang, W.; Chao, B.F. Interannual oscillations in earth rotation. J. Geophys. Res. Solid Earth 2019, 124, 13404–13414. [Google Scholar] [CrossRef]
- Afroosa, M.; Rohith, B.; Paul, A.; Durand, F.; Bourdallé-Badie, R.; Sreedevi, P.V.; Shenoi, S.S.C. Madden-Julian oscillation winds excite an intraseasonal see-saw of ocean mass that affects Earth’s polar motion. Commun. Earth Environ. 2001, 2, 139. [Google Scholar] [CrossRef]
- Trofimov, D.A.; Petrov, S.D.; Movsesyan, P.V.; Zheltova, K.V.; Kiyaev, V.I. Recent acceleration of the Earth rotation in the summer of 2020: Possible causes and effects. J. Phys. Conf. Ser. 2021, 2103, 012039. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lopes, F.; Courtillot, V.; Gibert, D.; Mouël, J.-L.L. On Two Formulations of Polar Motion and Identification of Its Sources. Geosciences 2022, 12, 398. https://doi.org/10.3390/geosciences12110398
Lopes F, Courtillot V, Gibert D, Mouël J-LL. On Two Formulations of Polar Motion and Identification of Its Sources. Geosciences. 2022; 12(11):398. https://doi.org/10.3390/geosciences12110398
Chicago/Turabian StyleLopes, Fernando, Vincent Courtillot, Dominique Gibert, and Jean-Louis Le Mouël. 2022. "On Two Formulations of Polar Motion and Identification of Its Sources" Geosciences 12, no. 11: 398. https://doi.org/10.3390/geosciences12110398
APA StyleLopes, F., Courtillot, V., Gibert, D., & Mouël, J.-L. L. (2022). On Two Formulations of Polar Motion and Identification of Its Sources. Geosciences, 12(11), 398. https://doi.org/10.3390/geosciences12110398






