Two-Dimensional Attenuation and Velocity Tomography of Iran
Abstract
1. Introduction

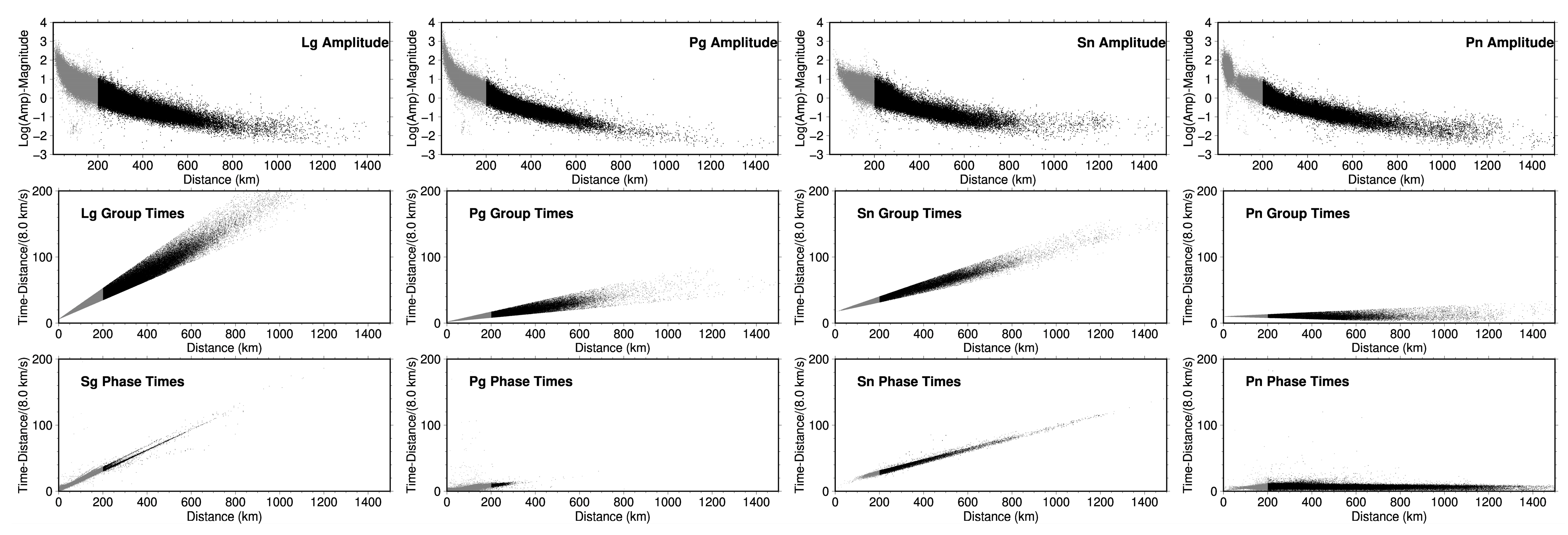
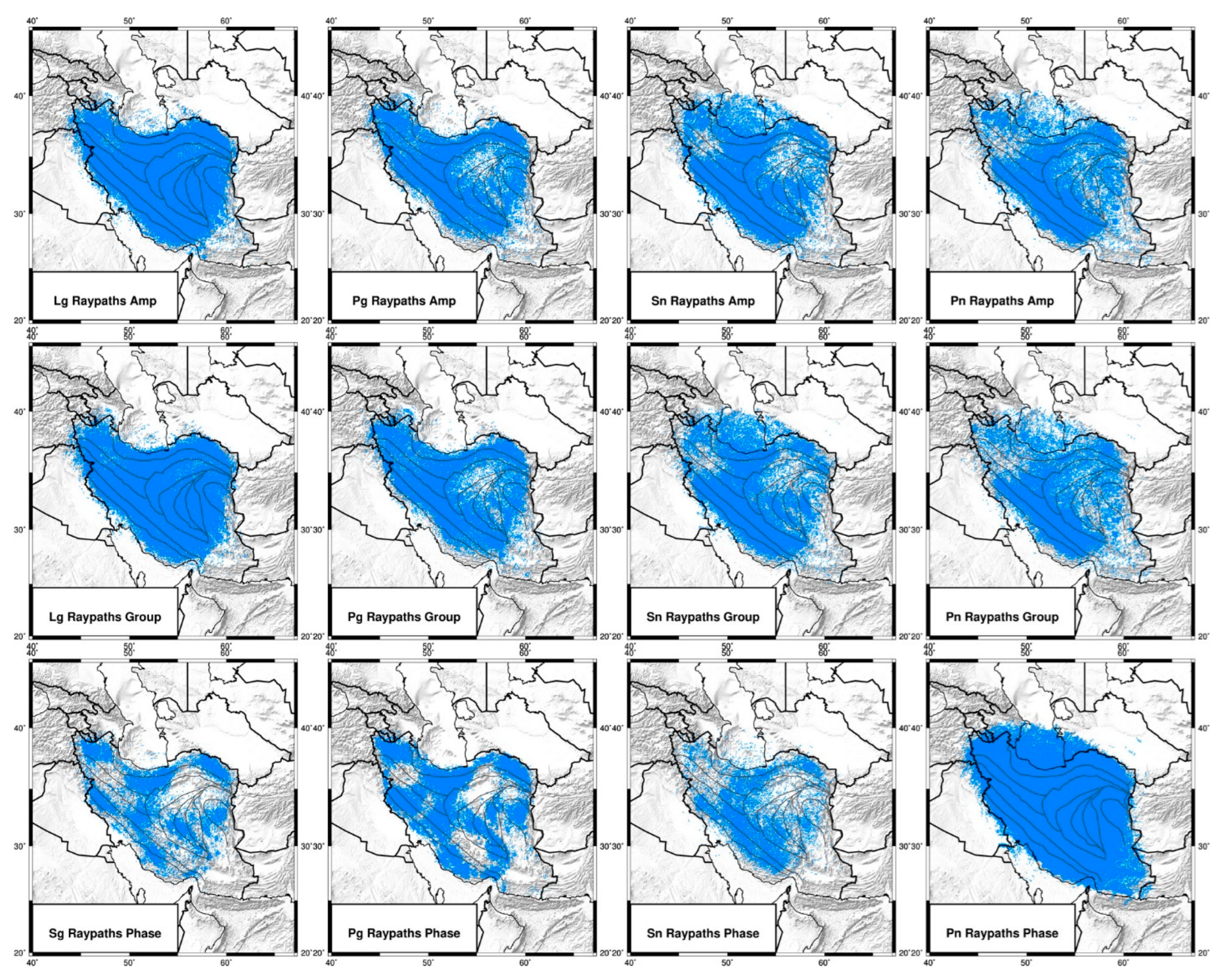
2. Data
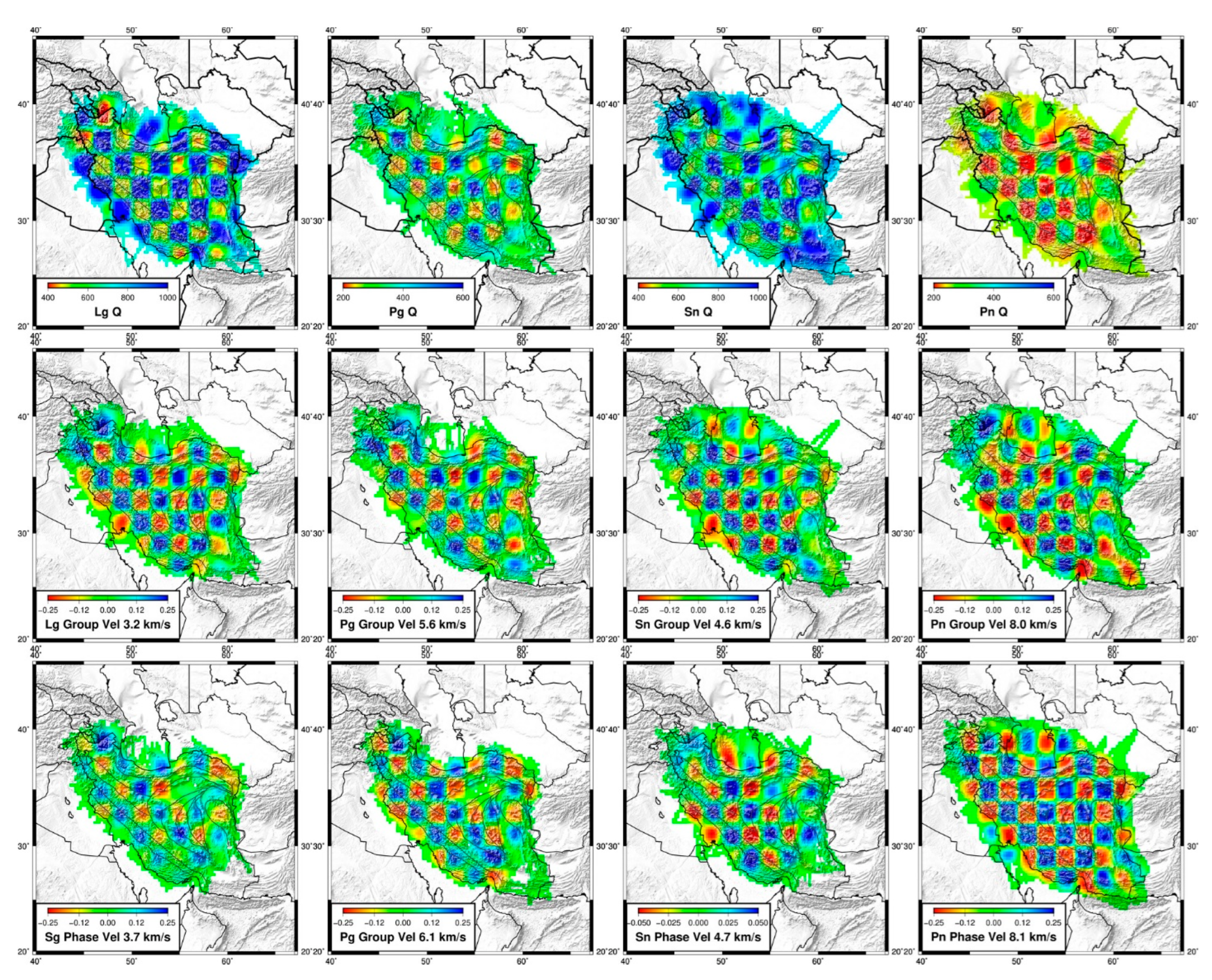
3. Method and Initial Results
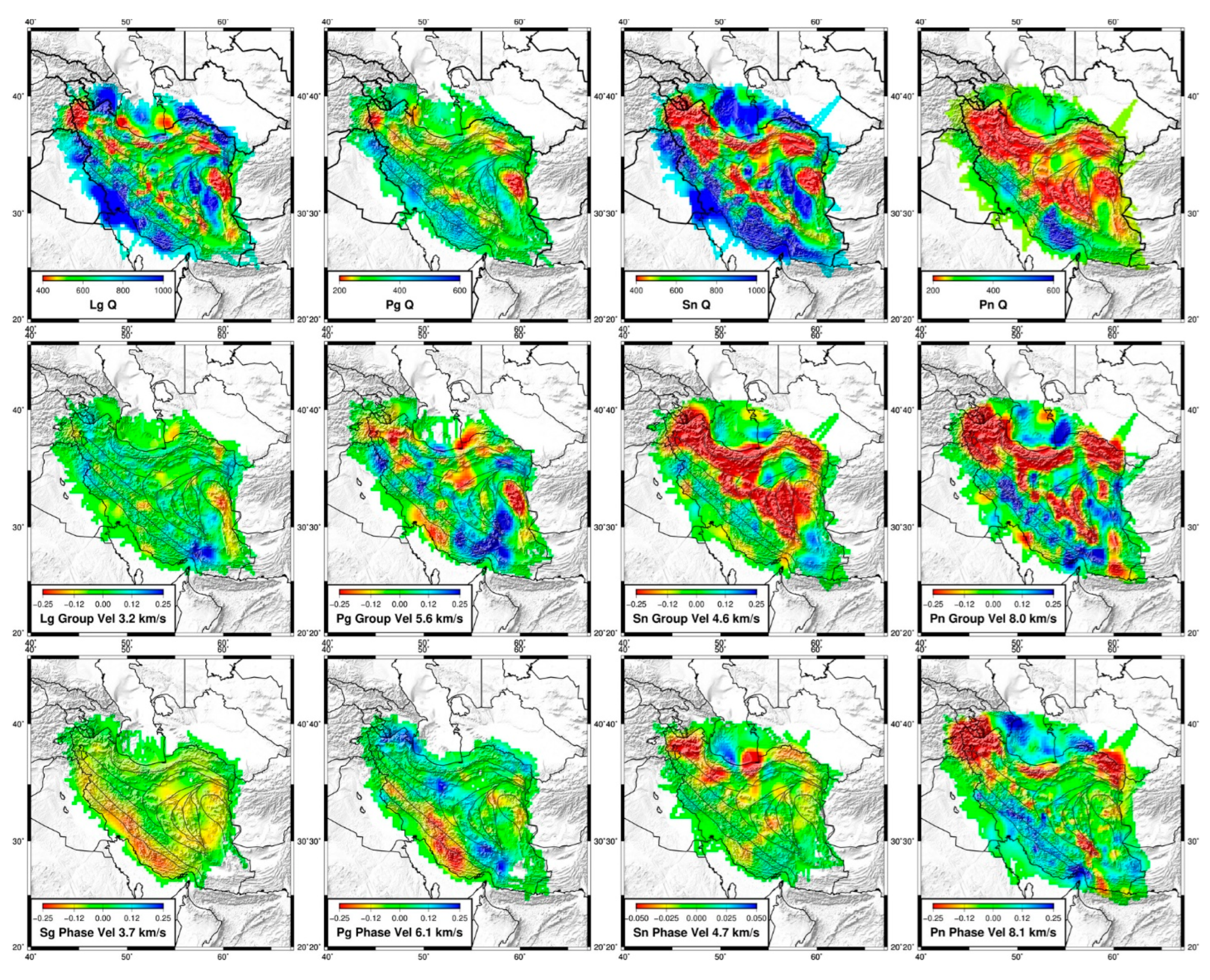
4. Results
4.1. Lg
4.2. Pg
4.3. Sn
4.4. Pn
5. Geophysical Interpretation
6. Geological Interpretation
7. Conclusions
Supplementary Materials
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Pei, S. ML Amplitude Tomography in North China. Bull. Seism. Soc. Am. 2006, 96, 1560–1566. [Google Scholar] [CrossRef]
- Hearn, T.M.; Wang, S.; Pei, S.; Xu, Z.; Ni, J.F.; Yu, Y. Seismic amplitude tomography for crustal attenuation beneath China. Geophys. J. Int. 2008, 174, 223–234. [Google Scholar] [CrossRef]
- Hearn, T.M. Crustal attenuation from USArray ML amplitude tomography. Geophys. J. Int. 2021, 224, 199–206. [Google Scholar] [CrossRef]
- Allen, M.B.; Jackson, J.; Walker, R. Late Cenozoic reorganization of the Arabia-Eurasia collision and the comparison of short-term and long-term deformation rates. Tectonics 2004, 23, 1–16. [Google Scholar] [CrossRef]
- Agard, P.; Omrani, J.; Jolivet, L.; Whitechurch, H.; Vrielynck, B.; Spakman, W.; Monié, P.; Meyer, B.; Wortel, R. Zagros orogeny: A subduction-dominated process. Geol. Mag. 2011, 148, 692–725. [Google Scholar] [CrossRef]
- Keskin, M. Magma generation by slab steepening and breakoff beneath a subduction-accretion complex: An alternative model for collision-related volcanism in Eastern Anatolia, Turkey. Geophys. Res. Lett. 2003, 30. [Google Scholar] [CrossRef]
- Barazangi, M.; Sandvol, E.; Seber, D. Structure and tectonic evolution of the Anatolian plateau in eastern Turkey. In Postcollisional Tectonics and Magmatism in the Mediterranean Region and Asia; Special Paper of the Geological Society of America; Geological Society of America: Boulder, CO, USA, 2006. [Google Scholar] [CrossRef]
- Hafkenscheid, E.; Wortel, M.J.R.; Spakman, W. Subduction history of the Tethyan region derived from seismic tomography and tectonic reconstructions. J. Geophys. Res. Earth Surf. 2006, 111. [Google Scholar] [CrossRef]
- Neill, I.; Meliksetian, K.; Allen, M.B.; Navasardyan, G.; Kuiper, K. Petrogenesis of mafic collision zone magmatism: The Armenian sector of the Turkish–Iranian Plateau. Chem. Geol. 2015, 403, 24–41. [Google Scholar] [CrossRef]
- Berberian, M.; King, G.C.P. Towards a paleogeography and tectonic evolution of Iran. Can. J. Earth Sci. 1980, 18, 210–265. [Google Scholar] [CrossRef]
- Nouri, F.; Azizi, H.; Asahara, Y.; Stern, R.J. A new perspective on Cenozoic calc-alkaline and shoshonitic volcanic rocks, eastern Saveh (central Iran). Int. Geol. Rev. 2021, 63, 476–503. [Google Scholar] [CrossRef]
- Tirrul, R.; Bell, I.R.; Griffis, R.J.; Camp, V.E. The Sistan suture zone of eastern Iran. GSA Bull. 1983, 94, 134. [Google Scholar] [CrossRef]
- Mokhtari, M.; Farahbod, A.M.; Lindholm, C.; Alahyarkhani, M.; Bungum, H. An Approach to a Comprehensive Moho Depth Map and Crust and Ipper Mantle Velocity Model for Iran. Iran. Int. J. Sci. 2004, 5, 223–244. [Google Scholar]
- Manaman, N.S.; Shomali, H.; Koyi, H. New constraints on upper-mantle S-velocity structure and crustal thickness of the Iranian plateau using partitioned waveform inversion. Geophys. J. Int. 2011, 184, 247–267. [Google Scholar] [CrossRef]
- Snyder, D.B.; Barazangi, M. Deep Crustal Structure and Flexure of the Arabian Plate beneath the Zagros Conllisional Mountain Belt as Inferred from Gravity Observations. Tectonics 1986, 5, 361–373. [Google Scholar] [CrossRef]
- Taghizadeh-Farahmand, F.; Afsari, N.; Sodoudi, F. Crustal Thickness of Iran Inferred from Converted Waves. Pure Appl. Geophys. 2014, 172, 309–331. [Google Scholar] [CrossRef]
- Ashena, Z.B.; Ardestani, V.E.; Camacho, A.G.; Dehghani, A.; Fernández, J. Moho depth determination beneath the Zagros Mountains from 3D inversion of gravity data. Arab. J. Geosci. 2018, 11, 52. [Google Scholar] [CrossRef]
- Shiranzaei, G.; Nasrabadi, A.; Sepahvand, M. Moho depth variations and Vp/Vs ratio in the Zagros (Iran) from teleseismic converted waves. J. Seism. 2021, 25, 671–682. [Google Scholar] [CrossRef]
- Paul, A.; Kaviani, A.; Hatzfeld, D.; Vergne, J.; Mokhtari, M. Seismological evidence for crustal-scale thrusting in the Zagros mountain belt (Iran). Geophys. J. Int. 2006, 166, 227–237. [Google Scholar] [CrossRef]
- Paul, A.; Hatzfeld, D.; Kaviani, A.; Tatar, M.; Péquegnat, C. Seismic imaging of the lithospheric structure of the Zagros mountain belt (Iran). Geol. Soc. Lond. Spéc. Publ. 2010, 330, 5–18. [Google Scholar] [CrossRef]
- Motaghi, K.; Shabanian, E.; Kalvandi, F. Underplating along the northern portion of the Zagros suture zone, Iran. Geophys. J. Int. 2017, 210, 375–389. [Google Scholar] [CrossRef]
- Motaghi, K.; Shabanian, E.; Tatar, M.; Cuffaro, M.; Doglioni, C. The south Zagros suture zone in teleseismic images. Tectonophysics 2017, 694, 292–301. [Google Scholar] [CrossRef]
- Radjaee, A.; Rham, D.; Mokhtari, M.; Tatar, M.; Priestley, K.; Hatzfeld, D. Variation of Moho depth in the central part of the Alborz Mountains, northern Iran. Geophys. J. Int. 2010, 181, 173–184. [Google Scholar] [CrossRef]
- Zonenshain, L.; Pichon, X. Deep basins of the Black Sea and Caspian Sea as remnants of Mesozoic back-arc basins. Tectonophysics 1986, 123, 181–211. [Google Scholar] [CrossRef]
- Brunet, M.-F.; Korotaev, M.V.; Ershov, A.V.; Nikishin, A.M. The South Caspian Basin: A review of its evolution from subsidence modelling. Sediment. Geol. 2003, 156, 119–148. [Google Scholar] [CrossRef]
- Kaviani, A.; Paul, A.; Moradi, A.; Mai, P.M.; Pilia, S.; Boschi, L.; Rümpker, G.; Lu, Y.; Tang, Z.; Sandvol, E. Crustal and uppermost mantle shear wave velocity structure beneath the Middle East from surface wave tomography. Geophys. J. Int. 2020, 221, 1349–1365. [Google Scholar] [CrossRef]
- Movaghari, R.; Doloei, G.J. 3-D crustal structure of the Iran plateau using phase velocity ambient noise tomography. Geophys. J. Int. 2020, 220, 1555–1568. [Google Scholar] [CrossRef]
- Lu, Y.; Liu, B.; Pei, S.; Sun, Y.; Toksoz, M.N.; Zeng, X. Pn Tomographic Velocity and Anisotropy beneath the Iran Region. Bull. Seism. Soc. Am. 2012, 102, 426–435. [Google Scholar] [CrossRef]
- Pasyanos, M.E.; Tarabulsi, Y.M.; Al-Hadidy, S.Y.; Raddadi, W.W.; Mousa, A.D.; El-Hussain, I.; Al-Jeri, F.; Al-Shukri, H.; Gök, R. Improved lithospheric attenuation structure of the Arabian Peninsula through the use of national network data. Arab. J. Geosci. 2021, 14, 914. [Google Scholar] [CrossRef]
- Pasyanos, M.E.; Matzel, E.M.; Walter, W.R.; Rodgers, A.J. Broad-band Lg attenuation modelling in the Middle East. Geophys. J. Int. 2009, 177, 1166–1176. [Google Scholar] [CrossRef]
- Rodgers, A.J.; Ni, J.F.; Hearn, T.M. Propagation Characteristics of Short-Period Sn and Lg in the Middle East. Bull. Seismol. Soc. Am. 1997, 87, 396–413. [Google Scholar]
- Sandvol, E.; Al-Damegh, K.; Calvert, A.; Seber, D.; Barazangi, M.; Mohamad, R.; Gök, R.; Türkelli, N.; Gürbüz, C. Tomographic Imaging of Lg and Sn Propagation in the Middle East. Pure Appl. Geophys. 2001, 158, 1121–1163. [Google Scholar] [CrossRef]
- Al-Damegh, K.; Sandvol, E.; Al-Lazki, A.; Barazangi, M. Regional seismic wave propagation (Lg and Sn) and Pn attenuation in the Arabian Plate and surrounding regions. Geophys. J. Int. 2004, 157, 775–795. [Google Scholar] [CrossRef]
- Kaviani, A.; Sandvol, E.; Ku, W.; Beck, S.L.; Türkelli, N.; Özacar, A.A.; Delph, J.R. Seismic attenuation tomography of the Sn phase beneath the Turkish-Iranian Plateau and the Zagros mountain belt. Geosphere 2022, 18, 1377–1393. [Google Scholar] [CrossRef]
- Sakineh, A. Foraminiferal-Based Paleobiogeographic Reconstructions in the Carboniferous of Iran and Its Implications for the Neo-Tethys Opening Time: A Synthesis. Geol. Acta 2017, 15, 1–17. [Google Scholar]
- Seber, D.; Vallvé, M.; Sandvol, E.; Steer, D.; Barazangi, M. Middle East Tectonics: Applications of Geographic Information Systems (GIS). GSA Today 1997, 7, 1–6. [Google Scholar]
- Maheri-Peyrov, M.; Ghods, A.; Abbasi, M.; Bergman, E.; Sobouti, F. ML shear wave velocity tomography for the Iranian Plateau. Geophys. J. Int. 2016, 205, 179–191. [Google Scholar] [CrossRef]
- Aki, K.; Richards, P. Quantitative Seismology, 2nd ed.; W.H. Freeman and Company: San Francisco, CA, USA, 2002; ISBN 0935702962. [Google Scholar]
- Takahashi, T.; Sato, H.; Nishimura, T.; Obara, K. Strong inhomogeneity beneath Quaternary volcanoes revealed from the peak delay analysis of S-wave seismograms of microearthquakes in northeastern Japan. Geophys. J. Int. 2007, 168, 90–99. [Google Scholar] [CrossRef]
- Takahashi, T.; Sato, H.; Nishimura, T. Recursive formula for the peak delay time with travel distance in von Kármán type non-uniform random media on the basis of the Markov approximation. Geophys. J. Int. 2008, 173, 534–545. [Google Scholar] [CrossRef]
- Sato, H.; Fehler, M.C.; Maeda, T. Seismic Wave Propagation and Scattering in the Heterogeneous Earth, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar] [CrossRef]
- Sato, H. Broadening of seismogram envelopes in the randomly inhomogeneous lithosphere based on the parabolic approximation: Southeastern Honshu, Japan. J. Geophys. Res. Earth Surf. 1989, 94, 17735. [Google Scholar] [CrossRef]
- Obara, K.; Sato, H. Regional differences of random inhomogeneities around the volcanic front in the Kanto-Tokai area, Japan, revealed from the broadening of S wave seismogram envelopes. J. Geophys. Res. Earth Surf. 1995, 100, 2103–2121. [Google Scholar] [CrossRef]
- Takahashi, T.; Sato, H.; Nishimura, T.; Obara, K. Tomographic inversion of the peak delay times to reveal random velocity fluctuations in the lithosphere: Method and application to northeastern Japan. Geophys. J. Int. 2009, 178, 1437–1455. [Google Scholar] [CrossRef]
- Takahashi, T.; Obana, K.; Yamamoto, Y.; Nakanishi, A.; Kodaira, S.; Kaneda, Y. The 3-D distribution of random velocity inhomogeneities in southwestern Japan and the western part of the Nankai subduction zone. J. Geophys. Res. Solid Earth 2013, 118, 2246–2257. [Google Scholar] [CrossRef]
- Takahashi, T.; Obana, K.; Kodaira, S.; Suetsugu, D.; Takahashi, N.; Kamiya, S.; Tamura, Y. Random inhomogeneities in the northern Izu-Bonin arc estimated by tomographic inversion of peak delay times of Swave seismograms. J. Geophys. Res. Earth Surf. 2011, 116. [Google Scholar] [CrossRef]
- Ranjan, P.; Konstantinou, K.; Andinisari, R. Spatial Distribution of Random Velocity Inhomogeneities in the Southern Aegean From Inversion of S Wave Peak Delay Times. J. Geophys. Res. Solid Earth 2019, 124, 10393–10412. [Google Scholar] [CrossRef]
- Calvet, M.; Sylvander, M.; Margerin, L.; Villaseñor, A. Spatial variations of seismic attenuation and heterogeneity in the Pyrenees: Coda Q and peak delay time analysis. Tectonophysics 2013, 608, 428–439. [Google Scholar] [CrossRef]
- Nuttli, O.W. Seismic wave attenuation and magnitude relations for eastern North America. J. Geophys. Res. Earth Surf. 1973, 78, 876–885. [Google Scholar] [CrossRef]
- Rezapour, M. Magnitude Scale in the Tabriz Seismic Network. J. Earth Space Phys. 2005, 31, 13021. [Google Scholar]
- Hearn, T.M. Pn travel times in southern California. J. Geophys. Res. Earth Surf. 1984, 89, 1843–1855. [Google Scholar] [CrossRef]
- Campillo, M. Propagation and attenuation characteristics of the crustal phaseLg. Pure Appl. Geophys. 1990, 132, 1–19. [Google Scholar] [CrossRef]
- Dalton, C.A.; Ekström, G.; Göran, E. Constraints on global maps of phase velocity from surface-wave amplitudes. Geophys. J. Int. 2006, 167, 820–826. [Google Scholar] [CrossRef]
- Bormann, P.; Dewey, J.W.; Gabstarova, I.; Gregersen, S.; Gusev, A.A.; Kim, W.-Y.; Patton, H.; Presgrave, B.; Ruifeng, L.; Saul, J.; et al. Summary of Magnitude Working Group Recommendations on Standard Procedures for Determining Earthquake Magnitudes from Digital Data. 2013. Available online: https://docplayer.net/15210031-Summary-of-magnitude-working-group-recommendations-on-determining-earthquake-magnitudes-from-digital-data.html (accessed on 16 October 2022).
- Hutton, L.K.; Boore, D.M. The ML Scale in Southern California. Bull. Seismol. Soc. Am. 1987, 77, 2074–2094. [Google Scholar] [CrossRef]
- Askari, R.; Ghods, A.; Sobouti, F. Calibration of an ML Scale in the Alborz Region, Northern Iran. Bull. Seism. Soc. Am. 2009, 99, 268–276. [Google Scholar] [CrossRef]
- Rezapour, M.; Rezaei, R. Empirical Distance Attenuation and the Local Magnitude Scale for Northwest Iran. Bull. Seism. Soc. Am. 2011, 101, 3020–3031. [Google Scholar] [CrossRef]
- Emami, R.; Rezaie, R.; Rezapour, M. Determination of Empirical Distance Attuation and the Local-Magnitude Scale for Northwest Iran with JHD Technique. Sci. Q. J. Geosci. 2014, 23, 85–92. [Google Scholar]
- Shoja-Taheri, J.; Naserieh, S.; Ghofrani, H. ML and MW Scales in the Iranian Plateau Based on the Strong-Motion Records. Bull. Seism. Soc. Am. 2007, 97, 661–669. [Google Scholar] [CrossRef][Green Version]
- Shoja-Taheri, J.; Naserieh, S.; Ghafoorian-Nasab, A.H. An ML Scale in Northeastern Iran. Bull. Seism. Soc. Am. 2008, 98, 1975–1982. [Google Scholar] [CrossRef]
- Zhang, T.-R.; Lay, T. Why the Lg Phase Does Not Traverse Oceanic Crust. Bull. Seismol. Soc. Am. 1995, 85, 1665–1678. [Google Scholar]
- Rahimi, H.; Hamzehloo, H.; Kamalian, N. Estimation of coda and shear wave attenuation in the volcanic area in SE Sabalan Mountain, NW Iran. Acta Geophys. 2009, 58, 244–268. [Google Scholar] [CrossRef]
- Campillo, M.; Bouchon, M.; Massinon, B. Theoretical study of the excitation, spectral characteristics, and geometrical attenuation of regional seismic phases. Bull. Seism. Soc. Am. 1984, 74, 79–90. [Google Scholar] [CrossRef]
- Akinci, A.; Ibáñez, J.; del Pezzo, E.; Morales, J. Geometrical spreading and attenuation of Lg waves: A comparison between western Anatolia (Turkey) and southern Spain. Tectonophysics 1995, 250, 47–60. [Google Scholar] [CrossRef]
- Mahood, M.; Hamzehloo, H. Variation of intrinsic and scattering attenuation of seismic waves with depth in the Bam region, East-Central Iran. Soil Dyn. Earthq. Eng. 2011, 31, 1338–1346. [Google Scholar] [CrossRef]
- Naghavi, M.; Shomali, Z.H.; Zare, M. Lg Coda Variations in North-Central Iran. Int. J. Geophys. 2012, 2012, 673506. [Google Scholar] [CrossRef][Green Version]
- Meghdadi, A.; Shoja-Taheri, J. Ground-Motion Attenuation and Source Spectral Shape for Earthquakes in Eastern Iran. Bull. Seism. Soc. Am. 2014, 104, 624–633. [Google Scholar] [CrossRef]
- Kaviani, A.; Sandvol, E.; Bao, X.; Rümpker, G.; Gök, R. The structure of the crust in the Turkish–Iranian Plateau and Zagros using Lg Q and velocity. Geophys. J. Int. 2015, 200, 1254–1268. [Google Scholar] [CrossRef]
- Bajestani, E.M.; Ansari, A.; Karkooti, E. Local and regional P-wave spectral attenuation model for Iran. Geophys. J. Int. 2020, 224, 241–256. [Google Scholar] [CrossRef]
- Motaghi, K.; Ghods, A. Attenuation of Ground-Motion Spectral Amplitudes and Its Variations across the Central Alborz Mountains. Bull. Seism. Soc. Am. 2012, 102, 1417–1428. [Google Scholar] [CrossRef]
- Maheri-Peyrov, M.; Ghods, A.; Donner, S.; Akbarzadeh-Aghdam, M.; Sobouti, F.; Motaghi, K.; Hassanzadeh, M.; Mortezanejad, G.; Talebian, M.; Chen, L. Upper crustal structure of NW Iran revealed by regional 3-D Pg velocity tomography. Geophys. J. Int. 2020, 222, 1093–1108. [Google Scholar] [CrossRef]
- Rezaeifar, M.; Kissling, E. Regional 3-D lithosphere structure of the northern half of Iran by local earthquake tomography. Geophys. J. Int. 2020, 223, 1956–1972. [Google Scholar] [CrossRef]
- Bavali, K.; Motaghi, K.; Sobouti, F.; Ghods, A.; Abbasi, M.; Priestley, K.; Mortezanejad, G.; Rezaeian, M. Lithospheric structure beneath NW Iran using regional and teleseismic travel-time tomography. Phys. Earth Planet. Inter. 2016, 253, 97–107. [Google Scholar] [CrossRef]
- Mostafanejad, A.; Shomali, Z.H.; Mottaghi, A.A. 3-D velocity structure of Damavand volcano, Iran, from local earthquake tomography. J. Southeast Asian Earth Sci. 2011, 42, 1091–1096. [Google Scholar] [CrossRef]
- Rezaeifar, M.; Kissling, E.; Shomali, Z.H.; Shahpasand-Zadeh, M. 3D Crustal Structure of the Northwest Alborz Region (Iran) from Local Earthquake Tomography. Swiss J. Geosci. 2016, 109, 389–400. [Google Scholar] [CrossRef]
- Lü, Y.; Chen, L. Upper crustal P-wave velocity structure beneath two volcanic areas in northern Iran. Sci. China Earth Sci. 2017, 60, 786–795. [Google Scholar] [CrossRef]
- Pei, S.; Sun, Y.; Toksöz, M.N. Tomographic Pn and Sn velocity beneath the continental collision zone from Alps to Himalaya. J. Geophys. Res. Earth Surf. 2011, 116. [Google Scholar] [CrossRef][Green Version]
- Zhu, T.; Chun, K.-Y.; West, G.F. Geometrical Spreading and Q of Pn Waves: An Investigative Studey in Eastern Canada. Bull. Seism. Soc. Am. 1991, 3, 882–896. [Google Scholar] [CrossRef]
- Amini, S.; Shomali, Z.H.; Koyi, H.; Roberts, R.G. Tomographic upper-mantle velocity structure beneath the Iranian Plateau. Tectonophysics 2012, 554–557, 42–49. [Google Scholar] [CrossRef]
- Kumar, R.; Gupta, S.C.; Kumar, A. Attenuation Characteristics of Seismic Body Waves for the Crust of Lower Siang Region of Arunachal Himalaya. Int. J. Adv. J. 2014, 2, 742–755. [Google Scholar]
- Kumar, R.; Gupta, S.C.; Singh, S.P.; Kumar, A. The Attenuation of High-Frequency Seismic Waves in the Lower Siang Region of Arunachal Himalaya: Qα, Qβ, Qc, Qi, and Qs. Bull. Seism. Soc. Am. 2016, 106, 1407–1422. [Google Scholar] [CrossRef]
- Gusev, A.; Abubakirov, I.R. Vertical profile of effective turbidity reconstructed from broadening of incoherent body-wave pulses-II.Application to Kamchatka data. Geophys. J. Int. 1999, 136, 309–323. [Google Scholar] [CrossRef][Green Version]
- Milton, D.J. Qal’eh hasan ali maars, central Iran. Bull. Volcanol. 1977, 40, 201–208. [Google Scholar] [CrossRef]
- Maggi, A.; Priestley, K. Surface waveform tomography of the Turkish-Iranian plateau. Geophys. J. Int. 2005, 160, 1068–1080. [Google Scholar] [CrossRef]
- Alinaghi, A.; Koulakov, I.; Thybo, H. Seismic tomographic imaging of P- and S-waves velocity perturbations in the upper mantle beneath Iran. Geophys. J. Int. 2007, 169, 1089–1102. [Google Scholar] [CrossRef]
- Mahmoodabadi, M.; Yaminifard, F.; Tatar, M.; Kaviani, A.; Motaghi, K. Upper-mantle velocity structure beneath the Zagros collision zone, Central Iran and Alborz from nonlinear teleseismic tomography. Geophys. J. Int. 2019, 218, 414–428. [Google Scholar] [CrossRef]
- Mottaghi, A.A.; Rezapour, M.; Korn, M. Ambient noise surface wave tomography of the Iranian Plateau. Geophys. J. Int. 2013, 193, 452–462. [Google Scholar] [CrossRef][Green Version]
- Dehghani, G.A.; Makris, J. The Gravity Field and Crustal Structure of Iran. Neues Jahrb. Geol. Paläontologie 1984, 168, 215–229. [Google Scholar] [CrossRef]
- Jiménez-Munt, I.; Fernàndez, M.; Saura, E.; Vergés, J.; Garcia-Castellanos, D. 3-D lithospheric structure and regional/residual Bouguer anomalies in the Arabia-Eurasia collision (Iran). Geophys. J. Int. 2012, 190, 1311–1324. [Google Scholar] [CrossRef]
- Babazadeh, S.A. Suture Zone (Neo-Tethyan Margin) in Eastern Iran. Geodyn. Res. Int. Bull. 2013, 1, 1–7. [Google Scholar]
- Kaz’Min, V.G.; Verzhbitskii, E.V. Age and origin of the South Caspian Basin. Oceanology 2011, 51, 131–140. [Google Scholar] [CrossRef]
- Rahimi, H.; Motaghi, K.; Mukhopadhyay, S.; Hamzehloo, H. Variation of coda wave attenuation in the Alborz region and central Iran. Geophys. J. Int. 2010, 181, 1643–1654. [Google Scholar] [CrossRef]
- Wessel, P.; Luis, J.F.; Uieda, L.; Scharroo, R.; Wobbe, F.; Smith, W.H.F.; Tian, D. The Generic Mapping Tools Version 6. Geochem. Geophys. Geosyst. 2019, 20, 5556–5564. [Google Scholar] [CrossRef]

| Authors | Region | Amplitude Type | Spreading Coefficient | Attenuation Coefficient | Constant Term |
|---|---|---|---|---|---|
| This study | Iran r > 150 km r < 1500 km | Velocity | 2.95 | −0.000150 | 6.98 |
| Iranian Seismological Center | Iran r > 106 km r < 600 km | Velocity/4π | 2.5 | - | −1.8 |
| Rezapour 2005 [50] | Iran r > 170 km r < 1000 km | Velocity/4π | 2.6 | - | −2.2 |
| Askari et al., 2009 [56] | Central Alborz | Displacement | 1.1725 | 0.0021 | −0.4450 |
| Rezapour and Rezaei 2011 [57] | NW Iran | Displacement | 0.9252 (vert.) 0.9993 (horiz.) | 0.0030 0.0029 | 0.8496 0.7114 |
| Emami, Rezaei, and Rezapour 2014 [58] | NW Iran | Displacement | 1.4050 | 0.0019 | 3 |
| Shoja-Taheri et al., 2007 [59] | Iran r < 96 km 96 < r < 131 km r > 131 km | Displacement | 1.01 −0.14 0.14 | 0.0002 0.0002 0.00020 | - |
| Bormann et al., 2013; Hutton and Boore 1987 [54,55] | Southern California <1000 km | Displacement | 1.11 | 0.00189 | −2.09 |
| Shoja-Taheri et al., 2008 [60] | NE Iran > 0 km 0 < r < 106 106 < r < 347 r > 347 | Displacement | 1.37 1.38 0.597 0.415 | 0.002 0.0033 0.0033 0.0033 | - |
| Phase | Phase Velocity | Group Velocity | Spreading | Q | Phase Intercept | Group Intercept |
|---|---|---|---|---|---|---|
| Lg | 3.7 km/s (Sg) | 3.2 km/s | 1.21 | 694 | 3.96 s (Sg) | 2.11 s |
| Pg | 6.1 km/s | 5.6 km/s | 0.97 | 314 | 1.8 s | 1.7 s |
| Sn | 4.7 km/s | 4.6 km/s | 1.69 | 733 | 12.7 s | 19.9 s |
| Pn | 8.1 km/s | 8.0 km/s | 0.99 | 254 | 8.6 s | 11.9 s |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hearn, T.M. Two-Dimensional Attenuation and Velocity Tomography of Iran. Geosciences 2022, 12, 397. https://doi.org/10.3390/geosciences12110397
Hearn TM. Two-Dimensional Attenuation and Velocity Tomography of Iran. Geosciences. 2022; 12(11):397. https://doi.org/10.3390/geosciences12110397
Chicago/Turabian StyleHearn, Thomas M. 2022. "Two-Dimensional Attenuation and Velocity Tomography of Iran" Geosciences 12, no. 11: 397. https://doi.org/10.3390/geosciences12110397
APA StyleHearn, T. M. (2022). Two-Dimensional Attenuation and Velocity Tomography of Iran. Geosciences, 12(11), 397. https://doi.org/10.3390/geosciences12110397









