Stability Analysis of Lava Tunnels on Santa Cruz Island (Galapagos Islands, Ecuador) Using Rock Mass Classifications: Empirical Approach and Numerical Modeling
Abstract
:1. Introduction
2. Materials and Methods
- Visit the cave for: geometric data, geomechanical station to determine the simple compression of the rock, discontinuity properties, quality index, and Geological Strength Index (GSI). Observation and data collection of eventual instabilities.
- Cabinet work for:
- 2.1.
- Determination of the massif quality indices: RMR, Q, and CGI;
- 2.2.
- 2.3.
- Elaboration of stress–strain models by numerical modeling using boundary elements;
- 2.4.
- Discussion and comparison of results.
- Geomechanical classification of the rock mass using Barton’s Q index and RMR [15]
- Cave steady state qualification using CGI
3. Results
4. Discussion
5. Conclusions
| RMR | Rock Mass Rating |
|---|---|
| Q | Q index |
| CGI | Cave Geomechanical Index |
| CS | Ceiling shape |
| CT | Ceiling thickness |
| Weight assigned to the rock mass rating for the CGI classification | |
| Weight assigned to the hydraulic radius for the CGI classification | |
| Weight assigned to the ceiling shape for the CGI classification | |
| Weight assigned to the ceiling thickness for the CGI classification | |
| Jn | Joint set number |
| Jr | Joint roughness number |
| Ja | Joint alteration number |
| Jw | Joint water reduction |
| SRF | Stress reduction factor |
| RMR1 | Strength of intact rock material (rating assigned for the RMR) |
| RMR2 | Rock quality designation (rating assigned for the RMR) |
| RMR3 | Spacing of discontinuities (rating assigned for the RMR) |
| RMR4 | Condition of discontinuities (rating assigned for the RMR) |
| RMR5 | Groundwater condition (rating assigned for the RMR) |
| RMRb | Basic RMR |
| RMRi | RMR of geomechanical station i |
| EG# | Geomechanical station number # |
| Em | Rock elastic modulus, Young modulus |
| GSI | Geological Strength Index |
| mi | Parameter used in Hoek–Brown failure criterion |
| F.S | Factor of safety |
| D | Disturbance factor used in Hoek–Brown criterion |
| S F | Safety factor (same as FS) |
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Jordà-Bordehore, L. Stability Assessment of Natural Caves Using Empirical Approaches and Rock Mass Classifications. Rock Mech. Rock Eng. 2017, 50, 2143–2154. [Google Scholar] [CrossRef]
- Grenier, C. Conservation against nature. The Galapagos Islands. In Conservation against Nature. The Galapagos Islands; Abya Yala: Quito, Ecuador, 2007. [Google Scholar] [CrossRef]
- Addison, A. Galapagos—Caving the Equator. Natl. Speleol. Soc. News 2011, 69, 8–17. [Google Scholar]
- Jordà-Bordehore, L.; Toulkeridis, T. Stability assessment of volcanic natural caves—Lava tunnels—Using both empirical and numerical approach, case studies of Galapagos Islands (Ecuador) and Lanzarote Island (Canary—Spain). In Proceedings of the ISRM International Symposium—EUROCK, Ürgüp, Turkey, 29–31 August 2016; pp. 835–839. [Google Scholar] [CrossRef]
- Geist, D.; Diefenbach, B.A.; Fornari, D.J.; Kurz, M.D.; Harpp, K.; Blusztajn, J. Construction of the Galápagos platform by large submarine volcanic terraces. Geochem. Geophys. Geosyst. 2008, 9, 1–27. [Google Scholar]
- Vallejo, S. Distribution of Late-Holocene Volcanic Ash on the Coast of Ecuador. Ph.D. Thesis, 2011. [Google Scholar]
- Sauro, F.; Pozzobon, R.; Massironi, M.; De Berardinis, P.; Santagata, T.; De Waele, J. Lava tubes on Earth, Moon and Mars: A review on their size and morphology revealed by comparative planetology. Earth-Sci. Rev. 2020, 209, 103288. [Google Scholar]
- Jordà-Bordehore, L.; García, R.; Alonso-Zarza, A.; Romero, C. Stability assessment of shallow limestone caves through an empirical approach: Application of the stability graph method to the Castañar Cave study site (Spain). Bull. Eng. Geol. Environ. 2016, 75, 1469–1483. [Google Scholar]
- Calvari, S.; Pinkerton, H. Lava tube morphology on Etna and evidence for lava flow emplacement mechanisms. J. Volcanol. Geotherm. Res. 1999, 90, 263–280. [Google Scholar]
- Mulas, M.; Cioni, R.; Andronico, D.; Mundula, F. The explosive activity of the 1669 Monti Rossi eruption at Mt. Etna (Italy). J. Volcanol. Geotherm. Res. 2016, 328, 115–133. [Google Scholar]
- Waltham, A.C.; Park, H.D. Roads over lava tubes in Cheju Island, South Korea. Eng. Geol. 2002, 66, 53–64. [Google Scholar]
- Jordá-Bordehore, L.; Toulkeridis, T.; Romero-Crespo, P.L.; Jordá-Bordehore, R.; García- Garizabal, I. Stability assessment of volcanic lava tubes in the Galápagos using engineering rock mass classifications and an empirical approach. Int. J. Rock Mech. Min. Sci. 2016, 89, 55–67. [Google Scholar] [CrossRef]
- Barton, N.; Grimstad, E. The Q System following thirty years of development and application in tunneling projects. In Proceedings of the ISRM Regional Symposium EUROCK 2004 & 53rd Geomechanics Colloquy, Salzburg, Austria, 7–9 October 2004; Schubert, W., Ed.; Verlag Glückauf: Essen, Germany, 2004. [Google Scholar]
- Barton, N.; Grimstad, E. Q-System—An Illustrated Guide following Forty Years in Tunneling. 2014. Available online: www.nickbarton.com (accessed on 20 May 2022).
- Bieniawski, Z.T. Engineering Rock Mass Classification; John Wiley & Sons: Hoboken, NJ, USA, 1989. [Google Scholar] [CrossRef]
- Bieniawski, Z. Errors in the Application of Geomechanical Classifications and Their Correction; Ingeopres: Madrid, Spain, 2011; Volume 208, pp. 10–21. [Google Scholar]
- Barton, N.; Bieniawski, Z. RMR and Q-setting records straight. Tunn. Tunn. Int. 2008, 2, 26–29. [Google Scholar]
- Grimstad, E.; Barton, N. Updating of the Q-System fr NTM. In Proceedings of the International Symposium on Sprayed Concrete-Moderns Use of Wet Mix Sprayed Concrete for Underground Support, Fagernes, Norway, 22–26 October 1993; Kompen, C., Opshal, S.L., Berg, S.L., Eds.; Norwegian Concrete Association: Oslo, Norway, 1993. [Google Scholar]
- Hoek, E. Practical Rock Engineering. Set of Course Notes. Shear Strength of Discontinuities. 2014. Available online: www.rocscience.com/education/hoeks_corner (accessed on 20 May 2022).
- Romana-Ruiz, M. Excavation and Support Recommendations for Tunnels; Jornadas Hispano-Lusas sobre Obras Subterráneas: Madrid, Spain, 2003; pp. 105–120. [Google Scholar]
- Bieniawski, Z. Engineering Classification of jointed rock masses. Civ. Eng. S. Afr. 1973, 15, 333–343. [Google Scholar]
- Brandi, I.V.; Barbosa, M.R.; Barata, A.; de Paula, R.G.; Correa, T.; Mota, H.; Lima, D.; Osborne, R.A.; Vale, S.A.; Lima, N.; et al. Cave Geomechanical Index (CGI). Classification and Contribution to the Conservation of Natural Caves in the Iron Mines. Geoconserv. Res. 2021, 3, 134–161. [Google Scholar] [CrossRef]
- Bieniawski, Z. The geomechanics classification in rock engineering applications. In Proceedings of the 4th Congress of the International Society for Rock Mechanics, Montreux, Switzerland, 2–8 September 1979; OnePetro: Richardson, TX, USA, 1979. [Google Scholar]
- Milne, D.M. Underground Design and Deformation Based on Surface Geometry. Master’s Thesis, University of British Columbia, Vancouver, BC, Canada, 1997. [Google Scholar]
- Laubscher, D.H. Geomechanics classification of jointed rock masses: Mining applications. Inst. Min. Metall. Trans. Sect. 1977, 86, 1–8. [Google Scholar]
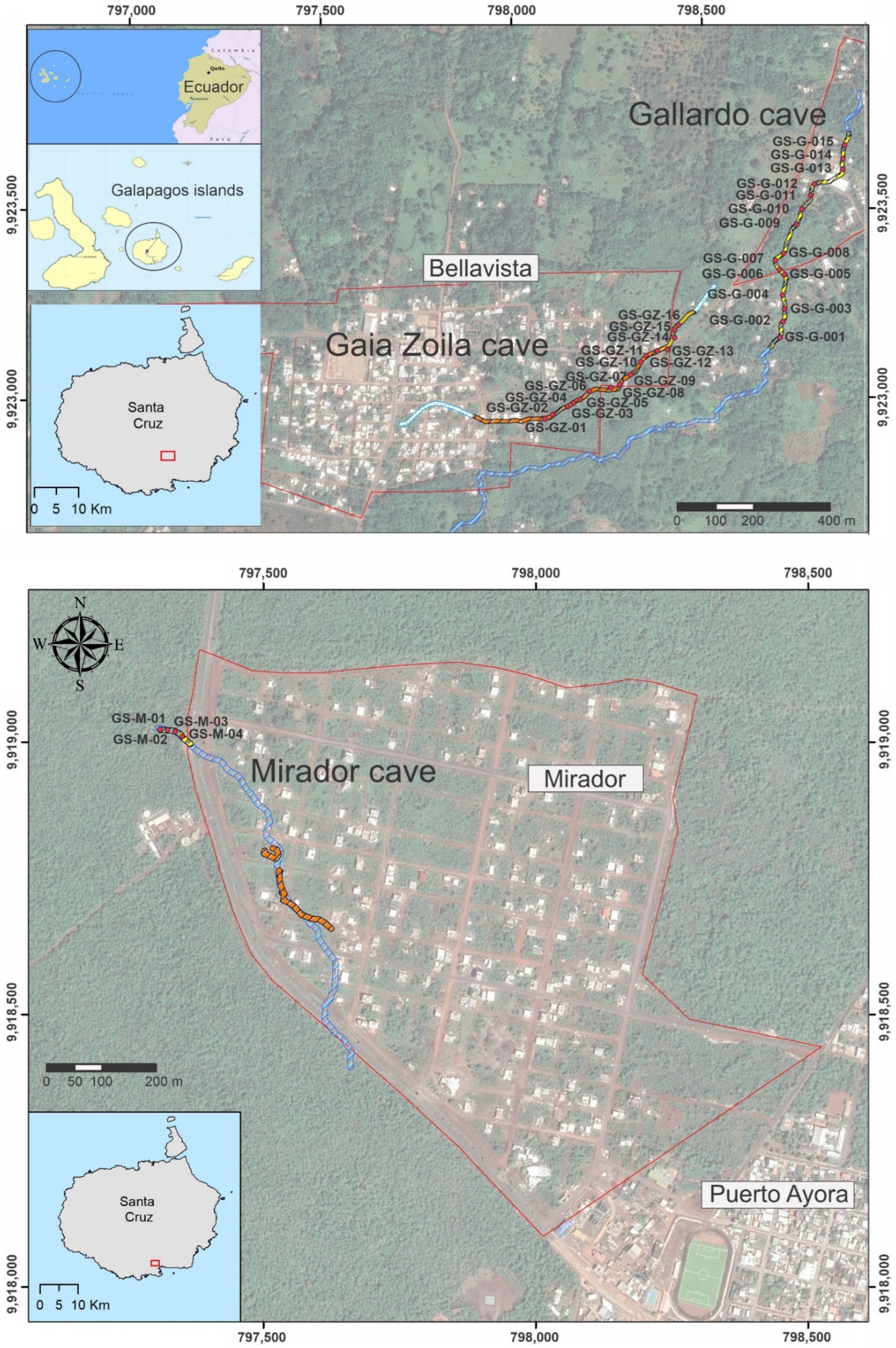
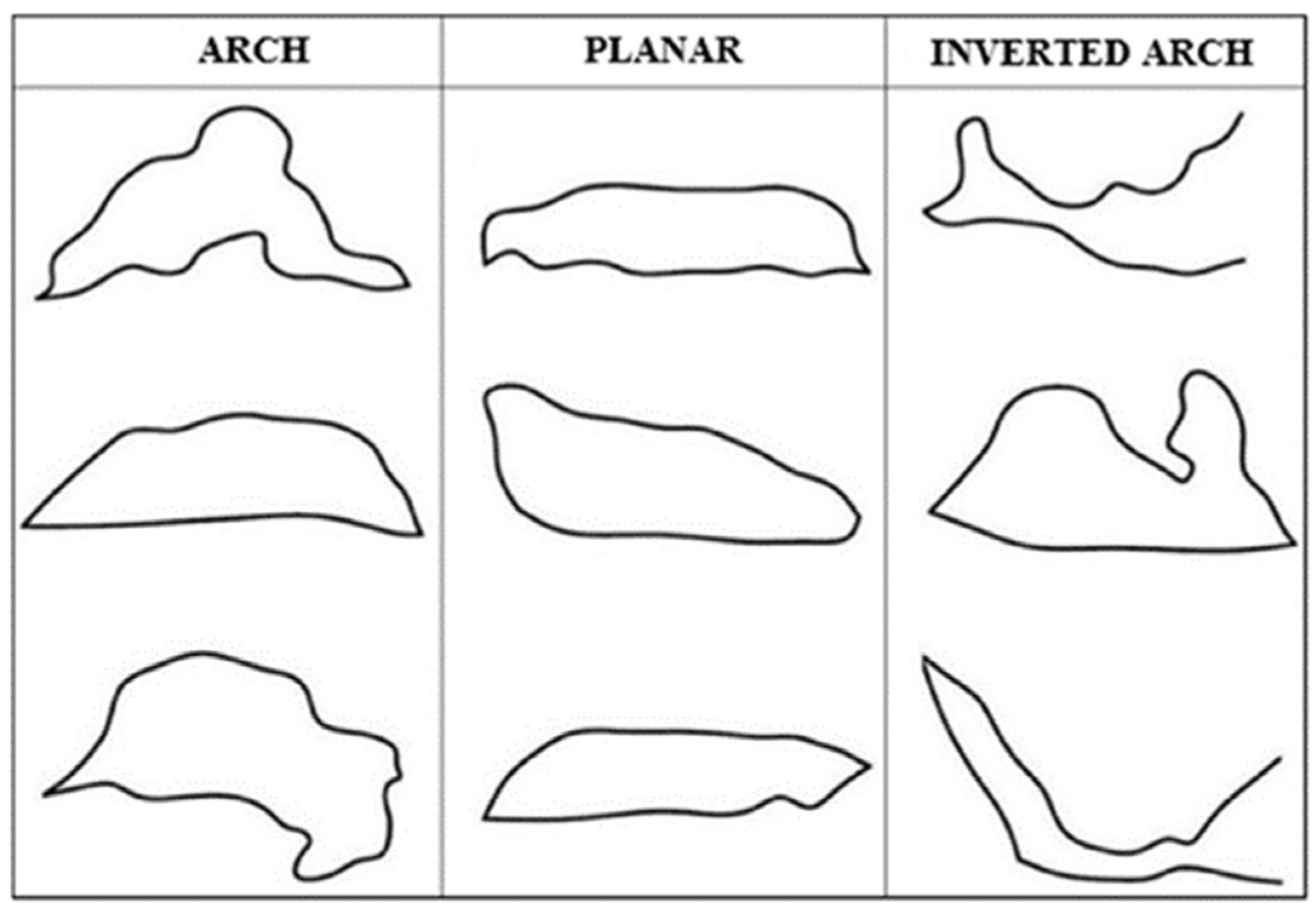
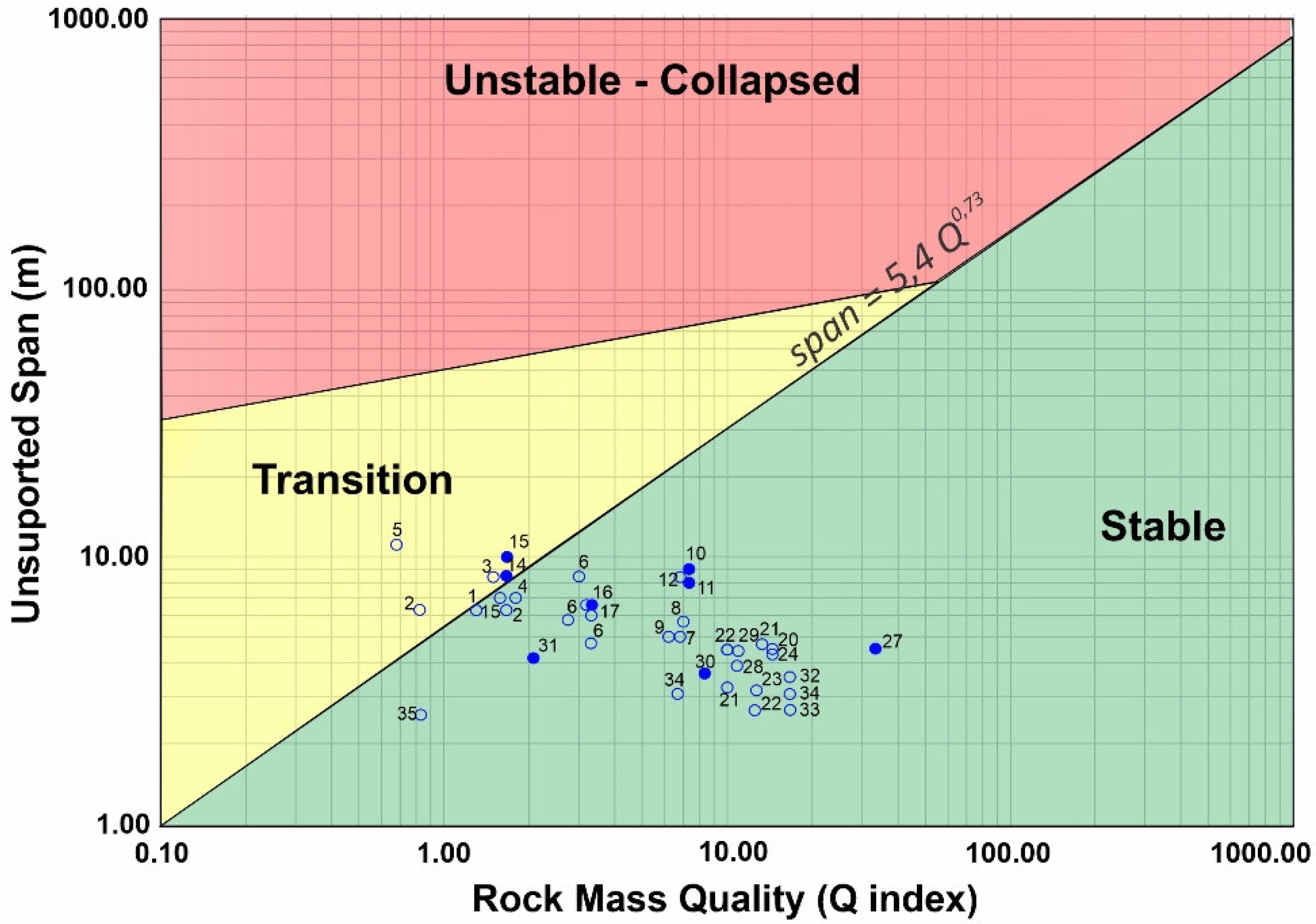
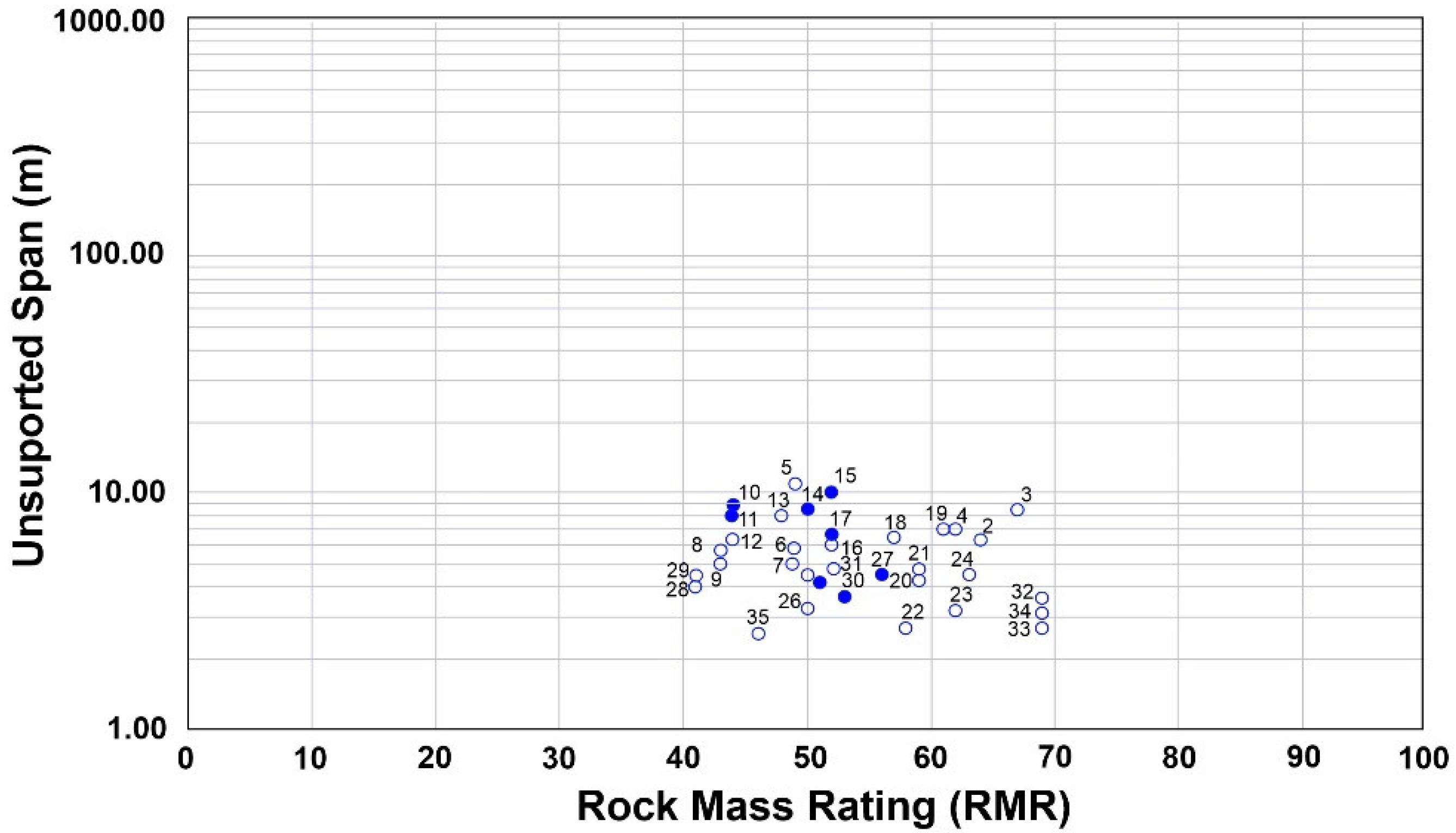

| Sum | 100–81 | 80–61 | 60–41 | 40–21 | <20 |
| Class number | I | II | III | IV | V |
| Description | Very good | Good | Regular | Poor | Very Poor |
| Class Range | 1.83–3 m | 0.92–1.82 m | 0.00–0.91 m |
| Class | Large | Regular | Small |
| Class Range | 7.65–10 m | 3.32–7.64 m | 0.00–3.31 m |
| Class | Large | Regular | Small |
| Cave | Geo- Mechanical Station | Dimensions | Rock Mass Quality Q Index | Code | Visual Description Type | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Width (m) | RQD | Jn | Jr | Ja | Jw | SRF | Q | ||||
| (%) | |||||||||||
| Mirador | GS-M-01 | 6.4 | 78 | 2 × 9 | 3 | 2 | 1 | 5 | 1.3 | 1 | Stable |
| GS-M-02 | 6.3 | 50 | 2 × 9 | 3 | 2 | 1 | 5 | 0.83 | 2 | Stable | |
| GS-M-02b | 6.3 | 50 | 2 × 9 | 3 | 1 | 1 | 5 | 1.67 | 2 | Stable | |
| GS-M-03 | 8.4 | 90 | 2 × 9 | 3 | 2 | 1 | 5 | 1.5 | 3 | Stable | |
| GS-M-03b | 8.4 | 90 | 2 × 9 | 3 | 1 | 1 | 5 | 3 | 3 | Stable | |
| GS-M-04 | 7 | 80 | 9 | 3 | 3 | 1 | 5 | 1.78 | 4 | Stable | |
| Gallardo | GS-G-01 | 11 | 82 | 2 × 9 | 3 | 4 | 1 | 5 | 0.68 | 5 | Stable |
| GS-G-02 | 5.8 | 82 | 9 | 3 | 4 | 1 | 2.5 | 2.73 | 6 | Stable | |
| GS-G-03 | 5 | 82 | 9 | 3 | 4 | 1 | 1 | 6.83 | 7 | Stable | |
| GS-G-04 | 5.8 | 85 | 9 | 3 | 4 | 1 | 1 | 7.1 | 8 | Stable | |
| GS-G-05 | 5 | 75 | 9 | 3 | 4 | 1 | 1 | 6.25 | 9 | Stable | |
| GS-G-06 | 8.9 | 88 | 9 | 3 | 4 | 1 | 1 | 7.33 | 10 | Stable | |
| GS-G-07 | 8 | 88 | 9 | 3 | 4 | 1 | 1 | 7.33 | 11 | Stable | |
| GS-G-08 | 6.4 | 88 | 9 | 3 | 4 | 1 | 1 | 7.33 | 12 | Stable | |
| GS-G-09 | 8 | 88 | 9 | 3 | 4 | 1 | 1 | 7.33 | 13 | Stable | |
| GS-G-10 | 8.5 | 100 | 9 | 3 | 4 | 1 | 1 | 1.67 | 14 | Unstable | |
| GS-G-11 | 10 | 100 | 9 | 3 | 4 | 1 | 5 | 1.67 | 15 | Unstable | |
| GS-G-12 | 4.8 | 100 | 9 | 3 | 4 | 1 | 5 | 3.33 | 16 | Stable | |
| GS-G-12b | 6 | 100 | 9 | 3 | 4 | 1 | 2.5 | 3.33 | 16 | Stable | |
| GS-G-13 | 6.6 | 100 | 9 | 3 | 4 | 1 | 2.5 | 3.33 | 17 | Unstable | |
| GS-G-14 | 6.5 | 95 | 9 | 3 | 4 | 1 | 2.5 | 3.17 | 18 | Stable | |
| GS-G-15 | 7 | 95 | 2x9 | 3 | 2 | 1 | 5 | 1.58 | 19 | Stable | |
| Galla-Zoila | GS-GZ-01 | 4.3 | 85 | 9 | 3 | 2 | 1 | 1 | 14.2 | 20 | Stable |
| GS-GZ-02 | 4.8 | 80 | 9 | 3 | 2 | 1 | 1 | 13.33 | 21 | Stable | |
| GS-GZ-03 | 2.7 | 75 | 9 | 3 | 2 | 1 | 1 | 12.5 | 22 | Stable | |
| GS-GZ-04 | 3.2 | 75 | 9 | 3 | 2 | 1 | 1 | 12.5 | 23 | Stable | |
| GS-GZ-05 | 4.5 | 87 | 9 | 3 | 2 | 1 | 1 | 14.5 | 24 | Stable | |
| GS-GZ-06 | 4.5 | 60 | 9 | 3 | 2 | 1 | 1 | 10 | 25 | Stable | |
| GS-GZ-07 | 3.3 | 60 | 9 | 3 | 2 | 1 | 1 | 10 | 26 | Stable | |
| GS-GZ-08 | 4.5 | 100 | 9 | 3 | 1 | 1 | 1 | 33.33 | 27 | Unstable | |
| GS-GZ-09 | 4 | 65 | 9 | 3 | 2 | 1 | 1 | 10.83 | 28 | Stable | |
| GS-GZ-10 | 4.5 | 65 | 9 | 3 | 2 | 1 | 1 | 10.83 | 29 | Stable | |
| GS-GZ-11 | 3.7 | 75 | 9 | 3 | 3 | 1 | 1 | 8.33 | 30 | Unstable | |
| GS-GZ-12 | 4.2 | 75 | 9 | 1 | 4 | 1 | 1 | 2 | 31 | Unstable | |
| GS-GZ-13 | 3.6 | 100 | 9 | 3 | 2 | 1 | 1 | 17 | 32 | Stable | |
| GS-GZ-14 | 2.7 | 100 | 9 | 3 | 2 | 1 | 1 | 17 | 33 | Stable | |
| GS-GZ-15 | 3.1 | 100 | 9 | 3 | 2 | 1 | 2.5 | 7 | 34 | Stable | |
| GS-GZ-15b | 3.1 | 100 | 9 | 3 | 2 | 1 | 1 | 17 | 34 | Stable | |
| GS-GZ-16 | 2.57 | 50 | 2 × 9 | 3 | 4 | 1 | 2.5 | 0.83 | 35 | Stable | |
| Cave | Rock Mass Rating RMR | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Geo- Mechanical Station | Dimensions | RMR1 | RMR2 | RMR3 | RMR4 | RMR5 | RMRb | Code | Visual Description Type | |
| Width (m) | (%) | |||||||||
| Mirador | GS-M-01 | 6.4 | 3 | 16 | 8 | 15 | 15 | 57 | 1 | Stable |
| GS-M-02 | 6.3 | 12 | 10 | 8 | 19 | 15 | 64 | 2 | Stable | |
| GS-M-03 | 8.4 | 4 | 18 | 10 | 20 | 15 | 67 | 3 | Stable | |
| GS-M-04 | 7 | 4 | 16 | 9 | 18 | 15 | 62 | 4 | Stable | |
| Gallardo | GS-G-01 | 11 | 3 | 16 | 10 | 10 | 10 | 49 | 5 | Stable |
| GS-G-02 | 5.8 | 3 | 16 | 10 | 10 | 10 | 49 | 6 | Stable | |
| GS-G-03 | 5 | 3 | 16 | 10 | 10 | 10 | 49 | 7 | Stable | |
| GS-G-04 | 5.8 | 3 | 17 | 9 | 10 | 4 | 43 | 8 | Stable | |
| GS-G-05 | 5 | 3 | 15 | 11 | 10 | 4 | 43 | 9 | Stable | |
| GS-G-06 | 8.9 | 2 | 17 | 10 | 11 | 4 | 44 | 10 | Stable | |
| GS-G-07 | 8 | 2 | 17 | 10 | 11 | 4 | 44 | 11 | Stable | |
| GS-G-08 | 6.4 | 2 | 17 | 10 | 11 | 4 | 44 | 12 | Stable | |
| GS-G-09 | 8 | 3 | 17 | 10 | 11 | 7 | 48 | 13 | Stable | |
| GS-G-10 | 8.5 | 3 | 20 | 11 | 11 | 5 | 50 | 14 | Unstable | |
| GS-G-11 | 10 | 3 | 20 | 11 | 11 | 7 | 52 | 15 | Unstable | |
| GS-G-12 | 4.8 | 3 | 20 | 11 | 11 | 7 | 52 | 16 | Stable | |
| GS-G-12b | 6 | 3 | 20 | 11 | 11 | 7 | 52 | 16 | Stable | |
| GS-G-13 | 6.6 | 3 | 20 | 11 | 11 | 7 | 52 | 17 | Unstable | |
| GS-G-14 | 6.5 | 3 | 19 | 16 | 12 | 7 | 57 | 18 | Stable | |
| GS-G-15 | 7 | 3 | 19 | 10 | 22 | 7 | 61 | 19 | Stable | |
| Galla-Zoila | GS-GZ-01 | 4.3 | 4 | 16 | 20 | 20 | 4 | 59 | 20 | Stable |
| GS-GZ-02 | 4.8 | 4 | 16 | 20 | 20 | 4 | 59 | 21 | Stable | |
| GS-GZ-03 | 2.7 | 4 | 15 | 20 | 20 | 4 | 58 | 22 | Stable | |
| GS-GZ-04 | 3.2 | 4 | 15 | 21 | 21 | 7 | 62 | 23 | Stable | |
| GS-GZ-05 | 4.5 | 2 | 17 | 19 | 19 | 10 | 63 | 24 | Stable | |
| GS-GZ-06 | 4.5 | 2 | 12 | 19 | 19 | 10 | 50 | 25 | Stable | |
| GS-GZ-07 | 3.3 | 2 | 12 | 19 | 19 | 10 | 50 | 26 | Stable | |
| GS-GZ-08 | 4.5 | 2 | 20 | 16 | 16 | 7 | 56 | 27 | Unstable | |
| GS-GZ-09 | 4 | 2 | 13 | 12 | 12 | 7 | 41 | 28 | Stable | |
| GS-GZ-10 | 4.5 | 2 | 13 | 12 | 12 | 7 | 41 | 29 | Stable | |
| GS-GZ-11 | 3.7 | 2 | 15 | 14 | 14 | 7 | 53 | 30 | Unstable | |
| GS-GZ-12 | 4.2 | 4 | 15 | 18 | 18 | 7 | 51 | 31 | Unstable | |
| GS-GZ-13 | 3.6 | 4 | 20 | 20 | 20 | 10 | 69 | 32 | Stable | |
| GS-GZ-14 | 2.7 | 4 | 20 | 20 | 20 | 10 | 69 | 33 | Stable | |
| GS-GZ-15 | 3.1 | 4 | 20 | 20 | 20 | 10 | 69 | 34 | Stable | |
| GS-GZ-16 | 2.57 | 4 | 10 | 18 | 18 | 7 | 46 | 35 | Stable | |
| Cave | Geo- Mechanical Station | Dimensions | Cave Geomechanical Index | Code | Type | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Width | Span Length | Area | Perimeter | Hydraulic Radius | Ceiling Shape | Ceiling Thickness | RMR Weight | CT Weight | HR Weight | CS Weight | CGI | ||||
| (m) | (m) | (m2) | (m) | (m) | (m) | (%) | |||||||||
| Mirador | GS-M-01 | 6.4 | 19 | 121.6 | 50.8 | 2.394 | Planar | 1 | 30 | 0 | 0 | 4 | 34 | 1 | Stable |
| GS-M-02 | 6.3 | 19 | 119.7 | 50.6 | 2.366 | Planar | 3.6 | 45 | 2 | 0 | 4 | 51 | 2 | Stable | |
| GS-M-03 | 8.4 | 24.8 | 208.32 | 66.4 | 3.137 | Arch | 1.5 | 45 | 0 | 0 | 10 | 55 | 3 | Stable | |
| GS-M-04 | 7 | 48 | 336 | 110 | 3.055 | Planar | 1 | 45 | 0 | 0 | 4 | 49 | 4 | Stable | |
| 11 | 25 | 275 | 72 | 3.819 | Planar | 5 | 30 | 2 | 0 | 4 | 36 | Stable | |||
| Gallardo | GS-G-01 | 5.8 | 61 | 353.8 | 133.6 | 2.648 | Arch | N/A | 30 | N/A | 0 | 10 | N/A | 5 | |
| GS-G-02 | 5 | N/A | N/A | N/A | N/A | Planar | N/A | 30 | N/A | N/A | 4 | N/A | 6 | ||
| GS-G-03 | 5.8 | 100 | 580 | 211.6 | 2.741 | Planar | N/A | 30 | N/A | 0 | 4 | N/A | 7 | ||
| GS-G-04 | 5 | 50 | 250 | 110 | 2.273 | Planar | N/A | 30 | N/A | 0 | 4 | N/A | 8 | ||
| GS-G-05 | 8.9 | 47 | 418.3 | 111.8 | 3.742 | Planar | 10 | 30 | 5 | 0 | 4 | 39 | 9 | Unstable | |
| GS-G-06 | 8 | N/A | N/A | N/A | N/A | Arch | 10.5 | 30 | 5 | N/A | 10 | N/A | 10 | ||
| GS-G-07 | 6.4 | N/A | N/A | N/A | N/A | Planar | 9.8 | 30 | 5 | N/A | 4 | N/A | 11 | ||
| GS-G-08 | 6.4 | N/A | N/A | N/A | N/A | Planar | 10.5 | 30 | 5 | N/A | 4 | N/A | 12 | ||
| GS-G-09 | 8 | N/A | N/A | N/A | N/A | Planar | 9.8 | 30 | 5 | N/A | 4 | N/A | 13 | ||
| GS-G-10 | 8.5 | 55 | 467.5 | 127 | 3.681 | Planar | 5.6 | 30 | 2 | 0 | 4 | 36 | 14 | Unstable | |
| GS-G-11 | 10 | N/A | N/A | N/A | N/A | Planar | 5.6 | 30 | 2 | N/A | 4 | N/A | 15 | ||
| GS-G-12 | 4.8 | 40 | 192 | 89.6 | 2.143 | Arch | 5.6 | 30 | 2 | 0 | 10 | 42 | 16 | Stable | |
| 6 | 40 | 240 | 92 | 2.609 | Arch | 5.6 | 30 | 2 | 0 | 10 | 42 | 16 | Stable | ||
| GS-G-13 | 6.6 | N/A | N/A | N/A | N/A | Planar | 5.5 | 30 | 2 | N/A | 4 | N/A | 17 | ||
| GS-G-14 | 6.5 | 80 | 520 | 173 | 3.006 | Arch | 5.3 | 30 | 2 | 0 | 10 | 42 | 18 | Stable | |
| GS-G-15 | 7 | 40 | 280 | 94 | 2.979 | Arch | 2 | 45 | 0 | 0 | 10 | 55 | 19 | Stable | |
| 7 | 40 | 280 | 94 | 2.979 | Arch | 3.8 | 45 | 2 | 0 | 10 | 57 | 19 | Stable | ||
| Galla-Zoila | GS-GZ-01 | 4.3 | 40 | 172 | 88.6 | 1.941 | Arch | 14.6 | 30 | 5 | 0 | 10 | 45 | 20 | Stable |
| GS-GZ-02 | 4.8 | 40 | 192 | 89.6 | 2.143 | Arch | 14.7 | 30 | 5 | 0 | 10 | 45 | 21 | Stable | |
| GS-GZ-03 | 2.7 | 40 | 108 | 85.4 | 1.265 | Arch | 18.8 | 30 | 5 | 15 | 10 | 60 | 22 | Stable | |
| GS-GZ-04 | 3.2 | 40 | 128 | 86.4 | 1.481 | Arch | 15.2 | 45 | 5 | 15 | 10 | 75 | 23 | Stable | |
| GS-GZ-05 | 4.5 | N/A | N/A | N/A | N/A | Arch | 12.2 | 45 | 5 | N/A | 10 | N/A | 24 | ||
| GS-GZ-06 | 4.5 | N/A | N/A | N/A | N/A | Arch | 9.6 | 30 | 5 | N/A | 10 | N/A | 25 | ||
| GS-GZ-07 | 3.3 | N/A | N/A | N/A | N/A | Arch | 12.8 | 30 | 5 | N/A | 10 | N/A | 26 | ||
| GS-GZ-08 | 4.5 | 10 | 45 | 29 | 1.552 | Arch | 13.1 | 30 | 5 | 15 | 10 | 60 | 27 | Unstable | |
| GS-GZ-09 | 4 | N/A | N/A | N/A | N/A | Arch | 9.7 | 30 | 5 | N/A | 10 | N/A | 28 | ||
| GS-GZ-10 | 4.5 | N/A | N/A | N/A | N/A | N/A | 4.7 | 30 | 2 | N/A | N/A | N/A | 29 | ||
| GS-GZ-11 | 3.7 | 30 | 111 | 67.4 | 1.647 | Arch | 9.9 | 30 | 5 | 15 | 10 | 60 | 30 | Unstable | |
| GS-GZ-12 | 4.2 | N/A | N/A | N/A | N/A | Arch | 10.6 | 30 | 5 | 0 | 10 | 45 | 31 | Unstable | |
| GS-GZ-13 | 3.6 | 40 | 144 | 87.2 | 1.651 | Planar | 11.4 | 45 | 5 | 15 | 4 | 69 | 32 | Stable | |
| GS-GZ-14 | 2.7 | N/A | N/A | N/A | N/A | Arch | 7.5 | 45 | 2 | N/A | 10 | N/A | 33 | ||
| GS-GZ-15 | 3.1 | 40 | 124 | 86.2 | 1.439 | Arch | 2.6 | 45 | 0 | 15 | 10 | 70 | 34 | Stable | |
| GS-GZ-16 | 2.57 | 40 | 102.8 | 85.14 | 1.207 | N/A | 3.5 | 30 | 2 | 15 | N/A | N/A | 35 | ||
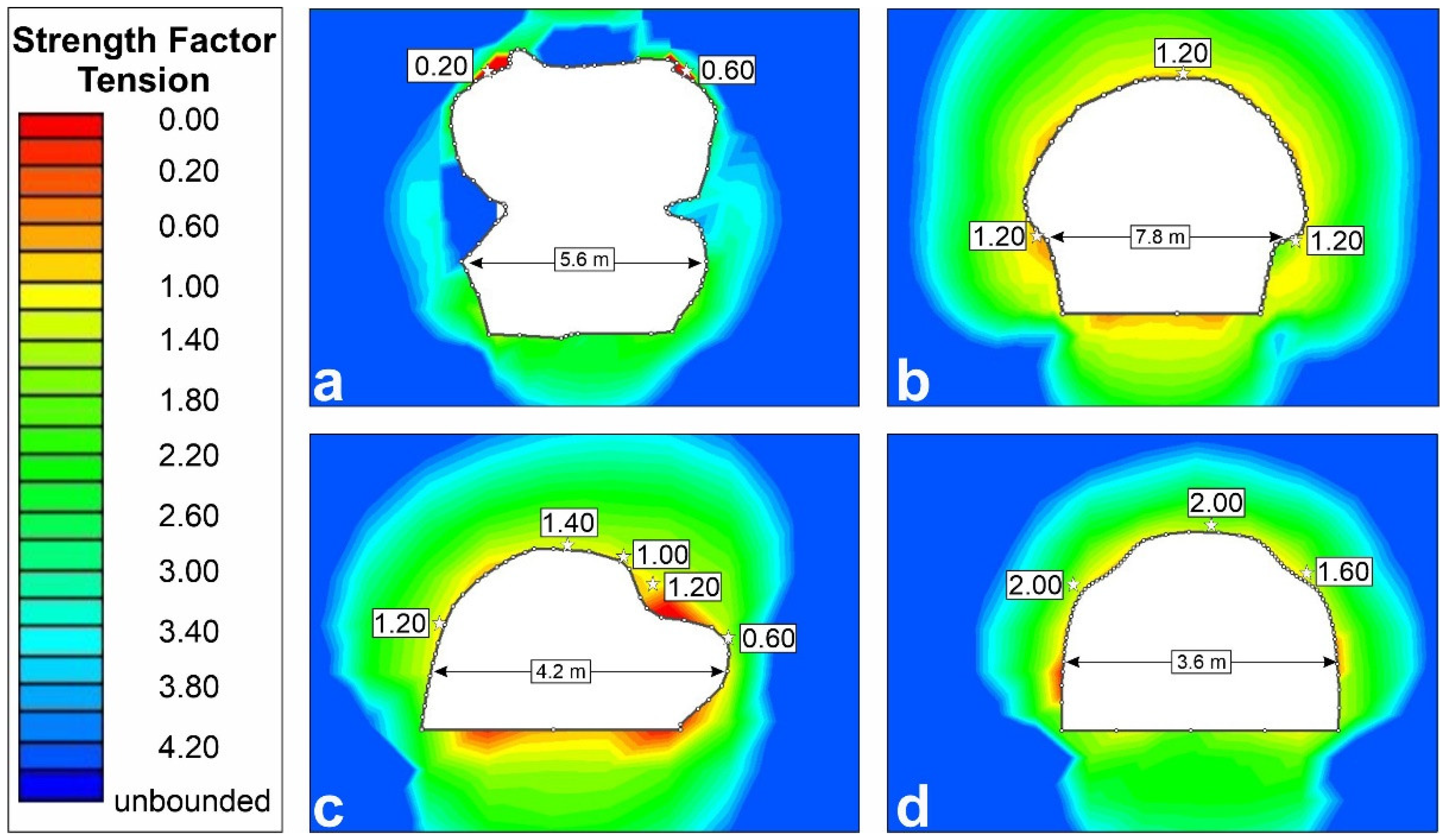
| Cave | Width (m) | Height (m) | Ceiling | Overburden Unit Weight (MN/m3) | Em (MPa) | Poisson Ratio | Intact Comp. Strength (MPa) | GSI | mi | D | F. S |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Thickness (m) | |||||||||||
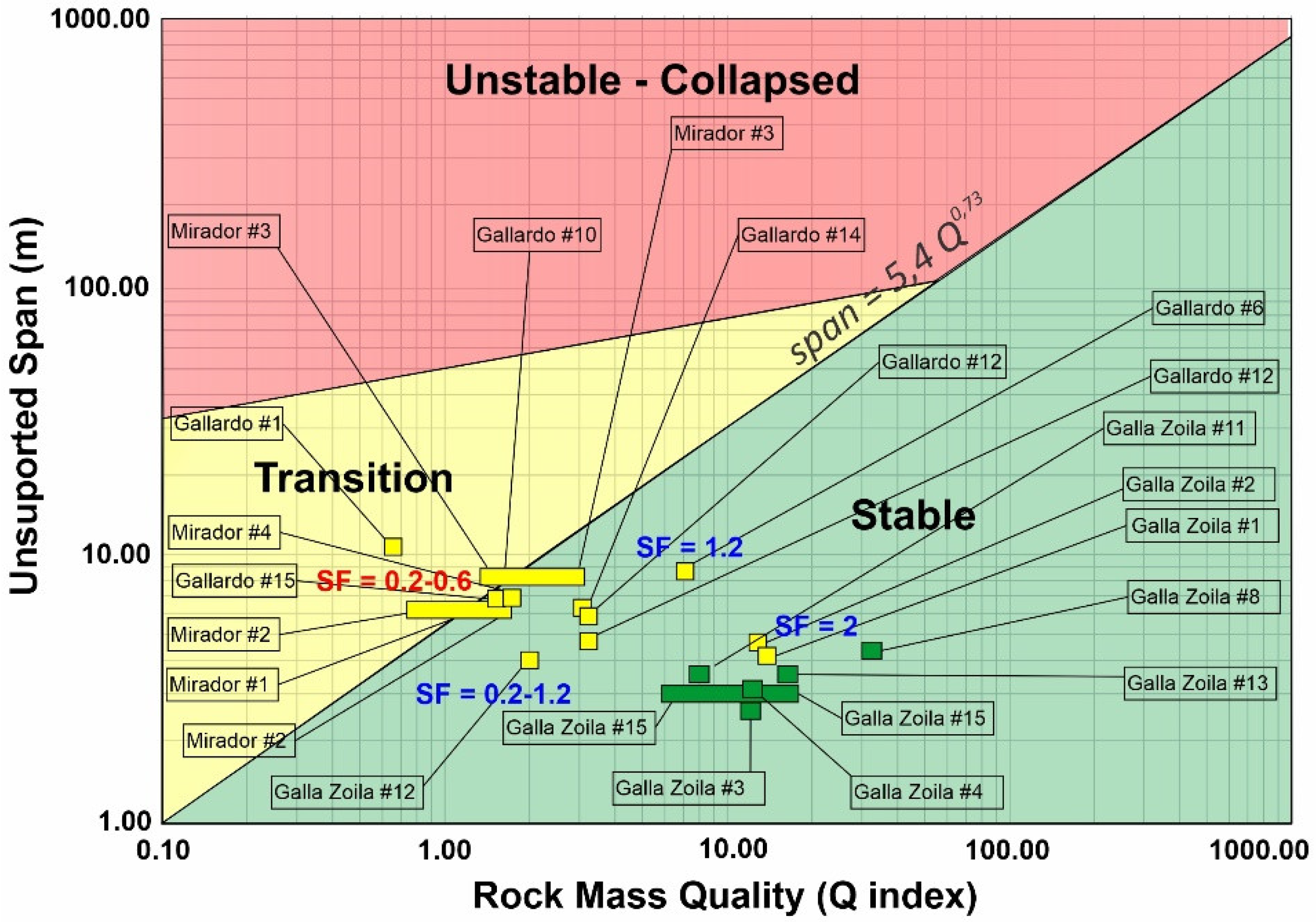
| Mirador GS-M-02 | 6.3 | 6.6 | 3.6 | 0.026 | 12,000 | 0.32 | 45 | 79 | 25 | 0 | 0.2–0.6 |
| Gallardo GS-G-06 | 8.9 | 7.5 | 10 | 0.028 | 6668 | 0.32 | 37.3 | 60 | 25 | 0 | 1.2 |
| Galla-Zoila GS-GZ-12 | 4.2 | 2.6 | 10.6 | 0.028 | 6668 | 0.32 | 27.5 | 70 | 25 | 0 | 0.2–1.2 |
| Galla-Zoila GS-GZ-13 | 3.6 | 2.6 | 11.4 | 0.028 | 6668 | 0.32 | 27.5 | 80 | 25 | 0 | 2 |
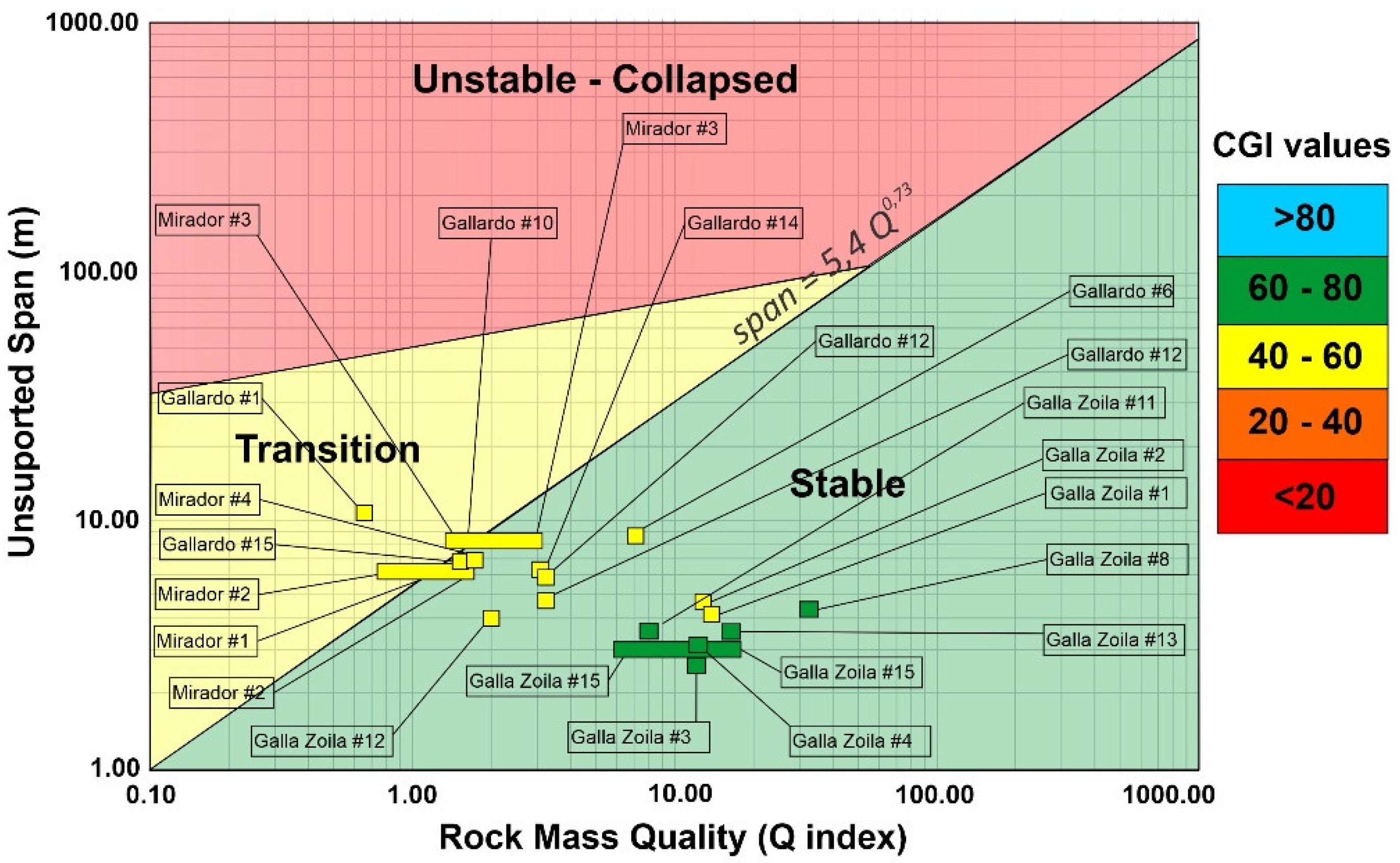
| Cave | Geo- Mechanical Station | Q Index | CGI | Numerical Modeling | Visual Description Type |
|---|---|---|---|---|---|
| Mirador | GS-M-01 | Stable | Unstable | N/A | Stable |
| GS-M-02 | Stable | Transition | Unstable | Stable | |
| GS-M-03 | Transition | Transition | N/A | Stable | |
| GS-M-04 | Stable | Transition | N/A | Stable | |
| Gallardo | GS-G-01 | Transition | Unstable | N/A | Stable |
| GS-G-02 | Stable | N/A | N/A | Stable | |
| GS-G-03 | Stable | N/A | N/A | Stable | |
| GS-G-04 | Stable | N/A | N/A | Stable | |
| GS-G-05 | Stable | N/A | N/A | Stable | |
| GS-G-06 | Stable | Unstable | Stable | Stable | |
| GS-G-07 | Stable | N/A | N/A | Stable | |
| GS-G-08 | Stable | N/A | N/A | Stable | |
| GS-G-09 | Stable | N/A | N/A | Stable | |
| GS-G-10 | Transition | Unstable | N/A | Unstable | |
| GS-G-11 | Transition | N/A | N/A | Unstable | |
| GS-G-12 | Stable | Transition | N/A | Stable | |
| GS-G-13 | Stable | N/A | N/A | Unstable | |
| GS-G-14 | Stable | Transition | N/A | Stable | |
| GS-G-15 | Stable | Transition | N/A | Stable | |
| Galla-Zoila | GS-GZ-01 | Stable | Transition | N/A | Stable |
| GS-GZ-02 | Stable | Transition | N/A | Stable | |
| GS-GZ-03 | Stable | Stable | N/A | Stable | |
| GS-GZ-04 | Stable | Stable | N/A | Stable | |
| GS-GZ-05 | Stable | N/A | N/A | Stable | |
| GS-GZ-06 | Stable | N/A | N/A | Stable | |
| GS-GZ-07 | Stable | N/A | N/A | Stable | |
| GS-GZ-08 | Stable | Stable | N/A | Unstable | |
| GS-GZ-09 | Stable | N/A | N/A | Stable | |
| GS-GZ-10 | Stable | N/A | N/A | Stable | |
| GS-GZ-11 | Stable | Stable | N/A | Unstable | |
| GS-GZ-12 | Stable | Transition | Unstable | Unstable | |
| GS-GZ-13 | Stable | Stable | Stable | Stable | |
| GS-GZ-14 | Stable | N/A | N/A | Stable | |
| GS-GZ-15 | Stable | Stable | N/A | Stable | |
| GS-GZ-16 | Stable | N/A | N/A | Stable |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bastidas, G.; Soria, O.; Mulas, M.; Loaiza, S.; Bordehore, L.J. Stability Analysis of Lava Tunnels on Santa Cruz Island (Galapagos Islands, Ecuador) Using Rock Mass Classifications: Empirical Approach and Numerical Modeling. Geosciences 2022, 12, 380. https://doi.org/10.3390/geosciences12100380
Bastidas G, Soria O, Mulas M, Loaiza S, Bordehore LJ. Stability Analysis of Lava Tunnels on Santa Cruz Island (Galapagos Islands, Ecuador) Using Rock Mass Classifications: Empirical Approach and Numerical Modeling. Geosciences. 2022; 12(10):380. https://doi.org/10.3390/geosciences12100380
Chicago/Turabian StyleBastidas, Gilmar, Oliver Soria, Maurizio Mulas, Silvia Loaiza, and Luis Jordá Bordehore. 2022. "Stability Analysis of Lava Tunnels on Santa Cruz Island (Galapagos Islands, Ecuador) Using Rock Mass Classifications: Empirical Approach and Numerical Modeling" Geosciences 12, no. 10: 380. https://doi.org/10.3390/geosciences12100380
APA StyleBastidas, G., Soria, O., Mulas, M., Loaiza, S., & Bordehore, L. J. (2022). Stability Analysis of Lava Tunnels on Santa Cruz Island (Galapagos Islands, Ecuador) Using Rock Mass Classifications: Empirical Approach and Numerical Modeling. Geosciences, 12(10), 380. https://doi.org/10.3390/geosciences12100380








