Theoretical Investigation Applied to Scattering Water Waves by Rectangular Submerged Obstacles/and Submarine Trenches
Abstract
1. Introduction
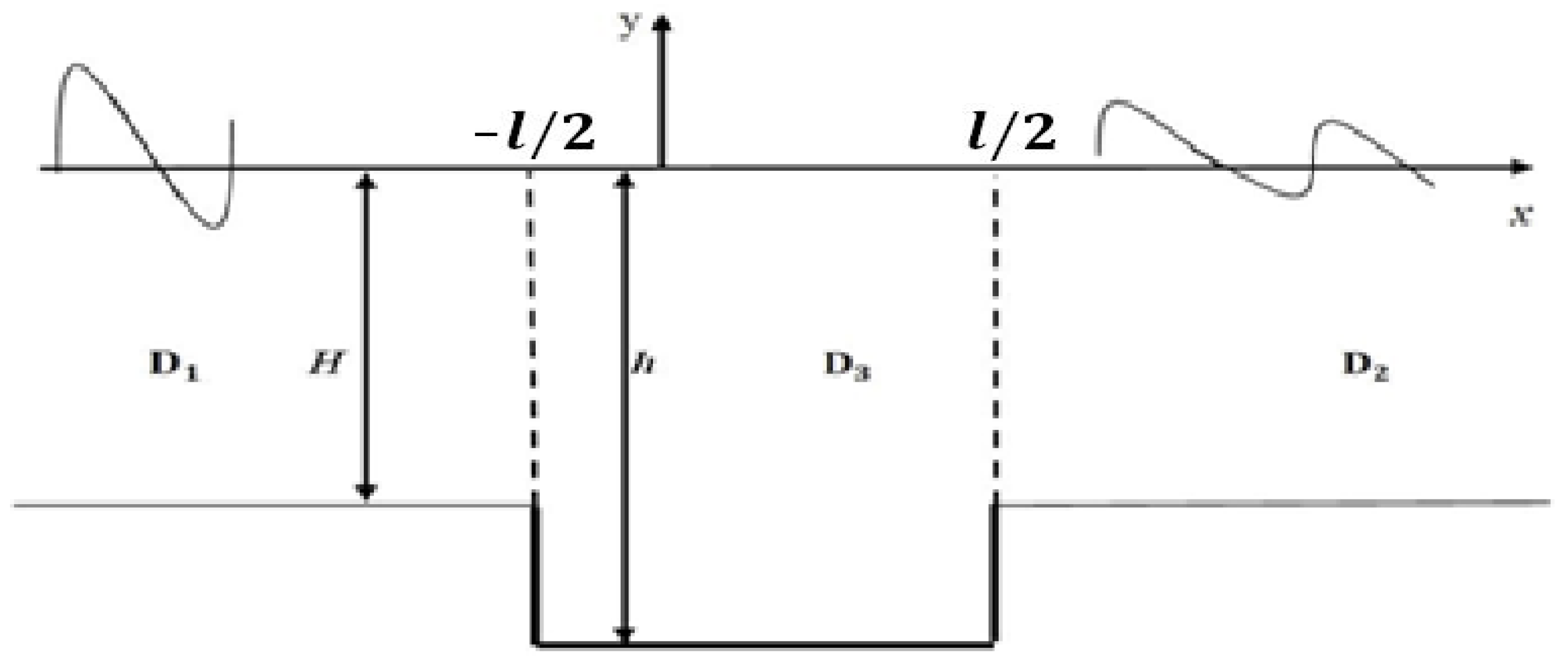
2. Theoretical Formulation of the Problem
- -
- at the subdomain
- -
- at the subdomain
- -
- at the subdomain
- At the position x =
- At the position x =
3. Results and Discussions
3.1. Validation Tests
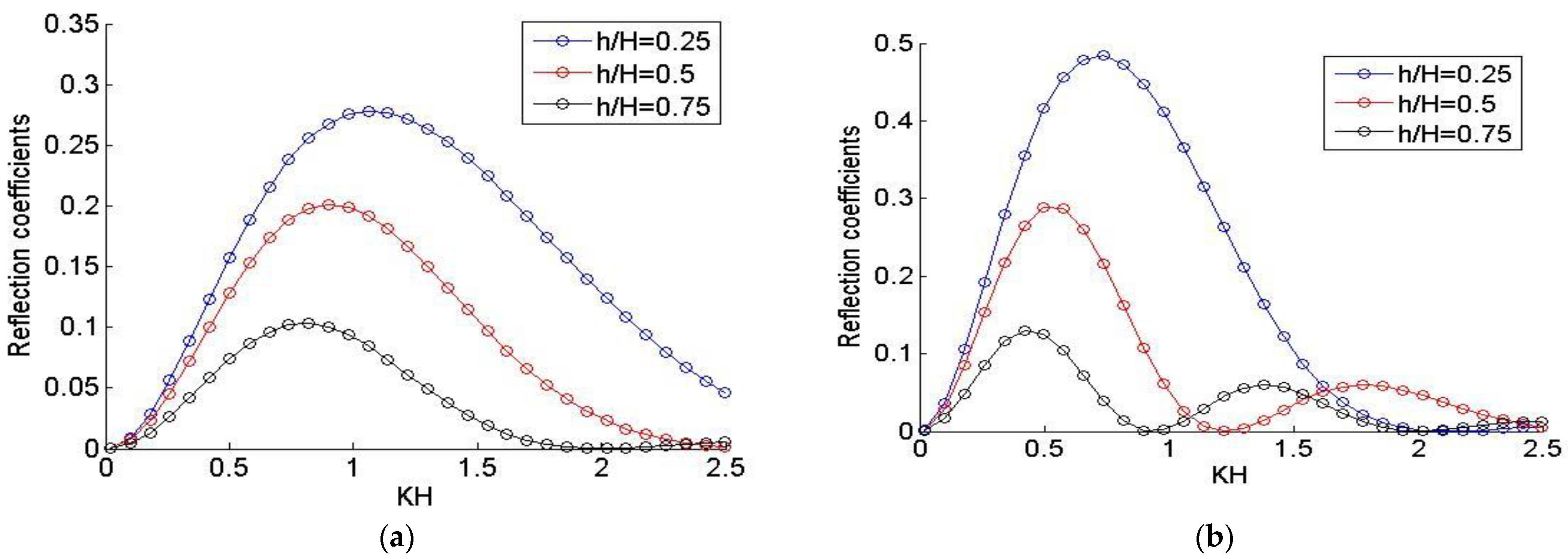
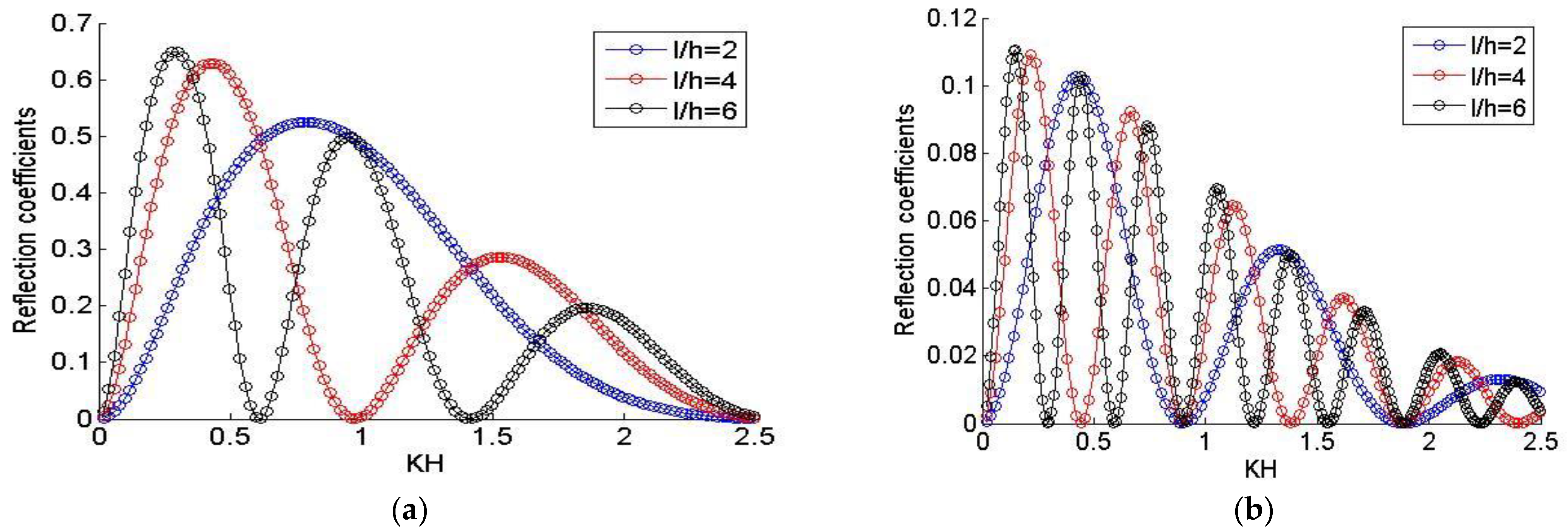
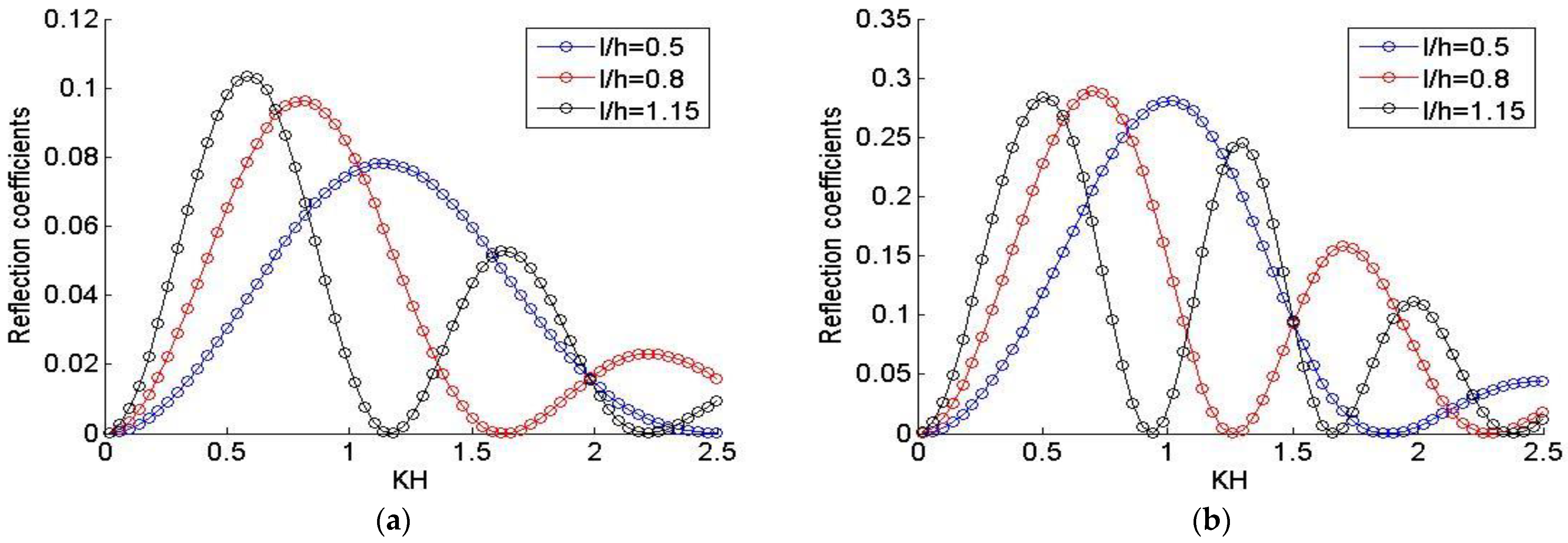
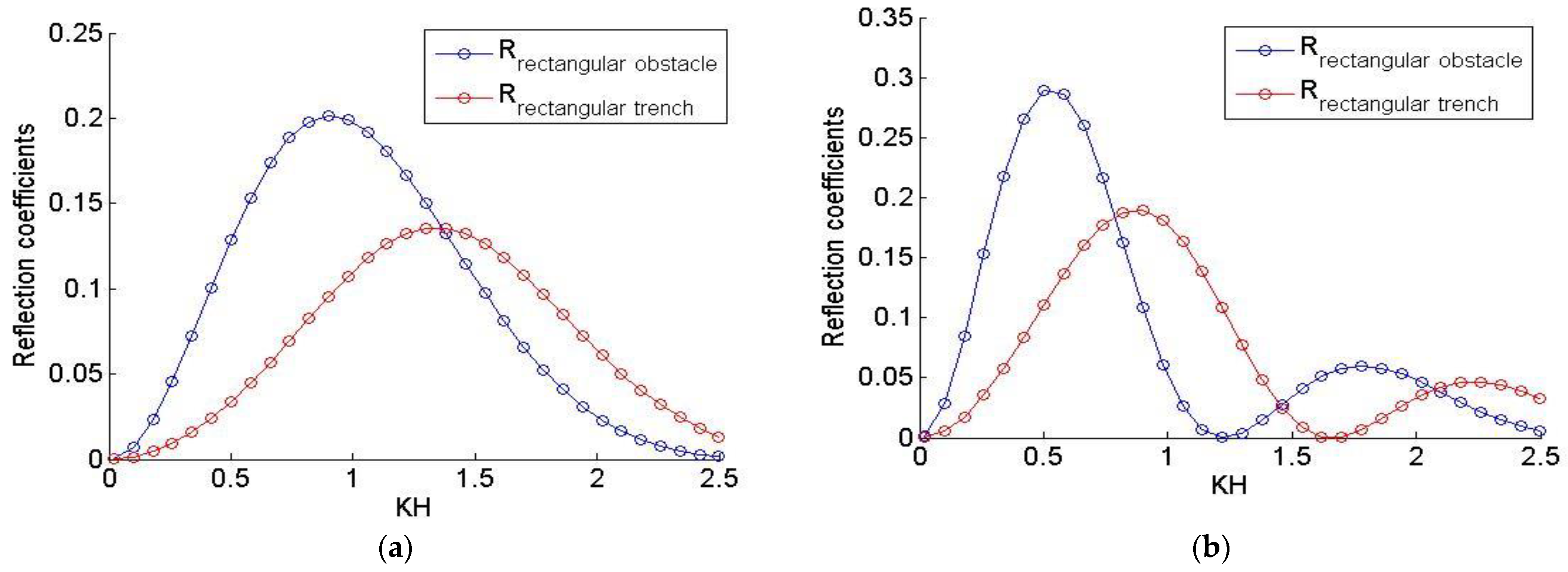
3.2. Reflection Coefficients Investigations
4. Conclusions and Perspectives
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Errifaiy, M.; Naasse, S.; Chahine, C. Analytical determination of the reflection coefficient by the evanescent modes model during the wave6—Solar Academy). Comptes Rendus Mécan. 2016, 344, 479–486. [Google Scholar] [CrossRef]
- Naasse, S.; Errifaiy, M.; Chakib, C. Analytical study of the effect of the geometrical parameters during the interaction of regular wavehorizontal plate-current. Acta Oceanol. Sin. 2019, 38, 10–20. [Google Scholar] [CrossRef]
- Loukili, M.; Dutykh, D.; Kotrasova, K.; Ning, D. Numerical Stability Investigations of the Method of Fundamental Solutions Applied to Wave-Current Interactions Using Generating-Absorbing Boundary Conditions. Symmetry 2021, 13, 1153. [Google Scholar] [CrossRef]
- Loukili, M.; Kotrasova, K.; Bouaine, A. A Generating—Absorbing Boundary Condition Applied to Wave—Current Interactions Using the Method of Fundamental Solutions. Civ. Environ. Eng. 2021, 17, 343–352. [Google Scholar] [CrossRef]
- Loukili, M.; Kotrasova, K.; Mouhid, M. Computerized Decision Aid Applied to Meshless Method for the Use Case: Wave-Structure Interactions. In Proceedings of the International Conference on Decision Aid Sciences and Application (DASA), Online, 8–9 November 2020; pp. 33–36. [Google Scholar]
- Brossard, J.; Chagdali, M. Experimental investigation of the harmonic generation by waves over a submerged plate. Coast. Eng. 2001, 42, 277–290. [Google Scholar] [CrossRef]
- Brossard, J.; Perret, G.; Blonce, L.; Diedhiou, A. Higher harmonics induced by a submerged horizontal plate and a submergedrectangular step in a wave flume. Coast. Eng. 2009, 56, 11–22. [Google Scholar] [CrossRef]
- Szmidt, K. Finite difference analysis of surface wave scattering by underwater rectangular obstacles. Arch. Hydro-Eng. Environ. Mech. 2010, 57, 179–198. [Google Scholar]
- Ouyang, H.T.; Chen, K.H.; Tsai, C.M. Wave characteristics of Bragg reflections from a train of submerged bottom breakwaters. J. Hydro-Environ. Res. 2016, 11, 91–100. [Google Scholar] [CrossRef]
- Hsu, T.W.; Chang, H.K.; Hsieh, C.M. Bragg reflection of waves by different shapes of artificial bars. China Ocean. Eng. 2002, 16, 21–30. [Google Scholar]
- Chioukh, N.; Çevik, E.; Yüksel, Y. Reflection and transmission of regular waves from/through single and double perforated thinwalls. China Ocean Eng. 2017, 31, 466–475. [Google Scholar] [CrossRef]
- Loukili, M.; Dutykh, D.; Nadjib, C.; Ning, D.; Kotrasova, K. Analytical and Numerical Investigations Applied to Study the Reflections and Transmissions of a Rectangular Breakwater Placed at the Bottom of a Wave Tank. Geosciences 2021, 11, 430. [Google Scholar] [CrossRef]
- Kreisel, H. Surface waves. Q. Appl. Math. 1949, 7, 21–44. [Google Scholar] [CrossRef]
- Lassiter, J.B. The Propagation of Water Waves over Sediment Pockets. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 1972. [Google Scholar]
- Lee, J.J.; Ayer, R.M. Wave propagation over a rectangular trench. J. Fluid Mech. 1981, 110, 335–347. [Google Scholar] [CrossRef]
- Miles, J.W. On surface wave diffraction by a trench. J. Fluid Mech. 1982, 115, 315–325. [Google Scholar] [CrossRef]
- Bender, C.J.; Dean, R.G. Wave transformation by two dimensional bathymetric anomalies with sloped transitions. Coast. Eng. 2003, 56, 61–84. [Google Scholar] [CrossRef]
- Xie, J.J.; Liu, H.W.; Lin, P. Analytical solution for long wave reflection by a rectangular obstacle with two scour trenches. ASCE J. Eng. Mech. 2011, 137, 919–930. [Google Scholar] [CrossRef]
- Liu, H.W.; Fu, D.J.; Sun, X.L. Analytic solution to the modified mild-slope equation for reflection by a rectangular breakwaterwith scour trenches. ASCE J. Eng. Mech. 2013, 139, 39–58. [Google Scholar] [CrossRef]
- Paul, S.; Sasmal, A.; De, S. Interaction of oblique waves with an ice sheet over an asymmetric trench. Ocean Eng. 2019, 193, 106613. [Google Scholar] [CrossRef]
- Sasmal, A.; De, S. Analysis of oblique wave diffraction by rectangular thick barrier in the presence of surface tension. Indian J. Phys. 2022, 96, 2051–2063. [Google Scholar] [CrossRef]
- Yang, X. Chapter 2—Analysis of Algorithms. In Nature-Inspired Optimization Algorithms; Elsevier: Amsterdam, The Netherlands, 2014; pp. 23–44. [Google Scholar]
- Kirby, J.T.; Dalrymple, R.A. Propagation of obliquely incident water waves over a trench. J. Fluid Mech. 1983, 133, 47–63. [Google Scholar] [CrossRef]
- Chakraborty, R.; Mandal, B.N. Water wave scattering by a rectangular trench. J. Eng. Math. 2014, 89, 101–112. [Google Scholar] [CrossRef]


| Kirby and Dalrymple [23] | Rumpa Chakraborty [24] | Present Work | |||||||
|---|---|---|---|---|---|---|---|---|---|
| kh | T | R | T | R | T | R | |||
| 0.341 | 0.888 | 0.459 | 0.999 | 0.889 | 0.469 | 1.010 | 0.8905 | 0.497 | 1.040 |
| 0.723 | 0.955 | 0.296 | 1.000 | 0.956 | 0.295 | 1.001 | 0.9575 | 0.302 | 1.008 |
| 1.296 | 0.999 | 0.030 | 0.999 | 0.998 | 0.031 | 0.997 | 0.9985 | 0.034 | 0.998 |
| Numerical [12] | Analytical | ||||||
|---|---|---|---|---|---|---|---|
| h/H | kh | T | R | T | R | ||
| 0.25 | 0.100 | 0.938 | 0.345 | 0.998 | 0.932 | 0.332 | 0.979 |
| 0.360 | 0.810 | 0.585 | 0.998 | 0.832 | 0.540 | 0.984 | |
| 0.780 | 0.958 | 0.284 | 0.998 | 0.969 | 0.242 | 0.999 | |
| 0.940 | 0.877 | 0.479 | 0.998 | 0.869 | 0.453 | 0.961 | |
| 0.5 | 0.100 | 0.991 | 0.133 | 0.999 | 0.978 | 0.099 | 0,968 |
| 0.360 | 0.947 | 0.320 | 0.999 | 0.950 | 0.292 | 0.989 | |
| 0.780 | 0.992 | 0.125 | 0.999 | 0.982 | 0.101 | 0.975 | |
| 0.94 | 1.000 | 0.006 | 1.000 | 0.970 | 0.004 | 0.941 | |
| 0.75 | 0.100 | 0.998 | 0.049 | 0.998 | 0.993 | 0.030 | 0.987 |
| 0.360 | 0.991 | 0.128 | 0.998 | 0.986 | 0.099 | 0.982 | |
| 0.780 | 0.995 | 0.090 | 0.998 | 0.981 | 0.069 | 0.969 | |
| 0.940 | 0.998 | 0.060 | 0.999 | 0.993 | 0.058 | 0.991 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Loukili, M.; Dutykh, D.; Pincemin, S.; Kotrasova, K.; Abcha, N. Theoretical Investigation Applied to Scattering Water Waves by Rectangular Submerged Obstacles/and Submarine Trenches. Geosciences 2022, 12, 379. https://doi.org/10.3390/geosciences12100379
Loukili M, Dutykh D, Pincemin S, Kotrasova K, Abcha N. Theoretical Investigation Applied to Scattering Water Waves by Rectangular Submerged Obstacles/and Submarine Trenches. Geosciences. 2022; 12(10):379. https://doi.org/10.3390/geosciences12100379
Chicago/Turabian StyleLoukili, Mohammed, Denys Dutykh, Sandrine Pincemin, Kamila Kotrasova, and Nizar Abcha. 2022. "Theoretical Investigation Applied to Scattering Water Waves by Rectangular Submerged Obstacles/and Submarine Trenches" Geosciences 12, no. 10: 379. https://doi.org/10.3390/geosciences12100379
APA StyleLoukili, M., Dutykh, D., Pincemin, S., Kotrasova, K., & Abcha, N. (2022). Theoretical Investigation Applied to Scattering Water Waves by Rectangular Submerged Obstacles/and Submarine Trenches. Geosciences, 12(10), 379. https://doi.org/10.3390/geosciences12100379











