Probability Methods for Stability Design of Open Pit Rock Slopes: An Overview
Abstract
:1. Introduction
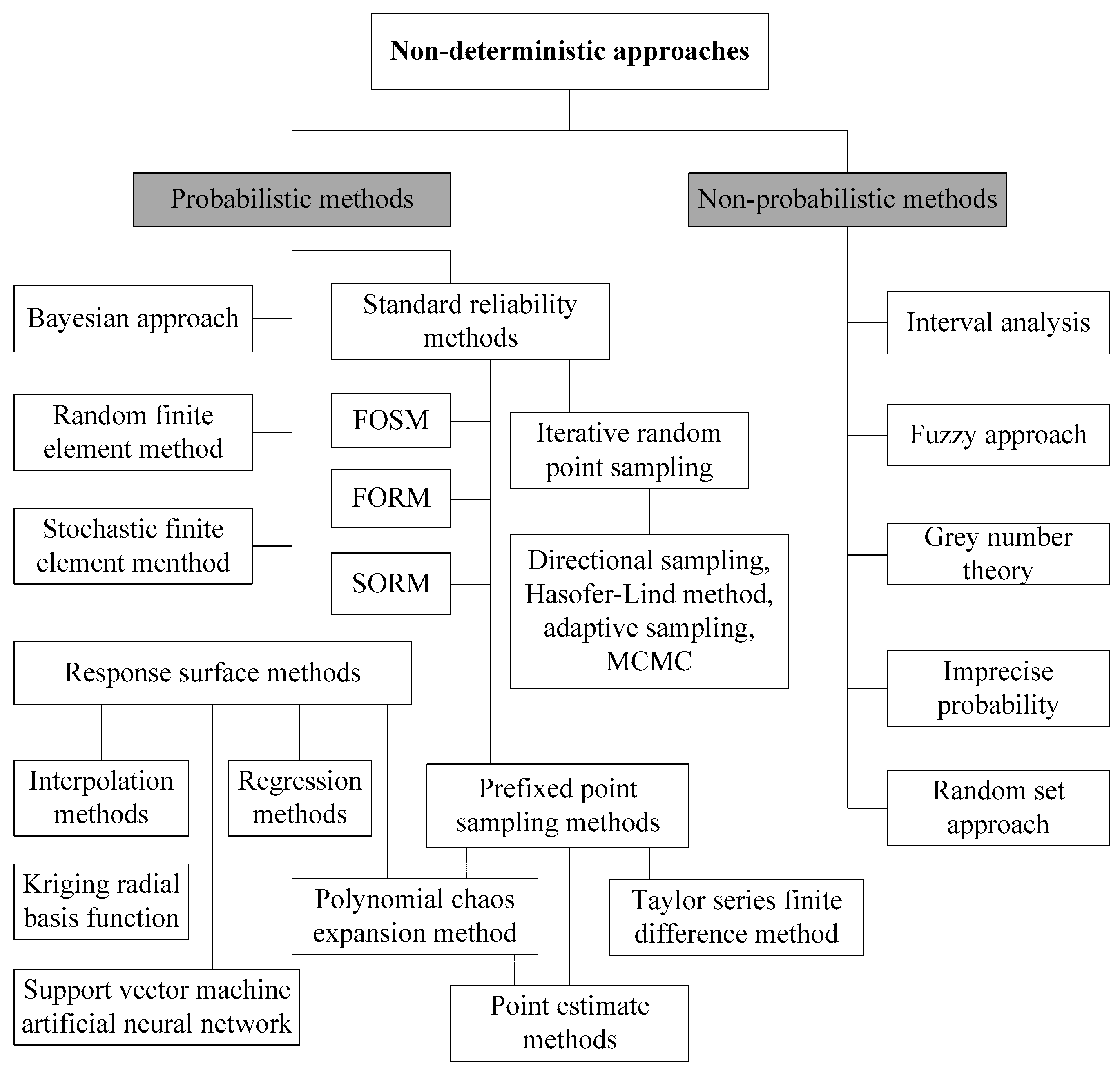
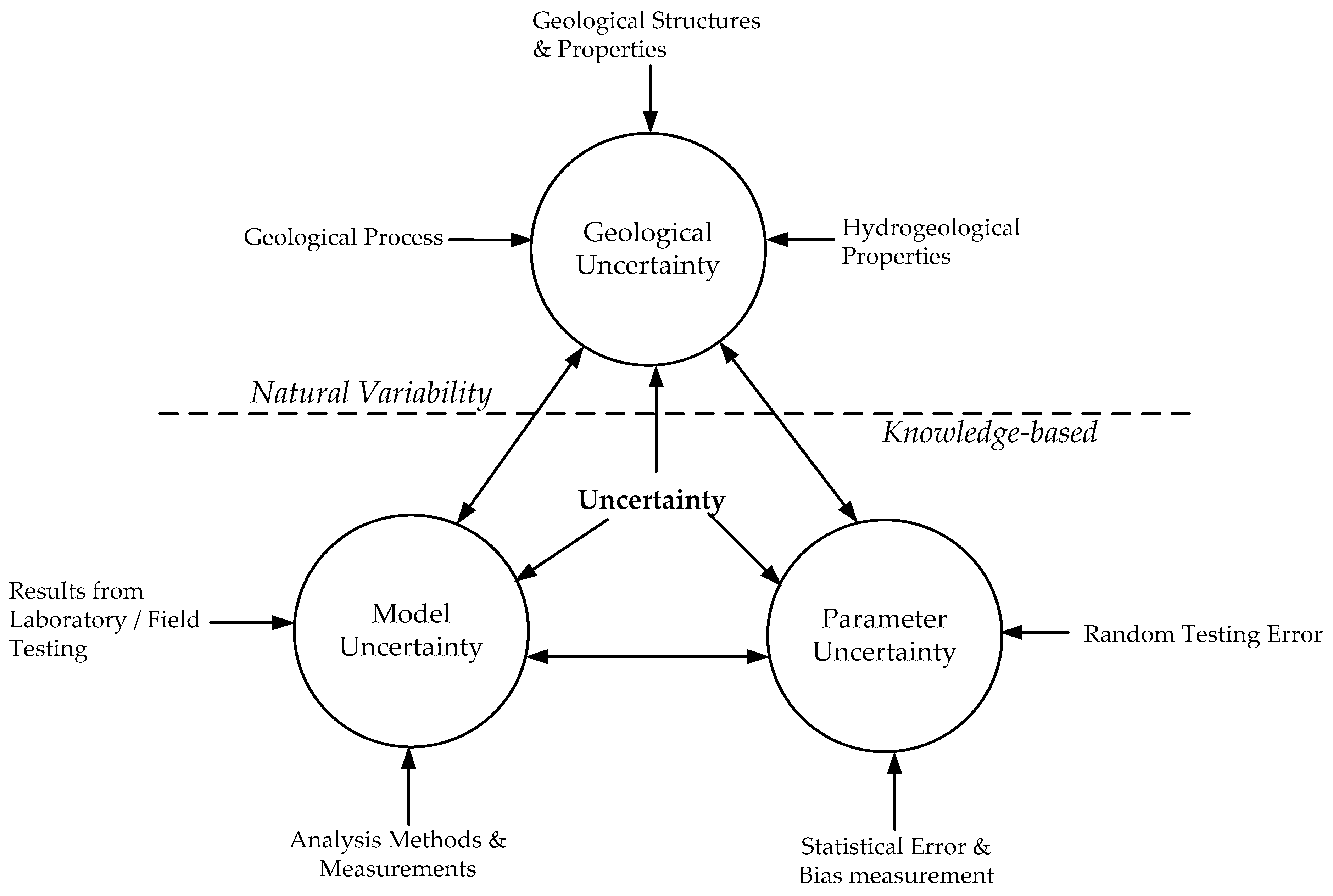
2. Uncertainty in Rock Slope Engineering and Methods of Quantification
3. Review of Reliability-Based Methods
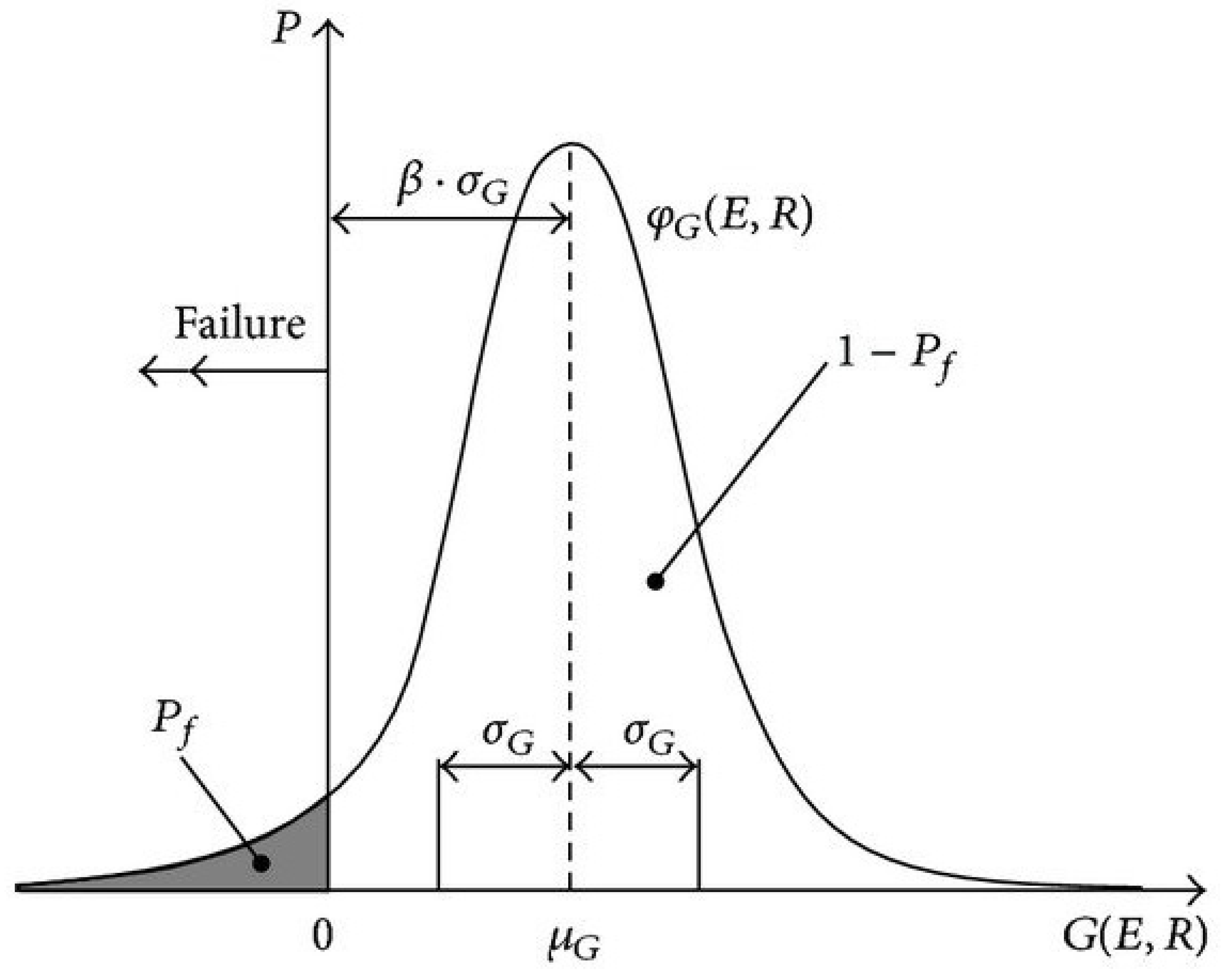
3.1. Reliability Index and Probability of Failure
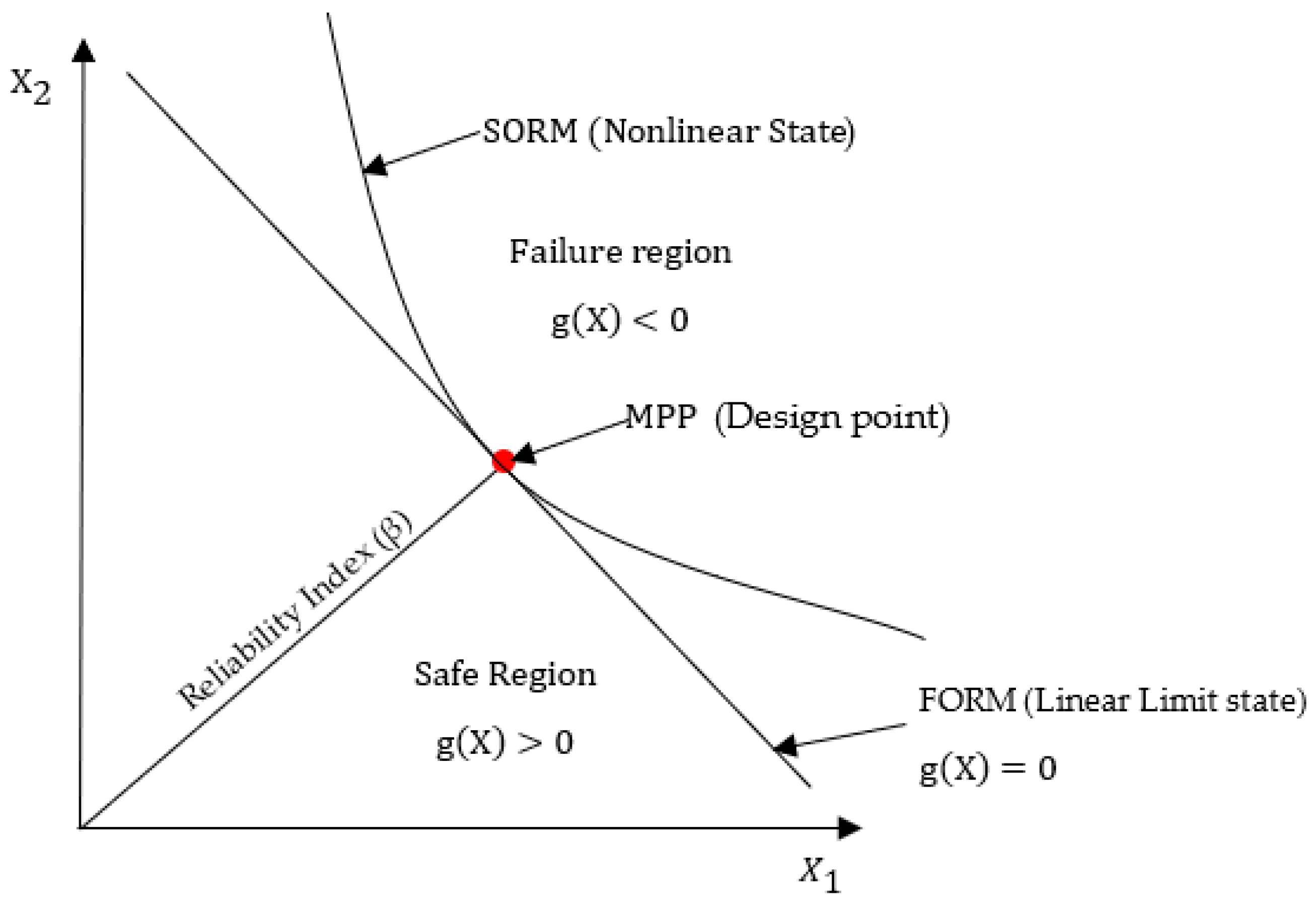
3.2. Reliability Methods
3.2.1. First Order Second Moment
3.2.2. Second-Order Reliability Method
3.2.3. Point Estimate Method
3.2.4. Monte Carlo Simulation
3.2.5. Response Surface Method
4. Overview of Cuckoo and Particle Search Optimization
4.1. Cuckoo Search Optimization Method
- Each cuckoo lays one egg at a time and dumps it in a randomly chosen nest.
- The best nests with high-quality eggs will carry over to the next generations.
- The number of available host nests is fixed, and hosts can discover an alien egg with a probability pa ∈ (0, 1).
4.2. Particle Swarm Optimization Method
5. Adopted Methods
5.1. Limit Equilibrium Analysis with Monte Carlo Simulation and the Response Surface Method
5.2. Finite Element Shear Strength Reduction Method
6. Application to Case Study
7. Results
8. Discussion
9. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Steffen, O.K.H.; Contreras, L.F.; Terbrugge, P.J.; Venter, J. A Risk Evaluation Approach for Pit Slope Design. In Proceedings of the 42nd U.S. Rock Mechanics Symposium (USRMS), San Francisco, CA, USA, 29 June–2 July 2008. [Google Scholar]
- Ali, M.A.; Morteza, O. Determination and stability analysis of ultimate open-pit slope under geomechanical uncertainty. Int. J. Min. Sci. Technol. 2014, 24, 105–110. [Google Scholar] [CrossRef]
- Zevgolis, I.E.; Deliveris, A.V.; Koukouzas, N.C. Probabilistic design optimization and simplified geotechnical risk analysis for large open pit excavations. Comput. Geotech. 2018, 103, 153–164. [Google Scholar] [CrossRef]
- Obregon, C.; Mitri, H. Probabilistic approach for open pit bench slope stability analysis—A mine case study. Int. J. Min. Sci. Technol. 2019, 29, 629–640. [Google Scholar] [CrossRef]
- Basahel, H.; Mitri, H. Probabilistic assessment of rock slopes stability using the response surface approach—A case study. Int. J. Min. Sci. Technol. 2019, 29, 357–370. [Google Scholar] [CrossRef]
- Santos, T.B.D.; Lana, M.S.; Pereira, T.M.; Canbulat, I. Quantitative hazard assessment system (Has-Q) for open pit mine slopes. Int. J. Min. Sci. Technol. 2019, 29, 419–427. [Google Scholar] [CrossRef]
- Christian, J.T.; Ladd, C.C.; Baecher, G.B. Reliability applied to slope stability analysis. J. Geotech. Eng. 1994, 120, 2180–2207. [Google Scholar] [CrossRef]
- Low, B.K. Reliability analysis of rock wedges. J. Geotech. Geoenviron. Eng. 1997, 123, 498–505. [Google Scholar] [CrossRef]
- Low, B.K. Reliability analysis of rock slopes involving correlated non-normals. Int. J. Rock Mech. Min. 2007, 44, 922–935. [Google Scholar] [CrossRef]
- Park, H.; West, T.R. Development of a probabilistic approach for rock wedge failure. Eng. Geol. 2001, 59, 233–251. [Google Scholar] [CrossRef]
- Jimenez-Rodriguez, R.; Sitar, N.; Chacon, J. System reliability approach to rock slope stability. Int. J. Rock Mech. Min. Sci. 2006, 43, 847–859. [Google Scholar] [CrossRef]
- Johari, A.; Javadi, A.A. Reliability assessment of infinite slope stability using jointly distributed random variable. Sci. Iran. A 2012, 19, 423–429. [Google Scholar] [CrossRef] [Green Version]
- Aladejare, A.E.; Akeju, V.O. Design and Sensitivity Analysis of Rock Slope Using Monte Carlo Simulation. Geotech. Geol. Eng. 2020, 38, 573–585. [Google Scholar] [CrossRef] [Green Version]
- Baecher, G.B.; Christian, J.T. Reliability and Statistics in Geotechnical Engineering; Wiley: Chichester, UK, 2003. [Google Scholar]
- Duzgun, H.S.B.; Yucemen, M.S.; Karpuz, C. A probabilistic model for the assessment of uncertainties in the shear strength of rock discontinuities. Int. J. Rock Mech. Min. Sci. 2002, 39, 743–754. [Google Scholar] [CrossRef]
- Duzgun, H.S.B.; Yucemen, M.S.; Karpuz, C. A methodology for reliability-based design of rock slopes. Rock Mech. Rock Eng. 2003, 36, 95–120. [Google Scholar] [CrossRef]
- Kirsten, H.A.D. Significance of the probability of failure in slope engineering. Civ. Eng. S. Afr. 1983, 25, 17–29. [Google Scholar]
- Rackwitz, R. Reliability analysis—A review and some perspectives. Struct. Saf. 2001, 23, 365–395. [Google Scholar] [CrossRef]
- Miller, S.M.; Whyatt, J.K.; McHugh, E.L.; Australian Geomechanics Society. Applications of Point Estimate Method for Stochastic Rock Slope Engineering. In Proceedings of the Gulf Rocks 2014, 6th North America Rock Mechanics Symposium (NARMS), Houston, TX, USA, 5–9 June 2004; pp. 1–12. [Google Scholar]
- Park, H.J.; West, T.R.; Woo, I. Probabilistic analysis of rock slope stability and random properties of discontinuity parameters. Interstate Highway 40, Western North Carolina, USA. Eng. Geol. 2005, 79, 230–250. [Google Scholar] [CrossRef]
- Duzgun, H.S.B.; Bhasin, R.K. Probabilistic stability evaluation of Oppstadhornet rock slope Norway. Rock Mech. Rock Eng. 2009, 42, 724–749. [Google Scholar] [CrossRef]
- US Army Corps of Engineers. Engineering and Design: Introduction to Probability and Reliability Methods for Use in Geotechnical Engineering; Engineer Technical Letter 1110-2-547; Department of the Army: Washington, DC, USA, 1997. [Google Scholar]
- Reale, C.; Xue, J.; Pan, Z.; Gavin, K. Deterministic and probabilistic multi-modal analysis of slope stability. Comput. Geotech. 2015, 66, 172–179. [Google Scholar] [CrossRef]
- Bastidas-Arteaga, E.; Soubra., A.-H. Doctoral School—Stochastic Analysis and In-Verse Modelling; Reliability Analysis, Methods; Hicks, M.A., Cristina, J., Eds.; HAL Archives-Ouverters: Lyon, France, 2014; pp. 53–77. Available online: http://alertgeomaterials.eu (accessed on 25 May 2021).
- Hasofer, A.M.; Lind, N.C. Exact and invariance second moment code forma. J. Eng. Mech. 1974, 100, 111–121. [Google Scholar]
- Tang, W.H.; Yuecemen, M.S.; Ang, A.H.S. Probability-based short-term design of slopes. Can. Geotech. J. 1976, 13, 201–215. [Google Scholar] [CrossRef]
- Venmarcke, E.H. Reliability of earth slopes. J. Geotech. Eng. Div. 1977, 103, 1227–1246. [Google Scholar]
- Chowdhury, R.N.; Xu, D.W. Geotechnical system reliability of slopes. Reliab. Eng. Syst. Saf. 1995, 47, 141–151. [Google Scholar] [CrossRef]
- Low, B.K.; Tang, W.H. Efficient reliability evaluation using spreadsheet. J. Eng. Mech. 1997, 123, 749–752. [Google Scholar] [CrossRef]
- Low, B.K.; Gilbert, R.B.; Wright, S.G. Slope reliability analysis using generalized method of slices. J. Geotech. Geoenviron. Eng. 1998, 124, 350–362. [Google Scholar] [CrossRef]
- Low, B.K. Practical probabilistic slope stability analysis. Proc. Soil. Rock Am. 2003, 2, 2777–2784. [Google Scholar]
- Cho, S.E. Probabilistic stability analyses of slopes using the ANN-based response surface. Comput. Geotech. 2009, 36, 787–797. [Google Scholar] [CrossRef]
- Low, B.K. FORM, SORM, and spatial modeling in geotechnical engineering. Struct. Saf. 2014, 49, 56–64. [Google Scholar] [CrossRef]
- Fenton, G.A.; Griffiths, D.V. Risk Assessment in Geotechnical Engineering; Wiley: Hoboken, NJ, USA, 2008. [Google Scholar]
- Chowdhury, R.N.; Xu, D.W. Rational polynomial technique in slope stability analysis. J. Geotech. Eng. 1993, 119, 1910–1928. [Google Scholar] [CrossRef]
- Dai, Y.; Fredlund, D.G.; Stolte, W.J. A probabilistic slope stability analysis using deterministic computer software. In Probabilistic Methods in Geotechnical Engineering; CRC Press: Canberra, Australia, 1993; pp. 267–274. [Google Scholar]
- El-Ramly, H.; Morgenstern, N.R.; Cruden, D.M. Probabilistic slope stability analysis for practice. Can. Geotech. J. 2002, 39, 665–683. [Google Scholar] [CrossRef]
- Park, H.J.; Jeong, U.J.; Han, B.H.; Ro, B.D.; Shin, K.H.; Kim, J.K. The evaluation of the probability of rock wedge failure using the point estimate method and maximum likelihood estimation method. In Proceedings of the 10th IAEG International Congress, Nottingham, UK, 6–10 September 2006; p. 485. [Google Scholar]
- Park, H.J.; Um, J.G.; Woo, I.; Kim, J.W. The evaluation of the probability of rock wedge failure using the point estimate method. Environ. Earth Sci. 2011, 65, 353–361. [Google Scholar] [CrossRef]
- Xu, B.; Low, B.K. Probabilistic stability analyses of embankments based on finite-element method. J. Geotech. Geoenviron. Eng. 2006, 132, 1444–1454. [Google Scholar] [CrossRef]
- Zhang, J.; Zhang, L.M.; Tang, W.H. Slope reliability analysis considering site-specific performance information. J. Geotech. Geoenviron. Eng. 2011, 137, 227–238. [Google Scholar] [CrossRef]
- Tobutt, D.C. Monte Carlo simulation methods for slope stability. Comput. Geosci. 1982, 8, 199–208. [Google Scholar] [CrossRef]
- Husein Malkawi, A.I.; Hassan, W.F.; Abdulla, F.A. Uncertainty and reliability analysis applied to slope stability. Struct. Saf. 2000, 22, 161–187. [Google Scholar] [CrossRef]
- McMahon, B.K. A Statistical Method for the Design of Rock Slopes. In Proceedings of the 1st Australia-New Zealand Conference on Geomechanics, Melbourne, Australia, 9 August 1971; pp. 314–321. [Google Scholar]
- McMahon, B.K. Geotechnical Design in the Face of Uncertainty; Memorial Lecture; Davis, E.H., Ed.; Australian Geomechanics Society: Sydney, Australia, 1985. [Google Scholar]
- Chowdhury, R.N. Geomechanics risk model for multiple failures along rock discontinuities. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1986, 23, 337–346. [Google Scholar] [CrossRef]
- Genske, D.D.; Walz, B. Probabilistic assessment of the stability of rock slopes. Struct. Saf. 1991, 9, 179–195. [Google Scholar] [CrossRef]
- Low, B.K.; Einstein, H.H. Simplified Reliability Analysis for Wedge Mechanisms in Rock Slopes. In Proceedings of the 6th International Symposium on Landslides, Rotterdam, The Netherlands, 10 February 1992; pp. 499–507. [Google Scholar]
- Madsen, H.O.; Krenk, N.C. Methods of Structural Safety; Prentice Hall: Hoboken, NJ, USA, 1986. [Google Scholar]
- Melchers, R.E. Structural Reliability Analysis and Predictions, 2nd ed.; Wiley: Chichester, UK, 1996. [Google Scholar]
- Straub, D. (Ed.) Reliability and Optimization of Structural Systems, 1st ed.; CRC Press: Boca Raton, FL, USA, 2010. [Google Scholar] [CrossRef]
- Bishop, A.W. The use of the slip circle in the stability analysis of earth slope. Geotechnique 1995, 5, 7–17. [Google Scholar] [CrossRef]
- Samui, P.; Kumar, R.; Yadav, U.; Kumari, S.; Bui, D.T. Reliability Analysis of Slope Safety Factor by Using GPR and GP. Geotech. Geol. Eng. 2019, 37, 2245–2254. [Google Scholar] [CrossRef]
- Griffiths, D.V. Stability Analysis of Highly Variable Soils by Elasto-Plastic Finite Elements (1999); Phase2 user’s guide Version 2.1; Rocscience Inc.: Toronto, ON, Canada, 2002. [Google Scholar]
- Schweiger, H.F.; Peschl, G.M. Reliability analysis in geotechnics with the random set finite element method. Comput. Geotech. 2005, 32, 422–435. [Google Scholar] [CrossRef]
- Read, J. Data Uncertainty: Guidelines for Open Pit Slope Design; Read, J., Stacey, P., Eds.; CSIRO Publishing: Clayton, Australia, 2009; pp. 214–220. [Google Scholar]
- Bedi, A.; Harrison, J.P. Characterisation and Propagation of Epistemic Uncertainty in Rock Engineering: A Slope Stability Example. In Proceedings of the International Symposium of the ISRM, Wroclaw, Poland, 21–26 September 2013. [Google Scholar]
- Abdulai, M.; Sharifzadeh, M. Uncertainty and reliability analysis of open pit rock slopes: A critical review of methods of analysis. Geotech. Geol. Eng. 2019. [Google Scholar] [CrossRef]
- Abbaszadeh, M.; Shahriar, K.; Sharifzadeh, M.; Heydari, M. Uncertainty and reliability analysis to slope stability: A case study from Sungun Copper Mine. Geotech. Geol. Eng. 2011, 29, 581–596. [Google Scholar] [CrossRef]
- Langford, J.C.; Diederichs, M.S. Application of Reliability Methods in Geological Engineering Design. In Proceedings of the Pan-Am Canadian Geotechnical Conference, Toronto, ON, Canada, 2–6 October 2011. [Google Scholar]
- Zadeh, L.A. Fuzzy sets. Inf. Control 1965, 8, 338–353. [Google Scholar] [CrossRef] [Green Version]
- Shrestha, B.; Duckstein, L. A fuzzy reliability measure for engineering applications. In Uncertainty Modeling and Analysis in Civil Engineering; CRC Press: Boca Raton, FL, USA, 1998; pp. 121–135. [Google Scholar]
- Tonon, F.; Bernardini, A.; Mammino, A. Determination of parameters range in rock engineering by means of random set theory. Reliab. Eng. Syst. Saf. 2000, 70, 241–261. [Google Scholar] [CrossRef]
- Tonon, F.; Bernardini, A.; Mammino, A. Reliability analysis of rock mass response by means of random set theory. Reliab. Eng. Syst. Saf. 2000, 70, 263–282. [Google Scholar] [CrossRef]
- Peschl, G.M. Reliability Analyses in Geotechnics with the Random Set Finite Element Method. Ph.D. Thesis, Institute for Soil Mechanics and Foundation Engineering, Graz University of Technology, Graz, Austria, 2004. [Google Scholar]
- Shen, H. Non-Deterministic Analysis of Slope Stability Based on Numerical Simulation. Ph.D. Thesis, Freiberg University of Mining and Technology, Freiberg, Germany, 2012. [Google Scholar]
- Low, B.K.; Tang, W.H. Reliability analysis using object-oriented constrained optimization. Struct. Saf. 2004, 26, 68–89. [Google Scholar] [CrossRef]
- Cornell, C.A. First-Order Uncertainty Analysis of Soils Deformation and Stability; University of Hong Kong Press: Hong Kong, China, 1971; pp. 129–144. [Google Scholar]
- Duncan, J.M. Factors of safety and reliability in geotechnical engineering. J. Geotech. Geoenviron. Eng. 2000, 126, 307–316. [Google Scholar] [CrossRef]
- Christian, J.T. Geotechnical engineering reliability: How well do we know what we are doing? J. Geotech. Geoenviron. Eng. 2004, 130, 985–1003. [Google Scholar] [CrossRef]
- Rackwitz, R.; Fiessler, B. Structural reliability under combined random load sequences. Comput. Struct. 1978, 9, 489–494. [Google Scholar] [CrossRef]
- Breitung, K. Asymptotic approximations for multinormal integrals. ASCE J. Eng. Mech. 1984, 110, 357–366. [Google Scholar] [CrossRef] [Green Version]
- Zhang, J.; Du, X. A second-order reliability method with first-order efficiency. J. Mech. Des. 2010, 132, 1–8. [Google Scholar] [CrossRef]
- Dadashzadeh, N.; Duzgun, H.S.B.; Gheibi, S. A Second-Order Reliability Analysis of Rock Slope Stability in Amasya, Turkey. In Proceedings of the 13th International ISRM Congress, International Symposium on Rock Mechanics, Montreal, QC, Canada, 10–13 May 2015. [Google Scholar]
- Der Kiureghian, A.; De Stefano, M. Efficient algorithm for second-order reliability analysis. J. Eng. Mech. 1991, 117, 2904–2927. [Google Scholar] [CrossRef]
- Zhao, Y.G.; Ono, T. New approximations for SORM: Part 1. J. Eng. Mech. 1999, 125, 79–85. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Y.G.; Ono, T. Moment methods for structural reliability. Struct. Saf. 2001, 23, 47–75. [Google Scholar] [CrossRef]
- Tvedt, L. Two Second Order Approximations to the Failure Probability; Veritas Report DIV/20-004-83; Det Norske Veritas: Bærum, Norway, 1983. [Google Scholar]
- Rosenblueth, E. Point Estimates for Probability Moments. Proc. Natl. Acad. Sci. USA 1975, 72, 3812–3814. [Google Scholar] [CrossRef] [Green Version]
- Rosenblueth, E. Two-point estimates in probabilities. Appl. Math. Model. 1981, 5, 329–335. [Google Scholar] [CrossRef]
- Langford, J.C. Application of Reliability Methods to the Design of Underground Structures. Ph.D. Thesis, Queen’s University, Kingston, ON, Canada, 2013. [Google Scholar]
- Langford, J.C.; Diederichs, M.S. Quantifying uncertainty in Hoek-Brown intact strength envelopes. Int. J. Rock Mech. Min. Sci. 2015, 74, 91–102. [Google Scholar] [CrossRef]
- Tsai, C.W.; Franceschini, S. Evaluation of probabilistic point estimate methods in uncertainty analysis for environmental engineering applications. J. Environ. Eng. 2005, 131, 387–395. [Google Scholar] [CrossRef]
- Wang, Y.; Cao, Z.; Au, S.K. Practical reliability analysis of slope stability by advanced Monte Carlo simulations in a spreadsheet. Can. Geotech. J. 2011, 48, 162–172. [Google Scholar] [CrossRef]
- Gibson, W. Probabilistic methods for slope analysis and design. Aust. Geomech. 2011, 46, 29. [Google Scholar]
- Hammah, R.E.; Yacoub, T.E.; Curran, J.H. Probabilistic Slope Analysis with the Finite Element Method. In Proceedings of the 43rd US Rock Mechanics Symposium and 4th US-Canada Rock Mechanics Symposium, Asheville, NC, USA, 28 June–1 July 2009. [Google Scholar]
- Shamekhi, E.; Tannant, D.D. Probabilistic assessment of rock slope stability using response surfaces determined from finite element models of geometric realizations. Comput. Geotech. 2015, 69, 70–81. [Google Scholar] [CrossRef] [Green Version]
- Hill, W.J.; Hunter, W.G. A review of response surface methodology: A literature survey. Technometrics 1966, 8, 571–590. [Google Scholar] [CrossRef]
- Sayed, S.; Dodagoudara, G.R.; Rajagopala, K. Finite element reliability analysis of reinforced retaining walls. Geomech. Geoeng. 2010, 5, 187–197. [Google Scholar] [CrossRef]
- Zhang, J.; Huang, H.W.; Phoon, K.K. Application of the Kriging-based response surface method to the system reliability of soil slopes. J. Geotech. Geoenviron. 2013, 139, 651–655. [Google Scholar] [CrossRef]
- Li, D.Q.; Jiang, S.H.; Cao, Z.J.; Zhou, W.; Zhou, C.B.; Zhang, L.M. A multiple response surface method for slope reliability analysis considering spatial variability of soil properties. Eng. Geol. 2015, 187, 60–72. [Google Scholar] [CrossRef]
- Li, D.; Chen, Y.; Lu, W.; Zhou, C. Stochastic response surface method for reliability analysis of rock slopes involving correlated non-normal variables. Comput. Geotech. 2011, 38, 58–68. [Google Scholar] [CrossRef]
- Bucher, C.G.; Bourgund, U. A fast and efficient response surface approach for structural reliability problems. Struct. Saf. 1990, 7, 57–66. [Google Scholar] [CrossRef]
- Rocscience Inc. Slide Version 7.0-2D Limit Equilibrium Slope Stability Analysis; Rocscience Inc.: Toronto, ON, Canada, 2015; Available online: www.rocscience.com (accessed on 7 June 2021).
- Choi, S.K.; Grandhi, R.V.; Canfield, R.A.; Pettit, C.L. Polynomial chaos expansion with latin hypercube sampling for estimating response variability. AIAA J. 2004, 42, 1191–1198. [Google Scholar] [CrossRef]
- Yang, X.S.; Deb, S. Cuckoo Search via Lévy Flights. In Proceedings of the 2009 World Congress on Nature & Biologically Inspired Computing (NaBIC), Coimbatore, India, 9–11 December 2009; pp. 210–214. [Google Scholar] [CrossRef]
- Wu, A. Locating General Failure Surfaces in Slope Analysis via CUCKOO Search. 2012. Available online: https://www.rocscience.com/help/slide2/pdf_files/theory/Cuckoo_Search.pdf (accessed on 21 May 2021).
- Gandomi, A.H.; Yang, X.S.; Talatahari, S.; Alavi, A.H. Metaheuristic Algorithms in Modeling and optimization. In Metaheuristic Applications in Structures and Infrastructures; Elsevier: Amsterdam, The Netherlands, 2013; pp. 1–24. [Google Scholar] [CrossRef]
- Azizi, M.A.; Marwanza, I.; Hartanti, N.A.; Ghifari, M.K.; Anugrahadi, A. Application of Cuckoo Search Method in 3D Slope Stability Analysis for Limestone Quarry Mine. Indones. Min. J. 2020, 23, 57–65. [Google Scholar]
- McQuillan, A.; Canbulat, I.; Oh, J.; Gale, S.; Yacoub, T. Case Study: Comparing Slide3 Models to Actual Slope Failure in an Open Cut Coal Mine. 2018. Available online: https://www.rocscience.com/documents/pdfs/rocnews/2018spring/Slide3CaseStudy.pdf (accessed on 10 June 2021).
- Mohamad, A.B.; Zain, A.M.; Bazin, N.E.N. Cuckoo Search Algorithm for Optimization Problems—A Literature Review and its Applications. Appl. Artif. Intell. 2014, 28, 419–448. [Google Scholar] [CrossRef]
- Yang, X.S.; Deb, S. Engineering optimisation by cuckoo search. Int. J. Math. Model. Numer. Optim. 2010, 1, 330–343. [Google Scholar] [CrossRef]
- Eberhart, R.; Kennedy, J. New Optimizer Using Particle Swarm Theory. In Proceedings of the 6th International Symposium on Micro Machine and Human Science, Nagoya, Japan, 4–6 October 1995; pp. 39–43. [Google Scholar]
- Kennedy, J.; Eberhart, R. Particle Swarm Optimization. In Proceedings of the IEEE International Conference on Neural Networks, Perth, WA, Australia, 27 November–1 December 1995; pp. 1942–1948. [Google Scholar]
- Shi, Y.; Eberhart, R.C. Empirical Study of Particle Swarm Optimization. In Proceedings of the 1999 Congress on Evolutionary Computation-CEC99 Cat, Washington, DC, USA, 6–9 July 1999. [Google Scholar]
- Eberhart, R.C.; Shi, Y. Particle Swarm Optimization: Developments, Application and Resources. In Proceedings of the 2001 Congress on Evolutionary Computation (IEEE Cat. No.01TH8546), Seoul, Korea, 27–30 May 2001; pp. 81–86. [Google Scholar]
- Wang, G.; Sun, F.; Tang, Q. Reliability Analysis of Rock Slope Excavation Considering the Stochasticity and Finite Persistence of Wedges. Period. Polytech. Civ. Eng. 2018, 62, 660–669. [Google Scholar] [CrossRef] [Green Version]
- Gholizadeh, S.; Salajegheh, E. Optimal design of structures subjected to time history loading by swarm intelligence and an advanced metamodel. Comput. Methods Appl. Mech. Eng. 2009, 198, 2936–2949. [Google Scholar] [CrossRef]
- Li, Y.; Tian, Y.; Oyang, Z.; Wang, L.; Xu, T.; Yang, P.; Zhao, H. Analysis of soil erosion characteristics in small watersheds with particle swarm optimization, support vector machine, and artificial neuronal networks. Environ. Earth Sci. 2010, 60, 1559–1568. [Google Scholar]
- Poitras, G.; Lefrançois, G.; Cormier, G. Optimization of steel floor systems using particle swarm optimization. J. Constr. Steel Res. 2011, 67, 1225–1231. [Google Scholar] [CrossRef]
- Kuok, K.K.; Harun, S.; Shamsuddin, S.M. Particle swarm optimization feedforward neural network for hourly rainfall runoff modeling in Bedup Basin, Malaysia. Int. J. Civ. Environ. Eng. 2010, 9, 9–18. [Google Scholar]
- Kuok, K.K.; Harun, S.; Shamsuddin, S.M. Particle swarm optimization feedforward neural network for modeling runoff. Int. J. Environ. Sci. Technol. 2010, 7, 67–78. [Google Scholar] [CrossRef] [Green Version]
- Chen, B.R.; Feng, X.T. CSV-PSO and Its Application in Geotechnical Engineering. In Swarm Intelligence: Focus on Ant and Particle Swarm Optimization; Chan, F.T.S., Tiwari, M.K., Eds.; BoD—Books on Demand: Norderstedt, Germany, 2007; pp. 263–288. [Google Scholar]
- Bharat, T.V.; Sivapullaiah, P.V.; Allam, M.M. Swarm intelligence-based solver for parameter estimation of laboratory through-diffusion transport of contaminants. Comput. Geotech. 2009, 36, 984–992. [Google Scholar] [CrossRef]
- Yazdi, J.S.; Kalantary, F.; Yazdi, H.S. Calibration of soil model parameters using particle swarm optimization. Int. J. Geomech. 2012, 12, 229–238. [Google Scholar] [CrossRef]
- Zhao, H.B.; Zou, Z.S.; Ru, Z.L. Chaotic particle swarm optimization for non-circular critical slip surface identification in slope stability analysis. In Boundaries of Rock Mechanics Recent Advances and Challenges for the 21st Century: Proceedings of the International Young Scholars’ Symposium on Rock Mechanics, Beijing, China, 28 April–2 May 2008; CRC Press: Boca Raton, FL, USA; pp. 585–588.
- Cheng, Y.M.; Li, L.; Sun, Y.J.; Au, S.K. A coupled particle swarm and harmony search optimization algorithm for difficult geotechnical problems. Struct. Multidiscip. Optim. 2012, 45, 489–501. [Google Scholar] [CrossRef]
- Kalatehjari, R.; Ali, N.; Kholghifard, M.; Hajihassani, M. The effects of method of generating circular slip surfaces on determining the critical slip surface by particle swarm optimization. Arab. J. Geosci. 2014, 7, 1529–1539. [Google Scholar] [CrossRef]
- Kalatehjari, R.; Safuan, A.; Rashid, A.; Ali, N.; Hajihassani, M. The Contribution of Particle Swarm Optimization to Three-Dimensional Slope Stability Analysis. Sci. World J. 2014, 2014, 973093. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jiang, J.C.; Yamagami, T.; Baker, R. Three-dimensional slope stability analysis based on nonlinear failure envelope. Chin. J. Rock Mech. Eng. 2003, 22, 1017–1023. [Google Scholar]
- Cheng, Y.M.; Li, L.; Chi, S.C. Performance studies on six heuristic global optimization methods in the location of critical slip surface. Comput. Geotech. 2007, 34, 462–484. [Google Scholar] [CrossRef]
- Javadzadeh, E.; Javadzadeh, R. Bishop’s Simplified Method and Particle Swarm Optimization for Location the Critical Failure Surface in Rock Slope Stability Analysis. In Proceedings of the ISRM International Symposium—5th Asian Rock Mechanics Symposium (ARMS5), Tehran, Iran, 24–26 November 2008. [Google Scholar]
- Millonas, M.M. Swarms, Phase Transitions, and Collective Intelligence. In Computational Intelligence: A Dynamic System Perspective; IEEE Press: Piscataway, NJ, USA, 1994; pp. 137–151. [Google Scholar]
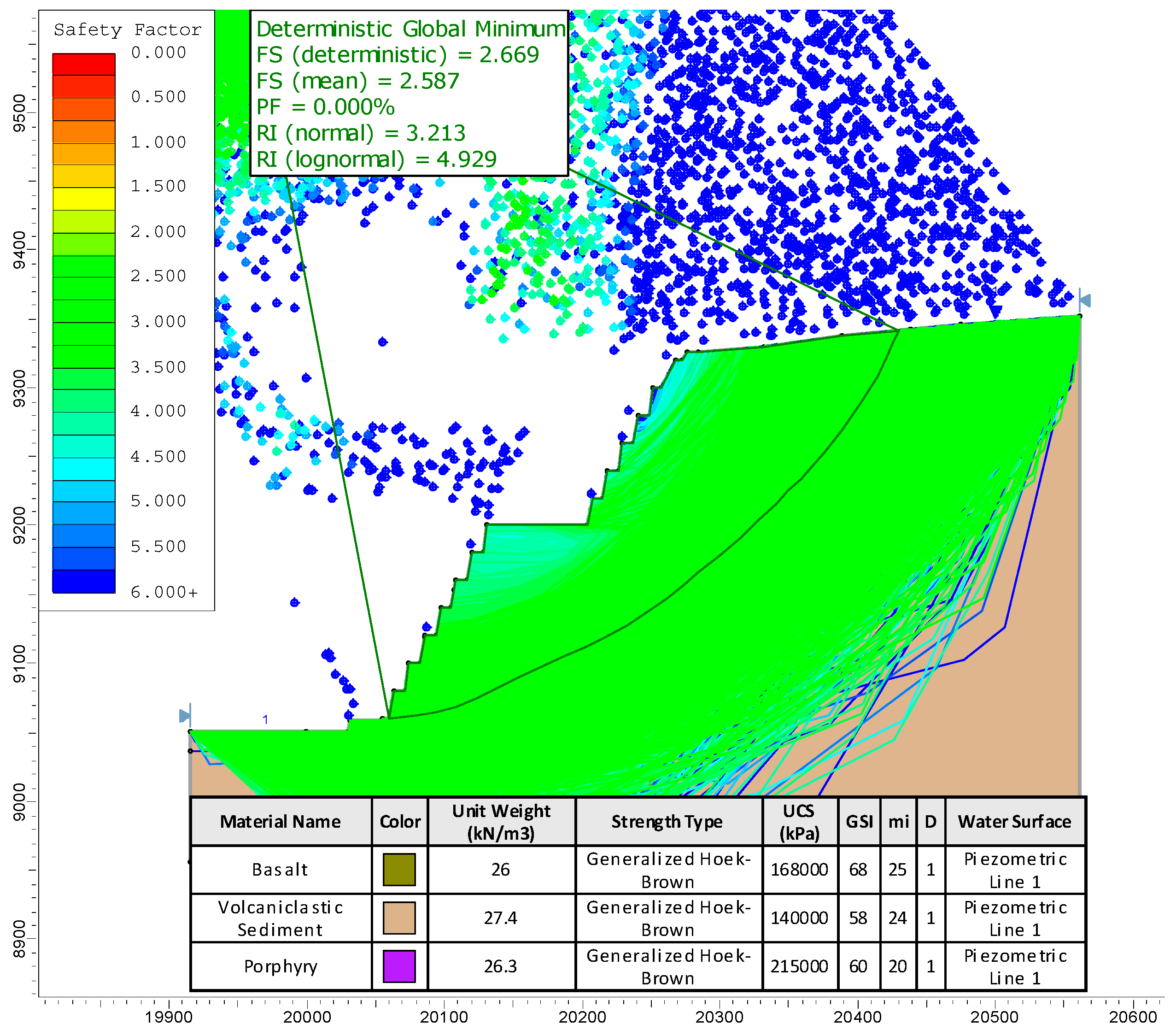
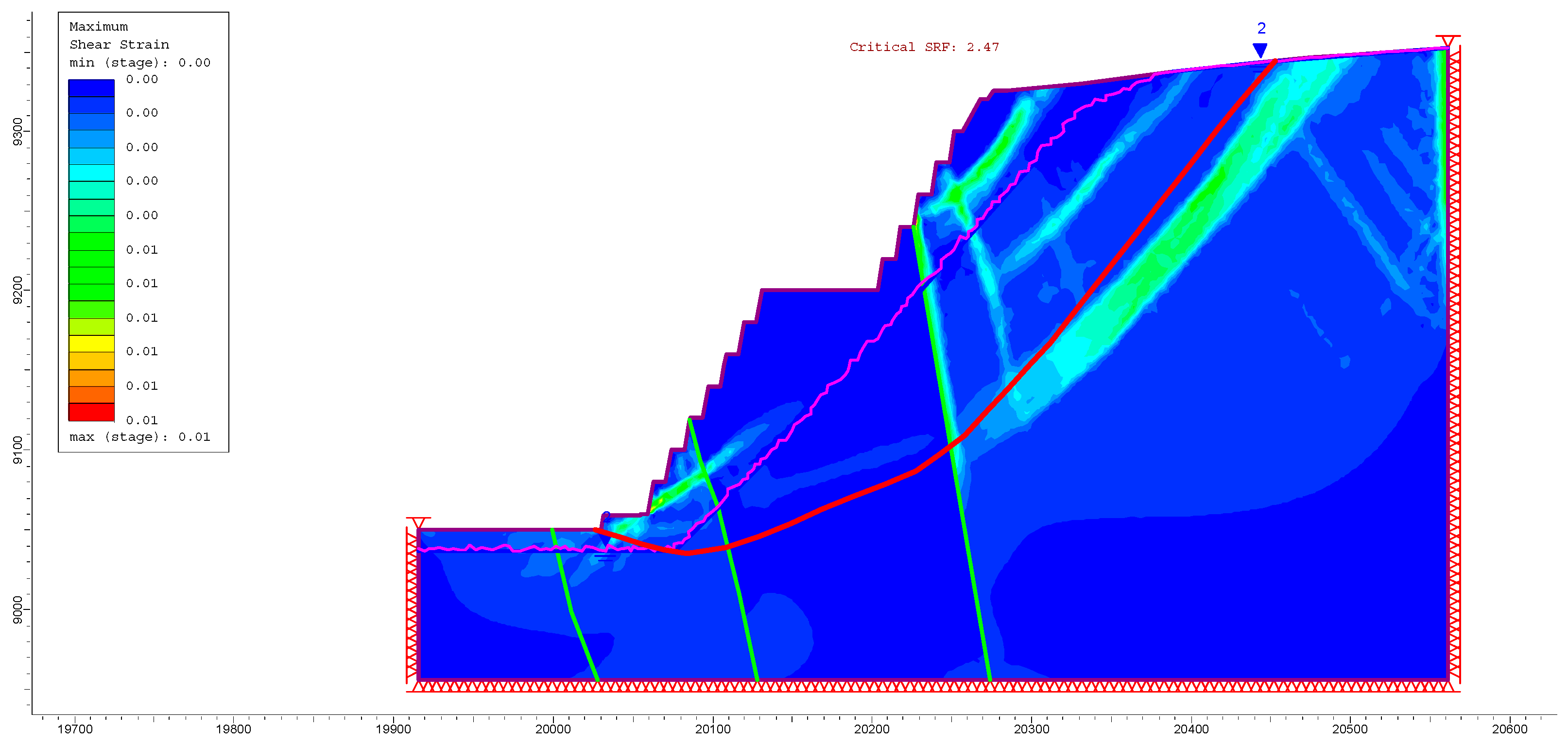
| Reliability Index β | Probability of Failure Pf =Φ(−β) | Expected Performance Level |
|---|---|---|
| 1.0 | 0.16 | Hazardous |
| 1.5 | 0.07 | Unsatisfactory |
| 2.0 | 0.023 | Poor |
| 2.5 | 0.006 | Below average |
| 3.0 | 0.001 | Above average |
| 4.0 | 0.00003 | Good |
| 5.0 | 0.0000003 | High |
| Rock Type | Unit Weight (kN/m3) | Generalized Hoek–Brown | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| UCS (MPa) | GSI | mi | D | Young’s Modulus (GPa) | Poisson Ratio | σUCS (MPa) | σGSI | Distribution | ||
| Volcaniclastic Sediment | 27.4 | 140 | 58 | 24 | 1.0 | 2.78 | 0.3 | 34.5 | 13.3 | Normal |
| Basalt | 28.8 | 168 | 68 | 25 | 1.0 | 4.93 | 0.2 | 32.0 | 10.0 | Normal |
| Porphyry | 26.3 | 215 | 60 | 20 | 1.0 | 3.34 | 0.2 | 53.9 | 12.0 | Normal |
| Probability Method | FS | PF (%) | RI |
|---|---|---|---|
| MCS | 2.587 | 0 | 3.2 |
| RSM | 2.902 | 0.2 | 2.7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdulai, M.; Sharifzadeh, M. Probability Methods for Stability Design of Open Pit Rock Slopes: An Overview. Geosciences 2021, 11, 319. https://doi.org/10.3390/geosciences11080319
Abdulai M, Sharifzadeh M. Probability Methods for Stability Design of Open Pit Rock Slopes: An Overview. Geosciences. 2021; 11(8):319. https://doi.org/10.3390/geosciences11080319
Chicago/Turabian StyleAbdulai, Musah, and Mostafa Sharifzadeh. 2021. "Probability Methods for Stability Design of Open Pit Rock Slopes: An Overview" Geosciences 11, no. 8: 319. https://doi.org/10.3390/geosciences11080319
APA StyleAbdulai, M., & Sharifzadeh, M. (2021). Probability Methods for Stability Design of Open Pit Rock Slopes: An Overview. Geosciences, 11(8), 319. https://doi.org/10.3390/geosciences11080319