Beach Deployment of a Low-Cost GNSS Buoy for Determining Sea-Level and Wave Characteristics
Abstract
:1. Introduction
1.1. Measuring Sea Level
1.2. Measuring Waves
2. Materials and Method
2.1. GNSS Buoy Set-Up and Mooring Design
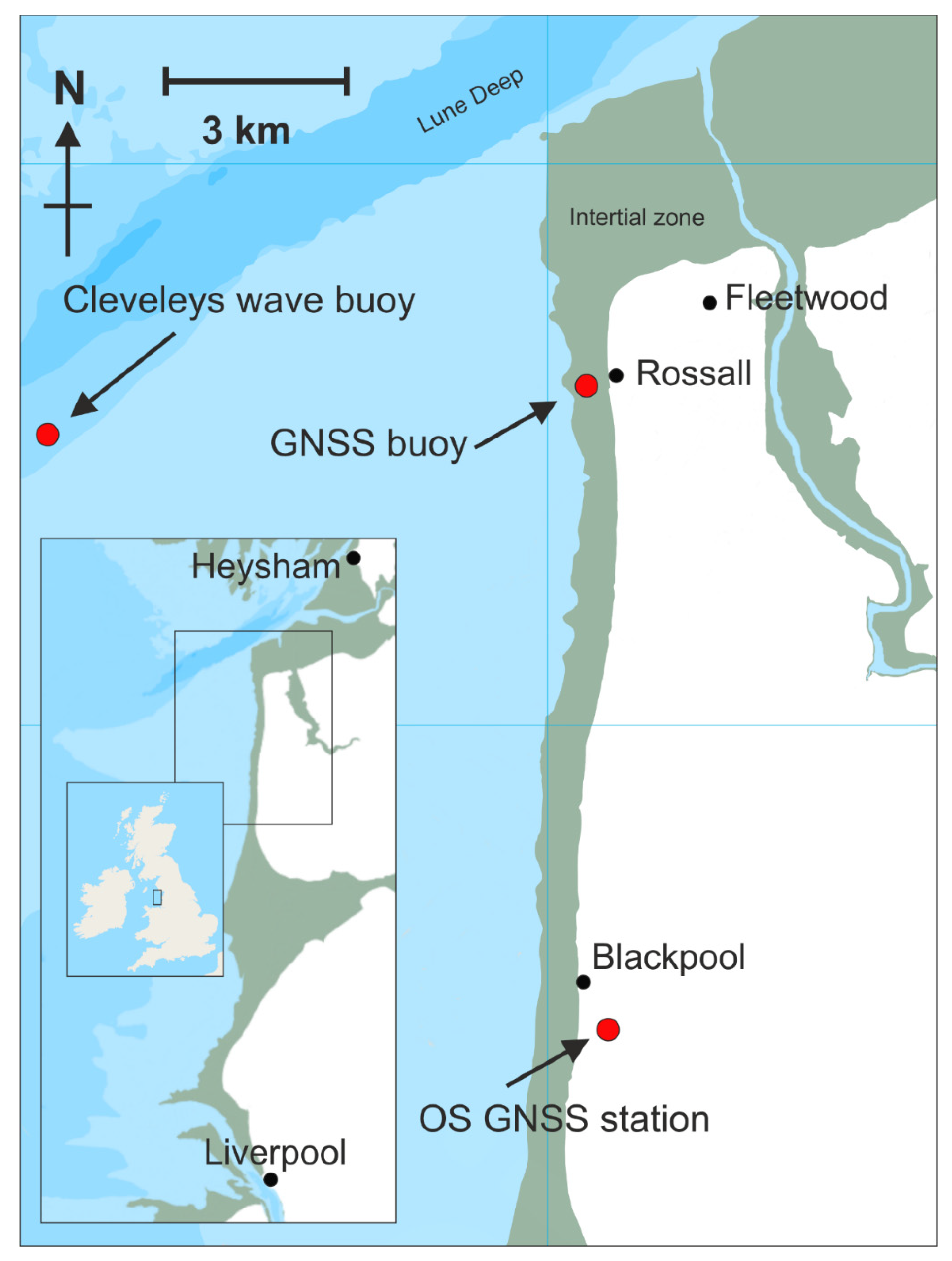
2.2. Buoy Testing at Rossall
2.3. Local Tide and Storm Surge Data
2.4. Offshore Wave Data
2.5. GNSS Base Station and Satellite Ephemerides Data
3. Results and Interpretation
- tidal cycle A refers to data between 18:00 on the 28 August 02:00 on the 29 August.
- tidal cycle B refers to data between 06:00–14:30 on the 29 August.
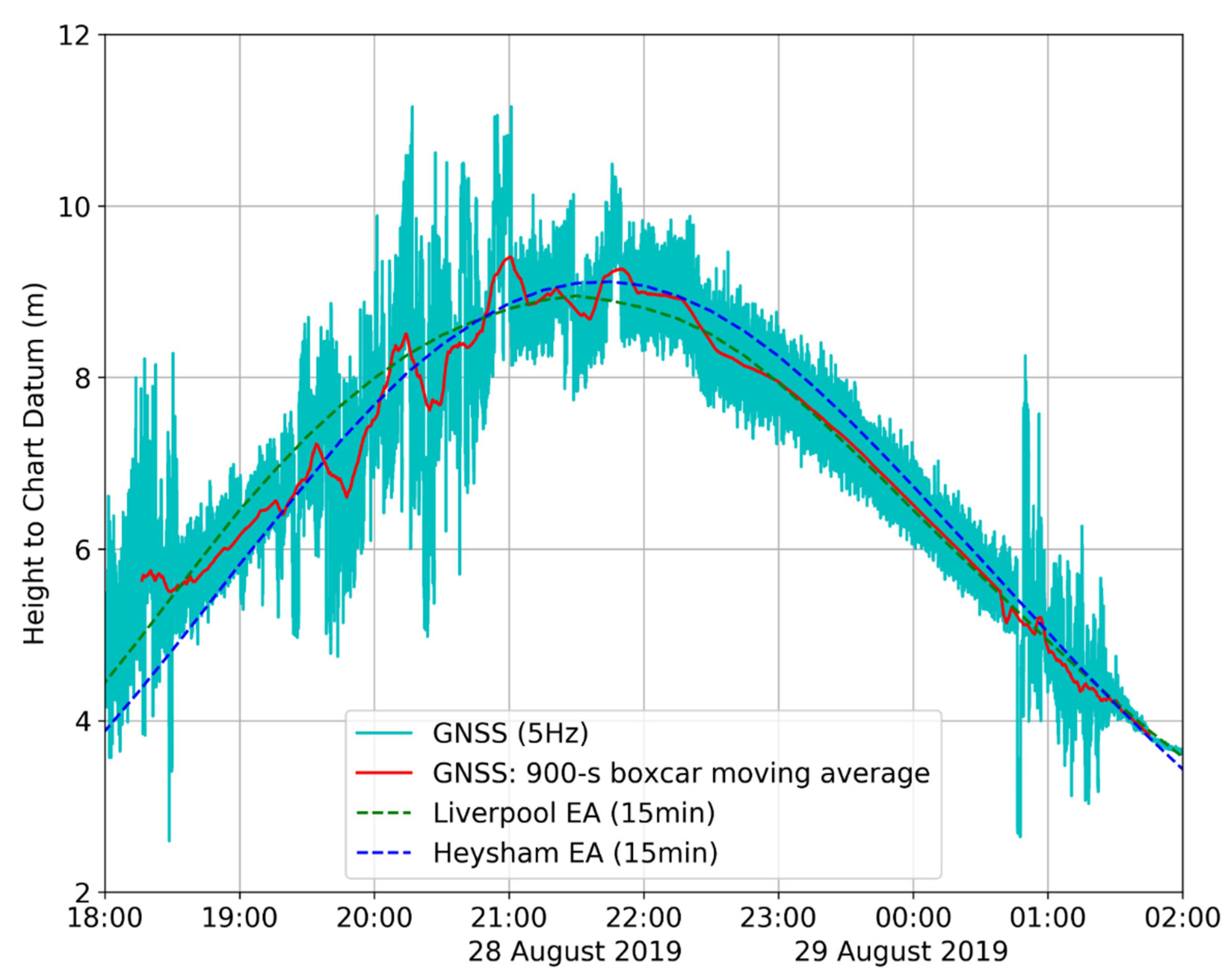
3.1. GNSS-Derived Tidal Heights
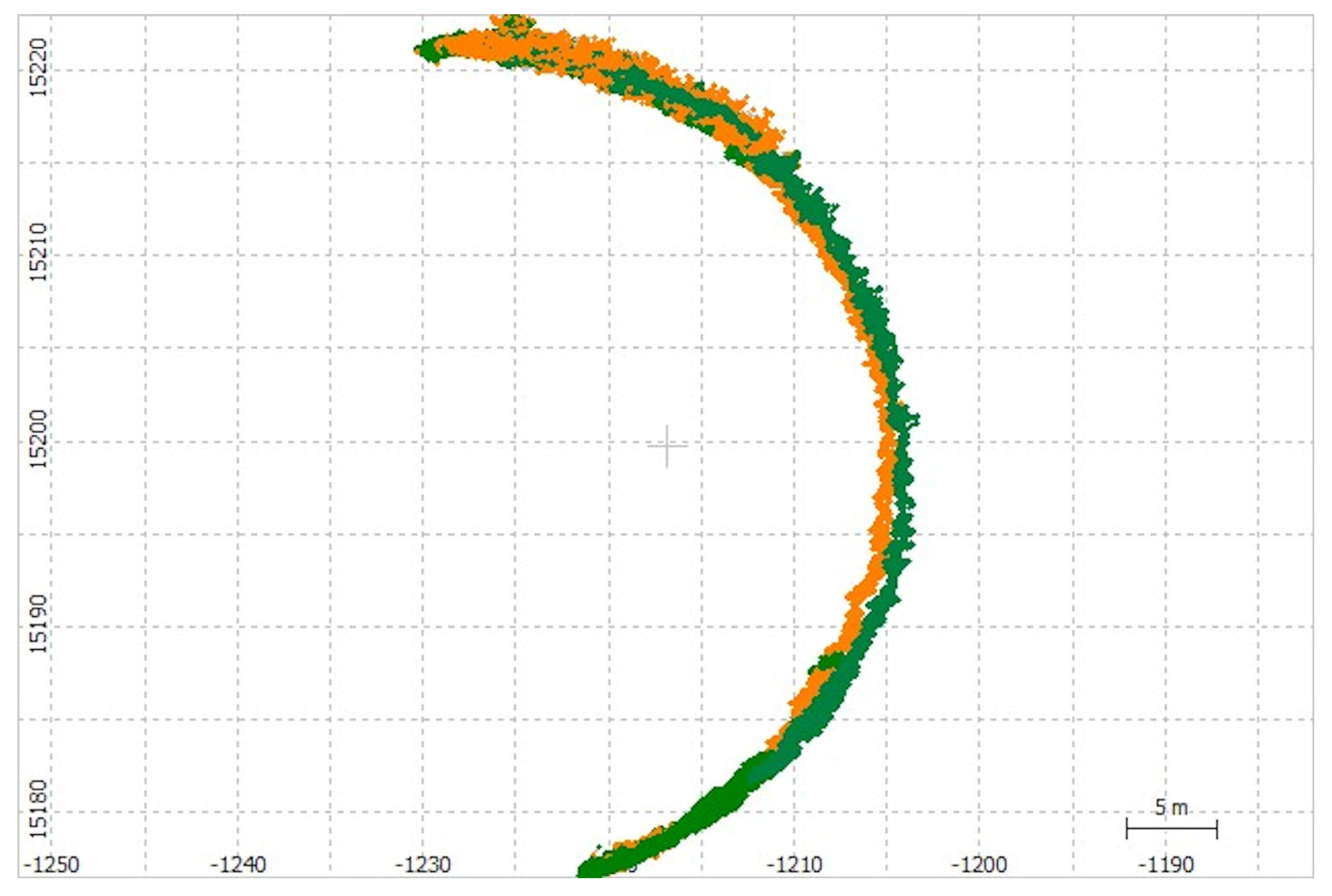
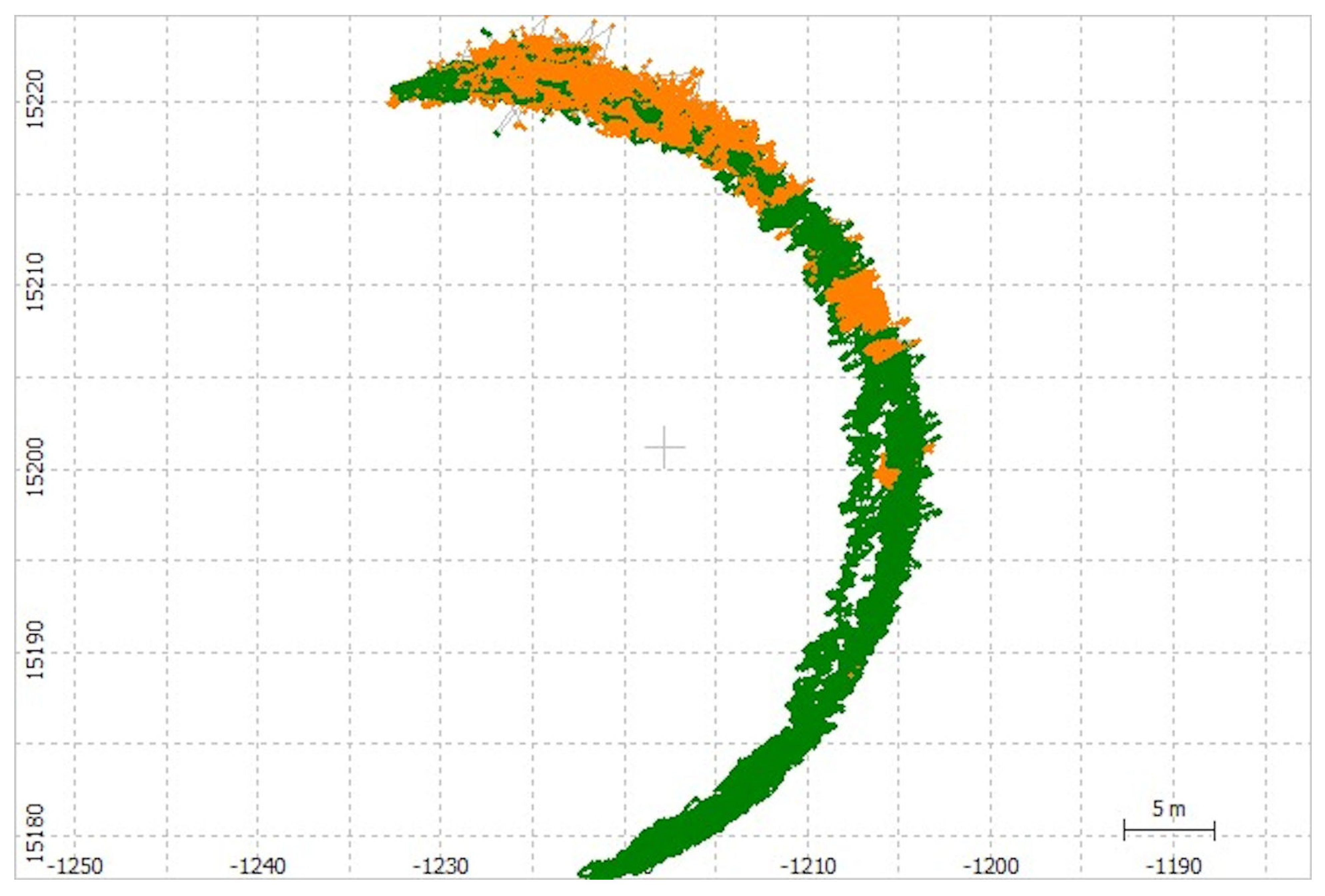
3.2. GNSS Buoy Track
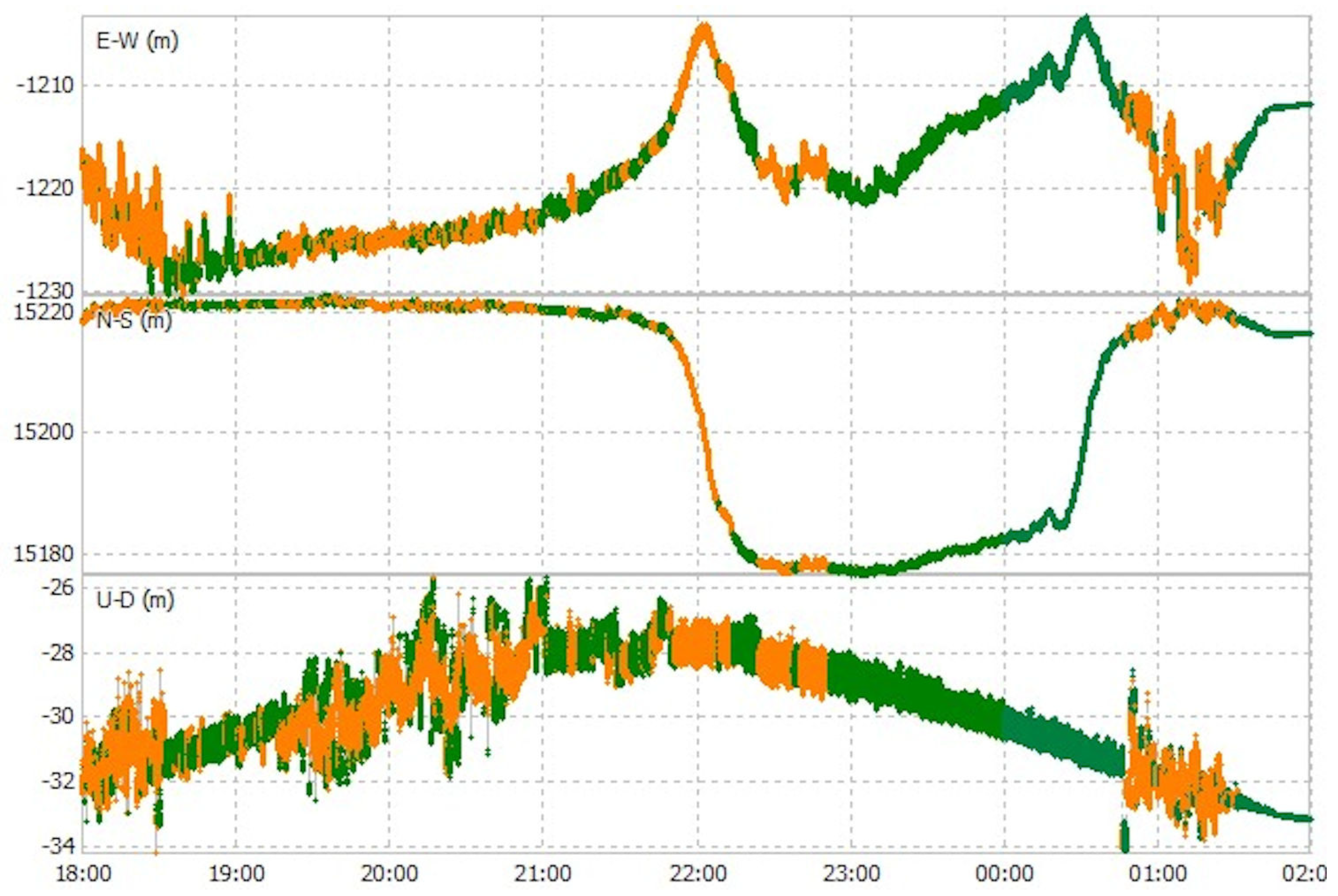
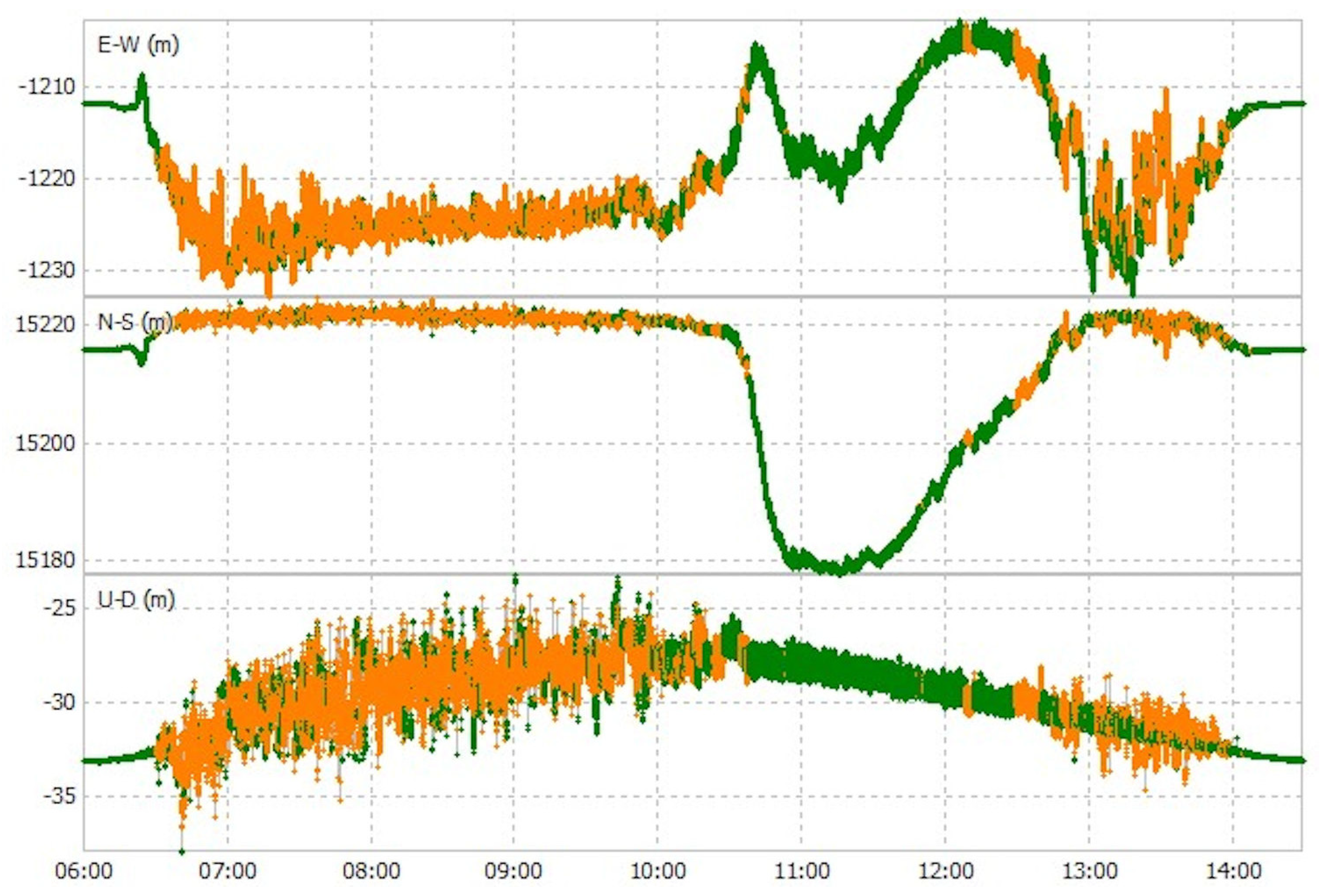
3.3. GNSS Buoy Positional Components
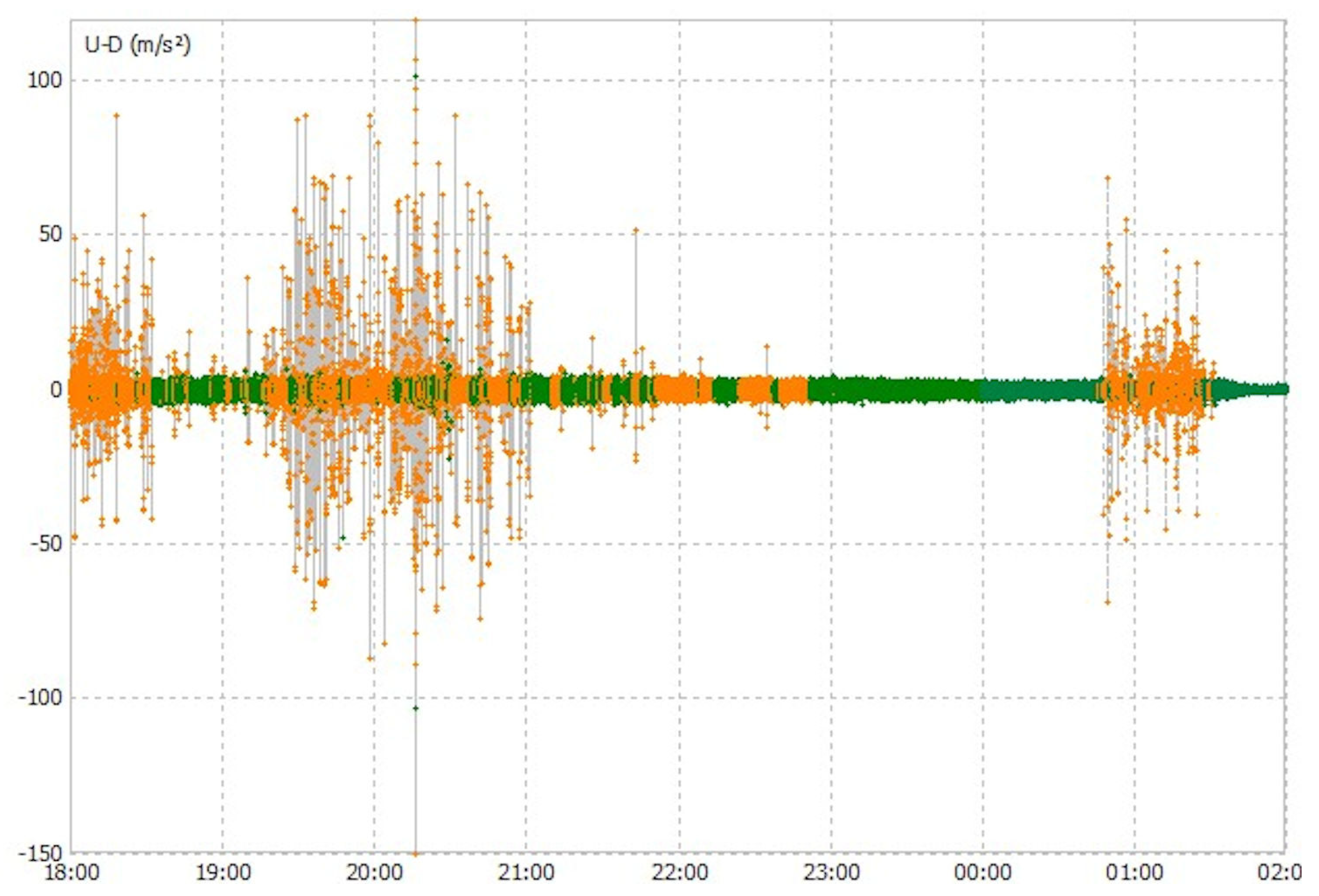
3.4. GNSS Buoy Vertical Accelerations
4. Analysis and Discussion
4.1. Buoy Performance
4.2. GNSS Receiver Performance
4.3. Sea Levels
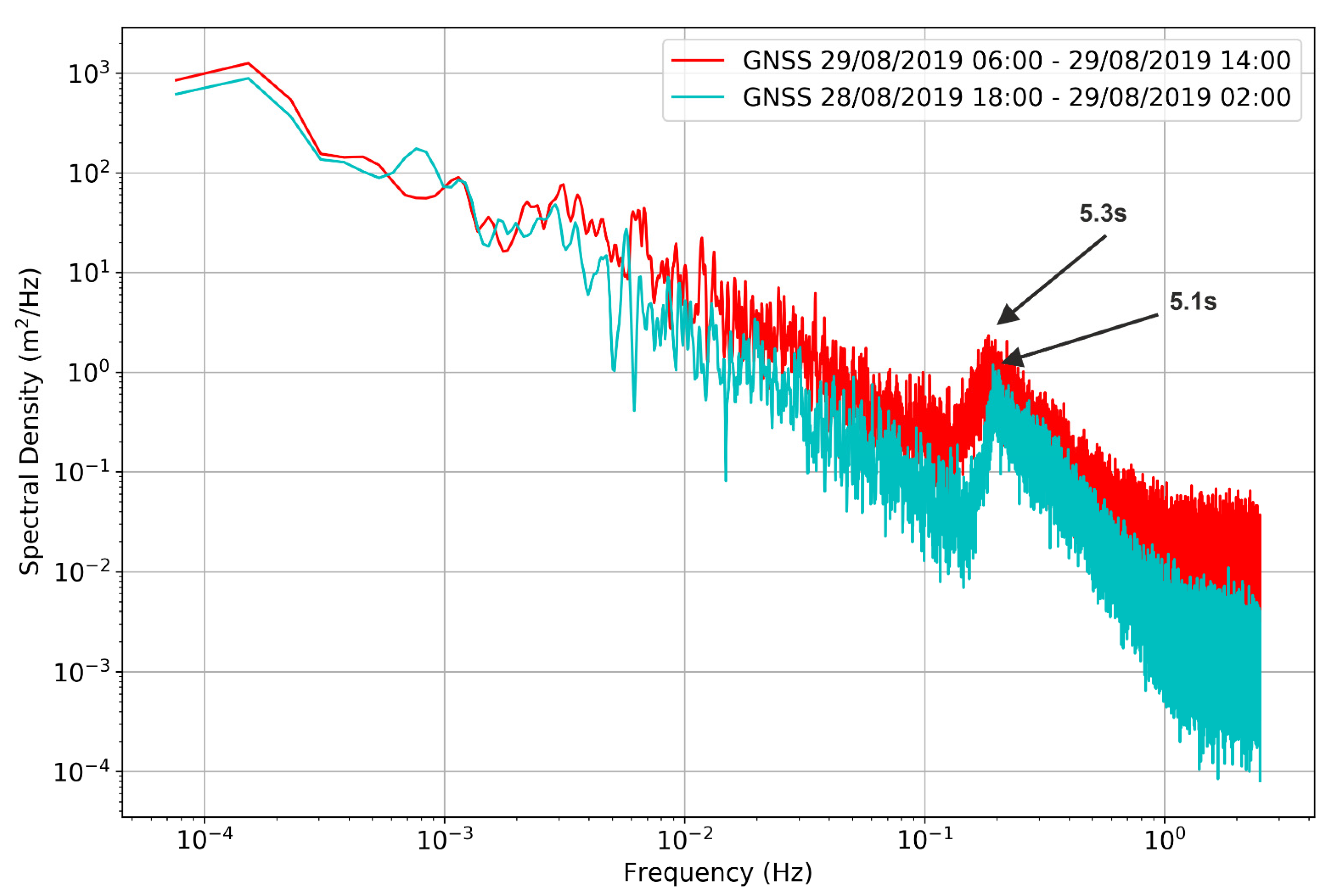
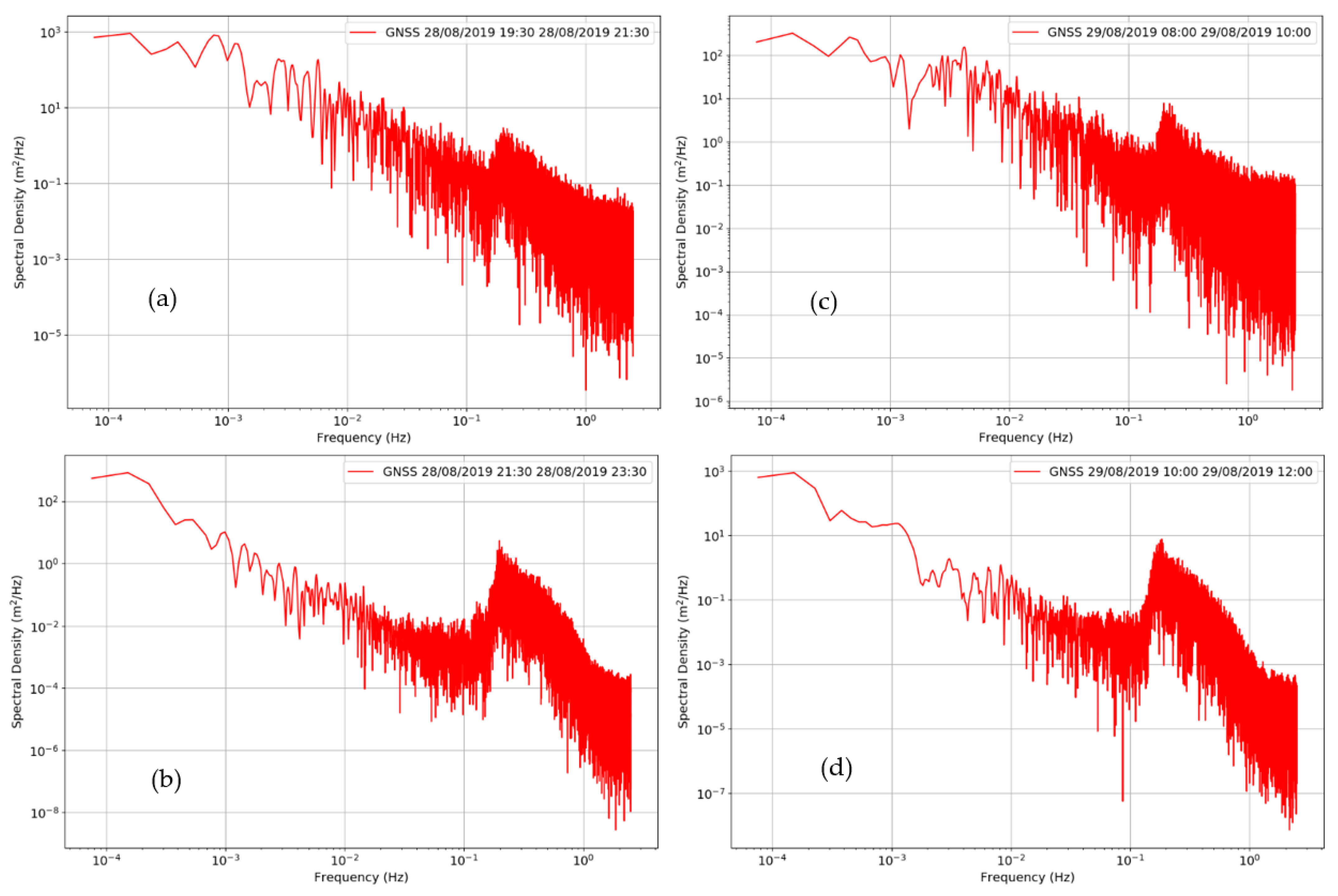
4.4. Wave Characteristics
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bird, C.O.; Bell, P.S.; Plater, A.J. Application of marine radar to monitoring seasonal and event-based changes in intertidal morphology. Geomorphology 2017, 285, 1–15. [Google Scholar] [CrossRef] [Green Version]
- Nicholls, R.J.; Wong, P.P.; Burkett, V.; Codignotto, J.; Hay, J.; McLean, R.; Ragoonaden, S.; Woodroffe, C.D.; Abuodha, P.; Arblaster, J. Coastal Systems and Low-Lying Areas. 2007. Available online: https://ro.uow.edu.au/scipapers/164 (accessed on 5 July 2021).
- Spodar, A.; Héquette, A.; Ruz, M.-H.; Cartier, A.; Grégoire, P.; Sipka, V.; Forain, N. Evolution of a beach nourishment project using dredged sand from navigation channel, Dunkirk, northern France. J. Coast. Conserv. 2018, 22, 457–474. [Google Scholar] [CrossRef]
- Habel, S.; Fletcher, C.H.; Barbee, M.; Anderson, T.R. The influence of seasonal patterns on a beach nourishment project in a complex reef environment. Coast. Eng. 2016, 116, 67–76. [Google Scholar] [CrossRef]
- RTKLIB: An Open Source Program Package for GNSS Positioning. Available online: http://www.rtklib.com/ (accessed on 10 January 2019).
- Pugh, D.; Woodworth, P. Sea-Level Science: Understanding Tides, Surges, Tsunamis and Mean Sea-Level Changes; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar]
- Williams, S.D.; Bell, P.S.; McCann, D.L.; Cooke, R.; Sams, C. Demonstrating the potential of low-cost GPS units for the remote measurement of tides and water levels using interferometric reflectometry. J. Atmos. Ocean. Technol. 2020, 37, 1925–1935. [Google Scholar] [CrossRef]
- Knight, P.J.; Bird, C.O.; Sinclair, A.; Plater, A.J. A low-cost GNSS buoy platform for measuring coastal sea levels. Ocean. Eng. 2020, 203, 107198. [Google Scholar] [CrossRef]
- André, G.; Míguez, B.M.; Ballu, V.; Testut, L.; Wöppelmann, G. Measuring sea level with GPS-equipped buoys: A multi-instruments experiment at Aix Island. Int. Hydrogr. Rev. 2013, 10, 27–38. [Google Scholar]
- Stal, C.; Poppe, H.; Vandenbulcke, A.; De Wulf, A. Study of post-processed GNSS measurements for tidal analysis in the Belgian North Sea. Ocean. Eng. 2016, 118, 165–172. [Google Scholar] [CrossRef]
- Fiorentino, L.A.; Heitsenrether, R.; Krug, W. Wave Measurements From Radar Tide Gauges. Front. Mar. Sci. 2019, 6, 586. [Google Scholar] [CrossRef]
- Martins, K.; Blenkinsopp, C.E.; Power, H.E.; Bruder, B.; Puleo, J.A.; Bergsma, E.W. High-resolution monitoring of wave transformation in the surf zone using a LiDAR scanner array. Coast. Eng. 2017, 128, 37–43. [Google Scholar] [CrossRef]
- Datawell, Directional Waverider MkIII, Brochure. Available online: https://www.datawell.nl/Portals/0/Documents/Brochures/datawell_brochure_dwr-mk3_b-09-09.pdf (accessed on 5 July 2021).
- MacIsaac, C.; Naeth, S. TRIAXYS next wave II directional wave sensor the evolution of wave measurements. In Proceedings of the 2013 OCEANS-San Diego, San Diego, CA, USA, 23–27 September 2013; pp. 1–8. [Google Scholar]
- Andrews, E.; Peach, L. An evaluation of current and emerging in-situ ocean wave monitoring technology. In Proceedings of the Australasian Coasts and Ports 2019 Conference: Future Directions from 40 [Degrees] S and Beyond, Hobart, Australia, 10–13 September 2019; p. 34. [Google Scholar]
- Datawell, Mini Directional Waverider GPS. Available online: https://www.datawell.nl/Portals/0/Documents/Brochures/datawell_brochure_dwr-g4_b-06-10.pdf (accessed on 5 July 2021).
- Spotter: The Agile Metocean Buoy. Available online: https://www.sofarocean.com/products/spotter (accessed on 5 July 2021).
- Brown, A.C.; Paasch, R.K. The Accelerations of a Wave Measurement Buoy Impacted by Breaking Waves in the Surf Zone. J. Mar. Sci. Eng. 2021, 9, 214. [Google Scholar] [CrossRef]
- Emlid Reach M+ RTK GNSS Module for Precise Navigation and UAV Mapping. Available online: https://emlid.com/ (accessed on 16 April 2019).
- U-blox, Product Details for M8T Series GNSS Receivers. Available online: https://www.u-blox.com/en/product/neolea-m8t-series (accessed on 16 April 2019).
- Ublox, Application Note: GPS Antennas, RF Design Considerations for U-blox GPS Receivers. Available online: https://www.u-blox.com/sites/default/files/products/documents/GPS-Antenna_AppNote_(GPS-X-08014).pdf (accessed on 24 May 2019).
- Scott, T.; Masselink, G.; Russell, P. Morphodynamic characteristics and classification of beaches in England and Wales. Mar. Geol. 2011, 286, 1–20. [Google Scholar] [CrossRef] [Green Version]
- Wright, L.D.; Short, A.D. Morphodynamic variability of surf zones and beaches: A synthesis. Mar. Geol. 1984, 56, 93–118. [Google Scholar] [CrossRef]
- UK National Tide Gauge Network. Available online: https://www.bodc.ac.uk/data/hosted_data_systems/sea_level/uk_tide_gauge_network/ (accessed on 1 October 2019).
- Woodworth, P.L.; Smith, D.E. A one year comparison of radar and bubbler tide gauges at Liverpool. Int. Hydrogr. Rev. 2003, 4, 42–49. [Google Scholar]
- National Oceanography Centre POLTIPS-3: Coastal Tidal Software. Available online: http://noc.ac.uk/business/marine-data-products/coastal (accessed on 29 March 2020).
- Archived Model Surge Outputs. Available online: https://www.ntslf.org/storm-surges (accessed on 19 April 2021).
- NERC, British Isles Continuous GNSS Facility (BIGF). Available online: http://www.bigf.ac.uk/ (accessed on 3 May 2021).
- CDDIS, NASA’s Archive of Space Geodesy Data. Available online: https://cddis.nasa.gov/Data_and_Derived_Products/GNSS/orbit_products.html (accessed on 18 April 2021).
- Noll, C.E. The Crustal Dynamics Data Information System: A resource to support scientific analysis using space geodesy. Adv. Space Res. 2010, 45, 1421–1440. [Google Scholar] [CrossRef] [Green Version]





| Liverpool | Blackpool | Heysham | ||||
|---|---|---|---|---|---|---|
| Time | Ht (m) | Time | Ht (m) | Time | Ht (m) | |
| Wed 28 August | 15:52 | 2.00 LW | 15:38 | 2.17 LW | 15:51 | 1.97 LW |
| 21:32 | 8.92 HW | 21:23 | 8.87 HW | 21:42 | 9.10 HW | |
| Thu 29 August | 04:30 | 1.49 LW | 04:14 | 1.60 LW | 04:29 | 1.49 LW |
| 10:00 | 9.12 HW | 09:55 | 9.01 HW | 10:11 | 9.33 HW | |
| 16:48 | 1.49 LW | 16:32 | 1.61 LW | 16:45 | 1.49 LW | |
| Parameter | Value |
|---|---|
| Solution | Kinematic |
| Frequency | L1 |
| Sensor dynamics | On |
| Earth tides | Off |
| Ionosphere | Broadcast |
| Troposphere | Saastamoinen |
| Ephemerides | Precise |
| Satellite system | GPS + GLONASS |
| Ambiguities GPS | Fix & Hold |
| Ambiguities GLONASS | On |
| Fixed Q1% | Float Q2% | |
|---|---|---|
| Tidal cycle A | 57.0 | 43.0 |
| Tidal cycle B | 48.4 | 51.6 |
| Significant Wave Height | Spectral Peak Period | ||||
|---|---|---|---|---|---|
| GNSS Buoy | Cleveleys Buoy | GNSS Buoy | Cleveleys Buoy | ||
| Tidal cycle A | 2 hr period before HW | 1.1 m | 0.9 m | 4.8 s | 5.0 s |
| 2 hr period after HW | 1.0 m | 1.0 m | 5.0 s | 4.8 s | |
| Tidal cycle B | 2 hr period before HW | 1.5 m | 1.5 m | 4.7 s | 4.8 s |
| 2 hr period after HW | 1.3 m | 1.6 m | 5.4 s | 5.3 s | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Knight, P.J.; Bird, C.O.; Sinclair, A.; Higham, J.; Plater, A.J. Beach Deployment of a Low-Cost GNSS Buoy for Determining Sea-Level and Wave Characteristics. Geosciences 2021, 11, 494. https://doi.org/10.3390/geosciences11120494
Knight PJ, Bird CO, Sinclair A, Higham J, Plater AJ. Beach Deployment of a Low-Cost GNSS Buoy for Determining Sea-Level and Wave Characteristics. Geosciences. 2021; 11(12):494. https://doi.org/10.3390/geosciences11120494
Chicago/Turabian StyleKnight, Philip J., Cai O. Bird, Alex Sinclair, Jonathan Higham, and Andrew J. Plater. 2021. "Beach Deployment of a Low-Cost GNSS Buoy for Determining Sea-Level and Wave Characteristics" Geosciences 11, no. 12: 494. https://doi.org/10.3390/geosciences11120494
APA StyleKnight, P. J., Bird, C. O., Sinclair, A., Higham, J., & Plater, A. J. (2021). Beach Deployment of a Low-Cost GNSS Buoy for Determining Sea-Level and Wave Characteristics. Geosciences, 11(12), 494. https://doi.org/10.3390/geosciences11120494






