Comparison of Applications to Evaluate Groundwater Recharge at Lower Kelantan River Basin, Malaysia
Abstract
1. Introduction
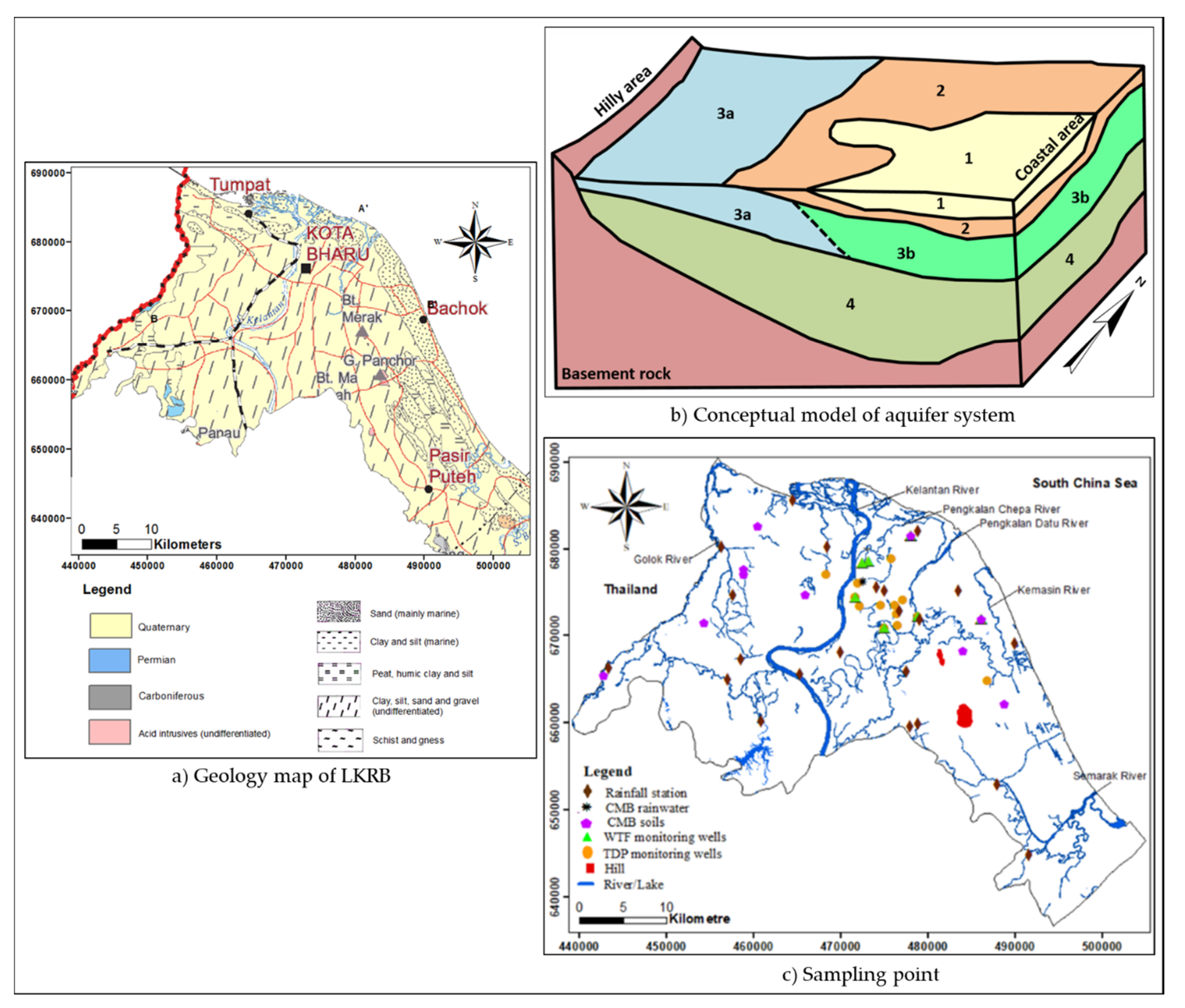
2. Study Area
2.1. Location and Climate Condition
2.2. Geology and Hydrogeology Conditions
3. Materials and Methods
3.1. Chloride Mass Balance, CMB
3.2. Water Level Fluctuation, WTF
3.3. Temperature-Depth Profiles, TDP
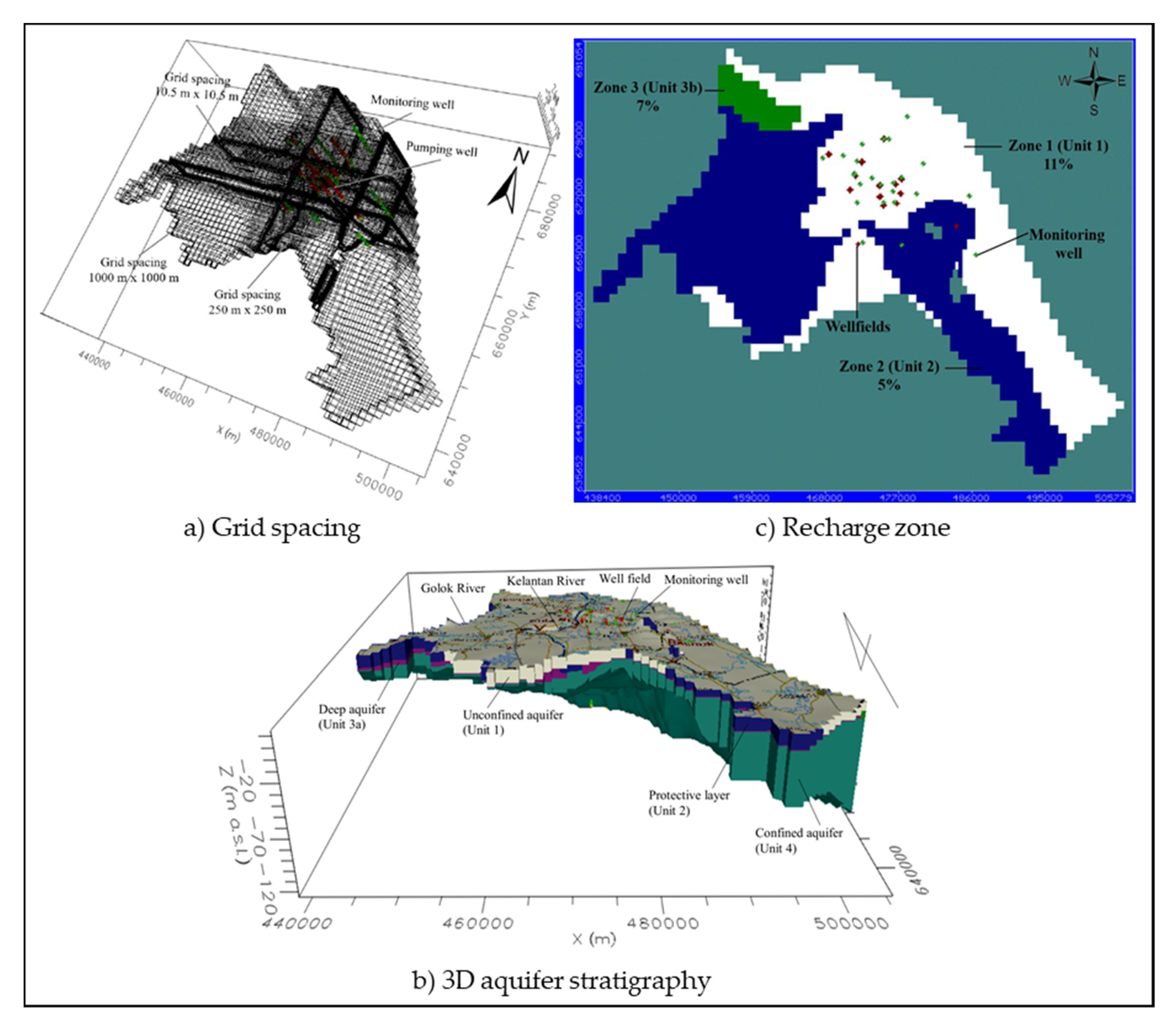
3.4. Groundwater Modelling, GM(WB)
4. Results
4.1. Chloride Mass Balance, CMB
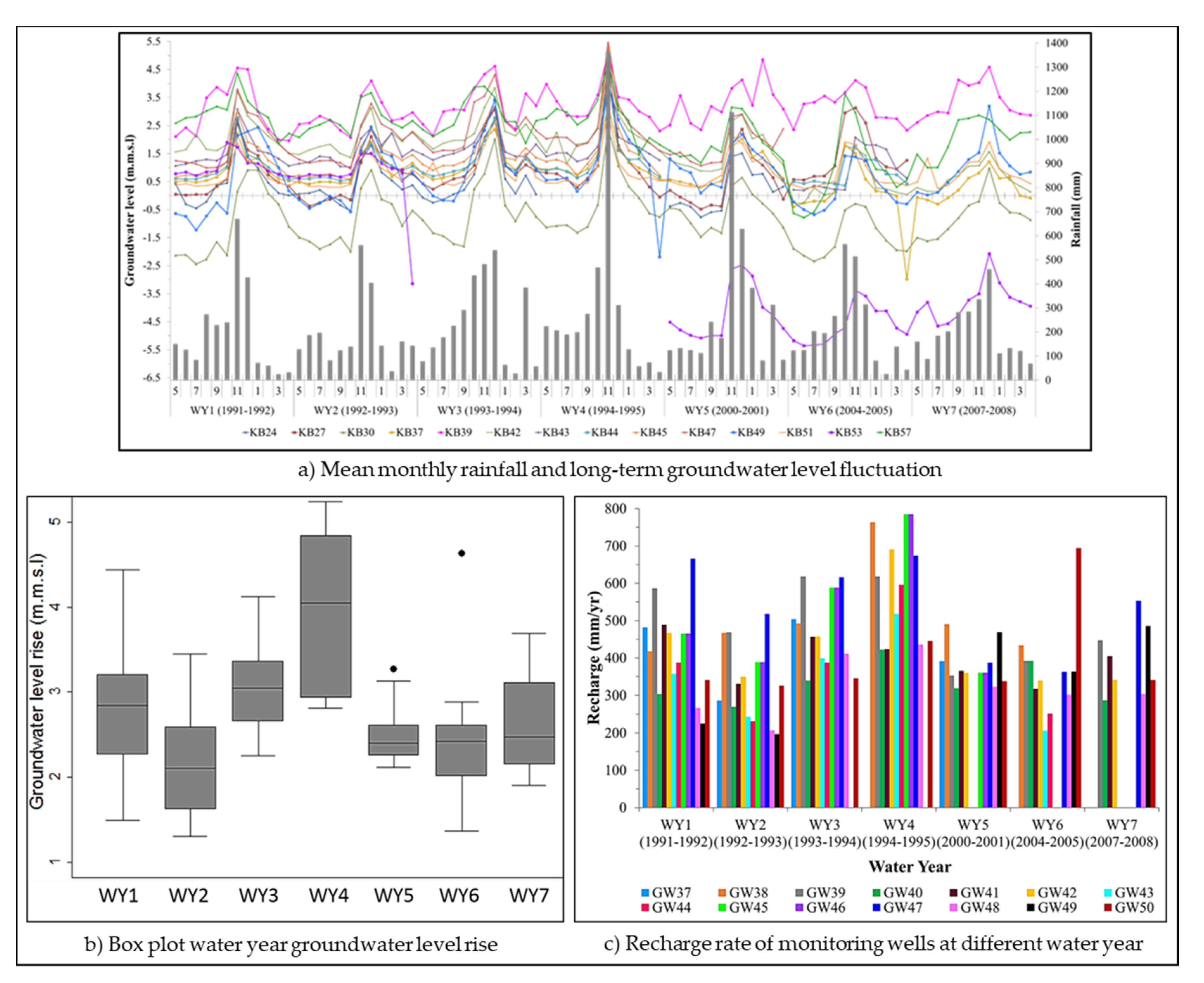
4.2. Water Level Fluctuation, WTF
4.3. Temperature–Depth Profiles, TDP
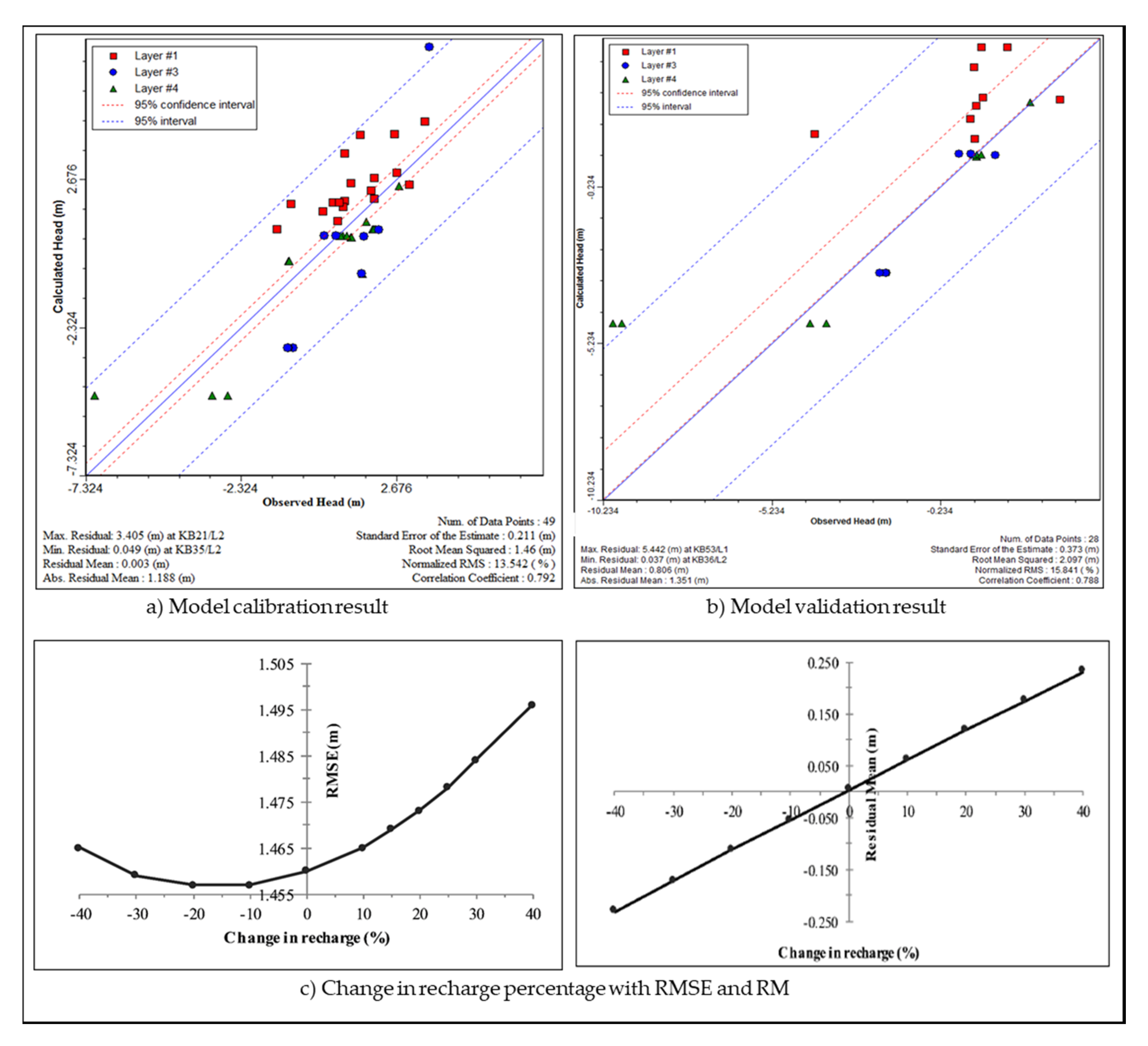
4.4. Groundwater Modelling, GM(WB)
5. Discussion
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- UNESCO. Water for a Sustainable World: Facts and Figures. In The United Nations World Water Development Report 2015; Franek, E.K.A., Connor, R., Hunziker, D., Eds.; United Nations World Water Assessment Programme: Perugia, Italy, 2015. [Google Scholar]
- NGWA. Facts about Global Groundwater Usage. National Groundwater Association, 2016. Available online: http://www.ngwa.org/Fundamentals/Documents/global-groundwater-use-fact-sheet.pdf (accessed on 20 April 2018).
- Famiglietti, J.S. The Global Groundwater Crisis. Nat. Clim. Chang. 2014, 4, 945–948. [Google Scholar] [CrossRef]
- Elias, M.; Turmon, M.; Reager, J.; Hobbs, J.; Liu, Z.; David, C.H. Cascading Dynamics of the Hydrologic Cycle in California Explored through Observations and Model Simulations. Geosciences 2020, 10, 71. [Google Scholar]
- Zhen, L.; Liu, P.W.; Massoud, E.; Farr, T.G.; Lundgren, P.; Famiglietti, J.S. Monitoring Groundwater Change in California’s Central Valley Using Sentinel-1 and Grace Observations. Geosciences 2019, 9, 436. [Google Scholar]
- Massoud, E.C.; Purdy, A.J.; Miro, M.E.; Famiglietti, J.S. Projecting Groundwater Storage Changes in California’s Central Valley. Sci. Rep. 2018, 8, 12917. [Google Scholar] [CrossRef] [PubMed]
- Babel, M.S.; Gupta, A.D.; Domingo, N.D.S. Land Subsidence: A Consequence of Groundwater over-Exploitation in Bangkok, Thailand. Int. Rev. Environ. Strateg. 2006, 6, 307–328. [Google Scholar]
- Delinom, R.M. Groundwater Management Issues in the Greater Jakarta. In Proceedings of the International Workshop on Integrated Watershed Management for Sustainable Water Use in a Humid Tropical Region, JSPS-DGHE Joint Research Project, University of Tsukuba, Tsukuba, Japan, 31 October 2007. [Google Scholar]
- Taylor, R.G.; Burgess, W.G.; Shamsudduha, M.; Zahid, A.; Lapworth, D.J.; Ahmed, K.; Mukherjee, A.; Nowreen, S. Deep Groundwater in the Bengal Mega Delta: New Evidence of Aquifer Hydraulics and the Influence of Intensive Abstraction. In Groundwater Science Programme; British Geological Survey: Nottingham, UK, 2014; Volume 14. [Google Scholar]
- Ha, K.C.; Ngoc, N.T.M.; Lee, E.H.; Jayakumar, R. Current Status and Issues of Groundwater in the Mekong River Basin; Korea Institute of Geoscience and Mineral Resources: Daejeon, Korea, 2016; Volume 121. [Google Scholar]
- Wada, Y.; Bierkens, M.F. Sustainability of Global Water Use: Past Reconstruction and Future Projections. Environ. Res. Lett. 2014, 9, 104003. [Google Scholar] [CrossRef]
- Kim, S.H.; Hejazi, M.; Liu, L.; Calvin, K.; Clarke, L.; Edmonds, J. Balancing Global Water Availability and Use at Basin Scale in an Integrated Assessment Model. Clim. Chang. 2016, 136, 217–231. [Google Scholar] [CrossRef]
- Turner, S.W.D.; Hejazi, M.; Yonkofski, C.; Kim, S.H.; Kyle, P. Influence of Groundwater Extraction Costs and Resource Depletion Limits on Simulated Global Nonrenewable Water Withdrawals over the Twenty-First Century. Earth’s Future 2019, 7, 123–135. [Google Scholar] [CrossRef]
- de Vries, J.J.; Simmers, I. Groundwater Recharge: An Overview of Processes and Challenges. Hydrogeol. J. 2002, 10, 5–17. [Google Scholar] [CrossRef]
- Nimmo, J.R.; Healy, R.W.; Stonestrom, D.A. Aquifer Recharge. In Encyclopedia of Hydrological Science: Part 13. Groundwater; Anderson, M.G., Bear Anderson, J., Eds.; Wiley: Chichester, UK, 2005; pp. 2229–2246. [Google Scholar]
- Healy, R.W. Estimating Groundwater Recharge; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Simmers, I. Groundwater Recharge Principles, Problems and Developments. In Recharge of Phreatic Aquifers in (Semi-)Arid Areas; Simmers, I., Ed.; A.A. Balkema: Rotterdam, The Netherlands, 1997; pp. 1–18. [Google Scholar]
- Scanlon, B.R.; Healy, R.W.; Cook, P.G. Choosing Appropriate Techniques for Quantifying Groundwater Recharge. Hydrogeol. J. 2002, 10, 18–39. [Google Scholar] [CrossRef]
- Dripps, W.R.; Bradbury, K.R. A Simple Daily Soil–Water Balance Model for Estimating the Spatial and Temporal Distribution of Groundwater Recharge in Temperate Humid Areas. Hydrogeol. J. 2007, 15, 433–444. [Google Scholar] [CrossRef]
- Barron, O.V.; Crosbie, R.S.; Dawes, W.R.; Charles, S.P.; Pickett, T.; Donn, M.J. Climatic Controls on Diffuse Groundwater Recharge across Australia. Hydrol. Earth Syst. Sci. 2012, 16, 4557–4570. [Google Scholar] [CrossRef]
- Moeck, C.; Grech-Cumbo, N.; Podgorski, J.; Bretzler, A.; Gurdak, J.J.; Berg, M.; Schirmer, M. A Global-Scale Dataset of Direct Natural Groundwater Recharge Rates: A Review of Variables, Processes and Relationships. Sci. Total Environ. 2020, 717, 137042. [Google Scholar] [CrossRef] [PubMed]
- Jayakumar, R.; Lee, E. Climate Change and Groundwater Conditions in the Mekong Region–a Review. J. Groundw. Sci. Eng. 2017, 5, 14–30. [Google Scholar]
- Hepburn, E.; Cendón, D.I.; Bekele, D.; Currell1, M. Environmental Isotopes as Indicators of Groundwater Recharge, Residence Times and Salinity in a Coastal Urban Redevelopment Precinct in Australia. Hydrogeol. J. 2019, 28, 503–520. [Google Scholar] [CrossRef]
- Minnig, M.; Moeck, C.; Radny, D.; Schirmer, M. Impact of Urbanization on Groundwater Recharge Rates in Dübendorf, Switzerland. J. Hydrol. 2018, 563, 1135–1146. [Google Scholar] [CrossRef]
- Dillon, P.; Stuyfzand, P.; Grischek, T.; Lluria, M.; Pyne, R.D.G.; Jain, R.C.; Bear, J.; Schwarz, J.; Wang, W.; Fernandez, E.; et al. Sixty Years of Global Progress in Managed Aquifer Recharge. Hydrogeol. J. 2019, 27, 1–30. [Google Scholar] [CrossRef]
- Sallwey, J.; Valverde, J.P.B.; López, F.V.; Junghanns, R.; Stefan, C. Suitability Maps for Managed Aquifer Recharge: A Review of Multi-Criteria Decision Analysis Studies. Environ. Rev. 2019, 27, 138–150. [Google Scholar] [CrossRef]
- Lerner, D.N.; Issar, A.; Simmers, I. Groundwater Recharge; a Guide to Understanding and Estimating Natural Recharge; International Contributions to Hydrogeology; Heise: Hannover, Germany, 1990; Volume 8. [Google Scholar]
- Hendrickx, J.M.H.; Walker, G.R. Recharge from Precipitation. In Recharge of Phreatic Aquifers in (Semi-) Arid Areas; Simmers, I., Ed.; A.A. Belkema: Rotterdam, The Netherlands; Brookfield, VT, USA, 1997. [Google Scholar]
- Delin, G.N.; Risser, D.W. Ground-Water Recharge in Humid Areas of the United States—A Summary of Ground-Water Resources Program Studies, 2003–2006; U.S. Department of the Interior, U.S. Geological Survey, Eds.; U.S. Geological Survey: Reston, VA, USA, 2007.
- Takounjou, A.F.; Ngoupayou, J.R.N.; Riotte, J.; Takem, G.E.; Mafany, G.; Maréchal, J.C.; Ekodeck, G.E. Estimation of Groundwater Recharge of Shallow Aquifer on Humid Environment in Yaounde, Cameroon Using Hybrid Water-Fluctuation and Hydrochemistry Methods. Environ. Earth Sci. 2011, 64, 107–118. [Google Scholar] [CrossRef]
- Majumder, R.K.; Shimada, J.; Taniguchi, M. Groundwater Flow Systems in the Bengal Delta, Bangladesh, Inferred from Subsurface Temperature Readings. Songklanakarin J. Sci. Technol. 2013, 35, 99–106. [Google Scholar]
- Vishal, V.; Kumar, S.; Singhal, D.C. Estimation of Groundwater Recharge in National Capital Territory, Delhi Using Groundwater Modeling. J. Indian Water Resour. Soc. 2014, 34, 15–23. [Google Scholar]
- Flint, A.L.; Flint, L.E.; Kwicklis, E.M.; Fabryka-Martin, J.T.; Bodvarsson, G.S. Estimating Recharge at Yucca Mountain, Nevada, USA: Comparison of Methods. Hydrogeol. J. 2002, 10, 180–204. [Google Scholar] [CrossRef]
- Verma, P.; Singh, P.; Srivastava, S.K. Development of Spatial Decision-Making for Groundwater Recharge Suitability Assessment by Considering Geoinformatics and Field Data. Arabian J. Geosci. 2020, 13, 306. [Google Scholar] [CrossRef]
- Senthilkumar, M.; Gnanasundar, D.; Arumugam, R. Identifying Groundwater Recharge Zones Using Remote Sensing & Gis Techniques in Amaravathi Aquifer System, Tamil Nadu, South India. Sustain. Environ. Res. 2019, 29, 15. [Google Scholar]
- Walker, D.; Parkin, G.; Schmitter, P.; Gowing, J.; Tilahun, S.A.; Haile, A.T.; Yimam, A.Y. Insights from a Multi-Method Recharge Estimation Comparison Study. Groundwater 2018, 57, 245–258. [Google Scholar] [CrossRef]
- DID. National Water Resources Study 2000–2050 (Malaysian Peninsula). Groundwater Studies; Department of Irrigation and Drainage Malaysia: Kuala Lumpur, Malaysia, 2000. [Google Scholar]
- Wan Ismail, W.M.Z. Groundwater for Public Water Supply. In Proceedings of the National Groundwater Conference 2019, Concarde Hotel, Shah Alam, Selangor, Malaysia, 2–3 July 2019. [Google Scholar]
- Md Hashim, M.A. Geologi Dan Geomorfologi Kawasan Kuala Besar Hingga Kuala Pengkalan Besar Dengan Penekanan Permatang-Permatang Holosen Dan Perubahan Pantai. Ph.D. Thesis, University of Malaya, Kuala Lumpur, Malaysia, 2002. [Google Scholar]
- Soh, Z.A. The Geomorphology of Kelantan Delta, Malaysia. Ph.D. Thesis, University of Malaya, Kuala Lumpur, Malaysia, 1972. [Google Scholar]
- Noor, I.M. Prefeasibility Study of Potential Groundwater Development in Kelantan, Malaysia. Ph.D. Thesis, University of Birmingham, Birmingham, UK, 1980. [Google Scholar]
- Suratman, S. Groundwater Protection in North Kelantan, Malaysia: An Integrated Mapping Approach Using Modeling and Gis. Ph.D. Thesis, University of Upon Tyne, Newcastle Upon Tyne, UK, 1997. [Google Scholar]
- Udie Lmasudin, M.I. Kajian Taburan Enapan Resen Dan Perubahan Pesisir Pantai, Kota Bharu—Kuala Telong, Kelantan Darul Naim. Ph.D. Thesis, University of Malaya, Kuala Lumpur, Malaysia, 2000. [Google Scholar]
- Tjia, H.D. Quaternary. In Geology of Peninsular Malaysia; Hutchison, C.S., Tan, D.N.K., Eds.; University of Malaya and Geological Society of Malaysia: Kuala Lumpur, Malaysia, 1973. [Google Scholar]
- MGD. Hydrogeological Map; Minerals and Geoscience Deaprtment, Malaysia (Ministry of Natural Resources and Environment): Kuala Lumpur, Malaysia, 2008. [Google Scholar]
- Sofner, B. Groundwater Monitoring and Groundwater Protection at the Geological Survey Department (Gsd) of Malaysia; Geological Survey Department of Malaysia: Kuala Lumpur, Malaysia, 1992. [Google Scholar]
- MGD. Geological Map of Peninsular Malaysia, 9th ed.; Minerals and Geoscience Malaysia: Kuala Lumpur, Malaysia, 2014. [Google Scholar]
- Bazuhair, A.S.; Wood, W.W. Chloride Mass-Balance Method for Estimating Ground Water Recharge in Arid Areas: Examples from Western Saudi Arabia. J. Hydrol. 1996, 186, 153–159. [Google Scholar] [CrossRef]
- Wood, W.W.; Sanford, W.E. Chemical and Isotopic Methods for Quantifying Groundwater Recharge in a Regional, Semiarid Environment. Ground Water 1995, 33, 458–468. [Google Scholar] [CrossRef]
- Subyani, A.M. Use of Chloride-Mass Balance and Environmental Isotopes for Evaluation of Groundwater Recharge in the Alluvial Aquifer, Wadi Tharad, Western Saudi Arabia. Environ. Geol. 2004, 46, 741–749. [Google Scholar] [CrossRef]
- Dassi, L. Use of Chloride Mass Balance and Tritium Data for Estimation of Groundwater Recharge and Renewal Rate in an Unconfined Aquifer from North Africa: A Case Study from Tunisia. Environ. Earth Sci. 2010, 60, 861–871. [Google Scholar] [CrossRef]
- Eriksson, E.; Khunakasem, V. Chloride Concentration in Groundwater, Recharge Rate and Rate of Deposition of Chloride in Israel Coastal Plain. J. Hydrol. 1969, 7, 178–197. [Google Scholar] [CrossRef]
- Ifediegwu, I.S. Groundwater Recharge Estimation Using Chloride Mass Balance: A Case Study of Nsukka Local Government Area of Enugu State, Southeastern, Nigeria. Model. Earth Syst. Environ. 2020, 6, 799–810. [Google Scholar] [CrossRef]
- Ting, C.S.; Kerh, T.; Liao, C.J. Estimation of Groundwater Recharge Using the Chloride Mass-Balance Method, Pingtung Plain, Taiwan. Hydrogeol. J. 1998, 6, 282–292. [Google Scholar] [CrossRef]
- Carrier, M.A.; Lefebvre, R.; Asare, E.B. Groundwater Recharge Assessment in Northern Ghana Using Soil Moisture Balance and Chloride Mass Balance. In Proceedings of the 61st Canadian Geotechnical Conference and 9th Joint CGS/IAH-CNC Groundwater Conference, Edmonton, AB, Canada, 21–24 September 2008. [Google Scholar]
- Scanlon, B.R.; Stonestrom, D.A.; Reedy, R.C.; Leaney, F.W.; Gates, J.; Cresswell, R.G. Inventories and Mobilization of Unsaturated Zone Sulfate, Fluoride, and Chloride Related to Land Use Change in Semiarid Regions, Southwestern United States and Australia. Water Resour. Res. 2009, 45, W00A18. [Google Scholar] [CrossRef]
- Bresciani, E.; Ordens, C.M.; Werner, A.D.; Batelaan, O.; Guan, H.; Post, V.E.A. Spatial Variability of Chloride Deposition in a Vegetated Coastal Area: Implications for Groundwater Recharge Estimation. J. Hydrol. 2014, 519, 1177–1191. [Google Scholar] [CrossRef]
- Guan, H.D.; Love, A.J.; Simmons, C.T.; Makhnin, O.; Kayaalp, A.S. Factors Influencing Chloride Deposition in a Coastal Hilly Area and Application to Chloride Deposition Mapping. Hydrol. Earth Syst. Sci. 2010, 14, 801–813. [Google Scholar] [CrossRef]
- Gobinddass, M.L.; Molinie, J.; Richard, S.; Panechou, K.; Jeannot, A.; Jean-Louis, S. Coastal Sea Salt Chlorine Deposition Linked to Intertropical Convergence Zone (Itcz) Oscillation in French Guiana. J. Atmos. Sci. 2020, 77, 1723–1731. [Google Scholar] [CrossRef]
- Gaye, C.B.; Edmunds, W.M. Groundwater Recharge Estimation Using Chloride, Stable Isotopes and Tritium Profiles in the Sands of Northwestern Senegal. Environ. Geol. 1996, 27, 246–251. [Google Scholar] [CrossRef]
- Mensah, F.O.; Alo, C.; Yidana, S.M. Evaluation of Groundwater Recharge Estimates in a Partially Metamorphosed Sedimentary Basin in a Tropical Environment: Application of Natural Tracers. Sci. World J. 2014, 2014, 419508. [Google Scholar]
- Wood, W. Interactive Comment on “Theory of the Generalized Chloride Mass Balance Method for Recharge Estimation in Groundwater Basins Characterised by Point and Diffuse Recharge” by N. Somaratne and K. R. J. Smettem. Hydrol. Earth Syst. Sci. Discuss. 2014, 11, C19–C21. [Google Scholar]
- Scanlon, B. Evaluation of Moisture Flux from Chloride Data in Desert Soils. J. Hydrol. 1991, 128, 137–156. [Google Scholar] [CrossRef]
- Meinzer, O.E. The Occurence of Groundwater in the United States with a Discussion of Principles. (Water Supply Paper 489); United States Government Printing Office: Washington, DC, USA, 1923. [Google Scholar]
- Delin, G.N.; Healy, R.W.; Lorenz, D.L.; Nimmo, J.R. Comparison of Local- to Regional-Scale Estimates of Ground-Water Recharge in Minnesota, USA. J. Hydrol. 2007, 334, 231–249. [Google Scholar] [CrossRef]
- Hall, B.; Currell, M.; Webb, J. Using Multiple Lines of Evidence to Map Groundwater Recharge in a Rapidly Urbanising Catchment: Implications for Future Land and Water Management. J. Hydrol. 2020, 580, 124265. [Google Scholar] [CrossRef]
- Crosbie, R.S.; Binning, P.; Kalma, J.D. A Time Series Approach to Inferring Groundwater Recharge Using the Water Table Fluctuation Method. Water Resour. Res. 2005, 41, 1–21. [Google Scholar] [CrossRef]
- Obuobie, E.; Diekkrueger, B.; Agyekum, W.; Agodzo, S. Groundwater Level Monitoring and Recharge Estimation in the White Volta River Basin of Ghana. J. Afr. Earth Sci. 2012, 71–72, 80–86. [Google Scholar] [CrossRef]
- Moon, S.K.; Woo, N.C.; Lee, K.S. Statistical Analysis of Hydrographs and Water-Table Fluctuation to Estimate Groundwater Recharge. J. Hydrol. 2004, 292, 198–209. [Google Scholar] [CrossRef]
- Healy, R.W.; Cook, P.G. Using Groundwater Levels to Estimate Recharge. Hydrogeol. J. 2002, 10, 91–109. [Google Scholar] [CrossRef]
- Labrecque, G.; Chesnaux, R.; Boucher, M. Water-Table Fluctuation Method for Assessing Aquifer Recharge: Application to Canadian Aquifers and Comparison with Other Methods. Hydrogeol. J. 2020, 28, 521–533. [Google Scholar] [CrossRef]
- Varni, M.R. Application of Several Methodologies to Estimate Groundwater Recharge in the Pampeano Aquifer, Argentina. Tecnol. Y Cienc. Del Agua 2013, 4, 67–85. [Google Scholar]
- Hung Vu, V.; Merkel, B.J. Estimating Groundwater Recharge for Hanoi, Vietnam. Sci. Total Environ. 2019, 651, 1047–1057. [Google Scholar] [CrossRef]
- Yang, L.; Qi, Y.; Zheng, C.; Andrews, C.B.; Yue, S.; Lin, S.; Li, Y.; Wang, C.; Xu, Y.; Li, H.A. A Modified Water-Table Fluctuation Method to Characterize Regional Groundwater Discharge. Water 2018, 10, 503. [Google Scholar] [CrossRef]
- Suzuki, K. Percolation Measurements Based on Heat Flow through Soil with Special Reference to Paddy Fields. J. Geophys. Res. 1960, 65, 2883–2885. [Google Scholar] [CrossRef]
- Bredehoeft, J.D.; Papadopulos, I.S. Rates of Vertical Groundwater Movement Estimated from Earth’s Thermal Profile. Water Resour. Res. 1965, 1, 325–328. [Google Scholar] [CrossRef]
- Stallman, R.W. Steady One Dimensional Fluid Flow in the Semi-Infinate Porous Medium with Sinusoidal Surface Temperature. J. Geophys. Res. 1965, 70, 2821–2827. [Google Scholar] [CrossRef]
- Carslaw, H.S.; Jaeger, J.C. Conduction of Heat in Solids, 2nd ed.; Oxford University Press: Oxford, UK, 1959. [Google Scholar]
- Constantz, J. Interaction between Streambed Temperature, Streamflow, and Groundwater Exchanges in Alpine Streams. Water Resour. Res. 1998, 34, 1609–1615. [Google Scholar] [CrossRef]
- Hatch, C.E.; Fischer, A.T.; Revenaugh, J.S.; Constantz, J.; Ruehl, C. Quantifying Surface Water/Groundwater Interaction Using Time Series Analysis of Streambed Thermal Records: Method Development. Water Resour. Res. 2006, 42, W10410. [Google Scholar] [CrossRef]
- Glose, T.J.; Lowry, C.S.; Hausner, M.B. Limits on Groundwater-Surface Water Fluxes Derived from Temperature Time Series: Defining Resolution-Based Thresholds. Water Resour. Res. 2019, 55, 1–12. [Google Scholar] [CrossRef]
- Taniguchi, M. Estimations of the Past Groundwater Recharge Rate from Deep Borehole Temperature Data. Catena 2002, 48, 39–51. [Google Scholar] [CrossRef]
- Taniguchi, M.; Shimada, J.; Tanaka, T.; Kayane, I.; Sakura, Y.; Shimano, Y.; Dapaah-Siakwan, S.; Kawashima, S. Disturbances of Temperature-Depth Profilesdue to Surface Climate Change and Subsurface Water Flow: (1) an Effect of Linear Increase in Surface Temperature Caused by Global Worming and Urbanization in the Tokyo Metropolitan Area, Japan. Water Resour. Res. 1999, 35, 1507–1517. [Google Scholar] [CrossRef]
- Taniguchi, M.; Williamson, D.R.; Peck, A.J. Disturbances of Temperature–Depth Profiles Due to Surface Climate-Change and Subsurface Water Flow: (2) an Effect of Step Increase in Surface Temperature Caused by Forest Clearing in Southwest of Western Australia. Water Resour. Res. 1999, 35, 1519–1529. [Google Scholar] [CrossRef]
- Dong, L.; Fu, C.; Liu, J.; Wang, Y. Disturbances of Temperature-Depth Profiles by Surface Warming and Groundwater Flow Convection in Kumamoto Plain, Japan. Geofluids 2018, 2018, 8451276. [Google Scholar] [CrossRef]
- Irvine, D.J.; Kurylyk, B.L.; Cartwright, I.; Bonham, M.; Post, V.E.A.; Banks, E.W.; Simmons, C.T. Groundwater Flow Estimation Using Temperature-Depth Profiles in a Complex Environment and a Changing Climate. Sci. Total Environ. 2017, 574, 272–281. [Google Scholar] [CrossRef] [PubMed]
- Dapaah-Siakwan, S.; Kayane, I. Estimation of Vertical Water and Heat Fluxes in the Semi-Confined Aquifers in Tokyo Metropolitan Area, Japan. Hydrol. Process. 1995, 9, 143–160. [Google Scholar] [CrossRef]
- Taniguchi, M.; Shimada, J.; Uemura, T. Transient Effects of Surface Temperature Anf Groundwater Flow on Subsurface Temperature in Kumamoto Plain, Japan. Phys. Chem. Earth 2003, 28, 477–486. [Google Scholar] [CrossRef]
- Li, S.; Dong, L.; Chen, J.; Li, R.; Yang, Z.; Liang, Z. Vertical Groundwater Flux Estimation from Borehole Temperature Profiles by a Numerical Model, Rflux. Hydrol. Process. 2019, 33, 1542–1552. [Google Scholar] [CrossRef]
- Taniguchi, M. Evaluation of Vertical Groundwater Fluxes and Thermal Properties of Aquifer Based on Transient Temperature-Depth Profiles. Water Resour. Res. 1993, 29, 2021–2026. [Google Scholar] [CrossRef]
- Ingebritsen, S.E.; Sanford, W.E.; Neuzil, C.E. Groundwater in Geological Processes, 2nd ed.; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Kurylyk, B.L.; Irvine, D.J.; Carey, S.K.; Briggs, M.A.; Werkema, D.D.; Bonham, M. Heat as a Groundwater Tracer in Shallow and Deep Heterogeneous Media: Analytical Solution, Spreadsheet Tool, and Field Applications. Hydrol. Process. 2017, 31, 2648–2661. [Google Scholar] [CrossRef]
- Suzuki, A.; Ikhwanda, F.; Yamaguchi, A.; Hashida, T. Estimations of Fracture Surface Area Using Tracer and Temperature Data in Geothermal Fields. Geosciences 2019, 9, 425. [Google Scholar] [CrossRef]
- Madon, M. Regional Tectonics and Sedimentary Basins of Malaysia: Basin Types, Tectono-Stratigraphy Provinces, Structural Styles. In The Petroleum Geology and Resorces of Malaysia; PETRONAS, Ed.; PETRONAS: Kuala Lumpur, Malaysia, 1999; pp. 79–111. [Google Scholar]
- Taniguchi, M.; Shimano, Y.; Kayane, I. Groundwater Flow Analysis Using the Temperature in the Upland Areas at the Western Foot of Aso Volcanoes. Journal of Japan Assoc. Hydrol. Sci. 1989, 19, 171–179. [Google Scholar]
- Kurylyk, B.L.; Irvine, D.J.; Bense, V.F. Theory, Tools, and Multidisciplinary Applications for Tracing Groundwater Fluxes from Temperature Profiles. WIREs Water 2019, 6, 1329. [Google Scholar] [CrossRef]
- Sudhakar, S.; Verma, M.K.; Soumya, S. Application of Gis and Modflow to Ground Water Hydrology—A Review. Int. J. Eng. Res. Appl. 2016, 6, 36–42. [Google Scholar]
- Hariharan, V.; Shankar, M.U. A Review of Visual Modflow Applications in Groundwater Modelling. In IOP Conf. Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2017; p. 032025. [Google Scholar]
- Pathak, R.; Awasthi, M.K.; Sharma, S.K.; Hardaha, M.K.; Nema, R.K. Ground Water Flow Modelling Using Modflow—A Review. Int. J. Curr. Microbiol. Appl. Sci. 2018, 7, 83–88. [Google Scholar] [CrossRef]
- Panda, P.; Narasimham, M.L. A Review on Modelling and Simulation of Ground Water Resources in Urban Regions. Infokara Res. 2020, 9, 235–244. [Google Scholar]
- Harbaugh, A.W. Modflow-2005: The U.S. Geological Survey Modular Ground-Water Model—The Ground-Water Flow Process. In Techniques and Methods 6–A16; US Geological Survey: Reston, VA, USA, 2005. [Google Scholar]
- Waterloo Hydrogeologic. Visual Modflow Help. Waterloo Hydrogeologic. Available online: https://www.waterloohydrogeologic.com/help/vmod/ (accessed on 27 June 2020).
- Thompson, C.E. Hydrologic Functioning of Glacial Moraine Landscapes within Alberta’s Boreal Plains. Ph.D. Thesis, University of Alberta, Edmonton, AB, Canada, 2019. [Google Scholar]
- Hydrogeologic Inc. (HGL). Modhms/Modflow-Surfact: A Comprehensive Modflow-Based Hydrologic Modeling System; HydroGeoLogic, Inc.: Reston, VA, USA, 2015. [Google Scholar]
- Waterloo Hydrogeologic. Visual Modflow V. 4.1 User’s Manual; Waterloo Hydrogeologic: Waterloo, ON, Canada, 2005. [Google Scholar]
- Hussin, N.H. Hydrogeochemical Study and Iron Removal of Groundwater in North Kelantan. Ph.D. Thesis, University of Malaya, Kuala Lumpur, Malaysia, 2011. [Google Scholar]
- ASTM. Standard Guide for Application of a Groundwater Flow Model to a Site-Specific Problem. In Astm Standards Related to Environment Site Characterization, 3rd ed.; Arendt, S.A., Bailey, S.J., Baldini, N.C., Eds.; ASTM: Baltimore, MD, USA, 2006. [Google Scholar]
- Anderson, M.P.; Woessner, W.W. Applied Groundwater Modelling: Simulation of Flow and Advective Transport; Academic Press: San Diego, CA, USA, 1992. [Google Scholar]
- Liu, G.Q.; Wang, Y.S.; Zhang, Y.F.; Song, T. Application of Chloride Profile and Water Balance Methods in Estimating Groundwater Recharge in Luanjing Irrigation Area, Inner Mongolia. Hydrol. Sci. J. 2009, 54, 961–973. [Google Scholar] [CrossRef]
- Huang, T.M.; Pang, Z.H. Estimating Groundwater Recharge Following Land-Use Change Using Chloride Mass Balance of Soil Profiles: A Case Study at Guyuan and Xifeng in the Loess Plateau of China. Hydrogeol. J. 2011, 19, 177–186. [Google Scholar] [CrossRef]
- Tesfaldet, Y.T.; Puttiwongrak, A.; Arpornthip, T. Spatial and Temporal Variation of Groundwater Recharge in Shallow Aquifer in the Thepkasattri of Phuket, Thailand. J. Groundw. Sci. Eng. 2019, 8, 10–19. [Google Scholar]
- Weeks, E.P. The Lisse Effect Revisited. Groundwater 2002, 40, 652–656. [Google Scholar] [CrossRef]
- Awadalla, S.; Noor, I.B.M.; Ibrahim, I. Comparative Model Study for Groundwater Resources Evaluation in Kelantan Malaysia; Part 1: Model Evaluation. In Proceedings of the International Workshop on Appropriate Methodologies for Development and Management of Groundwater Resources in Developing Countries, Hyderabad, India, 28 February–4 March 1989. [Google Scholar]
- Hansen, J.; Ruedy, R.; Sato, M.; Lo, K. Global Surface Temperature Change. Rev. Geophys. 2010, 48, 1–29. [Google Scholar] [CrossRef]
- Gosnold, W.D.; Todhunter, P.E.; Schmidt, W. The Borehoe Temperature Record of Climate Warming in the Mid-Continent of North America. Glob. Planet. Chang. 1997, 15, 33–45. [Google Scholar] [CrossRef]
- Gunawardhana, L.N.; Kazama, S. Statistical and Numerical Analyses of the Influence of Climate Variability on Aquifer Water Levels and Groundwater Temperatures: The Impacts of Climate Change on Aquifer Thermal Regimes. Glob. Planet. Chang. 2012, 86–87, 66–78. [Google Scholar] [CrossRef]
- Taniguchi, M.; Turner, J.V.; Smith, A.J. Evaluation of Groundwater Discharge Rates from Subsurface Temperature in Cockburn Sound, Western Australia. Biogeochemistry 2003, 66, 111–124. [Google Scholar] [CrossRef]
- Salem, Z.E.-S. Subsurface Thermal Regime to Delineate the Paleo-Groundwater Flow System in an Arid Area, Al Kufra, Libya. J. Astron. Geophys. 2016, 5, 451–462. [Google Scholar] [CrossRef]
- Domenico, A.P.; Palciauskus, V.V. Theoretical Analysis of Forced Convective Heat Transfer in Regional Groundwater Flow. Geol. Soc. Am. Bull. 1973, 84, 3803–3814. [Google Scholar] [CrossRef]
- Hiscock, K.M.; Bense, V.F. Hydrogeology: Principles and Practice, 2nd ed.; John Wiley & Sons, Ltd.: West Sussex, UK, 2014. [Google Scholar]
- Colombani, N.; Giambastiani, B.M.S.; Mastrocicco, M. Use of Shallow Groundwater Temperature Proflies to Infer Climate and Land Use Change: Interpretation and Measurement Challenges. Hydrol. Process. 2016, 30, 10805. [Google Scholar] [CrossRef]
- Bense, V.F.; Kooi, H. Temporal and Spatial Variations of Shallow Subsurface Temperature as a Record of Lateral Variations in Groundwater Flow. J. Geophys. Res. 2004, 9, B04103. [Google Scholar] [CrossRef]
- Harris, R.N.; Gosnold, W.D. Comparisons of Borehole Temperature-Depth Profiles and Surface Air Temperatures in the Northern Plain of the USA. Geophys. J. Int. 1999, 138, 541–548. [Google Scholar] [CrossRef]
- Lenhart, T.; Eckhardt, K.; Fohrer, N.; Frede, H.-G. Comparison of Two Different Approaches of Sensitivity Analysis. Phys. Chem. Earth Part B-Hydrol. Oceans Atmos. 2002, 27, 645–654. [Google Scholar] [CrossRef]
- Sukhija, B.S.; Reddy, D.V.; Nagabhushanam, P.; Hussain, S. Recharge Processes: Piston Flow Vs Preferential Flow in Semi-Arid Aquifers of India. Hydrogeol. J. 2003, 11, 387–395. [Google Scholar] [CrossRef]
- Deng, L.; Wang, W.; Hu, A. Estimation of Groundwater Recharge by Chloride Mass Balance (Cmb) Method in a Typical Semiarid Climate of China. In Proceedings of the International Symposium on Water Resource and Environmental Protection, Xi’an, China, 20–22 May 2011. [Google Scholar]
- MGD. Groundwater Modelling for Lower Kelantan River Basin. In 10th Malaysian Plan of National Groundwater Resources Study; Minerals and Geoscience Department of Malaysia: Kelantan, Malaysia, 2014. [Google Scholar]
- Che Sulaiman, M.Y. Pemantauan Pemendapan Tanah Di Kawasan Lembangan Sungai Kelantan. In Rancangan Malaysia Ke Sembilan Kajian Sumber Air Tanah Negara. Kementerian Sumber Asli dan Alam Sekitar; Jabatan Mineral dan Geosains Malaysia: Kota Bharu, Kelantan, Malaysia, 2010. [Google Scholar]
- Song, J.; Chen, X. Variation of Specific Yield with Depth in an Alluvial Aquifer of the Platte River Valley, USA. Int. J. Sediment Res. 2010, 25, 185–193. [Google Scholar] [CrossRef]
- Uchida, Y.; Hayashi, T. Effects of Hydrogeological and Climate Change on the Subsurface Thermal Regime in the Sendai Plain. Phys. Earth Planet. Inter. 2005, 152, 292–304. [Google Scholar] [CrossRef]
- Zhou, Y.; Li, W. A Review of Regional Groundwater Flow Modeling. Geosci. Front. 2011, 2, 205–214. [Google Scholar] [CrossRef]
- Scanlon, B.R.; Keese, K.E.; Flint, A.L.; Flint, L.E.; Gaye, C.B.; Edmunds, W.M.; Simmers, I. Global Synthesis of Groundwater Recharge in Semiarid and Arid Regions. Hydrol. Process. 2006, 20, 3335–3370. [Google Scholar] [CrossRef]

| Code | Depth to Water Level (m) | Chloride Concentration, Cuz (mg/L) | Particle Size Distribution (%) | ||
|---|---|---|---|---|---|
| Sand | Silt | Clay | |||
| 2013 | |||||
| S1 | 1.71 | 8.38–11.60 | 92.47–98.85 | 0.68–1.83 | 0.47–5.70 |
| S2 | 0.65 | 13.27–17.12 | 48.26–98.12 | 0.28–17.13 | 1.60–34.61 |
| S3 | 1.30 | 2.83–10.36 | 94.78–97.45 | 0.37–0.58 | 2.18–4.82 |
| S4 | 0.80 | 5.88–10.89 | 87.63–96.74 | 0.63–3.07 | 2.63–9.30 |
| S5 | 1.26 | 1.66–3.34 | 97.83–98.86 | 1.02–2.03 | 0.12–0.31 |
| 2015 | |||||
| S1 | 1.42 | 2.06–13.27 | 91.42–98.47 | 0.39–1.95 | 1.02–6.63 |
| S2 | 0.51 | 1.40–7.63 | 39.05–99.53 | 0.10–19.44 | 0.37–41.51 |
| S3 | 1.5 | 0.21–15.04 | 93.44–97.14 | 0.22–0.50 | 2.54–6.12 |
| S5 | 1.13 | 0.53–17.60 | 78.91–98.39 | 0.16–6.34 | 1.45–14.75 |
| S6 | 1.65 | 0.05–10.16 | 43.25–98.99 | 0.19–18.97 | 0.82–51.38 |
| S7 | 1.96 | 0.23–6.00 | 31.75–99.80 | 0.02–27.82 | 0.18–50.06 |
| S8 | 0.76 | 0.57–4.97 | 6.61–51.90 | 8.10–26.56 | 34.56–81.98 |
| S9 | 1.22 | 0.46–9.82 | 9.18–80.24 | 3.39–35.17 | 12.56–55.65 |
| S10 | 0.71 | 0.99–6.71 | 63.34–93.50 | 1.04–9.63 | 5.47–27.02 |
| Code | “Mean Chloride in Unsaturated Zone, Cuz (mg/L)” | Mean Weighted Chloride in Rainwater, CP (mg/L) | Rainfall * (mm) | Recharge (mm/yr) | Percent of Rainfall (%) |
|---|---|---|---|---|---|
| S1 | 7.48 | 1.18 | 2235.77 | 352.70 | 16 |
| S2 | 9.31 | 2235.77 | 283.37 | 13 | |
| S3 | 7.51 | 2235.77 | 351.29 | 16 | |
| S4 | 2.15 | 2538.97 | 1393.00 | 55 | |
| S5 | 8.12 | 2235.77 | 324.90 | 15 | |
| S6 | 2.18 | 1932.56 | 1046.06 | 54 | |
| S7 | 1.74 | 1932.56 | 1310.59 | 68 | |
| S8 | 2.07 | 1932.56 | 1101.30 | 57 | |
| S9 | 2.97 | 1932.56 | 767.82 | 40 | |
| S10 | 3.72 | 1932.56 | 612.48 | 32 | |
| Mean | 754.35 | 36 | |||
| Code | Rainfall (mm) | Recharge (mm/yr) | Percent of Rainfall (%) |
|---|---|---|---|
| GW37 | 2771 | 416 | 15 |
| GW38 | 2866 | 510 | 18 |
| GW39 | 2803 | 497 | 18 |
| GW40 | 2803 | 333 | 12 |
| GW41 | 2803 | 398 | 14 |
| GW42 | 2803 | 429 | 15 |
| GW43 | 2737 | 345 | 13 |
| GW44 | 2737 | 370 | 14 |
| GW45 | 2774 | 502 | 18 |
| GW46 | 2921 | 540 | 18 |
| GW47 | 2803 | 540 | 19 |
| GW48 | 2803 | 321 | 11 |
| GW49 | 2641 | 348 | 13 |
| GW50 | 2803 | 404 | 14 |
| Mean | 2790 | 425 ± 79 | 15 |
| Well ID | Aquifer Layer | Flux Rate, U (mm/yr) | Type * | RMSE * |
|---|---|---|---|---|
| 2014 | ||||
| GW47 | L1 | 250 | R | 0.49 |
| GW41 | L1 | 200 | R | 0.99 |
| GW43 | L1 | 300 | R | 1.24 |
| GW53 | L2 | 110 | R | 0.29 |
| GW23 | L2 | 190 | R | 0.38 |
| GW33 | L3 | 100 | R | 0.35 |
| GW51 | L3 | 100 | R | 0.27 |
| GW52 | L3 | 110 | R | 0.21 |
| 2015 | ||||
| GW19 | L2 | 100 | R | 0.10 |
| GW31 | L3 | 100 | R | 0.15 |
| GW55 | L3 | 150 | R | 0.29 |
| GW54 | L3 | 140 | R | 0.26 |
| GW30 | L3 | 120 | R | 0.31 |
| GW34 | L3 | 180 | R | 0.85 |
| GW56 | L3 | 150 | R | 0.22 |
| GW35 | L3 | 100 | R | 0.18 |
| Method | Recharge, % (Percentage of Rainfall) * |
|---|---|
| CMB | 13–68 (36) |
| WTF | 11–19 (15) |
| TDP | 8–11 (10) |
| GM(WB) | 6.6–12.1 [11] |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hussin, N.H.; Yusoff, I.; Raksmey, M. Comparison of Applications to Evaluate Groundwater Recharge at Lower Kelantan River Basin, Malaysia. Geosciences 2020, 10, 289. https://doi.org/10.3390/geosciences10080289
Hussin NH, Yusoff I, Raksmey M. Comparison of Applications to Evaluate Groundwater Recharge at Lower Kelantan River Basin, Malaysia. Geosciences. 2020; 10(8):289. https://doi.org/10.3390/geosciences10080289
Chicago/Turabian StyleHussin, Nur Hayati, Ismail Yusoff, and May Raksmey. 2020. "Comparison of Applications to Evaluate Groundwater Recharge at Lower Kelantan River Basin, Malaysia" Geosciences 10, no. 8: 289. https://doi.org/10.3390/geosciences10080289
APA StyleHussin, N. H., Yusoff, I., & Raksmey, M. (2020). Comparison of Applications to Evaluate Groundwater Recharge at Lower Kelantan River Basin, Malaysia. Geosciences, 10(8), 289. https://doi.org/10.3390/geosciences10080289




