Constraining Basin Parameters Using a Known Subsidence History
Abstract
1. Introduction
2. Numerical Model
- (1)
- Uniform instantaneous stretching for the initial phase is by pure shear;
- (2)
- A constant asthenospheric temperature is maintained at a fixed depth equal to initial position of the base of the lithosphere;
- (3)
- Radiogenic heat production is ignored.
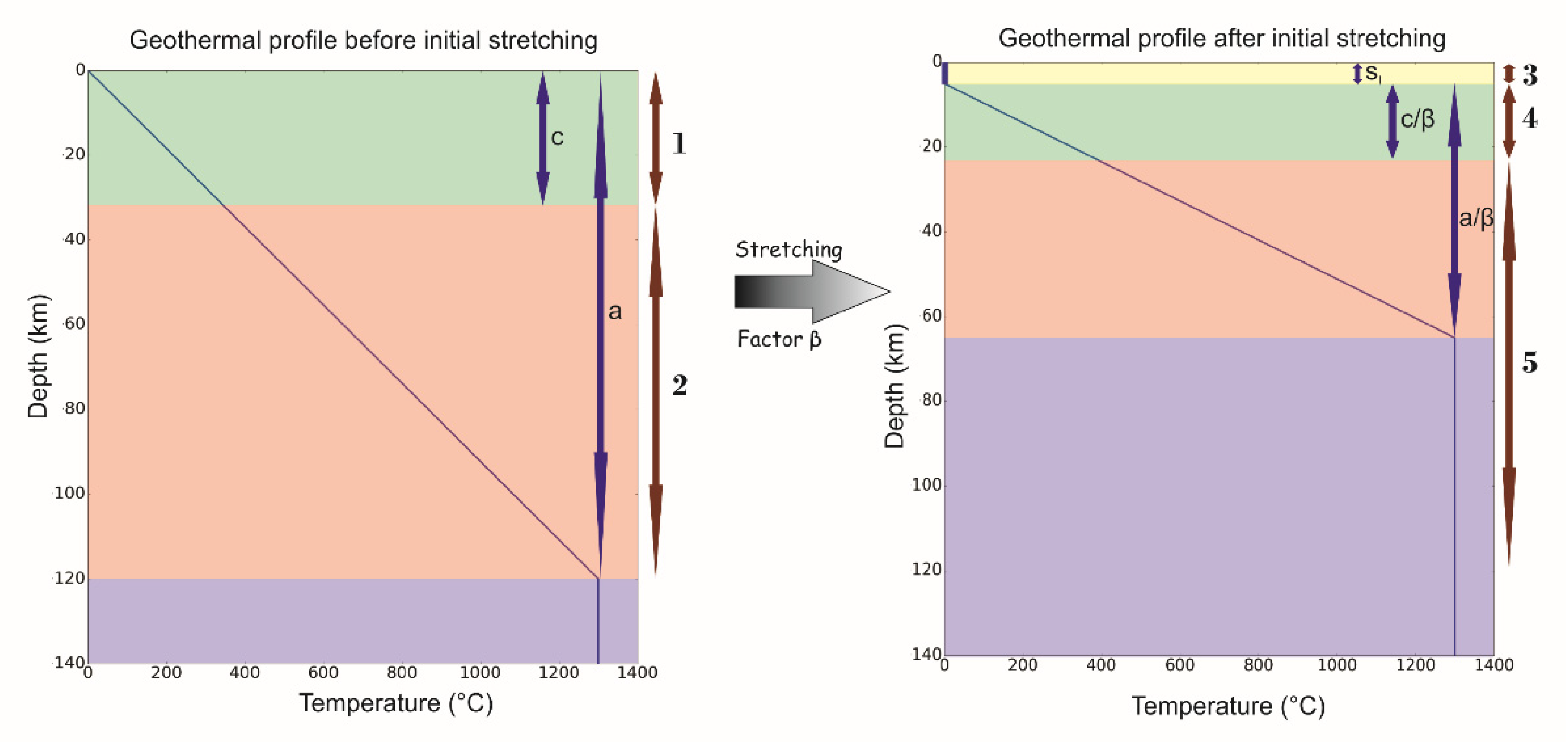
2.1. Initial Stretching
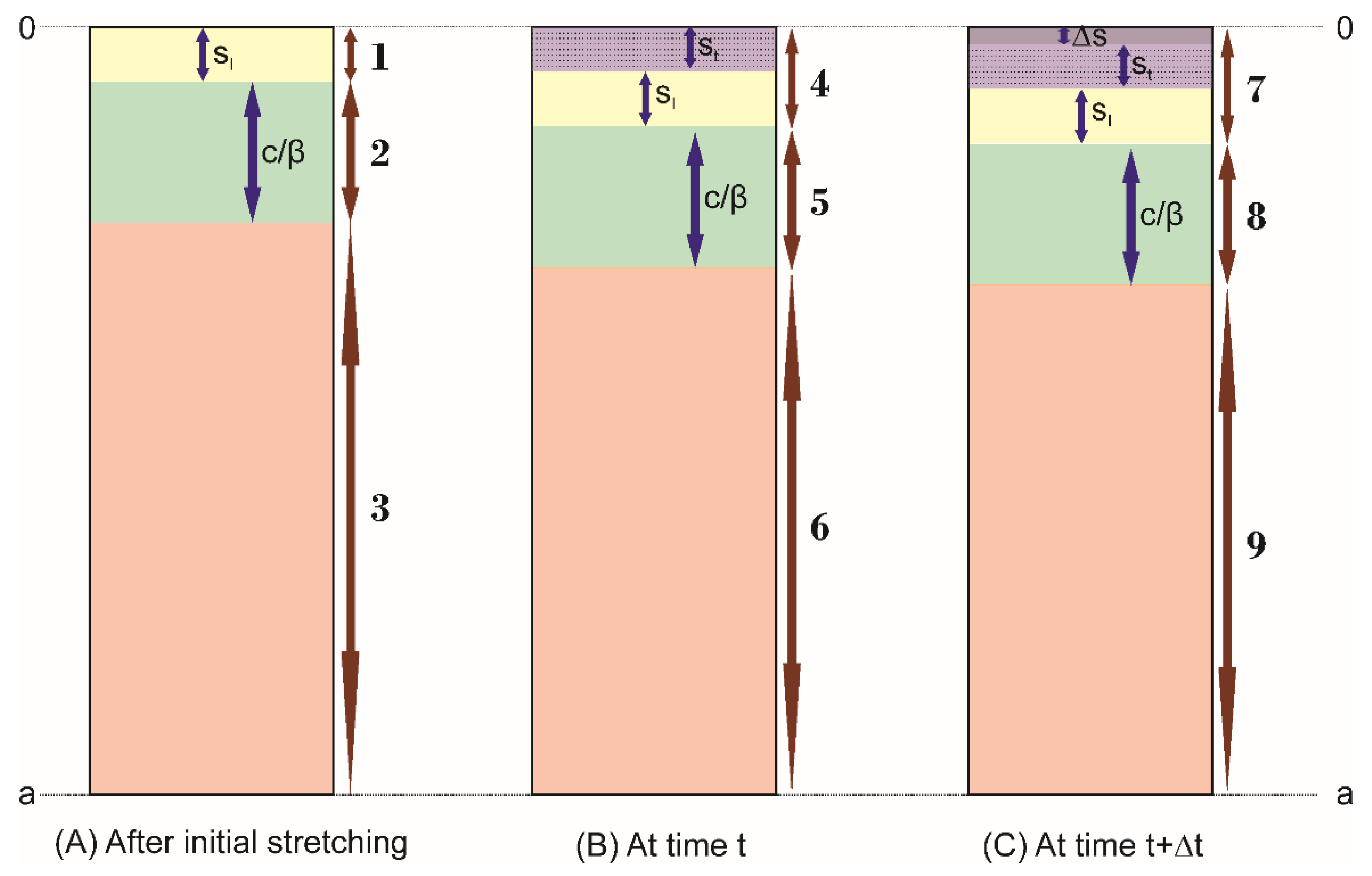
2.2. Gradual Subsidence
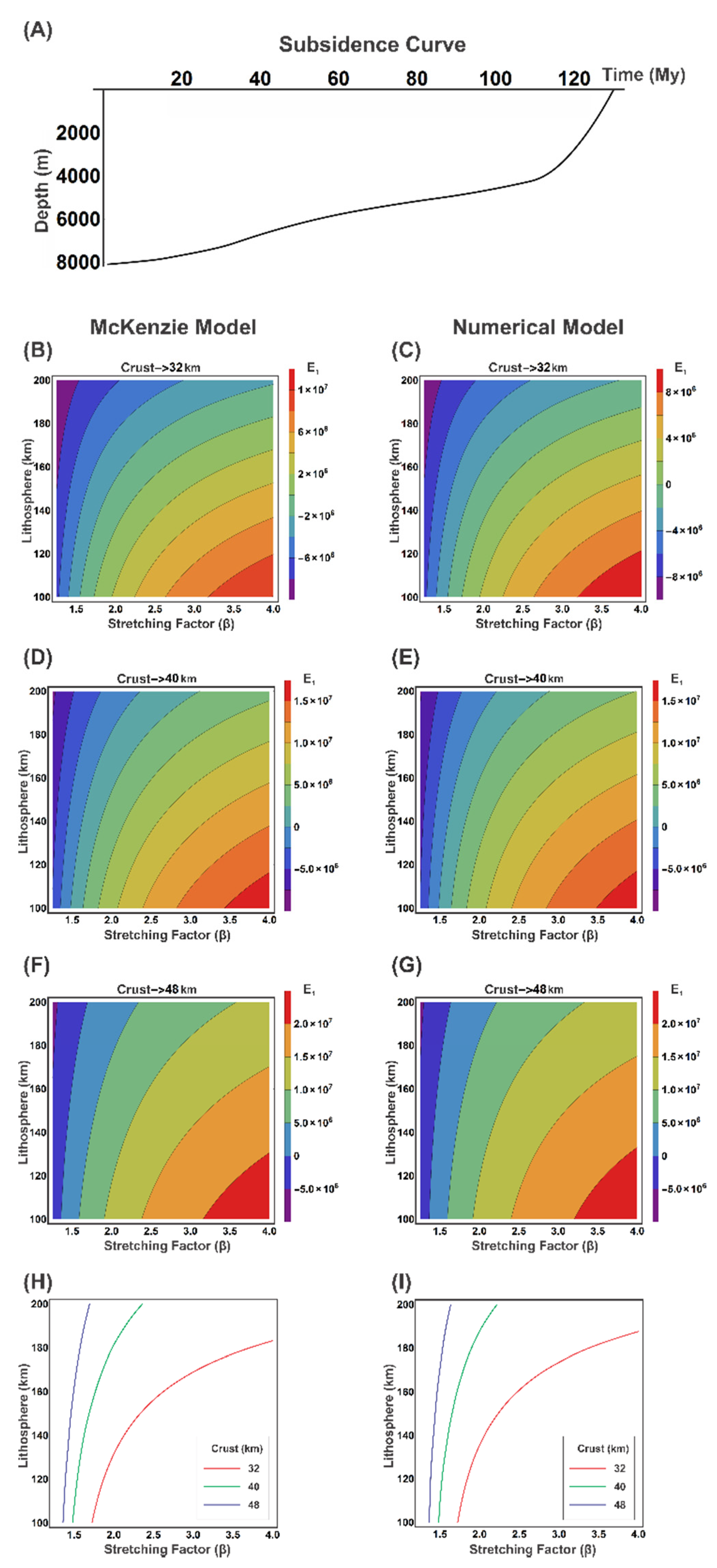
3. Curve Fitting Method
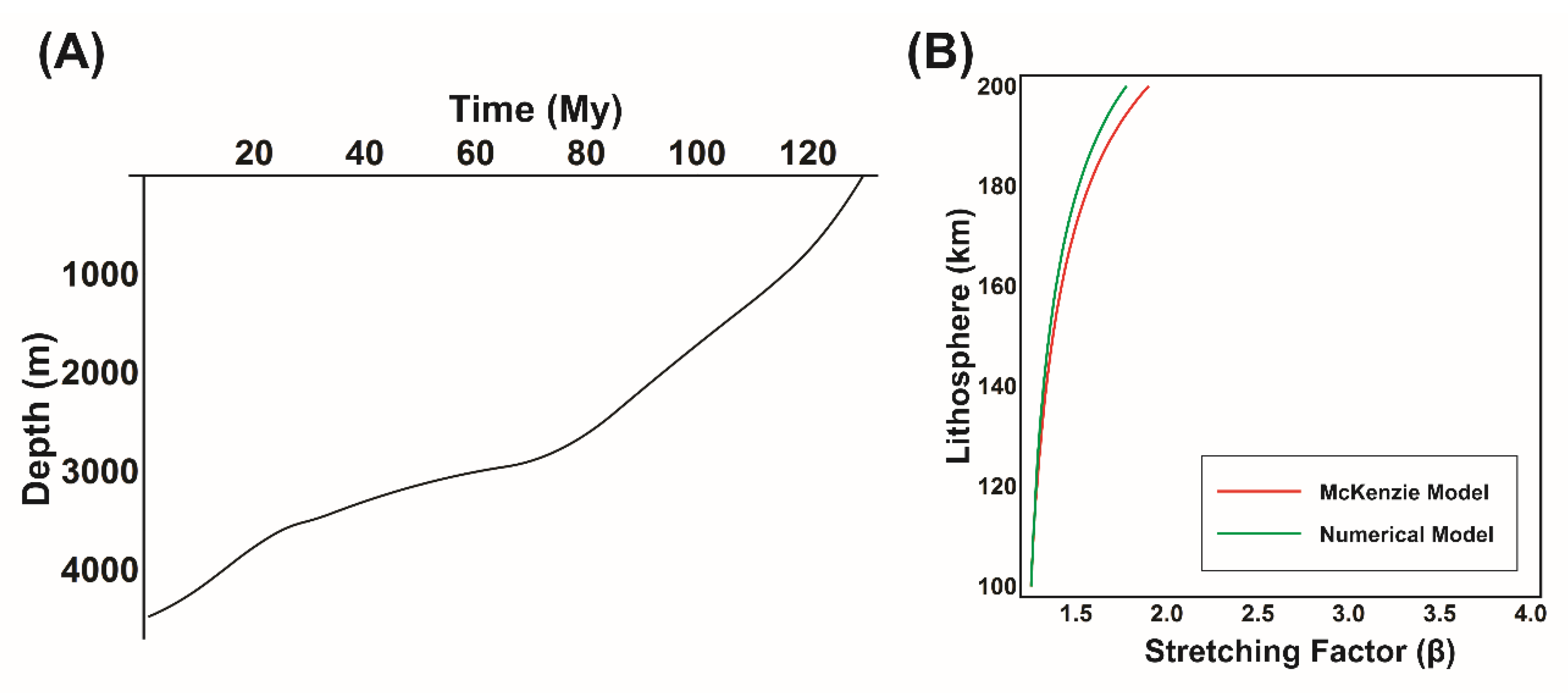
4. Case Study
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- McKenzie, D. Some remarks on the development of sedimentary basins. Earth Planet. Sci. Lett. 1978, 40, 25–32. [Google Scholar] [CrossRef]
- Wernicke, B. Low-angle normal faults in the Basin and Range Province: Nappe tectonics in an extending orogen. Nature 1981, 291, 645–648. [Google Scholar] [CrossRef]
- Wernicke, B. Uniform-sense normal simple shear of the continental lithosphere. Can. J. Earth Sci. 1985, 22, 108–125. [Google Scholar] [CrossRef]
- Kusznir, N.J.; Marsden, G.; Egan, S.S. A flexural-cantilever simple-shear/pure-shear model of continental lithosphere extension: Applications to the Jeanne d’Arc Basin, Grand Banks and Viking Graben, North Sea. Geol. Soc. Lond. Spec. Publ. 1991, 56, 41–60. [Google Scholar] [CrossRef]
- Rowley, D.B.; Sahagian, D. Depth-dependent stretching: A different approach. Geology 1986, 14, 32–35. [Google Scholar] [CrossRef]
- Royden, L.; Keen, C.E. Rifting process and thermal evolution of the continental margin of Eastern Canada determined from subsidence curves. Earth Planet. Sci. Lett. 1980, 51, 343–361. [Google Scholar] [CrossRef]
- Jarvis, G.T.; McKenzie, D.P. Sedimentary basin formation with finite extension rates. Earth Planet. Sci. Lett. 1980, 48, 42–52. [Google Scholar] [CrossRef]
- Lucazeau, F.; Le Douaran, S. The blanketing effect of sediments in basins formed by extension: A numerical model. Application to the Gulf of Lion and Viking graben. Earth Planet. Sci. Lett. 1985, 74, 92–102. [Google Scholar] [CrossRef]
- Wangen, M. The blanketing effect in sedimentary basins. Basin Res. 1995, 7, 283–298. [Google Scholar] [CrossRef]
- Hantschel, T.; Kauerauf, A.I. Fundamentals of Basin and Petroleum Systems Modeling; Springer Science & Business Media: Berlin, Germany, 2009. [Google Scholar]
- White, R.; McKenzie, D. Magmatism at rift zones: The generation of volcanic continental margins and flood basalts. J. Geophys. Res. Solid Earth 1989, 94, 7685–7729. [Google Scholar] [CrossRef]
- Stephenson, R.A.; Nakiboglu, S.M.; Kelly, M.A. Effects of asthenosphere melting, regional thermoisostasy, and sediment loading on the thermomechanical subsidence of extensional sedimentary basins. In Origin and Evolution of Sedimentary Basins and Their Energy and Mineral Resources; American Geophysical Union: Washington, DC, USA, 1989; pp. 17–27. [Google Scholar]
- Watts, A.B.; Karner, G.D.; Steckler, M.S. Lithospheric flexure and the evolution of sedimentary basins. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Sci. 1982, 305, 249–281. [Google Scholar] [CrossRef]
- Kooi, H.; Cloetingh, S.; Burrus, J. Lithospheric necking and regional isostasy at extensional basins 1. Subsidence and gravity modeling with an application to the Gulf of Lions Margin (SE France). J. Geophys. Res. Solid Earth 1992, 97, 17553–17571. [Google Scholar] [CrossRef]
- Van Balen, R.T.; van der Beek, P.A.; Cloetingh, S.A.P.L. The effect of rift shoulder erosion on stratal patterns at passive margins: Implications for sequence stratigraphy. Earth Planet. Sci. Lett. 1995, 134, 527–544. [Google Scholar] [CrossRef]
- Wangen, M. Physical Principles of Sedimentary Basin Analysis; Cambridge University Press: Cambridge, UK, 2010; p. 527. [Google Scholar]
- Burov, E.; Poliakov, A. Erosion and rheology controls on synrift and postrift evolution: Verifying old and new ideas using a fully coupled numerical model. J. Geophys. Res. Solid Earth 2001, 106, 16461–16481. [Google Scholar] [CrossRef]
- Hölzel, M.; Faber, R.; Wagreich, M. DeCompactionTool: Software for subsidence analysis including statistical error quantification. Comput. Geosci. 2008, 34, 1454–1460. [Google Scholar] [CrossRef]
- Steckler, M.S.; Watts, A.B. Subsidence of the Atlantic-type continental margin off New York. Earth Planet. Sci. Lett. 1978, 41, 1–13. [Google Scholar] [CrossRef]
- van Wees, J.D.; Stephenson, R.A.; Stovba, S.M.; Shymanovskyi, V.A. Tectonic variation in the Dniepr-Donets Basin from automated modelling of backstripped subsidence curves. Tectonophysics 1996, 268, 257–280. [Google Scholar] [CrossRef]
- Van Wees, J.D.; van Bergen, F.; David, P.; Nepveu, M.; Beekman, F.; Cloetingh, S.; Bonté, D. Probabilistic tectonic heat flow modeling for basin maturation: Assessment method and applications. Mar. Pet. Geol. 2009, 26, 536–551. [Google Scholar] [CrossRef]
- Beglinger, S.E.; van Wees, J.-D.; Cloetingh, S.; Doust, H. Tectonic subsidence history and source-rock maturation in the Campos Basin, Brazil. Pet. Geosci. 2012, 18, 153–172. [Google Scholar] [CrossRef]
- Chen, L.; Zhang, Z.; Song, H.; Li, F.; Franke, D. Numerical modeling of extensional sedimentary basin formation with MATLAB: Application to the northern margin of the South China Sea. Comput. Geosci. 2013, 51, 153–165. [Google Scholar] [CrossRef]
- Lee, E.Y.; Novotny, J.; Wagreich, M. BasinVis 1.0: A MATLAB®-based program for sedimentary basin subsidence analysis and visualization. Comput. Geosci. 2016, 91, 119–127. [Google Scholar] [CrossRef]
- Contreras, J.; Zühlke, R.; Bowman, S.; Bechstädt, T. Seismic stratigraphy and subsidence analysis of the southern Brazilian margin (Campos, Santos and Pelotas basins). Mar. Pet. Geol. 2010, 27, 1952–1980. [Google Scholar] [CrossRef]
- Holmes, M.H. Introduction to Numerical Methods in Differential Equations; Springer: New York, NY, USA, 2007; p. 239. [Google Scholar]
- Lebedev, S.; Adam, J.M.C.; Meier, T. Mapping the Moho with seismic surface waves: A review, resolution analysis, and recommended inversion strategies. Tectonophysics 2013, 609, 377–394. [Google Scholar] [CrossRef]
- Mohriak, W.U.; Hobbs, R.; Dewey, J.F. Basin-forming processes and the deep structure of the Campos Basin, offshore Brazil. Mar. Pet. Geol. 1990, 7, 94–122. [Google Scholar] [CrossRef]
- Allen, P.A.; Allen, J.R. Basin Analysis: Principles and Application to Petroleum Play Assessment, 3rd ed.; Wiley-Blackwell: Hoboken, NJ, USA, 2013; p. 619. [Google Scholar]
- Xie, X.; Heller, P. Plate tectonics and basin subsidence historyBasin subsidence. GSA Bull. 2009, 121, 55–64. [Google Scholar] [CrossRef]
- Guardado, L.R.; Gamboa, L.A.; Lucchesi, C.F. Petroleum geology of the Campos Basin, Brazil, a model for a producing atlantic type basin: Part 1. In Divergent/Passive Margin Basins; Edwards, J.D., Santogrossi, P.A., Eds.; American Association of Petroleum Geologists: Tulsa, OK, USA, 1989; pp. 3–80. [Google Scholar]
- Mohriak, W.U.; Mello, M.R.; Dewey, J.F.; Maxwell, J.R. Petroleum geology of the Campos Basin, offshore Brazil. Geol. Soc. Lond. Spec. Publ. 1990, 50, 119–141. [Google Scholar] [CrossRef]
- Karner, G.D.; Driscoll, N.W. Tectonic and stratigraphic development of the West African and eastern Brazilian Margins: Insights from quantitative basin modelling. Geol. Soc. Lond. Spec. Publ. 1999, 153, 11–40. [Google Scholar] [CrossRef]
- Cainelli, C.; Mohriak, W.U. General evolution of the eastern Brazilian continental margin. Lead. Edge 1999, 18, 800–861. [Google Scholar] [CrossRef]
- Mohriak, W.; Nemčok, M.; Enciso, G. South Atlantic divergent margin evolution: Rift-border uplift and salt tectonics in the basins of SE Brazil. Geol. Soc. Lond. Spec. Publ. 2008, 294, 365–398. [Google Scholar] [CrossRef]

© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tunwal, M.; Mulchrone, K.F.; Meere, P.A. Constraining Basin Parameters Using a Known Subsidence History. Geosciences 2020, 10, 263. https://doi.org/10.3390/geosciences10070263
Tunwal M, Mulchrone KF, Meere PA. Constraining Basin Parameters Using a Known Subsidence History. Geosciences. 2020; 10(7):263. https://doi.org/10.3390/geosciences10070263
Chicago/Turabian StyleTunwal, Mohit, Kieran F. Mulchrone, and Patrick A. Meere. 2020. "Constraining Basin Parameters Using a Known Subsidence History" Geosciences 10, no. 7: 263. https://doi.org/10.3390/geosciences10070263
APA StyleTunwal, M., Mulchrone, K. F., & Meere, P. A. (2020). Constraining Basin Parameters Using a Known Subsidence History. Geosciences, 10(7), 263. https://doi.org/10.3390/geosciences10070263




