Mixed Models in Nonlinear Regression for Description of the Growth of Nelore Cattle
Abstract
Simple Summary
Abstract
1. Introduction
2. Materials and Methods
2.1. Animal Registration
2.2. Body Weight
2.3. Quality of Adjustment Evaluators of Nonlinear Models
2.4. Statistical Analysis
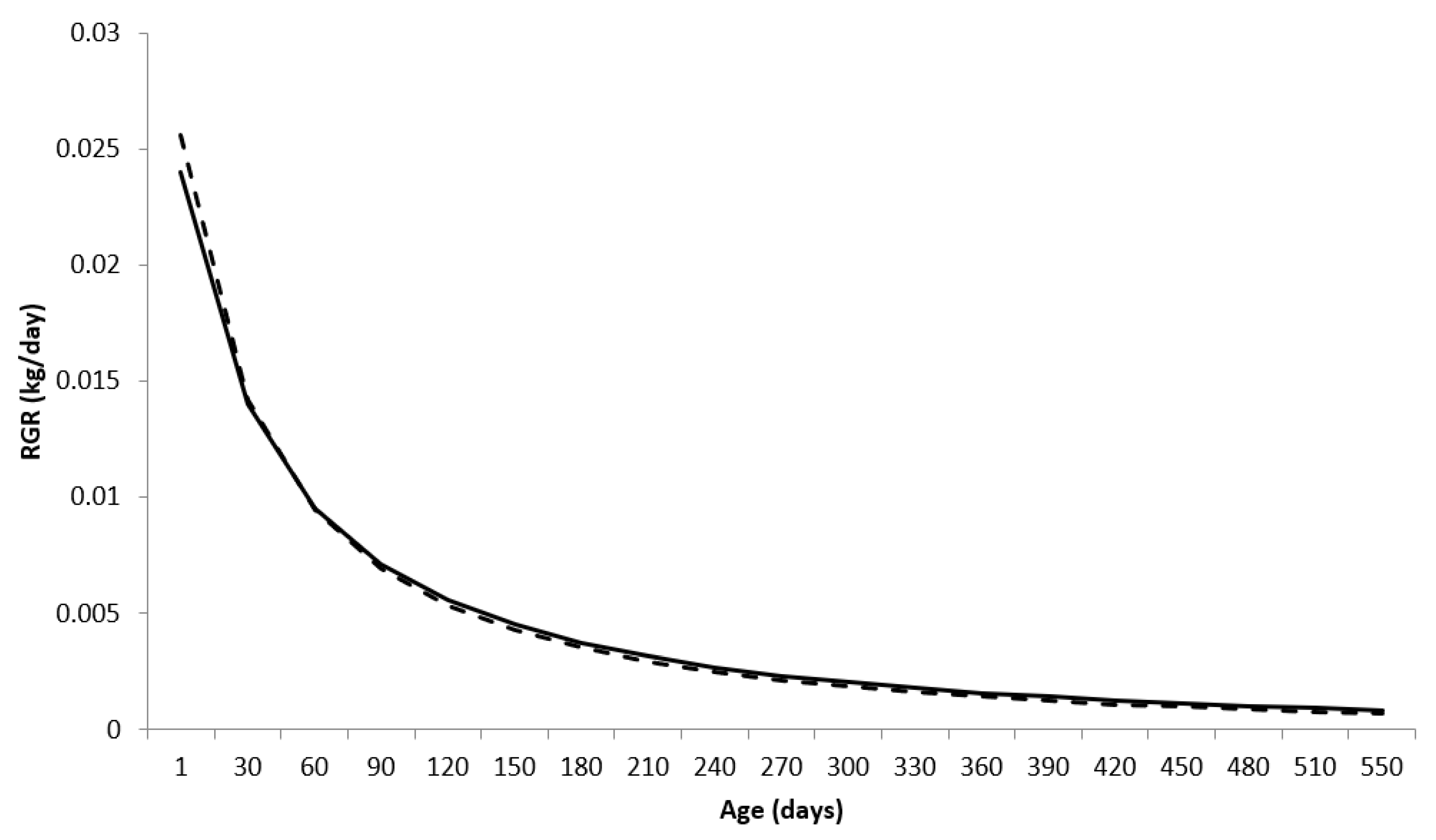
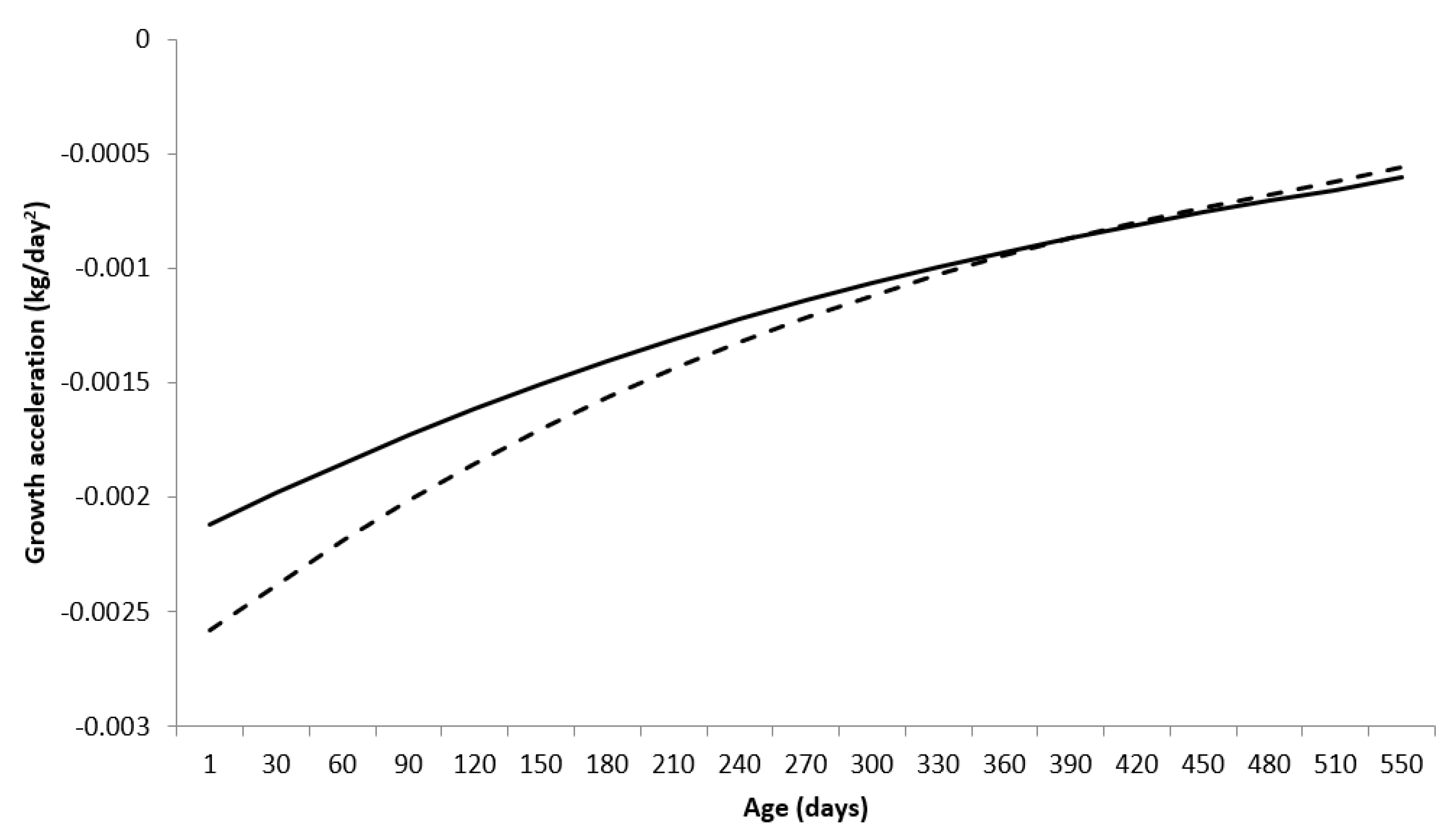
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gonçalves, T.D.M.; Dias, M.A.D.; Azevedo Junior, J.; Rodriguez, M.A.P.; Timpani, V.D.; Oliveira, A.I.G.D. Curvas de crescimento de fêmeas da raça Nelore e seus cruzamentos. Ciência Agrotecnologia 2011, 35, 582–590. [Google Scholar] [CrossRef]
- Hartati, H.; Putra, W.P.B. Predicting the growth curve of body weight in madura cattle. Kafkas Üniversitesi Veteriner Fakültesi Dergisi 2021, 27, 431–437. [Google Scholar]
- Alves, R.F.S.; Pereira, K.D.; Carneiro, A.P.S.; Emiliano, P.C.; Carneiro, P.L.S.; Malhado, C.H.M.; Martins Filho, R. Nonlinear mixed effects models for comparing growth curves for Guzerá cattle. Rev. Bras. Saúde Produção Anim. 2020, 21, e210132020. [Google Scholar] [CrossRef]
- Amaral, M.T.R. Abordagem Bayesiana Para Curva de Crescimento COM restrições Nos Parâmetros. Master’s Thesis, Universidade Federal Rural de Pernambuco, Recife, Brazil, 2008. [Google Scholar]
- Carvalho, L.R.D.; Pereira, G.M.S.; Silva, H.D.O.F.; Mischan, M.M.; Furtado, E.L. Ajustes de modelos não lineares de efeitos fixos, com ponderação e misto-aplicações. Rev. Bras. Biom. 2014, 1, 296–307. [Google Scholar]
- SAS Institute Inc. SAS/STAT User’s Guide; Version 9.2; SAS Institute Inc.: Cary, NC, USA, 2009. [Google Scholar]
- Bauman, D.E.; Currie, W.B. Partitioning of nutrients during pregnancy and lactation: A review of mechanisms involving homeostasis and homeorhesis. J. Dairy Sci. 1980, 63, 1514. [Google Scholar] [CrossRef] [PubMed]
- Faria, H.A. Nova Função Para Descrever o Crescimento Corporal de Fêmeas Nelore e Cruzadas. Master’s Thesis, Universidade Federal de Lavras, Lavras, MG, Brazil, 2008; p. 46. [Google Scholar]
- Boligon, A.A.; Albuquerque, L.G.; Mercadante, M.E.Z.; Lôbo, R.B. Associação genética entre a idade ao primeiro parto, ganhos em peso e peso adulto em animais da raça Nelore. In Reunião Anual da Sociedade Brasileira de Zootecnia; Sociedade Brasileira de Zootecnia: Brasilia, Brazil, 2009; p. 46. [Google Scholar]
- Lopes, F.B.; Silva, M.C.; Marques, E.G.; Ferreira, J.L. Ajuste de curvas de crescimento em bovinos Nelore da região Norte de Brasil. Rev. Bras. Saúde Produção Anim. 2011, 12, 607–617. [Google Scholar]
- McManus, C.; Evangelista, C.; Fernandes, L.A.C.; Miranda, R.M.D.; Moreno-Bernal, F.E.; Santos, N.R.D. Curvas de crescimento de ovinos Bergamácia criados no Distrito Federal. Rev. Bras. Zootec. 2003, 32, 1207–1212. [Google Scholar] [CrossRef][Green Version]
- Malhado, C.; Ramos, A.; Carneito, P.; Azevedo, D.; Mello, P.R.; Pereira, D. Martins Filho, R. Modelos não lineares utilizados para descrever o crescimento de bovinos da raça Nelore no Estado da Bahia: 1. Efeito ambiental. Rev. Bras. Saúde Produção Anim. 2009, 10, 1189. [Google Scholar]
- Souza, L.A.; Caires, D.N.; Carneiro, P.L.S.; Malhado, C.H.M.; Martins Filho, R. Curvas de crescimento em bovinos da raça Indubrasil criados no Estado do Sergipe. Rev. Ciências Agron. 2010, 41, 671–676. [Google Scholar] [CrossRef]
- Silva, N.D.; Lima, R.R.; Silva, F.F.; Muniz, J.A. Modelo hierárquico bayesiano aplicado na avaliação genética de curvas de crescimento de bovinos de corte. Arq. Bras. Med. Veterinária Zootec. 2010, 62, 409–418. [Google Scholar] [CrossRef][Green Version]
- Santoro, K.R.; Barbosa, S.B.P.; Brasil, L.H.D.A.; Santos, E.D.S. Estimativas de parâmetros de curvas de crescimento de bovinos Zebu, criados no Estado de Pernambuco. Rev. Bras. Zootec. 2005, 34, 2262–2279. [Google Scholar] [CrossRef]
- Giese, J. Modelos Mistos em Regressão Não Linear Para Descrição Do Crescimento de Bovinos da Raça Nelore. Master’s Dissertation, Universidade Federal do Mato Grosso, Cuiabá, Brazil, 2015; p. 55. [Google Scholar]
- Arango, J.A.; Van Vleck, L.D. Size of beef cows: Early ideas, new developments. Genet. Mol. Res. 2002, 50, 51–63. [Google Scholar] [CrossRef]
- Santana, T.J.S. Novos Modelos de Curvas de Crescimento Para Bovinos de Corte. Doctoral Thesis, Universidade Federal de Lavras, Lavras, Brazil, 2013; p. 70. [Google Scholar]
- Mazzini, A.R.D.A.; Muniz, J.A.; Silva, F.F.; Aquino, L.H.D. Curva de crescimento de novilhos Hereford: Heterocedasticidade e resíduos autorregressivos. Ciência Rural 2005, 35, 422–427. [Google Scholar] [CrossRef]
- Moreira, K.F.; Regadas Filho, J.G.L.; Oliveira, T.S.; Brito, L.F.; Soares, V.R.C.; Rodrigues, M.T. Modelos não linear misto no ajuste de curvas de crescimento de caprinos leiteiros. In Reunião Anual da Sociedade Brasileira de Zootecnia; Sociedade Brasileira de Zootecnia: Brasilia, Brazil, 2012; p. 49. [Google Scholar]


| Model | Equation |
|---|---|
| Brody | Yt = A (1 − Be−kt) + Ɛ |
| Gompertz | Yt = + Ɛ |
| Logistic | Yt = A (1 + Be−kt)−1 + Ɛ |
| Richards | Yt = A (1 − Be−kt)m + Ɛ |
| Von Bertalanffy | Yt = A (1 − Be−kt)3 + Ɛ |
| Von Bertalanffy modified | Yt = A (1 − Be−kt)2 + Ɛ |
| Meloun 1 | Yt = A − Be−kt+ Ɛ |
| Gender | Age | Average (Kg) | SD (Kg) | CV (%) |
|---|---|---|---|---|
| Male | WB | 32.72 | 3.19 | 9.76 |
| W120 | 131.52 | 16.75 | 12.74 | |
| W210 | 191.79 | 22.11 | 11.53 | |
| W365 | 241.08 | 27.07 | 11.23 | |
| W450 | 279.57 | 32.24 | 11.53 | |
| W550 | 332.63 | 41.15 | 12.37 | |
| Female | WB | 31.70 | 3.26 | 10.28 |
| W120 | 127.53 | 15.35 | 12.03 | |
| W210 | 184.48 | 20.85 | 11.30 | |
| W365 | 227.13 | 27.99 | 12.32 | |
| W450 | 257.84 | 29.39 | 11.40 | |
| W550 | 301.76 | 32.63 | 10.81 |
| Model | Gender | A | B | K |
|---|---|---|---|---|
| Brody | Male | 479.45 | 0.9216 | 0.0019 |
| Gompertz | 357.51 | 2.0002 | 0.0051 | |
| Logistic | 334.00 | 4.6810 | 0.0078 | |
| Meloun 1 | 479.45 | 441.08 | 0.0019 | |
| Von Bertalanffy | 376.06 | 0.5090 | 0.0040 | |
| Von Bertalanffy modified | 390.03 | 0.6695 | 0.0035 | |
| Brody | Female | 377.08 | 0.9067 | 0.0025 |
| Gompertz | 306.93 | 1.9472 | 0.0059 | |
| Logistic | 289.00 | 4.5291 | 0.0091 | |
| Meloun 1 | 377.10 | 341.90 | 0.0025 | |
| Von Bertalanffy | 319.30 | 0.4969 | 0.0048 | |
| Von Bertalanffy modified | 328.08 | 0.6548 | 0.0042 |
| Functions | Gender | EAS | ASD | ADR | R²aj | AI | −2log(L) | AIC | BIC |
|---|---|---|---|---|---|---|---|---|---|
| Male | |||||||||
| Brody | 808.11 | 28.43 | 21.37 | 0.92 | 48.87 | 165.178 | 165.186 | 165.217 | |
| Gompertz | 930.15 | 30.50 | 24.28 | 0.91 | 53.87 | 167.615 | 167.623 | 167.654 | |
| Logistic | 1066.04 | 32.65 | 26.93 | 0.90 | 58.67 | 169.977 | 169.985 | 170.017 | |
| Meloun 1 | 808.11 | 28.43 | 21.37 | 0.92 | 48.87 | 165.178 | 165.186 | 165.217 | |
| V. B. 1 | 884.94 | 29.75 | 23.26 | 0.92 | 52.09 | 166.751 | 166.759 | 166.790 | |
| V. B. M. 2 | 863.51 | 29.39 | 22.74 | 0.92 | 51.21 | 166.326 | 166.334 | 166.365 | |
| Female | |||||||||
| Brody | 644.05 | 25.38 | 19.06 | 0.92 | 43.51 | 186.929 | 186.937 | 186.969 | |
| Gompertz | 740.39 | 27.21 | 21.41 | 0.91 | 47.71 | 189.729 | 189.737 | 189.769 | |
| Logistic | 855.74 | 29.25 | 23.76 | 0.90 | 52.12 | 192.638 | 192.646 | 192.677 | |
| Meloun 1 | 644.05 | 25.38 | 19.06 | 0.92 | 43.51 | 186.929 | 186.937 | 186.969 | |
| V. B. 1 | 703.73 | 26.53 | 20.53 | 0.92 | 46.14 | 188.709 | 188.717 | 188.749 | |
| V.B. M. 2 | 686.71 | 26.21 | 20.10 | 0.92 | 45.39 | 188.217 | 188.225 | 188.257 |
| Male | Female | |||||||
|---|---|---|---|---|---|---|---|---|
| Mixed | Fix | Mixed | Fix | |||||
| Parameter | Value | SE | Value | SE | Value | SE | Value | SE |
| A | 434.40 | 0.0033 | 479.45 | 0.0001 | 362.13 | 1.2951 | 377.08 | 2.1670 |
| B | 0.9242 | 0.0003 | 0.9216 | 0.0009 | 0.9123 | 0.0004 | 0.9067 | 0.0010 |
| K | 0.0023 | 0.0001 | 0.0019 | 0.0001 | 0.0028 | 0.0002 | 0.0025 | 0.0003 |
| 5.8662 | 28.4300 | 4.2804 | 25.3763 | |||||
| 27.8534 | 28.3107 | |||||||
| 0.0003 | 0.0003 | |||||||
| 0.0045 | 0.0043 | |||||||
| −2 Log(L) | 155.530 | 165.178 | 175.488 | 186.929 | ||||
| AIC | 155.544 | 165.186 | 175.502 | 186.937 | ||||
| BIC | 155.598 | 165.217 | 175.558 | 186.969 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Júnior, R.N.C.C.; Araújo, C.V.d.; Silva, W.C.d.; Araújo, S.I.d.; Lôbo, R.B.; Nakabashi, L.R.M.; Castro, L.M.d.; Menezes, F.L.; Maciel e Silva, A.G.; Silva, L.K.X.; et al. Mixed Models in Nonlinear Regression for Description of the Growth of Nelore Cattle. Animals 2023, 13, 101. https://doi.org/10.3390/ani13010101
Júnior RNCC, Araújo CVd, Silva WCd, Araújo SId, Lôbo RB, Nakabashi LRM, Castro LMd, Menezes FL, Maciel e Silva AG, Silva LKX, et al. Mixed Models in Nonlinear Regression for Description of the Growth of Nelore Cattle. Animals. 2023; 13(1):101. https://doi.org/10.3390/ani13010101
Chicago/Turabian StyleJúnior, Raimundo Nonato Colares Camargo, Cláudio Vieira de Araújo, Welligton Conceição da Silva, Simone Inoe de Araújo, Raysildo Barbosa Lôbo, Lílian Roberta Matimoto Nakabashi, Letícia Mendes de Castro, Flávio Luiz Menezes, André Guimarães Maciel e Silva, Lílian Kátia Ximenes Silva, and et al. 2023. "Mixed Models in Nonlinear Regression for Description of the Growth of Nelore Cattle" Animals 13, no. 1: 101. https://doi.org/10.3390/ani13010101
APA StyleJúnior, R. N. C. C., Araújo, C. V. d., Silva, W. C. d., Araújo, S. I. d., Lôbo, R. B., Nakabashi, L. R. M., Castro, L. M. d., Menezes, F. L., Maciel e Silva, A. G., Silva, L. K. X., Silva, J. A. R. d., Barbosa, A. V. C., Marques, J. R. F., & Lourenço Júnior, J. d. B. (2023). Mixed Models in Nonlinear Regression for Description of the Growth of Nelore Cattle. Animals, 13(1), 101. https://doi.org/10.3390/ani13010101






