Measurements versus Numerical Simulations for Slotted Synthetic Jet Actuator
Abstract
1. Introduction
2. Experimental Characterization
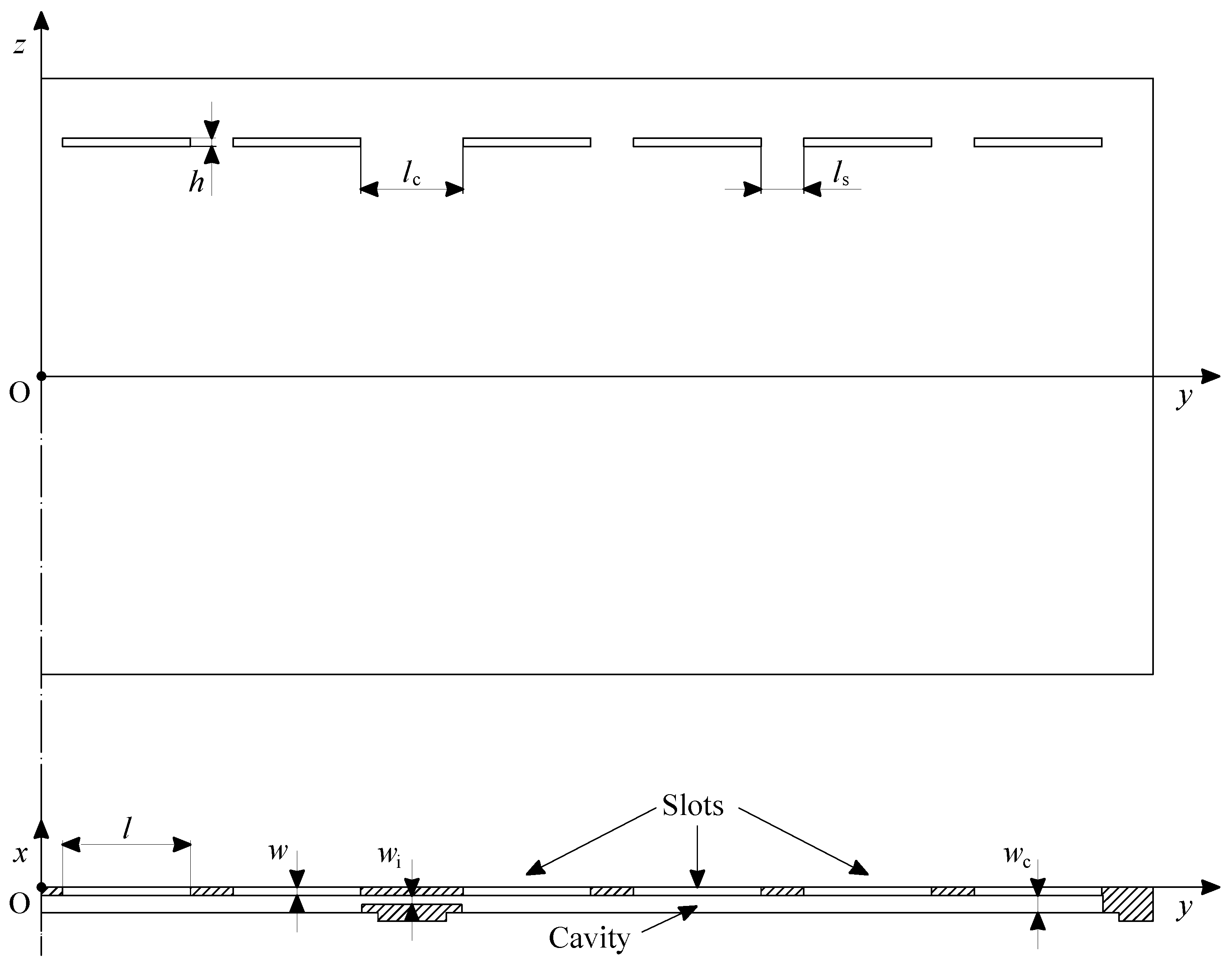
2.1. The SJ Device
2.2. Experimental Methodology
2.3. Non-Dimensional Parameters
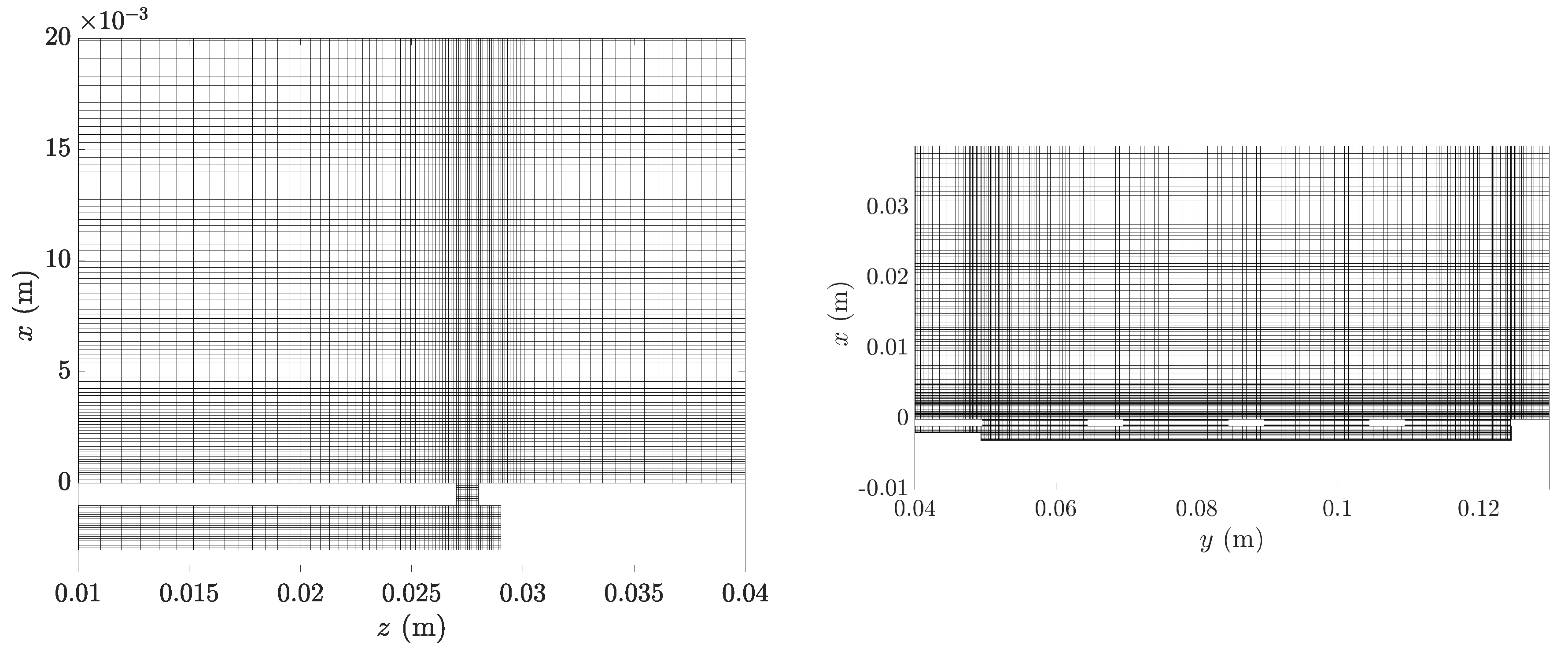
3. Computational Setup
4. Results
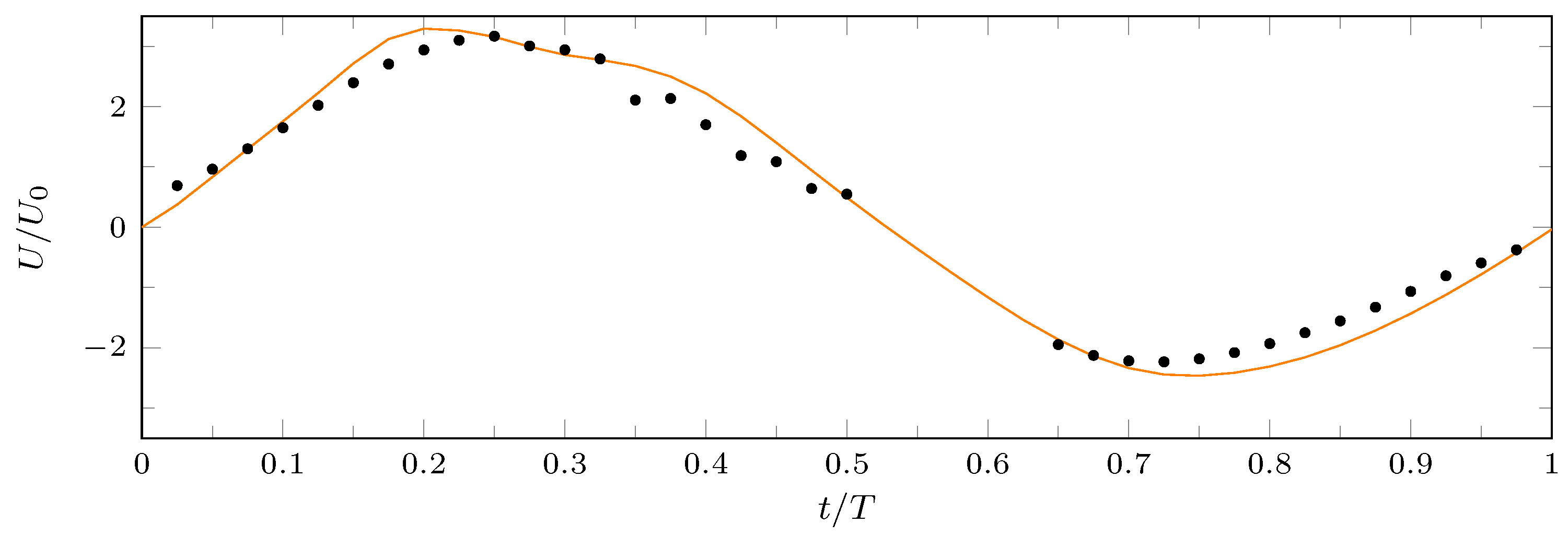
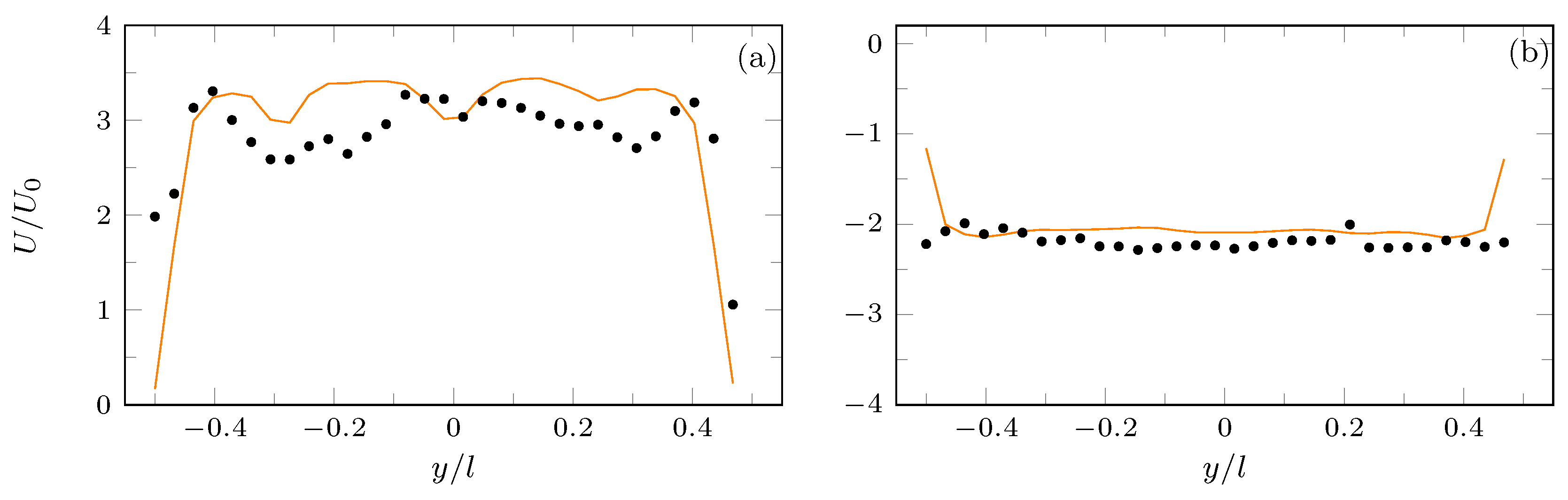
4.1. Validation
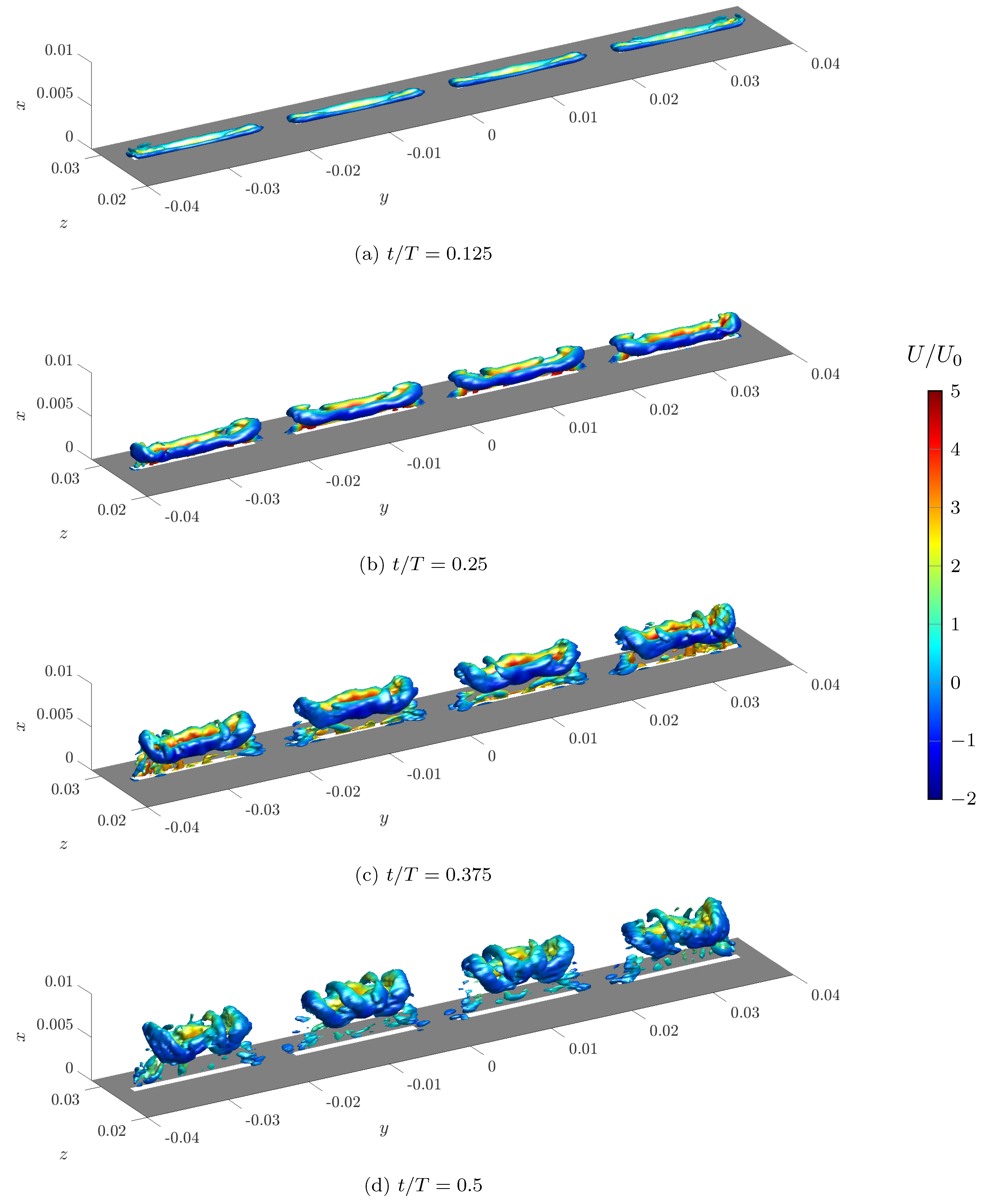
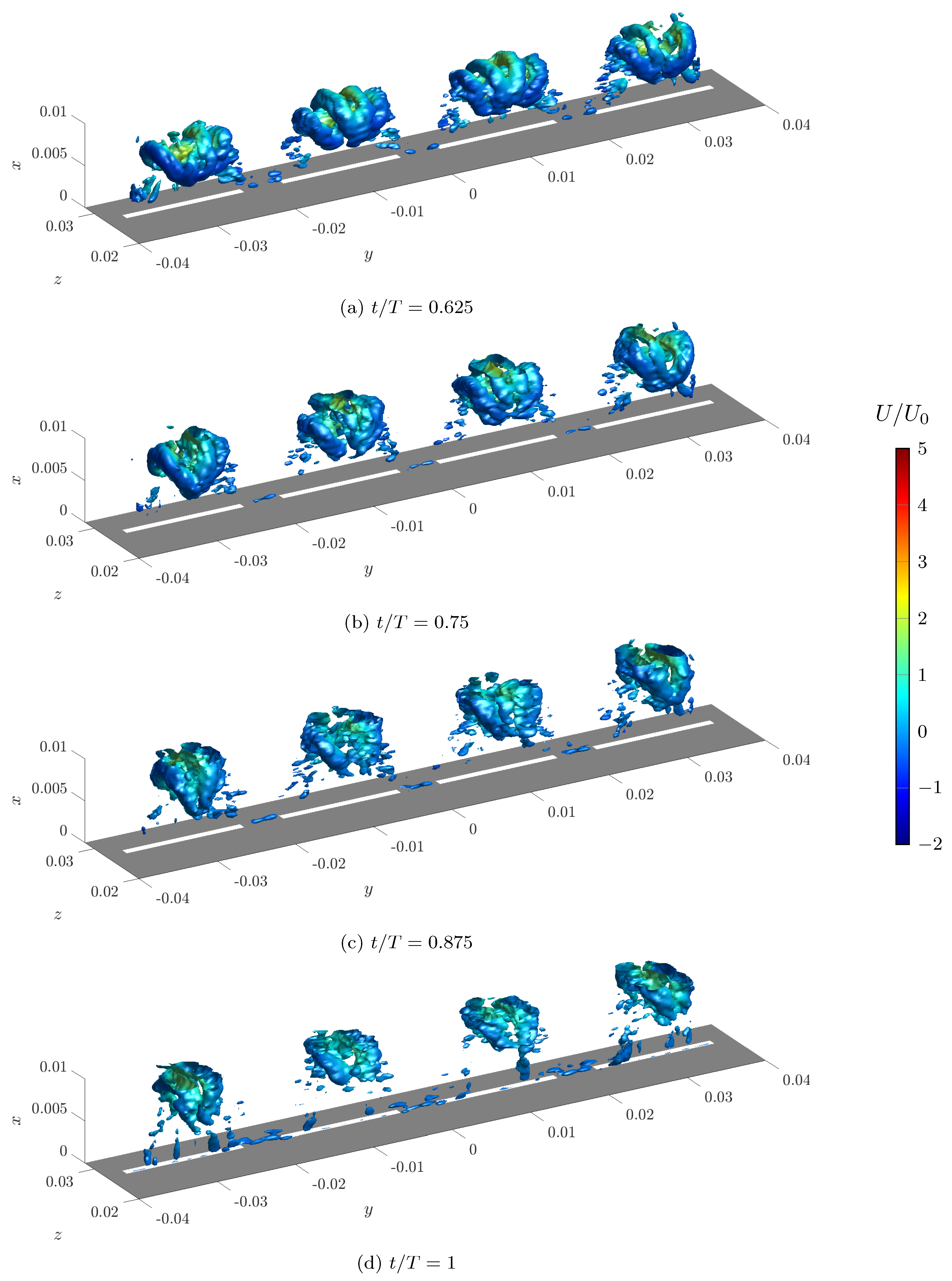
4.2. Further Insights on the External Field
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Glezer, A.; Amitay, M. Synthetic jets. Annu. Rev. Fluid Mech. 2002, 34, 503–529. [Google Scholar] [CrossRef]
- Mohseni, K.; Mittal, R. Synthetic Jets: Fundamentals and Applications; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Cattafesta, L.N., III; Sheplak, M. Actuators for active flow control. Annu. Rev. Fluid Mech. 2011, 43, 247–272. [Google Scholar] [CrossRef]
- Smith, B.; Swift, G. A comparison between synthetic jets and continuous jets. Exp. Fluids 2003, 34, 467–472. [Google Scholar] [CrossRef]
- He, X.; Lustbader, J.A.; Arik, M.; Sharma, R. Heat transfer characteristics of impinging steady and synthetic jets over vertical flat surface. Int. J. Heat Mass Transf. 2015, 80, 825–834. [Google Scholar] [CrossRef]
- Smith, B.L.; Glezer, A. Jet vectoring using synthetic jets. J. Fluid Mech. 2002, 458, 1–34. [Google Scholar] [CrossRef]
- Tamburello, D.; Amitay, M. Active manipulation of a particle-laden jet. Int. J. Multiph. Flow 2008, 34, 829–851. [Google Scholar] [CrossRef]
- Chiatto, M.; Marchitto, L.; Valentino, G.; de Luca, L. Influence of piezo-driven synthetic jet on water spray behavior. At. Sprays 2017, 27, 691–706. [Google Scholar] [CrossRef]
- Dandois, J.; Garnier, E.; Sagaut, P. Numerical simulation of active separation control by a synthetic jet. J. Fluid Mech. 2007, 574, 25–58. [Google Scholar] [CrossRef]
- Amitay, M.; Smith, D.R.; Kibens, V.; Parekh, D.E.; Glezer, A. Aerodynamic flow control over an unconventional airfoil using synthetic jet actuators. AIAA J. 2001, 39, 361–370. [Google Scholar] [CrossRef]
- You, D.; Moin, P. Study of Flow Separation over an Airfoil With Synthetic Jet Control Using Large-Eddy Simulation; Technical Report; Annual Research Brief, Center for Turbulence Research: Stanford, CA, USA, 2007; pp. 311–321. [Google Scholar]
- Krishnan, G.; Mohseni, K. An experimental and analytical investigation of rectangular synthetic jets. J. Fluids Eng. 2009, 131, 121101. [Google Scholar] [CrossRef]
- Van Buren, T.; Amitay, M. Comparison between finite-span steady and synthetic jets issued into a quiescent fluid. Exp. Therm. Fluid Sci. 2016, 75, 16–24. [Google Scholar] [CrossRef]
- Girfoglio, M.; Greco, C.S.; Chiatto, M.; de Luca, L. Modelling of efficiency of synthetic jet actuators. Sens. Actuators A Phys. 2015, 233, 512–521. [Google Scholar] [CrossRef]
- McDonald, P.; Persoons, T. Numerical Characterisation of Active Drag and Lift Control for a Circular Cylinder in Cross-Flow. Appl. Sci. 2017, 7, 1166. [Google Scholar] [CrossRef]
- Crowther, W.J.; Gomes, L.T. An evaluation of the mass and power scaling of synthetic jet actuator flow control technology for civil transport aircraft applications. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2008, 222, 357–372. [Google Scholar] [CrossRef]
- Mohamed, A.M.; Crowther, W.; Nabawy, M. Development of Valveless Resonant Micropumps for Liquid Applications. In Proceedings of the 2018 AIAA Aerospace Sciences Meeting, Kissimmee, FL, USA, 8–12 January 2018; p. 0580. [Google Scholar]
- Smith, B.L.; Glezer, A. The formation and evolution of synthetic jets. Phys. Fluids 1998, 10, 2281–2297. [Google Scholar] [CrossRef]
- Yao, C.; Chen, F.J.; Neuhart, D. Synthetic jet flowfield database for computational fluid dynamics validation. AIAA J. 2006, 44, 3153–3157. [Google Scholar] [CrossRef]
- Van Buren, T.; Whalen, E.; Amitay, M. Vortex formation of a finite-span synthetic jet: effect of rectangular orifice geometry. J. Fluid Mech. 2014, 745, 180–207. [Google Scholar] [CrossRef]
- Xia, X.; Mohseni, K. Transitional region of a round synthetic jet. Phys. Rev. Fluids 2018, 3, 011901. [Google Scholar] [CrossRef]
- Wang, L.; Feng, L.H.; Wang, J.J.; Li, T. Parameter influence on the evolution of low-aspect-ratio rectangular synthetic jets. J. Vis. 2018, 21, 105–115. [Google Scholar] [CrossRef]
- Kral, L.D.; Donovan, J.F.; Cain, A.B.; Cary, A.W. Numerical simulation of synthetic jet actuators. In Proceedings of the 4th Shear Flow Control Conference, Shear Flow Conference, Snowmass Village, CO, USA, 29 June–2 July 1997; p. 1824. [Google Scholar]
- Rizzetta, D.P.; Visbal, M.R.; Stanek, M.J. Numerical Investigation of Synthetic-Jet Flow Fields. AIAA J. 1999, 37, 919–927. [Google Scholar] [CrossRef]
- Kotapati, R.B.; Mittal, R.; Cattafesta, L.N., III. Numerical study of a transitional synthetic jet in quiescent external flow. J. Fluid Mech. 2007, 581, 287–321. [Google Scholar] [CrossRef]
- Gallas, Q.; Holman, R.; Nishida, T.; Carroll, B.; Sheplak, M.; Cattafesta, L. Lumped element modeling of piezoelectric-driven synthetic jet actuators. AIAA J. 2003, 41, 240–247. [Google Scholar] [CrossRef]
- Chiatto, M.; Capuano, F.; Coppola, G.; de Luca, L. LEM Characterization of Synthetic Jet Actuators Driven by Piezoelectric Element: A Review. Sensors 2017, 17, 1216. [Google Scholar] [CrossRef] [PubMed]
- Feero, M.A.; Lavoie, P.; Sullivan, P.E. Influence of cavity shape on synthetic jet performance. Sens. Actuators A Phys. 2015, 223, 1–10. [Google Scholar] [CrossRef]
- Kordík, J.; Trávníček, Z. Optimal diameter of nozzles of synthetic jet actuators based on electrodynamic transducers. Exp. Therm. Fluid Sci. 2017, 86, 281–294. [Google Scholar] [CrossRef]
- Chiatto, M.; Capuano, F.; de Luca, L. Numerical and experimental characterization of a double-orifice synthetic jet actuator. Meccanica 2018, 53, 2883–2896. [Google Scholar] [CrossRef]
- Sahni, O.; Wood, J.; Jansen, K.; Amitay, M. Three-dimensional interactions between a finite-span synthetic jet and a crossflow. J. Fluid Mech. 2011, 671, 254–287. [Google Scholar] [CrossRef]
- Jabbal, M.; Liddle, S.; Potts, J.; Crowther, W. Development of design methodology for synthetic jet actuator array for flow separation control applications. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2013, 227, 110–124. [Google Scholar] [CrossRef]
- McGlynn, E.; Tran, S.; Sahni, O. Large Eddy Simulation of Flow Interactions of Segmented Synthetic Jets on an Airfoil. In Proceedings of the 47th AIAA Fluid Dynamics Conference, Denver, CO, USA, 5–9 June 2017; p. 3310. [Google Scholar]
- Invigorito, M.; Ceglia, G. SyntHetic Jet AcTuators for Flow Control (SHAFT), WP2 Progress Meeting; Technical Report; CIRA: Capua, Italy, 2016. [Google Scholar]
- Yavuzkurt, S. A guide to uncertainty analysis of hot-wire data. J. Fluids Eng. Trans. ASME 1984, 106, 181–186. [Google Scholar] [CrossRef]
- OpenFOAM and The OpenFOAM Foundation. Available online: http://www.openfoam.org (accessed on 27 July 2018).
- Palumbo, A.; Capuano, F.; de Luca, L. Performances of two open-source solvers in the numerical simulation of synthetic jets. In Proceedings of the 7th European Conference on Computational Fluid Dynamics (ECCM-ECFD 2018), Glasgow, UK, 11–15 June 2018. [Google Scholar]
- de Luca, L.; Girfoglio, M.; Chiatto, M.; Coppola, G. Scaling properties of resonant cavities driven by piezo-electric actuators. Sens. Actuators A Phys. 2016, 247, 465–474. [Google Scholar] [CrossRef]
- MARCONI, the New Tier-0 System. Available online: http://www.hpc.cineca.it/hardware/marconi (accessed on 27 July 2018).
- Hunt, J.C.; Wray, A.A.; Moin, P. Eddies, Streams, and Convergence Zones in Turbulent Flows; Technical Report CTR-S88; Center for Turbulence Research: Stanford, CA, USA, 1988. [Google Scholar]
- Zong, H.; Kotsonis, M. Formation, evolution and scaling of plasma synthetic jets. J. Fluid Mech. 2018, 837, 147–181. [Google Scholar] [CrossRef]


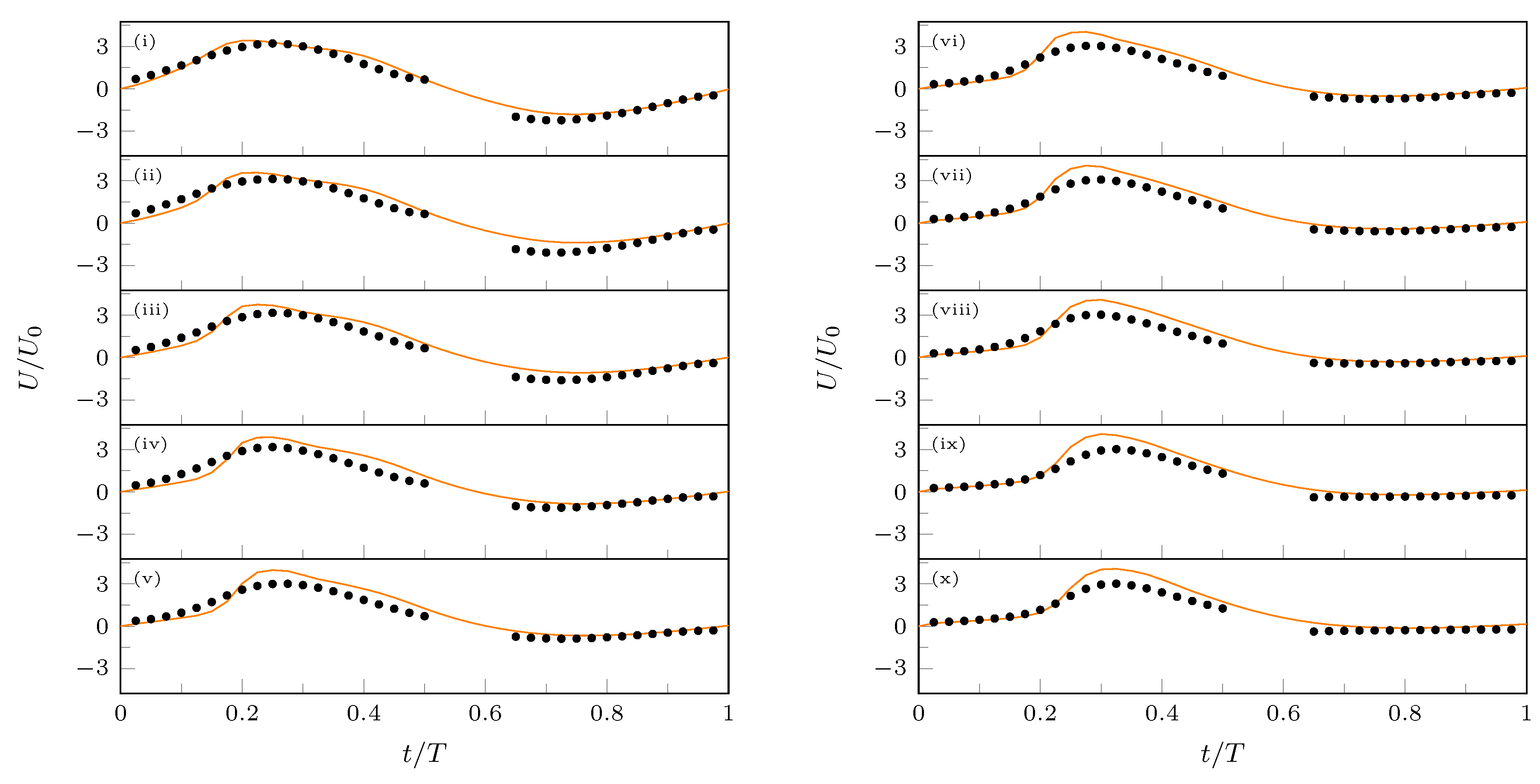

| Parameter | Experimental | Numerical |
|---|---|---|
| Actuation frequency, f (Hz) | 250 | 250 |
| Slot width, h (mm) | 1 | 1 |
| Slot spanwise length, l (mm) | 15 | 15 |
| Average jet velocity, (m/s) | 2.85 | 2.95 |
| Maximum exit velocity, (m/s) | 9.1 | 9.5 |
| Reynolds number, | 190 | 197 |
| Strouhal number, | 0.0877 | 0.0842 |
| Stokes number, S | 10.23 | 10.23 |
| Dimensionless stroke length, | 11.41 | 11.86 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Palumbo, A.; Chiatto, M.; De Luca, L. Measurements versus Numerical Simulations for Slotted Synthetic Jet Actuator. Actuators 2018, 7, 59. https://doi.org/10.3390/act7030059
Palumbo A, Chiatto M, De Luca L. Measurements versus Numerical Simulations for Slotted Synthetic Jet Actuator. Actuators. 2018; 7(3):59. https://doi.org/10.3390/act7030059
Chicago/Turabian StylePalumbo, Andrea, Matteo Chiatto, and Luigi De Luca. 2018. "Measurements versus Numerical Simulations for Slotted Synthetic Jet Actuator" Actuators 7, no. 3: 59. https://doi.org/10.3390/act7030059
APA StylePalumbo, A., Chiatto, M., & De Luca, L. (2018). Measurements versus Numerical Simulations for Slotted Synthetic Jet Actuator. Actuators, 7(3), 59. https://doi.org/10.3390/act7030059






