Scalloping and Stress Concentration in DRIE-Manufactured Comb-Drives
Abstract
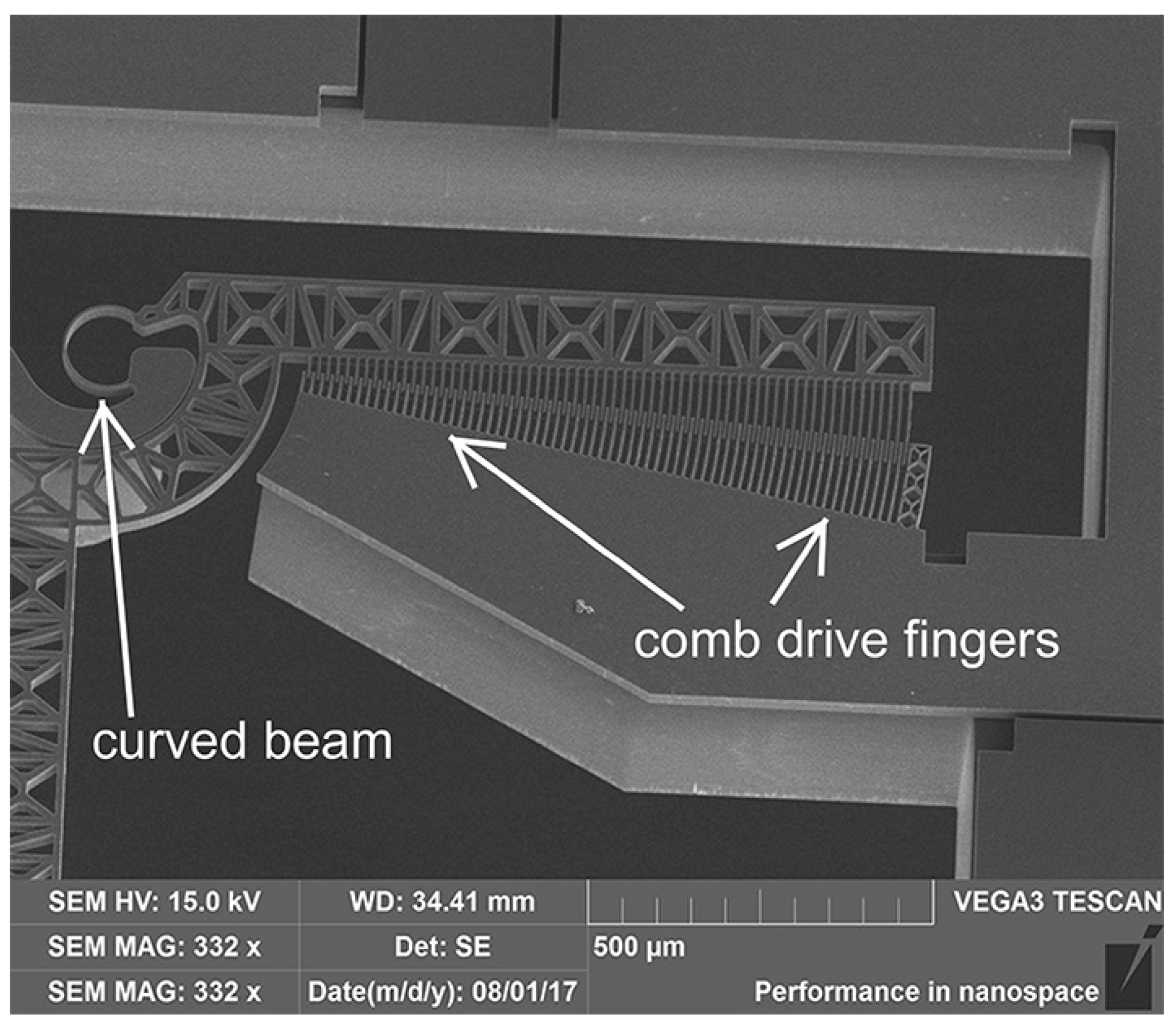
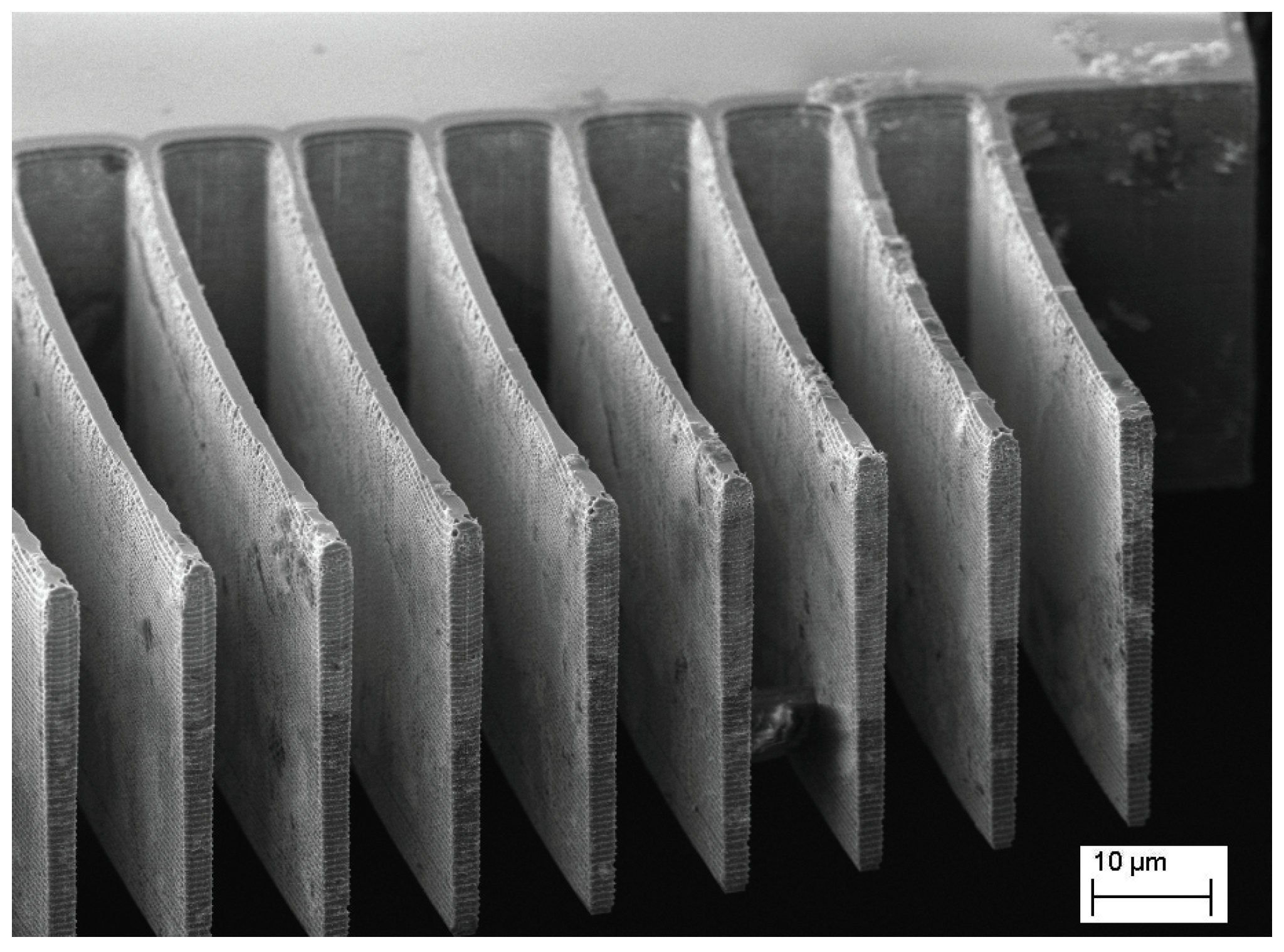
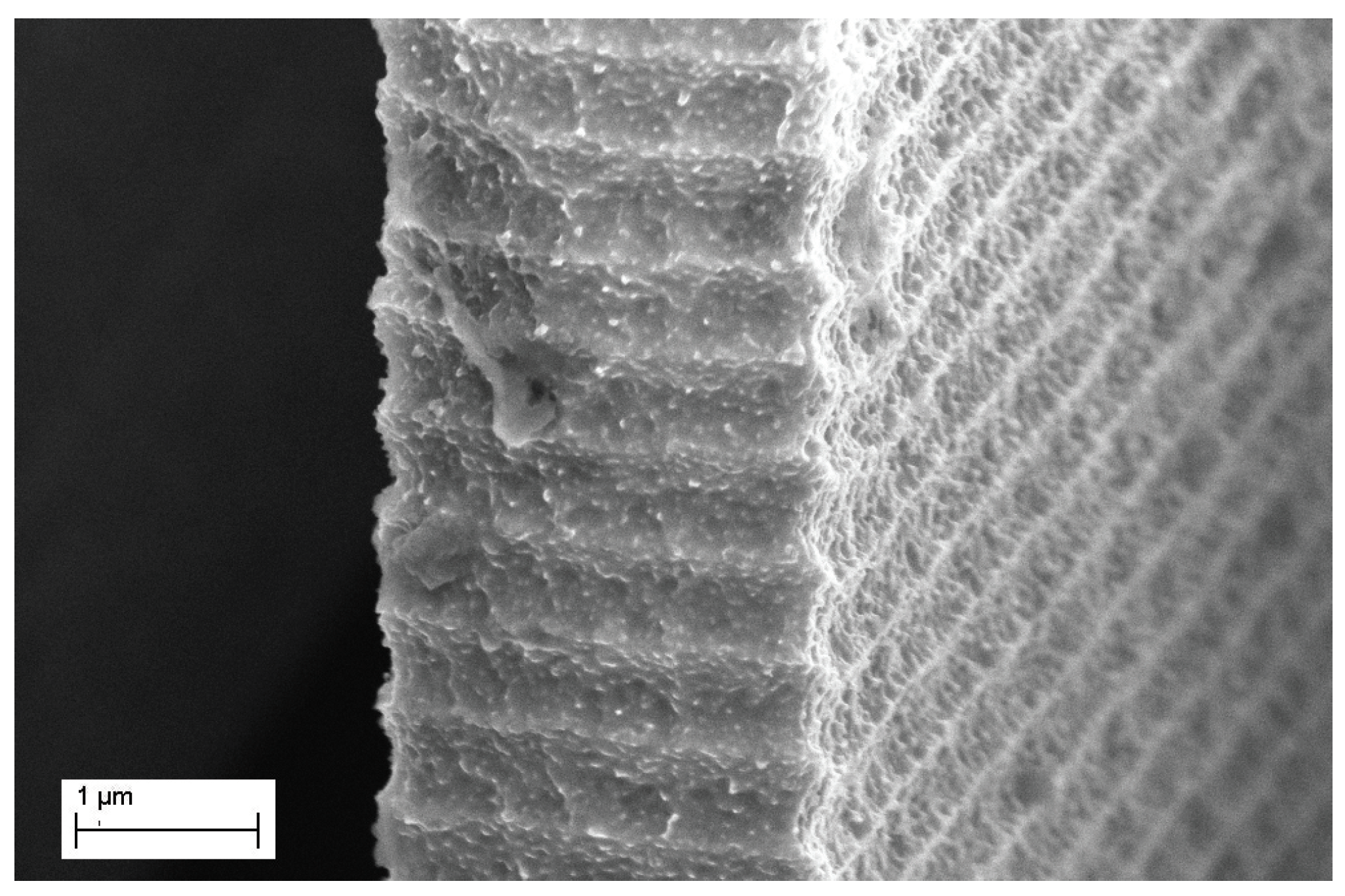
1. Introduction
2. Motivation of This Work
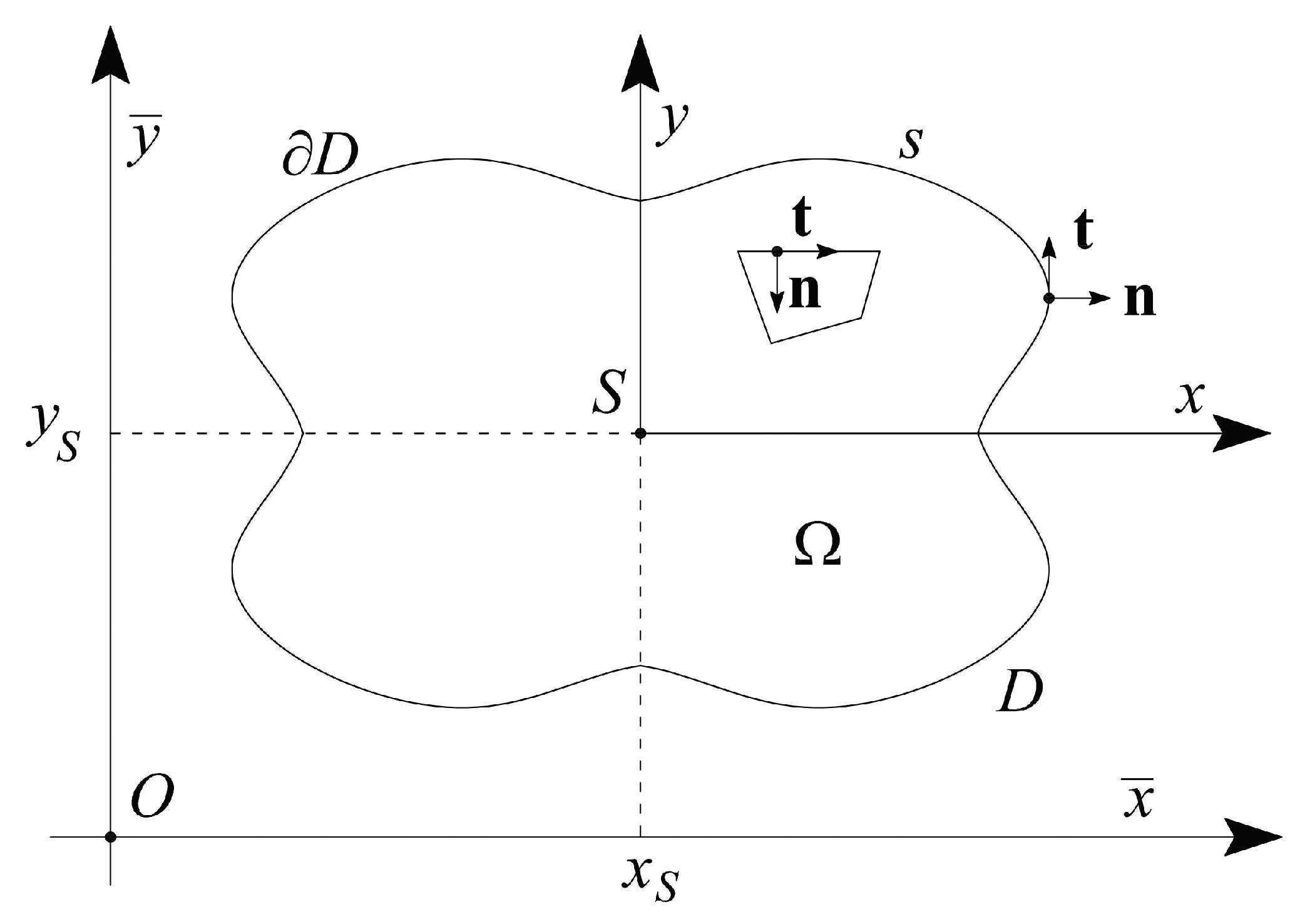
3. Theoretical Background
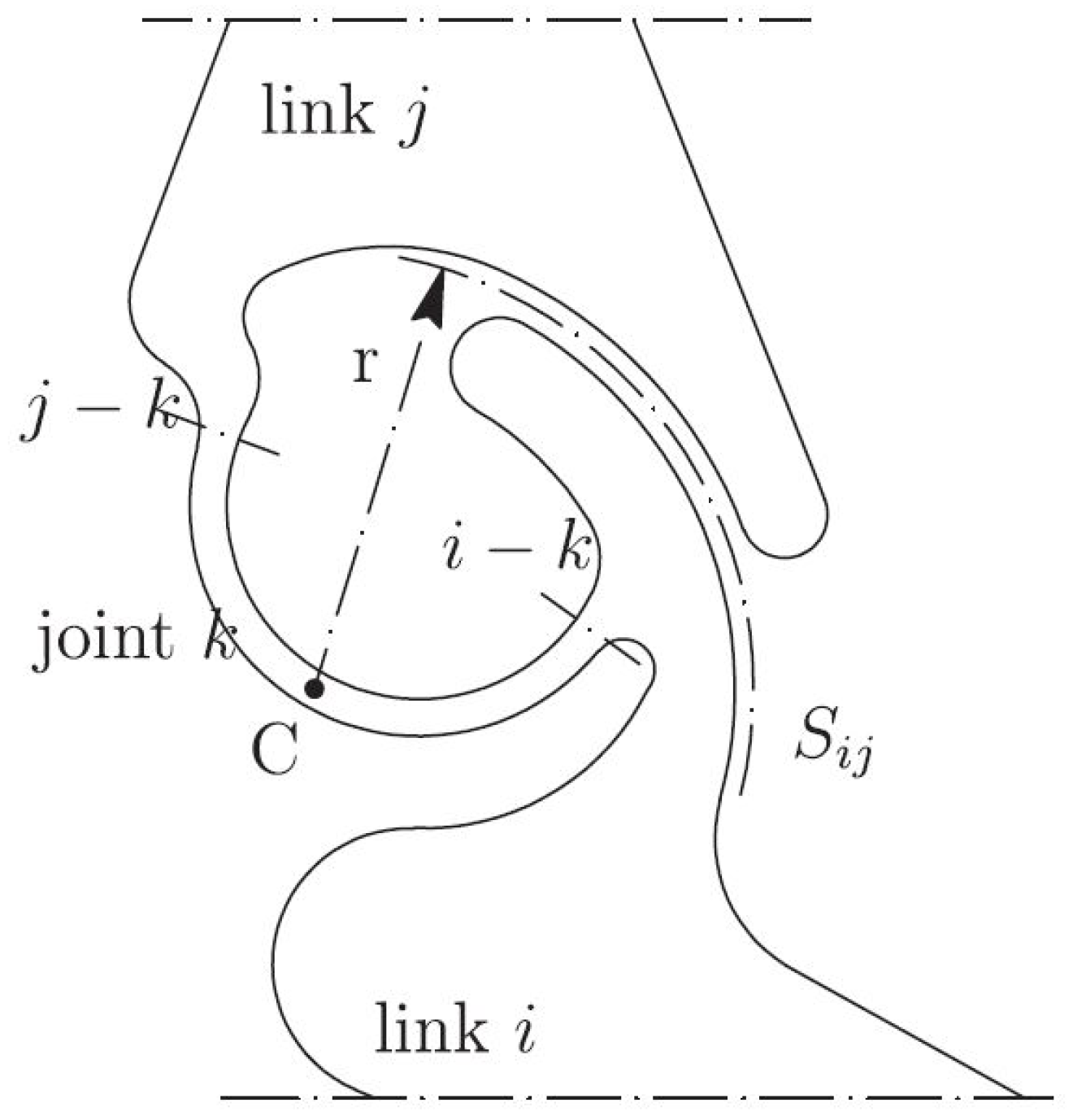
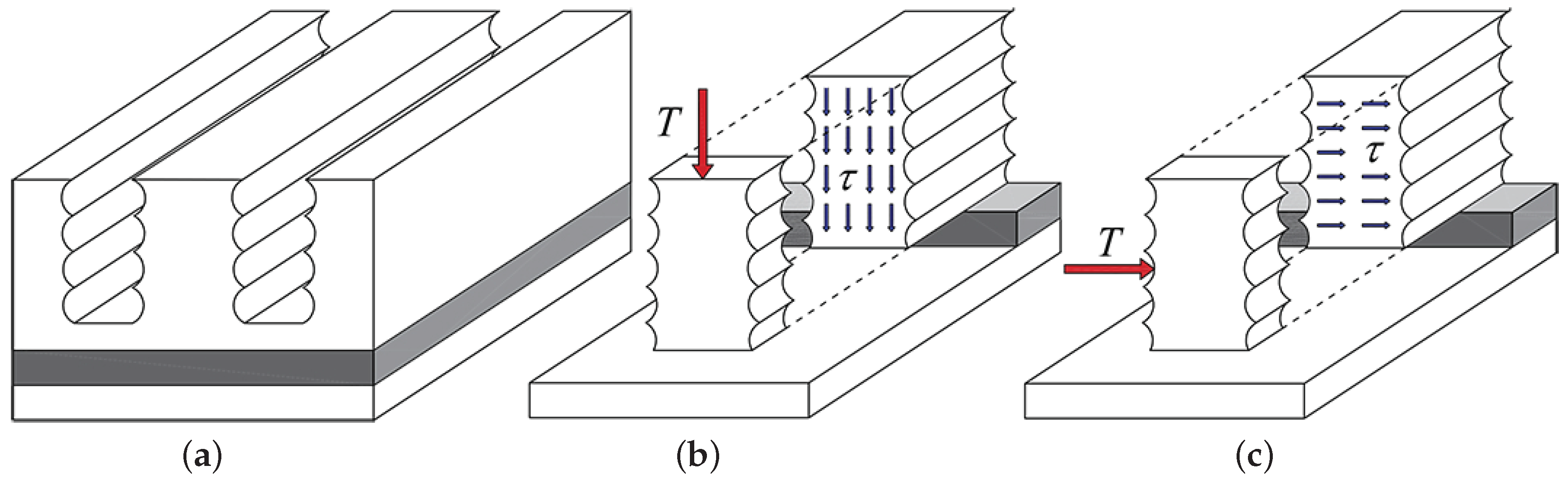
4. Shear Stresses in Torsion
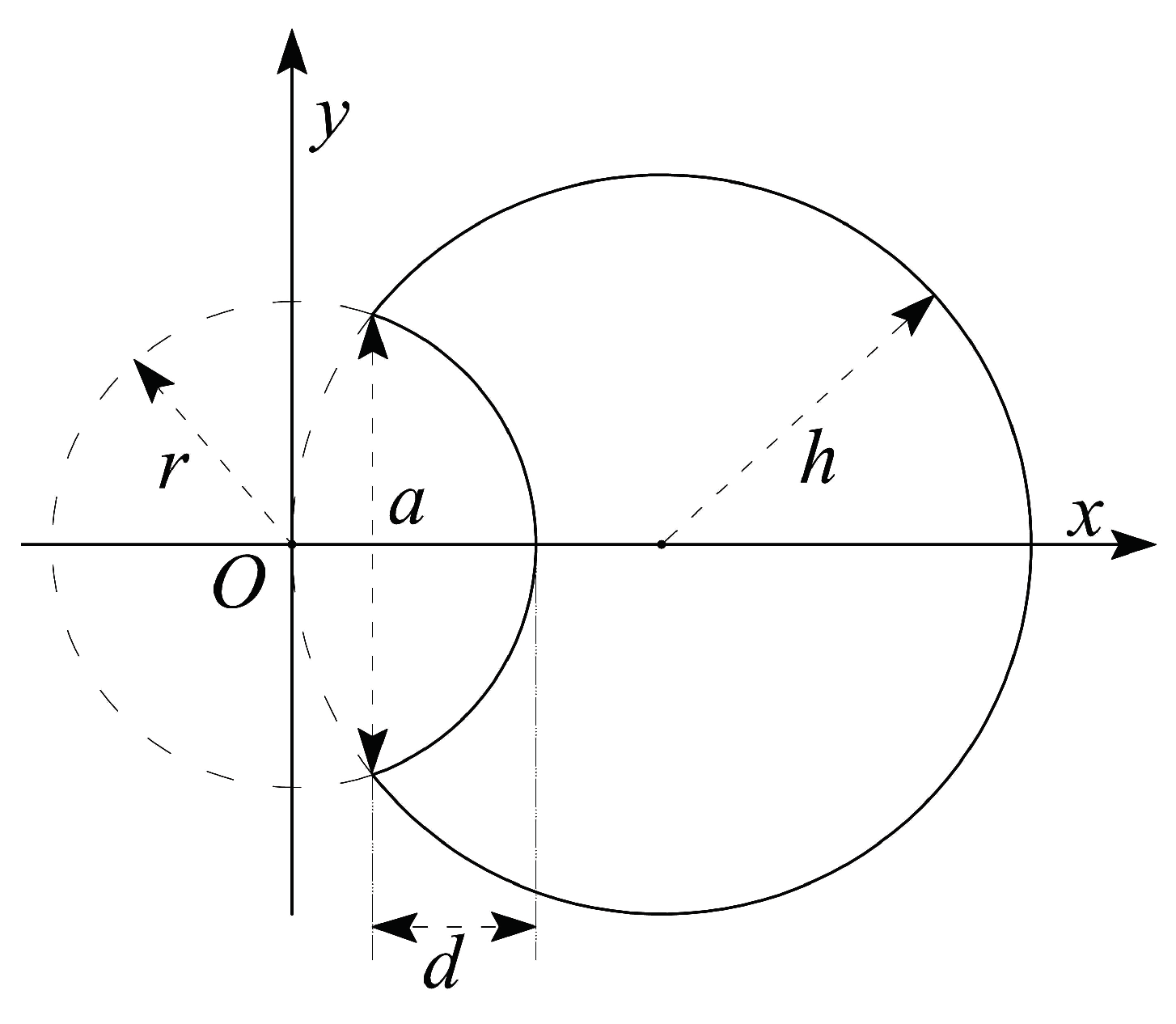
5. Circular Notched Cross-Section
6. Theoretical Aspects in the Finite Element Formulation
7. Stress Analysis
7.1. Analytical Solution
- beam radius, m
- notch radius, m
- GPa
- torsion relative angle rad
- beam length m
- twist of the centroidal fiber
7.2. Numerical Solution via Finite Difference Discretization
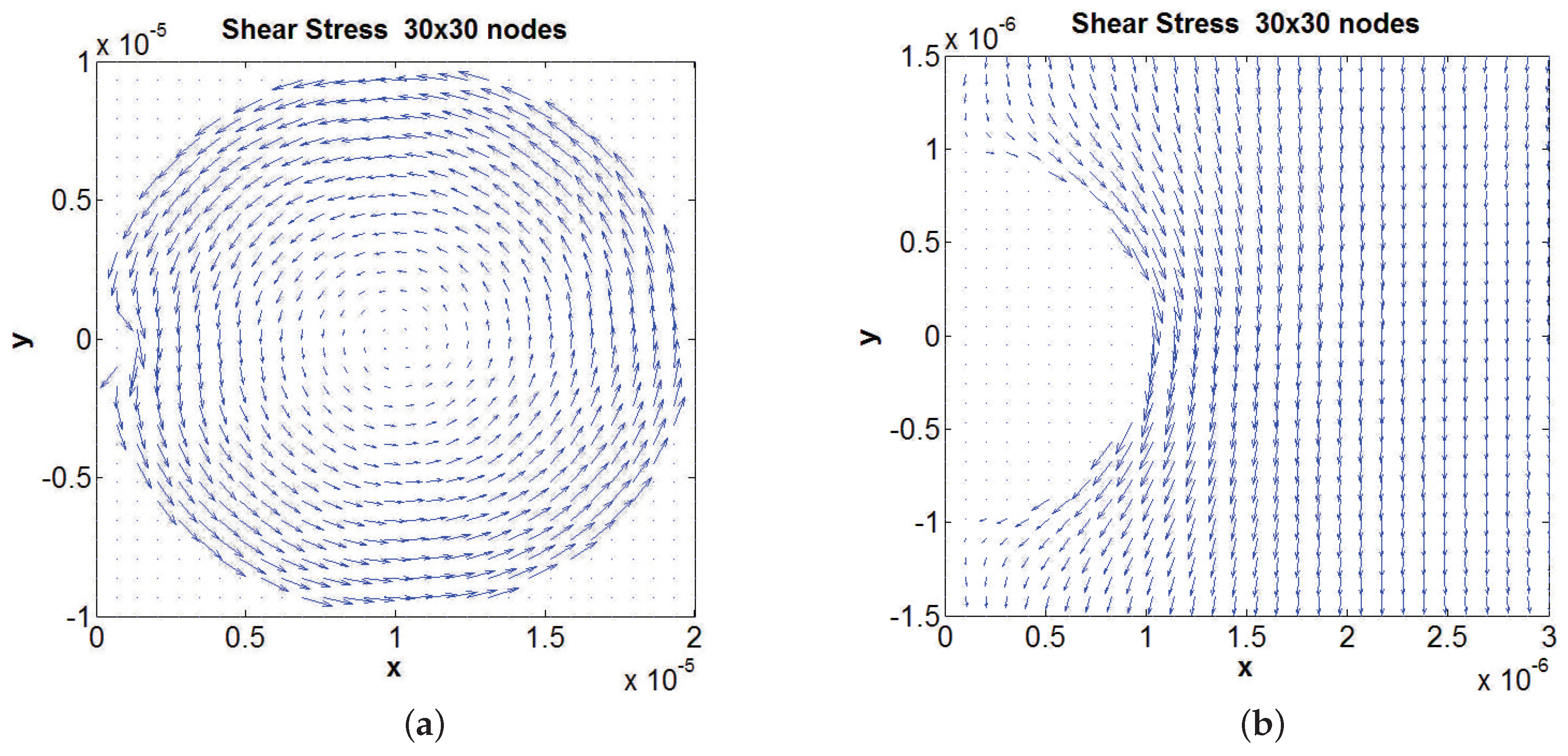
7.3. Numerical Solution via Finite Element Analysis
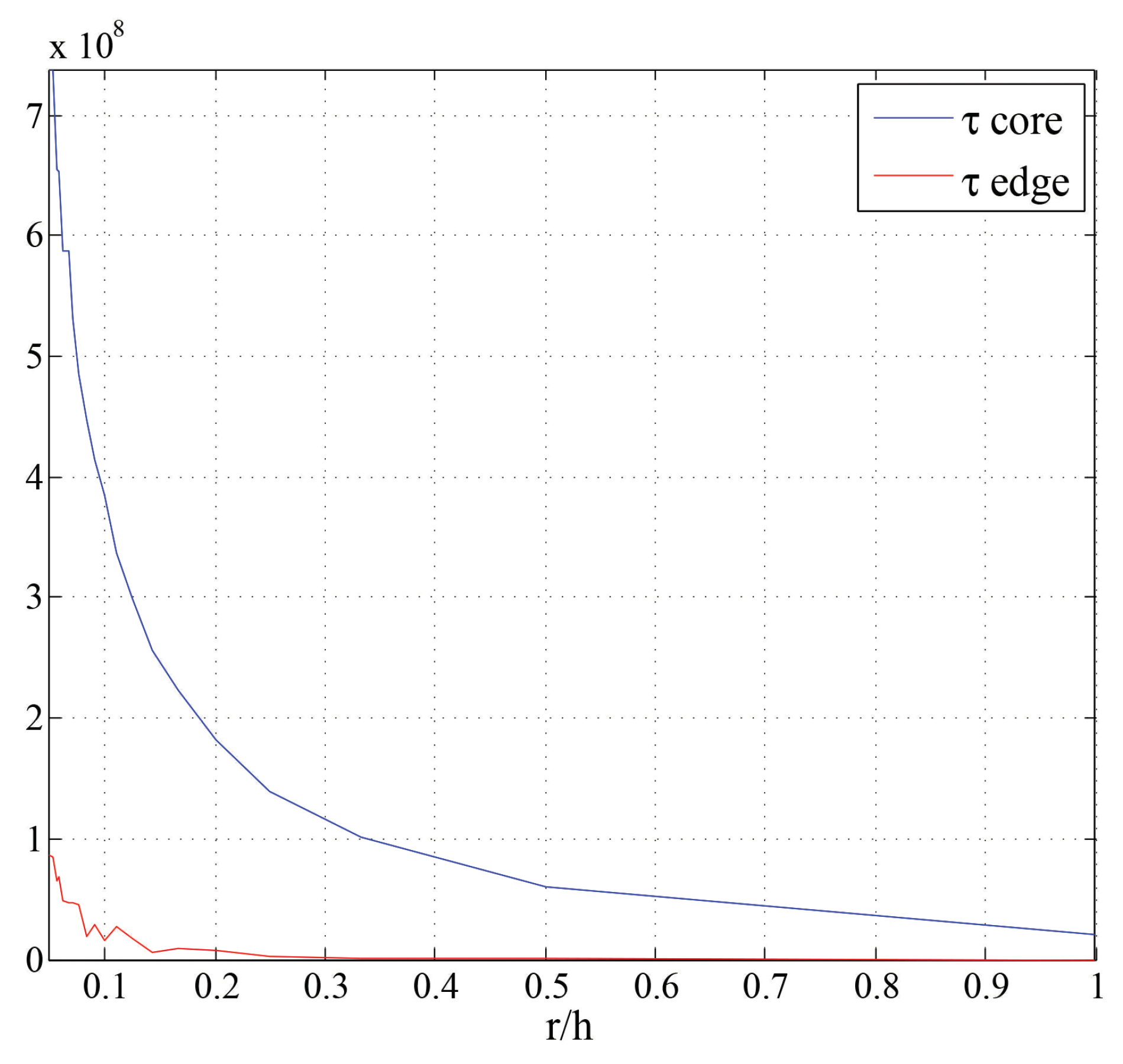
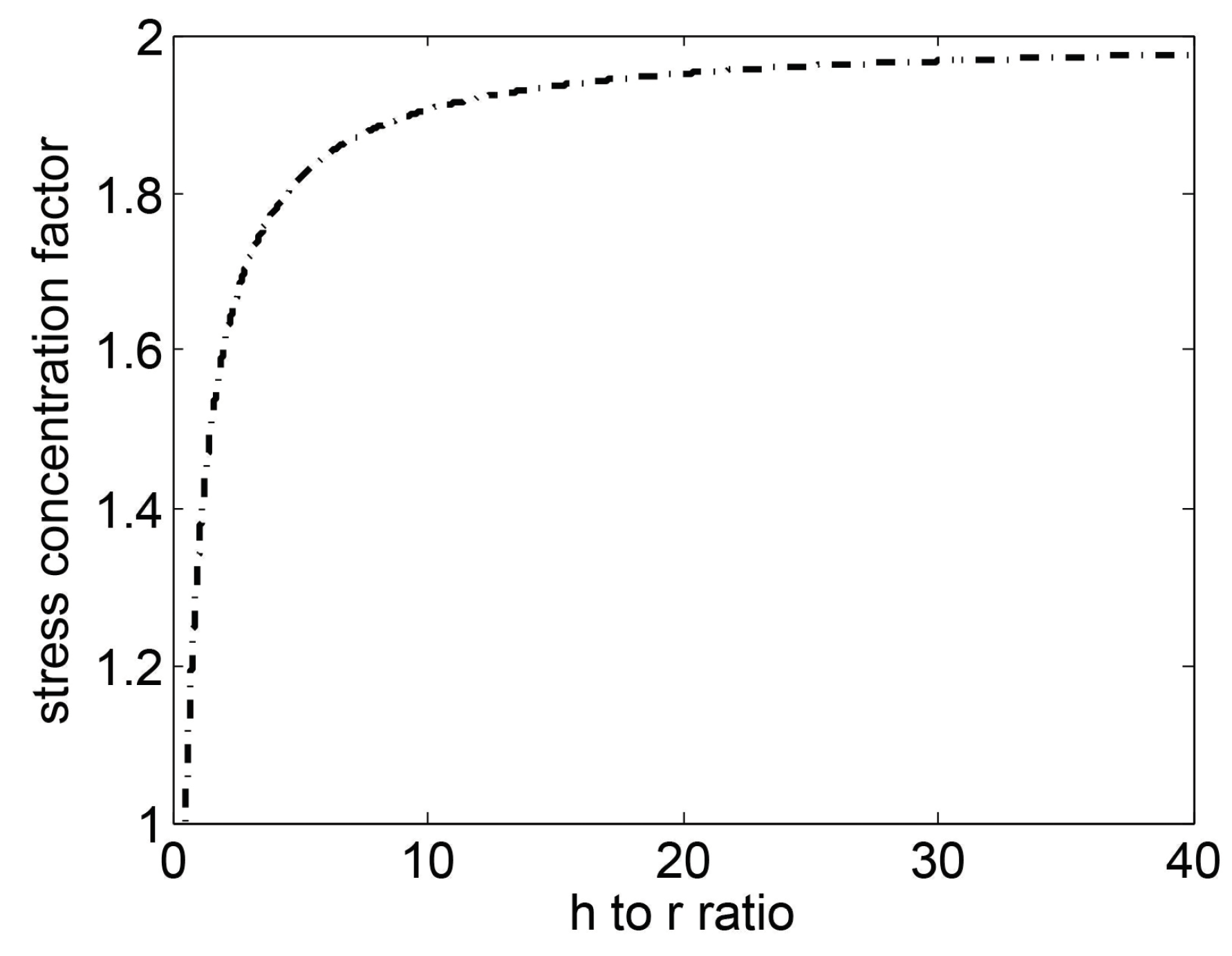
7.4. Discussion about the Achieved Results
- the introduction of a reliable index of the severity of stress concentration.
7.4.1. Adopted Equivalence Criterion
7.4.2. Stress Concentration Factor
8. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Elman, N.; Ho Duc, H.; Cima, M. An implantable MEMS drug delivery device for rapid delivery in ambulatory emergency care. Biomed. Microdevices 2009, 11, 625–631. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Shawgo, R.; Tyler, B.; Henderson, P.; Vogel, J.; Rosenberg, A.; Storm, P.; Langer, R.; Brem, H.; Cima, M. In vivo release from a drug delivery MEMS device. J. Control. Release 2004, 100, 211–219. [Google Scholar] [CrossRef] [PubMed]
- Lo, R.; Li, P.Y.; Saati, S.; Agrawal, R.; Humayun, M.; Meng, E. A passive MEMS drug delivery pump for treatment of ocular diseases. Biomed. Microdevices 2009, 11, 959–970. [Google Scholar] [CrossRef] [PubMed]
- Voskerician, G.; Shive, M.; Shawgo, R.; Von Recum, H.; Anderson, J.; Cima, M.; Langer, R. Biocompatibility and biofouling of MEMS drug delivery devices. Biomaterials 2003, 24, 1959–1967. [Google Scholar] [CrossRef]
- Xie, Y.; Xu, B.; Gao, Y. Controlled transdermal delivery of model drug compounds by MEMS microneedle array. Nanomed. Nanotechnol. Biol. Med. 2005, 1, 184–190. [Google Scholar] [CrossRef] [PubMed]
- Ibrahim, D. Using MEMS accelerometers in aerospace and defence electronics. Electron. World 2012, 118, 16–21. [Google Scholar]
- Leclerc, J. MEMs for aerospace navigation. IEEE Aerosp. Electron. Syst. Mag. 2007, 22, 31–36. [Google Scholar] [CrossRef]
- Hsu, H.; Hariz, A.; Omari, T.; Teng, M.; Sii, D.; Chan, S.; Lau, L.; Tan, S.; Lin, G.; Haskard, M.; et al. Development of a MEMS based manometric catheter for diagnosis of functional swallowing disorders. J. Phys. Conf. Ser. 2006, 34, 955–960. [Google Scholar] [CrossRef]
- Sun, H.; Fu, G.; Xie, H. A MEMS accelerometer-based real-time motion-sensing module for urological diagnosis and treatment. J. Med. Eng. Technol. 2013, 37, 127–134. [Google Scholar] [CrossRef] [PubMed]
- Horvath, I.; Panayotatos, P.; Lu, Y. Si MEMS microbearing with integrated safety sensors for surgical applications. Microelectron. J. 2001, 32, 1–9. [Google Scholar] [CrossRef]
- Lou, L.; Ramakrishna, K.; Shao, L.; Park, W.; Yu, D.; Lim, L.; Wee, Y.; Kripesh, V.; Feng, H.; Chua, B.; et al. Sensorized guidewires with MEMS tri-axial force sensor for minimally invasive surgical applications. In Proceedings of the 2010 Annual International Conference of the IEEE Engineering in Medicine and Biology, Buenos Aires, Argentina, 31 August–4 September 2010; pp. 6461–6464. [Google Scholar]
- To, G.; Qu, W.; Mahfouz, M. ASIC Design for Wireless Surgical MEMS Device and Instrumentation. In Proceedings of the 2006 International Conference of the IEEE Engineering in Medicine and Biology Society, New York, NY, USA, 30 August–3 September 2006; pp. 5892–5895. [Google Scholar]
- Chronis, N.; Lee, L. Polymer mems-based microgripper for single cell manipulation. In Proceedings of the 17th IEEE International Conference on Micro Electro Mechanical Systems (MEMS), Maastricht, The Netherlands, 25–29 January 2004; pp. 17–20. [Google Scholar]
- Kim, K.; Liu, X.; Zhang, Y.; Sun, Y. Nanonewton force-controlled manipulation of biological cells using a monolithic MEMS microgripper with two-axis force feedback. J. Micromech. Microeng. 2008, 18, 055013. [Google Scholar] [CrossRef]
- Belfiore, N.P.; Verotti, M.; Crescenzi, R.; Balucani, M. Design, optimization and construction of MEMS-based micro grippers for cell manipulation. In Proceedings of the ICSSE 2013—IEEE International Conference on System Science and Engineering, Budapest, Hungary, 4–6 July 2013; pp. 105–110. [Google Scholar]
- Balucani, M.; Belfiore, N.P.; Crescenzi, R.; Verotti, M. The development of a MEMS/NEMS-based 3 D.O.F. compliant micro robot. Int. J. Mech. Control 2011, 12, 3–10. [Google Scholar]
- Belfiore, N.P.; Simeone, P. Inverse kinetostatic analysis of compliant four-bar linkages. Mech. Mach. Theory 2013, 69, 350–372. [Google Scholar] [CrossRef]
- Verotti, M.; Dochshanov, A.; Belfiore, N.P. Compliance Synthesis of CSFH MEMS-Based Microgrippers. J. Mech. Des.-Trans. ASME 2017, 139, 022301. [Google Scholar] [CrossRef]
- Belfiore, N.P.; Broggiato, G.; Verotti, M.; Crescenzi, R.; Balucani, M.; Bagolini, A.; Bellutti, P.; Boscardin, M. Development of a MEMS technology CSFH based microgripper. In Proceedings of the 23rd International Conference on Robotics in Alpe-Adria-Danube Region, Smolenice, Slovakia, 3–5 September 2014; Institute of Electrical and Electronics Engineers Inc.: Piscataway Township, NJ, USA, 2015. [Google Scholar]
- Bagolini, A.; Ronchin, S.; Bellutti, P.; Chistè, M.; Verotti, M.; Belfiore, N.P. Fabrication of Novel MEMS Microgrippers by Deep Reactive Ion Etching With Metal Hard Mask. J. Microelectromech. Syst. 2017, 26, 926–934. [Google Scholar] [CrossRef]
- Belfiore, N.P.; Scaccia, M.; Ianniello, F.; Presta, M. Selective Compliance Hinge. U.S. Patent 8,191,204 B2, 5 June 2012. [Google Scholar]
- Balucani, M.; Belfiore, N.P.; Crescenzi, R.; Genua, M.; Verotti, M. Developing and modeling a plane 3 DOF compliant micromanipulator by means of a dedicated MBS code. In Proceedings of the 2011 NSTI Nanotechnology Conference and Expo, NSTI-Nanotech, At Boston, MA, USA, 13–16 June 2011; Volume 2, pp. 659–662. [Google Scholar]
- Belfiore, N.P.; Balucani, M.; Crescenzi, R.; Verotti, M. Performance analysis of compliant mems parallel robots through pseudo-rigid-body model synthesis. In Proceedings of the ASME 2012 11th Biennial Conference on Engineering Systems Design and Analysis, Nantes, France, 2–4 July 2012; Volume 3, pp. 329–334. [Google Scholar]
- Belfiore, N.P.; Emamimeibodi, M.; Verotti, M.; Crescenzi, R.; Balucani, M.; Nenzi, P. Kinetostatic optimization of a MEMS-based compliant 3 DOF plane parallel platform. In Proceedings of the ICCC 2013—IEEE 9th International Conference on Computational Cybernetics, Tihany, Hungary, 8–10 July 2013; pp. 261–266. [Google Scholar]
- Nenzi, P.; Crescenzi, R.; Dolgyi, A.; Klyshko, A.; Bondarenko, V.; Belfiore, N.P.; Balucani, M. High density compliant contacting technology for integrated high power modules in automotive applications. In Proceedings of the Electronic Components and Technology Conference, San Diego, CA, USA, 29 May–1 June 2012; pp. 1976–1983. [Google Scholar]
- Dochshanov, A.; Verotti, M.; Belfiore, N.P. A Comprehensive Survey on Microgrippers Design: Operational Strategy. J. Mech. Des.-Trans. ASME 2017, 139, 070801. [Google Scholar] [CrossRef]
- Verotti, M.; Dochshanov, A.; Belfiore, N.P. A Comprehensive Survey on Microgrippers Design: Mechanical Structure. J. Mech. Des.-Trans. ASME 2017, 139, 060801. [Google Scholar] [CrossRef]
- Di Giamberardino, P.; Bagolini, A.; Bellutti, P.; Rudas, I.; Verotti, M.; Botta, F.; Belfiore, N.P. New MEMS tweezers for the viscoelastic characterization of soft materials at the microscale. Micromachines 2017, 9, 15. [Google Scholar] [CrossRef]
- Belfiore, N.P.; Prosperi, G.; Crescenzi, R. A simple application of conjugate profile theory to the development of a silicon micro tribometer. In Proceedings of the ASME 2014 12th Biennial Conference on Engineering Systems Design and Analysis, Copenhagen, Denmark, 25–27 July 2014; Volume 2. [Google Scholar]
- Laermer, F.; Schlip, A. Method of Anisotropically Etching Silicon. U.S. Patent 5,501,893, 26 March 1996. [Google Scholar]
- Ayön, A.A.; Chen, K.S.; Lohner, K.A.; Spearing, S.M.; Sawin, H.H.; Schmidt, M.A. Deep Reactive Ion Etching of Silicon. In Proceedings of the Symposium AA Materials Science of Microelectromechanical Systems (MEMS), Boston, MA, USA, 1–2 December 1998; Volume 546. [Google Scholar]
- Chen, K.S.; Ayön, A.A.; Zhang, X.; Spearing, S. Effect of process parameters on the surface morphology and mechanical performance of silicon structures after deep reactive ion etching (DRIE). J. Microelectromech. Syst. 2002, 11, 264–275. [Google Scholar] [CrossRef]
- Fricker, H. Why does charge concentrate on points? Phys. Educ. 1989, 24, 157. [Google Scholar] [CrossRef]
- Crescenzi, R.; Balucani, M.; Belfiore, N.P. Operational characterization of CSFH MEMS technology based hinges. J. Micromech. Microeng. 2018, 28, 055012. [Google Scholar] [CrossRef]
- Mita, Y.; Sugiyama, M.; Kubota, M.; Marty, F.; Bourouina, T.; Shibata, T. Aspect Ratio Dependent Scalloping Attenuation in Drie and an Application to Low-Loss Fiber-Optical Switches. In Proceedings of the 19th IEEE International Conference on Micro Electro Mechanical Systems, Istanbul, Turkey, 22–26 January 2006; pp. 114–117. [Google Scholar]
- Pham, P.; Dao, D.; Amaya, S.; Kitada, R.; Sugiyama, S. Fabrication and characterization of smooth Si mold for hot embossing process. IEEJ Trans. Sens. Micromach. 2007, 127, 187–191. [Google Scholar] [CrossRef]
- Song, I.H.; Peter, Y.A.; Meunier, M. Smoothing dry-etched microstructure sidewalls using focused ion beam milling for optical applications. J. Micromech. Microeng. 2007, 17, 1593–1597. [Google Scholar] [CrossRef]
- Defforge, T.; Song, X.; Gautier, G.; Tillocher, T.; Dussart, R.; Kouassi, S.; Tran-Van, F. Scalloping removal on DRIE via using low concentrated alkaline solutions at low temperature. Sens. Actuators A-Phys. 2011, 170, 114–120. [Google Scholar] [CrossRef]
- Hung, Y.J.; Lee, S.L.; Thibeault, B.J.; Coldren, L.A. Realization of silicon nanopillar arrays with controllable sidewall profiles by holography lithography and a novel single-step deep reactive ion etching. In Symposia P Q R Low-Dimensional Functional Nanostructures-Fabrication, Characterization and Applications; Materials Research Society Online Proceedings Library Archive, Cambridge University Press: Cambridge, UK, 2010; Volume 1258. [Google Scholar]
- Inagaki, N.; Sasaki, H.; Shikida, M.; Sato, K. Selective removal of micro-corrugation by anisotropic wet etching. In Proceedings of the TRANSDUCERS 2009—2009 International Solid-State Sensors, Actuators and Microsystems Conference, Denver, CO, USA, 21–25 June 2009; pp. 1865–1868. [Google Scholar]
- Phinney, L.; McKenzie, B.; Ohlhausen, J.; Buchheit, T.; Shul, R. Characterization of SOI MEMS sidewall roughness. In Proceedings of the ASME 2011 International Mechanical Engineering Congress and Exposition, Denver, CO, USA, 11–17 November 2011; Volume 11, pp. 187–193. [Google Scholar]
- Chekurov, N.; Grigoras, K.; Peltonen, A.; Franssila, S.; Tittonen, I. The fabrication of silicon nanostructures by local gallium implantation and cryogenic deep reactive ion etching. Nanotechnology 2009, 20, 065307. [Google Scholar] [CrossRef] [PubMed]
- De Boer, M.; Gardeniers, J.; Jansen, H.; Smulders, E.; Gilde, M.J.; Roelofs, G.; Sasserath, J.; Elwenspoek, M. Guidelines for etching silicon MEMS structures using fluorine high-density plasmas at cryogenic temperatures. J. Microelectromech. Syst. 2002, 11, 385–401. [Google Scholar] [CrossRef]
- Murakami, K.; Wakabayashi, Y.; Minami, K.; Esashi, M. Cryogenic dry etching for high aspect ratio microstructures. In Proceedings of the IEEE Micro Electro Mechanical Systems, Fort Lauderdale, FL, USA, 10 February 1993; IEEE: Piscataway, NJ, USA, 1993; pp. 65–70. [Google Scholar]
- Sainiemi, L.; Franssila, S. Mask material effects in cryogenic deep reactive ion etching. J. Vac. Sci. Technol. B 2007, 25, 801–807. [Google Scholar] [CrossRef]
- Morikawa, Y.; Murayama, T.; Sakuishi, T.; Nakamura, T.; Kurimoto, T.; Nakamuta, Y.; Kimura, I.; Suu, K. Scallop Free si Etching and Low Cost Integration Technologies for 2.5D Si Interposer; International Microelectronics Assembly and Packaging Society: Research Triangle Park, NC, USA, 2012; pp. 998–1000. [Google Scholar]
- Guo, M.; Chou, X.; Mu, J.; Liu, B.; Xiong, J. Fabrication of micro-trench structures with high aspect ratio based on DRIE process for MEMS device applications. Microsyst. Technol. 2013, 19, 1097–1103. [Google Scholar] [CrossRef]
- Miller, K.; Li, M.; Walsh, K.; Fu, X.A. The effects of DRIE operational parameters on vertically aligned micropillar arrays. J. Micromech. Microeng. 2013, 23, 035039. [Google Scholar] [CrossRef]
- Ma, Z.; Jiang, C.; Yuan, W.; He, Y. Large-scale patterning of hydrophobic silicon nanostructure arrays fabricated by dual lithography and deep reactive ion etching. Nano-Micro Lett. 2013, 5, 7–12. [Google Scholar] [CrossRef]
- Wang, Z.; Jiang, F.; Yu, D.; Zhang, W. Si Etching for TSV Formation. ECS Trans. 2014, 60, 407–412. [Google Scholar] [CrossRef]
- Zhao, Y.P.; Wang, L.S.; Yu, T.X. Mechanics of adhesion in MEMS—A review. J. Adhes. Sci. Technol. 2003, 17, 519–546. [Google Scholar] [CrossRef]
- Fraeijs de Veubeke, B.M. A Course in Elasticity; Springer: New York, NY, USA, 1979; pp. 135–200. [Google Scholar]
- Boresi, A.; Schmidt, R. Advanced Mechanics of Materials; John Wiley & Sons: Hoboken, NJ, USA, 2003. [Google Scholar]
- Dell’Isola, F.; Ruta, G.C. Outlooks in Saint-Venant theory III. Torsion and flexure in sections of variable thickness by formal expansions. Arch. Mech. 1997, 49, 321–343. [Google Scholar]
- Andreaus, U.; Ruta, G. A review of the problem of the shear centre(s). Contin. Mech. Thermodyn. 1998, 10, 369–380. [Google Scholar] [CrossRef]
- Paolone, A.; Ruta, G.; Vidoli, S. Torsion in multi-cell thin-walled girders. Acta Mech. 2009, 206, 163–171. [Google Scholar] [CrossRef]
- Ruta, G. On the flexure of a Saint-Venant cylinder. J. Elast. 1998, 52, 99–110. [Google Scholar] [CrossRef]
- Ruta, G. On Kelvin’s formula for torsion of thin cylinders. Mech. Res. Commun. 1999, 26, 591–596. [Google Scholar] [CrossRef]
- Saint-Venant, B. Mémoire sur la torsion des prismes. Mem. Savants Etrang. 1855, 14, 233–560. [Google Scholar]
- Clebsch, R.F.A. Theorie der Elasticität Fester Körper; B. G. Teubner: Leipzig, Germany, 1862. [Google Scholar]
- Iesan, D. On Saint-Venant’s problem. Arch. Ration. Mech. Anal. 1986, 91, 363–373. [Google Scholar] [CrossRef]
- Muskhelishvili, N.I. Some Basic Problems of the Mathematical Theory of Elasticity; Noordhoff Ltd.: Groningen, The Netherlands, 1963; pp. 571–607. [Google Scholar]
- Gruttman, F.; Sauer, R.; Wagner, W. Shear Stresses in Prismatic Beams with Arbitrary Cross-Sections. Int. J. Numer. Methods Eng. 1999, 45, 865–889. [Google Scholar] [CrossRef]
- Cecchi, R.; Verotti, M.; Capata, R.; Dochshanov, A.; Broggiato, G.; Crescenzi, R.; Balucani, M.; Natali, S.; Razzano, G.; Lucchese, F.; et al. Development of micro-grippers for tissue and cell manipulation with direct morphological comparison. Micromachines 2015, 6, 1710–1728. [Google Scholar] [CrossRef]
- Timoshenko, S.; Goodier, J. Theory of Elasticity; McGraw-Hill: New York, NY, USA, 1970. [Google Scholar]
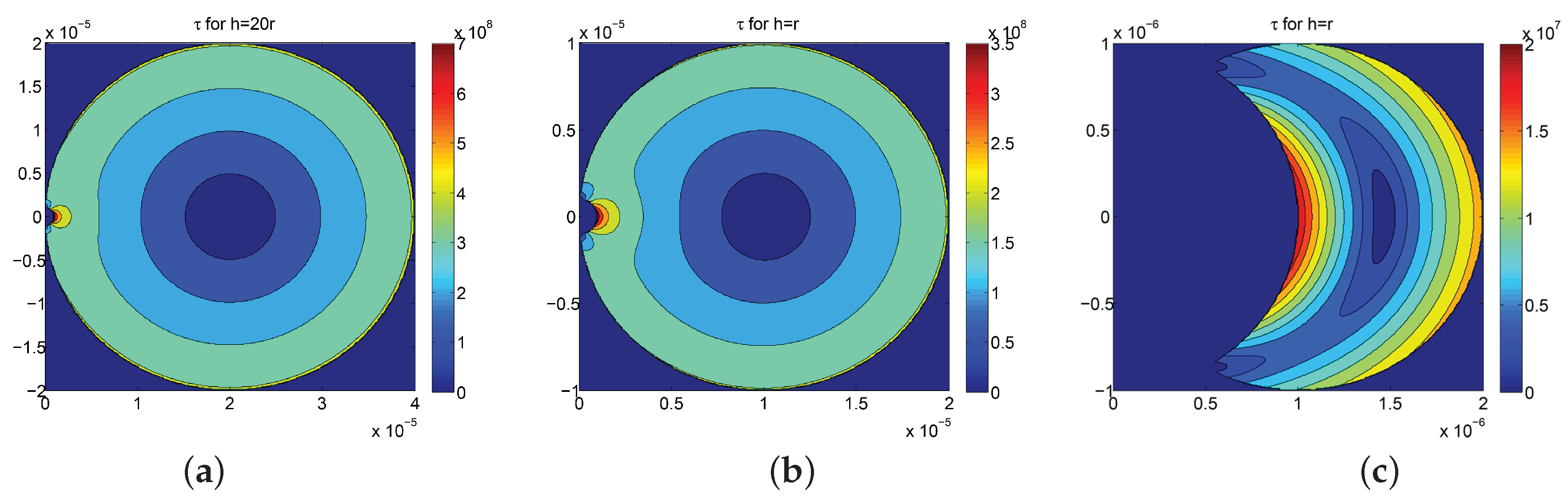
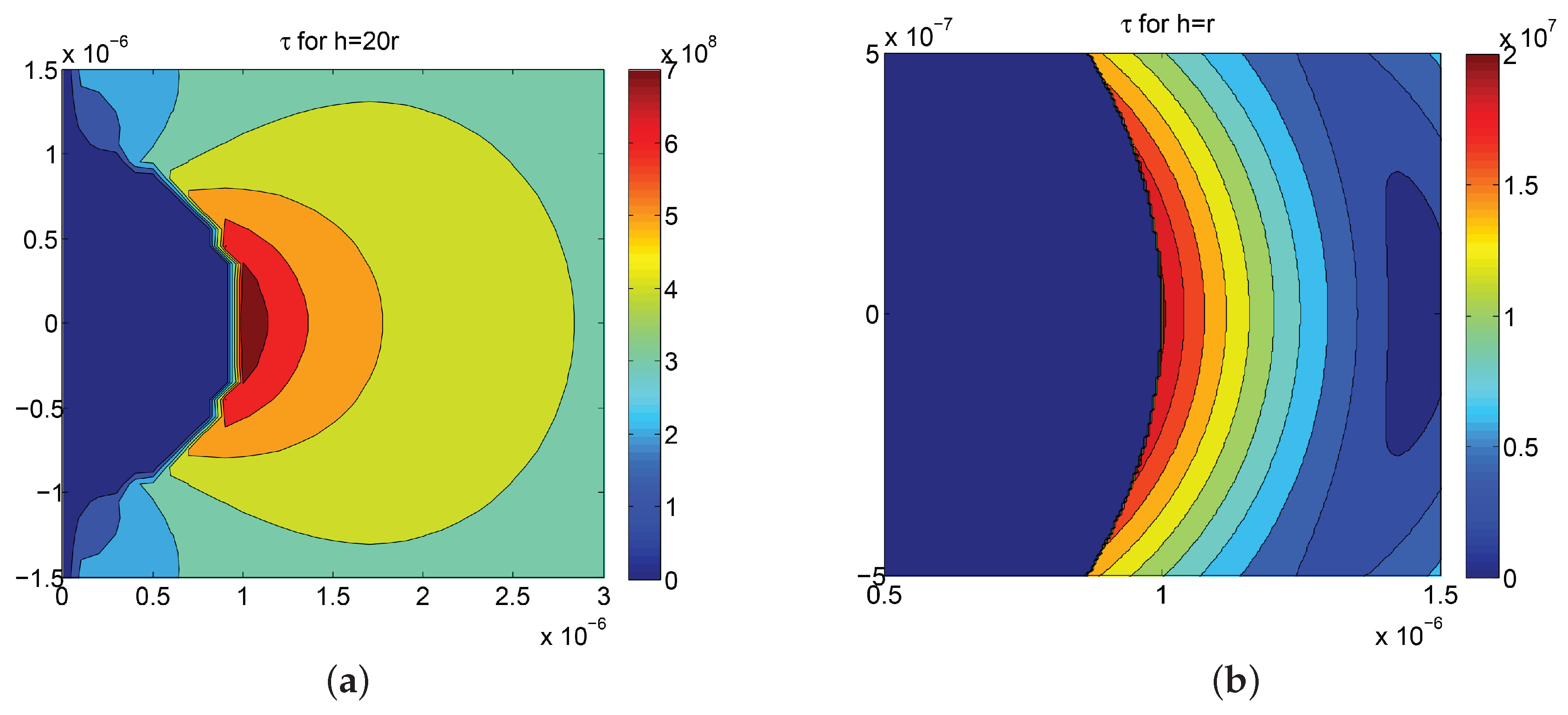
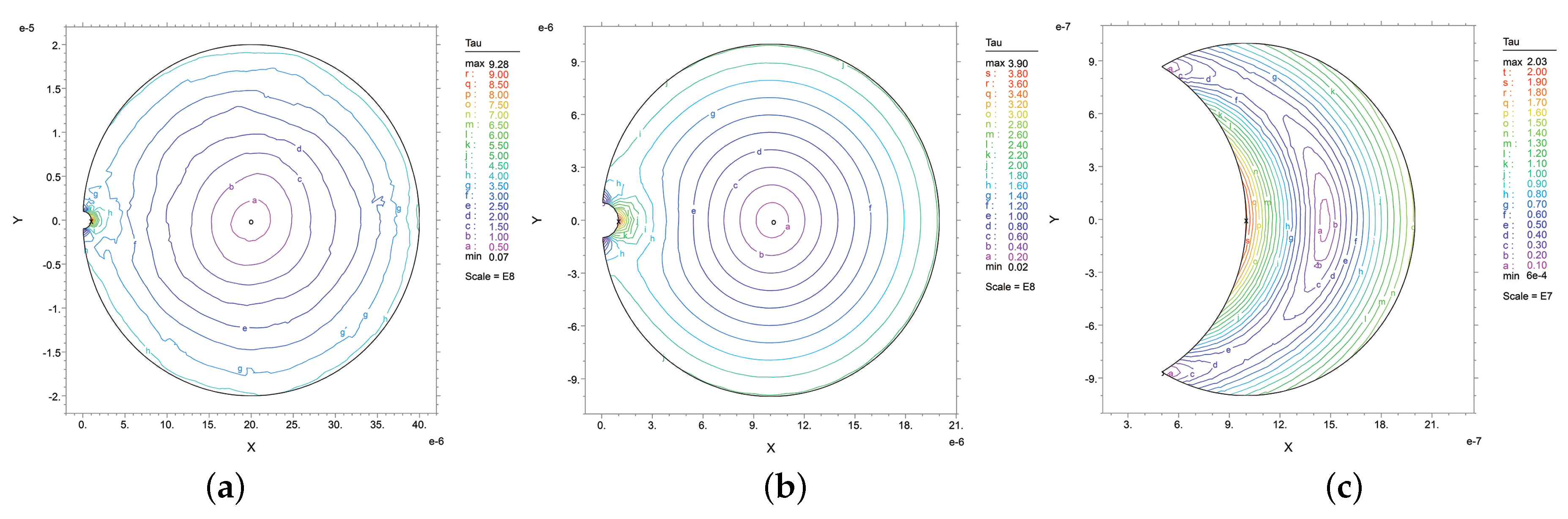
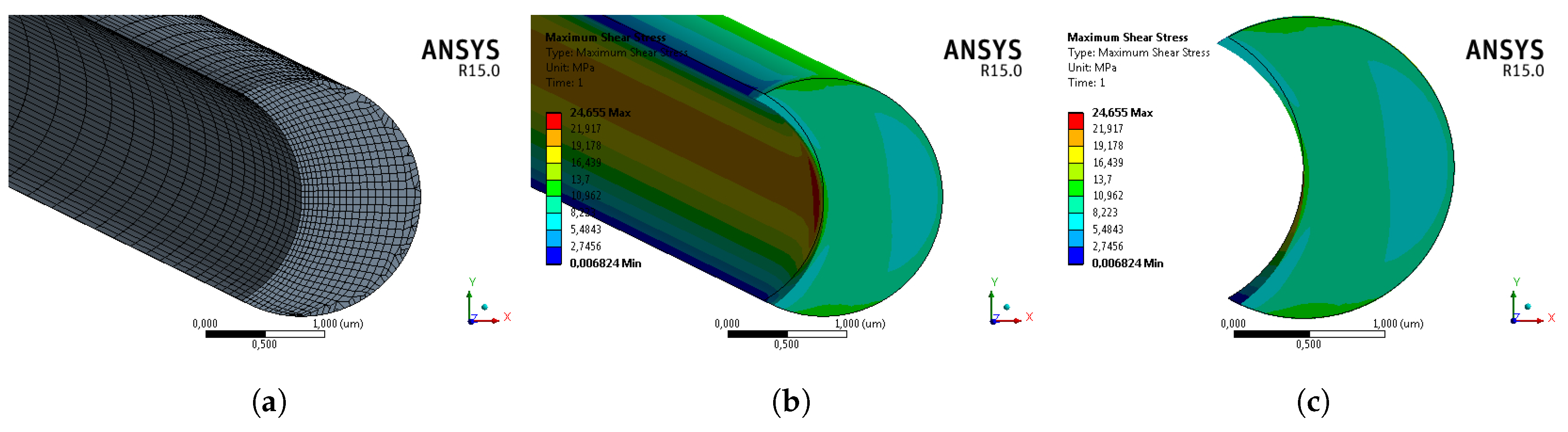
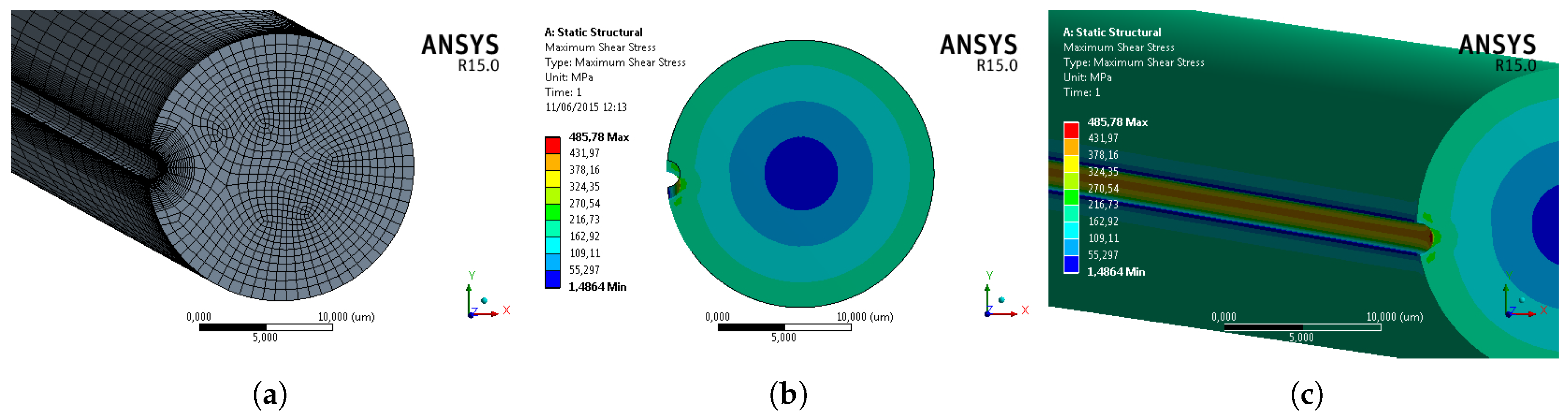
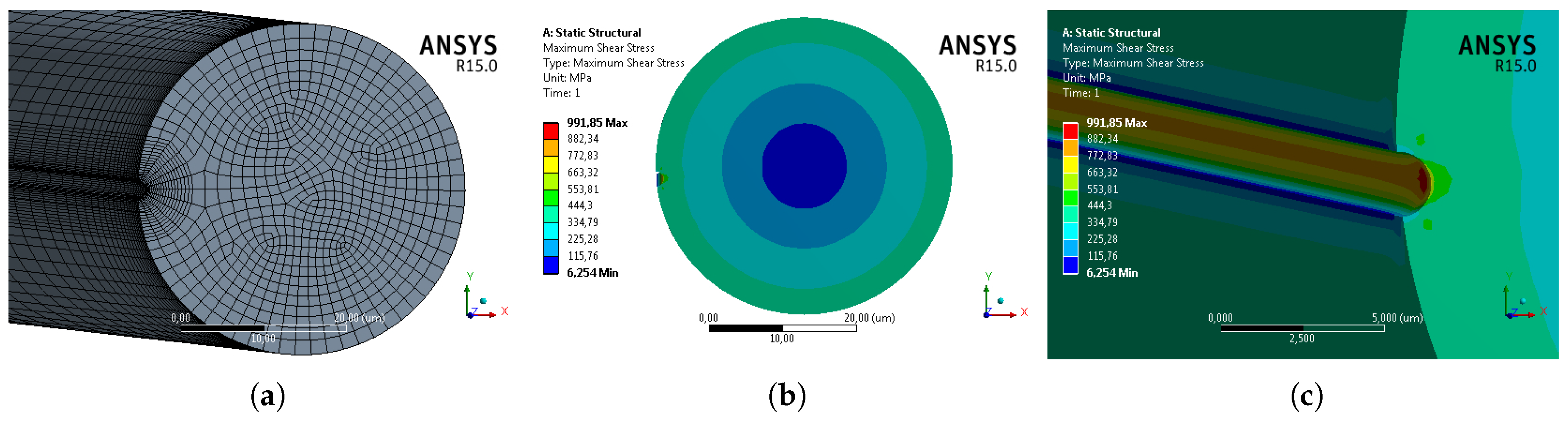
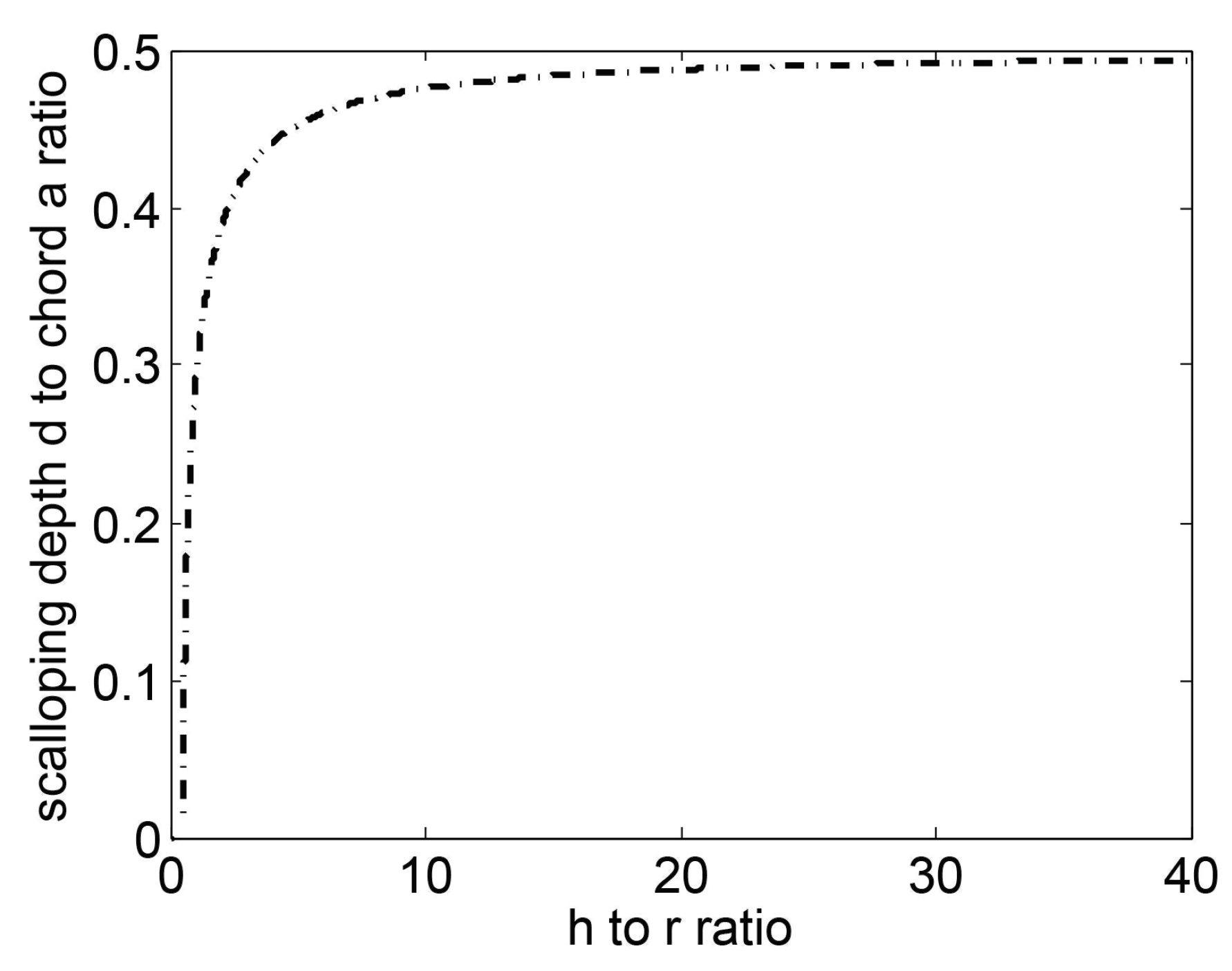
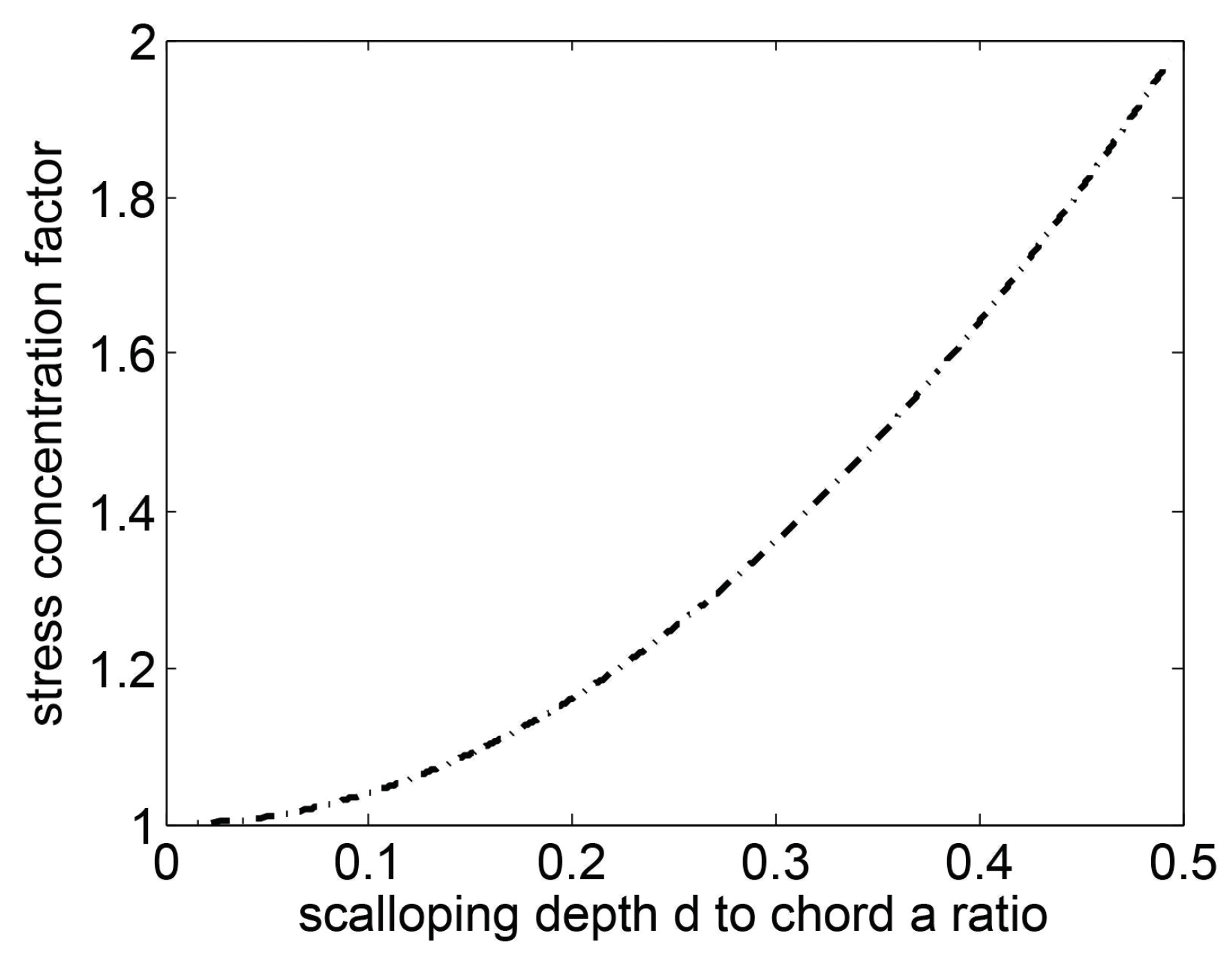
| h | Point | Position | (MPa) | ||||
|---|---|---|---|---|---|---|---|
| x | y | Analytical | Flex PDE | FEA | |||
| mid* | min, max* | ||||||
| r | Core | r | 0 | 19.9 | 20.3 | 20.37 | 24.66 |
| Edge | 0 | <6.0 | |||||
| Core | r | 0 | 356 | 390 | |||
| Edge | 0 | <20 | |||||
| Core | r | 0 | 727 | 928 | |||
| Edge | 0 | <150 | |||||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bertini, S.; Verotti, M.; Bagolini, A.; Bellutti, P.; Ruta, G.; Belfiore, N.P. Scalloping and Stress Concentration in DRIE-Manufactured Comb-Drives. Actuators 2018, 7, 57. https://doi.org/10.3390/act7030057
Bertini S, Verotti M, Bagolini A, Bellutti P, Ruta G, Belfiore NP. Scalloping and Stress Concentration in DRIE-Manufactured Comb-Drives. Actuators. 2018; 7(3):57. https://doi.org/10.3390/act7030057
Chicago/Turabian StyleBertini, Silvia, Matteo Verotti, Alvise Bagolini, Pierliugi Bellutti, Giuseppe Ruta, and Nicola Pio Belfiore. 2018. "Scalloping and Stress Concentration in DRIE-Manufactured Comb-Drives" Actuators 7, no. 3: 57. https://doi.org/10.3390/act7030057
APA StyleBertini, S., Verotti, M., Bagolini, A., Bellutti, P., Ruta, G., & Belfiore, N. P. (2018). Scalloping and Stress Concentration in DRIE-Manufactured Comb-Drives. Actuators, 7(3), 57. https://doi.org/10.3390/act7030057