Novel Nozzle Shapes for Synthetic Jet Actuators Intended to Enhance Jet Momentum Flux
Abstract
1. Introduction
2. Problem Parameters
2.1. Basic Synthetic Jet Dimensionless Parameters
2.2. Synthetic Jet Integral Quantities
2.3. Parameters for Lumped Element Model
3. Measurement Methods
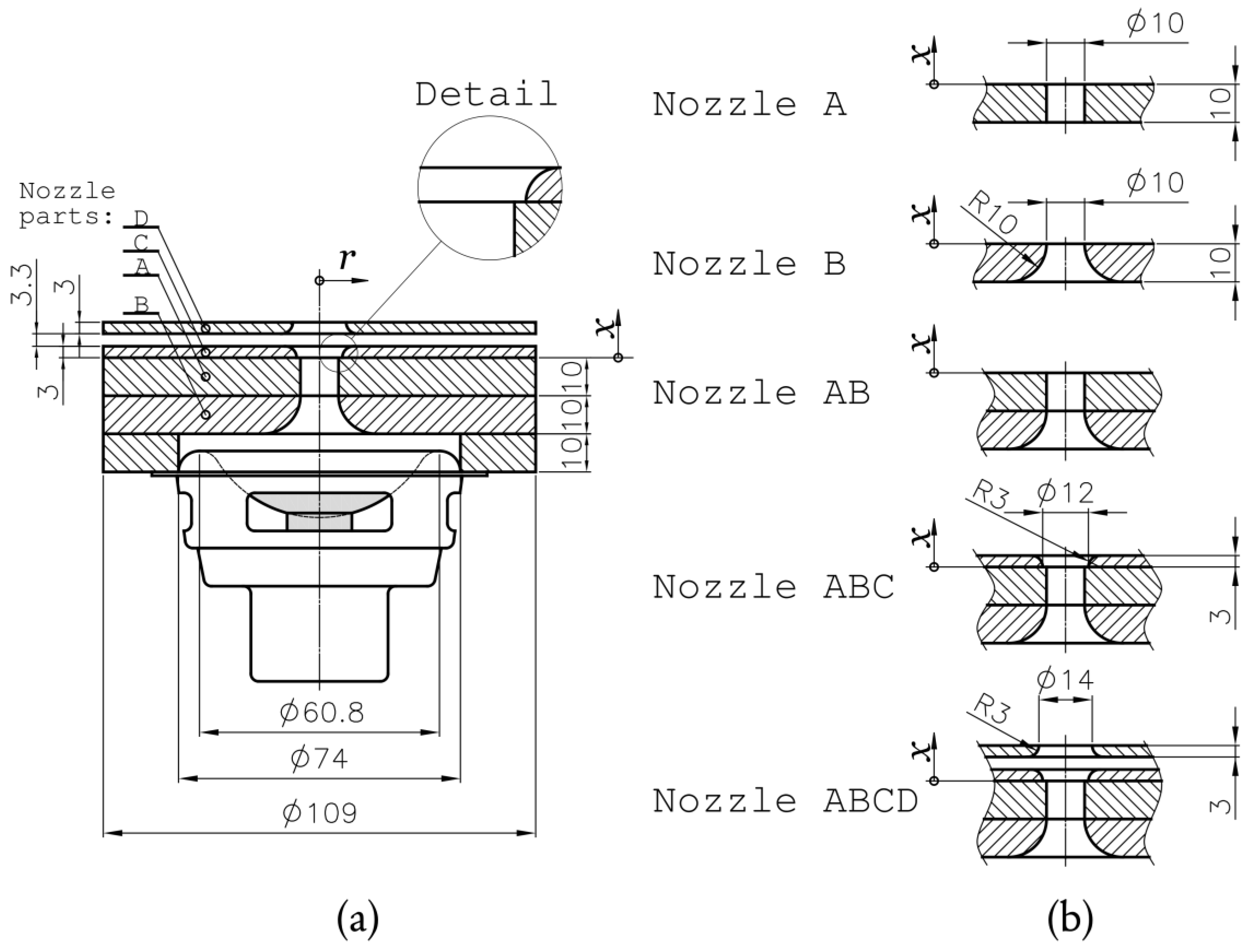
3.1. The Synthetic Jet Actuator
- Nozzle AB was a combination of the previous nozzles. Note that an experimental study of similar nozzle geometries was done, e.g., by Nani and Smith [32].
- The fourth nozzle was denoted as ABC (it consists of three nozzle plates A+B+C, see Figure 1a) and had a special novel design with rounded lips at both inner and outer nozzle ends. A similar shape was tested by Lee and Goldstein [36] for a slot-type nozzle. However, this study’s nozzle was circular and equipped with a small step at the end of the straight nozzle section. This step promoted flow separation and prevented flow reattachment during extrusion stroke.
- The ABCD nozzle combined the design by Rylatt and O’Donovan [37] with the ABC nozzle. The nozzle contained an auxiliary outer nozzle—denoted as part D, see Figure 1a. The auxiliary nozzle had a greater diameter (12.0 mm) than Dn = 10.0 mm and was placed 3.3 mm downstream from the exit of nozzle ABC, see Figure 1a. The rounded edge of the part D was oriented towards the actuator outlet, helping to direct the radially dispersed momentum flux along the x-axis.
3.2. Data Acquisition and Measurement Methods
3.2.1. Measurement of the Jet Thrust Using Precision Scales
- For relatively weak SJs at the dimensionless stroke length of L0/Dn from 0.5 to 5 (i.e., including the vicinity of the SJ existence threshold), the maximum deviation is within ±5.4%.
- For stronger SJs at L0/Dn ≥ 5, the maximum deviation is only ±3.4%.
3.2.2. Hot-Wire and Cavity Pressure Measurements
4. Experimental Results
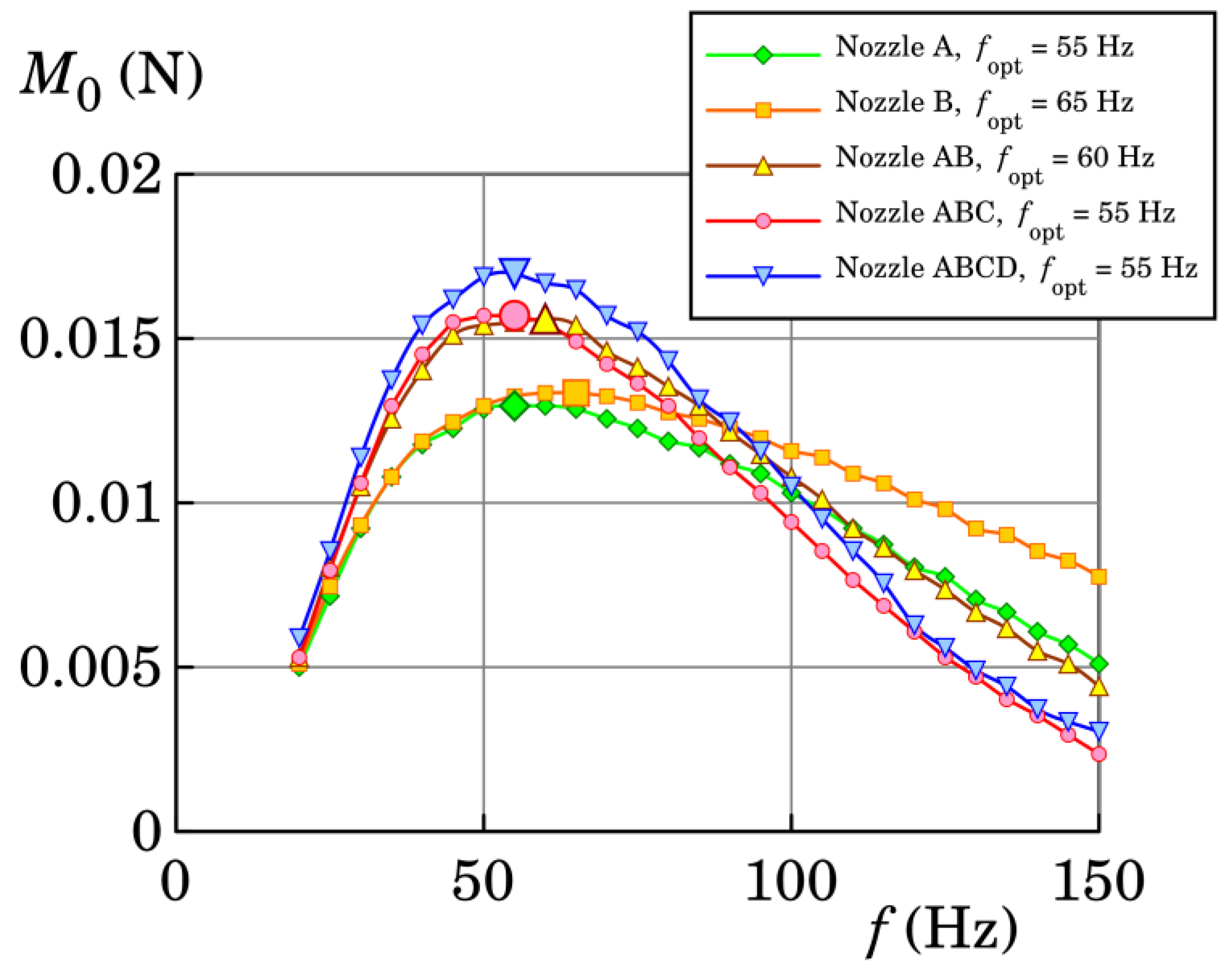
4.1. Frequency Characteristics
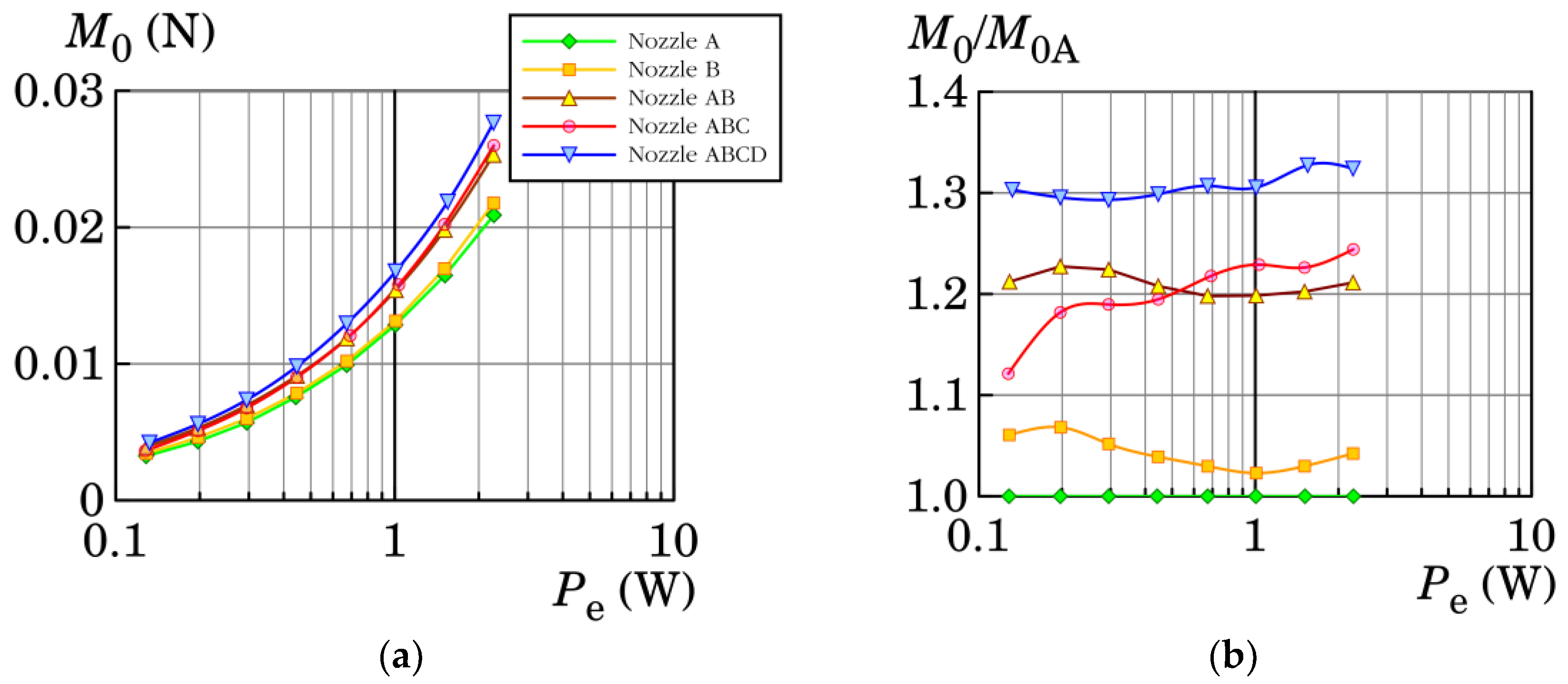
4.2. Synthetic Jet Thrust Measurement
4.3. Measurement of Velocity Profiles
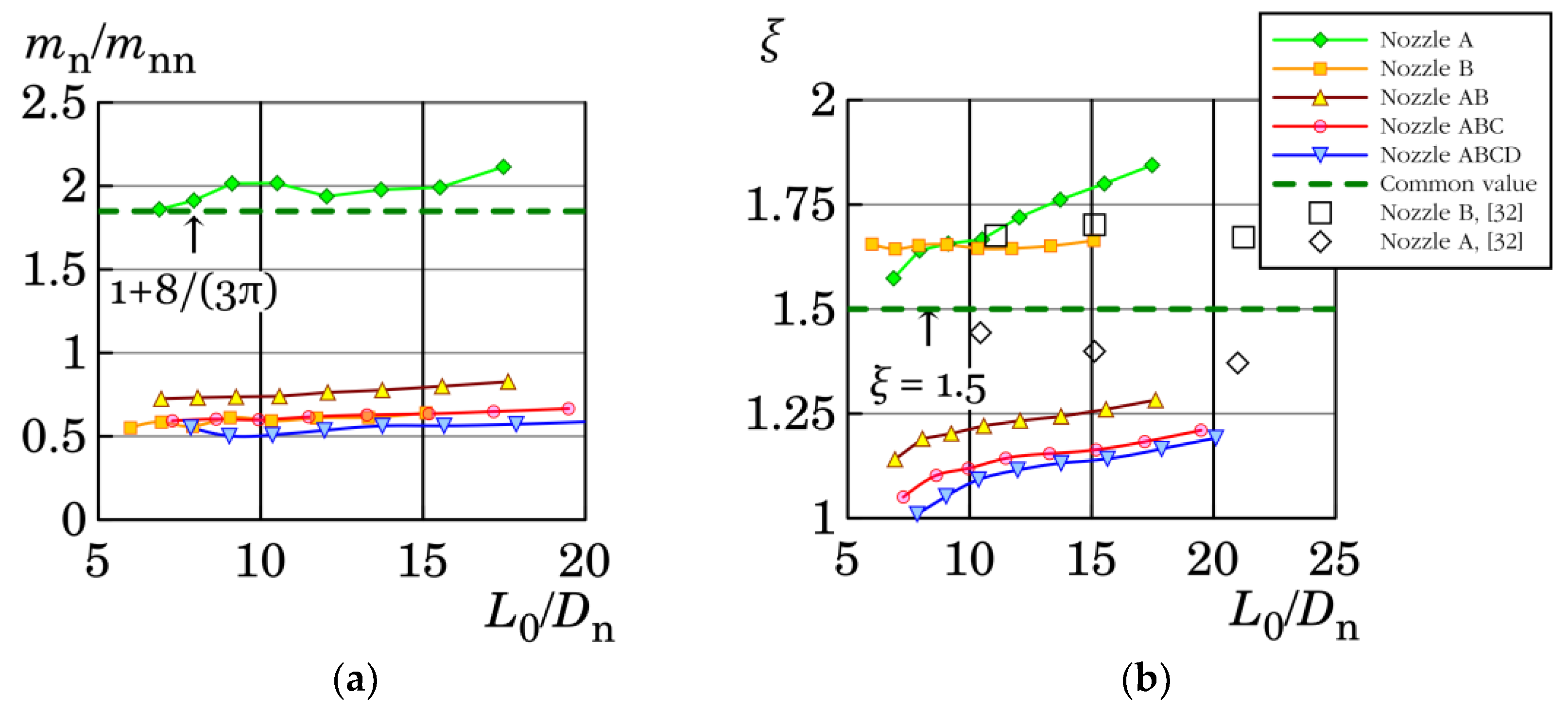
4.4. Evaluation of mn and ξ
5. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
Nomenclature
| D | diameter, m |
| e | electrical voltage, V |
| f | driving frequency, Hz |
| g | magnitude of the gravitational acceleration, m/s2 |
| i | electrical current, A |
| L | length, m |
| M | momentum flux, N |
| m | mass, kg |
| p | pressure, Pa |
| P | input power, W |
| r | radial coordinate, m |
| Re | Reynolds number, 1 |
| S | cross-sectional area, m2 |
| T | time period, s |
| t | time, s |
| u | velocity, m/s |
| x | axial coordinate, m |
| ρ | density, kg/m3 |
| Subscripts | |
| 0 | averaging in time and space |
| A | related to the nozzle A |
| b | barometric |
| c | cavity |
| d | diaphragm |
| e | electrical |
| E | extrusion stroke |
| n | nozzle |
| nn | related to nozzle internal |
| Superscripts | |
| * | dimensionless |
Appendix A. Estimation of mn and ξ
References
- Smith, B.L.; Glezer, A. The formation and evolution of synthetic jets. Phys. Fluids 1998, 10, 2281–2297. [Google Scholar] [CrossRef]
- Cater, J.E.; Soria, J. The evolution of round zero-net-mass-flux jets. J. Fluid Mech. 2002, 472, 167–200. [Google Scholar] [CrossRef]
- Lardeau, S.; Leschziner, M.A. The interaction of round synthetic jets with a turbulent boundary layer separating from a rounded ramp. J. Fluid Mech. 2011, 683, 172–211. [Google Scholar] [CrossRef]
- Bernardini, C.; Carnevale, M.; Manna, M.; Martelli, F.; Simoni, D.; Zunino, P. Turbine blade boundary layer separation suppression via synthetic jet: An experimental and numerical study. J. Therm. Sci. 2012, 21, 404–412. [Google Scholar] [CrossRef]
- Jabbal, M.; Zhong, S. Particle image velocimetry measurements of the interaction of synthetic jets with a zero-pressure gradient laminar boundary layer. Phys. Fluids 2010, 22, 063603. [Google Scholar] [CrossRef]
- Sahni, O.; Wood, J.; Jansen, K.E.; Amitay, M. Three-dimensional interactions between a finite-span synthetic jet, a crossflow. J. Fluid Mech. 2011, 671, 254–287. [Google Scholar] [CrossRef]
- Xia, X.; Mohseni, K. Transitional region of a round synthetic jet. Phys. Rev. Fluids 2018, 3, 011901. [Google Scholar] [CrossRef]
- Chiatto, M.; Capuano, F.; de Luca, L. Numerical and experimental characterization of a double-orifice synthetic jet actuator. Meccanica 2018, 53, 2883–2896. [Google Scholar] [CrossRef]
- Seo, J.H.; Cadieux, F.; Mittal, R.; Deem, E.; Cattafesta, L. Effect of synthetic jet modulation schemes on the reduction of a laminar separation bubble. Phys. Rev. Fluids 2018, 3, 033901. [Google Scholar] [CrossRef]
- Xia, Z.-X.; Luo, Z.-B. Physical factors of primary jet vectoring control using synthetic jet actuators. Appl. Math. Mech. 2007, 28, 907–920. [Google Scholar] [CrossRef]
- Ben Chiekh, M.; Ferchichi, M.; Béra, J.-C.C. Aerodynamic flow vectoring of a wake using asymmetric synthetic jet actuation. Exp. Fluids 2012, 53, 1797–1813. [Google Scholar] [CrossRef]
- Trávníček, Z.; Němcová, L.; Kordík, J.; Tesař, V.; Kopecký, V. Axisymmetric impinging jet excited by a synthetic jet system. Int. J. Heat Mass Transf. 2012, 55, 1279–1290. [Google Scholar] [CrossRef]
- McGuinn, A.; Farrelly, R.; Persoons, T.; Murray, D.B. Flow regime characterisation of an impinging axisymmetric synthetic jet. Exp. Therm. Fluid Sci. 2013, 47, 241–251. [Google Scholar] [CrossRef]
- Lee, A.; Yeoh, G.H.; Timchenko, V.; Reizes, J.A. Heat transfer enhancement in micro-channel with multiple synthetic jets. Appl. Therm. Eng. 2012, 48, 275–288. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, P.; Xie, Y. Numerical investigation of heat transfer characteristics of impinging synthetic jets with different waveforms. Int. J. Heat Mass Transf. 2018, 125, 1017–1027. [Google Scholar] [CrossRef]
- Trávníček, Z.; Vít, T.; Tesař, V. Hybrid synthetic jets as the nonzero-net-mass-flux synthetic jets. Phys. Fluids 2006, 18, 081701. [Google Scholar] [CrossRef]
- Trávníček, Z.; Tesař, V.; Kordík, J. Performance of synthetic jet actuators based on hybrid and double-acting principles. J. Vis. 2008, 11, 221–229. [Google Scholar] [CrossRef]
- Kordík, J.; Trávníček, Z. Novel fluidic diode for hybrid synthetic jet actuator. J. Fluids Eng. 2013, 135, 101101. [Google Scholar] [CrossRef]
- Kordík, J.; Broučková, Z.; Trávníček, Z. Impinging jet-based fluidic diodes for hybrid synthetic jet actuators. J. Vis. 2015, 18, 449–458. [Google Scholar] [CrossRef]
- Gallas, Q.; Holman, R.; Nishida, T.; Carroll, B.; Sheplak, M.; Cattafesta, L. Lumped element modeling of piezoelectric-driven synthetic jet actuators. AIAA J. 2003, 41, 240–247. [Google Scholar] [CrossRef]
- Kim, B.H.; Williams, D.R.; Emo, S.; Acharya, M. Modeling pulsed-blowing systems for flow control. AIAA J. 2005, 43, 314–325. [Google Scholar] [CrossRef]
- Kordík, J.; Trávníček, Z. Axisymmetric synthetic jet actuators with large streamwise dimensions. AIAA J. 2013, 51, 2862–2877. [Google Scholar] [CrossRef]
- De Luca, L.; Girfoglio, M.; Coppola, G. Modeling and experimental validation of the frequency response of synthetic jet actuators. AIAA J. 2014, 52, 1733–1748. [Google Scholar] [CrossRef]
- Sharma, R.N. Fluid-dynamic-based analytical model for synthetic jet actuation. AIAA J. 2007, 45, 1841–1847. [Google Scholar] [CrossRef]
- Chiatto, M.; Capuano, F.; Coppola, G.; de Luca, L. LEM characterization of synthetic jet actuators driven by piezoelectric element: A review. Sensors 2017, 17, 1216. [Google Scholar] [CrossRef] [PubMed]
- Broučková, Z.; Trávníček, Z. Visualization study of hybrid synthetic jets. J. Vis. 2015, 18, 581–593. [Google Scholar] [CrossRef]
- Mohseni, K.; Mittal, R. Synthetic Jets: Fundamentals and Applications; CRC Press, Taylor & Francis: Boca Raton, FL, USA, 2015. [Google Scholar]
- Persoons, T.; O’Donovan, T.S. A pressure-based estimate of synthetic jet velocity. Phys. Fluids 2007, 19, 128104. [Google Scholar] [CrossRef]
- Persoons, T. General reduced-order model to design and operate synthetic jet actuators. AIAA J. 2012, 50, 916–927. [Google Scholar] [CrossRef]
- Kordík, J.; Trávníček, Z. Optimal diameter of nozzles of synthetic jet actuators based on electrodynamic transducers. Exp. Therm. Fluid Sci. 2017, 86, 281–294. [Google Scholar] [CrossRef]
- Kordík, J.; Broučková, Z.; Vít, T.; Pavelka, M.; Trávníček, Z. Novel methods for evaluation of the Reynolds number of synthetic jets. Exp. Fluids 2014, 55, 1757. [Google Scholar] [CrossRef]
- Nani, D.J.; Smith, B.L. Effect of orifice inner lip radius on synthetic jet efficiency. Phys. Fluids 2012, 24, 115110. [Google Scholar] [CrossRef]
- Kordík, J.; Trávníček, Z.; Timchenko, V.; Ismail, N.A. The predominant effect of stroke length on velocity profiles at the exit of axisymmetric synthetic jet actuators. Int. J. Heat Fluid Flow 2017, 66, 197–208. [Google Scholar] [CrossRef]
- Tesař, V.; Kordík, J. Quasi-similarity model of synthetic jets. Sens. Actuators A Phys. 2009, 149, 255–265. [Google Scholar] [CrossRef]
- Tesař, V.; Kordík, J. Time-mean structure of axisymmetric synthetic jets. Sens. Actuators A Phys. 2010, 161, 217–224. [Google Scholar] [CrossRef]
- Lee, C.Y.; Goldstein, D.B. Two-dimensional synthetic jet simulation. AIAA J. 2002, 40, 510–516. [Google Scholar] [CrossRef]
- Rylatt, D.I.; O’Donovan, T.S. Heat transfer enhancement to a confined impinging synthetic air jet. Appl. Therm. Eng. 2013, 51, 468–475. [Google Scholar] [CrossRef]
- Kordík, J.; Trávníček, Z. Non-harmonic excitation of synthetic jet actuators based on electrodynamic transducers. Int. J. Heat Fluid Flow 2018, 73, 154–162. [Google Scholar] [CrossRef]
- Trávníček, Z.; Fedorchenko, A.I.; Wang, A.-B. Enhancement of synthetic jets by means of an integrated valve-less pump, Part I: Design of the actuator. Sens. Actuator A Phys. 2005, 120, 232–240. [Google Scholar] [CrossRef]
- Kooijman, G.; Ouweltjes, O. Finite difference time domain electroacoustic model for synthetic jet actuators including nonlinear flow resistance. J. Acoust. Soc. Am. 2009, 125, 1911–1918. [Google Scholar] [CrossRef] [PubMed]
- Trávníček, Z.; Broučková, Z. A synthetic jet issuing from a bio-inspired actuator with an oscillating nozzle lip. J. Fluids Eng. 2018, 140, 101104. [Google Scholar] [CrossRef]
- Kordík, J.; Trávníček, Z. Integral quantities of axisymmetric synthetic jets evaluated from a direct jet thrust measurement. Flow Turbul. Combust. 2018. submitted. [Google Scholar]
- Kordík, J. Theoretical and Experimental Analysis of Synthetic and Hybrid Synthetic Jet Actuators. Ph.D. Thesis, Czech Technical University, Prague, Czech Republic, 2011. [Google Scholar]

| Quantity | Symbol | Value | Unit |
|---|---|---|---|
| Synthetic jet actuator | |||
| Loudspeaker type | Aurasound NS3-193-8A | ||
| Nozzle diameter | Dn | 10.0 | (mm) |
| Diaphragm effective diameter | Dd | 60.8 | (mm) |
| Mass of air inside the nozzle volume | mnn | 0.92, 2.1, 3.0, 3.5, 4.1 | (mg) |
| Fluid: air | |||
| Ambient temperature | Ta | 24.2–26.6 | (°C) |
| Barometric pressure | pb | 98 000–98 900 | (Pa) |
| Density | ρ | 1.14–1.15 | (kg/m3) |
| Forcing | |||
| Input real power | Pe | 0.13, 0.20, 0.30, 0.45, 0.68, 1.02, 1.53, and 2.30 | (W) |
| Actuating frequency | f | 55–65 | (Hz) |
| Nozzle A | 55 Hz |
| Nozzle B | 65 Hz |
| Nozzle AB | 60 Hz |
| Nozzle ABC | 55 Hz |
| Nozzle ABCD | 55 Hz |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kordík, J.; Trávníček, Z. Novel Nozzle Shapes for Synthetic Jet Actuators Intended to Enhance Jet Momentum Flux. Actuators 2018, 7, 53. https://doi.org/10.3390/act7030053
Kordík J, Trávníček Z. Novel Nozzle Shapes for Synthetic Jet Actuators Intended to Enhance Jet Momentum Flux. Actuators. 2018; 7(3):53. https://doi.org/10.3390/act7030053
Chicago/Turabian StyleKordík, Jozef, and Zdeněk Trávníček. 2018. "Novel Nozzle Shapes for Synthetic Jet Actuators Intended to Enhance Jet Momentum Flux" Actuators 7, no. 3: 53. https://doi.org/10.3390/act7030053
APA StyleKordík, J., & Trávníček, Z. (2018). Novel Nozzle Shapes for Synthetic Jet Actuators Intended to Enhance Jet Momentum Flux. Actuators, 7(3), 53. https://doi.org/10.3390/act7030053





