A V-Shaped Actuator Utilizing Electrostatic Force
Abstract
:1. Introduction
2. Materials and Methods
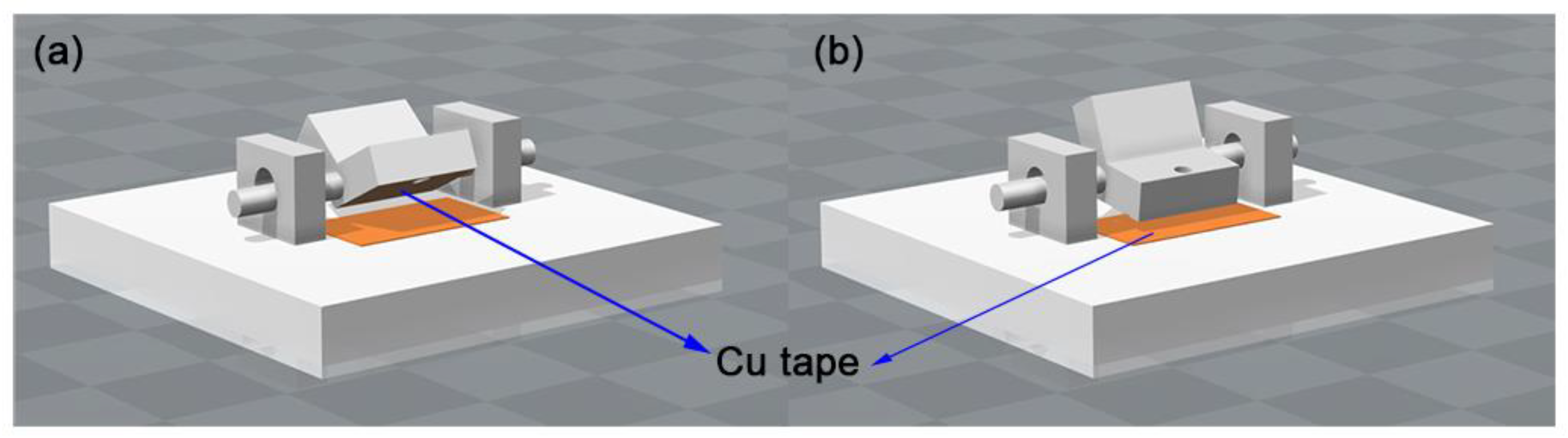
2.1. Model Fabrication by 3D Printing
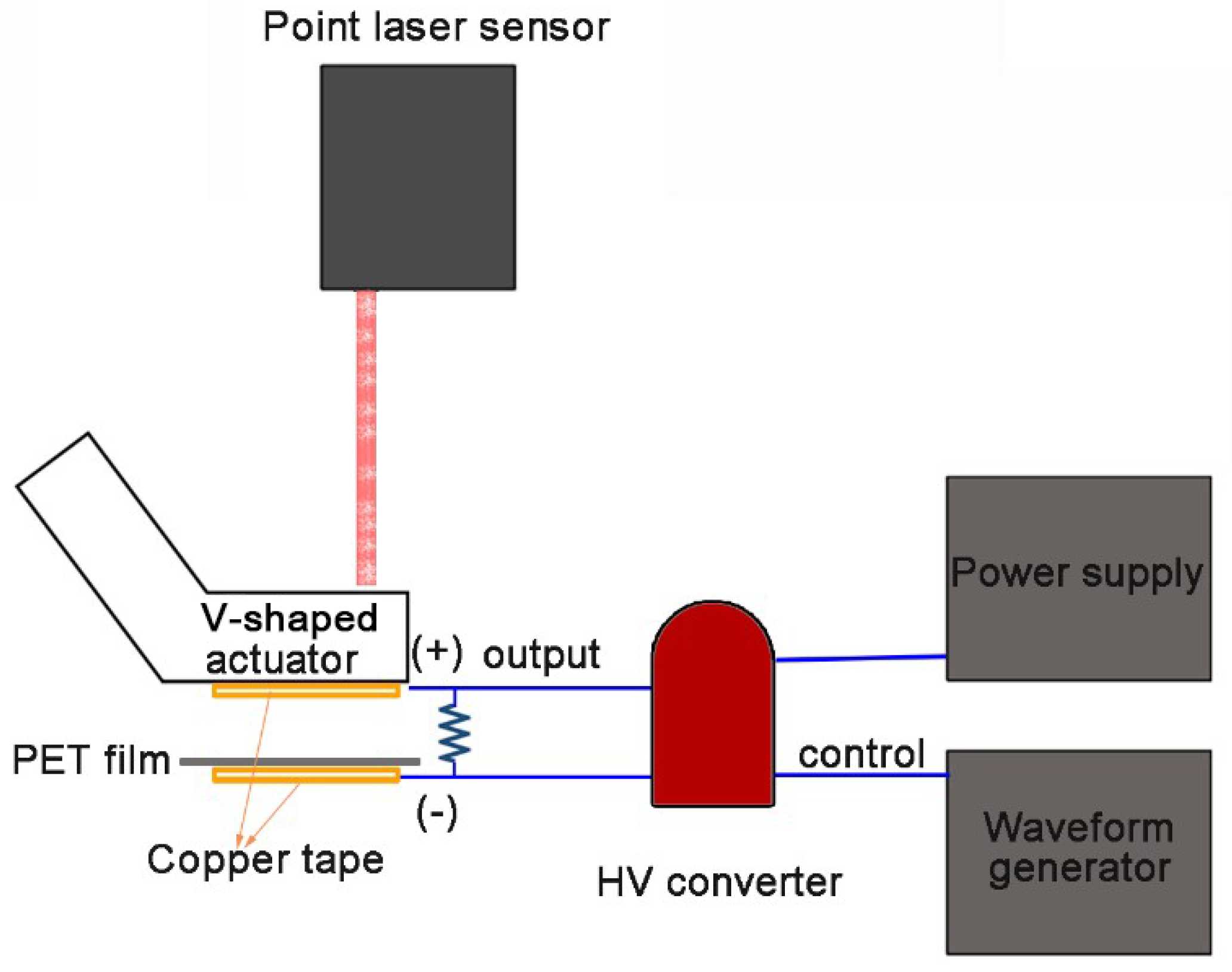
2.2. Circuit Configuration and Set Up
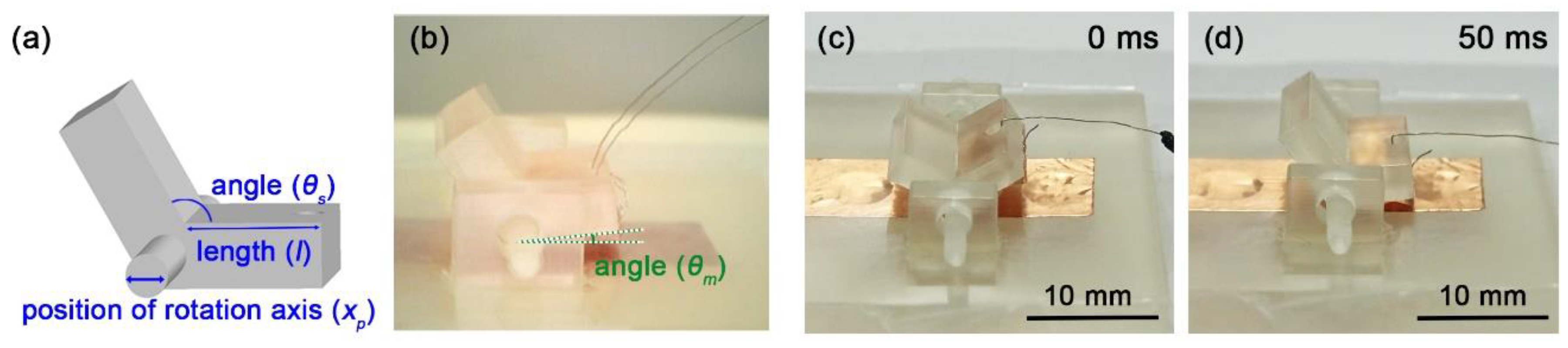
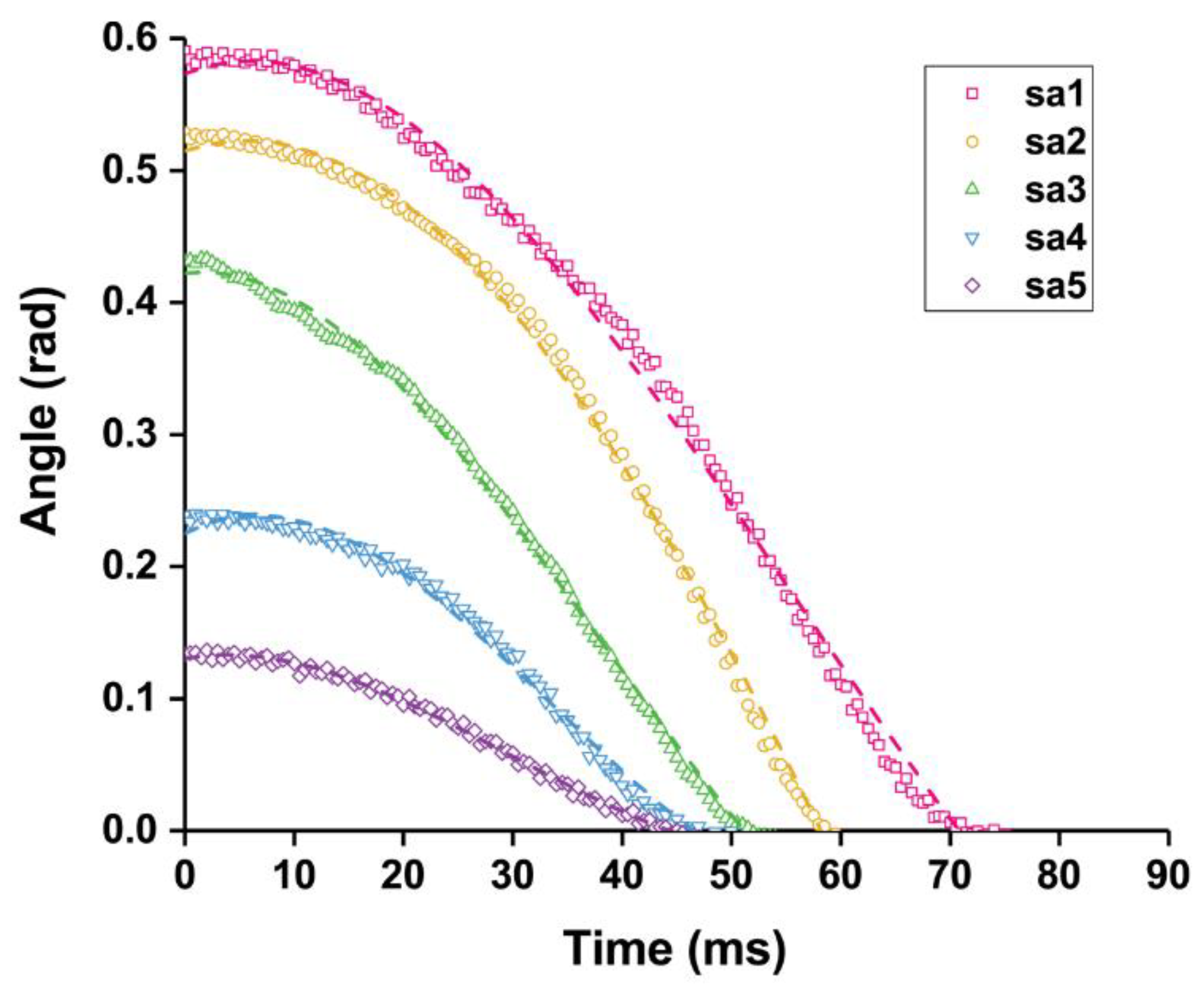
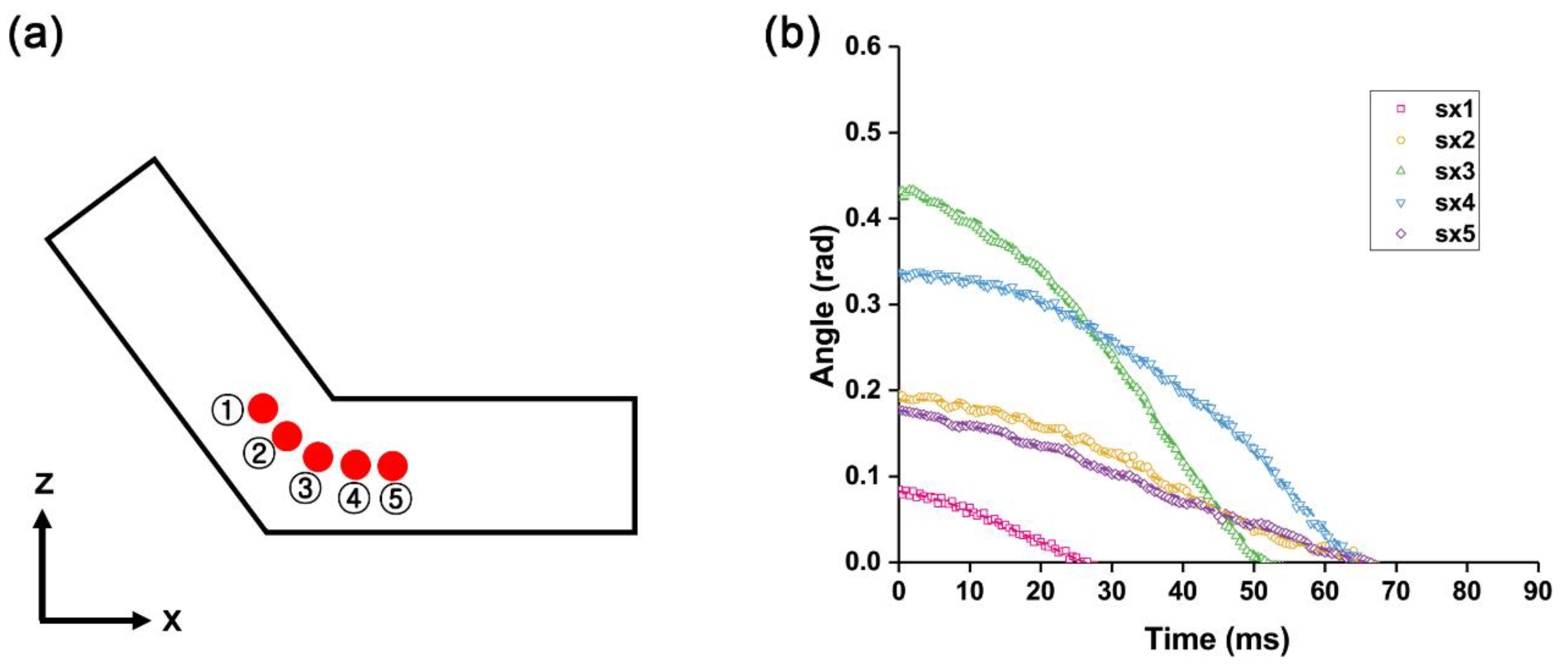
2.3. Motion Tracing and Angle Measurement
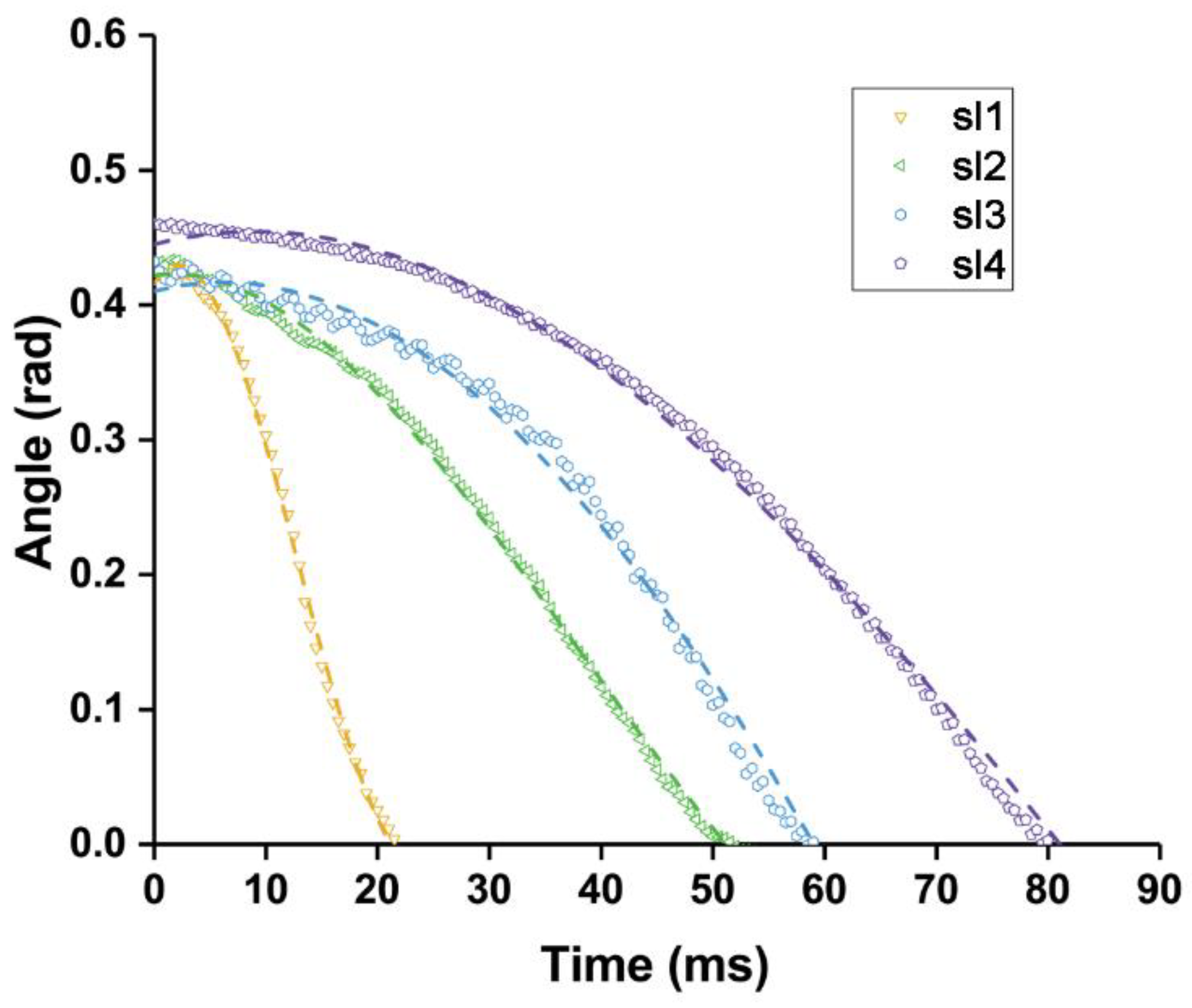
3. Results
4. Discussion
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Zupan, M.; Ashby, M.F.; Fleck, N.A. Actuator classification and selection—The development of a database. Adv. Eng. Mater. 2002, 4, 933–940. [Google Scholar] [CrossRef]
- Jones, L.A.; Held, D.A. Characterization of tactors used in vibrotactile displays. J. Comput. Inf. Sci. Eng. 2008, 8, 044501. [Google Scholar] [CrossRef]
- Yao, H.-Y.; Hayward, V. Design and analysis of a recoil-type vibrotactile transducer. J. Acoust. Soc. Am. 2010, 128, 619–627. [Google Scholar] [CrossRef] [PubMed]
- Velazquez, R.; Pissaloux, E.; Hafez, M.; Szewczyk, J. A low-cost highly-portable tactile display based on shape memory alloy micro-actuators. In Proceedings of the IEEE International Conference on Virtual Environments, Human-Computer Interfaces, and Measurement Systems, Giardini Naxos, Italy, 18–20 July 2005; IEEE: Giardini Naxos, Italy, 2005; p. 6. [Google Scholar]
- Koo, I.M.; Jung, K.; Koo, J.C.; Nam, J.-D.; Lee, Y.K.; Choi, H.R. Development of soft-actuator-based wearable tactile display. IEEE Trans. Robot. 2008, 24, 549–558. [Google Scholar] [CrossRef]
- Park, S.; Abdel-Rahman, E. Low voltage electrostatic actuation and displacement measurement through resonant drive circuit. In Proceedings of the ASME 2012 International Design Engineering Technical Conferences and Computers and Information in Engineering Conferences, Chicago, IL, USA, 12–15 August 2012; American Society of Mechanical Engineers: Chicago, IL, USA, 2012; pp. 119–126. [Google Scholar]
- Velosa-Moncada, L.A.; Aguilera-Cortés, L.A.; González-Palacios, M.A.; Raskin, J.-P.; Herrera-May, A.L. Design of a novel MEMS microgripper with rotatory electrostatic comb-drive actuators for biomedical applications. Sensors 2018, 18, 1664. [Google Scholar] [CrossRef] [PubMed]
- Masurkar, N.; Jamil, K.; Arava, L.M.R. Environmental effects on the polypyrrole tri-layer actuator. Actuators 2017, 6, 17. [Google Scholar] [CrossRef]
- Scott, J.; Gray, R. A comparison of tactile, visual, and auditory warnings for rear-end collision prevention in simulated driving. Hum. Factors 2008, 50, 264–275. [Google Scholar] [CrossRef] [PubMed]
- Koskinen, E.; Kaaresoja, T.; Laitinen, P. Feel-good touch: Finding the most pleasant tactile feedback for a mobile touch screen button. In Proceedings of the 10th International Conference on Multimodal Interfaces, 20–22 October 2008; ACM: New York, NY, USA, 2008; pp. 297–304. [Google Scholar]
- Lee, M.H.; Nicholls, H.R. Review article tactile sensing for mechatronics—A state of the art survey. Mechatronics 1999, 9, 1–31. [Google Scholar] [CrossRef]
- Eltaib, M.; Hewit, J. Tactile sensing technology for minimal access surgery—A review. Mechatronics 2003, 13, 1163–1177. [Google Scholar] [CrossRef]
- Shin, B.; Ha, J.; Lee, M.; Park, K.; Park, G.H.; Choi, T.H.; Cho, K.-J.; Kim, H.-Y. Hygrobot: A self-locomotive ratcheted actuator powered by environmental humidity. Sci. Robot. 2018, 3, eaar2629. [Google Scholar] [CrossRef]
- Jones, L.A. Perspectives on the evolution of tactile, haptic, and thermal displays. Presence (Camb.) 2016, 25, 247–252. [Google Scholar] [CrossRef]
- McGrath, B.; McKinley, A.; Duistermaat, M.; Carlander, O.; Brill, C.; Zets, G.; Van Erp, J. Tactile actuator technology. In Tactile Displays for Orientation, Navigation and Communication in Air, Sea and Land Environments; National Technical Information Service: Springfield, VA, USA, 2008; Volume 4. [Google Scholar]
- Bargatin, I.; Myers, E.; Aldridge, J.; Marcoux, C.; Brianceau, P.; Duraffourg, L.; Colinet, E.; Hentz, S.; Andreucci, P.; Roukes, M. Large-scale integration of nanoelectromechanical systems for gas sensing applications. Nano Lett. 2012, 12, 1269–1274. [Google Scholar] [CrossRef] [PubMed]
- Horsley, D.A.; Wongkomet, N.; Horowitz, R.; Pisano, A.P. Precision positioning using a microfabricated electrostatic actuator. IEEE Trans. Magn. 1999, 35, 993–999. [Google Scholar] [CrossRef]
- Liu, C.-C. Dynamic behavior analysis of cantilever-type nano-mechanical electrostatic actuator. Int. J. Non-Linear Mech. 2016, 82, 124–130. [Google Scholar] [CrossRef]
- Schenk, H.; Conrad, H.; Gaudet, M.; Uhlig, S.; Kaiser, B.; Langa, S.; Stolz, M.; Schimmanz, K. A novel electrostatic micro-actuator class and its application potential for optical mems. In Proceedings of the Optical MEMS and Anophotonics (OMN), Singapore, 31 July–4 August 2016; IEEE: Singapore, 2016; pp. 1–2. [Google Scholar]
- Rutherglen, C.; Jain, D.; Burke, P. Nanotube electronics for radiofrequency applications. Nat. Nanotechnol. 2009, 4, 811–819. [Google Scholar] [CrossRef] [PubMed]
- Van Der Wijngaart, W.; Ask, H.; Enoksson, P.; Stemme, G. A high-stroke, high-pressure electrostatic actuator for valve applications. Sens. Actuators A Phys. 2002, 100, 264–271. [Google Scholar] [CrossRef]
- Tsuchiya, T.; Hemmi, T.; Suzuki, J.-Y.; Hirai, Y.; Tabata, O. Tensile strength of silicon nanowires batch-fabricated into electrostatic MEMS testing device. Appl. Sci. 2018, 8, 880. [Google Scholar] [CrossRef]
- Yamaguchi, M.; Kawamura, S.; Minami, K.; Esashi, M. Distributed electrostatic micro actuator. In Proceedings of the An Investigation of Micro Structures, Sensors, Actuators, Machines and Systems, Fort Lauderdale, FL, USA, USA, 10 February 1993; IEEE: Fort Lauderdale, FL, USA, 1993; pp. 18–23. [Google Scholar]
- Agarwal, A.K.; Nammi, K.; Kaczmarek, K.A.; Tyler, M.E.; Beebe, D.J. A hybrid natural/artificial electrostatic actuator for tactile stimulation. In Proceedings of the 2nd Annual International IEEE-EMB Special Topic Conference on Microtechnologies in Medicine & Biology, Madison, WI, USA, 2–4 May 2002; IEEE: Madison, WI, USA, 2002; pp. 341–345. [Google Scholar]
- Meyer, D.J.; Peshkin, M.A.; Colgate, J.E. Fingertip friction modulation due to electrostatic attraction. In Proceedings of the World Haptics Conference, Daejeon, Korea, 14–17 April 2013; IEEE: Daejeon, Korea, 2013; pp. 43–48. [Google Scholar]
- Jungmann, M.; Schlaak, H.F. Miniaturised electrostatic tactile display with high structural compliance. In Proceedings of the Conference Eurohaptics, 15–17 October 2002, Edinburgh, UK.
- Song, K.; Cha, Y. Fe3O4–silicone mixture as flexible actuator. Materials 2018, 11, 753. [Google Scholar] [CrossRef] [PubMed]
- Crowley, J.M. Electrostatic Fundamentals; Marcel Dekker Inc.: New York, NY, USA, 1995. [Google Scholar]
- Tao, K.; Lye, S.W.; Miao, J.; Tang, L.; Hu, X. Out-of-plane electret-based mems energy harvester with the combined nonlinear effect from electrostatic force and a mechanical elastic stopper. J. Micromech. Microeng. 2015, 25, 104014. [Google Scholar] [CrossRef]
- Chiu, Y.; Lee, Y.-C. Flat and robust out-of-plane vibrational electret energy harvester. J. Micromech. Microeng. 2012, 23, 015012. [Google Scholar] [CrossRef]
- Tao, K.; Tang, L.; Wu, J.; Lye, S.W.; Chang, H.; Miao, J. Investigation of multimodal electret-based mems energy harvester with impact-induced nonlinearity. J. Microelectromech. Syst. 2018, 27, 276–288. [Google Scholar] [CrossRef]
- Simoneau, M.; Corbeil, P. The effect of time to peak ankle torque on balance stability boundary: Experimental validation of a biomechanical model. Exp. Brain Res. 2005, 165, 217–228. [Google Scholar] [CrossRef] [PubMed]
- Pilkey, W.D.; Pilkey, W.D. Formulas for Stress, Strain, and Structural Matrices; John Wiley & Sons: New York, NY, USA, 1994. [Google Scholar]
- Luukkonen, T. Modelling and Control of Quadcopter; Aalto University: Espoo, Finland, 2011; Volume 22. [Google Scholar]
- Tay, F.; Jun, X.; Liang, Y.; Logeeswaran, V.; Yufeng, Y. The effects of non-parallel plates in a differential capacitive microaccelerometer. J. Micromech. Microeng. 1999, 9, 283. [Google Scholar] [CrossRef]
- Ak, C.; Yildiz, A. A new analytical model to estimate the voltage value and position of the pull-in limit of a mems cantilever. Micromachines 2016, 7, 53. [Google Scholar] [CrossRef]
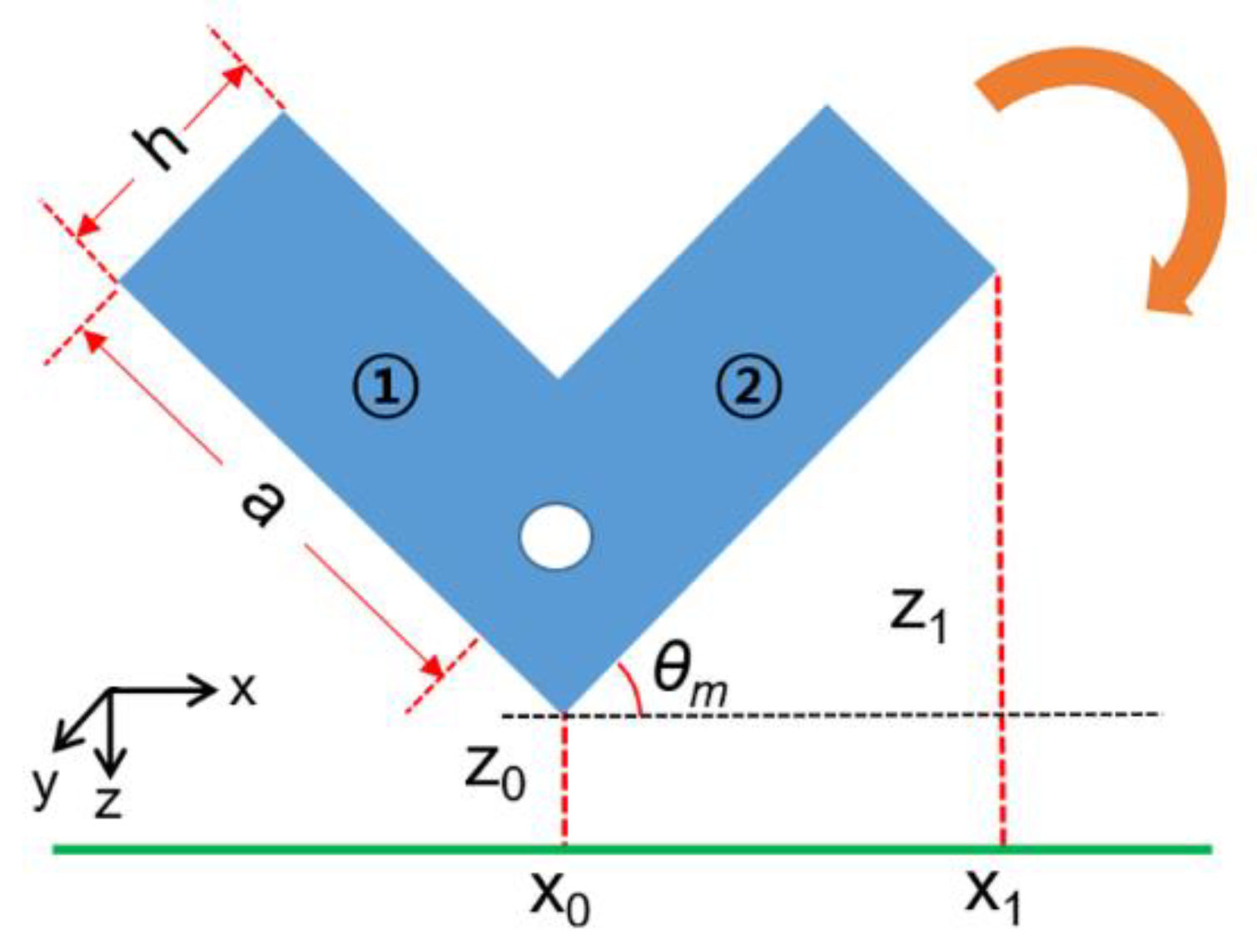

| Symbol | Definition | Value |
|---|---|---|
| ε0 | Electric permittivity of free space | 8.85 × 10−12 F/m |
| ΔV | Voltage difference | 5 kV |
| z0 | Gap between the bottom and the V-shaped actuator | 1 × 10−3 m |
| a | Length of panel | 5 × 10−3 m |
| mt | Mass of panel | 0.43 × 10−3 kg |
| g | Gravitational force | 9.80 m/s2 |
| h | Thickness of panel | 3 × 10−3 m |
| dcom | Distance between the center of mass and position of rotational axis in x-direction | 1.43 × 10−3 m |
| θs | Angle between two panel | 2.09 rad |
| x1 | The orthogonal length of the electrostatic attraction | 7 × 10−3 m |
| y1 | Width of panel in y-axis | 10 × 10−3 m |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, K.; Lee, H.; Cha, Y. A V-Shaped Actuator Utilizing Electrostatic Force. Actuators 2018, 7, 30. https://doi.org/10.3390/act7020030
Song K, Lee H, Cha Y. A V-Shaped Actuator Utilizing Electrostatic Force. Actuators. 2018; 7(2):30. https://doi.org/10.3390/act7020030
Chicago/Turabian StyleSong, Kahye, Hyeongyu Lee, and Youngsu Cha. 2018. "A V-Shaped Actuator Utilizing Electrostatic Force" Actuators 7, no. 2: 30. https://doi.org/10.3390/act7020030
APA StyleSong, K., Lee, H., & Cha, Y. (2018). A V-Shaped Actuator Utilizing Electrostatic Force. Actuators, 7(2), 30. https://doi.org/10.3390/act7020030






