Concept of a Series-Parallel Elastic Actuator for a Powered Transtibial Prosthesis
Abstract
:1. Introduction
2. Stiff Set-Up or Servomotor
2.1. Reference Data of Winter

2.2. Motor Selection in Stiff Set-Up
3. SEA Set-Up
3.1. SEA Agonistic Drum-Drum Set-Up
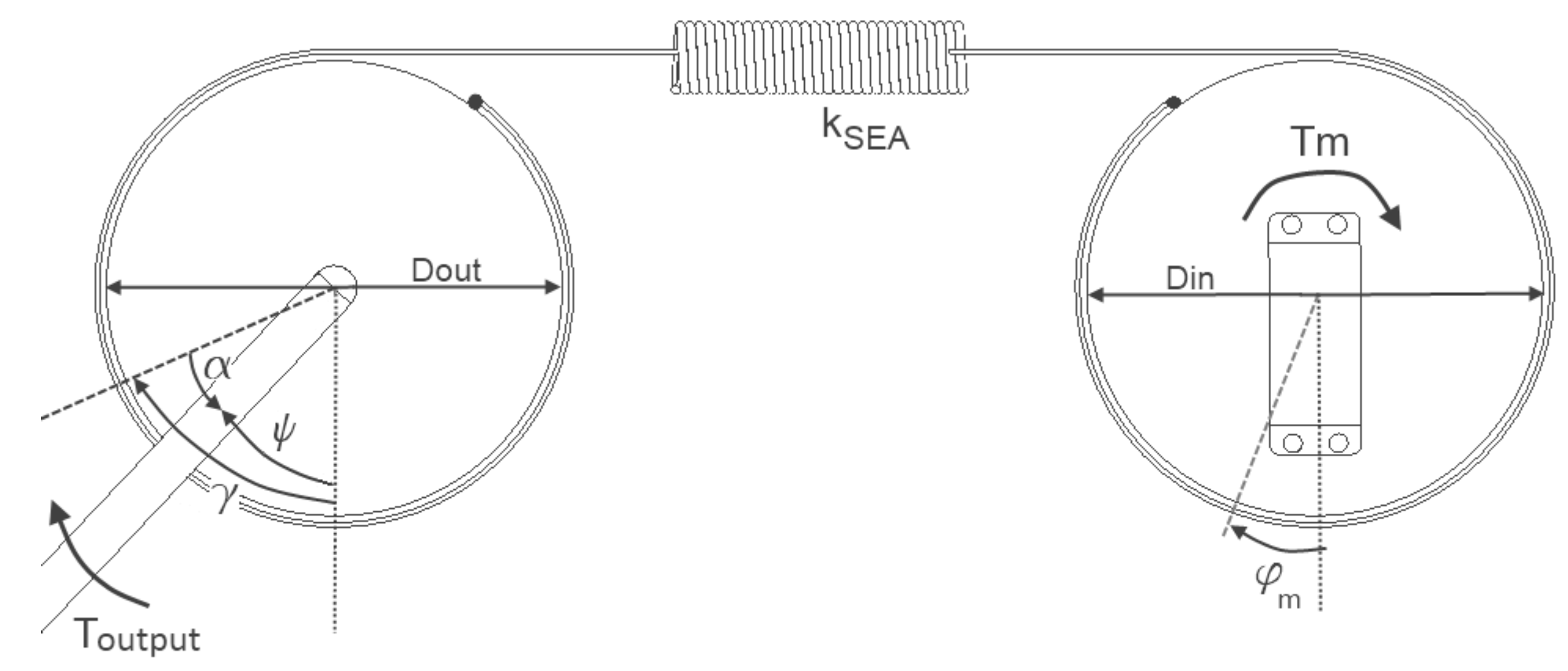

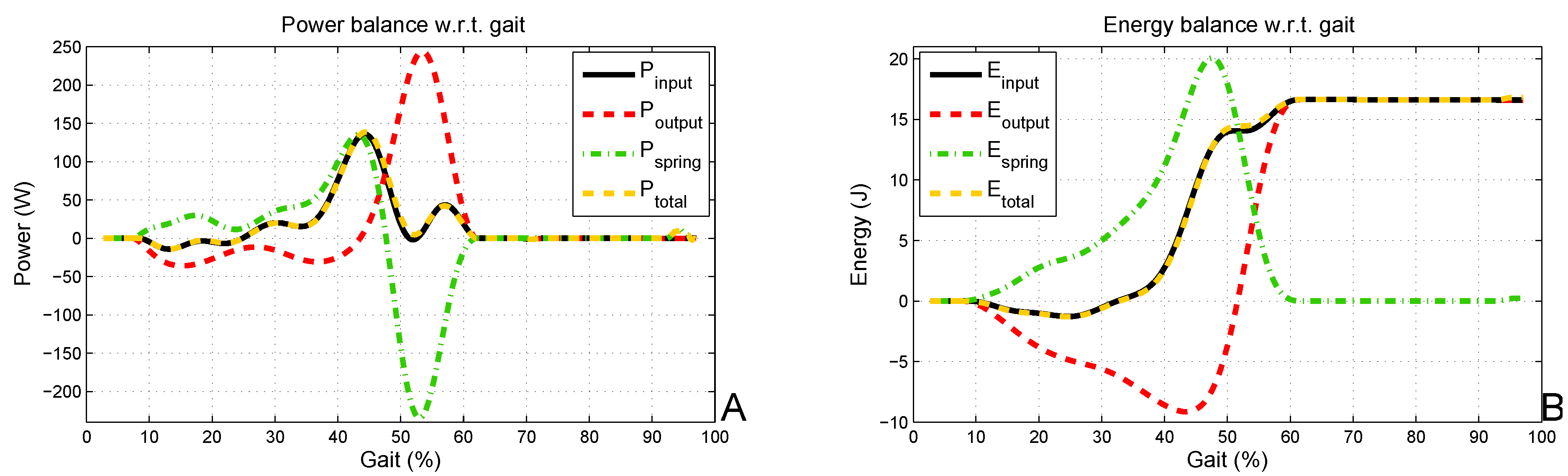
3.2. Motor Selection in SEA Setup
4. SPEA Set-Up
4.1. SPEA Agonistic Drum-Drum Set-Up
- The unpretensioned phase: the intermittent mechanism is locked, the spring is at its rest length and fixed to the ground link and the output link; all forces that are exerted will not pass through the motor, since it is not present in the force path (shaded motor);
- The pretensioned phase: the intermittent mechanism is locked, the spring is fully extended and fixed to the ground link and the output link; all forces that are exerted will not pass through the motor, since it is not present in the force path (shaded motor);
- The pretensioning phase: the intermittent mechanism is unlocked, the motor controls the length of the spring and brings it from the unpretensioned phase to the pretensioned phase or vice versa (solid black motor).

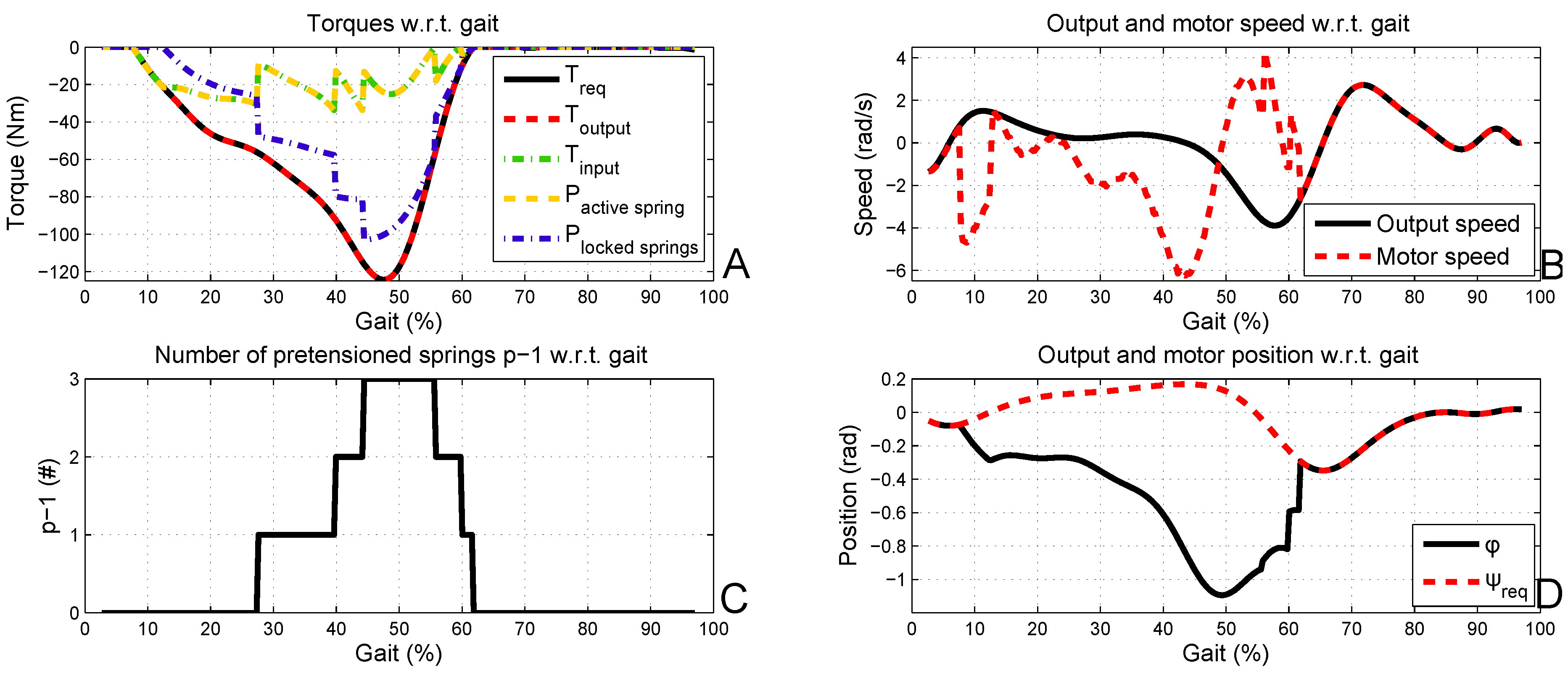
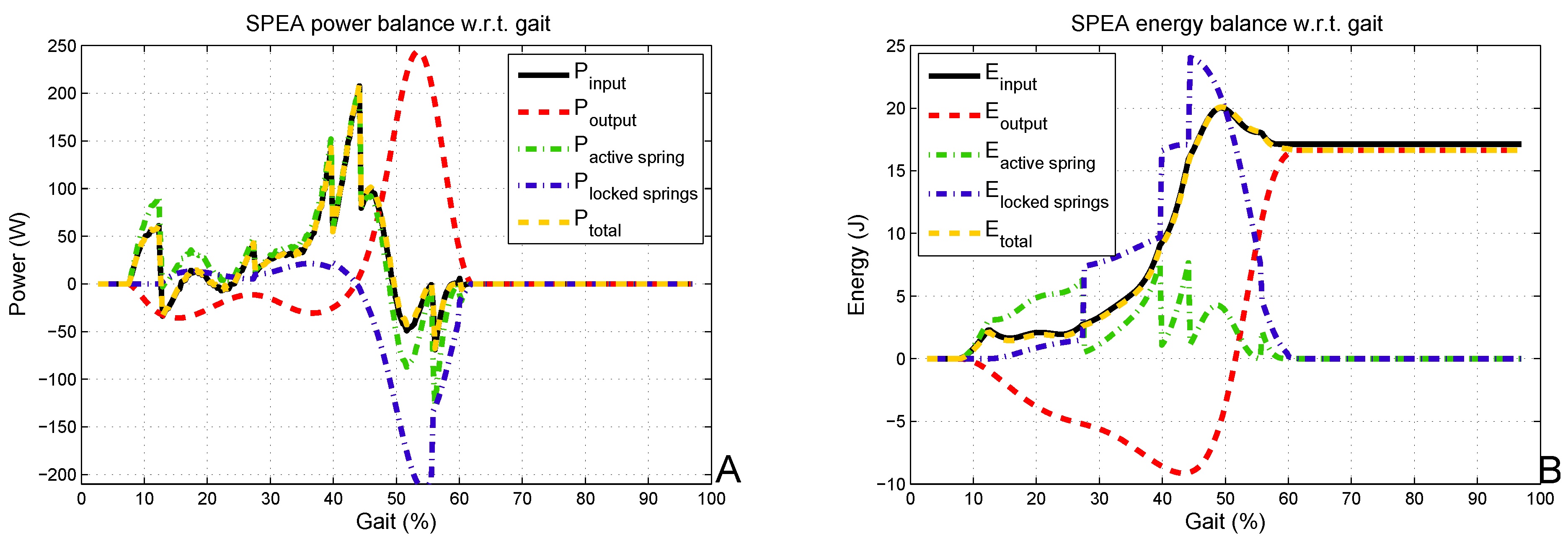
4.2. Motor Selection in SPEA Setup
5. Discussion
| Req | Maxon Motor | Maxon Gear | # | Total | +Custom Gear | +Extra | |
|---|---|---|---|---|---|---|---|
| Stiff | 250 W | EC-4pole 45 | GP42C 24:1 | 2 | ≈ | 4:1 | |
| 125 Nm | 300 W & 1.13 kg | 0.40 kg | 3.0kg | ||||
| 43 rpm | Nom: 0.635 Nm | 15 Nm | 60 Nm | ||||
| Nom: 3580 rpm | 150 rpm | 37.5 rpm | |||||
| SEA | 95 W | EC-4pole 30 | GP 42C 235:1 | 2 | ≈ | 4:1 | 1 spring |
| 125 Nm | 100 W & 0.21 kg | 0.56 kg | 1.6kg | ||||
| 23 rpm | Nom: 0.064 Nm | 15 Nm | 60 Nm | ||||
| Nom: 16,700 rpm | 71 rpm | 17.8 rpm | |||||
| SPEA | 208 W | EC-4pole 30 | GP 42C 142:1 | 1 | ≈ | 2:1 | 4 springs |
| 32 Nm | 200 W & 0.3 kg | 0.460 kg | 0.8kg | ||||
| 61 rpm | Nom: 0.112 Nm | 15 Nm | 30 Nm | Intermittent | |||
| Nom: 16,200 rpm | 114 rpm | 57 rpm | mechanism |
6. Conclusions
Acknowledgments
Conflict of Interest
References
- Winter, D. Biomechanics of Human Movement; John Wiley & Sons: Toronto, Canada, 1979. [Google Scholar]
- Rao, S.; Boyd, L.; Mulroy, S.; Bontrager, E.; Gronley, J.; Perry, J. Segment velocities in normal and transtibial amputees: Prosthetic design implications. IEEE Trans. Rehabil. Eng. 1998, 6, 219–226. [Google Scholar] [CrossRef] [PubMed]
- Perry, J.; Boyd, L.; Rao, S.; Mulroy, S. Prosthetic weight acceptance mechanics in transtibial amputees wearing the single axis, seattle lite, and flex foot. IEEE Trans. Rehabil. Eng. 1997, 5, 283–289. [Google Scholar] [CrossRef] [PubMed]
- Snyder, R.D.; Powers, C.M.; Fountain, C.; Perry, J. The effect of five prosthetic feet on the gait and loading of the sound limb in dysvascular below-knee amputees. J. Rehabil. Res. Dev. 1995, 32, 309–315. [Google Scholar] [PubMed]
- Pratt, G.A.; Williamson, M.M. Series Elastic Actuators. In Proceedings of IEEE/RSJ International Conference on Intelligent Robots and Systems 95, Human Robot Interaction and Cooperative Robots, Pittsburgh, PA, USA, 5–9 August 1995; Volume 1, pp. 399–406.
- Everarts, C.; Dehez, B.; Ronsse, R. Variable Stiffness Actuator Applied to an Active Ankle Prosthesis: Principle, Energy-efficiency, and Control. In Proceedings of 2012 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Vilamoura, Portugal, 7–12 October 2012; pp. 323–328.
- Hitt, J.; Merlo, J.; Johnston, J.; Holgate, M.; Boehler, A.; Hollander, K.; Sugar, T. Bionic Running for Unilateral Transtibial Military Amputees. Technical report, DTIC Document. 2010. [Google Scholar]
- Hollander, K.W.; Ilg, R.; Sugar, T.G.; Herring, D. An efficient robotic tendon for gait assistance. J. Biomechan. Eng. 2006, 128, 788–791. [Google Scholar] [CrossRef] [PubMed]
- Hitt, J.K.; Sugar, T.G.; Holgate, M.; Bellman, R. An active foot-ankle prosthesis with biomechanical energy regeneration. J. Med. Devices 2010, 4, 011003:1–011003:9. [Google Scholar] [CrossRef]
- Au, S.; Herr, H. Powered ankle-foot prosthesis. IEEE Robot. Autom. Mag. 2008, 15, 52–59. [Google Scholar] [CrossRef]
- Au, S.K.; Weber, J.; Herr, H. Powered ankle–foot prosthesis improves walking metabolic economy. IEEE Trans. Robot. 2009, 25, 51–66. [Google Scholar] [CrossRef]
- Versluys, R.; Desomer, A.; Lenaerts, G.; Pareit, O.; Vanderborght, B.; Perre, G.; Peeraer, L.; Lefeber, D. A biomechatronical transtibial prosthesis powered by pleated pneumatic artificial muscles. Int. J. Modell. Identif. Control 2008, 4, 394–405. [Google Scholar] [CrossRef]
- Brackx, B.; van Damme, M.; Matthys, A.; Vanderborght, B.; Lefeber, D. Passive ankle-foot prosthesis prototype with extended push-off. Int. J. Adv. Robot. Syst. 2013. [Google Scholar] [CrossRef]
- Cherelle, P.; Grosu, V.; Matthys, A.; Vanderborght, B.; Lefeber, D. Design and validation of the ankle mimicking prosthetic (AMP-) foot 2.0. IEEE Trans. Neural Syst. Rehabil. Eng. 2013, in press. [Google Scholar] [CrossRef] [PubMed]
- Marden, J.H. Scaling of maximum net force output by motors used for locomotion. J. Exp. Biol. 2005, 208, 1653–1664. [Google Scholar] [CrossRef] [PubMed]
- Caprari, G.; Estier, T.; Siegwart, R. Fascination of down scaling alice the sugar cube robot. J. Micromechatron. 2001, 1, 177–189. [Google Scholar] [CrossRef]
- Grimmer, M.; Eslamy, M.; Gliech, S.; Seyfarth, A. A Comparison of Parallel-and Series Elastic Elements in an Actuator for Mimicking Human Ankle Joint in Walking and Running. In Proceedings of 2012 IEEE International Conference on Robotics and Automation (ICRA), Saint Paul, MN, USA, 14–18 May 2012; pp. 2463–2470.
- Mettin, U.; La Hera, P.X.; Freidovich, L.B.; Shiriaev, A.S. Parallel elastic actuators as a control tool for preplanned trajectories of underactuated mechanical systems. Int. J. Robot. Res. 2010, 29, 1186–1198. [Google Scholar] [CrossRef]
- Haeufle, D.F.B.; Taylor, M.D.; Schmitt, S.; Geyer, H. A Clutched Parallel Elastic Actuator Concept: Towards Energy Efficient Powered Legs in Prosthetics and Robotics. In Proceedings of the 2012 4th IEEE RAS and EMBS International Conference on Biomedical Robotics and Biomechatronics (BioRob), Rome, Italy, 24–27 June 2012; pp. 1614–1619.
- Henneman, E. Relation between size of neurons and their susceptibility to discharge. Science 1957, 126, 1345–1347. [Google Scholar] [CrossRef] [PubMed]
- Mathijssen, G.; Brackx, B.; van Damme, M.; van Ham, R.; Lefeber, D.; Vanderborght, B. Novel Design of a Series-Parallel Elastic Actuator. In Proceedings of the Workshop IEEE International Conference on Robotics and Automation (ICRA), Karlsruhe, Germany, 6–10 May 2013.
- Mathijssen, G.; Lefeber, D.; Vanderborght, B. A novel compliant actuation concept: Series-Parallel Elastic Actuators (SPEA). IEEE/ASME Trans. Mechatron. 2013. under review. [Google Scholar]
- Tesar, D. Overview of the long term objectives of the journal actuators. Actuators 2012, 1, 1–11. [Google Scholar] [CrossRef]
- Vanderborght, B.; Verrelst, B.; Van Ham, R.; van Damme, M.; Beyl, P.; Lefeber, D. Development of a compliance controller to reduce energy consumption for bipedal robots. Auton. Robot. 2008, 24, 419–434. [Google Scholar] [CrossRef]
- Vanderborght, B.; Tsagarakis, N.G.; van Ham, R.; Thorson, I.; Caldwell, D.G. MACCEPA 2.0: Compliant actuator used for energy efficient hopping robot Chobino1D. Auton. Robot. 2011, 31, 55–65. [Google Scholar] [CrossRef]
- Wolf, S.; Hirzinger, G. A New Variable Stiffness Design: Matching Requirements of the Next Robot Generation. In Proceedings of the IEEE International Conference on Robotics and Automation, Pasadena, CA, USA, 19–23 May 2008; pp. 1741–1746.
- Bickford, J. Mechanisms for Intermittent Motion; Industrial Press: New York, NY, USA, 1972. [Google Scholar]
- Haddadin, S.; Mansfeld, N.; Albu-Schaffer, A. Rigid vs. Elastic Actuation: Requirements & Performance. In Proceedings of the 2012 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Vilamoura, Portugal, 7–12 October 2012; pp. 5097–5104.
© 2013 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Mathijssen, G.; Cherelle, P.; Lefeber, D.; Vanderborght, B. Concept of a Series-Parallel Elastic Actuator for a Powered Transtibial Prosthesis. Actuators 2013, 2, 59-73. https://doi.org/10.3390/act2030059
Mathijssen G, Cherelle P, Lefeber D, Vanderborght B. Concept of a Series-Parallel Elastic Actuator for a Powered Transtibial Prosthesis. Actuators. 2013; 2(3):59-73. https://doi.org/10.3390/act2030059
Chicago/Turabian StyleMathijssen, Glenn, Pierre Cherelle, Dirk Lefeber, and Bram Vanderborght. 2013. "Concept of a Series-Parallel Elastic Actuator for a Powered Transtibial Prosthesis" Actuators 2, no. 3: 59-73. https://doi.org/10.3390/act2030059
APA StyleMathijssen, G., Cherelle, P., Lefeber, D., & Vanderborght, B. (2013). Concept of a Series-Parallel Elastic Actuator for a Powered Transtibial Prosthesis. Actuators, 2(3), 59-73. https://doi.org/10.3390/act2030059





