Innovative Device to Control Self-Induced Instabilities Associated with the Swirling Flow from the Discharge Cone of Hydraulic Turbines
Abstract
1. Introduction
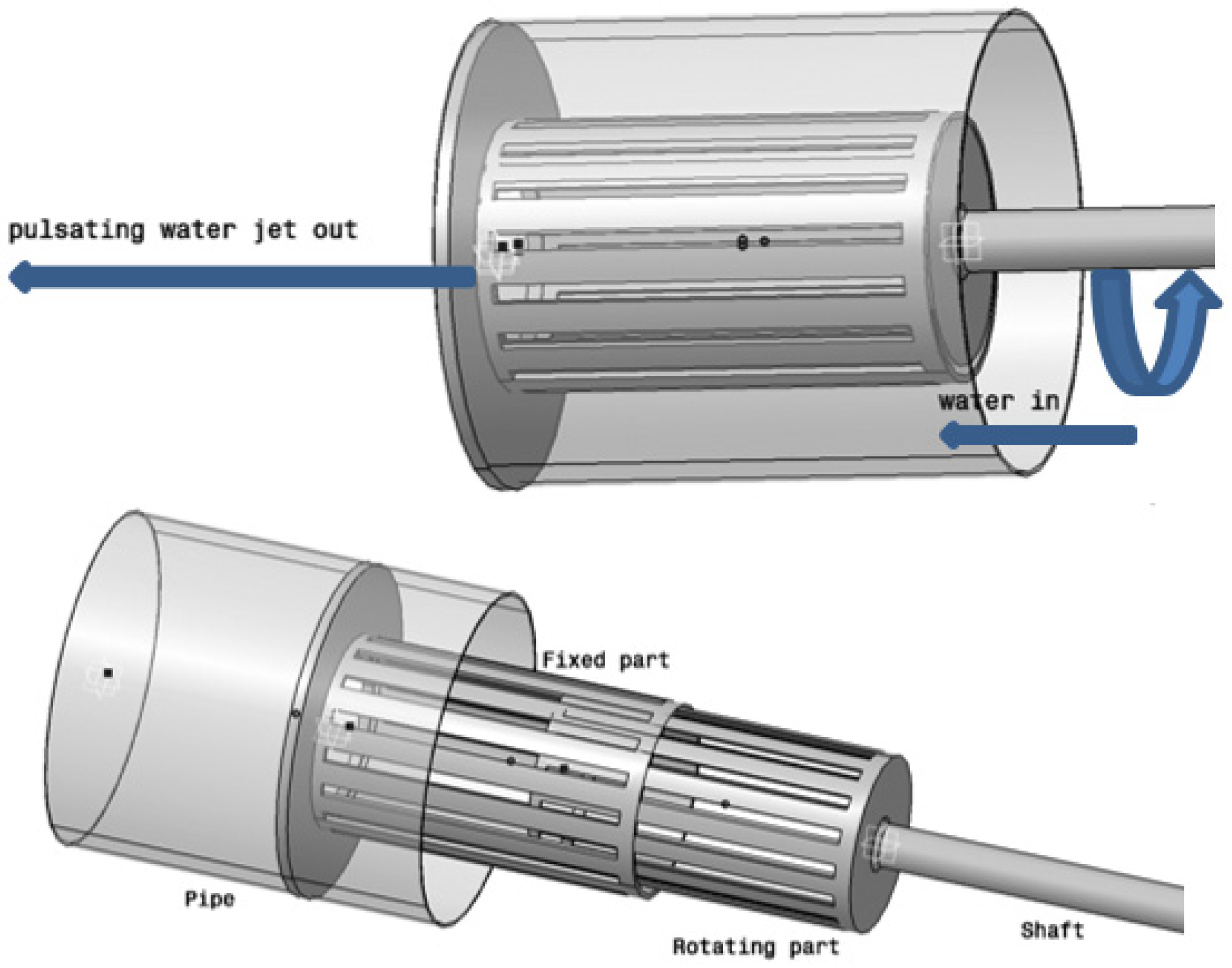
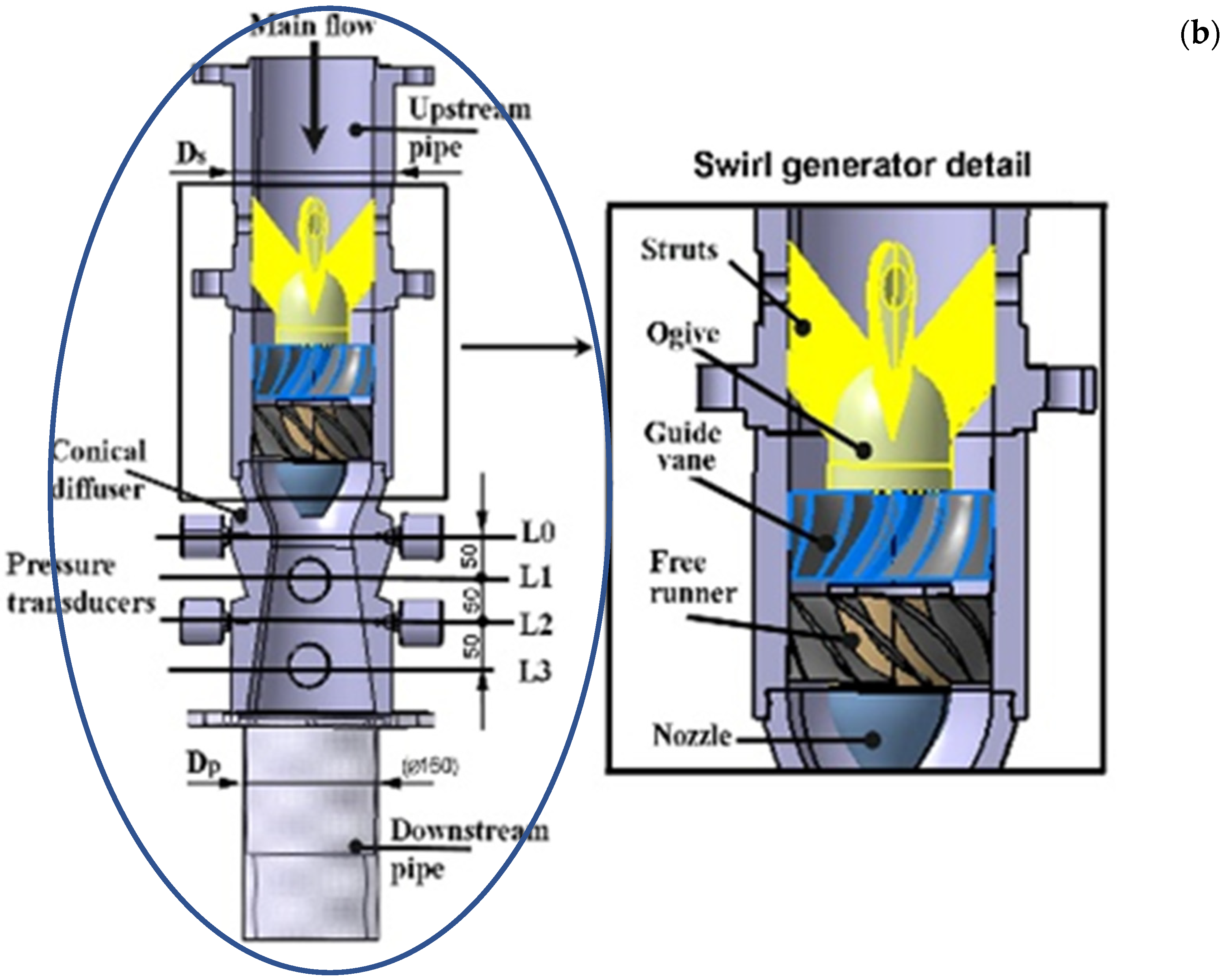
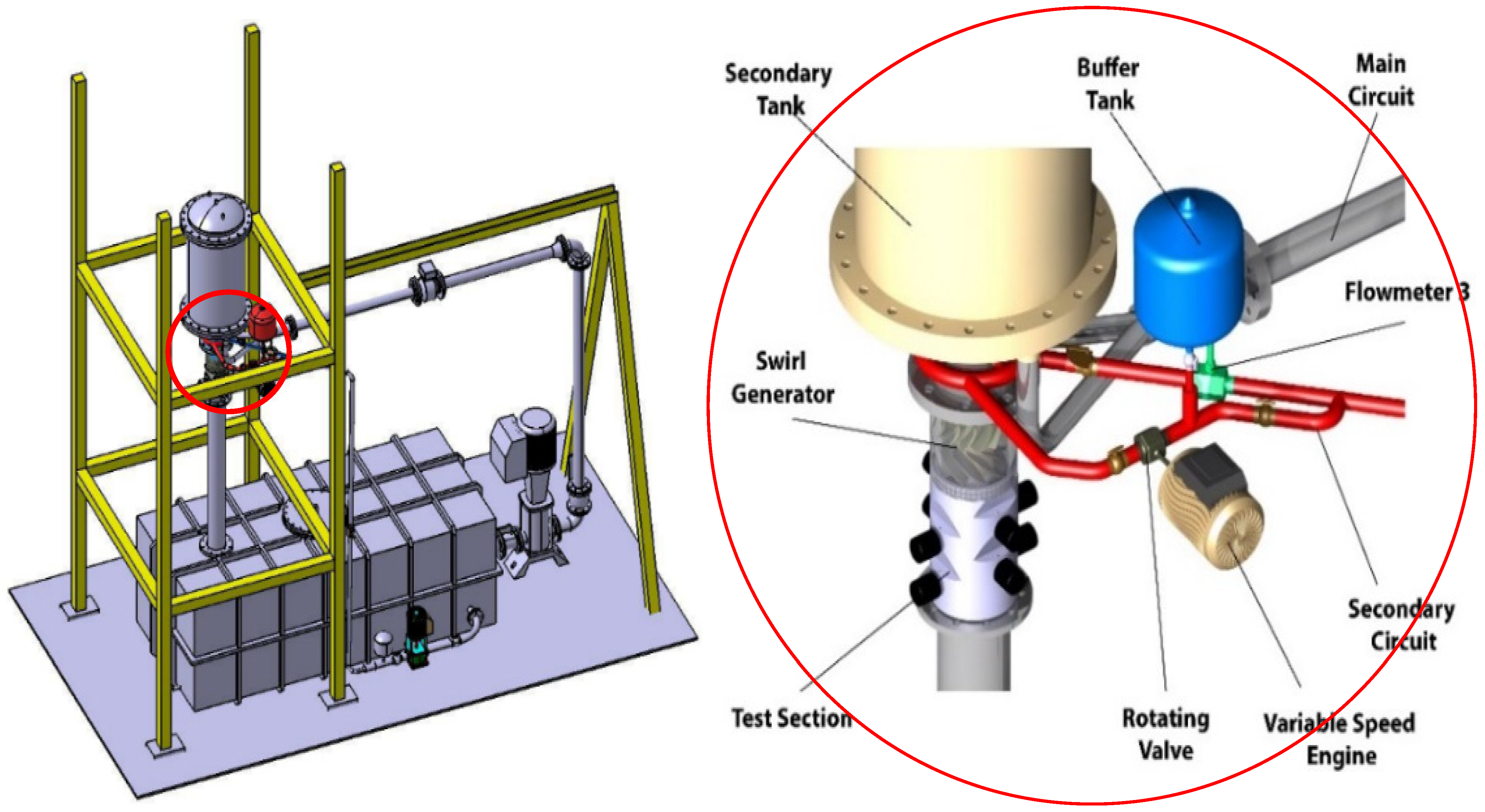
2. Materials and Methods
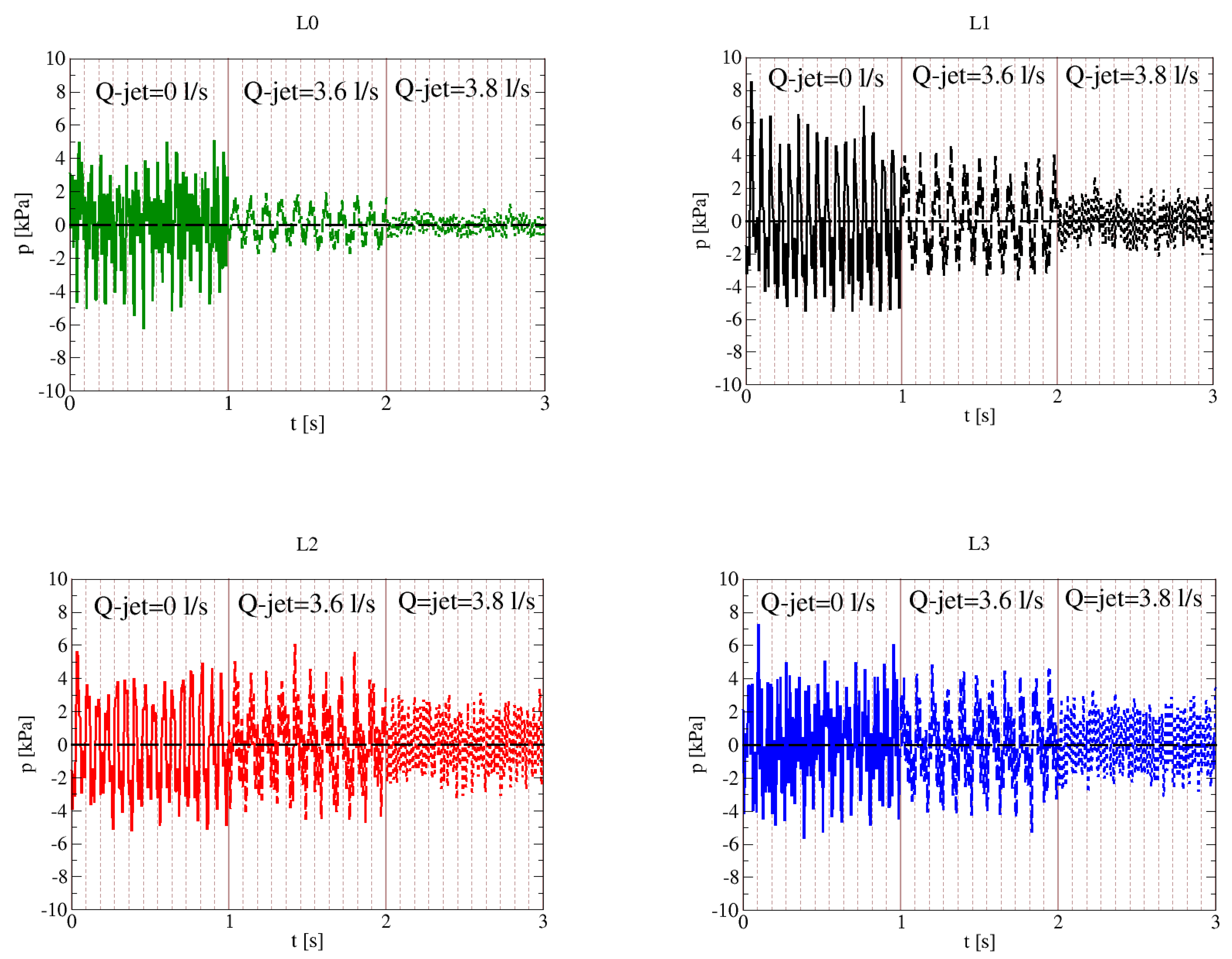
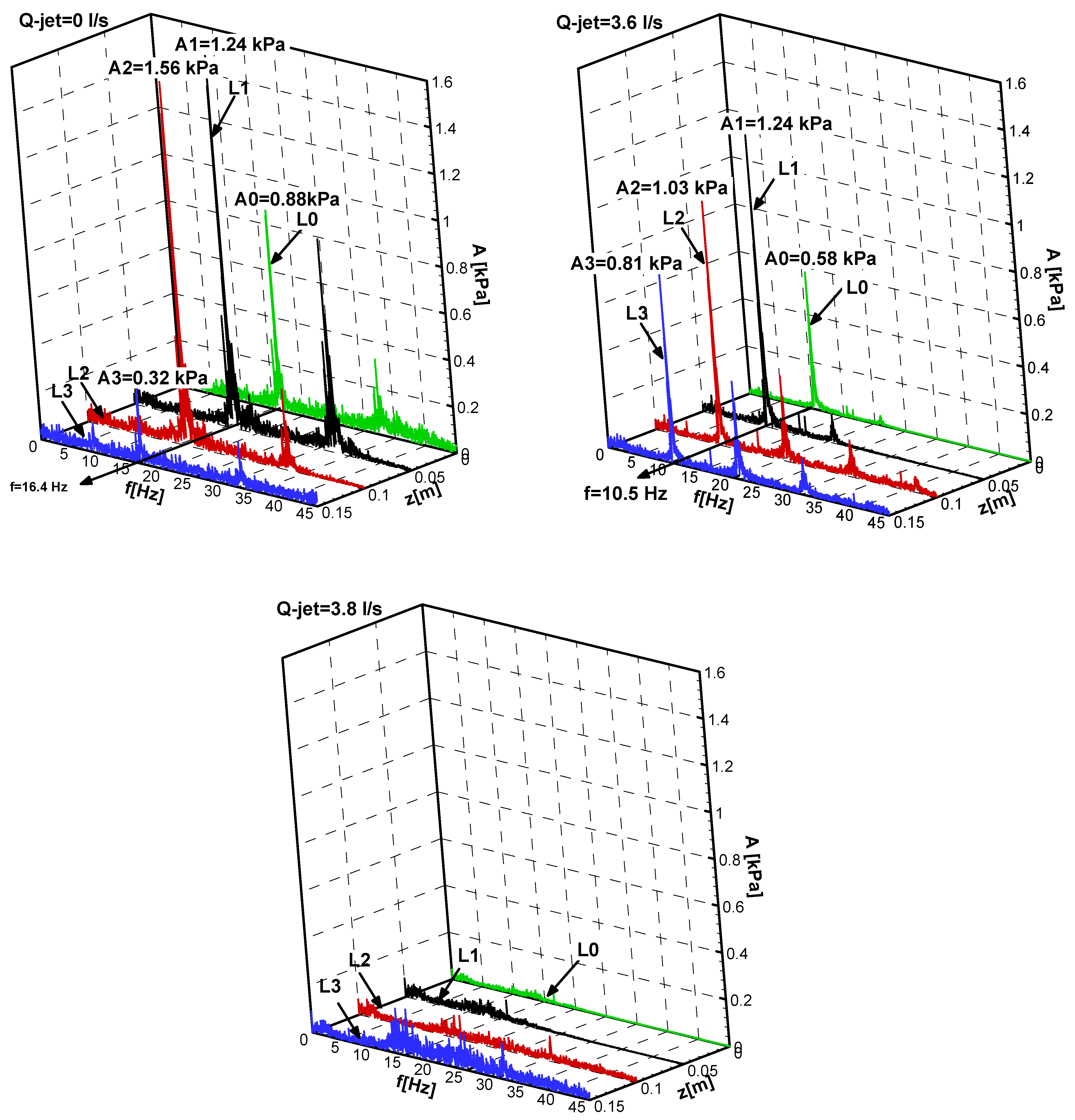
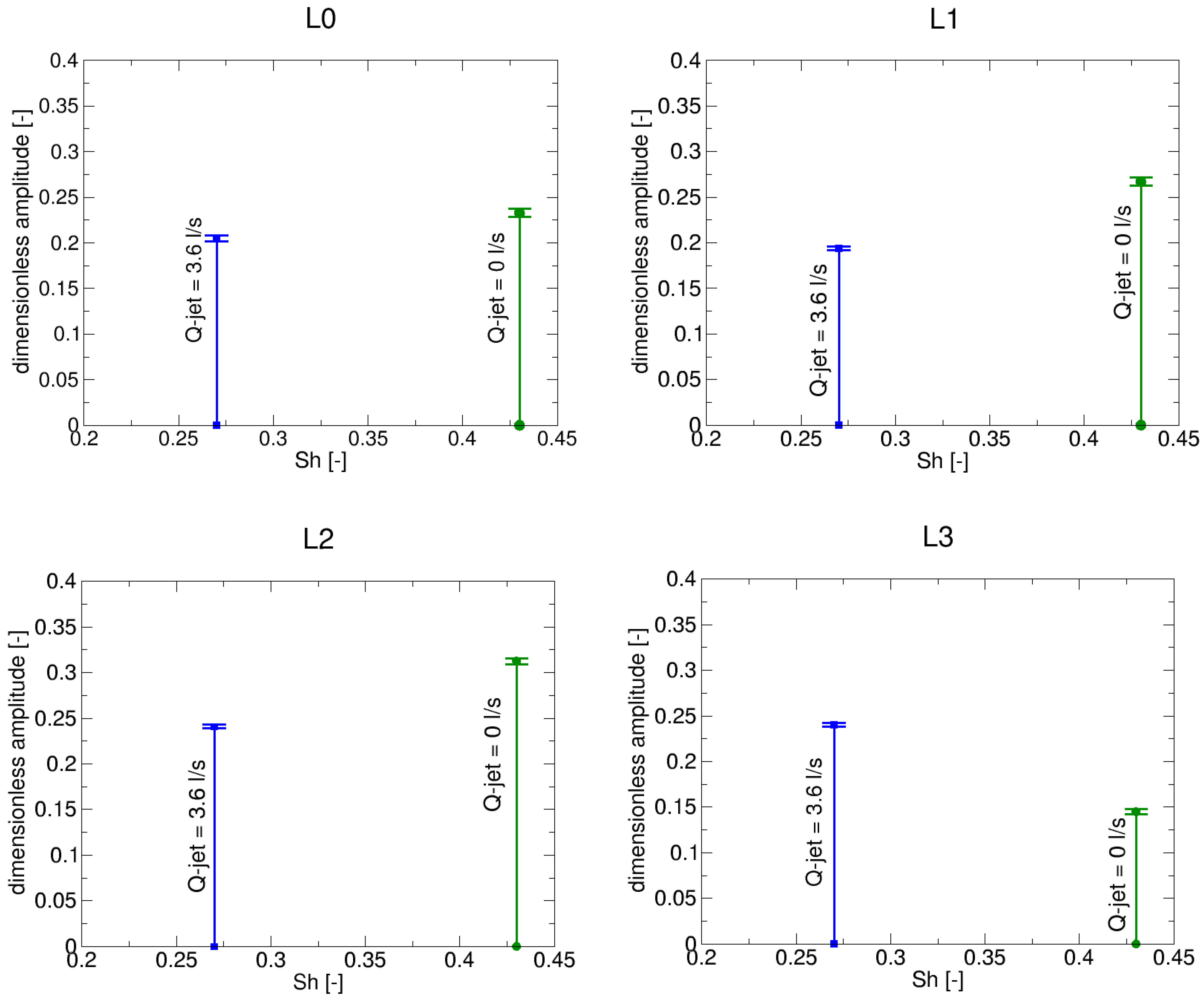
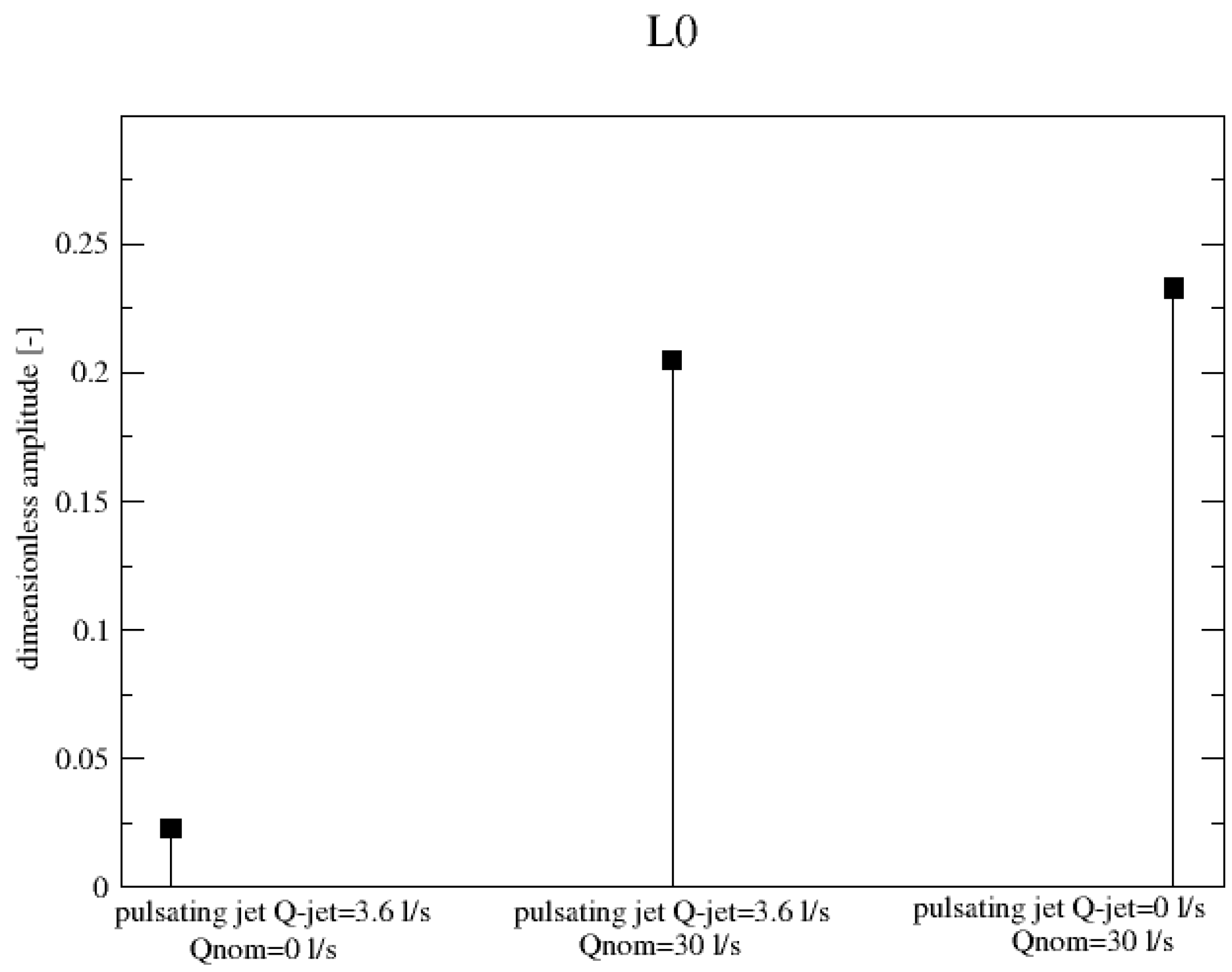
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| Dthroat | [m] | Diameter, corresponding to throat test section |
| [-] | Number of samples acquired for each point on the survey axis | |
| nom | [l/s] | Nominal discharge from the main hydraulic circuit |
| Q-jet | [l/s] | Pulsating jet discharge |
| Sh | [-] | Strouhal number |
| f | [Hz] | Frequency |
| Aeq | [-] | Equivalent amplitude |
| vthroat | [m/s] | Velocity from the throat of the test section |
| pRMS | [kPa] | Root mean square of the part of the fluctuating pressure signal |
| p | [kPa] | Pressure |
| VO | New device used to generate the pulsating jet | |
| q | [-] | Flow rate coefficient |
References
- March, P. Flexible Operation of Hydropower Plants; EPRI Document No. 3002011185; Electric Power Research Institute (EPRI): Palo Alto, CA, USA, 2017. [Google Scholar]
- Monette, C.; Marmont, H.; Chamberland-Lauzon, J.; Skagerstrand, A.; Coutu, A.; Carlevi, J. Cost of Enlarged Operating Zone for an Existing Francis Runner. In Proceedings of the 28th IAHR Symposium on Hydraulic Machinery and Systems, Grenoble, France, 4–8 July 2016; International Association for Hydro-Environment Engineering and Research: Beijing, China. [Google Scholar]
- Stark, G.; Dhulipala, S.C.; Brinkman, G. Storage Effectiveness in Enabling Variable Generation and Avoiding Fossil Emissions; NREL/TP-5700-86751; National Renewable Energy Laboratory: Golden, CO, USA, 2023. Available online: https://www.nrel.gov/docs/fy23osti/86751.pdf (accessed on 28 February 2025).
- U.S. Energy Information Administration. Electricity: Preliminary Monthly Electric Generator Inventory (Based on Form EIA-860M As a Supplement to Form EIA-860); EIA Independent Statistics and Analysis: Washington, DC, USA, 2023. Available online: https://www.eia.gov/electricity/data/eia860m/ (accessed on 28 February 2025).
- Litvinov, I.; Shtork, S.; Gorelikov, E.; Mitryakov, A.; Hanjalic, K. Unsteady regimes and pressure pulsations in draft tube of a model hydro turbine in a range of off-design conditions. Exp. Therm. Fluid Sci. 2018, 91, 410–422. [Google Scholar] [CrossRef]
- Nishi, M.; Matsunaga, S.; Kubota, T.; Senoo, Y. 1982, Flow Regimes in an Elbow-Type Draft Tube. In Proceedings of the 11th IAHR Symposium on Hydraulic Machinery and Systems, Amsterdam, The Netherlands, 13–17 September 1982; p. 38. [Google Scholar]
- Nishi, M.; Matsunaga, S.; Okamoto, M.; Uno, M.; Nishitani, K. Measurements of Three-Dimensional Periodic Flow in a Conical Draft Tube at Surging Condition, Flows in Non Rotating Turbomachinery Components. FED ASME 1988, 69, 81–88. [Google Scholar]
- Smith, W.J. Draft Tube Surges and Resonance in Generators and Pipelines; Canadian Electrical Association: Ottawa, ON, Canada, 1954. [Google Scholar]
- Momčilović, D.; Odanović, Z.; Mitrović, R.; Atanasovska, I.; Vuherer, T. Failure analysis of hydraulic turbine shaft. Eng. Fail. Anal. 2012, 20, 54–66. [Google Scholar] [CrossRef]
- Kurokawa, J.; Kajigaya, A.; Matusi, J.; Imamura, H. Suppression of Swirl in a Conical Diffuser by Use of J-Groove. In Proceedings of the 20th IAHR Symposium on Hydraulic Machinery and Systems, Charlotte, NC, USA, 6–9 August 2000; p. DY-01. [Google Scholar]
- Tanasa, C.; Susan-Resiga, R.F.; Muntean, S.; Bosioc, A.I. Flow-feedback method for mitigating the vortex rope in decelerated swirling flows. ASME J. Fluids Eng. 2013, 135, 061304. [Google Scholar] [CrossRef]
- Thicke, R.H. Practical solutions for draft tube instability. Water Power Dam Constr. 1981, 33, 31–37. [Google Scholar]
- Pappilon, B.; Sabourin, M.; Couston, M.; Deschenes, C. Methods for Air Admission in Hydro Turbines. In Proceedings of the XXIst IAHR Symposium on Hydraulic Machinery and Systems, Lausanne, Switzerland, 9–12 September 2002; pp. 1–6. [Google Scholar]
- Nishi, M.; Wang, X.M.; Yoshida, K.; Takahashi, T.; Tsukamoto, T. An experimental study on fins, their role in control of the draft tube surging. In Hydraulic Machinery and Cavitation; Kluwer Academic Publishers: Dordrech, the Netherlands, 1996; pp. 905–914. [Google Scholar]
- Qian, Z.-D.; Yang, J.-D.; Huai, W.-X. Numerical simulation and analysis of pressure pulsation in francis hydraulic turbine with air admission. J. Hydr. 2007, 19, 467–472. [Google Scholar] [CrossRef]
- Kjeldsen, M.; Olsen, K.; Nielsen, T.; Dahlhaug, O. Water Injection for the Mitigation of Draft Tube Pressure Pulsations. In Proceedings of the International Meeting on Cavitation and Dynamic Problems in Hydraulic Machinery and Systems, Barcelona, Spain, 28–30 June 2006. [Google Scholar]
- Susan-Resiga, R.; Vu, T.C.; Muntean, S.; Ciocan, G.D.; Nennemann, B. Jet control of the draft tube in francis turbines at partial discharge. In Proceedings of the 23rd IAHR Symposium on Hydraulic Machinery and Systems, Yokohama, Japan, 17–21 October 2006; p. 192. [Google Scholar]
- Bosioc, A.; Susan-Resiga, R.; Muntean, S.; Tanasa, C. Unsteady pressure analysis of a swirling flow with vortex rope and axial water injection in a discharge cone. ASME J. Fluids Eng. 2012, 134, 081104. [Google Scholar] [CrossRef]
- Gitan, A.A.; Zulkifli, R.; Abdullah, S.; Sopian, K. Development of Pulsating Twin Jets Mechanism for Mixing Flow Heat Transfer Analysis. Sci. World J. 2014, 2014, 767614. [Google Scholar] [CrossRef] [PubMed]
- Binder, G.; Favre-Marinet, M. Some Characteristics of Pulsating or Flapping Jets. In Unsteady Turbulent Shear Flows: Symposium Toulouse, France, May 5–8, 1981; Springer: Berlin/Heidelberg, Germany, 1981; pp. 370–379. [Google Scholar] [CrossRef]
- Hirata, K.; Kubo, T.; Hatanaka, Y.; Matsushita, M.; Shobu, K.; Funaki, J. An Experimental Study of Amplitude and Frequency Effects upon a Pulsating Jet. J. Fluid Sci. Technol. 2009, 4, 578–589. [Google Scholar] [CrossRef][Green Version]
- Abdolahipour, S.; Mani, M.; Teleghani, S. Parametric study of a frequency-modulated pulse jet by measurements of flow characteristics. Phys. Scr. 2012, 96, 125012. [Google Scholar] [CrossRef]
- Abdolahipour, S. Effects of low and high frequency actuation on aerodynamic performance of a supercritical airfoil. Front. Mech. Eng. 2023, 9, 1290074. [Google Scholar] [CrossRef]
- Abdolahipour, S. Review on flow separation control: Effects of excitation frequency and momentum coefficient. Front. Mech. Eng. 2024, 10, 1380675. [Google Scholar] [CrossRef]
- Taleghani, A.S.; Hesabi, A.; Esfahanian, V. Numerical Study of Flow Control to Increase Vertical Tail Effectiveness of an Aircraft by Tangential Blowing. Int. J. Aeronaut. Space Sci. 2024, 26, 785–799. [Google Scholar] [CrossRef]
- Athanassiadis, G.A.; Hart, D.P. Effects of multijet coupling on propulsive performance in underwater pulsed jets. Phys. Rev. Fluids 2016, 1, 034501. [Google Scholar] [CrossRef]
- Edelman, L. The Pulsating Jet Engine-Its Evolution and Future Prospects; SAE Technical Paper 470212; SAE International: Warrendale, PA, USA, 1947. [Google Scholar] [CrossRef]
- Zhang, J.-Y.; Peng, W.-Q.; Luo, Z.-B.; Zhao, Z.-J.; Gong, J.-Y.; Dong, Z.-F. Dual Synthetic Jets Actuator and Its Applications Part V: Novel Valveless Continuous Micropump Based on Dual Synthetic Jets with a Tesla Structure. Actuators 2023, 12, 226. [Google Scholar] [CrossRef]
- Liu, Z.; Pan, T.; Wang, S.; Yan, Z. Characteristics of a Fluidic Oscillator with Low Frequency and Low Speed and Its Application to Stall Margin Improvement. Actuators 2022, 11, 341. [Google Scholar] [CrossRef]
- Tănasă, C.; Stuparu, A.; Bosioc, A.; Susan-Resiga, R. Numerical Analysis of Pulsating Water Jet Method for Mitigating the Vortex Rope. In Proceedings of the International Conference on Energy and Environment (CIEM), Timisoara, Romania, 18–19 October 2019; pp. 423–428. [Google Scholar] [CrossRef]
- Tanasa, C.; Szakal, R.; Mos, D.; Ciocan, T.; Muntean, S. Experimental and numerical analysis of decelerated swirling flow from the discharge cone of hydraulic turbines using pulsating jet technique. In Proceedings of the 29th IAHR Symposium on Hydraulic Machinery and Systems, Kyoto, Japan, 17–21 September 2018; IOP Conference Series: Earth and Environmental Science, Volume 240. IOP Publishing: Bristol, UK, 2019. [Google Scholar] [CrossRef]
- Avellan, F. Flow investigation in a Francis draft tube: The FLINDT project. In Proceedings of the 20th IAHR Symposium on Hydraulic Machinery and Systems, Charlotte, NC, USA, 6–9 August 2000; p. DY-03. [Google Scholar]
- Wei, L.; Ling, Z.; Wei-Dong, S.; Leilei, J.; Yongfei, Y.; Xiaofan, Z. PIV experiment of the unsteady flow field in mixed-flow pump under part loading condition. Exp. Therm. Fluid Sci. 2017, 83, 191–199. [Google Scholar] [CrossRef]
- Bosioc, A.I.; Muntean, S.; Tanasa, C.; Susan-Resiga, R.; Vékás, L. Unsteady pressure measurements of decelerated swirling flow in a discharge cone at lower runner speeds. IOP Conf. Ser. Earth Environ. Sci. 2014, 22, 032008. [Google Scholar] [CrossRef]
- Tanasa, C.; Bosioc, A.; Muntean, S.; Susan-Resiga, R. Flow-Feedback Control Technique for Vortex Rope Mitigation from Conical Diffuser of Hydraulic Turbines Draft Tube. Proc. Rom. Acad. Ser. A 2011, 12, 125–132. [Google Scholar]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tănasă, C.; Stuparu, A.C.; Bosioc, A.; Terteci, C.; Belgiu, G.; Nanu, S. Innovative Device to Control Self-Induced Instabilities Associated with the Swirling Flow from the Discharge Cone of Hydraulic Turbines. Actuators 2025, 14, 126. https://doi.org/10.3390/act14030126
Tănasă C, Stuparu AC, Bosioc A, Terteci C, Belgiu G, Nanu S. Innovative Device to Control Self-Induced Instabilities Associated with the Swirling Flow from the Discharge Cone of Hydraulic Turbines. Actuators. 2025; 14(3):126. https://doi.org/10.3390/act14030126
Chicago/Turabian StyleTănasă, Constantin, Adrian Ciprian Stuparu, Alin Bosioc, Cristina Terteci, George Belgiu, and Sorin Nanu. 2025. "Innovative Device to Control Self-Induced Instabilities Associated with the Swirling Flow from the Discharge Cone of Hydraulic Turbines" Actuators 14, no. 3: 126. https://doi.org/10.3390/act14030126
APA StyleTănasă, C., Stuparu, A. C., Bosioc, A., Terteci, C., Belgiu, G., & Nanu, S. (2025). Innovative Device to Control Self-Induced Instabilities Associated with the Swirling Flow from the Discharge Cone of Hydraulic Turbines. Actuators, 14(3), 126. https://doi.org/10.3390/act14030126







