Development of a Basilar Membrane-Inspired Mechanical Spectrum Analyzer Using Metastructures for Enhanced Frequency Selectivity
Abstract
1. Introduction
2. Metastructure Design and Study of Absorption Coefficients
2.1. Finite Element (FE) Model of the Host Beam
2.2. FE Model of the Host Structure with DVRs
2.3. Study of Absorption Coefficients in a Metastructure
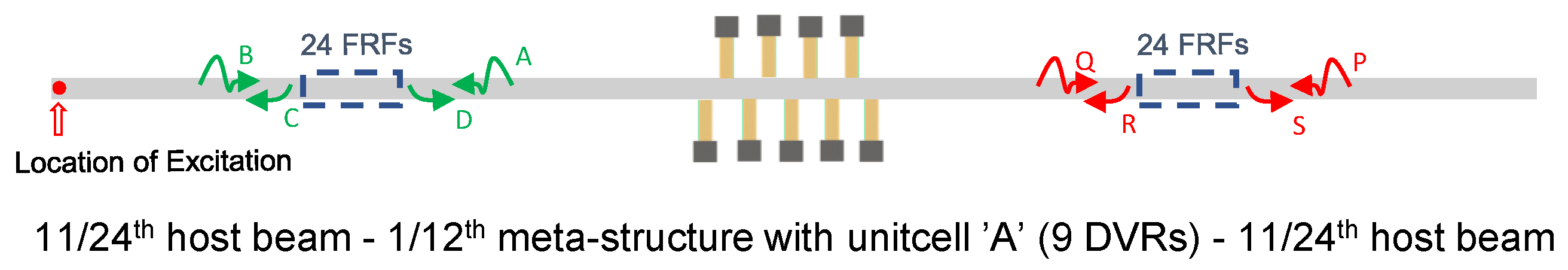
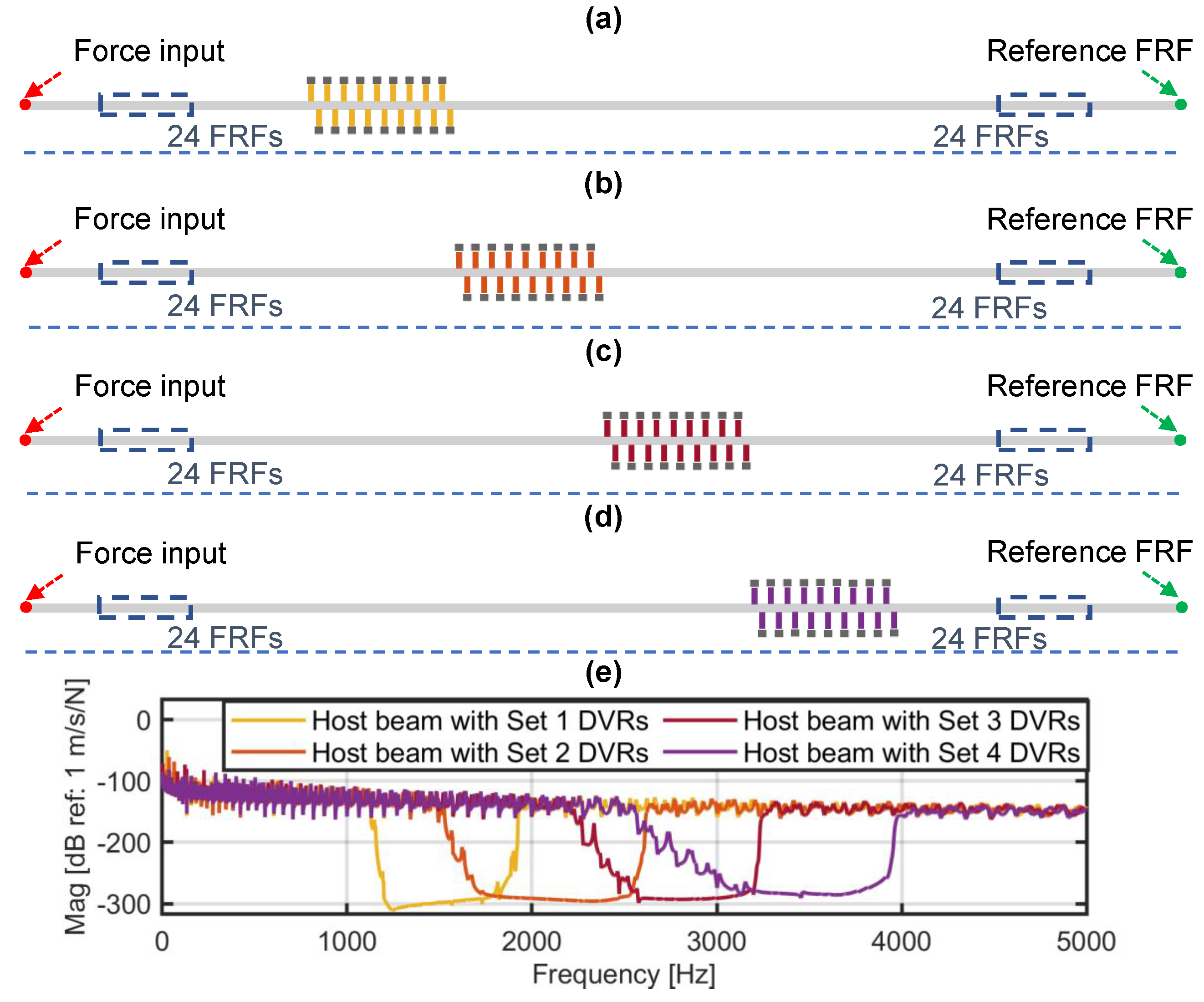
2.3.1. Metastructural Design Specimens Considered for Study
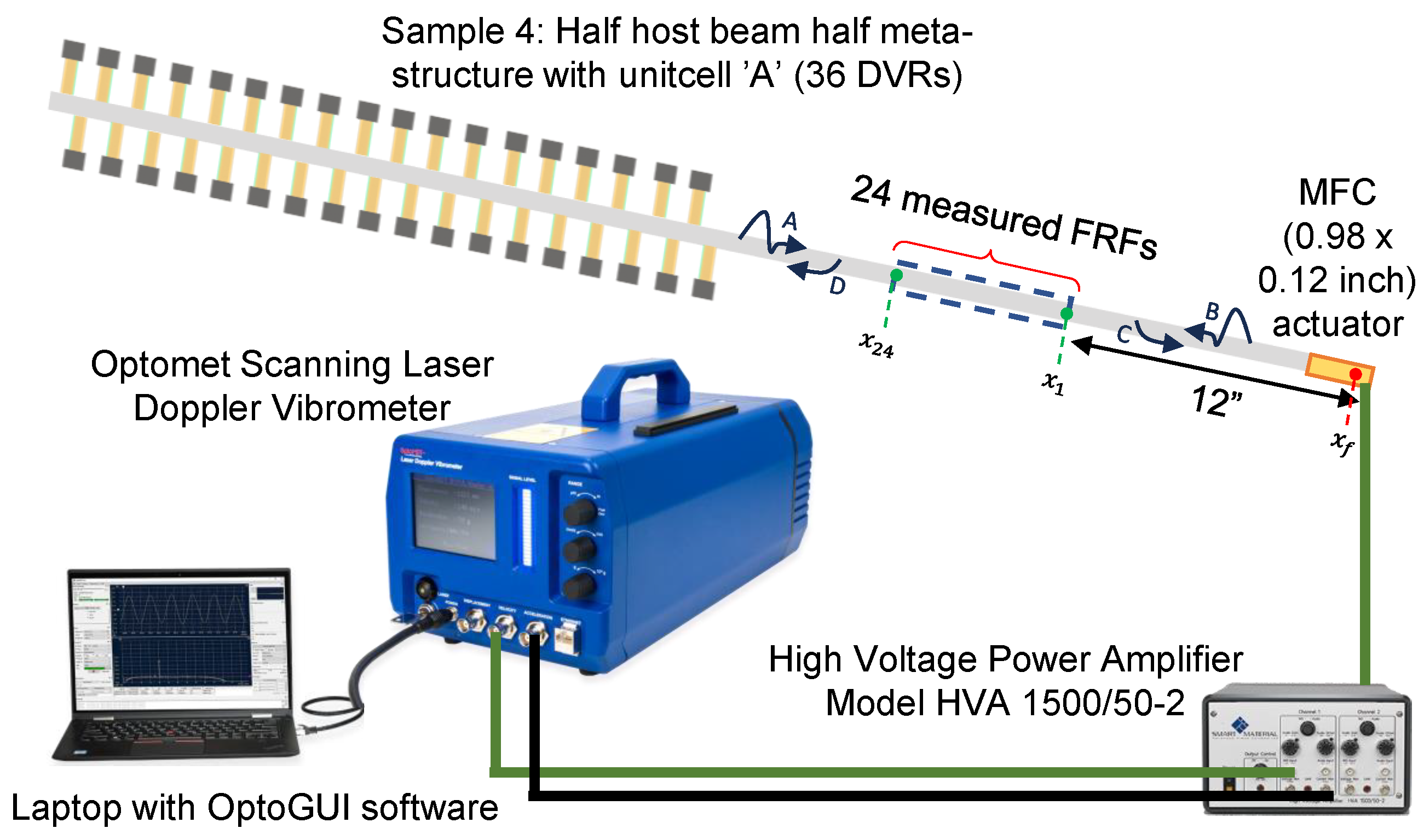
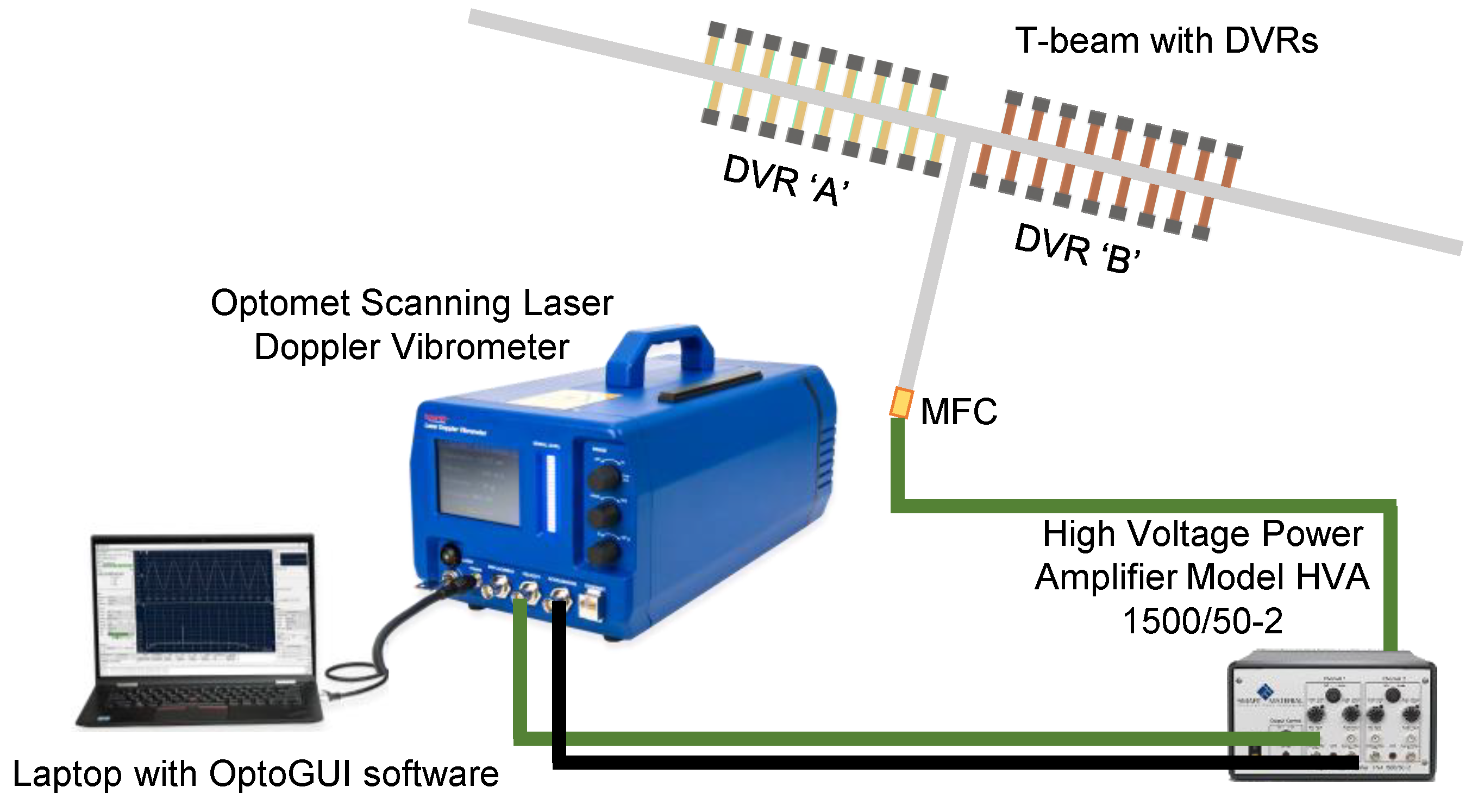
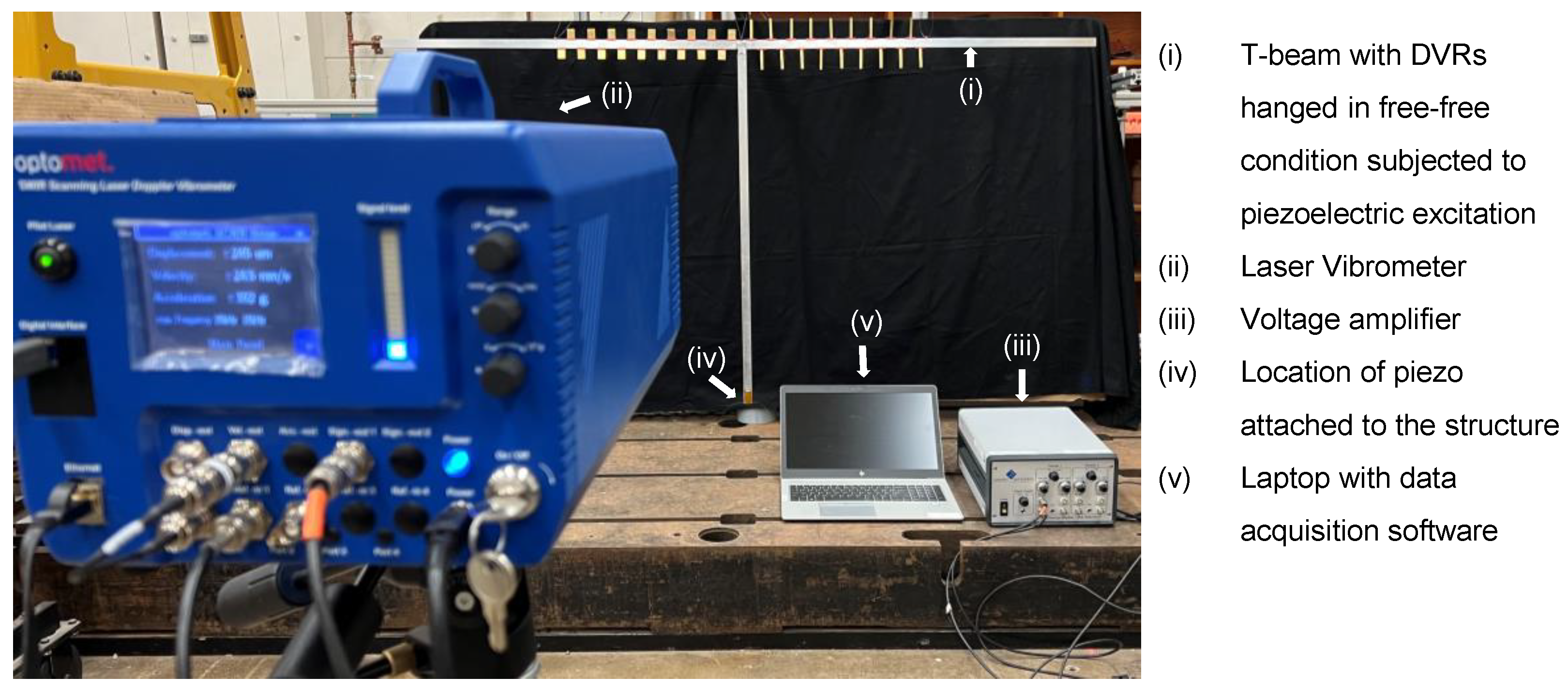
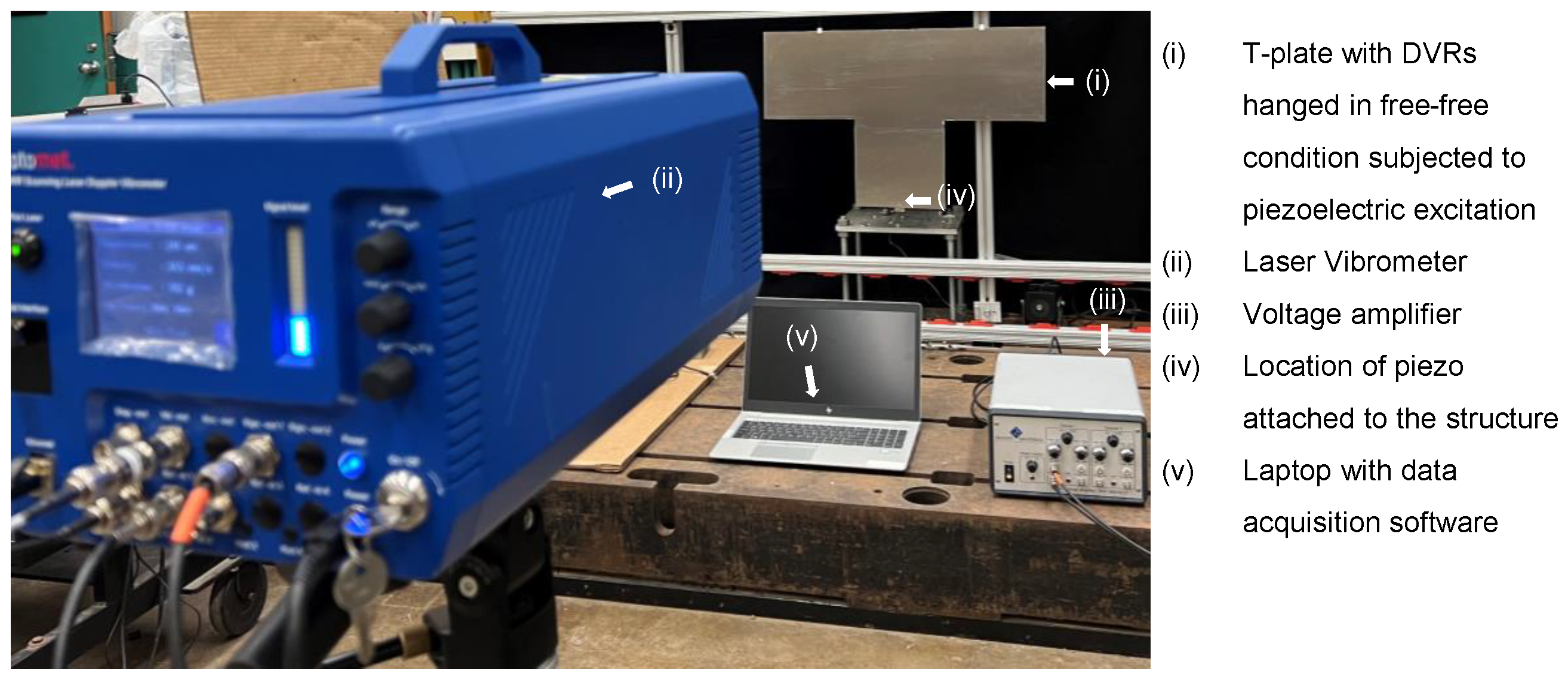
2.3.2. Experimental Setup
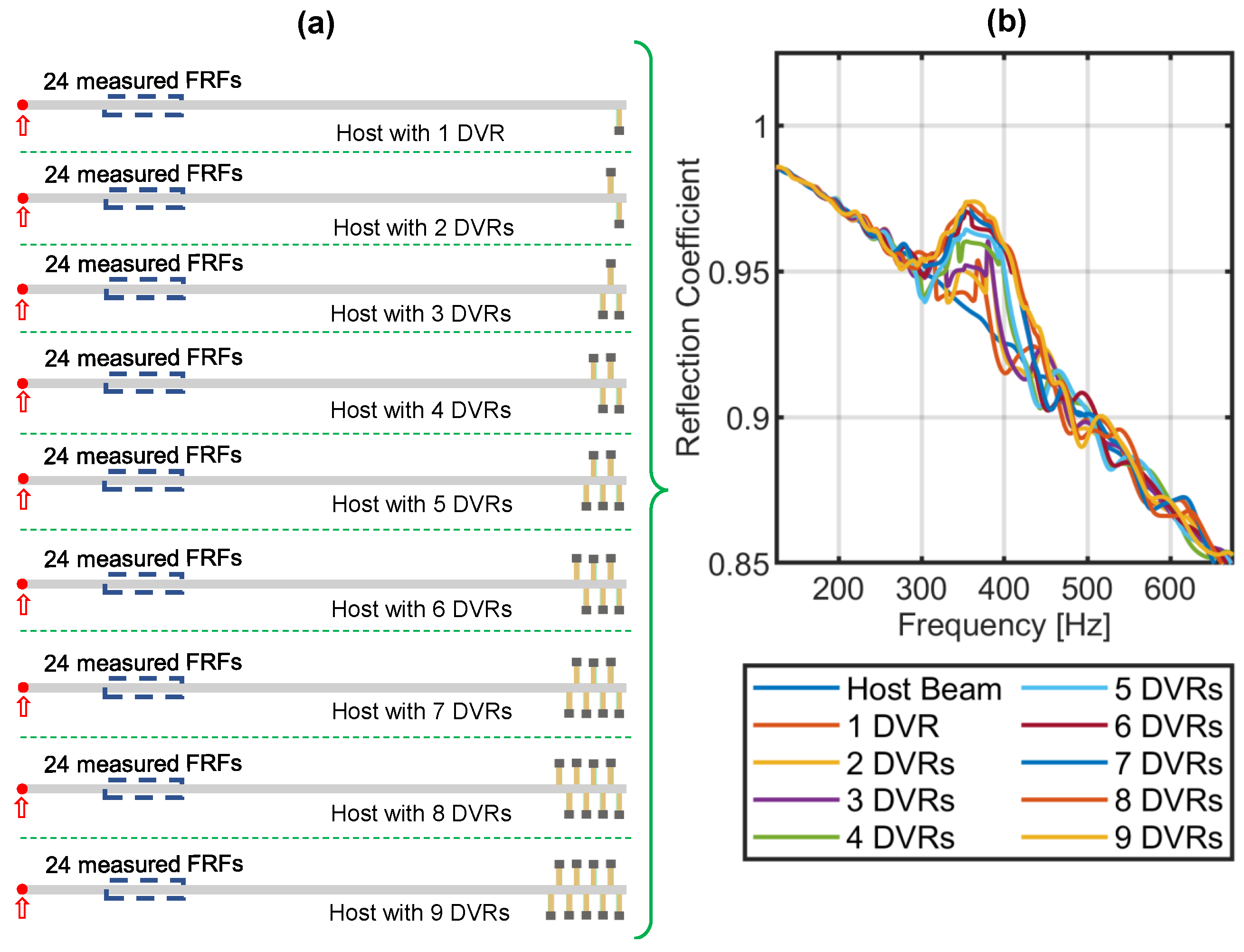
2.3.3. Estimation of Reflection Coefficients
2.3.4. Reflection Coefficient Calculations from Simulations
2.3.5. Reflection Coefficient Calculations from Experiments
2.3.6. Reflection Coefficient Calculations for Host Beam Attached with One to Nine DVRs
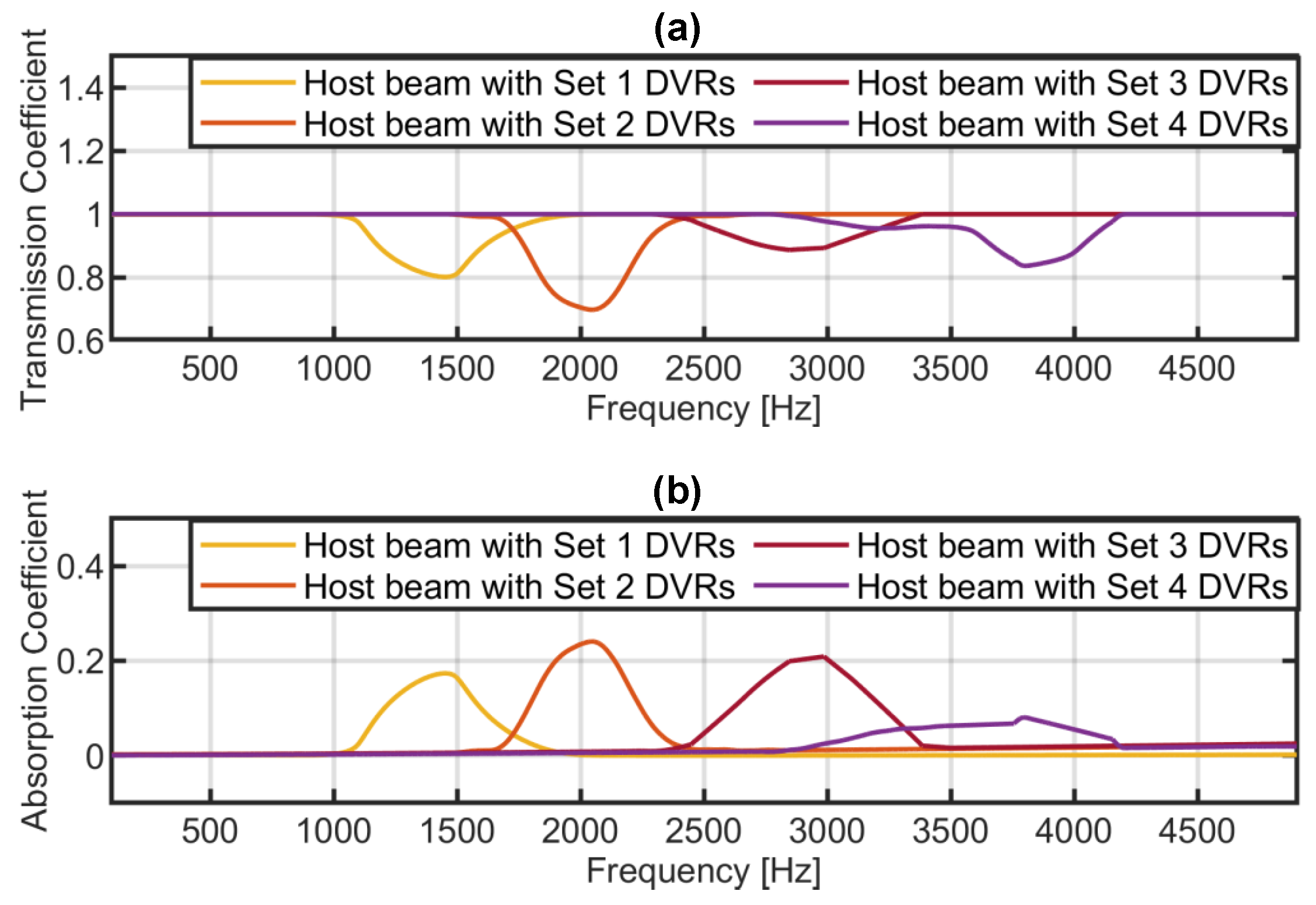
2.3.7. Transmission and Absorption Coefficient Calculations from Simulations
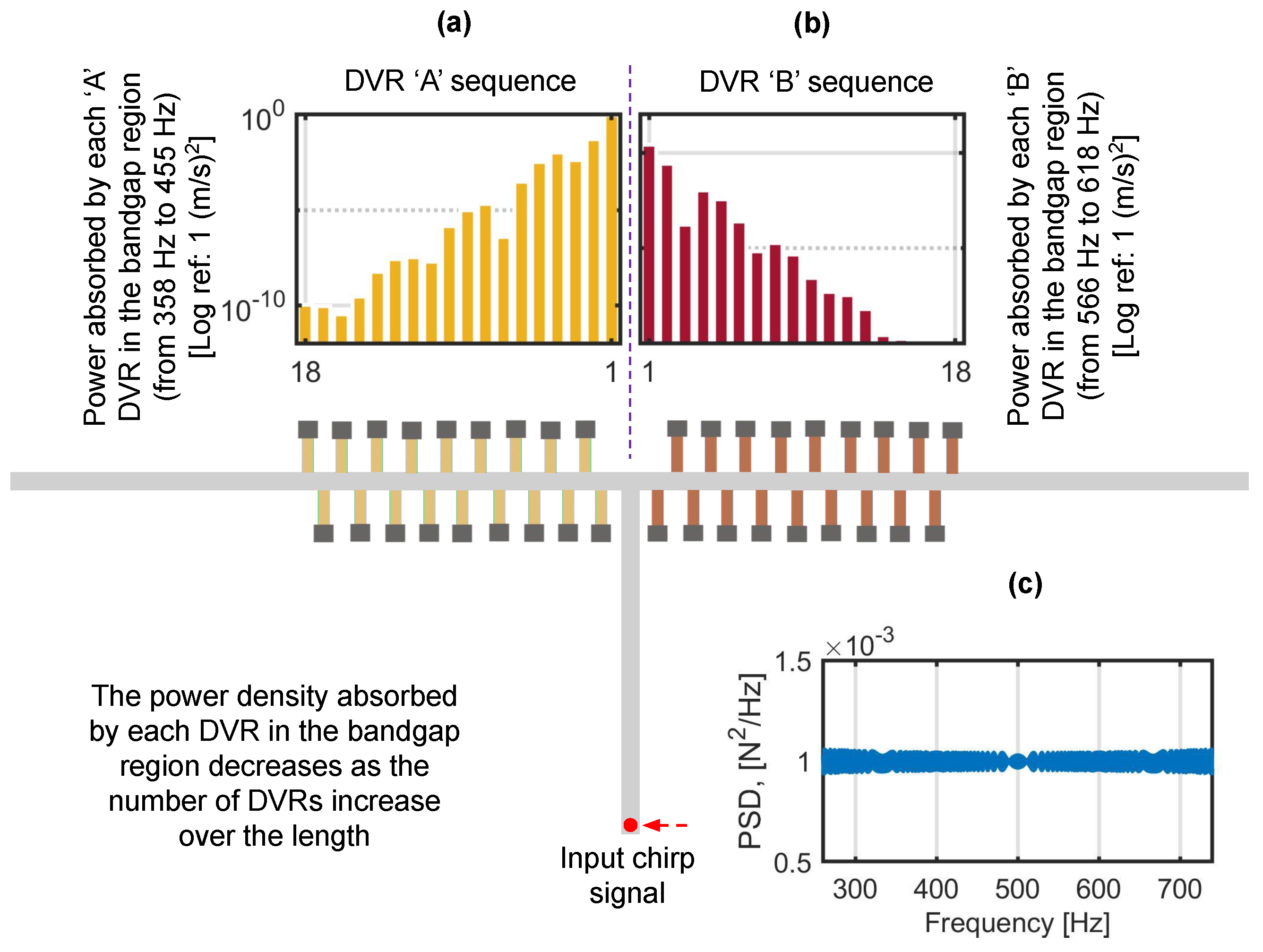
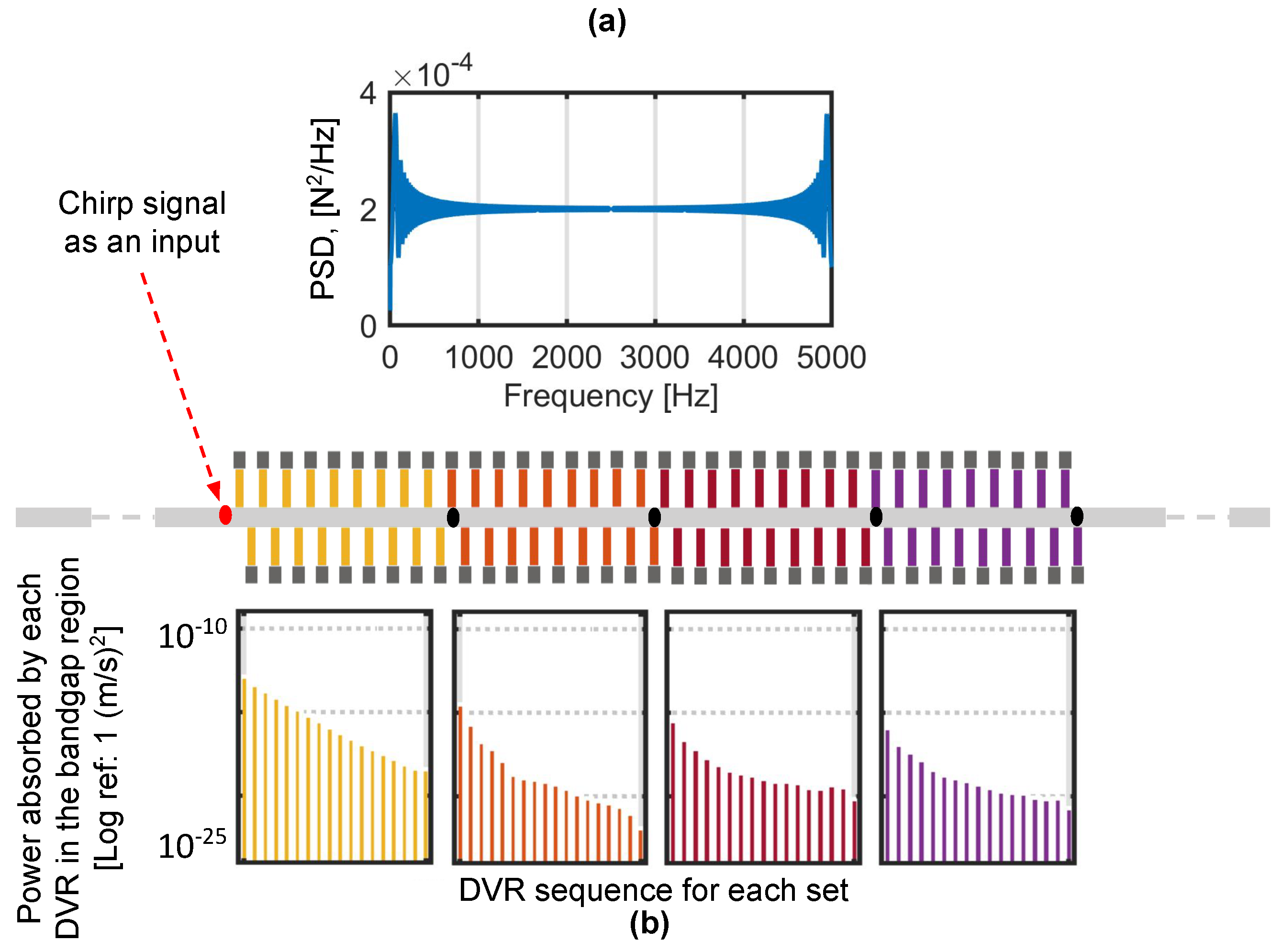
2.3.8. Power Absorbed by Each DVR in the Bandgap Region
3. Modeling and Experimental Validation of Host T-Beam with DVRs
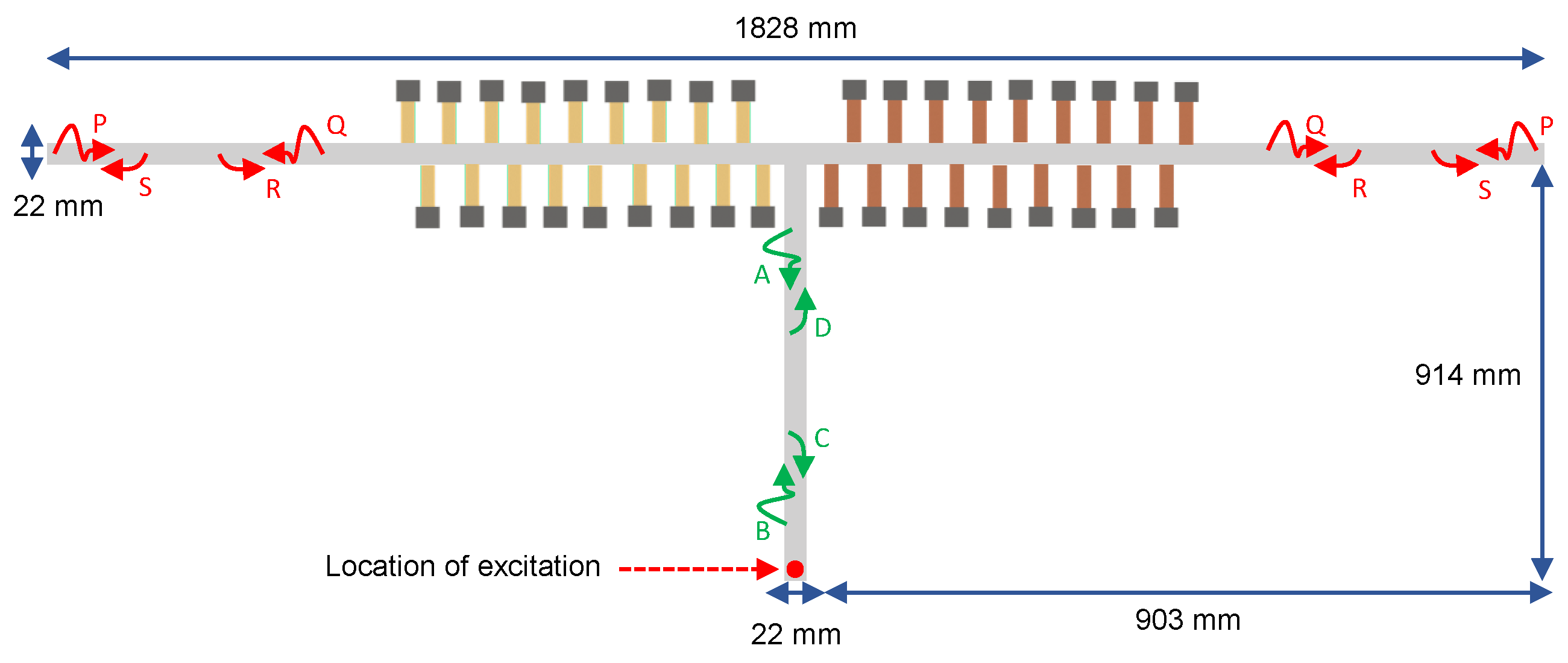
3.1. FE Model of the Host Structure
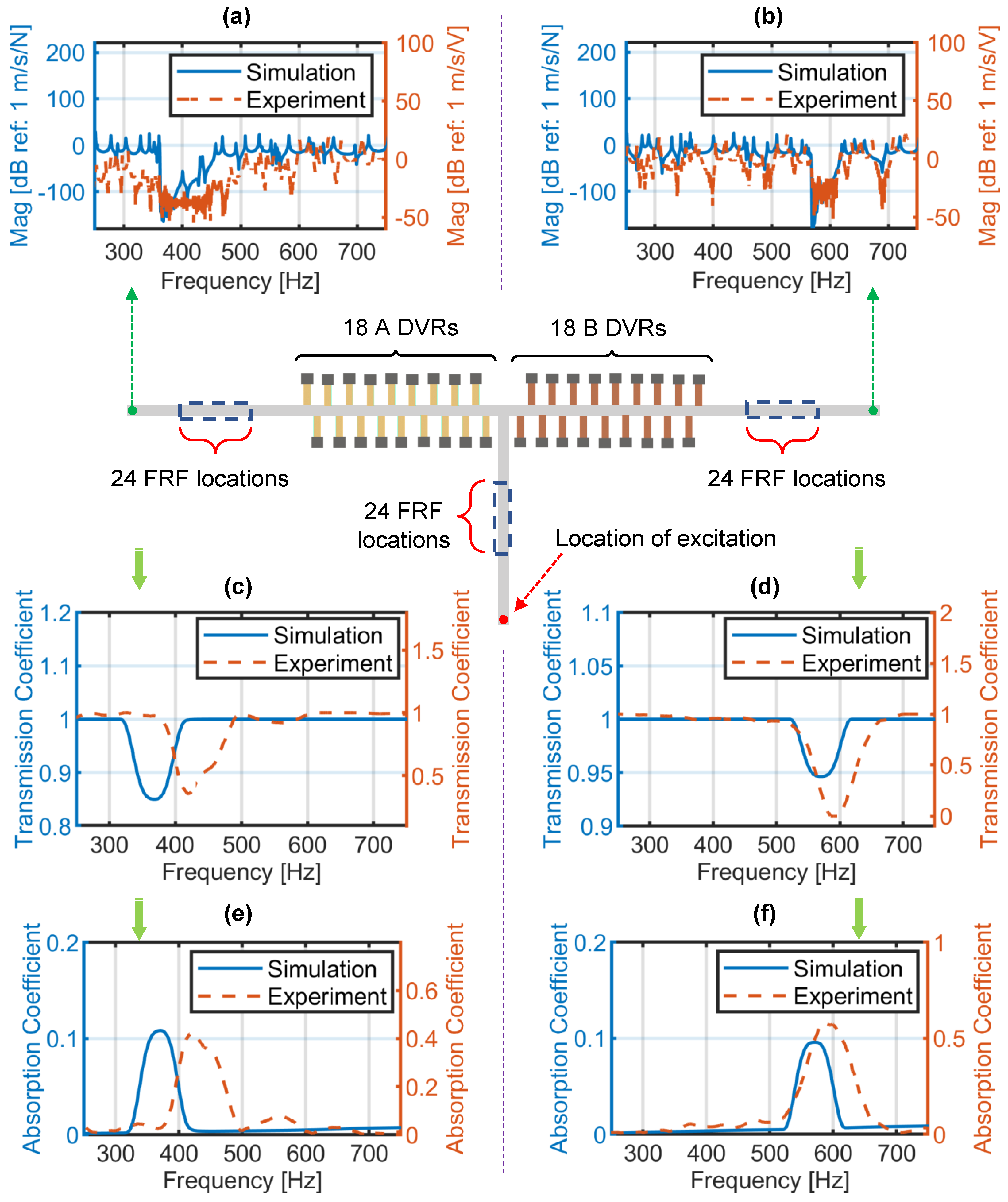
3.2. Experimental Validation of T-Beam with DVRs
3.3. Estimation of Absorption Coefficients on Each Arm of the T-Beam
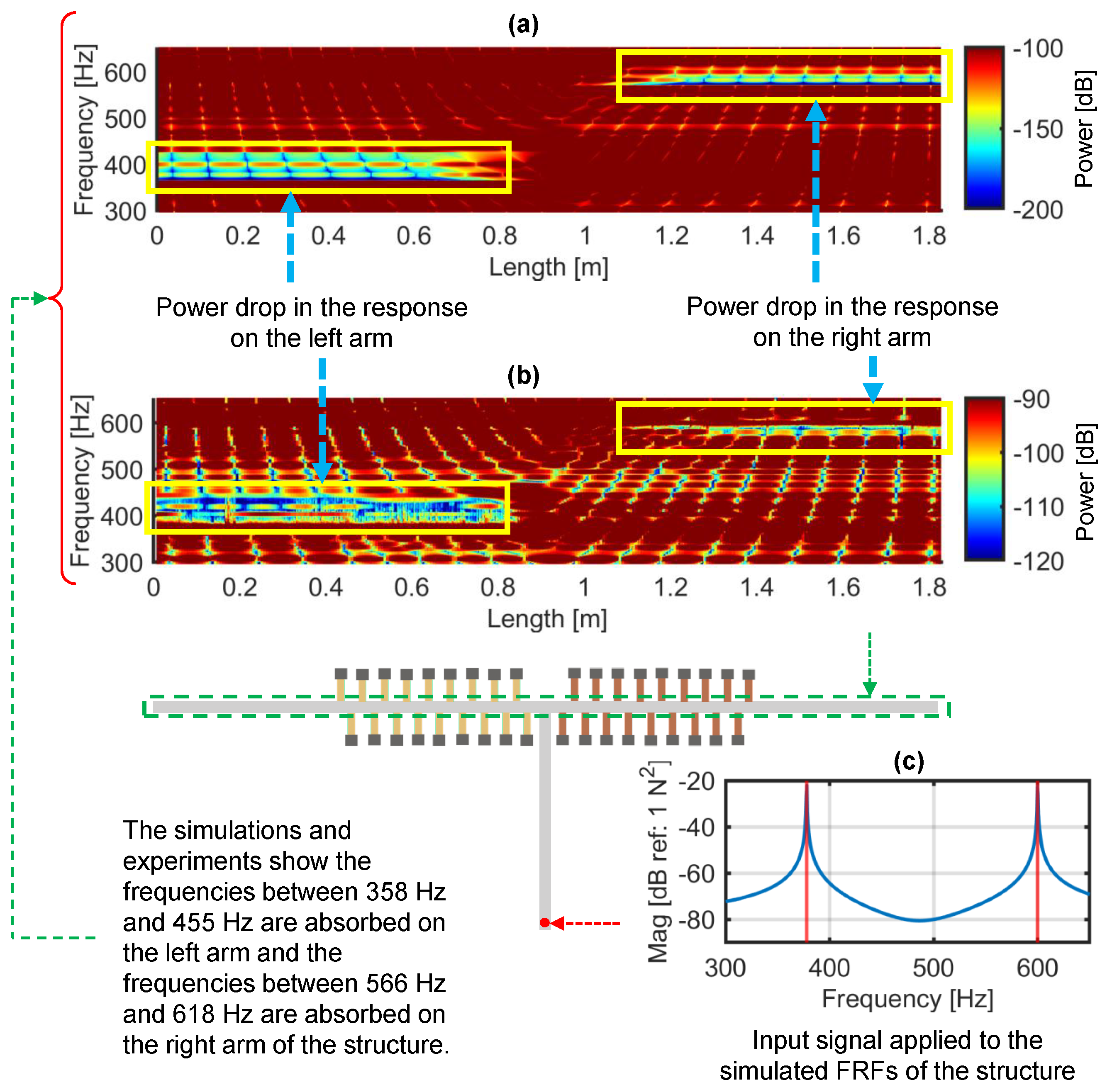
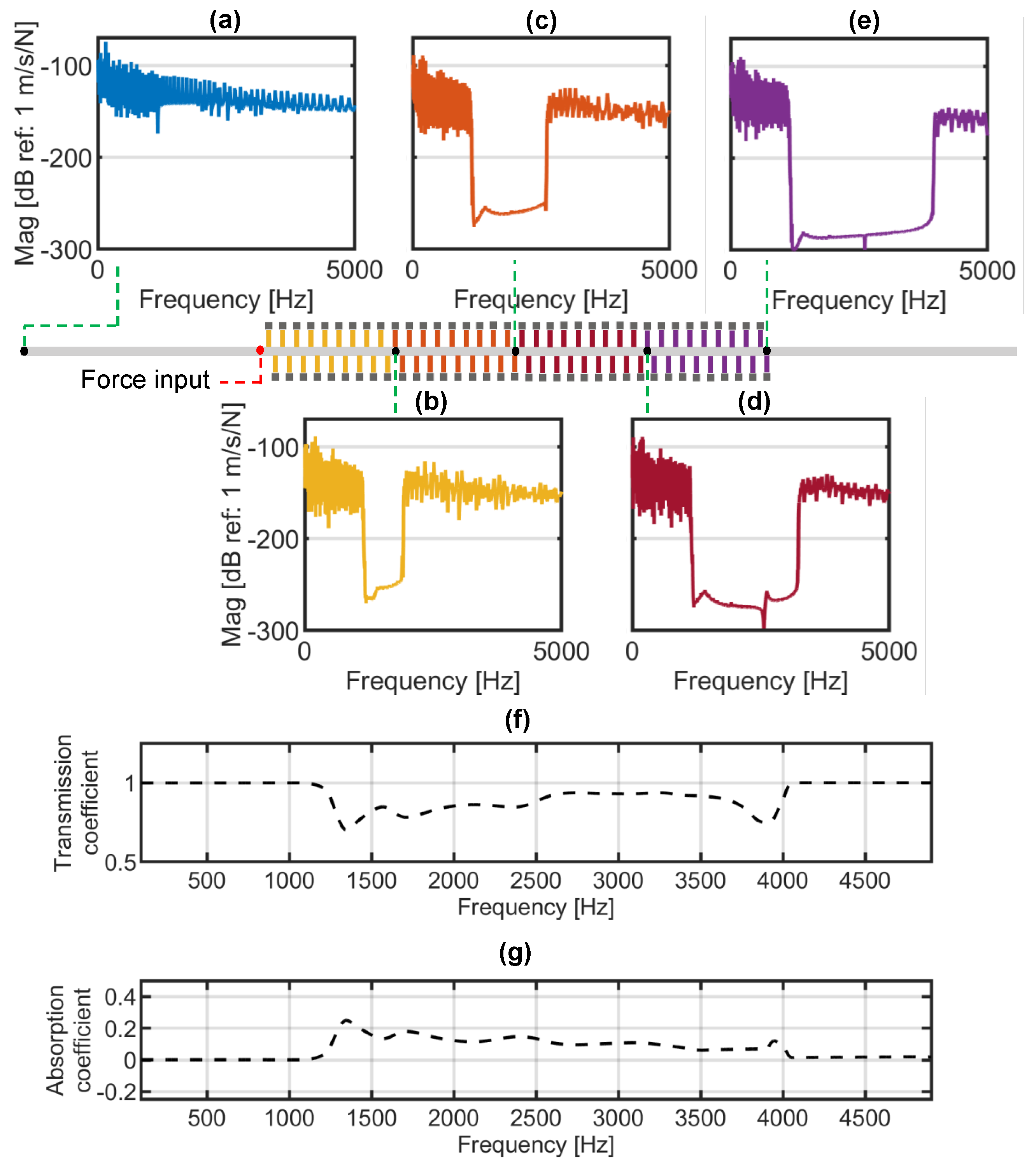
Power Absorbed by Each DVR in Selective Bandgaps
4. Selective Frequency Transmission in the T-Beam
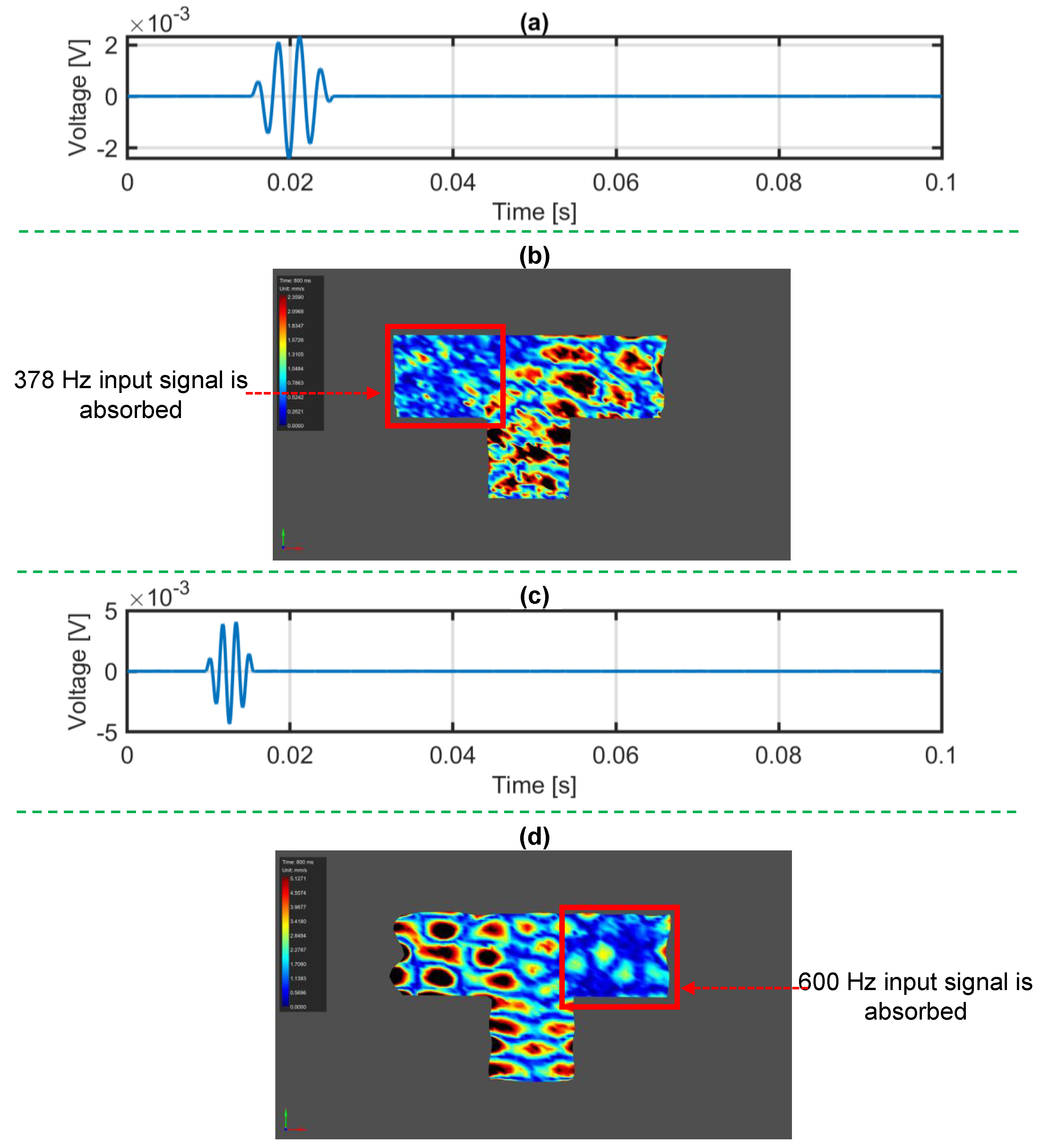
4.1. Wave Propagation at 378 Hz and 600 Hz
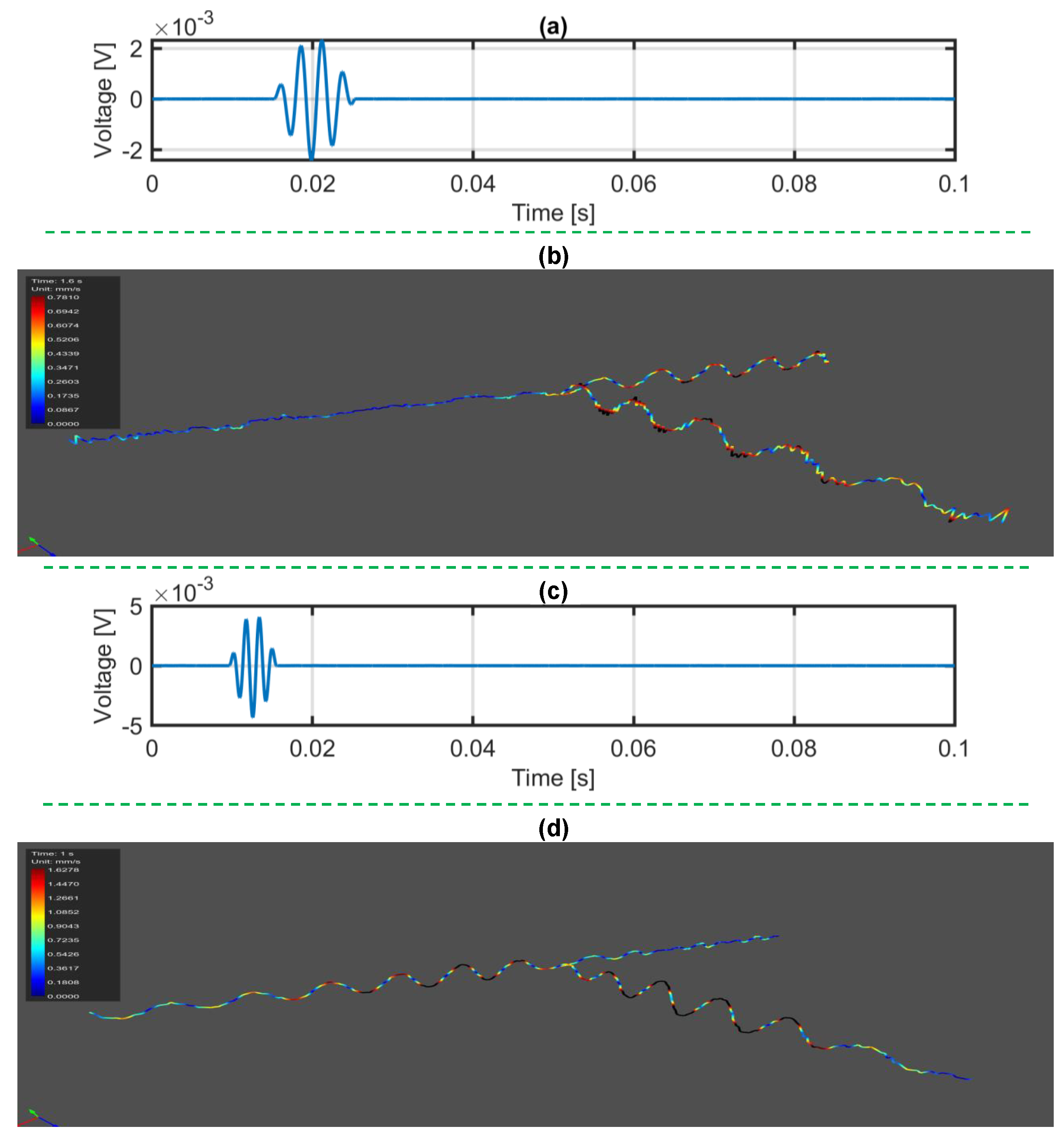
4.1.1. Simulation
4.1.2. Experiments
5. Basilar Membrane-Inspired Mechanical Spectrum Analyzer
Power Absorbed by Each DVR in Selective Bandgaps
6. Extension of Selective Frequency Transmission in 2D Structures and Future Work
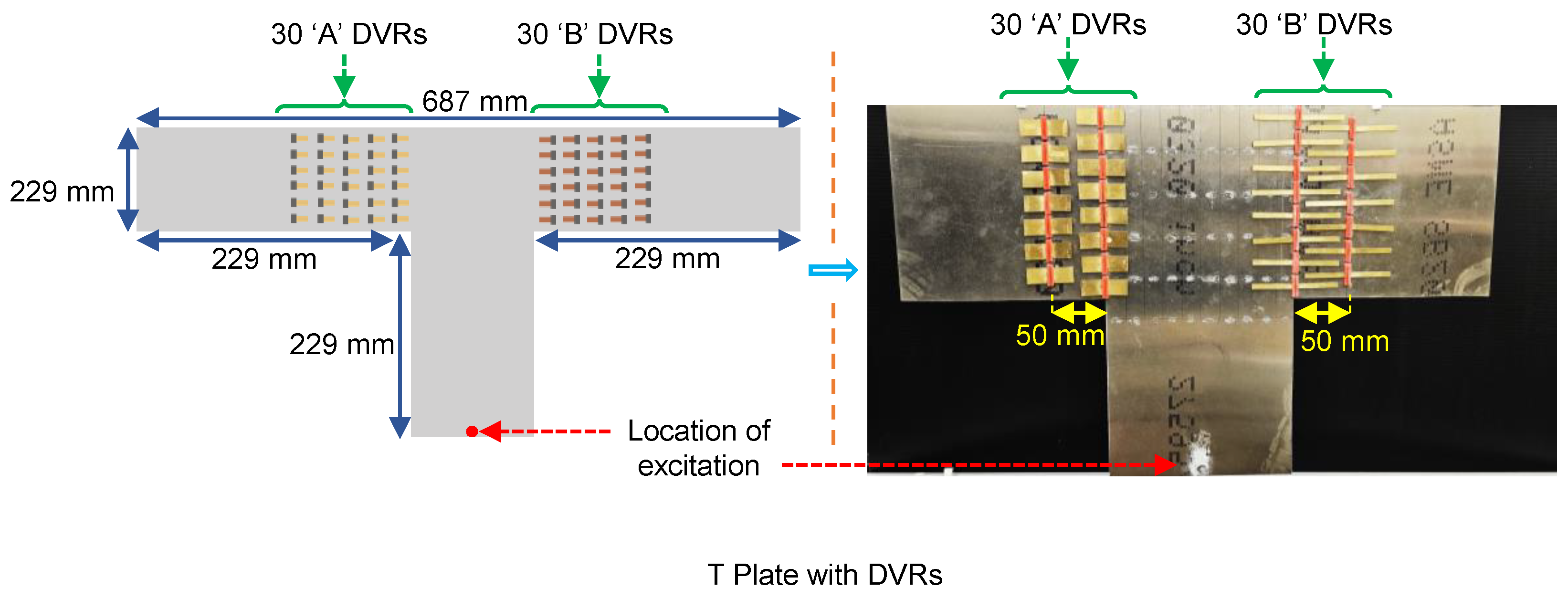
6.1. Selective Frequency Transmission in a T-Plate
Experiments
7. Conclusions
Declaration of Generative AI and AI-Assisted Technologies in the Writing Process
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Eggermont, J.J. Cochlea and auditory nerve. Handb. Clin. Neurol. 2019, 160, 437–449. [Google Scholar] [PubMed]
- Davis, R.L. Gradients of neurotrophins, ion channels, and tuning in the cochlea. Neuroscientist 2003, 9, 311–316. [Google Scholar] [CrossRef] [PubMed]
- Robles, L.; Ruggero, M.A. Mechanics of the mammalian cochlea. Physiol. Rev. 2001, 81, 1305–1352. [Google Scholar] [CrossRef] [PubMed]
- Zwislocki, J. Analysis of the middle-ear function. Part I: Input impedance. J. Acoust. Soc. Am. 1962, 34, 1514–1523. [Google Scholar] [CrossRef]
- Holmes, M.; Cole, J. Pseudo-resonance in the cochlea. In Mechanics of Hearing: Proceedings of the IUTAM/ICA Symposium Held at Delft University of Technology The Netherlands 13–15 July 1983; Springer: Berlin/Heidelberg, Germany, 1983; pp. 45–52. [Google Scholar]
- Oghalai, J.S. The cochlear amplifier: Augmentation of the traveling wave within the inner ear. Curr. Opin. Otolaryngol. Head Neck Surg. 2004, 12, 431. [Google Scholar] [CrossRef][Green Version]
- Kim, S.; Song, W.J.; Jang, J.; Jang, J.H.; Choi, H. Characterization and modeling of an acoustic sensor using AlN thin-film for frequency selectivity. Electron. Mater. Lett. 2014, 10, 299–303. [Google Scholar] [CrossRef]
- Song, W.J.; Jang, J.; Kim, S.; Choi, H. Influence of mechanical coupling by SiO2 membrane on the frequency selectivity of microfabricated beam arrays for artificial basilar membranes. J. Mech. Sci. Technol. 2015, 29, 963–971. [Google Scholar] [CrossRef]
- Shintaku, H.; Kobayashi, T.; Zusho, K.; Kotera, H.; Kawano, S. Wide-range frequency selectivity in an acoustic sensor fabricated using a microbeam array with non-uniform thickness. J. Micromech. Microeng. 2013, 23, 115014. [Google Scholar] [CrossRef]
- Jang, J.; Jang, J.H.; Choi, H. Biomimetic artificial basilar membranes for next-generation cochlear implants. Adv. Healthc. Mater. 2017, 6, 1700674. [Google Scholar] [CrossRef]
- Shintaku, H.; Nakagawa, T.; Kitagawa, D.; Tanujaya, H.; Kawano, S.; Ito, J. Development of piezoelectric acoustic sensor with frequency selectivity for artificial cochlea. Sens. Actuators A Phys. 2010, 158, 183–192. [Google Scholar] [CrossRef]
- Inaoka, T.; Shintaku, H.; Nakagawa, T.; Kawano, S.; Ogita, H.; Sakamoto, T.; Hamanishi, S.; Wada, H.; Ito, J. Piezoelectric materials mimic the function of the cochlear sensory epithelium. Proc. Natl. Acad. Sci. USA 2011, 108, 18390–18395. [Google Scholar] [CrossRef] [PubMed]
- White, R.D.; Grosh, K. Microengineered hydromechanical cochlear model. Proc. Natl. Acad. Sci. USA 2005, 102, 1296–1301. [Google Scholar] [CrossRef] [PubMed]
- Wittbrodt, M.J.; Steele, C.R.; Puria, S. Developing a physical model of the human cochlea using microfabrication methods. Audiol. Neurotol. 2006, 11, 104–112. [Google Scholar] [CrossRef] [PubMed]
- Jung, Y.; Kwak, J.H.; Lee, Y.H.; Kim, W.D.; Hur, S. Development of a multi-channel piezoelectric acoustic sensor based on an artificial basilar membrane. Sensors 2013, 14, 117–128. [Google Scholar] [CrossRef]
- Lee, H.S.; Chung, J.; Hwang, G.T.; Jeong, C.K.; Jung, Y.; Kwak, J.H.; Kang, H.; Byun, M.; Kim, W.D.; Hur, S.; et al. Flexible inorganic piezoelectric acoustic nanosensors for biomimetic artificial hair cells. Adv. Funct. Mater. 2014, 24, 6914–6921. [Google Scholar] [CrossRef]
- Chavan, S.H.; Malladi, S.S.; Malladi, V.V.S. Reinforcement Learning approach of switching bi-stable oscillators to adapt bandgaps of 1D-meta-structures. Mech. Syst. Signal Process. 2023, 191, 110151. [Google Scholar] [CrossRef]
- He, H.; Fan, H. Explosion vibration mitigation of meta-plate with mass–spring metastructures. Extrem. Mech. Lett. 2021, 42, 101108. [Google Scholar] [CrossRef]
- An, X.; Lai, C.; Fan, H.; Zhang, C. 3D acoustic metamaterial-based mechanical metalattice structures for low-frequency and broadband vibration attenuation. Int. J. Solids Struct. 2020, 191, 293–306. [Google Scholar] [CrossRef]
- An, X.; Lai, C.; He, W.; Fan, H. Three-dimensional meta-truss lattice composite structures with vibration isolation performance. Extrem. Mech. Lett. 2019, 33, 100577. [Google Scholar] [CrossRef]
- An, X.; Fan, H.; Zhang, C. Elastic wave and vibration bandgaps in two-dimensional acoustic metamaterials with resonators and disorders. Wave Motion 2018, 80, 69–81. [Google Scholar] [CrossRef]
- An, X.; Fan, H.; Zhang, C. Wave dispersion in one-dimensional periodic graded metacomposites. J. Sound Vib. 2017, 409, 217–226. [Google Scholar] [CrossRef]
- Ghasemi, F.; Salari, E.; Salari, A.; Rastgoo, A.; Li, D.; Deng, J. Integrating analytical and machine learning methods for investigating nonlinear bending and post-buckling behavior of 3D-printed auxetic tubes. In Engineering with Computers; Springer: Berlin/Heidelberg, Germany, 2024; pp. 1–38. [Google Scholar]
- Nasri, M.R.; Salari, E.; Salari, A.; Vanini, S.A.S. Nonlinear bending and buckling analysis of 3D-printed meta-sandwich curved beam with auxetic honeycomb core. Aerosp. Sci. Technol. 2024, 152, 109339. [Google Scholar] [CrossRef]
- Barnard, A.R.; Rao, M.D. Measurement of sound transmission loss using a modified four microphone impedance tube. In Proceedings of the ASME Noise Control and Acoustics Division (Noise-Con’04), Baltimore, MD, USA, 12–14 July 2004. [Google Scholar]
- Deshpande, S.P.; Rao, M.D. Development of a low-cost impedance tube to measure acoustic absorption and transmission loss of materials. In Proceedings of the 2014 ASEE Annual Conference & Exposition, Indianapolis, IN, USA, 15–18 June 2014; pp. 24–417. [Google Scholar]
- Jung, S.S.; Kim, Y.T.; Lee, Y.B.; Cho, S.I.; Lee, J.K. Measurement of sound transmission loss by using impedance tubes. J. Korean Phys. Soc. 2008, 53, 596–600. [Google Scholar] [CrossRef]
- Mei, C.; Mace, B.R. Wave Reflection and Transmission in Timoshenko Beams and Wave Analysis of Timoshenko Beam Structures. J. Vib. Acoust. 2004, 127, 382–394. [Google Scholar] [CrossRef]
- Cremer, L.; Heckl, M. Structure-Borne Sound: Structural Vibrations and Sound Radiation at Audio Frequencies; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Miller, D.W.; Von Flotow, A. A travelling wave approach to power flow in structural networks. J. Sound Vib. 1989, 128, 145–162. [Google Scholar] [CrossRef]
- Mace, B. Wave reflection and transmission in beams. J. Sound Vib. 1984, 97, 237–246. [Google Scholar] [CrossRef]
- Wang, C.; Rose, L. Wave reflection and transmission in beams containing delamination and inhomogeneity. J. Sound Vib. 2003, 264, 851–872. [Google Scholar] [CrossRef]
- Denis, V.; Gautier, F.; Pelat, A.; Poittevin, J. Measurement and modelling of the reflection coefficient of an acoustic black hole termination. J. Sound Vib. 2015, 349, 67–79. [Google Scholar] [CrossRef]
- Mace, B.; Halkyard, C.; El-Khatib, H. Real-time measurement of wave components and intensity in a beam in the presence of a near field. J. Sound Vib. 2005, 286, 507–527. [Google Scholar] [CrossRef]
- Cao, S.; Lee, H.P.; Lim, K.M. Estimation of wave reflection coefficient by semi-analytical method in an acoustic black hole beam. Int. J. Appl. Mech. 2020, 12, 2050004. [Google Scholar] [CrossRef]
- Feurtado, P.; Conlon, S. Investigation of boundary-taper reflection for acoustic black hole design. Noise Control Eng. J. 2015, 63, 460–466. [Google Scholar] [CrossRef]
- Huang, W.; Zhang, H.; Inman, D.J.; Qiu, J.; Cesnik, C.E.; Ji, H. Low reflection effect by 3D printed functionally graded acoustic black holes. J. Sound Vib. 2019, 450, 96–108. [Google Scholar] [CrossRef]
- Leng, J.; Romero-García, V.; Pelat, A.; Pico, R.; Groby, J.P.; Gautier, F. Interpretation of the acoustic black hole effect based on the concept of critical coupling. J. Sound Vib. 2020, 471, 115199. [Google Scholar] [CrossRef]
- Magliacano, D.; Ouisse, M.; de Rosa, S.; Franco, F.; Khelif, A. Investigations about the modelling of acoustic properties of periodic porous materials with the shift cell approach. In Proceedings of the SMART 2019: IX ECCOMAS Thematic Conference on Smart Structures and Materials, Paris, France, 8–11 July 2019. [Google Scholar]
- Meirovitch, L. Principles and Techniques of Vibrations; Prentice Hall: Englewood Cliffs, NJ, USA, 1997; Volume 1. [Google Scholar]
- Inman, D.J. Engineering Vibration; Prentice Hall: Englewood Cliffs, NJ, USA, 1994; Volume 3. [Google Scholar]
- Albakri, M.I.; Malladi, V.V.S.; Gugercin, S.; Tarazaga, P.A. Estimating dispersion curves from frequency response functions via vector-fitting. Mech. Syst. Signal Process. 2020, 140, 106597. [Google Scholar] [CrossRef]
- Rao, S.S. The Finite Element Method in Engineering; Butterworth-Heinemann: Oxford, UK, 2017. [Google Scholar]
- Malladi, V.V.S.; Albakri, M.I.; Krishnan, M.; Gugercin, S.; Tarazaga, P.A. Estimating experimental dispersion curves from steady-state frequency response measurements. Mech. Syst. Signal Process. 2022, 164, 108218. [Google Scholar] [CrossRef]
- Penrose, R. A generalized inverse for matrices. Math. Proc. Camb. Philos. Soc. 1955, 51, 406–413. [Google Scholar] [CrossRef]





| Geometric Properties | Material Properties | |||||
|---|---|---|---|---|---|---|
| (mm × mm × mm) | (GPa) | (kg/m3) | (GPa) | |||
| Host beam | 66 | 2700 | 0.33 | 24 | 0.93 | |
| DVR “A” | 110 | 8730 | 0.34 | 77 | 0.85 | |
| DVR “B” | 110 | 8730 | 0.34 | 77 | 0.85 | |
| Reduced Order Model | DVR “A” | DVR “B” |
|---|---|---|
| Targeted natural frequency (Hz) | 352.68 | |
| Mass (kg) | 0.005 | 0.0045 |
| Stiffness (N/m) | 24,028.8 | 56,170.5 |
| Damping (Ns/m) | 0.4889 | 0.8267 |
| Geometric Properties | Material Properties | |||||
|---|---|---|---|---|---|---|
| (mm × mm × mm) | (GPa) | (kg/m3) | (GPa) | |||
| Host beam | 66 | 2700 | 0.33 | 24 | 0.93 | |
| Natural Frequency | Mass | Stiffness | |
|---|---|---|---|
| (Hz) | (kg) | (N/m) | |
| DVR A | 1225 | 0.0024 | 142,182 |
| DVR B | 1750 | 0.002 | 244,641 |
| DVR C | 2600 | 0.000923 | 246,345 |
| DVR D | 3200 | 0.000923 | 373,162 |
| Spatial Location | Start Frequency | End Frequency | |
|---|---|---|---|
| (mm) | (Hz) | (Hz) | |
| Set 1 DVRs | 1828 to 2286 | 1130 | 1925 |
| Set 2 DVRs | 2286 to 2743 | 1570 | 2610 |
| Set 3 DVRs | 2743 to 3200 | 2250 | 3230 |
| Set 4 DVRs | 3200 to 3658 | 2660 | 3960 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chavan, S.H.; Malladi, V.V.N.S. Development of a Basilar Membrane-Inspired Mechanical Spectrum Analyzer Using Metastructures for Enhanced Frequency Selectivity. Actuators 2025, 14, 63. https://doi.org/10.3390/act14020063
Chavan SH, Malladi VVNS. Development of a Basilar Membrane-Inspired Mechanical Spectrum Analyzer Using Metastructures for Enhanced Frequency Selectivity. Actuators. 2025; 14(2):63. https://doi.org/10.3390/act14020063
Chicago/Turabian StyleChavan, Shantanu H., and Vijaya V. N. Sriram Malladi. 2025. "Development of a Basilar Membrane-Inspired Mechanical Spectrum Analyzer Using Metastructures for Enhanced Frequency Selectivity" Actuators 14, no. 2: 63. https://doi.org/10.3390/act14020063
APA StyleChavan, S. H., & Malladi, V. V. N. S. (2025). Development of a Basilar Membrane-Inspired Mechanical Spectrum Analyzer Using Metastructures for Enhanced Frequency Selectivity. Actuators, 14(2), 63. https://doi.org/10.3390/act14020063






