Dynamics Study of Hybrid Support Flywheel Energy Storage System with Damping Ring Device
Abstract
1. Introduction
2. Design Theory of FESS with DR
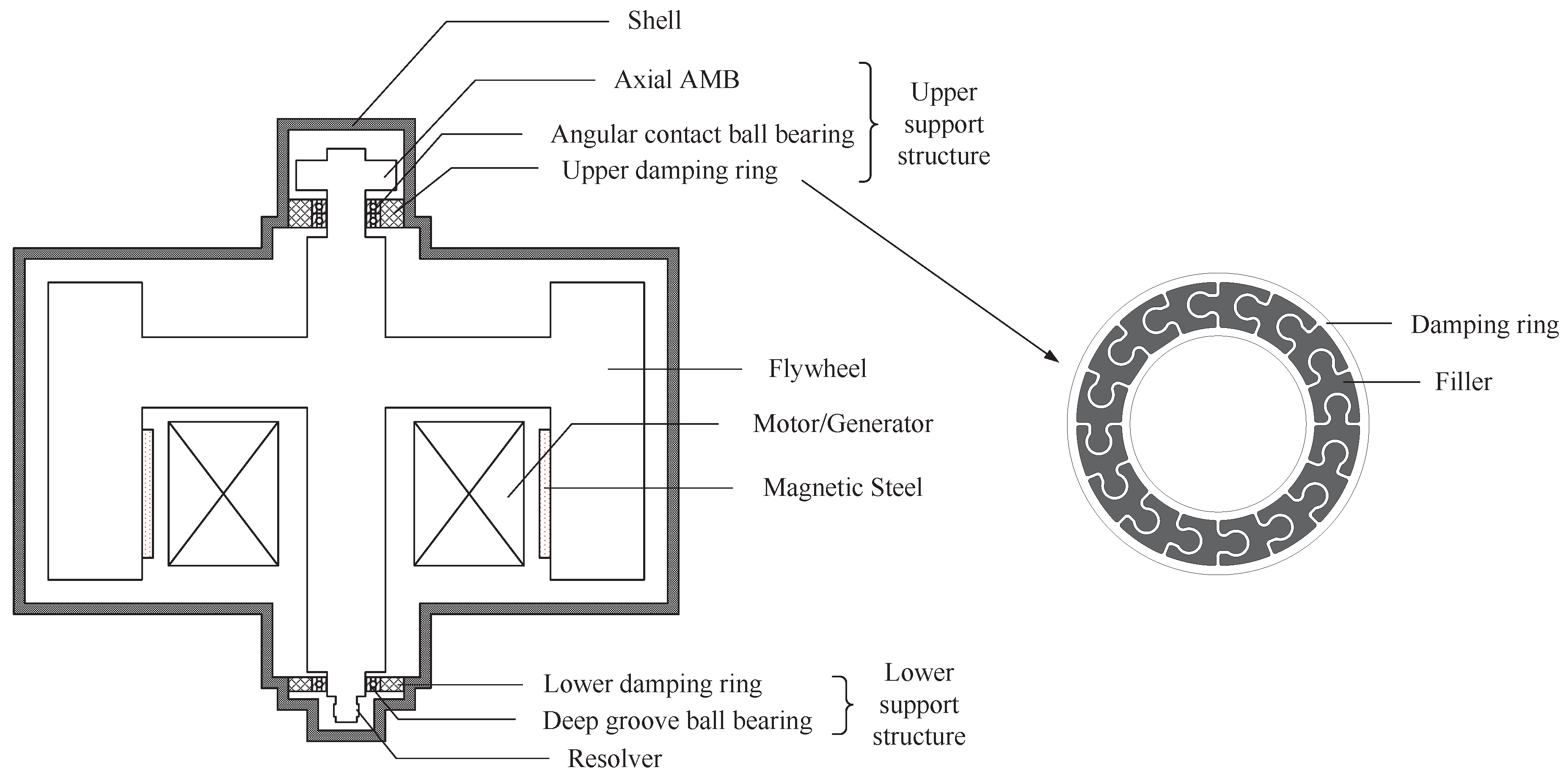
2.1. Structure Introduction of FESS
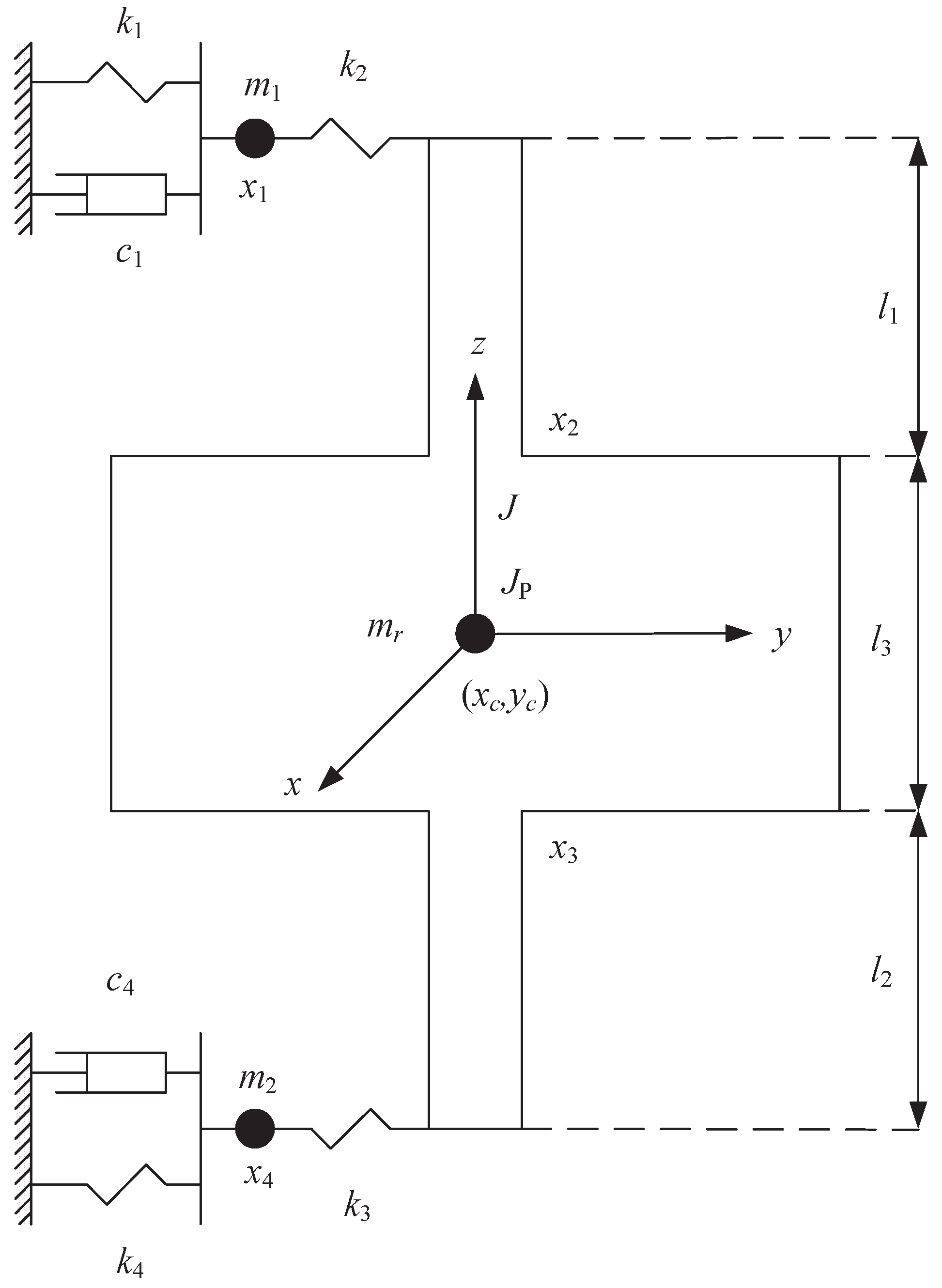
2.2. Dynamics Modeling of FESS
2.3. Method of Free Vibration Attenuation
3. Analysis of Dynamic Characteristics
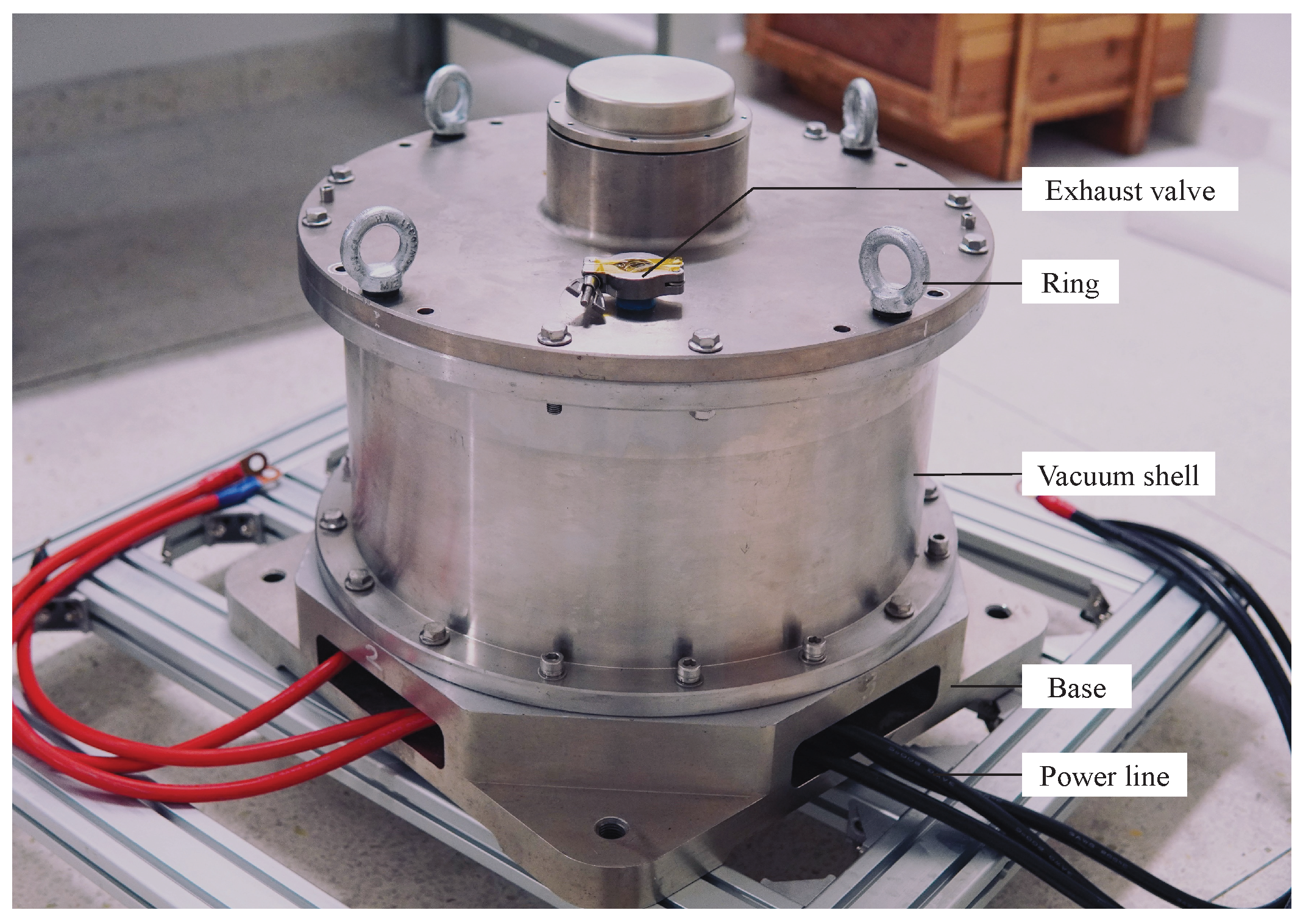
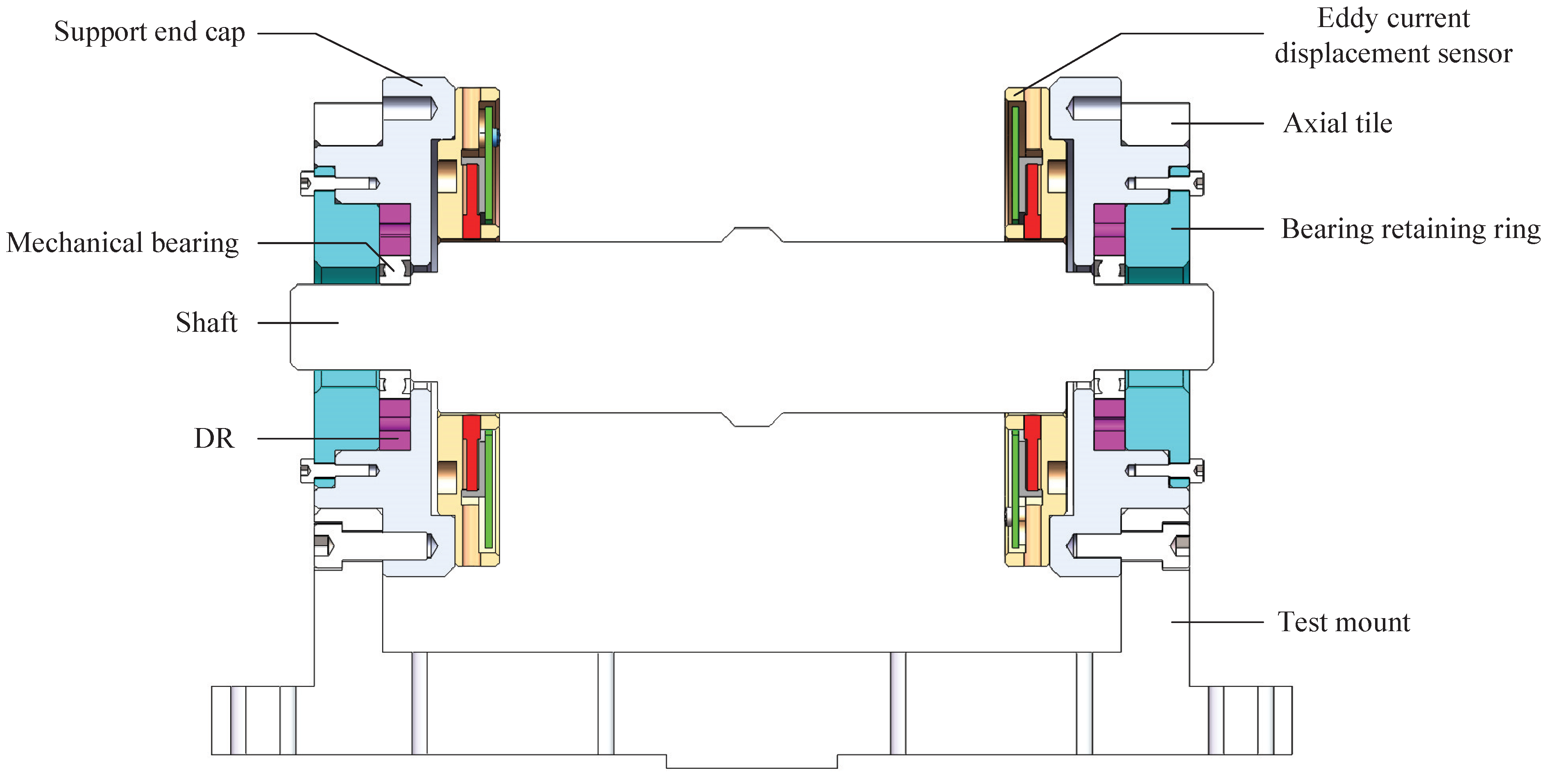
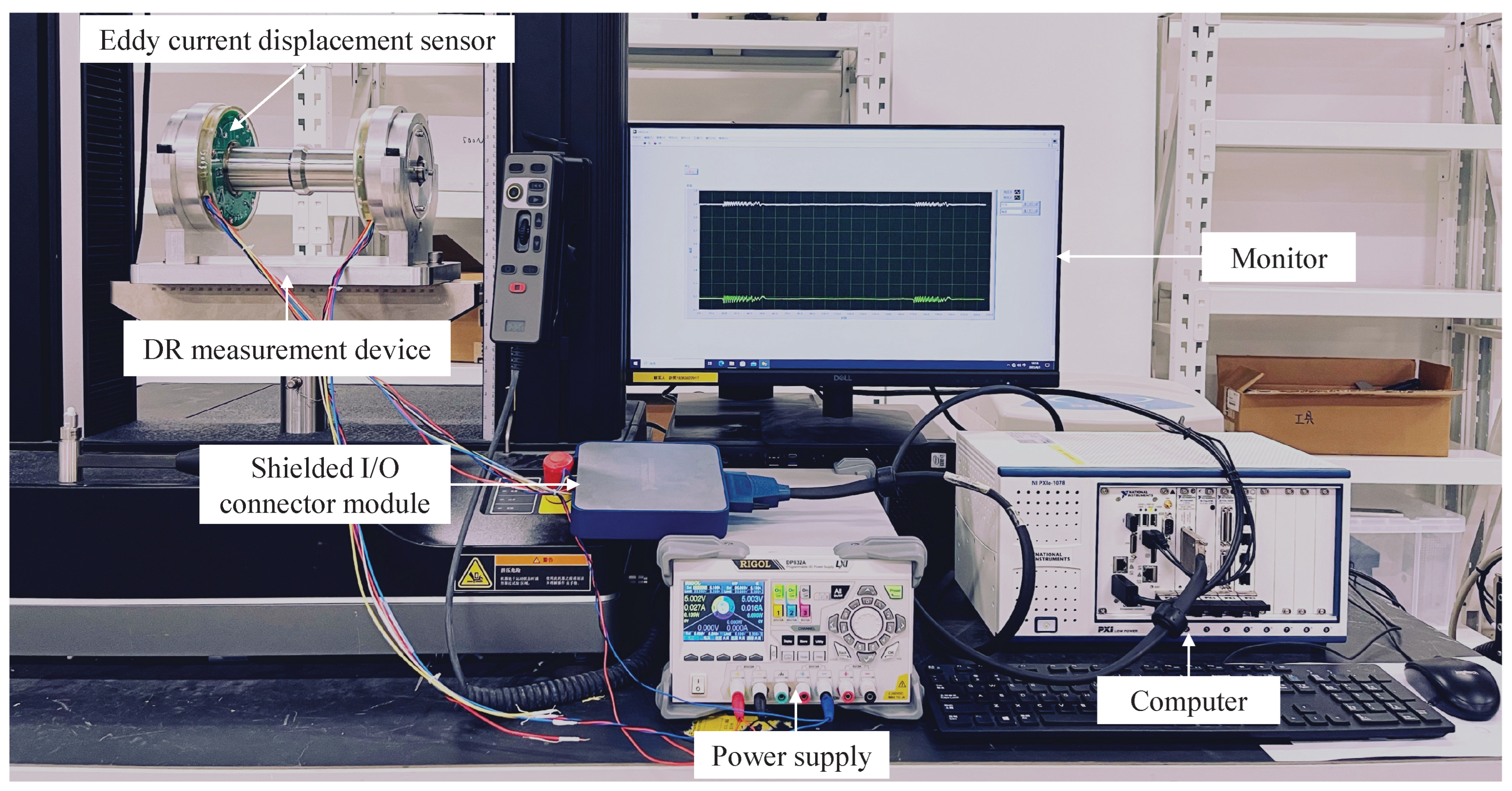
3.1. Materials and Methods
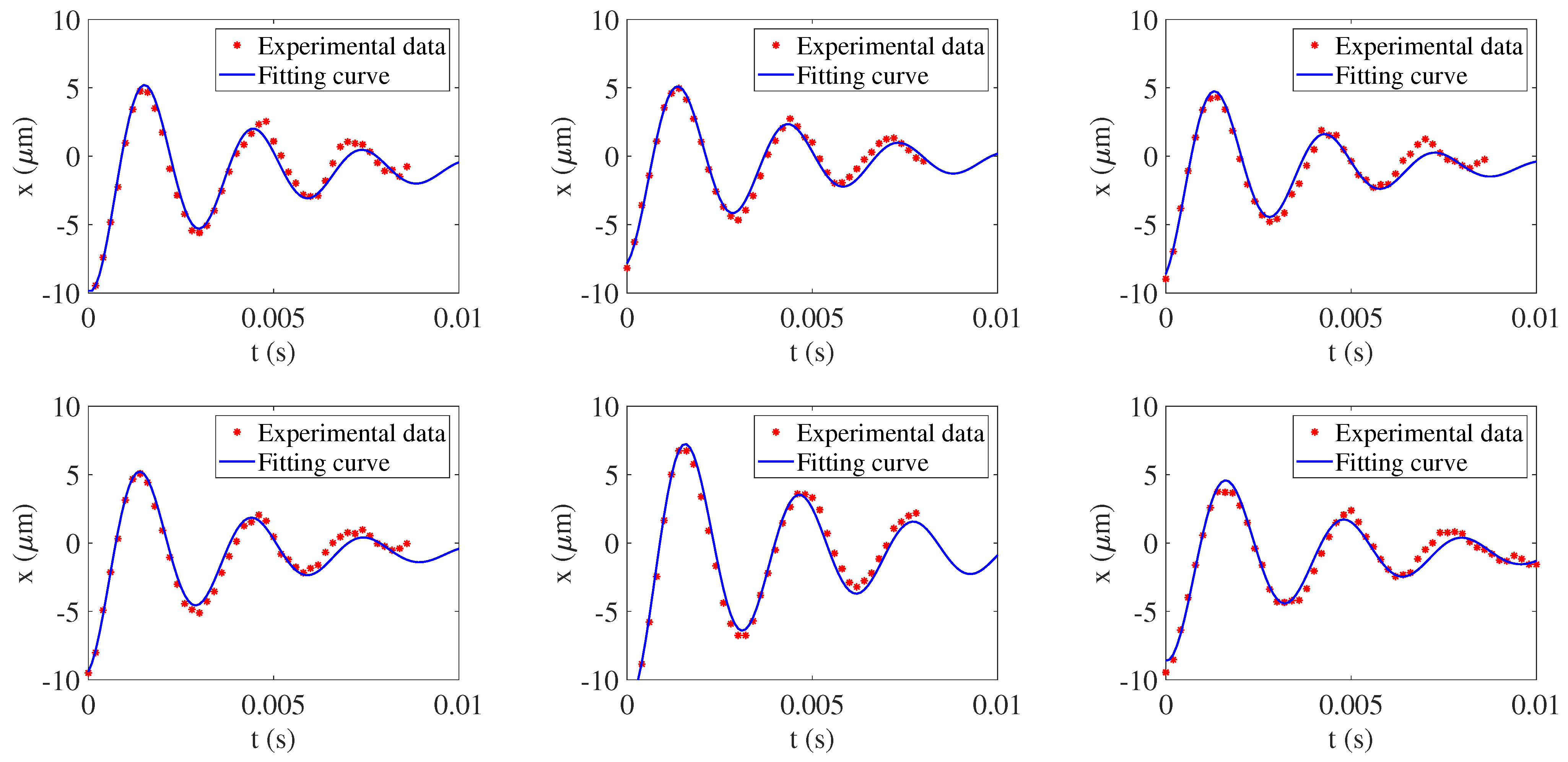
3.2. Stiffness and Damping Experiment of DR
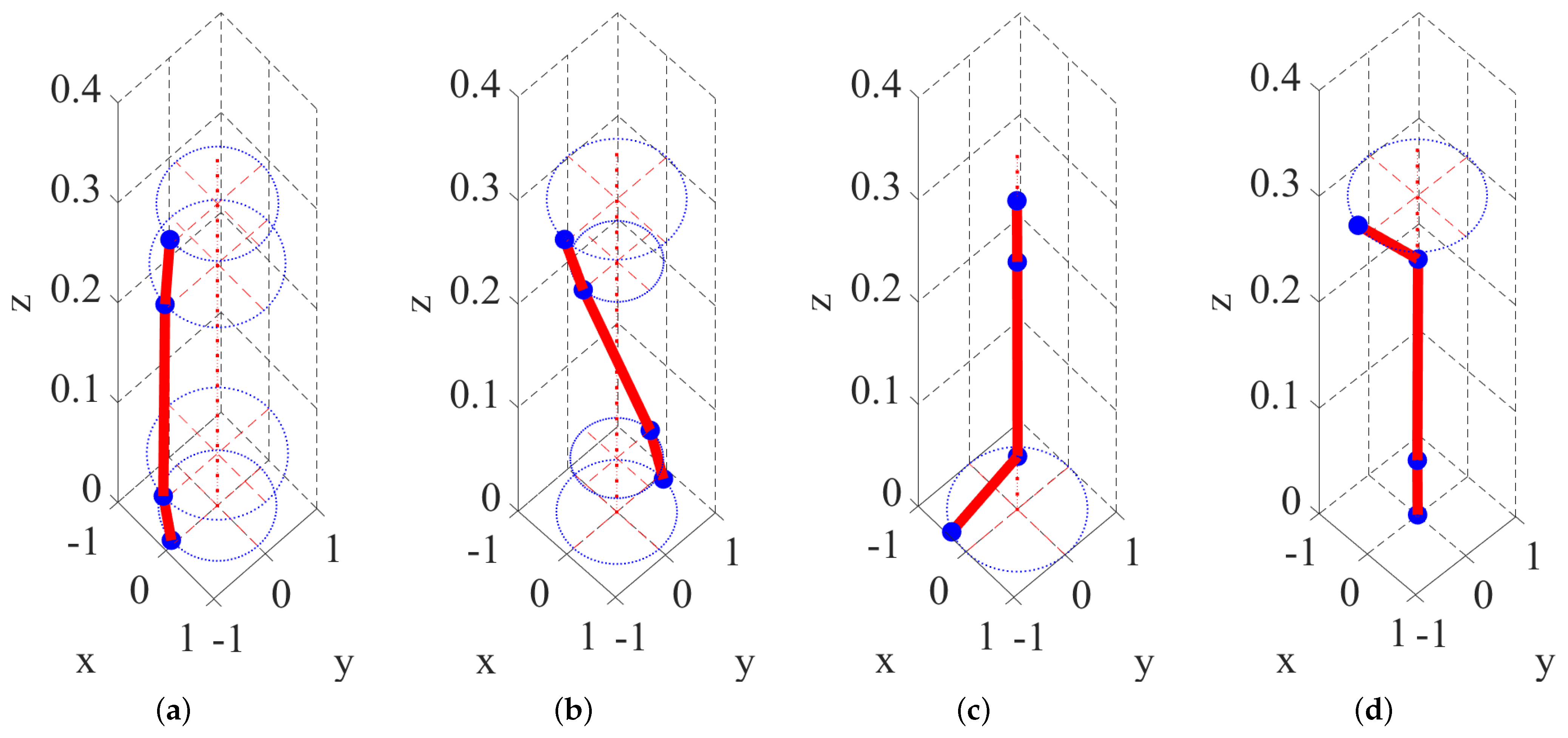
3.3. Dynamics of the Rotor–Bearing System
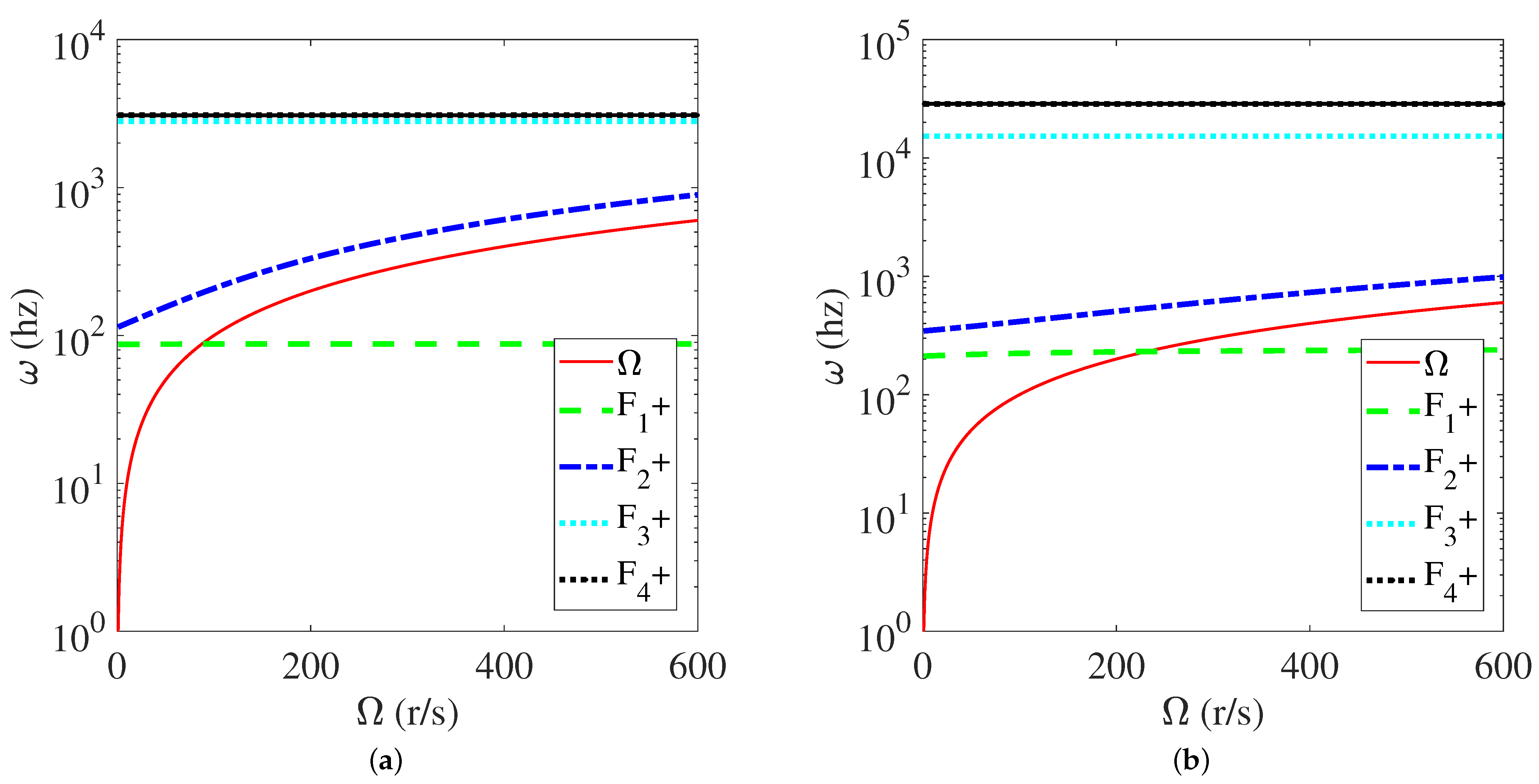
3.4. Critical Speed
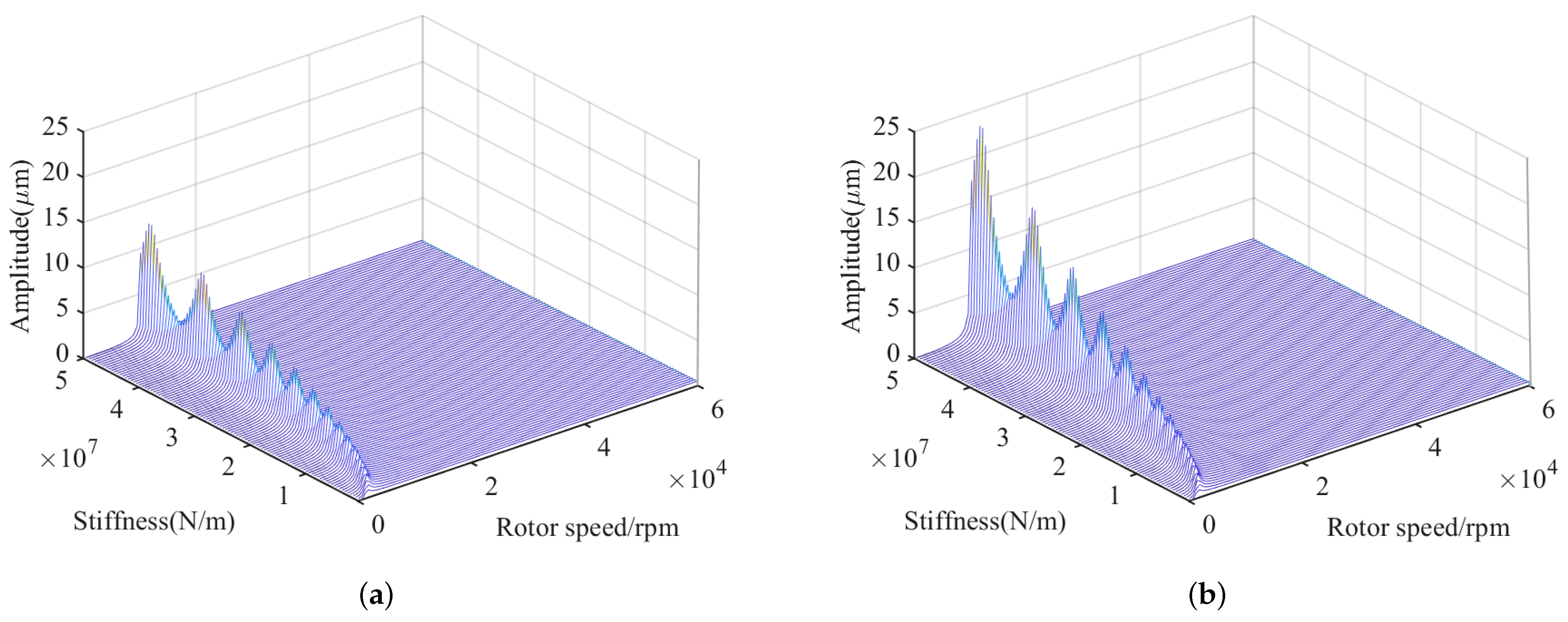
3.5. Unbalanced Response
3.6. Comparison with FESS of Mechanical Bearings
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| FESS | Flywheel energy storage system |
| PMB | Permanent magnet bearing |
| AMB | Active magnetic bearing |
| SMB | Superconducting magnetic bearing |
| REB | Rolling element bearing |
| DR | Damping ring |
| Mass of the flywheel rotor | |
| Mass of the upper damping ring after filling | |
| Mass of the lower damping ring after filling | |
| Stiffness of upper damping ring | |
| Stiffness of the upper mechanical bearing | |
| Stiffness of the lower mechanical bearing | |
| Stiffness of lower damping ring | |
| Damping coefficient of upper damping ring | |
| Damping coefficient of lower damping ring | |
| Polar moment inertia of the flywheel rotor | |
| J | Diameter moment inertia of the flywheel rotor |
| Length from upper mechanical bearing to upper end of flywheel | |
| Length from lower mechanical bearing to lower end of flywheel | |
| Length between the upper and lower ends of the flywheel | |
| Rotating speed of the rotor | |
| Modal frequency or exciting frequency | |
| x | Vibration in the direction of x-axis |
| y | Vibration in the direction of y-axis |
References
- Naderi, E.; Bibek, K.C.; Ansari, M.; Asrari, A. Experimental validation of a hybrid storage framework to cope with fluctuating power of hybrid renewable energy-based systems. IEEE Trans. Energy Convers. 2021, 36, 1991–2001. [Google Scholar] [CrossRef]
- Ershad, N.; Mehrjardi, R.; Ehsani, M. High-performance 4WD electric powertrain with flywheel kinetic energy recovery. IEEE Trans. Power Electron. 2021, 36, 772–784. [Google Scholar] [CrossRef]
- Hu, H.; Liu, K.; Wang, H.; Wei, J. A wide bandwidth GaN switching power amplifier of active magnetic bearing for a flywheel energy storage system. IEEE Trans. Power Electron. 2023, 38, 2589–2605. [Google Scholar] [CrossRef]
- Masouleh, M.; Limebeer, D. Fuel minimization for a vehicle equipped with a flywheel and battery on a three-dimensional track. IEEE Trans. Intell. Veh. 2017, 2, 161–174. [Google Scholar] [CrossRef]
- Hu, H.; Wei, J.; Wang, H.; Xiao, P.; Zeng, Y.; Liu, K. Analysis of the Notch Filter Insertion Position for Natural Frequency Vibration Suppression in a Magnetic Suspended Flywheel Energy Storage System. Actuators 2023, 12, 22. [Google Scholar] [CrossRef]
- Haidl, P.; Buchroithner, A. Design of a Low-Loss, Low-Cost Rolling Element Bearing System for a 5 kWh/100 kW Flywheel Energy Storage System. Energies 2021, 14, 7195. [Google Scholar] [CrossRef]
- Buchroithner, A.; Brandstatter, A.; Recheis, M. Mobile Flywheel Energy Storage Systems: Determining Rolling Element Bearing Loads to Expand Possibilities. IEEE Veh. Technol. Mag. 2017, 12, 83–94. [Google Scholar] [CrossRef]
- Cole, M.; Hawkins, L. Model-Based Analysis of Friction-Induced Subsynchronous Whirl for a Rotor Contacting with Clearance Bearings Under Axial Load. ASME J. Eng. Gas Turbines Power 2016, 138, 072507. [Google Scholar] [CrossRef]
- Ginzinger, L.; Ulbrich, H. Simulation-Based Controller Design for an Active Auxiliary Bearing. J. Syst. Des. Dyn. 2009, 3, 607–616. [Google Scholar] [CrossRef][Green Version]
- Qiu, Y.; Jiang, S. Suppression of low-frequency vibration for rotor-bearing system of flywheel energy storage system. Mech. Syst. Signal Process. 2019, 121, 496–508. [Google Scholar] [CrossRef]
- Qiu, Y.; Jiang, S. Dynamics of Flywheel Energy Storage System with Permanent Magnetic Bearing and Spiral Groove Bearing. J. Dyn. Syst. Meas. Control 2018, 140, 021006. [Google Scholar] [CrossRef]
- Xiang, B.; Liu, H. Dynamic Vibration Absorbing Performance of 5-DoF Magnetically Suspended Momentum Wheel Based on Damping Regulation. Actuators 2023, 12, 152. [Google Scholar] [CrossRef]
- Hopf, T.; Richter, M.; Schüßler, B.; Rinderknecht, S. Control Strategies for Highly Gyroscopic Outer Rotors with Diametral Enlargement in Active Magnetic Bearings. Actuators 2022, 11, 91. [Google Scholar] [CrossRef]
- Lee, H.; Jung, S.; Cho, Y. Peak Power Reduction and Energy Efficiency Improvement with the Superconducting Flywheel Energy Storage in Electric Railway System. Phys. C Supercond. 2013, 494, 246–249. [Google Scholar] [CrossRef]
- Komori, M.; Kato, H.; Asami, K.-I. Suspension-Type of Flywheel Energy Storage System Using High Tc Superconducting Magnetic Bearing (SMB). Actuators 2022, 11, 215. [Google Scholar] [CrossRef]
- Breńkacz, Ł.; Witanowski, Ł.; Drosińska-Komor, M.; Szewczuk-Krypa, N. Research and applications of active bearings: A state-of-the-art review. Mech. Syst. Signal Process. 2021, 151, 107423. [Google Scholar] [CrossRef]
- Fang, J.; Tang, E.; Zheng, S. Optimum Damping Control of the Flexible Rotor in High Energy Density Magnetically Suspended Motor. ASME J. Eng. Gas Turbines Power 2015, 137, 082505. [Google Scholar] [CrossRef]
- Sato, D.; Itoh, J.; Watanabe, T.; Kawagoe, K.; Yamada, N.; Kato, K. 2015 Development of magnetic coupling with variable thrust structure for flywheel energy storage system in long lifetime UPS. In Proceedings of the IEEE International Telecommunications Energy Conference (INTELEC), Osaka, Japan, 18–22 October 2015. [Google Scholar]
- Yang, D.; Qin, H.; Xie, X.; Zhang, Z. Suppression of lateral vibration in a shafting system via an auxiliary active magnetic bearing with position control. Proc. Inst. Mech. Eng. C J. Mech. Eng. Sci. 2023, 237, 1581–1594. [Google Scholar] [CrossRef]
- Recheis, M.; Buchroithner, A.; Andrasec, I.; Gallien, T.; Schweighofer, B.; Bader, M.; Wegleiter, H. Improving kinetic energy storage for vehicles through the combination of rolling element and active magnetic bearings. In Proceedings of the IECON 2013-39th Annual Conference of the IEEE Industrial Electronics Society, Vienna, Austria, 10–13 November 2013. [Google Scholar]
- Xu, K.; Guo, Y.; Lei, G.; Zhu, J. A Review of Flywheel Energy Storage System Technologies. Energies 2023, 16, 6462. [Google Scholar] [CrossRef]
- Kang, X.; Palazzolo, A. Simulation, Test, and Mitigation of ½× Forward Whirl Following Rotor Drop Onto Auxiliary Bearings. ASME J. Eng. Gas Turbines Power 2020, 142, 041020. [Google Scholar] [CrossRef]
- Haidl, P.; Zisser, M.; Buchroithner, A.; Schweighofer, B.; Wegleiter, H.; Bader, M. Improved Test Rig Design for Vibration Control of a Rotor Bearing System. In Proceedings of the 23rd International Congress on Sound and Vibration, Athens, Greece, 10–14 July 2016. [Google Scholar]
- Buchroithner, A.; Haan, A.; Preßmair, R.; Bader, M.; Schweighofer, B.; Wegleiter, H.; Edtmayer, H. Decentralized low-cost flywheel energy storage for photovoltaic systems. In Proceedings of the 2016 International Conference on Sustainable Energy Engineering and Application (ICSEEA), Jakarta, Indonesia, 3–5 October 2016. [Google Scholar]
- Jiang, S.; Wang, H.; Wen, S. Flywheel Energy Storage System with a Permanent Magnet Bearing and a Pair of Hybrid Ceramic Ball Bearings. J. Mech. Sci. Technol. 2014, 28, 5043–5053. [Google Scholar] [CrossRef]
- Wang, J. Vibration Measurement and Analysis of Energy Storage Flywheel System. Master’s Thesis, Tsinghua University, Beijing, China, 2008; pp. 18–19. [Google Scholar]
- Cheng, D. Mechanical Design Manual, 6th ed.; Chemical Industry Press: Beijing, China, 2017; Volume 2, p. 31. [Google Scholar]






| Item | Value | Item | Value | Item | Value | Item | Value |
|---|---|---|---|---|---|---|---|
| (kg) | 41.34 | (kg) | 0.198 | (kg) | 0.117 | J (kg · ) | 0.566 |
| (kg · ) | 0.835 | (m) | 0.06 | (m) | 0.052 | (m) | 0.19 |
| (N/m) | (N/m) | – | – | – | – |
| Number | SSE * | c (N · s/m) | k (N/m) | ||||
|---|---|---|---|---|---|---|---|
| 1 | 15.97 | 0.9673 | 246.9 | 2143 | 1655 | 7.7975 × | 0.1152 |
| 2 | 12.63 | 0.9672 | 240.6 | 1974 | 1612 | 6.6265 × | 0.1219 |
| 3 | 8.436 | 0.9743 | 237.4 | 2113 | 1591 | 7.5758 × | 0.1124 |
| 4 | 11.01 | 0.9657 | 264 | 2122 | 1769 | 7.662 × | 0.1244 |
| 5 | 12.23 | 0.9686 | 279.5 | 2082 | 1837 | 7.3944 × | 0.1342 |
| 6 | 20.74 | 0.979 | 224 | 2017 | 1501 | 6.9011 × | 0.1111 |
| Item | Value | Item | Value |
|---|---|---|---|
| (N/m) | 7.29 × | (N · s/m) | 1.66 × |
| (N/m) | 7.29 × | (N · s/m) | 1.66 × |
| Item | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
| REB * (0 rpm) | 211.57 Hz | 344.91 Hz | 15,285 Hz | 28,658 Hz |
| REB (12,000 rpm) | 229.91 Hz | 506.48 Hz | 15,285 Hz | 28,658 Hz |
| (0 rpm) | 87.14 Hz | 113.37 Hz | 2808 Hz | 3082 Hz |
| REB + DR (12,000 rpm) | 87.56 Hz | 331.76 Hz | 2808 Hz | 3083 Hz |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, M.; Liu, K.; Wei, J.; Hou, E.; Liu, D.; Zhao, X. Dynamics Study of Hybrid Support Flywheel Energy Storage System with Damping Ring Device. Actuators 2024, 13, 532. https://doi.org/10.3390/act13120532
Hu M, Liu K, Wei J, Hou E, Liu D, Zhao X. Dynamics Study of Hybrid Support Flywheel Energy Storage System with Damping Ring Device. Actuators. 2024; 13(12):532. https://doi.org/10.3390/act13120532
Chicago/Turabian StyleHu, Mingming, Kun Liu, Jingbo Wei, Eryong Hou, Duhe Liu, and Xi Zhao. 2024. "Dynamics Study of Hybrid Support Flywheel Energy Storage System with Damping Ring Device" Actuators 13, no. 12: 532. https://doi.org/10.3390/act13120532
APA StyleHu, M., Liu, K., Wei, J., Hou, E., Liu, D., & Zhao, X. (2024). Dynamics Study of Hybrid Support Flywheel Energy Storage System with Damping Ring Device. Actuators, 13(12), 532. https://doi.org/10.3390/act13120532






