Experimental Verification of a Two-Motor-Two-Pump Motor-Controlled Hydraulic Cylinder with Throttle-Free Passive Load-Holding Capability in Four-Quadrant Operations
Abstract
1. Introduction
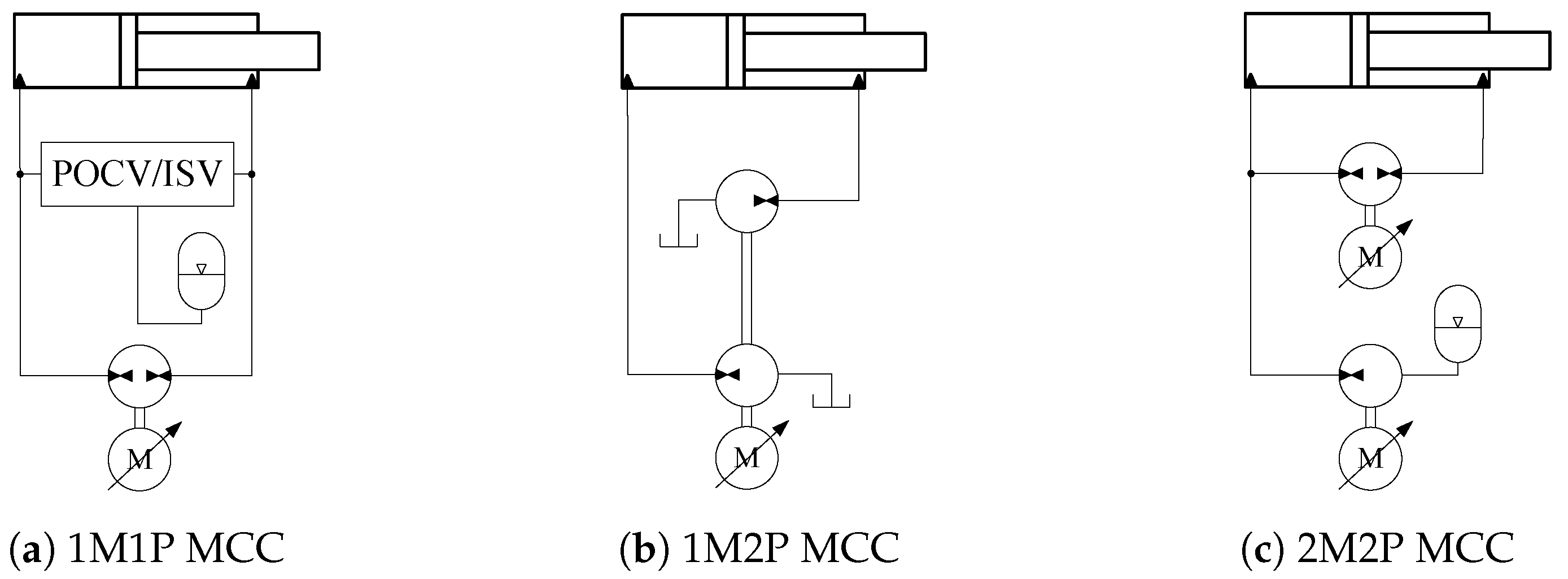
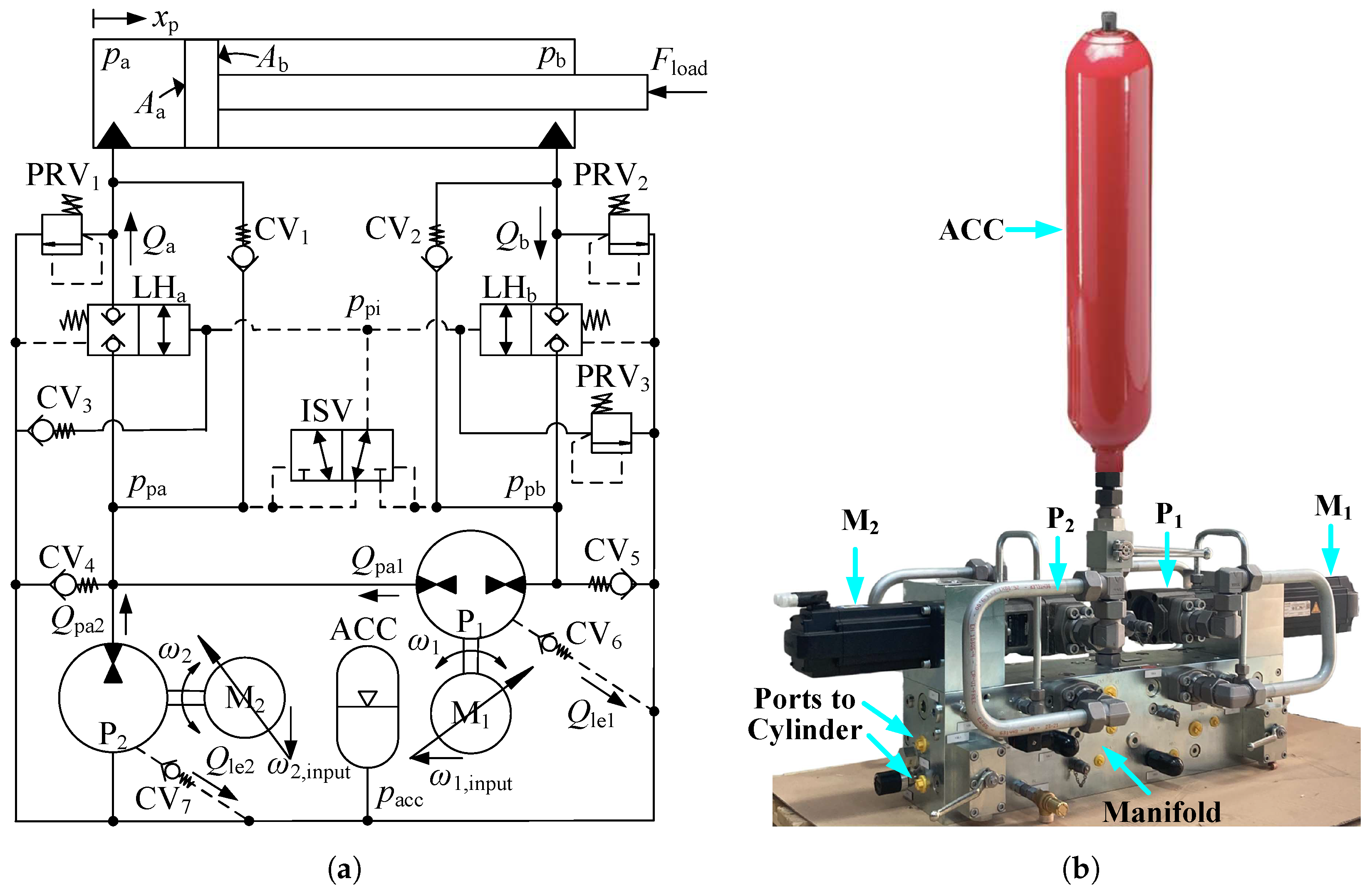
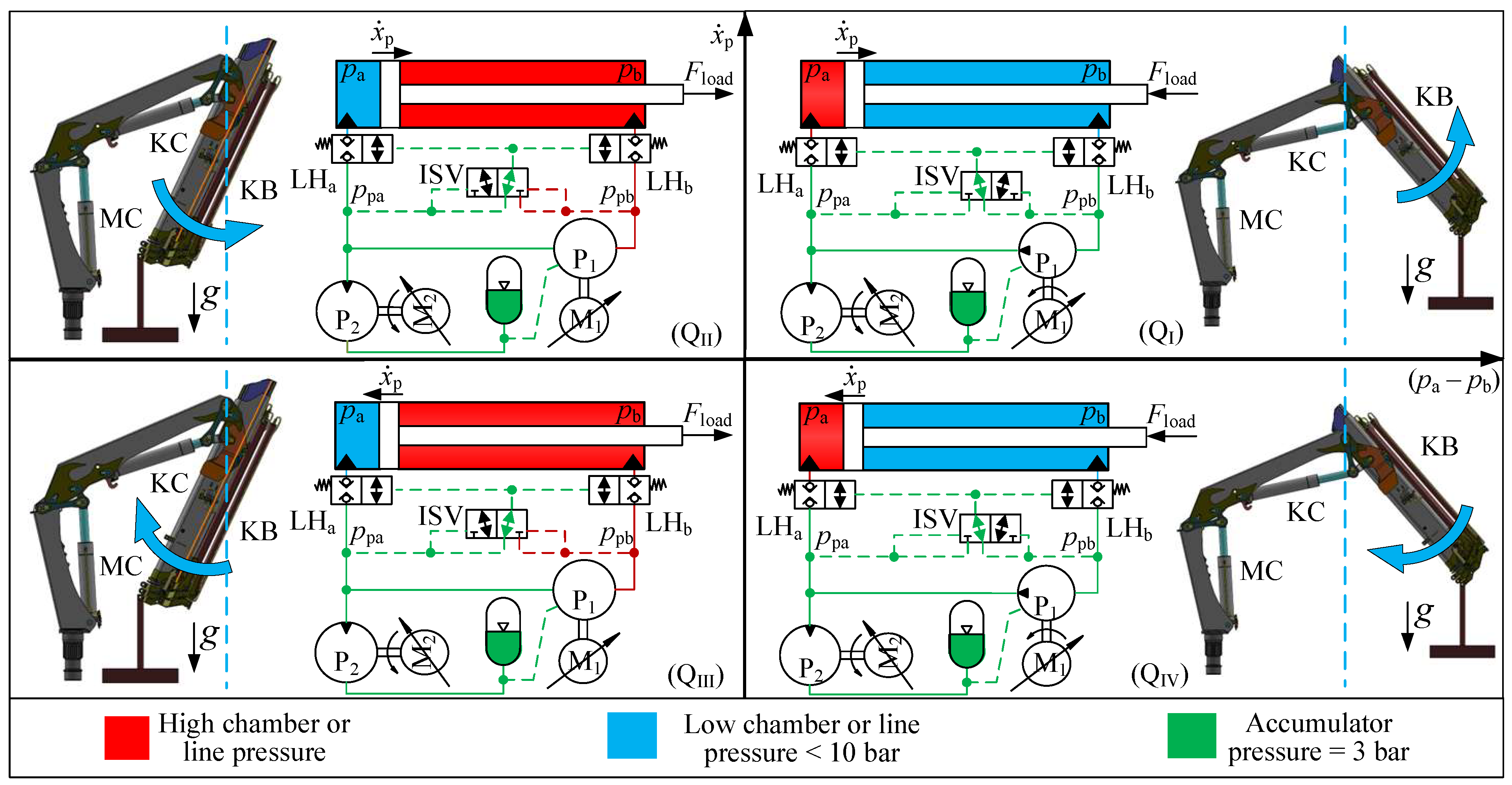
2. Proposed System
3. Experimental Analysis
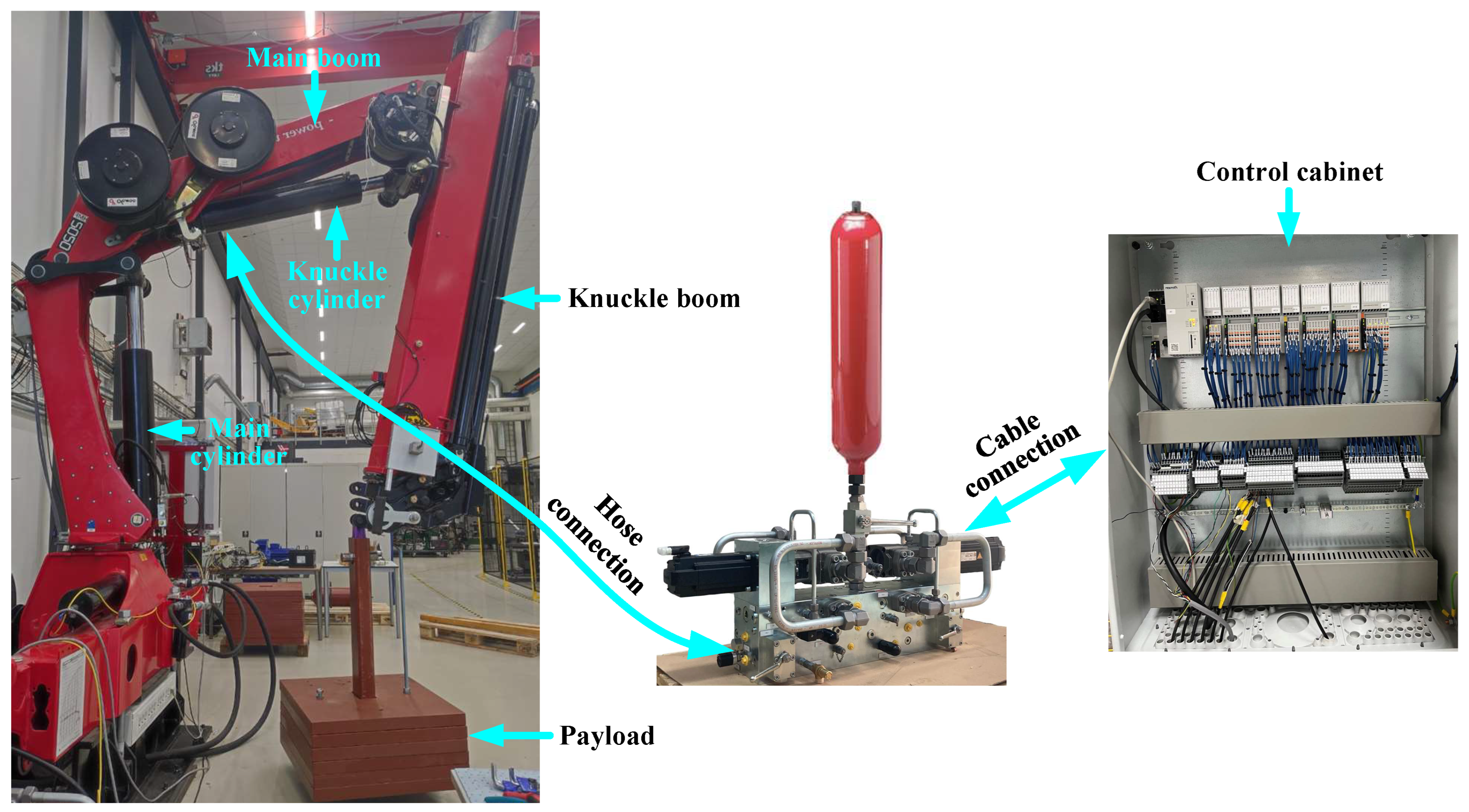
3.1. Experimental Setup
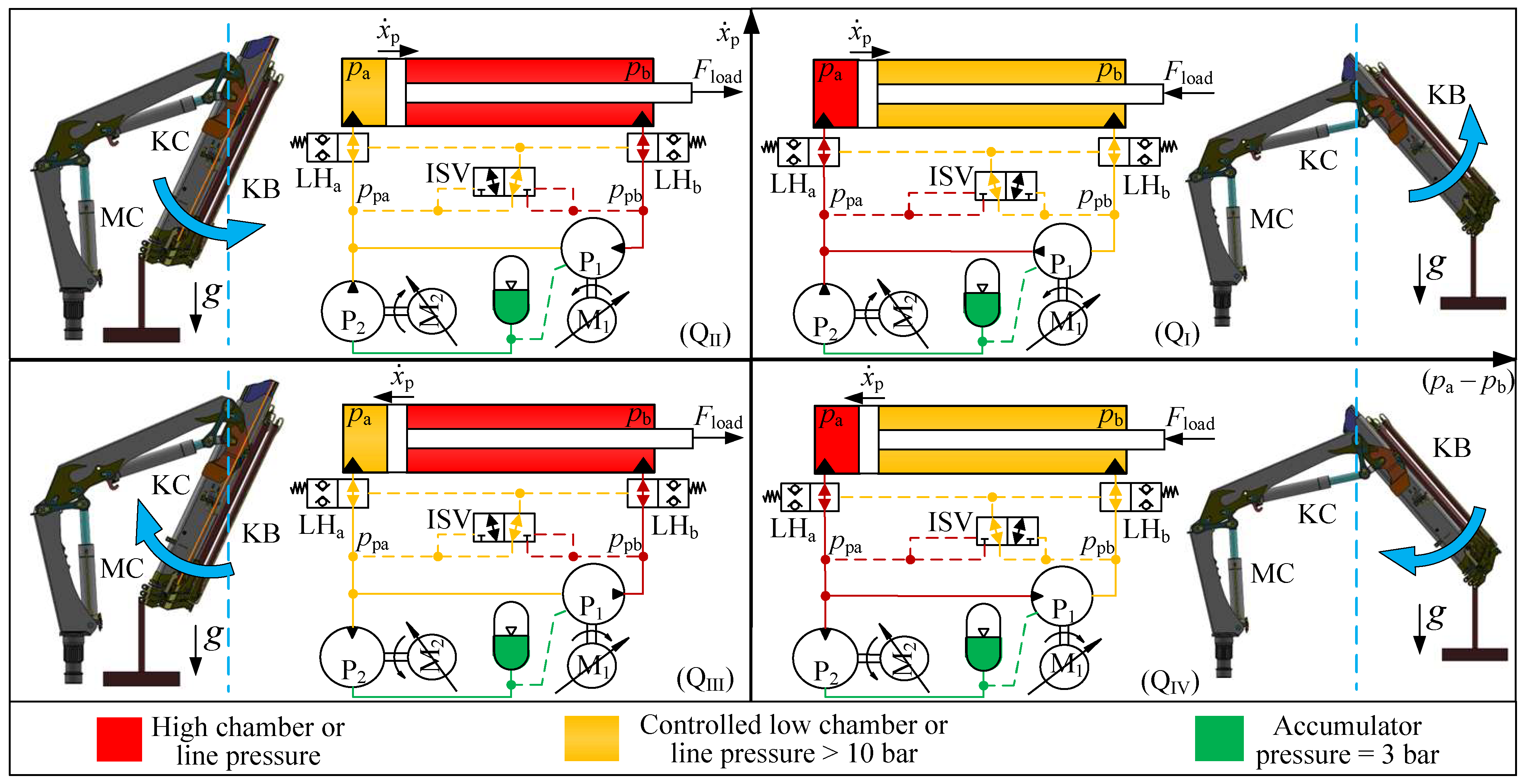
3.2. Four-Quadrant Operation Analysis
3.3. Passive Load-Holding Function in Four Quadrants
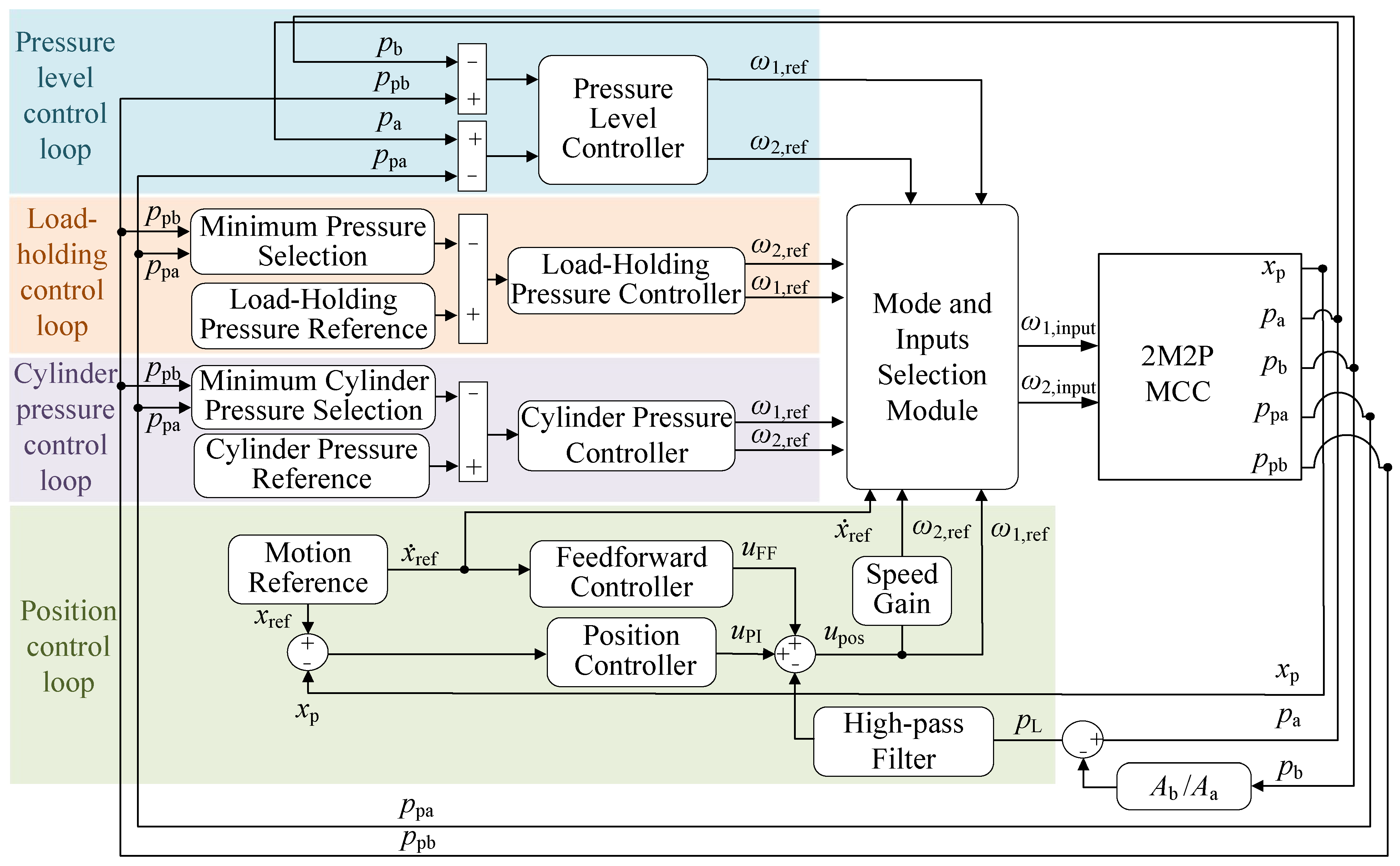
4. Control Algorithm
5. Results of Experimental Work
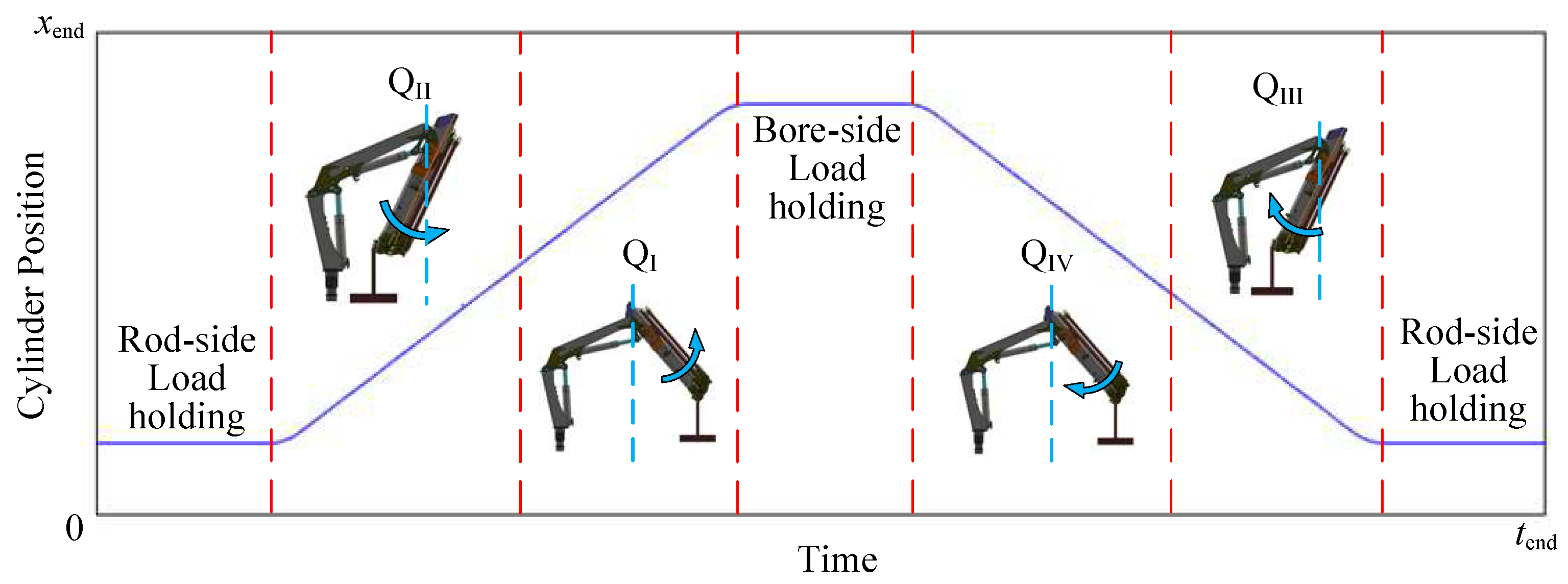
5.1. Reference Signals and Experiment Procedures
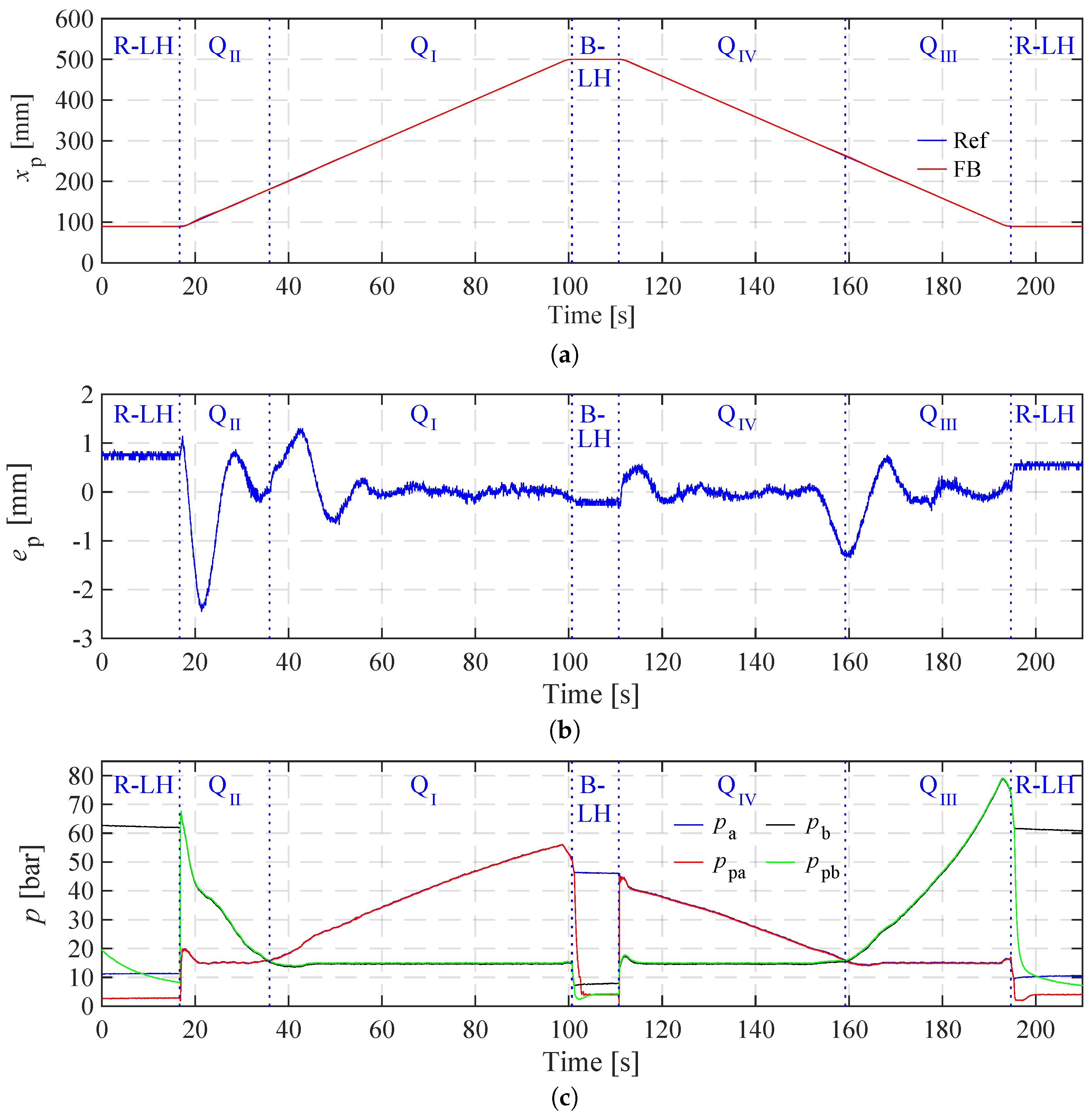
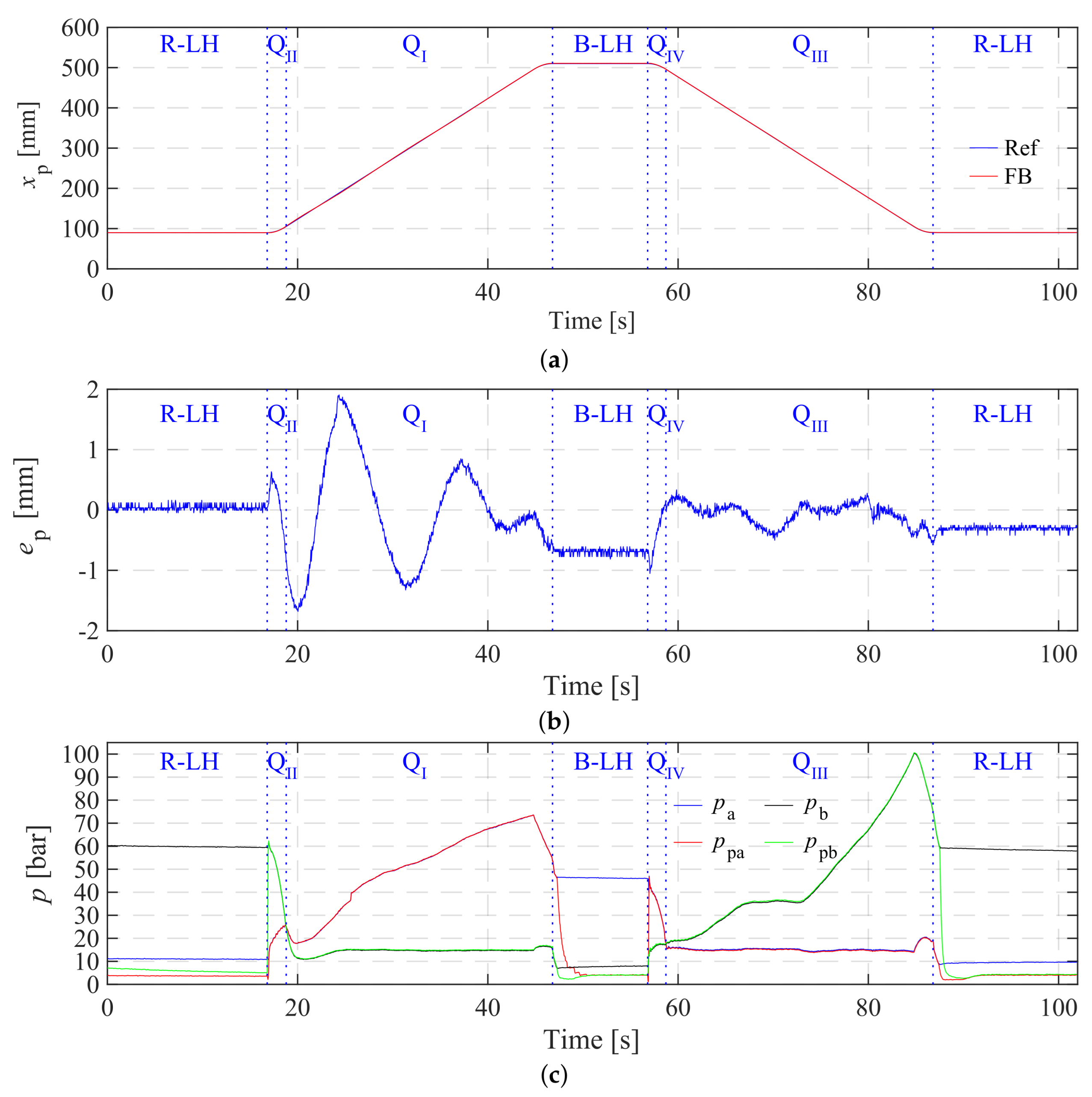
5.2. Case One
5.3. Case Two
5.4. Case Three
6. Discussion
7. Conclusions
- The system status and component motions of the proposed 2M2P MCC are comprehensively analyzed.
- A specialized experimental setup is constructed, comprising a knuckle boom crane and a 2M2P MCC prototype.
- A position reference involving four-quadrant operation and two types of load holding is developed.
- Experiments are conducted across three cases, with satisfactory position tracking performance and seamless pressure intersection during transitions between different quadrants of operation.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zimmerman, J.D.; Pelosi, M.; Williamson, C.A.; Ivantysynova, M. Energy Consumption of an LS Excavator Hydraulic System. In Proceedings of the ASME 2007 International Mechanical Engineering Congress and Exposition, Seattle, WA, USA, 11–15 November 2007; pp. 117–126. [Google Scholar]
- Quan, Z.; Quan, L.; Zhang, J. Review of energy efficient direct pump controlled cylinder electro-hydraulic technology. Renew. Sustain. Energy Rev. 2014, 35, 336–346. [Google Scholar] [CrossRef]
- Ketelsen, S.; Padovani, D.; Andersen, T.O.; Ebbesen, M.K.; Schmidt, L. Classification and Review of Pump-Controlled Differential Cylinder Drives. Energies 2019, 12, 1293. [Google Scholar] [CrossRef]
- Fassbender, D.; Zakharov, V.; Minav, T. Utilization of electric prime movers in hydraulic heavy-duty-mobile-machine implement systems. Autom. Constr. 2021, 132, 103964. [Google Scholar] [CrossRef]
- Zhao, W.; Ebbesen, M.K.; Andersen, T.O. Identifying The Future Research Trend for Using Speed-Controlled Hydraulic Cylinders in Offshore Applications through Literature Survey. In Proceedings of the 2022 IEEE Global Fluid Power Society PhD Symposium (Presented), Naples, Italy, 12–14 October 2022. [Google Scholar]
- Ivantysynova, M. The swash plate machine for displacement control unit with great development potentiality. In Proceedings of the First IFK, Aachen, Germany, 18–23 May 1998. [Google Scholar]
- Rahmfeld, R.; Ivantysynova, M. Displacement controlled linear actuator with differential cylinder—A way to save primary energy in mobile machines. In Proceedings of the Fifth International Conference on Fluid Power Transmission and Control, Hangzhou, China, 3–5 April 2001. [Google Scholar]
- Rahmfeld, R.; Ivantysynova, M. Energy Saving Hydraulic Displacement Controlled Linear Actuators in Industry Applications and Mobile Machine Systems. In Proceedings of the Fourth International Symposium on Linear Drives for Industry Applications, Birmingham, UK, 8–10 September 2003. [Google Scholar]
- Zimmerman, J.; Busquets, E.; Ivantysynova, M. 40% fuel savings by displacement control leads to lower working temperatures—A simulation study and measurements. In Proceedings of the IFPE, International Exposition for Power Transmission and Technical Conference, 2011, the 52nd NCFP, National Conference on Fluid Power, Las Vegas, NV, USA, 23–25 March 2011; National Fluid Power Association: Milwaukee, WI, USA, 2011; p. 1081. [Google Scholar]
- Helduser, S. Electric-hydrostatic drive—An innovative energy-saving power and motion control system. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 1999, 213, 427–437. [Google Scholar] [CrossRef]
- Rydberg, K.E. Energy Efficient Hydraulics—System solutions for loss minimization. In Proceedings of the National Conference on Fluid Power, Linköping, Sweden, 14–16 December 2015. [Google Scholar]
- Hagen, D.; Padovani, D.; Choux, M. A comparison study of a novel self-contained electro-hydraulic cylinder versus a conventional valve-controlled actuator—Part 2: Energy efficiency. Actuators 2019, 8, 78. [Google Scholar] [CrossRef]
- Hagen, D.; Padovani, D.; Choux, M. A Comparison Study of a Novel Self-Contained Electro-Hydraulic Cylinder versus a Conventional Valve-Controlled Actuator—Part 1: Motion Control. Actuators 2019, 8, 79. [Google Scholar] [CrossRef]
- Zhang, S.; Minav, T.; Pietola, M. Decentralized Hydraulics for Micro Excavator. In Proceedings of the 15th Scandinavian International Conference on Fluid Power, Linköping, Sweden, 7–9 June 2017; pp. 187–195. [Google Scholar]
- Ketelsen, S.; Schmidt, L.; Donkov, V.H.; Andersen, T.O. Energy saving potential in knuckle boom cranes using a novel pump controlled cylinder drive. Model. Identif. Control (Online) 2018, 39, 73–89. [Google Scholar] [CrossRef]
- Zhao, W.; Bhola, M. Comparing Compact and Remote Deployments of a Speed-Controlled Cylinder Drive Unit on an Offshore Knuckle Boom Crane. In Proceedings of the 18th Scandinavian International Conference on Fluid Power, Tampere, Finland, 30 May–1 June 2023; pp. 518–533. [Google Scholar]
- Williamson, C.; Ivantysynova, M. Pump Mode Prediction for Four-Quadrant Velocity Control of Valueless Hydraulic Actuators. In Proceedings of the JFPS International Symposium on Fluid Power, Toyama, Japan, 15–18 September 2008; pp. 323–328. [Google Scholar]
- Zhao, W.; Ebbesen, M.K.; Hansen, M.R.; Andersen, T.O. Enabling Passive Load-Holding Function and System Pressures Control in a One-Motor-One-Pump Motor-Controlled Hydraulic Cylinder: Simulation Study. Energies 2024, 17, 2484. [Google Scholar] [CrossRef]
- Kärnell, S.; Ericson, L. Control of an Asymmetric Cylinder with Two Individually Controlled Pump/Motors. In Proceedings of the ASME/BATH 2023 Symposium on Fluid Power and Motion Control, Sarasota, FL, USA, 16–18 October 2023. [Google Scholar]
- Helduser, S. Electric-Hydrostatic Drive Systems and Their Application in Injection Moulding Machines. Proc. JFPS Int. Symp. Fluid Power 1999, 1999, 261–266. [Google Scholar] [CrossRef][Green Version]
- Cho, S.H.; Räcklebe, S.; Helduser, S. Position tracking control of a clamp-cylinder for energy-saving injection moulding machines with electric-hydrostatic drives. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2009, 223, 479–491. [Google Scholar] [CrossRef]
- Hertz, R.A.; Therkelsen, O.; Kristiansen, S.; Christensen, J.K.; Helver, C.E.; Schmidt, L. Practical Implementation of Secondary Control Principles in an Electro-Hydraulic Speed-Variable Drive Applied to an Injection Moulding Machine. In Proceedings of the ASME/BATH 2023 Symposium on Fluid Power and Motion Control, Sarasota, FL, USA, 16–18 October 2023. [Google Scholar]
- Ketelsen, S.; Andersen, T.O.; Ebbesen, M.K.; Schmidt, L. A Self-Contained Cylinder Drive with Indirectly Controlled Hydraulic Lock. Model. Identif. Control. A Nor. Res. Bull. 2020, 41, 185–205. [Google Scholar] [CrossRef]
- Zhao, W.; Bhola, M.; Ebbesen, M.K.; Andersen, T.O. A Novel Control Design for Realizing Passive Load-Holding Function on a Two-Motor-Two-Pump Motor-Controlled Hydraulic Cylinder. Model. Identif. Control 2023, 44, 125–139. [Google Scholar] [CrossRef]

| Component | Manufacturer | Specifications |
|---|---|---|
| Electric Servo | Bosch Rexroth | = 16.3 Nm, = 2000 rpm |
| Electric Servo | Bosch Rexroth | = 7.2 Nm, = 2990 rpm |
| Axial Piston | Bosch Rexroth | = 6 cc/rev. |
| Axial Piston | Bosch Rexroth | = 3 cc/rev. |
| Shuttle valve | Bucher Hydraulics | = 350 bar, = 16 L/min |
| Load Holding Valve | Sun Hydraulics | = 345 bar, = 227 L/min, = 10 bar |
| Check Valve | Bosch Rexroth | = 420 bar, = 120 L/min, = 0.2 bar |
| Pressure Relief Valve | Bosch Rexroth | = 50 L/min, = 400 bar, = 200 or 80 bar |
| Hydraulic Cylinder | LJM Hydraulik | D = 65 mm, d = 43 mm, L = 400 mm |
| PLC | Bosch Rexroth | RAM 512 MB, SERCOS |
| Controllers | P | I |
|---|---|---|
| Position Controller | 30 rev/min/m | 0.08 rev/m |
| Cylinder Pressure Controller | 20 rev/min/bar | 0.08 rev/bar |
| Load-Holding Controller | 20 rev/min/bar | 0.07 rev/bar |
| Pressure Level Controller | 50 rev/min/bar (bore side) | 0 rev/bar (bore side) |
| Pressure Level Controller | 10 rev/min/bar (rod side) | 0 rev/bar (rod side) |
| Three Cases | Maximum Cylinder Speed | Payload on Knuckle Boom |
|---|---|---|
| Case one | 5 mm/s | 0 kg |
| Case two | 5 mm/s | 600 kg |
| Case three | 15 mm/s | 600 kg |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, W.; Ebbesen, M.K.; Hansen, M.R.; Andersen, T.O. Experimental Verification of a Two-Motor-Two-Pump Motor-Controlled Hydraulic Cylinder with Throttle-Free Passive Load-Holding Capability in Four-Quadrant Operations. Actuators 2024, 13, 372. https://doi.org/10.3390/act13090372
Zhao W, Ebbesen MK, Hansen MR, Andersen TO. Experimental Verification of a Two-Motor-Two-Pump Motor-Controlled Hydraulic Cylinder with Throttle-Free Passive Load-Holding Capability in Four-Quadrant Operations. Actuators. 2024; 13(9):372. https://doi.org/10.3390/act13090372
Chicago/Turabian StyleZhao, Wei, Morten Kjeld Ebbesen, Michael Rygaard Hansen, and Torben Ole Andersen. 2024. "Experimental Verification of a Two-Motor-Two-Pump Motor-Controlled Hydraulic Cylinder with Throttle-Free Passive Load-Holding Capability in Four-Quadrant Operations" Actuators 13, no. 9: 372. https://doi.org/10.3390/act13090372
APA StyleZhao, W., Ebbesen, M. K., Hansen, M. R., & Andersen, T. O. (2024). Experimental Verification of a Two-Motor-Two-Pump Motor-Controlled Hydraulic Cylinder with Throttle-Free Passive Load-Holding Capability in Four-Quadrant Operations. Actuators, 13(9), 372. https://doi.org/10.3390/act13090372








