Optimising Sodium Borohydride Reduction of Platinum onto Nafion-117 in the Electroless Plating of Ionic Polymer–Metal Composites
Abstract
1. Introduction
2. Materials and Methods
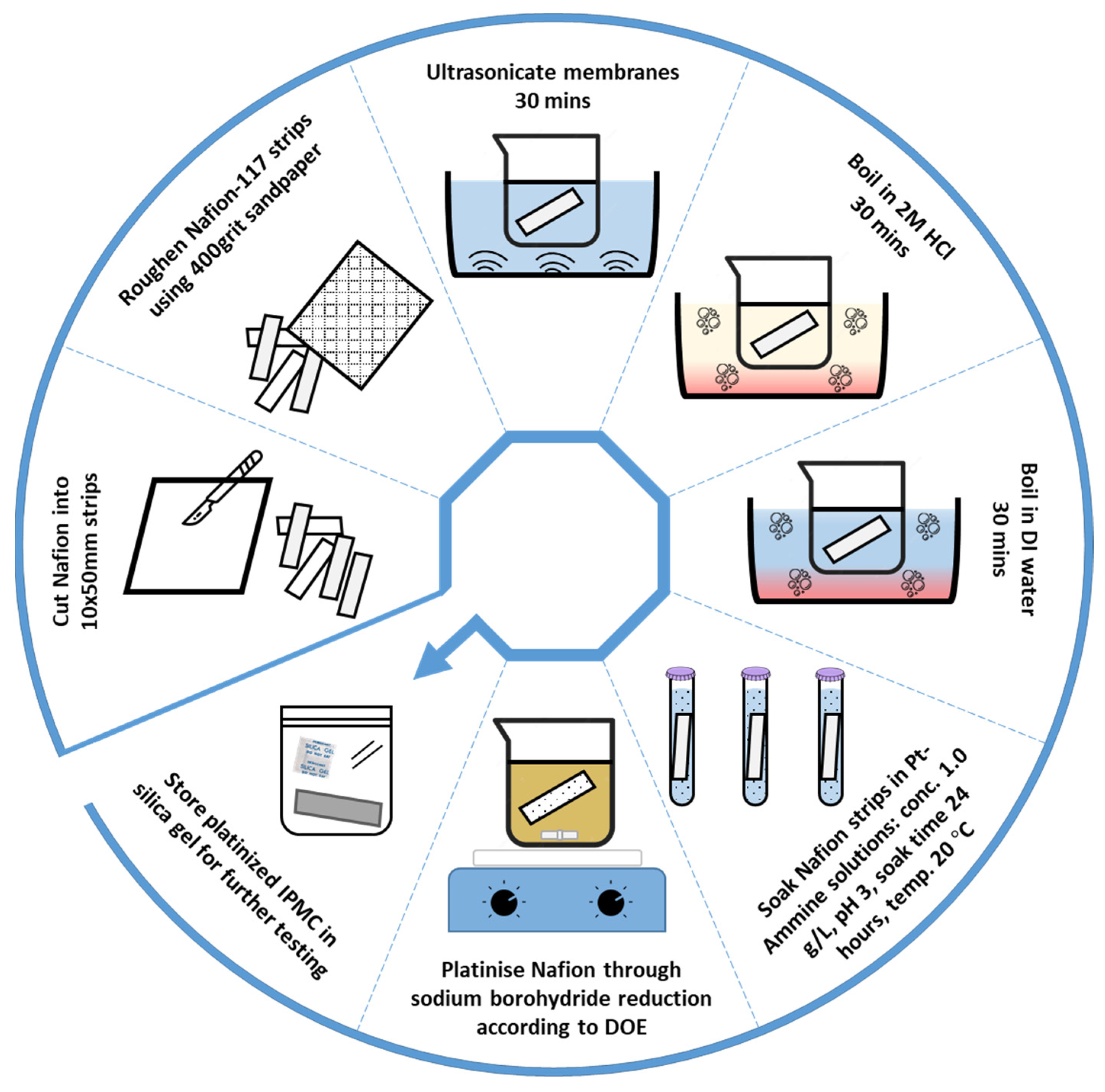
2.1. IPMC Preparation
2.2. Full Factorial Design for Sodium Borohydride Reduction
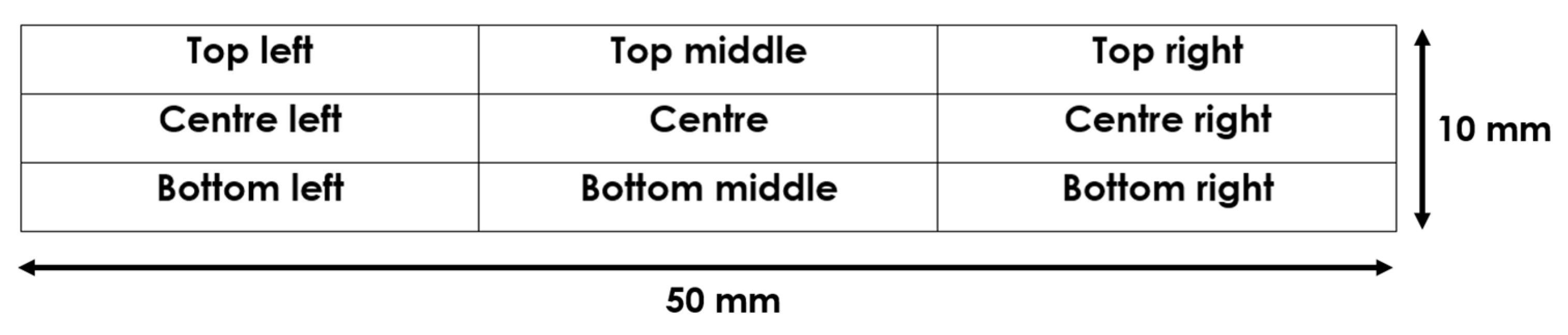
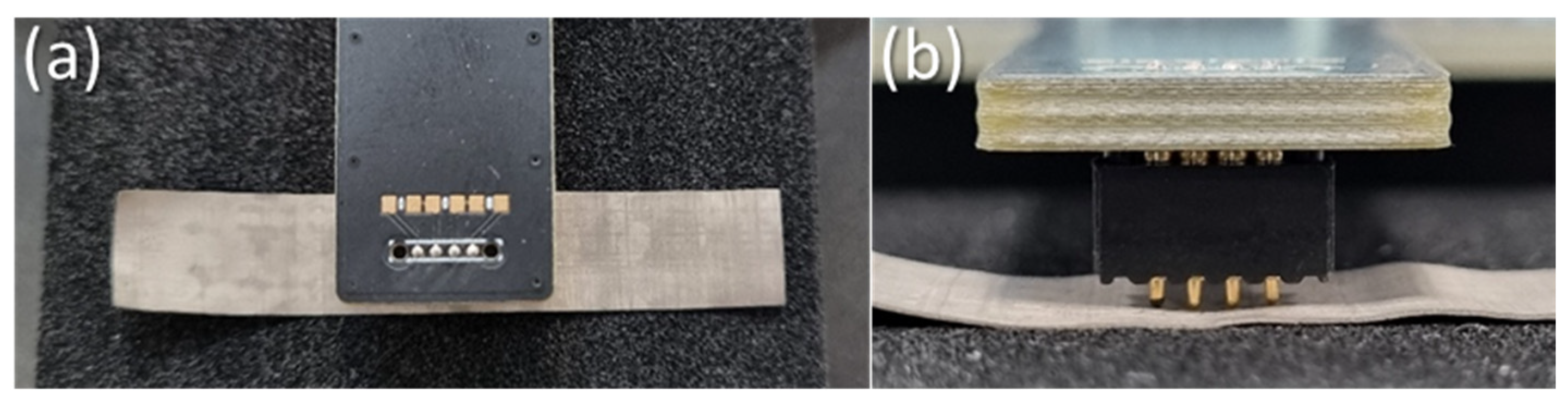
2.3. Surface Resistance Measurements
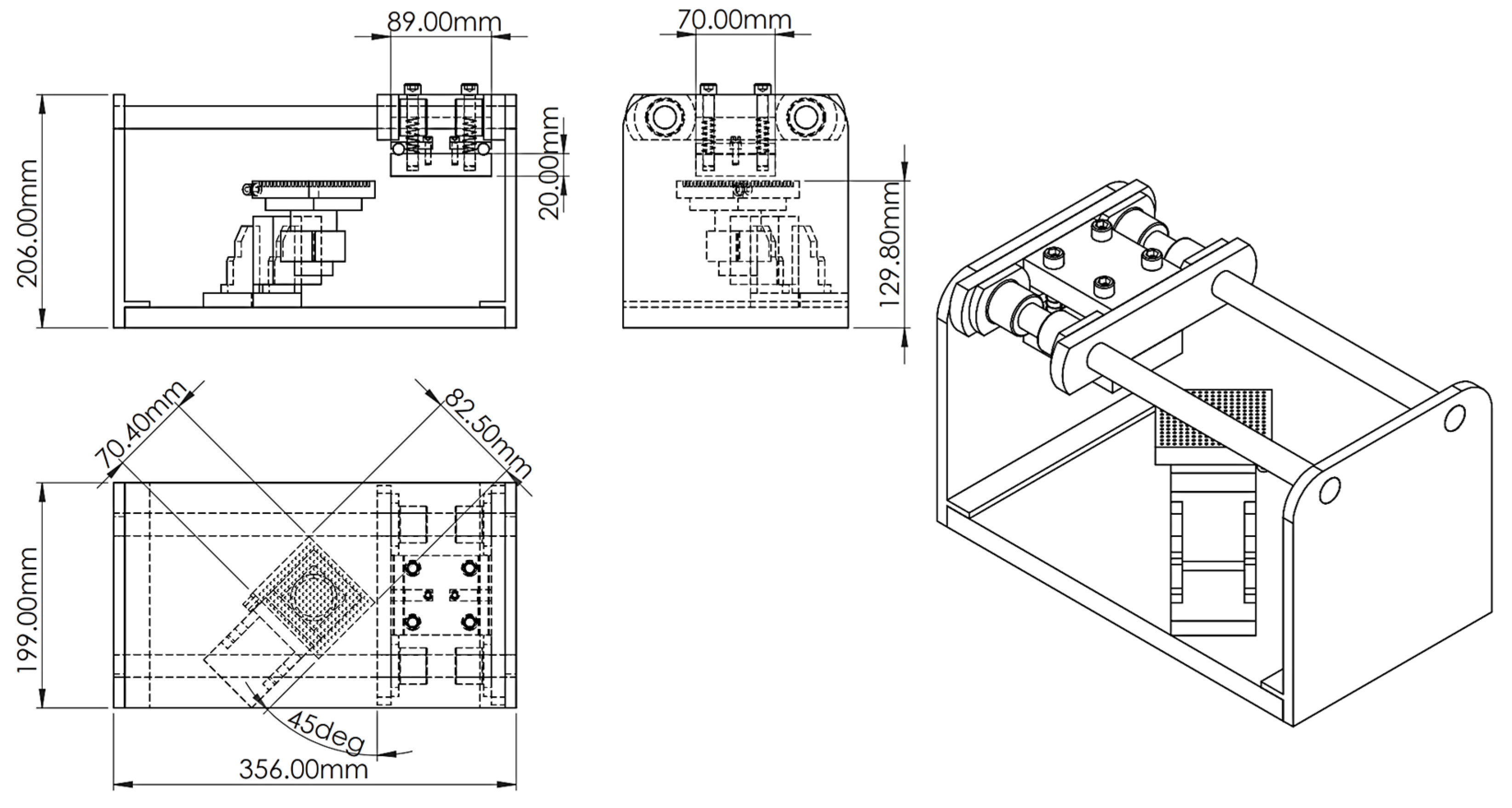
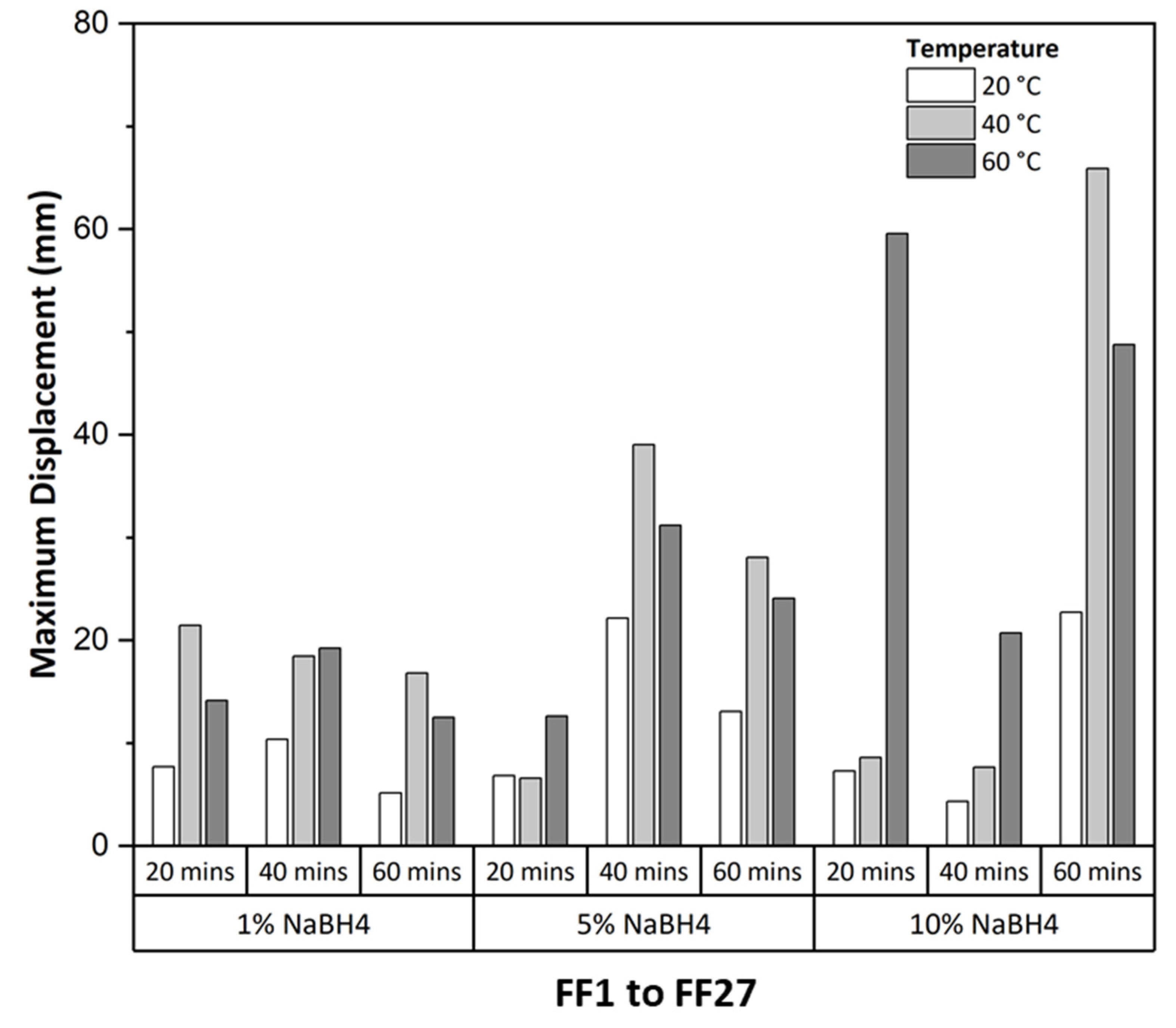
2.4. IPMC Displacement Measurement
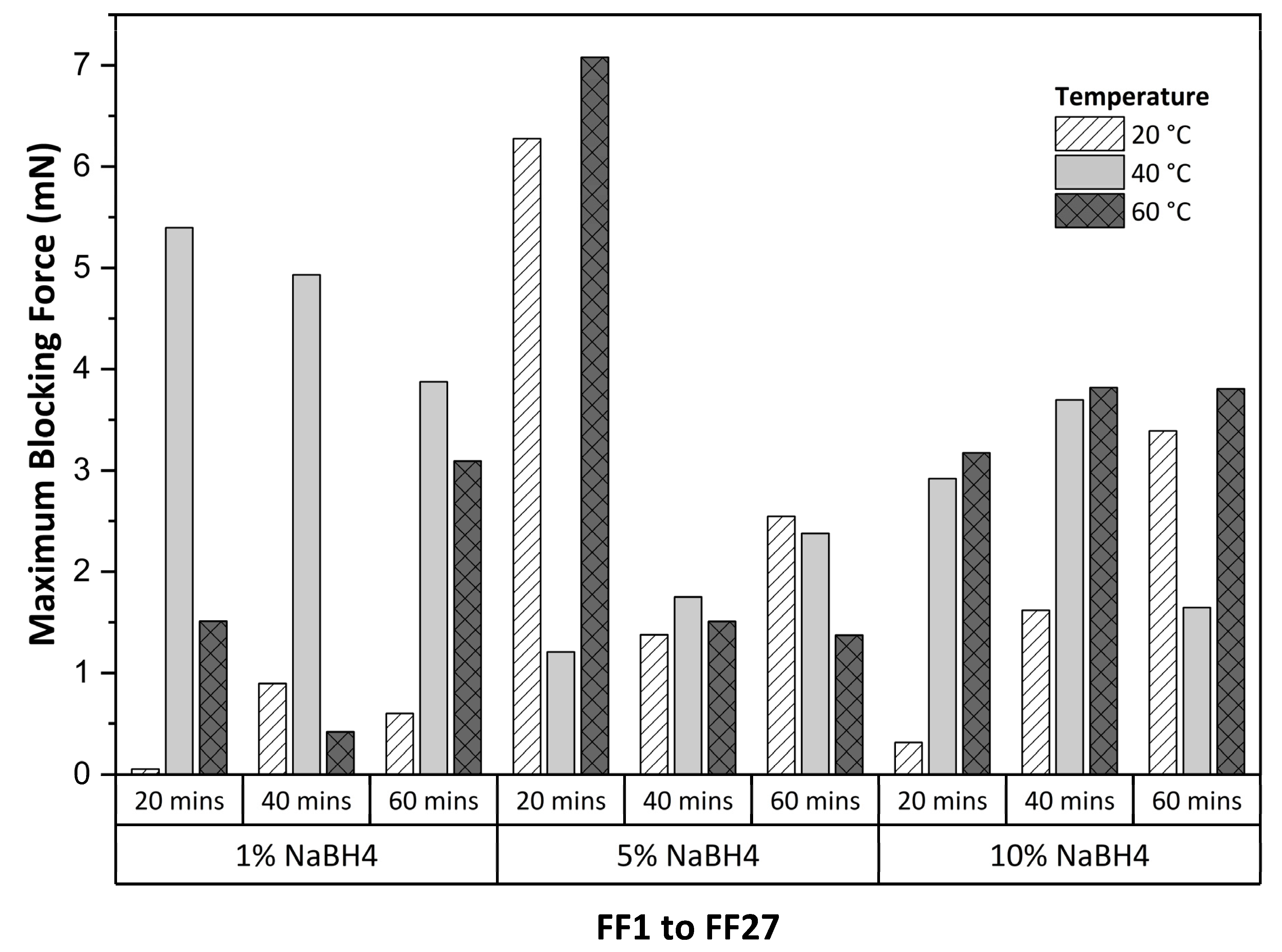
2.5. IPMC Blocking Force Measurement
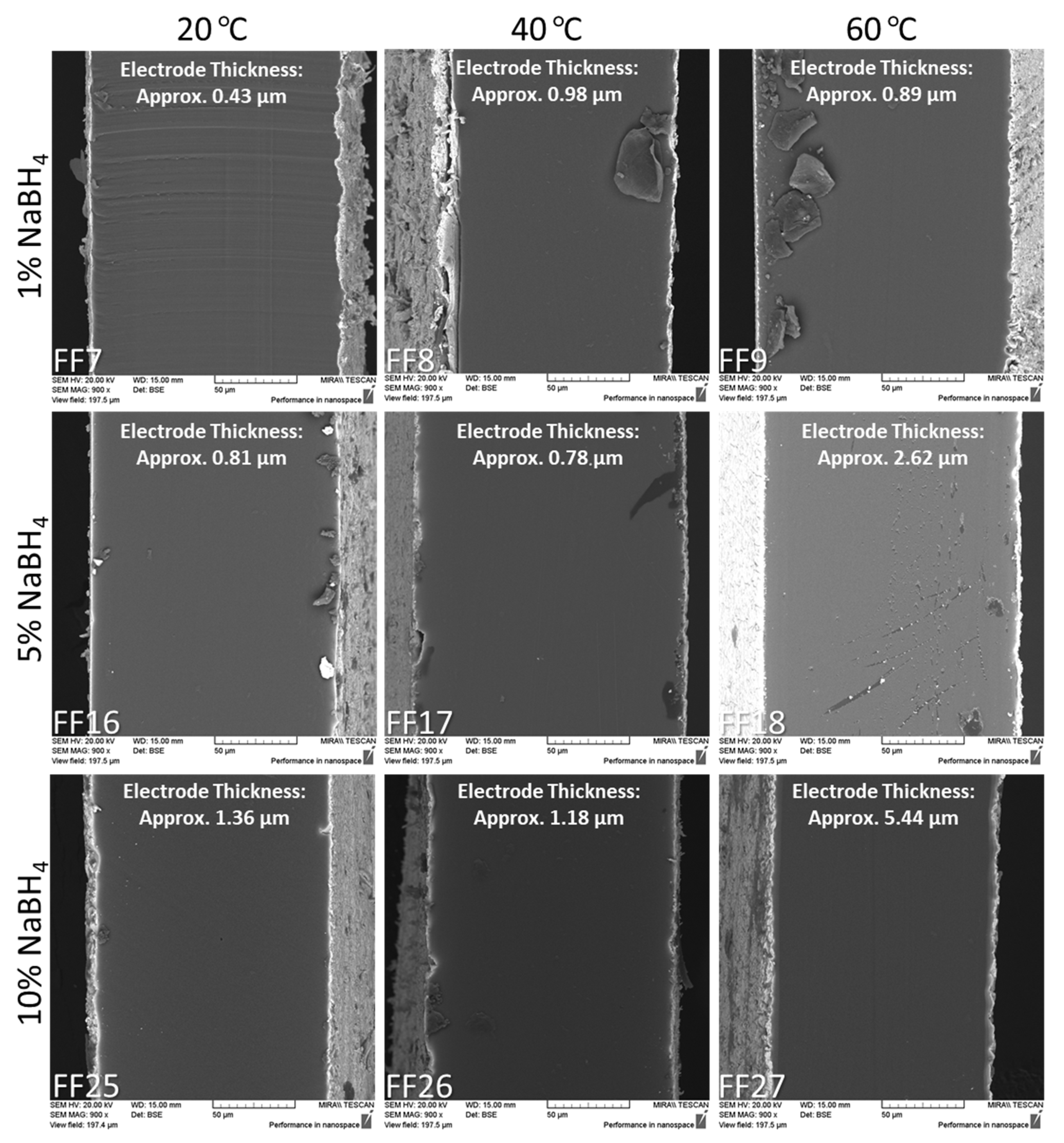
2.6. Scanning Electron Microscopy
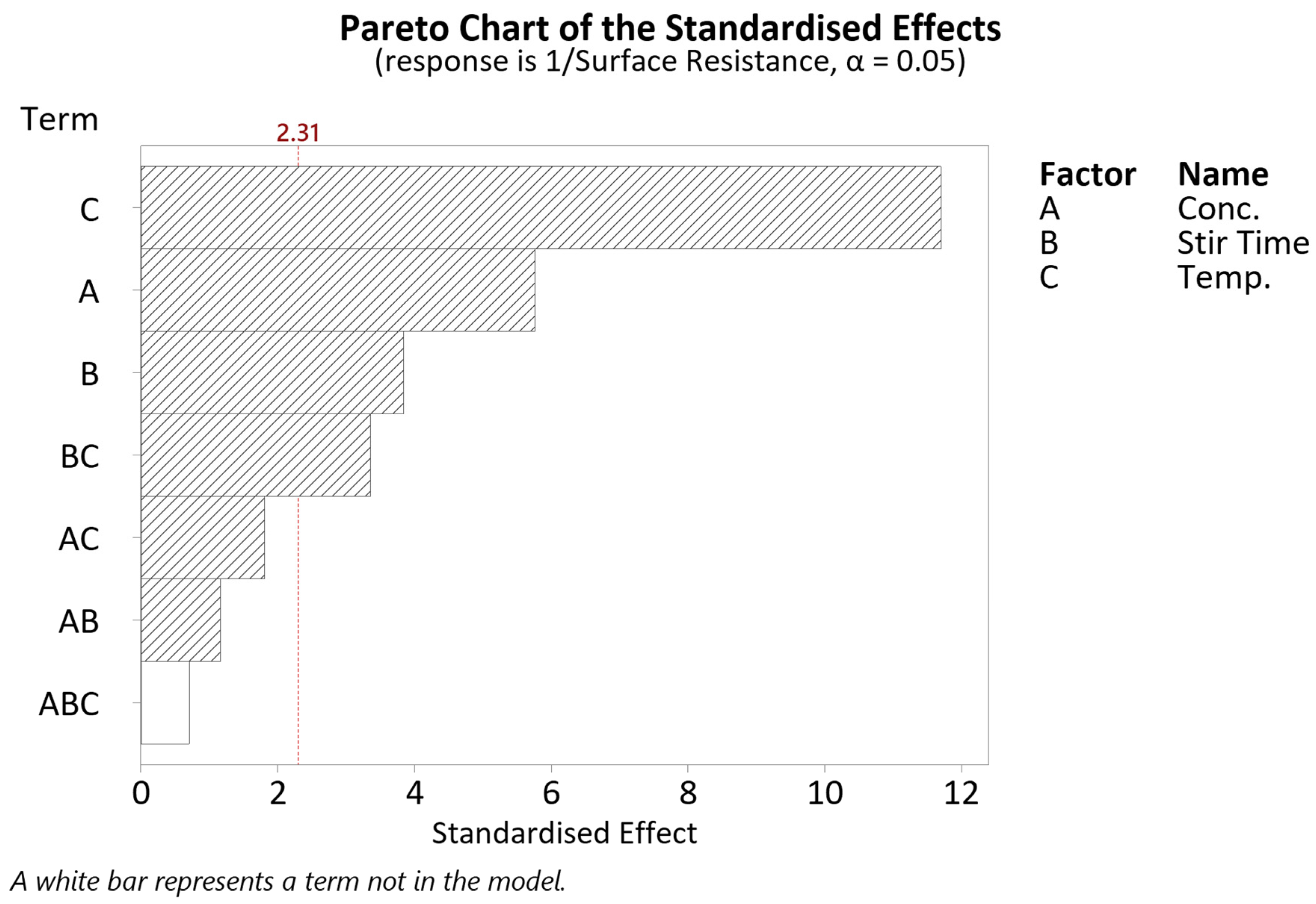
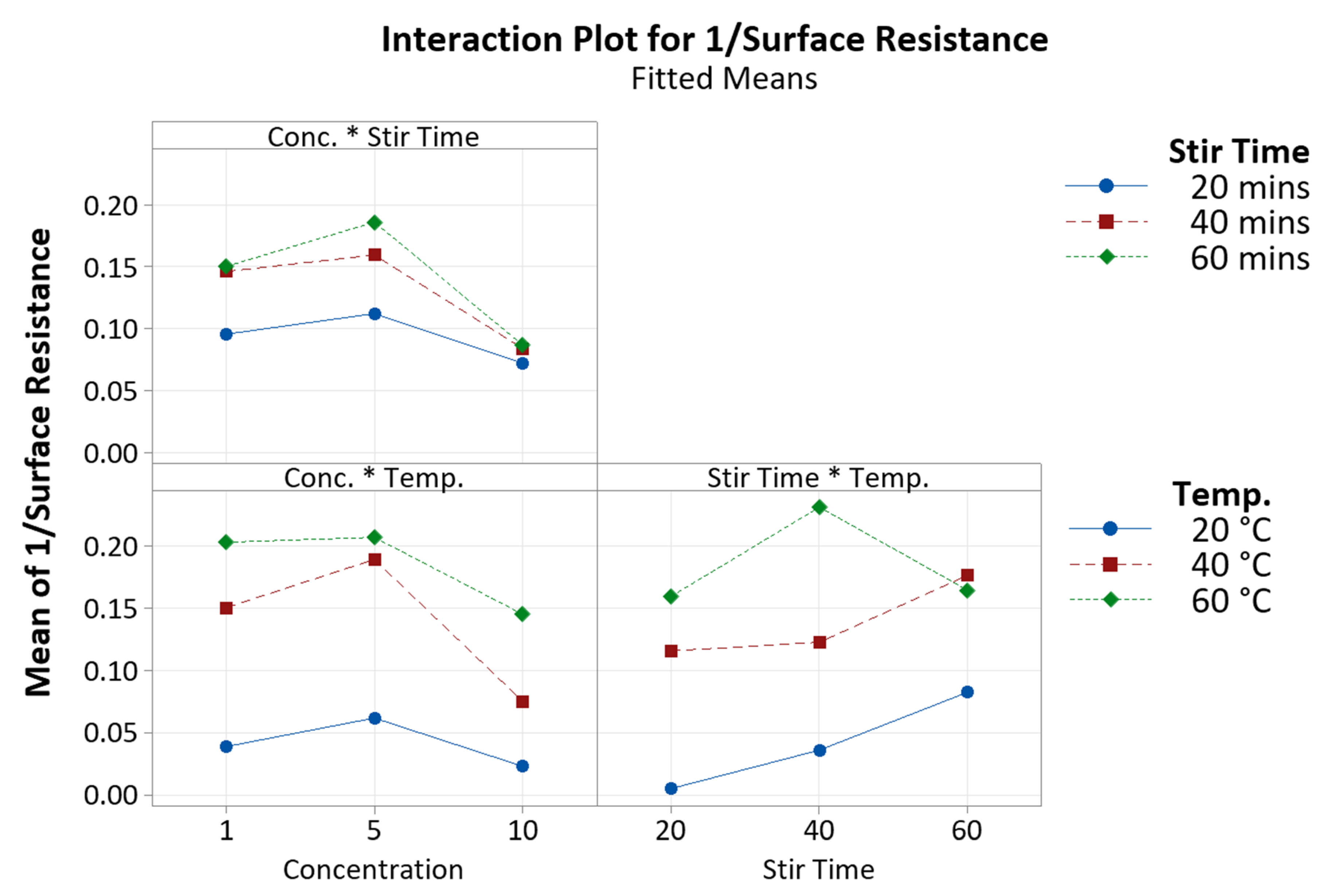
3. Results and Discussion
3.1. Concentration
3.2. Stir Time
3.3. Temperature
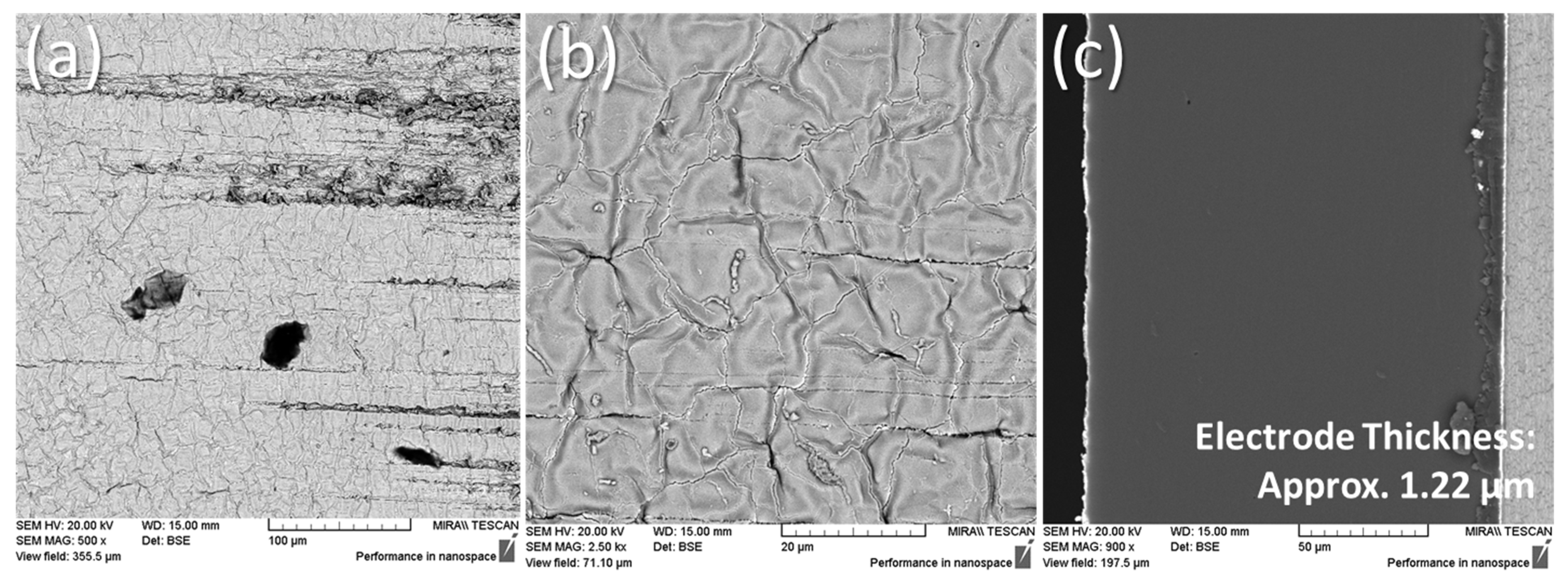
3.4. Scanning Electron Microscopy Results
3.5. IPMC Performance
4. Limitations and Recommendations
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| FF1 | FF2 | FF3 | ||||||
| 837,545.077 | 2,381,020.195 | 582.518 | 9.979 | 36.336 | 19.905 | 7.03 | 23.759 | 8.632 |
| 1,934,525.223 | 2,754,967.048 | 4682.585 | 7.927 | 21.9 | 23.526 | 7.927 | 11.611 | 8.898 |
| 905,504.633 | 2,730,937.747 | 3730.69 | 10.495 | 30.228 | 45.368 | 6.315 | 6.954 | 9.064 |
| FF4 | FF5 | FF6 | ||||||
| 34.263 | 214.228 | 31.932 | 10.336 | 32.989 | 6.436 | 7.278 | 4.138 | 96.579 |
| 28.979 | 33.535 | 64.008 | 10.044 | 21.962 | 7.183 | 5.43 | 11.311 | 25.654 |
| 28.316 | 90.202 | 24.116 | 12.933 | 17.517 | 8.518 | 5.944 | 14.772 | 17.937 |
| FF7 | FF8 | FF9 | ||||||
| 13.812 | 18.75 | 45 | 19.445 | 56.283 | 6.472 | 71.68 | 42.175 | 5.351 |
| 14.589 | 31.509 | 61.746 | 12.511 | 5.913 | 5.948 | 350.506 | 81.694 | 6.706 |
| 13.892 | 23.394 | 35.287 | 12.746 | 76.687 | 6.408 | 437.834 | 101.935 | 4.782 |
| FF10 | FF11 | FF12 | ||||||
| 99.046 | 2764.726 | 3,635,747.617 | 516.904 | 1584.862 | 7.218 | 36.547 | 19.005 | 5.826 |
| 362.044 | 2304.978 | 2,772,178.739 | 288,504.969 | 2033.538 | 6.666 | 35.392 | 20.318 | 5.68 |
| 422.902 | 12,713.805 | 417,848.278 | 122.99 | 3653.969 | 7.253 | 157.38 | 30.79 | 9.615 |
| FF13 | FF14 | FF15 | ||||||
| 32.033 | 95.217 | 31.292 | 19.392 | 13.049 | 6.616 | 60.777 | 16.914 | 4.254 |
| 30.569 | 59.846 | 25.576 | 29.207 | 10.838 | 5.875 | 551.801 | 11.003 | 3.716 |
| 26.267 | 476.744 | 25.895 | 26.432 | 9.943 | 6.501 | 22,889.61 | 25.629 | 3.888 |
| FF16 | FF17 | FF18 | ||||||
| 9.299 | 121.47 | 436.187 | 9.408 | 9.609 | 4.044 | 22.689 | 9.609 | 5.961 |
| 7.392 | 65.903 | 501.895 | 14.065 | 12.293 | 5.383 | 22.764 | 9.395 | 5.686 |
| 8.202 | 75.451 | 213.183 | 19.014 | 15.709 | 4.661 | 23.048 | 13.137 | 7.263 |
| FF19 | FF20 | FF21 | ||||||
| 8913.456 | 4071.78 | 10,484.77 | 1889.377 | 229.782 | 23.903 | 42.436 | 23.744 | 7.88 |
| 19,625.522 | 895.949 | 6240.57 | 1965.838 | 320.28 | 15.098 | 22.633 | 15.856 | 6.977 |
| 4701.204 | 415.075 | 5042.839 | 1485.666 | 1894.301 | 14.203 | 21.931 | 16.185 | 8.274 |
| FF22 | FF23 | FF24 | ||||||
| 6604.527 | 78,888.929 | 108.414 | 30.718 | 14,779.799 | 25,851.422 | 179.526 | 32.61 | 5.494 |
| 87,029.214 | 86,811.625 | 85.888 | 24.046 | 4073.054 | 28,441.475 | 81.878 | 30.471 | 5.452 |
| 11,530.769 | 22,689.887 | 37.741 | 31.446 | 1356.76 | 5928.397 | 327.947 | 41.231 | 5.78 |
| FF25 | FF26 | FF27 | ||||||
| 26.312 | 345.196 | 1209.541 | 47.927 | 34.306 | 14.474 | 9.89 | 15.467 | 16.268 |
| 54.266 | 110.342 | 2756.198 | N/A | 58.453 | 8.885 | 9.27 | 12.178 | 11.348 |
| 25.589 | 1229.963 | 3058.124 | 129.293 | 64.64 | 13.231 | 9.284 | 14.993 | 28.343 |
| Response | Regression Equation |
|---|---|
| 1/Surface Resistance | 0.12132 + 0.00928 Conc._1 + 0.03124 Conc._5 − 0.04052 Conc._10 − 0.02815 Stir Time_20 + 0.00851 Stir Time_40 + 0.01964 Stir Time_60 − 0.08043 Temp._20 + 0.01676 Temp._40 + 0.06367 Temp._60 − 0.00704 Conc.*Stir Time_1 20 + 0.00706 Conc.*Stir Time_1 40 − 0.00002 Conc.*Stir Time_1 60 − 0.01236 Conc.*Stir Time_5 20 − 0.00159 Conc.*Stir Time_5 40 + 0.01395 Conc.*Stir Time_5 60 + 0.01940 Conc.*Stir Time_10 20 − 0.00547 Conc.*Stir Time_10 40 − 0.01393 Conc.*Stir Time_10 60 − 0.01165 Conc.*Temp._1 20 + 0.00286 Conc.*Temp._1 40 + 0.00879 Conc.*Temp._1 60 − 0.01064 Conc.*Temp._5 20 + 0.01985 Conc.*Temp._5 40 − 0.00921 Conc.*Temp._5 60 + 0.02229 Conc.*Temp._10 20 − 0.02271 Conc.*Temp._10 40 + 0.00042 Conc.*Temp._10 60 − 0.00801 Stir Time*Temp._20 20 + 0.00559 Stir Time*Temp._20 40 + 0.00242 Stir Time*Temp._20 60 − 0.01372 Stir Time*Temp._40 20 − 0.02420 Stir Time*Temp._40 40 + 0.03791 Stir Time*Temp._40 60 + 0.02172 Stir Time*Temp._60 20 + 0.01860 Stir Time*Temp._60 40 − 0.04033 Stir Time*Temp._60 60 |
| Term | Coef | SE Coef | T-Value | p-Value | VIF |
|---|---|---|---|---|---|
| Constant | 0.12132 | 0.00434 | 27.94 | 0.000 | |
| Conc. | |||||
| 1 | 0.00928 | 0.00614 | 1.51 | 0.169 | 1.33 |
| 5 | 0.03124 | 0.00614 | 5.09 | 0.001 | 1.33 |
| Stir Time | |||||
| 20 | −0.02815 | 0.00614 | −4.58 | 0.002 | 1.33 |
| 40 | 0.00851 | 0.00614 | 1.39 | 0.203 | 1.33 |
| Temp. | |||||
| 20 | −0.08043 | 0.00614 | −13.10 | 0.000 | 1.33 |
| 40 | 0.01676 | 0.00614 | 2.73 | 0.026 | 1.33 |
| Conc.*Stir Time | |||||
| 1 20 | −0.00704 | 0.00868 | −0.81 | 0.441 | 1.78 |
| 1 40 | 0.00706 | 0.00868 | 0.81 | 0.440 | 1.78 |
| 5 20 | −0.01236 | 0.00868 | −1.42 | 0.192 | 1.78 |
| 5 40 | −0.00159 | 0.00868 | −0.18 | 0.859 | 1.78 |
| Conc.*Temp. | |||||
| 1 20 | −0.01165 | 0.00868 | −1.34 | 0.217 | 1.78 |
| 1 40 | 0.00286 | 0.00868 | 0.33 | 0.751 | 1.78 |
| 5 20 | −0.01064 | 0.00868 | −1.23 | 0.255 | 1.78 |
| 5 40 | 0.01985 | 0.00868 | 2.29 | 0.052 | 1.78 |
| Stir Time*Temp. | |||||
| 20 20 | −0.00801 | 0.00868 | −0.92 | 0.383 | 1.78 |
| 20 40 | 0.00559 | 0.00868 | 0.64 | 0.538 | 1.78 |
| 40 20 | −0.01372 | 0.00868 | −1.58 | 0.153 | 1.78 |
| 40 40 | −0.02420 | 0.00868 | −2.79 | 0.024 | 1.78 |
| FF | Conc. (%) | Soak Time (minutes) | Temp. (°C) | Min. Surf. Res. (Ω/square) | Max. Disp. (mm) | Max. Blocking Force (mN) |
|---|---|---|---|---|---|---|
| 1 | 1 | 20 | 20 | 582.52 | 7.71 | 0.052 |
| 2 | 1 | 20 | 40 | 7.93 | 21.45 | 5.396 |
| 3 | 1 | 20 | 60 | 6.31 | 14.12 | 1.51 |
| 4 | 1 | 40 | 20 | 24.12 | 10.34 | 0.895 |
| 5 | 1 | 40 | 40 | 6.44 | 18.46 | 4.932 |
| 6 | 1 | 40 | 60 | 4.14 | 19.22 | 0.418 |
| 7 | 1 | 60 | 20 | 13.81 | 5.13 | 0.6 |
| 8 | 1 | 60 | 40 | 5.91 | 16.81 | 3.876 |
| 9 | 1 | 60 | 60 | 4.78 | 12.49 | 3.092 |
| 10 | 5 | 20 | 20 | 99.05 | 6.83 | 6.277 |
| 11 | 5 | 20 | 40 | 6.67 | 6.58 | 1.206 |
| 12 | 5 | 20 | 60 | 5.68 | 12.6 | 7.079 |
| 13 | 5 | 40 | 20 | 25.58 | 22.16 | 1.377 |
| 14 | 5 | 40 | 40 | 5.87 | 39.02 | 1.749 |
| 15 | 5 | 40 | 60 | 3.72 | 31.17 | 1.509 |
| 16 | 5 | 60 | 20 | 7.39 | 13.06 | 2.545 |
| 17 | 5 | 60 | 40 | 4.04 | 28.06 | 2.377 |
| 18 | 5 | 60 | 60 | 5.69 | 24.09 | 1.372 |
| 19 | 10 | 20 | 20 | 415.08 | 7.26 | 0.313 |
| 20 | 10 | 20 | 40 | 14.2 | 8.58 | 2.918 |
| 21 | 10 | 20 | 60 | 6.98 | 59.54 | 3.176 |
| 22 | 10 | 40 | 20 | 37.74 | 4.32 | 1.618 |
| 23 | 10 | 40 | 40 | 24.05 | 7.65 | 3.697 |
| 24 | 10 | 40 | 60 | 5.45 | 20.7 | 3.817 |
| 25 | 10 | 60 | 20 | 25.59 | 22.74 | 3.389 |
| 26 | 10 | 60 | 40 | 8.89 | 65.9 | 1.646 |
| 27 | 10 | 60 | 60 | 9.27 | 48.74 | 3.805 |
References
- Yang, L.; Wang, H.; Zhang, X. Recent Progress in Preparation Process of Ionic Polymer-Metal Composites. Results Phys. 2021, 29, 104800. [Google Scholar] [CrossRef]
- Tarditi, A.M.; Bosko, M.L.; Cornaglia, L.M. 3.1 Electroless Plating of Pd Binary and Ternary Alloys and Surface Characteristics for Application in Hydrogen Separation. In Comprehensive Materials Finishing; Hashmi, M., Ed.; Elsevier: Oxford, UK, 2017; pp. 1–24. ISBN 978-0-12-803249-7. [Google Scholar]
- Takenaka, H.; Torikai, E. Production of an Ion-Exchange Membrane-Catalytic Electrode Bonded Material for Electrolytic Cells; NASA: Washington, DC, USA, 1980; pp. 147–150.
- Fedkiw, P.S.; Her, W.-H. An Impregnation-Reduction Method to Prepare Electrodes on Nafion SPE. J. Electrochem. Soc. 1989, 136, 899. [Google Scholar] [CrossRef]
- Kim, K.J.; Shahinpoor, M. Ionic Polymer Metal Composites: II. Manufacturing Techniques. Manufacturing Techniques. Smart Mater. Struct. 2003, 12, 65–79. [Google Scholar] [CrossRef]
- Yang, L.; Zhang, D.; Zhang, X.; Tian, A.; Wang, X. Models of Displacement and Blocking Force of Ionic-Polymer Metal Composites Based on Actuation Mechanism. Appl. Phys. Mater. Sci. Process. 2020, 126, 365. [Google Scholar] [CrossRef]
- Rashid, T.; Shahinpoor, M. Force Optimization of Ionic Polymeric Platinum Composite Artificial Muscles by Means of an Orthogonal Array Manufacturing Method. In Proceedings of the Smart Structures and Materials 1999: Electroactive Polymer Actuators and Devices, Newport Beach, CA, USA, 1–2 March 1999; SPIE: Bellingham, DC, USA, 1999; Volume 3669, pp. 289–298. [Google Scholar]
- Yip, J.; Feng, L.S.; Hang, C.W.; Marcus, Y.C.W.; Wai, K.C. Experimentally Validated Improvement of IPMC Performance through Alternation of Pretreatment and Electroless Plating Processes. Smart Mater. Struct. 2010, 20, 015009. [Google Scholar] [CrossRef]
- Rashid, M.; Jun, T.-S.; Kim, Y.S. Material Properties of the Pt Electrode Deposited on Nafion Membrane by the Impregnation–Reduction Method. J. Nanosci. Nanotechnol. 2013, 13, 3627–3633. [Google Scholar] [CrossRef]
- Shahinpoor, M. Chapter 2: Ionic Polymer Metal Composites (IPMCs) Optimal Manufacturing. In Ionic Polymer Metal Composites (IPMCs); Royal Society of Chemistry: London, UK, 2015; pp. 61–147. [Google Scholar]
- Liu, R.; Her, W.-H.; Fedkiw, P.S. In Situ Electrode Formation on a Nafion Membrane by Chemical Platinization. J. Electrochem. Soc. 1992, 139, 15. [Google Scholar] [CrossRef]
- Tian, A.; Sun, Y.; Wang, X.; Li, J.; Zhang, X.; Wang, H. Effects of Surface Roughening Method on the Performance of Ionic Polymer Metal Composition. In Proceedings of the 2021 IEEE International Symposium on Applications of Ferroelectrics (ISAF), Virtual, 16–21 May 2021; pp. 1–4. [Google Scholar]
- Palmre, V.; Pugal, D.; Kim, K.J.; Leang, K.K.; Asaka, K.; Aabloo, A. Nanothorn Electrodes for Ionic Polymer-Metal Composite Artificial Muscles. Sci. Rep. 2014, 4, 6176. [Google Scholar] [CrossRef]
- Kim, K.J.; Lee, D.-C.; Nam, J.; Hwang, T.S. Fabricating Ionic/Polyimtde Membranes. U.S. Patent 11,312,851, 26 April 2022. [Google Scholar]
- Oguro, K. Preparation Procedure Ion-Exchange Polymer Metal Composites (IPMC) Membranes. Available online: https://ndeaa.jpl.nasa.gov/nasa-nde/lommas/eap/IPMC_PrepProcedure.htm (accessed on 29 September 2021).
- Xu, B.; Wang, S.; Zhang, Z.; Ling, J.; Wu, X. Improving the Torsion Performance of IPMC by Changing the Electrode Separation. Sci. Rep. 2021, 11, 7639. [Google Scholar] [CrossRef]
- Ma, S.; Zhang, Y.; Liang, Y.; Ren, L.; Tian, W.; Ren, L. High-Performance Ionic-Polymer–Metal Composite: Toward Large-Deformation Fast-Response Artificial Muscles. Adv. Funct. Mater. 2020, 30, 1908508. [Google Scholar] [CrossRef]
- Yang, L.; Zhang, D.; Zhang, X.; Tian, A.; Ding, Y. Surface Roughening of Nafion Membranes Using Different Route Planning for IPMCs. Int. J. Smart Nano Mater. 2020, 11, 117–128. [Google Scholar] [CrossRef]
- Manaf, E.; Abdeali, G.; Reidy, S.; Higginbotham, C.L.; Lyons, J.G. Response Surface Modelling Nafion-117 Sorption of Tetraammineplatinum(II) Chloride in the Electroless Plating of IPMCs. Polymers 2024, 16, 2338. [Google Scholar] [CrossRef] [PubMed]
- Manaf, E.; Fitzgerald, K.; Higginbotham, C.L.; Lyons, J.G. Computer Vision System: Measuring Displacement and Bending Angle of Ionic Polymer-Metal Composites. Appl. Sci. 2022, 12, 6744. [Google Scholar] [CrossRef]
- Chen, J.; Wang, G.; Wang, X.; Yang, X.; Zhu, S.; Wang, R. Effect of NaBH4 Concentration and Synthesis Temperature on the Performance of Pd/C Catalyst for Formic Acid Electro-Oxidation. Mater. Express 2013, 3, 176–180. [Google Scholar] [CrossRef]
- Luty-Błocho, M.; Wojnicki, M.; Csapo, E.; Fitzner, K. On the Rate of Interaction of Sodium Borohydride with Platinum (IV) Chloride Complexes in Alkaline Media. Materials 2021, 14, 3137. [Google Scholar] [CrossRef]
- Lee, J.-W.; Nguyen, V.K.; Park, S.Y.; Yoo, Y. Effect of Reduction Temperature on Electrode Formation and Performance of Ionic Polymer Metal Composites. In Proceedings of the Smart Structures and Materials 2006: Electroactive Polymer Actuators and Devices (EAPAD), San Diego, CA, USA, 27 February–2 March 2006; SPIE: Bellingham, DC, USA, 2006; Volume 6168, pp. 602–610. [Google Scholar]
- Shahinpoor, M.; Kim, K.J. The Effect of Surface-Electrode Resistance on the Performance of Ionic Polymer-Metal Composite (IPMC) Artificial Muscles. Smart Mater. Struct. 2000, 9, 543–551. [Google Scholar] [CrossRef]
- Ninham, B.W.; Battye, M.J.; Bolotskova, P.N.; Gerasimov, R.Y.; Kozlov, V.A.; Bunkin, N.F. Nafion: New and Old Insights into Structure and Function. Polymers 2023, 15, 2214. [Google Scholar] [CrossRef]
- Chen, Q.; Xiong, K.; Bian, K.; Jin, N.; Wang, B. Preparation and Performance of Soft Actuator Based on IPMC with Silver Electrodes. Front. Mech. Eng. China 2009, 4, 436–440. [Google Scholar] [CrossRef]
- He, C.; Gu, Y.; Zhang, J.; Ma, L.; Yan, M.; Mou, J.; Ren, Y. Preparation and Modification Technology Analysis of Ionic Polymer-Metal Composites (IPMCs). Int. J. Mol. Sci. 2022, 23, 3522. [Google Scholar] [CrossRef]
- Wang, Y.; Chen, H.; Wang, Y.; Zhu, Z.; Li, D. Effect of Dehydration on the Mechanical and Physicochemical Properties of Gold- and Palladium-Ionomeric Polymer-Metal Composite (IPMC) Actuators. Electrochimica Acta 2014, 129, 450–458. [Google Scholar] [CrossRef]
- Cho, W.-H.; Kei, C.-C.; Liu, B.-H.; Yu, Y.-S.; Chen, F.-Z. Platinum Growth Analysis in Atomic Layer Deposition Using In-Situ Resistance Measurement. In Proceedings of the 2016 IEEE International Instrumentation and Measurement Technology Conference Proceedings, Taipei, Taiwan, 23–26 May 2016; pp. 1–4. [Google Scholar]
- De Luca, V.; Digiamberardino, P.; Di Pasquale, G.; Graziani, S.; Pollicino, A.; Umana, E.; Xibilia, M.G. Ionic Electroactive Polymer Metal Composites: Fabricating, Modeling, and Applications of Postsilicon Smart Devices. J. Polym. Sci. Part B Polym. Phys. 2013, 51, 699–734. [Google Scholar] [CrossRef]
- Schlupp, M.V.F.; Wehrle, M.M.; Kunze, K.; Remhof, A.; Vogt, U.F. Platinum Thin-Film Electrodes Prepared by a Cost-Effective Chemical Vapor Deposition Technique. Adv. Eng. Mater. 2016, 18, 1200–1207. [Google Scholar] [CrossRef]
- Tang, G.; Mei, D.; Zhao, X.; Zhao, C.; Li, L.; Wang, Y. A Comprehensive Survey of Ionic Polymer Metal Composite Transducers: Preparation, Performance Optimization and Applications. Soft Sci. 2023, 3, 9. [Google Scholar] [CrossRef]
- Zhang, H.; Lin, Z.; Hu, Y.; Ma, S.; Liang, Y.; Ren, L.; Ren, L. Low-Voltage Driven Ionic Polymer-Metal Composite Actuators: Structures, Materials, and Applications. Adv. Sci. 2023, 10, 2206135. [Google Scholar] [CrossRef] [PubMed]
- Balaraju, J.N.; Sankara Narayanan, T.S.N.; Seshadri, S.K. Electroless Ni–P Composite Coatings. J. Appl. Electrochem. 2003, 33, 807–816. [Google Scholar] [CrossRef]
- Kalantary, M.R.; Holbrook, K.A.; Wells, P.B. Optimisation of a Bath for Electroless Plating and Its Use for the Production of Nickel-Phosphorus-Silicon Carbide Coatings. Trans. IMF 1993, 71, 55–61. [Google Scholar] [CrossRef]






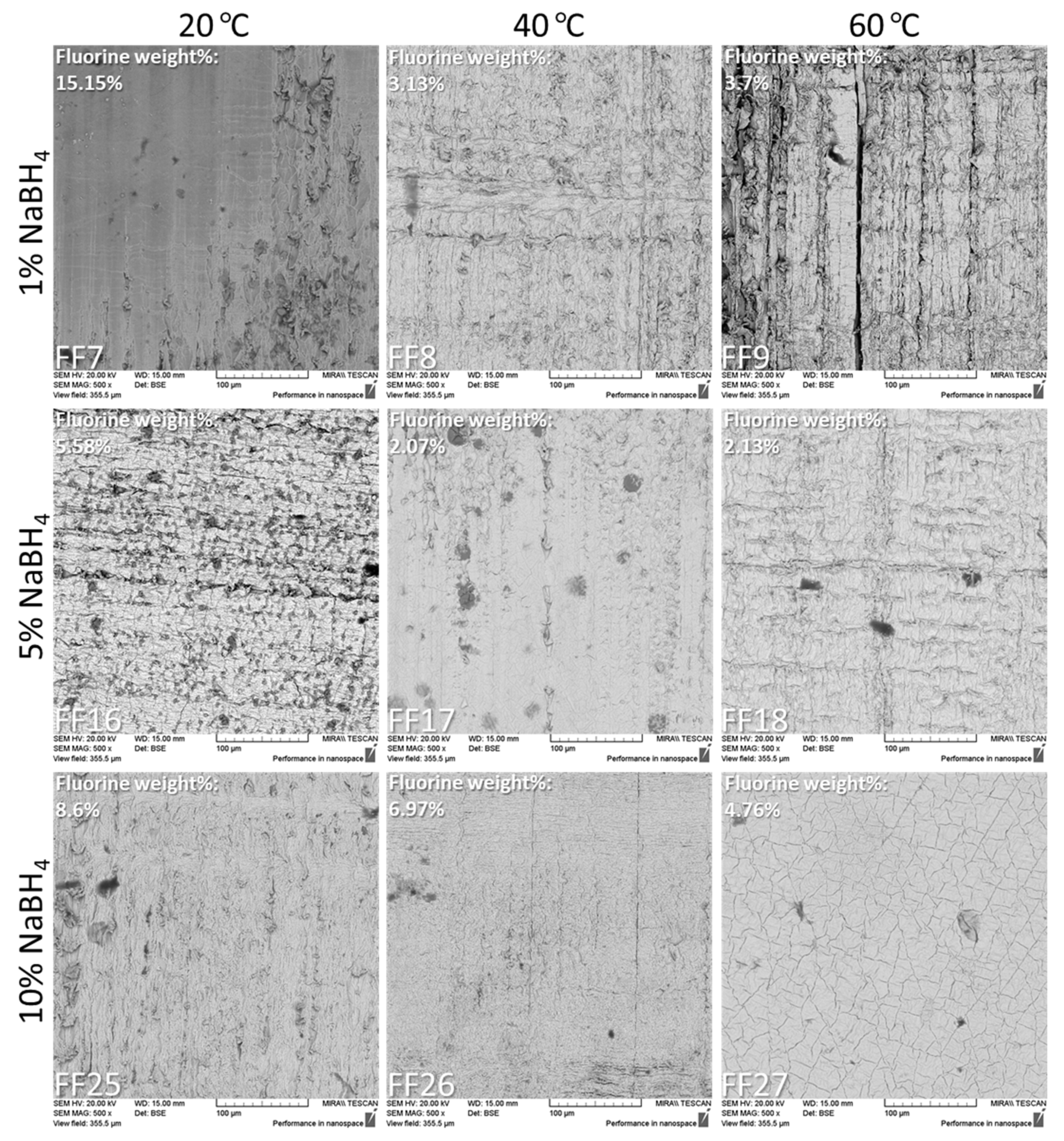
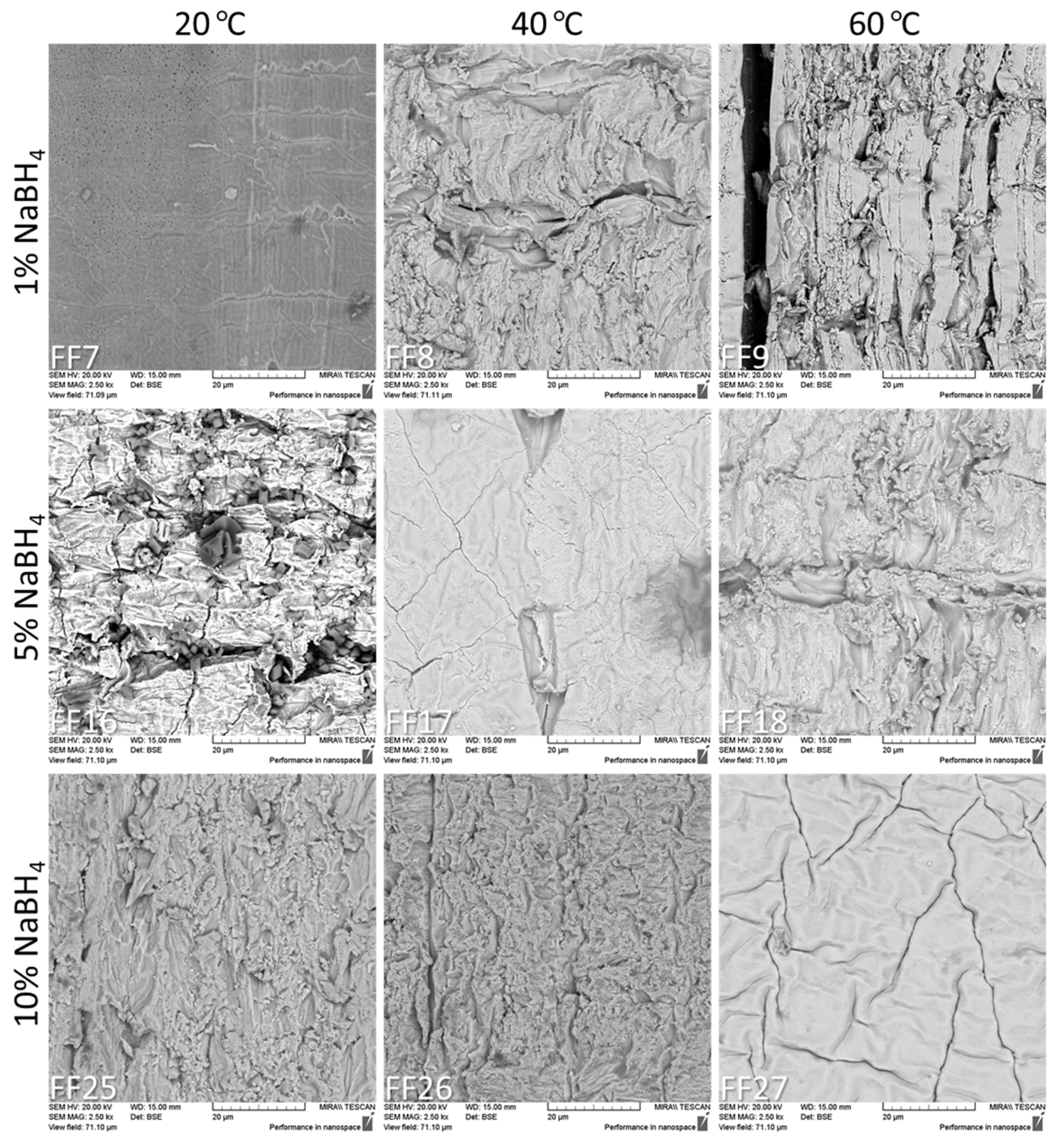


| Author (s) | Membrane Size | Reducing Agent | Concentration | Stir Time | Temp., °C |
|---|---|---|---|---|---|
| Kim et al., 2003 [5] | 5 × 5 cm | NaBH4 1 or LiBH4 2 | 5% | NS 3 | 60 |
| Shahinpoor, 2015 [10] | 5 × 5 cm | NaBH4 or LiBH4 | 5% | NS | 40–60 |
| Yip et al., 2011 [8] | 50 × 60 mm | NaBH4 | 5 wt% | 6.5 h | 40–60 |
| Liu et al., 1992 [11] | 1 in side hexagon | NaBH4 | 0.1 M | 2 h | 50 |
| Tian et al., 2021 [12] | 30 × 10 mm | NaBH4 | NS | NS | NS |
| Palmre et al., 2014 [13] | 50 × 10 mm | NaBH4 | 0.57 g/L | 2 h | 60 |
| Kim et al., 2022 [14] | 0.5 × 2.5 cm | NaBH4 | 0.57 g/L | 2 h | 60 |
| Yang et al., 2020 [6] | 10 × 50 mm | NaBH4 | 1% | >30 min | 40–60 |
| Oguro et al., 2000 [15] | 30 cm2 | NaBH4 | 5 wt% | 1.5 h | 40–60 |
| Xu et al., 2021 [16] | 30 × 29 mm | NaBH4 | 5% | 2 h | NS |
| Ma et al., 2020 [17] | 30 × 30 mm | NaBH4 | 5 wt% | 5 h (5 mL added every 30 min 10 times) | 40 |
| Rashid et al., 2013 [9] | 15 mm dia. circle | NaBH4 | 10–100 mM | 2 h (replaced every 20 min) | 5, 25, 50 |
| Full Factorial Configurations | |||||
|---|---|---|---|---|---|
| Factor | Unit | Notation | Factor Level | ||
| 1 | 2 | 3 | |||
| Concentration of NaBH4 | % | Conc. | 1 | 5 | 10 |
| Stir Time | minutes | ST | 20 | 40 | 60 |
| Temperature | °C | Temp. | 20 | 40 | 60 |
| FF1 86.7% | FF2 52.5% | FF3 50.6% |
| FF4 94.7% | FF5 57.4% | FF6 131.0% |
| FF7 54.2% | FF8 108.4% | FF9 122.7% |
| FF10 174.6% | FF11 274.4% | FF12 124.9% |
| FF13 155.4% | FF14 58.4% | FF15 273.8% |
| FF16 110.8% | FF17 47.2% | FF18 53.2% |
| FF19 82.1% | FF20 98.0% | FF21 56.9% |
| FF22 113.9% | FF23 119.5% | FF24 129.6% |
| FF25 115.0% | FF26 80.0% | FF27 39.9% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Manaf, E.; Fitzpatrick, D.P.; Higginbotham, C.L.; Lyons, J.G. Optimising Sodium Borohydride Reduction of Platinum onto Nafion-117 in the Electroless Plating of Ionic Polymer–Metal Composites. Actuators 2024, 13, 350. https://doi.org/10.3390/act13090350
Manaf E, Fitzpatrick DP, Higginbotham CL, Lyons JG. Optimising Sodium Borohydride Reduction of Platinum onto Nafion-117 in the Electroless Plating of Ionic Polymer–Metal Composites. Actuators. 2024; 13(9):350. https://doi.org/10.3390/act13090350
Chicago/Turabian StyleManaf, Eyman, Daniel P. Fitzpatrick, Clement L. Higginbotham, and John G. Lyons. 2024. "Optimising Sodium Borohydride Reduction of Platinum onto Nafion-117 in the Electroless Plating of Ionic Polymer–Metal Composites" Actuators 13, no. 9: 350. https://doi.org/10.3390/act13090350
APA StyleManaf, E., Fitzpatrick, D. P., Higginbotham, C. L., & Lyons, J. G. (2024). Optimising Sodium Borohydride Reduction of Platinum onto Nafion-117 in the Electroless Plating of Ionic Polymer–Metal Composites. Actuators, 13(9), 350. https://doi.org/10.3390/act13090350








