Design and Characterisation of a 3D-Printed Pneumatic Rotary Actuator Exploiting Enhanced Elastic Properties of Auxetic Metamaterials
Abstract
1. Introduction
2. Materials and Methods
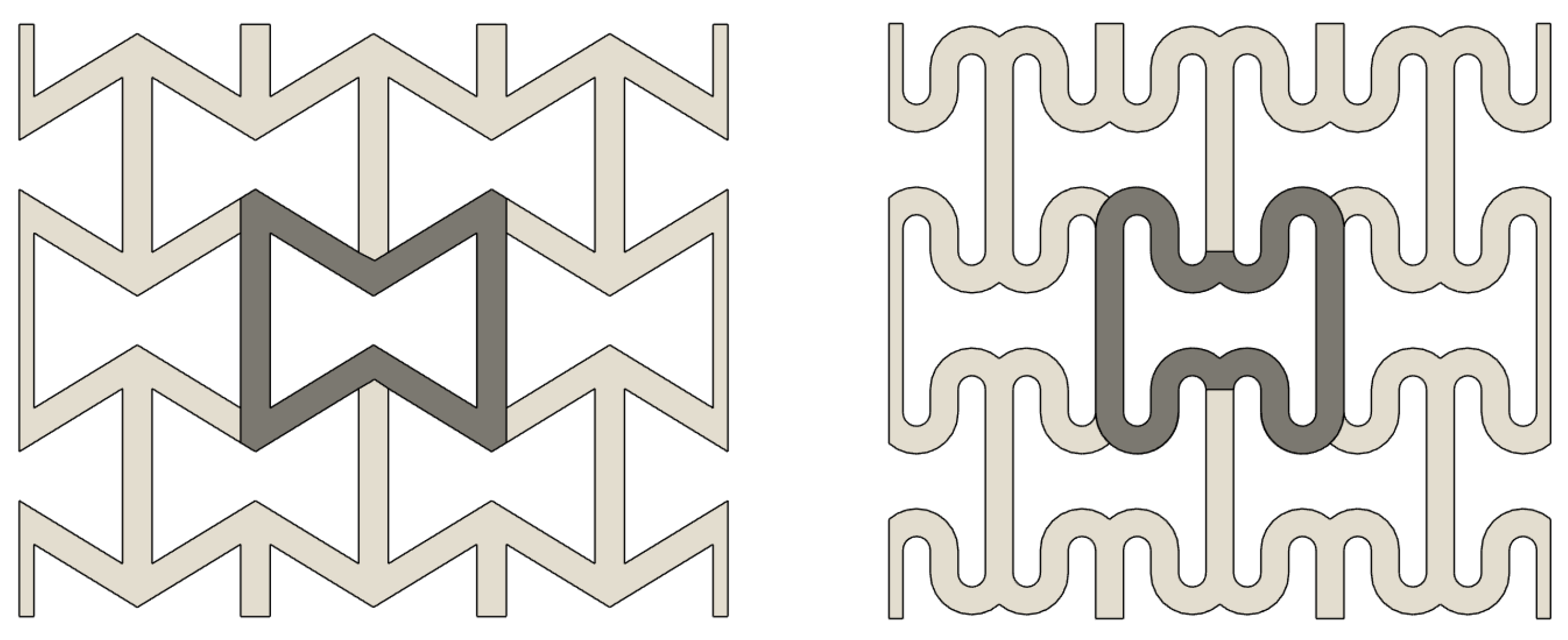
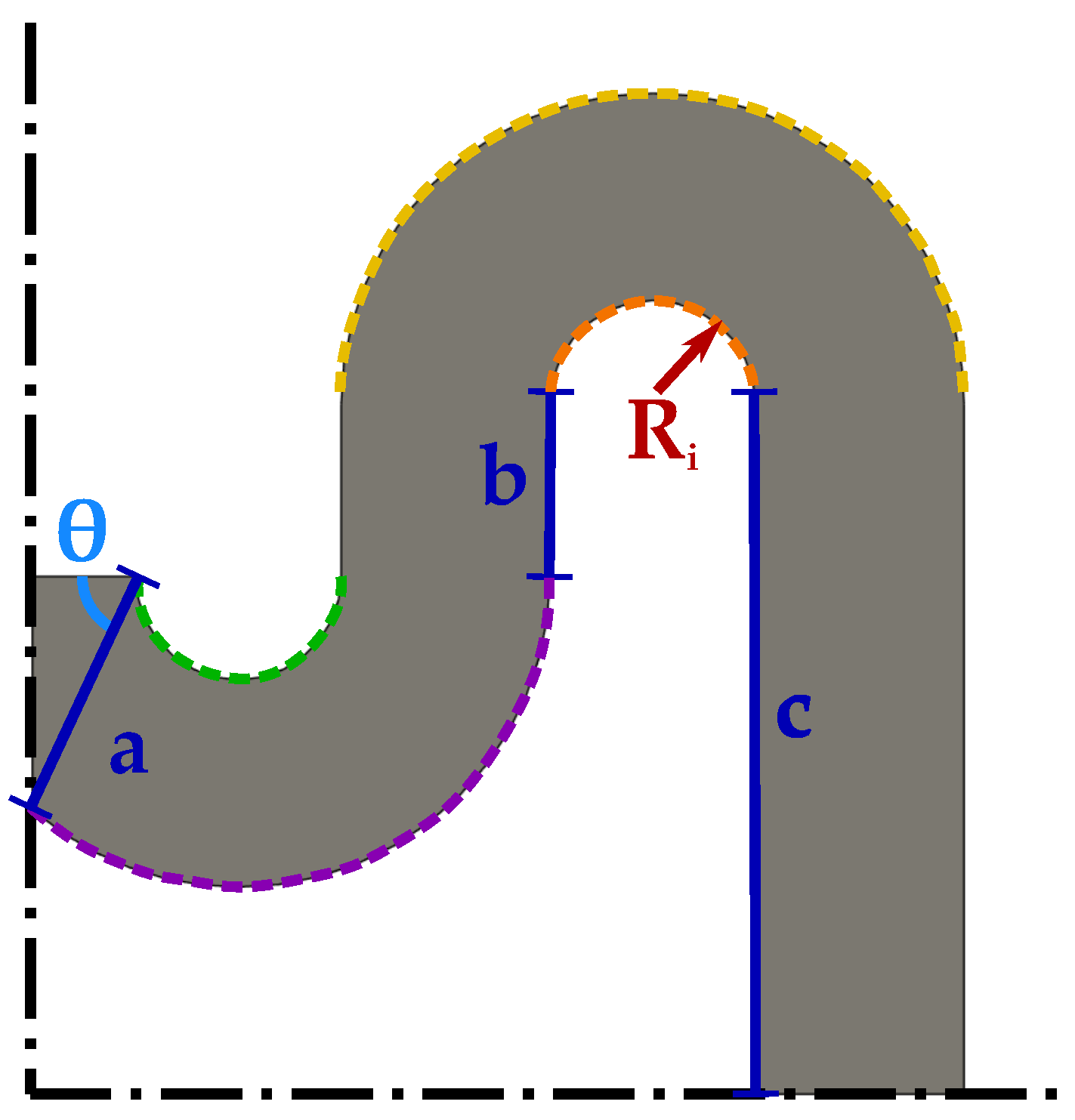
2.1. Actuation Principle Based on Auxetic Metamaterials
- for 2D structures;
- for 3D structures with isotropic behaviour;
- for 3D structures with anisotropic behaviour.
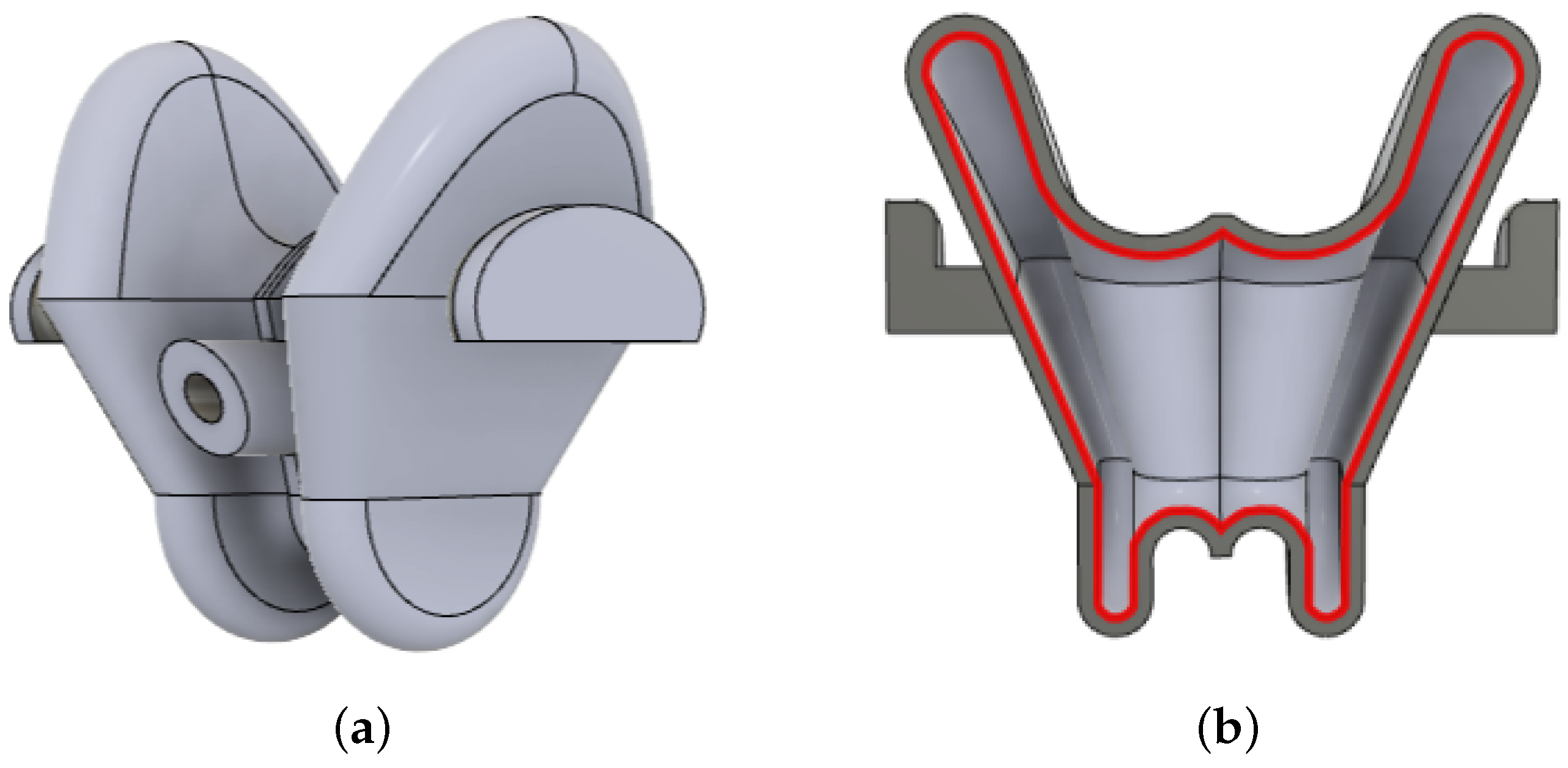
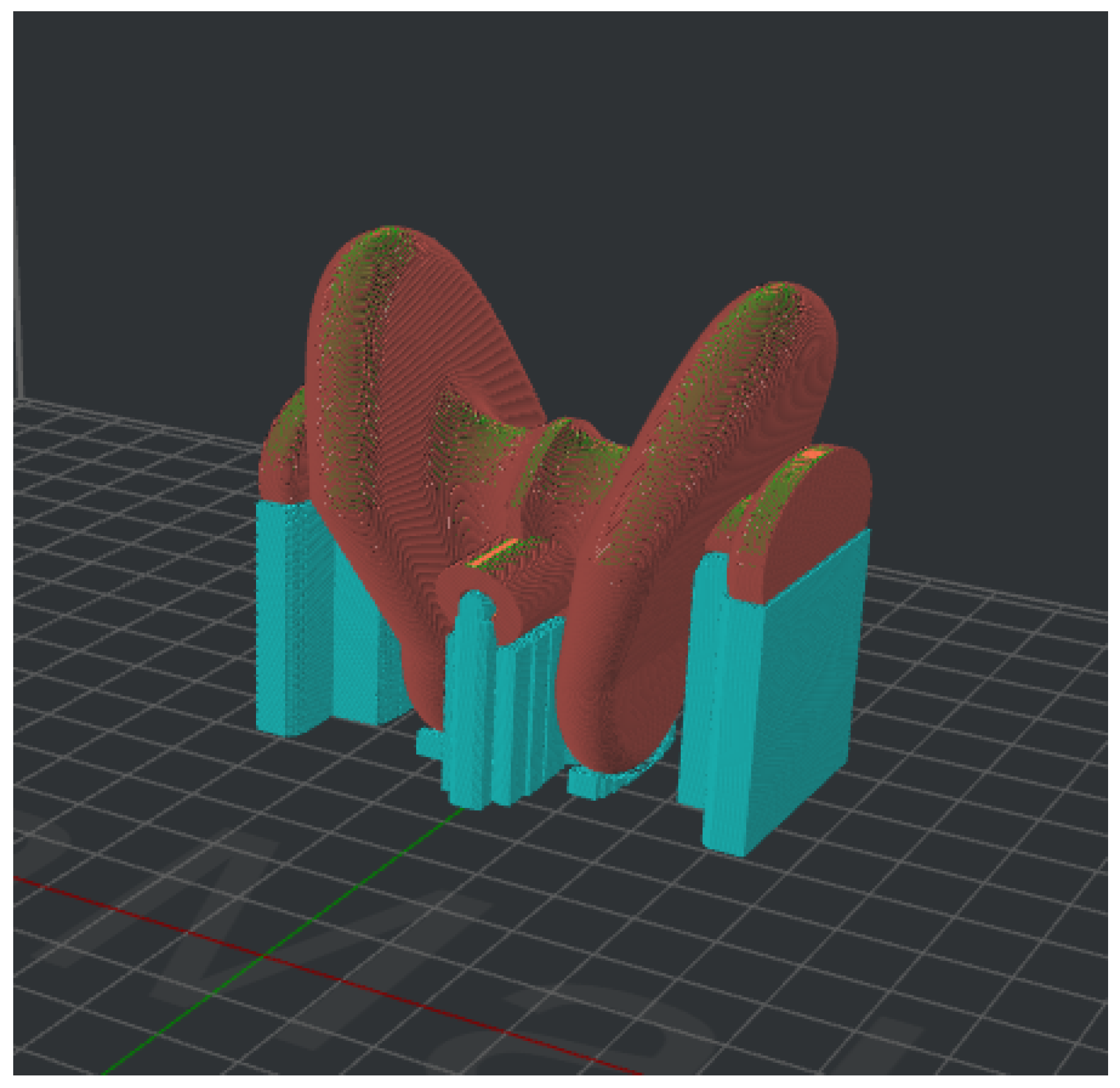
2.2. Three-Dimensional Design and Additive Manufacturing
3. Experimental Tests and Results
3.1. Experimental Setup
3.2. Identification of the Mechanical Response of the Actuator
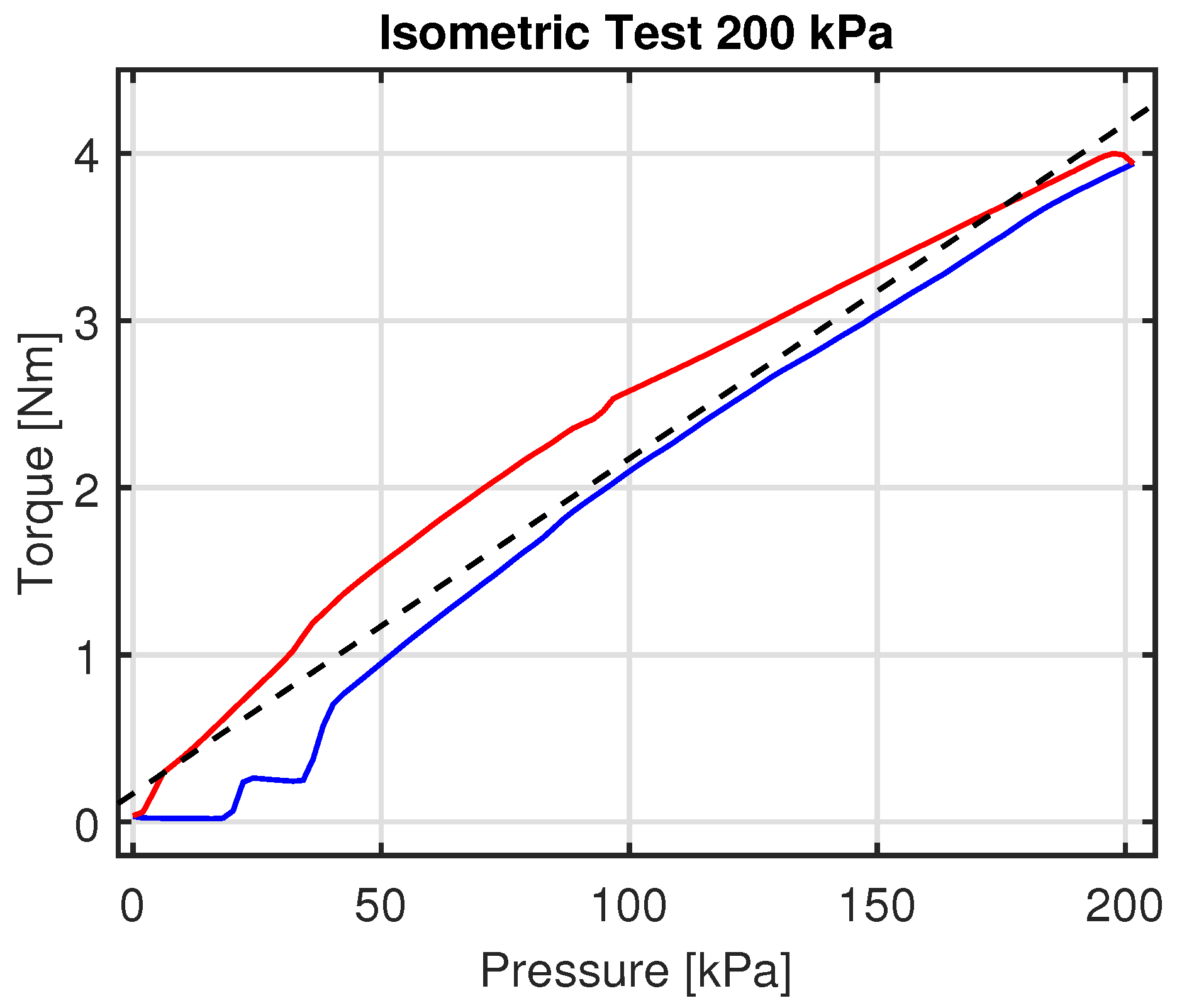
3.3. Isometric Tests
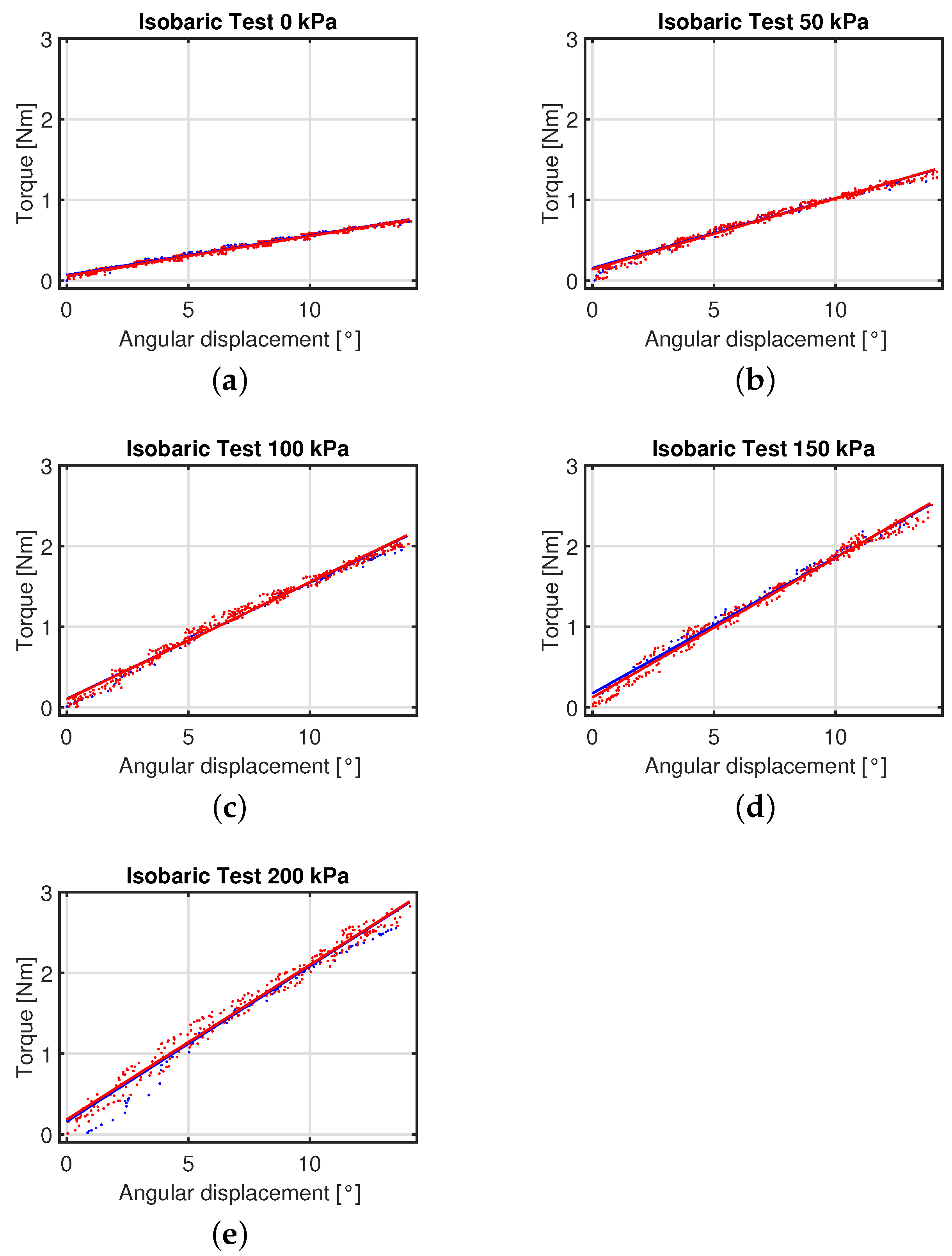
3.4. Isobaric Tests
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tawk, C.; Alici, G. A Review of 3D-Printable Soft Pneumatic Actuators and Sensors: Research Challenges and Opportunities. Adv. Intell. Syst. 2021, 3, 2000223. [Google Scholar] [CrossRef]
- El-Atab, N.; Mishra, R.B.; Al-Modaf, F.; Joharji, L.; Alsharif, A.A.; Alamoudi, H.; Diaz, M.; Qaiser, N.; Hussain, M.M. Soft Actuators for Soft Robotic Applications: A Review. Adv. Intell. Syst. 2020, 2, 2000128. [Google Scholar] [CrossRef]
- Xavier, M.S.; Tawk, C.D.; Zolfagharian, A.; Pinskier, J.; Howard, D.; Young, T.; Lai, J.; Harrison, S.M.; Yong, Y.K.; Bodaghi, M.; et al. Soft Pneumatic Actuators: A Review of Design, Fabrication, Modeling, Sensing, Control and Applications. IEEE Access 2022, 10, 59442–59485. [Google Scholar] [CrossRef]
- Manns, M.; Morales, J.; Frohn, P. Additive manufacturing of silicon based PneuNets as soft robotic actuators. Procedia CIRP 2018, 72, 328–333. [Google Scholar] [CrossRef]
- Yirmibesoglu, O.D.; Morrow, J.; Walker, S.; Gosrich, W.; Canizares, R.; Kim, H.; Daalkhaijav, U.; Fleming, C.; Branyan, C.; Menguc, Y. Direct 3D printing of silicone elastomer soft robots and their performance comparison with molded counterparts. In Proceedings of the 2018 IEEE International Conference on Soft Robotics (RoboSoft), Livorno, Italy, 24–28 April 2018; IEEE: Piscataway, NJ, USA, 2018. [Google Scholar] [CrossRef]
- Gul, J.Z.; Sajid, M.; Rehman, M.M.; Siddiqui, G.U.; Shah, I.; Kim, K.H.; Lee, J.W.; Choi, K.H. 3D printing for soft robotics – A review. Sci. Technol. Adv. Mater. 2018, 19, 243–262. [Google Scholar] [CrossRef]
- Conrad, S.; Speck, T.; Tauber, F.J. Tool changing 3D printer for rapid prototyping of advanced soft robotic elements. Bioinspiration Biomimetics 2021, 16, 055010. [Google Scholar] [CrossRef]
- Belforte, G.; Eula, G.; Ivanov, A.; Sirolli, S. Soft Pneumatic Actuators for Rehabilitation. Actuators 2014, 3, 84–106. [Google Scholar] [CrossRef]
- Su, H.; Hou, X.; Zhang, X.; Qi, W.; Cai, S.; Xiong, X.; Guo, J. Pneumatic Soft Robots: Challenges and Benefits. Actuators 2022, 11, 92. [Google Scholar] [CrossRef]
- Chen, Y.; Chung, H.; Chen, B.; Baoyinjiya. A lobster-inspired articulated shaft for minimally invasive surgery. Robot. Auton. Syst. 2020, 131, 103599. [Google Scholar] [CrossRef]
- Chen, Y.; Le, S.; Tan, Q.C.; Lau, O.; Song, C. A Lobster-Inspired Hybrid Actuator with Rigid and Soft Components. In Proceedings of the Volume 5B: 41st Mechanisms and Robotics Conference, Cleveland, OH, USA, 6–9 August 2017; American Society of Mechanical Engineers: New York, NY, USA, 2017. IDETC-CIE2017. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, T.; Wang, J.; Wang, M.Y.; Li, B.; Zhang, J.X.; Hong, J. Geometric Confined Pneumatic Soft–Rigid Hybrid Actuators. Soft Robot. 2020, 7, 574–582. [Google Scholar] [CrossRef]
- Chen, Y.; Wan, F.; Wu, T.; Song, C. Soft-rigid interaction mechanism towards a lobster-inspired hybrid actuator. J. Micromechan. Microeng. 2017, 28, 014007. [Google Scholar] [CrossRef]
- Chen, Y.; Chung, H.; Chen, B.; Bao, Y.; Sun, Y. The Lobster-inspired Antagonistic Actuation Mechanism towards a Bending Module. In Proceedings of the 2020 IEEE International Conference on Robotics and Automation (ICRA), Paris, France, 31 May–31 August 2020; IEEE: Piscataway, NJ, USA, 2020. [Google Scholar] [CrossRef]
- Chen, Y.; Le, S.; Tan, Q.C.; Lau, O.; Wan, F.; Song, C. A reconfigurable hybrid actuator with rigid and soft components. In Proceedings of the 2017 IEEE International Conference on Robotics and Automation (ICRA), Singapore, 29 May–3 June 2017; IEEE: Piscataway, NJ, USA, 2017. [Google Scholar] [CrossRef]
- Fras, J.; Noh, Y.; Wurdemann, H.; Althoefer, K. Soft fluidic rotary actuator with improved actuation properties. In Proceedings of the 2017 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Vancouver, BC, Canada, 24–28 September 2017; IEEE: Piscataway, NJ, USA, 2017. [Google Scholar] [CrossRef]
- Sun, Y.; Song, Y.S.; Paik, J. Characterization of silicone rubber based soft pneumatic actuators. In Proceedings of the 2013 IEEE/RSJ International Conference on Intelligent Robots and Systems, Tokyo, Japan, 3–7 November 2013; IEEE: Piscataway, NJ, USA, 2013. [Google Scholar] [CrossRef]
- Jamil, B.; Yoo, G.; Choi, Y.; Rodrigue, H. Proprioceptive Soft Pneumatic Gripper for Extreme Environments Using Hybrid Optical Fibers. IEEE Robot. Autom. Lett. 2021, 6, 8694–8701. [Google Scholar] [CrossRef]
- Babu, S.P.M.; Sadeghi, A.; Mondini, A.; Mazzolai, B. Antagonistic Pneumatic Actuators with Variable Stiffness for Soft Robotic Applications. In Proceedings of the 2019 2nd IEEE International Conference on Soft Robotics (RoboSoft), Seoul, Repulic of Korea, 14–18 April 2019; IEEE: Piscataway, NJ, USA, 2019. [Google Scholar] [CrossRef]
- Zhao, S.; Li, D.; Xiang, J. Design and application of PneuNets bending actuator. Aircr. Eng. Aerosp. Technol. 2020, 92, 1539–1546. [Google Scholar] [CrossRef]
- Baiden, D.; Wilkening, A.; Ivlev, O. Safety and handling concept for assistive robotic devices with pneumatic rotary soft-actuators. In Proceedings of the 2011 IEEE/ASME International Conference on Advanced Intelligent Mechatronics (AIM), Budapest, Hungary, 3–7 July 2011; IEEE: Piscataway, NJ, USA, 2011. [Google Scholar] [CrossRef]
- Pan, Q.; Chen, S.; Chen, F.; Zhu, X. Programmable soft bending actuators with auxetic metamaterials. Sci. China Technol. Sci. 2020, 63, 2518–2526. [Google Scholar] [CrossRef]
- Rafsanjani, A.; Bertoldi, K.; Studart, A.R. Programming soft robots with flexible mechanical metamaterials. Sci. Robot. 2019, 4, eaav7874. [Google Scholar] [CrossRef]
- Kelkar, P.U.; Kim, H.S.; Cho, K.H.; Kwak, J.Y.; Kang, C.Y.; Song, H.C. Cellular Auxetic Structures for Mechanical Metamaterials: A Review. Sensors 2020, 20, 3132. [Google Scholar] [CrossRef]
- Teng, X.C.; Ren, X.; Zhang, Y.; Jiang, W.; Pan, Y.; Zhang, X.G.; Zhang, X.Y.; Xie, Y.M. A simple 3D re-entrant auxetic metamaterial with enhanced energy absorption. Int. J. Mech. Sci. 2022, 229, 107524. [Google Scholar] [CrossRef]
- Lu, Z.; Wang, Q.; Li, X.; Yang, Z. Elastic properties of two novel auxetic 3D cellular structures. Int. J. Solids Struct. 2017, 124, 46–56. [Google Scholar] [CrossRef]
- Lei, M.; Hong, W.; Zhao, Z.; Hamel, C.; Chen, M.; Lu, H.; Qi, H.J. 3D Printing of Auxetic Metamaterials with Digitally Reprogrammable Shape. ACS Appl. Mater. Interfaces 2019, 11, 22768–22776. [Google Scholar] [CrossRef]
- Photiou, D.; Avraam, S.; Sillani, F.; Verga, F.; Jay, O.; Papadakis, L. Experimental and Numerical Analysis of 3D Printed Polymer Tetra-Petal Auxetic Structures under Compression. Appl. Sci. 2021, 11, 10362. [Google Scholar] [CrossRef]
- Lvov, V.; Senatov, F.; Korsunsky, A.; Salimon, A. Design and mechanical properties of 3D-printed auxetic honeycomb structure. Mater. Today Commun. 2020, 24, 101173. [Google Scholar] [CrossRef]
- Choudhry, N.K.; Biranchi Panda, S.K. In-plane energy absorption characteristics of a modified re-entrant auxetic structure fabricated via 3D printing. Compos. Part B Eng. 2022, 228, 109437. [Google Scholar] [CrossRef]
- Simons, M.F.; Digumarti, K.M.; Conn, A.T.; Rossiter, J. Tiled Auxetic Cylinders for Soft Robots. In Proceedings of the 2019 2nd IEEE International Conference on Soft Robotics (RoboSoft), Seoul, Republic of Korea, 14–18 April 2019; IEEE: Piscataway, NJ, USA, 2019. [Google Scholar] [CrossRef]
- Lakes, R. Foam Structures with a Negative Poisson’s Ratio. Science 1987, 235, 1038–1040. [Google Scholar] [CrossRef] [PubMed]
- Yang, L.; Harrysson, O.; West, H.; Cormier, D. Mechanical properties of 3D re-entrant honeycomb auxetic structures realized via additive manufacturing. Int. J. Solids Struct. 2015, 69–70, 475–490. [Google Scholar] [CrossRef]
- Mustahsan, F.; Khan, S.Z.; Zaidi, A.A.; Alahmadi, Y.H.; Mahmoud, E.R.I.; Almohamadi, H. Re-Entrant Honeycomb Auxetic Structure with Enhanced Directional Properties. Materials 2022, 15, 8022. [Google Scholar] [CrossRef] [PubMed]
- Grima, J.N.; Gatt, R.; Alderson, A.; Evans, K.E. On the potential of connected stars as auxetic systems. Mol. Simul. 2005, 31, 925–935. [Google Scholar] [CrossRef]
- Yang, L.; Ye, M.; Huang, Y.; Dong, J. Mechanics Characteristics of a 3D Star-Shaped Negative Poisson’s Ratio Composite Structure. Materials 2023, 16, 3950. [Google Scholar] [CrossRef]
- Nečemer, B.; Glodež, S.; Novak, N.; Kramberger, J. Numerical modelling of a chiral auxetic cellular structure under multiaxial loading conditions. Theor. Appl. Fract. Mech. 2020, 107, 102514. [Google Scholar] [CrossRef]
- Huang, H.H.; Wong, B.L.; Chou, Y.C. Design and properties of 3D-printed chiral auxetic metamaterials by reconfigurable connections. Phys. Status Solidi (b) 2016, 253, 1557–1564. [Google Scholar] [CrossRef]
- Guo, M.F.; Yang, H.; Ma, L. 3D lightweight double arrow-head plate-lattice auxetic structures with enhanced stiffness and energy absorption performance. Compos. Struct. 2022, 290, 115484. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, W.; Zhou, G.; Gao, Q.; Wang, C. Optimization of an auxetic jounce bumper based on Gaussian process metamodel and series hybrid GA-SQP algorithm. Struct. Multidiscip. Optim. 2017, 57, 2515–2525. [Google Scholar] [CrossRef]
- Roychoudhury, A.; Singamneni, S.; Das, S. Modification of a re-entrant sinusoidal auxetic structure with a central stiffener. Mater. Today Proc. 2023, in press. [CrossRef]
- Shankar, P.; Ju, J.; Summers, J.D.; Ziegert, J.C. Design of Sinusoidal Auxetic Structures for High Shear Flexure. In Proceedings of the Volume 3: 30th Computers and Information in Engineering Conference, Parts A and B. ASMEDC, Montreal, QC, Canada, 15–18 August 2010. [Google Scholar] [CrossRef]
- Elipe, J.C.Á.; Lantada, A.D. Comparative study of auxetic geometries by means of computer-aided design and engineering. Smart Mater. Struct. 2012, 21, 105004. [Google Scholar] [CrossRef]
- Jiang, F.; Yang, S.; Qi, C.; Liu, H.T. Two plateau characteristics of re-entrant auxetic honeycomb along concave direction. Thin-Walled Struct. 2022, 179, 109665. [Google Scholar] [CrossRef]
- Bora, K.M.; Varshney, S.K.; Kumar, C.S. Rounded corner thicken strut re-entrant auxetic honeycomb: Analytical and numerical modeling. Mech. Res. Commun. 2024, 136, 104246. [Google Scholar] [CrossRef]
- Scarpa, F. Auxetic materials for bioprostheses [In the Spotlight]. IEEE Signal Process. Mag. 2008, 25, 126–128. [Google Scholar] [CrossRef]
- Alomarah, A.; Masood, S.H.; Sbarski, I.; Faisal, B.; Gao, Z.; Ruan, D. Compressive properties of 3D printed auxetic structures: Experimental and numerical studies. Virtual Phys. Prototyp. 2019, 15, 1–21. [Google Scholar] [CrossRef]
- Evans, K.E.; Alderson, A. Auxetic Materials: Functional Materials and Structures from Lateral Thinking! Adv. Mater. 2000, 12, 617–628. [Google Scholar] [CrossRef]
- Cabras, L.; Brun, M. Auxetic two-dimensional lattices with Poisson’s ratio arbitrarily close to −1. Proc. R. Soc. A Math. Phys. Eng. Sci. 2014, 470, 20140538. [Google Scholar] [CrossRef]
- Fu, M.; Xu, O.; Hu, L.; Yu, T. Nonlinear shear modulus of re-entrant hexagonal honeycombs under large deformation. Int. J. Solids Struct. 2016, 80, 284–296. [Google Scholar] [CrossRef]
- Gibson, L.J.; Ashby, M.F. Cellular Solids; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar] [CrossRef]
- Yap, H.K.; Ng, H.Y.; Yeow, C.H. High-Force Soft Printable Pneumatics for Soft Robotic Applications. Soft Robot. 2016, 3, 144–158. [Google Scholar] [CrossRef]
- Arleo, L.; Stano, G.; Percoco, G.; Cianchetti, M. I-support soft arm for assistance tasks: A new manufacturing approach based on 3D printing and characterization. Prog. Addit. Manuf. 2020, 6, 243–256. [Google Scholar] [CrossRef]
- Dragone, D.; Randazzini, L.; Capace, A.; Nesci, F.; Cosentino, C.; Amato, F.; De Momi, E.; Colao, R.; Masia, L.; Merola, A. Design, Computational Modelling and Experimental Characterization of Bistable Hybrid Soft Actuators for a Controllable-Compliance Joint of an Exoskeleton Rehabilitation Robot. Actuators 2022, 11, 32. [Google Scholar] [CrossRef]
- Stano, G.; Arleo, L.; Percoco, G. Additive Manufacturing for Soft Robotics: Design and Fabrication of Airtight, Monolithic Bending PneuNets with Embedded Air Connectors. Micromachines 2020, 11, 485. [Google Scholar] [CrossRef]




| Main 3D-Printing Parameters for Soft Pneumatic Actuator | |
|---|---|
| Material | TPU Ninjaflex 85A (Ninjatek®) |
| 3D Printer | Raise 3D Pro 2 |
| Layer height | 0.15 mm |
| Nozzle diameter | 0.4 mm |
| Perimeter lines | 5 |
| Infill density | 100% |
| Infill pattern | Grid |
| Support | Touch platform only |
| Extrusion temperature | 220 °C |
| Print speed | 15 mm/s |
| Heated bed temperature | 0 °C |
| Printing time | 16 h 48 min |
| Main 3D-Printing Parameters for Rigid Frames | |
|---|---|
| Material | PLA (Raise3D®) |
| 3D Printer | Raise 3D Pro 2 |
| Layer height | 0.1 mm |
| Nozzle diameter | 0.6 mm |
| Infill density | 15% |
| Infill pattern | Grid |
| Support | Touch platform only |
| Extrusion temperature | 205 °C |
| Print speed | 50 mm/s |
| Heated bed temperature | 60 °C |
| Printing time | 4 h 26 min |
| Linear Fitting | |||
|---|---|---|---|
| Expansion | Compression | ||
| 0.932 | 0.898 | ||
| Pressure [kPa] | Linear Fitting | |||
|---|---|---|---|---|
| Expansion | Compression | |||
| 0 | 1.49 | 0.010 | ||
| 50 | 1.07 | 0.013 | ||
| 100 | 0.32 | 0.007 | ||
| 150 | 2.13 | 0.051 | ||
| 200 | 0.97 | 0.027 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Donadio, F.F.; Dragone, D.; Procopio, A.; Amato, F.; Cosentino, C.; Merola, A. Design and Characterisation of a 3D-Printed Pneumatic Rotary Actuator Exploiting Enhanced Elastic Properties of Auxetic Metamaterials. Actuators 2024, 13, 329. https://doi.org/10.3390/act13090329
Donadio FF, Dragone D, Procopio A, Amato F, Cosentino C, Merola A. Design and Characterisation of a 3D-Printed Pneumatic Rotary Actuator Exploiting Enhanced Elastic Properties of Auxetic Metamaterials. Actuators. 2024; 13(9):329. https://doi.org/10.3390/act13090329
Chicago/Turabian StyleDonadio, Francesca Federica, Donatella Dragone, Anna Procopio, Francesco Amato, Carlo Cosentino, and Alessio Merola. 2024. "Design and Characterisation of a 3D-Printed Pneumatic Rotary Actuator Exploiting Enhanced Elastic Properties of Auxetic Metamaterials" Actuators 13, no. 9: 329. https://doi.org/10.3390/act13090329
APA StyleDonadio, F. F., Dragone, D., Procopio, A., Amato, F., Cosentino, C., & Merola, A. (2024). Design and Characterisation of a 3D-Printed Pneumatic Rotary Actuator Exploiting Enhanced Elastic Properties of Auxetic Metamaterials. Actuators, 13(9), 329. https://doi.org/10.3390/act13090329











