Soft Electrohydraulic Bending Actuators for Untethered Underwater Robots
Abstract
1. Introduction
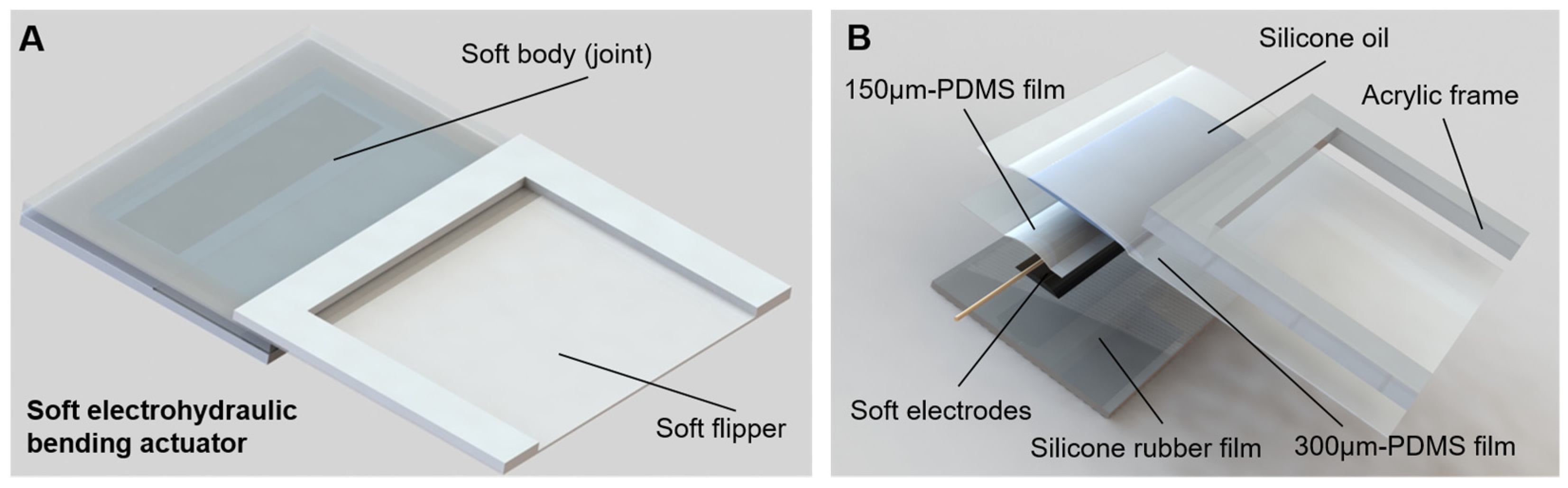
2. Soft Electrohydraulic Bending Actuator
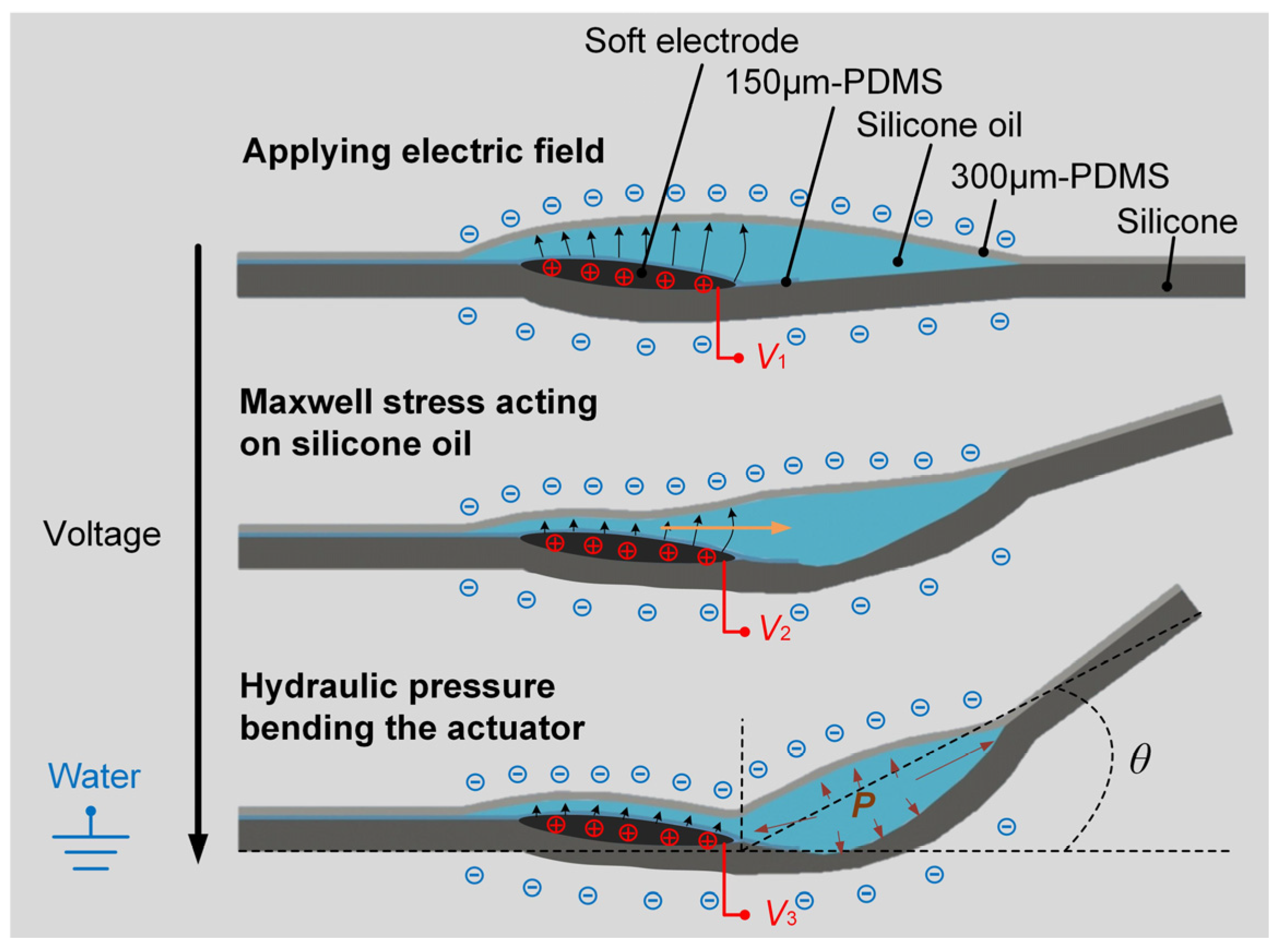
2.1. Actuator Design and Operating Principle
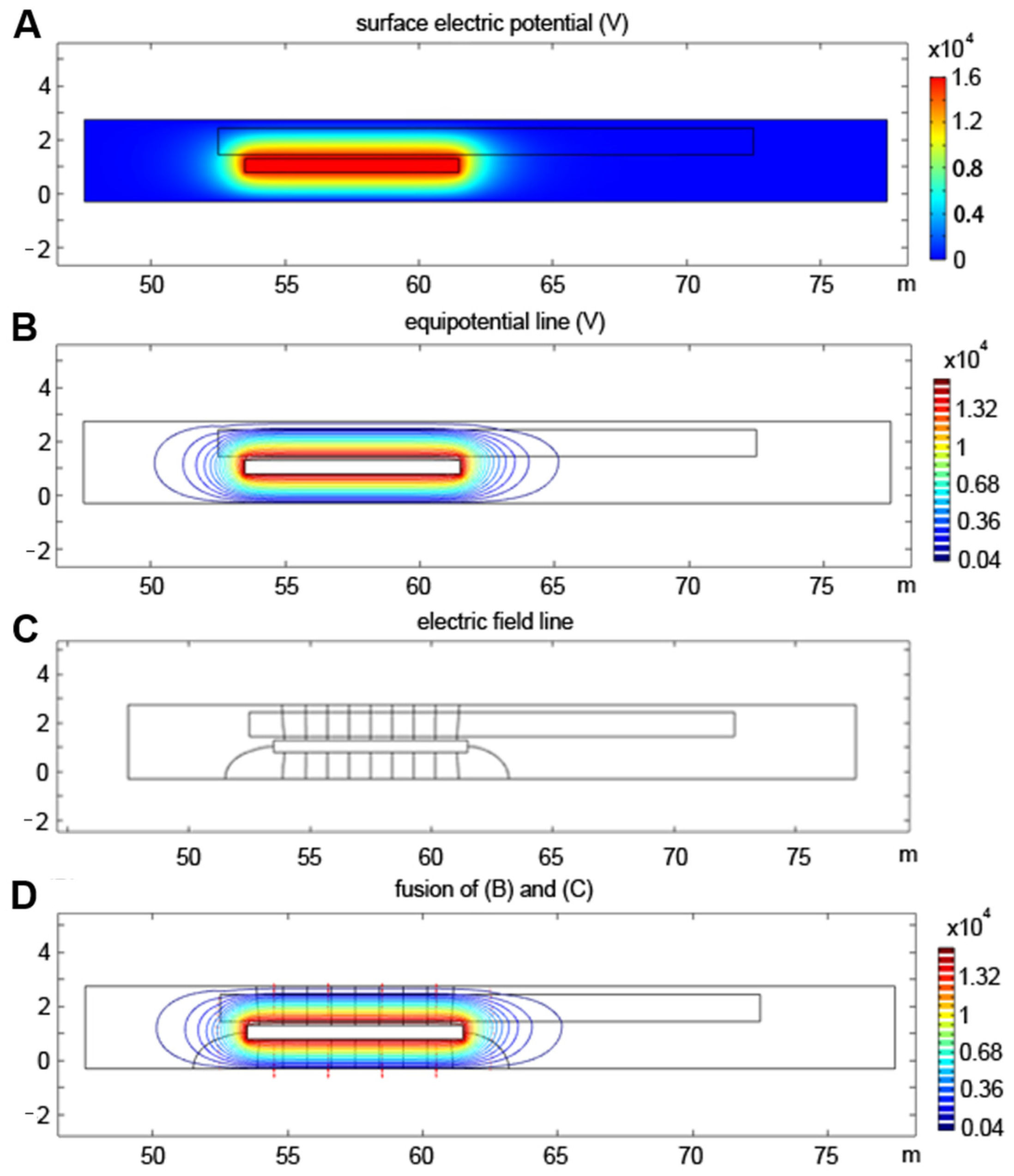
2.2. Simulations of the Actuator
2.3. Materials of the Actuator
2.4. Research and Testing of Parameters
2.4.1. Effect of Silicone Rubber Film Thickness on Actuator Bending Performance
2.4.2. Effect of Silicone Oil Volume on Actuator Bending Performance
2.5. Determination of Parameters
2.6. Fabrication of the Actuator
2.6.1. The Fabrication Process of 1.1 mm-Thick Silicone Rubber Film
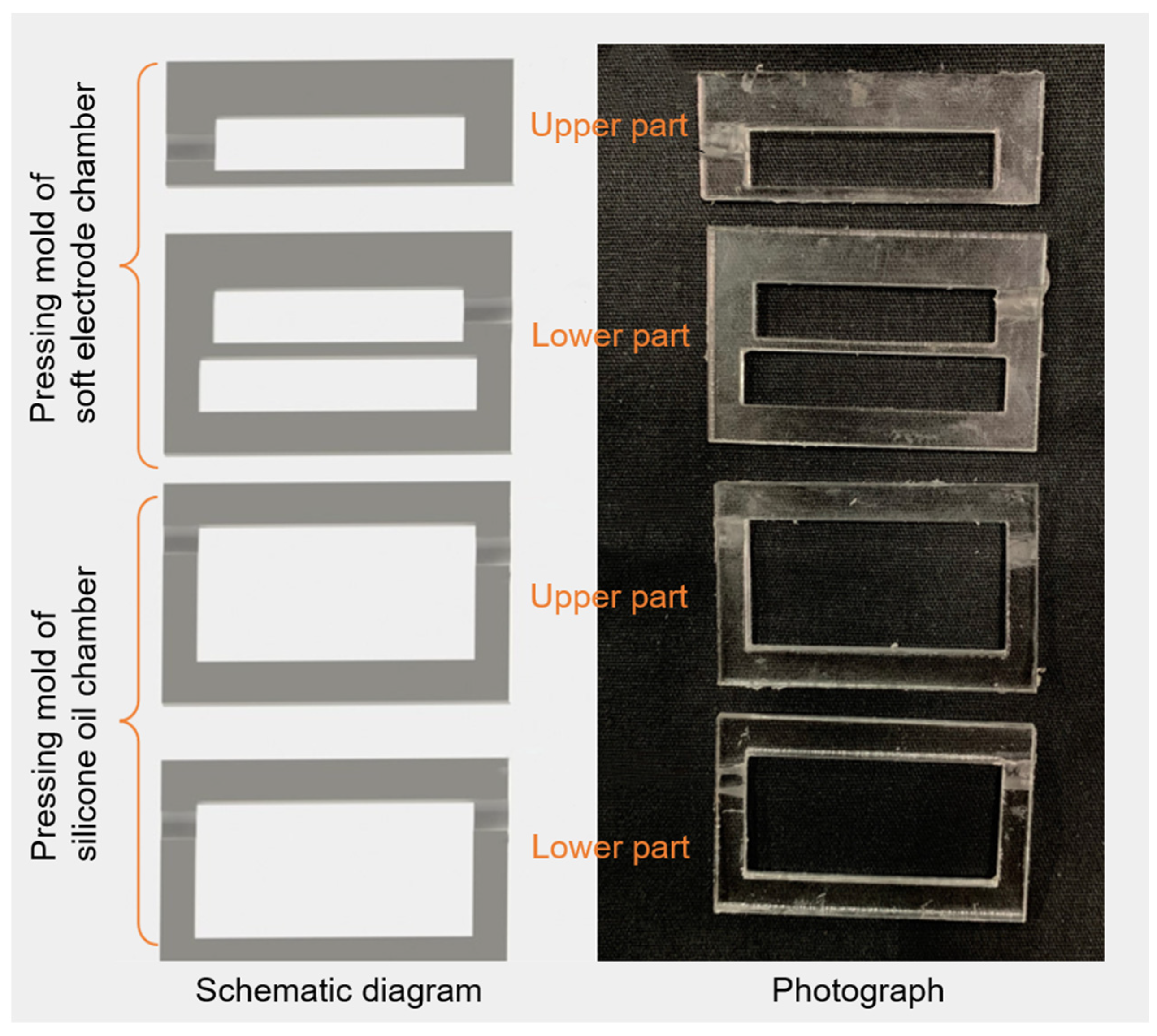
2.6.2. The Fabrication Process of the Pressing Mold
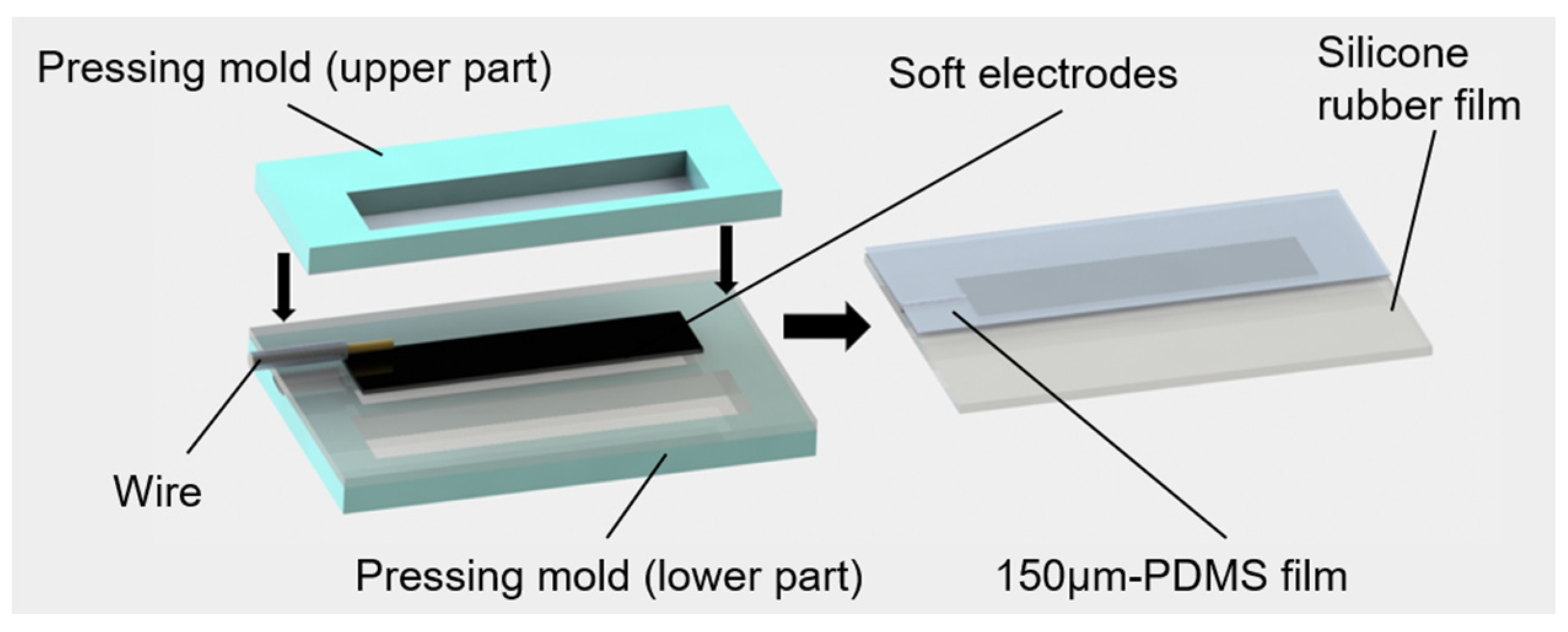
2.6.3. The Fabrication Process of the Soft Electrodes Chamber
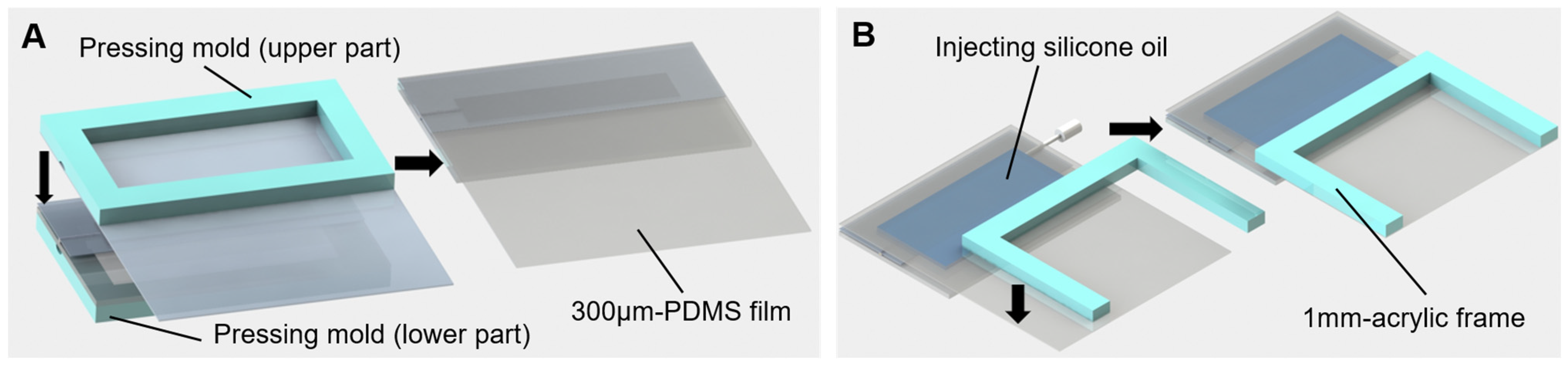
2.6.4. The Fabrication Process of the Silicone Oil Chamber
3. Untethered Underwater Robot
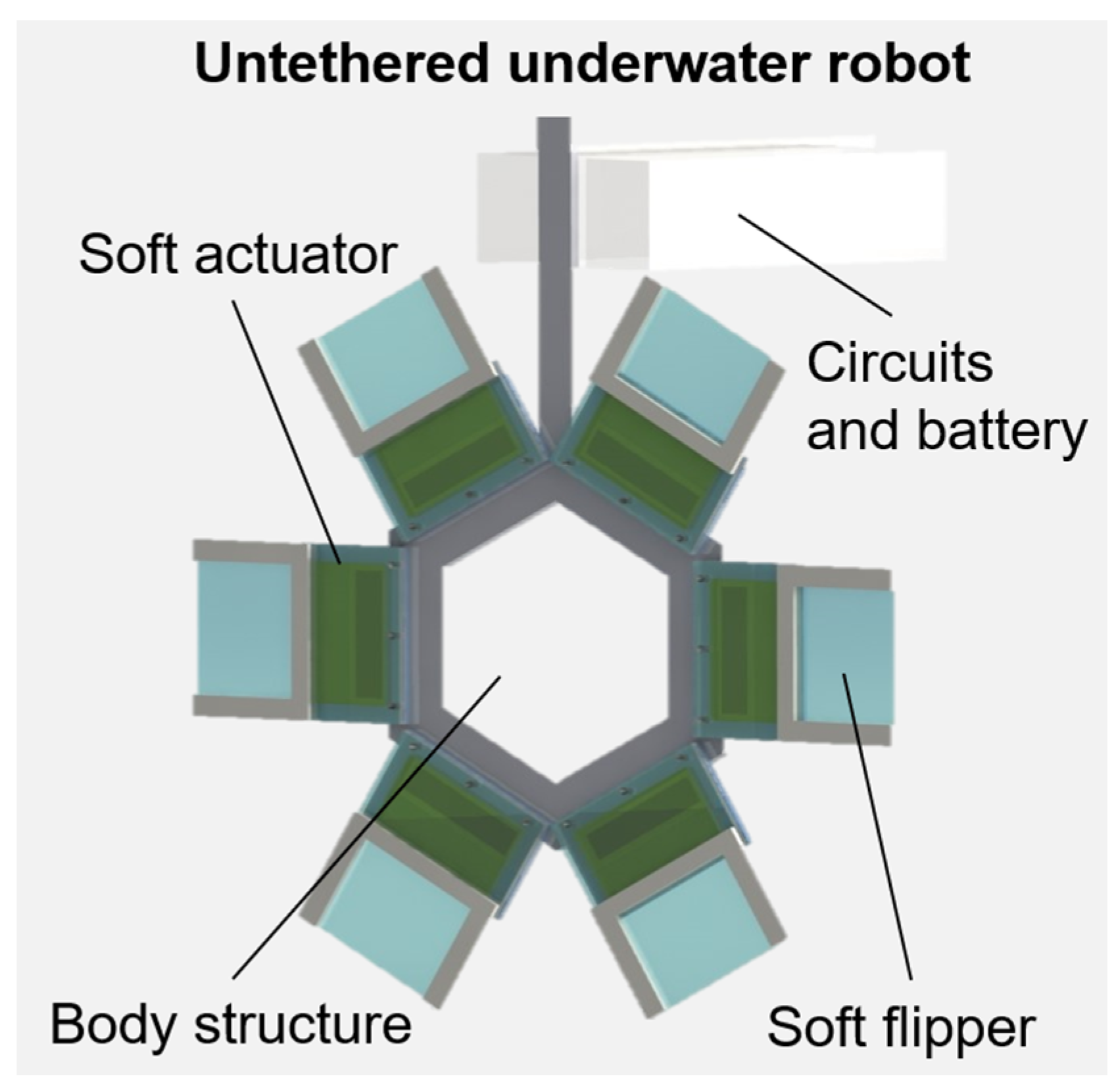
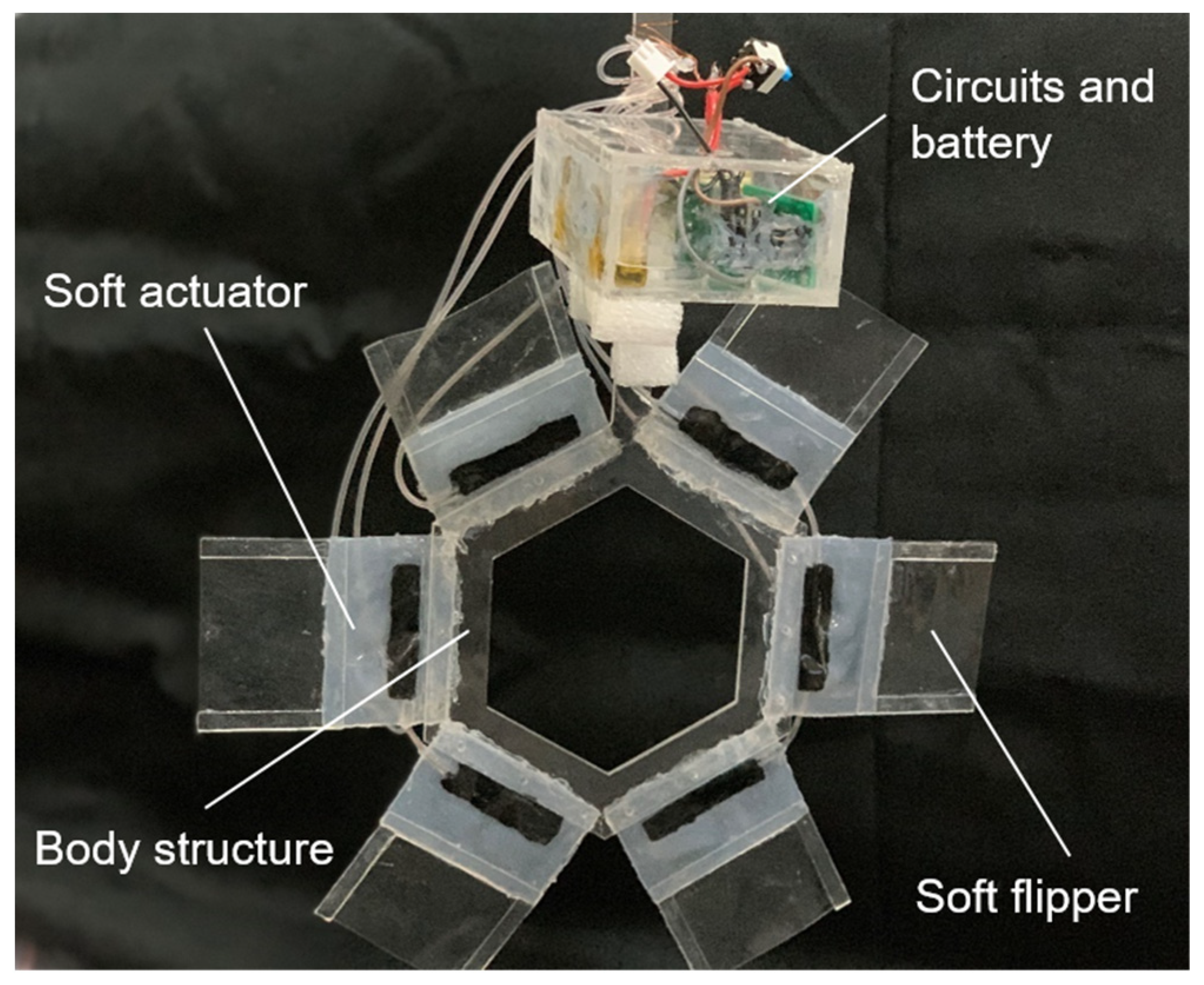
3.1. Structural Design of the Underwater Robot
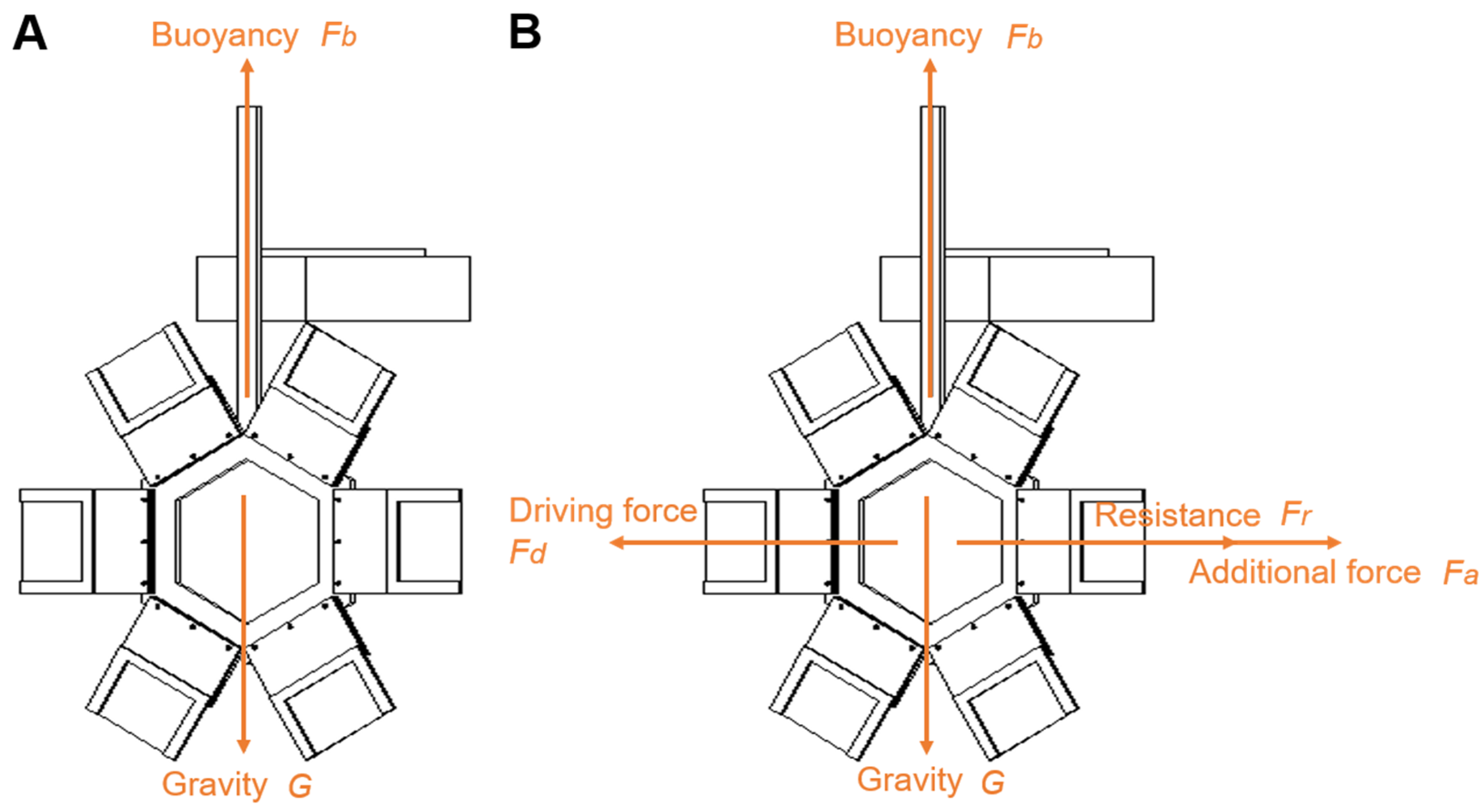
3.2. Force Analysis
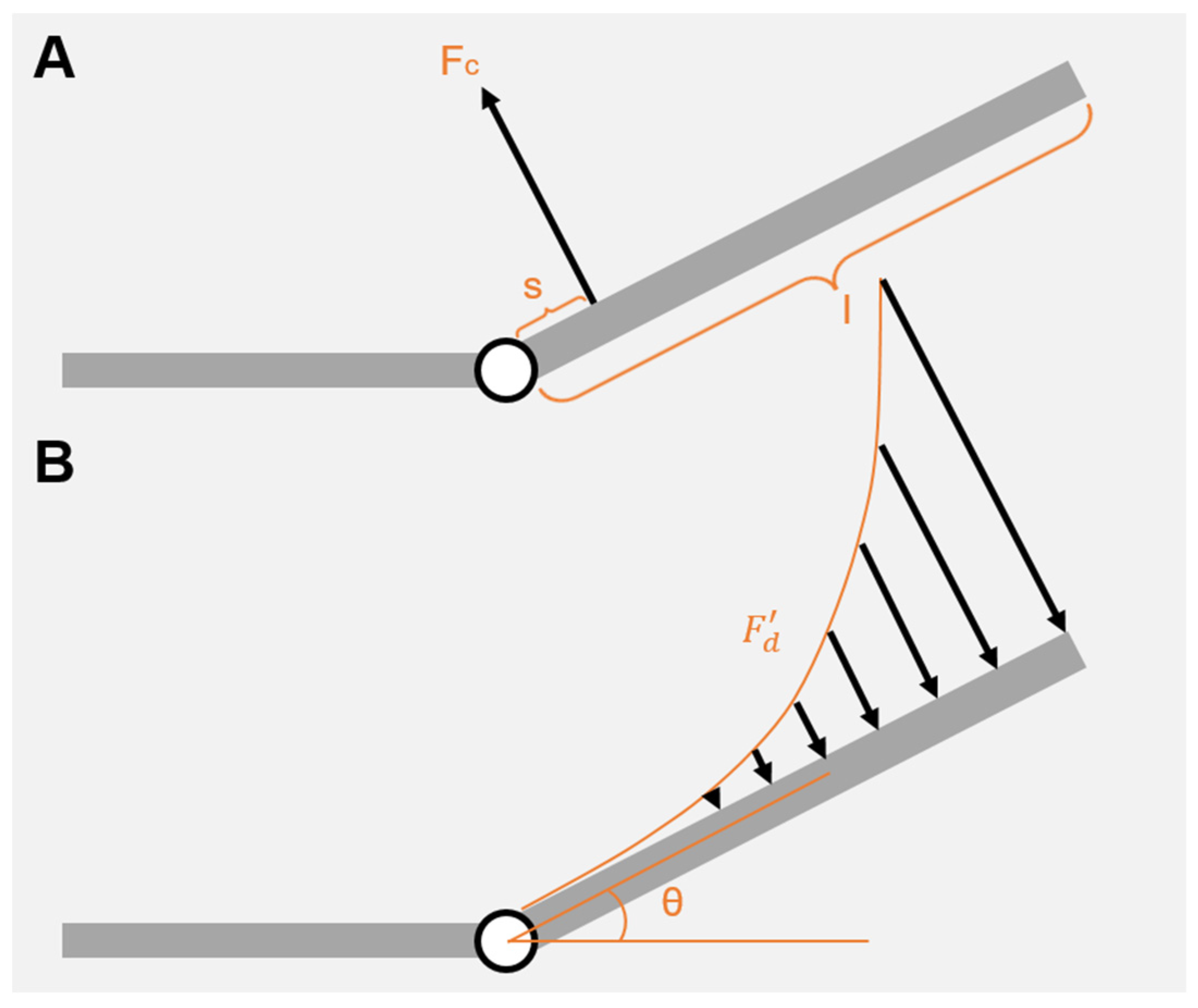
3.2.1. Static Force Analysis
3.2.2. Dynamic Force Analysis
3.3. Swimming Posture Design of the Underwater Robot
3.3.1. Static Suspension
3.3.2. Draining and Propelling
3.3.3. Decelerating and Drifting
3.3.4. Action Recovery
3.4. Design of Control Circuit
3.5. Production of the Underwater Robot
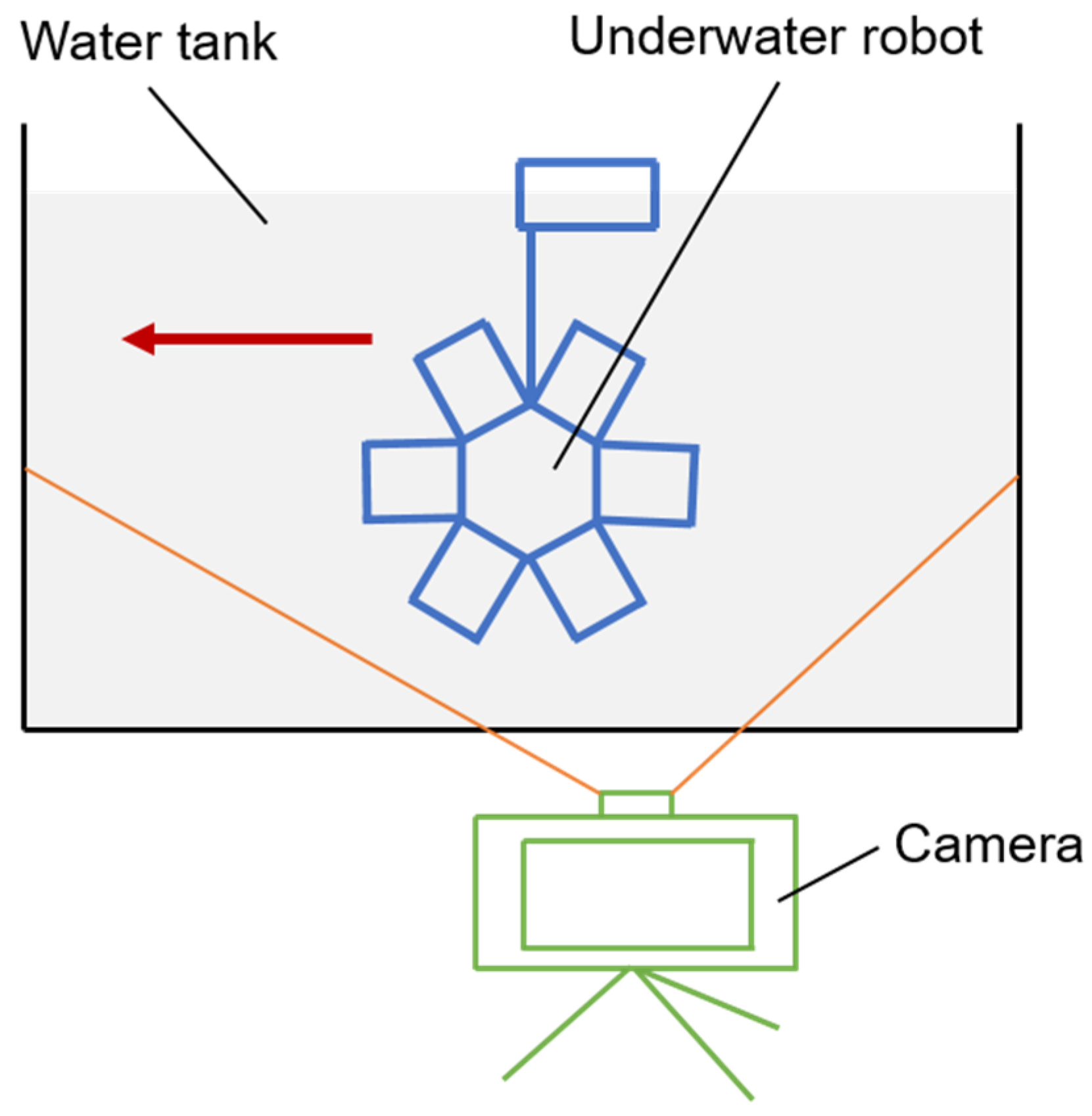
3.6. Underwater Robot Test Device
3.7. Swimming Test of the Underwater Robot
4. Conclusions
- (1)
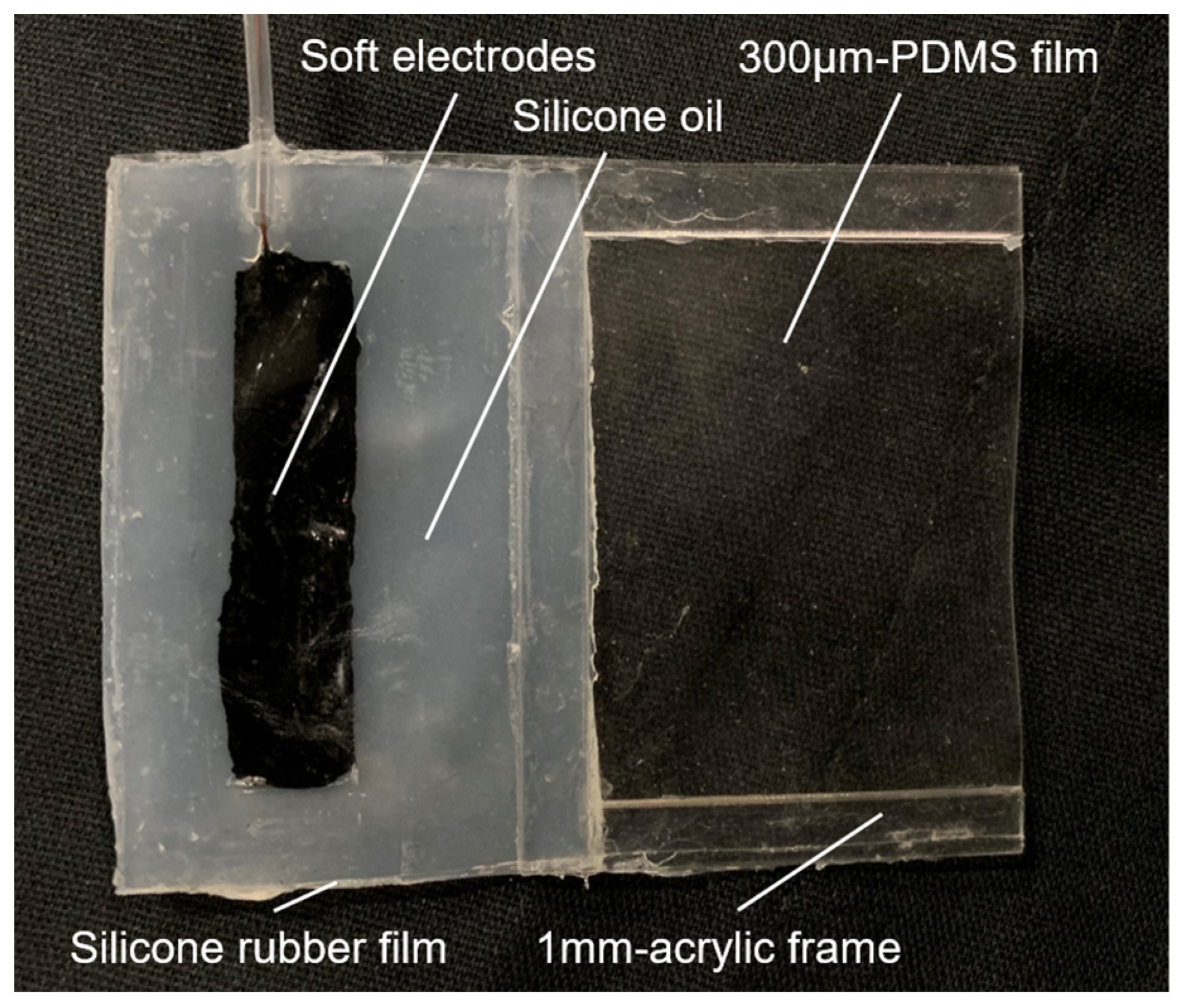
- We designed a type of soft electrohydraulic bending actuators, mostly consisting of five layers. A 5-A silicone rubber film made up the first layer. In the second layer, there were soft electrodes made of black carbon paste. The third layer fully enclosed the soft electrodes with a 150 μm-thick PDMS film. The fourth layer consisted of a closed chamber filled with dimethyl silicone oil, which was the working liquid that achieved bending during the actuation process. This silicone oil also acted as an insulating medium to protect the silicone film at the edge of the electrode from being broken down by the electric field. The final layer of the construction was a 300 μm-thick PDMS film. Furthermore, we used an acrylic frame and a soft flipper to amplify the actuator’s bending motion. In order to create an electrostatic field and squeeze the silicone oil into motion, which allowed the actuator to bend, the soft electrode served as a positive electrode and the surrounding water as a ground electrode. When a square wave voltage was applied, the soft electrohydraulic bending actuator could generate continuous flapping motions.
- (2)
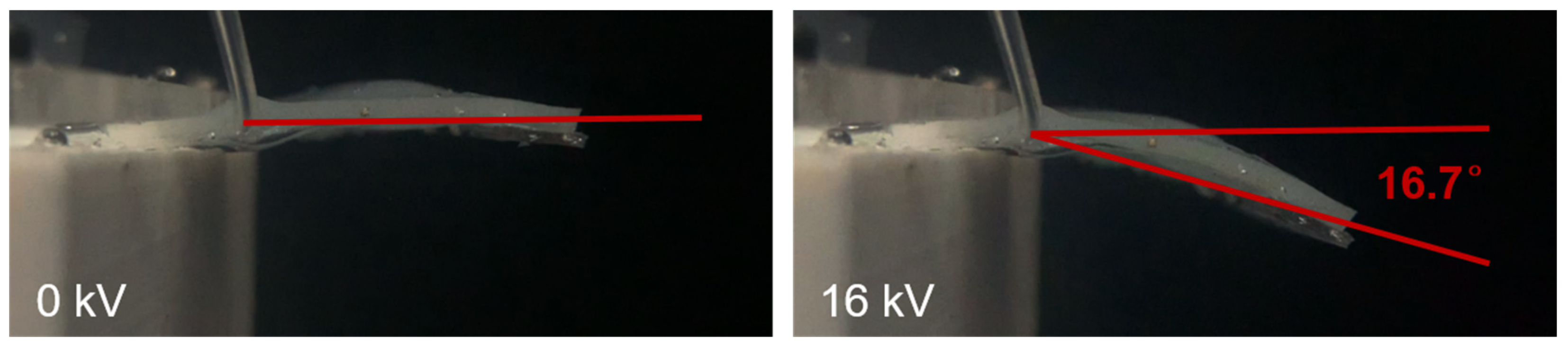
- Through electric field simulations and experiments, we determined the actuator’s basic parameters. We studied the effects of silicone rubber film thickness and silicone oil volume in the silicone oil chamber on the bending degree and output force of the actuator and obtained that the optimal silicone rubber film thickness was 1.1 mm and the optimal silicone oil volume was 0.8 mL. Combining the results of our research with the experimental data, we found that the highest input voltage for the actuator was 16 kV, and the maximum bending angle of the actuator was 16.7°. After that, we created a mold using a laser cutting machine and paired it with the mold pressing technique to produce an actuator prototype.
- (3)
- We created the primary construction of the underwater robot based on the swimming ways of Haliclystus auricula. This structure consisted of a body frame, six soft electrohydraulic bending actuators, and a box that housed lithium batteries and a control circuit. We constructed a mechanical model of the underwater robot, examined its force state when it was suspended and swimming underwater, obtained the mechanical equation that propelled the robot to swim, and used theoretical deduction to infer the underwater robot’s properties. It was concluded that there existed a positive correlation between the driving force and the maximum speed. When the driving force was stronger, the underwater robot could reach a faster maximum speed. The conclusions drawn from the analytical mechanics model provide a theoretical basis for robot swimming tests.
- (4)
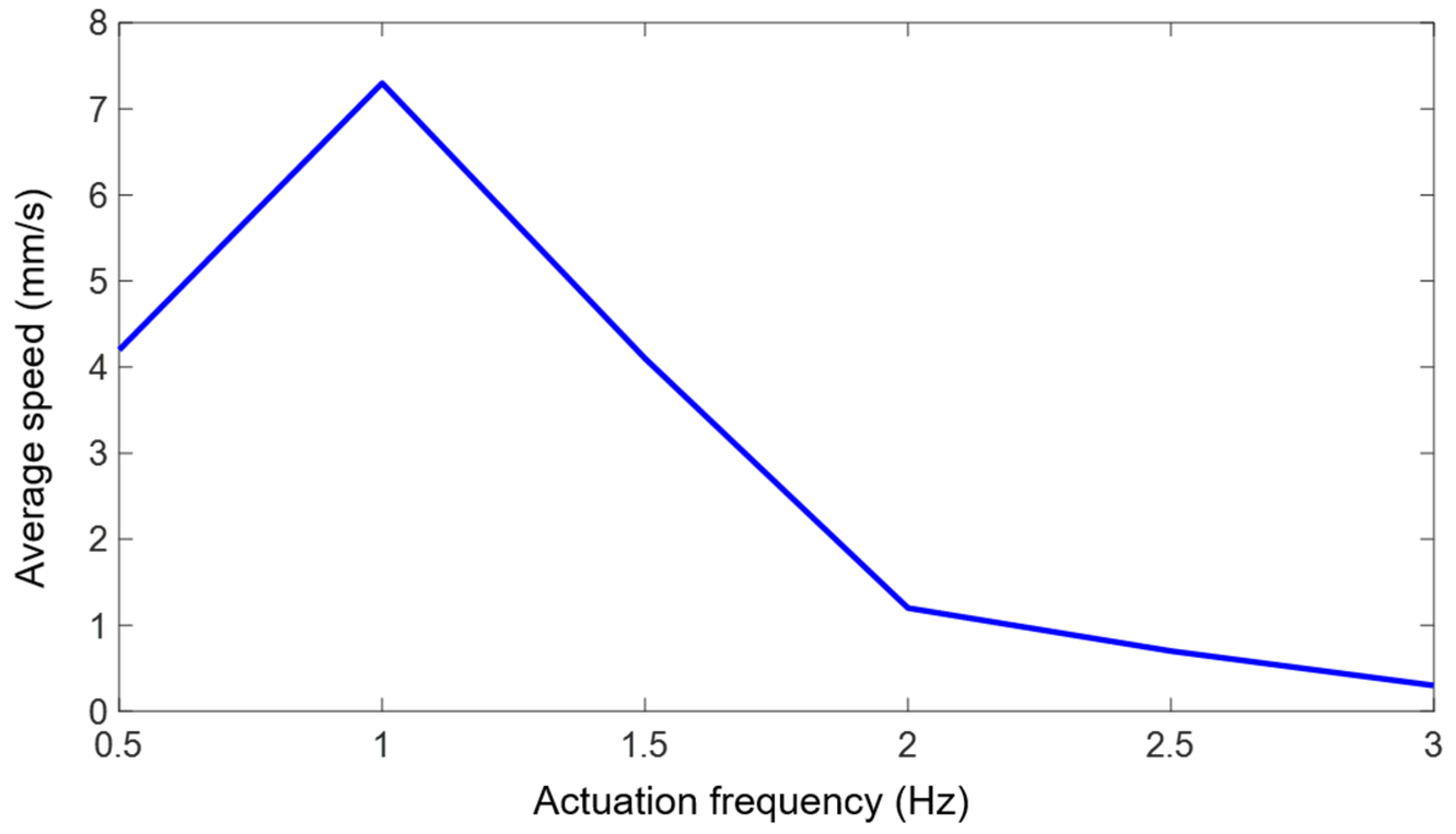
- We designed the swimming action of the underwater robot based on the swimming posture of Haliclystus auricula, which mainly included four parts. In order to realize the untethered motions of the robot, we designed a high-voltage circuit board for driving and controlling the robot, with a built-in 7.4 V lithium battery for power supply. The circuit board could both receive control signals from smart phones to enable remote control and can output high-voltage square wave signals to drive the robot. For testing, we built an underwater robot prototype and fed it signals at various frequencies. The experiment results demonstrate that the horizontal movement speed of the underwater robot could reach its maximum value (7.3 mm/s) when the input signal frequency was 1 Hz. This is because the signal frequency of 1 Hz is closest to the natural frequency of the actuator when moving underwater, which can maximize the displacement of the actuator within a certain period of time.
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, Y.; Li, Y.; Cheng, R.; Shen, S.; Yi, J.; Peng, X.; Ning, C.; Dong, K.; Wang, Z.L. Underwater Monitoring Networks Based on Cable-Structured Triboelectric Nanogenerators. Research 2022, 2022, 9809406. [Google Scholar] [CrossRef]
- Madricardo, F.; Ghezzo, M.; Nesto, N.; Mc Kiver, W.J.; Faussone, G.C.; Fiorin, R.; Riccato, F.; Mackelworth, P.C.; Basta, J.; De Pascalis, F.; et al. How to deal with seafloor marine litter: An overview of the state-of-the-art and future perspectives. Front. Mar. Sci. 2020, 7, 505134. [Google Scholar] [CrossRef]
- Wang, Y.; Chu, H.; Ma, R.; Bai, X.; Cheng, L.; Wang, S.; Tan, M. Learning-Based Discontinuous Path Following Control for a Biomimetic Underwater Vehicle. Research 2024, 7, 0299. [Google Scholar] [CrossRef]
- Panda, J.P.; Mitra, A.; Warrior, H.V. A review on the hydrodynamic characteristics of autonomous underwater vehicles. Proc. Inst. Mech. Eng. Part J. Eng. Marit. Environ. 2021, 235, 15–29. [Google Scholar] [CrossRef]
- Sahoo, A.; Dwivedy, S.K.; Robi, P.S. Advancements in the field of autonomous underwater vehicle. Ocean Eng. 2019, 181, 145–160. [Google Scholar] [CrossRef]
- Fan, R.; Yu, J.; Wang, L.; Xie, G.; Fang, Y.; Hu, Y. Optimized design and implementation of biomimetic robotic dolphin. In Proceedings of the 2005 IEEE International Conference on Robotics and Biomimetics (ROBIO), Hong Kong, China, 5–9 July 2005; pp. 484–489. [Google Scholar]
- Low, K.H.; Chong, C.W. Parametric study of the swimming performance of a fish robot propelled by a flexible caudal fin. Bioinspir. Biomim. 2010, 5, 046002. [Google Scholar] [CrossRef]
- Yao, G.; Liang, J.; Wang, T.; Yang, X.; Shen, Q.; Zhang, Y.; Wu, H.; Tian, W. Development of a Turtle-Like Underwater Vehicle Using Central Pattern Generator. In Proceedings of the 2013 IEEE International Conference on Robotics and Biomimetics (ROBIO), Shenzhen, China, 12–14 December 2013; pp. 44–49. [Google Scholar]
- Liao, L.; Gao, L.; Ngwa, M.; Zhang, D.; Du, J.; Li, B. Adaptive Super-Twisting Sliding Mode Control of Underwater Mechanical Leg with Extended State Observer. Actuators 2023, 12, 373. [Google Scholar] [CrossRef]
- Zhou, C.; Low, K.H. Design and Locomotion Control of a Biomimetic Underwater Vehicle with Fin Propulsion. IEEE-ASME Trans. Mech. 2012, 17, 25–35. [Google Scholar] [CrossRef]
- Li, Z.; Chao, X.; Hameed, I.; Li, J.; Zhao, W.; Jing, X. Biomimetic omnidirectional multi-tail underwater robot. Mech. Syst. Signal Pract. 2022, 173, 109056. [Google Scholar] [CrossRef]
- Yan, S.; Wu, Z.; Wang, J.; Tan, M.; Yu, J. Marine Autonomous Navigation for Biomimetic Underwater Robots Based on Deep Stereo Attention Network. In Proceedings of the 2021 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Prague, Czech Republic, 16 December 2021; pp. 8418–8423. [Google Scholar]
- Zhong, Y.; Tang, W.; Zhang, C.; Jiao, Z.; Wu, D.; Liu, W.; Yang, H.; Zou, J. Programmable thermochromic soft actuators with “two dimensional” bilayer architectures for soft robotics. Nano Energy 2022, 102, 107741. [Google Scholar] [CrossRef]
- Zhong, Y.; Tang, W.; Xu, H.; Qin, K.; Yan, D.; Fan, X.; Qu, Y.; Li, Z.; Jiao, Z.; Yang, H.; et al. Phase-transforming mechanical metamaterials with dynamically controllable shape-locking performance. Natl. Sci. Rev. 2023, 10, nwad192. [Google Scholar] [CrossRef]
- Wu, H.; Chen, Y.; Xu, W.; Xin, C.; Wu, T.; Feng, W.; Yu, H.; Chen, C.; Jiang, S.; Zhang, Y.; et al. High-performance Marangoni hydrogel rotors with asymmetric porosity and drag reduction profile. Nat. Commun. 2023, 14, 20. [Google Scholar] [CrossRef]
- Chen, Y.; Wu, H.; Li, R.; Jiang, S.; Zhou, S.; Cui, Z.; Tao, Y.; Zheng, X.; Zhang, Q.; Li, J.; et al. High-performance liquid metal electromagnetic actuator fabricated by femtosecond laser. Int. J. Extrem. Manuf. 2024, 6, 025503. [Google Scholar] [CrossRef]
- Zhang, L.; Zhao, S.; Zhou, X.; Jing, X.; Zhou, Y.; Wang, Y.; Zhu, Y.; Liu, X.; Zhao, Z.; Zhang, D.; et al. A Magnetic-Driven Multi-motion Robot with Position/Orientation Sensing Capability. Research 2023, 6, 0177. [Google Scholar] [CrossRef]
- Tang, W.; Zhang, C.; Zhong, Y.; Zhu, P.; Hu, Y.; Jiao, Z.; Wei, X.; Lu, G.; Wang, J.; Liang, Y.; et al. Customizing a self-healing soft pump for robot. Nat. Commun. 2021, 12, 2247. [Google Scholar] [CrossRef]
- Tang, W.; Lin, Y.; Zhang, C.; Liang, Y.; Wang, J.; Wang, W.; Ji, C.; Zhou, M.; Yang, H.; Zou, J. Self-contained soft electrofluidic actuators. Sci. Adv. 2021, 7, eabf8080. [Google Scholar] [CrossRef]
- Wang, R.; Zhang, C.; Zhang, Y.; Tan, W.; Chen, W.; Liu, L. Soft Underwater Swimming Robots Based on Artificial Muscle. Adv. Mater. Technol. 2023, 8, 2200962. [Google Scholar] [CrossRef]
- Qu, J.; Xu, Y.; Li, Z.; Yu, Z.; Mao, B.; Wang, Y.; Wang, Z.; Fan, Q.; Qian, X.; Zhang, M.; et al. Recent Advances on Underwater Soft Robots. Adv. Intell. Syst. 2024, 6, 2300299. [Google Scholar] [CrossRef]
- Lee, J.; Yoon, Y.; Park, H.; Choi, J.; Jung, Y.; Ko, S.H.; Yeo, W.-H. Bioinspired Soft Robotic Fish for Wireless Underwater Control of Gliding Locomotion. Adv. Intell. Syst. 2022, 4, 2100271. [Google Scholar] [CrossRef]
- Tang, W.; Zhong, Y.; Xu, H.; Qin, K.; Guo, X.; Hu, Y.; Zhu, P.; Qu, Y.; Yan, D.; Li, Z.; et al. Self-protection soft fluidic robots with rapid large-area self-healing capabilities. Nat. Commun. 2023, 14, 6430. [Google Scholar] [CrossRef]
- Li, T.; Li, G.; Liang, Y.; Cheng, T.; Dai, J.; Yang, X.; Liu, B.; Zeng, Z.; Huang, Z.; Luo, Y.; et al. Fast-moving soft electronic fish. Sci. Adv. 2017, 3, e1602045. [Google Scholar] [CrossRef]
- Ye, Z.; Zheng, L.; He, J.; Lin, J.; Chen, Y.; Yu, H.; Wang, Y.; Zhong, W.; Handschuh-Wang, S.; Niu, S.; et al. Liquid-metal soft electronics coupled with multi-legged robots for targeted delivery in the gastrointestinal tract. Device 2024, 2, 100181. [Google Scholar] [CrossRef]
- Chen, S.; Wang, H.-Z.; Liu, T.-Y.; Liu, J. Liquid Metal Smart Materials toward Soft Robotics. Adv. Intell. Syst. 2023, 5, 2200375. [Google Scholar] [CrossRef]
- Wang, Z.; Zhang, B.; He, Q.; Chen, H.; Wang, J.; Yao, Y.; Zhou, N.; Cui, W. Multimaterial Embedded 3D Printing of Composite Reinforced Soft Actuators. Research 2023, 6, 0122. [Google Scholar] [CrossRef]
- Guo, X.; Tang, W.; Qin, K.; Zhong, Y.; Xu, H.; Qu, Y.; Li, Z.; Sheng, Q.; Gao, Y.; Yang, H.; et al. Powerful UAV manipulation via bioinspired self-adaptive soft self-contained gripper. Sci. Adv. 2024, 10, eadn6642. [Google Scholar] [CrossRef]
- Jung, Y.; Kwon, K.; Lee, J.; Ko, S.H. Untethered soft actuators for soft standalone robotics. Nat. Commun. 2024, 15, 3510. [Google Scholar] [CrossRef]
- Feng, M.; Yang, D.; Ren, L.; Wei, G.; Gu, G. X-crossing pneumatic artificial muscles. Sci. Adv. 2023, 9, eadi7133. [Google Scholar] [CrossRef]
- Liu, Z.; Sun, B.; Hu, J.; Zhang, Y.; Lin, Z.; Liang, Y. Ethanol Phase Change Actuator Based on Thermally Conductive Material for Fast Cycle Actuation. Polymers 2021, 13, 4095. [Google Scholar] [CrossRef]
- Kapłon, T.; Milecki, A. Research on the Use of Silicon-Ethanol Composite in Actuators. Materials 2022, 15, 8570. [Google Scholar] [CrossRef]
- García-Samartín, J.F.; Rieker, A.; Barrientos, A. Design, Manufacturing, and Open-Loop Control of a Soft Pneumatic Arm. Actuators 2024, 13, 36. [Google Scholar] [CrossRef]
- Ridremont, T.; Singh, I.; Bruzek, B.; Jamieson, A.; Gu, Y.; Merzouki, R.; Wijesundara, M.B.J. Pneumatically Actuated Soft Robotic Hand and Wrist Exoskeleton for Motion Assistance in Rehabilitation. Actuators 2024, 13, 180. [Google Scholar] [CrossRef]
- Zou, J.; Feng, M.; Ding, N.; Yan, P.; Xu, H.; Yang, D.; Fang, N.X.; Gu, G.; Zhu, X. Muscle-fiber array inspired, multiple-mode, pneumatic artificial muscles through planar design and one-step rolling fabrication. Natl. Sci. Rev. 2021, 8, nwab048. [Google Scholar] [CrossRef]
- Xie, D.; Su, Y.; Li, X.; Chen, J.; Shi, X.; Liang, D.; Yip, J.; Liu, J.; Li, Z.; Tong, R.K.-Y. Fluid-Driven High-Performance Bionic Artificial Muscle with Adjustable Muscle Architecture. Adv. Intell. Syst. 2023, 5, 2200370. [Google Scholar] [CrossRef]
- Villeda-Hernandez, M.; Baker, B.C.; Romero, C.; Rossiter, J.M.; Faul, C.F.J. Soft alchemy: A comprehensive guide to chemical reactions for pneumatic soft actuation. Soft Sci. 2024, 4, 14. [Google Scholar] [CrossRef]
- Kim, J.; Luo, K.; Suo, Z. A Chemical Pump that Generates High-Pressure Gas by Transmitting Liquid Fuel against Pressure Gradient. Adv. Intell. Syst. 2022, 4, 2100246. [Google Scholar] [CrossRef]
- Wei, Q.; Ke, D.; Sun, Z.; Wu, Z.; Zhou, Y.; Zhang, D. A Structural Design and Motion Characteristics Analysis of an Inchworm-Inspired Soft Robot Based on Shape Memory Alloy Actuation. Actuators 2024, 13, 43. [Google Scholar] [CrossRef]
- Zhuang, P.; Wang, W.; Li, Y.; Han, M. Cyclic behavior of an adaptive seismic isolation system combining a double friction pendulum bearing and shape memory alloy cables. Smart Mater. Struct. 2021, 30, 075003. [Google Scholar]
- Song, S.-H.; Lee, J.-Y.; Rodrigue, H.; Choi, I.-S.; Kang, Y.J.; Ahn, S.-H. 35Hz shape memory alloy actuator with bending-twisting mode. Sci. Rep. 2016, 6, 21118. [Google Scholar] [CrossRef]
- Guo, D.-J.; Liu, R.; Cheng, Y.; Zhang, H.; Zhou, L.-M.; Fang, S.-M.; Elliott, W.H.; Tan, W. Reverse Adhesion of a Gecko-Inspired Synthetic Adhesive Switched by an Ion-Exchange Polymer–Metal Composite Actuator. ACS Appl. Mater. Interfaces 2015, 7, 5480–5487. [Google Scholar] [CrossRef]
- Li, Y.; Jin, R. Novel Ionic Polymer-Metal Composite Employing Sulfonated Polyimide as Ion-Exchange Membrane. Advanced Mater. Res. 2014, 912–914, 251–254. [Google Scholar] [CrossRef]
- Yun, J.S.; Yang, K.S.; Choi, N.-J.; Lee, H.-K.; Moon, S.E.; Kim, D.H. Microvalves Based on Ionic Polymer-Metal Composites for Microfluidic Application. J. Nanosci. Nanotechno. 2011, 11, 5975–5979. [Google Scholar] [CrossRef]
- Medina, H.; Farmer, C.; Liu, I. Dielectric Elastomer-Based Actuators: A Modeling and Control Review for Non-Experts. Actuators 2024, 13, 151. [Google Scholar] [CrossRef]
- Zhao, H.; Hussain, A.M.; Duduta, M.; Vogt, D.M.; Wood, R.J.; Clarke, D.R. Compact Dielectric Elastomer Linear Actuators. Adv. Funct. Mater. 2018, 28, 1804328. [Google Scholar] [CrossRef]
- Moretti, G.; Rosset, S.; Vertechy, R.; Anderson, I.; Fontana, M. A Review of Dielectric Elastomer Generator Systems. Adv. Intell. Syst. 2020, 2, 2000125. [Google Scholar] [CrossRef]
- Huang, C.; Lv, J.; Tian, X.; Wang, Y.; Yu, Y.; Liu, J. Miniaturized Swimming Soft Robot with Complex Movement Actuated and Controlled by Remote Light Signals. Sci. Rep. 2015, 5, 17414. [Google Scholar] [CrossRef]
- Che, S.; Zhang, J.; Mou, F.; Guo, X.; Kauffman, J.E.; Sen, A.; Guan, J. Light-Programmable Assemblies of Isotropic Micromotors. Research 2022, 2022, 9816562. [Google Scholar] [CrossRef]
- Acome, E.; Mitchell, S.K.; Morrissey, T.G.; Emmett, M.B.; Benjamin, C.; King, M.; Radakovitz, M.; Keplinger, C. Hydraulically amplified self-healing electrostatic actuators with muscle-like performance. Science 2018, 359, 61–65. [Google Scholar] [CrossRef]
- Kellaris, N.; Venkata, V.G.; Smith, G.M.; Mitchell, S.K.; Keplinger, C. Peano-HASEL actuators: Muscle-mimetic, electrohydraulic transducers that linearly contract on activation. Sci. Robot. 2018, 3, eaar3276. [Google Scholar] [CrossRef]
- Mitchell, S.K.; Wang, X.; Acome, E.; Martin, T.; Ly, K.; Kellaris, N.; Venkata, V.G.; Keplinger, C. An Easy-to-Implement Toolkit to Create Versatile and High-Performance HASEL Actuators for Untethered Soft Robots. Adv. Sci. 2019, 6, 1900178. [Google Scholar] [CrossRef]
- Kellaris, N.; Rothemund, P.; Zeng, Y.; Mitchell, S.K.; Smith, G.M.; Jayaram, K.; Keplinger, C. Spider-Inspired Electrohydraulic Actuators for Fast, Soft-Actuated Joints. Adv. Sci. 2021, 8, 2100916. [Google Scholar] [CrossRef]
- Shi, X.; Wang, Q.; Wang, C.; Wang, R.; Zheng, L.; Qian, C.; Tang, W. An AI-Based Curling Game System for Winter Olympics. Research 2022, 2022, 9805054. [Google Scholar] [CrossRef]




| Voltage (kV) | Bending Angle (°) When the Film Thickness (mm) Is: | ||||
|---|---|---|---|---|---|
| 0.8 | 0.9 | 1.0 | 1.1 | 1.2 | |
| 0~3 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 4 | 3.5 | 3.0 | 2.5 | 2.0 | 1.0 |
| 5 | 4.9 | 4.0 | 3.5 | 3.0 | 2.3 |
| … | … | … | … | … | … |
| 12 | 16.0 | 14.0 | 12.0 | 11.0 | 9.5 |
| 13 | Breakdown | 15.5 | 14.0 | 13.0 | 11.0 |
| 14 | Breakdown | Breakdown | 15.5 | 14.5 | 12.5 |
| 15 | Breakdown | Breakdown | 16.5 | 15.0 | 13.0 |
| 16 | Breakdown | Breakdown | Breakdown | 16.5 | 13.0 |
| 17 | Breakdown | Breakdown | Breakdown | Breakdown | 13.0 |
| 18 | Breakdown | Breakdown | Breakdown | Breakdown | 13.0 |
| Parameter | Value | Unit |
|---|---|---|
| Shore hardness of silicone rubber film | 5 | A |
| Length of the actuator | 64 | mm |
| Layers of film structure | 3 | unit |
| Number of chambers | 2 | unit |
| Volume of silicone oil | 0.8 | mL |
| Thickness of silicone rubber film | 1.1 | mm |
| Soft electrode dimensional parameters | 36 × 8 | mm × mm |
| Dimensional parameters of silicone oil chamber | 40 × 20 | mm × mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, H.; Chen, Y.; Tang, W. Soft Electrohydraulic Bending Actuators for Untethered Underwater Robots. Actuators 2024, 13, 214. https://doi.org/10.3390/act13060214
Lin H, Chen Y, Tang W. Soft Electrohydraulic Bending Actuators for Untethered Underwater Robots. Actuators. 2024; 13(6):214. https://doi.org/10.3390/act13060214
Chicago/Turabian StyleLin, Hao, Yihui Chen, and Wei Tang. 2024. "Soft Electrohydraulic Bending Actuators for Untethered Underwater Robots" Actuators 13, no. 6: 214. https://doi.org/10.3390/act13060214
APA StyleLin, H., Chen, Y., & Tang, W. (2024). Soft Electrohydraulic Bending Actuators for Untethered Underwater Robots. Actuators, 13(6), 214. https://doi.org/10.3390/act13060214




