The Study of the Salient Pole Geometry Optimization of the Flux Switching Permanent Magnet Machine
Abstract
1. Introduction
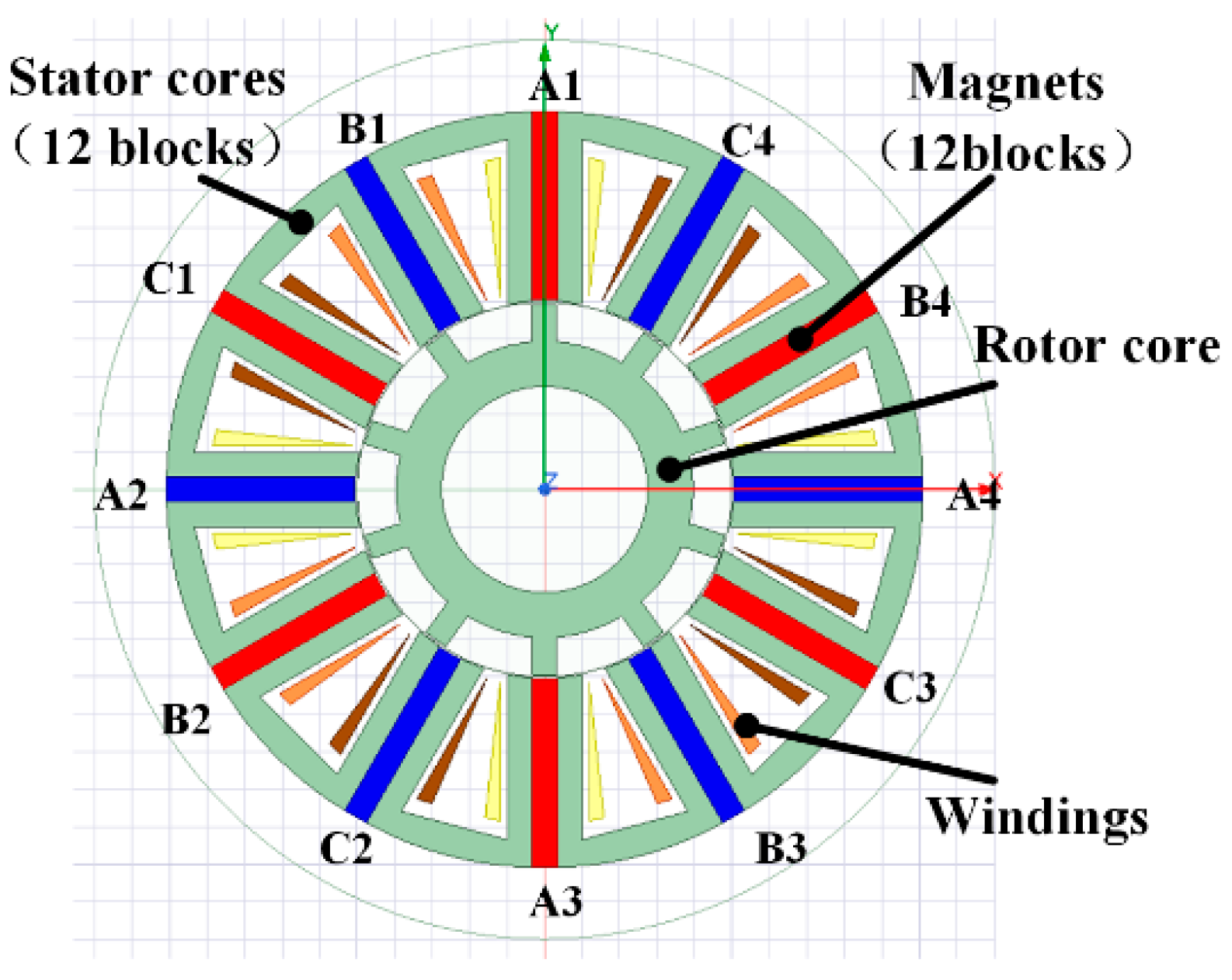
2. The Basis of the FSPM Motor
3. Analysis of Salient Pole Dimensions
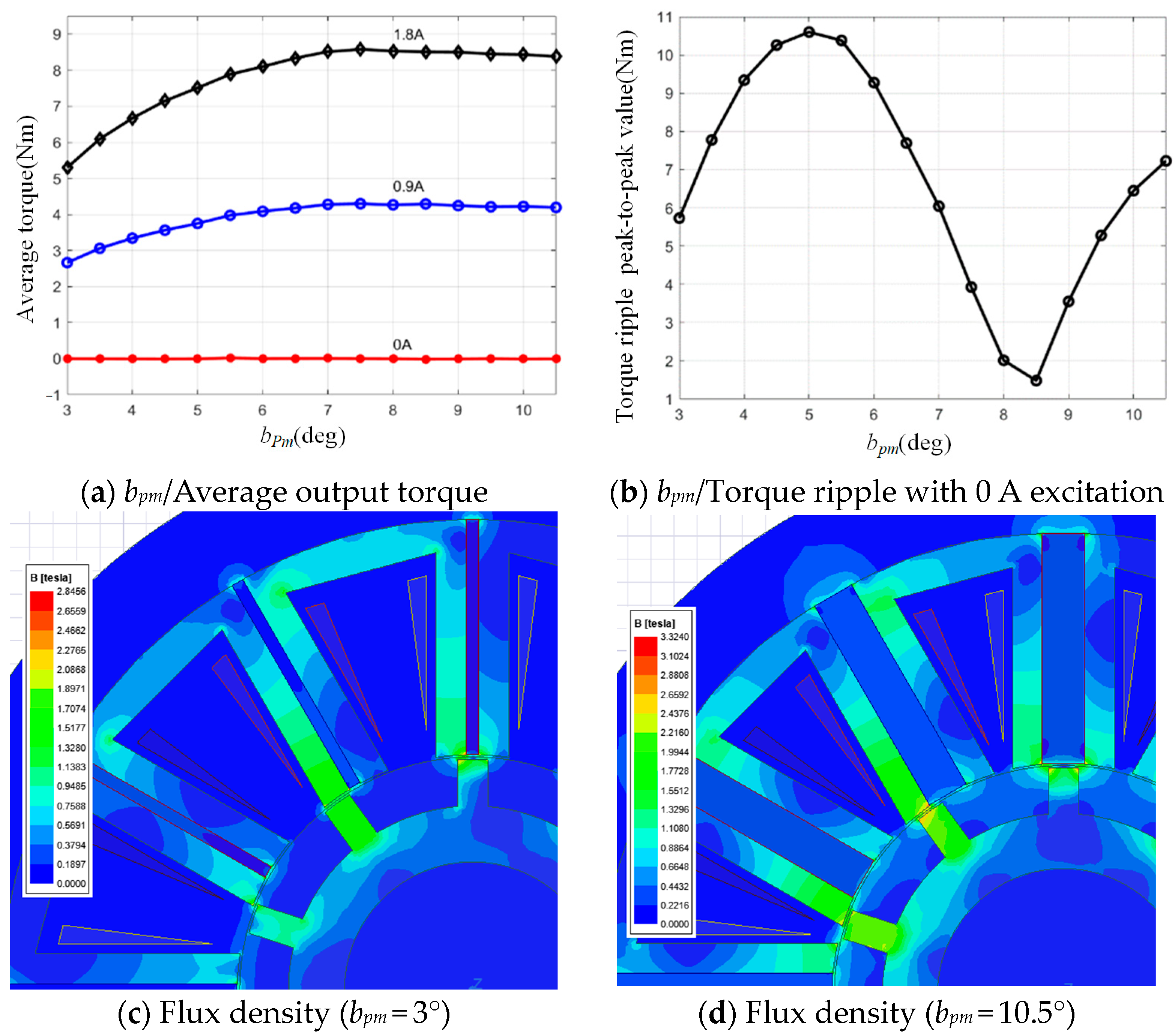
3.1. Effect of bpm on Motor Output Torque
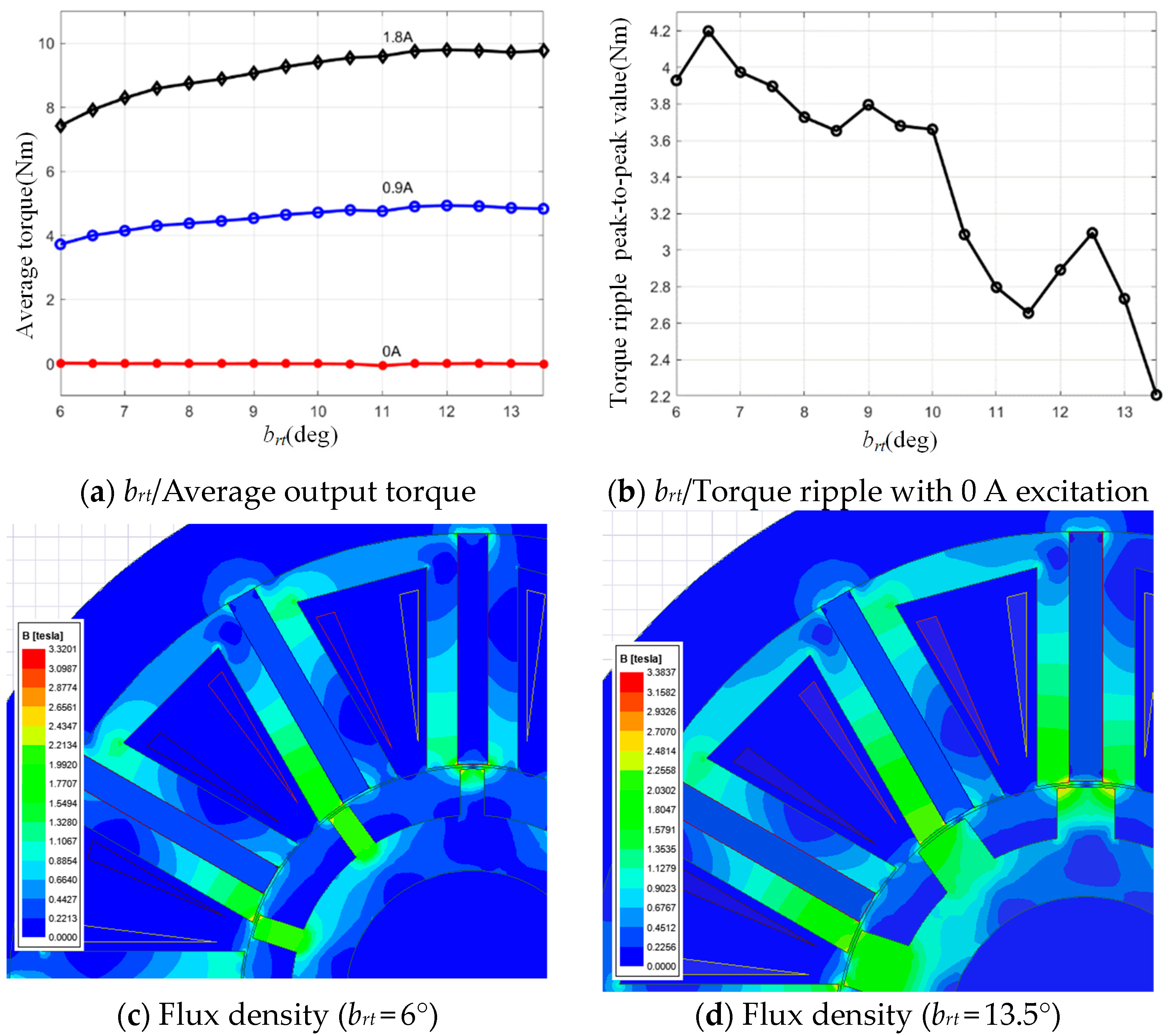
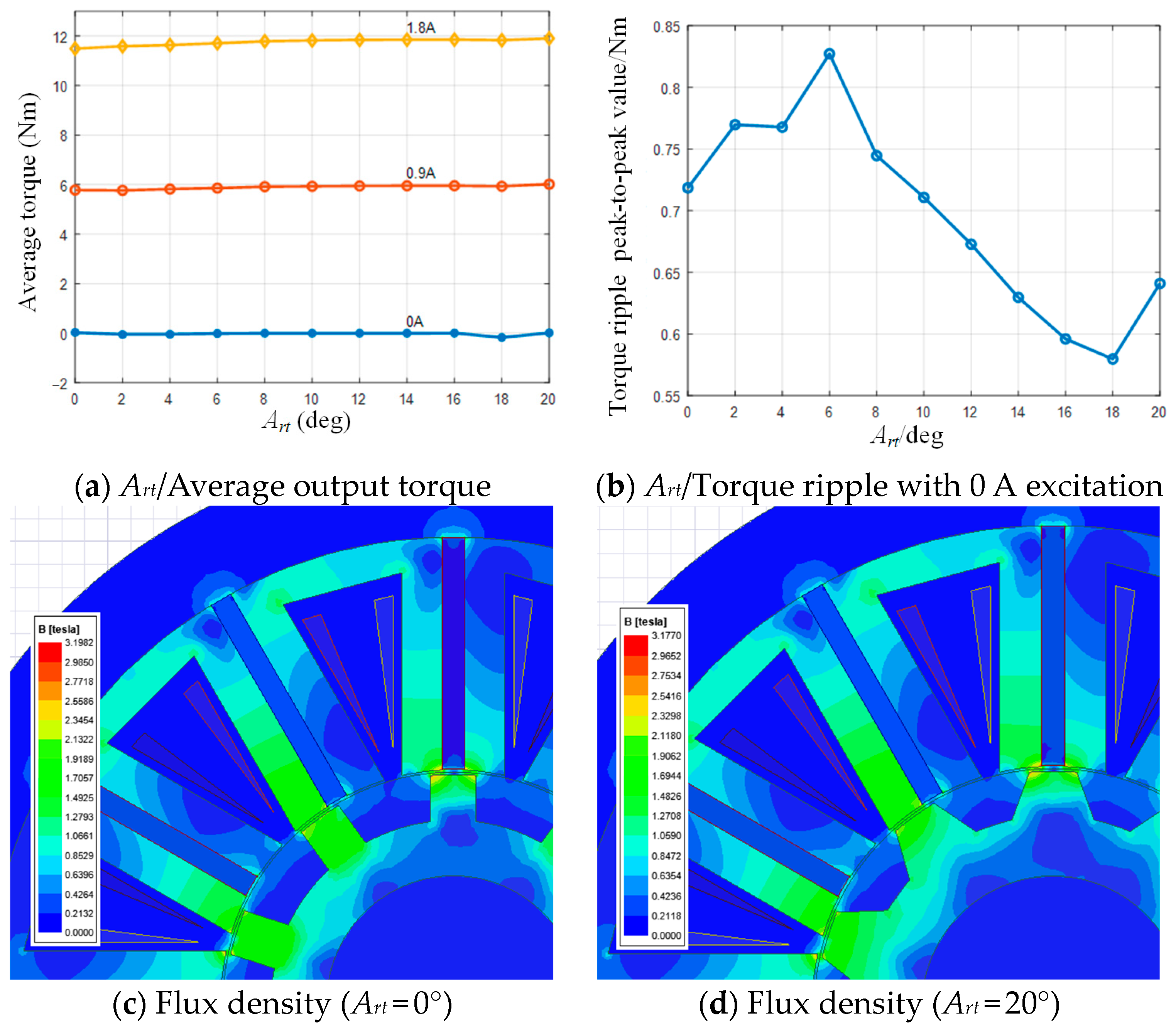
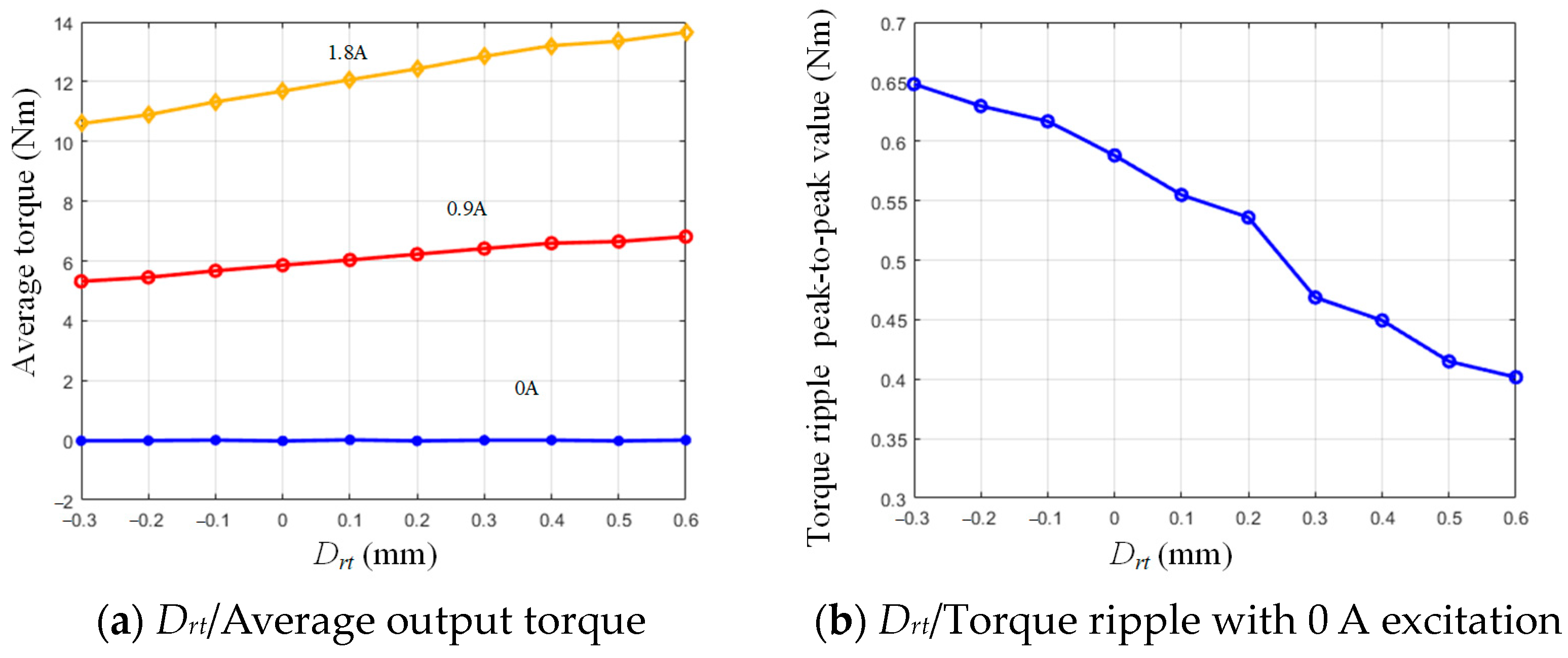
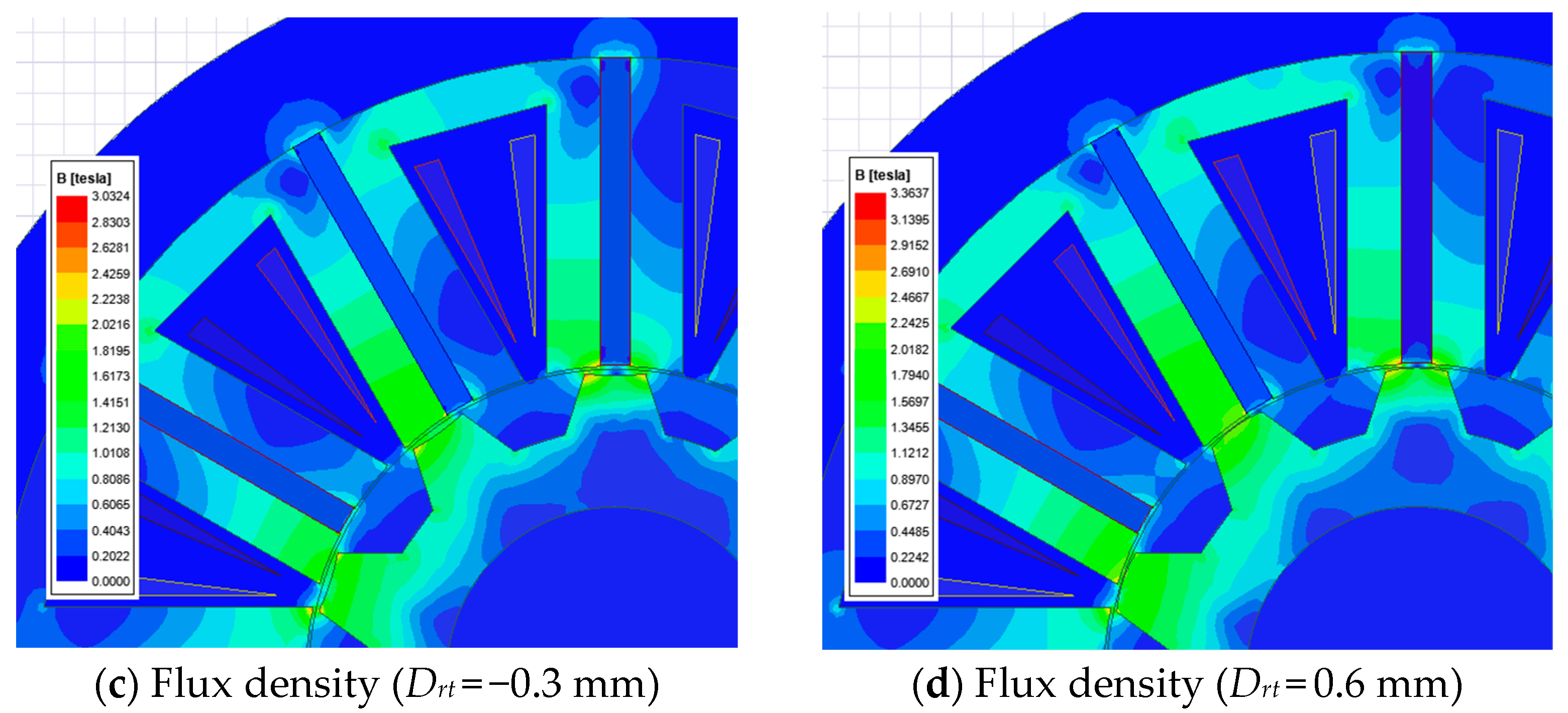
3.2. Effect of brt on Motor Performance
3.3. Effect of bst on Motor Performance
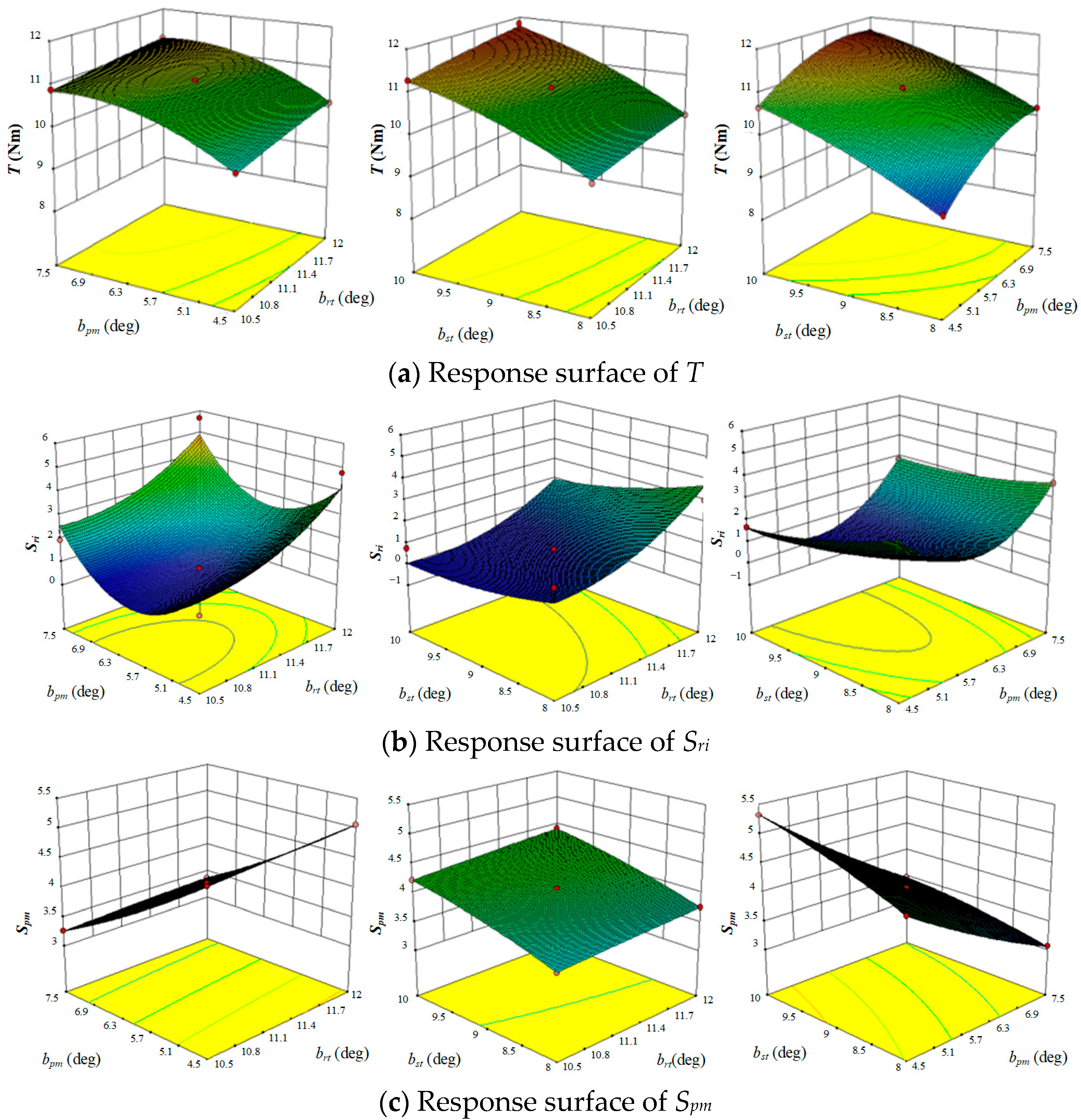
4. Motor Salient Pole Parameter Coupling Optimization
5. The Rotor Salient Pole Shape and the Torque Characteristic
6. Experiment
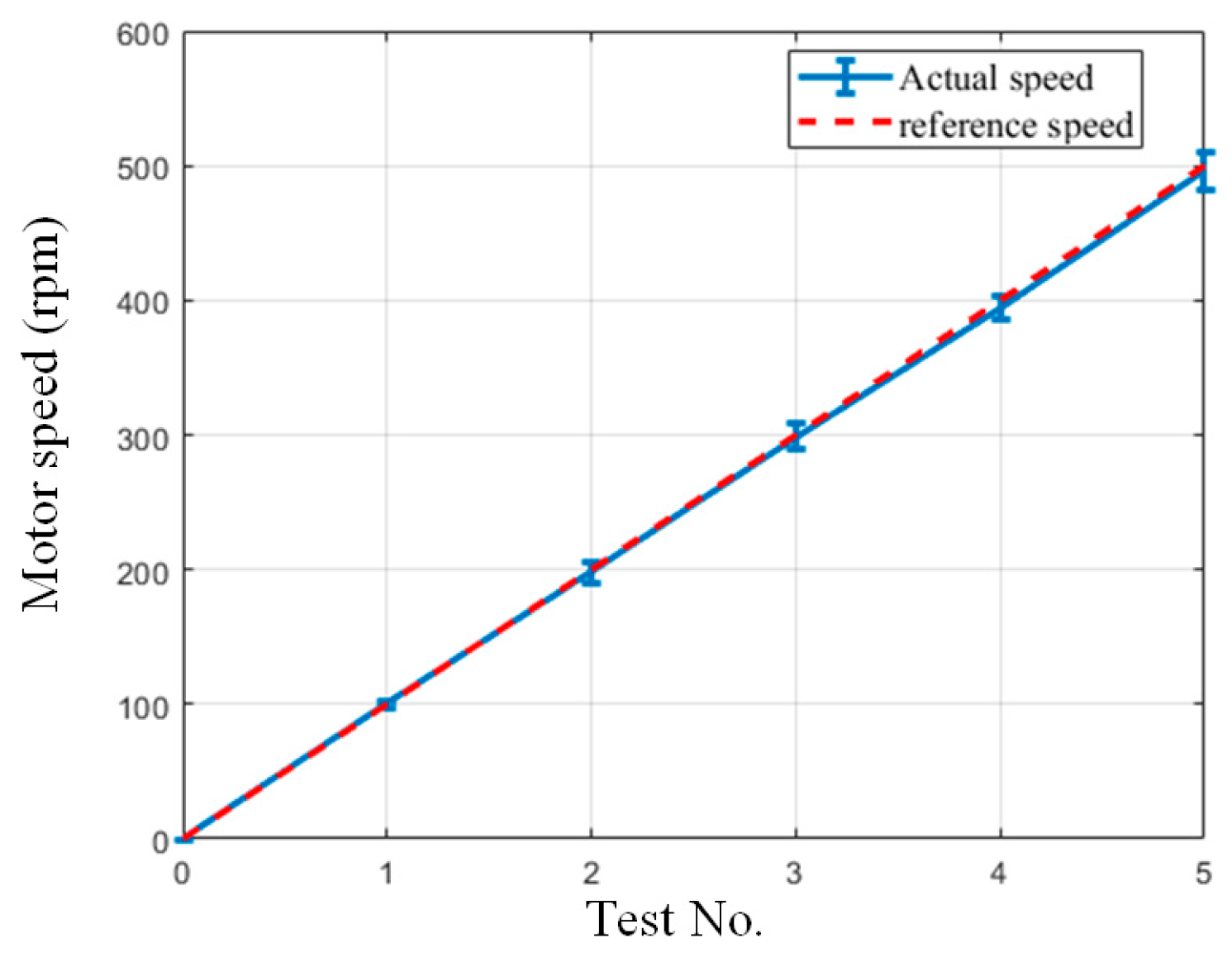
6.1. The No-Load EMF, Speed, and Torque Test
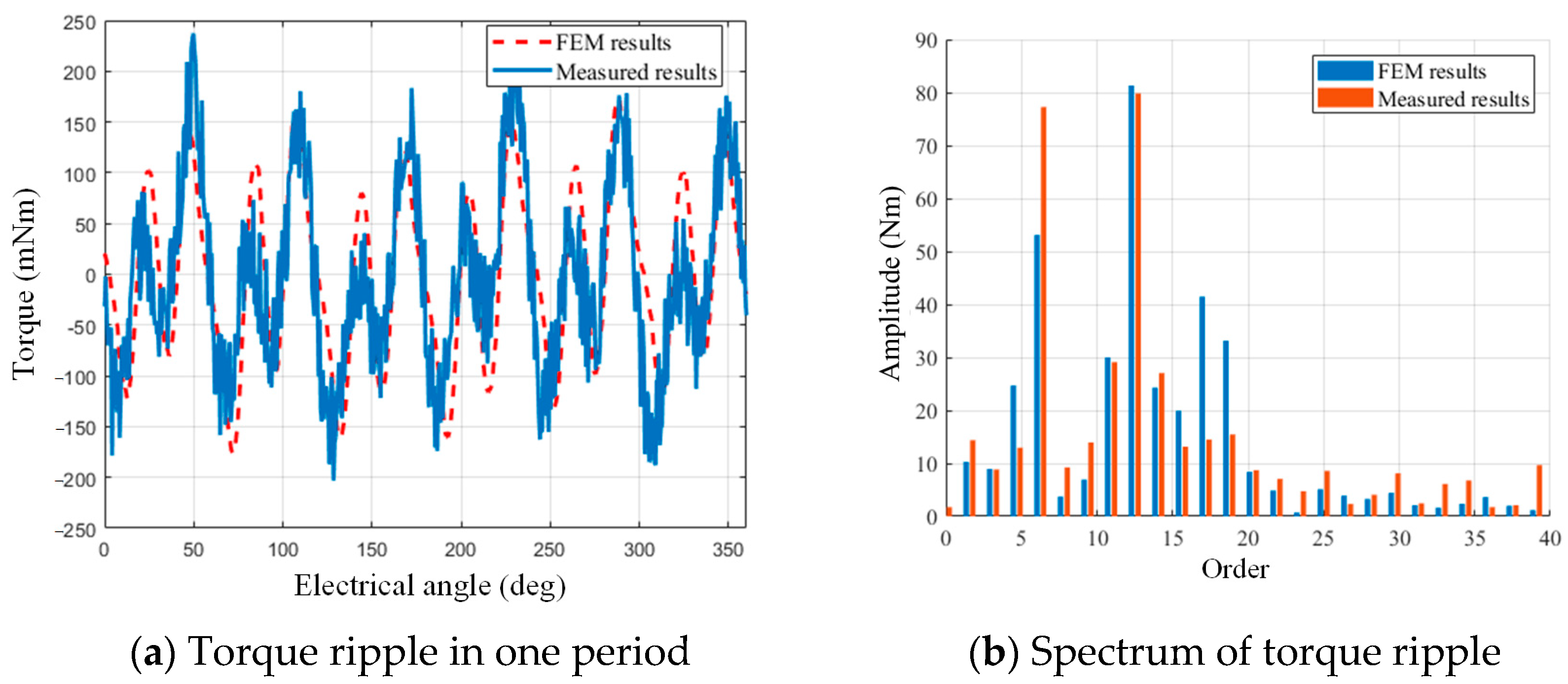
6.2. Torque Ripple Test
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Denkena, B.; Ahlborn, P. Linear-rotary direct drive for multi-functional machine tools. Cirp. Ann. Manuf. Technol. 2022, 71, 349–352. [Google Scholar] [CrossRef]
- Yang, X.; Li, J.; Xuan, J.; Zhao, W. Influence of the Machining Process on the Thrust Force and Mechanical Characteristics for the Direct Drive System. Processes 2023, 11, 17. [Google Scholar] [CrossRef]
- Park, J.; An, J.; Han, K.; Choi, H.-S.; Park, I.S. Enhancement of cooling performance in traction motor of electric vehicle using direct slot cooling method. Appl. Therm. Eng. 2022, 217, 15. [Google Scholar] [CrossRef]
- Wang, P.; Hua, W.; Wang, G.; Zhang, Z.; Hu, M. Comparative Study of Flux-Switching Permanent Magnet Machine with C- and E-Shaped Cores Based on Magnetic Field Modulation Theory. IEEJ Trans. Electr. Electron. Eng. 2023, 18, 270–277. [Google Scholar] [CrossRef]
- Zhu, J.; Wu, L.; Wen, H. Optimization and Comparison of Dual-Armature Flux-Switching Permanent Magnet Machines with Different Stator Core Shapes. IEEE Trans. Ind. Appl. 2022, 58, 314–324. [Google Scholar] [CrossRef]
- Zhu, Z.Q.; Chen, J.T. Advanced Flux-Switching Permanent Magnet Brushless Machines. IEEE Trans. Magn. 2010, 46, 1447–1453. [Google Scholar] [CrossRef]
- Zhou, J.; Xiao, X. Combined methods for torque variation reduction of high-speed FSPM. Energy Rep. 2023, 9, 199–206. [Google Scholar] [CrossRef]
- Jiang, M.; Zhu, X.; Xiang, Z.; Quan, L.; Que, H.; Yu, B. Suppression of Torque Ripple of a Flux-Switching Permanent Magnet Motor in Perspective of Flux-Modulation Principle. IEEE Trans. Transp. Electrif. 2022, 8, 1116–1127. [Google Scholar] [CrossRef]
- Soomro, I.A.; Sulaiman, E.B.; Ahmad, M.Z.B.; Azis, R. Performance Analysis and Comparison for Various Excitation Source Salient Rotor with Modular Rotor Permanent Magnet of Flux Switching Machine. J. Electr. Eng. Technol. 2023, 18, 2771–2782. [Google Scholar] [CrossRef]
- Yan, J.; Lin, H.; Feng, Y.; Zhu, Z.Q.; Jin, P.; Guo, Y. Cogging Torque Optimization of Flux-Switching Transverse Flux Permanent Magnet Machine. IEEE Trans. Magn. 2013, 49, 2169–2172. [Google Scholar] [CrossRef]
- Farahani, E.F.; Baker, N.J.; Mahmouditabar, F. An Innovative H-Type Flux Switching Permanent Magnet Linear Generator for Thrust Force Enhancement. Energies 2023, 16, 5976. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, H.; Chen, H. A new design approach for reduction of force ripple in permanent magnet flux-switching linear motor. IET Electr. Power Appl. 2023, 17, 1148–1158. [Google Scholar] [CrossRef]
- Ullah, W.; Khan, F.; Sulaiman, E.; Umair, M.; Ullah, N.; Khan, B. Analytical validation of novel consequent pole E-core stator permanent magnet flux switching machine. IET Electr. Power Appl. 2020, 14, 789–796. [Google Scholar] [CrossRef]
- Chen, Z.X.; Cui, Y.J. Numerical Simulation and Experimental Validation of a Flux Switching Permanent Magnet Memory Machine. IEEE Access 2020, 8, 194904–194911. [Google Scholar] [CrossRef]
- Torn, V.; Seangwong, P.; Fernando, N.; Siritaratiwat, A.; Khunkitti, P. Performance Improvement of Flux Switching Permanent Magnet Wind Generator Using Magnetic Flux Barrier Design. Sustainability 2023, 15, 8867. [Google Scholar] [CrossRef]
- Petkar, S.G.; Thippiripati, V.K. A Novel Duty-Controlled DTC of a Surface PMSM Drive with Reduced Torque and Flux Ripples. IEEE Trans. Ind. Electron. 2023, 70, 3373–3383. [Google Scholar] [CrossRef]
- Hua, W.; Cheng, M.; Zhu, Z.Q.; Howe, D. Analysis and optimization of back-EMF waveform of a novel flux-switching permanent magnet motor. In Proceedings of the 2007 IEEE International Electric Machines & Drives Conference, Antalya, Turkey, 3–5 May 2007; pp. 1025–1030. [Google Scholar]
- Zhu, D.; Shen, C.; Du, Y.; Xiao, F. Pole-Shape Optimization of Flux-Switching Permanent Magnet Machine; Institute of Electrical and Electronics Engineers Inc.: Hangzhou, China, 2014; pp. 1681–1684. [Google Scholar]
- Zhu, X.; Hua, W. Back-EMF Waveform Optimization of Flux-Switching Permanent Magnet Machines; Institute of Electrical and Electronics Engineers Inc.: Lausanne, Switzerland, 2016; pp. 2419–2425. [Google Scholar]
- Ma, Z.; Cheng, M.; Zhao, C. Analytical Investigation and Topology Evolution of Rotor Salient Pole Reluctance in the Flux-Switching Permanent Magnet Machine; Institute of Electrical and Electronics Engineers Inc.: Zhuhai, China, 2023; pp. 130–135. [Google Scholar]
- Ullah, W.; Khan, F.; Umair, M. Multi-Objective Optimization of High Torque Density Segmented PM Consequent Pole Flux Switching Machine with Flux Bridge. CES Trans. Electr. Mach. Syst. 2021, 5, 30–40. [Google Scholar] [CrossRef]


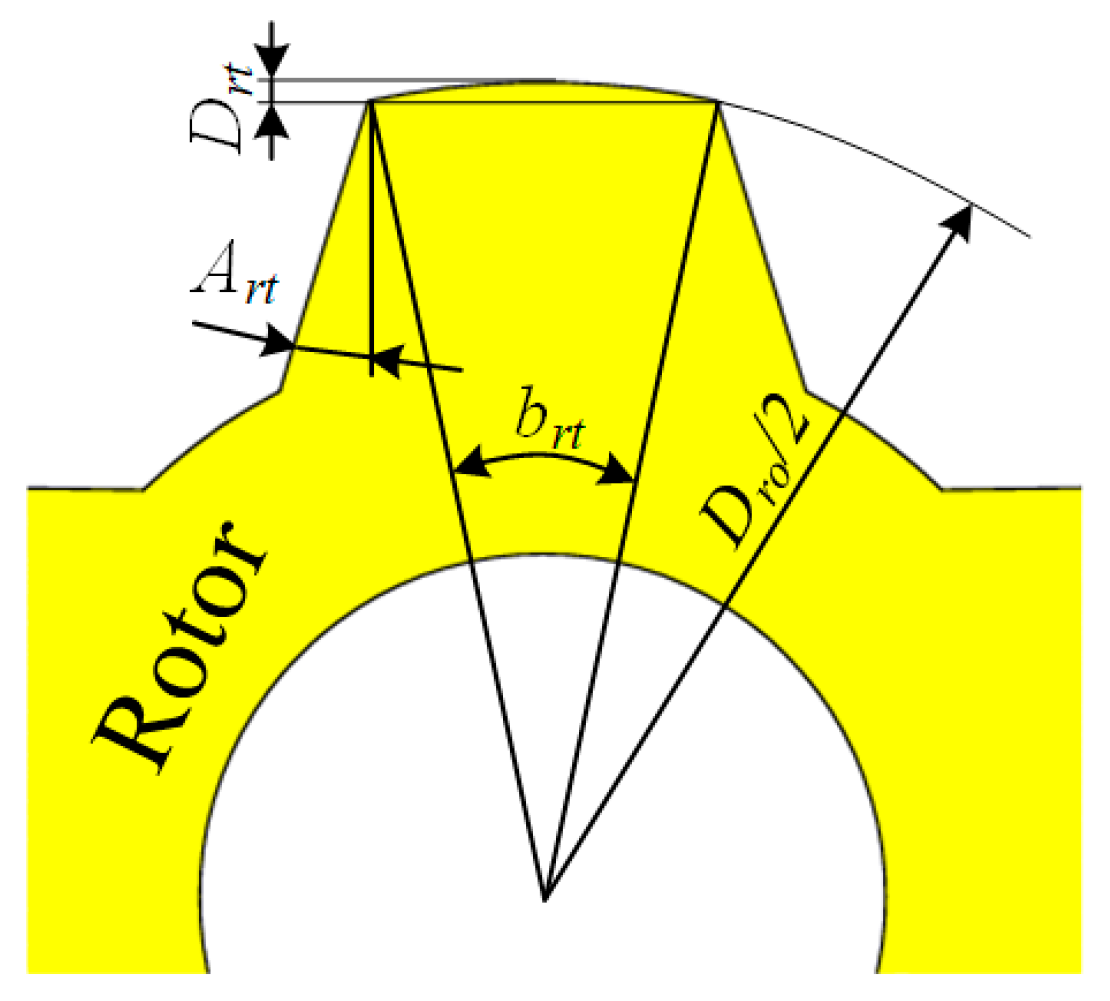
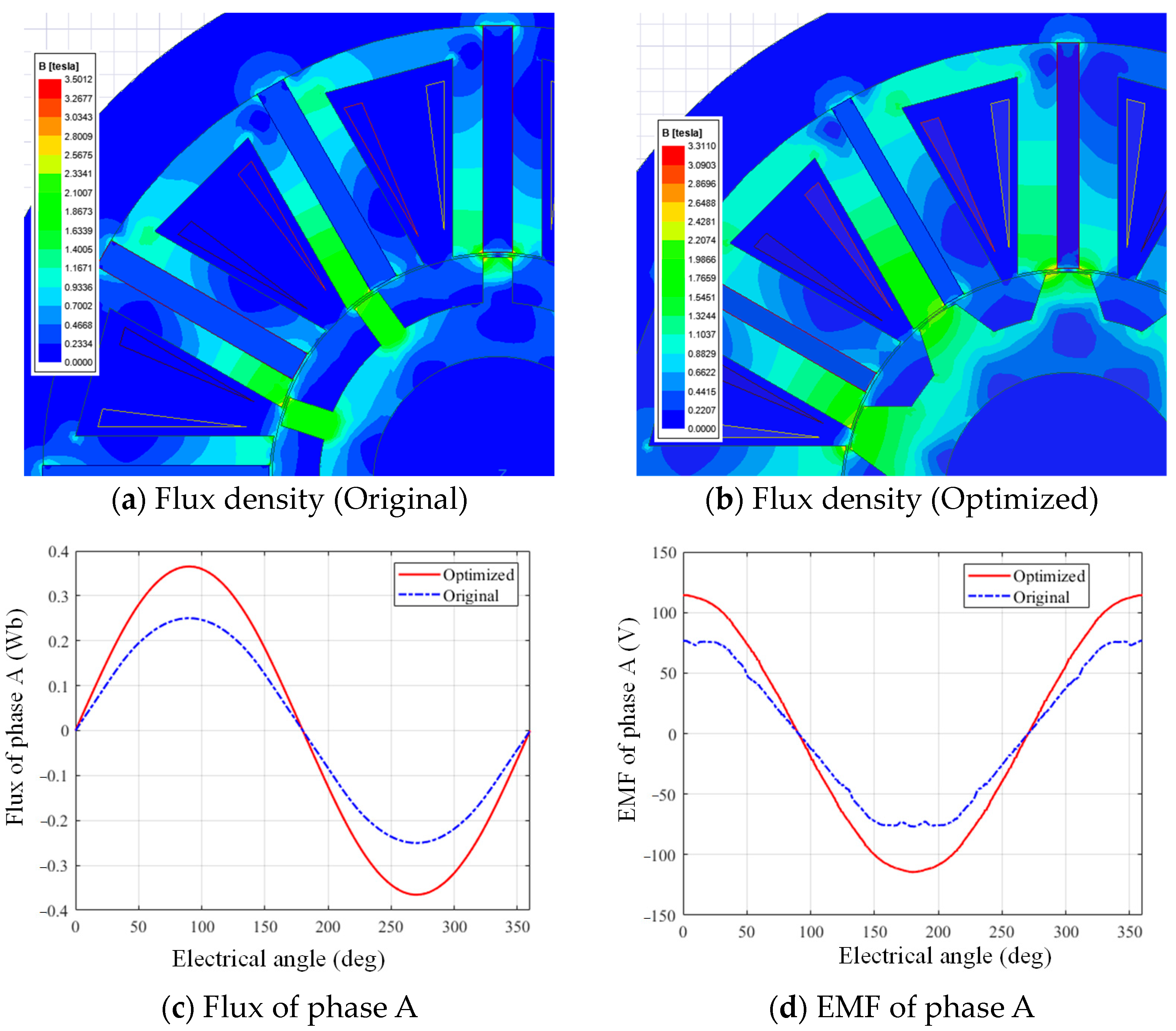

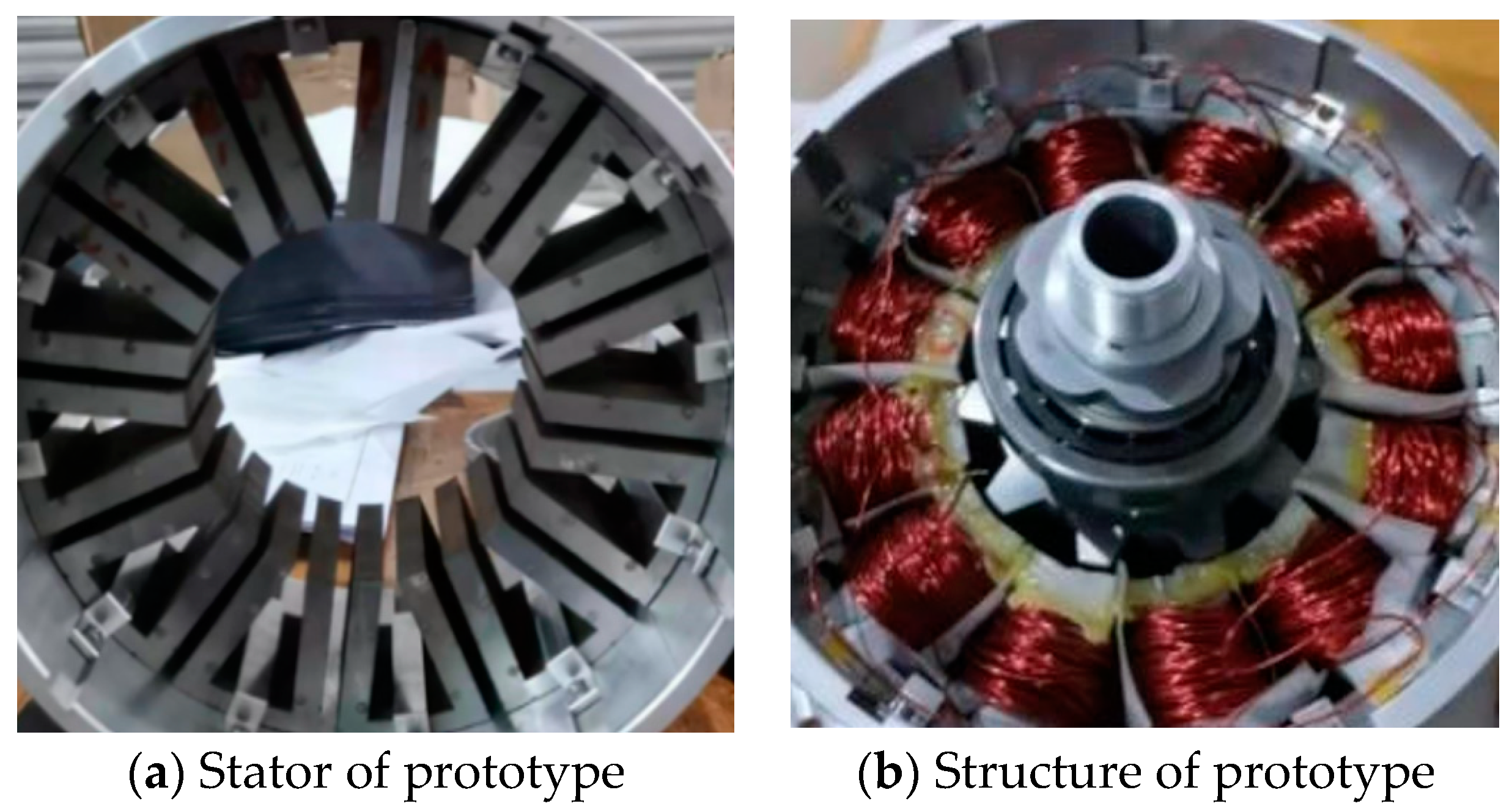
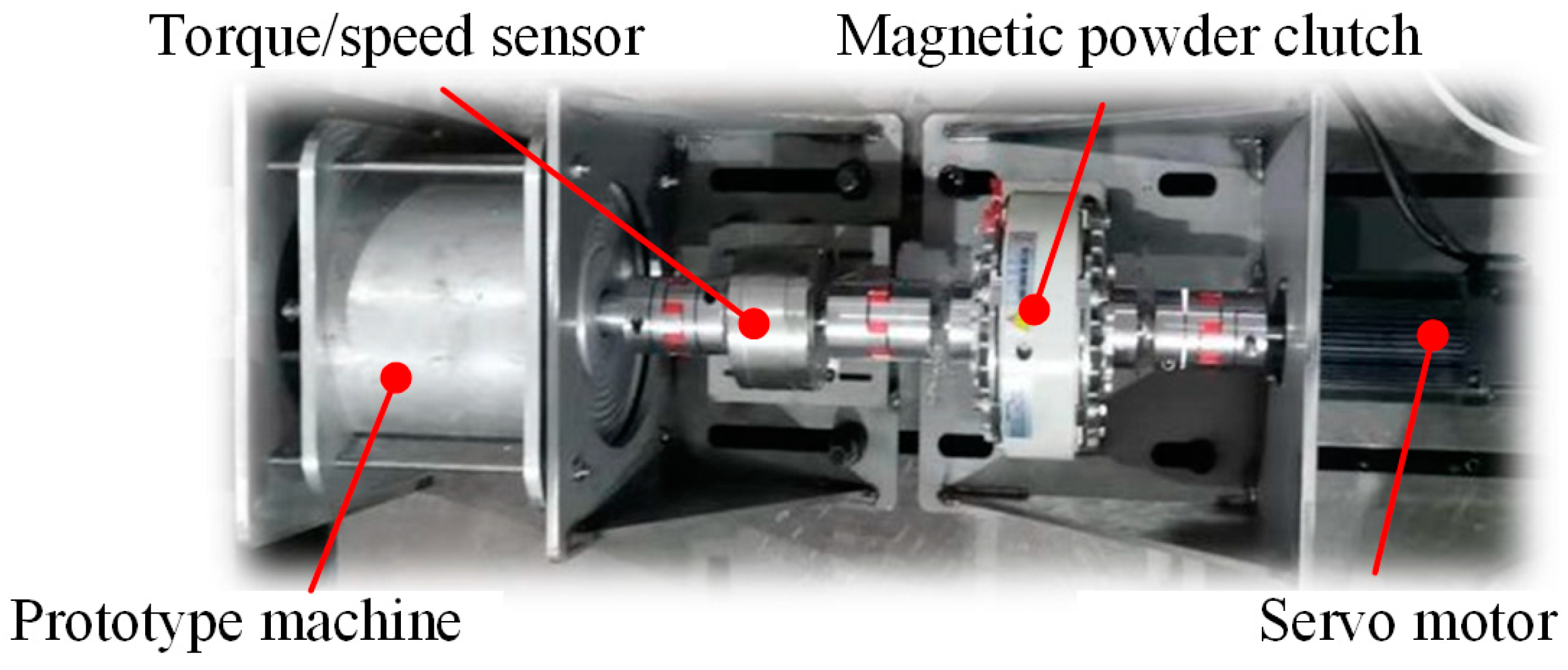
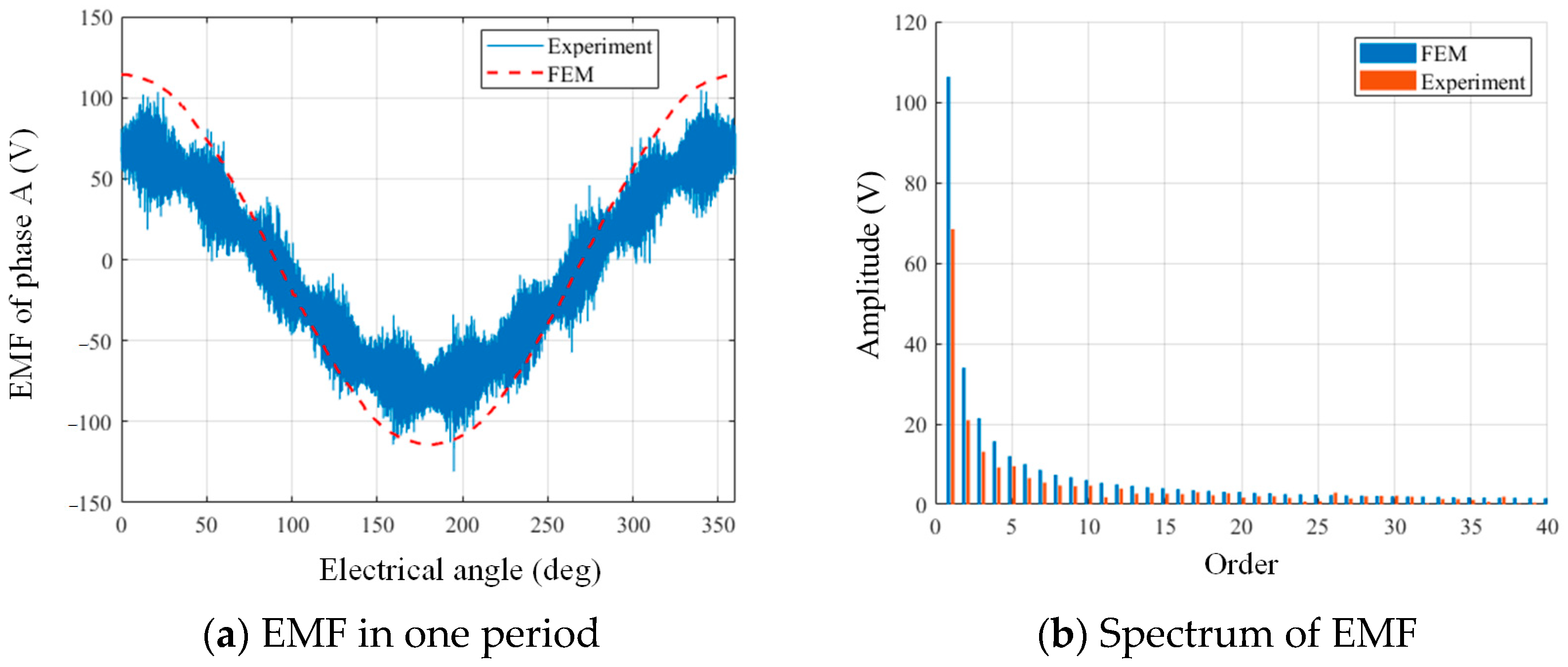

| Parameters | Symbols | Values |
|---|---|---|
| Stator outer diameter | Dso | 202 mm |
| Rotor outer diameter | Dro | 99 mm |
| Rotor inside diameter | Dri | 55 mm |
| Air gap length | δ | 1 mm |
| Stator salient pole angle | bst | 7.5° |
| Permanent magnet angle | bpm | 7.5° |
| Rotor salient pole angle | brt | 7.5° |
| Stator salient pole length | Hst | 44 mm |
| Rotor salient pole length | Hrt | 10 mm |
| Symbols | Parameters | Initial Value | Factor Level | ||
|---|---|---|---|---|---|
| −1 | 0 | 1 | |||
| brt | Rotor salient pole angle /° | 7.5 | 10.5 | 11.5 | 12 |
| bpm | Permanent magnet angle/° | 7.5 | 4.5 | 6 | 7.5 |
| bst | Stator salient pole angle /° | 7.5 | 8 | 9 | 10 |
| No. | Variable Factors | Response Results | ||||
|---|---|---|---|---|---|---|
| brt/° | bpm/° | bst/° | T/Nm | Sri | Spm | |
| 1 | 10.5 | 4.5 | 9 | 9.7346 | 1.1392 | 4.8601 |
| 2 | 12 | 6 | 8 | 10.0365 | 3.0147 | 3.7581 |
| 3 | 11.25 | 6 | 9 | 10.8813 | 0.6963 | 4.0744 |
| 4 | 10.5 | 6 | 10 | 11.2849 | 0.7638 | 4.2256 |
| 5 | 11.25 | 7.5 | 10 | 11.6070 | 2.8518 | 3.4769 |
| 6 | 10.5 | 7.5 | 9 | 11.2344 | 5.6508 | 3.3653 |
| 7 | 11.25 | 4.5 | 10 | 10.6550 | 1.6975 | 5.3196 |
| 8 | 11.25 | 7.5 | 9 | 10.8856 | 1.9387 | 3.2609 |
| 9 | 11.25 | 4.5 | 8 | 8.9879 | 3.6046 | 4.4873 |
| 10 | 11.25 | 7.5 | 8 | 10.2181 | 3.6887 | 3.0609 |
| 11 | 10.5 | 6 | 8 | 9.6700 | 1.6131 | 3.6209 |
| 12 | 12 | 4.5 | 9 | 10.1405 | 4.7839 | 5.0628 |
| 13 | 12 | 6 | 10 | 11.8310 | 1.3327 | 4.4300 |
| Optimal Factors | brt | bpm | bst | Optimal Results | ||
|---|---|---|---|---|---|---|
| T (Nm) | Sri | Spm | ||||
| 11.5° | 5.5° | 10.0° | 11.450 | 0.696 | 4.719 | |
| T (Nm) | Sri | Spm | |
|---|---|---|---|
| response surface model prediction | 11.450 | 0.696 | 4.719 |
| FEM simulation | 11.473 | 0.638 | 4.700 |
| Relative error | 0.2% | 9.1% | 0.4% |
| AVG Torque (Nm) | Torque Ripple (Nm) | Angle of PM (deg) | |
|---|---|---|---|
| Initial | 8.032 | 4.080 | 7.5 |
| Optimized | 13.354 | 0.349 | 5.5 |
| Difference | +66.2% | −91.4% | −26.7% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, C.; Xu, D.; Wu, W.; Yang, B. The Study of the Salient Pole Geometry Optimization of the Flux Switching Permanent Magnet Machine. Actuators 2024, 13, 398. https://doi.org/10.3390/act13100398
Liu C, Xu D, Wu W, Yang B. The Study of the Salient Pole Geometry Optimization of the Flux Switching Permanent Magnet Machine. Actuators. 2024; 13(10):398. https://doi.org/10.3390/act13100398
Chicago/Turabian StyleLiu, Chen, Dewei Xu, Wenwu Wu, and Bo Yang. 2024. "The Study of the Salient Pole Geometry Optimization of the Flux Switching Permanent Magnet Machine" Actuators 13, no. 10: 398. https://doi.org/10.3390/act13100398
APA StyleLiu, C., Xu, D., Wu, W., & Yang, B. (2024). The Study of the Salient Pole Geometry Optimization of the Flux Switching Permanent Magnet Machine. Actuators, 13(10), 398. https://doi.org/10.3390/act13100398




