Abstract
The recent commercial availability of stacked dielectric elastomer actuators (SDEAs) has unlocked new opportunities for their application as “artificial skeletal muscles” in rehabilitation robots and powered exoskeletons. Composed of multiple layers of thin, elastic capacitors, these actuators present a lightweight, soft, and acoustically noiseless alternative to traditional DC motor actuators commonly used in rehabilitation robotics, thereby enhancing the natural feel of such systems. Building on our previous research, this study aimed to evaluate the most recent version of commercial SDEAs to assess their potential for mechanizing rehabilitation robots. We quantified the stress and strain behavior and stiffness of these actuators in both single and 1 × 3 configurations (with three SDEAs connected in series). The actuators demonstrated the capability to generate up to 25 N of force and 115 KPa, a value surpassing human biceps, with a longitudinal strain measured at about 11%. The significant increase in force generation from 10 N in the previous version to 25 N and displacement from 3.3% to 11% substantially enhances the applicability of this actuator in rehabilitation robotics. SDEAs’ high force generation capability, combined with their strain and stress characteristics comparable to that of human biological muscles, make them ideal alternative actuators for biomimetic robots and applications where actuators must operate in the vicinity of the human body.
1. Introduction
Enhancing the effectiveness and acceptance of rehabilitation robots necessitates the development of actuator technology that feels more natural. Incorporating softness, defined as possessing Young’s modulus similar to that of biological tissues, can enhance the device’s safety, flexibility, and tactile sensation []. When softness is combined with acoustically noiseless operation and longitudinal contraction, it creates a muscle-like actuator ideal for rehabilitation applications, particularly in exoskeletons where the actuator operates near human limbs. The longitudinal actuation of such actuators allows them to align with the skeletal system, mimicking skeletal muscle, thus minimizing the bulkiness of the exoskeletal structure. Additionally, this linear movement, when paired with softness, adapts to complex shapes and allows for complex degrees of freedom with a single actuator []. For exoskeletons, it is advantageous for the actuator to generate forces and movements on par with those of mammalian skeletal muscle. Such an actuator, characterized by its softness, quiet operation, linear motion, and ability to match the force and displacement of skeletal muscle, is termed an artificial skeletal muscle.
Potential candidates for artificial skeletal muscles are generally categorized into two types: thermally driven and electroactive polymers. Thermally driven polymer actuators tend to be more powerful, particularly in terms of their strain capacity, compared to electroactive actuators. For instance, a 2 g ethanol-based phase-change actuator developed by Miriyev’s team was capable of exerting up to 120 N of force and achieving 140% strain []. In comparison to electroactive polymers’ high strain rate, thermally driven actuators exhibit slower actuation speeds (about 2.5%/s strain rate) and significantly lower efficiencies (0.2%). These characteristics present considerable drawbacks for exoskeleton applications, which require quick and repetitive longitudinal movements (contractions).
Soft electroactive polymer actuators like dielectric elastomer actuators (DEAs) provide a fast, efficient, and quiet alternative to the conventional DC motor actuators found in standard rehabilitation robotic systems. DEAs exhibit remarkable muscle-like properties, positioning them as highly promising and frequently studied soft actuators. Notably, mammalian skeletal muscle functions similarly to an electroactive polymer. Dielectric elastomer actuators are essentially composed of a compliant capacitor featuring an elastic dielectric material encased between two mechanically compliant electrodes (Figure 1A). When a DC voltage is applied across these electrodes, the resulting Maxwell pressure, electrostatic force-induced pressure between two oppositely charged plates, compresses the dielectric in the thickness direction. Equation (1) demonstrates the parabolic relationship between Maxwell pressure and voltage, where ε and represent the permittivity of the polymer and vacuum, respectively, V is the voltage applied across the electrodes, and d is the thickness.
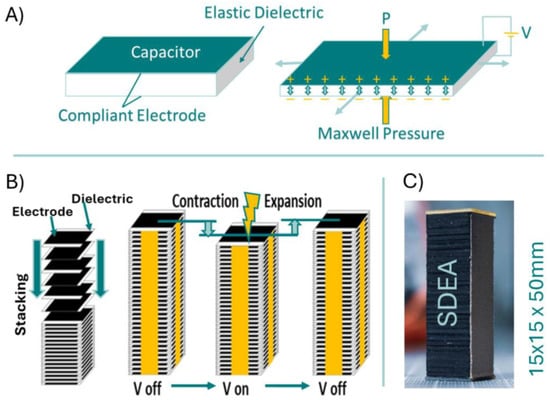
Figure 1.
(A) An elastic capacitor generates displacement through Maxwell pressure. Once the voltage is applied, the Maxwell pressure contracts the thickness of the elastic dielectric while its area expands, as demonstrated by the four arrows. (B) The stacked dielectric elastomer actuator (SDEA) configuration comprises multiple layers of elastic capacitors. (C) A typical SDEA may contain approximately 2500 capacitor layers.
Dielectric elastomer actuators (DEAs) possess several distinct characteristics, including high power-to-mass ratios that can exceed those of human skeletal muscles, rapid responsiveness, length self-sensing, and the ability to recuperate energy [,]. These actuators are notably efficient. When the capacitor is fully charged—meaning the DEA is completely contracted—the primary source of energy consumption is minimal dielectric leakage currents, typically in the microampere range []. However, the requirement for high driving voltages (over 1 KV) can be problematic, as it not only restricts the selection of compatible electrical components but also raises significant safety concerns. Despite these high voltages, the low power requirements of DEAs (usually a few watts, due to their low operational currents) generally keep them within safe operational limits, particularly if adequate insulation measures are in place. Moreover, under a constant DC voltage, the rate at which a capacitor charges, and thus the contraction speed of the DEA, is governed by the applied electrical current, which can be adjusted to safe levels depending on the required actuation speed. Additionally, the discharge rate of the DEA is a critical factor in safety assessments. Zhang et al. conducted simulations of their electrical system, demonstrating the safety of their DEA-based glove-like force feedback device in compliance with IEC TS 60479-2 standards [,].
DEAs have shown significant potential as artificial skeletal muscles in orthotic and prosthetic applications. Carpi et al. pioneered the use of multilayer DEAs to mechanize a hand orthosis, demonstrating their practical utility in this domain []. Another promising application of DEAs in rehabilitation is in the design of ankle-foot orthoses (AFOs) aimed at assisting individuals with ankle control deficits during walking. For instance, we have developed a DEA-based ankle-foot orthosis (DE-AFO) specifically tailored for children with cerebral palsy []. Additionally, Allen et al. have designed an innovative DE-powered AFO, achieving an impressive 49% dorsiflexion during use [].
DEAs are primarily available in two configurations: (1) freestanding DEAs, and (2) DEAs integrated with rigid frames. Rigid frames can be less suitable where flexibility and softness are required, often resulting in heavier and more cumbersome designs compared to their freestanding counterparts.
In applications for artificial skeletal muscles, freestanding configurations are particularly favored for their ability to impart a more natural feel to the design. Various freestanding configurations have been developed, including tubular [], helical [], folded [], stacked [], and rolled DEAs [] (Table 1). DEAs have been constructed into cylindrical rolls, achieving strain, shape, and performance characteristics akin to natural skeletal muscles. Kunze, Julian, et al. developed compact, high-energy-density rolled DEAs for soft robotics and artificial muscles. Made from thin silicone films with flexible electrodes, these actuators show a blocking force change of 0.18 N and a 2.5% stroke at 2 N. The design is smaller than conventional DEAs, enabling compact soft robotic systems. Future work will optimize the design, eliminate the hollow core, and develop models to enhance performance. One rolled actuator was designed to resemble the shape of a biceps muscle and was implemented to function within a life-size skeletal arm model [].

Table 1.
Properties of freestanding DEA configurations: strain values are no-load; forces show isometric force; dimensions in parentheses indicate active length.
Among the free-standing configurations, the stacked DEAs developed by Kovacs et al. [] stand out as highly powerful and versatile, bearing the closest resemblance to human skeletal muscle among the freestanding DEA configurations. Kovacs et al. innovatively layered multiple thin, enhanced DEAs to craft this advanced actuator. In this setup, each layer functions analogously to a sarcomere within a skeletal muscle’s myofibril. This assembly of tensile actuators was capable of generating 32 N of isometric force and 15% strain while managing a 1.1 kg tensile load []. These features have enabled these stacked DEAs to be the first and only commercially available model produced by a company named CTsystems []; we call their DEAs CT-SDEAs in this study.
Previously, we measured the mechanical properties of the CT-SDEA (model CT25.0-15-15-71, Compliant Transducer Systems, Dubendorf, Switzerland) and compared them to the reported values provided by CTsystems, as shown in Figure 2. In this study, we benchmarked the latest version of this design, now manufactured by Dätwyler Schweiz AG (Schattdorf, Switzerland), to be incorporated as motor units in powered exoskeletons, including our latest ankle exoskeleton, DE-AFO—a comfortable ankle-foot orthosis (AFO) powered by SDEA-based artificial muscles []. The dimensions of the evaluated actuator in this study are 15 × 15 × 50 mm, slightly different from the CT-SDEA’s 30 × 17 × 17 mm. We refer to these actuators as SDEA-1550.
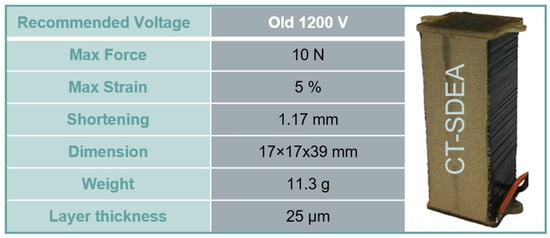
Figure 2.
The reported mechanical property of CTsystems’ CT25.0-1515-71 (CT-SDEA).
We measured the axial stiffness of SDEA-1550 and evaluated its stress and strain capabilities under three conditions: free-standing, isometric (constant length), and isotonic (constant tensile load). Additionally, we configured an artificial muscle by connecting three of these actuators in series (1 × 3 configuration) and tested it under the same conditions. To further assess the reliability of these actuators in producing force and displacement, we conducted a comparative analysis of 10 actuators.
2. Methods
2.1. Testing Procedure
To evaluate the performance of the SDEAs, tests were conducted under three conditions: free-standing, isometric, and isotonic contraction conditions. The actuators were activated using different driving voltages, from 0 to 1200 V, in 100 V increments.
2.2. Strain-Stress Testing Setup
A comprehensive test rig was designed and implemented to evaluate the performance of the SDEAs under all three aforementioned conditions: isometric, isotonic, and free-standing contractions. The main components of this test rig include the aluminum mainframe, National Instrument’s (NI’s) data acquisition (DAQ) boards, laser displacement sensor, load cell, and precision translation stages.
A laser displacement sensor, model ILD 142025 (Micro-Epsilon, Ortenburg, Germany), was used to measure the longitudinal contraction of the actuators (Figure 3). This sensor offers a precision of 1 μm, guaranteeing highly accurate displacement measurements. To measure both active and passive forces exerted by the SDEA-1550s, a load cell model LSB302 (FUTEK Advanced Sensor Technology, Inc. in Irvine, CA, USA), capable of handling up to 25 lb, was utilized. This load cell provides precise force measurements.
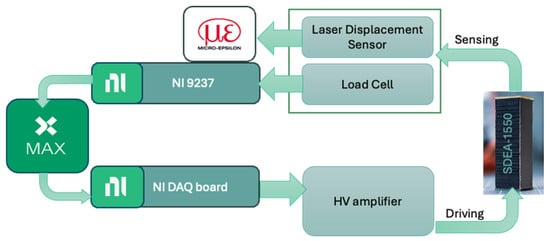
Figure 3.
The control and data acquisition block diagram of the test rig is illustrated. NI MAX was used to control the HV amplifier that drove the actuators (SDEAs) and to read the force data via the NI 9237 module. Displacement data were directly recorded through the Micro-Epsilon software using a laser sensor.
The setup also included three precision translational stages with 10 μm graduation and a 102 N axial load capacity, which allowed for fine-tuning the position of the load cell to ensure accurate alignment and load application. To provide the necessary driving voltage to the SDEAs, we employed a high voltage (HV) fast amplifier (HA51U-1.6P10-3, hivolt.de, Hamburg, Germany) with a slew rate of 80 V/μs. Additionally, a 10 MΩ discharge resistor, recommended by CTsystems, was used for faster discharge of the actuator. This resistor choice ensured an appropriate discharge rate, further contributing to the accuracy and reliability of the hysteresis measurements.
Load cell data were collected via an H-Bridge Input Module (NI 9237, National Instruments in Austin, TX, USA) connected to NI’s compact RIO (cRIO-9045), and the HV amplifier was controlled via an NI DAQ Data Acquisition board (NI USB-6343, National Instruments in Austin, TX, USA). The displacement data of the laser sensor were collected via Micro-Epsilon’s homegrown software (sensorTOOL 1.12.0.1370). Figure 3 demonstrates the testing setup block diagram.
NI MAX (version 2024 Q2) was employed to generate control voltages ranging from 0.625 to 7.5 V, which were subsequently amplified to 100 to 1200 V by the HV amplifier (1:160 ratio). Additionally, NI MAX was used to acquire force data from the load cell.
By integrating these high-precision sensors and advanced data acquisition systems, the test rig allowed for a comprehensive evaluation of the SDEAs’ behavior under different loading scenarios, providing valuable insights into their capabilities and performance.
2.3. Isometric Condition
The experiments were conducted in three steps: (1) securing the SDEA between the load cell and the main frame, (2) precisely adjusting the SDEAs to a neutral position, and (3) applying the driving voltages and recording the load cell data. A set of 10 single SDEAs were tested under this condition.
Three precision translation stages—two at the base for adjusting the X and Y directions and one at the top for adjusting the actuator along the Z-axis (compression-extension)—provide the flexibility needed to precisely position the SDEAs under test (Figure 4). Laser beams were employed as visual guides for vertical alignment. First, a laser beam was emitted onto the front plane of the actuator, and the X-axis precision stage was manually adjusted to align the actuator with the laser. A similar procedure was then followed for the sagittal plane of the actuator, with alignment achieved by adjusting the Y-axis precision stage. Before activation, the Z-axis precision stage was carefully adjusted to a virtual neutral position, ensuring the actuator was neither under tension nor compression. Then, the generated force under each activation voltage was recorded.

Figure 4.
The figure illustrates an actuator under isometric conditions, with one fixed end at the bottom and the other end connected to a load cell. It is crucial for the actuator to remain vertical; therefore, we used a laser balance to ensure proper alignment and adjusted the actuator using two precision translation stages.
Using the data collected, maximum stress was calculated by dividing the averaged maximum force (reported as mean and one standard deviation) of the ten SDEA-1550s by the area of the SDEA’s endplate (225 mm2).
2.4. Isotonic Condition
In the isotonic condition, as shown in Figure 5, the SDEAs were tested while suspended from the Z stage using a 3D-printed bracket, with a series of tensile loads consisting of seven loads (100 to 700 g in 100 g increments) attached to the actuators. The contraction of the SDEA (Figure 5a) and the 1 × 3 configuration (Figure 5b) under these loaded conditions were measured by applying the activation voltages (from 100 V to 1200 V). The laser displacement sensor was beaming at the extended endplate of the lowest SDEA.
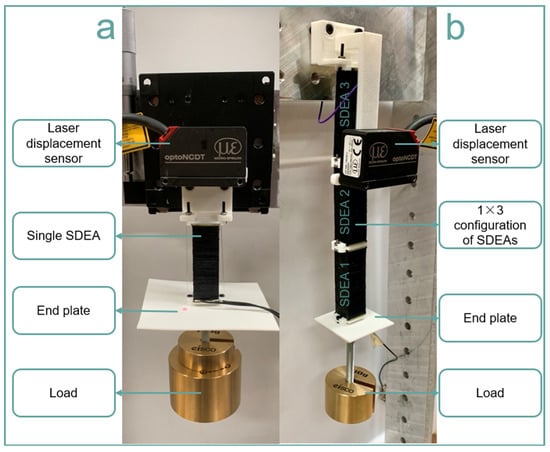
Figure 5.
The figure depicts isotonic conditions, which include: (a) fixing the actuator at the top end to the Z stage, hanging various loads from the bottom end, and measuring contraction using a displacement laser sensor; and (b) fixing a 1 × 3 configuration of SDEAs, hanging various loads from the bottom end, and measuring contraction using a displacement laser sensor.
Using the data collected from the isotonic condition, the maximum longitudinal strain was calculated by dividing the averaged maximum longitudinal contraction (reported as mean and standard deviation) of the SDEAs by their normal length of 50 mm.
2.5. Free-Standing Condition
In the free-standing condition, as shown in Figure 6, we fixed the actuator vertically to the base of the test rig’s mainframe. This setup ensured that the actuator remained stable throughout the testing process. The displacement generated by each driving voltage was then precisely measured using the laser displacement sensor. This sensor was mounted directly on top of the SDEAs. Single SDEA (Figure 6a) and 1 × 3 configurations (Figure 6b) were evaluated under this condition.
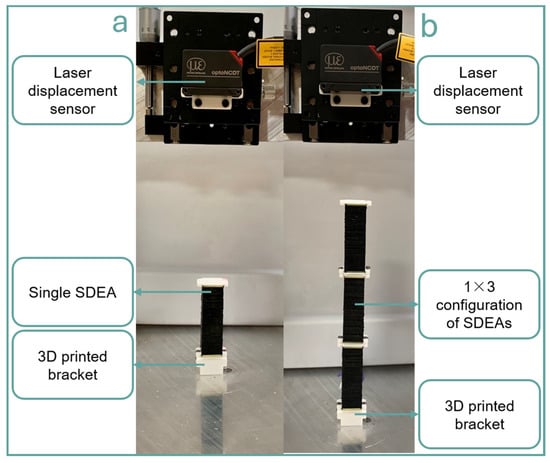
Figure 6.
The figure depicts SDEAs under free-standing conditions, which include: (a) applying different voltages to an actuator fixed at the bottom and measuring contraction using a laser displacement sensor; and (b) applying different voltages to a 1 × 3 configuration of SDEAs and measuring contraction using the laser displacement sensor.
For comparisons between actuators, a set of 10 SDEA-1550 went through the isometric, isotonic, and free-standing conditions to compare the reliability of the actuators in producing displacement.
2.6. Stiffness
We evaluated a single DEA’s stiffness using a Shimadzu Universal Electromechanical Test Frame. The test was conducted under two orientations: vertical and horizontal, as shown in Figure 7. The objective was to determine the stiffness of the actuator when subjected to a constant displacement of 1 mm while applying different voltages.
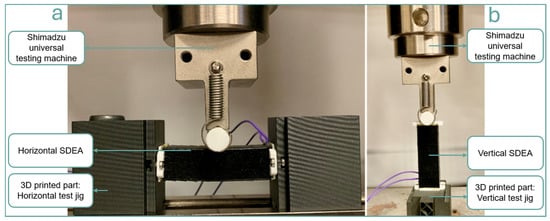
Figure 7.
The figure depicts an actuator under stiffness testing, (a) in a horizontal orientation and (b) in a vertical orientation. A Shimadzu universal testing machine applies a 1 mm displacement to the actuator at different voltages, and the feedback force is measured using the machine’s load cell.
The procedure involved placing the actuator on the test frame and applying loads vertically and horizontally. The stiffness, k, was calculated using the following equation:
where F is the force applied by the Shimadzu Universal Electromechanical Test Frame, and d is the constant displacement of 1 mm.
This testing approach allowed us to assess the actuator’s stiffness characteristics under varying driving voltages, providing valuable insights into its mechanical properties.
The force and displacement data for both single actuators and the assembled artificial muscle are graphed. For the comparison between actuators, we report the range, mean, and standard deviation (mean ± SD) of force and displacement, as well as the maximum and mean values of stress and strain. Note that 1200 V was the recommended voltage for the old version, and 800 V is the recommended voltage for the latest model; therefore, we focused on these two values more closely throughout the Section 3.
3. Results
3.1. Isometric Condition
In the first experiment, conducted under isometric conditions, we tested a set of 10 randomly selected actuators. The results, shown in Figure 8, indicate that at the recommended voltage of 800 V, the measured force ranged from 9.37 N to 12.21 N, with an average of 10.92 ± 0.73 N. Based on the actuator’s surface area of 225 mm2, the minimum and maximum stresses were calculated as 41.64 KPa and 54.27 KPa, respectively.
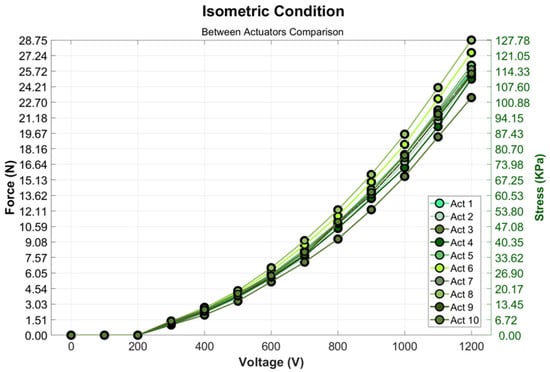
Figure 8.
The graph shows the force and the stress that each of the 10 actuators can generate in isometric condition at voltages up to 1200 V.
By increasing the voltage to 1200 V, recommended for the previous version (CT-SDEA), a significant rise in force was observed, with forces ranging from 23.15 N to 28.75 N and a mean of 25.84 ± 1.50 N. The mean and maximum stresses were calculated at 114.84 kPa and 127.78 kPa, respectively.
3.2. Isotonic Condition
Figure 9 presents the displacements of the actuator under constant loads and different voltage levels for a single actuator. The data indicate that the maximum displacement occurred with the minimum load of 100 g, as the actuator was able to compress more easily under a lighter load. As shown in Figure 9, the maximum shortening (displacement) at 1200 V occurs with a 100 g load at −5.79 mm, while the minimum shortening is observed with a 700 g load at −3.77 mm. For the recommended voltage (800 V), the maximum and minimum shortening at 100 g and 700 g loads were −2.19 mm and −0.39 mm, respectively.

Figure 9.
The graph shows seven separate experiments on one actuator at different voltages, up to 1200 V, using loads ranging from 100 g to 700 g.
We compared the shortening of ten actuators under varying voltage levels while subjected to a constant 200 g load. As shown in Figure 10, at 1200 V, the range of shortening displacement is from −6.09 mm to −4.17 mm, with a mean value of −5.30 ± 0.65 mm. The maximum strain observed at 1200 V is 12%. The mean shortening at 800 V was −1.98 ± 0.14 mm with a maximum strain of 4.4%.
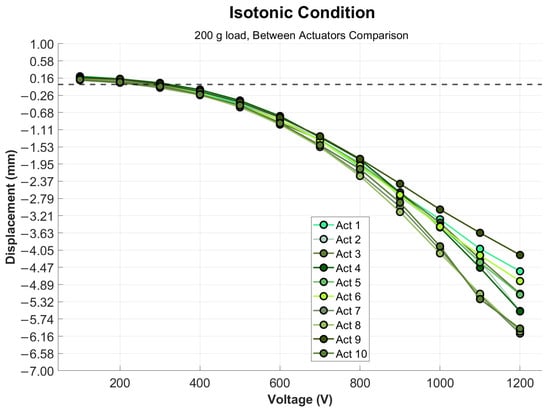
Figure 10.
The graph shows the shortening of ten different actuators under a 200 g load and various voltages up to 1200 V.
The result of the artificial muscle shortening under isotonic conditions is illustrated in Figure 11. As expected, the graph shows that the maximum displacement occurs at 1200 V, ranging from −17.92 mm to −14.04 mm for 100 g and 700 g loads, respectively, with a mean displacement of −16.43 ± 1.48 mm.
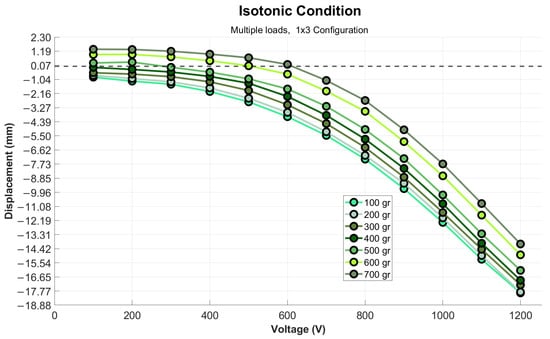
Figure 11.
The graph shows the shortening of a 1 × 3 configuration of DEAs under different loads, ranging from 100 g to 700 g, and voltages up to 1200 V.
3.3. Free-Standing Condition
As Figure 12 shows, at 1200 V, under free-standing conditions, the displacements ranged from −6.15 mm to −4.4 mm, with a mean displacement of −5.45 ± 0.53 mm. The mean shortening at 800 V (the recommended voltage) was −2.25 ± 0.24 mm. As the voltage increases, a significant difference of 1.75 mm is observed between actuators’ shortening at 1200 V.
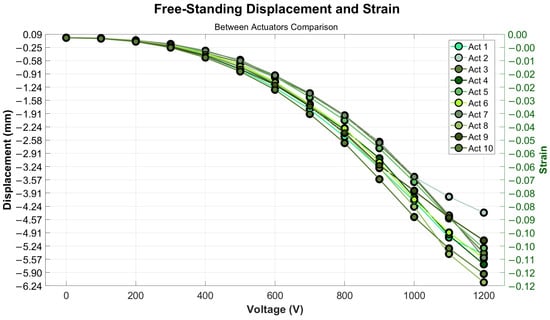
Figure 12.
The graph shows the shortening and the strain of ten different actuators under voltages up to 1200 V in a no-load condition.
Table 2 presents the mean ± SD for isometric, isotonic, and free-standing conditions for the comparisons between actuators at the driving voltages ranging from 100 to 1200 V. By increasing the voltage, the variability between actuators increases, as reflected in SDs; this is specifically the case past 800 V, the recommended voltage of SDEA-1550.

Following the experiments conducted on ten individual actuators, we further tested a 1 × 3 artificial muscles. The objective was to assess the combined displacement behavior of this configuration under varying voltage levels.
The results of these tests are presented in Figure 13, which illustrates the displacement of the series-connected actuators across a range of voltages from 0 to 1200 V in freestanding conditions. As depicted in Figure 13, the displacement increases with the applied voltage, reaching a maximum value of −16.44 mm at 1200 V, while the shortening at 800 V was −6.9 mm.
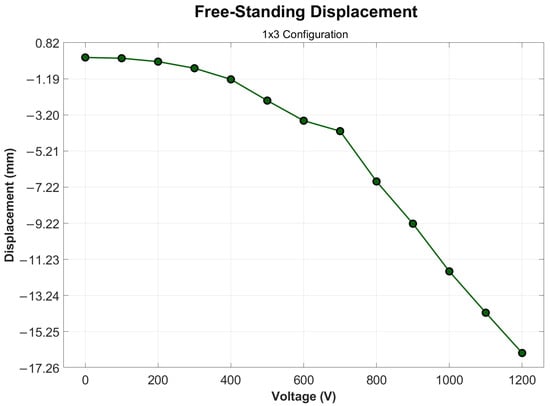
Figure 13.
The graph shows the shortening of a 1 × 3 configuration of DEAs under voltages up to 1200 V in a no-load condition.
3.4. Stiffness
We tested the stiffness of an actuator along the X (horizontal) and Y (vertical) directions under different voltages, ranging from 800 V to 1200 V. The results are presented in Figure 14. As shown in Figure 14, the actuator exhibits greater stiffness in the Y (vertical) direction, with a maximum stiffness of 1.99 MPa at 1200 V. The stiffness in the X (horizontal) direction ranged from 0.034 MPa at 800 V to 0.086 MPa at 1200 V.
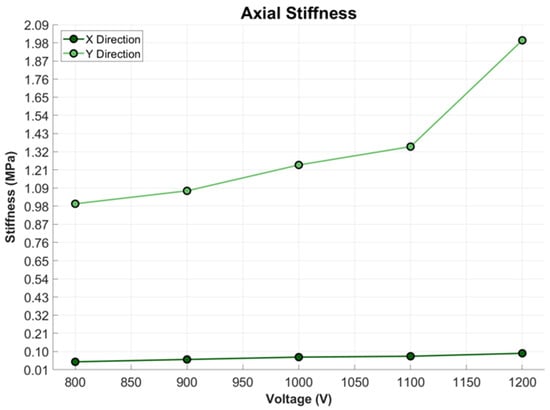
Figure 14.
The graph shows the stiffness of one actuator in the Y (vertical) and X (horizontal) directions under different voltages, ranging from 800 V to 1200 V.
The summary of the measured mechanical properties of SDEA-1550 at 800 V and 1200 V is presented in Figure 15. The values are compared with those measured in our previous study []. The shortening and strain values reported in the table are from the free-standing condition.
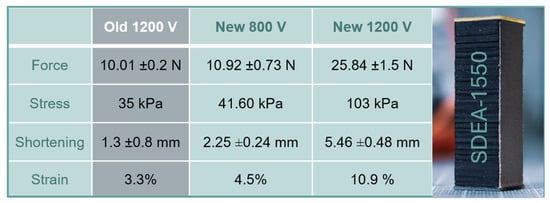
Figure 15.
The measured mechanical property of SDEA-1550 at 800 V (the recommended voltage) and 1200 V (the recommended voltage for CT-SDEA) in comparison to our reported value for CT-SDEA []. The presented values are the mean values; the force and shortening values are presented as mean ± SD.
4. Discussion
In this study, we benchmarked the latest version of the commercially available SDEA-1550, revealing substantial improvements over the previous CT-SDEA model we evaluated in our earlier research []. The SDEA-1550 demonstrated a threefold enhancement in strain and stress, increasing from 3.3% to 10.9% strain and from 35 kPa to 115 kPa stress under the recommended driving voltage of the CT-SDEA, 1200 V. Notably, the recommended voltage for the SDEA-1550 has been reduced by approximately 30%, down to 800 V, which is a significant advantage in terms of compatibility with electronics, safety, and reduced power consumption. At 800 V, the SDEA-1550 exhibited 4.5% strain and 41.6 KPa stress. It is important to note that the pressure between the electrodes of each layer of the SDEA (each compliant capacitor stacked in parallel) is proportional to the square of the applied voltage (as described in Equation (1)). This relationship explains the substantial increase in both stress and strain when the voltage is increased from 800 V to 1200 V, as reflected in the logarithmic shape of the resulting graphs.
The mechanical properties of the SDEAs are approaching those of biological skeletal muscle, as highlighted in our previous work [,]. Typical strain and stress values reported for human biceps muscle are around 20% and 100 kPa, respectively []. Although the 11% strain measured for the SDEA-1550 is lower (slightly more than half) than that of the biceps, it represents a significant improvement—more than three times the value of previous iterations. Moreover, the stress generated by the SDEA-1550, 115 KPa, now surpasses that of the biceps, which is a remarkable achievement in the development of biomimetic actuators. The substantial improvement is likely due to enhanced production quality of each layer, particularly the electrodes of the compliant capacitors. Since each actuator consists of hundreds of such capacitors, even slight improvements are magnified, resulting in a significant enhancement of the actuator’s overall performance.
The stress and strain capabilities of the SDA1550 demonstrate significant potential for rehabilitation robotics, particularly in exoskeleton applications. Building upon this, we aim to refine the design of our robotic ankle exoskeleton, the DE-AFO. The DE-AFO is a biomimetic robot powered by artificial muscles configured using SDEA. Figure 16 illustrates the DE-AFO, a comfortable robotic exoskeleton designed to facilitate walking in children with cerebral palsy (CP) []. The artificial muscles in this design will actuate in synchrony with the ankle muscles, utilizing a finite-state controller that detects different phases of the gait cycle []. Such designs could significantly benefit various populations with neuromotor pathologies, including individuals with cerebral palsy [], stroke [], and multiple sclerosis []. Leveraging the varying stiffness and length self-sensing capabilities of SDEAs, we see the potential for these actuators to be incorporated into the upper limb [] and hand exoskeletons and grippers with haptic control [], which is another avenue we plan to investigate. Previously, we developed an elbow exoskeleton powered by CT-SDEA-based artificial muscles. By leveraging the enhanced stress and strain capabilities of the SDEA1550, we expect a significant improvement in the exoskeleton’s performance. The previous design was capable of generating 19° of elbow flexion under no load [], and the updated SDEA1550 actuators are anticipated to further increase this range and improve overall functionality.
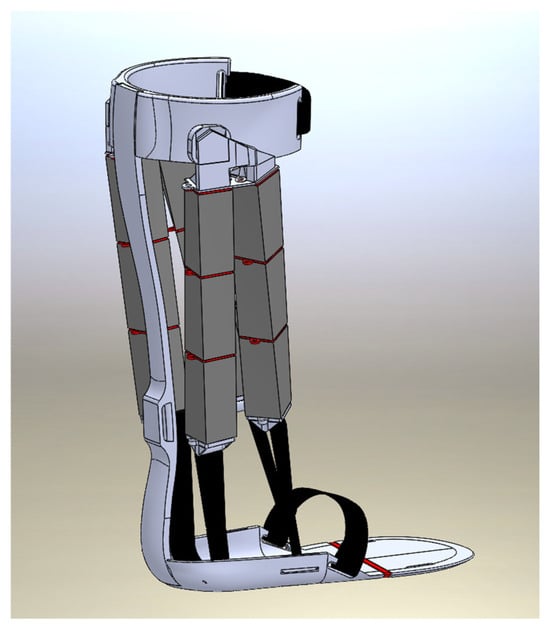
Figure 16.
The biomimetic AFO, which is powered by SDEA-based artificial muscles.
5. Conclusions
SDEAs are emerging as strong candidates for constructing artificial skeletal muscles, particularly in the context of biomimetic rehabilitation robots where the natural feel is a crucial component for user compliance. In this study, we evaluated the latest commercially available SDEAs produced by Dätwyler Schweiz AG and compared them to their previous versions produced by CTsystems. Our findings reveal that the new SDEAs exhibit substantially improved strain and stress, increased by more than 300%. The stress value now surpasses the typical value reported for the biceps of humans. Combined with their muscle-like characteristics—such as longitudinal contraction, softness, and noiseless operation—these enhancements further establish SDEAs as increasingly comparable to biological human muscles. This progress underscores their potential as soft motor units in artificial skeletal muscles, reinforcing their suitability for next-generation rehabilitation robotics.
Author Contributions
Conceptualization, A.B. and S.C.K.L.; methodology, A.B. and V.M.; software, M.T. and V.M.; validation, A.B. and V.M.; formal analysis, V.M. and S.M.G.; investigation, A.B. and V.M.; resources, A.B.; data curation, M.T., V.M. and S.M.G.; writing—original draft preparation, V.M.; writing—review and editing, A.B., V.M. and S.C.K.L.; visualization, V.M. and M.T.; supervision, A.B.; project administration, A.B. and S.C.K.L.; funding acquisition, A.B. All authors have read and agreed to the published version of the manuscript.
Funding
This research was funded by Shriners Hospitals for Children—Philadelphia, Grant No. 71011-PHI; the National Science Foundation I-Corps, Grant No. 1906128; the University Science Center’s QED award, Project No. 1803; and the National Institute of Health DE-CTR ACCEL, Grant No. U54-GM104941. This research was supported by the National Institutes of Health (NIH) under Grants P20GM109090 and P20GM152301.
Data Availability Statement
Data are contained within the article.
Acknowledgments
We would like to express our sincere gratitude to the NIH for their generous funding, which made this project possible. The resources and support provided through these grants have been instrumental in advancing our research on rehabilitation robotics and artificial skeletal muscles.
Conflicts of Interest
The corresponding authors are founders of Elasthetics LLC, which was established to potentially commercialize DE-AFO in the future.
References
- Rus, D.; Tolley, M.T. Design, fabrication and control of soft robots. Nature 2015, 521, 467–475. [Google Scholar] [CrossRef] [PubMed]
- Verl, A.; Albu-Schäffer, A.; Brock, O.; Raatz, A. (Eds.) Soft Robotics: Transferring Theory to Application; Springer: Berlin/Heidelberg, Germany, 2015; Available online: https://link.springer.com/10.1007/978-3-662-44506-8 (accessed on 25 August 2024).
- Miriyev, A.; Stack, K.; Lipson, H. Soft material for soft actuators. Nat. Commun. 2017, 8, 596. [Google Scholar] [CrossRef]
- Yoo, I.S.; Reitelshöfer, S.; Landgraf, M.; Franke, J. Artificial Muscles, Made of Dielectric Elastomer Actuators—A Promising Solution for Inherently Compliant Future Robots. In Soft Robotics; Verl, A., Albu-Schäffer, A., Brock, O., Raatz, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2015; pp. 33–41. Available online: https://link.springer.com/10.1007/978-3-662-44506-8_4 (accessed on 25 August 2024).
- Carpi, F.; De Rossi, D.; Kornbluh, R.; Pelrine, R.E.; Sommer-Larsen, P. (Eds.) Dielectric Elastomers as Electromechanical Transducers; Elsevier Science: Amsterdam, The Netherlands, 2011. [Google Scholar]
- Hoffstadt, T.; Griese, M.; Maas, J. Online identification algorithms for integrated dielectric electroactive polymer sensors and self-sensing concepts. Smart Mater. Struct. 2014, 23, 104007. [Google Scholar] [CrossRef]
- Zhang, R.; Kunz, A.; Lochmatter, P.; Kovacs, G. Dielectric Elastomer Spring Roll Actuators for a Portable Force Feedback Device. In Proceedings of the 2006 14th Symposium on Haptic Interfaces for Virtual Environment and Teleoperator Systems, Alexandria, VA, USA, 25–26 March 2006; IEEE: Alexandria, VA, USA, 2006; pp. 347–353. Available online: http://ieeexplore.ieee.org/document/1627137/ (accessed on 25 August 2024).
- IEC. 60479-2: 2007 Effects of Current on Human Beings and Livestock. Part. Available online: https://webstore.iec.ch/en/publication/63392 (accessed on 23 September 2024).
- Carpi, F.; Frediani, G.; Gerboni, C.; Gemignani, J.; De Rossi, D. Enabling variable-stiffness hand rehabilitation orthoses with dielectric elastomer transducers. Med. Eng. Phys. 2014, 36, 205–211. [Google Scholar] [CrossRef] [PubMed]
- Mohammadi, V.; Tajdani, M.; Masaei, M.; Mohammadi Ghalehney, S.; Lee, S.C.K.; Behboodi, A. DE-AFO: A Robotic Ankle Foot Orthosis for Children with Cerebral Palsy Powered by Dielectric Elastomer Artificial Muscle. Sensors 2024, 24, 3787. [Google Scholar] [CrossRef] [PubMed]
- Allen, D.P.; Little, R.; Laube, J.; Warren, J.; Voit, W.; Gregg, R.D. Towards an ankle-foot orthosis powered by a dielectric elastomer actuator. Mechatronics 2021, 76, 102551. [Google Scholar] [CrossRef]
- Sarban, R.; Jones, R.W.; Mace, B.R.; Rustighi, E. A tubular dielectric elastomer actuator: Fabrication, characterization and active vibration isolation. Mech. Syst. Signal Process. 2011, 25, 2879–2891. [Google Scholar] [CrossRef]
- Carpi, F.; Migliore, A.; Serra, G.; Rossi, D.D. Helical dielectric elastomer actuators. Smart Mater. Struct. 2005, 14, 1210–1216. [Google Scholar] [CrossRef]
- Carpi, F.; Salaris, C.; Rossi, D.D. Folded dielectric elastomer actuators. Smart Mater. Struct. 2007, 16, S300–S305. [Google Scholar] [CrossRef]
- Kovacs, G.; Düring, L.; Michel, S.; Terrasi, G. Stacked dielectric elastomer actuator for tensile force transmission. Sens. Actuators A Phys. 2009, 155, 299–307. [Google Scholar] [CrossRef]
- Kunze, J.; Prechtl, J.; Bruch, D.; Nalbach, S.; Motzki, P.; Seelecke, S.; Rizzello, G. Design and fabrication of silicone-based dielectric elastomer rolled actuators for soft robotic applications. In Electroactive Polymer Actuators and Devices (EAPAD) XXII; Bar-Cohen, Y., Anderson, I.A., Shea, H.R., Eds.; Online Only; SPIE: Bellingham, WA, USA, 2020; p. 80. Available online: https://www.spiedigitallibrary.org/conference-proceedings-of-spie/11375/2558444/Design-and-fabrication-of-silicone-based-dielectric-elastomer-rolled-actuators/10.1117/12.2558444.full (accessed on 25 August 2024).
- Gabor Kovacs, L.D. CTSystem Swiss Compliant Transducer. 2016. Available online: http://www.ct-systems.ch/ (accessed on 27 September 2024).
- Behboodi, A.; Lee, S.C.K. Benchmarking of a Commercially Available Stacked Dielectric Elastomer as an Alternative Actuator for Rehabilitation Robotic Exoskeletons. In Proceedings of the 2019 IEEE 16th International Conference on Rehabilitation Robotics (ICORR), Toronto, ON, Canada, 24–28 June 2019; IEEE: Toronto, ON, Canada, 2019; pp. 499–505. Available online: https://ieeexplore.ieee.org/document/8779378/ (accessed on 25 August 2024).
- Pappas, G.P.; Asakawa, D.S.; Delp, S.L.; Zajac, F.E.; Drace, J.E. Nonuniform shortening in the biceps brachii during elbow flexion. J. Appl. Physiol. 2002, 92, 2381–2389. [Google Scholar] [CrossRef]
- Behboodi, A.; Zahradka, N.; Wright, H.; Alesi, J.; Lee, S.C.K. Real-Time Detection of Seven Phases of Gait in Children with Cerebral Palsy Using Two Gyroscopes. Sensors 2019, 19, 2517. [Google Scholar] [CrossRef]
- Jiang, H.; Zhou, X.; Li, X.; Chen, Z.; Du, Q.; Xie, L. Flexible Lower Limb Exoskeleton Robot for Rehabilitation Training of Children with Cerebral Palsy. J Shanghai Jiaotong Univ. Sci. 2024. [Google Scholar] [CrossRef]
- Awad, L.N.; Kudzia, P.; Revi, D.A.; Ellis, T.D.; Walsh, C.J. Walking Faster and Farther with a Soft Robotic Exosuit: Implications for Post-Stroke Gait Assistance and Rehabilitation. IEEE Open J. Eng. Med. Biol. 2020, 1, 108–115. [Google Scholar] [CrossRef]
- Ajami, H.; Kargar Nigjeh, M.; Umbaugh, S.E. Unsupervised white matter lesion identification in multiple sclerosis (MS) using MRI segmentation and pattern classification: A novel approach with CVIPtools. In Applications of Digital Image Processing XLVI; Tescher, A.G., Ebrahimi, T., Eds.; SPIE: San Diego, CA, USA, 2023; p. 59. Available online: https://www.spiedigitallibrary.org/conference-proceedings-of-spie/12674/2688268/Unsupervised-white-matter-lesion-identification-in-multiple-sclerosis-MS-using/10.1117/12.2688268.full (accessed on 28 August 2024).
- Maciejasz, P.; Eschweiler, J.; Gerlach-Hahn, K.; Jansen-Troy, A.; Leonhardt, S. A survey on robotic devices for upper limb rehabilitation. J. Neuroeng. Rehabil. 2014, 11, 3. Available online: https://jneuroengrehab.biomedcentral.com/articles/10.1186/1743-0003-11-3 (accessed on 16 September 2024). [CrossRef] [PubMed]
- Mohammadi, V.; Shahbad, R.; Hosseini, M.; Gholampour, M.H.; Shiry Ghidary, S.; Najafi, F.; Behboodi, A. Development of a Two-Finger Haptic Robotic Hand with Novel Stiffness Detection and Impedance Control. Sensors 2024, 24, 2585. [Google Scholar] [CrossRef] [PubMed]
- Behboodi, A.; DeSantis, C.; Lubsen, J.; Lee, S.C.K. A Mechanized Pediatric Elbow Joint Powered by a De-Based Artificial Skeletal Muscle. In Proceedings of the 2020 42nd Annual International Conference of the IEEE Engineering in Medicine & Biology Society (EMBC), Montreal, QC, Canada, 20–24 July 2020; IEEE: Montreal, QC, Canada, 2020; pp. 4930–4935. Available online: https://ieeexplore.ieee.org/document/9176332/ (accessed on 16 September 2024).
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).