Dynamic Response Analysis of a Magnetically Suspended Dual-Rotor System Considering the Uncertainty of Interference-Fit Value
Abstract
1. Introduction
2. Dual-Rotor Model and Its Motion Equation
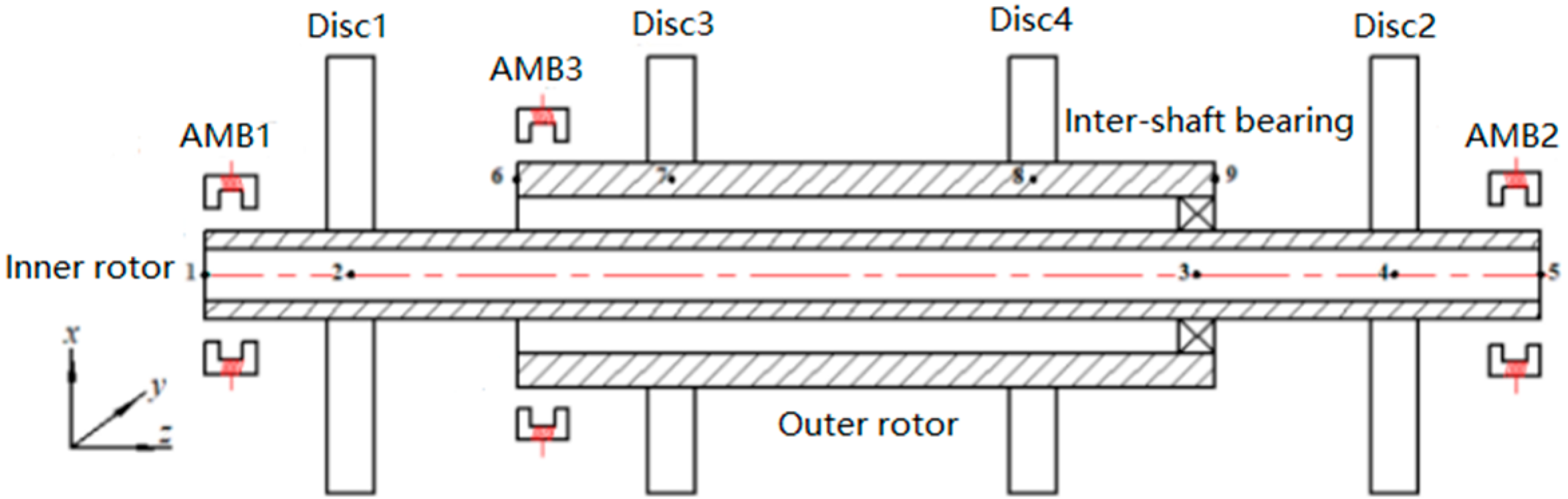
2.1. Structure of the Magnetically Suspended Dual-Rotor System
2.2. Dynamics Equations of the System
3. Interval Method Model with Uncertainty of Interference Value
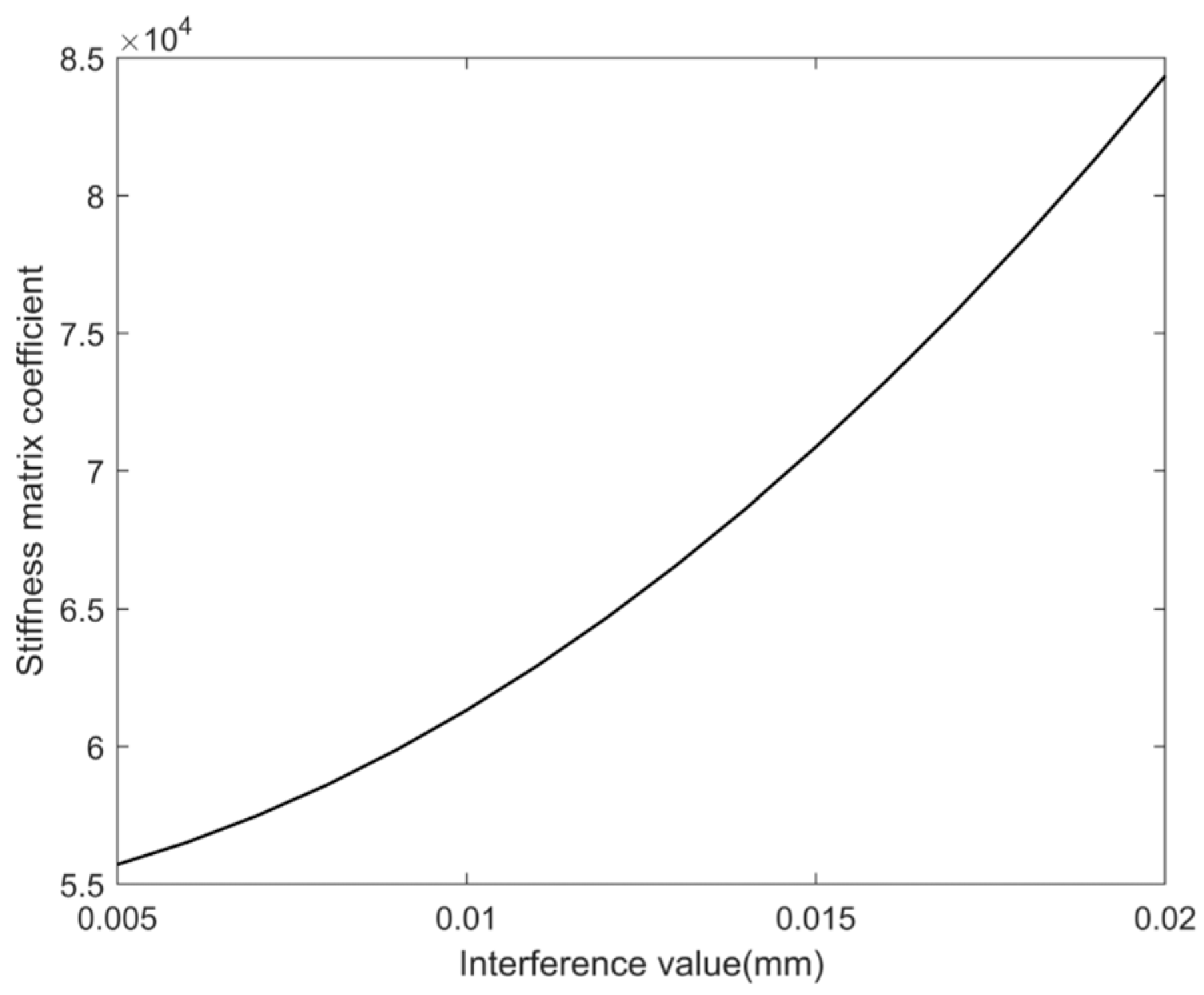
3.1. Effect of Interference Value on Rotor Stiffness
3.2. Interval Method Model Based on Chebyshev Polynomials
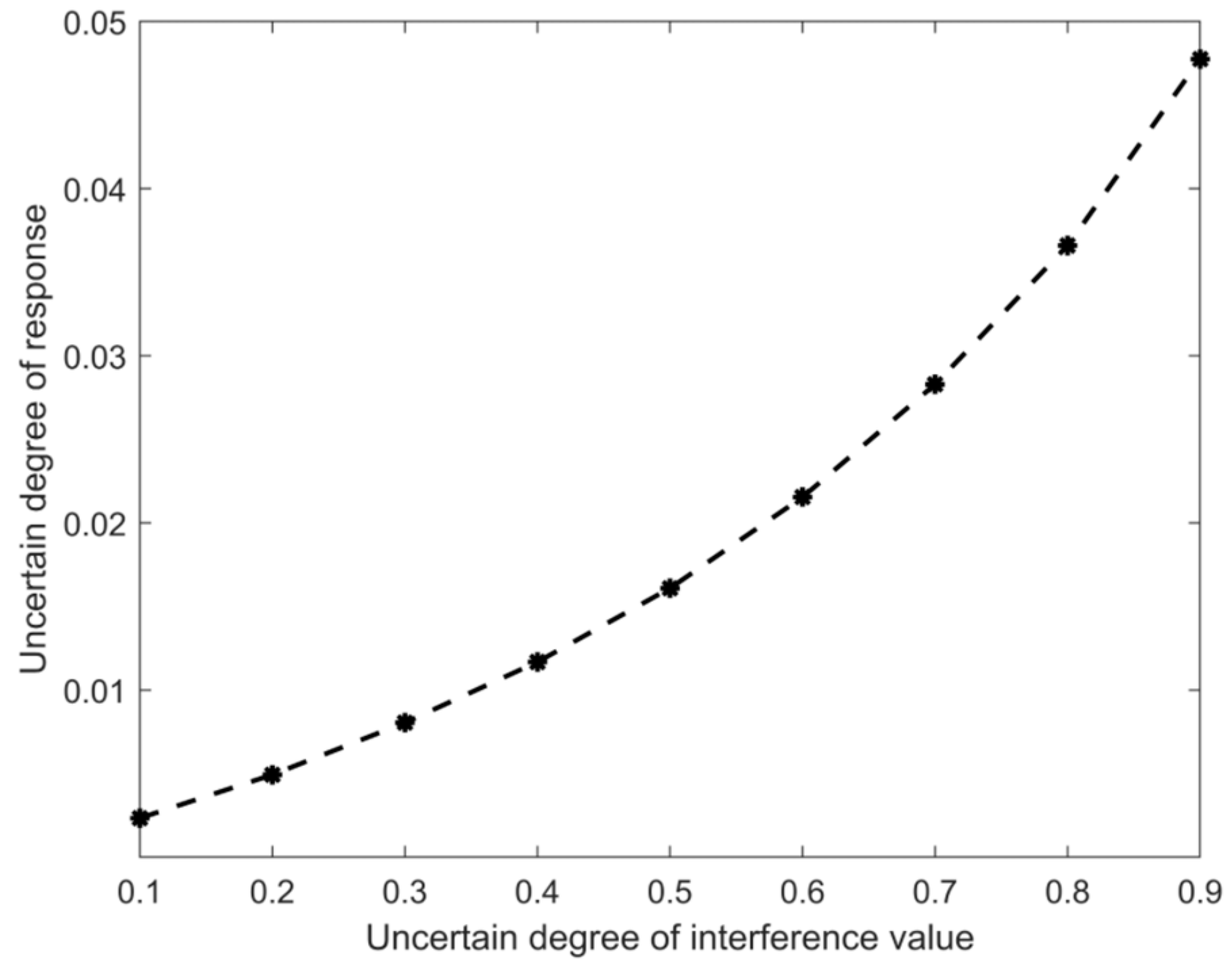
4. Numerical Results
4.1. Verification of Stiffness Equation
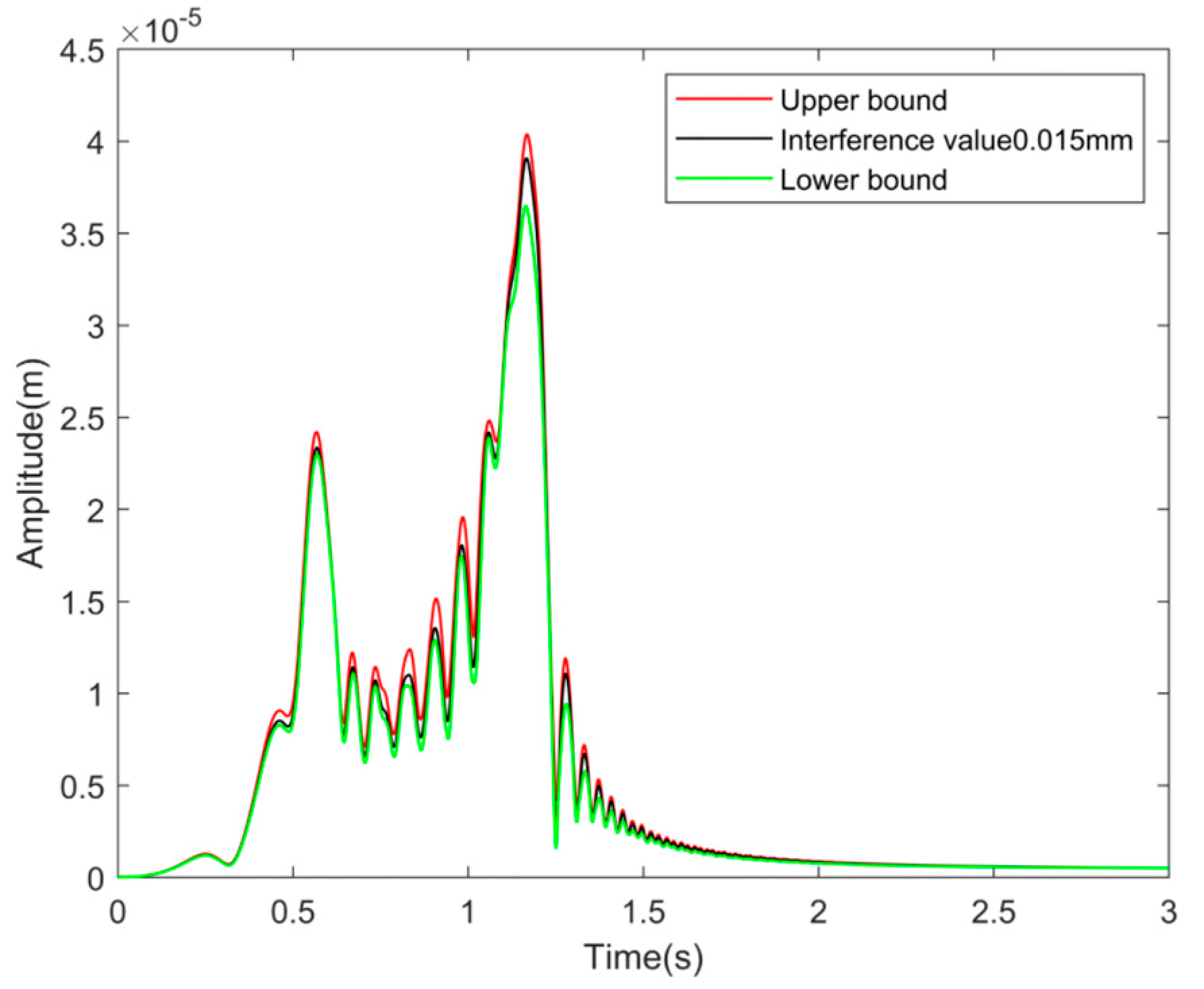
4.2. Transient Response Analysis
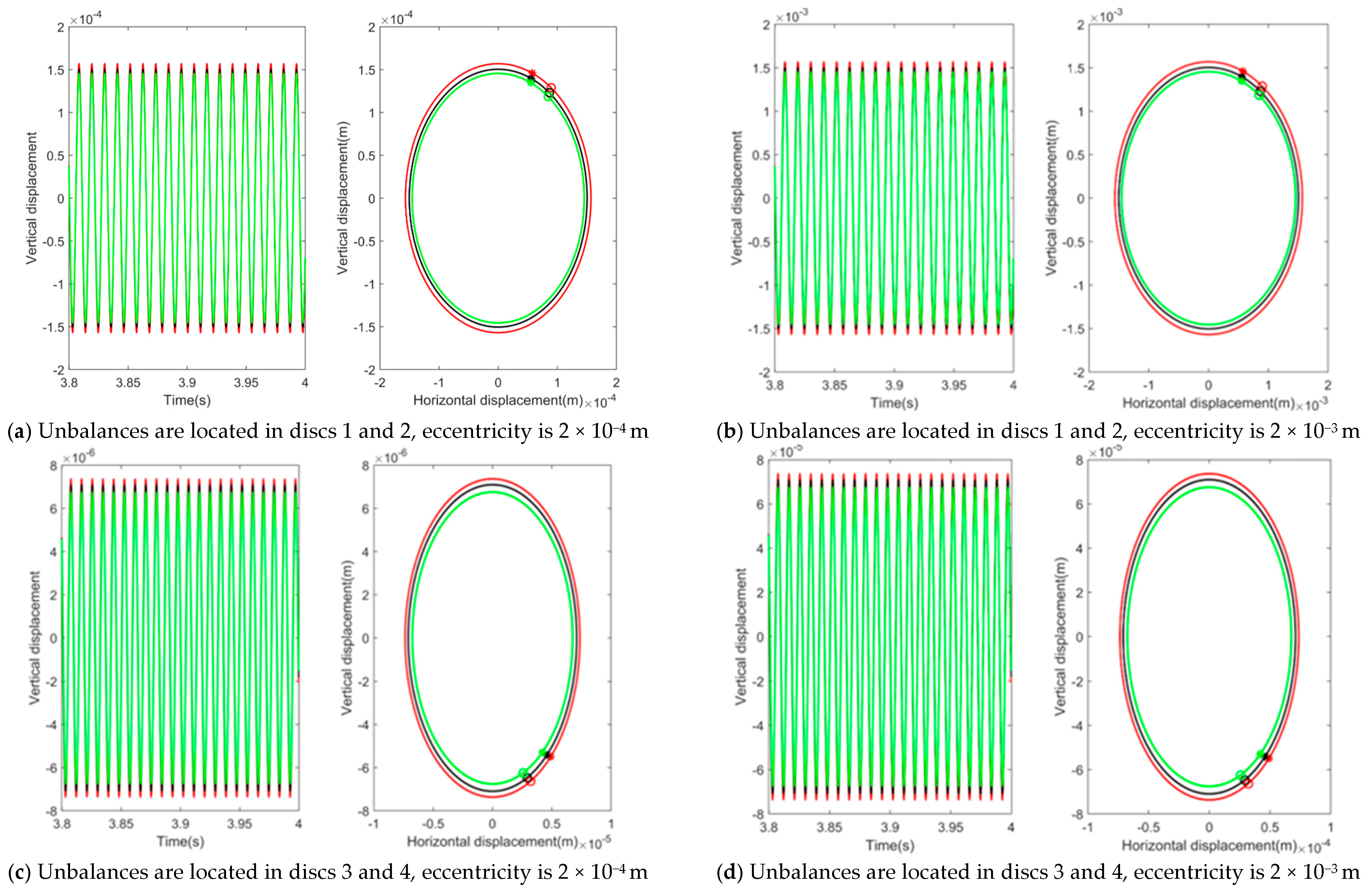
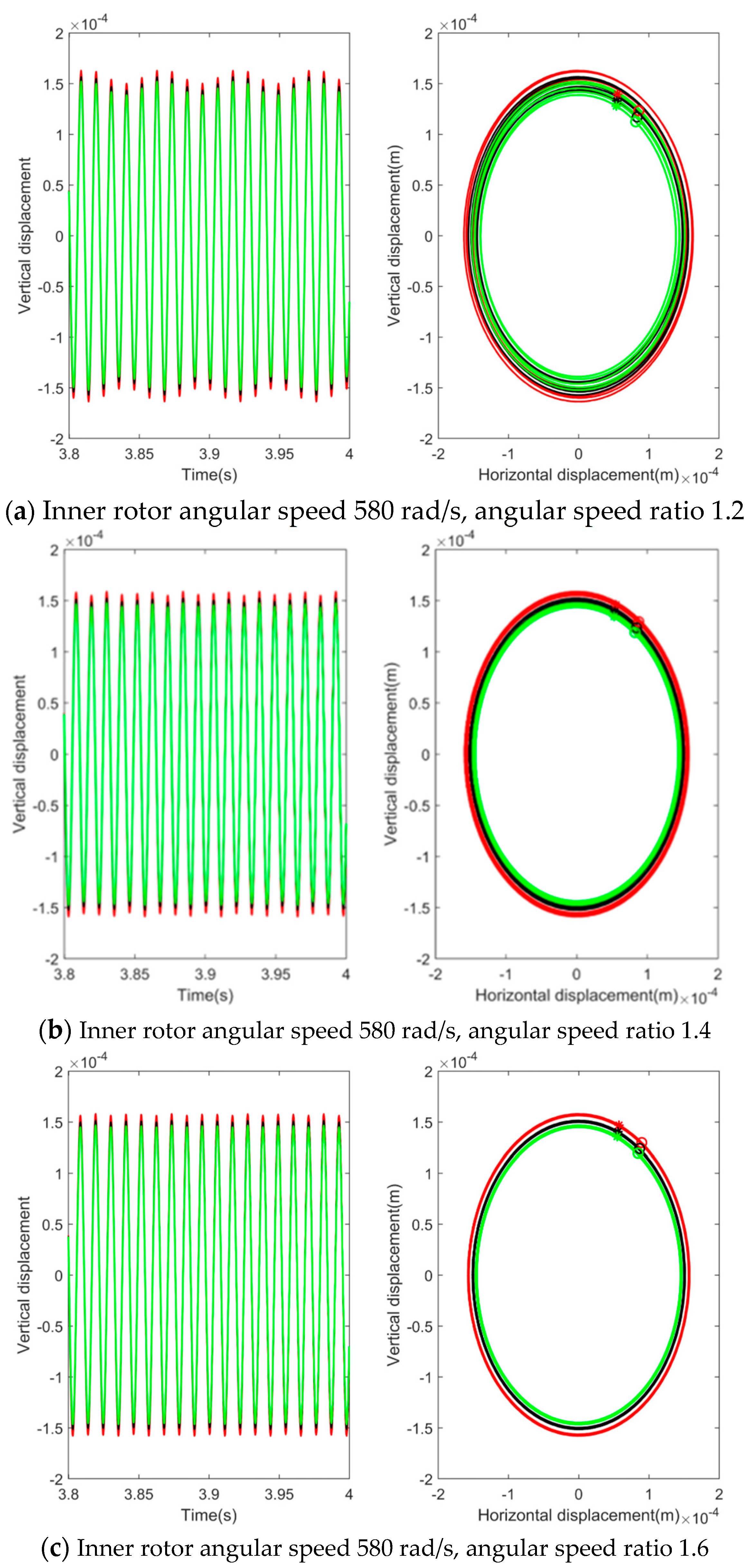
4.3. Steady-State Response Analysis
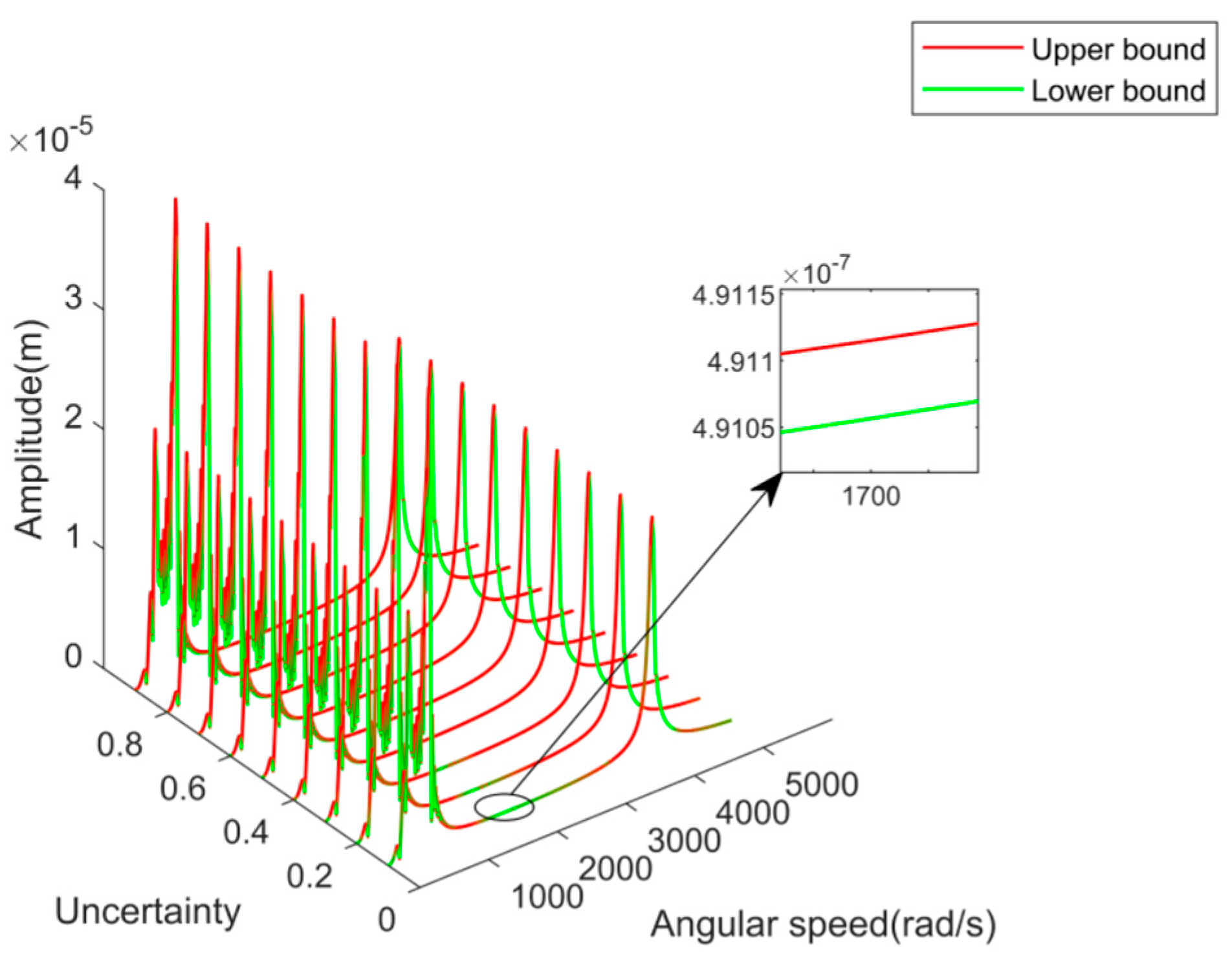
4.3.1. Influence of Unbalance on Steady-State Response
4.3.2. Influence of Angular Speed Ratio on Steady-State Response
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hu, Y.; Zhou, D.; Jiang, Z. Basic Theory and Application of Magnetic Bearings; Mechanical Industry Press: Beijing, China, 2006; pp. 7–8. [Google Scholar]
- Li, S.; He, Z.; Zhao, P. Study on the design method of interference fit between gear and shaft of automobile transmission. J. Phys. Conf. Ser. 2021, 1885, 052067. [Google Scholar] [CrossRef]
- Hu, C.; Yu, H.; Xia, Z.; Gu, B. Flexible assembly research for cylindrical interference fit with form error in shaft-hole structures. Proc. Inst. Mech. Eng. 2023, 237, 203–215. [Google Scholar] [CrossRef]
- Liu, S.; Chen, X.; Song, C.; Zhu, C.; Bai, H.; Fuentes-Aznar, A. Influence of gear-shaft interference fit assembly on the meshing characteristics of cylindrical gears considering comprehensive modifications. Mech. Mach. Theory 2023, 182, 105247. [Google Scholar] [CrossRef]
- Suo, H.; Wei, Z.; Luo, B.; Wang, L.; Zhang, K.; Liang, B.; Deng, K.; Cheng, H. Interfacial wear damage mechanism between Ti-alloy and Al-alloy in interference-fit joint and influence of surface coatings: Experimental and numerical study. Eng. Fail. Anal. 2023, 143, 106931. [Google Scholar] [CrossRef]
- Shu, Y.; Yang, G.; Liu, Z. Experimental study on fretting damage in the interference fit area of high-speed train wheels and axles based on specimen. Eng. Fail. Anal. 2022, 141, 106619. [Google Scholar] [CrossRef]
- Lanoue, F.; Vadean, A.; Sanschagrin, B. Finite element analysis and contact modelling considerations of interference fits for fretting fatigue strength calculations. Simul. Model. Pract. Theory 2009, 17, 1587–1602. [Google Scholar] [CrossRef]
- Wang, Z.; Wang, Z.; Bai, X.; Zhang, X.; Wang, Y. Effect of interference fit on dynamic characteristics of spindle rotor system. J. Braz. Soc. Mech. Sci. Eng. 2022, 44, 316. [Google Scholar] [CrossRef]
- Güven, F. Effect of design parameters on stresses occurring at the tooth root in a spur gear pressed on a shaft. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2021, 235, 1164–1174. [Google Scholar] [CrossRef]
- Pedersen, N.L. On optimization of interference fit assembly. Struct. Multidisc. Optim. 2016, 54, 349–359. [Google Scholar] [CrossRef]
- Gruescu, C.M.; Davidescu, A.; Sticlaru, C.; Lovasz, E.C. Interference Fits. Bearing Capacity Under Complex Loading-FEM Analysis. New Adv. Mech. Mech. Transm. Robot. 2020, 88, 403–414. [Google Scholar]
- Storti, G.C.; Fonçatti, L.G. Rotordynamic Influence of a Spider Mounted on Shaft with Interference Fit in an Electric Machine Rotor. In Proceedings of the 10th International Conference on Rotor Dynamics-IFToMM; Springer International Publishing: Berlin/Heidelberg, Germany, 2018; Volume 61, pp. 530–546. [Google Scholar]
- Kovan, V. Separation frequency analysis of interference fitted hollow shaft-hub connections by finite element method. Adv. Eng. Softw. 2011, 42, 644–648. [Google Scholar] [CrossRef]
- Vishwakarma, N.; Renuke, A.; Phalle, V. Effect of Tapered Interference Fit between Impeller and Shaft in Turbo Machines. Stroj. Cas. J. Mech. Eng. 2018, 68, 25–32. [Google Scholar] [CrossRef]
- Wang, J.; Yang, Y.; Zheng, Q.; Deng, W.; Zhang, D.; Fu, C. Dynamic Response of Dual-Disk Rotor System with Uncertainties Based on Chebyshev Convex Method. Appl. Sci. 2021, 11, 9146. [Google Scholar] [CrossRef]
- Zhao, S.; Ren, X.; Zheng, Q.; Lu, K.; Fu, C.; Yang, Y. Transient dynamic balancing of the rotor system with uncertainty. Mech. Syst. Signal Process. 2022, 171, 108894. [Google Scholar] [CrossRef]
- Jia, Z.; Yang, Y.; Zheng, Q.; Deng, W. Dynamic analysis of Jeffcott rotor under uncertainty based on Chebyshev convex method. Mech. Syst. Signal Process. 2022, 167, 108603. [Google Scholar] [CrossRef]
- Wang, C.; Ma, Y.; Zhang, D.; Hong, J. Interval Analysis on Aero-Engine Rotor System with Misalignment. In Proceedings of the ASME Turbo Expo 2015: Turbine Technical Conference and Exposition, Montreal, QC, Canada, 15–19 June 2015; Volume 7A, pp. 15–19. [Google Scholar]
- Fu, C.; Ren, X.; Yang, Y.; Xia, Y.; Deng, W. An interval precise integration method for transient unbalance response analysis of rotor system with uncertainty. Mech. Syst. Signal Process. 2018, 107, 137–148. [Google Scholar] [CrossRef]
- Fu, C.; Ren, X.; Yang, Y.; Lu, K.; Qin, W. Steady-state response analysis of cracked rotors with uncertain-but-bounded parameters using a polynomial surrogate method. Commun. Nonlinear Sci. Numer. Simul. 2019, 68, 240–256. [Google Scholar] [CrossRef]
- Chen, Y.; Guo, C. An interval fault diagnosis method for rotor cracks. Comput. Electr. Eng. 2020, 87, 106752. [Google Scholar] [CrossRef]
- Ma, Y.; Wang, Y.; Wang, C.; Hong, J. Interval analysis of rotor dynamic response based on Chebyshev polynomials. Chin. J. Aeronaut. 2020, 33, 2342–2356. [Google Scholar] [CrossRef]
- Ma, Y.; Wang, Y.; Wang, C.; Hong, J. Nonlinear interval analysis of rotor response with joints under uncertainties. Chin. J. Aeronaut. 2020, 33, 205–218. [Google Scholar] [CrossRef]
- Lara-Molina, F.A.; Cavalini, A.A., Jr.; Koroishi, E.H.; Steffen, V., Jr. Sensitivity Analysis of Flexible Rotor Subjected to Interval Uncertainties. Lat. Am. J. Solids Struct. 2019, 16. [Google Scholar] [CrossRef]
- Hao, Y.; Chen, M.; Hong, J.; Ma, Y. Interval analysis method of rotordynamics based on Taylor expansion method. J. Aerosp. Power 2014, 29, 571–577. [Google Scholar]
- Barbosa, P.C.P.F.; Lara-Molina, F.A.; da Silva, I.B.; Cavalini, A.A., Jr.; Steffen, V. Uncertain and sensitivity analyses of a composite shaft. Meccanica 2020, 55, 35–48. [Google Scholar] [CrossRef]
- Yang, Y.; Cao, D.; Wang, D.; Jiang, G. Fixed-point rubbing characteristic analysis of a dual-rotor system based on the Lankarani-Nikravesh model. Mech. Mach. Theory 2016, 103, 202–221. [Google Scholar] [CrossRef]
- Xu, Z. Elasticity; Higher Education Press: Beijing, China, 1984. [Google Scholar]
- Zhuo, W. Applied Elastoplastic Mechanics; Science Press: Beijing, China, 2013. [Google Scholar]


| Title | Value | Title | Value |
|---|---|---|---|
| Inner rotor length(m) | 0.706 | Outer rotor length(m) | 0.5011 |
| Inner rotor outer radius(m) | 0.0125 | Outer rotor outer radius(m) | 0.02 |
| Inner rotor inner radius(m) | 0.0075 | Outer rotor inner radius(m) | 0.015 |
| Inner rotor density (kg/m3) | 7850 | Outer rotor density(kg/m3) | 7850 |
| Elastic modulus of the inner rotor (Pa) | 2.1 × 1011 | Elastic modulus of the outer rotor(Pa) | 2.1 × 1011 |
| Poisson ratio of inner rotor | 0.3 | Poisson ratio of outer rotor | 0.3 |
| Inner rotor disc density(kg/m3) | 7928.56 | Outer rotor disc density(kg/m3) | 7928.56 |
| Inner rotor disc thickness(m) | 0.0273 | Outer rotor disc thickness(m) | 0.0273 |
| Outer radius of disc 1(m) | 0.125 | Outer radius of disc 3(m) | 0.125 |
| Inner radius of disc 1(m) | 0.0125 | Inner radius of disc 3(m) | 0.02 |
| Outer radius of disc 2(m) | 0.125 | Outer radius of disc 4(m) | 0.125 |
| Inner radius of disc 2(m) | 0.0125 | Inner radius of disc 4(m) | 0.02 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, N.; Tao, W.; Liu, M.; Nai, Y. Dynamic Response Analysis of a Magnetically Suspended Dual-Rotor System Considering the Uncertainty of Interference-Fit Value. Actuators 2024, 13, 33. https://doi.org/10.3390/act13010033
Wang N, Tao W, Liu M, Nai Y. Dynamic Response Analysis of a Magnetically Suspended Dual-Rotor System Considering the Uncertainty of Interference-Fit Value. Actuators. 2024; 13(1):33. https://doi.org/10.3390/act13010033
Chicago/Turabian StyleWang, Nianxian, Wenqiang Tao, Mingzheng Liu, and Yunfei Nai. 2024. "Dynamic Response Analysis of a Magnetically Suspended Dual-Rotor System Considering the Uncertainty of Interference-Fit Value" Actuators 13, no. 1: 33. https://doi.org/10.3390/act13010033
APA StyleWang, N., Tao, W., Liu, M., & Nai, Y. (2024). Dynamic Response Analysis of a Magnetically Suspended Dual-Rotor System Considering the Uncertainty of Interference-Fit Value. Actuators, 13(1), 33. https://doi.org/10.3390/act13010033





