Design and Experimental Study of Cavity Structure of Pneumatic Soft Actuator
Abstract
1. Introduction
2. Experimental Methods for Soft Actuators
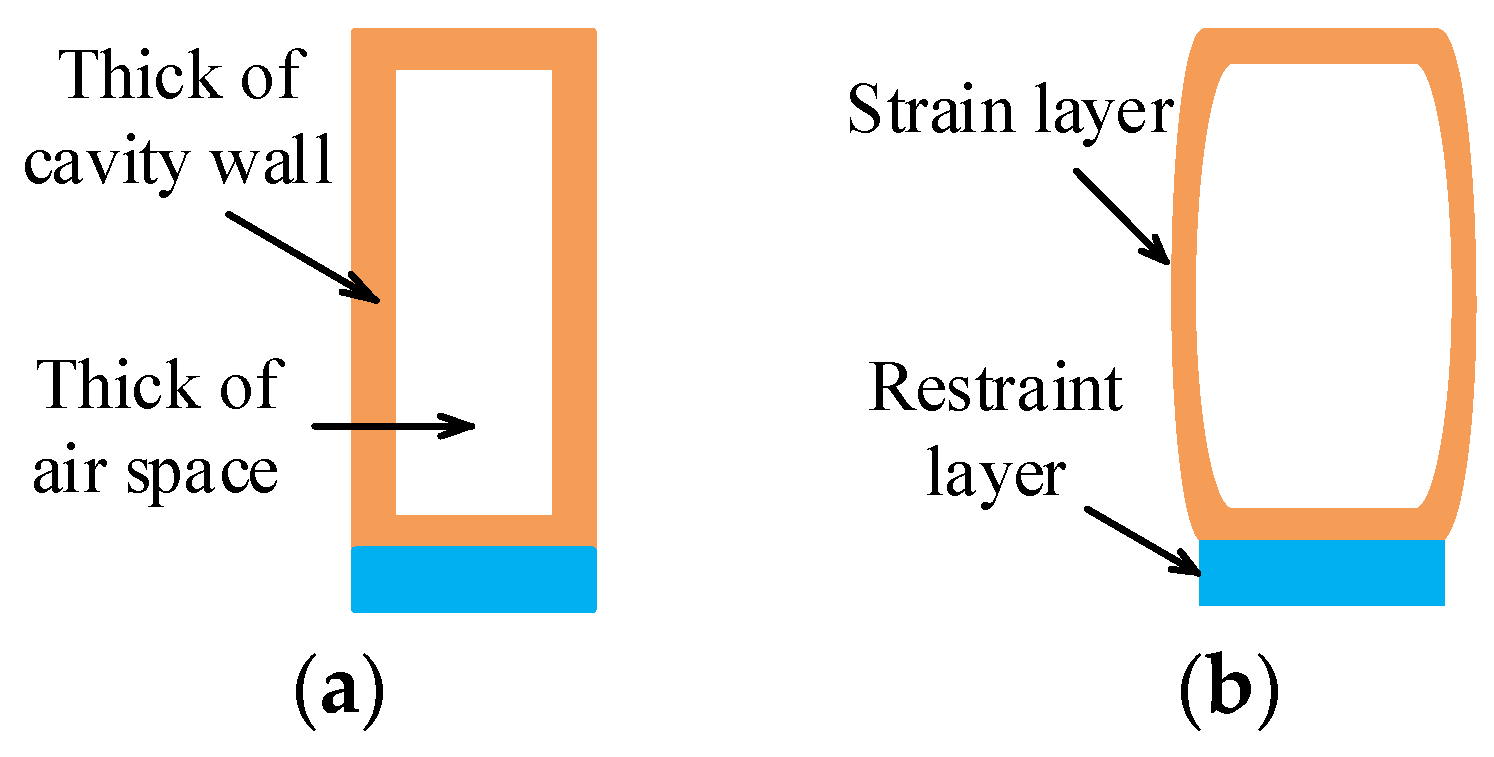
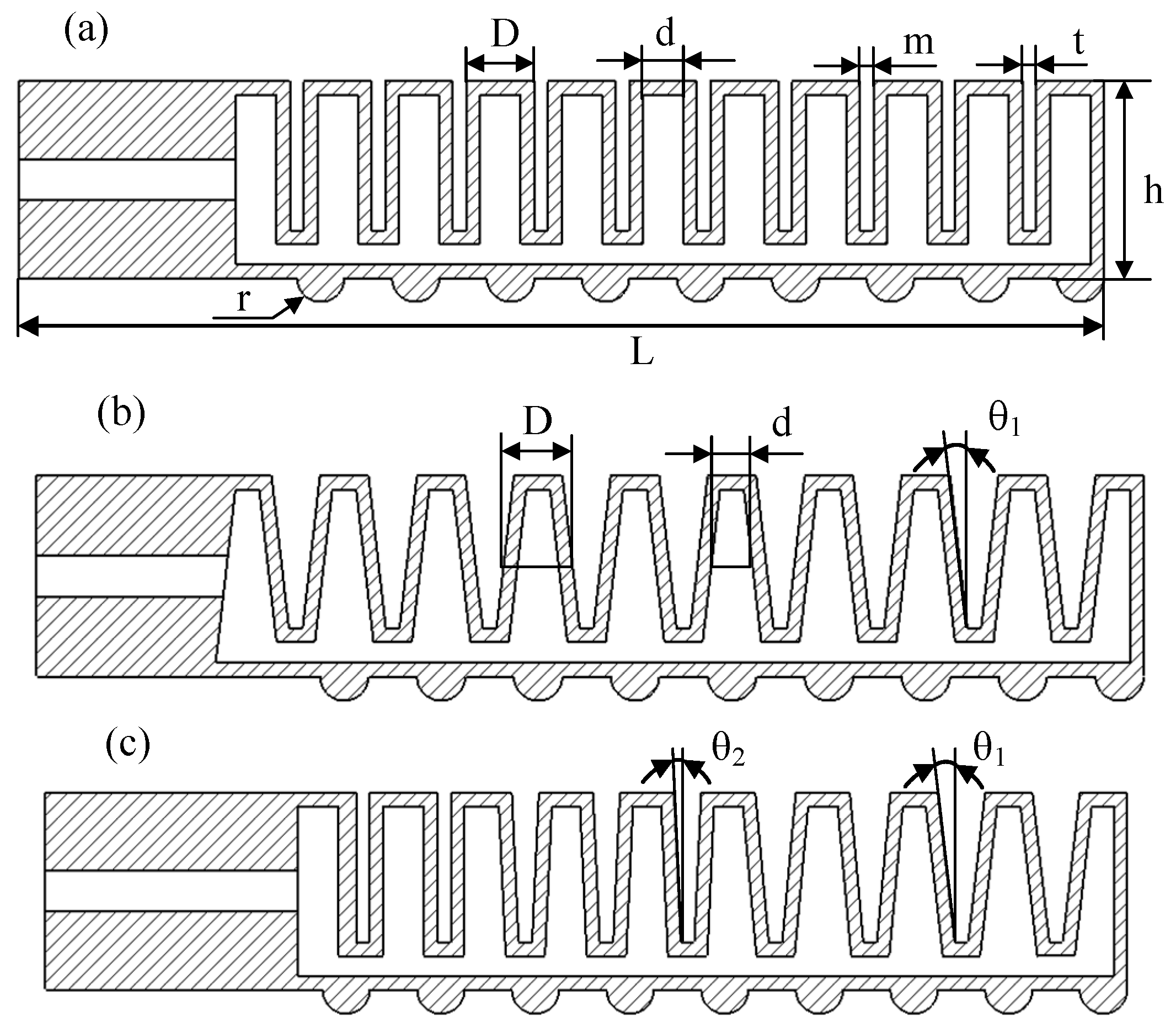
2.1. Structural Design
2.2. Material Design
2.3. Preparation Method
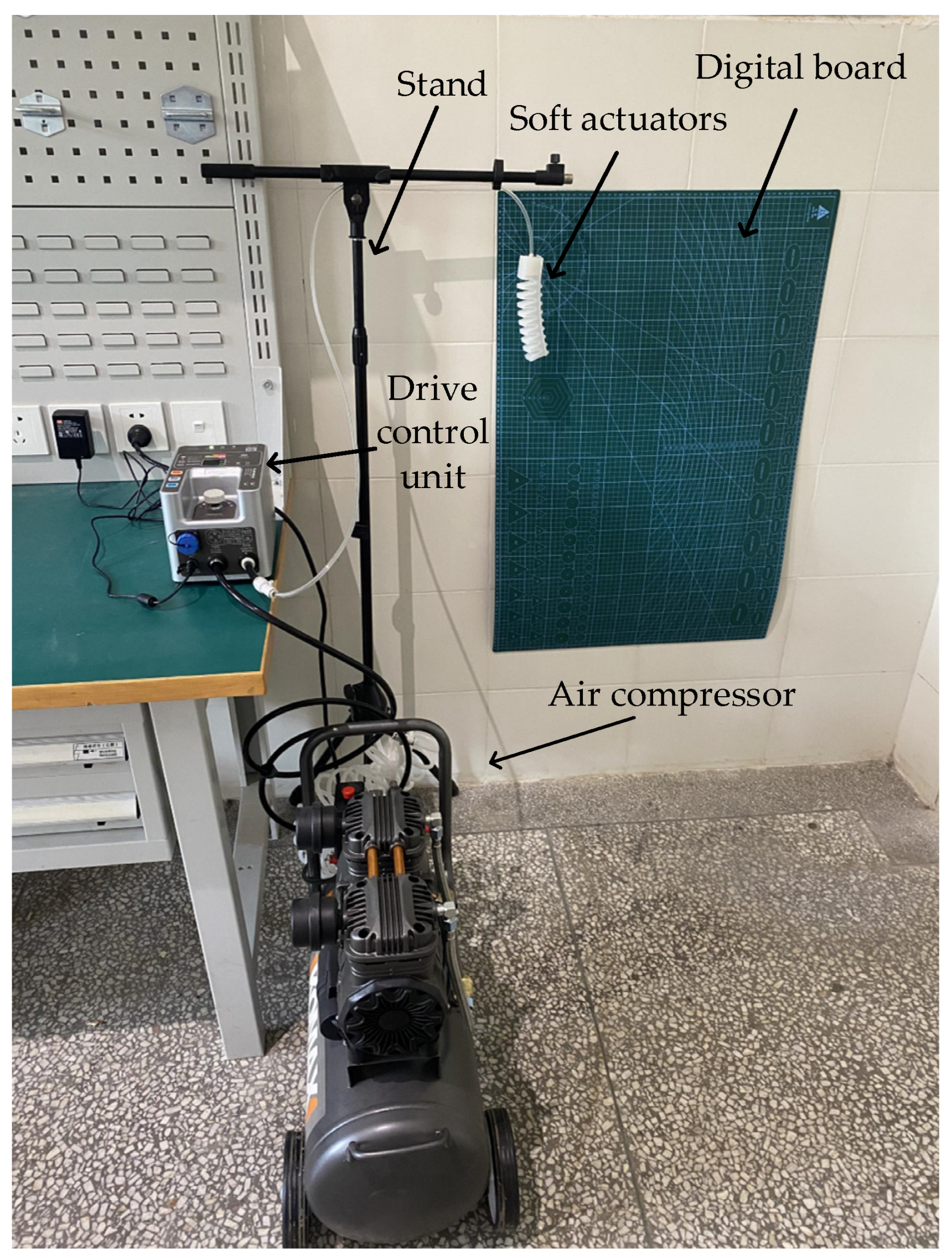
2.4. Experimental Platform
3. Finite Element Simulation Model
3.1. Constitutive Model of Hyperelastic Materials
3.2. Parameter Setting of Simulation Model
3.3. Mesh Sensitivity and Quality Analysis
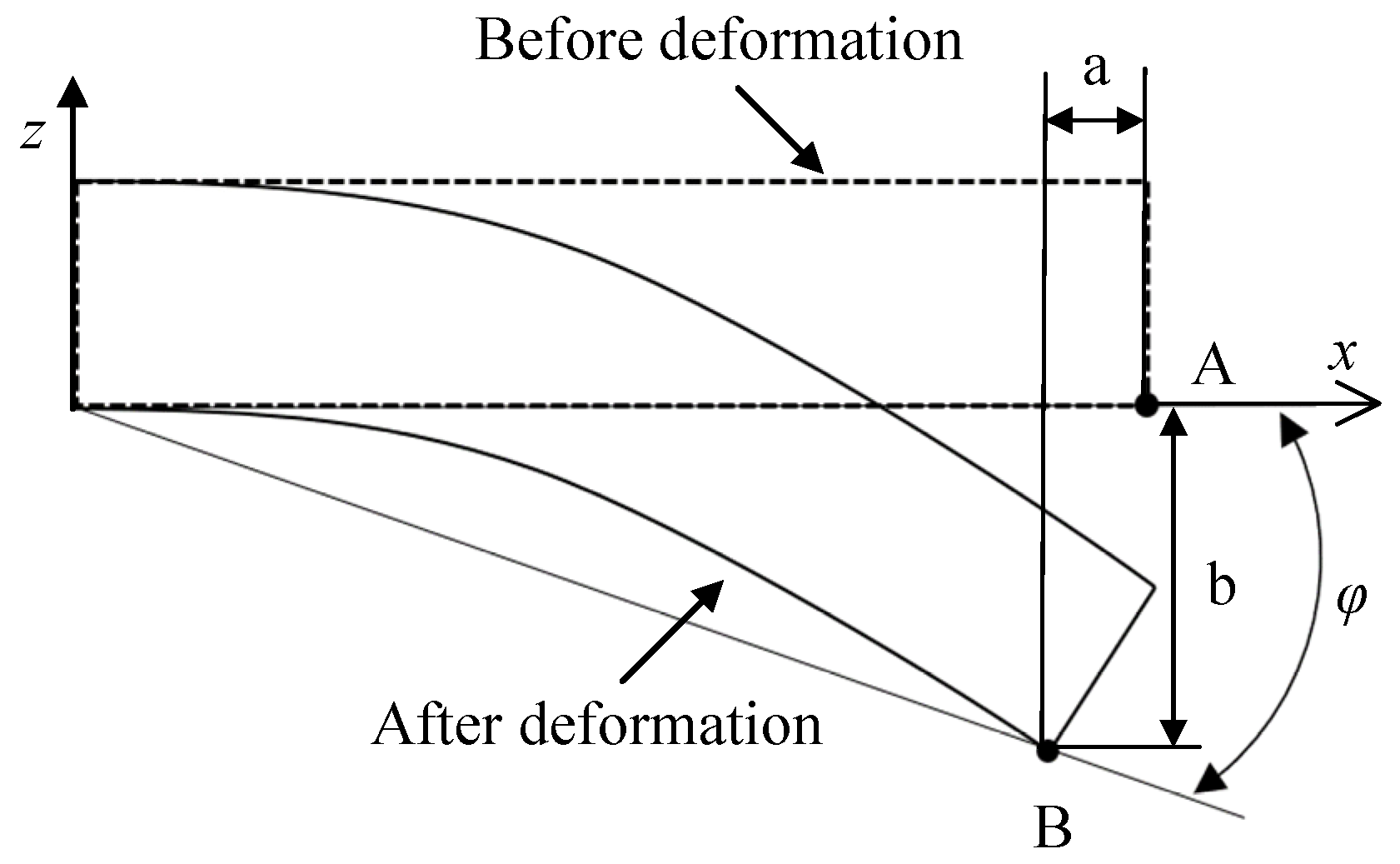
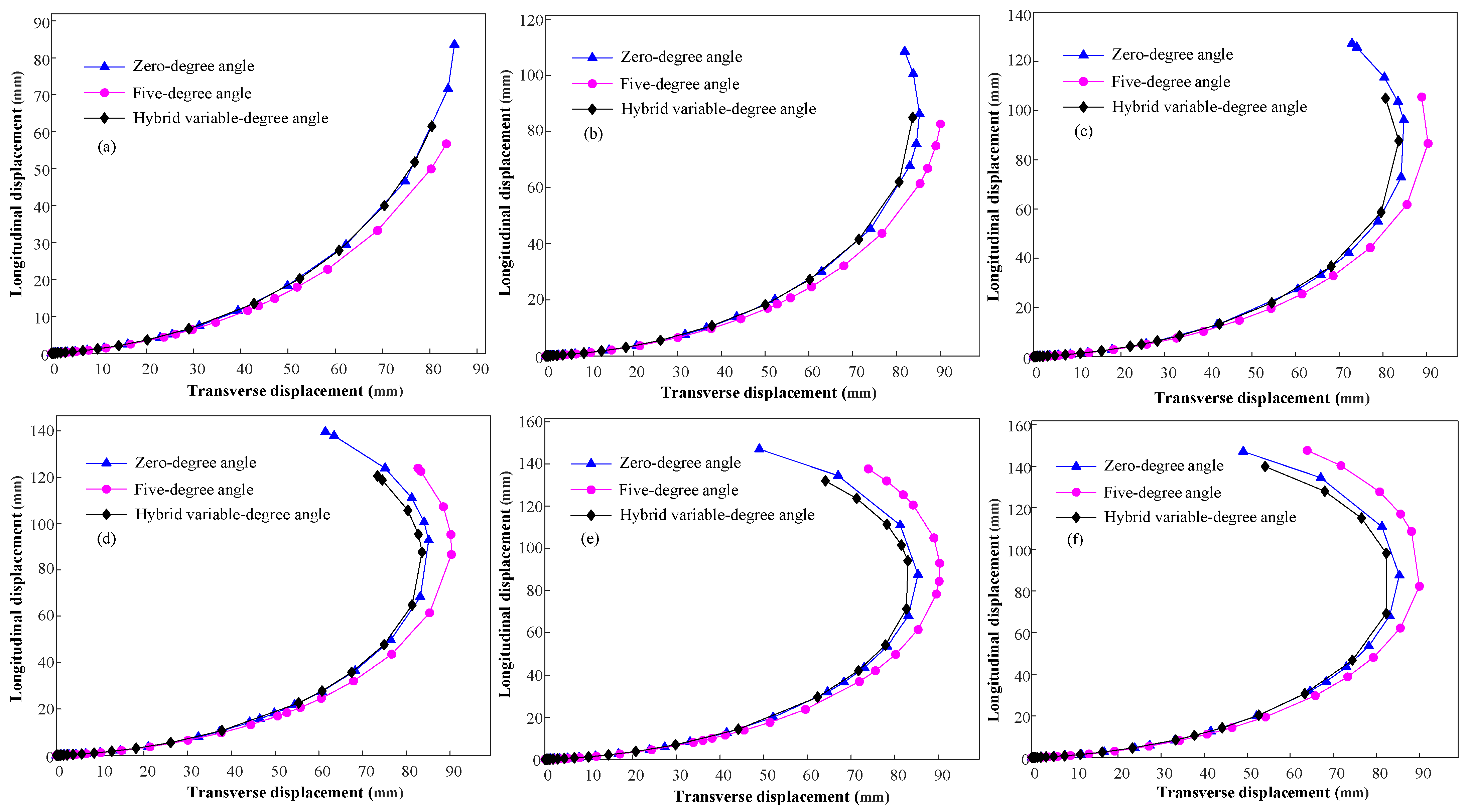
4. Analysis of Experimental and Finite Element Simulation Results
5. Conclusions
- (1)
- Three different soft actuators were fabricated using 3D printing technology and silicone pouring technology, a testing experimental platform of soft actuators was built, and the relationship between the gas pressure and bending angle of the three soft actuators considered in the experiment was investigated. At the same time, ABAQUS software was used to carry out finite element analysis of the soft actuators, and the relationship between the gas pressure and the bending angle of the three kinds of soft actuators under finite element simulation was obtained.
- (2)
- The experimental and simulation results of the three kinds of soft actuators were compared and analyzed, and the mean absolute error between the experimental and simulation results of the zero-degree-angle soft actuator was 0.926, the mean absolute error between the experimental and simulation results of the five-degree-angle soft actuator was 1.472, and the mean absolute error between the experimental and simulation results of the hybrid variable-degree-angle soft actuator was 1.22. The feasibility of the finite element simulation modeling method proposed in this paper was verified through experiments.
- (3)
- The bending performance of three soft actuators with different cavity structures was analyzed, and it was found that the five-degree-angle soft actuator had the largest range of bending angle variation under the same pressure load. Under the same longitudinal displacement, the transverse displacement of the five-degree-angle soft actuator was larger than that of the zero-degree-angle soft actuator and the hybrid variable-degree-angle soft actuator. The bending performance of the soft actuator was significantly improved by changing the cavity tilt angle.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A


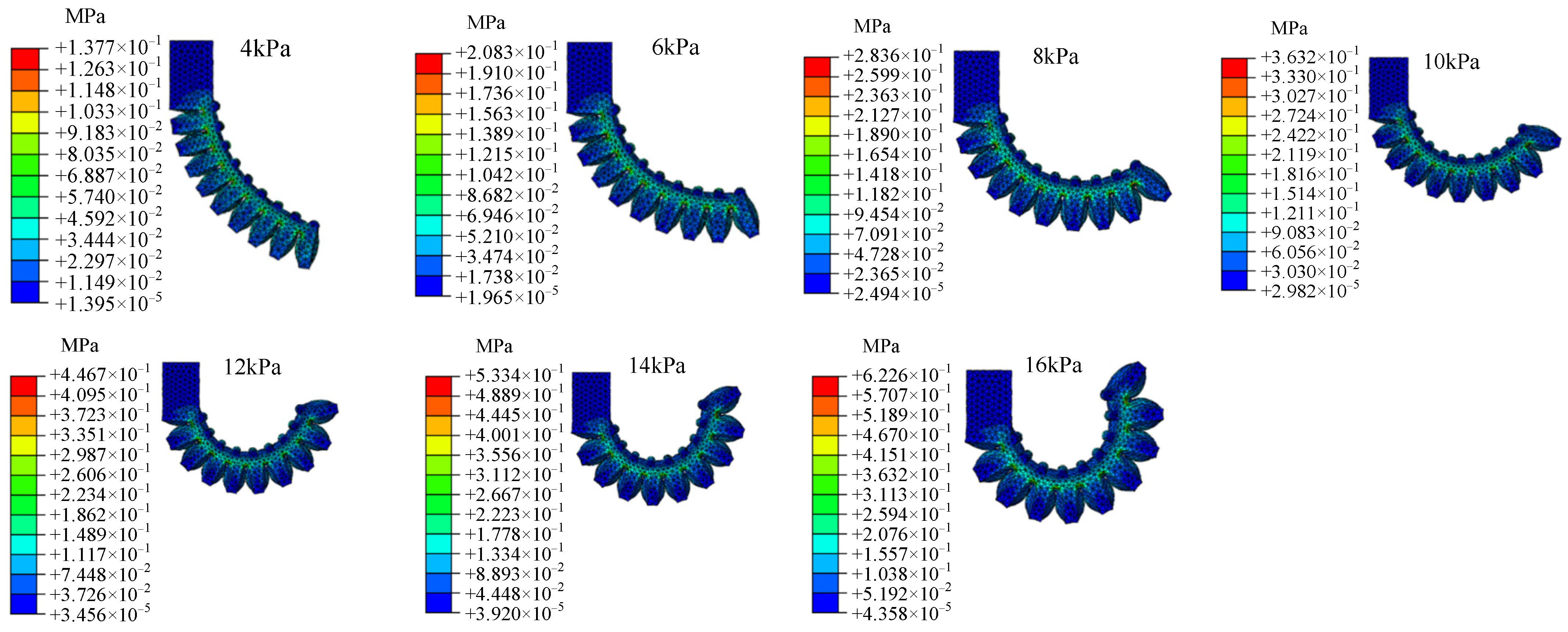
Appendix B
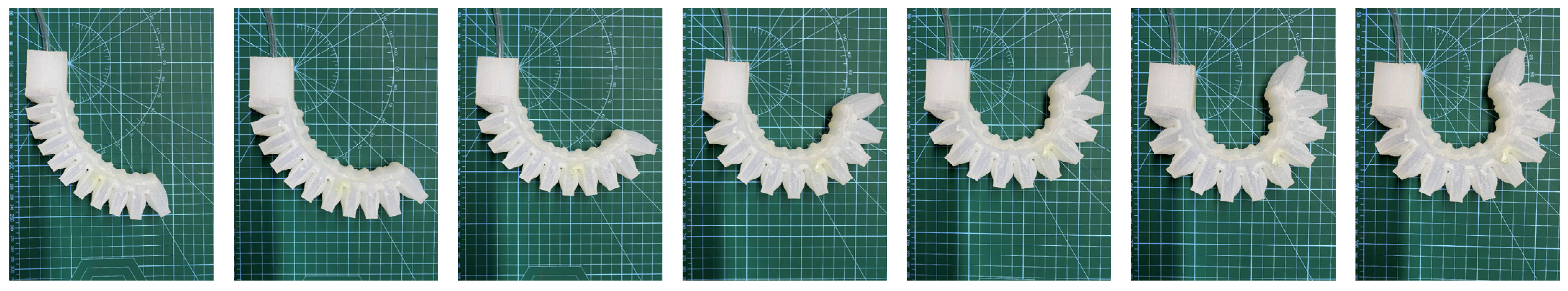
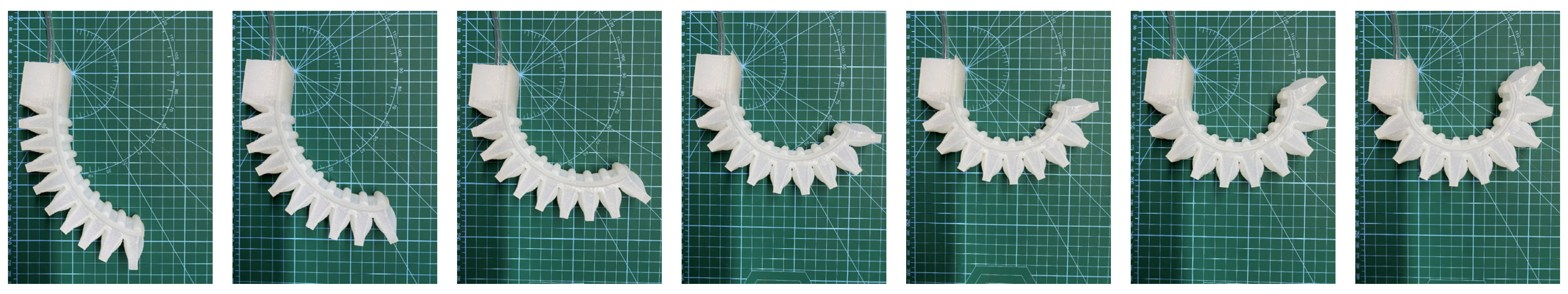
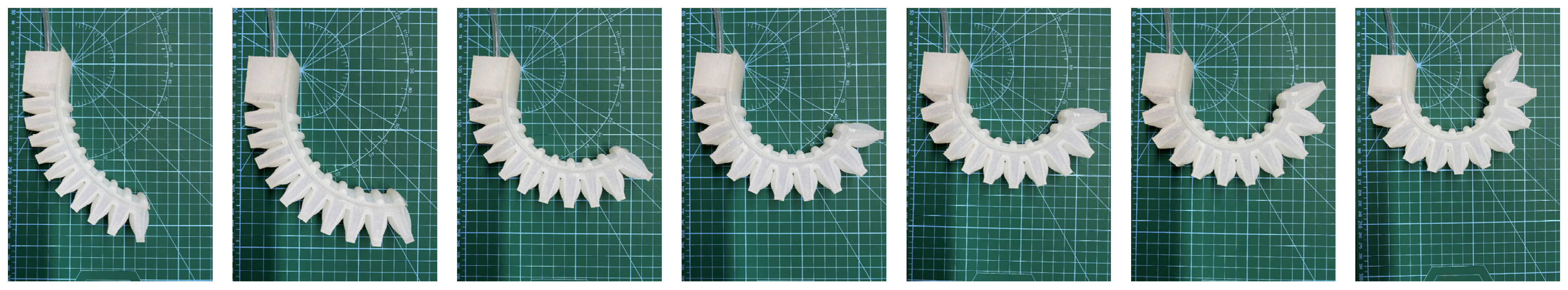
References
- Shen, H. Meet the soft, cuddly robots of the future. Nature 2016, 530, 24–26. [Google Scholar] [CrossRef] [PubMed]
- Rus, D.; Tolley, M.T. Design, fabrication and control of soft robots. Nature 2015, 521, 467–475. [Google Scholar] [CrossRef] [PubMed]
- Liu, K.; Chen, W.; Yang, W.; Jiao, Z.; Yu, Y. Review of the Research Progress in Soft Robots. Appl. Sci. 2023, 13, 120. [Google Scholar] [CrossRef]
- TolleyMichael, T.; ShepherdRobert, F.; GallowayKevin, C.; WoodRobert, J.; WhitesidesGeorge, M. A resilient, untethered soft robot. Soft Robot. 2014, 1, 213–223. [Google Scholar] [CrossRef]
- Chen, Z.; Liang, X.; Wu, T.; Yin, T.H.; Xiang, Y.H.; Qu, S.X. Pneumatically Actuated Soft Robotic Arm for Adaptable Grasping. Acta Mech. Solida Sin. 2018, 31, 608–622. [Google Scholar] [CrossRef]
- Laschi, C.; Cianchetti, M. Soft robotics: New perspectives for robot bodyware and control. Front. Bioeng. Biotechnol. 2014, 2, 3. [Google Scholar] [CrossRef]
- Whitesides, G.M. Soft Robotics. Angew. Chem.-Int. Ed. 2018, 57, 4258–4273. [Google Scholar] [CrossRef]
- Shintake, J.; Cacucciolo, V.; Floreano, D.; Shea, H. Soft Robotic Grippers. Adv. Mater. 2018, 30, 1707035. [Google Scholar] [CrossRef]
- Wallin, T.J.; Pikul, J.; Shepherd, R.F. 3D printing of soft robotic systems. Nat. Rev. Mater. 2018, 3, 84–100. [Google Scholar] [CrossRef]
- Yap, Y.L.; Sing, S.L.; Yeong, W.Y. A review of 3D printing processes and materials for soft robotics. Rapid Prototyp. J. 2020, 26, 1345–1361. [Google Scholar] [CrossRef]
- Filippova, O.V.; Maksimkin, A.V.; Dayyoub, T.; Larionov, D.I.; Telyshev, D.V. Sustainable Elastomers for Actuators: “Green” Synthetic Approaches and Material Properties. Polymers 2023, 15, 2755. [Google Scholar] [CrossRef] [PubMed]
- Gafford, J.; Ding, Y.; Harris, A.; McKenna, T.; Polygerinos, P.; Holland, D.; Moser, A.; Walsh, C. Shape Deposition Manufacturing of a Soft, Atraumatic, Deployable Surgical Grasper. ASME J. Med. Devices 2014, 8, 030927. [Google Scholar] [CrossRef]
- Zhao, H.C.; O’Brien, K.; Li, S.; Shepherd, R.F. Optoelectronically innervated soft prosthetic hand via stretchable optical waveguides. Sci. Robot. 2016, 1, eaai7529. [Google Scholar] [CrossRef]
- Tawk, C.; Spinks, G.M.; Panhuis, M.I.H.; Alici, G. 3D Printable Linear Soft Vacuum Actuators: Their Modeling, Performance Quantification and Application in Soft Robotic Systems. IEEE/ASME Trans. Mechatron. 2019, 24, 2118–2129. [Google Scholar] [CrossRef]
- Liao, W.; Yang, Z.Q. 3D printing programmable liquid crystal elastomer soft pneumatic actuators. Mater. Horiz. 2022, 10, 576–584. [Google Scholar] [CrossRef]
- Li, T.; Zou, Z.; Mao, G.; Yang, X.; Liang, Y.; Li, C.; Qu, S.; Suo, Z.; Yang, W. Agile and resilient insect-scale robot. Soft Robot. 2019, 6, 133–141. [Google Scholar] [CrossRef]
- Trimmer, B.A.; Lin, H.T. Bone-Free: Soft Mechanics for Adaptive Locomotion. Integr. Comp. Biol. 2014, 54, 1122–1135. [Google Scholar] [CrossRef]
- Jin, H.; Dong, E.; Xu, M.; Liu, C.S.; Alici, G.; Jie, Y. Soft and smart modular structures actuated by shape memory alloy (SMA) wires as tentacles of soft robots. Smart Mater. Struct. 2016, 25, 085026. [Google Scholar] [CrossRef]
- Ranzani, T.; Gerboni, G.; Cianchetti, M.; Menciassi, A. A bioinspired soft manipulator for minimally invasive surgery. Bioinspiration Biomim. 2015, 10, 035008. [Google Scholar] [CrossRef]
- Pi, J.; Liu, J.; Zhou, K.; Qian, M. An Octopus-Inspired Bionic Flexible Gripper for Apple Grasping. Agriculture 2021, 11, 1014. [Google Scholar] [CrossRef]
- Li, J.; Luan, Z.B.; Wang, Y.W.; Huang, M.Z.; Yan, J.; Wang, Y.H. Analysis modeling and experiment of bionic winding soft actuator inspired by plant tendrils. Smart Mater. Struct. 2023, 32, 035023. [Google Scholar] [CrossRef]
- Paek, J.W.; Cho, I.; Kim, J. Microrobotic tentacles with spiral bending capability based on shape-engineered elastomeric microtubes. Sci. Rep. 2015, 5, 10768. [Google Scholar] [CrossRef] [PubMed]
- Wall, V.; Deimel, R.; Brock, O. Selective Stiffening of Soft Actuators Based on Jamming. In Proceedings of the 2015 IEEE International Conference on Robotics and Automation (ICRA), Seattle, WA, USA, 26–30 May 2015; pp. 252–257. [Google Scholar] [CrossRef]
- An, S.Q.; Li, W.H.; Li, J.H.; Zou, H.L.; Deng, Z.C. Tuning Stiffness with Granular Chain Structures for Versatile Soft Robots. Soft Robot. 2023, 10, 493–503. [Google Scholar] [CrossRef]
- Elgeneidy, K.; Neumann, G.; Pearson, S.; Jackson, M.; Lohse, N. Contact Detection and Size Estimation Using a Modular Soft Gripper with Embedded Flex Sensors. In Proceedings of the 2018 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Madrid, Spain, 1–5 October 2018; pp. 498–503. [Google Scholar] [CrossRef]
- Yamaguchi, A.; Takemura, K.; Yokota, S.; Edamura, K. A robot hand using electro-conjugate fluid: Grasping experiment with balloon actuators inducing a palm motion of robot hand. Sens. Actuators A Phys. 2012, 174, 181–188. [Google Scholar] [CrossRef]
- Zhou, J.S.; Chen, S.; Wang, Z. A Soft-Robotic Gripper with Enhanced Object Adaptation and Grasping Reliability. IEEE Robot. Autom. Lett. 2017, 2, 2287–2293. [Google Scholar] [CrossRef]
- Mosadegh, B.; Polygerinos, P.; Keplinger, C.; Wennstedt, S.; Shepherd, R.F.; Gupta, U.; Shim, J.; Bertoldi, K.; Walsh, C.J.; Whitesides, G.M. Pneumatic Networks for Soft Robotics that Actuate Rapidly. Adv. Funct. Mater. 2014, 24, 2163–2170. [Google Scholar] [CrossRef]
- Chen, X.Q.; Zhang, X.; Liu, H.W.; Huang, Y.Y. Design and development of a soft robotic manipulator. Int. J. Mech. Mater. Des. 2020, 16, 309–321. [Google Scholar] [CrossRef]
- Wang, Y.X.; Xu, Q.S. Design and Fabrication of a New Dual-Arm Soft Robotic Manipulator. Actuators 2019, 8, 5. [Google Scholar] [CrossRef]
- Li, X.L.; Zheng, T.J.; Sui, D.B.; Lin, N.G.; Zhang, Q.H.; Zhao, J.; Zhu, Y.H. A 3D printed variable cross-section pneumatic soft manipulator with high-precision positioning capability: Design and control implementation. Sens. Actuators A Phys. 2022, 342, 113644. [Google Scholar] [CrossRef]
- Koivikko, A.; Raei, E.S.; Sariola, V.; Mosallaei, M.; Mantysalo, M. Soft Actuators with Screen-Printed Curvature Sensors; IEEE Sensors: Glasgow, UK, 2017; pp. 1–3. [Google Scholar] [CrossRef]
- Liu, S.F.; Wang, F.J.; Liu, Z.; Zhang, W.; Tian, Y.L.; Zhang, D.W. A Two-Finger Soft-Robotic Gripper with Enveloping and Pinching Grasping Modes. IEEE/ASME Trans. Mechatron. 2021, 26, 146–155. [Google Scholar] [CrossRef]
- Lotfiani, A.; Zhao, H.C.; Shao, Z.F. Torsional Stiffness Improvement of a Soft Pneumatic Finger Using Embedded Skeleton. J. Mech. Robot.-Trans. ASME 2020, 12, 011016. [Google Scholar] [CrossRef]
- Íñiguez-Macedo, S.; Lostado-Lorza, R.; Escribano-García, R.; Martínez-Calvo, M.Á. Finite Element Model Updating Combined with Multi-Response Optimization for Hyper-Elastic Materials Characterization. Materials 2019, 12, 1019. [Google Scholar] [CrossRef] [PubMed]
- Xu, Q.P.; Liu, J.Y. Modeling and Simulation of Pneumatic Soft Actuator with Multiple Chambers. J. Shanghai Jiaotong Univ. 2020, 54, 551–561. Available online: https://xuebao.sjtu.edu.cn/CN/Y2020/V54/I6/551 (accessed on 20 July 2023).




| Model | Hardness/A | Tensile Strength /MPa | Tear Strength KN/m | Elongation /% | Density Kg/m3 |
|---|---|---|---|---|---|
| E615 | 15 ± 2 | 4.2 | 12 ± 2 | 470 | 1.05 × 10−9 |
| E620 | 20 ± 2 | 4.7 | 20 ± 2 | 520 | 1.05 × 10−9 |
| E626 | 25 ± 2 | 5.2 | 20 ± 2 | 470 | 1.07 × 10−9 |
| Size [mm] | Elements | Average Aspect Ratio | Nodes | Time [s] | MAE [°] |
|---|---|---|---|---|---|
| (Linear/Quadratic) | (Linear/Quadratic) | (Linear/Quadratic) | |||
| 2 | 88,542 | 1.49 | 88,542/146,475 | 326/2080 | 14.04/2 |
| 3 | 21,488 | 1.58 | 7055/44,348 | 76/540 | 23.56/3.26 |
| 4 | 14,953 | 1.65 | 5430/28,077 | 48/350 | 25.18/2.8 |
| 5 | 8234 | 2.06 | 2150/15,515 | 54/158 | 29.1/0.02 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, Y.; Fu, T. Design and Experimental Study of Cavity Structure of Pneumatic Soft Actuator. Actuators 2023, 12, 314. https://doi.org/10.3390/act12080314
Yu Y, Fu T. Design and Experimental Study of Cavity Structure of Pneumatic Soft Actuator. Actuators. 2023; 12(8):314. https://doi.org/10.3390/act12080314
Chicago/Turabian StyleYu, Yang, and Tao Fu. 2023. "Design and Experimental Study of Cavity Structure of Pneumatic Soft Actuator" Actuators 12, no. 8: 314. https://doi.org/10.3390/act12080314
APA StyleYu, Y., & Fu, T. (2023). Design and Experimental Study of Cavity Structure of Pneumatic Soft Actuator. Actuators, 12(8), 314. https://doi.org/10.3390/act12080314





