The Impact Modeling and Experimental Verification of a Launch Vehicle with Crushing-Type Landing Gear
Abstract
:1. Introduction
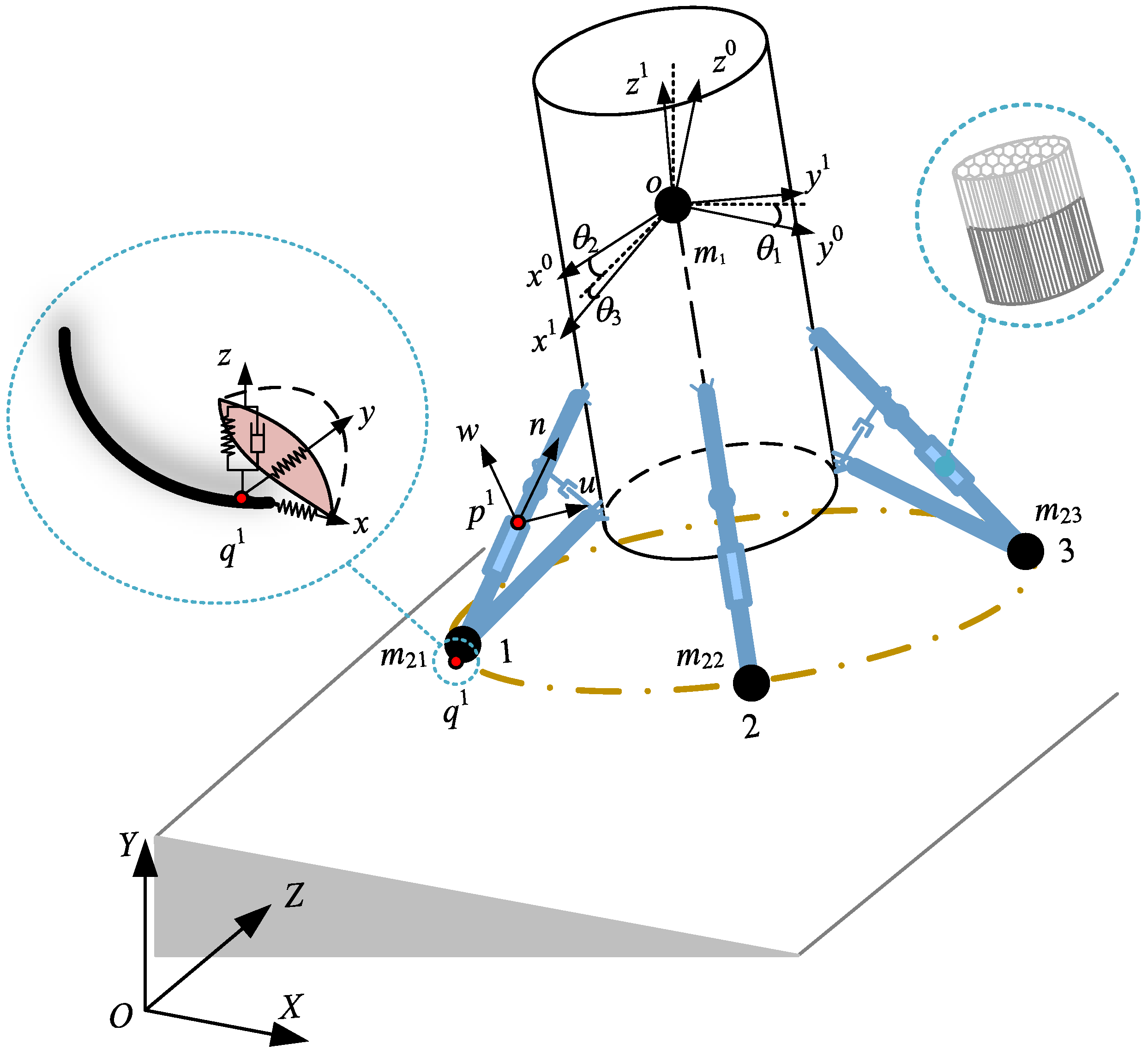
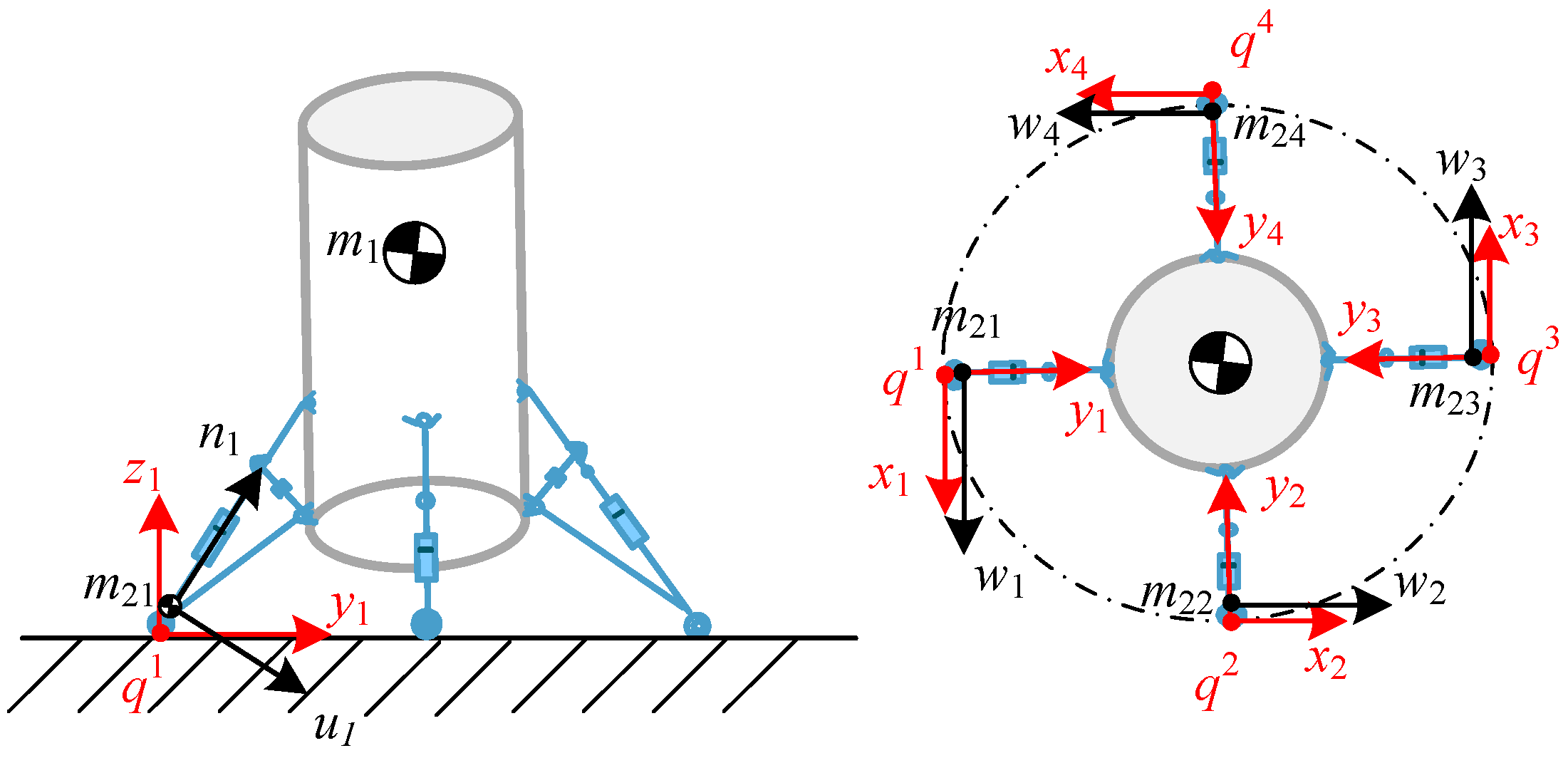
2. Landing Model of a Vehicle Considering the Impact–Buffering Coupling Effect
2.1. Vertical Landing Process of the Vehicle
- Nonlinear system states.
- Rigid-body system states.
2.2. Three-Dimensional Impact Model with Friction
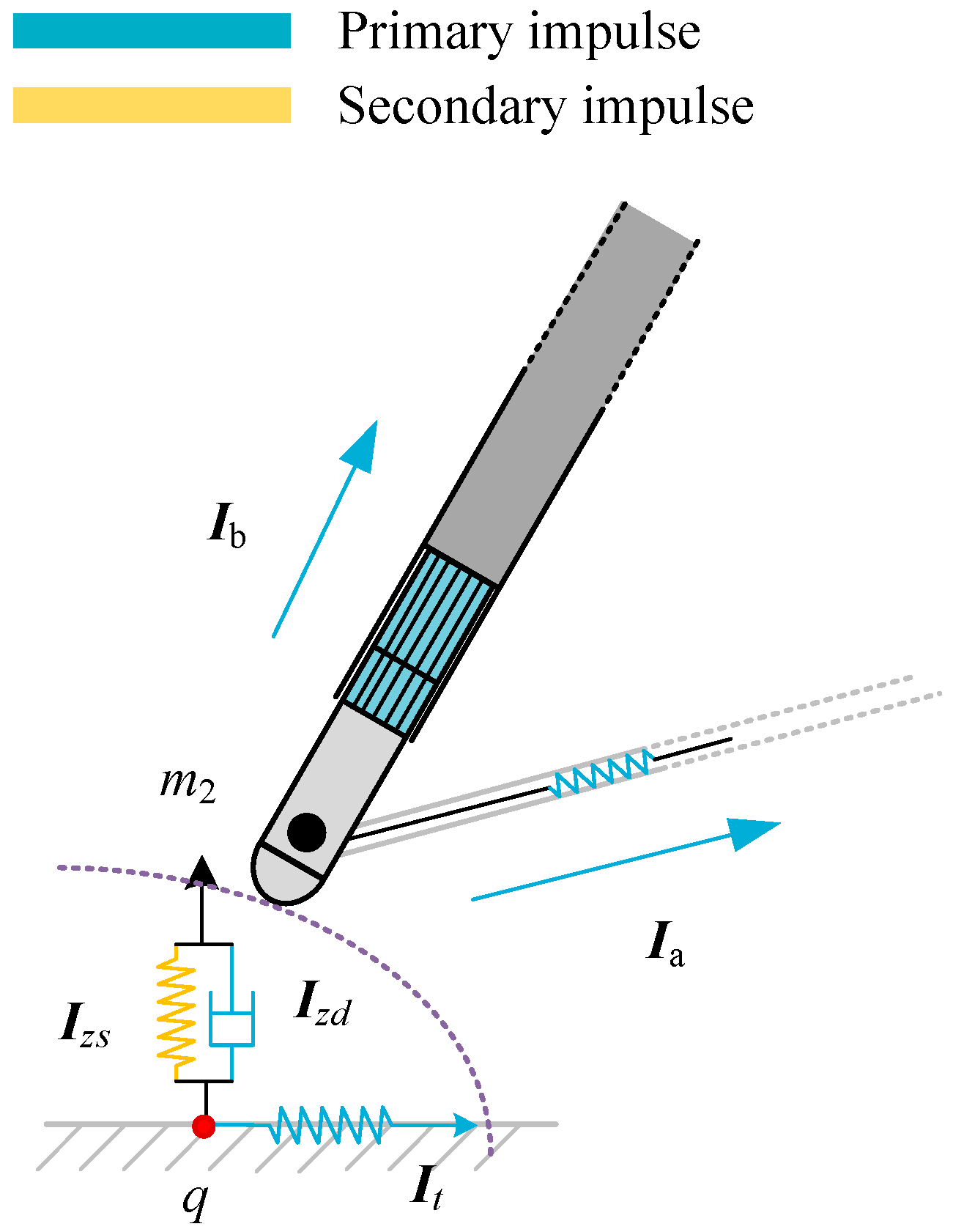
2.2.1. Impulses of the Three-Dimensional Impact
2.2.2. Tangential Motion Patterns
2.2.3. Tangential Velocities
- Stick.
- 2.
- Slip.
2.2.4. Determination of the Tangential Patterns
2.3. Theoretical Model of Multistage Aluminum Honeycomb
2.4. Coupling of the Multi-Impact and Buffering
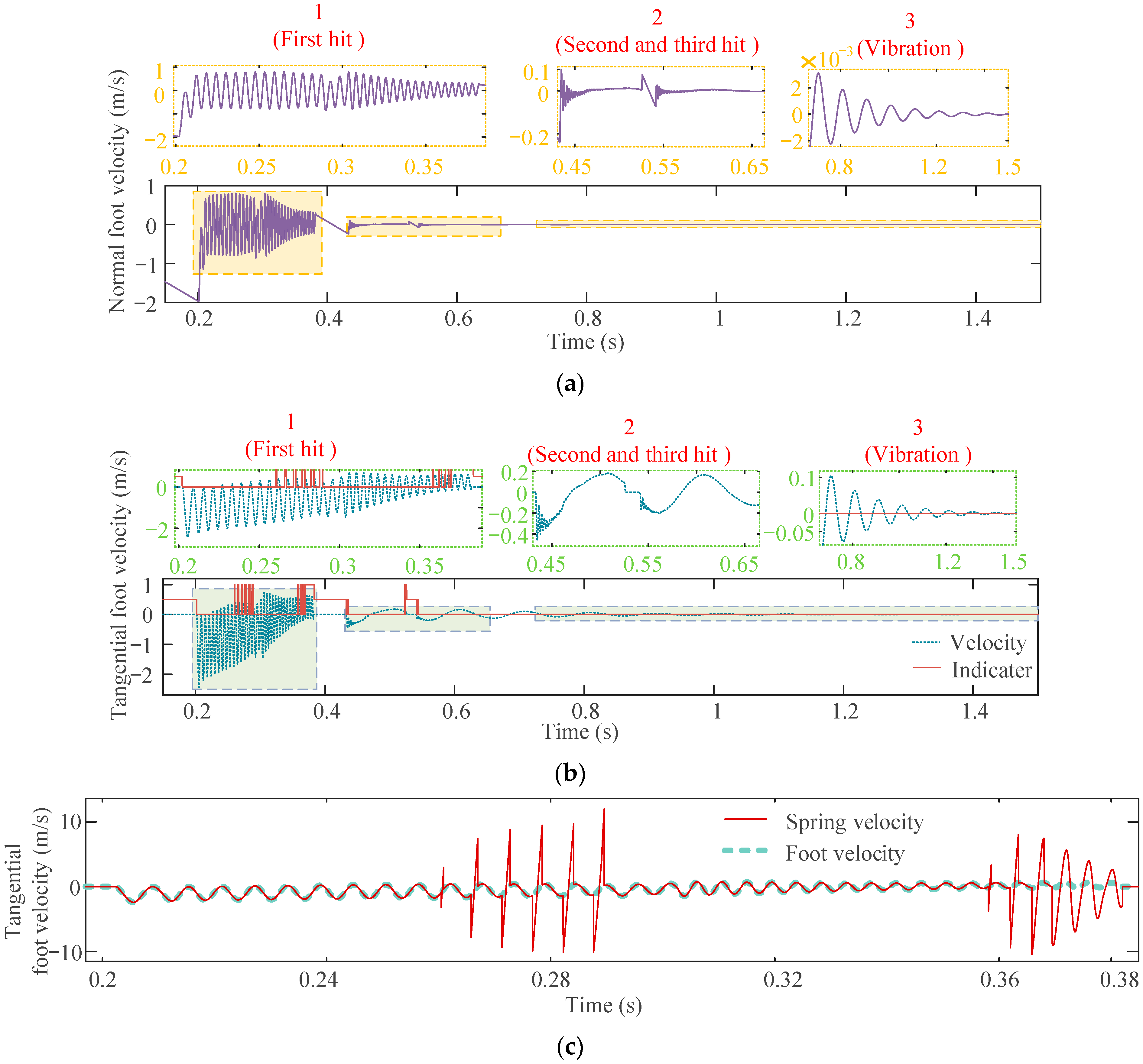
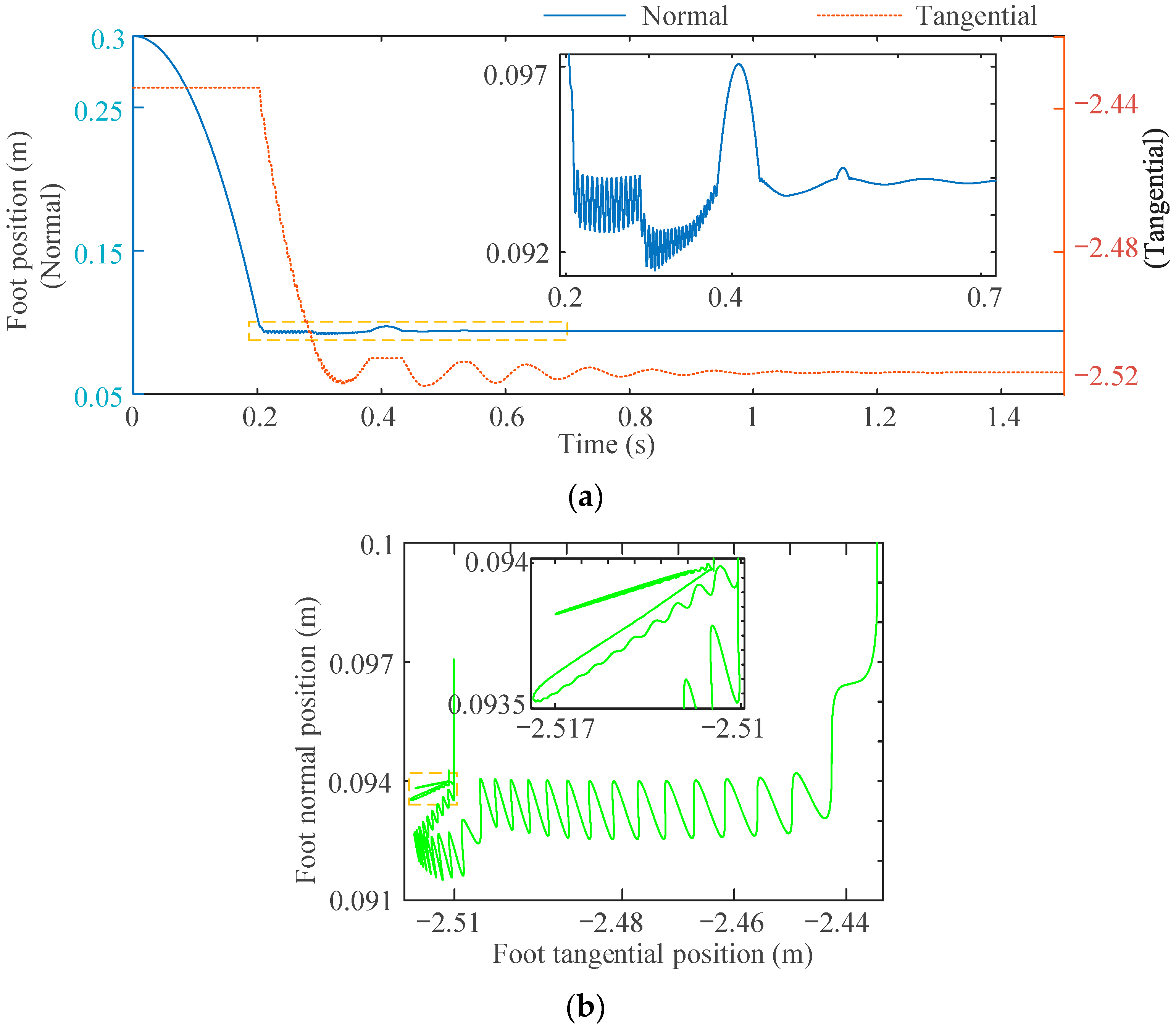
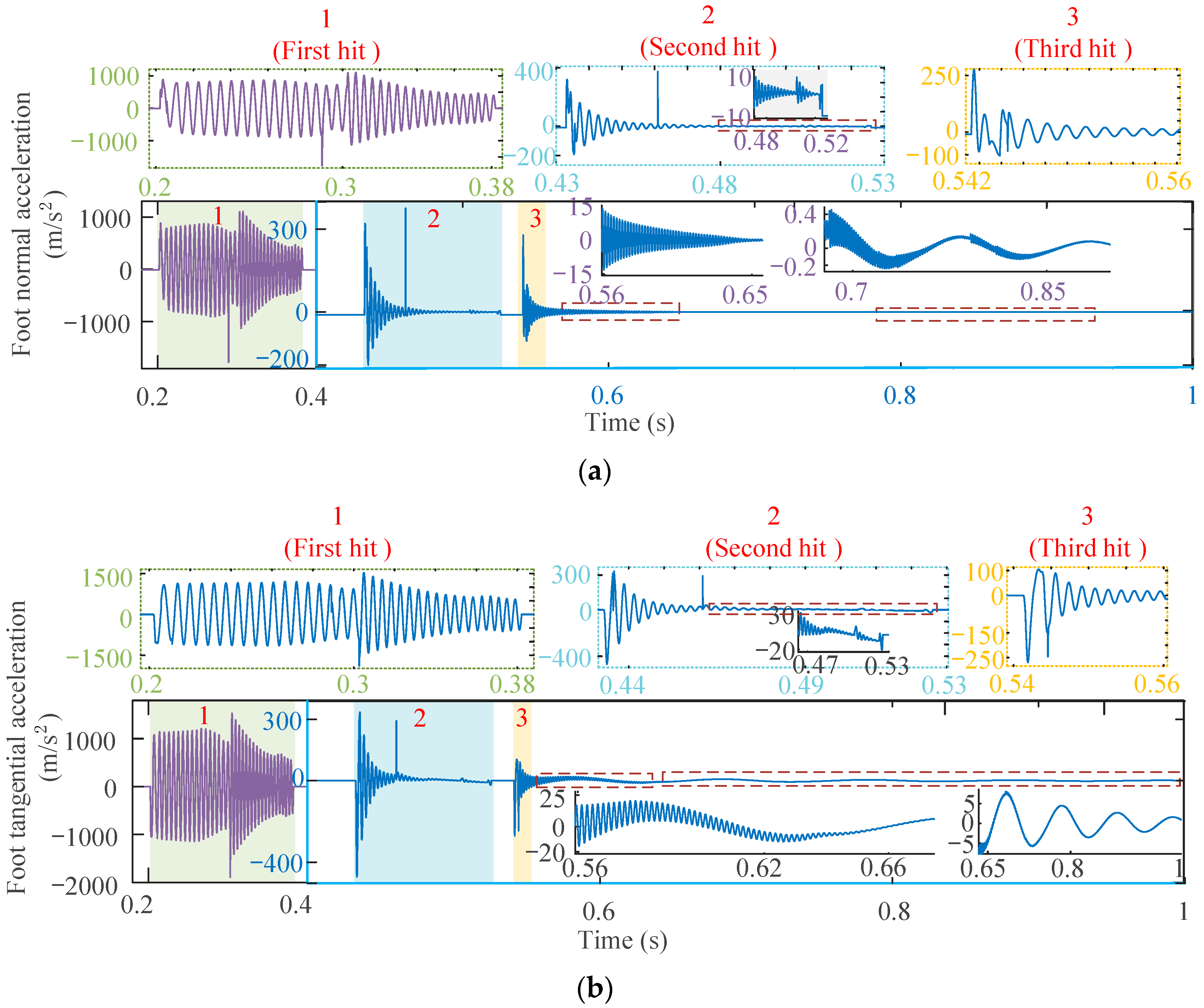
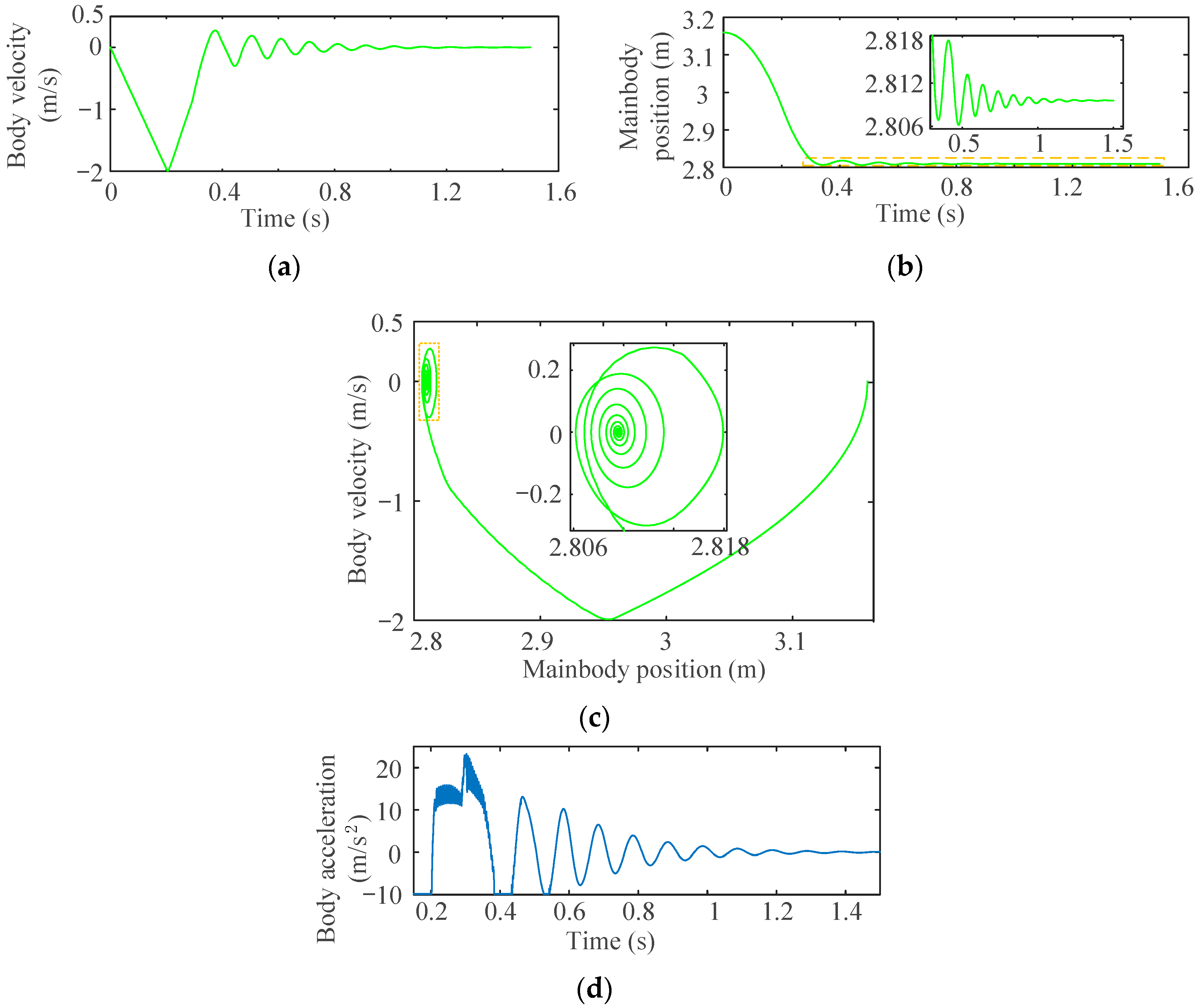
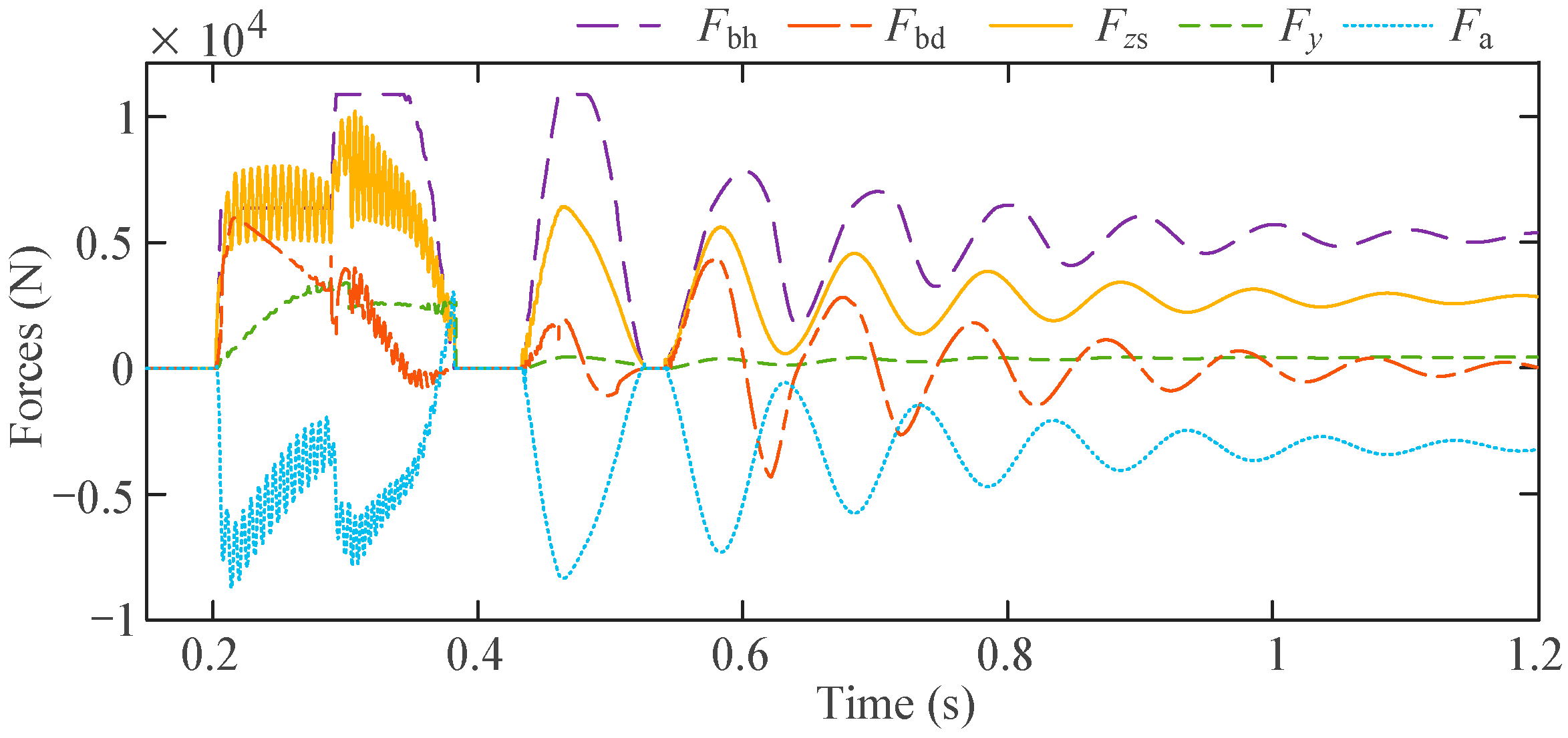
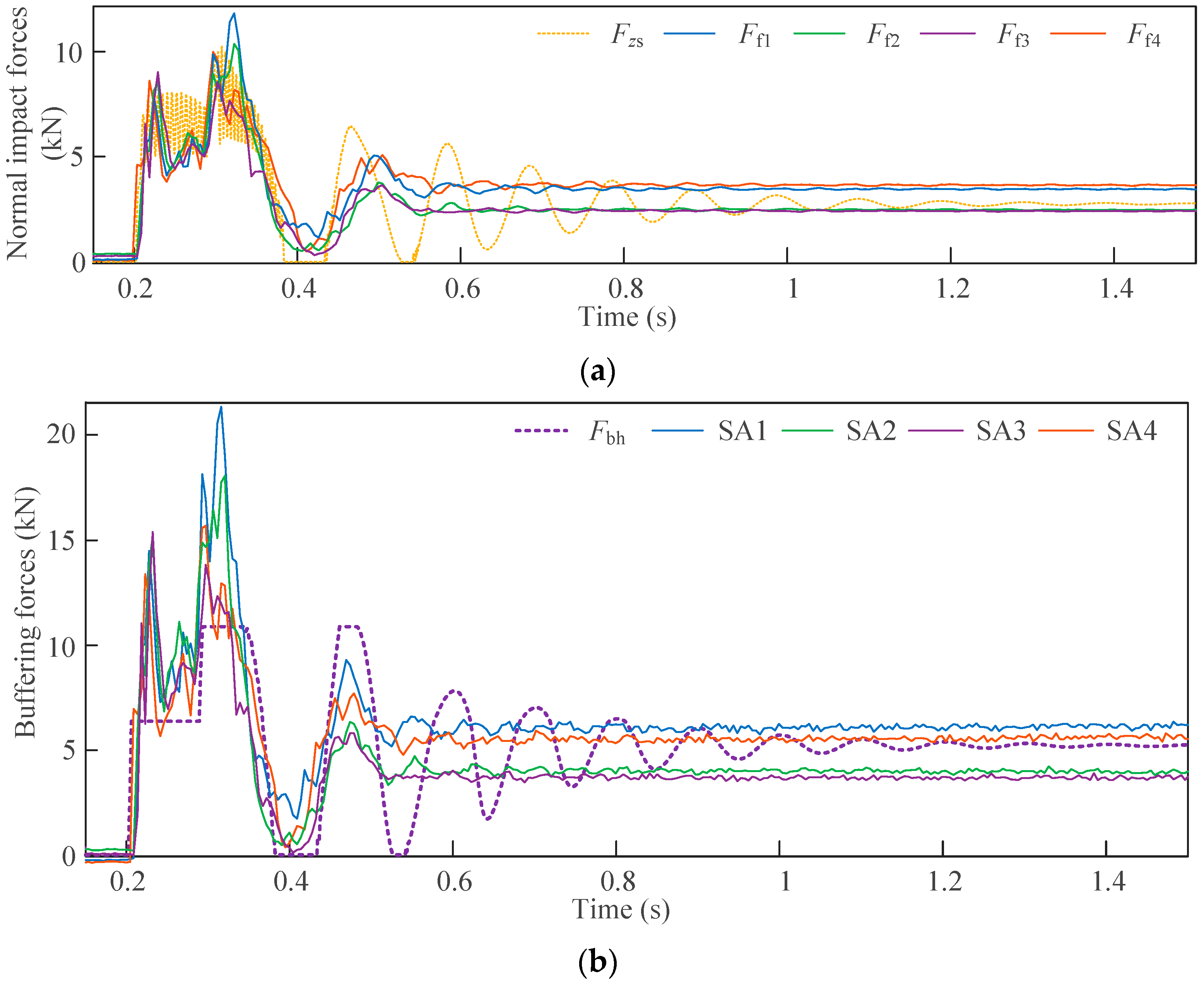
3. Implementation and Results of Simulation and Experiment of the Vertical Landing Process of the Vehicle
3.1. Simulation of the Vertical Landing Process
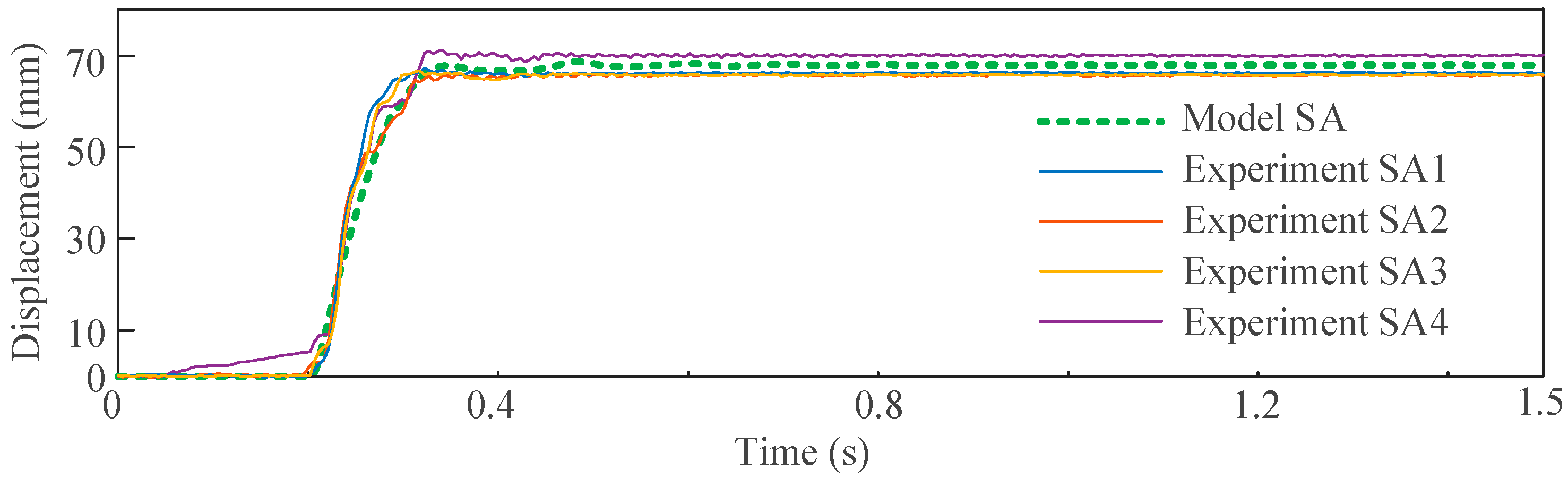
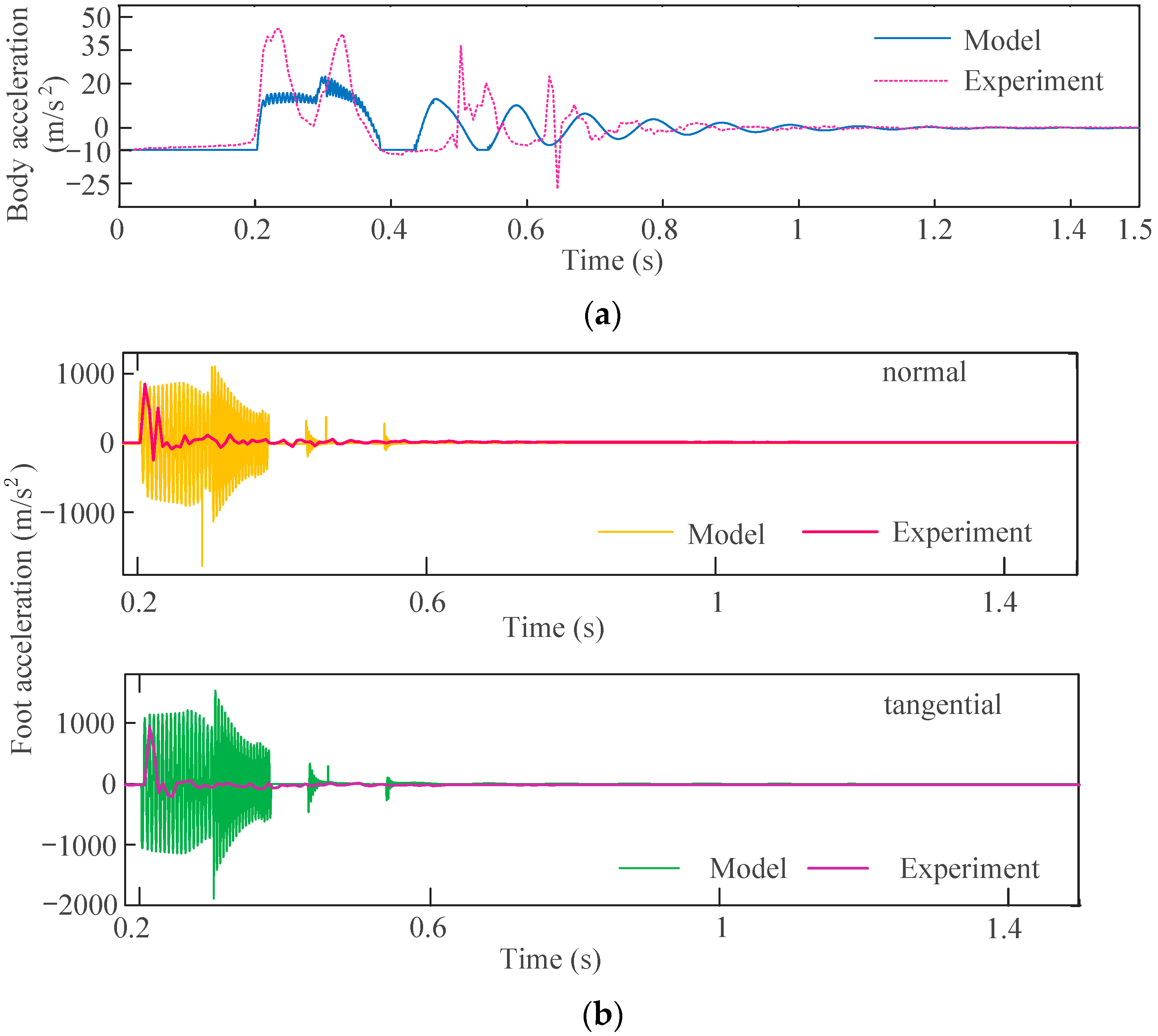
3.2. Implementation of the Vertical Landing Experiment of the Physical Vehicle
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Z, Y, X | The geodetic coordinate system |
| z0, y0, x0 | The main body’s initial companion coordinate system |
| z1, y1, x1 | The main body’s companion coordinate system |
| zj, yj, xj | The coordinate system of the impact between the foot i and the ground |
| z, y, x | The displacement of the z, y, x visual spring of the foot–ground impact or the coordinate of the foot–ground impact (mm) |
| n, w, u | The coordinate of the main pillar |
| θk | k ∈ [1, 3], respectively, represents the rotation angle of each coordinate axis (°) |
| m1 | The mass of the main body (kg) |
| m2i | The mass of the foot i (kg) |
| qi | The impact point between the foot i and the ground |
| pi | The origin of the coordinate of the main pillar i |
| Ebi, Fbi, Ibi | The buffer energy, force, and impulse of leg i, respectively (J, N, N·s) |
| Ezsi, Fzsi, Izsi | The energy, force, and impulse of the normal spring of the foot–ground impact (J, N, N·s) |
| Etan | The elastic energy of the tangential springs (J) |
| vzi | The normal velocity of the foot i (m/s) |
| vbi | The buffering velocity of leg i (m/s) |
| Vtan | The tangential velocity of the touchdown foot (m/s) |
| Vs | The slipping velocity of the touchdown foot (m/s) |
| Zfi | The normal displacement of the foot i (m) |
| Fli | The transverse force exerted on the main body by the touchdown foot i (N) |
| , | Indicator of the x and y virtual spring states; 1 means the spring is compressed. Otherwise, it will be set as −1 |
| Izd | The normal damping impulse of the foot–ground impact (N·s) |
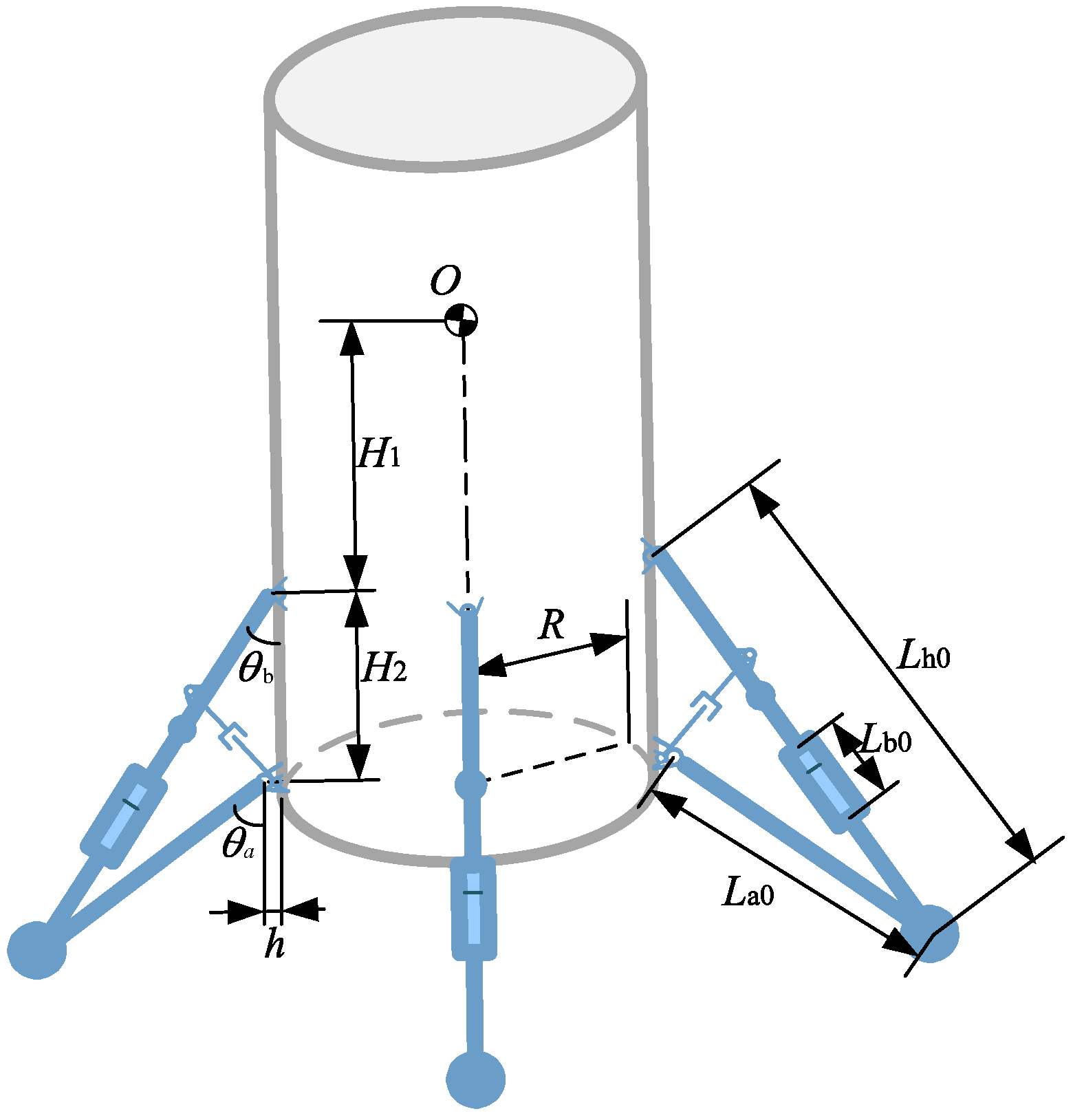
| θbi | The angle between main pillar i axis and main body axis (°) |
| θai | The angle between auxiliary pillar i axis and main body axis (°) |
| h | The relative lateral distance of the auxiliary strut (m) |
| H1 | The longitudinal distance from the center of mass of the main body to the main pillar (m) |
| H2 | The longitudinal distance from main strut to auxiliary pillar (m) |
| R | The radius of the body (m) |
| xb | The stroke of the buffer |
| kb1, kb2, kb3, kb4 | The stiffness coefficients of elastic and plastic section of the two-stage aluminum honeycomb |
| Lh0 | The initial length of the main pillar (m) |
| Lb0 | The maximum stroke of the buffer device (m) |
| La0 | The initial length of the auxiliary pillar (m) |
| xL1 | The elastic section length of the primary aluminum honeycomb (mm) |
| xL2 | The length of primary aluminum honeycomb (mm) |
| xL3 | The length from the initial buffer point to the elastic section of the secondary aluminum honeycomb (mm) |
| xL4 | The length of two stages of aluminum honeycomb (mm) |
| k | The coefficient of stiffness of the normal spring |
| ktan | The coefficient of stiffness of the tangential spring |
| μ | The coefficient of friction |
| γ | 1 minus the coefficient of restitution |
| χ | The coefficient of damping of foot–ground impact |
| Db | The coefficient of damping of structure of the landing gear |
| Hheight | Free-falling height (m) |
References
- Zhou, H.; Wang, X.; Wang, Y.; Zhan, J.; Zhang, J. Research on a coupler with arc surface contact and its effect on a train collision. J. Rail Rapid Transit 2023, 237, 479–489. [Google Scholar] [CrossRef]
- Zhou, H.; Wang, Y.; Wang, X.; Zhan, J.; Zhang, J. Influence of the coupler and buffer device on the train collision safety. J. Rail Rapid Transit 2023, 237, 385–393. [Google Scholar]
- Su, Y.; Hou, X.; Li, L.; Cao, G.; Chen, X.; Jin, T.; Jiang, S.; Li, M. Study on impact energy absorption and adhesion of biomimetic buffer system for space robots. Adv. Space Res. 2020, 65, 1353–1366. [Google Scholar] [CrossRef]
- Yin, K.; Sun, Q.; Gao, F.; Zhou, F. Lunar surface soft-landing analysis of a novel six-legged mobile lander with repetitive landing capacity. J. Mech. Eng. Sci. 2022, 236, 1214–1233. [Google Scholar]
- Hou, W.; Hao, Y.; Wang, C.; Chen, L.; Li, G.; Zhao, B.; Wang, H.; Wei, Q.; Xu, S.; Feng, K.; et al. Theoretical and experimental investigations on high-precision micro-low gravity simulation technology for lunar mobile vehicle. Sensors 2023, 23, 3458. [Google Scholar]
- Lin, Q.; Ren, J. Investigation on the horizontal landing velocity and pitch angle impact on the soft-landing dynamic characteristics. Int. J. Aerosp. Eng. 2022, 16, 3277581. [Google Scholar] [CrossRef]
- Yin, K.; Qi, C.; Gao, Y.; Sun, Q.; Gao, F. Landing control method of a lightweight four-legged landing and walking robot. Front. Mech. Eng. 2022, 17, 51. [Google Scholar]
- Zhang, Z.Q.; Chen, D.S.; Chen, K.W. Analysis and comparison of three leg models for bionic locust Robot based on landing buffering performance. Technol. Sci. 2016, 59, 1413–1427. [Google Scholar]
- Chen, D.S.; Zhang, Z.Q.; Chen, K.W. Dynamic model and performance analysis of landing buffer for bionic locust mechanism. Acta Mech Sin. 2016, 32, 551–565. [Google Scholar]
- Ji, S.; Liang, S. DEM-FEM-MBD coupling analysis of landing process of lunar lander considering landing mode and buffering mechanism. Adv. Space Res. 2021, 68, 1627–1643. [Google Scholar]
- Ding, J.; Liu, X.; Dong, Y.; Wang, C. Stability analysis of mars soft landing under uncertain landing conditions and two landing strategies. Aircr. Eng. Aerosp. Technol. 2022, 94, 1883–1891. [Google Scholar]
- Yu, H.; Tian, B.; Yan, Z.; Gao, H.; Zhang, H.; Wu, H.; Wang, Y.; Shi, Y.; Deng, Z. Watt linkage-based legged deployable landing mechanism for reusable launch vehicle: Principle, prototype design, and experimental validation. Engineering 2023, 20, 120–133. [Google Scholar] [CrossRef]
- Aghili, F. Modeling and analysis of multiple impacts in multibody systems under unilateral and bilateral constrains based on linear projection operators. Multibody Syst. Dyn. 2019, 46, 41–62. [Google Scholar]
- Natsiavas, S.; Passas, P.; Paraskevopoulos, E. A time-stepping method for multibody system with frictional impacts based on a return map and boundary layer theory. Int. J. Non Linear Mech. 2021, 131, 103683. [Google Scholar] [CrossRef]
- Lei, B.; Zhang, M.; Lin, H.; Nie, H. Optimization design containing dimension and buffer parameters of landing legs for reusable landing vehicle. Chin. J. Aeronaut. 2022, 35, 234–249. [Google Scholar]
- Dong, Y.; Ding, J.; Wang, C.; Wang, H.; Liu, X. Soft landing stability analysis of a Mars Lander under uncertain terrain. Chin. J. Aeronaut. 2022, 35, 377–388. [Google Scholar] [CrossRef]
- Arailopoulos, A.; Giagopoulos, D. Nonlinear constitutive force model selection, update and uncertainty quantification for periodically sequential impact applications. Nonlinear Dyn. 2020, 99, 2623–2646. [Google Scholar]
- Poursina, A.-M.; Nikravesh, P.-E. Optimal damping coefficient for a class of continuous contact models. Multibody Syst. Dyn. 2020, 50, 169–188. [Google Scholar]
- Shen, Y.; Kuang, Y.; Wang, W.; Zhao, Y.; Yang, J.; Tian, A. Frictional impact analysis of an elastoplastic multi-link robotic system using a multi-timescale modelling approach. Nonlinear Dyn. 2019, 98, 1999–2018. [Google Scholar]
- Wang, H.; He, T.; Wang, C. A comprehensive performance optimization method for the honeycomb buffer of a legged-type lander. Aircr. Eng. Aerosp. Technol. 2021, 93, 821–831. [Google Scholar]
- Xie, S.; Du, X.; Zhou, H.; Wang, J.; Chen, P. Crashworthiness of Nomex honeycomb-filled anti-climbing energy absorbing devices. Int. J. Crashworthiness 2021, 26, 121–132. [Google Scholar] [CrossRef]
- Zhou, D.; Wang, X.; Zheng, Q.; Fu, T.; Wu, M.; Sun, X. A nonlinear occupant-restraint system model for human injuries caused by vertical impact. Nonlinear Dyn. 2021, 105, 3093–3115. [Google Scholar] [CrossRef]
- Yuan, Q.; Chen, H.; Nie, H.; Zheng, G.; Wang, C.; Hao, L. Soft-landing dynamic analysis of a manned lunar lander em-ploying energy absorption materials of carbon nanotube buckypaper. Materials 2021, 14, 6202. [Google Scholar] [CrossRef]
- Zhou, J.; Jia, S.; Qian, J.; Chen, M.; Chen, J. Improving the buffer energy absorption characteristics of movable lander-numerical and experimental studies. Materials 2020, 13, 3340. [Google Scholar] [CrossRef] [PubMed]
- Zhou, J.; Ma, H.; Jia, S.; Tian, S. Mechanical properties of multilayer combined gradient cellular structure and its application in the WLL. Heliyon 2023, 9, e14825. [Google Scholar] [CrossRef] [PubMed]
- Jia, Y.B. Three-dimensional impact: Energy-based modeling of tangential compliance. Int. J. Robot. Res. 2013, 32, 56–83. [Google Scholar] [CrossRef]
- Jia, Y.B.; Mason, M.-T.; Erdmann, M.-A. Multiple impacts: A state transition diagram approach. Int. J. Robot. Res. 2013, 32, 84–114. [Google Scholar] [CrossRef] [Green Version]








| Stage Category | Length (mm) | Diameter of Section (mm) | Cell Size (mm) | Sheet Thickness (mm) |
|---|---|---|---|---|
| Primary honeycomb | 72 | 98 | 8.66 | 0.07 |
| Secondary honeycomb | 125 | 98 | 5.20 | 0.06 |
| Symbol | Value | Symbol | Value |
|---|---|---|---|
| 58.70 | 57.60 | ||
| 35.11 | 58.23 | ||
| 0.092 | 183.23 | ||
| 0.25 | k | 2 × 106 | |
| 1.55 | 7 × 104 | ||
| 0.60 | 0.50 | ||
| 3.19 | 0.50 | ||
| 0.18 | 2 × 10−4 | ||
| 2.04 | 1.50 | ||
| 0.47 | Hheight | 0.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Yu, H.; Xie, J.; Yan, Z.; Tian, B.; Gao, H. The Impact Modeling and Experimental Verification of a Launch Vehicle with Crushing-Type Landing Gear. Actuators 2023, 12, 307. https://doi.org/10.3390/act12080307
Wang Y, Yu H, Xie J, Yan Z, Tian B, Gao H. The Impact Modeling and Experimental Verification of a Launch Vehicle with Crushing-Type Landing Gear. Actuators. 2023; 12(8):307. https://doi.org/10.3390/act12080307
Chicago/Turabian StyleWang, Yingchao, Haitao Yu, Jianghui Xie, Zhen Yan, Baolin Tian, and Haibo Gao. 2023. "The Impact Modeling and Experimental Verification of a Launch Vehicle with Crushing-Type Landing Gear" Actuators 12, no. 8: 307. https://doi.org/10.3390/act12080307
APA StyleWang, Y., Yu, H., Xie, J., Yan, Z., Tian, B., & Gao, H. (2023). The Impact Modeling and Experimental Verification of a Launch Vehicle with Crushing-Type Landing Gear. Actuators, 12(8), 307. https://doi.org/10.3390/act12080307






