Dynamic Vibration Absorbing Performance of 5-DoF Magnetically Suspended Momentum Wheel Based on Damping Regulation
Abstract
1. Introduction
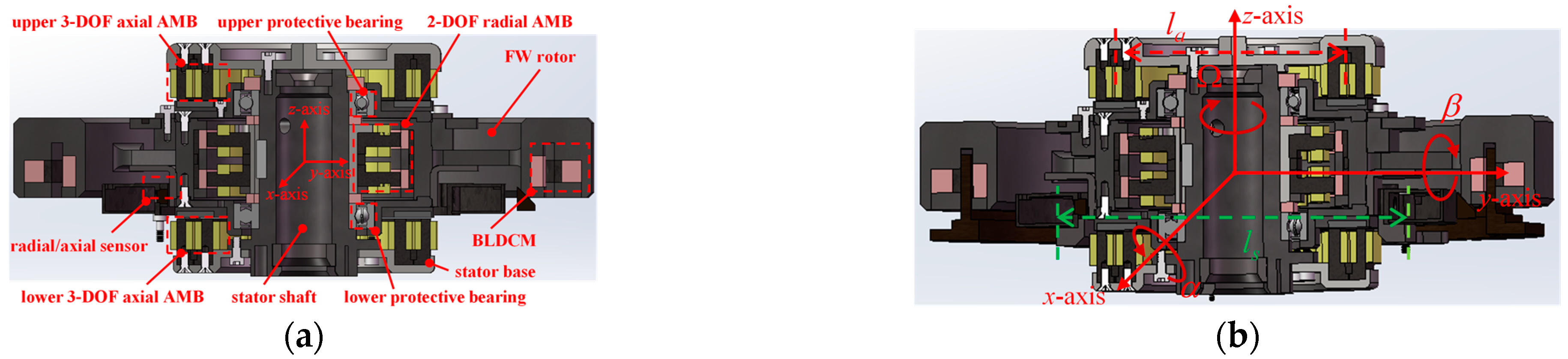
2. Force Modelling of 5-DoF MSMW
2.1. System View of 5-DoF MSMW
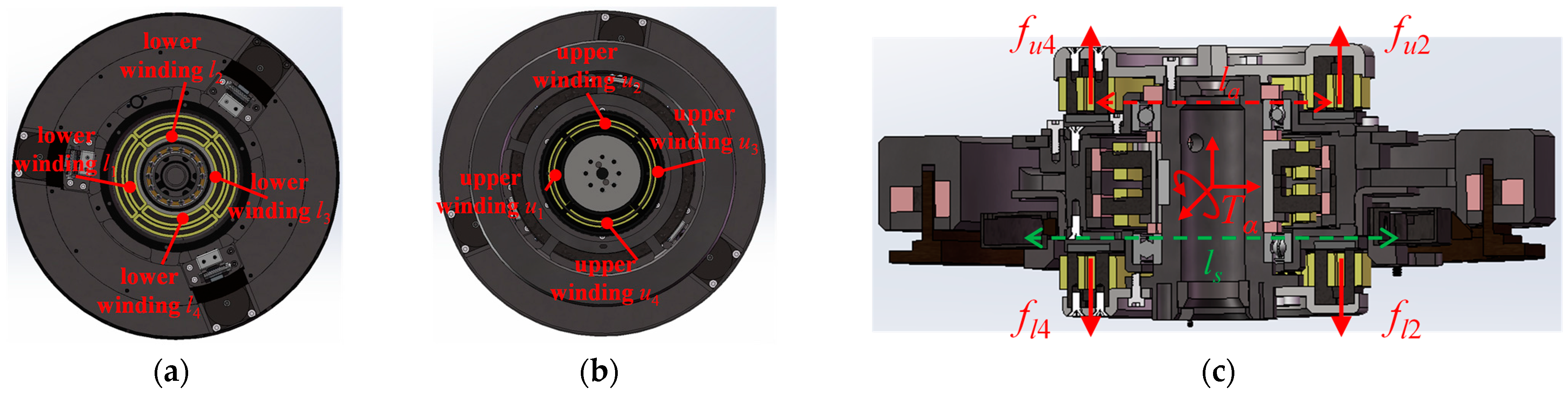
2.2. Displacement and Force Coordinates of 5-DoF MSMW
2.3. Translational Stiffness and Damping Characteristics of 5-DoF MSMW
2.4. Torsional Stiffness and Damping Characteristics of 5-DoF MSMW
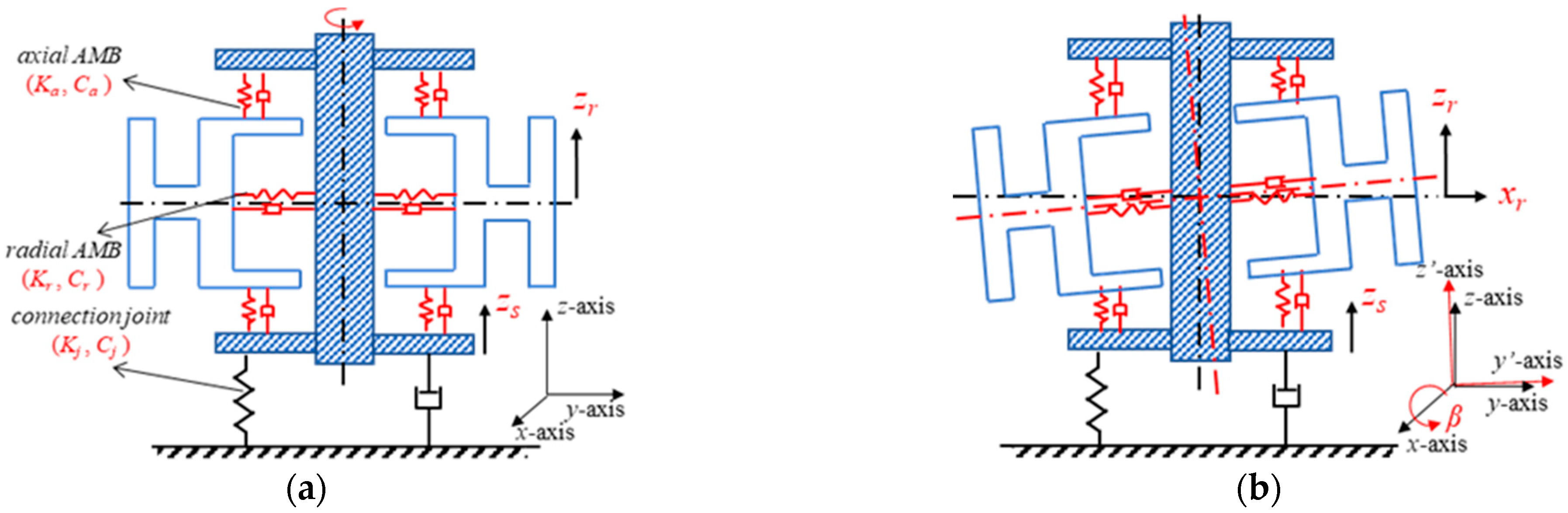
3. Translational Vibration Modelling of 5-DoF MSMW
3.1. Translational Vibration Model of 5-DoF MSMW
3.2. Translational Vibration Model of 5-DoF MSMW with Active Controllable Stiffness and Damping
4. Torsional Vibration Modelling of 5-DoF MSMW
4.1. Torsional Vibration Model of 5-DoF MSMW
4.2. Torsional Vibration Model of 5-DoF MSMW with Active Controllable Stiffness and Damping
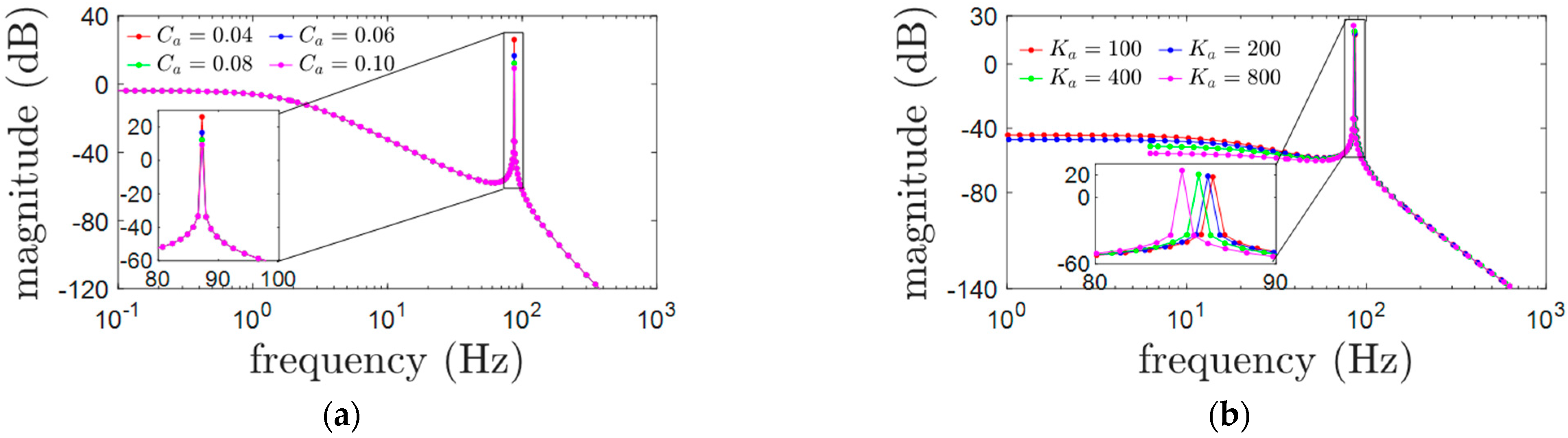
5. Numerical Simulation
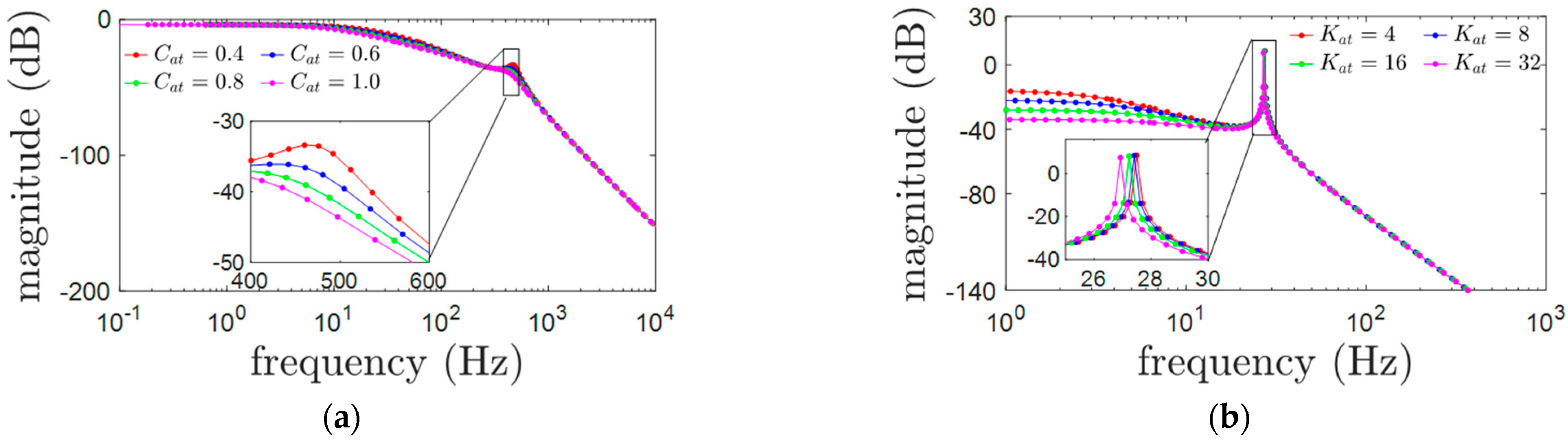
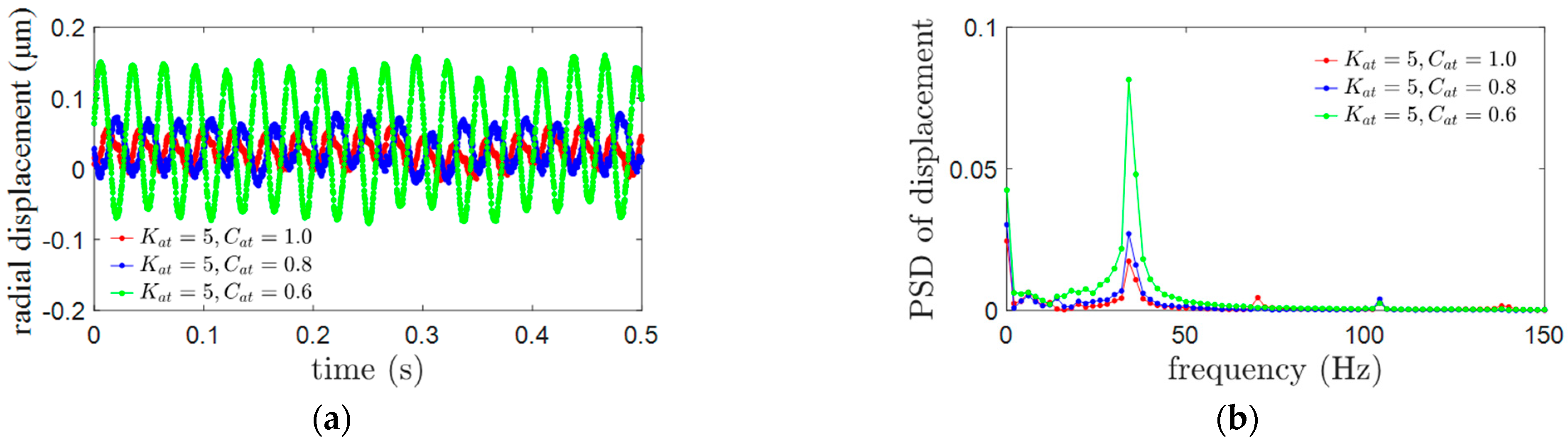
5.1. Translational Vibration of 5-DoF MSMW
5.2. Torsional Vibration of 5-DoF MSMW
6. Experimental Validation
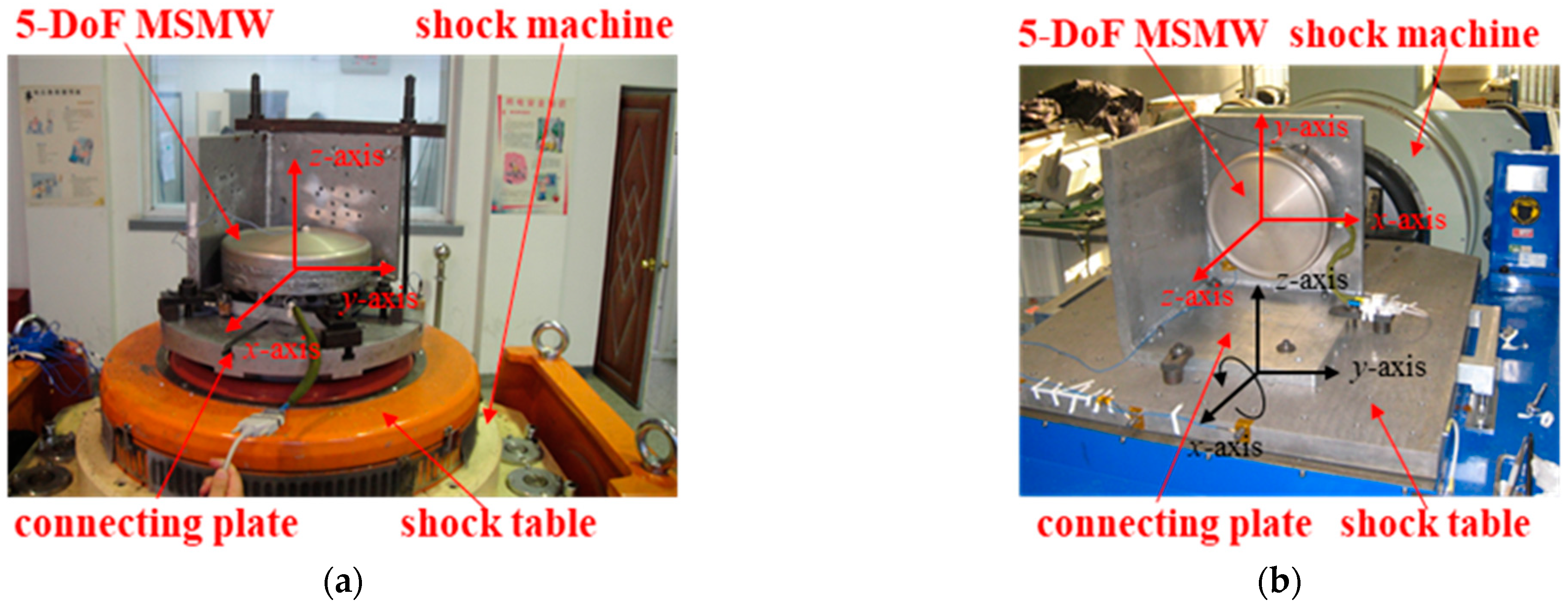
6.1. Vibration Measurement System of 5-DoF MSMW
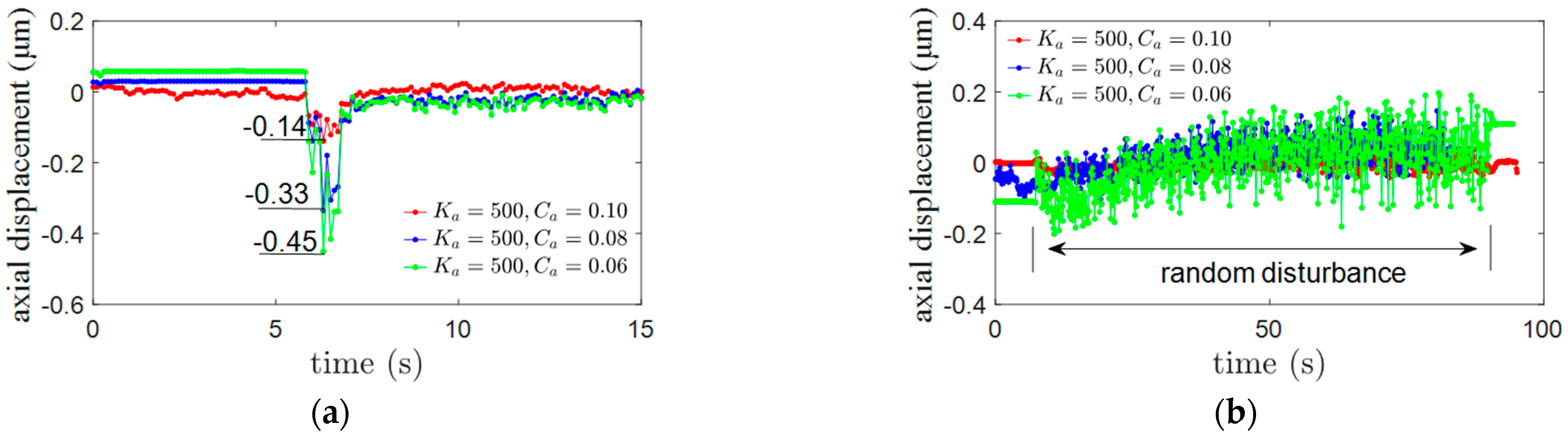
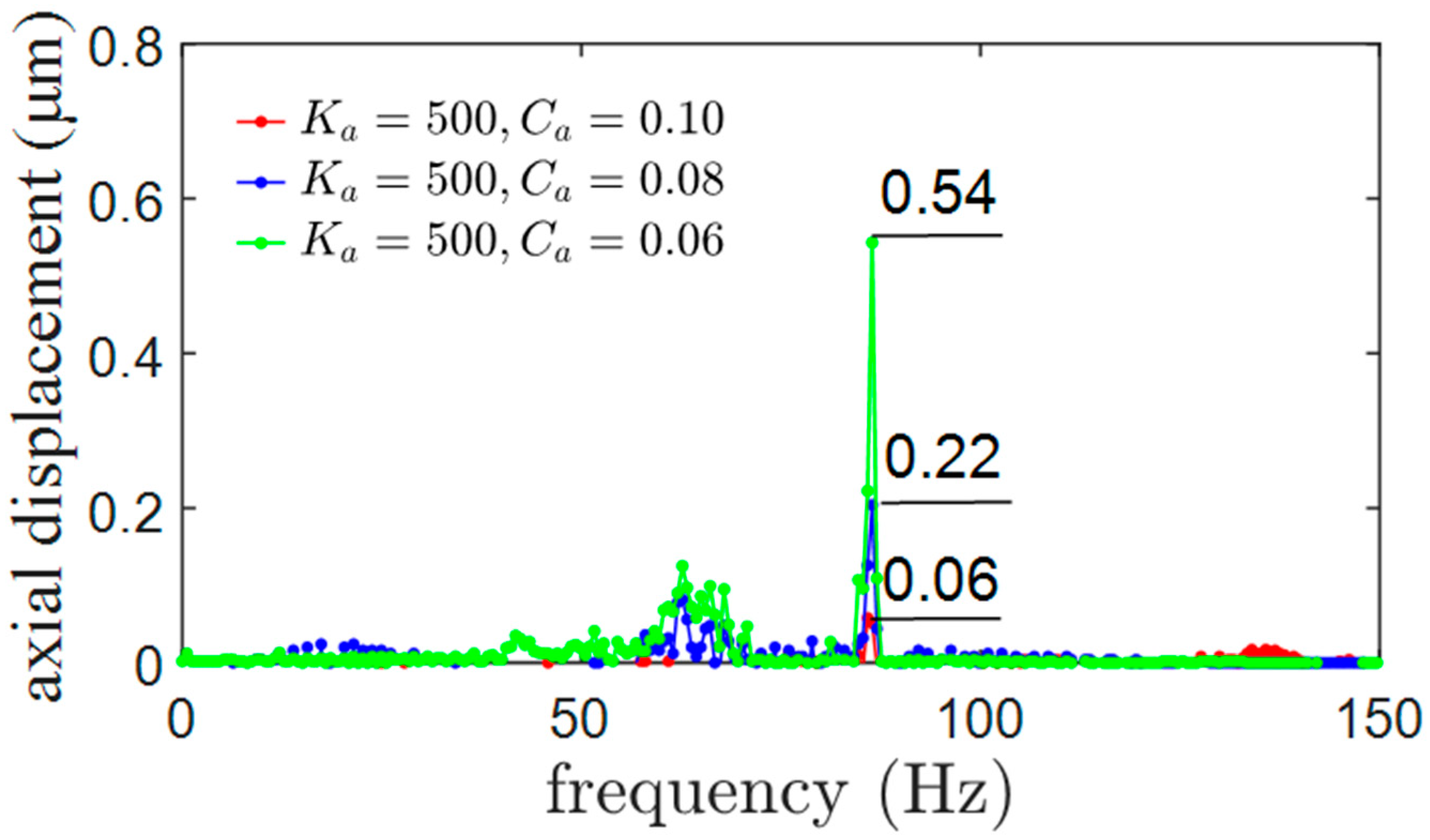
6.2. Translational Vibration Experiment of 5-DoF MSMW
6.3. Torsional Vibration Experiment of 5-DoF MSMW
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Mahfouz, A.; Pritykin, D.; Biggs, J. Hybrid Attitude Control for Nano-Spacecraft: Reaction Wheel Failure and Singularity Handling. J. Guid. Control. Dyn. 2021, 44, 548–558. [Google Scholar] [CrossRef]
- Ismail, Z.; Varatharajoo, R. A study of reaction wheel configurations for a 3-axis satellite attitude control. Adv. Space Res. 2009, 45, 750–759. [Google Scholar] [CrossRef]
- Kumar, K.D.; Godard; Abreu, N.; Sinha, M. Fault-tolerant attitude control of miniature satellites using reaction wheels. Acta Astronaut. 2018, 151, 206–216. [Google Scholar] [CrossRef]
- Abd-Elhay, A.-E.R.; Murtada, W.A.; Yosof, M.I. A high accuracy modeling scheme for dynamic systems: Spacecraft reaction wheel model. J. Eng. Appl. Sci. 2022, 69, 1–22. [Google Scholar] [CrossRef]
- Fu, C.; Sinou, J.-J.; Zhu, W.; Lu, K.; Yang, Y. A state-of-the-art review on uncertainty analysis of rotor systems. Mech. Syst. Signal Process. 2023, 183, 109619. [Google Scholar] [CrossRef]
- Jia, Q.; Li, H.; Chen, X.; Zhang, Y. Observer-based reaction wheel fault reconstruction for spacecraft attitude control systems. Aircr. Eng. Aerosp. Technol. 2019, 91, 1268–1277. [Google Scholar] [CrossRef]
- Tuysuz, A.; Achtnich, T.; Zwyssig, C.; Kolar, J.W. A 300 000-r/min Magnetically Levitated Reaction Wheel Demonstrator. IEEE Trans. Ind. Electron. 2018, 66, 6404–6407. [Google Scholar] [CrossRef]
- Xiang, B.; Liu, H.; Yu, Y. Gimbal effect of magnetically suspended flywheel with active deflection of Lorentz-force magnetic bearing. Mech. Syst. Signal Process. 2022, 173, 109081. [Google Scholar] [CrossRef]
- Zhai, L.; Han, B.; Liu, X.; Zhao, J. Losses estimation, thermal-structure coupled simulation analysis of a magnetic-bearing reaction wheel. Int. J. Appl. Electromagn. Mech. 2019, 60, 33–53. [Google Scholar] [CrossRef]
- Xiang, B.; Wen, T.; Liu, Z. Vibration analysis, measurement and balancing of flywheel rotor suspended by active magnetic bearing. Measurement 2022, 197, 111305. [Google Scholar] [CrossRef]
- Dagnaes-Hansen, N.A.; Santos, I.F. Magnetically suspended flywheel in gimbal mount-Test bench design and experimental validation. J. Sound Vib. 2019, 448, 197–210. [Google Scholar] [CrossRef]
- Dagnaes-Hansen, N.A.; Santos, I.F. Magnetically suspended flywheel in gimbal mount–Nonlinear modelling and simulation. J. Sound Vib. 2018, 432, 327–350. [Google Scholar] [CrossRef]
- Saeed, N.A.; El-Shourbagy, S.M.; Kamel, M.; Raslan, K.R.; Awrejcewicz, J.; Gepreel, K.A. On the Resonant Vibrations Control of the Nonlinear Rotor Active Magnetic Bearing Systems. Appl. Sci. 2022, 12, 8300. [Google Scholar] [CrossRef]
- Soni, T.; Dutt, J.K.; Das, A. Dynamic behavior and stability of energy efficient electro-magnetic suspension of rotors involving time delay. Energy 2021, 231, 120906. [Google Scholar] [CrossRef]
- Zheng, S.; Wang, C. Rotor Balancing for Magnetically Levitated TMPs Integrated With Vibration Self-Sensing of Magnetic Bearings. IEEE/ASME Trans. Mechatron. 2021, 26, 3031–3039. [Google Scholar] [CrossRef]
- Li, X.; Dietz, D.; An, J.; Erd, N.; Gemeinder, Y.; Binder, A. Manufacture and Testing of a Magnetically Suspended 0.5 kWh-Flywheel Energy Storage System. IEEE Trans. Ind. Appl. 2022, 58, 1–11. [Google Scholar] [CrossRef]
- Li, X.; Palazzolo, A.; Wang, Z. A Combination 5-DOF Active Magnetic Bearing for Energy Storage Flywheels. IEEE Trans. Transp. Electrif. 2021, 7, 2344–2355. [Google Scholar] [CrossRef]
- Cole, M.O.T.; Fakkaew, W. An Active Magnetic Bearing for Thin-Walled Rotors: Vibrational Dynamics and Stabilizing Control. IEEE/ASME Trans. Mechatron. 2018, 23, 2859–2869. [Google Scholar] [CrossRef]
- Xiang, B.; Wong, W. Electromagnetic vibration absorber for torsional vibration in high speed rotational machine. Mech. Syst. Signal Process. 2020, 140, 106639. [Google Scholar] [CrossRef]
- Lusty, C.; Keogh, P. Active Vibration Control of a Flexible Rotor by Flexibly Mounted Internal-Stator Magnetic Actuators. IEEE/ASME Trans. Mechatron. 2018, 23, 2870–2880. [Google Scholar] [CrossRef]
- Hutterer, M.; Wimmer, D.; Schrödl, M. Control of magnetically levitated rotors using stabilizing effects of gyroscopes. Mech. Syst. Signal Process. 2021, 166, 108431. [Google Scholar] [CrossRef]
- Numanoy, N.; Srisertpol, J. Vibration Reduction of an Overhung Rotor Supported by an Active Magnetic Bearing Using a Decoupling Control System. Machines 2019, 7, 73. [Google Scholar] [CrossRef]
- Xiang, B.; Wong, W. Decoupling control of magnetically suspended motor rotor with heavy self-weight and great moment of inertia based on internal model control. J. Vib. Control 2021, 28, 1591–1604. [Google Scholar] [CrossRef]
- Gallego, G.B.; Rossini, L.; Achtnich, T.; Araujo, D.M.; Perriard, Y. Novel Generalized Notch Filter for Harmonic Vibration Suppression in Magnetic Bearing Systems. IEEE Trans. Ind. Appl. 2021, 57, 6977–6987. [Google Scholar] [CrossRef]
- Peng, C.; He, J.; Deng, Z.; Liu, Q. Parallel mode notch filters for vibration control of magnetically suspended flywheel in the full speed range. IET Electr. Power Appl. 2020, 14, 1672–1678. [Google Scholar] [CrossRef]
- Gong, L.; Zhu, C. Synchronous Vibration Control for Magnetically Suspended Rotor System Using a Variable Angle Compensation Algorithm. IEEE Trans. Ind. Electron. 2020, 68, 6547–6559. [Google Scholar] [CrossRef]



| Symbol | Quantity | Value |
|---|---|---|
| mr | Mass of flywheel rotor | 4.2 kg |
| ms | Mass of stator base | 12 kg |
| Je | Equatorial moment of inertia | 0.02865 kgm2 |
| Jp | Polar moment of inertia | 0.01508 kgm2 |
| kix | Current stiffness of radial AMB unit | 132.26 N/A |
| kdx | Displacement stiffness of radial AMB unit | −352 N/mm |
| kiz | Current stiffness of axial AMB unit | 128 N/A |
| kdz | Displacement stiffness of axial AMB unit | −132.7 N/mm |
| ks | Displacement sensitivity of eddy-current sensor | 4 V/mm |
| kw | Amplification coefficient of power system | 3.6 A/V |
| Kj | Translational stiffness coefficient of connection joint | 600 |
| Cj | Translational damping coefficient of connection joint | 0.02 |
| Kjt | Torsional stiffness coefficient of connection joint | 58 |
| Cjt | Torsional damping coefficient of connection joint | 0.025 |
| Frequency | Vibration Amplitude | Speed | Imposing Axis |
|---|---|---|---|
| 10–20 Hz | 10 mm | 2 octave/min | z-axis |
| 20–100 Hz | 16 g | 2 octave/min | z-axis |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiang, B.; Liu, H. Dynamic Vibration Absorbing Performance of 5-DoF Magnetically Suspended Momentum Wheel Based on Damping Regulation. Actuators 2023, 12, 152. https://doi.org/10.3390/act12040152
Xiang B, Liu H. Dynamic Vibration Absorbing Performance of 5-DoF Magnetically Suspended Momentum Wheel Based on Damping Regulation. Actuators. 2023; 12(4):152. https://doi.org/10.3390/act12040152
Chicago/Turabian StyleXiang, Biao, and Hu Liu. 2023. "Dynamic Vibration Absorbing Performance of 5-DoF Magnetically Suspended Momentum Wheel Based on Damping Regulation" Actuators 12, no. 4: 152. https://doi.org/10.3390/act12040152
APA StyleXiang, B., & Liu, H. (2023). Dynamic Vibration Absorbing Performance of 5-DoF Magnetically Suspended Momentum Wheel Based on Damping Regulation. Actuators, 12(4), 152. https://doi.org/10.3390/act12040152







