1. Introduction
The need for alternative fuels for conventional internal combustion engines (ICEs) has grown as a result of depletion of crude oil reserves, global warming and more rigorous emission regulations. Natural gas (NG), generally containing more than 90% methane, is considered one of the most promising alternative fuels owing to its advantages of abundant reserves, cleaner combustion and lower cost [
1]. According to the International Energy Agency, the importance of NG can be compared with that of gasoline in terms of power generation, transportation, etc. [
2]. Driven by current sustainability development policies, over one-quarter of global power generation is provided by NG [
3]. In terms of road transport, NG has replaced other alternative fuels as the preferred choice for vehicles [
4].
In recent years, natural-gas vehicles (NGVs) have been vigorously developed and employed around the world [
5]. Until 2019, there were more than 28.5 million NGVs worldwide (including all land-based motor vehicles, from two-wheeled to off-road). Asian countries led the world in this respect with 20.5 million NGVs, followed by Latin American countries with 5.4 million NGVs [
6]. In particular, NG is the main alternative fuel for long-haul and heavy trucks, such as long-distance transport vehicles and fleets that require centralized refueling [
4].
The fuel supply system plays an important role in the performance of NG-fueled engines. Based on the supply mode, fuel supply systems can be classified as out-cylinder premixed method, port fuel injection (PFI) and direct injection (DI). The premixed method, which is the oldest NG supply method, works through the incorporation of a Venturi tube [
7]. NG is premixed with air in the Venturi tube, and then the mixture is drawn into the cylinder because of the in-cylinder subatmospheric pressure during intake. This method leads to great power reduction, although it has the best mixing uniformity. The PFI mode can be divided into single-point injection (SPI) and multipoint injection (MPI). For the former mode, NG is injected near the intake manifold near the gas injector, while for the latter, NG is injected into the intake port of each cylinder, closer to the cylinder than in SPI. At present, the PFI mode is the most common fuel supply system for compressed natural gas (CNG) spark-ignition engines. However, it will lead to torque cutting due to reductions in volume efficiency. In contrast, for the DI mode, this problem can be avoided via injecting gaseous fuel after the intake-valve closing time (IVC).
The transient injection characteristics of a gas fuel injection device, as the terminal executive component of the PFI or DI mode, will affect the key performance of an NG engine directly. One outward-opening NG injector, driven with a piezo actuator, was developed by Siemens under support from the New Integrated Combustion System for Future Passenger Car Engines project (during the period of 2004 to 2012) [
8,
9]. Considering that the piezo actuator’s stroke was rather small, a hydraulic stroke amplifier unit was designed to achieve the desired needle lift (about 200 μm) and mass flow rate. Based on this injector, Baratta et al. [
10] used planar laser-induced fluorescence (PLIF) and the computational fluid dynamics (CFD) method to investigate the differences of transient NG jet patterns between different engine cycles. The NG jets were identified as having “cloud-like” and “umbrella-like” shapes.
In recent years, research of NG injectors (or NG injection devices) mainly focused on electromagnetic characteristics, structural design and structural optimization based on steady internal-flow characteristics [
11,
12,
13,
14]. A novel cascade control algorithm was used by Tan et al. to achieve multiobjective optimization of control parameters for electromagnetic linear actuators [
11]. One new sensorless electronic closed-loop antibounce solution was proposed by Glasmachers et al. to effectively reduce bouncing and provide robust, soft landing for fuel injectors [
12]. Single-shot X-ray radiography was used by Swantek et al. to investigate the steady-state behavior of an outward-opening gas injector [
13]. Several CFD simulations of steady-state gas flow through various poppet-valve geometries were performed by Kim et al. to suggest design improvements for obtaining more efficient poppet valves with reduced stagnation pressure loss [
14].
The transient injection characteristics of the gas fuel injector have, however, received little attention. The transient gas flow development of one outward-opening injector was studied by Deshmukh et al. [
15,
16] with the large eddy simulation (LES) method. The research results showed that an NG jet’s features (such as axial penetration length, maximum jet width and volume) and subsequent mixture-formation process would be seriously affected by the transient motion process of an injector. In addition, there would be about a 30% disparity between the data of an LES simulation and the corresponding experiment if the motion process of an NG injector was ignored. One high-pressure NG injector was developed by Rogers et al. [
17], based on BOSCH’s gasoline injector, and the transient injection process of this NG injector was studied with the particle image velocimetry method. It was found that the injector would have a large degree of seating bounce when its valve was seated at the valve seat or the upper stop position because of the undesirable force characteristics of a traditional solenoid. A solenoid is generally characterized with greater force at both ends of the stroke, and it is difficult to achieve accurate displacement control with it. The maximum bouncing lift was almost 80% larger than the expected stable lift. In addition, the duration of the seating bounce was about 16% of the whole injection-pulse width of the NG injector [
17]. It is obvious that these on/off transition processes of NG injectors will play a vital role in the process of gas fuel injection and subsequent in-cylinder mixture formation.
However, this research of the effects of transient valve motion on gas jet patterns is not comprehensive enough because the effects of key factors (such as injector-valve vibration magnitude, period and vibration time) have not been analyzed. As had been discussed in the literature cited above [
11,
12,
13,
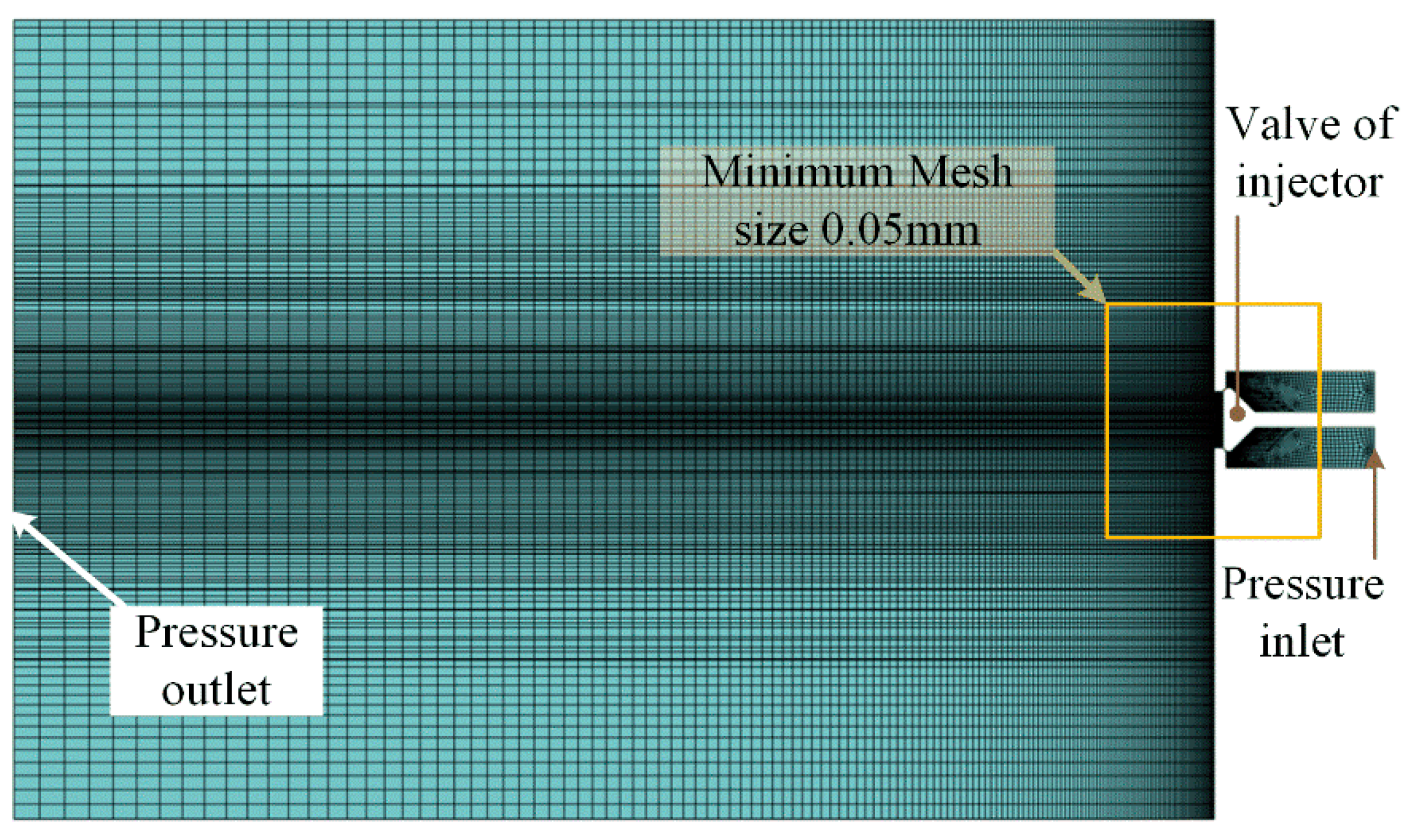
14], researchers were accustomed to trying to reduce the seating impact of the nozzle valve in order to improve the control accuracy of the fuel supply of the gas fuel engine. Problems such as how much impact the valve-seating-bounce process has on the transient injection mass flow rate and which factor among vibration magnitude, period and vibration time has the greatest impact on jet pattern have not been discussed. In this paper, the affecting laws of these factors will be given focus. In order to explore the effects of injector-valve transient vibration on transient injection characteristics, one transient CFD model of an NG fuel injection device of the DI mode is proposed. Factors such as valve vibration amplitude, period, frequency and velocity are discussed. Finally, the CFD model is validated in both the aspects of transient gas fuel jet pattern and cumulative mass flow rate. The research results of this paper will provide guidance for future research on seating control of gas fuel injection devices.
3. Results and Discussion
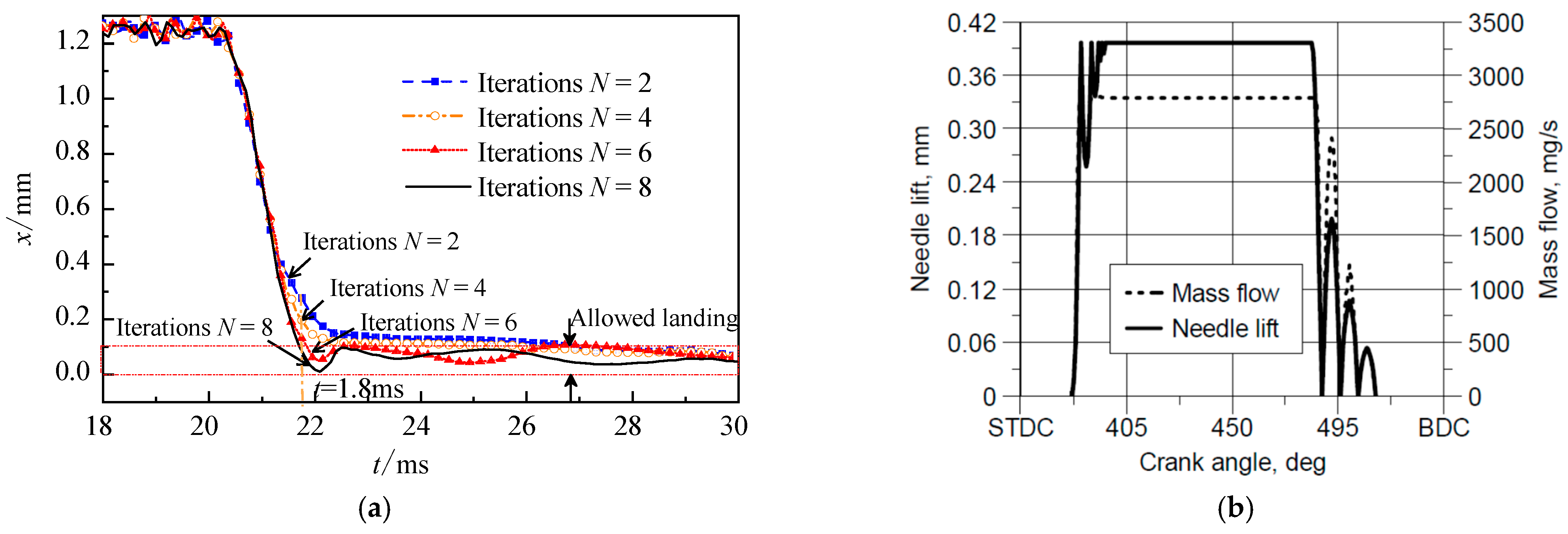
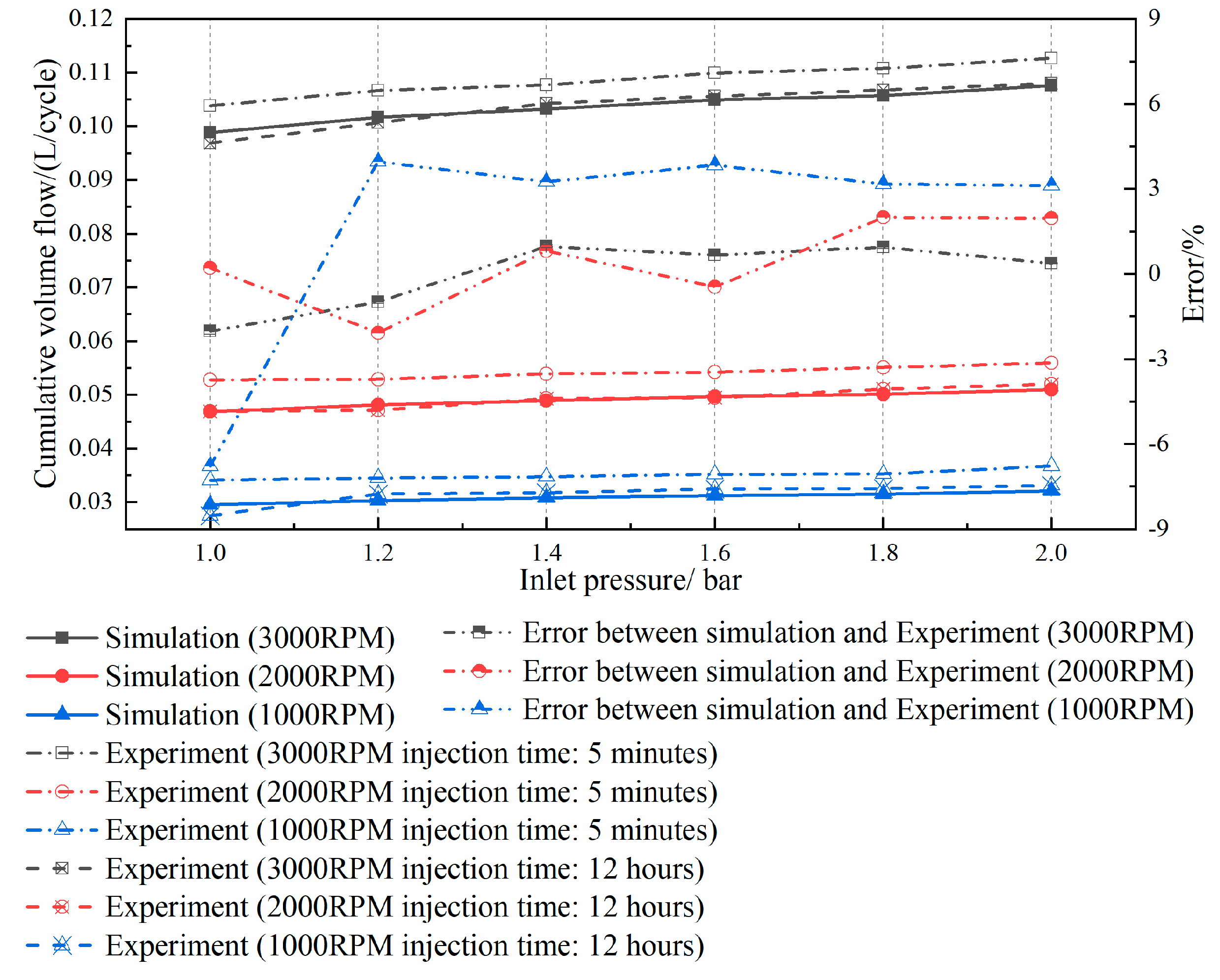
The flow fluctuation after the end of the valve lift vibration (called delayed flow fluctuation (DFF), as shown in
Figure 7a) is the most important focus of this study. DFF caused by lift vibration would have a serious impact on the calibration quantity of gas fuel under certain engine working conditions because DFF is usually uncontrollable. In order to facilitate a comparison, the ending times of the valve vibration processes for all discussed cases (as shown in
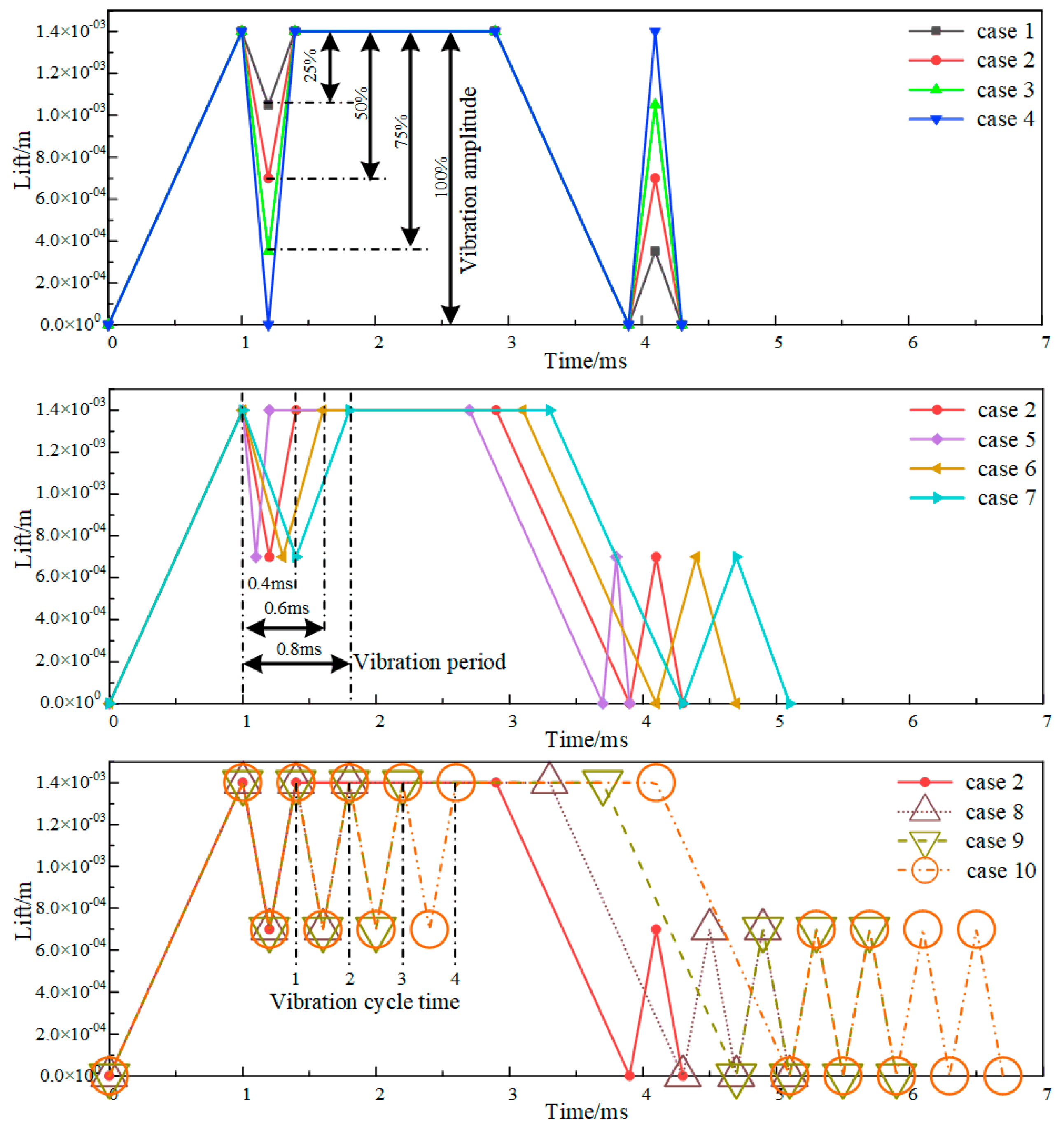
Table 3 and
Figure 2) were normalized to the ending times (1.4 ms) of the valve vibrations for cases 1–4. Only the DFF near the maximum valve lift will be discussed in this study, because there was no DFF near the zero-lift position.
Firstly, the effect of vibration amplitude was discussed as cases 1–4 were compared, and the NG flow-rate fluctuation processes through the injection device’s throat in these four cases are presented in
Figure 7. It was found that there was an almost linear correlation between the transient flow rate and instantaneous lift vibration during the lift-vibration process (from 1.0 ms to 1.4 ms), as shown in
Figure 7a. Case 4 had the strongest DFF, and the strengths of the DFFs for case 3, case 2 and case 1 showed a gradual downward trend, as shown in
Figure 7b. The cycle periods of the DFF for cases 1–4 were all the same. For a more comprehensive comparison, a case with no valve lift vibration (called no vibration in figure) was also taken into consideration. It was easily found that the case with no vibration had the weakest DFF and the shortest cycle period when compared with cases 1–4. It is obvious that higher vibration amplitude brings stronger DFF.
Secondly, the effect of the vibration period was discussed as cases 2 and 5–7 were compared, and the NG flow-rate fluctuation processes of these four cases are presented in
Figure 8. The raw data during the lift-vibration process (for example, case 7: from 0.6 ms to 1.4 ms (normalized)) were almost the same as those when the vibration-amplitude factor was concerned. The linear relationships between the transient flow rate and instantaneous lift vibration still existed for case 6 and case 7. However, for case 5, which had the shortest vibration period, this linear relationship was somewhat broken, as shown in
Figure 8a. It is obvious that the transient developing process of the supersonic gas jet was influenced by the faster velocity of valve vibration in case 5, and the transient mass flow rate was affected in turn.
When the vibration-period factor was considered, case 5 (period: 0.2 ms) had the strongest DFF, and the strengths of the DFFs for case 2 (period: 0.4 ms), case 7 (period: 0.8 ms) and case 6 (period: 0.6 ms) showed a gradual downward trend, as shown in
Figure 8b. Case 5 had the longest DFF period, case 2 took the second place, case 7 took the third place and case 6 had the shortest value, as shown in
Figure 8c. There seems to be some correlation among them: cases 2 and 5–7, which had shorter lift-vibration periods, had stronger DFFs and longer DFF periods. This rule is not applicable to case 6 or case 7, however. Actually, case 6, case 7 and the case with no vibration had some crossing areas when the case with no vibration was taken into consideration, as shown in
Figure 8c. Their DFF strengths and periods were almost the same. Therefore, it can be concluded that the effect of valve vibration period on DFF will be rather little if the vibration period is long enough (the boundary was about 0.6 ms (case 6) in the example presented in this paper).
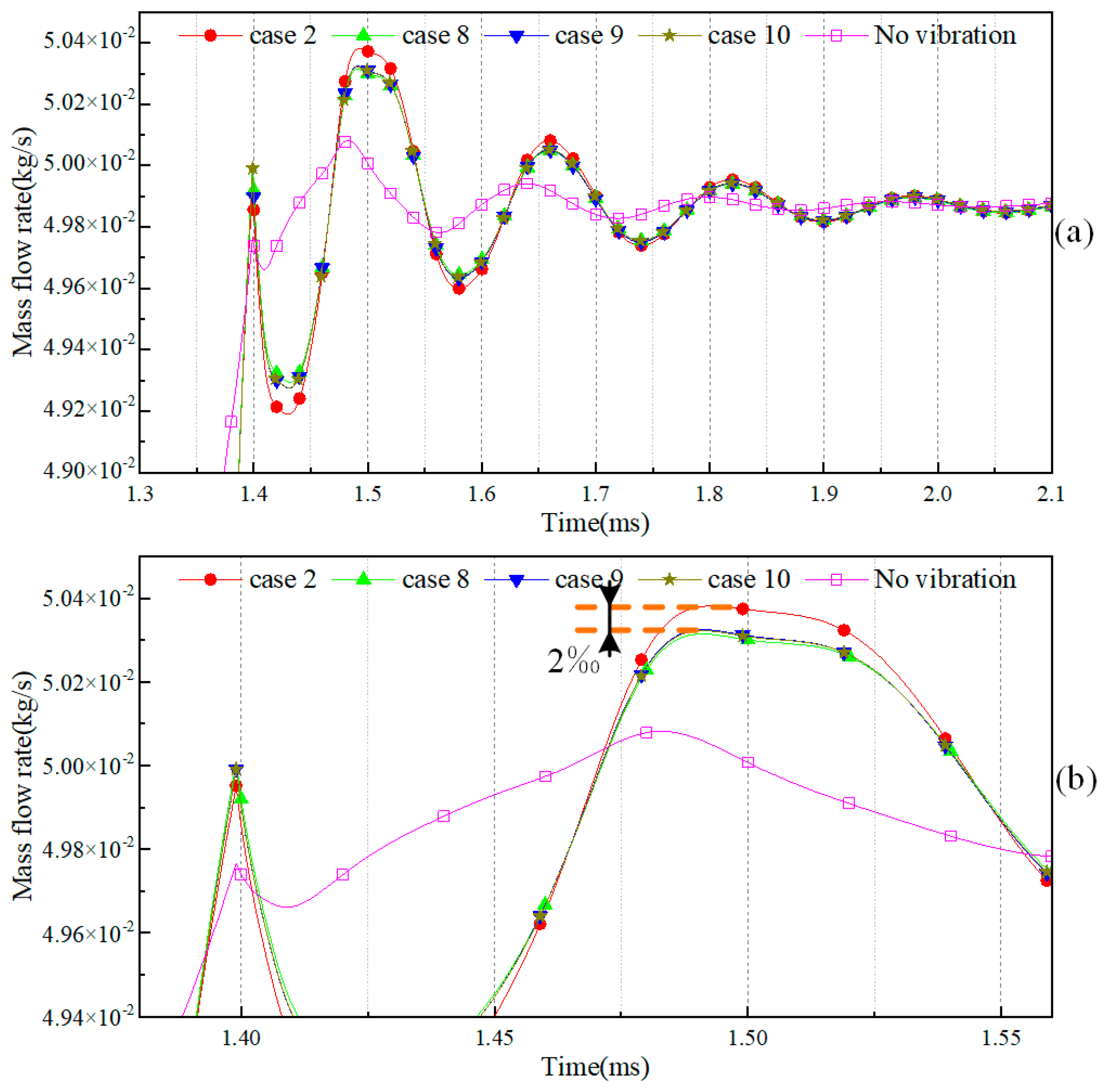
At last, the effect of vibration-cycle time was discussed as cases 2 and 8–10 were compared, as shown in
Figure 9. It was found that the DFF of case 2 (cycle time: 1) was the strongest, and the DFFs of cases 8–10 were almost the same. What is more important is that the DFF strengths of the last three cases were slightly weaker (about 1.2‰ at the peak point of the flow rate) than that of case 2, as shown in
Figure 9b. It can be concluded that more vibration time tends to bring weaker DFF, although the influence is quite little.
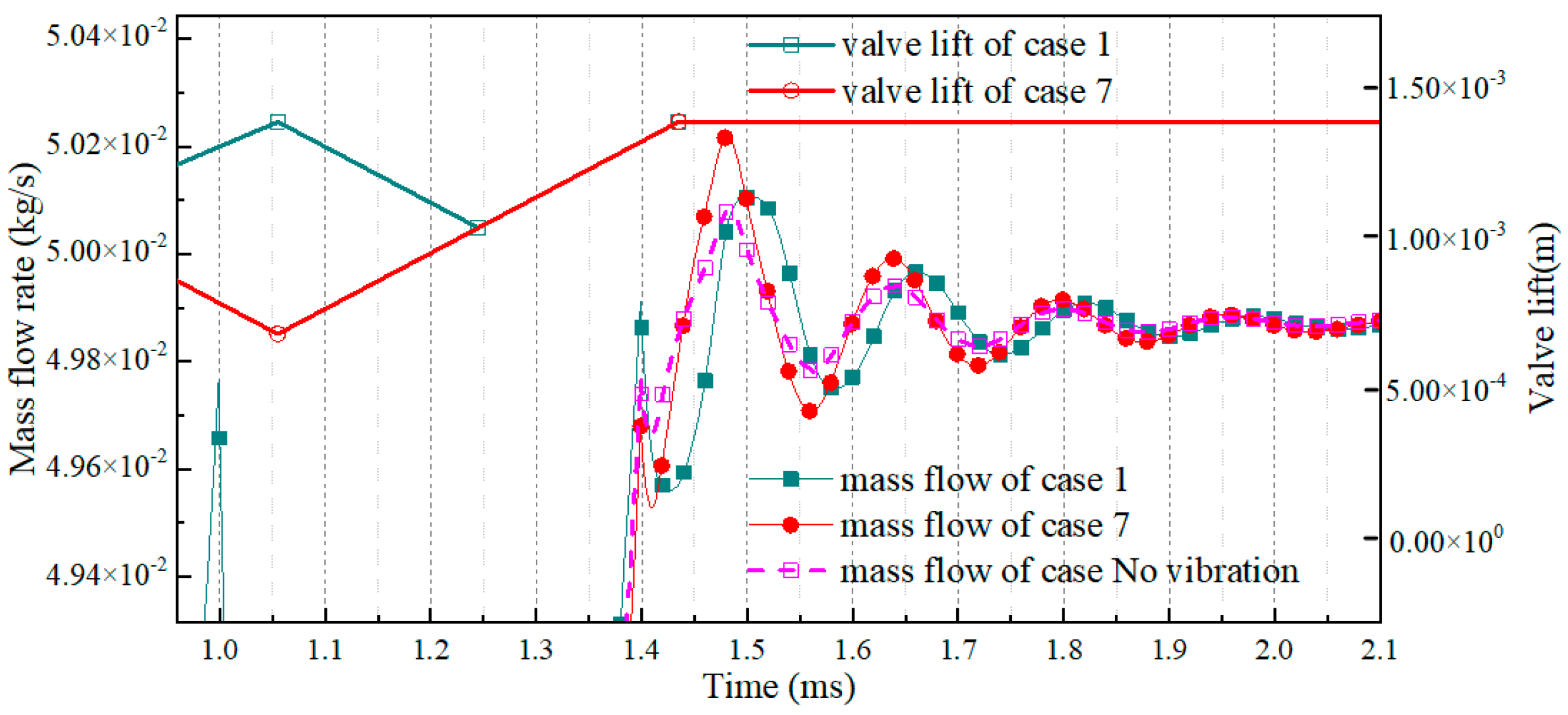
By now, the influences of injector-valve lift-vibration amplitude, period and cycle time on transient gas jet have been discussed in cases 1–10, above. It was interesting to find that case 4 had the same valve vibration speed (slope of valve lift-vibration curve) as case 5, as shown in
Figure 2. This situation also existed for case 1 and case 7. Therefore, it is necessary to discuss these two cases further. Their valve lifts and corresponding mass flow rates are presented in
Figure 10 and
Figure 11, respectively. It can be seen that case 5, which had a smaller lift-vibration amplitude, shows a stronger DFF than case 4, and that case 1, which had a smaller lift-vibration amplitude, shows a weaker DFF than case 7. These seem to lead to quite different conclusions. However, it is worth noting that the DFF strengths of case 1 and case 7 are actually almost the same as each other. The biggest difference between case 1 and case 7 is smaller than 4‰, and these two cases’ DFFs were almost the same as that of the case with no vibration, as shown in
Figure 7 and
Figure 8, because their lift-vibration speeds were rather slow (that is: their vibration periods were long enough). Shorter vibration tends to cause greater DFF when the vibration speed is the same.
According to the mass-flow-rate formula of one-dimensional isentropic flow [
17], the flow fluctuation of a jet nozzle generally results from pressure-ratio (ratio of pressure downstream of the nozzle throat and pressure upstream of the nozzle throat) fluctuation. Therefore, in order to reveal the reason for DFF in this paper, the pressure fluctuation situations downstream and upstream of the NG injection device’s throat are presented in
Figure 12. Based on the mass flow-rate fluctuation curves (
Figure 7,
Figure 8 and
Figure 9) after the end the of valve lift vibration, three peak points and three valley points of each fluctuation curve were selected as the inner pressure analyzing points. Firstly, the throat near-field pressure of the case with no vibration was presented because the DFF of this case could not come from valve lift vibration. The analyzing points were 1.009 ms, 1.082 ms, 1.162 ms, 1.239 ms, 1.320 ms and 1.400 ms. Secondly, as discussed above, case 2 was used as a base case for comparison, and its near-field pressure needed to be presented. The analyzing points were 1.430 ms, 1.492 ms, 1.580 ms, 1.657 ms, 1.741 ms and 1.820 ms.
It could be intuitively obtained from the near-field pressure distribution that a smaller pressure-concentration zone is formed on the back face of an injector’s valve for the valley point (for example: case with no vibration, 1.162 ms-valley) when compared with that for the nearby peak points (for example: case with no vibration, 1.082 ms-peak and 1.239 ms-peak). In addition, the volume of the pressure concentration area tends to increase as injection time goes by. On the other hand, for the peak point (for example: case 2, 1.657 ms-peak), a larger-pressure concentration area is formed when compared with that for nearby valley points (for example: case 2, 1.580 ms-valley and 1.741 ms-valley), and the volume tends to decrease. In addition, the differences of pressure distribution between nearby valley and peak points tend to be gradually smaller. For example, the difference between the 1.320 ms point and the 1.400 ms point was much smaller than that between the 1.162 ms point and the 1.239 ms point. This rule existed for both the case with no vibration and case 2. This gradually decreased pressure difference leads to a smaller amplitude of DFF, and so the gas jet would finally be stabilized. It was also found that the difference between the nearby valley and peak points of the case with no vibration was smaller than that of case 2, resulting in a lower amplitude of DFF in the former case, as shown in
Figure 7b.
4. Conclusions
The transient CFD model of a DI NG injector’s transient injection process was established in this paper. Based on this CFD model, the influences of injector valve vibration amplitude, period, cycle time and velocity on transient injection characteristics were investigated. Flow fluctuation after the end of valve lift vibration was a focus. The main conclusions are as follows:
There is an almost linear correlation between the transient flow rate and instantaneous lift vibration during the lift-vibration process (from 1.0 ms to 1.4 ms for cases 1–4), and this linear correlation will be somewhat broken if the valve’s vibration velocity is high enough or the vibration period is short enough.
A higher value of vibration amplitude tends to bring stronger DFF. In studying the seating control of gas fuel injectors, efforts should be made to reduce valve lift-vibration amplitude because it has a significant impact on flow during and after the injection period.
Under the condition of the same amplitude, a shorter valve vibration period means stronger DFF and a longer period of DFF. In addition, the effect of the valve vibration period on DFF is rather little if the vibration period is long enough (the boundary was about 0.6 ms in the example presented in this paper).
The influence of vibration-cycle time on DFF is quite little. More vibration time tends to bring weaker DFF. When studying the seating control of gas fuel injectors, we should try to avoid too-fast valve lift-vibration speed. However, vibration-cycle time does not need too much attention when only DFF is considered.
The throat near-field pressure fluctuation caused by valve lift vibration results in DFF.