Numerical Study on Unbalance Response of Dual-Rotor System Based on Nonlinear Bearing Characteristics of Active Magnetic Bearings
Abstract
1. Introduction
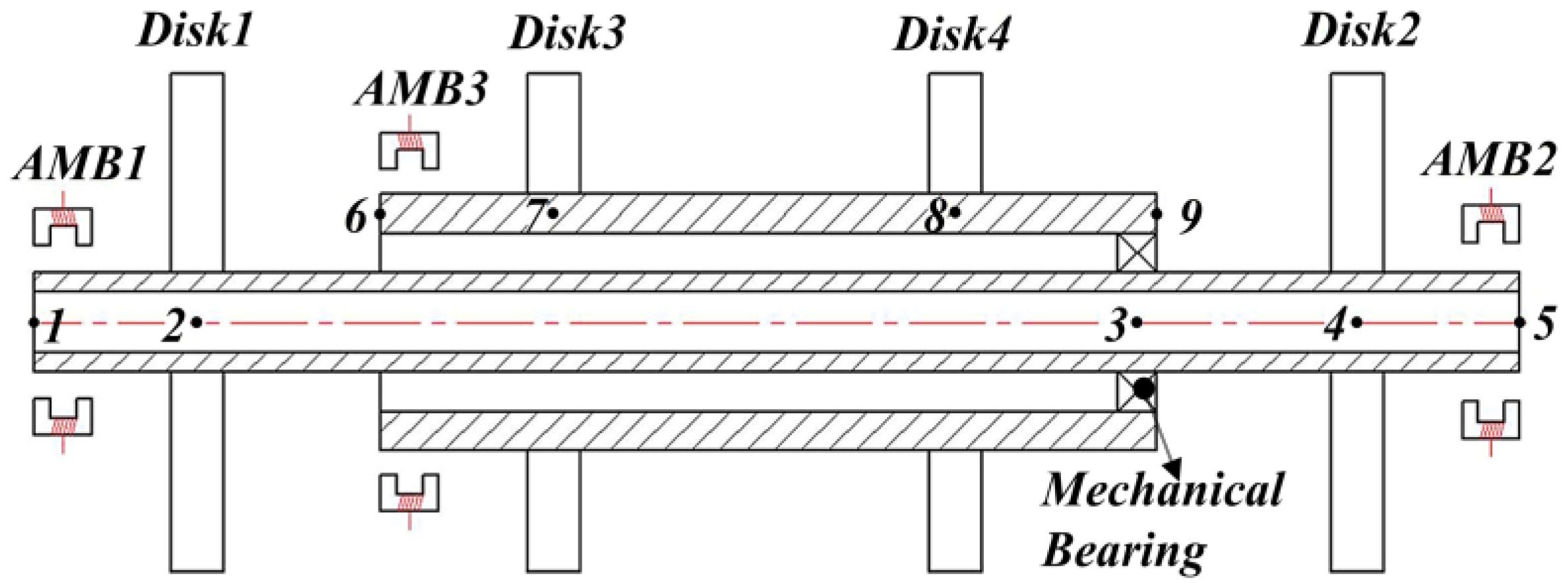
2. System Model
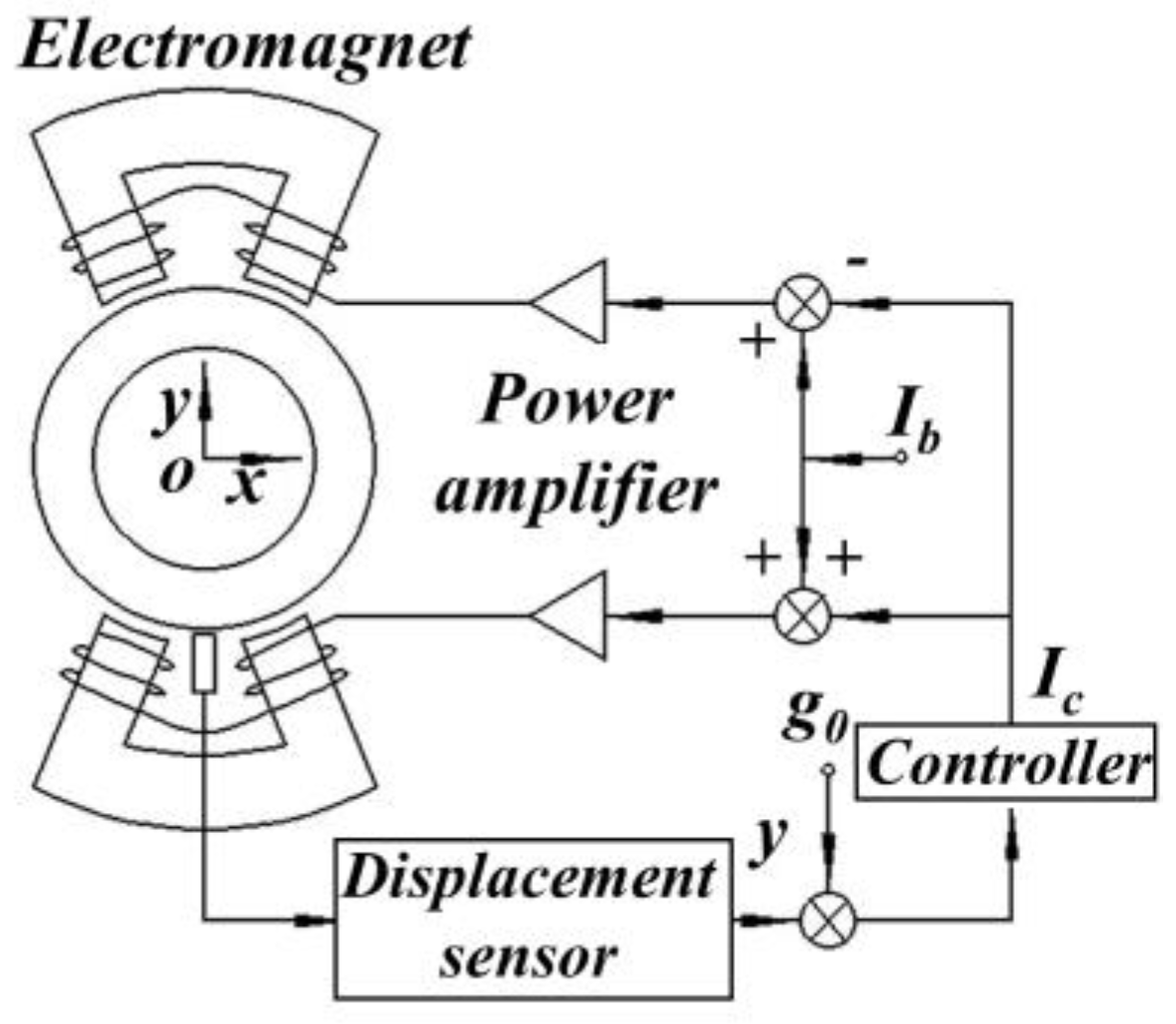
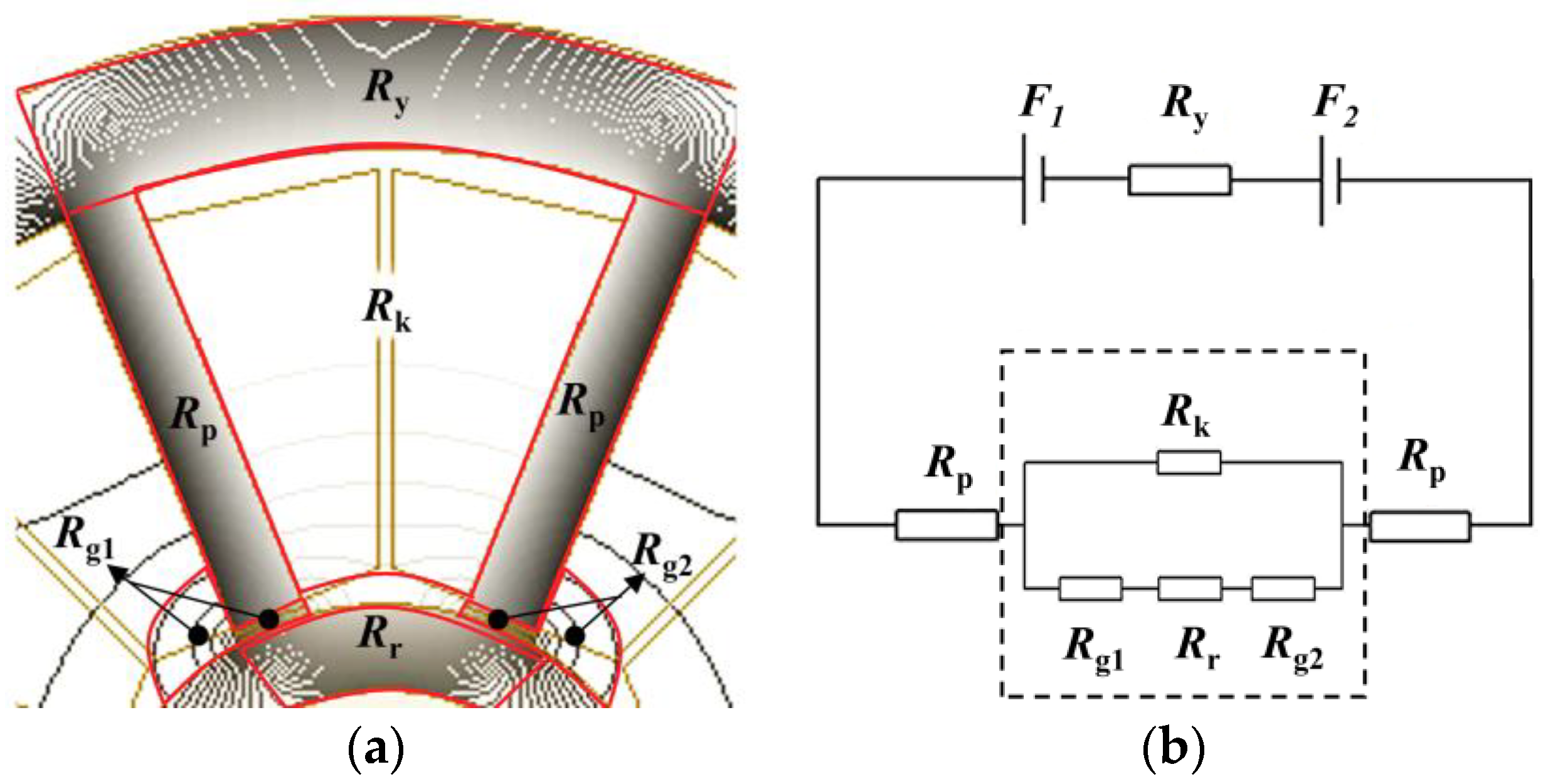
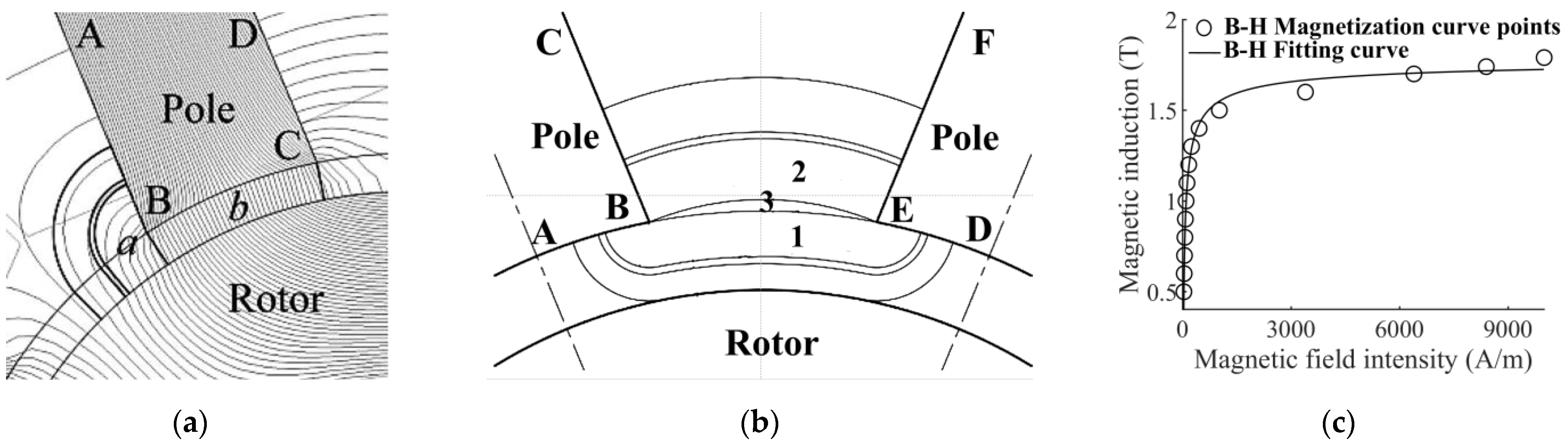
2.1. Magnetic Bearing Modeling
2.2. Dynamical Equations of the MSDS
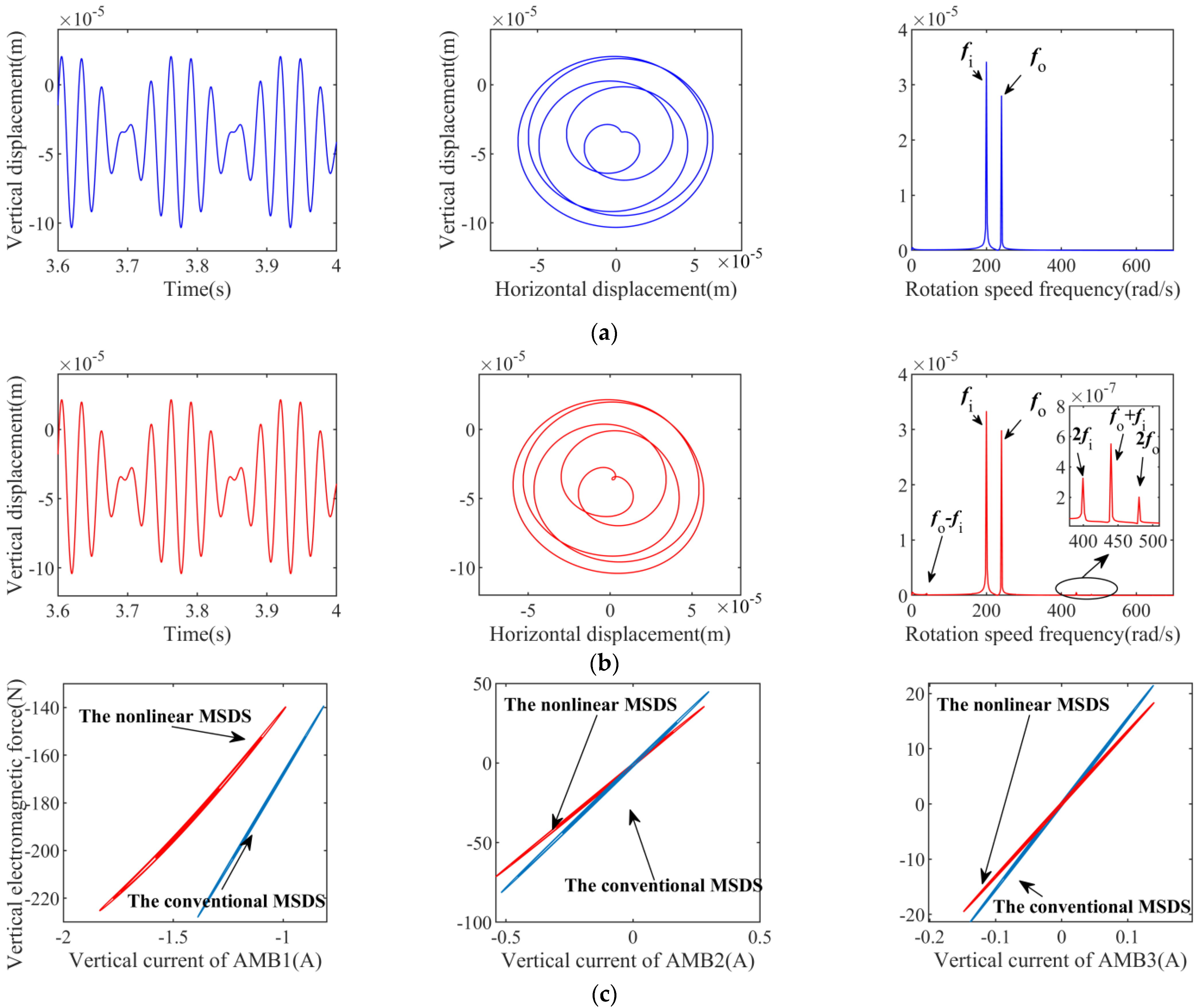
3. Model Validation
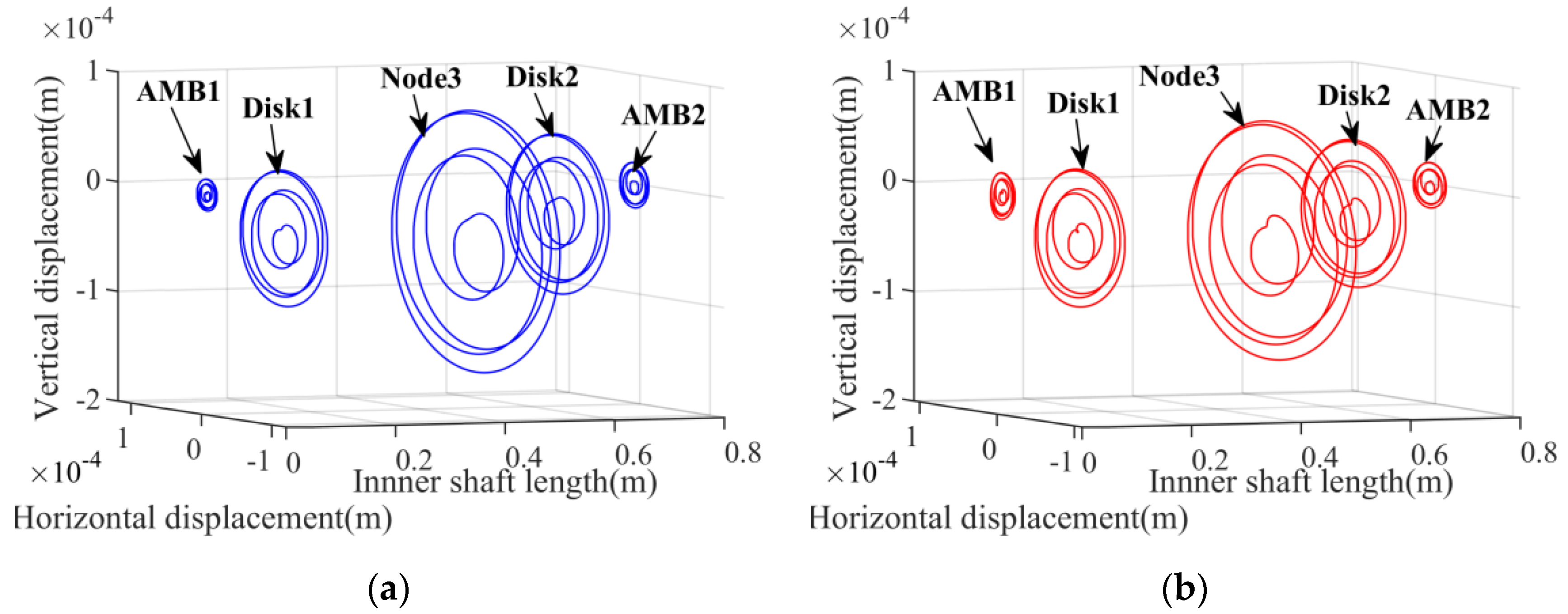
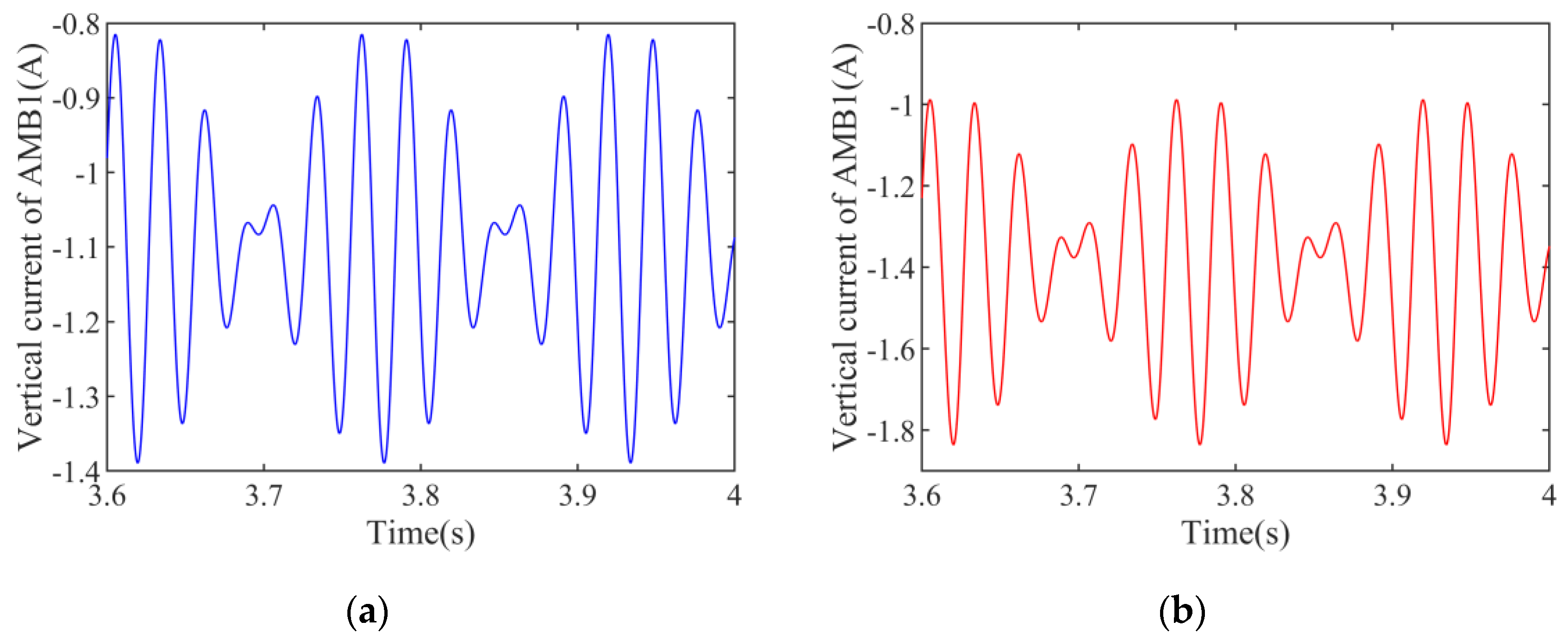
4. Unbalance Response Analysis
4.1. The Effect of Load on the System
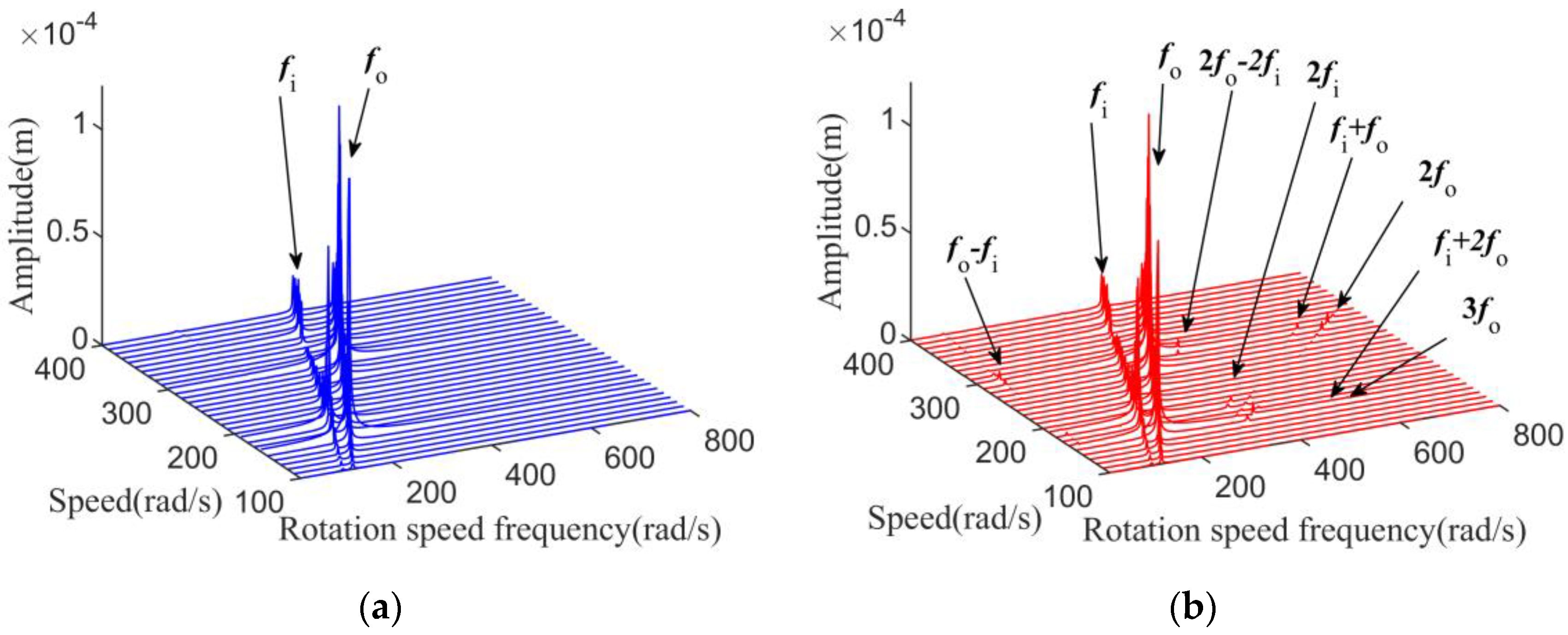
4.2. The Effect of Operation Parameters
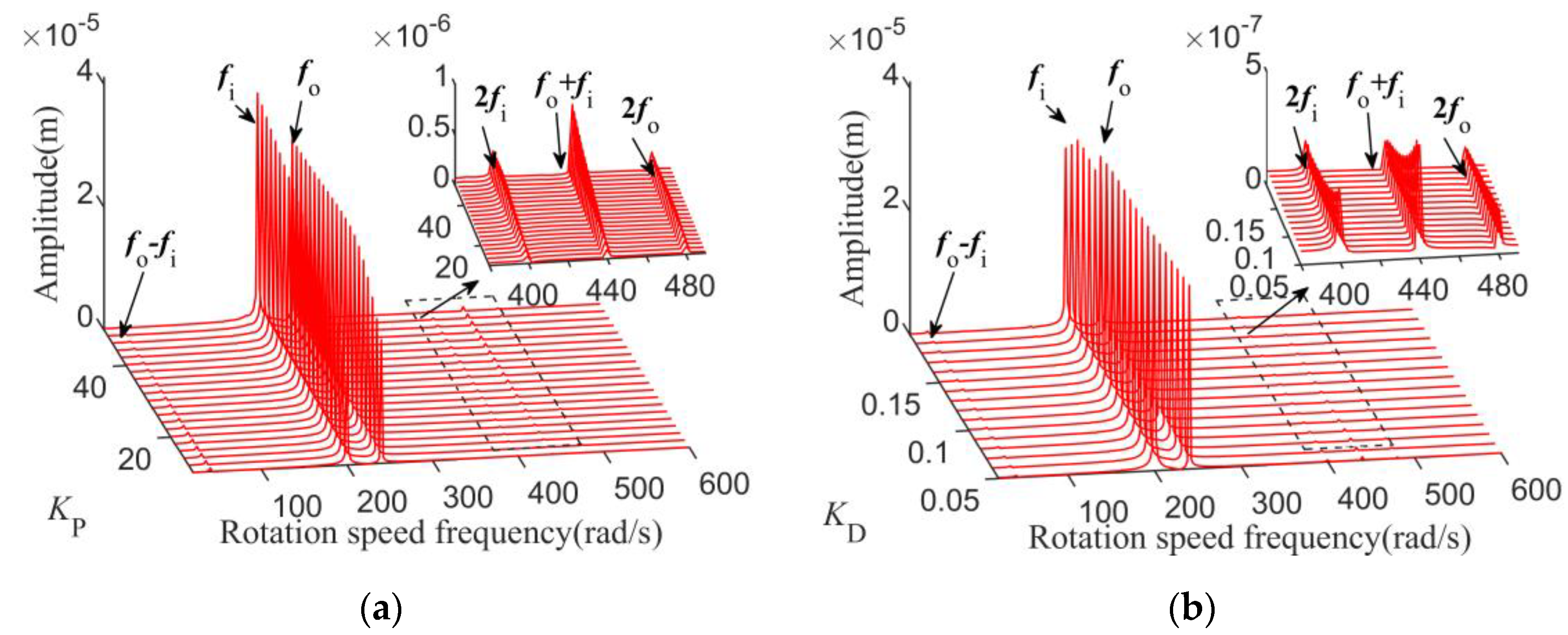
4.3. The Effect of Control Parameters
5. Conclusions
- (1)
- Combination frequencies (mfo ± nfi) may exist in the responses of the nonlinear MSDS under heavy loading conditions, which could excite the nonlinear bearing characteristics of the AMBs, and the effects of the inner and outer rotors’ disks that are loaded are basically identical. Moreover, the stability of the nonlinear MSDS is worse than that of the conventional MSDS under the same operating conditions.
- (2)
- KP has an influence on the amplitude of the nonlinear system; when the amplitude of the system becomes larger, the unbalance response of the system becomes more complex, and a small KD will lead to more combination frequencies. The results obtained in this paper may contribute to the construction of models based on the nonlinear bearing characteristics of AMBs and restraining the negative effect of nonlinear bearing characteristics on the system.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Maslen, E.H.; Schweitzer, G.; Bleuler, H.; Cole, M.; Traxler, A. Magnetic Bearings—Theory, Design, and Application to Rotating Machinery; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Wang, D.X.; Wang, N.X.; Chen, K.S.; Ye, C. Dynamic Characteristics of Magnetic Suspended Dual-Rotor System by Riccati Transfer Matrix Method. Shock. Vib. 2019, 2019, 9843732. [Google Scholar] [CrossRef]
- Yu, C.M.; Deng, Z.Q.; Mei, L.; Peng, C.; Cao, X.; Chen, S.S.; Ding, Q. Evaluation Criteria of Material Selection on 3-DOF Hybrid Magnetic Bearing. IEEE Trans. Ind. Appl. 2021, 57, 4733–4744. [Google Scholar] [CrossRef]
- Yu, C.; Deng, Z.; Mei, L.; Peng, C.; Cao, X. Multiobjective optimization of 3-DOF magnetic bearing considering eddy current effects and saturation. Mech. Syst. Signal Process. 2023, 182, 109538. [Google Scholar] [CrossRef]
- Zhang, W.Y.; Yang, H.K.; Cheng, L.; Zhu, H.Q. Modeling Based on Exact Segmentation of Magnetic Field for a Centripetal Force Type-Magnetic Bearing. IEEE Trans. Ind. Electron. 2020, 67, 7691–7701. [Google Scholar] [CrossRef]
- Wang, D.X.; Wang, N.X.; Ye, C.; Chen, K.S. Research on analytical bearing capacity model of active magnetic bearings based on magnetic saturation. IET Electr. Power Appl. 2017, 11, 1548–1557. [Google Scholar] [CrossRef]
- Wajnert, D.; Tomczuk, B. Nonlinear magnetic equivalent circuit of the hybrid magnetic bearing. Compel-Int. J. Comput. Math. Electr. Electron. Eng. 2019, 38, 1190–1203. [Google Scholar] [CrossRef]
- Lu, Z.Y.; Hou, L.; Chen, Y.S.; Sun, C.Z. Nonlinear response analysis for a dual-rotor system with a breathing transverse crack in the hollow shaft. Nonlinear Dyn. 2016, 83, 169–185. [Google Scholar] [CrossRef]
- Wang, D.; Wang, N.; Chen, K. Fixed-point Rubbing Characteristics of Magnetic Suspended Dual-rotor Systems. China Mech. Eng. 2021, 32, 1686–1699. [Google Scholar]
- Zhang, S.Y.; Zhou, J.; Wu, H.T.; Zhang, Y. Dynamic Analysis of Active Magnetic Bearing Rotor System Considering Alford Force. J. Vib. Eng. Technol. 2021, 9, 1147–1154. [Google Scholar] [CrossRef]
- Zhang, S.Y.; Zhou, J.; Han, X.M.; Ma, Y.C. Analysis of Nonlinear Characteristics and the Factors Affecting the Operation of the Active Magnetic Bearings Rotor System Considering Alford Force. Appl. Comput. Electromagn. Soc. 2022, 37, 253–261. [Google Scholar] [CrossRef]
- Su, Y.X.; Gu, Y.P.; Keogh, P.S.; Yu, S.Y.; Ren, G.X. Nonlinear dynamic simulation and parametric analysis of a rotor-AMB-TDB system experiencing strong base shock excitations. Mech. Mach. Theory 2021, 155, 104071. [Google Scholar] [CrossRef]
- Yi, H.; Hou, L.; Gao, P.; Chen, Y. Nonlinear resonance characteristics of a dual-rotor system with a local defect on the inner ring of the inter-shaft bearing. Chin. J. Aeronaut. 2021, 34, 110–124. [Google Scholar] [CrossRef]
- Gao, P.; Chen, Y.S.; Hou, L. Nonlinear thermal behaviors of the inter-shaft bearing in a dual-rotor system subjected to the dynamic load. Nonlinear Dyn. 2020, 101, 191–209. [Google Scholar] [CrossRef]
- Wang, F.; Luo, G.H.; Yan, S.; Cui, H.T. A Comparison Study on Co- and Counterrotating Dual-Rotor System with Squeeze Film Dampers and Intermediate Bearing. Shock. Vib. 2017, 2017, 5493763. [Google Scholar] [CrossRef]
- Ji, J.C.; Hansen, C.H. Non-Linear Oscillations of a Rotor in Active Magnetic Bearings. J. Sound Vib. 2001, 240, 599–612. [Google Scholar] [CrossRef]
- Zhang, G.; Xi, G. Vibration Control of a Time-Delayed Rotor-Active Magnetic Bearing System by Time-Varying Stiffness. Int. J. Appl. Mech. 2022, 14, 2250007. [Google Scholar] [CrossRef]
- Zhang, W.; Wu, R.Q.; Siriguleng, B. Nonlinear Vibrations of a Rotor-Active Magnetic Bearing System with 16-Pole Legs and Two Degrees of Freedom. Shock. Vib. 2020, 2020, 5282904. [Google Scholar] [CrossRef]
- Wu, R.Q.; Zhang, W.; Yao, M.H. Nonlinear dynamics near resonances of a rotor-active magnetic bearings system with 16-pole legs and time varying stiffness. Mech. Syst. Signal Process. 2018, 100, 113–134. [Google Scholar] [CrossRef]
- Zhang, X.S.; Fan, T.P.; Sun, Z.; Zhao, L.; Yan, X.S.; Zhao, J.L.; Shi, Z.G. Nonlinear Analysis of Rotor-AMB System with Current Saturation Effect. Appl. Comput. Electromagn. Soc. J. 2019, 34, 557–566. [Google Scholar]
- Sun, Z.; Zhang, X.; Fan, T.; Yan, X.; Zhao, J.; Zhao, L.; Shi, Z. Nonlinear dynamic characteristics analysis of active magnetic bearing system based on cell mapping method with a case study. Mech. Syst. Signal Process. 2019, 117, 116–137. [Google Scholar] [CrossRef]
- Kang, K.; Palazzolo, A. Homopolar Magnetic Bearing Saturation Effects on Rotating Machinery Vibration. IEEE Trans. Magn. 2012, 48, 1984–1994. [Google Scholar] [CrossRef]
- Soni, T.; Dutt, J.K.; Das, A.S. Dynamic behavior and stability of energy efficient electro-magnetic suspension of rotors involving time delay. Energy 2021, 231, 120906. [Google Scholar] [CrossRef]
- Yang, Y.; Cao, D.; Yu, T.; Wang, D.; Li, C. Prediction of dynamic characteristics of a dual-rotor system with fixed point rubbing—Theoretical analysis and experimental study. Int. J. Mech. Sci. 2016, 115, 253–261. [Google Scholar] [CrossRef]

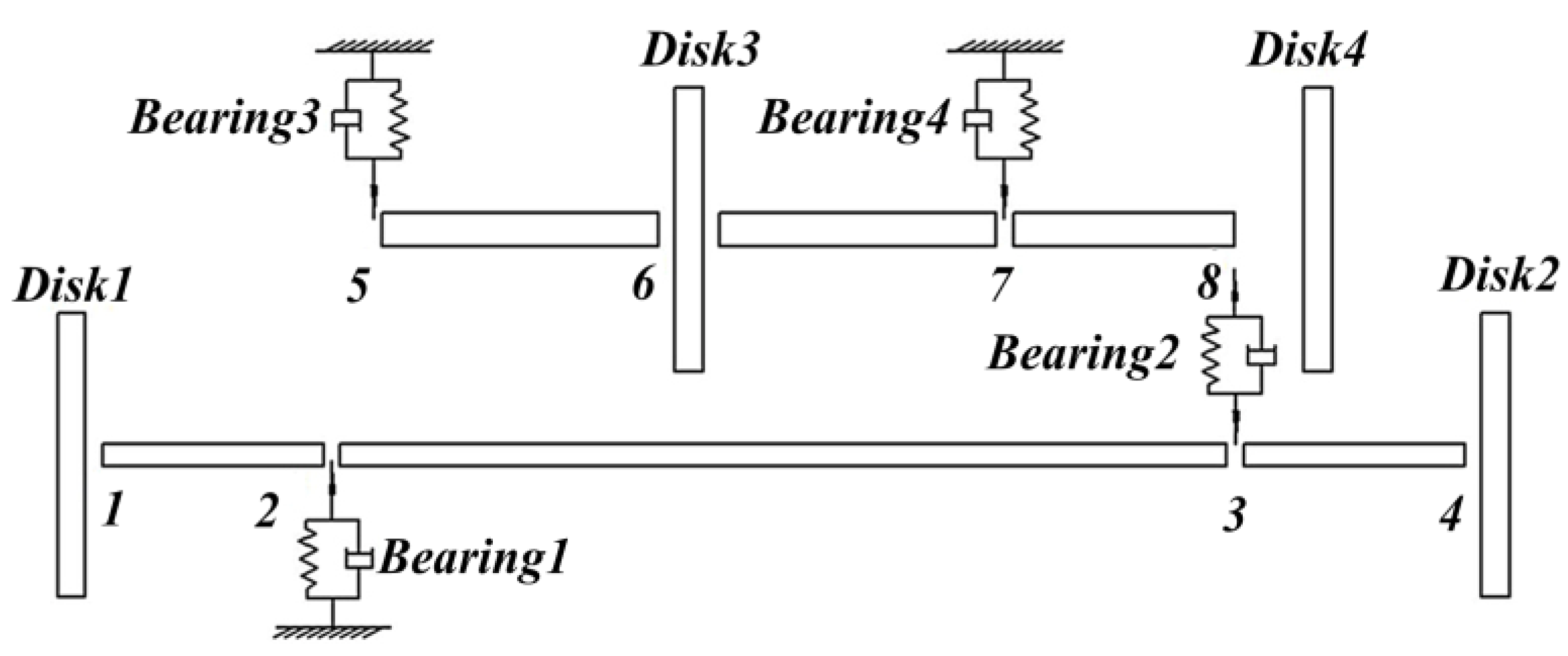
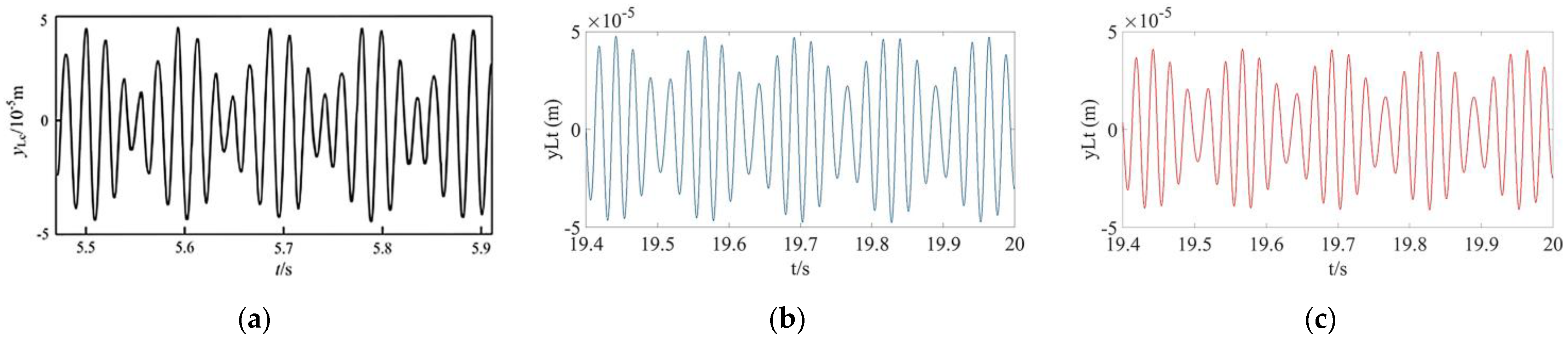
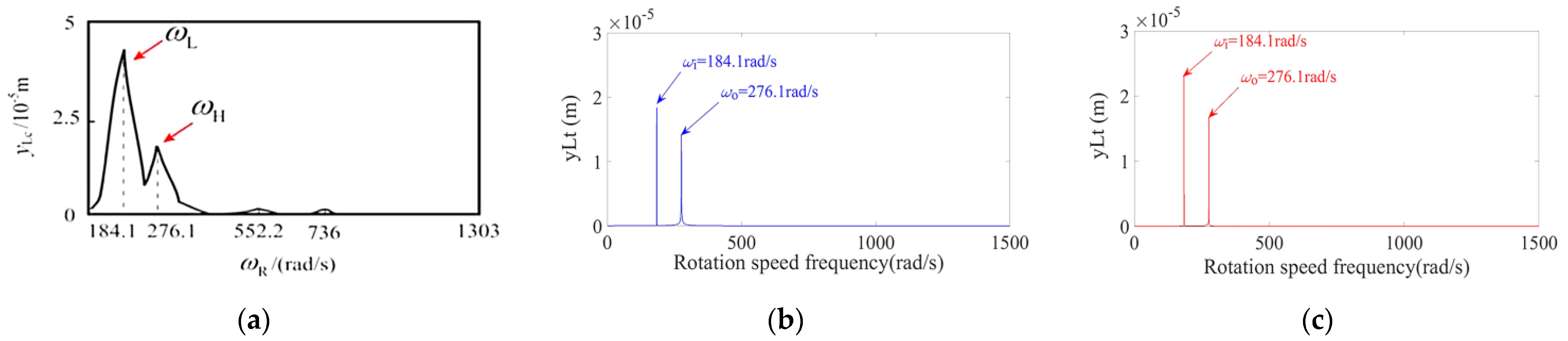
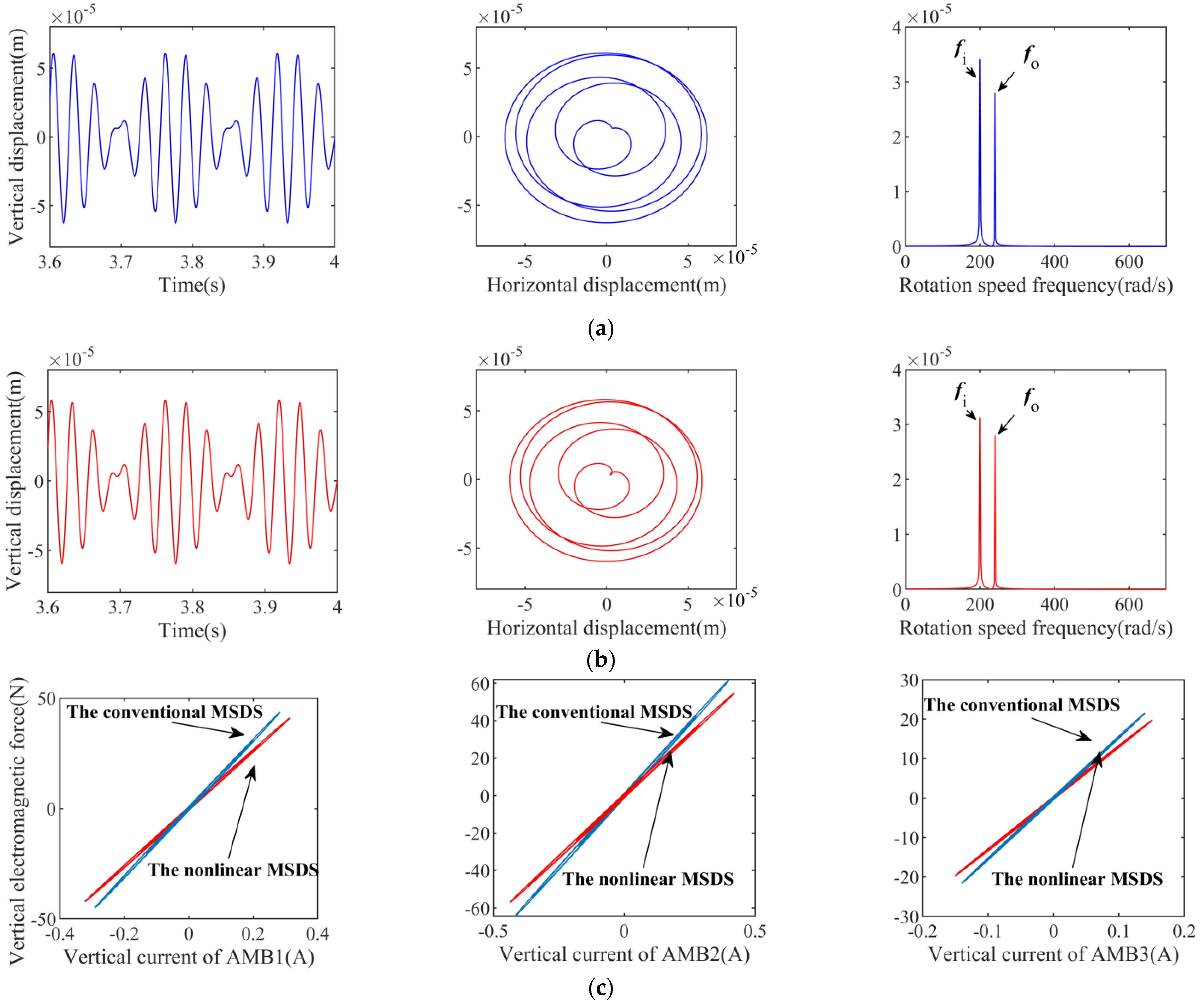


| Physical Parameter | Value | Physical Parameter | Value |
|---|---|---|---|
| Length of inner rotor (m) | 0.706 | Outside and inside radius of disk 2 (m) | 0.125, 0.0125 |
| Length of outer rotor (m) | 0.5011 | Outside and inside radius of disk 3 (m) | 0.125, 0.02 |
| Outside and inside radius of inner rotor (m) | 0.0125, 0.0075 | Outside and inside radius of disk 4 (m) | 0.125, 0.02 |
| Outside and inside radius of outer rotor (m) | 0.02, 0.015 | Thickness of disk (m) | 0.0273 |
| Density of rotating shaft (kg/m3) | 7850 | Density of disk (kg/m3) | 7928.56 |
| Elastic modulus (Pa) | 2.1 × 1011 | Eccentric distance of disk 2 and disk 4 (m) | 6 × 10−5, 5 × 10−5 |
| Poisson’s ratio | 0.3 | Inter-shaft bearing stiffness (N/m) | 1 × 106 |
| Outside and inside radius of disk 1 (m) | 0.125, 0.0125 | Inter-shaft bearing damping (N·s/m) | 100 |
| Name | Parameters | Name | Parameters |
|---|---|---|---|
| Theoretical value of maximum bearing capacity Fmax (N) | 500 | Inner diameter of the stator D (mm) | 50 |
| Theoretical value of saturated magnetic flux density Bpmax (T) | 1.3 | The width of the pole WP (mm) | 10 |
| g0 (mm) | 0.5 | The height of the pole h (mm) | 23 |
| Coil turn N | 172 | The width of the stator yoke Wy (mm) | 12 |
| Ib (A) | 3 | Axial length of the stator La (mm) | 42 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, N.; Liu, M.; Yao, J.; Ge, P.; Wu, H. Numerical Study on Unbalance Response of Dual-Rotor System Based on Nonlinear Bearing Characteristics of Active Magnetic Bearings. Actuators 2023, 12, 86. https://doi.org/10.3390/act12020086
Wang N, Liu M, Yao J, Ge P, Wu H. Numerical Study on Unbalance Response of Dual-Rotor System Based on Nonlinear Bearing Characteristics of Active Magnetic Bearings. Actuators. 2023; 12(2):86. https://doi.org/10.3390/act12020086
Chicago/Turabian StyleWang, Nianxian, Mingzheng Liu, Junfu Yao, Pingping Ge, and Huachun Wu. 2023. "Numerical Study on Unbalance Response of Dual-Rotor System Based on Nonlinear Bearing Characteristics of Active Magnetic Bearings" Actuators 12, no. 2: 86. https://doi.org/10.3390/act12020086
APA StyleWang, N., Liu, M., Yao, J., Ge, P., & Wu, H. (2023). Numerical Study on Unbalance Response of Dual-Rotor System Based on Nonlinear Bearing Characteristics of Active Magnetic Bearings. Actuators, 12(2), 86. https://doi.org/10.3390/act12020086






