Research on the Residual Vibration Suppression of Delta Robots Based on the Dual-Modal Input Shaping Method
Abstract
1. Introduction
2. Model of the Delta Robot
3. Elastic Dynamics Modeling and Modal Analysis
3.1. Elastic Dynamic Modeling
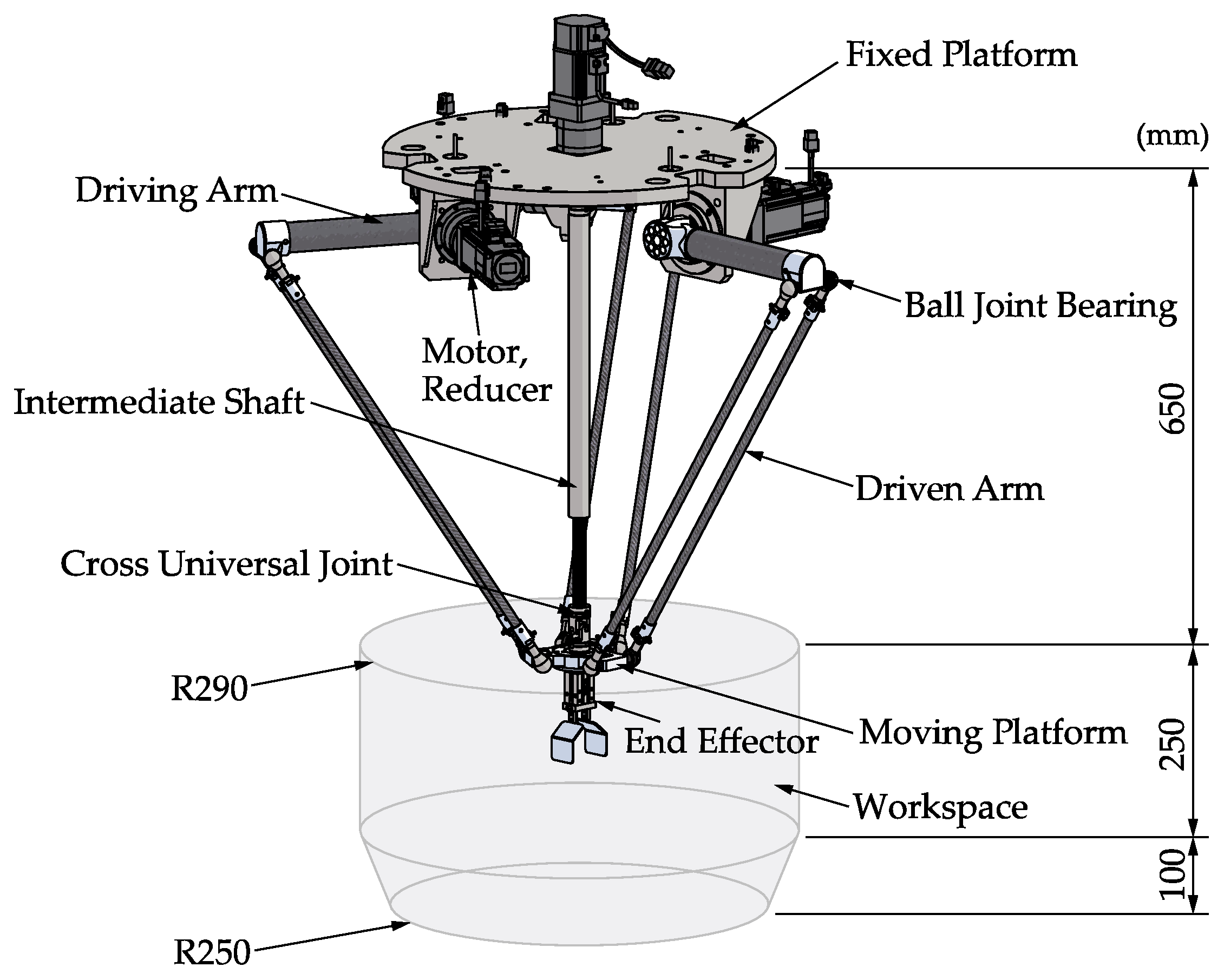
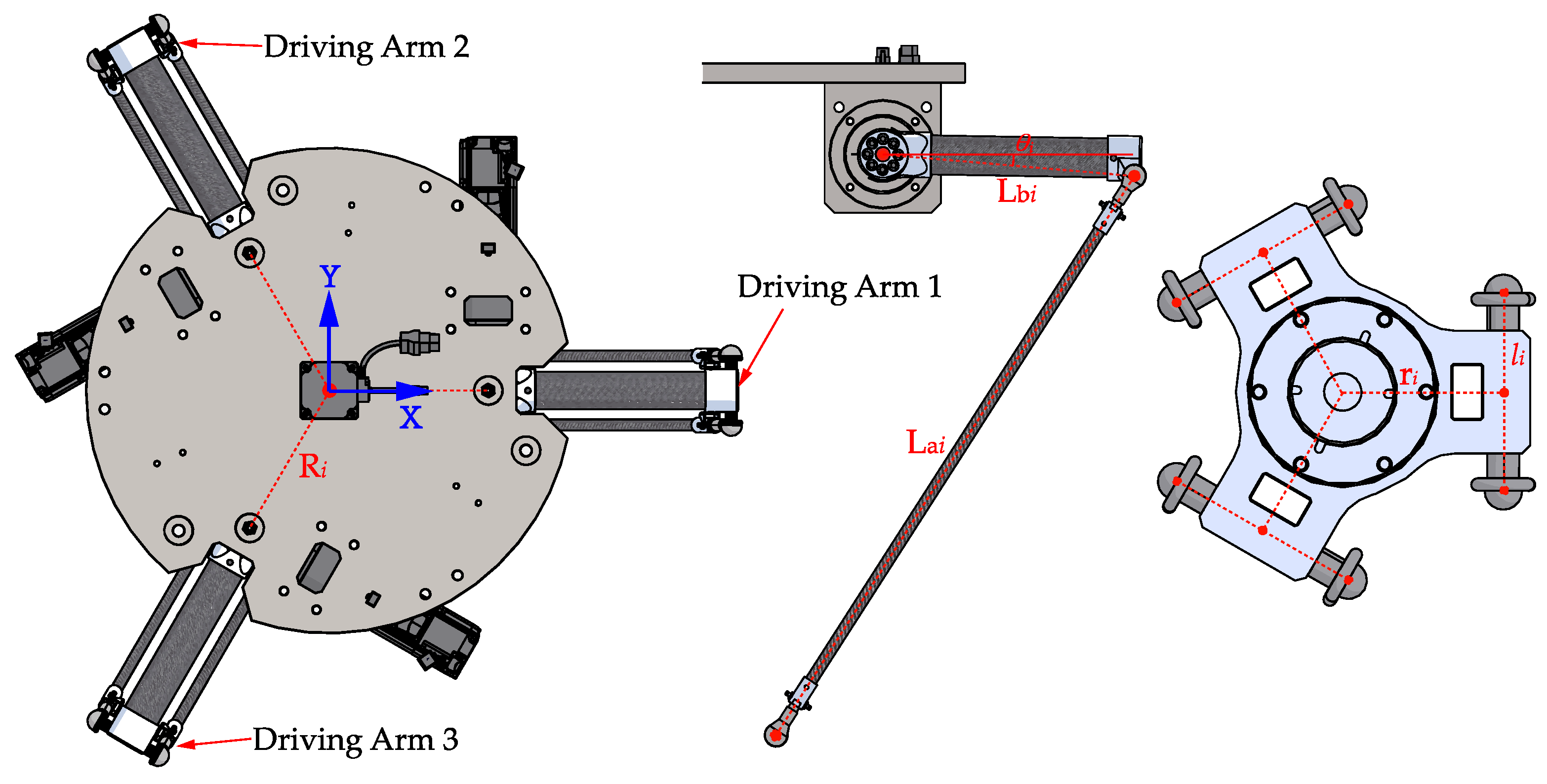
- Establish a parametric geometric model of the Delta robot according to actual needs, as shown in Figure 1.
- Take the Delta robot model shown in Figure 1 as the verification object, simplify its geometric model, and import it into ANSYS workbench in the x_t standard format.
- Import the component features (material and unit type) and connection features (joint contact type/fixed support) into the finite element software, perform mesh division, and establish the finite element model of the robot.
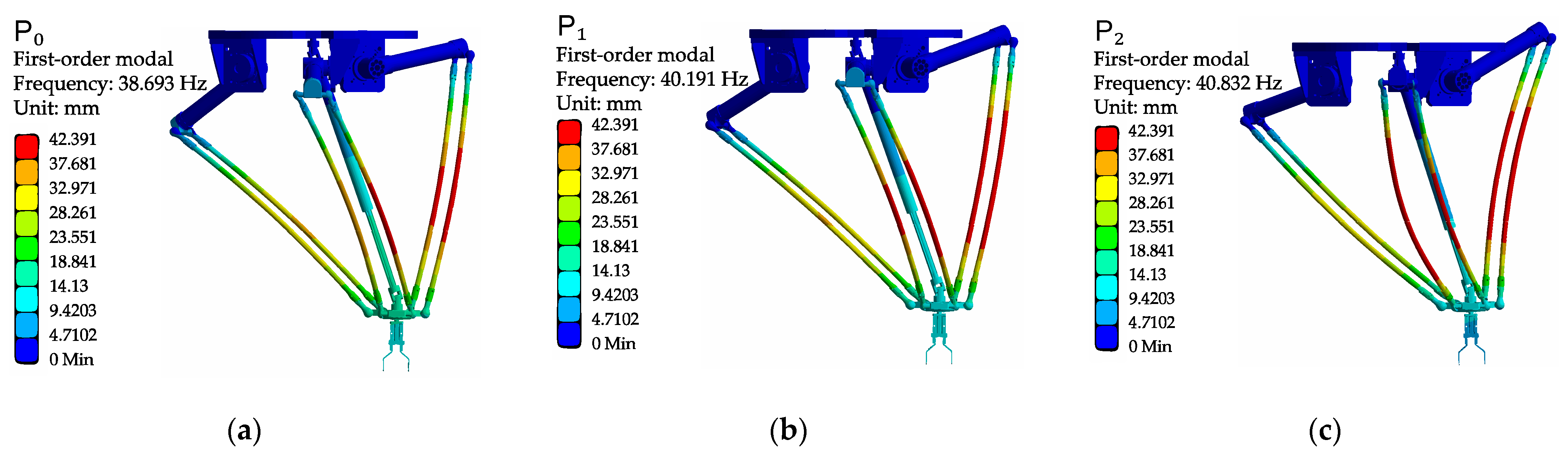
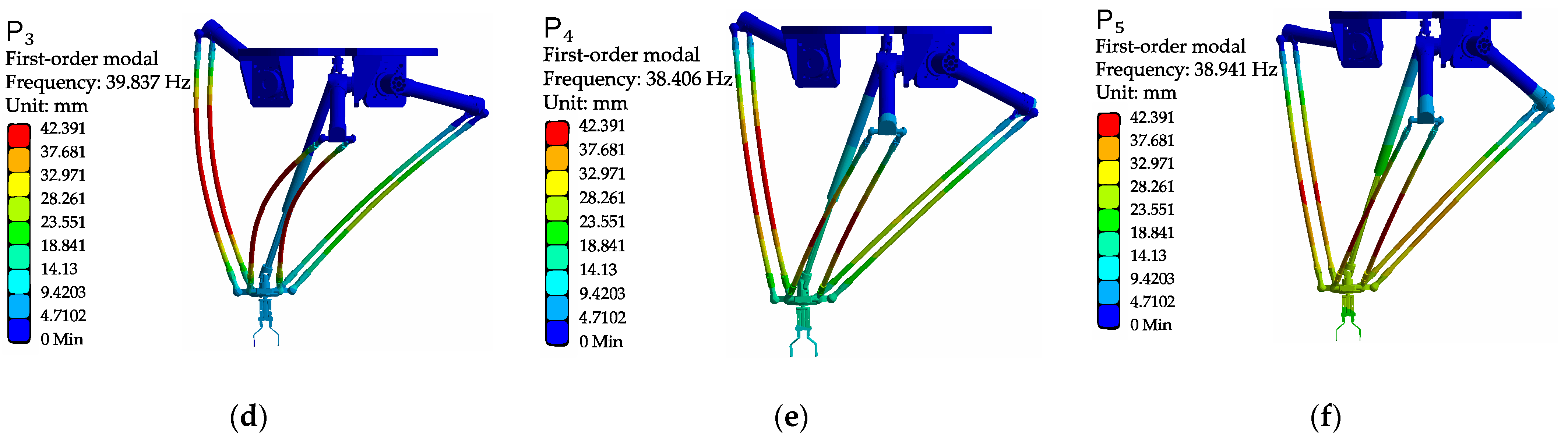
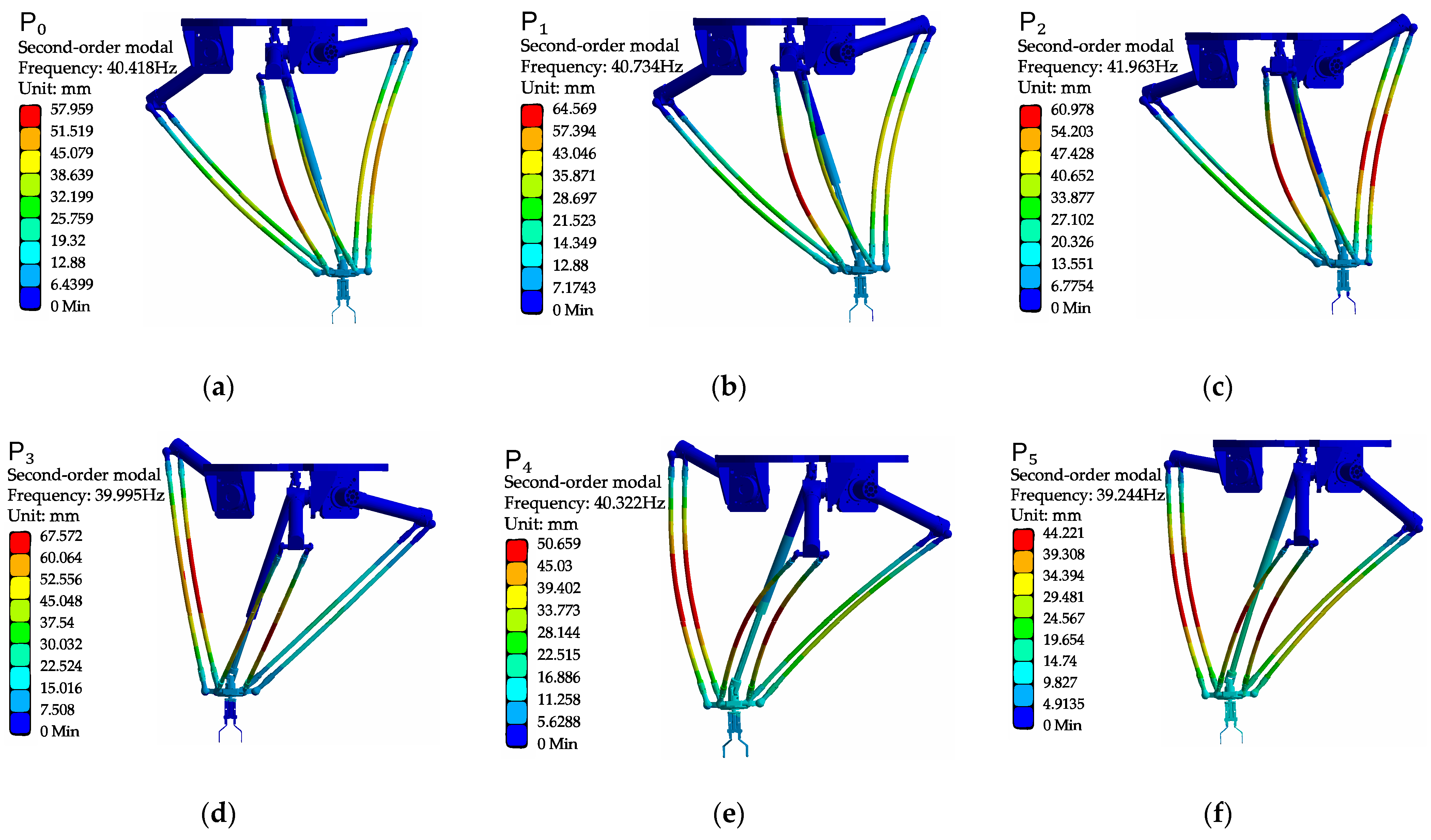
3.2. Delta Robot Modal Analysis
4. Design of the Input Shaping Controller
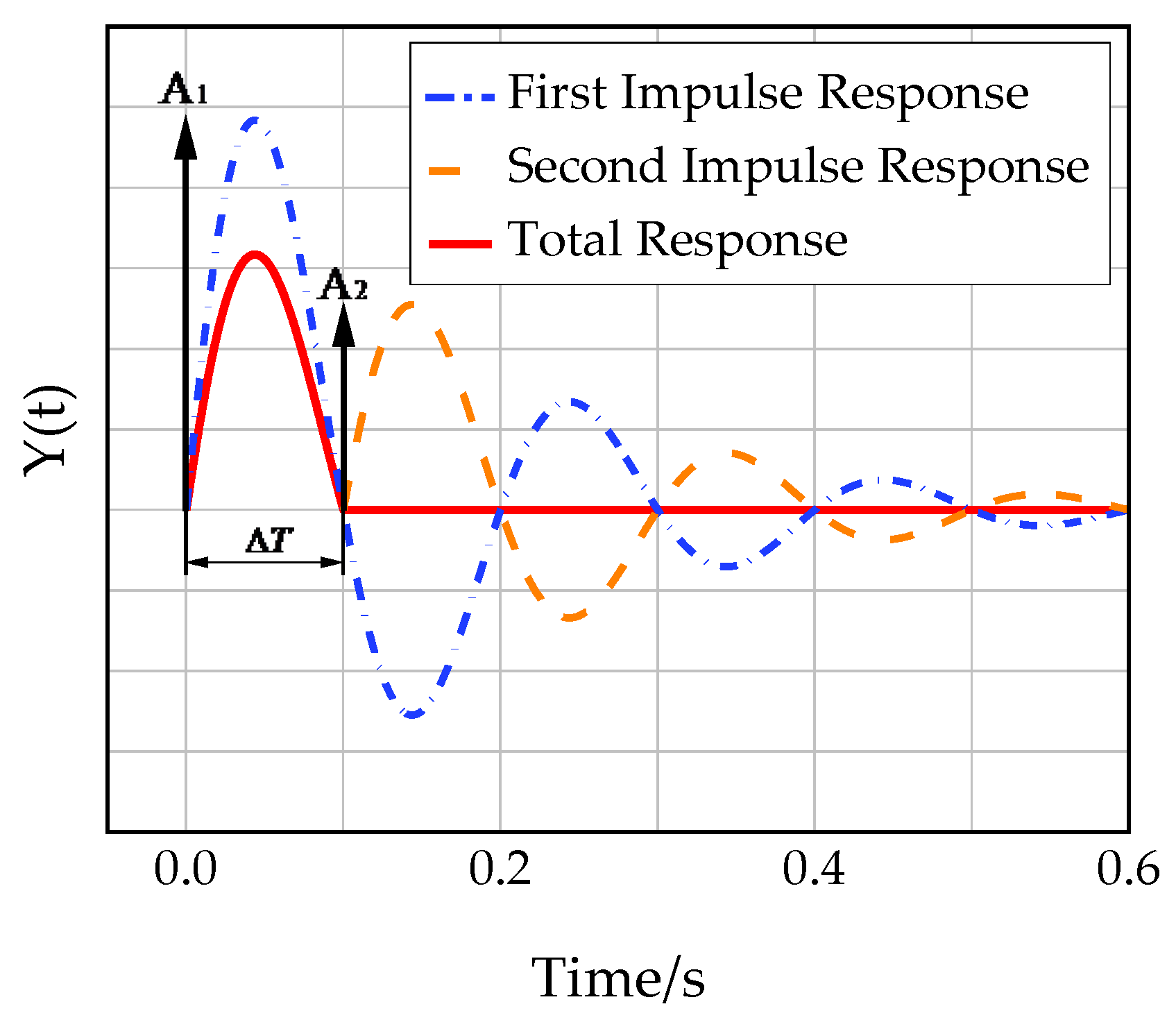
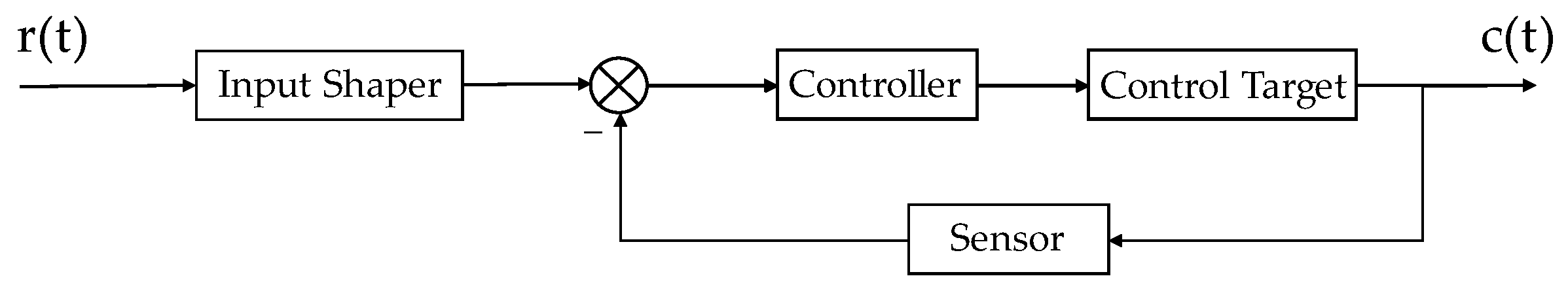
4.1. Principle of Input Shaping Technology
4.2. Construction of a Single-Modal Input Shaper
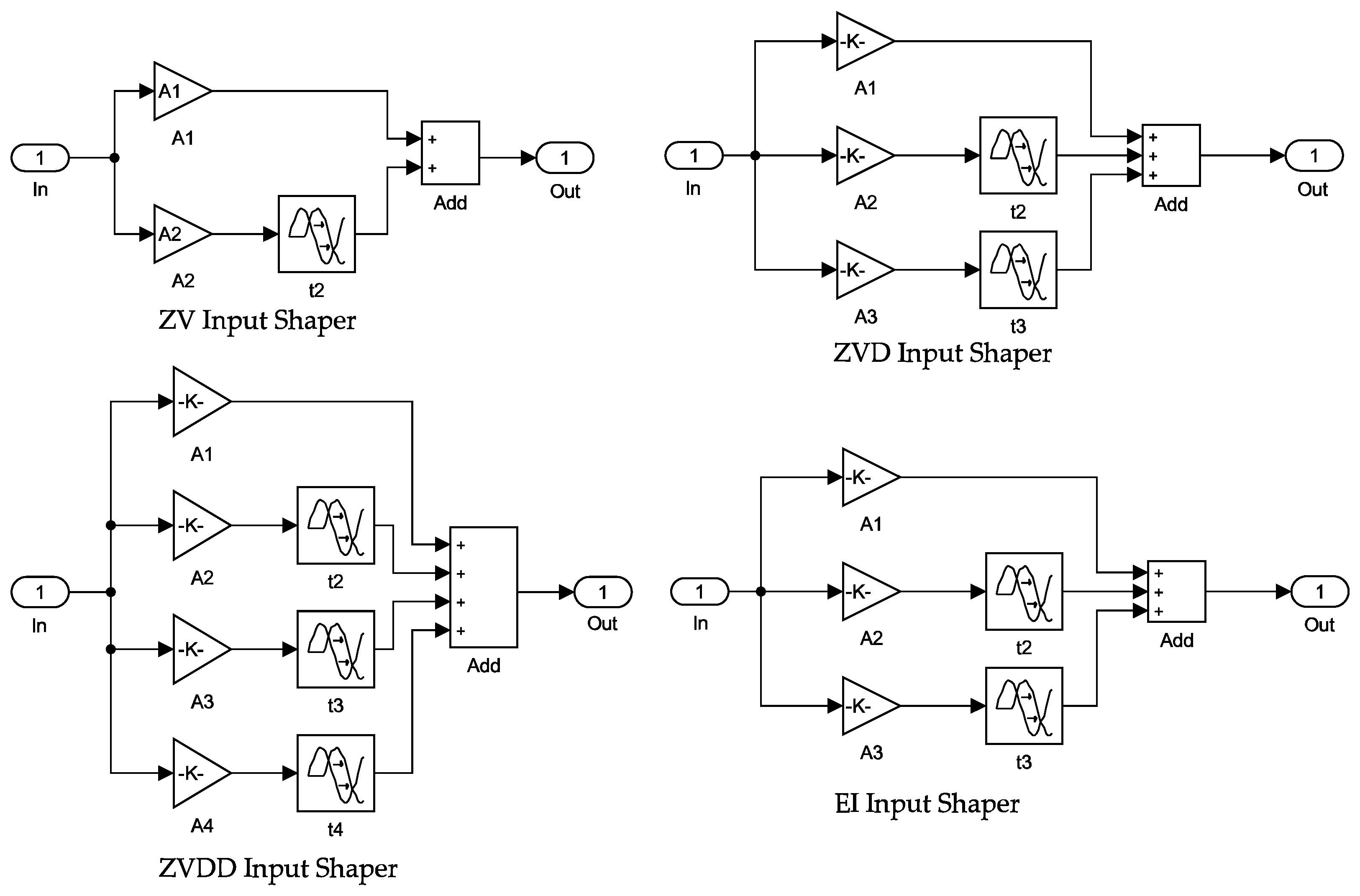
4.2.1. ZV Input Shaper
4.2.2. ZVD Input Shaper
4.2.3. ZVDD Input Shaper
4.2.4. EI Input Shaper
4.3. Simulink Implementation of a Single-Modal Input Shaper
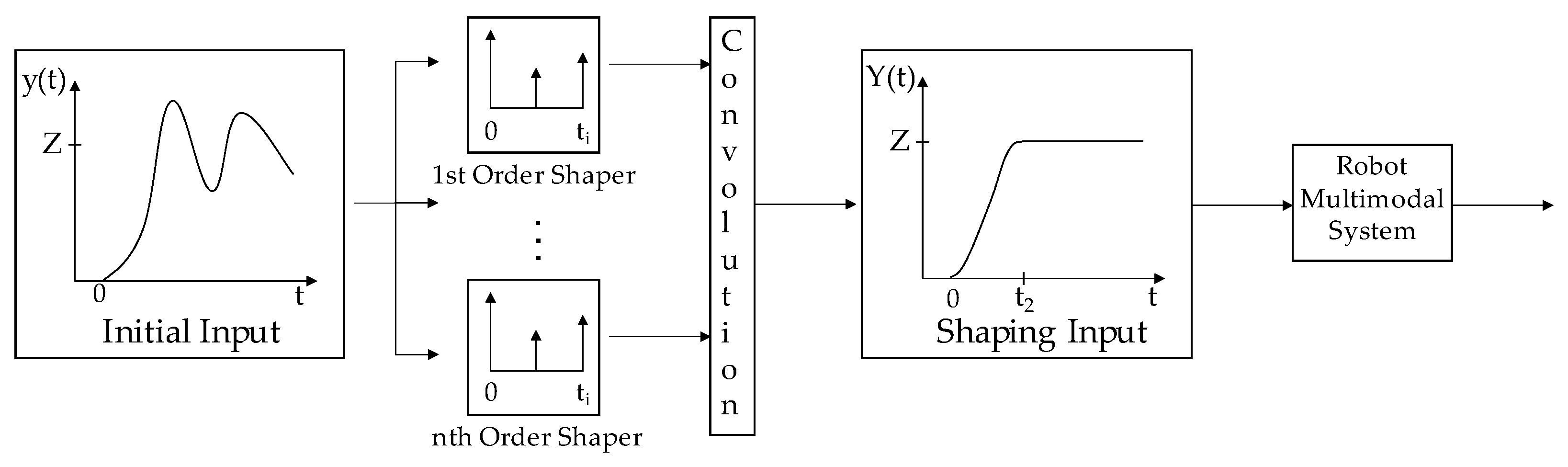
4.4. Multimodal Input Shaper
4.5. Design of a Dual-Modal Input Shaper
5. Simulation and Analysis
5.1. Design of a Simulink Block Diagram
5.2. Robustness Analysis of the Dual-Modal Input Shaper
5.3. Verification of the ZVD-EI Dual-Modal Input Shaper
5.4. Selection Principles of Dual-Modal Input Shapers
6. Conclusions
- (1)
- A design method of a dual-modal input shaper was presented to solve the poor effect of the traditional input shaper on residual vibration suppression caused by the change of the dynamic characteristics of the Delta robot in its workspace. Through simulation analysis, it is found that among the six dual-modal input shapers established in this paper, the ZVD-EI dual-modal input shaper has the best robustness and residual vibration suppression performance.
- (2)
- The simulation verification in Simulink shows that compared with the traditional ZVD single-modal input shaper, when the system modal parameters are accurate, the adjustment time of the ZVD-EI dual-mode input shaper is reduced by about 24%; when the parameter error value is 10%, the adjustment time of the ZVD-EI dual-modal input shaper is reduced by about 17%. Compared with other input shapers, the ZVD-EI dual-modal input shaper has better robustness and comprehensive performance and can effectively reduce the residual vibration of the Delta robot.
- (3)
- By analyzing the usage principles of the established dual-modal input shaper, different dual-modal input shapers in different robots and industrial equipment can be selected for residual vibration suppression according to their system characteristics. In the future, the ZVD-EI dual-modal input shaper algorithm will be applied to the Delta robot prototype to further improve its residual vibration suppression performance and make this method apply better to actual engineering.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Clavel, R. DELTA, a fast robot with parallel geometry. In Proceedings of the 18th International Symposium on Industrial Robots, Lausanne, Switzerland, 26−28 April 1988; pp. 91–100. [Google Scholar]
- Carabin, G.; Scalera, L.; Wongratanaphisan, T.; Vidoni, R. An energy-efficient approach for 3D printing with a Linear Delta Robot equipped with optimal springs. Robot. Comput. Integr. Manuf. 2021, 67, 102045. [Google Scholar] [CrossRef]
- Scalera, L.; Boscariol, P.; Carabin, G.; Vidoni, R.; Gasparetto, A. Enhancing energy efficiency of a 4-DOF parallel robot through task-related analysis. Machines 2020, 8, 10. [Google Scholar] [CrossRef]
- Wu, M.; Mei, J.; Zhao, Y.; Niu, W. Vibration reduction of delta robot based on trajectory planning. Mech. Mach. Theory 2020, 153, 104004. [Google Scholar] [CrossRef]
- Cheng, H.; Li, W. Reducing the Frame Vibration of Delta Robot in Pick and Place Application: An Acceleration Profile Optimization Approach. Shock Vib. 2018, 2018, 2945314. [Google Scholar] [CrossRef]
- Dai, Z.; Sheng, X.; Hu, J.; Wang, H.; Zhang, D. Design and implementation of Bézier curve trajectory planning in DELTA parallel robots. In Proceedings of the International Conference on Intelligent Robotics and Applications, Portsmouth, UK, 24–27 August 2015; Springer International Publishing: Cham, Switzerland, 2015; pp. 420–430. [Google Scholar]
- Zu, Q.; Liu, Q.; Wu, J. Dynamic Pick and Place Trajectory of Delta Parallel Manipulator. In Proceedings of the 6th International Conference on Human Centered Computing, Virtual Event, 14–15 December 2020; Springer International Publishing: Cham, Switzerland, 2021; pp. 1–11. [Google Scholar]
- Ni, J.; Mei, J.; Ding, Y.; Yu, D.; Duan, Y.; Le, Y. A trajectory planning approach for Delta robots considering both motion smoothness and dynamic stress. J. Mech. Robot. 2022, 15, 041012. [Google Scholar] [CrossRef]
- Zhao, R.; Wu, L.; Chen, Y.H. Robust Control for Nonlinear Delta Parallel Robot With Uncertainty: An Online Estimation Approach. IEEE Access 2020, 8, 97604–97617. [Google Scholar] [CrossRef]
- Singer, N.C.; Seering, W.P. Preshaping Command Inputs to Reduce System Vibration. J. Dyn. Syst. Meas. Control 1990, 112, 76–82. [Google Scholar] [CrossRef]
- Chatlatanagulchai, W.; Kijdech, D.; Benjalersyarnon, T.; Damyot, S. Quantitative Feedback Input Shaping for Flexible-Joint Robot Manipulator. J. Dyn. Syst. Meas. Control 2016, 138, 061006. [Google Scholar] [CrossRef]
- Piedrafita, R.; Comín, D.; Beltrán, J.R. Simulink implementation and industrial test of Input Shaping techniques. Control Eng. Pract. 2018, 79, 1–21. [Google Scholar] [CrossRef]
- Yu, Z.; Chen, W.; Te, T.; Tomizuka, M. Zero time delay input shaping for smooth settling of industrial robots. In Proceedings of the 2016 IEEE International Conference on Automation Science and Engineering (CASE), Fort Worth, TX, USA, 21–25 August 2016; pp. 620–625. [Google Scholar]
- Li, Z.; Wang, H.; Zhao, H.; Ding, H. Force impact suppression of contact transition state in robot grinding and polishing of industrial blades. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2022, 236, 7387–7397. [Google Scholar] [CrossRef]
- Ha, M.T.; Kang, C.G. Experimental analysis of natural frequency error to residual vibration in ZV, ZVD, and ZVDD shapers. In Proceedings of the 2013 10th International Conference on Ubiquitous Robots and Ambient Intelligence (URAI), Jeju, Republic of Korea, 30 October–2 November 2013; pp. 195–199. [Google Scholar]
- Masoud, Z.; Alhazza, K. Frequency-modulation input shaping for multimode systems. J. Vib. Control 2014, 22, 3439–3451. [Google Scholar] [CrossRef]
- Thomsen, D.K.; Søe-Knudsen, R.; Balling, O.; Zhang, X. Vibration control of industrial robot arms by multi-mode time-varying input shaping. Mech. Mach. Theory 2021, 155, 104072. [Google Scholar] [CrossRef]
- Singhose, W.; Crain, E.; Seering, W. Convolved and simultaneous two-mode input shapers. IEEE Proc. Control Theory Appl. 1997, 144, 515–520. [Google Scholar] [CrossRef]
- Zheng, K. Research on intelligent vibration suppression control of high-speed lightweight Delta robot. J. Vib. Control 2022, 28, 3042–3057. [Google Scholar] [CrossRef]
- Shan, X.; Li, Y.; Liu, H.; Huang, T. Residual Vibration Reduction of High-Speed Pick-and-Place Parallel Robot Using Input Shaping. Chin. J. Mech. Eng. 2022, 35, 16. [Google Scholar] [CrossRef]
- Wang, H.; Liu, Q.; Ma, Y.; Liu, S. Residual Vibration Suppression of the Diamond Robot Based on the Input Shaping. Mach. Des. Res. 2022, 38, 75–80. (In Chinese) [Google Scholar] [CrossRef]
- Smith, O.J.M. Posicast Control of Damped Oscillatory Systems. Proc. IRE 1957, 45, 1249–1255. [Google Scholar] [CrossRef]
- Hung, J.Y. Feedback control with Posicast. IEEE Trans. Ind. Electron. 2003, 50, 94–99. [Google Scholar] [CrossRef]
- Li, L. Vibration Suppression of Flexible Load System of Robot Based on Actor-Critic Algorithm. Master’s Thesis, University of Chinese Academy of Sciences, Beijing, China, 2020. (In Chinese). [Google Scholar]
- Singer, N.; Singhose, W.; Kriikku, E. An input shaping controller enabling cranes to move without sway. In Proceedings of the American Nuclear Society Topical Meeting on Robotics and Remote Systems, Augusta, GA, USA, 27 April–1 May 1997. [Google Scholar]
- Singhose, W.E.; Seering, W.P.; Singer, N.C. Shaping inputs to reduce vibration: A vector diagram approach. In Proceedings of the IEEE International Conference on Robotics and Automation, Cincinnati, OH, USA, 13–18 May 1990; Volume 922, pp. 922–927. [Google Scholar]
- Li, B.; Xie, L.; Wei, Y.; Zhao, J.; Wang, L. Residual Vibration Suppression of a 3-DOF Parallel Robot Using Multimodal Input Shaping. Machinery 2010, 48, 21–25. [Google Scholar] [CrossRef]






| Description | Notation | Value |
|---|---|---|
| Radius of the fixed platform | 0.17 m | |
| Radius of the moving platform | 0.06 m | |
| Length of the i-th driven arm | 0.68 m | |
| Length of the i-th driving arm | 0.26 m | |
| Width of the branch chain of the i-th driven arm | 0.074 m | |
| Angle of the i-th active joint | −35°~75° |
| Description | Value |
|---|---|
| Shear modulus of elasticity of the driving arm and driven arm | |
| Young’s modulus of the driving arm and driven arm | |
| Poisson coefficient | 0.2 |
| Friction coefficient | 0.11 |
| Mass of the i-th driving arm | 0.693 kg |
| Mass of the i-th driven arm | 0.175 kg |
| Mass of moving platform | 0.52 kg |
| Load mass | 1 kg |
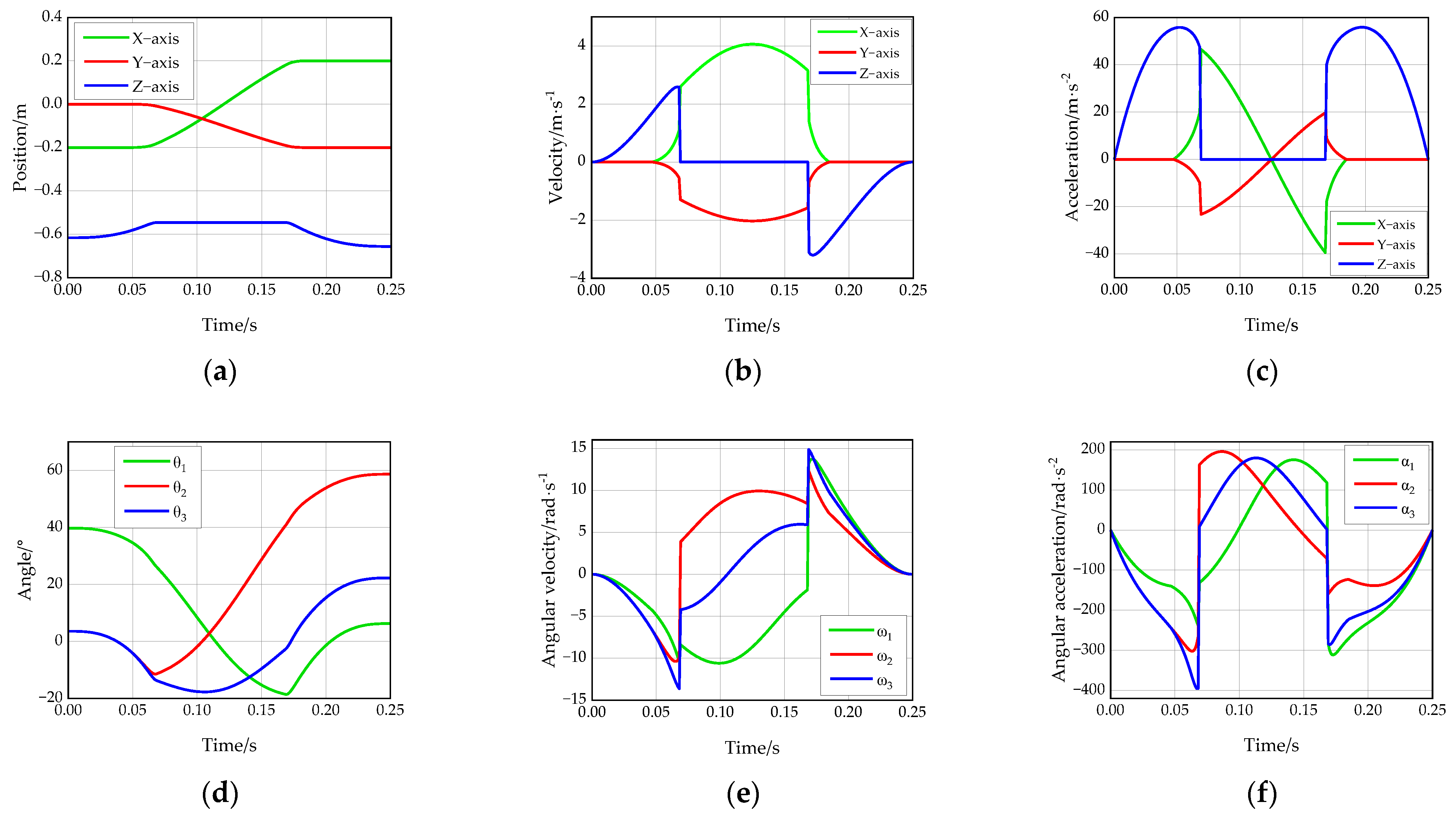
| Position Point | X (mm) | Y (mm) | Z (mm) |
|---|---|---|---|
| P0 | −200 | 0 | −740 |
| P1 | −200 | 0 | −710 |
| P2 | −182 | −9 | −670 |
| P3 | 182 | −191 | −670 |
| P4 | 200 | −200 | −710 |
| P5 | 200 | −200 | −780 |
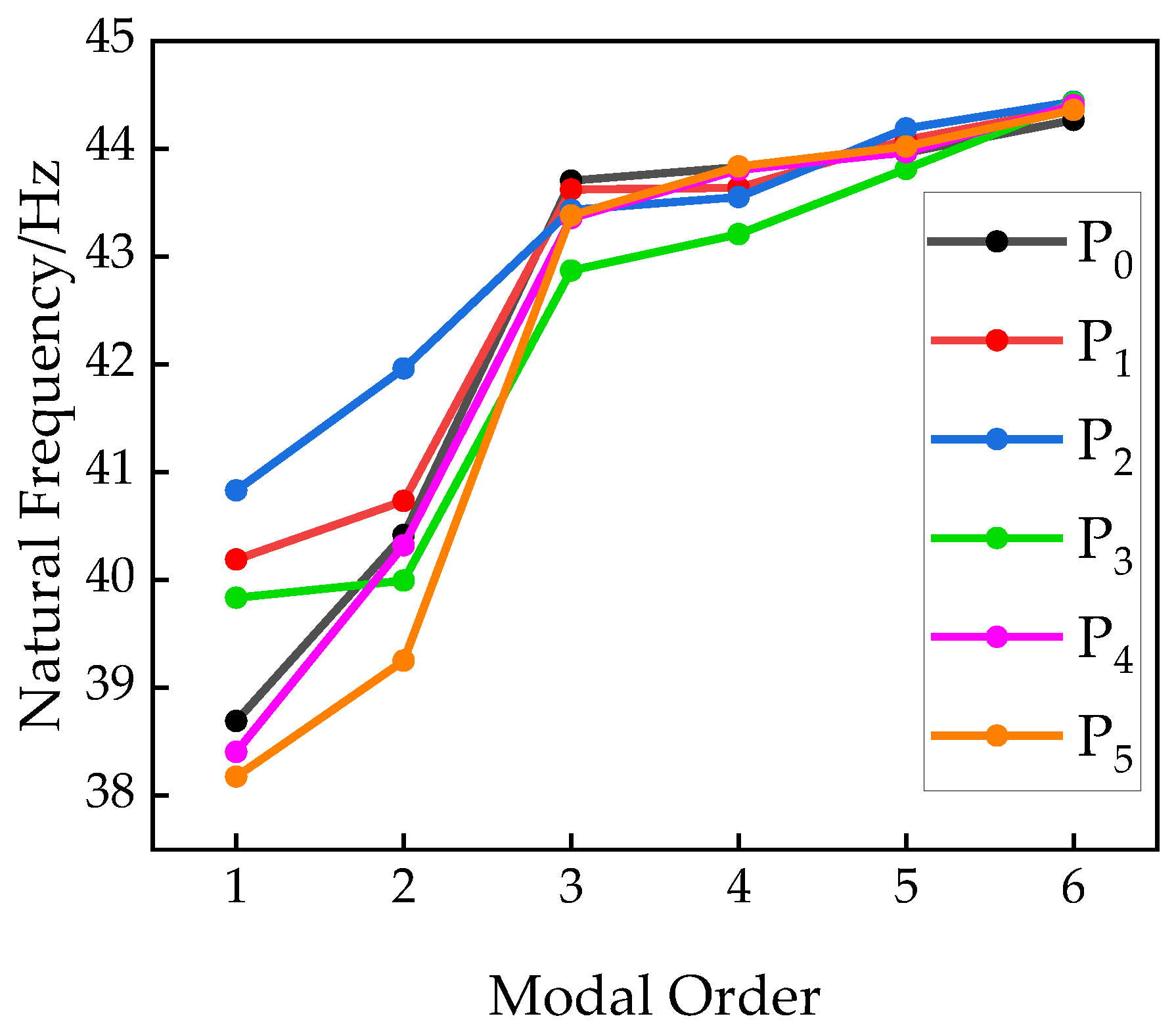
| Order | Maximum Frequency/Hz | Minimum Frequency/Hz | Modal Shape Description |
|---|---|---|---|
| 1 | 40.832 | 38.177 | The moving platform is twisted along the XY plane |
| 2 | 41.963 | 39.253 | The moving platform is twisted along the XY plane |
| 3 | 43.706 | 42.871 | Deflection of a driven arm chain along its parallelogram plane |
| 4 | 43.836 | 43.208 | The deflection deformation of two driven arm chains along their parallelogram planes |
| 5 | 44.19 | 43.813 | The deflection deformation of two driven arm chains perpendicular to their parallelogram plane |
| 6 | 44.437 | 44.268 | Two driven arm chains deflect along its parallelogram plane, and one driven arm chain deflects perpendicularly to its parallelogram plane |
| Input Shaper | Overshoot/% | Adjustment Time/s |
|---|---|---|
| ZV | 12.66 | 0.606 |
| ZVD | 2.28 | 0.156 |
| ZVDD | 0.04 | 0.244 |
| EI(V=5%) | 2.05 | 0.166 |
| ZV-ZV | 11.33 | 0.606 |
| ZV-ZVD | 11.53 | 0.682 |
| ZV-EI(V=5%) | 12.22 | 0.687 |
| ZVD-ZVD | 1.35 | 0.156 |
| ZVD-EI(V=5%) | 0.16 | 0.158 |
| EI(V=5%)-EI(V=5%) | 3.92 | 0.170 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, Z.; Zhang, J.; Zhang, P. Research on the Residual Vibration Suppression of Delta Robots Based on the Dual-Modal Input Shaping Method. Actuators 2023, 12, 84. https://doi.org/10.3390/act12020084
Guo Z, Zhang J, Zhang P. Research on the Residual Vibration Suppression of Delta Robots Based on the Dual-Modal Input Shaping Method. Actuators. 2023; 12(2):84. https://doi.org/10.3390/act12020084
Chicago/Turabian StyleGuo, Zhongfeng, Jianqiang Zhang, and Peisen Zhang. 2023. "Research on the Residual Vibration Suppression of Delta Robots Based on the Dual-Modal Input Shaping Method" Actuators 12, no. 2: 84. https://doi.org/10.3390/act12020084
APA StyleGuo, Z., Zhang, J., & Zhang, P. (2023). Research on the Residual Vibration Suppression of Delta Robots Based on the Dual-Modal Input Shaping Method. Actuators, 12(2), 84. https://doi.org/10.3390/act12020084





