Improvement in Position Response of Laser Focus Controlled Magnetic Actuator Based on Mixed Sensitivity Robust Control
Abstract
1. Introduction
2. Structural and Mathematical Models
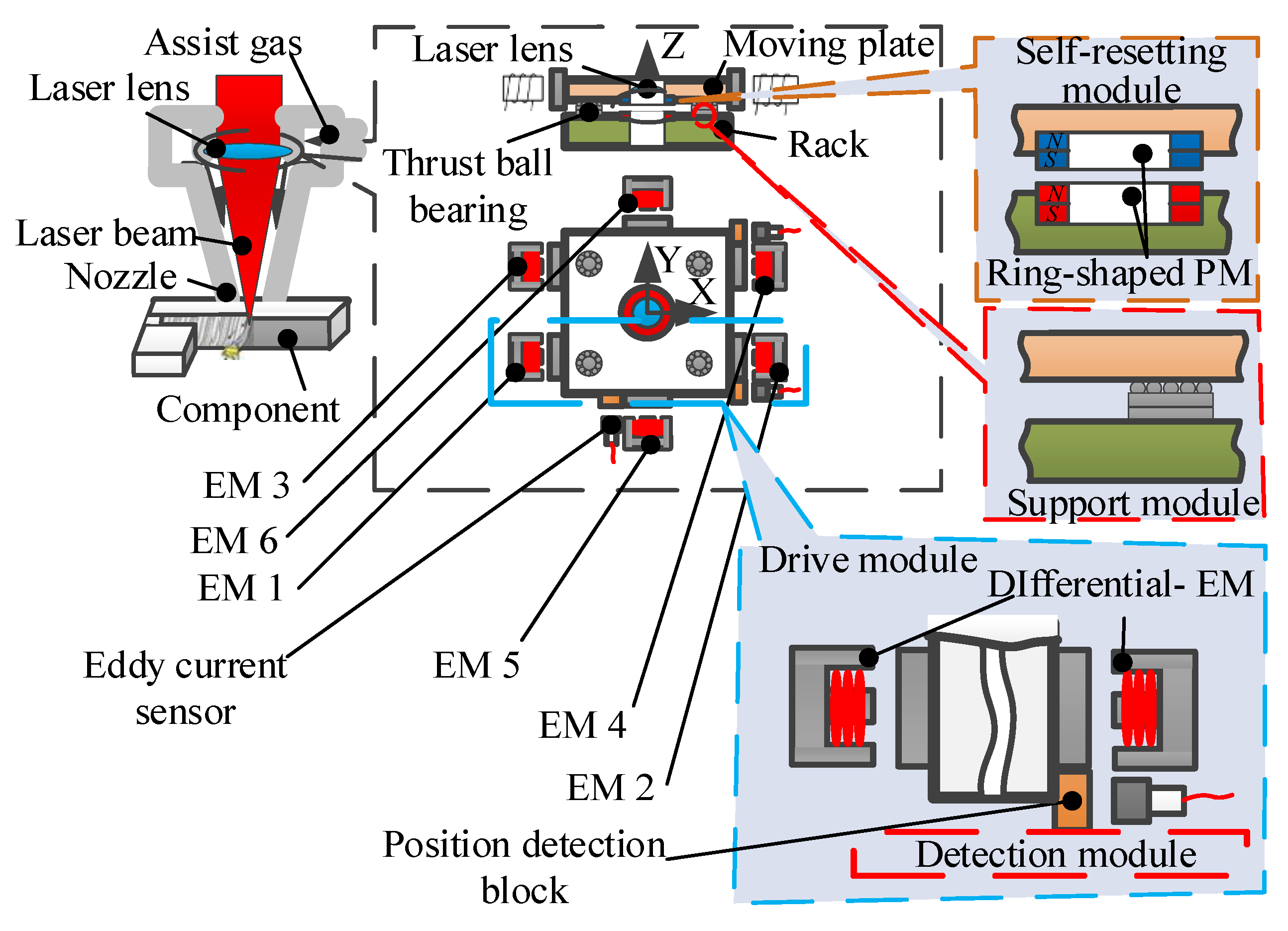
2.1. Structure of the Actuator
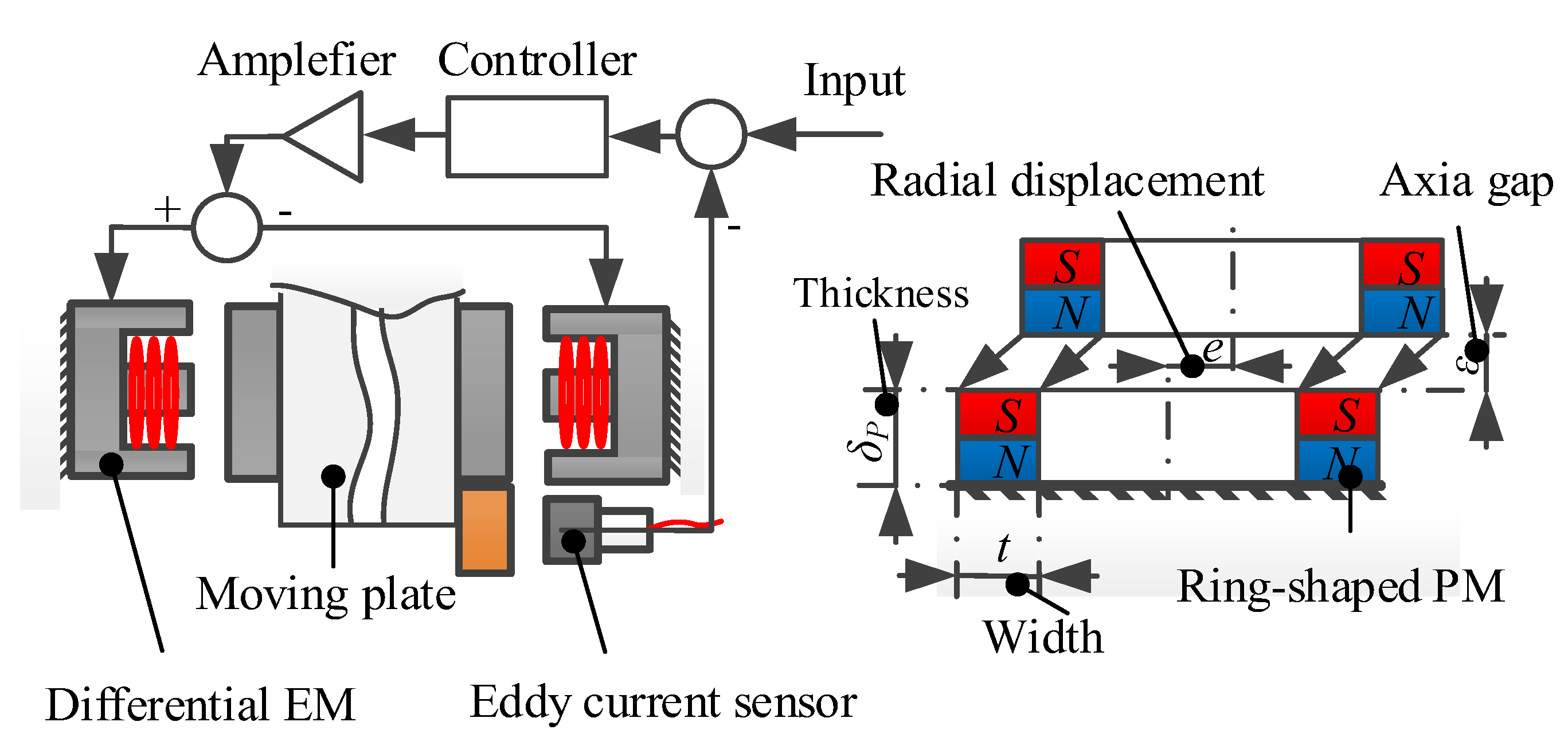
2.2. The Principle of the Actuator
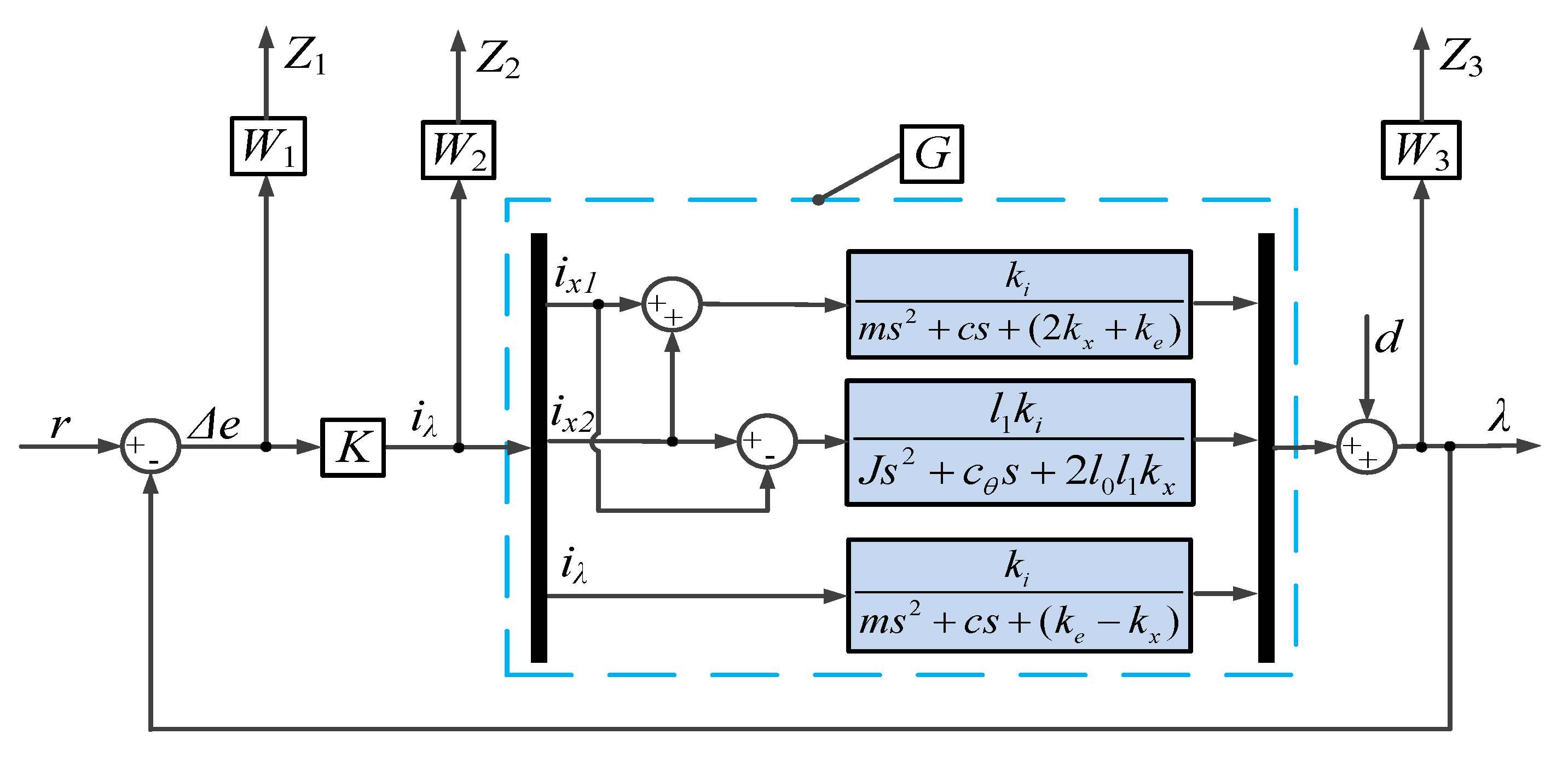
2.3. The Mathematical Model of the Actuator
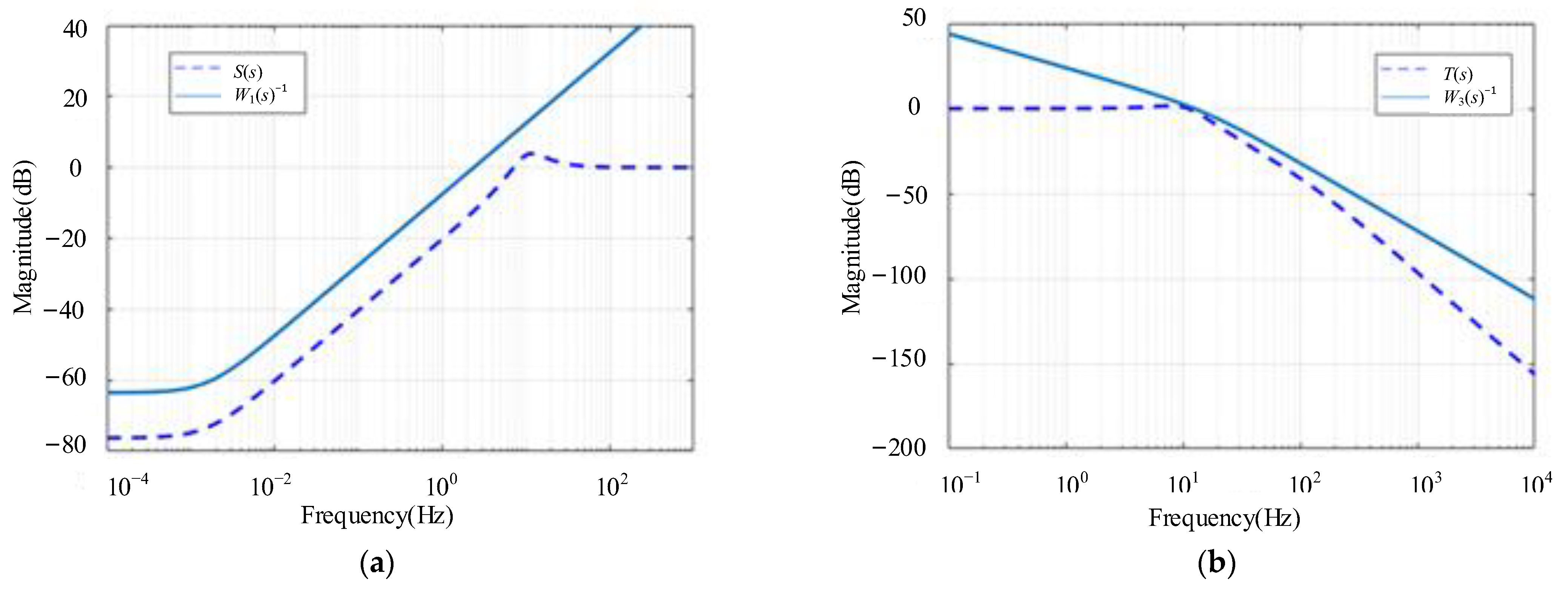
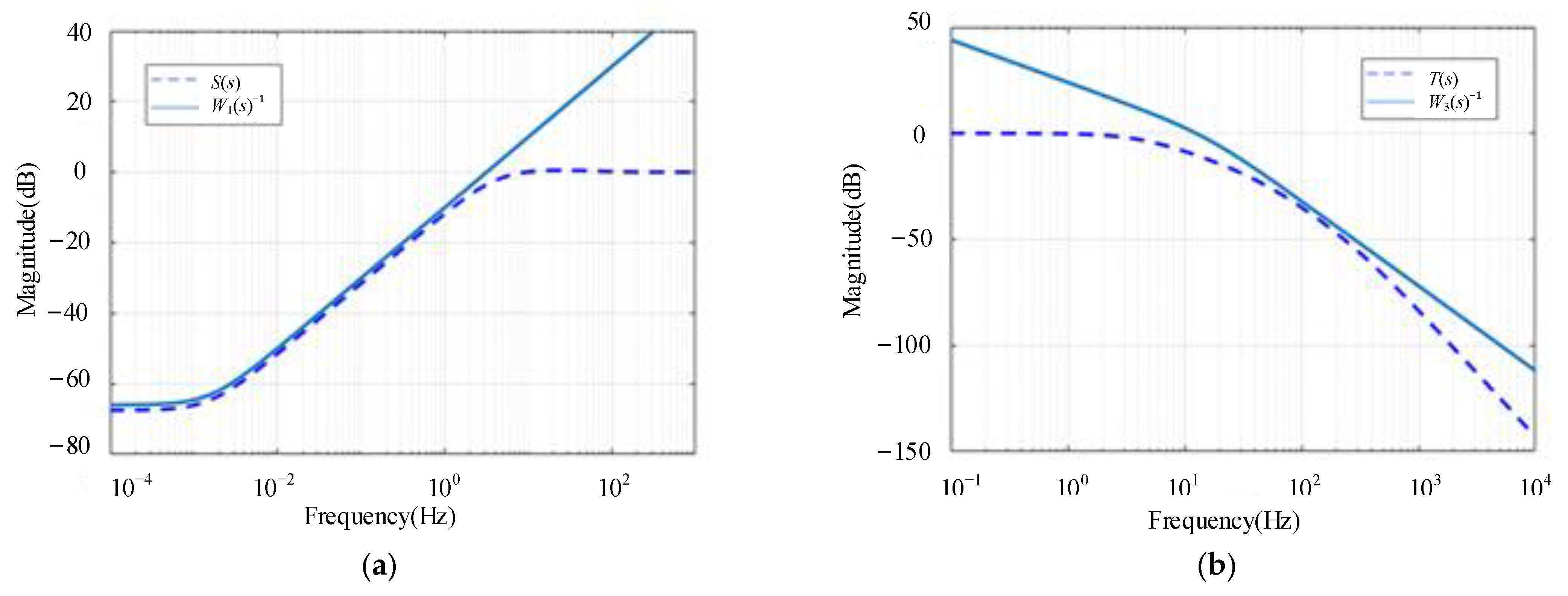
3. Mixed Sensitivity Robust Controller Design
4. Simulation Analysis
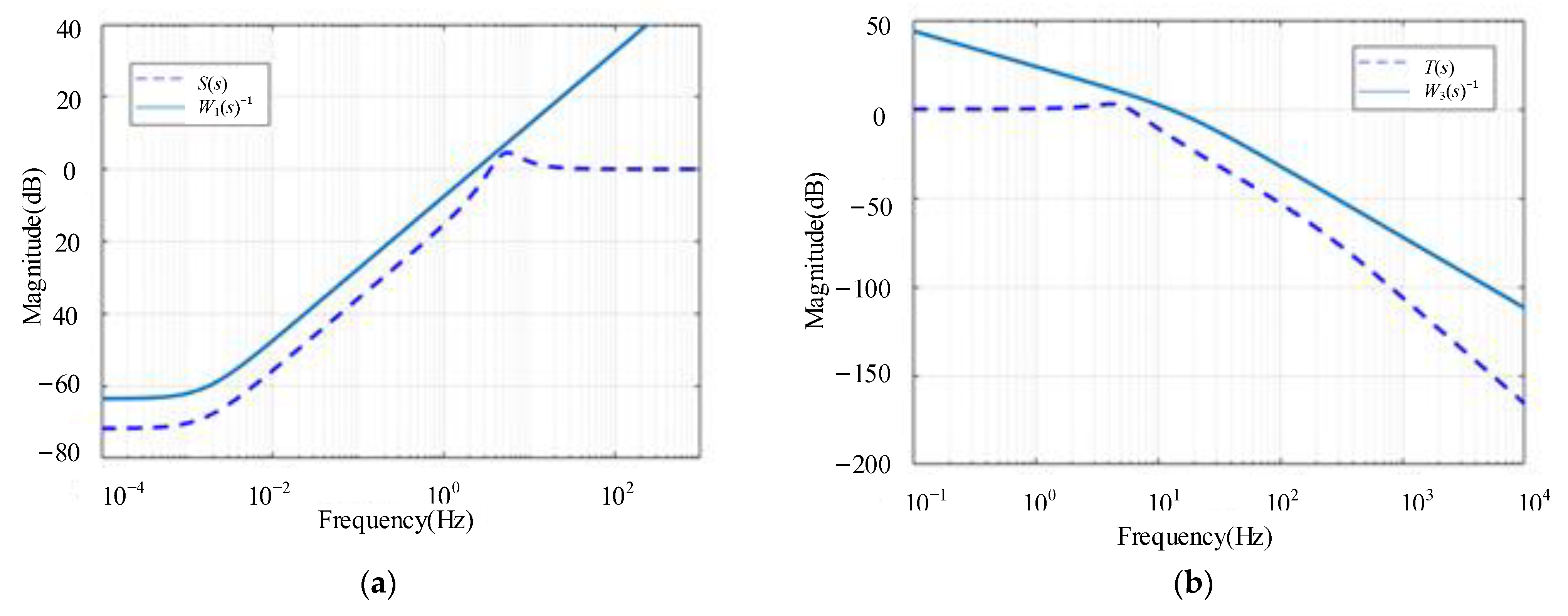
4.1. Analysis of the Performance of the Control system
4.1.1. Analysis of the Performance of the Y Direction Control System
4.1.2. Analysis of the Performance of the X Direction Control System
4.1.3. Analysis of the Performance of the θ Direction Control System
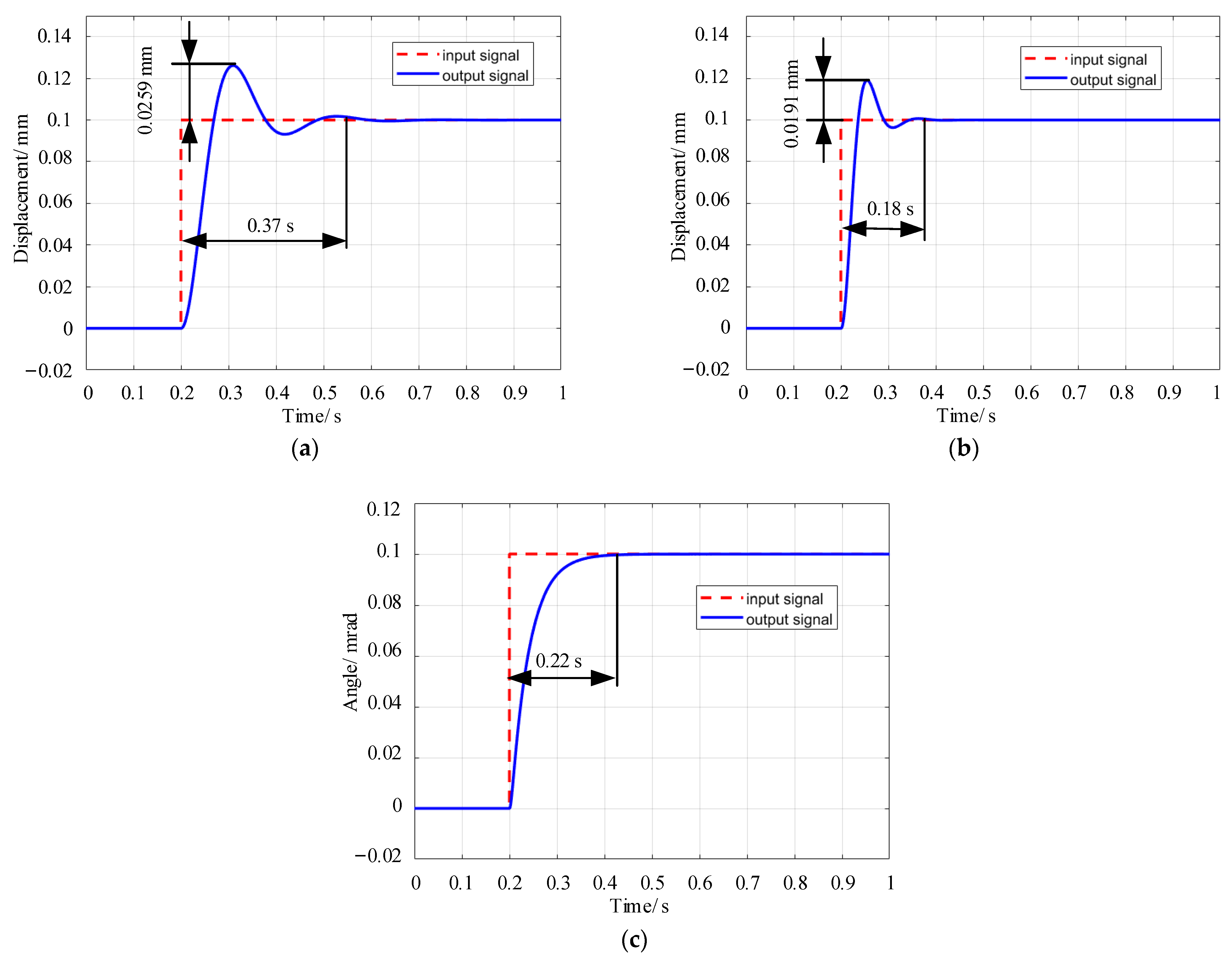
4.2. Analysis of the System Response of the Standard Model
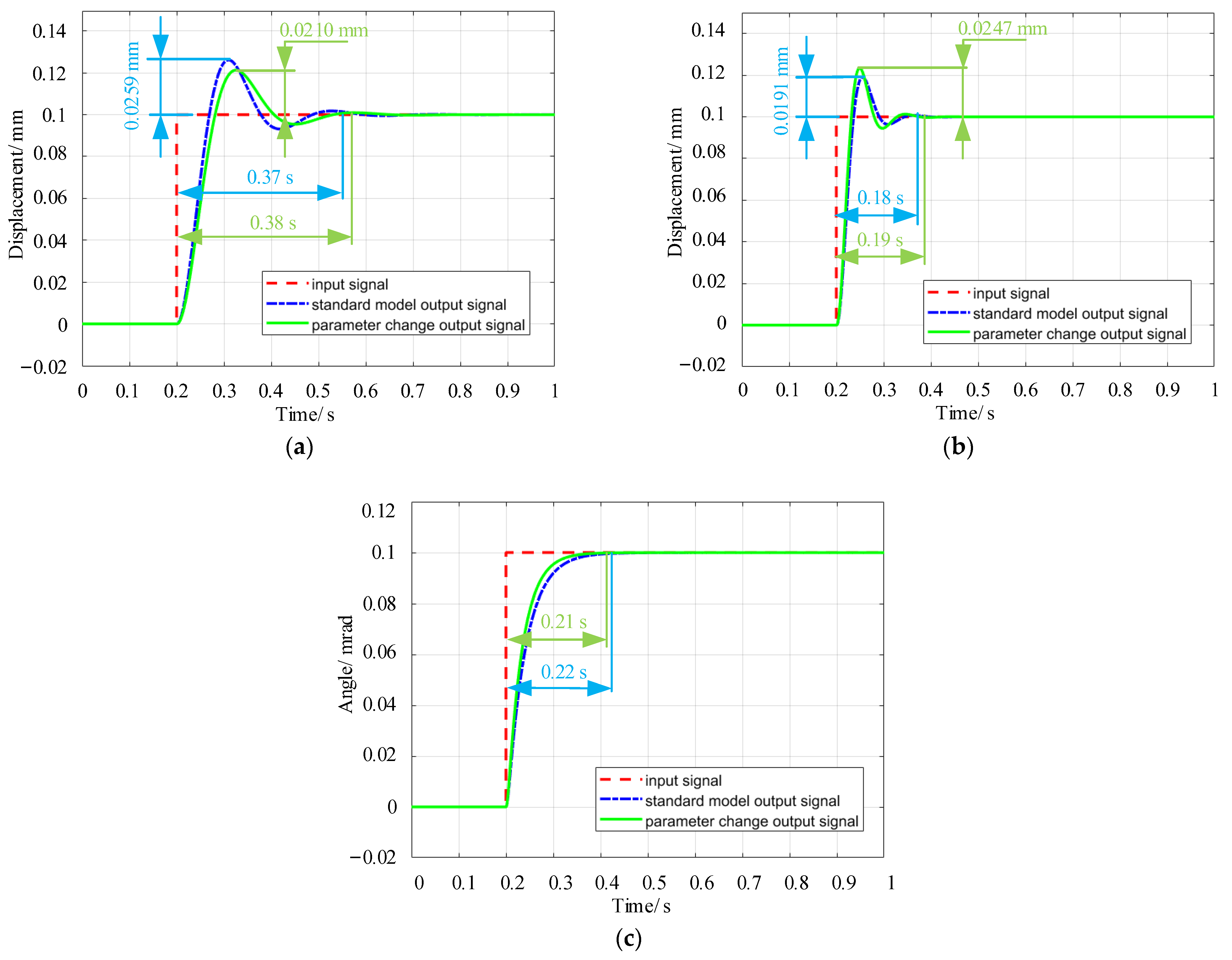
4.3. Analysis of System Response to Parameter Disturbance
4.4. Analysis of System Response to Impulse External Disturbance
5. Experimental Verification
5.1. Experimental Equipment
5.2. Experimental Results
6. Conclusions
- 1.
- The mixed sensitivity controller of the system is calculated by a reasonable selection of the weighting function, and the simulation analysis of the position step response in Y, X, and θ directions is carried out, and the steady-state errors are all 0. It shows that the designed controller has good position response characteristics.
- 2.
- Based on the standard model, 20% parameter variation is introduced, and the original controller simulates and analyzes the system. In the simulation of position step response in Y, X, and θ directions, the response characteristics are consistent with the results of the standard model. There are slight differences in overshoot and adjustment time between the above, and the steady-state error is 0. It shows that the system has better position response characteristics when the model is inaccurate.
- 3.
- When the external pulse disturbance is added to the system, the steady-state values of Y, X, and θ can be quickly restored in the position step experiment. It shows that the controller has good anti-interference properties.
- 4.
- There is a coupling phenomenon in the direction of X and θ, so both show certain coupling characteristics in the step response experiment. Still, the moving plate can be quickly adjusted to the steady-state value. The steady-state errors in the three directions are all less than 25%, which meets the requirement of machine tool accuracy of 0.025 mm.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- He, Y.; Xie, H.; Ge, Y.; Lin, Y.; Yao, Z.; Wang, B.; Jin, M.; Liu, J.; Chen, X.; Sun, Y. Laser Cutting Technologies and Corresponding Pollution Control Strategy. Processes 2022, 10, 732. [Google Scholar] [CrossRef]
- Singh, Y.; Singh, J.; Sharma, S.; Sharma, A.; Chohan, J. Process parameter optimization in laser cutting of Coir fiber reinforced Epoxy composite—A review. Mater. Today Proc. 2021, 48, 1021–1027. [Google Scholar] [CrossRef]
- Sim, M.; Lee, C. Determination of optimal laser power according to the tool path inclination angle of a titanium alloy workpiece in laser-assisted machining. Int. J. Adv. Manuf. Technol. 2016, 83, 1717–1724. [Google Scholar] [CrossRef]
- Jain, A.; Agarwal, A.; Chauhan, P.S. Optimization of laser cutting quality characteristics for basalt–glass hybrid composite using hybrid approach. Mater. Today Proc. 2022, 62, 12. [Google Scholar] [CrossRef]
- Wang, H.; Yang, T. A review on laser drilling and cutting of silicon. J. Eur. Ceram. Soc. 2021, 41, 4997–5015. [Google Scholar] [CrossRef]
- Marimuthu, S.; Dunleavey, J.; Liu, Y.; Antar, M.; Smith, B. Laser cutting of aluminium-alumina metal matrix composite. Opt. Laser Technol. 2019, 117, 251–259. [Google Scholar] [CrossRef]
- Sharifi, M.; Akbari, M. Experimental investigation of the effect of process parameters on cutting region temperature and cutting edge quality in laser cutting of AL6061T6 alloy. Optik 2019, 184, 457–463. [Google Scholar] [CrossRef]
- Mushtaq, R.T.; Wang, Y.; Rehman, M.; Khan, A.M.; Mia, M. State-Of-The-Art and Trends in CO2 Laser Cutting of Polymeric Materials—A Review. Materials 2020, 13, 3839. [Google Scholar] [CrossRef]
- Li, M.; Han, H.; Jiang, X.; Zhang, X.; Chen, Y. Surface morphology and defect characterization during high-power fiber laser cutting of SiC particles reinforced aluminum metal matrix composite. Opt. Laser Technol. 2022, 155, 108419. [Google Scholar] [CrossRef]
- Li, M.; Li, S.; Yang, X.; Zhang, Y.; Liang, Z. Effect of lay-up configuration and processing parameters on surface quality during fiber laser cutting of CFRP laminates. Int. J. Adv. Manuf. Technol. 2019, 100, 623–635. [Google Scholar] [CrossRef]
- Elsheikh, A.; Deng, W.; Showaib, E. Improving laser cutting quality of polymethylmethacrylate sheet: Experimental investigation and optimization. J. Mater. Res. Technol. 2020, 9, 1325–1339. [Google Scholar] [CrossRef]
- Vora, J.; Chaudhari, R.; Patel, C.; Pimenov, D.; Patel, V.; Giasin, K.; Sharma, S. Experimental investigations and Pareto optimization of fiber laser cutting process of Ti6Al4V. Metals 2021, 11, 1461. [Google Scholar] [CrossRef]
- Hong, L.; Zhang, Y.; Mi, C. Technological study of laser cutting silicon steel controlled by rotating gas flow. Opt. Laser Technol. 2009, 41, 328–333. [Google Scholar] [CrossRef]
- Quintero, F.; Pou, J.; Fernandez, J.L.; Doval, A.F.; Lusquiños, F.; Boutinguiza, M.; Soto, R.; Pérez-Amor, M. Optimization of an off-axis nozzle for assist gas injection in laser fusion cutting. Opt. Lasers Eng. 2006, 44, 1158–1171. [Google Scholar] [CrossRef]
- Riveiro, A.; Quintero, F.; Boutinguiza, M.; Del Val, J.; Comesaña, R.; Lusquiños, F.; Pou, J. Laser cutting: A review on the influence of assist gas. Materials 2019, 12, 157. [Google Scholar] [CrossRef]
- Riveiro, A.; Quintero, F.; Lusquiños, F.; Comesaña, R.; Pou, J. Effects of processing parameters on laser cutting of aluminium–copper alloys using off-axial supersonic nozzles. Appl. Surf. Sci. 2011, 257, 5393–5397. [Google Scholar] [CrossRef]
- Riveiro, A.; Quintero, F.; del Val, J.; Boutinguiza, M.; Comesaña, R.; Lusquiños, F.; Pou, J. Laser cutting using off-axial supersonic rectangular nozzles. Precis. Eng. 2018, 51, 78–87. [Google Scholar] [CrossRef]
- Yagi, A.; Kadonaga, S.; Okamoto, Y.; Ishiguro, H.; Ito, R.; Sugiyama, A.; Okawa, H.; Fujta, R.; Okada, A. Fundamental study on reduction of dross in fiber laser cutting of steel by shifting nozzle axis. J. Laser Appl. 2021, 33, 012022. [Google Scholar] [CrossRef]
- Murakami, I.; Zhao, Y.; Tashiro, T. Stabilization of a Magnetic Repulsive Levitation Flywheel System Using a High-Efficiency Superconducting Magnetic Bearing. Actuators 2022, 11, 180. [Google Scholar] [CrossRef]
- Soni, T.; Dutt, J.; Das, A.S. Parametric stability analysis of active magnetic bearing supported rotor system with a novel control law subject to periodic base motion. IEEE Trans. Ind. Electron. 2019, 67, 1160–1170. [Google Scholar] [CrossRef]
- Sillanpää, T.; Smirnov, A.; Jaatinen, P.; Vuojolainen, J.; Nevaranta, N.; Jastrzebski, R.; Pyrhönen, O. Three-axis inductive displacement sensor using phase-sensitive digital signal processing for industrial magnetic bearing applications. Actuators 2021, 10, 115. [Google Scholar] [CrossRef]
- Cao, S.; Niu, P.; Wang, W.; Zhao, T.; Liu, Q.; Bai, J.; Sheng, S. Novel Magnetic Suspension Platform with Three Types of Magnetic Bearings for Mass Transfer. Energies 2022, 15, 5691. [Google Scholar] [CrossRef]
- Javed, A.; Mizuno, T.; Takasaki, M.; Ishino, Y.; Hara, M.; Yamaguchi, D. Lateral Vibration Suppression by Varying Stiffness Control in a Vertically Active Magnetic Suspension System. Actuators 2018, 7, 21. [Google Scholar] [CrossRef]
- Ren, G.P.; Chen, Z.; Zhang, H.; Wu, Y.; Meng, H.; Wu, D.; Ding, H. Design of interval type-2 fuzzy controllers for active magnetic bearing systems. IEEE/ASME Trans. Mechatron. 2020, 25, 2449–2459. [Google Scholar] [CrossRef]
- Fan, X.; Yin, J.; Lu, Q. Design and Analysis of a Novel Composited Electromagnetic Linear Actuator. Actuators 2022, 11, 6. [Google Scholar] [CrossRef]
- Xu, Y.; Jiang, Q.; Yang, K.; Zhou, J.; Guo, Q. A novel ultra-high-resolution inclination sensor based on diamagnetic levitation. Sens. Actuators A Phys. 2022, 343, 113686. [Google Scholar] [CrossRef]
- Zhang, X.; Shinshi, T.; Fukuoka, T.; Nakai, T. Development of a 2-DOF Controlled Magnetic Drive Actuator for Laser Beam Cutting. J. Adv. Mech. Des. Syst. Manuf. 2013, 7, 448–457. [Google Scholar] [CrossRef][Green Version]
- He, D.; Shinshi, T.; Nakai, T. Development of a maglev lens driving actuator for off-axis control and adjustment of the focal point in laser beam machining. Precis. Eng. 2013, 37, 255–264. [Google Scholar] [CrossRef]
- He, D.; Shinshi, T.; Nakai, T. Development of a Lens Driving Maglev Actuator for Laser Beam Off-Axis Cutting and Deep Piercing. In Key Engineering Materials; Trans Tech Publications Ltd.: Bäch, Switzerland, 2012; Volume 523, pp. 774–779. [Google Scholar] [CrossRef]
- Morimoto, Y.; Shinshi, T.; Nakai, T. A two-DOF controlled lens drive actuator for off-axis laser beam cutting. J. Adv. Mech. Des. Syst. Manuf. 2012, 6, 875–884. [Google Scholar] [CrossRef]
- Gasmi, N.; Boutayeb, M.; Thabet, A.; Bel Haj Frej, G.; Aoun, M. Robust Control of a Class of Nonlinear Discrete-Time Systems: Design and Experimental Results on a Real-Time Emulator. Actuators 2021, 10, 303. [Google Scholar] [CrossRef]
- Seyfi, N.; Khalaji, A. Robust control of a cable-driven rehabilitation robot for lower and upper limbs. ISA Trans. 2022, 125, 268–289. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.; Ma, K.; Wei, Y.; Han, Y. Finite-time robust H∞ control for high-speed underwater vehicles subject to parametric uncertainties and disturbances. J. Mar. Sci. Technol. 2017, 22, 201–218. [Google Scholar] [CrossRef]
- Bu, X.; Wu, X.; Wei, D.; Huang, J. Neural-approximation-based robust adaptive control of flexible air-breathing hypersonic vehicles with parametric uncertainties and control input constraints. Inf. Sci. 2016, 346, 29–43. [Google Scholar] [CrossRef]
- Gosiewski, Z.; Mystkowski, A. Robust control of active magnetic suspension: Analytical and experimental results. Mech. Syst. Signal Process. 2008, 22, 1297–1303. [Google Scholar] [CrossRef]
- Zhang, Q.; Yu, R.; Li, C.; Chen, Y.-H.; Gu, J. Servo Robust Control of Uncertain Mechanical Systems: Application in a Compressor/PMSM System. Actuators 2022, 11, 42. [Google Scholar] [CrossRef]
- Zhang, K.; Scorletti, G.; Ichchou, M.; Mieyeville, F. Quantitative robust linear parameter varying H∞ vibration control of flexible structures for saving the control energy. J. Intell. Mater. Syst. Struct. 2015, 26, 1006–1027. [Google Scholar] [CrossRef]
- Ko, Y.; Kim, T. Feedforward Plus Feedback Control of an Electro-Hydraulic Valve System Using a Proportional Control Valve. Actuators 2020, 9, 45. [Google Scholar] [CrossRef]
- Isbeih, Y.; El Moursi, M.; Xiao, W.; El-Saadany, E. H∞ mixed-sensitivity robust control design for damping low-frequency oscillations with DFIG wind power generation. IET Gener. Transm. Distrib. 2019, 13, 4274–4286. [Google Scholar] [CrossRef]
- Wu, X.; Xu, C.; Wei, B.; Xia, C.; Li, X. H∞ mixed sensitivity robust control method of relay ICPT system for output voltage regulation. Electr. Eng. 2021, 103, 781–792. [Google Scholar] [CrossRef]
- Xu, Z.; Zhang, Q.; Sun, F. Analysis of Wave Characteristics of Laser Focus Magnetic Drive Platform. Modul. Mach. Tool Autom. Manuf. Tech. 2020, 3, 13–15. [Google Scholar] [CrossRef]
- Tian, L.; Ai, X.P.; Tian, Y. Analytical model of magnetic force for axial stack permanent-magnet bearings. IEEE Trans. Magn. 2012, 48, 2592–2599. [Google Scholar] [CrossRef]





| System Parameters | Symbol | Value |
|---|---|---|
| Air permeability | μ0 (H/m) | 4π × 10−7 |
| Relative permeability | μr | 1.05 |
| Material remanence | Br (T) | 1.18 |
| Number of permanent magnets | n | 2 |
| Permanent magnet thickness | δP (mm) | 4 |
| Permanent magnet inner diameter | r1 (mm) | 15 |
| Permanent magnet outer diameter | r2 (mm) | 20.5 |
| Permanent magnet axial air gap | ε (mm) | 4 |
| Permanent magnet radial displacement | e (mm) | 0 < e < 4 |
| System Parameters | Symbol | Value |
|---|---|---|
| Moving plate weight | m (kg) | 1.3995 |
| The distance between two pairs of electromagnet force centers in the X direction | l1 (mm) | 44.5 |
| The X direction sensor measures the distance from the point to the center | l0 (mm) | 167 |
| The rotational inertia of the moving plate | J (kg·mm2) | 0.004972 |
| The magnetic permeability of air | μ0 (H/m) | 4π × 10−7 |
| Number of coil turns | N | 128 |
| The cross-sectional area of the magnetic circuit | S (mm2) | 912 |
| Magnetic circuit reluctance coefficient | δ | 1.38 |
| X direction damping | cx | 10 |
| Y direction damping | cy | 10 |
| θ direction damping | cθ | 10 |
| Initial current | i0 (A) | 1.2 |
| Initial gap | d0 (mm) | 2 |
| Permanent magnet stiffness | ke (N/m) | 3032.7 |
| Direction | Maximum Overshoot | Regulation Time | Steady-State Error |
|---|---|---|---|
| Y direction | 25.9% | 0.37 s | 0 |
| X direction | 19.1% | 0.18 s | 0 |
| θ direction | 0 | 0.22 s | 0 |
| Direction | Maximum Overshoot | Regulation Time | Steady-State Error |
|---|---|---|---|
| Y direction | 21% | 0.38 s | 0 |
| X direction | 24.7% | 0.19 s | 0 |
| θ direction | 0 | 0.21 s | 0 |
| Direction | Maximum Overshoot | Regulation Time | Steady-State Error |
|---|---|---|---|
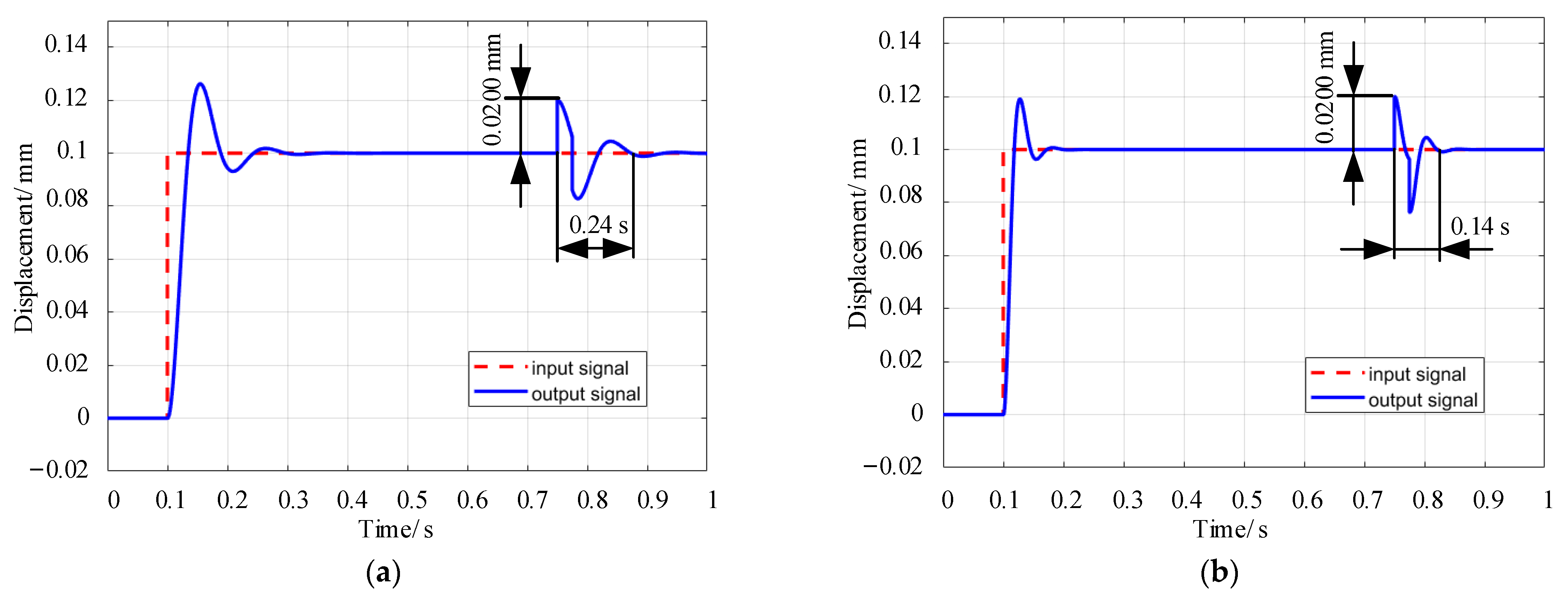
| Y direction | 20% | 0.24 s | 0 |
| X direction | 20% | 0.14 s | 0 |
| θ direction | 20% | 0.16 s | 0 |
| Direction | Maximum Overshoot | Regulation Time | Steady-State Error |
|---|---|---|---|
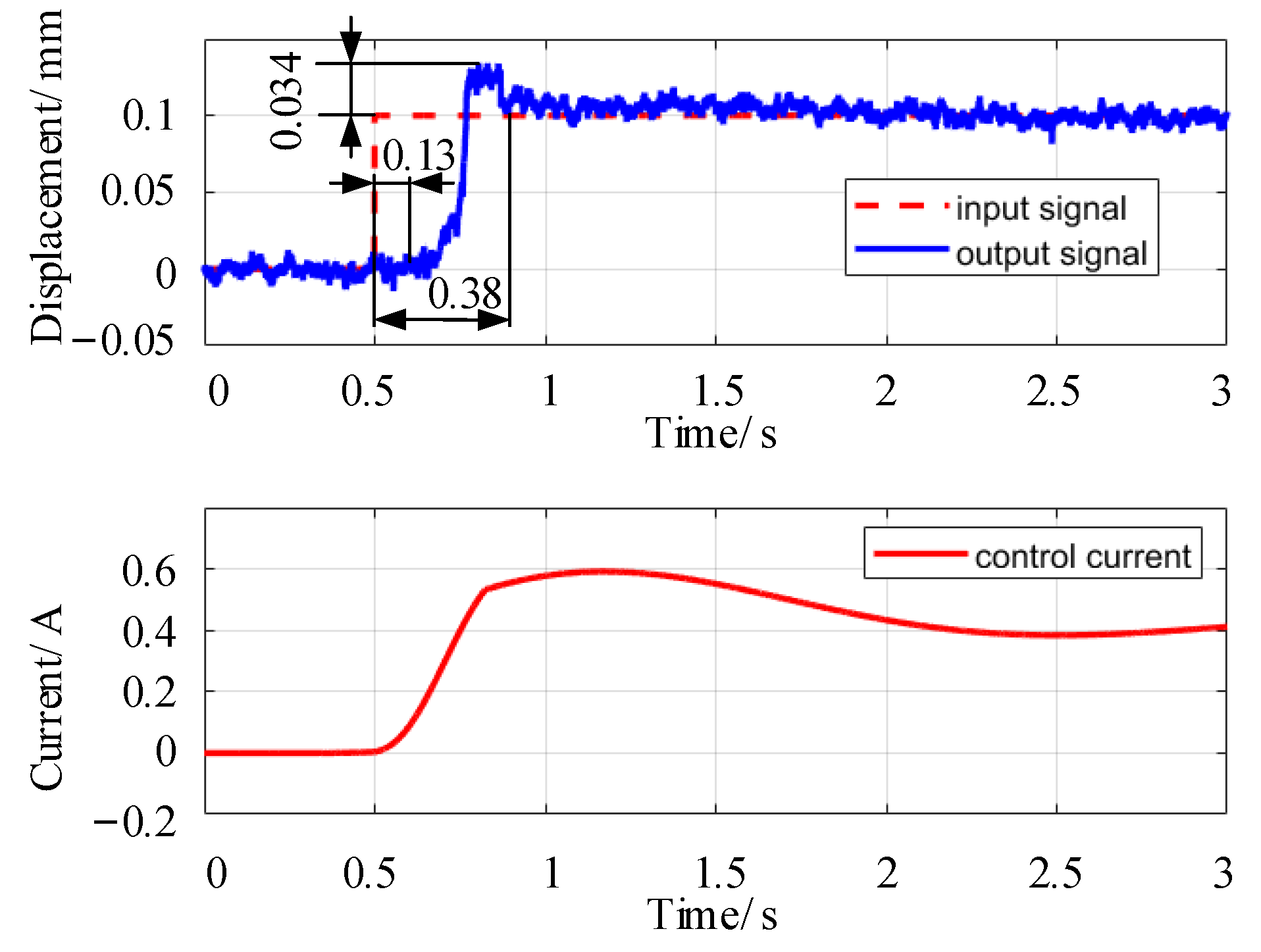
| Y direction | 34% | 0.38 s | 0.39% |
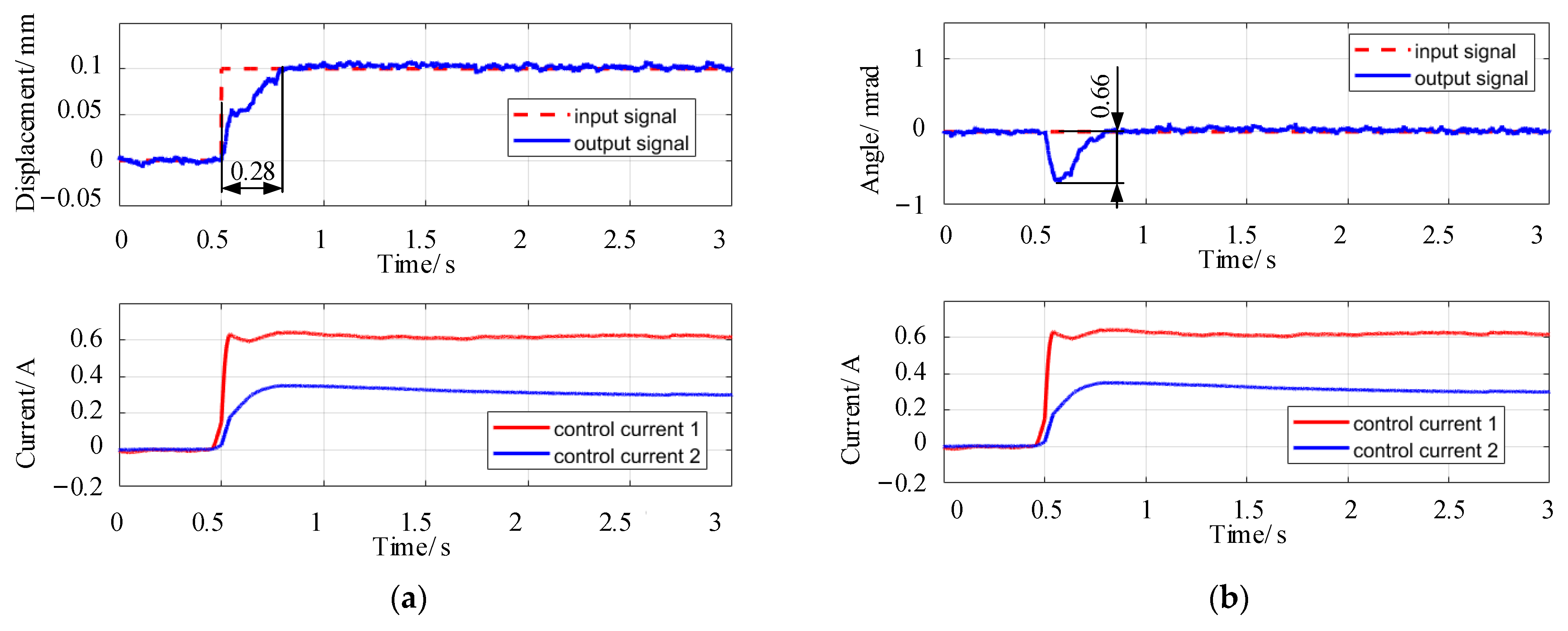
| X direction | 3.1% | 0.28 s | 1.6% |
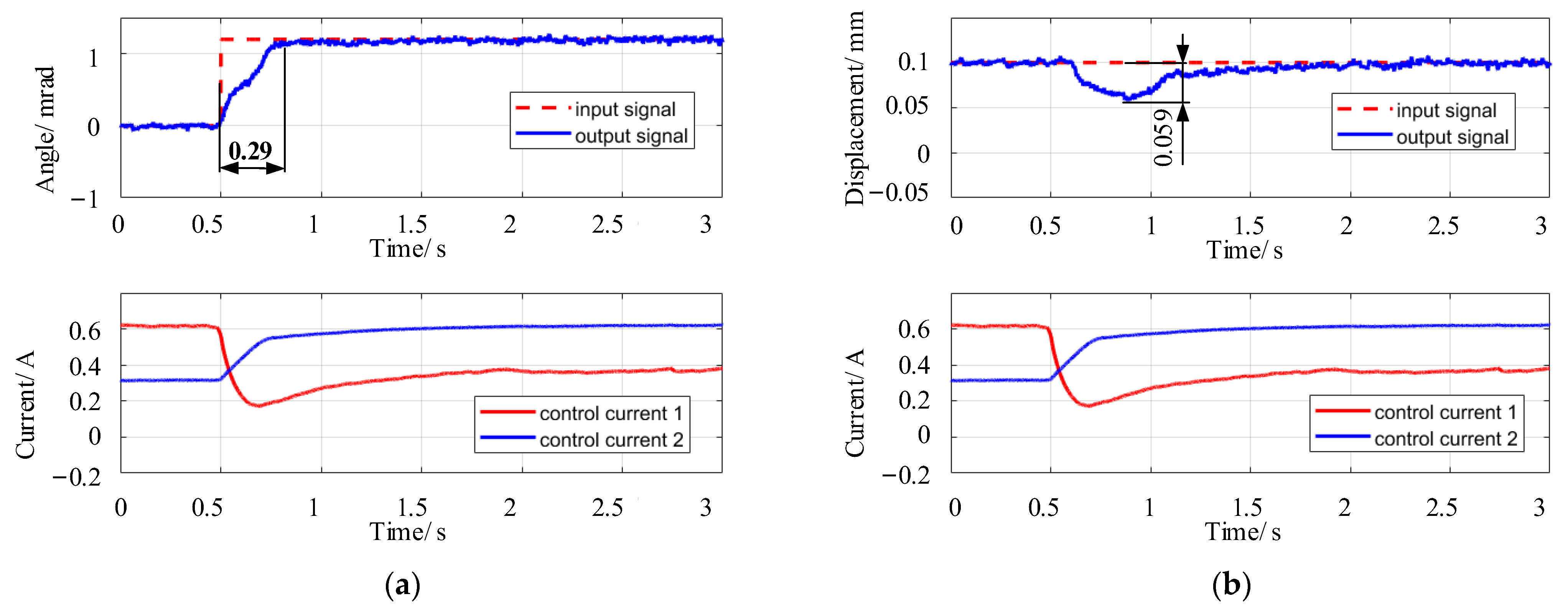
| θ direction | 0% | 0.29 s | 0.45% |
| Direction | Maximum Overshoot | Regulation Time | Steady-State Error | |
|---|---|---|---|---|
| Y direction | Simulation | 25.9% | 0.37 s | 0 |
| Experiment | 34% | 0.38 s | 0.39% | |
| |Difference value| | 8.1% | 0.01 s | 0.39% | |
| X direction | Simulation | 19.1% | 0.18 s | 0 |
| Experiment | 3.1% | 0.28 s | 1.60% | |
| |Difference value| | 16% | 0.10 s | 1.60% | |
| θ direction | Simulation | 0% | 0.22 s | 0 |
| Experiment | 0% | 0.29 s | 0.45% | |
| |Difference value| | 0% | 0.07 s | 0.45% | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, L.; Tong, L.; Yang, G.; Zhang, Q.; Xu, F.; Jin, J.; Zhang, X.; Sun, F. Improvement in Position Response of Laser Focus Controlled Magnetic Actuator Based on Mixed Sensitivity Robust Control. Actuators 2023, 12, 4. https://doi.org/10.3390/act12010004
Wu L, Tong L, Yang G, Zhang Q, Xu F, Jin J, Zhang X, Sun F. Improvement in Position Response of Laser Focus Controlled Magnetic Actuator Based on Mixed Sensitivity Robust Control. Actuators. 2023; 12(1):4. https://doi.org/10.3390/act12010004
Chicago/Turabian StyleWu, Liping, Ling Tong, Guang Yang, Qi Zhang, Fangchao Xu, Junjie Jin, Xiaoyou Zhang, and Feng Sun. 2023. "Improvement in Position Response of Laser Focus Controlled Magnetic Actuator Based on Mixed Sensitivity Robust Control" Actuators 12, no. 1: 4. https://doi.org/10.3390/act12010004
APA StyleWu, L., Tong, L., Yang, G., Zhang, Q., Xu, F., Jin, J., Zhang, X., & Sun, F. (2023). Improvement in Position Response of Laser Focus Controlled Magnetic Actuator Based on Mixed Sensitivity Robust Control. Actuators, 12(1), 4. https://doi.org/10.3390/act12010004






