1. Introduction
In order for the robot to perform various manipulation tasks, it is of utmost importance that the robot’s end-effector, or gripper, is adaptable to the types of handled objects and task conditions. In common places, picking, holding, or catching objects are uses of traditional factory robots or modern collaborative robots, where arbitrarily-shaped objects may have to be grasped robustly in a partially known, or unknown environment. As an inherent and dependable solution for such applications, adaptive grippers that passively conform their grasping postures to the shapes of objects being grasped have gained attention and become one of the preferred options in the robotics community.
Early robotic grippers beginning in the 1960s were developed for industrial applications. Those industrial robot grippers were improved through the adoption of various driving actuators and mechanisms [
1]. Traditional robot grippers that have been developed are often classified by the type of actuator, the mechanism of the actuator, the number of gripper fingers, etc. [
2,
3]. A robot gripper classification study was conducted by the methods of actuation; please refer to [
4]. Recently, industrial gripper companies are classifying their products into 2-finger, 3-finger, and adaptive functionalities [
5,
6].
The robot grippers evolved from a gripper with a simple mechanism to a robotic hand with complex control laws and grasp methods. Among a large number of research works, notable ones can be listed as follows: Okada hand [
7], Utah/MIT hand [
8], shadow hand [
9], stanford/jpl [
10], DLR Hand [
11], LMS hand [
12], and NASA robonaut hand [
13], etc. Since the robot hand is composed of a complex mechanical structure and many sensors, it can perform more delicate tasks with various postures and control laws. However, although a human-like robot hand capable of complex functionalities can perform a variety of tasks, it can hardly produce agile movements; moreover, the complex structure and sophisticated controller reduce its flexibility and controllability, and thus, increase the cost of the robotic hand, making it unsuitable for large-scale use.
In contrast, the simple industrial grippers motioned by the usual 1-DOF mechanism could not create sufficient functionalities. Accordingly, an under-actuated gripper capable of performing a variety of tasks using a small number of actuators became used as a compromise between a human-like robotic hand and an industrial gripper. Using an under-actuated hand means reducing the number of actuators required for a variety of mechanical motions. Representative studies related to this include the grasper hand [
14], DIES-DIEM hand [
15], Cassino Finger [
16], and SSL hand [
17].
The differential mechanism is one of the elements for configuring under-actuated driving systems. The differential gear mechanisms, which distribute a single input into two output shafts, has been used to reduce the number of actuators while increasing the adaptability and operational flexibility of the output. Additionally, this mechanism had expanded into robotic applications as well. For example, the self-adaptive gripper using a differential gear was used to move the multi-link finger [
18] with a smaller number of actuators than its degrees of freedom, so that the gripper could deform passively to the shape of objects when performing an encompassing grasp. Another example is a gripper that was constructed using differential gears in a reverse manner to produce one output motion with two input actuators [
19]. However, these examples were not intended for adaptability for common parallel graspings such as pinch or spread.
To construct an under-actuated gripper, passive springs or passive mechanical elements must be incorporated with instead of the additional actuators required for the gripper’s mechanism. The so-called self-adaptive passive mechanism was first used in the under-actuated gripper design that changed its structure according to the shape of the grasped object [
20]. Birglen and their group later defined and presented the kinetostatics of finger mechanism [
21], force analysis of minimal variation of 4-bar linkage [
22], and more. Then, variants of self-adaptive grippers from Birglen’s work had been developed subsequently, such as [
23,
24,
25].
The aforementioned self-adaptive gripper mechanisms worked based on a conventional 4-bar linkage structure, providing three representative grasp methods: encompassing, pinch, and spread grasps. However, the 4-bar linkage structure in those self-adaptive grippers cannot produce perfectly parallel jaw motions due to the non-linear movement of the gripper tips, which would be a drawback. For example, the self-adaptive grippers cannot grasp a flat object safely due to the nonlinear travel path of the two jaws during the pinch grasping. A study was conducted to overcome such a disadvantage by using an additional actuator, allowing perfectly parallel grasping [
26], though the adaptability during the parallel grasping was not offered.
Studies to apply the adaptive finger to the traditional parallel type gripper were also conducted. An adaptive finger with a linkage structure capable of encompassing and pinch grasping was proposed for an industrial parallel gripper [
27,
28]. Another design was proposed to define the contact point that occurs in the encompassing grasp of the adaptive gripper finger of the parallel type and to understand its stability. Therefore, an adaptive finger was developed to perform encompassing and pinch grasping [
29]. However, these parallel type self-adaptive grippers again provide only the shape adaptability during encompassing and pinch grasp methods. Therefore, a simple yet expandable gripper with a larger adaptability than the simple shape adaptability is required that facilitates grasping in various environments and uncertain conditions.
In this paper, we present a novel self-adaptive gripper that enables two ways of self-adaptive grasping: the first one is the adaptation in the parallel grasping by using a differential gear system and the second one is the adaptation in the encompassing grasping by using exoskeletal–internal separable 4-bar linkages with spring elements. While performing the pinch grasping, the differential gear system works to adaptively hold the object safely even when external load or resistance is applied to the object. Concerning the encompassing grasping, a modified 4-bar linkage is used with a spring reaction to the internal linkage while the exoskeletal body remains unchanged. Kinematic modeling and contact force analysis are performed and grasp performance is verified through simulations and experiments.
2. Design of Two-Way Self-Adaptive Gripper
In this section, we address two sub mechanisms used in the proposed gripper from a mechanical point of view. One is an actuator mechanism that overcomes a jammed situation using a differential gear. Another is a shape-adaptive mechanism of the internal linkage that provides encompassing, spread, and pinch grasping.
In the case of a conventional gripper, shape adaptiveness of the gripper finger is possible. However, the uncertainty caused by the load applied during the parallel pinch grasping could not be adapted. Therefore, in this paper, we propose an improved gripper design by adding adaptability to the external load during the linear grasping motion using differential gear together with the conventional adaptiveness to the object shape being grasped.
Another issue related to the conventional adaptive grippers to indicate is the non-linear movement during the jaw opening/closing. Because the gripper’s fingertips exhibit non-linear movements, it is difficult to perform a perfect pinch grasp. Accordingly, the fingers of the parallel-type adaptive gripper increased the number of actuators to make a grasp motion, or was only used for encompassing pinch grasping due to the structural characteristics of the finger. The proposed two-way adaptive gripper allows perfect parallel movements with three possible grasp methods with a single actuator.
The gripper concept proposed in this paper is extensibility. Load adaptiveness using differential gear is added to the three adaptive grasps of a conventional gripper to create an extended adaptive gripper. In addition, the gripper configured in the form of a module expands the task capability by the combination of modules according to the working environment [
30,
31].
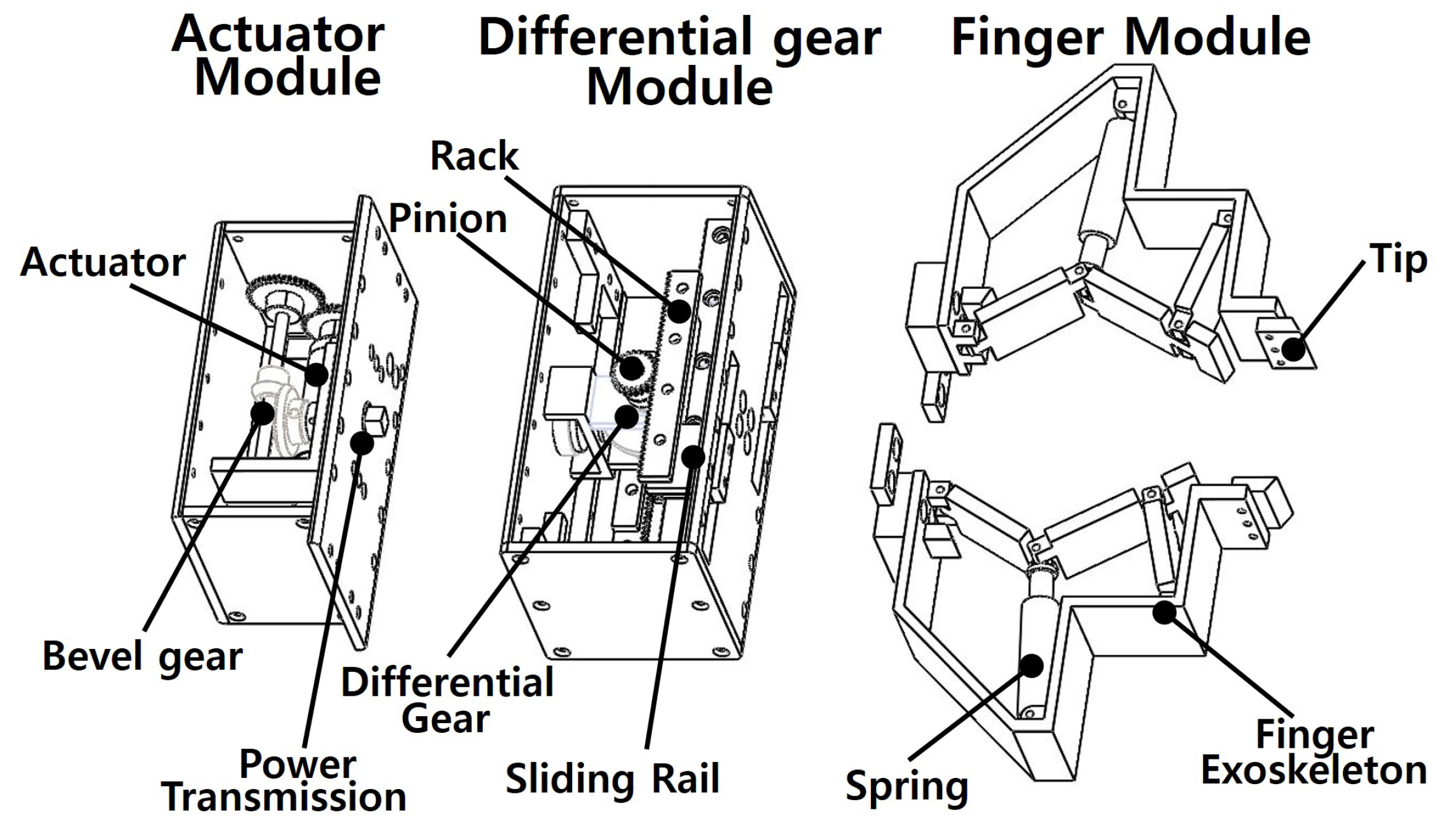
Figure 1 shows the overall image of the designed gripper. The gripper is composed of three modules: an actuator module, a differential module, and a self-adaptive finger module [
32].
The differential module converts the output axes of the differential gear into linear motion and is applied to the gripper finger slide motion. A single differential gear is used to transmit one input power to two output axes. At this time, if loads (friction, external force, etc.) act on one output axis and cause it to stop or slows down, it has a gear train whose rotation amount of the opposite output axis is automatically adjusted. Accordingly, load adaptation using a differential gear is possible. In this paper, the gripper finger slide motion is applied by changing the differential gear’s output axes into linear motion. Load adaptiveness is possible when one of the gripper fingers is jammed on an object or a finger load is applied. Therefore, even if a load is applied to one finger by the differential gear, resulting in different movement speeds or rotation amounts, adaptation by motion is possible because the reduced movement speed and rotation amount are compensated for on the opposite side.
2.1. Differential Actuating Mechanism
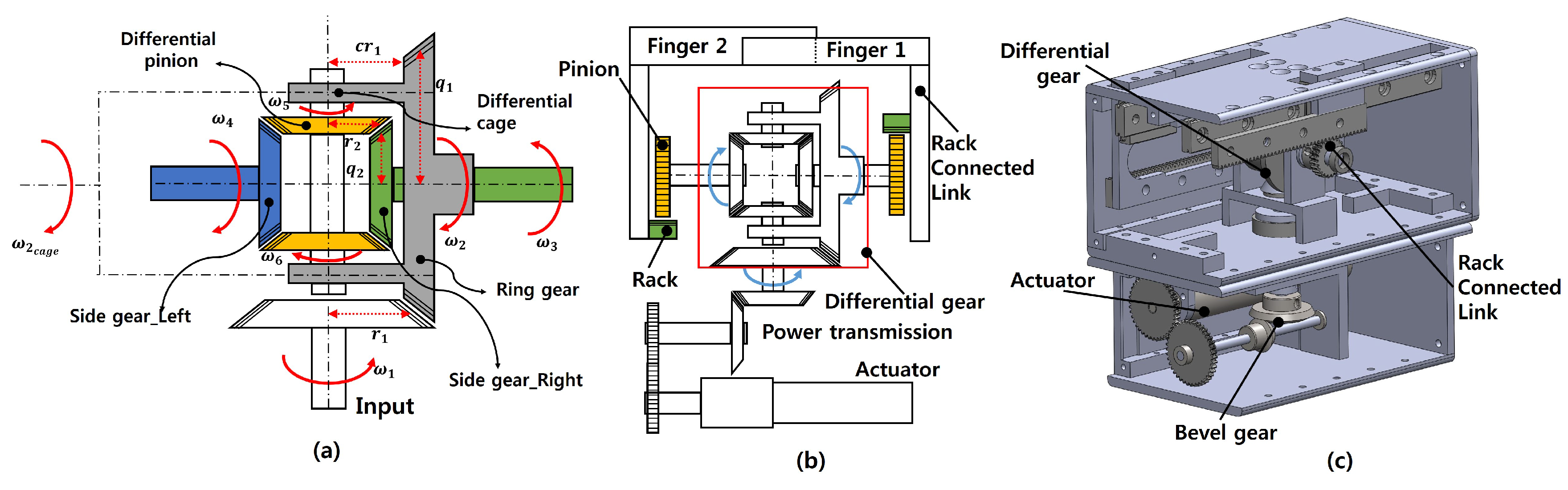
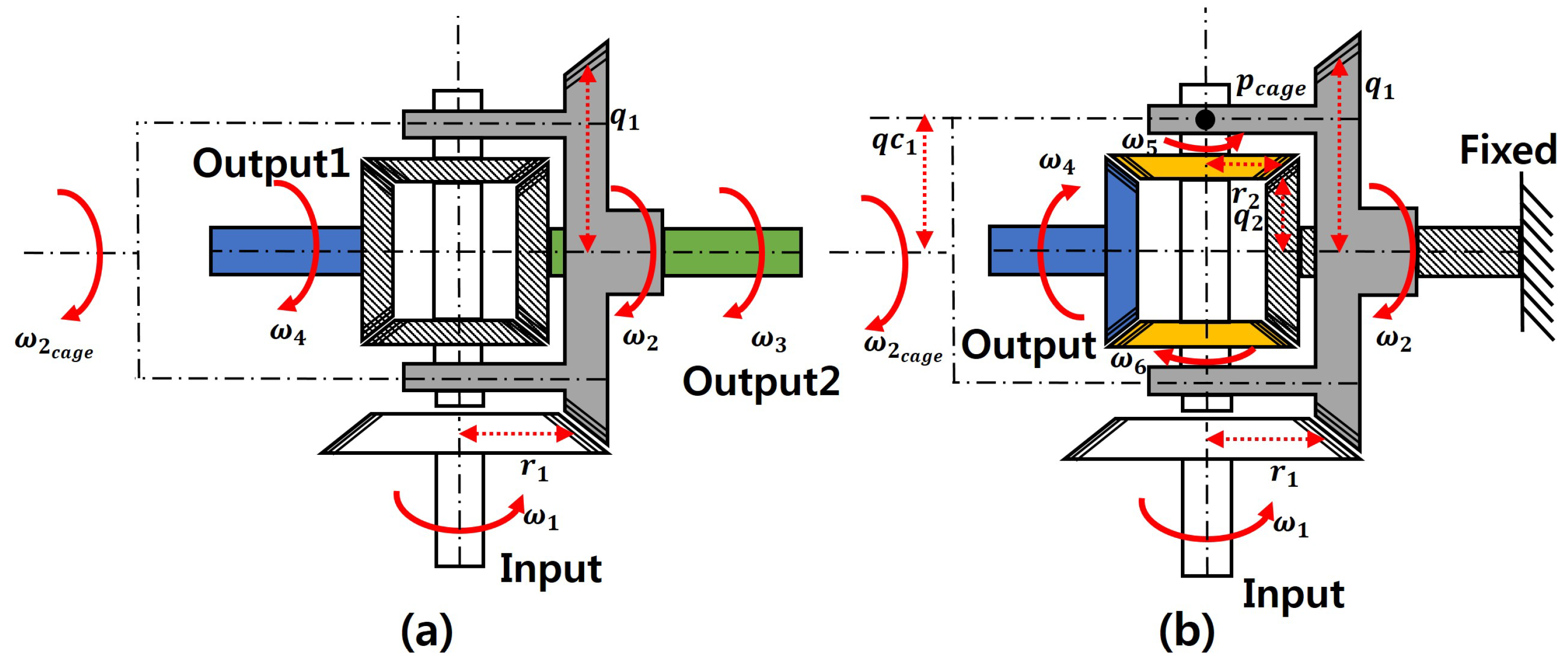
Figure 2a shows the structure of the differential gear; at this time,
Figure 2b shows the schematic for driving two fingers by putting one actuator in the differential mechanism. The power generated by the actuator changes the position of the power vertically through the bevel gear, and the power is connected to the input of the differential gear. As shown in
Figure 2b, the differential gear consists of a ring gear and an internal bevel gear, which is the same as the gear structure commonly used in automobiles. A linear motion is produced by rack and pinion on both output axes of the differential gear.
Figure 2c shows the designed combination of the differential gear module and actuator module. The parallel differential adaptive gripper proposed in this paper expands the scalability of the gripper in the form of a module.
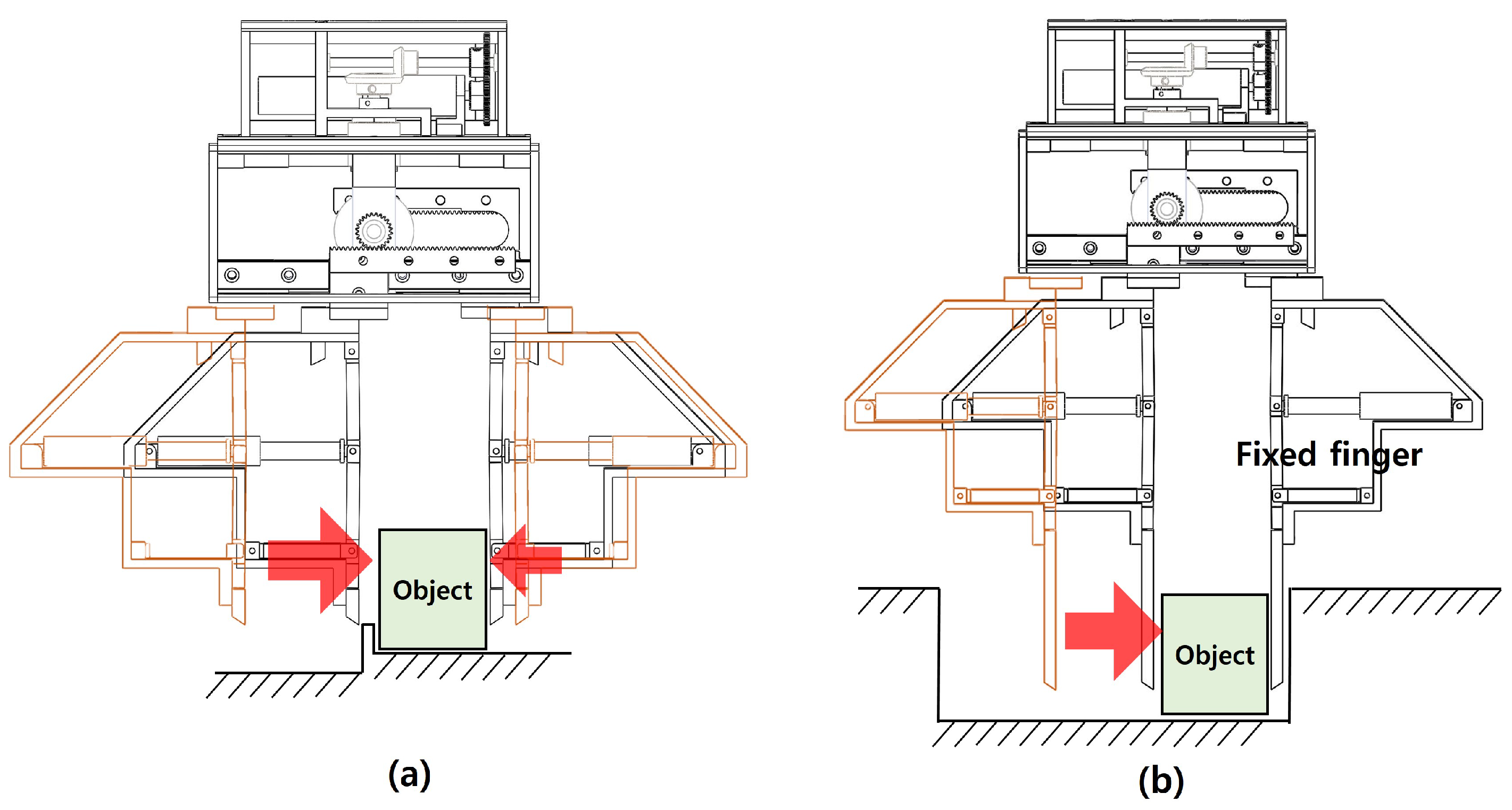
Figure 3a shows the adaptive function of load added through differential gear. It shows that grasping is possible due to mutual compensation of both fingers, even if the gripper is not positioned in the center of the gripper when grasping an object.
Figure 3b shows an example of grasping that can be realized when there is un immobility constraint on one finger. In this situation, It is possible to grasp un object by moving the other unconstrained finger. This functionality may be useful when the gripping space of one side is narrow.
2.2. Architecture of Adaptive Gripper Finger Mechanism
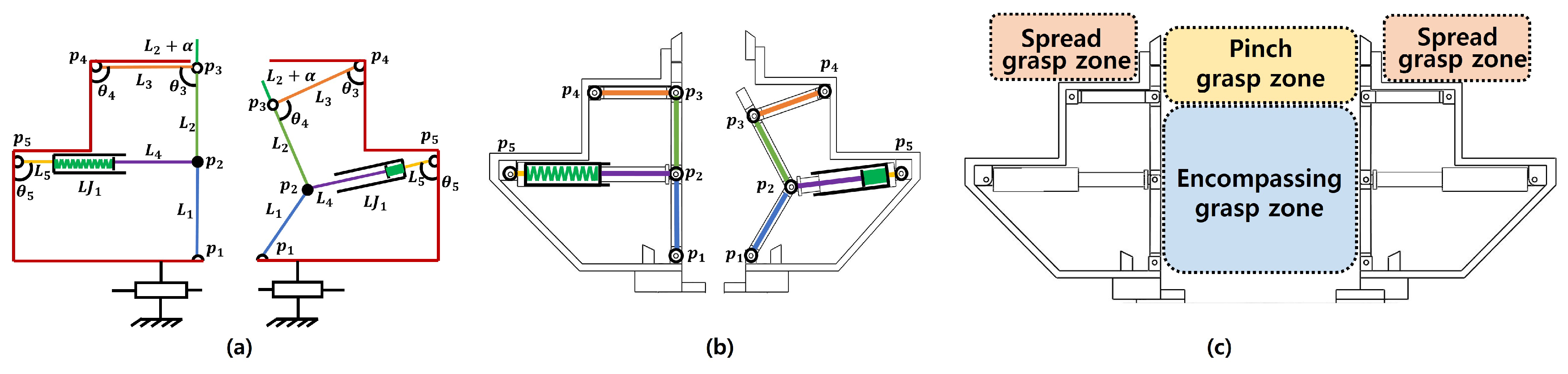
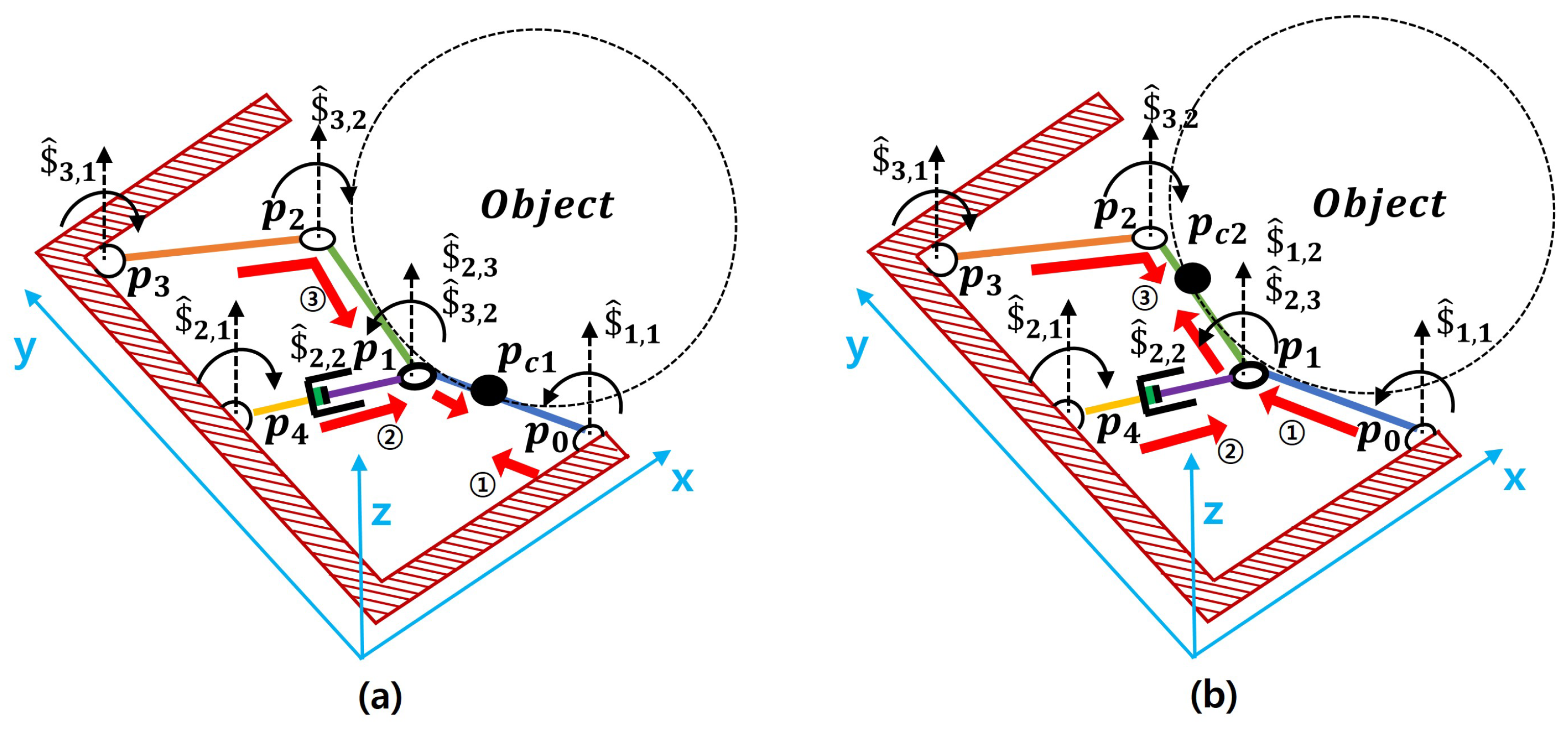
Figure 4 shows the structure of the gripper finger.
Figure 4a shows the schematic of the finger,
Figure 4b shows the structure of the gripper finger, and
Figure 4c indicates the area that can be grasped.
The shape adaptability of the grasping is realized by the gripper’s finger structure. And basically, the gripper considers the jaw-type parallel grasping motion. As shown in
Figure 4a, the proposed self-adaptive grasping can be performed by combining the exoskeleton of the finger in red line and the inner linkages. Each of them consists of 5 links, 5 rotation joints and 1 spring.
,
and
are serially connected, and the initial posture is trying to be maintained by the spring of
.
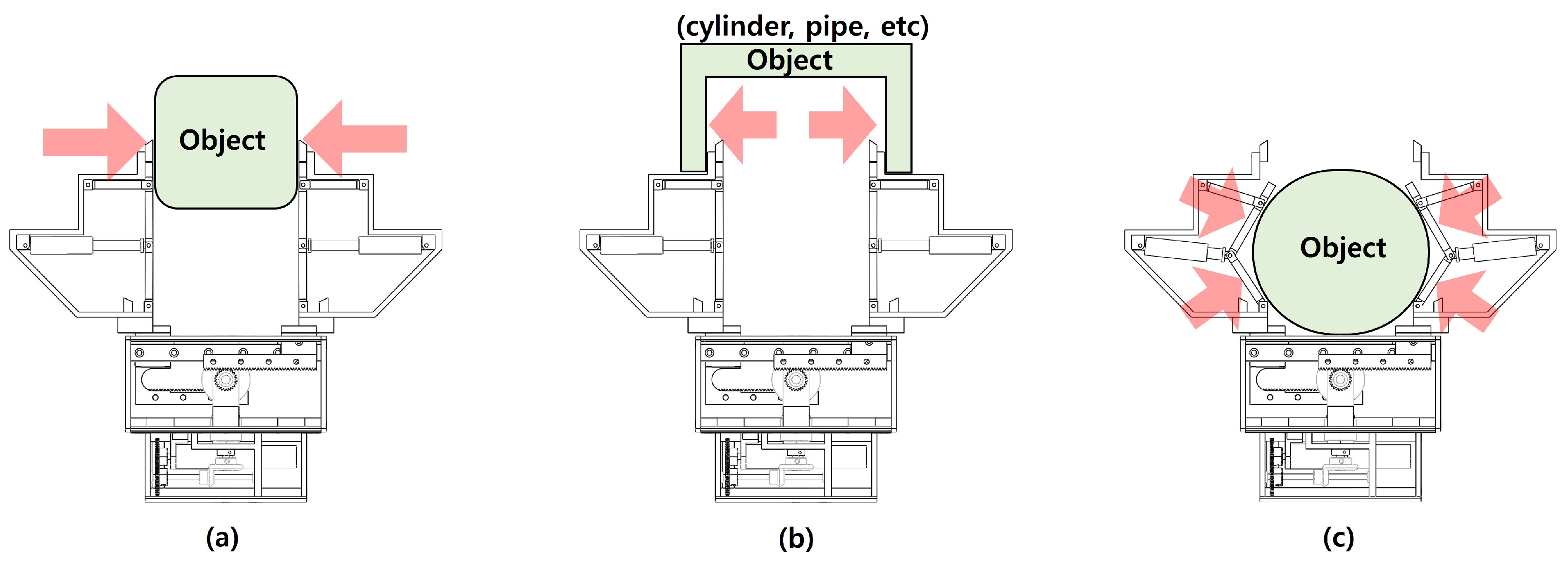
Figure 5a–c shows the proposed grasp method of the parallel type adaptive finger. In detail,
Figure 5a,
Figure 5b and
Figure 5c, respectively, shows pinch, spread, and encompassing graspings through parallel finger movement. Therefore, the proposed gripper shows the parallel-type gripper operates linearly, and when a grasp object enters the gripper’s grasp zone (as shown in
Figure 4c), it performs grasping according to each grasp mode.
Figure 5a shows a pinch grasp. The finger’s internal link
performs gripping with the exoskeleton supported.
Figure 5b shows the spread grasp. By the exoskeleton of the finger, the parallel gripper operates to the outside and grips an object.
Figure 5c represents the encompassing grasp. The inner links of the exoskeleton perform grasp according to the shape of the grasp object by organic action.
4. Prototype and Grasping Experiments
4.1. Prototype and Hardware Setup
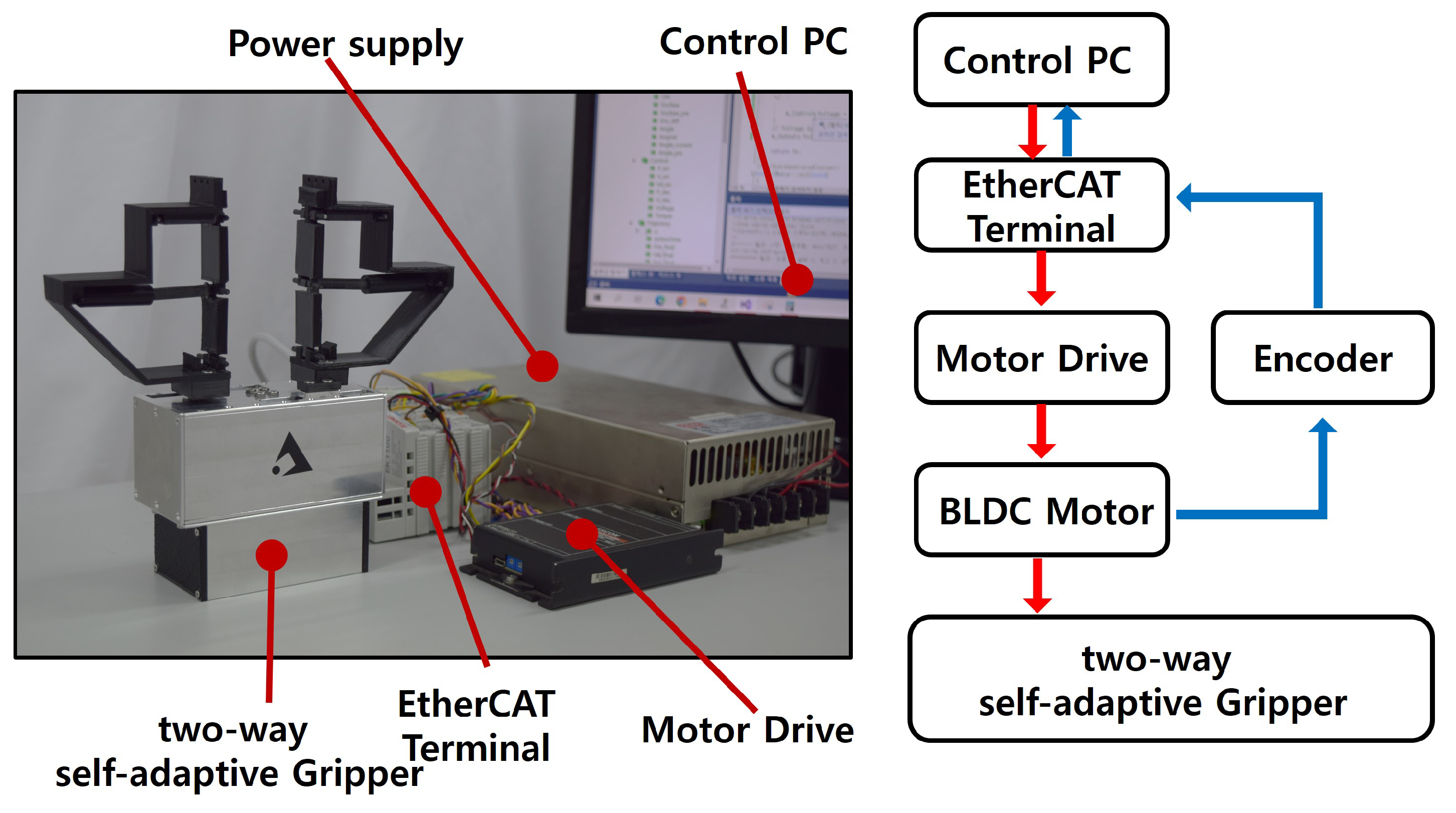
The gripper prototype system built by the proposed design is shown in
Figure 10. The motor used for the gripper is a single brushless DC motor from Maxon (model ECXSP13M). This motor is 70 mm in length and 13 mm in diameter. An optical encoder with a resolution of 1024 pulse/rev is installed in it. An IBM-compatible PC is used to control the motor through EtherCAT real-time communication.
Table 1 shows the hardware specifications and performance values of the prototype. With the torque of the motor being 4.3 mNm, the proposed gripper can produce a maximum grasping force of 154.2 N in accordance with the gear ratios in
Table 1. Additionally, by the stall speed of 63,000 rpm of the motor, the proposed gripper allows a maximum linear speed of 200.96 mm/s. Additionally, by the design, the finger-to-finger maximum grasping stroke is 90 mm.
The size of the differential gear module is 114 × 59 × 53.2 mm, and the size of the actuator module is 93 × 59 × 41 mm. Additionally, the contact links and are 35 mm each, and the length from to for installing the spring is only 70 mm. The manufactured gripper prototype is made of aluminum and plastic material with a total weight of 690 g. particular, the gripper fingers are made of a plastic material that was 3D printed using a PLA (Poly Lactic Acid) material.
The numerical values of the grasp zones (as illustrated in
Figure 4c) of the prototype is also shown in
Table 1. In the case of the encompassing zone, it is a rectangular shape with a width of 90 mm, height of 70 mm, and depth of 20 mm. The zone of the pinch grasp is a rectangular shape with a width of 90 mm, height of 16 mm, and depth of 27 mm as the finger’s exoskeleton depth. The zone of the spread grasp starts in the gripper’s closed state but has the same dimension as the pinch grasp case.
Using the prototype, in this paper, a series of grasp tests were performed to verify the two-way adaptability and reliability of the proposed gripper design.
4.2. Parallel Grasping Using Differential Gear Mechanism
When using conventional grippers, the grasping of an object always occurs at the center of the gripper stroke due to the identical closing speed of the two fingers. Such grippers do not allow a fair amount of tolerance in the positioning error of the object being grasped because otherwise the grasping would become unstable. To hold and turn a door handle or to grasp a heavy body, the positioning error of the robot may also cause significant damage to the robot and the gripper as well. Such a disadvantage can be mitigated by imposing adaptability on the asymmetric loading condition on both fingertips during the linear pinch grasping.
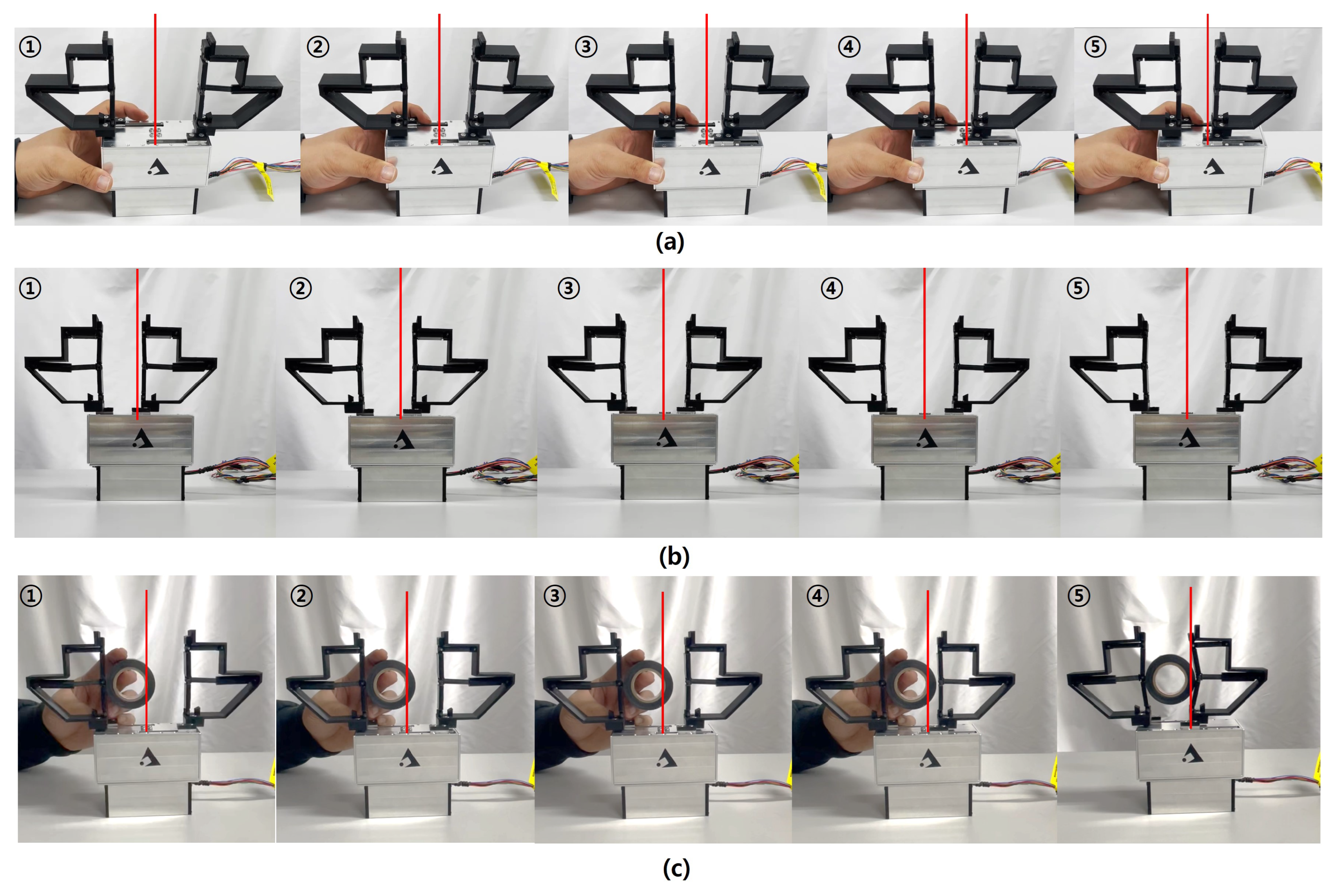
The proposed gripper enables load adaptability in linear parallel grasping by employing the differential gear mechanism, as shown in
Figure 11, where the red lines indicate the center of the gripper.
Figure 11a shows the sequential steps of grasping; when one finger was held by a human hand, the grasping was completed with the other finger. Therefore, the gripper operated asymmetrically, but even if an external load was applied to one of the fingers, the object could be gripped safely by compensating for the closing action with the other finger.
Figure 11b shows the returning phases of the gripper fingers back to their original position. Even if the initial gripper posture was not symmetric due to the adaptive grasping, it could manage to return to the home position on its own.
Figure 11c shows the scenes when actually grasping a circular object. The left finger was intentionally held by a human hand, but the right finger completed the grasping any way. In this situation, inner links were bent according to the shape of the grasped object. This result shows the two kinds of adaptability of the gripper during the parallel encompassing grasping: the load adaptability and the shape adaptability. (More detailed demonstration of the shape adaptability shall be given in the next subsection). After all, the proposed gripper, different from the conventional grippers, did not have to complete the grasping at the center, but could deal with the external load (including jamming or one-finger-fixed situations) in an adapted manner to finish the required grasping. Therefore, the results verified that the gripper using differential gear offers adaptability in the parallel grasping motion.
4.3. Finger Shape Adaptation
The finger-shape adaptive grasping is performed by applying the three grasping methods: encompassing, pinch, and spread according to the shape of the object. In encompassing and pinching, the gripper adaptively performs grasping by passively deforming the linkage in accordance with the shape of the object’s contact surface.
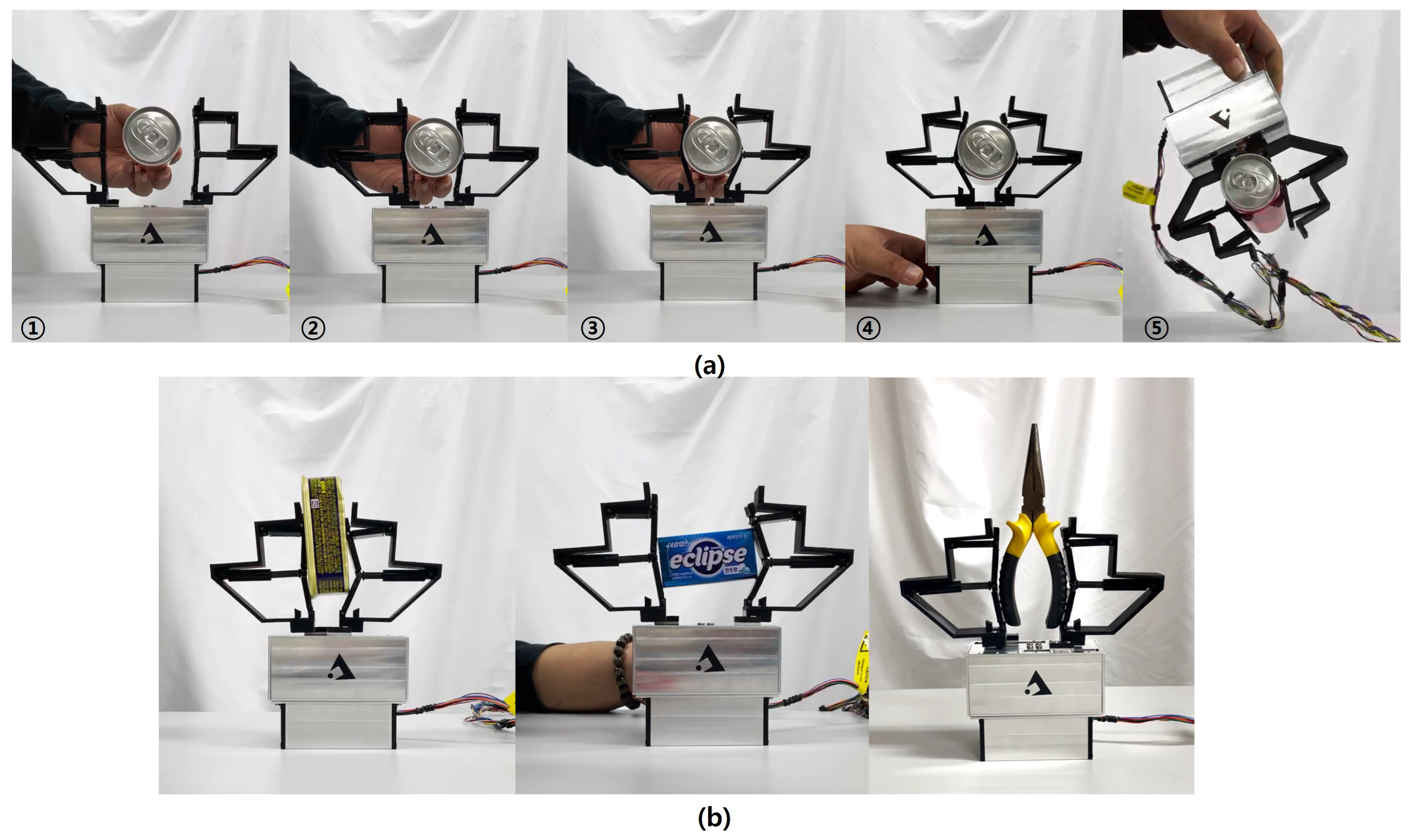
Figure 12 shows the experimental results for encompassing grasping.
Figure 12a shows the process of grasping a circular soda can. When the grasping was started, the soda can was placed in the encompassing grasp zone, and the gripper fingers on both sides approached in parallel to grasp the object. At this time, the internal links began to encompass the object with lateral linkage being bent passively to safely hold the can.
Figure 12b shows the screenshots of holding various objects other than the circular can by the encompassing grasp. The leftmost case was holding an object that was located across the pinch grasp zone and the encompassing grasp zones. In the middle of
Figure 12b, a rectangular (50.36 × 95.2 × 31.6 mm) object lying in the encompassing zone was held adaptively. The rightmost case shows grasping a tool with a weight of 166 g. In all these cases, the internal linkage became complied with the shape of the objects being grasped.
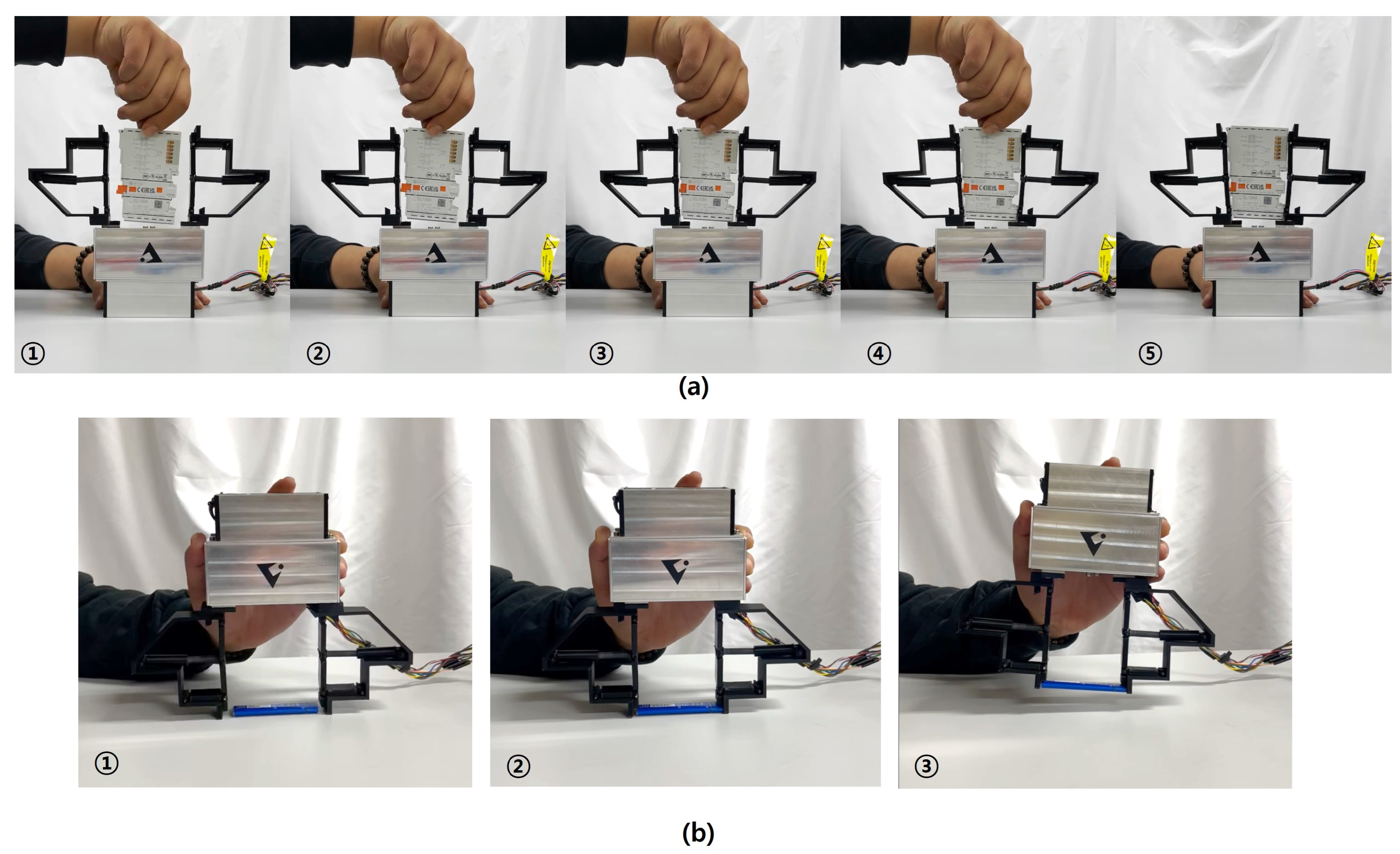
Figure 13 shows the experimental results by using parallel pinch grasping.
Figure 13a shows the sequential steps of grasping an electronic part having a flat shape. As expected, the gripper could hold the object without difficulty.
Figure 13b shows the sequential step of grasping a thin plastic card lying on a desktop. Grasping a thin card is considered a difficult task by the conventional adaptive grippers due to their non-linear finger movement, whereas the proposed self-adaptive gripper can manage to pick it up.
Figure 14 shows the experimental results by using the spread grasping. Note that the spread grasping is performed by driving the fingertips in the opposite direction, and therefore it is suited for holding objects with open empty holes. As shown in the experimental test, the paper cup was grasped safely by the spread grasping, which shows another possible grasping method of the proposed gripper design.
In summary, through the experimental results in this section, we verified that the two-way self-adaptive capability, i.e., the load adaptability in the parallel grasping motion and shape adaptability in the encompassing grasping using only a single actuator. In particular, due to the load adaptability, we could confirm that the grasping can be completed in the jammed situation using the differential gear mechanism, which is our notable contribution as no previous conventional self-adaptive grippers can guarantee this. We also confirmed that load and shape adaptability can be simultaneously achieved. Enabling the perfect parallel fingertip movement was additional merit for the proposed gripper, while the conventional adaptive grippers, mostly adopting the 4-bar linkage structure, do not provide perfect parallel fingertip movement. A consequent disadvantage of the conventional adaptive grippers is the significant reduction in the graspable zone during parallel grasping, whereas the proposed gripper fully uses its whole stroke distance to pick up the objects located within it.