Design and Analysis of a Novel Variable Stiffness Joint for Robot
Abstract
1. Introduction
- (1)
- In the second part of the paper, the overall structure and the variable stiffness structure of the VSJ-RP are introduced. Based on the variable stiffness principle, the stiffness mathematical model of the VSJ-RP is derived;
- (2)
- In the third part of the paper, the VSJ-RP dynamic model is constructed, and the VSJ-RP system is simulated in MATLAB/Simulink;
- (3)
- In the fourth part of the paper, the VSJ-RP experimental platform is built, and the compliance, safety, and energy storage and release characteristics of the variable stiffness joint are verified by a location experiment, a torque test experiment, a hitting ball experiment, and a security test experiment, respectively.
- (4)
- The fifth part is the conclusion of this paper.
2. Mechanical Structure of the VSJ-RP
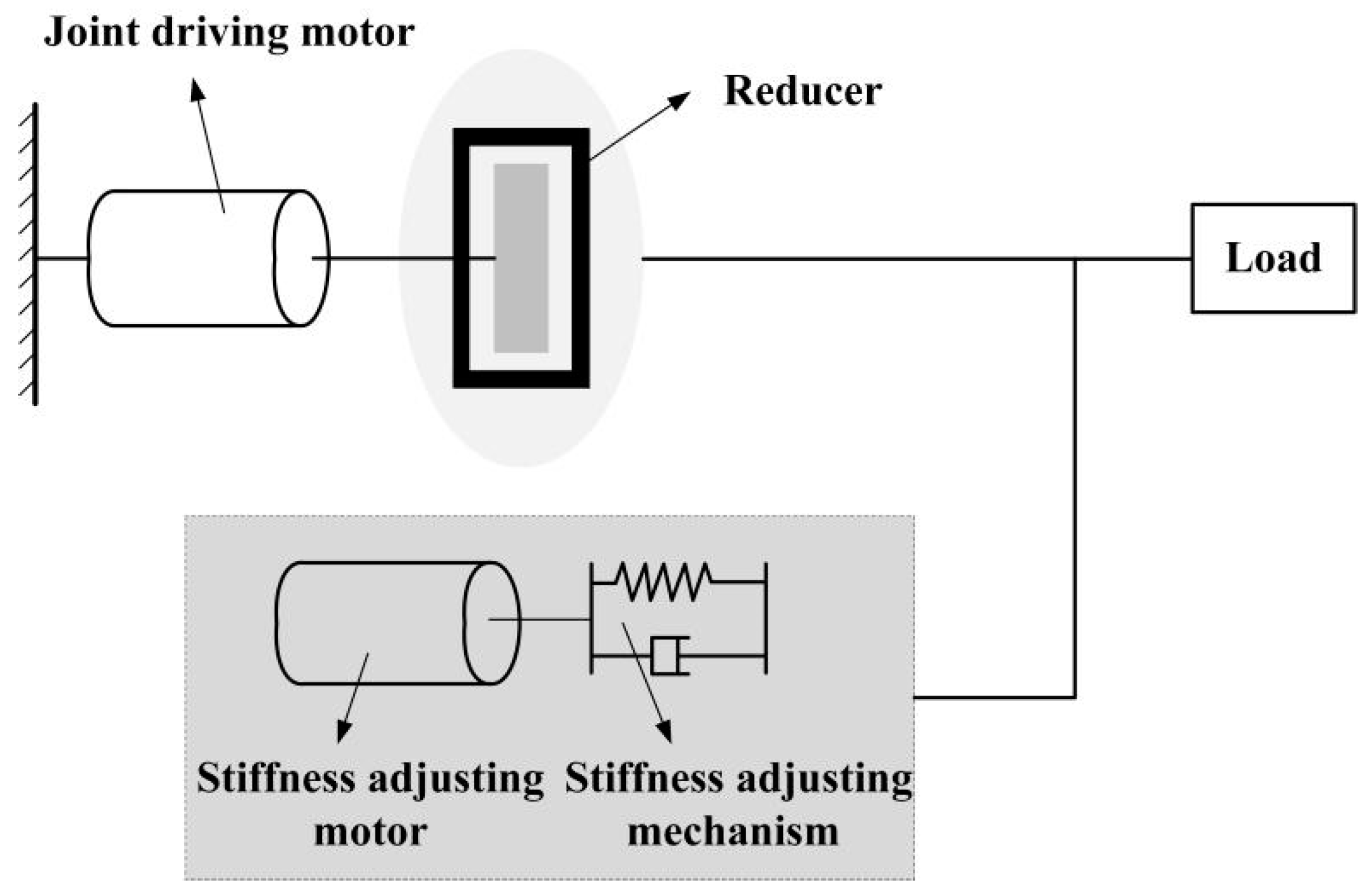
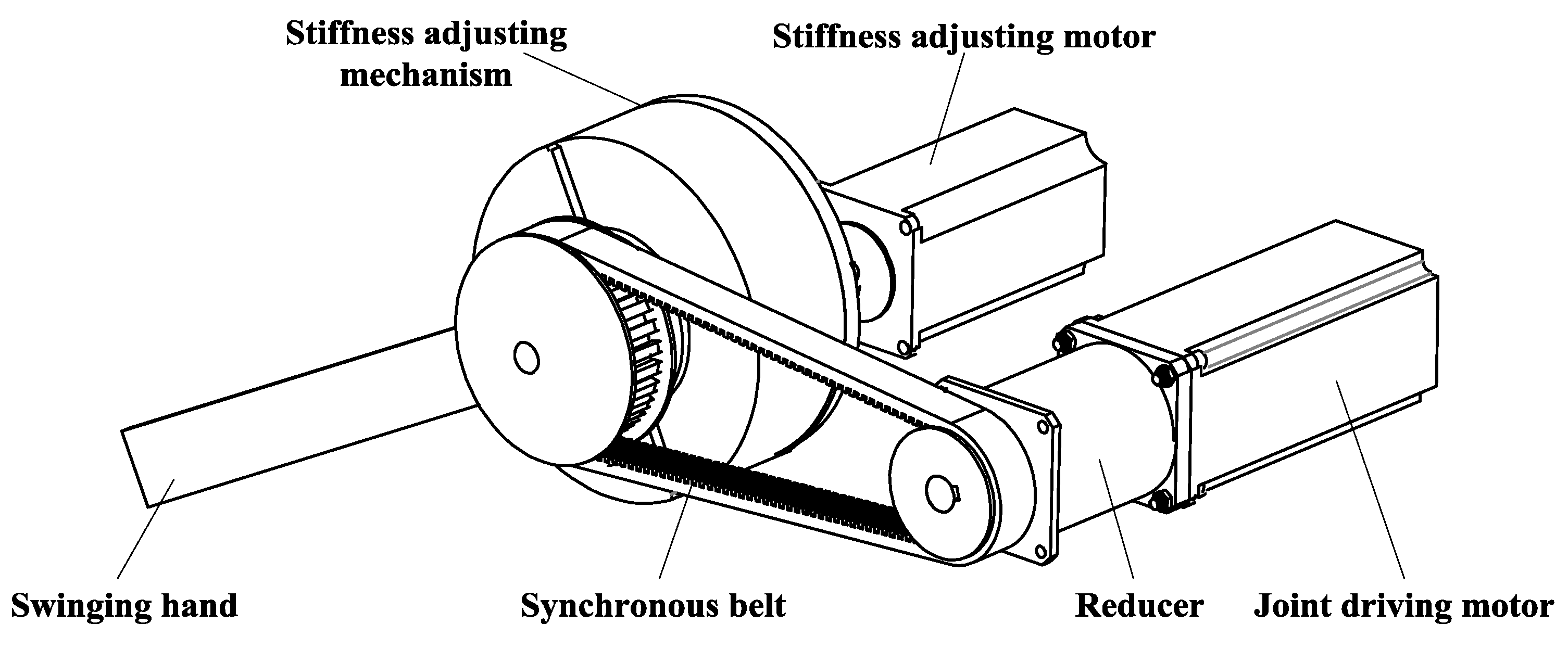
2.1. The Overall Structure of the VSJ-RP
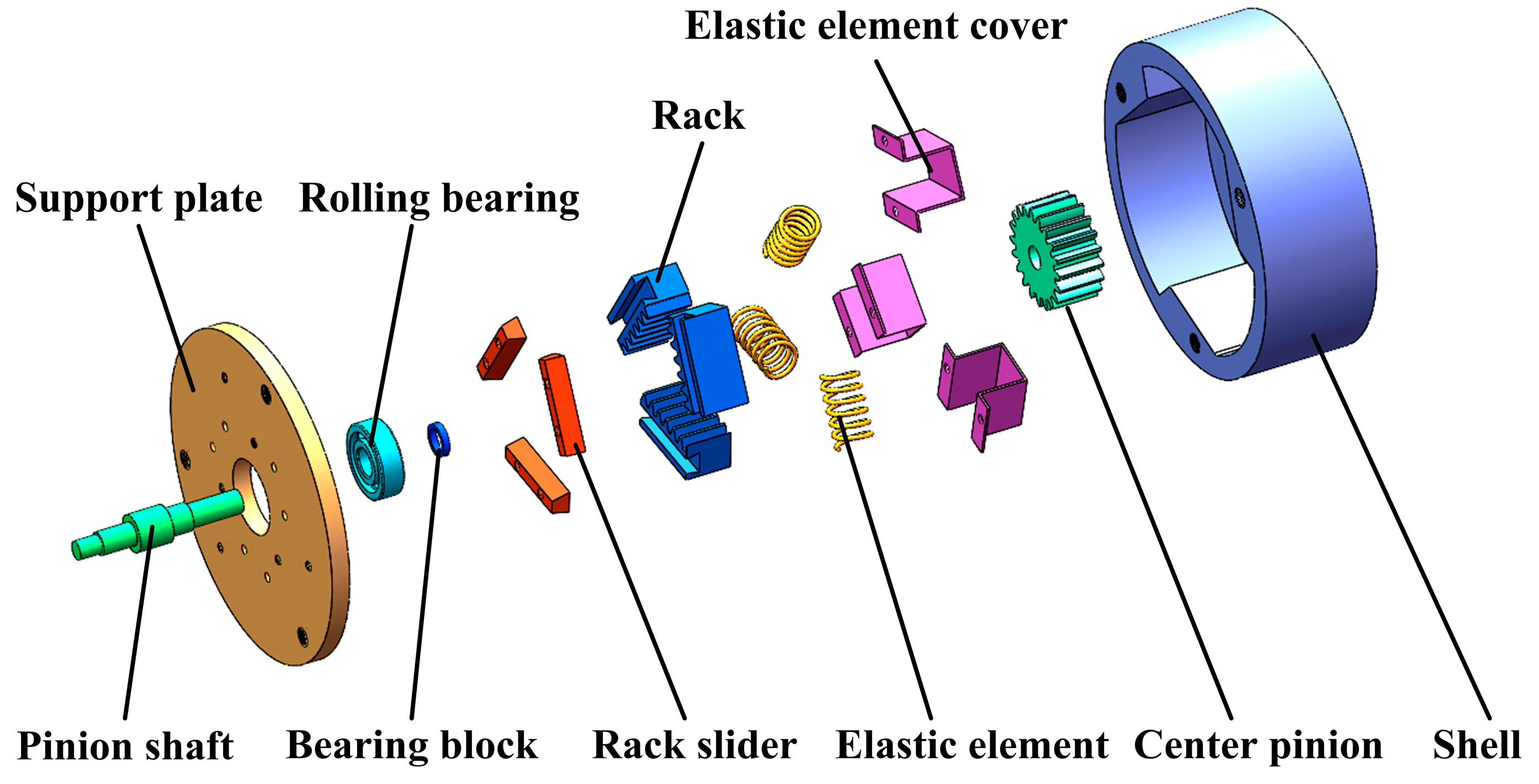
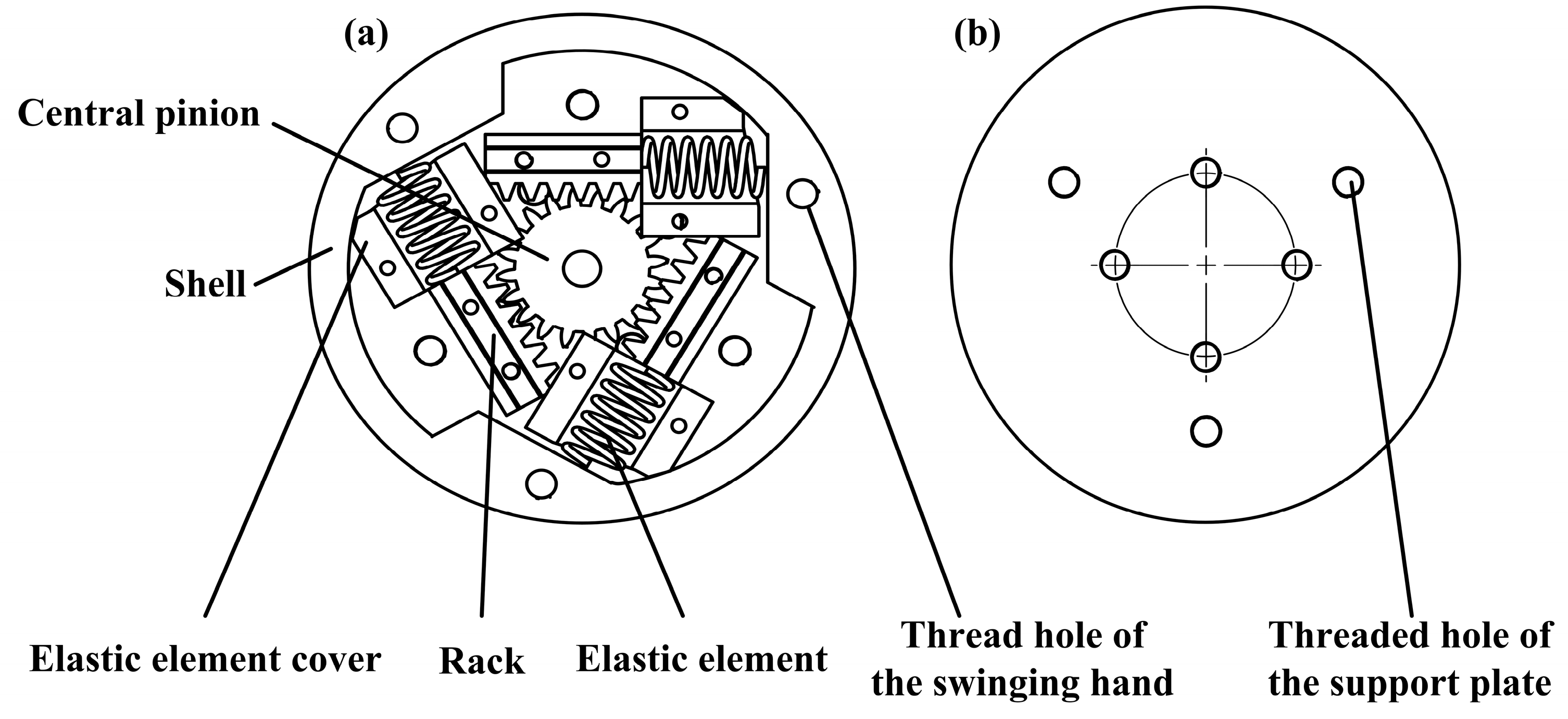
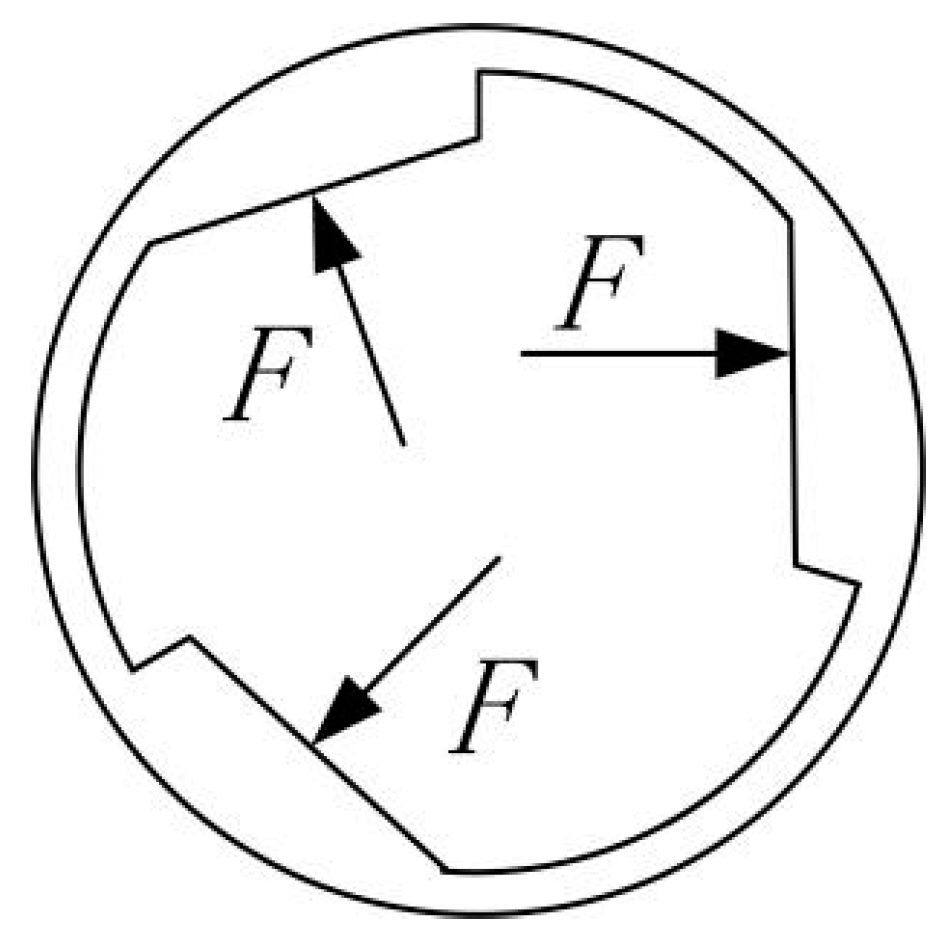
2.2. Mechanical Structure of Stiffness-Adjusting Mechanism
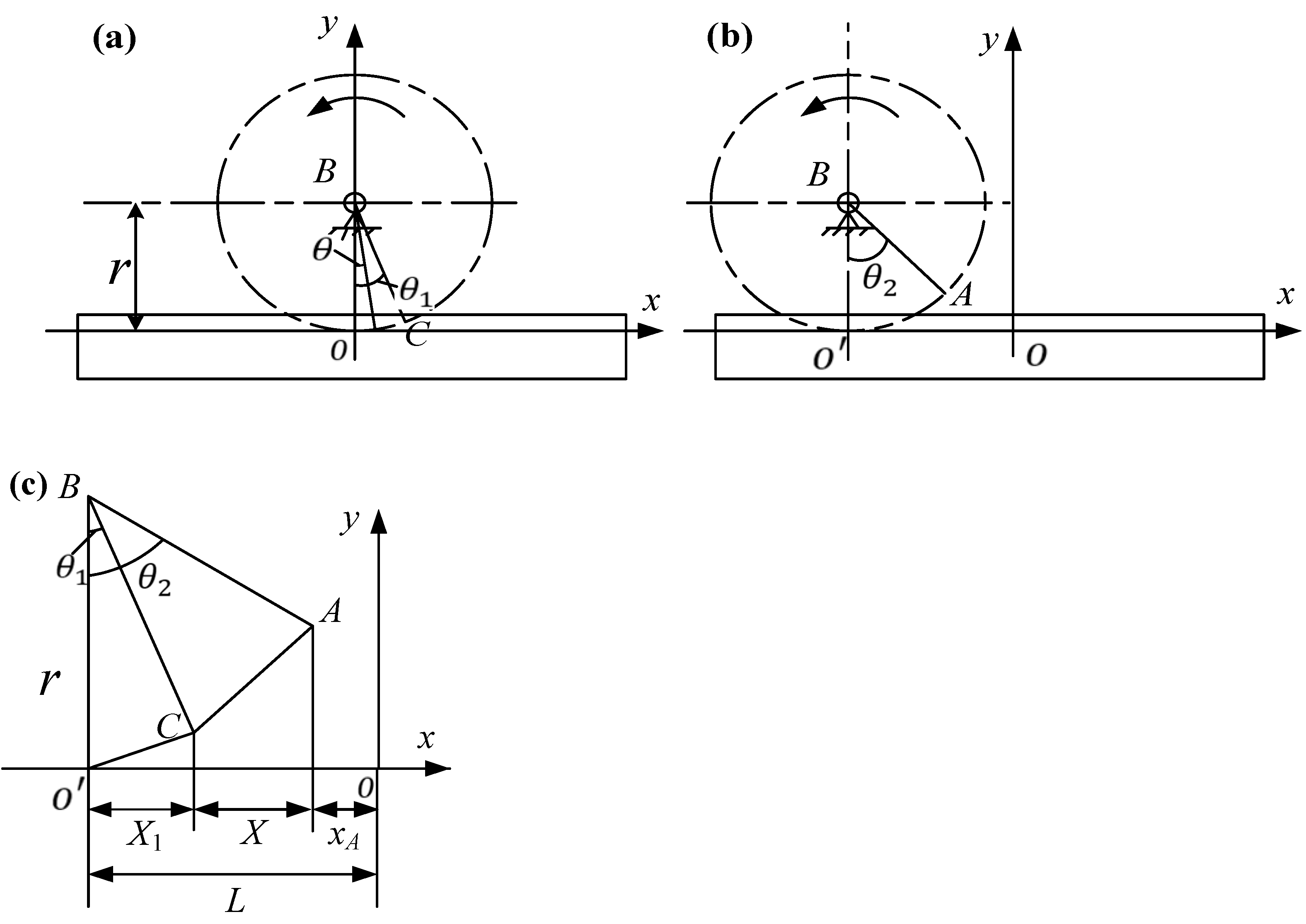
2.3. The VSJ-RP Mathematical Model
- (1)
- The load torque and the stiffness characteristics of the VSJ-RP are mainly determined by the spring type, pinion, and rack parameters. Therefore, different stiffness component types can meet different requirements;
- (2)
- After the parameters of the spring and pinion of the VSJ-RP are determined, the stiffness is mainly affected by the deformation of the spring and the offset angle of the joint.
3. Dynamics Analysis of the VSJ-RP
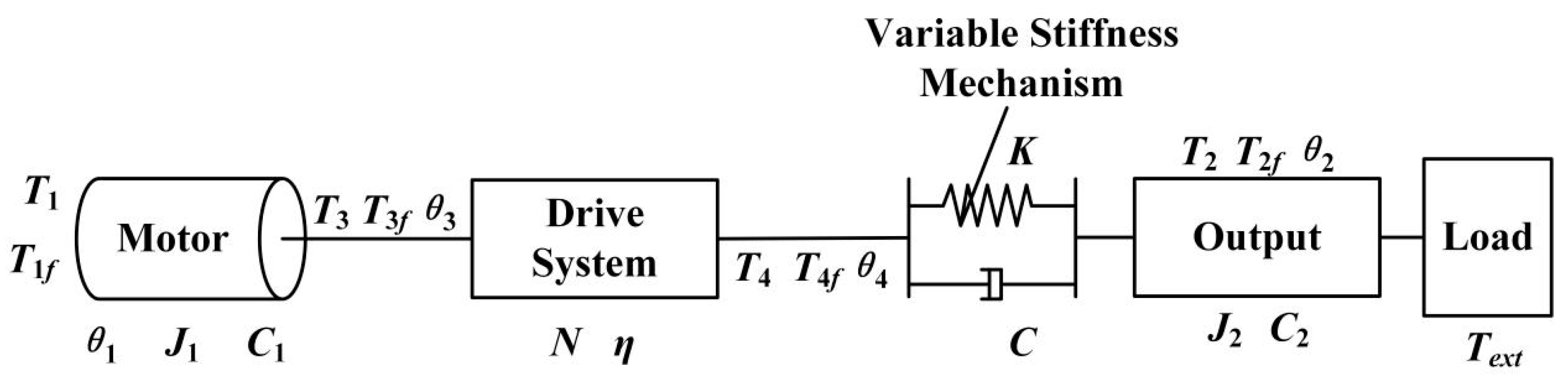
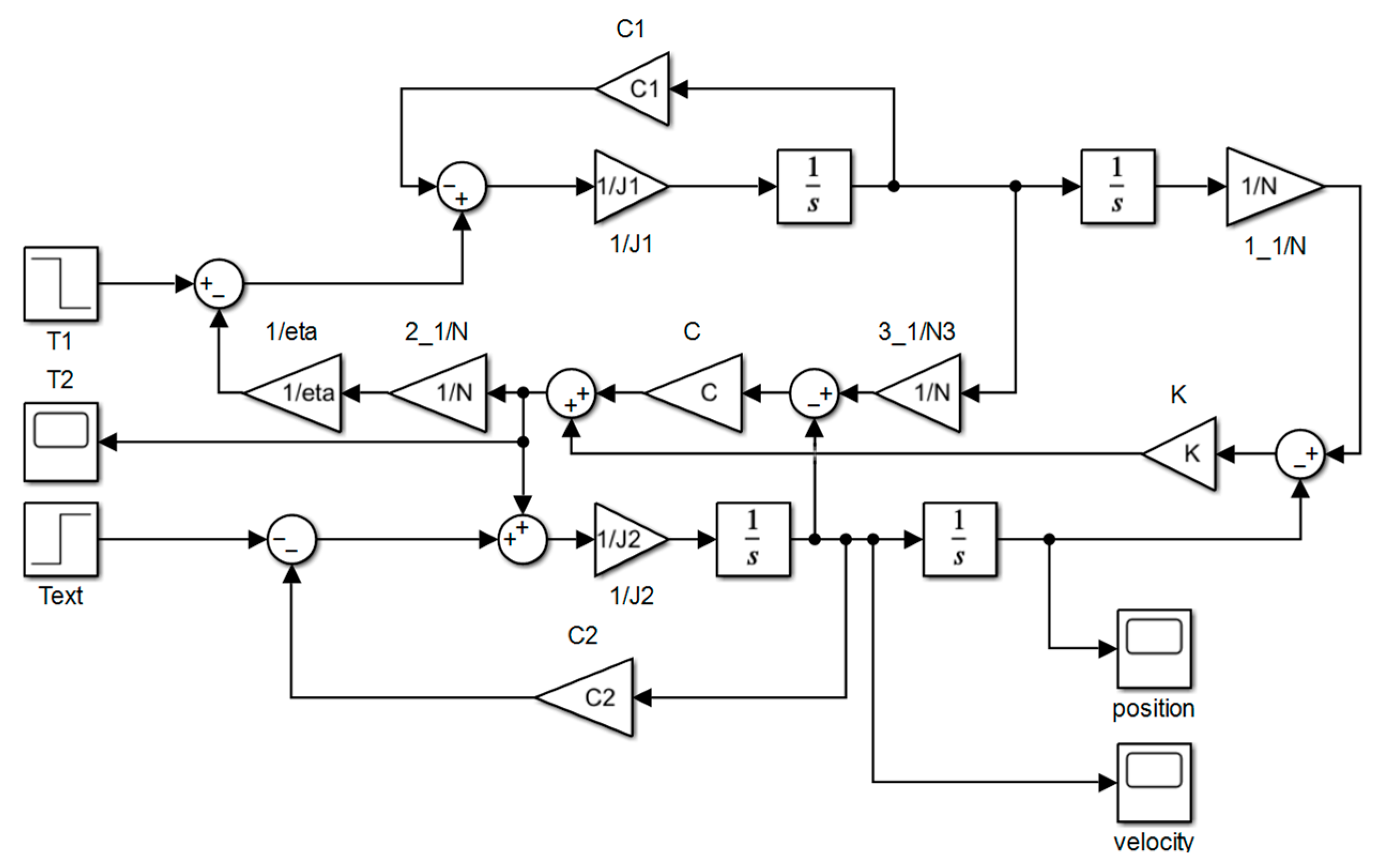
3.1. The VSJ-RP’s Dynamics Model
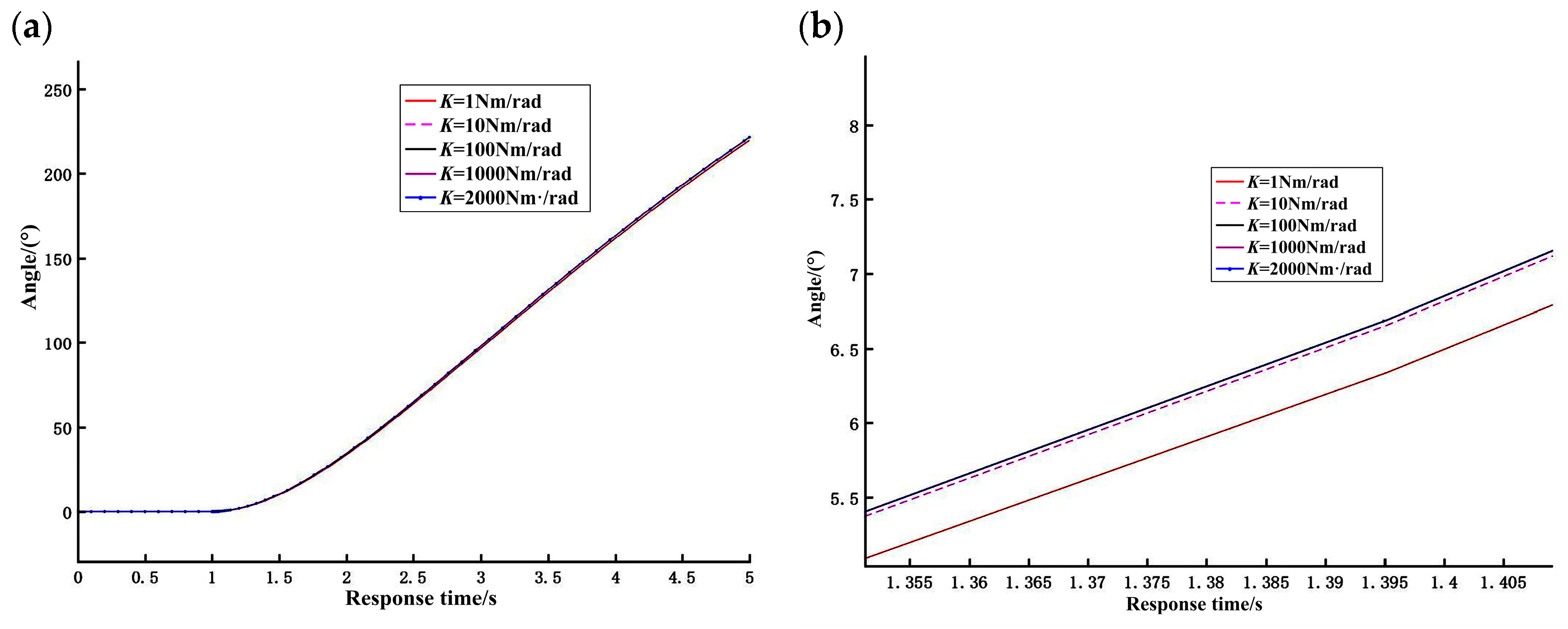
3.2. Dynamics Simulation of the VSJ-RP
4. Experimental Analysis the VSJ-RP Prototype
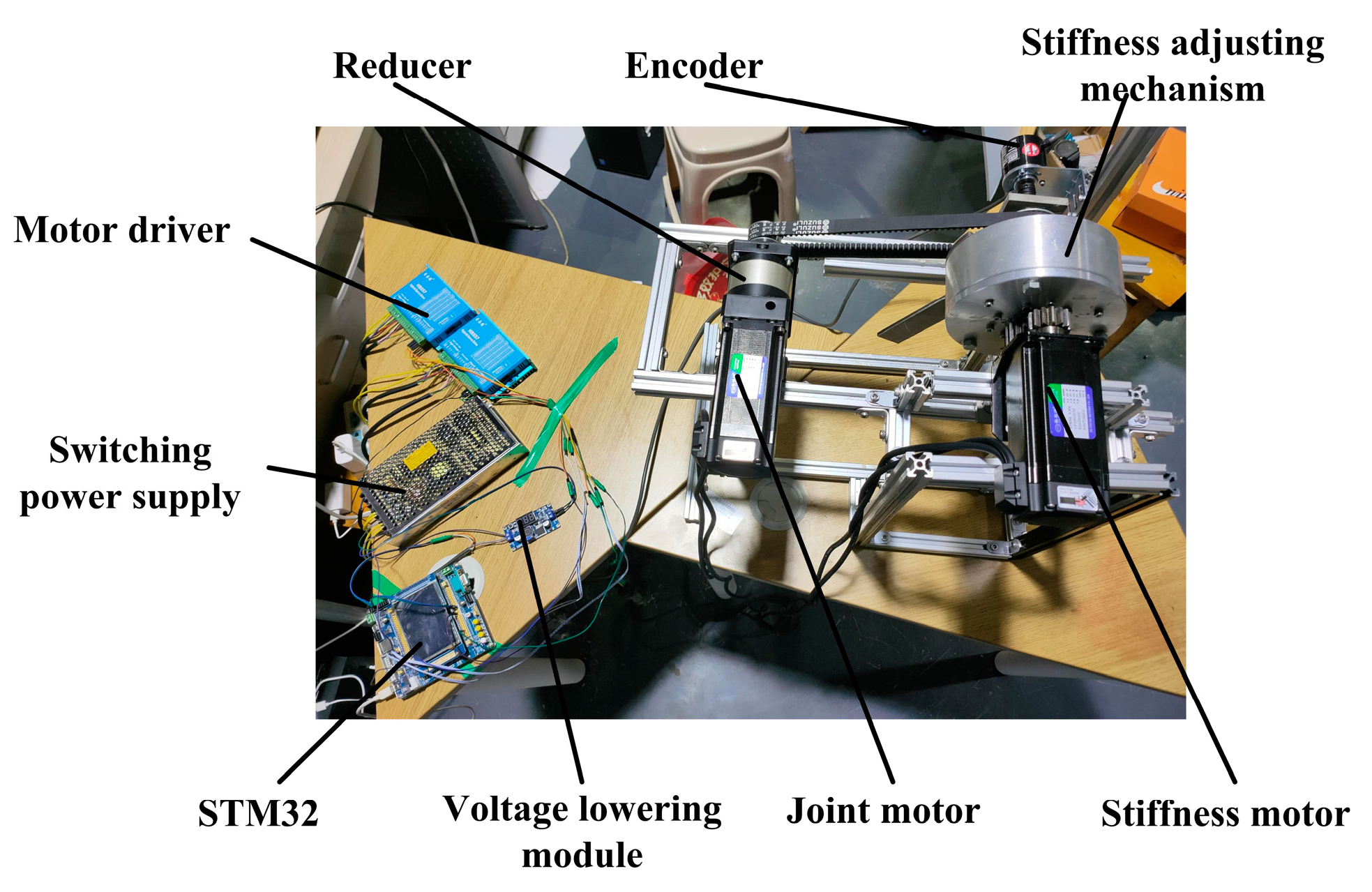
4.1. The Construction of the Experimental Platform
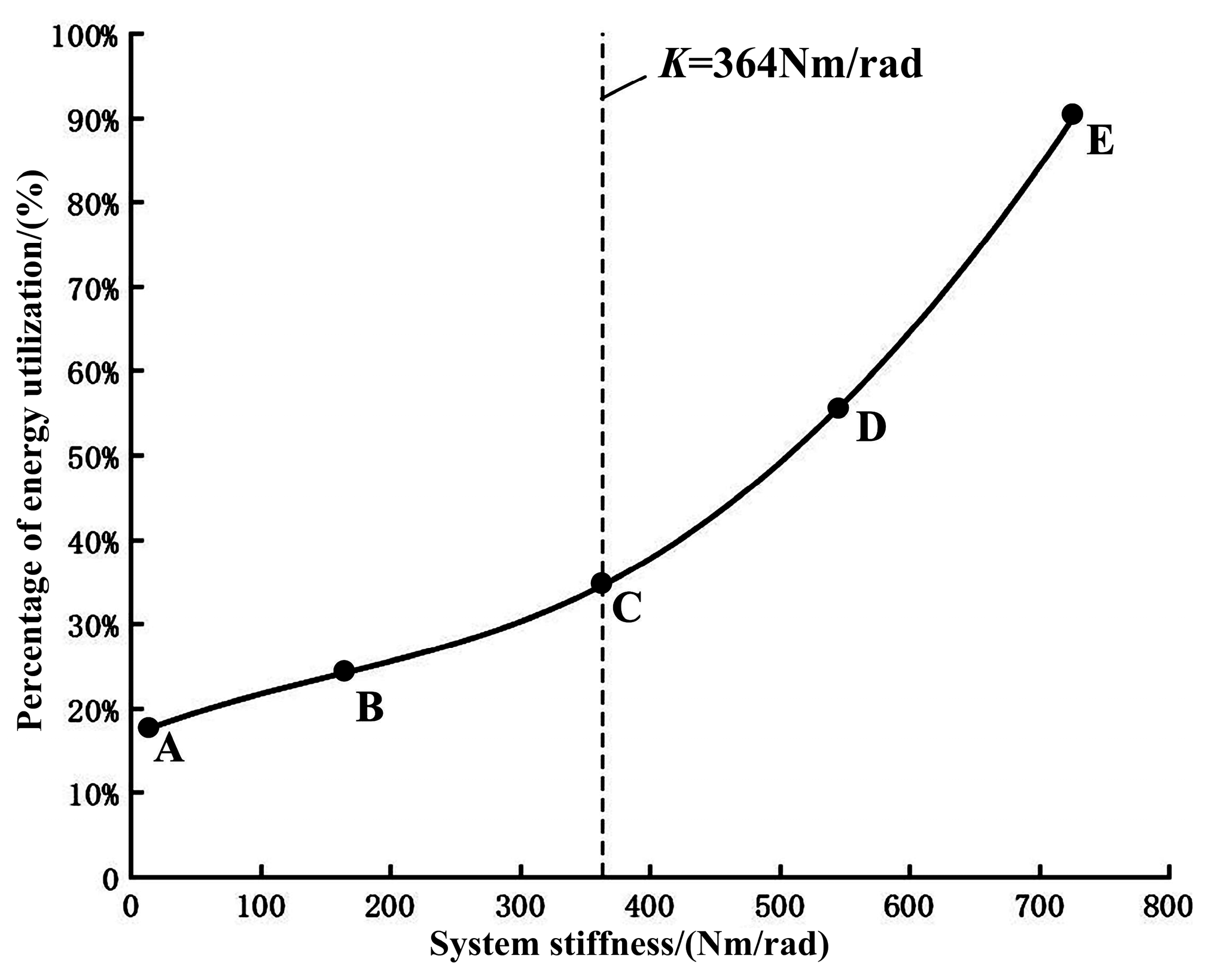
4.2. Joint Stiffness Characteristics’ Verification Experiment
4.3. Location Experiment
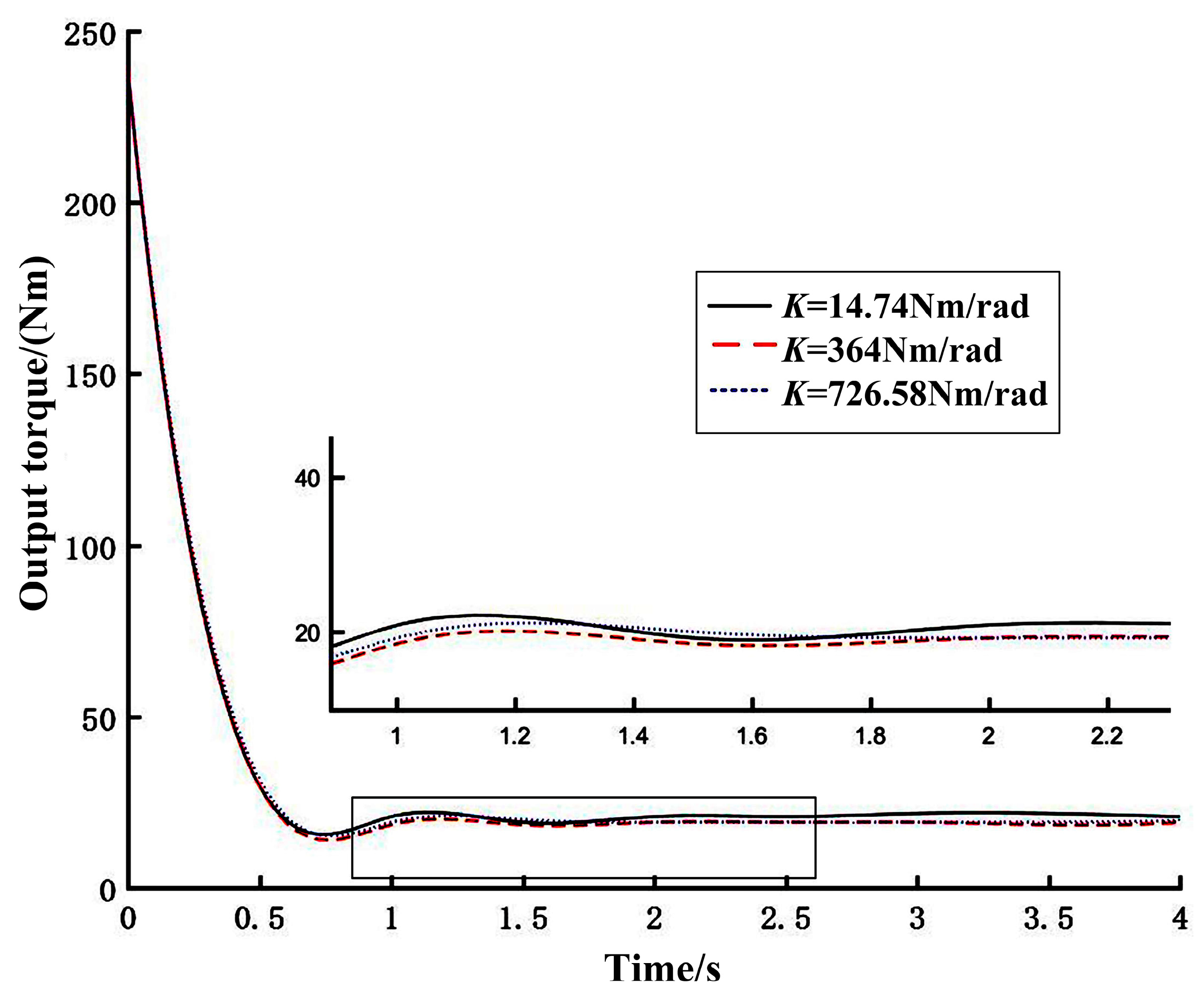
4.4. Torque Test Experiment
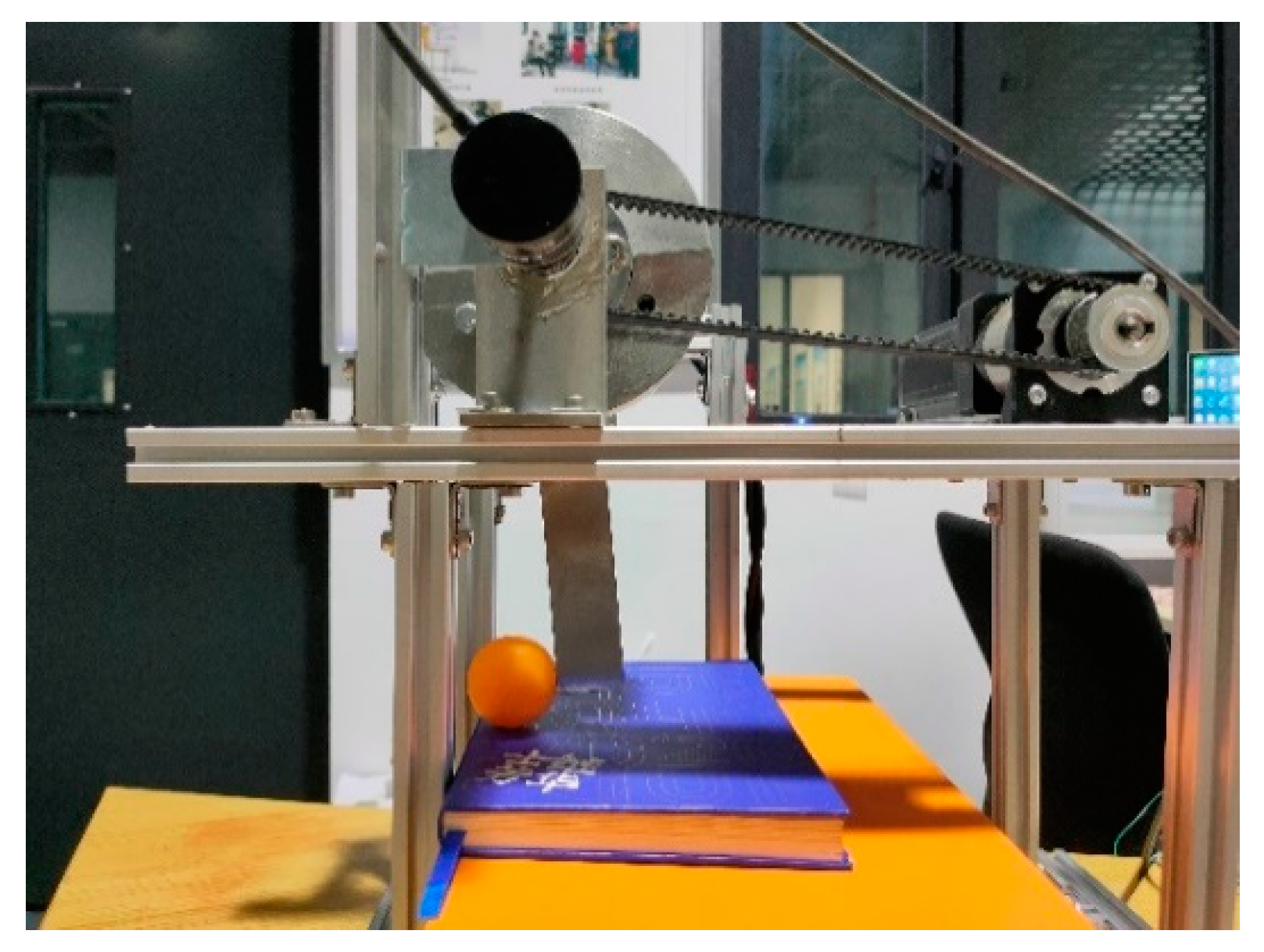
4.5. Hitting Ball Experiment
4.6. Security Test Experiment
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhang, M.; Fang, L.J.; Sun, F.; Sun, X.W. Design and decoupling control method of variable stiffness flexible robot joints. J. Electr. Mach. Control 2019, 23, 120–128. [Google Scholar]
- Xiong, J.; Sun, Y.X.; Zheng, J.; Dong, D.B.; Bai, L. Design and experiment of a SMA-based continuous-stiffness-adjustment torsional elastic component for variable stiffness actuators. Smart Mater. Struct. 2021, 30, 105201. [Google Scholar] [CrossRef]
- Li, M.H.; Ma, Y.Y.; Zhang, M.L. Review of key technologies of bionic flexible joints. Mech. Des. 2018, 35, 1–9. [Google Scholar]
- Baek, S.G.; Moon, H.; Choi, H.R.; Koo, J. A new cam-follower safety joint mechanism design based on variable-length four-bar linkage for robot safety. J. Mech. Robot. 2022, 14, 011004. [Google Scholar] [CrossRef]
- Cai, S.B.; Bao, G.J.; Xu, F.; Zhang, L.B. A review of robot compliant joint research. High-Tech Commun. 2018, 28, 233–243. [Google Scholar]
- Wang, Y.; Fang, L.J.; Zhou, S.Q. Principle and design of mechanically musculoskeletal variable-stiffness mechanism. Robot 2015, 37, 506–512. [Google Scholar]
- Wolf, S.; Grioli, G.; Eiberger, O.; Friedl, W.; Grebenstein, M.; Höppner, H.; Burdet, E.; Caldwell, D.G.; Carloni, R.; Catalano, M.G.; et al. Variable stiffness actuators: Review on design and components. IEEE/ASME Trans. Mechatron. 2016, 21, 2418–2430. [Google Scholar] [CrossRef]
- Sardellitti, I.; Medrano-Cerda, G.A.; Tsagarakis, N.; Jafari, A.; Caldwell, D.G. Gain scheduling control for a class of variable stiffness actuators based on lever mechanisms. IEEE Trans. Robot. 2013, 29, 791–798. [Google Scholar] [CrossRef]
- Zhakatayev, A.; Rubagotti, M.; Varol, H.A. Closed-loop control of variable stiffness actuated robots via nonlinear model predictive control. IEEE Access 2015, 3, 235–248. [Google Scholar] [CrossRef]
- Hollander, K.; Ilg, R.; Sugar, T.G.; Herring, D. An efficient robotic tendon for gait assistance. J. Biomech. Eng. 2006, 10, 788–791. [Google Scholar] [CrossRef]
- Au, S.K.; Herr, H.; Weber, J.; Martinez-Villalpando, E.C. Powered ankle-foot prosthesis for the improvement of amputee ambulation. In Proceedings of the Annual International Conference of the IEEE Engineering in Medicine and Biology Society (EMBC), Lyon, France, 23–26 August 2007; IEEE: Lyon, France, 2007; pp. 3020–3026. [Google Scholar]
- Fumagallim, M.; Barrett, E.; Stramigioli, S.; Carloni, R. The mVSA-UT: A miniaturized differential mechanism for a continuous rotational variable stiffness actuator. In Proceedings of the 2012 4th IEEE RAS & EMBS International Conference on Biomedical Robotics and Biomechatronics (BioRob), Rome, Italy, 24–27 June 2012; pp. 1943–1948. [Google Scholar]
- Groothuis, S.S.; Rusticelli, G.; Zucchelli, A.; Stramigioli, S.; Carloni, R. The vsaUT-II: A novel rotational variable stiffness actuator. In Proceedings of the 2012 IEEE International Conference on Robotics and Automation, St. Paul, MN, USA, 14–19 May 2012; pp. 3355–3360. [Google Scholar]
- Guo, Z.; Pan, Y.P.; Wee, L.B.; Yu, H. Design and control of a novel compliant differential shape memory alloy actuator. Sens. Actuators A Phys. 2015, 225, 71–80. [Google Scholar] [CrossRef]
- Schutter, J.D. A study of active compliant motion control methods for rigid manipulators based on a generic scheme. In Proceedings of the International Conference on Robotics and Automation, Raleigh, NC, USA, 31 March–3 April 1987; pp. 1060–1065. [Google Scholar]
- Lee, Y.T.; Choi, H.R.; Chung, W.K.; Youm, Y. Stiffness control of a coupled tendon-driven robot hand. IEEE Control Syst. Mag. 1994, 14, 10–19. [Google Scholar]
- Shi, Y.L.; Zhang, X.J.; Zhang, M.L. Design and analysis of active-passive compound variable stiffness flexible joint. Chin. J. Mech. Eng. 2018, 54, 55–62. [Google Scholar] [CrossRef]
- Yin, P.; Li, M.T.; Guo, W.; Wang, P.; Fei, S.L. Design and performance test of a new flexible joint with adjustable stiffness for foot robot. Robotics 2014, 36, 322–329. [Google Scholar]
- Shi, H.R.; Xu, Y.; Zhang, Q.Q.; Jiang, X.; Liu, J.; Dong, F.; Zhao, C.; Wang, Y. Mechanism optimization design of explosive-driven monopod bouncing robot. Mech. Des. Res. 2021, 37, 71–76. [Google Scholar]
- Li, M.H.; Ma, Y.Y.; Zhang, M.L.; Zhang, J.H. Design and analysis of variable stiffness bionic flexible joint based on cam mechanism. Instrumentation 2019, 40, 213–222. [Google Scholar]
- Wang, Y.; Fang, L. J Design and experiment of bionic variable stiffness Joint. J. Agric. Mach. 2018, 49, 390–396. [Google Scholar]
- Folgheraiter, M.; Aubakir, B.; Varol, H.A. Thermally-controlled coiled polymeric wire as a novel variable elastic element. In Proceedings of the 2017 IEEE International Conference on Advanced Intelligent Mechatronics (AIM), Munich, Germany, 3–7 July 2017; pp. 466–471. [Google Scholar]
- Shao, Y.X.; Zhang, W.X.; Ding, X.L. Configuration synthesis of variable stiffness mechanisms based on guide-bar mechanisms with length-adjustable links. Mech. Mach. Theory 2021, 156, 104153. [Google Scholar] [CrossRef]
- Fang, L.J.; Zhang, M.; Sun, F.; Koichi, O. Research on the method of compliance control and force compensation of rope-driven variable stiffness joints. Instrumentation 2019, 40, 234–241. [Google Scholar]
- Qu, X.X.; Cao, D.X.; Zhang, S. Design and research of a flexible joint with variable stiffness of torsion spring. Chin. J. Mech. Eng. 2021, 57, 1–10. [Google Scholar]
- Zhou, J.J.; Li, J.; Hu, T.; Shang, H.H. Design and characteristic analysis of joint actuator with antagonistic variable stiffness. Mech. Des. Manuf. 2018, 7, 16–22. [Google Scholar]
- Fu, X.Y.; Wang, W. Analysis and research on dynamic characteristics of variable stiffness joint actuator. Robotics 2017, 39, 466–473. [Google Scholar]







| Ordinal | Designation | Unit | Parameter |
|---|---|---|---|
| 1 | Swinging hand length | 270 | |
| 2 | Swinging hand rotation angle | ° | 0~360 |
| 3 | Synchronous belt length | 600 | |
| 4 | Reducer transmission ratio | / | 1:10 |
| 5 | Motor torque | 3 | |
| 6 | Shell diameter | Φ140 | |
| 7 | Shell height | 58 |
| Symbol | Explanation |
|---|---|
| C, C1, C2 | (Joint, motor, output) equivalent damping |
| K | Joint equivalent stiffness |
| T1, T3 | Motor input and output torque |
| T2 | Joint output torque |
| T4 | Drive system output torque |
| Text | Load torque |
| T1f, T2f, T3f, T4f | Friction torque |
| θ1, θ2, θ3, θ4 | Input and output angle |
| J1, J2 | Moment of inertia of motor and output end |
| Stiffness (Nm/rad) | K1 | K2 | K3 | K4 | K5 |
|---|---|---|---|---|---|
| 14.74 | 175 | 364 | 545 | 726.58 | |
| Hit ball displacement (mm) | 367 | 405 | 441 | 486 | 523 |
| 359 | 411 | 449 | 481 | 519 | |
| 369 | 408 | 447 | 485 | 525 | |
| 362 | 406 | 449 | 489 | 526 | |
| 370 | 409 | 442 | 488 | 520 | |
| Average (mm) | 365.4 | 407.8 | 445.6 | 485.8 | 522.6 |
| Initial velocity v0 (m/s) | 1.81 | 2.08 | 2.21 | 2.41 | 2.59 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jin, H.; Luo, M.; Lu, S.; He, Q.; Lin, Y. Design and Analysis of a Novel Variable Stiffness Joint for Robot. Actuators 2023, 12, 10. https://doi.org/10.3390/act12010010
Jin H, Luo M, Lu S, He Q, Lin Y. Design and Analysis of a Novel Variable Stiffness Joint for Robot. Actuators. 2023; 12(1):10. https://doi.org/10.3390/act12010010
Chicago/Turabian StyleJin, Hui, Mulin Luo, Shiqing Lu, Qingsong He, and Yuanchang Lin. 2023. "Design and Analysis of a Novel Variable Stiffness Joint for Robot" Actuators 12, no. 1: 10. https://doi.org/10.3390/act12010010
APA StyleJin, H., Luo, M., Lu, S., He, Q., & Lin, Y. (2023). Design and Analysis of a Novel Variable Stiffness Joint for Robot. Actuators, 12(1), 10. https://doi.org/10.3390/act12010010









