Sound Driven Actuator Using Helmholtz Resonance
Abstract
1. Introduction
2. Related Works
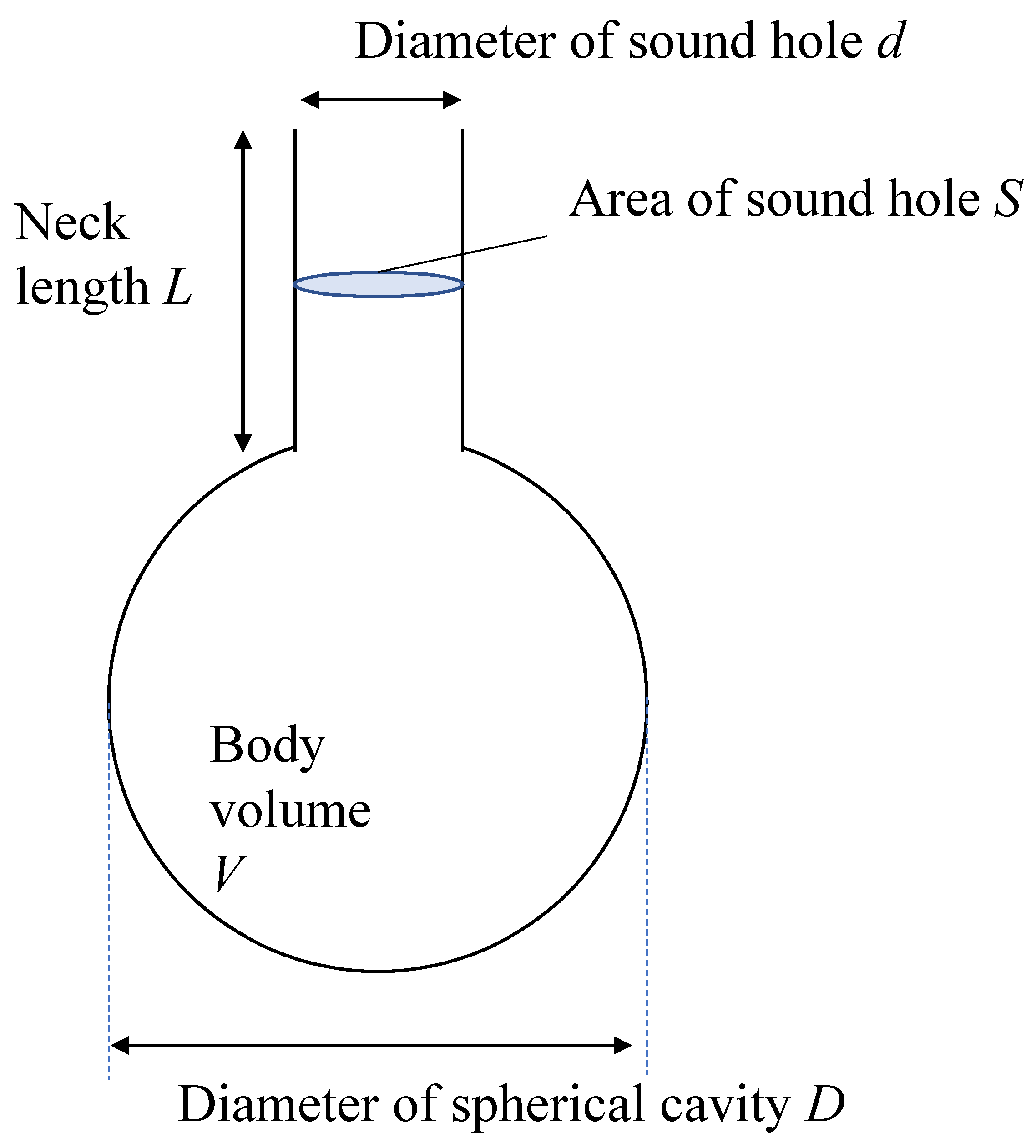
3. Physics of Helmholtz Resonance
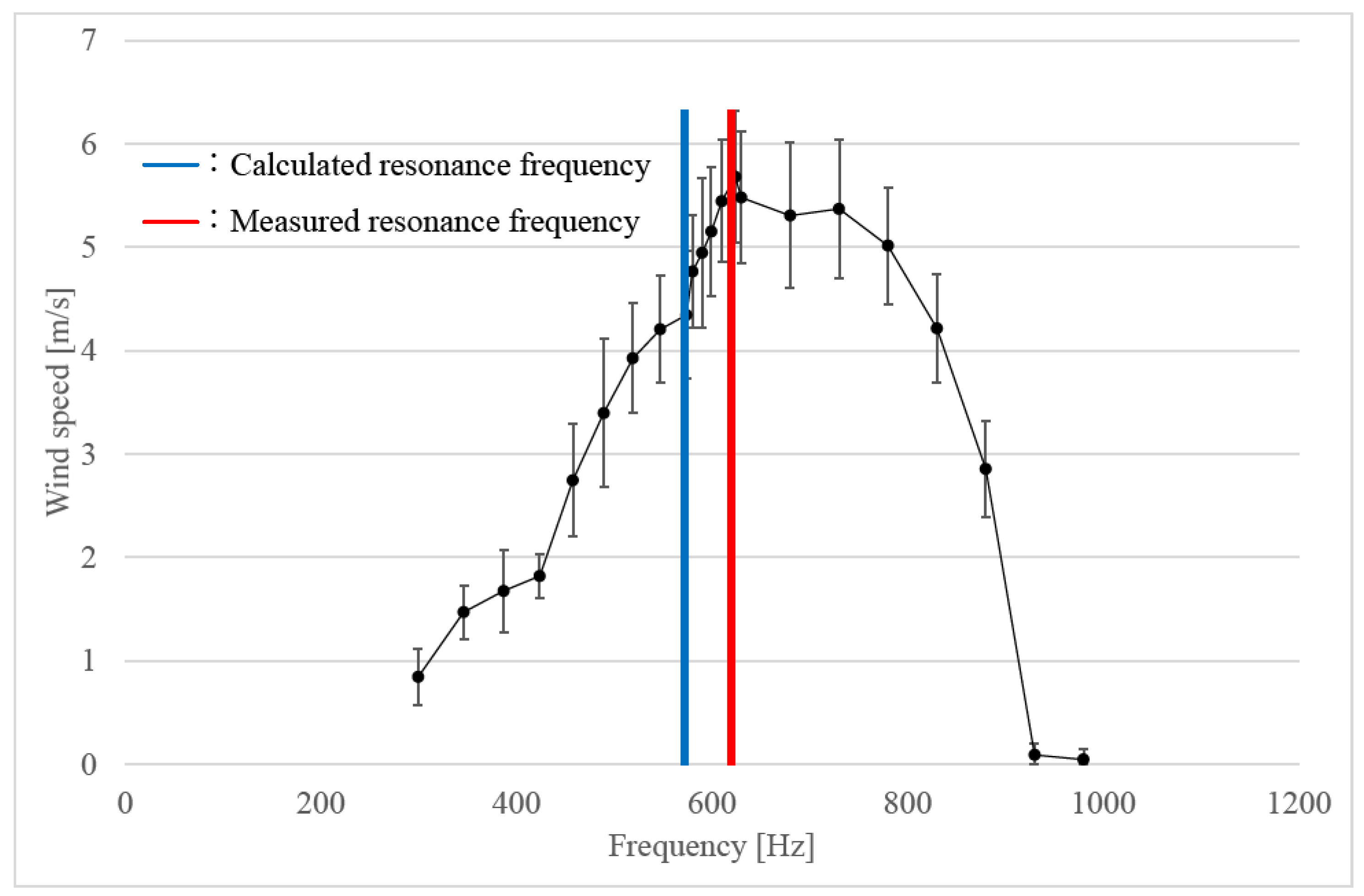
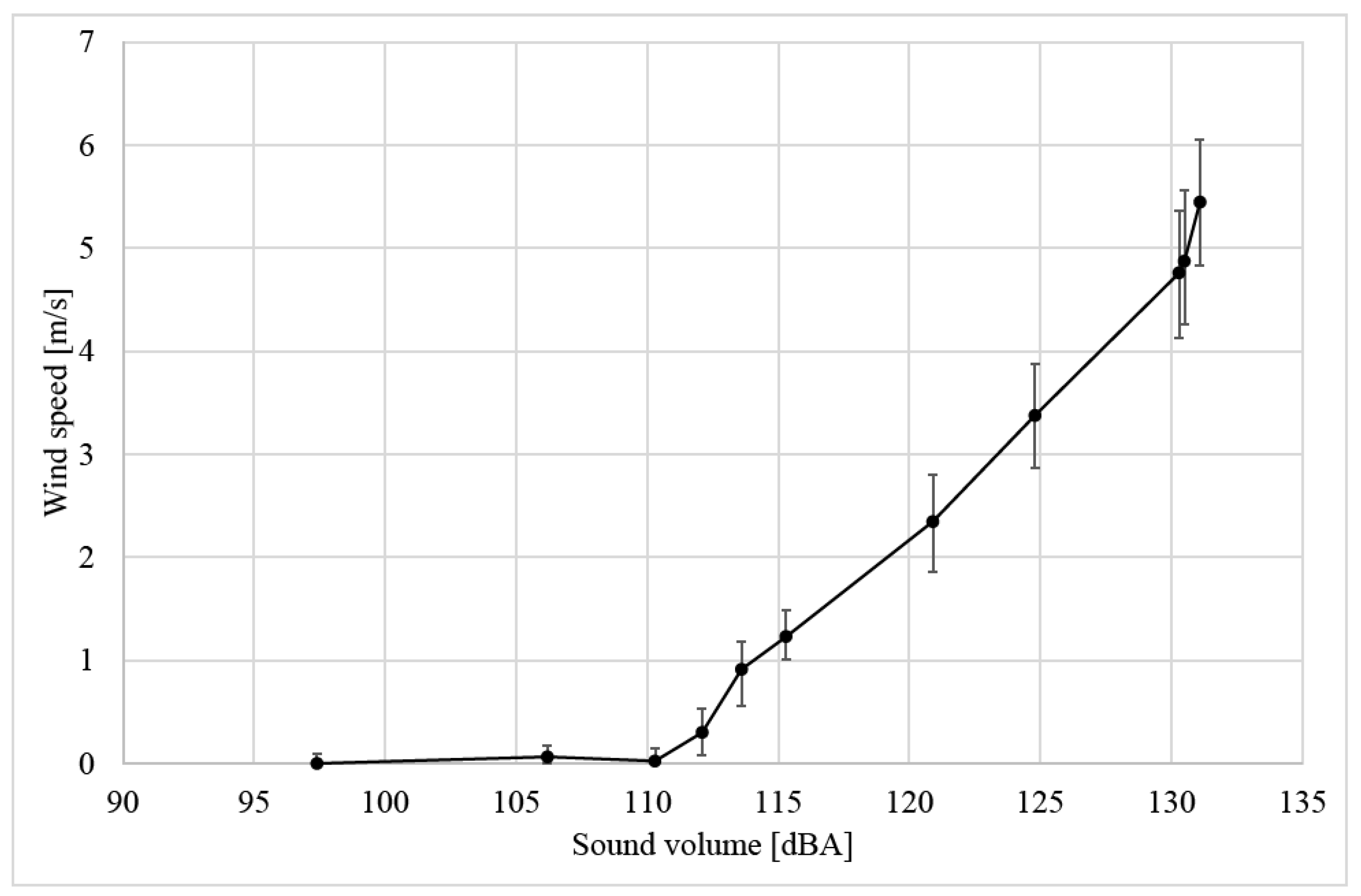
4. Measurement of Wind Speed Depending on Sound Frequency
5. Sound Driven Actuator
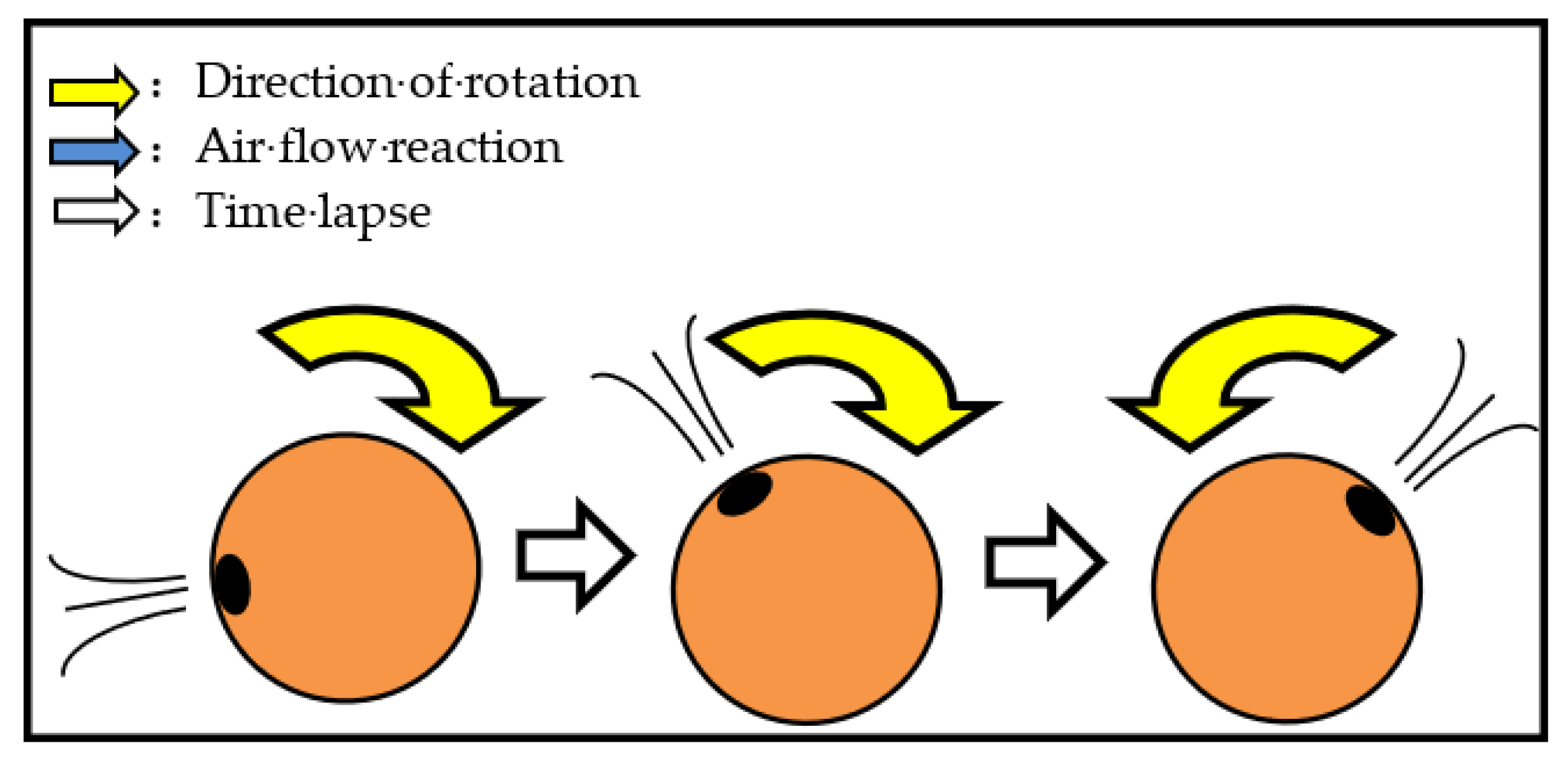
5.1. Physical Characteristics of the Ball as an Actuator
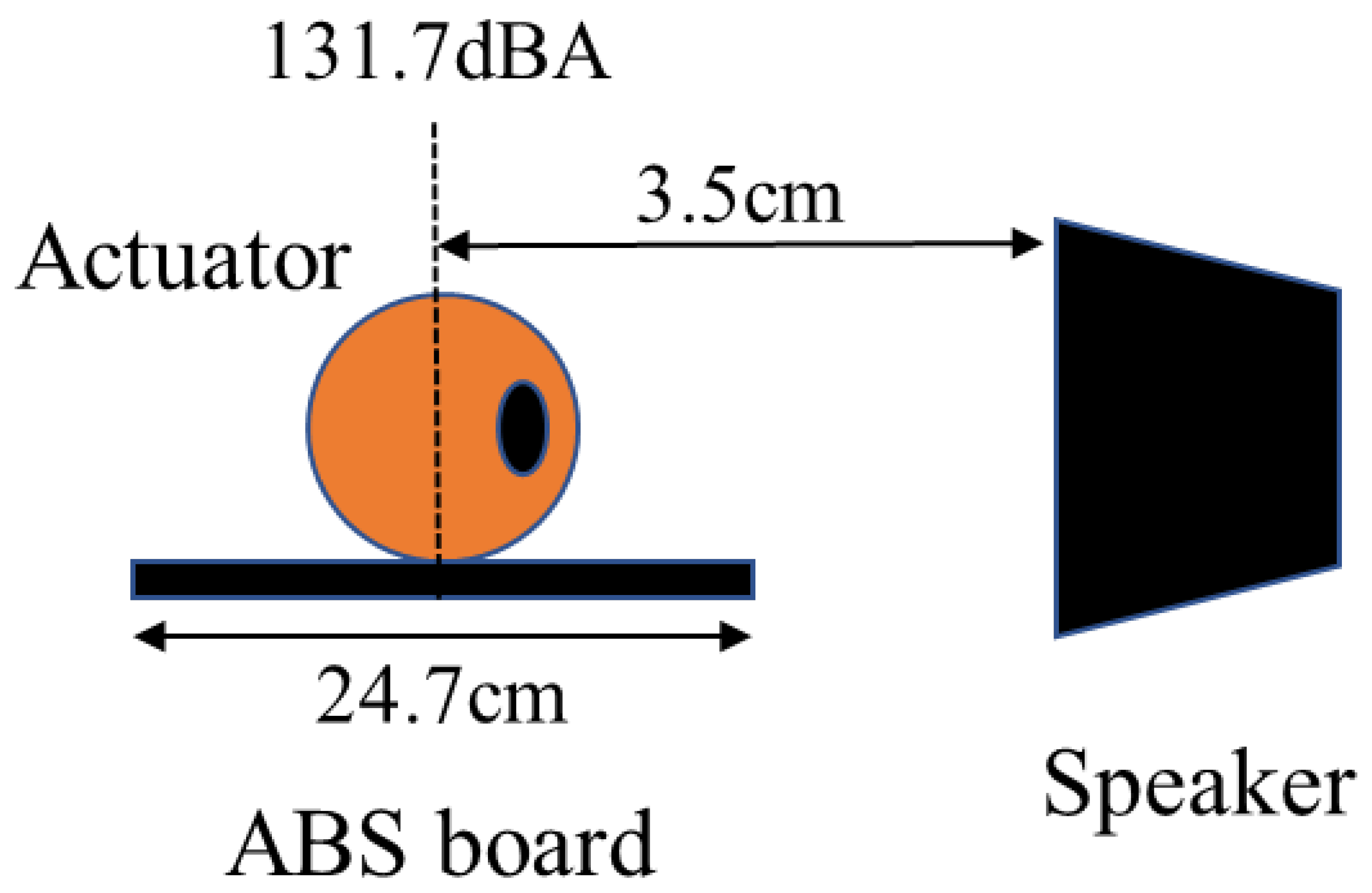
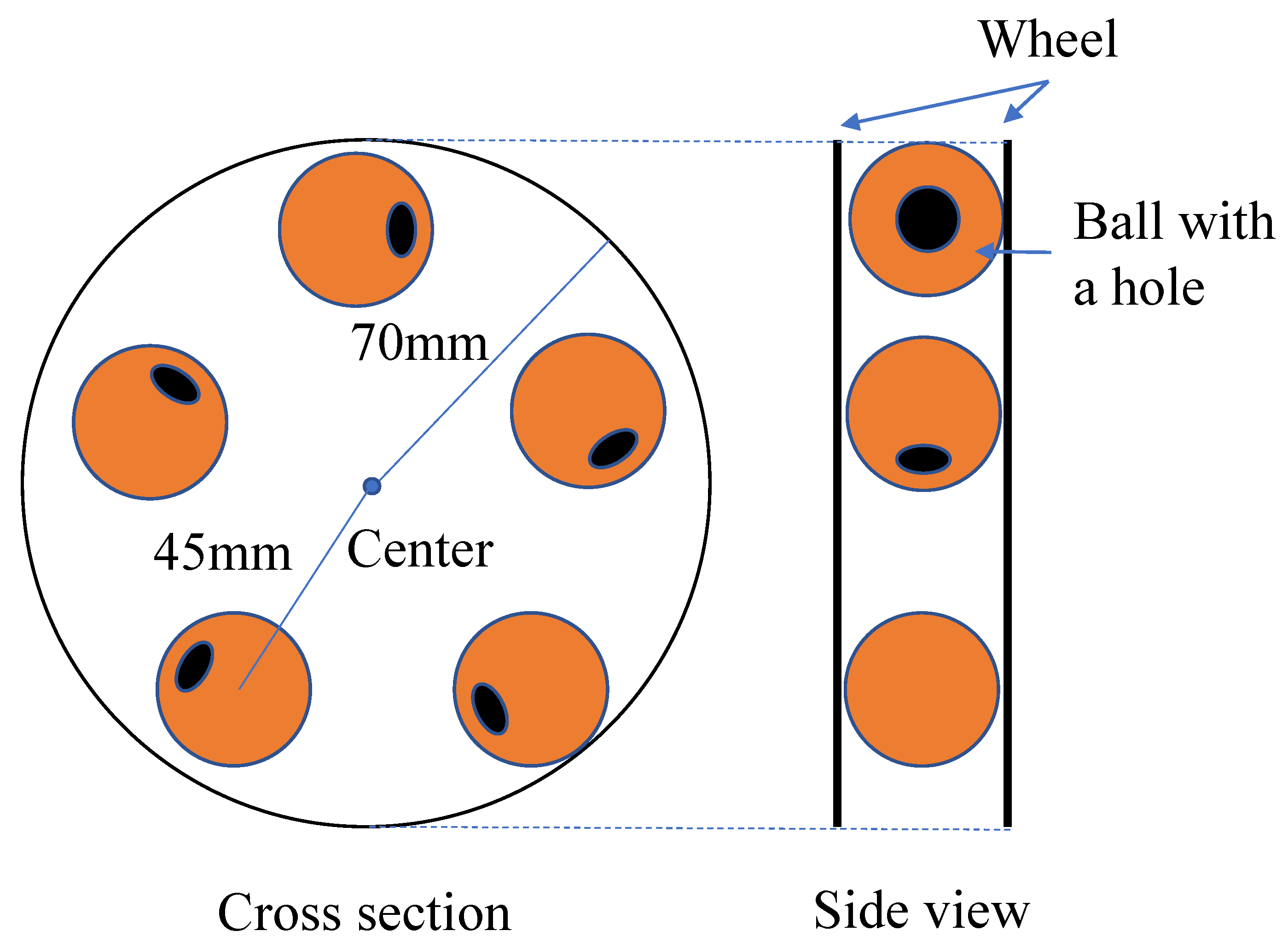
5.2. Design of the Actuator
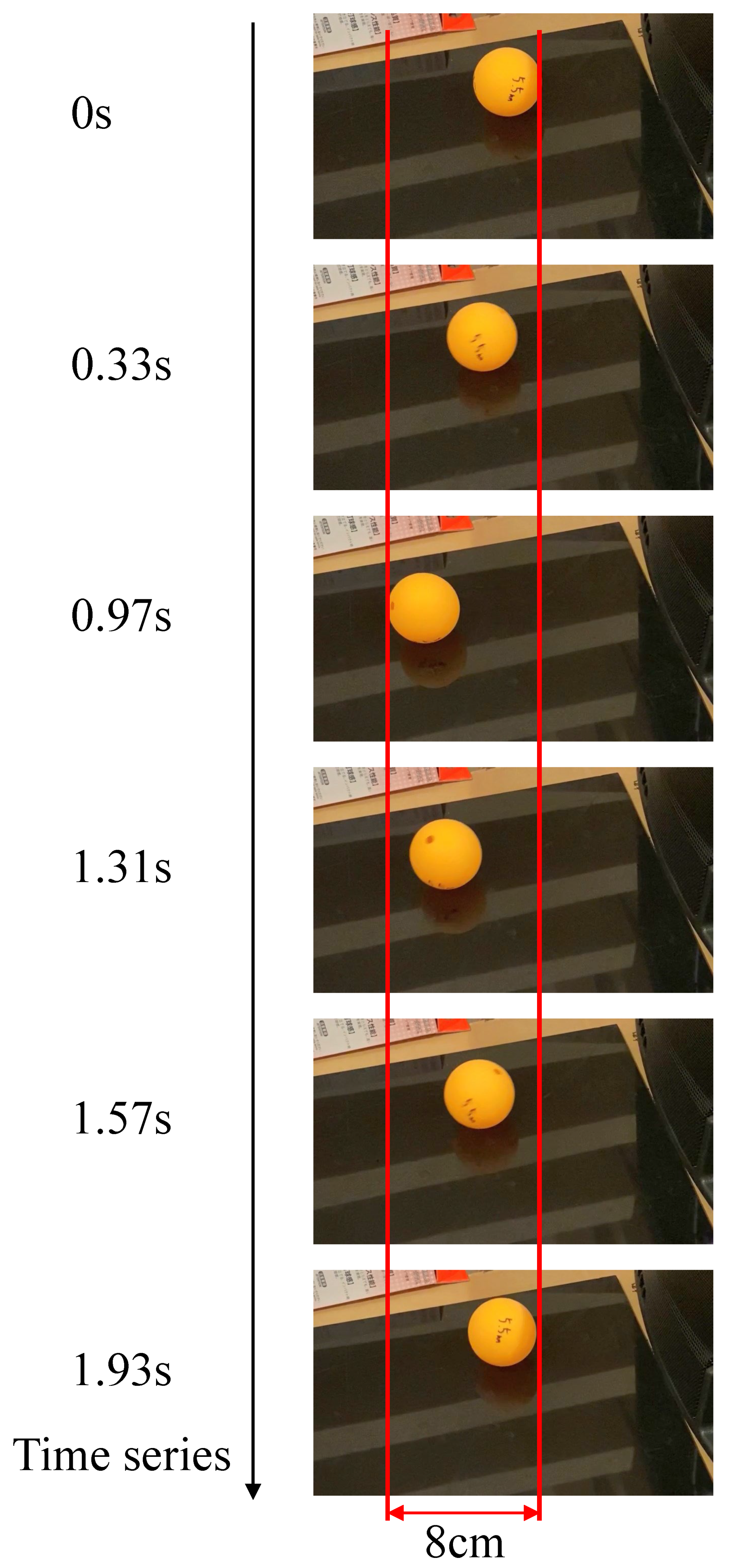
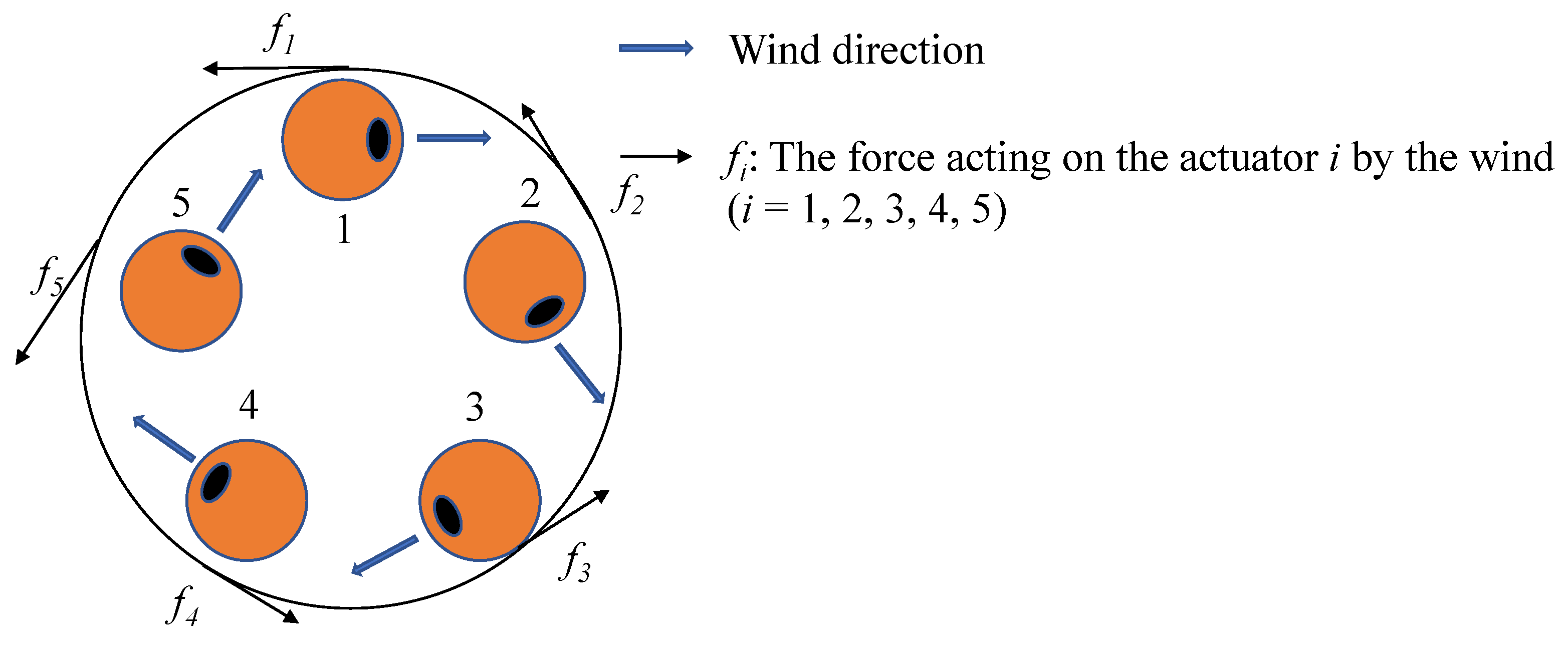
5.3. Results of the Migration Experiments
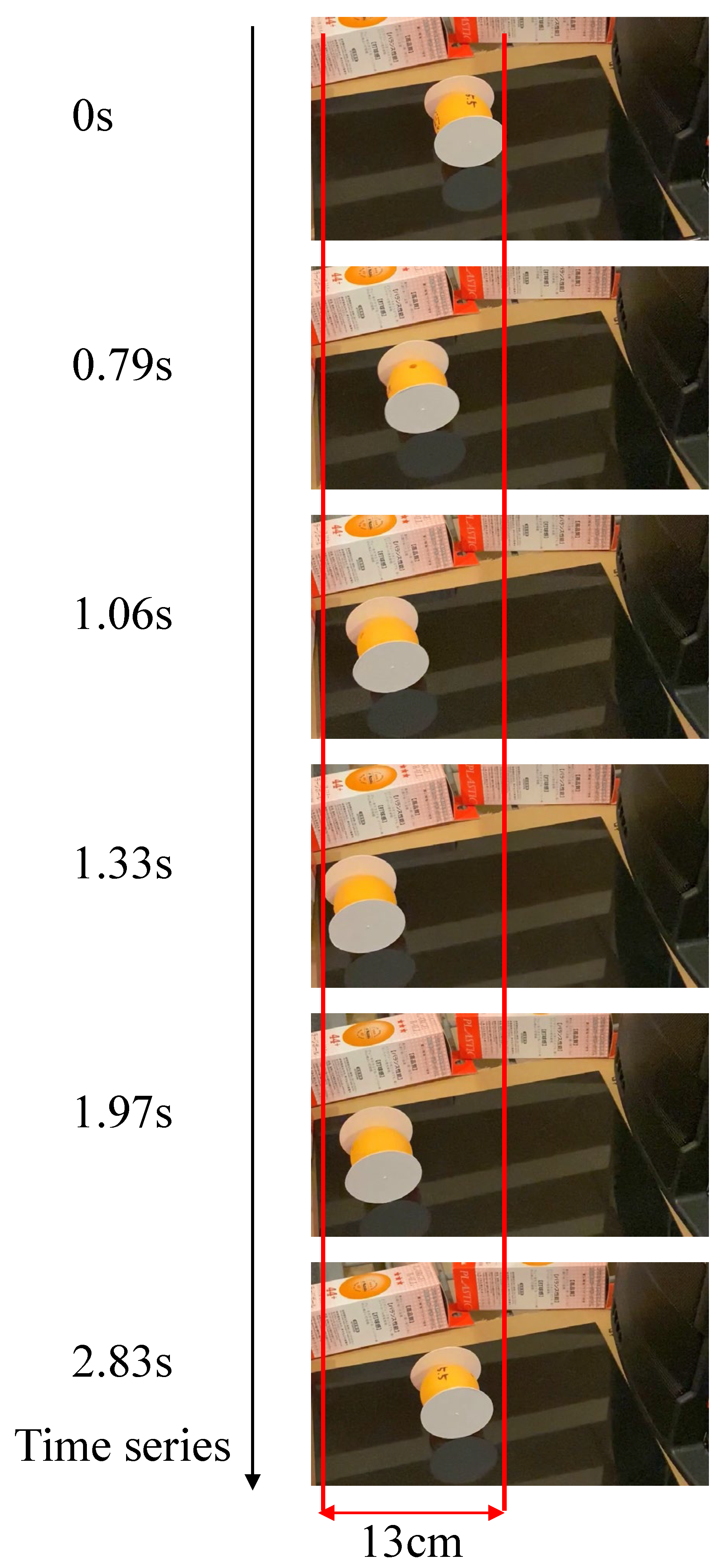
5.4. Redesign of the Actuator
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Gregory, J. Application of Multi-Robot Systems to Disaster-Relief Scenarios with Limited Communication. Field Serv. Robot. 2016, 113, 639–653. [Google Scholar]
- Kamegawa, T.; Akiyama, T.; Sakai, S.; Fujii, K.; Une, K.; Ou, E.; Matsumura, Y.; Kishutani, T.; Nose, E.; Yoshizaki, Y.; et al. Development of a separable search-and-rescue robot composed of a mobile robot and a snake robot. Adv. Robot. 2020, 34, 132–139. [Google Scholar] [CrossRef]
- Deng, W.; Huang, K.; Chen, X.; Zhou, Z.; Shi, C.; Guo, R.; Zhang, H. Semantic RGB-D SLAM for Rescue Robot Navigation. IEEE Access 2020, 8, 221320–221329. [Google Scholar] [CrossRef]
- ANNnewsCH, “[Nuclear Power] Domestic Robot Left Behind in Reactor Building (11/10/21)”. Available online: https://www.youtube.com/watch?v=9AV4i6-2Q4s (accessed on 14 March 2022).
- Kawauchi, N.; Shiotani, S.; Kanazawa, H.; Sasaki, T.; Tsuji, H. A plant maintenance humanoid robot system. In Proceedings of the IEEE International Conference on Robotics and Automation, Taipei, Taiwan, 14–19 September 2003; pp. 2973–2978. [Google Scholar]
- Shibata, T.; Sasaya, T.; Kawahara, N. Development of In-Pipe Microrobot Using Microwave Energy Transmission. Electron. Commun. Jpn. 2000, 84, 1–8. [Google Scholar] [CrossRef]
- Huruta, T.; Yoshida, T.; Nishimura, T.; Yamato, H. Development of the Exploring Robot toward Future Indoor Surveillance Missions in the Fukushima Daiichi Nuclear Power Plant. J. Robot. Soc. Jpn. 2014, 32, 92–97. [Google Scholar]
- Onishi, K.; Onishi, N.; Fujita, J.; Hara, K.; Hashimoto, T. Design and Development of Robots which Support Activities of Recovery from Nuclear Hazards. J. Robot. Soc. Jpn. 2014, 32, 816–824. [Google Scholar] [CrossRef][Green Version]
- Nagatani, K.; Kiribayashi, S.; Okada, Y.; Otake, K.; Yoshida, K.; Tadokoro, S.; Nishimura, T.; Yoshida, T.; Koyanag, E.; Fukushima, M.; et al. Emergency response to the nuclear accident at the Fukushima Daiichi Nuclear Power Plants using mobile rescue robots. J. Field Robot. 2013, 30, 44–63. [Google Scholar] [CrossRef]
- Tsiligiannis, G.; Touboul, A.; Bricas, G.; Maraine, T.; Boch, J.; Wrobel, F.; Michez, A.; Saigne, F.; Godot, A.; Etile, A.; et al. Evaluation and Analysis of Technologies for Robotic Platforms for the Nuclear Decommissioning. In Proceedings of the 15th International Conference on Design and Technology of Integrated Systems in Nanoscale Era, Marrakech, Morocco, 1–3 April 2020. [Google Scholar]
- Han, Z.; Duan, X.; Wang, Y.; Pan, Y.; Wu, Y. Robot System and Fastening Force Control for Sealing Blocking Plates of Steam Generator. In Proceedings of the 9th IEEE International Conference on CYBER Technology in Automation, Control, and Intelligent Systems, Suzhou, China, 29 July–2 August 2019. [Google Scholar]
- Nagatani, K.; Kiribayashi, S.; Okada, Y.; Tadokoro, S.; Nishimura, T.; Yoshida, T.; Koyanagi, E.; Hada, Y. Redesign of rescue mobile robot Quince. In Proceedings of the 2011 IEEE International Symposium on Safety, Security and Rescue Robotics, Kyoto, Japan, 1–5 November 2011. [Google Scholar]
- Dong, P.; Wang, X.; Xing, H.; Liu, Y.; Zhang, M. Design and control of a tracked robot for search and rescue in nuclear power plant. In Proceedings of the 2016 International Conference on Advanced Robotics and Mechatronics, Macau, China, 18–20 August 2016. [Google Scholar]
- Zolfagharian, A.; Durran, L.; Gharaie, S.; Rolfe, B.; Kaynak, A.; Bodaghi, M. 4D printing soft robots guided by machine learning and finite element models. Sens. Actuators A Phys. 2021, 328, 112774. [Google Scholar] [CrossRef]
- Wang, Z.; Hirai, S. Analytical Modeling of a Soft Pneu-Net Actuator Subjected to Planar Tip Contact. IEEE Trans. Robot. 2022, 1–14. [Google Scholar] [CrossRef]
- Zolfagharian, A.; Mahmud, M.A.P.; Gharaie, S.; Bodaghi, M.; Kouzani, A.Z.; Kaynak, A. 3D/4D-printed bending-type soft pneumatic actuators: Fabrication, modelling, and control. Virtual Phys. Prototyp. 2020, 15, 373–402. [Google Scholar] [CrossRef]
- Hawkes, E.W.; Blumenschein, L.H.; Greer, J.D.; Okamura, A.M. A soft robot that navigates its environment through growth. Sci. Robot. 2017, 2, eaan3028. [Google Scholar] [CrossRef] [PubMed]
- Nishikawa, Y.; Matsumoto, M. Lightweight indestructible soft robot. IEEJ Trans. Electr. Electron. Eng. 2018, 13, 652–653. [Google Scholar] [CrossRef]
- Tondu, B.; Lopez, P. Modeling and control of McKibben artificial muscle robot actuators. IEEE Control Syst. 2000, 20, 15–38. [Google Scholar] [CrossRef]
- Tondu, B. Modelling of the McKibben artificial muscle: A review. J. Intell. Mater. Syst. Struct. 2012, 23, 225–253. [Google Scholar] [CrossRef]
- Boxerbaum, A.; Chiel, H.J.; Quinn, R.D. A New Theory and Methods for Creating Peristaltic Motion in a Robotic Platform. In Proceedings of the IEEE International Conference on Robotics and Automation, Anchorage, AK, USA, 3–8 May 2010; pp. 1221–1227. [Google Scholar] [CrossRef]
- Rosendo, A.; Nakatsu, S.; Narioka, K.; Hosoda, K.; Pneupard, A. biomimetic musculoskeletal approach for a feline-inspired quadruped robot. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems, Tokyo, Japan, 3–7 November 2013; pp. 1452–1457. [Google Scholar] [CrossRef]
- Niiyama, R.; Nagakubo, A.; Kuniyoshi, Y.; Mowgli, A. Bipedal Jumping and Landing Robot with an Artificial Musculoskeletal System. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems, Rome, Italy, 10–14 April 2007; pp. 2546–2551. [Google Scholar] [CrossRef]
- Niiyama, R.; Nishikawa, S.; Kuniyoshi, Y. Athlete Robot with Applied Human Muscle Activation Patterns for Bipedal Running. In Proceedings of the IEEE-RAS International Conference on Humanoid Robots, Nashville, TN, USA, 6–8 December 2010; pp. 498–503. [Google Scholar] [CrossRef]
- Norioka, K.; Hosoda, K. Motor Development of an Pneumatic Musculoskeletal Infant Robot. In Proceedings of the IEEE International Conference on Robotics and Automation, Shanghai, China, 9–13 May 2011; pp. 963–968. [Google Scholar] [CrossRef]
- Steltz, E.; Mozeika, A.; Rodenberg, N.; Brown, E.; Jaeger, H.M. JSEL: Jamming Skin Enabled Locomotion. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems, St. Louis, MO, USA, 11–15 October 2009; pp. 5672–5677. [Google Scholar] [CrossRef]
- Shepherd, R.F.; Ilievski, F.; Choi, W.; Morin, S.A.; Stokes, A.A.; Mazzeo, A.D.; Chen, X.; Wang, M.; Whitesides, G.M. Multigait soft robot. Proc. Natl. Acad. Sci. USA 2011, 108, 20400–20403. [Google Scholar] [CrossRef]
- Li, S.; Daniel, M.V.; Rus, D.; Wood, R.J. Fluid-driven origami-inspired artificial muscles. Proc. Natl. Acad. Sci. USA 2017, 114, 13132–13137. [Google Scholar] [CrossRef]
- Yamagiwa, K.; Katoh, M.; Yoshida, M.; Ohkawa, A.; Ichijo, H. Temperature-Swing Column Adsorption of Nonionic Surfactant with Poly(vinylmethylether) Gel. J. Chem. Eng. Jpn. 2001, 34, 1317–1320. [Google Scholar] [CrossRef]
- Kim, Y.S.; Liu, M.; Ishida, Y.; Ebina, Y.; Osada, M.; Sasaki, T.; Hikima, T.; Takata, M.; Aida, T. Thermoresponsive actuation enabled by permittivity switching in an electrostatically anisotropic hydrogel. Nat. Mater. 2015, 14, 1002–1007. [Google Scholar] [CrossRef]
- Brannon-Peppas, L.; Peppas, N.A. Dynamic and equilibrium swelling behaviour of pH-sensitive hydrogels containing 2-hydroxyethyl methacrylate. Biomaterials 1990, 11, 635–644. [Google Scholar] [CrossRef]
- Fundueanu, G.; Constantin, M.; Bucatariu, S.; Ascenzi, P. pH/thermo-responsive poly(N-isopropylacrylamide-co-maleic acid) hydrogel with a sensor and an actuator for biomedical applications. Polymer 2017, 110, 177–186. [Google Scholar] [CrossRef]
- Osada, Y.; Okazaki, H.; Hori, H. A polymer gel with electrically driven mobility. Nature 1992, 355, 242–244. [Google Scholar] [CrossRef]
- Matsumoto, M. Water driven soft actuator. Appl. Syst. Innov. 2018, 1, 41. [Google Scholar] [CrossRef]
- Yu, Y.; Nakano, M.; Ikeda, T. Photomechanics: Directed bending of a polymer film by light. Nature 2003, 425, 145. [Google Scholar] [CrossRef] [PubMed]
- Otake, S.; Matsumoto, M. Investigation of driving principle of non-electrically driven robots using sound waves as a power source. In Proceedings of the 2021 IEEE International Conference on Mechatronics and Automation, Online, 8–11 August 2021; pp. 1261–1267. [Google Scholar]
- Helmholtz, H. On the Sensations of Tone as a Physiological Basis for the Theory of Music. Nature 1875, 12, 449–452. [Google Scholar]
- Fletcher, N.H.; Rossing, T.D. The Physics of Musical Instruments; Springer: Berlin/Heidelberg, Germany, 1998. [Google Scholar]
- Greene, C.A.; Argoiv, T.F.; Wilson, P.S. A Helmholtz resonator experiment for the Listen Up project. J. Acoust. Soc. Am. 2009, 5, 025001. [Google Scholar]
- Raichel, D.R. The Science and Applications of Acoustics; Springer: Berlin/Heidelberg, Germany, 2006; pp. 145–149. [Google Scholar]


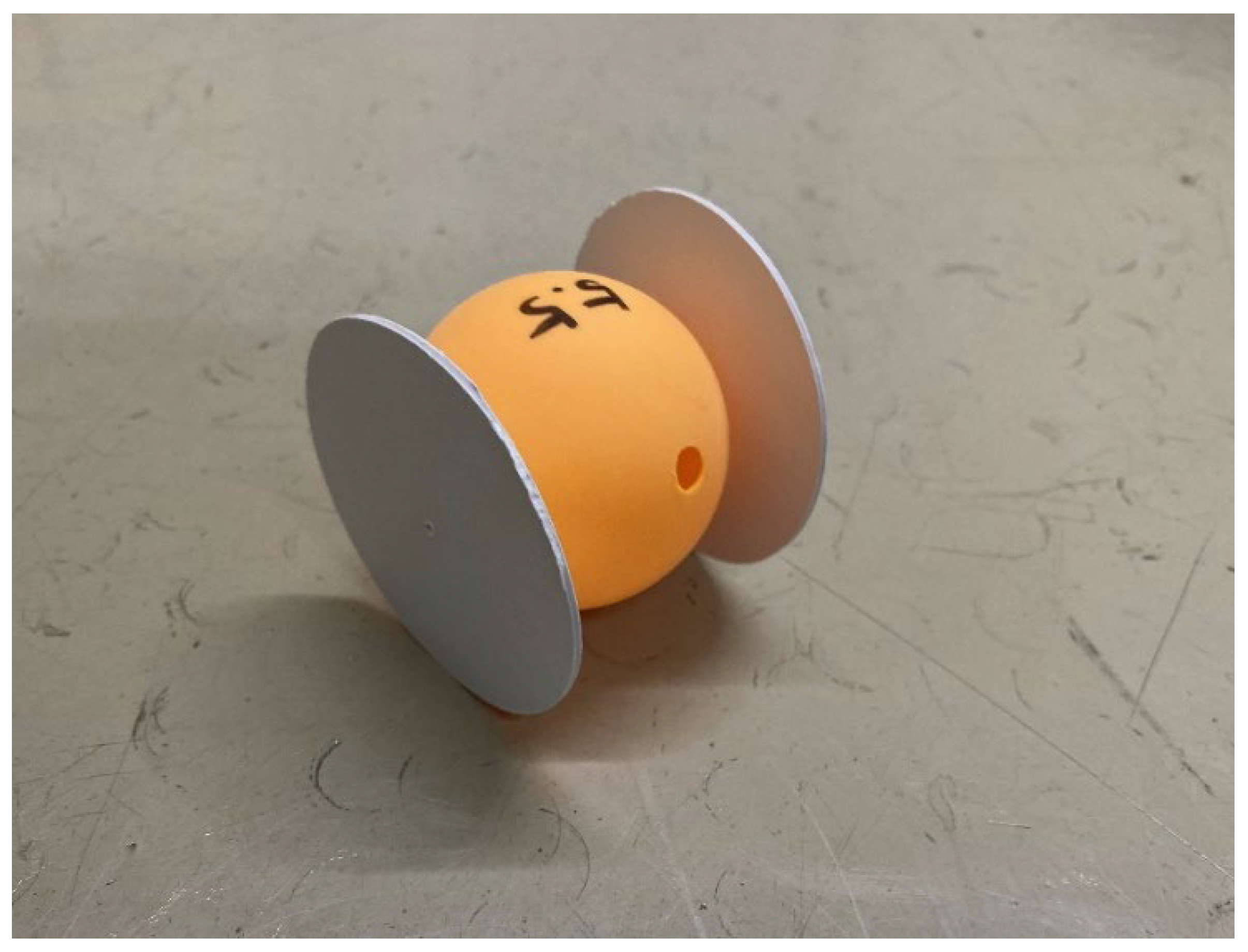



| Structure | Behavior |
|---|---|
| Normal table tennis ball | Reciprocation |
| Table tennis ball on wheels | Reciprocation |
| Table tennis ball with a fine-tipped cotton swab | Not moving |
| Table tennis ball with a round-tipped cotton swab | Not moving |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Niwano, T.; Matsumoto, M. Sound Driven Actuator Using Helmholtz Resonance. Actuators 2022, 11, 163. https://doi.org/10.3390/act11060163
Niwano T, Matsumoto M. Sound Driven Actuator Using Helmholtz Resonance. Actuators. 2022; 11(6):163. https://doi.org/10.3390/act11060163
Chicago/Turabian StyleNiwano, Takeru, and Mitsuharu Matsumoto. 2022. "Sound Driven Actuator Using Helmholtz Resonance" Actuators 11, no. 6: 163. https://doi.org/10.3390/act11060163
APA StyleNiwano, T., & Matsumoto, M. (2022). Sound Driven Actuator Using Helmholtz Resonance. Actuators, 11(6), 163. https://doi.org/10.3390/act11060163






