Dynamic Performance Analysis and Design of Vortex Array Grippers
Abstract
:1. Introduction
2. Numerical Method
3. Results and Discussions
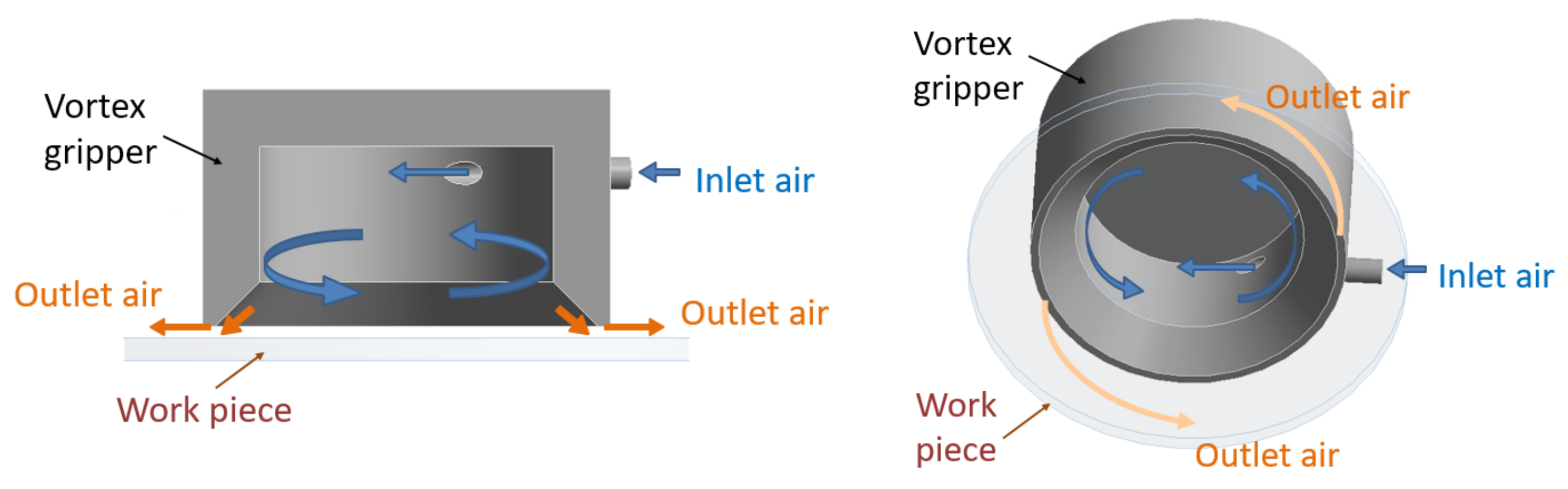
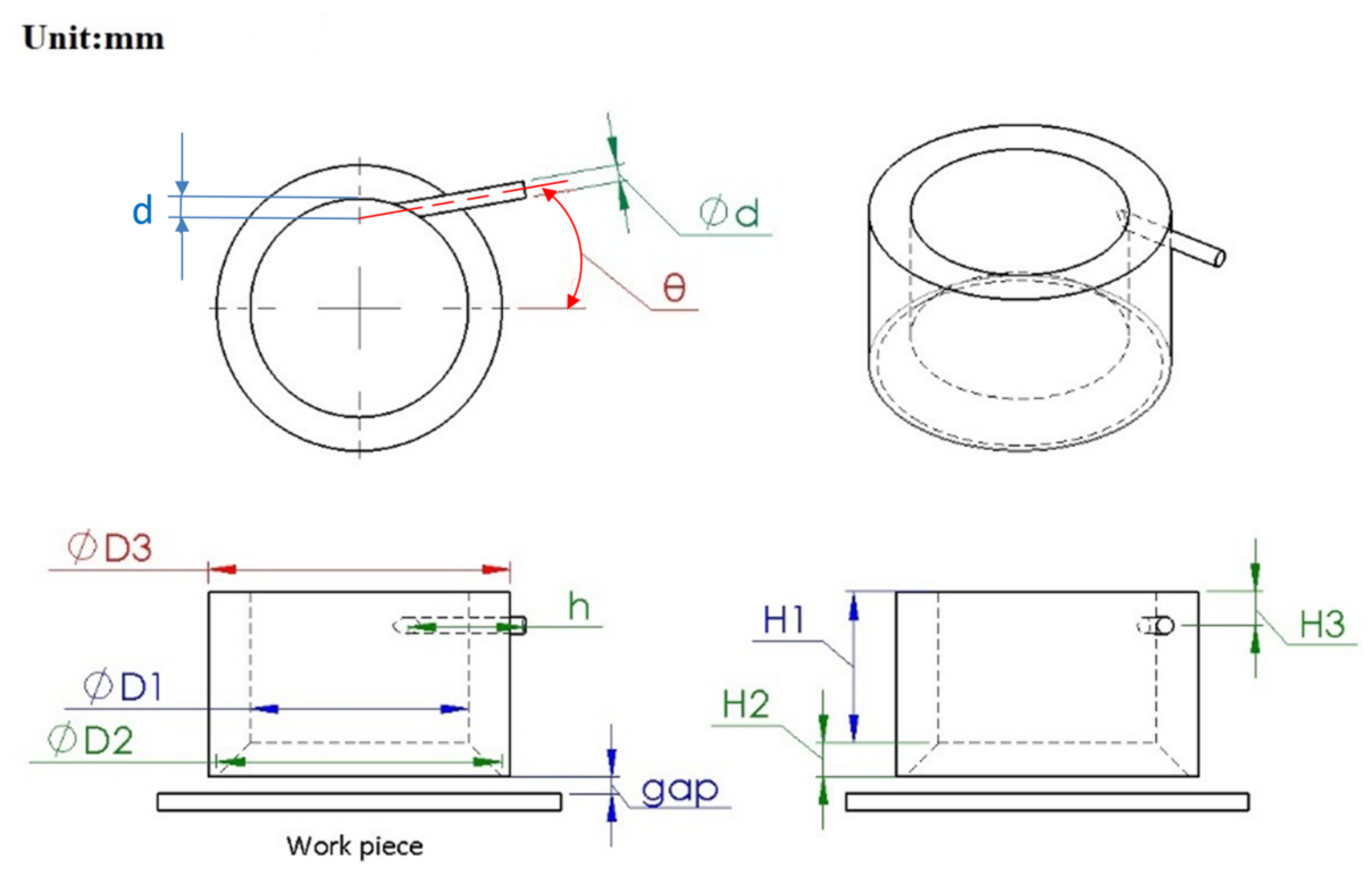
3.1. Geometric Parameters and Performance of a Single Vortex Gripper
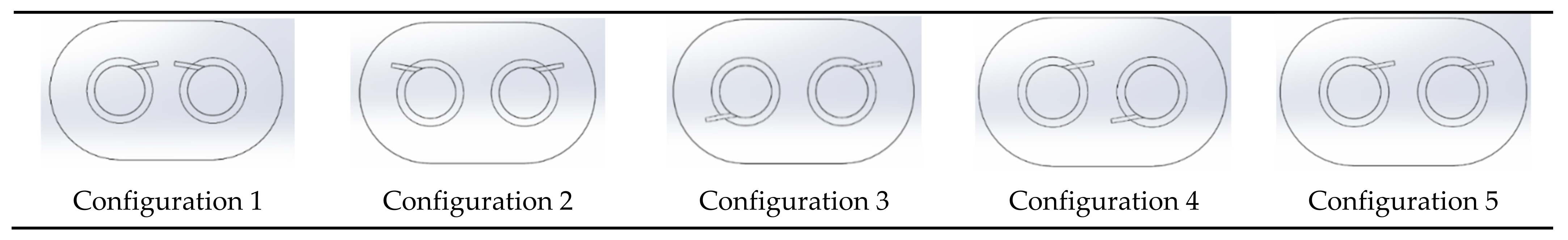
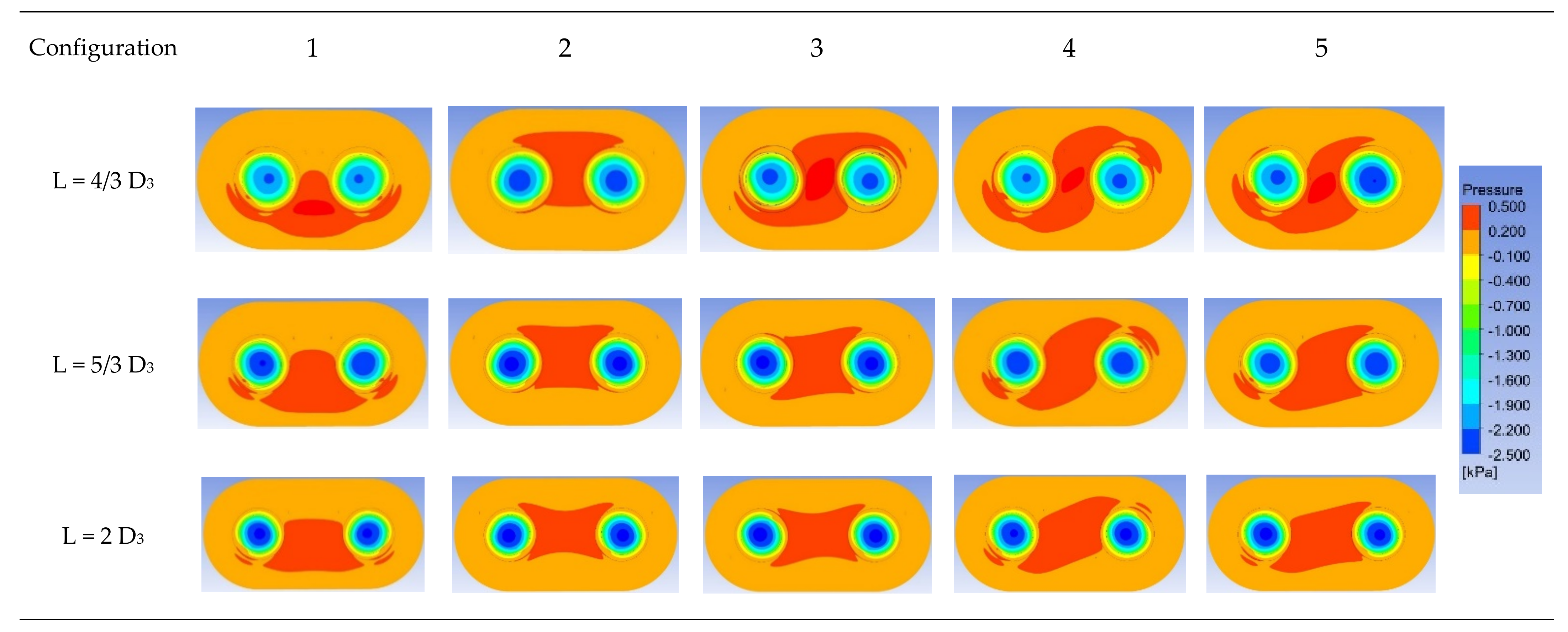
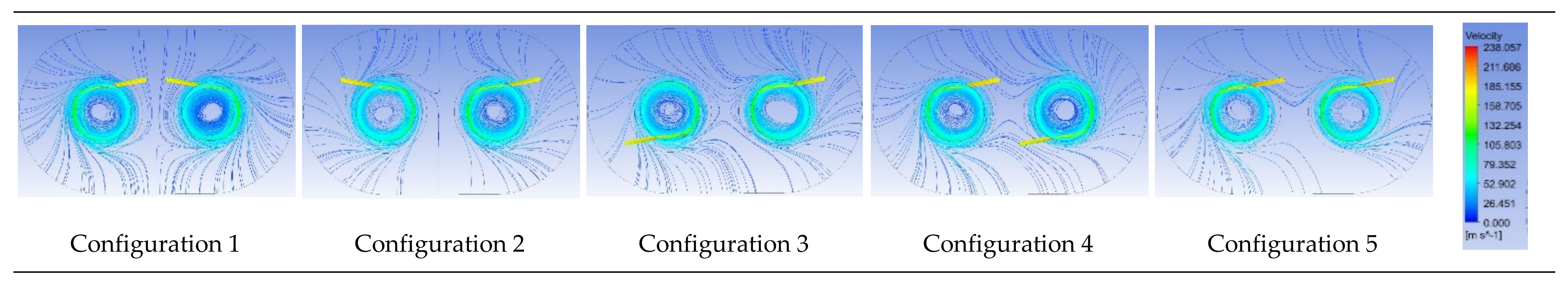
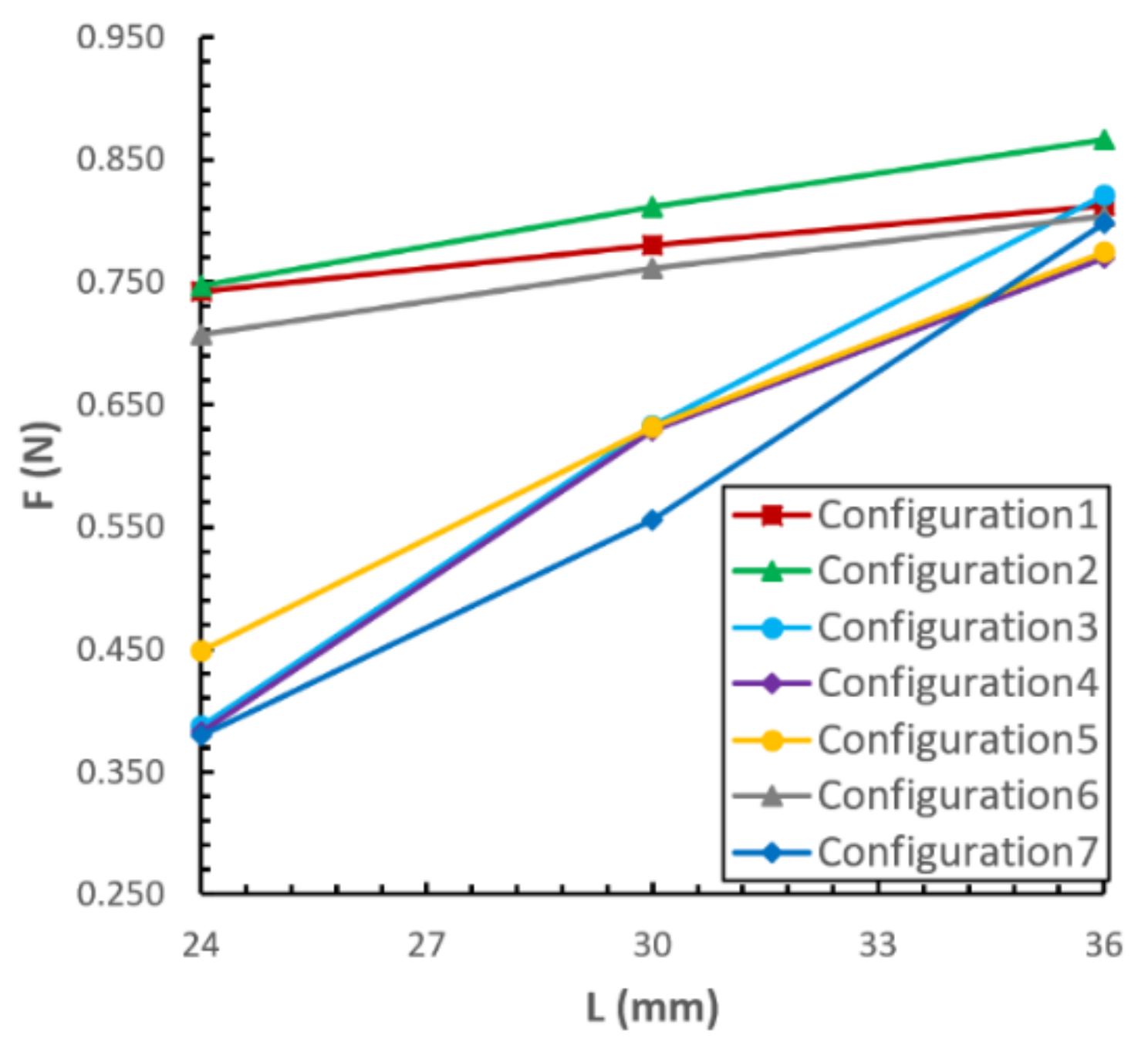
3.2. Dual-Vortex Grippers
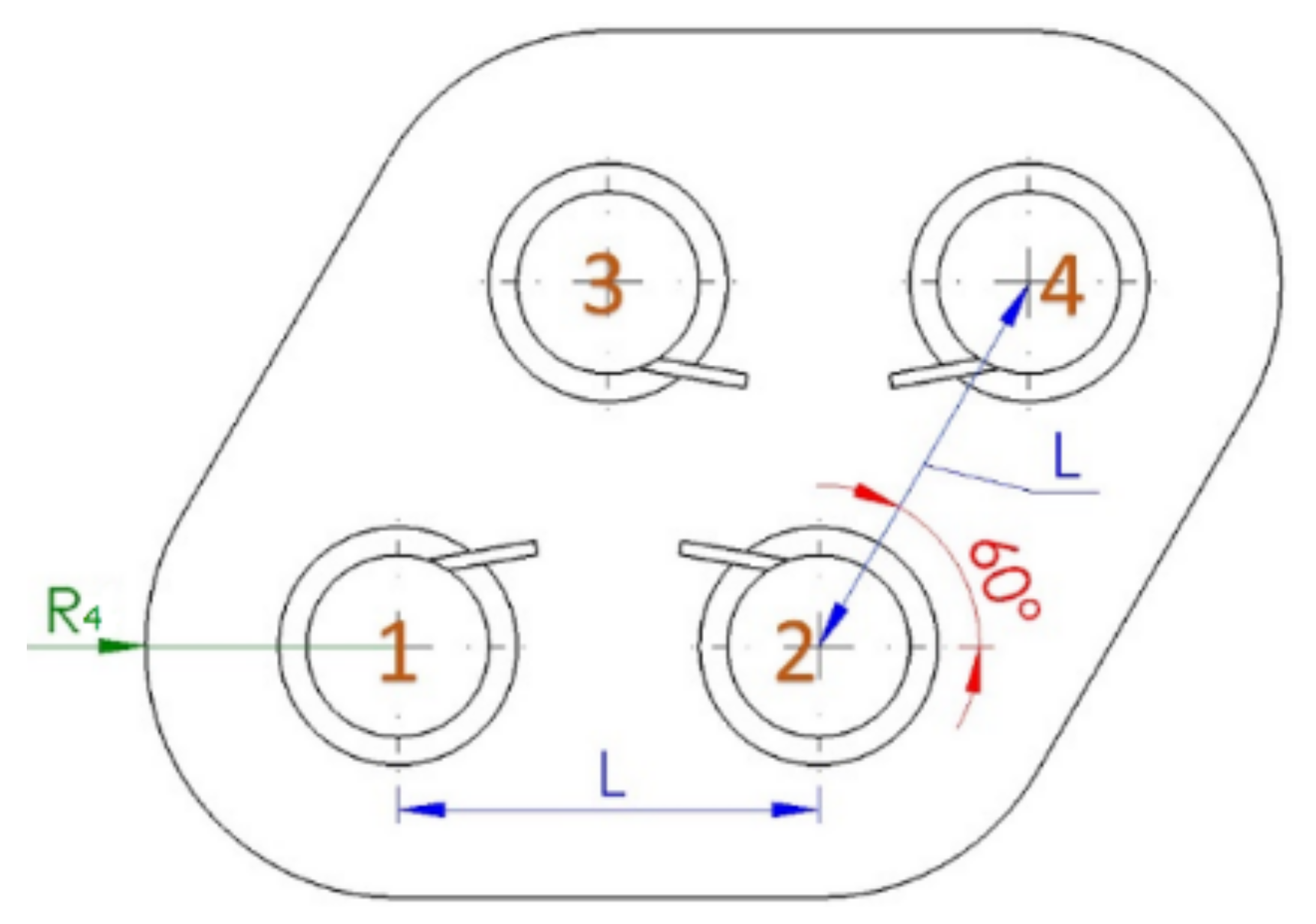
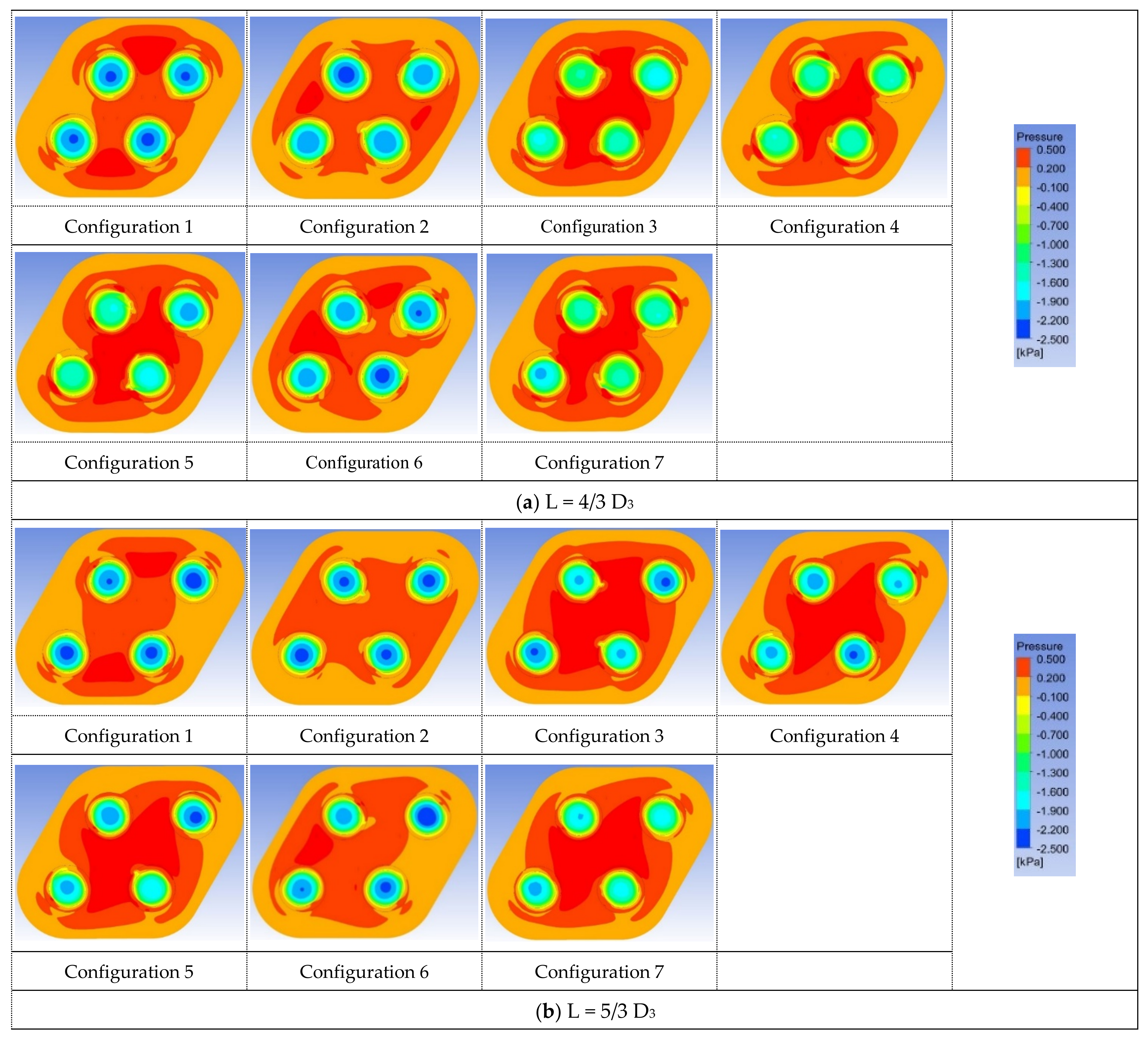
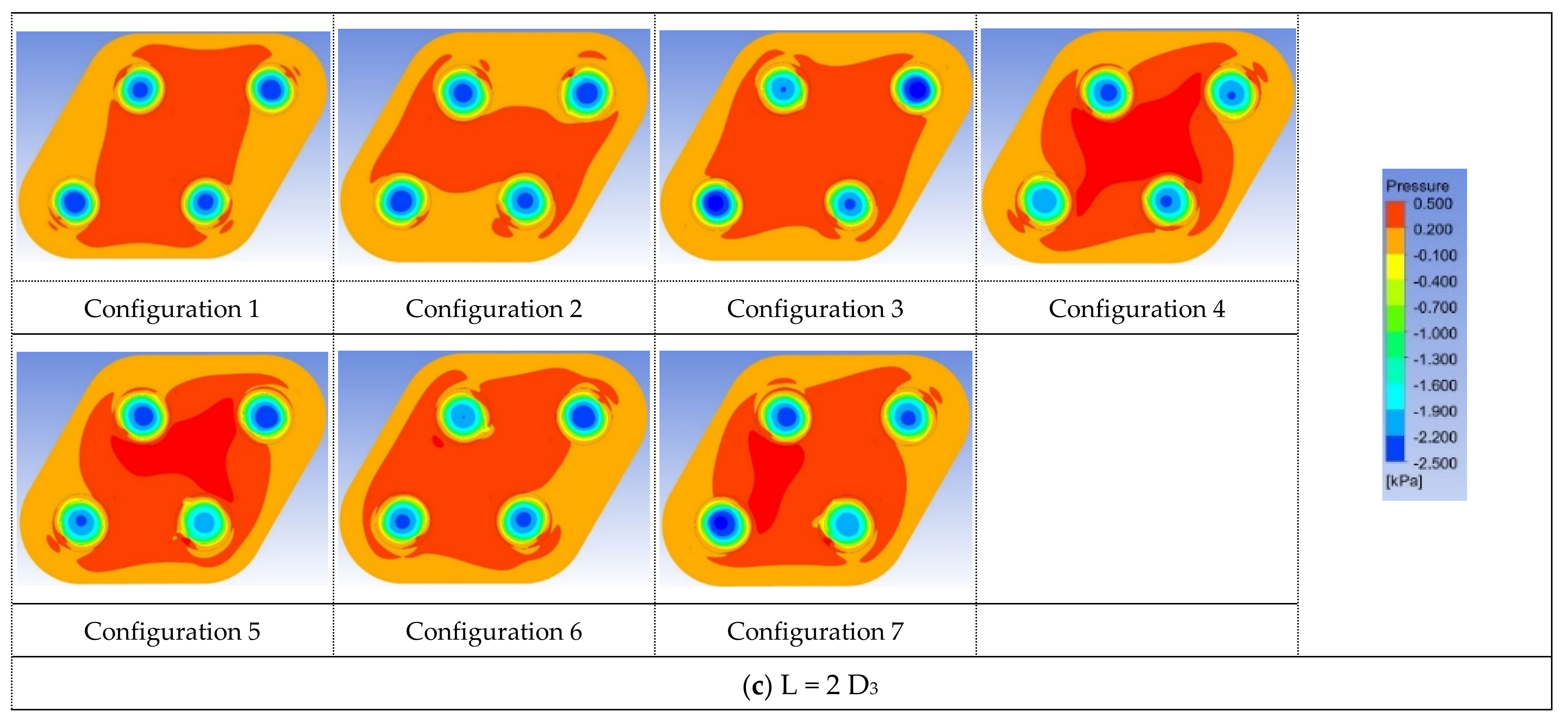
3.3. Quad-Vortex Array Grippers
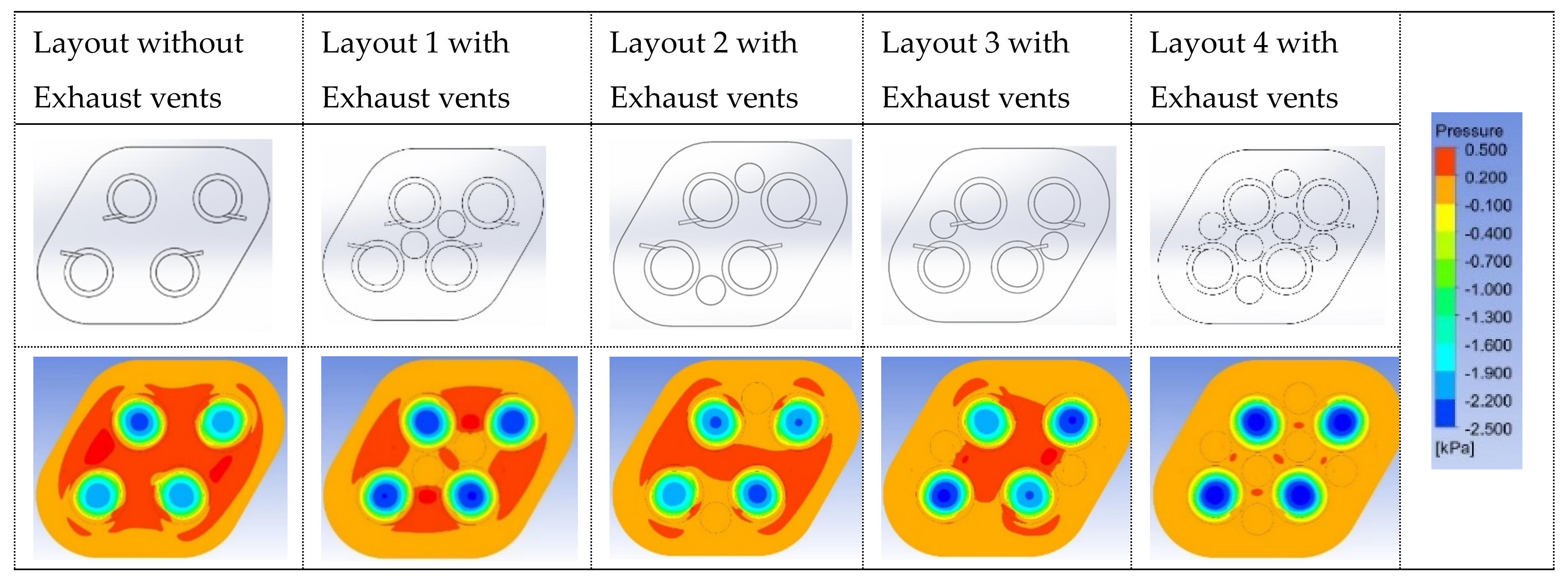
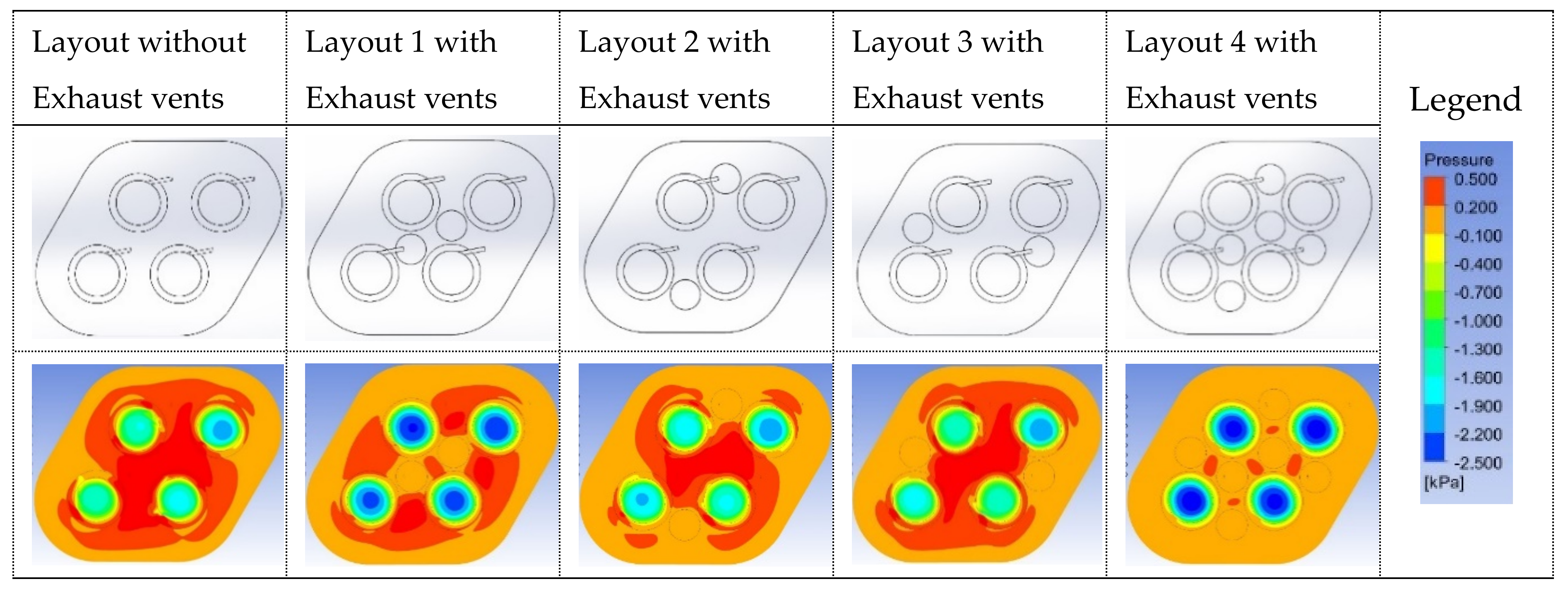
3.4. Quad-Vortex Array Grippers with Exhaust Vents
4. Conclusions
- (1)
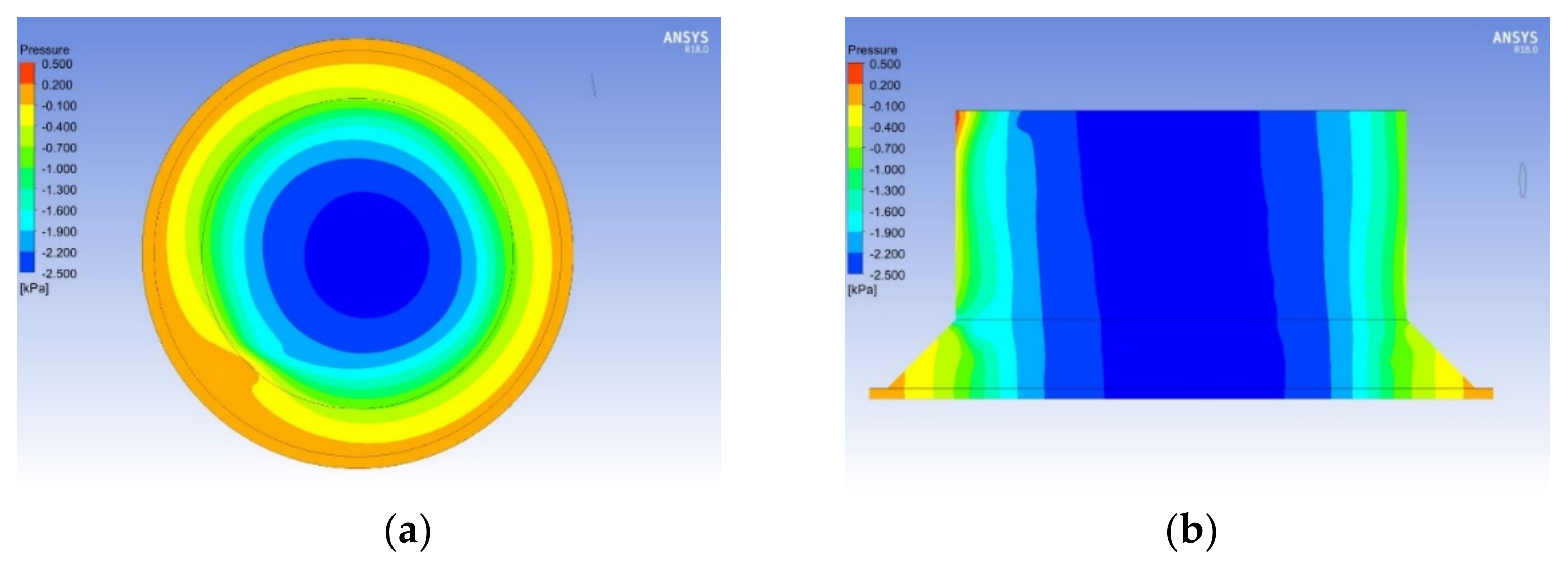
- The lower the height of the vortex chamber (H1), the better the suction force generated. However, a large numerical error is induced by a very small vortex chamber, and the pressure of the vortex chamber is highly sensitive to the gap between the gripper and workpiece when the vortex chamber is insufficient.
- (2)
- With regard to the vortex array grippers, the suction force of each gripper reaches a maximum when the vortex gripper distance is sufficiently large. However, the suction force per unit area may not be the largest, owing to the increase in the effective area.
- (3)
- For both dual-vortex grippers and quad-vortex array grippers, a mirror-like layout design with L = 24 mm (4/3 D3) generates a better suction force per unit area, and the suction forces of each gripper are similar to each other.
- (4)
- The suction force per unit area increases significantly when exhaust vents are applied to the quad-vortex array grippers. An appropriate number of exhaust vents can be introduced to obtain a better suction force per unit area.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Waltham, C.; Bendall, S.; Kotlicki, A. Bernoulli levitation. Am. J. Phys. 2003, 71, 176–179. [Google Scholar] [CrossRef] [Green Version]
- Brun, X.F.; Melkote, S.N. Modeling and prediction of the flow, pressure, and holding force generated by a Bernoulli handling device. J. Manuf. Sci. Eng. 2009, 131, 31018. [Google Scholar] [CrossRef]
- Davis, S.; Gray, J.O.; Caldwell, D.G. An end effector based on the Bernoulli principle for handling sliced fruit and vegetables. Robot. Cim. Int. Manuf. 2008, 24, 249–257. [Google Scholar] [CrossRef]
- Kagawa, T.; Li, X. Invited lecture: Vortex levitation. In Proceedings of the Fluid Control, Measurements, and Visualization Conference, Moscow, Russia, 18–19 August 2009. [Google Scholar]
- Li, X.; Kawashima, K.; Kagawa, T. Analysis of vortex levitation. Exp. Therm. Fluid Sci. 2008, 32, 1448–1454. [Google Scholar] [CrossRef]
- Ma, W.; Xu, L.; Yu, H. Study on Flow Field Characteristics of Non-Contact Vortex Negative Pressure Carrier. In Proceedings of the International Conference on Measuring Technology and Mechatronics Automation, Changsha, China, 13–14 March 2010; pp. 587–591. Available online: https://ieeexplore.ieee.org/document/5459112 (accessed on 6 May 2010).
- Feng, T.H.; Chen, C.K.; Tsai, K.T.; Liu, S.H. A Study of Geometry Parameters of Non-Contact Vortex Grippers. In Proceedings of the Annual Conference on Engineering and Information Technology Conference, Kyoto, Japan, 27–29 March 2018. [Google Scholar]
- Iio, S.; Umebachi, M.; Li, X.; Kagawa, T.; Ikeda, T. Performance of a non-contact handling device using swirling flow with various gap height. J. Visual. Jpn. 2010, 13, 319–326. [Google Scholar] [CrossRef] [Green Version]
- Li, X.; Iio, S.; Kawashima, K.; Kagawa, T. Computational fluid dynamics study of a noncontact handling device using air-swirling flow. J. Eng. Mech. 2011, 137, 400–409. [Google Scholar] [CrossRef]
- Li, X.; Kagawa, T. Development of a new noncontact gripper using swirl vanes. Robot. Cim. Int. Manuf. 2013, 29, 63–70. [Google Scholar] [CrossRef]






| θ (°) | D3 (mm) | F (N) | |
|---|---|---|---|
| H1 = 8.5 mm | H1 = 6 mm | ||
| 0 | 18 | 0.187 | 0.247 |
| 10 | 0.190 | 0.257 | |
| 20 | 0.183 | 0.245 | |
| 30 | 0.178 | 0.231 | |
| 40 | 0.177 | 0.247 | |
| Gap (mm) | H1 (mm) | D1 (mm) | F(N) | ||
|---|---|---|---|---|---|
| Q = 1 × 10−4 kg/s | Q = 2 × 10−4 kg/s | Q = 3 × 10−4 kg/s | |||
| 0.3 | 3 | 10 | 0.051 | 0.286 | 0.619 |
| 13 | 0.038 | 0.199 | 0.438 | ||
| 16 | 0.009 | 0.203 | N/A | ||
| 6 | 10 | 0.033 | 0.226 | 0.554 | |
| 13 | 0.035 | 0.257 | 0.660 | ||
| 16 | 0.023 | 0.192 | 0.473 | ||
| 9 | 10 | 0.026 | 0.152 | 0.369 | |
| 13 | 0.022 | 0.179 | 0.365 | ||
| 16 | 0.028 | 0.166 | 0.476 | ||
| 12 | 10 | 0.013 | 0.102 | 0.325 | |
| 13 | 0.011 | 0.114 | 0.370 | ||
| 16 | 0.019 | 0.130 | 0.355 | ||
| Layout | Without Vents | Layout 1 with Vents | Layout 2 with Vents | Layout 3 with Vents | Layout 4 with Vents |
|---|---|---|---|---|---|
| F/A (10−6 N/mm2) | 355.624 | 433.104 | 381.421 | 405.842 | 483.752 |
| Performance ratio | 100% | 121.79% | 107.25% | 114.12% | 136.03% |
| Layout | Without Vents | Layout 1 with Vents | Layout 2 with Vents | Layout 3 with Vents | Layout 4 with Vents |
|---|---|---|---|---|---|
| F/A (10−6 N/mm2) | 213.585 | 403.914 | 275.405 | 232.736 | 474.547 |
| Performance ratio | 100% | 189.11% | 128.94% | 108.97% | 222.18% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, H.-S.; Liu, C.-Y.; Chen, C.-L. Dynamic Performance Analysis and Design of Vortex Array Grippers. Actuators 2022, 11, 137. https://doi.org/10.3390/act11050137
Peng H-S, Liu C-Y, Chen C-L. Dynamic Performance Analysis and Design of Vortex Array Grippers. Actuators. 2022; 11(5):137. https://doi.org/10.3390/act11050137
Chicago/Turabian StylePeng, Huan-Sen, Chen-Yen Liu, and Chieh-Li Chen. 2022. "Dynamic Performance Analysis and Design of Vortex Array Grippers" Actuators 11, no. 5: 137. https://doi.org/10.3390/act11050137
APA StylePeng, H.-S., Liu, C.-Y., & Chen, C.-L. (2022). Dynamic Performance Analysis and Design of Vortex Array Grippers. Actuators, 11(5), 137. https://doi.org/10.3390/act11050137






