Design of Longitudinal-Bending Coupled Horn of a Giant Magnetostriction Transducer
Abstract
:1. Introduction
2. Materials and Methods
2.1. Design of Longitudinal Vibration Horn
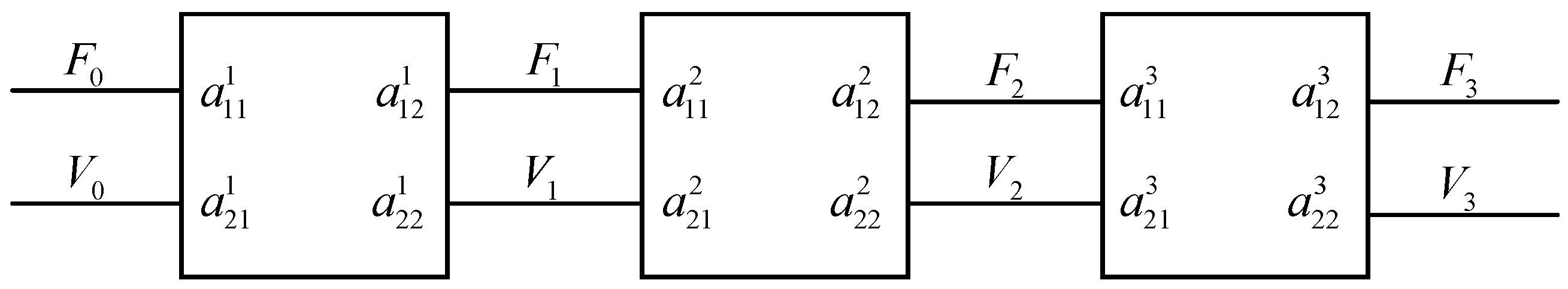
2.1.1. Design Theory Based on Equivalent Four-Terminal Network
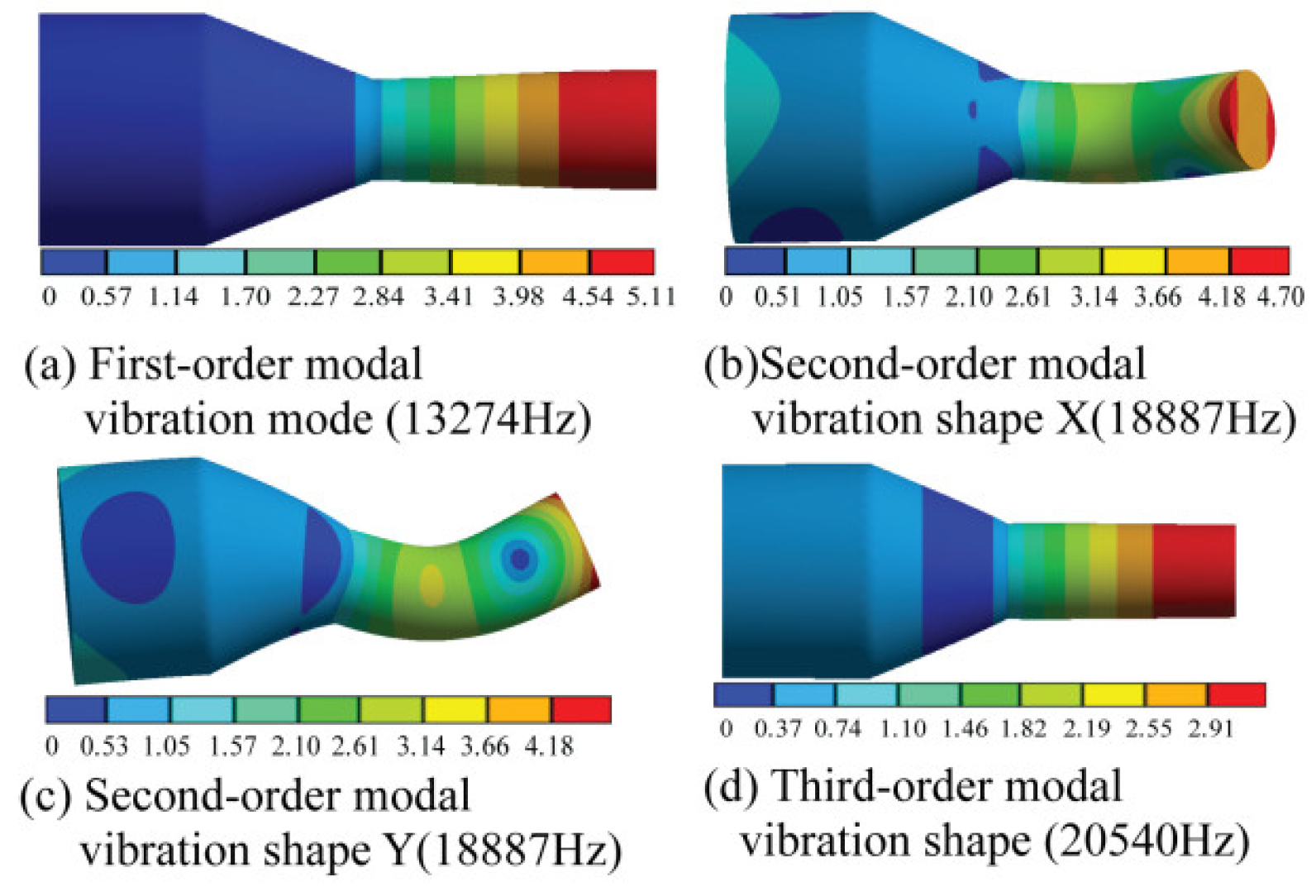
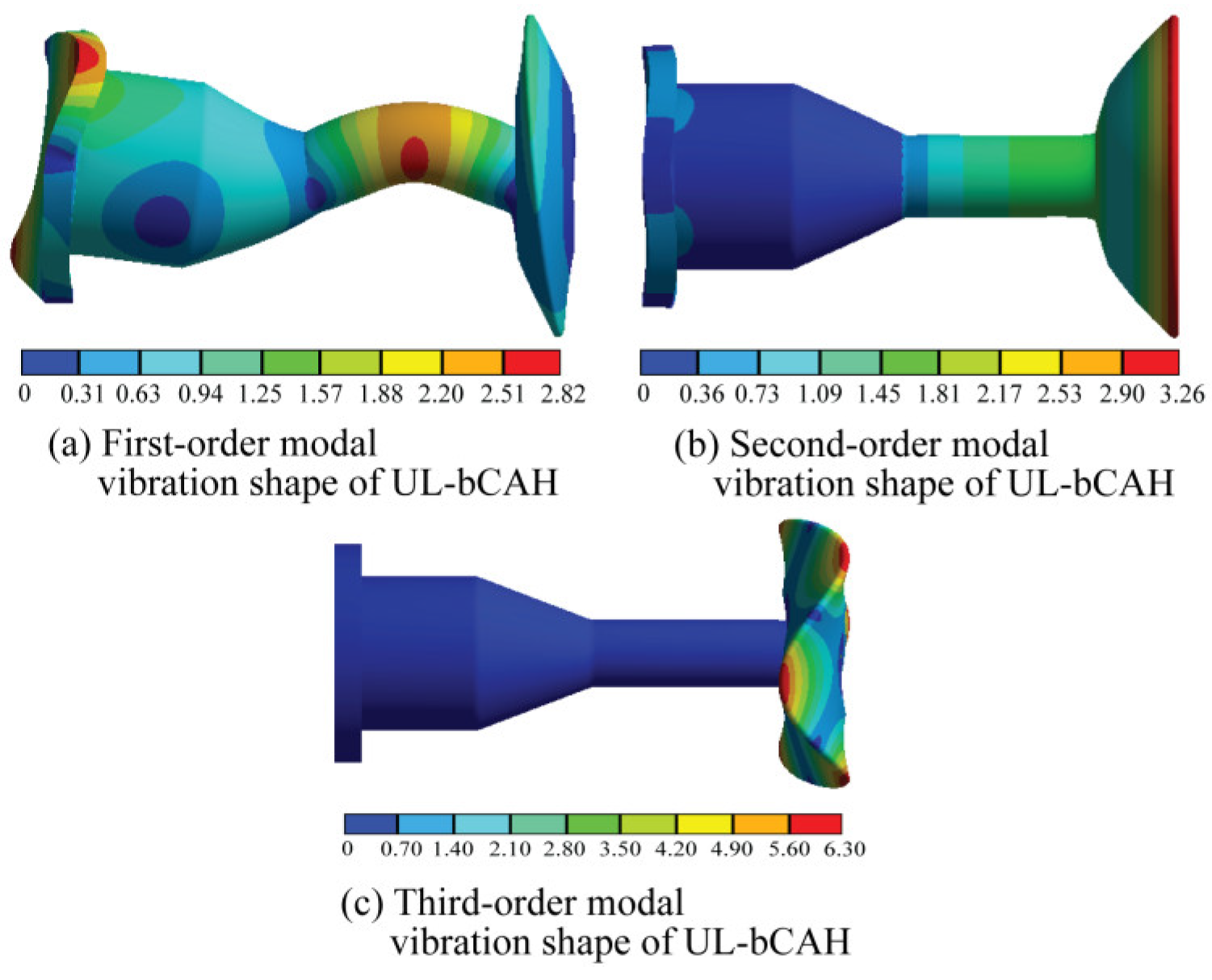
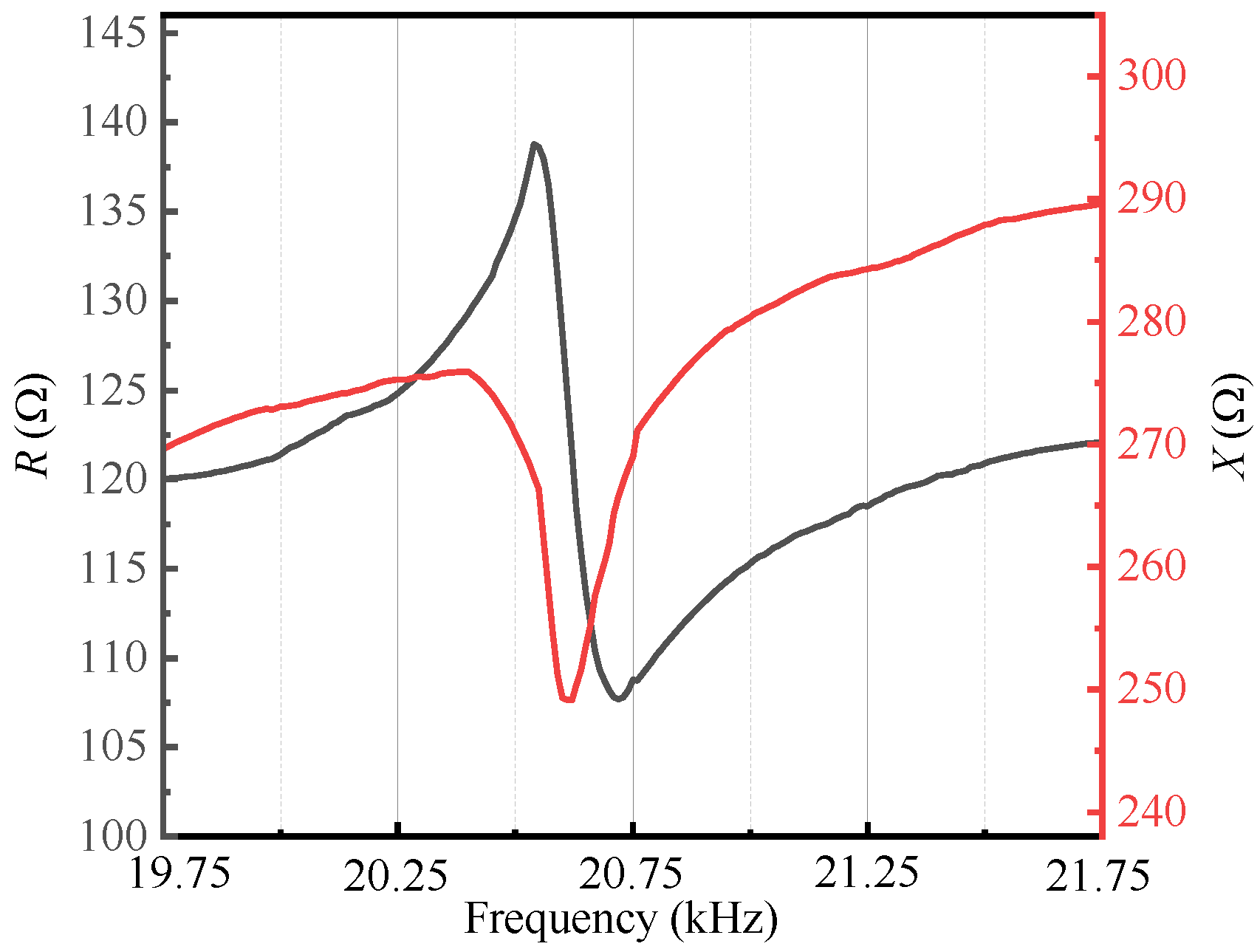
2.1.2. Modal Analysis
2.2. Design of Bending Vibration Disc
2.2.1. Design Theory
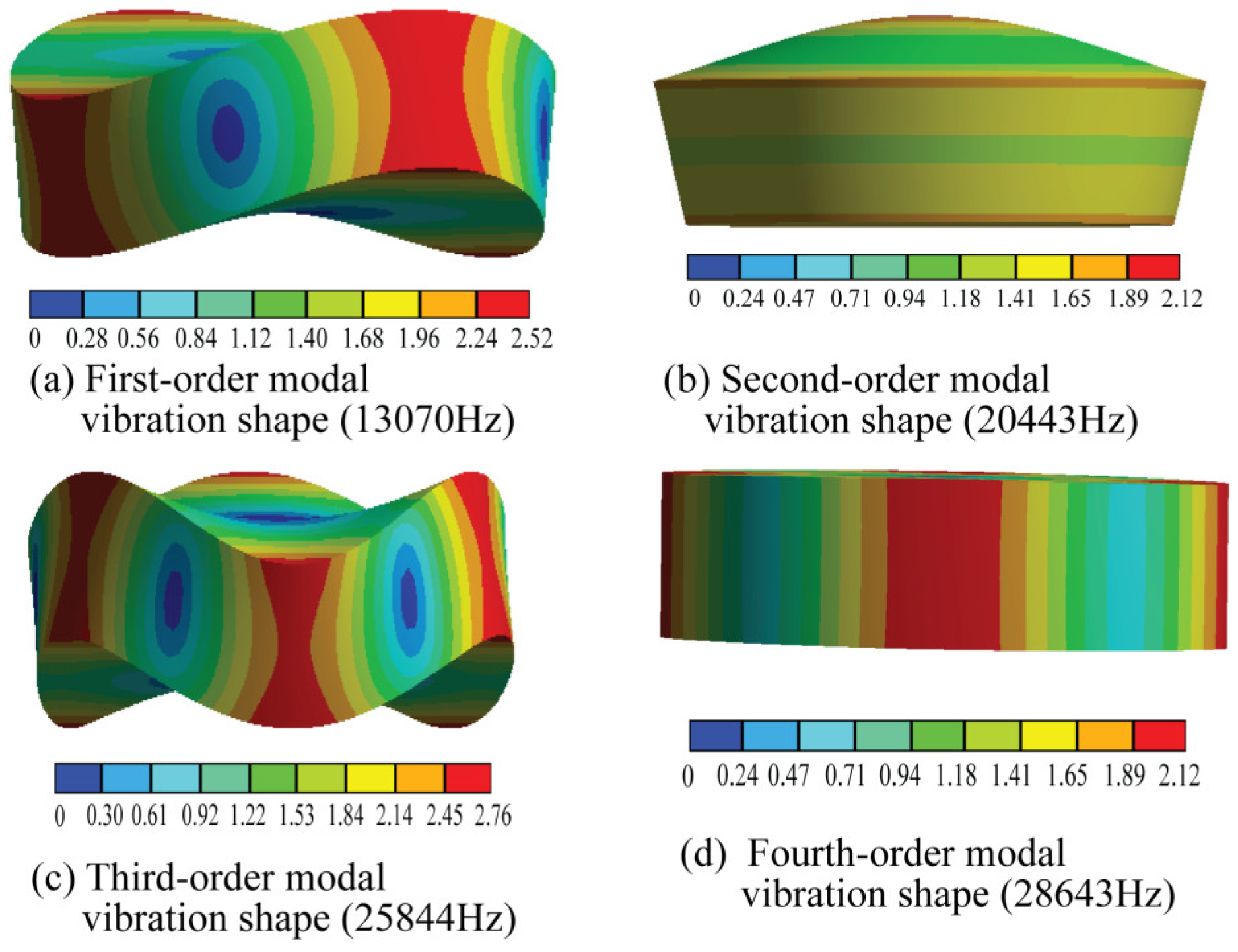
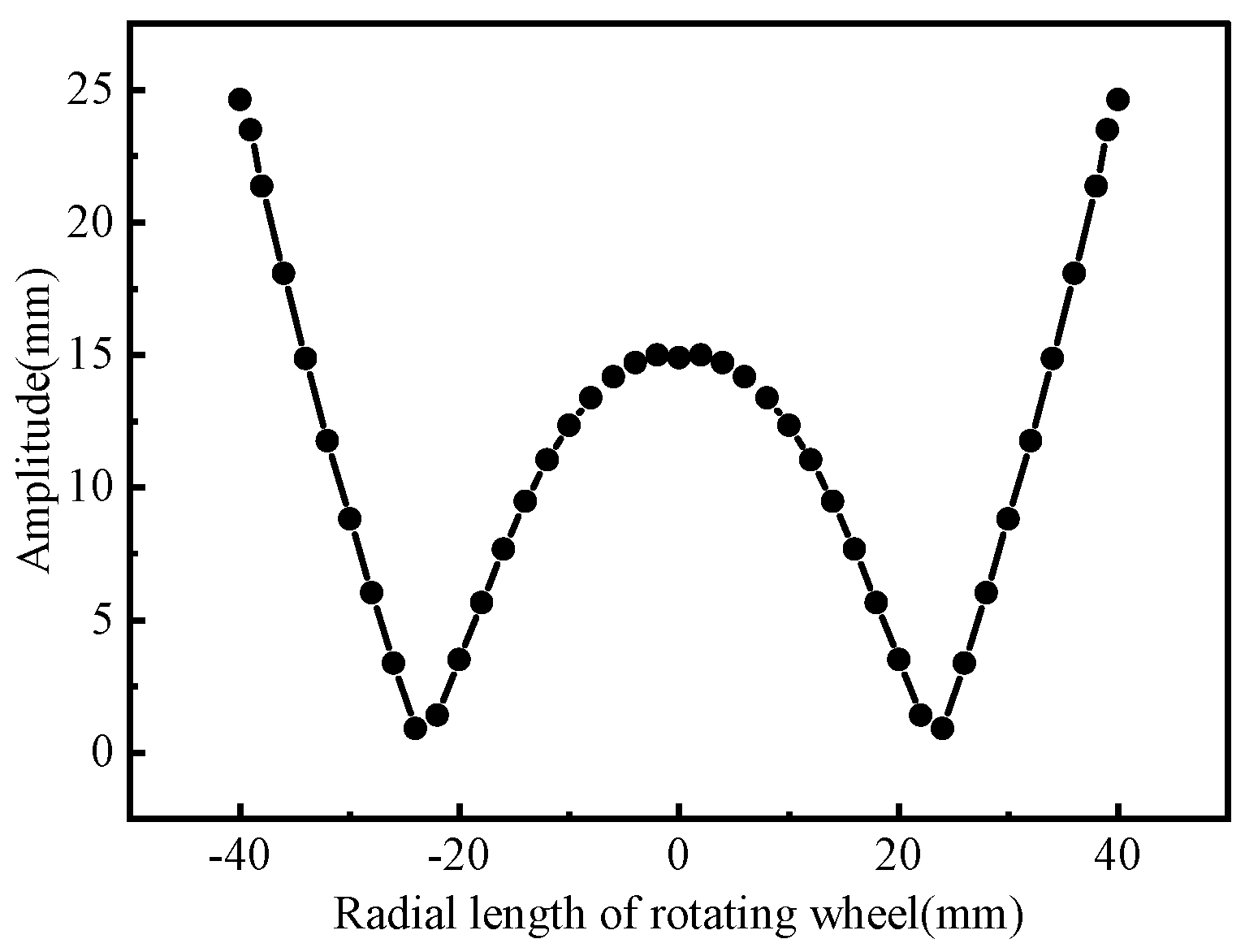
2.2.2. Analysis of Rotating Wheel Model
2.3. Dynamic Simulation of the L-BCH
2.3.1. The Influence of the Rotating Wheel’s Thickness on the Dynamic Characteristics
2.3.2. The Influence of the Spinning Wheel’s Large Diameter on the Dynamic Characteristics
2.3.3. Influence of the Spinning Wheel’s Small Diameter on Dynamic Characteristics
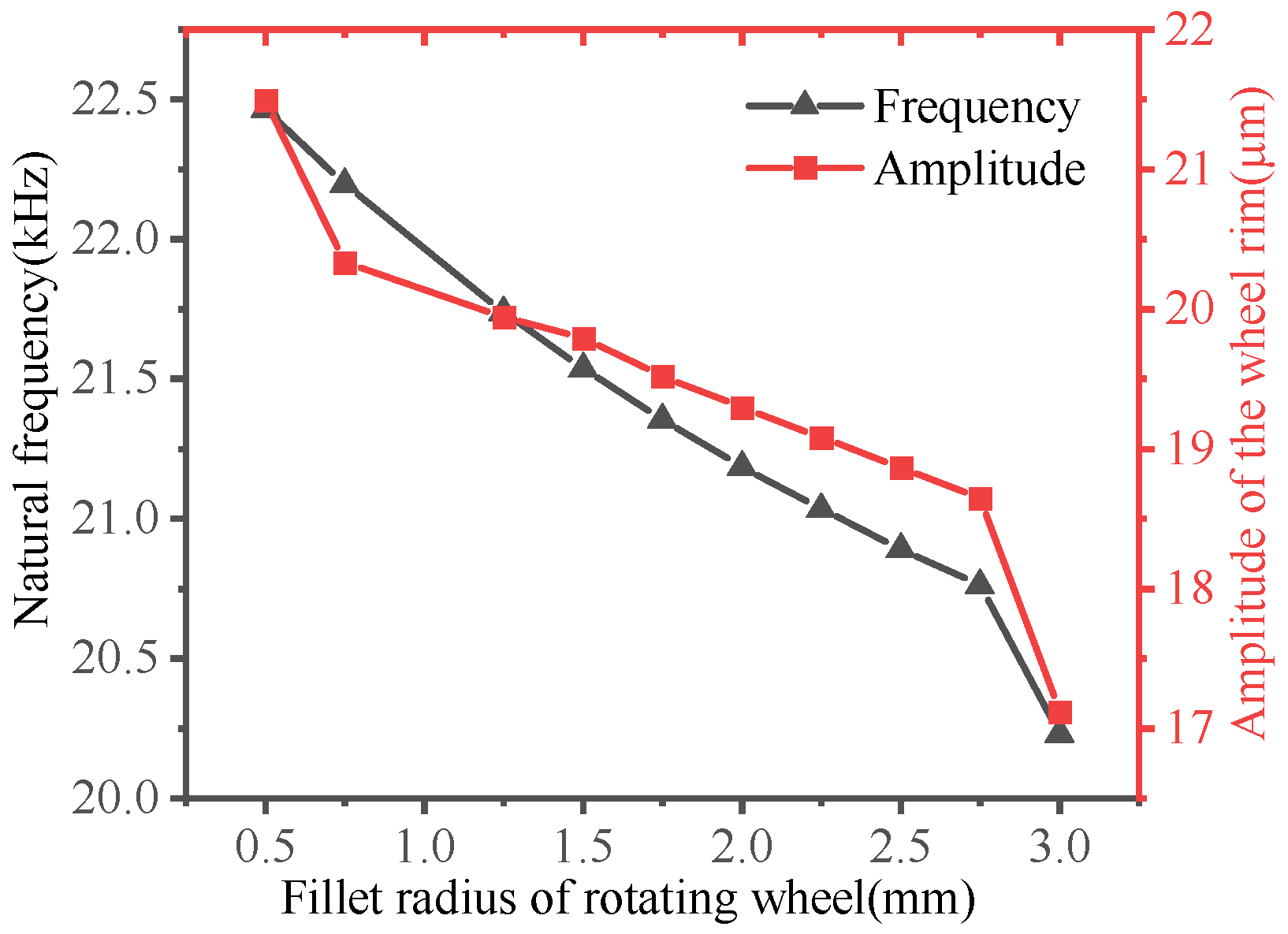
2.3.4. Influence of the Spinning Wheel’s Fillet Radius on Dynamic Characteristics
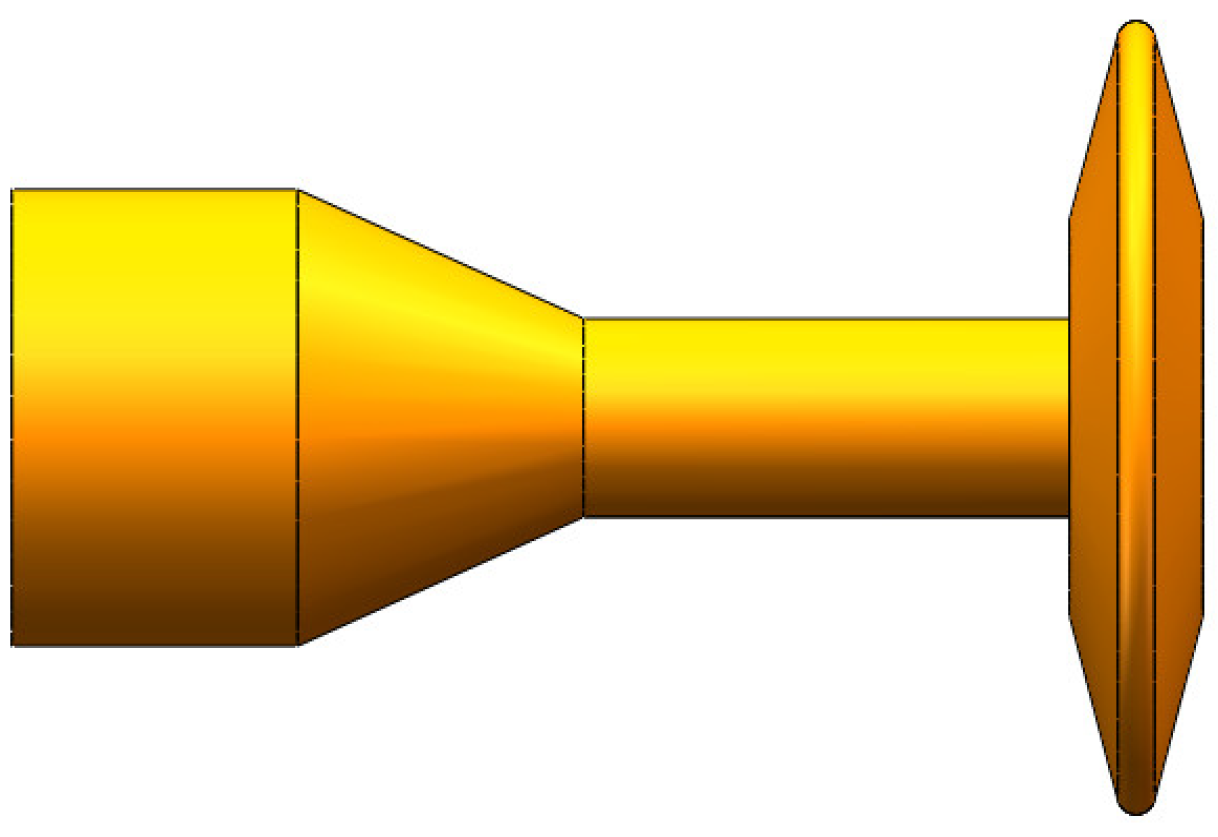
2.4. Determination of Structural Parameters of the L-BCH
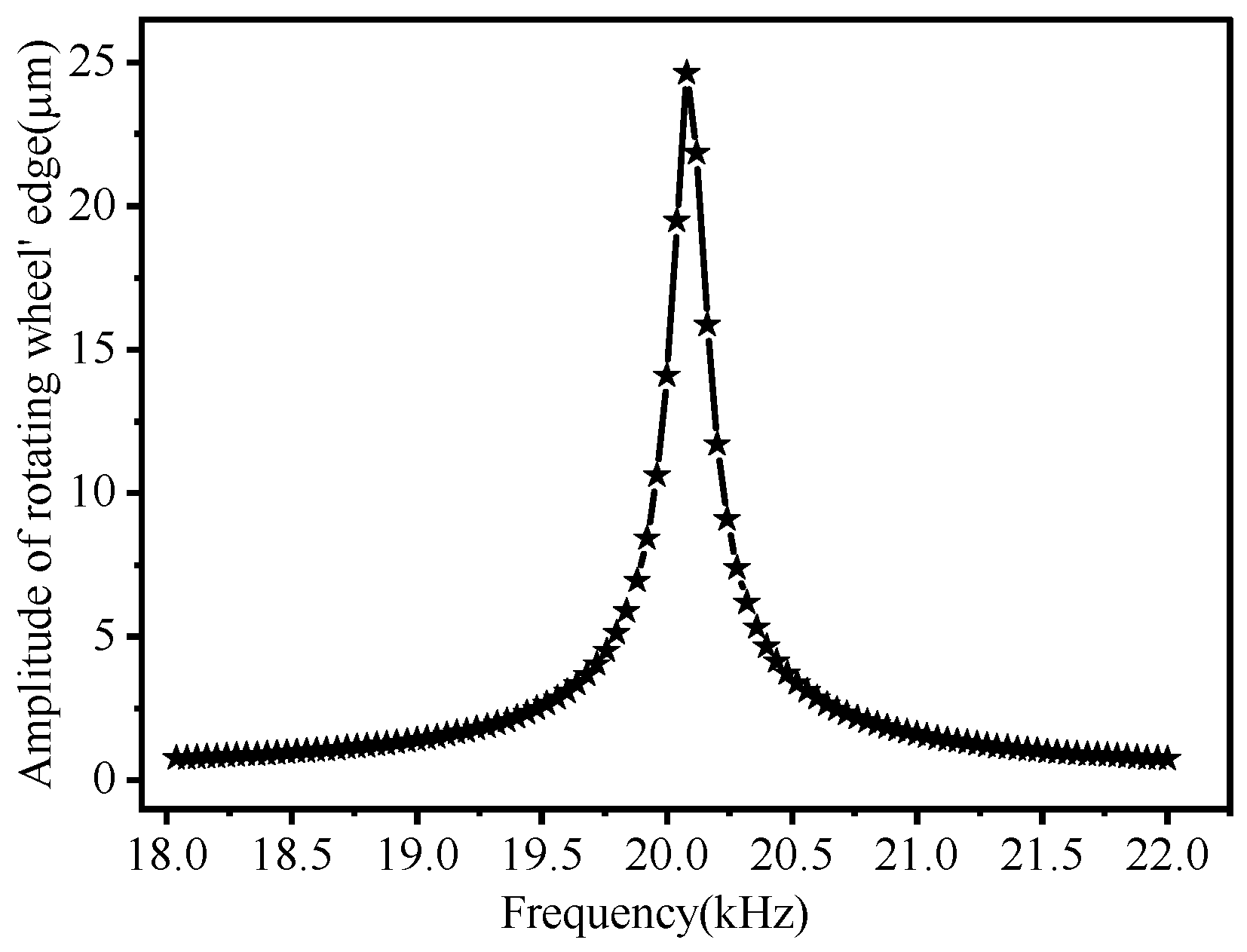
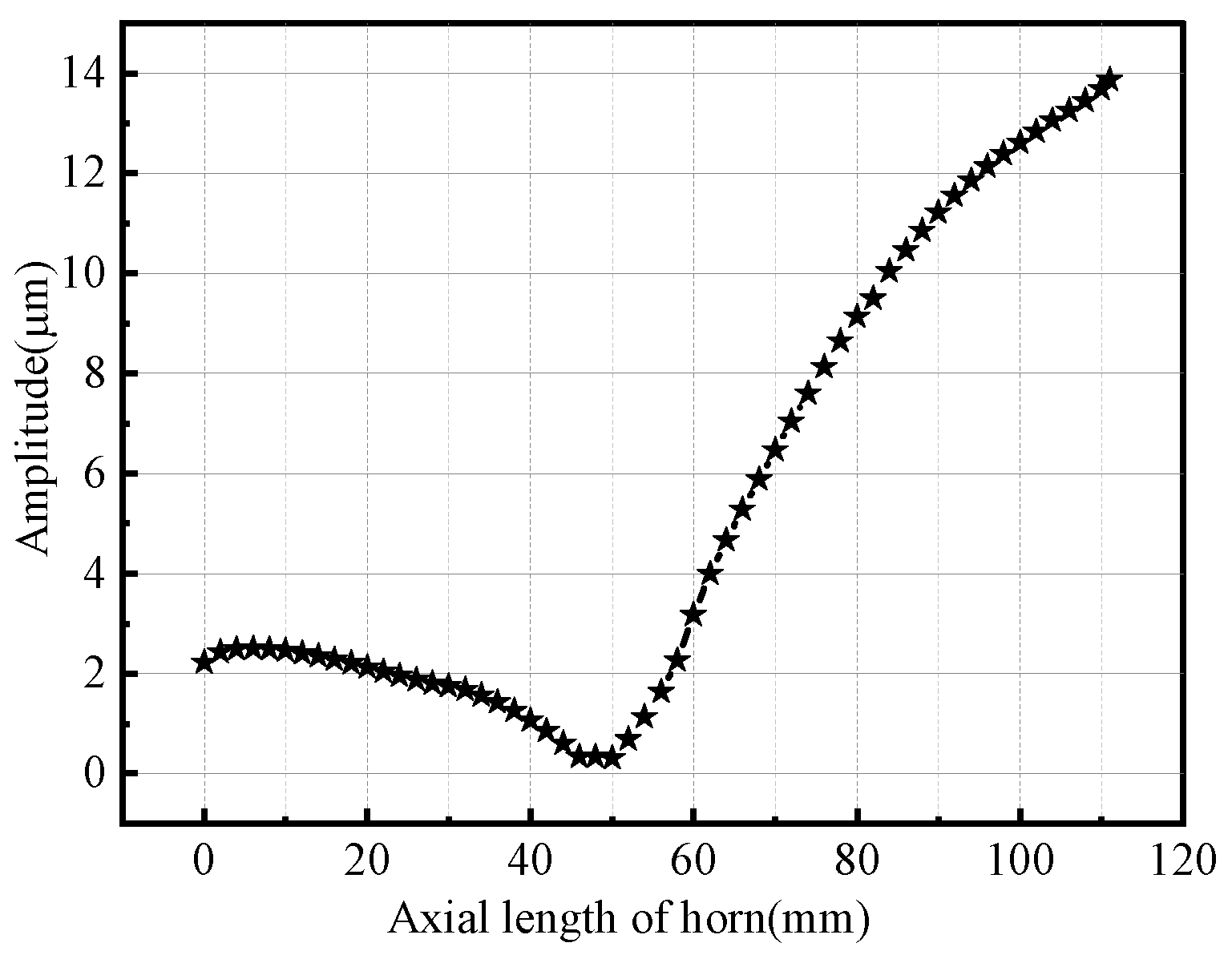
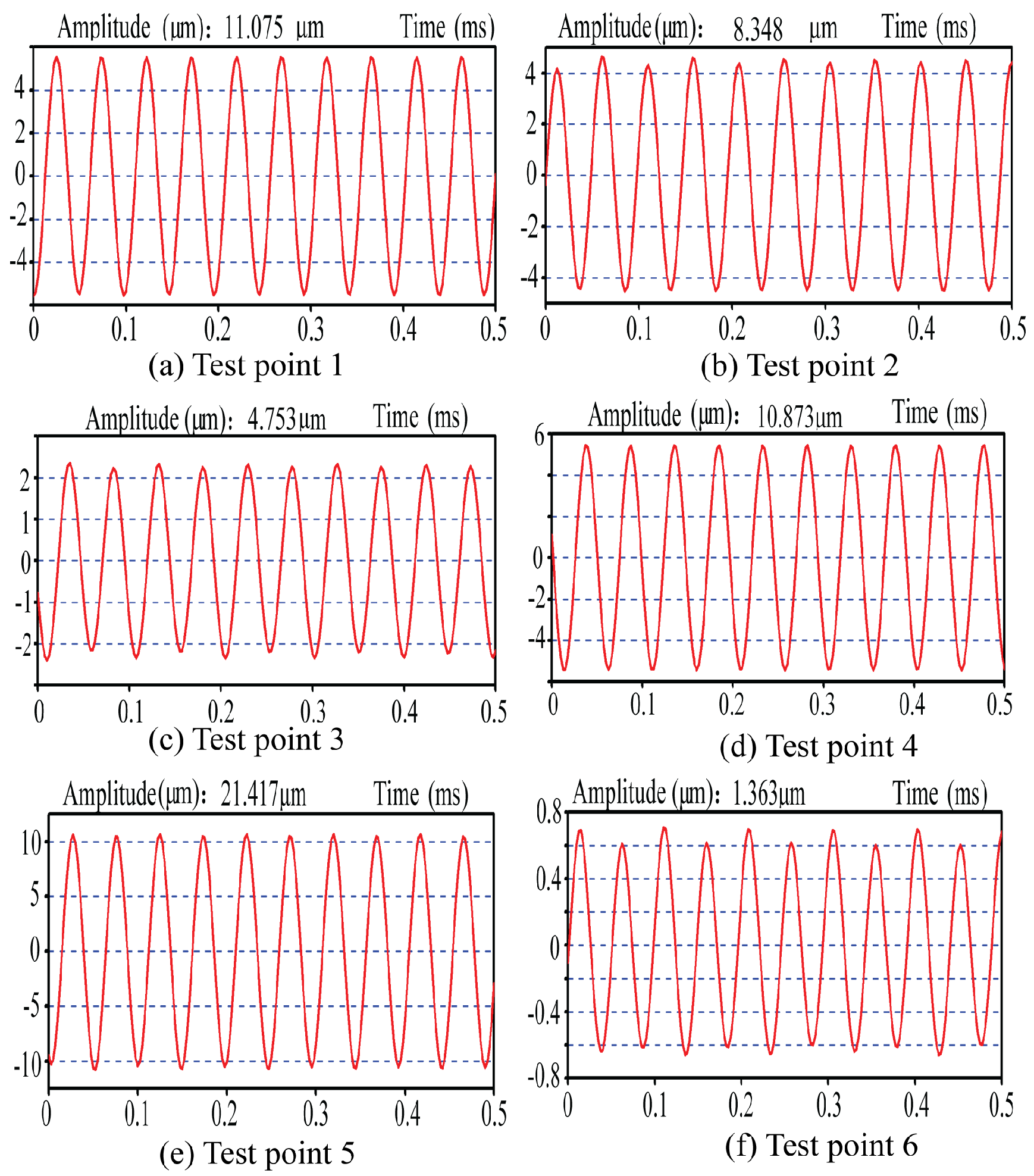
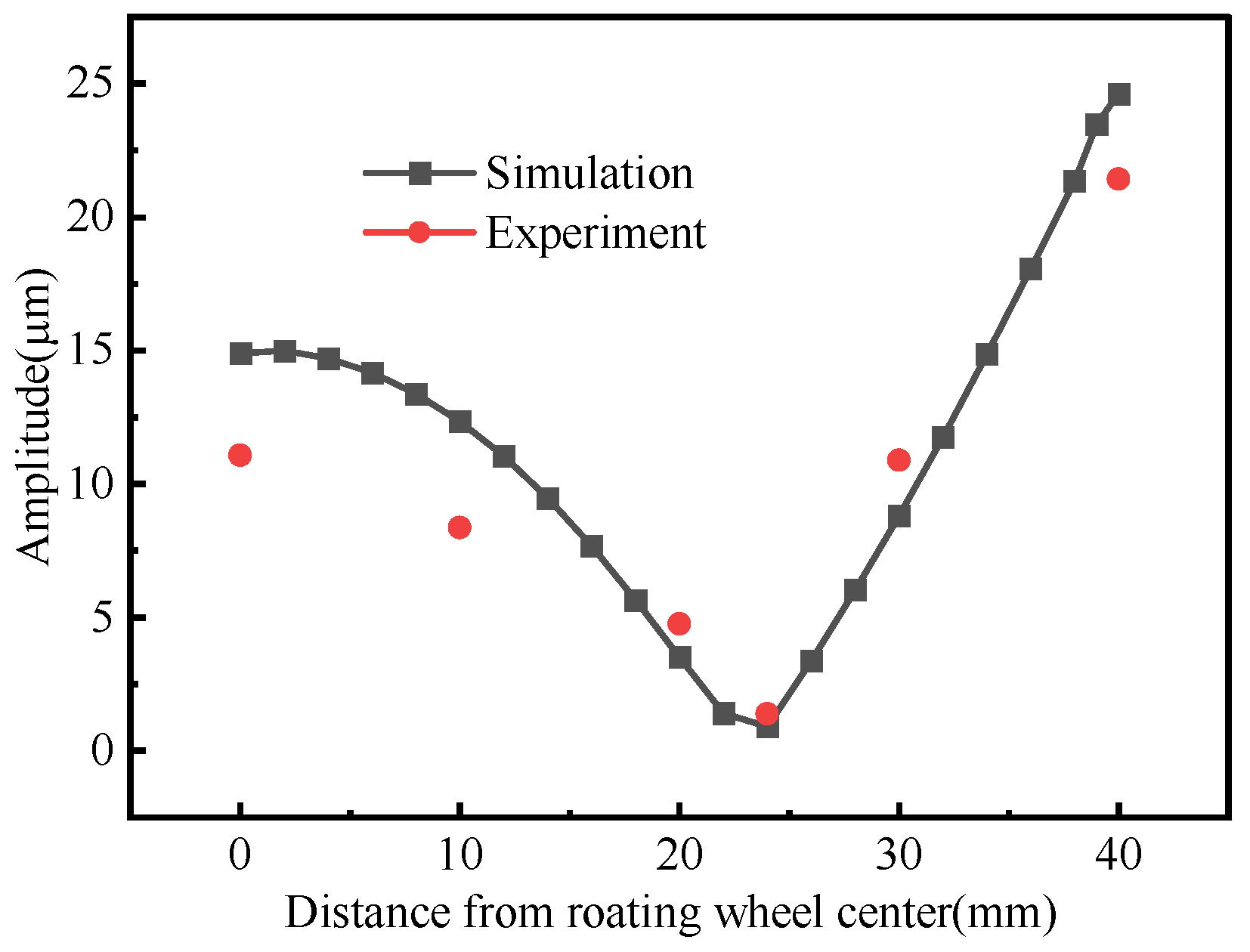
3. Result and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, H.; Ning, F.; Li, Y.; Hu, Y.; Cong, W. Scratching-induced Surface Characteristics and Material Removal Mechanisms in Rotary Ultrasonic Surface Machining of CFRP. Ultrasonics 2019, 97, 19–28. [Google Scholar] [CrossRef] [PubMed]
- Gondo, S.; Arai, H. Effect and control of path parameters on thickness distribution of cylindrical cups formed via multi-pass conventional spinning. J. Intell. Manuf. 2022, 33, 617–635. [Google Scholar] [CrossRef]
- Trzepiecinski, T. Recent Developments and Trends in Sheet Metal Forming. Metals 2020, 10, 779. [Google Scholar] [CrossRef]
- Luo, W.; Chen, F.; Xu, B.B.; Yang, Z.J.; Guo, Y.M.; Lu, B.; Huang, T. Study on compound spinning technology of large thin-walled parts with ring inner ribs and curvilinear generatrix. Int. J. Adv. Manuf. Technol. 2018, 98, 1199–1216. [Google Scholar] [CrossRef]
- Wu, H.; Xu, W.; Shan, D.; Jin, B.C. Mechanism of increasing spinnability by multi-pass spinning forming—Analysis of damage evolution using a modified GTN model. Int. J. Mech. Sci. 2019, 159, 1–19. [Google Scholar] [CrossRef]
- Wen, X.; Tan, J.P.; Li, X.H. Optimization of spinning process parameters for the large-diameter thin-walled cylinder based on the drum shape. Int. J. Adv. Manuf. Technol. 2020, 108, 2315–2335. [Google Scholar] [CrossRef]
- Li, P.Y.; He, J.; Liu, Q.; Yang, M.S.; Wang, Q.D.; Yuan, Q.L.; Li, Y. Evaluation of forming forces in ultrasonic incremental sheet metal forming. Aerosp. Sci. Technol. 2017, 63, 132–139. [Google Scholar] [CrossRef] [Green Version]
- Sun, J.; Kang, R.; Qin, Y.; Wang, Y.; Dong, Z. Simulated and Experimental Study on the Ultrasonic Cutting Mechanism of Aluminum Honeycomb by Disc Cutter. Compos. Struct. 2021, 275, 114431. [Google Scholar] [CrossRef]
- Lee, H.; Singh, R. Acoustic radiation from out-of-plane modes of an annular disk using thin and thick plate theories. J. Sound Vib. 2005, 282, 313–339. [Google Scholar] [CrossRef]
- Du, P.; Han, L.; Qiu, X.; Chen, W.; Deng, J.; Liu, Y.; Zhang, J. Development of a high-precision piezoelectric ultrasonic milling tool using longitudinal-bending hybrid transducer. Int. J. Mech. Sci. 2022, 222, 107239. [Google Scholar] [CrossRef]
- Liu, Y.X.; Wang, L.; Gu, Z.Z.; Quan, Q.Q.; Deng, J. Development of a Two-Dimensional Linear Piezoelectric Stepping Platform Using Longitudinal-Bending Hybrid Actuators. IEEE Trans. Ind. Electron. 2019, 66, 3030–3040. [Google Scholar] [CrossRef]
- Shen, Q.Q.; Liu, Y.X.; Wang, L.; Liu, J.K.; Li, K. A long stroke linear stepping piezoelectric actuator using two longitudinal bending hybrid transducers. Ceram. Int. 2018, 44, S104–S107. [Google Scholar] [CrossRef]
- Yang, X.; Liu, Y.; Chen, W.; Liu, J. Miniaturization of a longitudinal–bending hybrid linear ultrasonic motor. Ceram. Int. 2015, 41, S607–S611. [Google Scholar] [CrossRef]
- Liu, Y.; Yan, J.; Wang, L.; Chen, W. A Two-DOF Ultrasonic Motor Using a Longitudinal–Bending Hybrid Sandwich Transducer. IEEE Trans. Ind. Electron. 2019, 66, 3041–3050. [Google Scholar] [CrossRef]
- Narayanan, M.M.; Arjun, V.; Kumar, A.; Mukhopadhyay, C.K. Development of In-bore Magnetostrictive Transducer for Ultrasonic Guided Wave based-inspection of Steam Generator Tubes of PFBR. Ultrasonics 2020, 106, 106148. [Google Scholar] [CrossRef] [PubMed]
- Fang, S.; Zhang, Q.; Zhao, H.; Yu, J.; Chu, Y. The Design of Rare-Earth Giant Magnetostrictive Ultrasonic Transducer and Experimental Study on Its Application of Ultrasonic Surface Strengthening. Micromachines 2018, 9, 98. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ma, K.; Wang, J.; Zhang, J.; Feng, P.; Yu, D.; Ahmad, S. A highly temperature-stable and complete-resonance rotary giant magnetostrictive ultrasonic system. Int. J. Mech. Sci. 2022, 214, 106927. [Google Scholar] [CrossRef]
- Senjanovic, I.; Hadzic, N.; Vladimir, N.; Cho, D.S. Natural vibrations of thick circular plate based on the modified Mindlin theory. Arch. Mech. 2014, 66, 389–409. [Google Scholar]
- Lezgy-Nazargah, M. A high-performance parametrized mixed finite element model for bending and vibration analyses of thick plates. Acta Mech. 2016, 227, 3429–3450. [Google Scholar] [CrossRef]
- Farahmand, H. Analytical solutions of bending and free vibration of moderately thick micro-plate via two-variable strain gradient theory. J. Braz. Soc. Mech. Sci. Eng. 2020, 42, 251. [Google Scholar] [CrossRef]
- Usarov, D.; Turajonov, K.; Khamidov, S. Simulation of free vibrations of a thick plate without simplifying hypotheses. J. Phys. Conf. Ser. 2019, 1425, 012115. [Google Scholar] [CrossRef]
- Niu, Y.; Jiao, F.; Li, J.; Zhang, J.F. Study on the Effect of Vibration Amplitude in Two-Dimension Ultrasonic Vibration Cutting. Adv. Mater. Res. 2014, 1027, 131–135. [Google Scholar] [CrossRef]
- Niu, Y.; Jiao, F.; Tong, J.L.; Li, J.; Zhang, J.F. Research on Vibration Characteristics of Horn with Longitudinal Bending Vibration. Appl. Mech. Mater. 2014, 551, 121–126. [Google Scholar] [CrossRef]
- Shahid, M.B.; Jung, J.Y.; Park, D.S. Finite element analysis coupled artificial neural network approach to design the longitudinal-torsional mode ultrasonic welding horn. Int. J. Adv. Manuf. Technol. 2020, 107, 2731–2743. [Google Scholar] [CrossRef]
- Chen, T.; Liu, S.; Liu, W.; Wu, C. Study on a longitudinal-torsional ultrasonic vibration system with diagonal slits. Adv. Mech. Eng. 2017, 9, 168781401770634. [Google Scholar] [CrossRef]
- Li, P.Y.; Liu, Q.; Li, S.J.; Wang, Q.D.; Zhang, D.Y.; Li, Y. Design and numerical simulation of novel giant magnetostrictive ultrasonic transducer. Results Phys. 2017, 7, 3946–3954. [Google Scholar] [CrossRef]
- Chiu, Y.; Li, X.; Yu, G.; Yang, C.; Pan, J.; Chen, C. Research on effect of rings on coupling vibration in a rigid-disk rotor. J. Mech. Sci. Technol. 2020, 34, 521–530. [Google Scholar] [CrossRef]









| Parameters | Value |
|---|---|
| Large cylindrical segment length l1 (mm) | 30 |
| Large cylindrical segment diameter d1 (mm) | 46 |
| Conical segment length l2 (mm) | 30 |
| Small cylindrical segment diameter d2 (mm) | 20 |
| Young’s modulus E (GPa) | 193 |
| Density ρ (kg/m3) | 8000 |
| Poisson ratio ν | 0.28 |
| Parameters | Value |
|---|---|
| Corner radius, r (mm) | 2 |
| Small diameter, d (mm) | 40 |
| Large diameter, D (mm) | 80 |
| Thickness, t (mm) | 16 |
| Parameters | Value |
|---|---|
| Large cylindrical segment length l1 (mm) | 30 |
| Large cylindrical segment diameter d1 (mm) | 46 |
| Conical segment length l2 (mm) | 30 |
| Small cylindrical segment length l3 (mm) | 51 |
| Small cylindrical segment diameter d2 (mm) | 20 |
| Young’s modulus E (GPa) | 193 |
| Density ρ (kg/m3) | 8000 |
| Poisson ratio ν | 0.28 |
| Small diameter d (mm) | 40 |
| Large diameter D (mm) | 80 |
| Thickness t (mm) | 14 |
| corner radius r (mm) | 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, P.; Chen, Y.; Li, W.; Sun, J.; Li, J.; Wang, K. Design of Longitudinal-Bending Coupled Horn of a Giant Magnetostriction Transducer. Actuators 2022, 11, 110. https://doi.org/10.3390/act11040110
Li P, Chen Y, Li W, Sun J, Li J, Wang K. Design of Longitudinal-Bending Coupled Horn of a Giant Magnetostriction Transducer. Actuators. 2022; 11(4):110. https://doi.org/10.3390/act11040110
Chicago/Turabian StyleLi, Pengyang, Yunshuai Chen, Wei Li, Jian Sun, Jian Li, and Kai Wang. 2022. "Design of Longitudinal-Bending Coupled Horn of a Giant Magnetostriction Transducer" Actuators 11, no. 4: 110. https://doi.org/10.3390/act11040110
APA StyleLi, P., Chen, Y., Li, W., Sun, J., Li, J., & Wang, K. (2022). Design of Longitudinal-Bending Coupled Horn of a Giant Magnetostriction Transducer. Actuators, 11(4), 110. https://doi.org/10.3390/act11040110






