Design and Control of a Nonlinear Series Elastic Cable Actuator Based on the Hill Muscle Model
Abstract
:1. Introduction
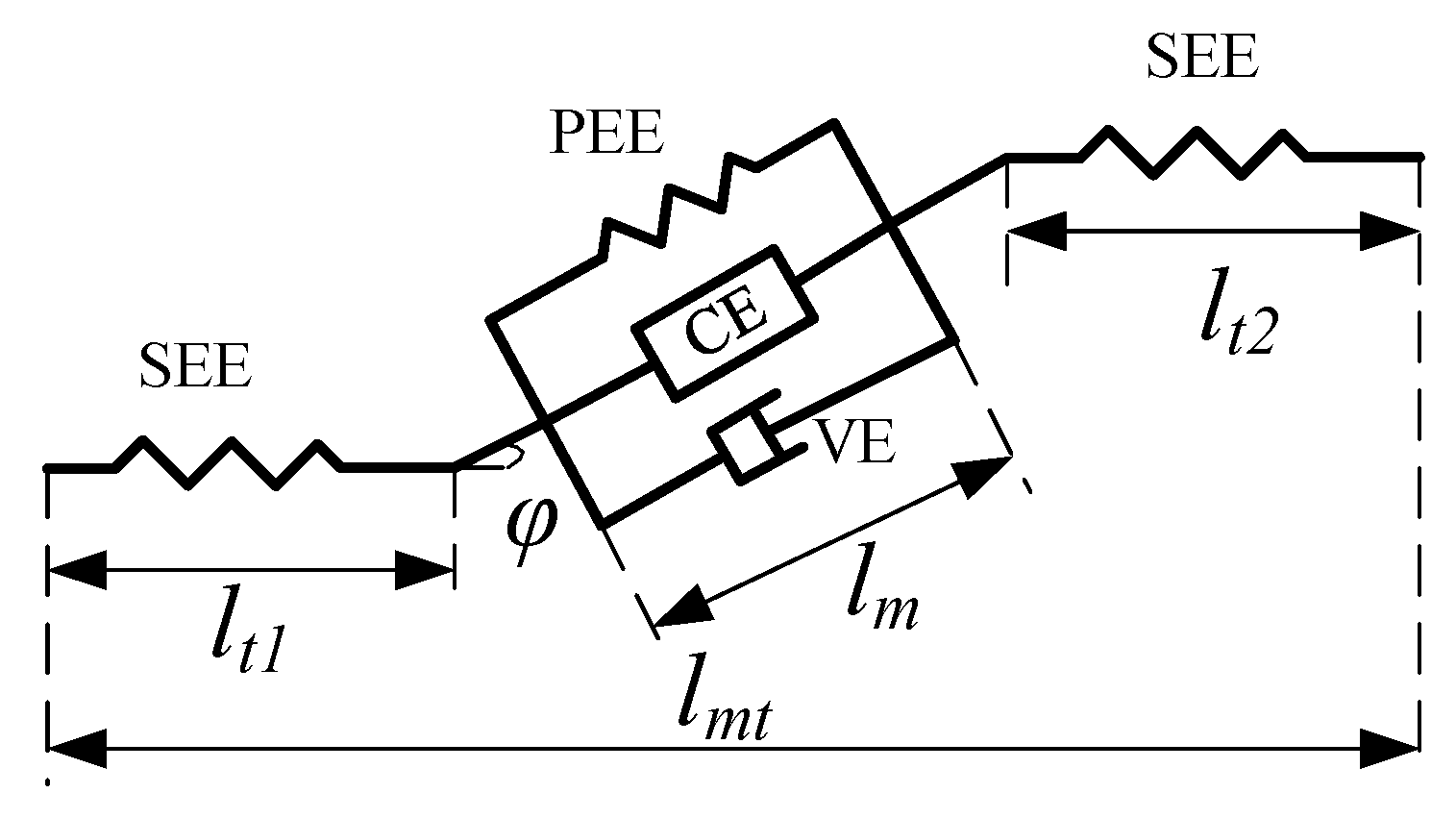
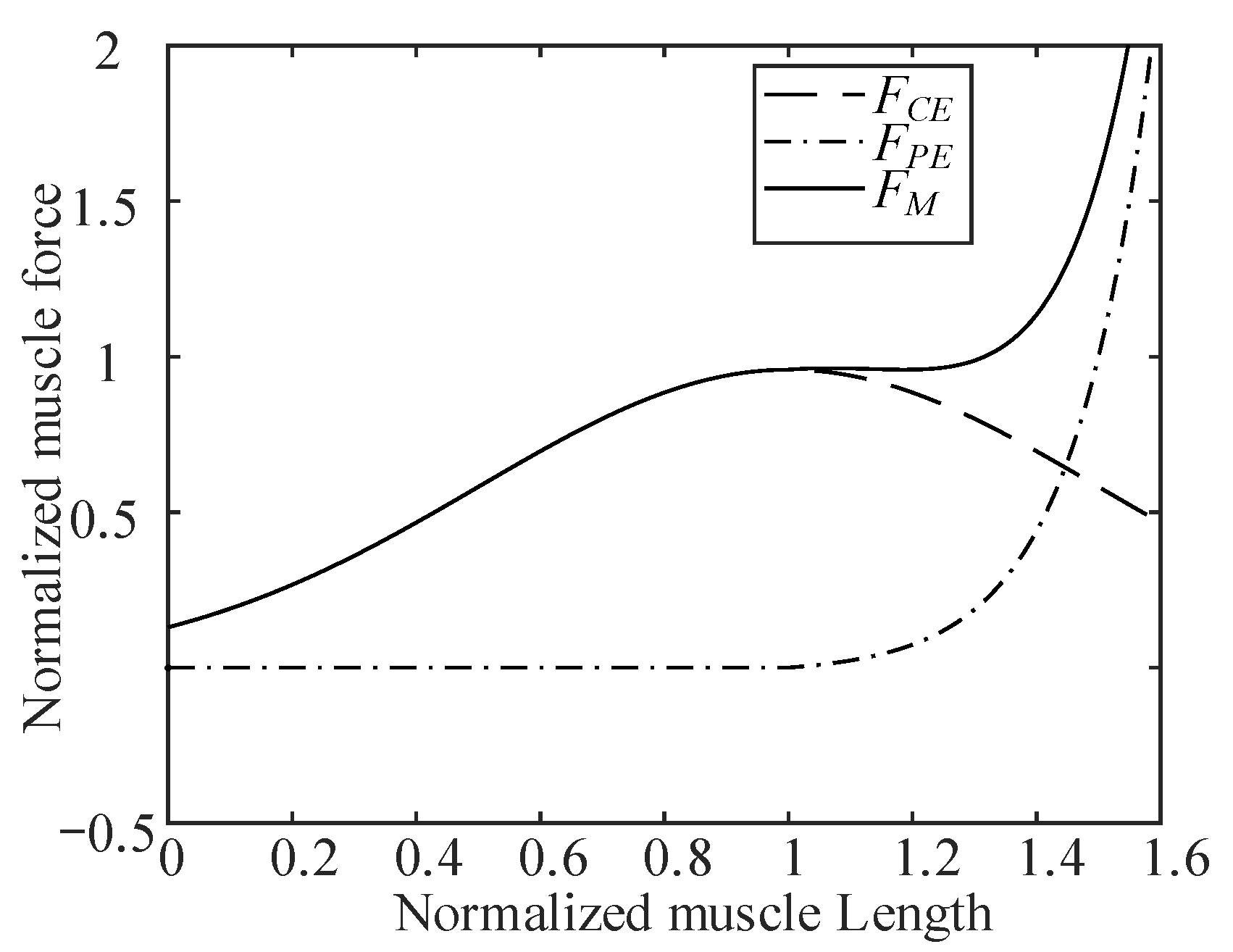
2. Muscle Model
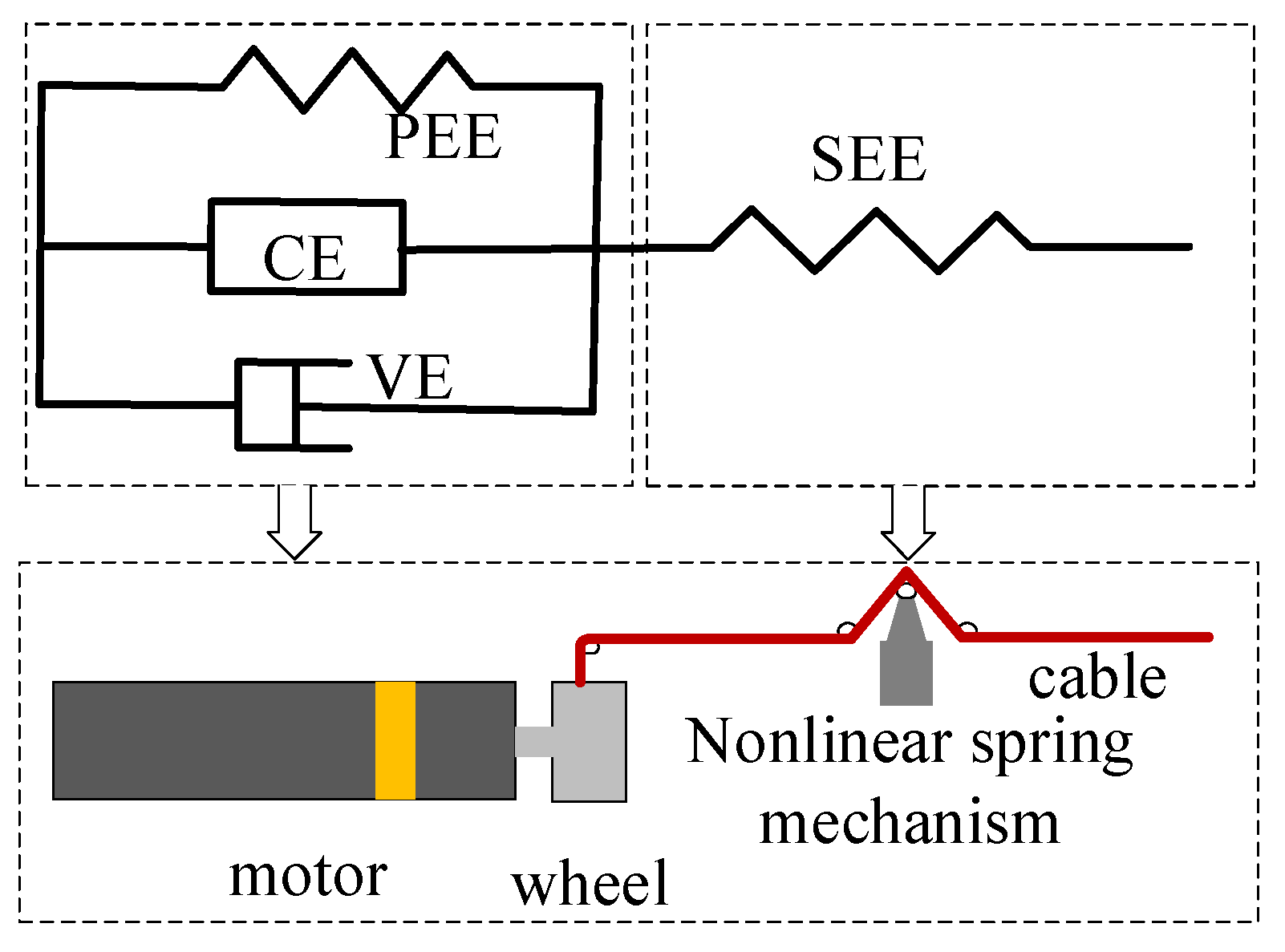
3. Mechanism of the Actuator
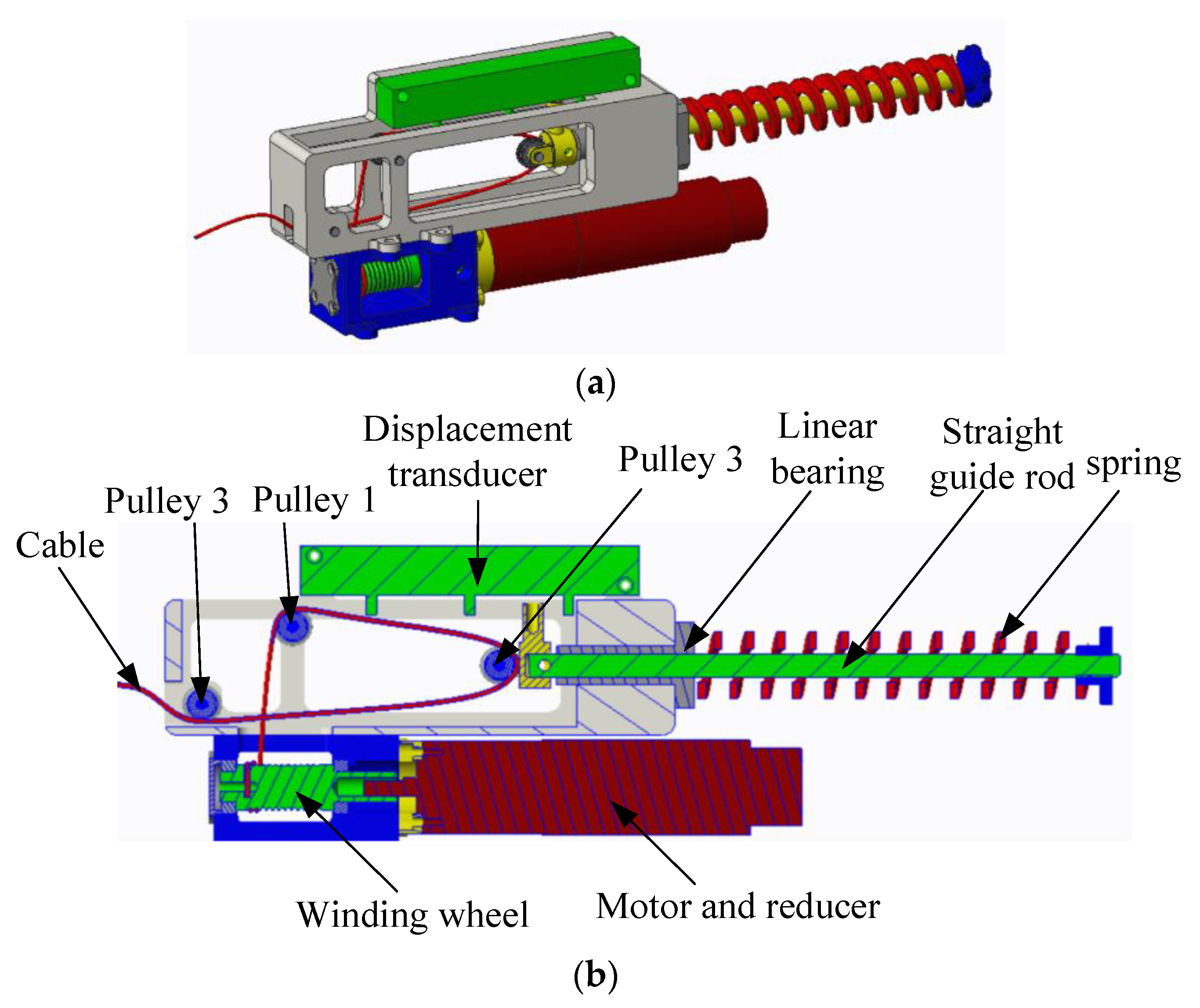
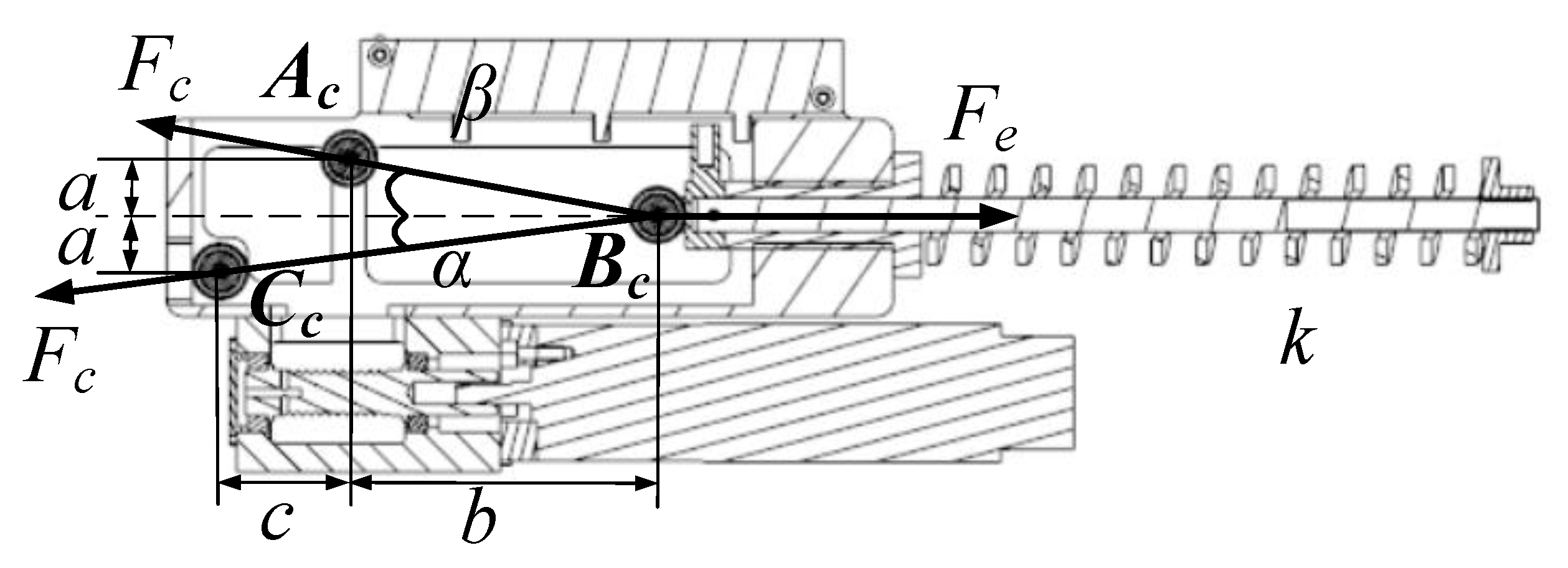
3.1. Mechanism Design
3.2. Nonlinear Elastic Analysis
3.3. Mechanism Parameter Optimization for Passive Elastic Imitation
4. Active Contraction Force Control
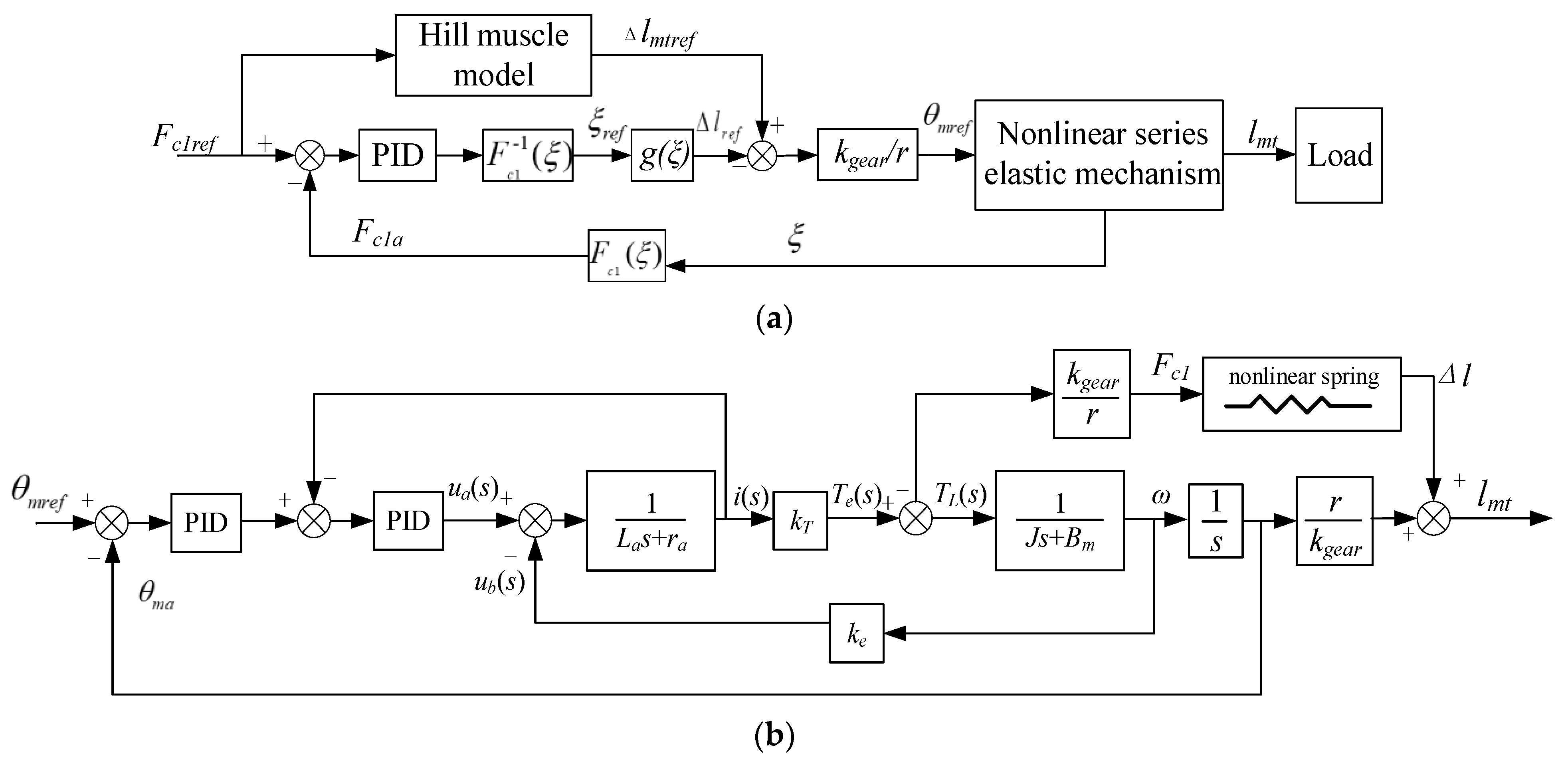
4.1. Modeling of the Actuator
4.2. Force Control Method of the Actuator
5. Experiments
5.1. Experiments Platform
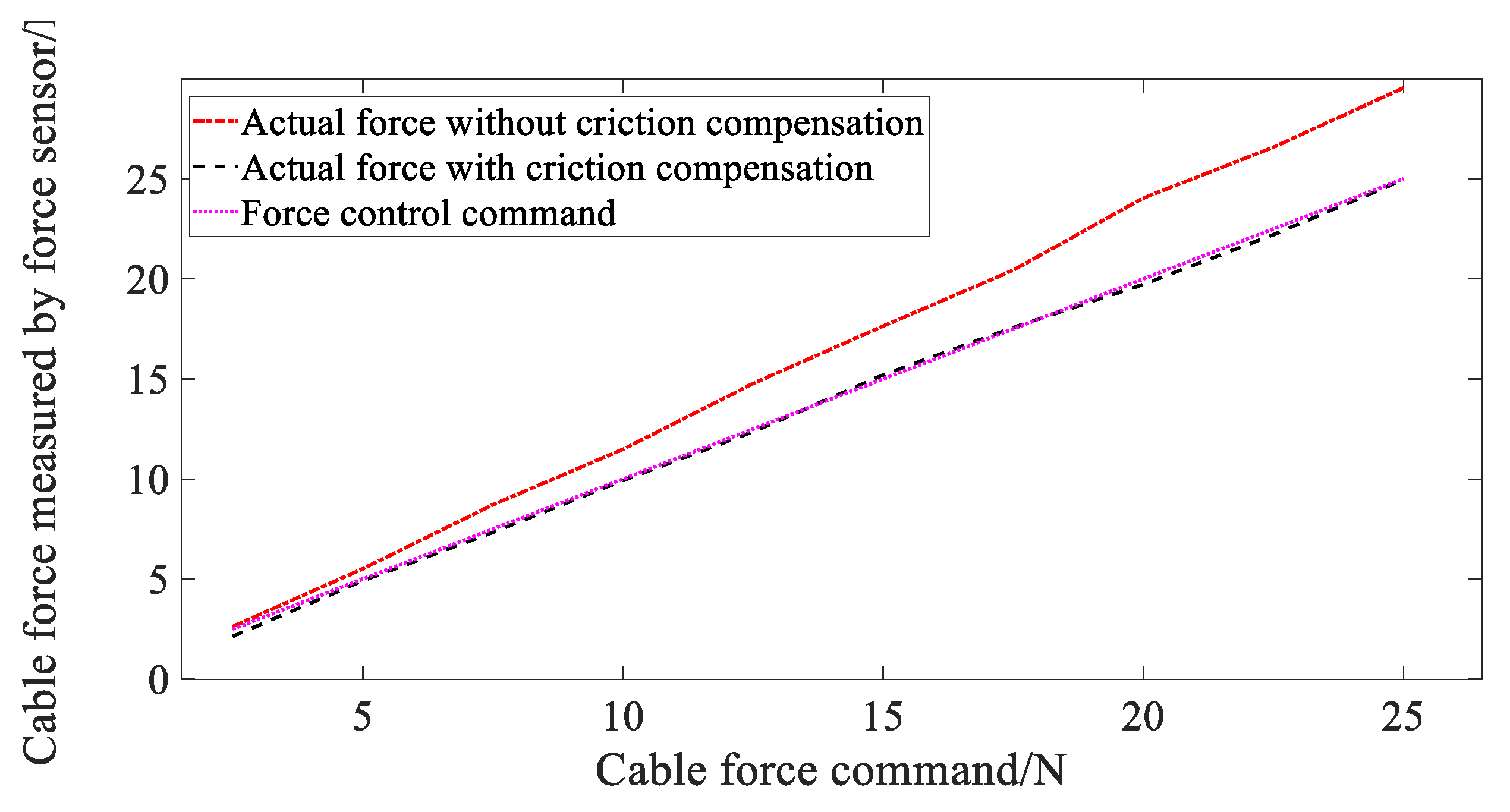
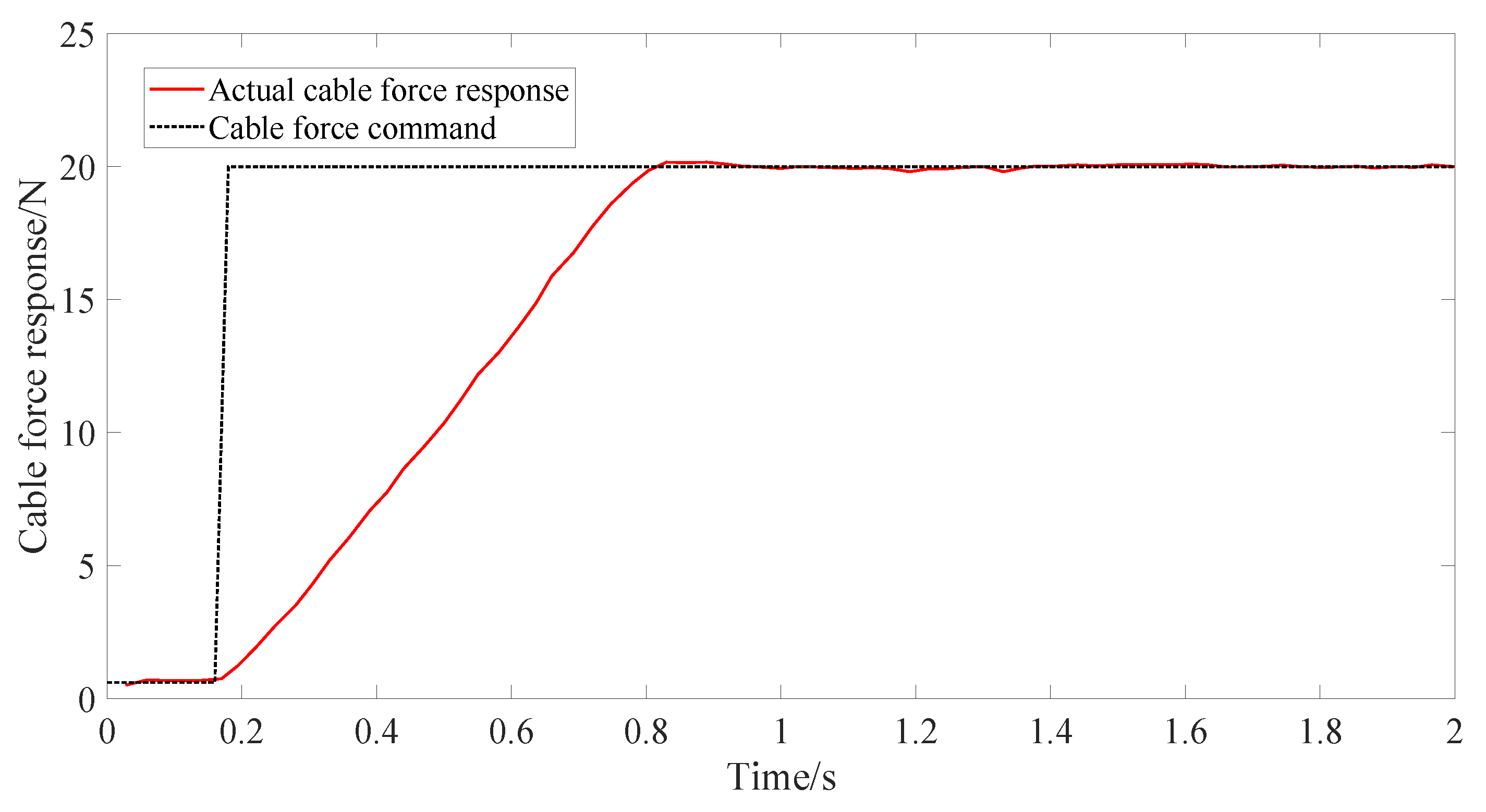
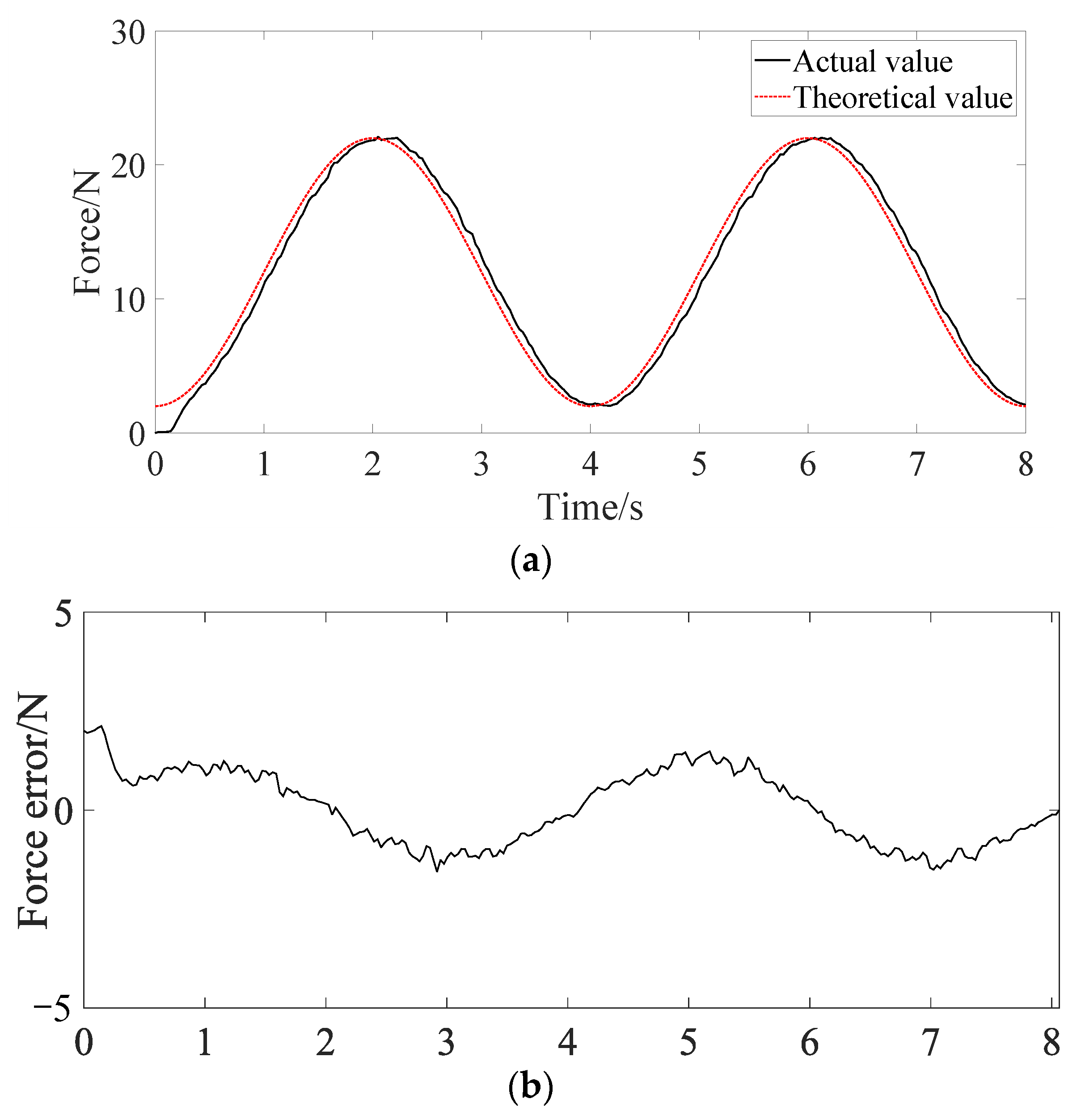
5.2. Force Control Experiments of the Actuator
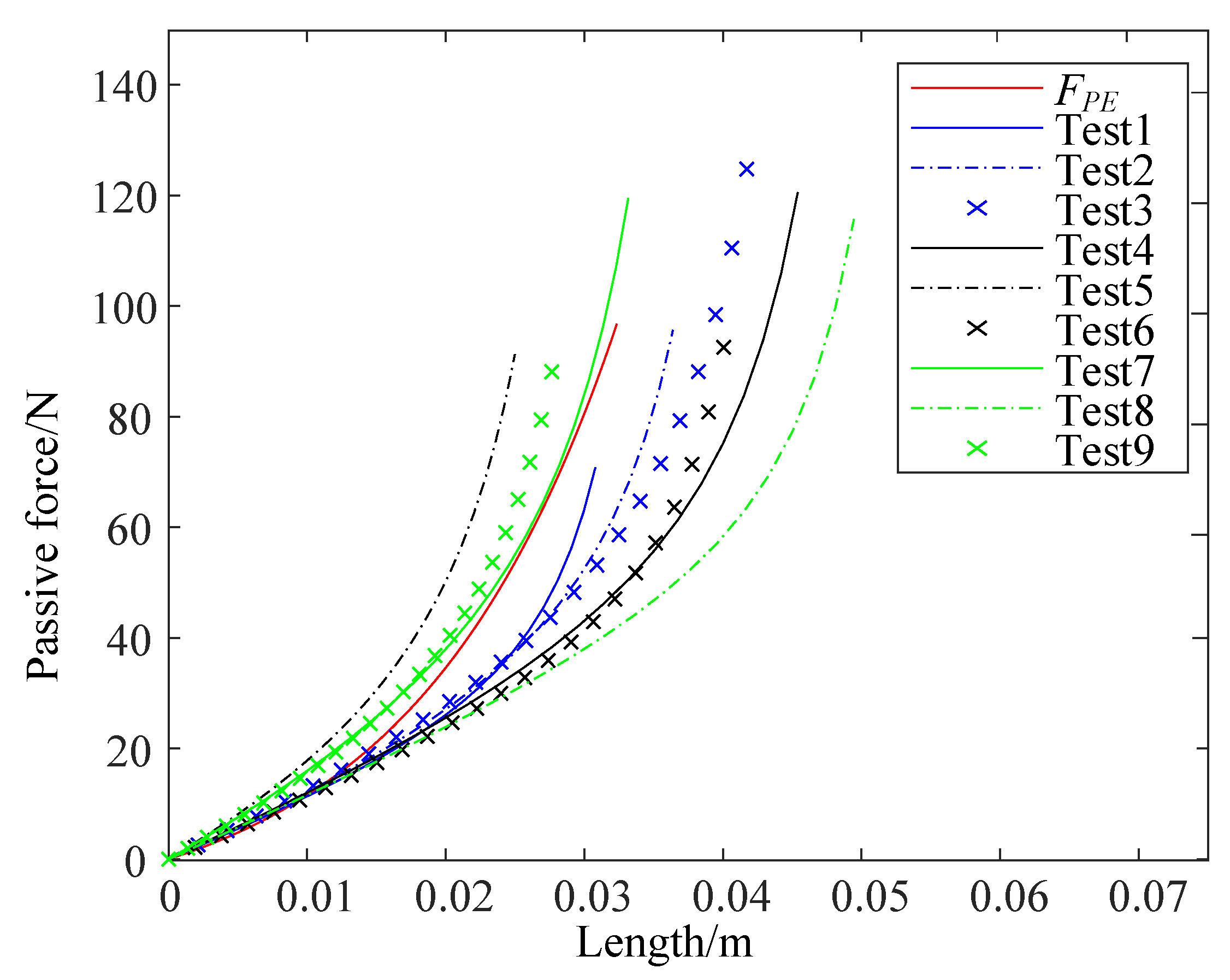
5.3. Passive Elastic Performance Verification
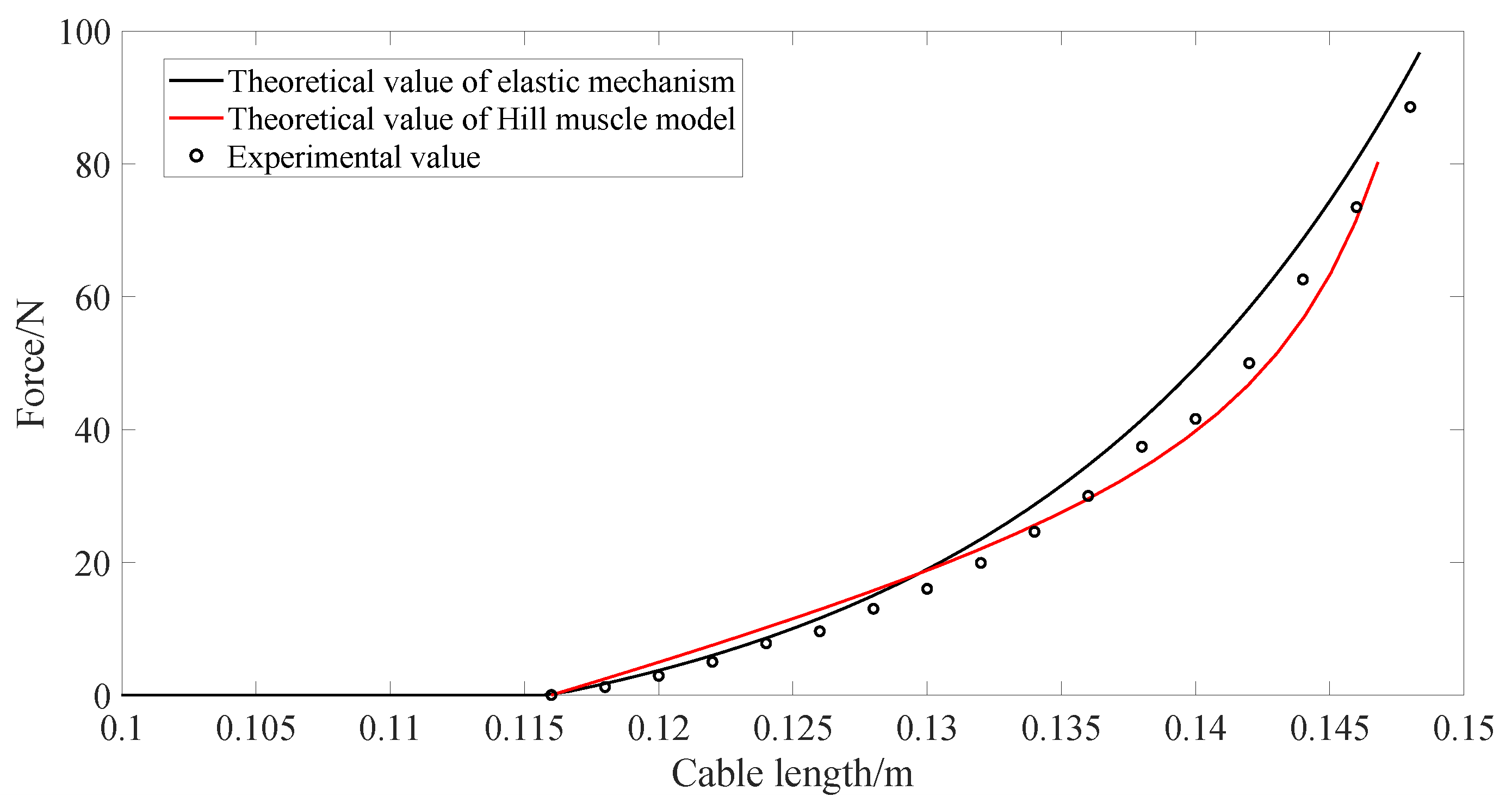
5.4. Contraction Force–Position Relationship Verification
6. Conclusions and Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations and Symbols
| NSECA | nonlinear series elastic cable actuator |
| SEE | series elastic element |
| PEE | passive elastic element |
| CE | contraction element |
| VE | viscous damping element |
| PID | Proportion-Integration-Differentiation |
| pinnate angle | |
| lmt | muscle length |
| lm | muscle fiber length |
| lt1, lt2 | the tendon lengths at both ends |
| muscle force | |
| FCE | active forces of the muscle fibers |
| FPE | passive forces of the muscle fibers |
| FVE | viscous damping forces |
| muscle activation degree | |
| fl | influence factor of the muscle fiber length |
| fv | influence factor of the muscle fiber contraction speed |
| F0 | maximum isometric contraction force at rest |
| u(t) | normalized EMG signal |
| A | nonlinearity degree |
| lm | real-time muscle fiber length |
| lmopt | resting muscle fiber length |
| γ | shape coefficient |
| vn | normalized contraction velocity |
| As | curve parameter |
| fM | maximum force of muscle fiber elongation |
| kPE | curve shape parameter |
| maximum passive strain | |
| Fe | spring force |
| Δl | change of the cable length caused by the spring deformation |
| k | elastic coefficient of the spring |
| Fc | the tension on the cable |
| Km | the equivalent stiffness of the actuator |
| τm | torque output of the motor |
| kgear | reduction ratio of the planetary reducer |
| r | the radius of the winding wheel |
| Jm | inertia moment of the motor |
| JW | inertia moment of the winding wheel |
| θm | angular displacement of the motor |
| Δlmt | the total displacement of the cable actuator |
| θm | the angular displacement of the motor |
| Δl(ξ) | cable length change caused by nonlinear spring deformation |
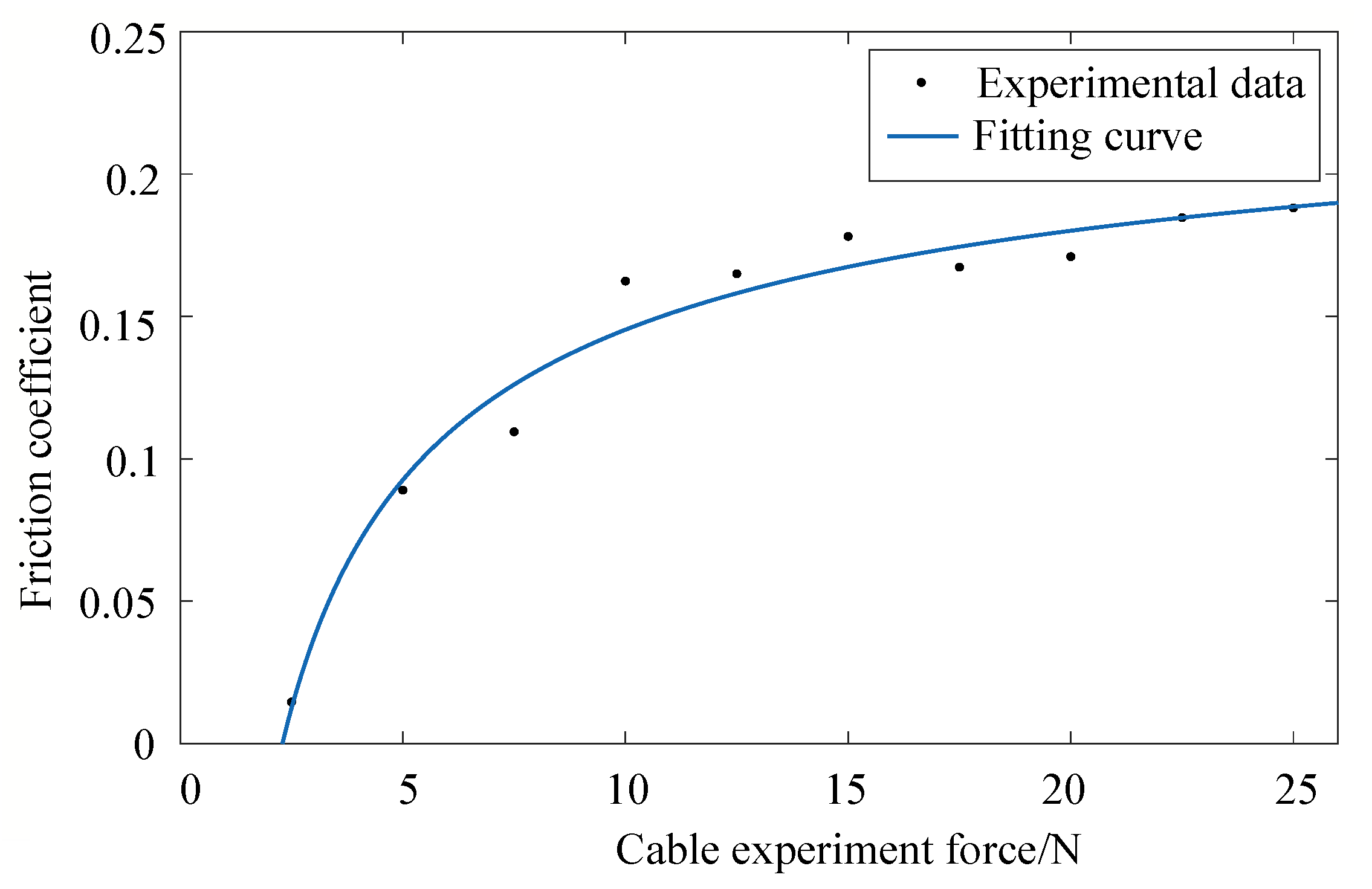
| k1 | load-related friction coefficient |
| Fc1 | the cable end tension after compensation. |
| ua | armature voltage of the motor |
| ra | interphase resistance |
| i | loop current |
| La | interphase inductance |
| kb | back electromotive force constant |
| kt | torque constant of the motor |
References
- Chen, W.; Xiong, C.; Wang, Y. Analysis and synthesis of underactuated compliant mechanisms based on transmission properties of motion and force. IEEE Trans. Robot. 2020, 36, 773–788. [Google Scholar] [CrossRef]
- Shao, Z.; Wu, Q.; Chen, B.; Wu, H.; Zhang, Y. Modeling and inverse control of a compliant single-tendon-sheath artificial tendon actuator with bending angle compensation. Mechatronics 2019, 63, 102262. [Google Scholar] [CrossRef]
- Chen, S.; Tan, M.W.M.; Gong, X.; Lee, P.S. Low-voltage soft actuators for Interactive human–machine interfaces. Adv. Intell. Syst. 2021, 4, 2100075. [Google Scholar] [CrossRef]
- Xu, Y.; Guo, K.; Sun, J.; Li, J. Design, modeling and control of a reconfigurable variable stiffness actuator. Mech. Syst. Signal Processing 2021, 160, 107883. [Google Scholar] [CrossRef]
- Higueras-Ruiz, D.R.; Shafer, M.W.; Feigenbaum, H.P. Cavatappi artificial muscles from drawing, twisting, and coiling polymer tubes. Sci. Robot. 2021, 6, eabd5383. [Google Scholar] [CrossRef]
- Sabarianand, D.; Karthikeyan, P.; Muthuramalingam, T. A review on control strategies for compensation of hysteresis and creep on piezoelectric actuators based micro systems. Mech. Syst. Signal Processing 2020, 140, 106634. [Google Scholar] [CrossRef]
- Zhao, S.; Li, D.; Zhou, J.; Sha, E. In Numerical and Experimental Study of a Flexible Trailing Edge Driving by Pneumatic Muscle Actuators. Actuators 2021, 10, 142. [Google Scholar] [CrossRef]
- Guan, J.H.; Pei, Y.C.; Wu, J.T. A driving strategy of shape memory alloy wires with electric resistance modeled by logistic function for power consumption reduction. Mech. Syst. Signal Processing 2021, 160, 107839. [Google Scholar] [CrossRef]
- Bombara, D.; Fowzer, S.; Zhang, J. Compliant, large-strain, and self-sensing twisted string actuators. Soft Robot. 2020, 9, 72–88. [Google Scholar] [CrossRef]
- Zhang, C.; Wei, W.; Sun, H.; Zhu, Q. Study on the properties of different dielectric elastomers applying to actuators. Sens. Actuators A Phys. 2021, 329, 112806. [Google Scholar] [CrossRef]
- Doregiraei, M.J.; Moeinkhah, H.; Sadeghi, J. A fractional order model for electrochemical impedance of IPMC actuators based on constant phase element. J. Intel. Mat. Syst. Str. 2021, 32, 880–888. [Google Scholar] [CrossRef]
- Seo, J.S.; Kim, D.; Hwang, S.; Shim, S.E. A review on recent development and applications of dielectric elastomers. Elastomers Compos. 2021, 56, 57–64. [Google Scholar]
- Zhang, C.; Zhu, P.; Lin, Y.; Jiao, Z.; Zou, J. Modular soft robotics: Modular units, connection mechanisms, and applications. Adv. Intell. Syst. 2020, 2, 1900166. [Google Scholar] [CrossRef] [Green Version]
- Thelen, D.G. Adjustment of muscle mechanics model parameters to simulate dynamic contractions in older adults. J. Biomech. Eng. 2003, 125, 70–77. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Q.; Yang, M.; Shen, X.; Tian, M.; Wang, X. Muscle-like contraction control of tendon-sheath artificial muscle. Mechatronics 2021, 77, 102584. [Google Scholar] [CrossRef]
- Shao, Y.; Zhang, W.; Su, Y.; Ding, X. Design and optimisation of load-adaptive actuator with variable stiffness for compact ankle exoskeleton. Mech. Mach. Theory 2021, 161, 104323. [Google Scholar] [CrossRef]
- Niehues, T.D.; Rao, P.; Deshpande, A.D. Compliance in parallel to actuators for improving stability of robotic hands during grasping and manipulation. Int. J. Robot. Res. 2015, 34, 256–269. [Google Scholar] [CrossRef]
- Plooij, M.; Wolfslag, W.; Wisse, M. Clutched elastic actuators. IEEE/ASME Trans. Mechatron. 2017, 22, 739–750. [Google Scholar] [CrossRef] [Green Version]
- Furnémont, R.; Mathijssen, G.; Verstraten, T.; Lefeber, D.; Vanderborght, B. Bi-directional series-parallel elastic actuator and overlap of the actuation layers. Bioinspir. Biomim. 2016, 11, 016005. [Google Scholar] [CrossRef] [Green Version]
- Moore, R.; Schimmels, J.M. Design of a quadratic, Antagonistic, Cable-driven, Variable stiffness actuator. J. Mech. Robot. 2021, 13, 031001. [Google Scholar] [CrossRef]
- Zhang, Q.; Wang, X.; Tian, M.; Shen, X.; Wu, Q. Modeling of novel compound tendon-sheath artificial muscle inspired by hill muscle model. IEEE T Ind. Electron. 2017, 65, 6372–6381. [Google Scholar] [CrossRef]
- Schmitt, S.; Haeufle, D.F.B.; Blickhan, R.; Günther, M. Nature as an engineer: One simple concept of a bio-inspired functional artificial muscle. Bioinspir. Biomim. 2012, 7, 036022. [Google Scholar] [CrossRef] [PubMed]
- Shen, X.; Wang, X.; Tian, M.; Zhang, Q. Modeling and sensorless force control of novel tendon-sheath artificial muscle based on hill muscle model. Mechatronics 2019, 62, 102243. [Google Scholar] [CrossRef]
- Shao, Z.; Wu, Q.; Chen, B.; Wu, H. Horce and deformation transmission characteristics of a compliant tendon–sheath actuation system based on Hill-type muscle model. Proc. Inst. Mech. Eng. Part H J. Eng. Med. 2019, 233, 695–705. [Google Scholar] [CrossRef] [PubMed]
- Gialias, N.; Matsuoka, Y. Muscle actuator design for the ACT Hand. In Proceedings of the IEEE International Conference on Robotics and Automation (ICRA), New Orleans, LA, USA, 26 April–1 May 2004; pp. 3380–3385. [Google Scholar]
- Richter, C.; Jentzsch, S.; Hostettler, R.; Garrido, J.A.; Ros, E.; Knoll, A.; Rohrbein, F.; van der Smagt, P.; Conradt, J. Musculoskeletal robots: Scalability in neural control. IEEE Robot. Autom. Mag. 2016, 23, 128–137. [Google Scholar] [CrossRef] [Green Version]
- Asano, Y.; Okada, K.; Inaba, M. Design principles of a human mimetic humanoid: Humanoid platform to study human intelligence and internal body system. Sci. Robot. 2017, 2, eaaq0899. [Google Scholar] [CrossRef] [Green Version]
- Shao, Q.; Bassett, D.N.; Manal, K.; Buchanan, T.S. An EMG-driven model to estimate muscle forces and joint moments in stroke patients. Comput. Biol. Med. 2009, 39, 1083–1088. [Google Scholar] [CrossRef] [Green Version]
- Holzbaur, K.R.; Murray, W.M.; Delp, S.L. A model of the upper extremity for simulating musculoskeletal surgery and analyzing neuromuscular control. Ann. Biomed. Eng. 2005, 33, 829–840. [Google Scholar] [CrossRef]
- Reinecke, J.; Chalon, M.; Friedl, W.; Grebenstein, M. Guiding effects and friction modeling for tendon driven systems. In Proceedings of the 2014 IEEE International Conference on Robotics and Automation (ICRA), Hong Kong, China, 31 May–7 June 2014; pp. 6726–6732. [Google Scholar]
- Wang, J.; Zhang, H.; Dong, H.; Zhao, J. Partial-state feedback based dynamic surface motion control for series elastic actuators. Mech. Syst. Signal Processing 2021, 160, 107837. [Google Scholar] [CrossRef]

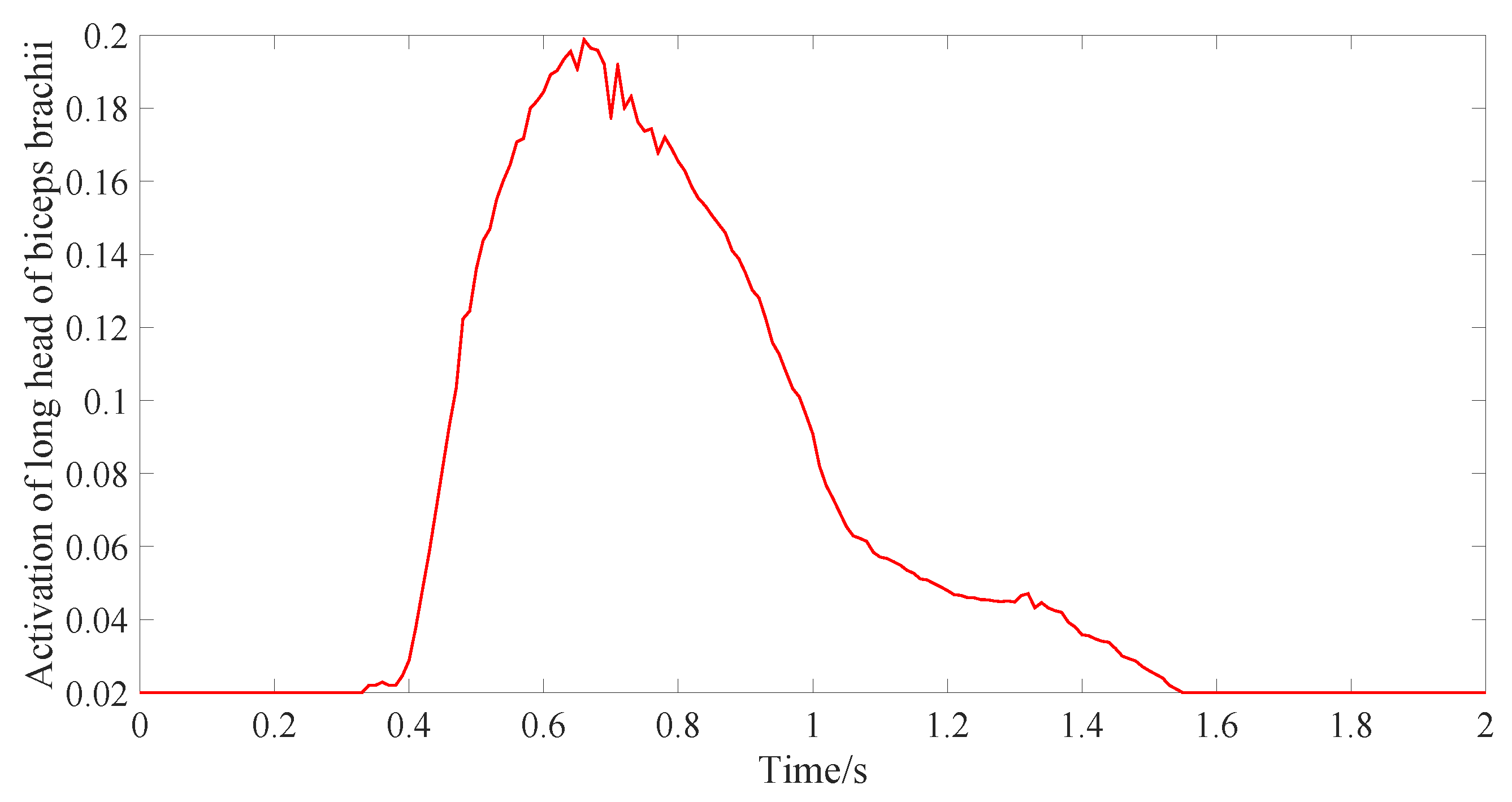
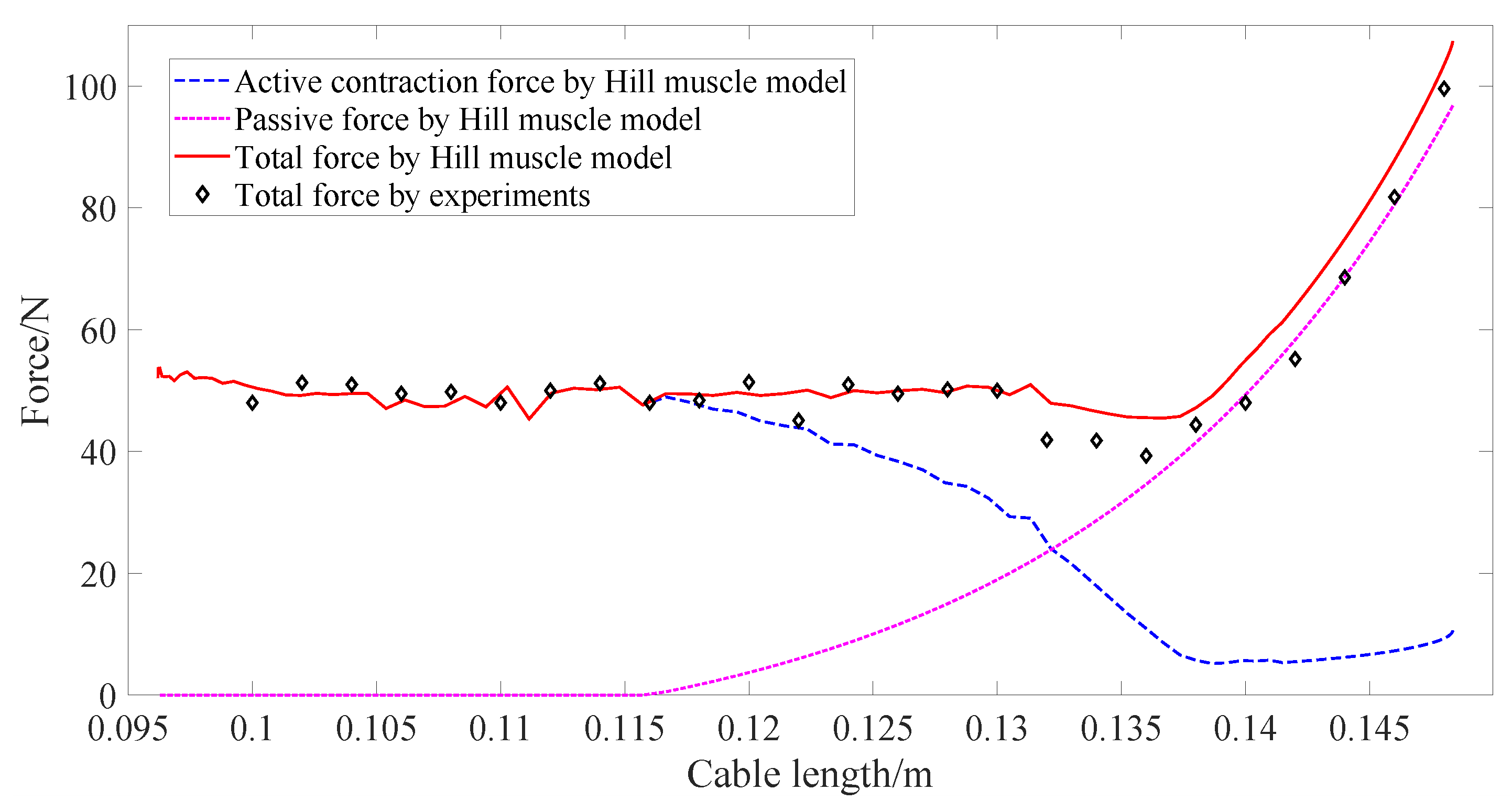
| Level | k/(N·m−1) | a/m | b/m |
|---|---|---|---|
| 1 | 3750 | 0.01 | 0.02 |
| 2 | 4000 | 0.015 | 0.025 |
| 3 | 4250 | 0.02 | 0.03 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Su, Y.; Zou, H.; Lu, H.; Hu, B.; Yu, H. Design and Control of a Nonlinear Series Elastic Cable Actuator Based on the Hill Muscle Model. Actuators 2022, 11, 68. https://doi.org/10.3390/act11030068
Su Y, Zou H, Lu H, Hu B, Yu H. Design and Control of a Nonlinear Series Elastic Cable Actuator Based on the Hill Muscle Model. Actuators. 2022; 11(3):68. https://doi.org/10.3390/act11030068
Chicago/Turabian StyleSu, Yingbing, Huaiwu Zou, Hongrun Lu, Bingshan Hu, and Hongliu Yu. 2022. "Design and Control of a Nonlinear Series Elastic Cable Actuator Based on the Hill Muscle Model" Actuators 11, no. 3: 68. https://doi.org/10.3390/act11030068
APA StyleSu, Y., Zou, H., Lu, H., Hu, B., & Yu, H. (2022). Design and Control of a Nonlinear Series Elastic Cable Actuator Based on the Hill Muscle Model. Actuators, 11(3), 68. https://doi.org/10.3390/act11030068






