Methodology for Shape Optimization of Magnetic Designs: Magnetic Spring Characteristic Tailored to Application Needs
Abstract
:1. Introduction
- weaving looms and other textile machinery featuring reciprocating motion;
- agricultural machines, e.g., reciprocating moving parts in combine harvesters;
- metal forming machines such as punching and bending machines;
- packaging machinery;
- pick and place robots;
- humanoid robots and exoskeletons;
- torque ripple in drivetrains with gears due to the variable gear meshing;
- internal combustion engines, particularly in hybrid drivetrain;
- fans, blowers and centrifugal compressors due to aerodynamic effects;
- other compressors and pumps, such as centrifugal, rotary lobe, piston, screw etc.;
- wind turbines due to pole shade effect.
2. Methodology
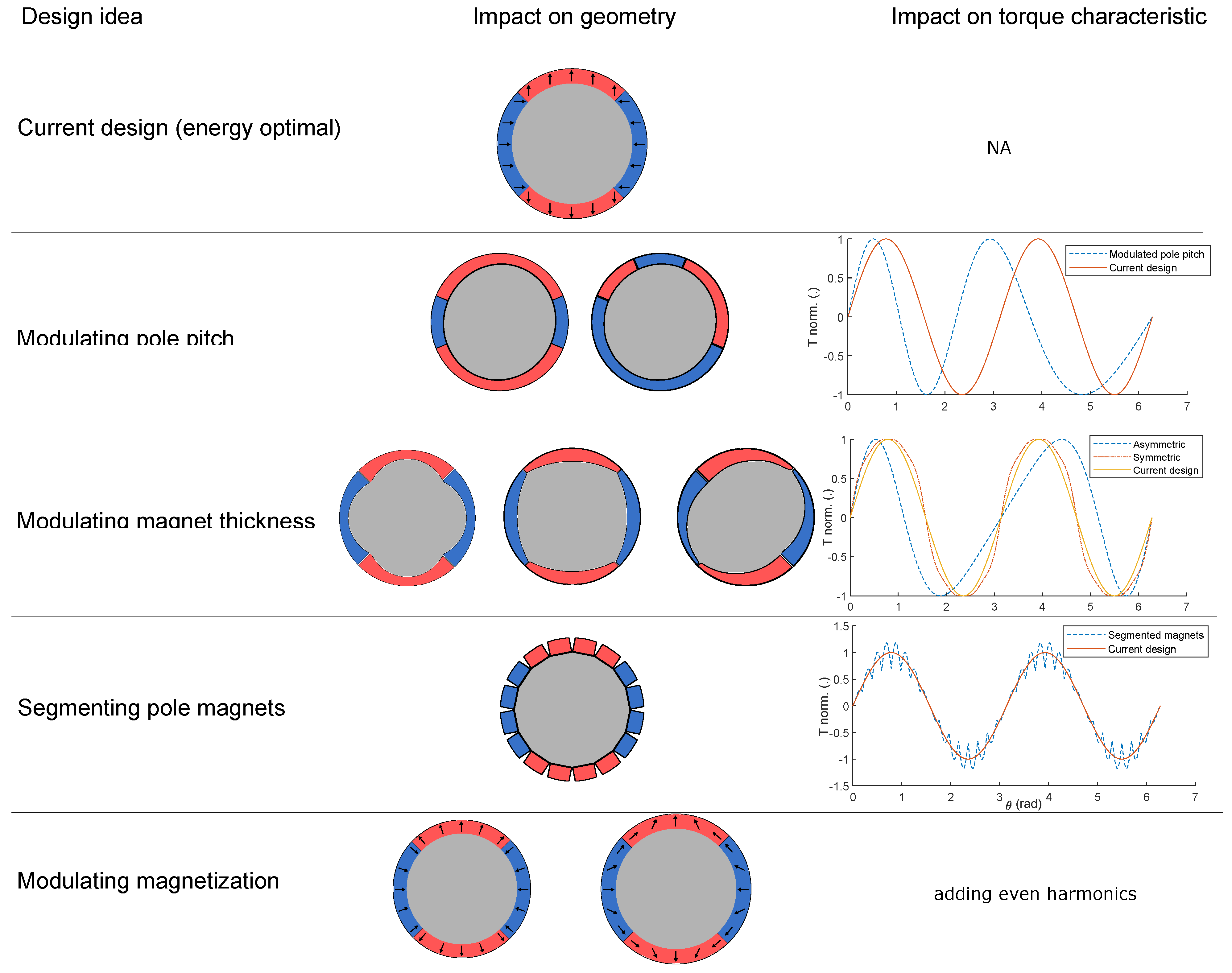
2.1. Decomposition Based Approach
- Manufacturing simplicity of the magnetic springs;
- Improved performance at compensating specific isolated orders, e.g., in applications where specific higher orders can be related to the individual physical effects such as gear meshing, ICE firing order, etc.
- Higher material cost, i.e., more rare-earth magnets required for same equivalent energy content as depending on the phase alignment and order, separate order torques partially cancel out;
- higher mechanical complexity, i.e., multiple stators and rotors need to be manufactured and assembled together;
- Difficulty of capturing all characteristics with limited number of orders (<3);
- Issues with high gradients torque characteristic due to the Gibbs phenomenon even with higher order.
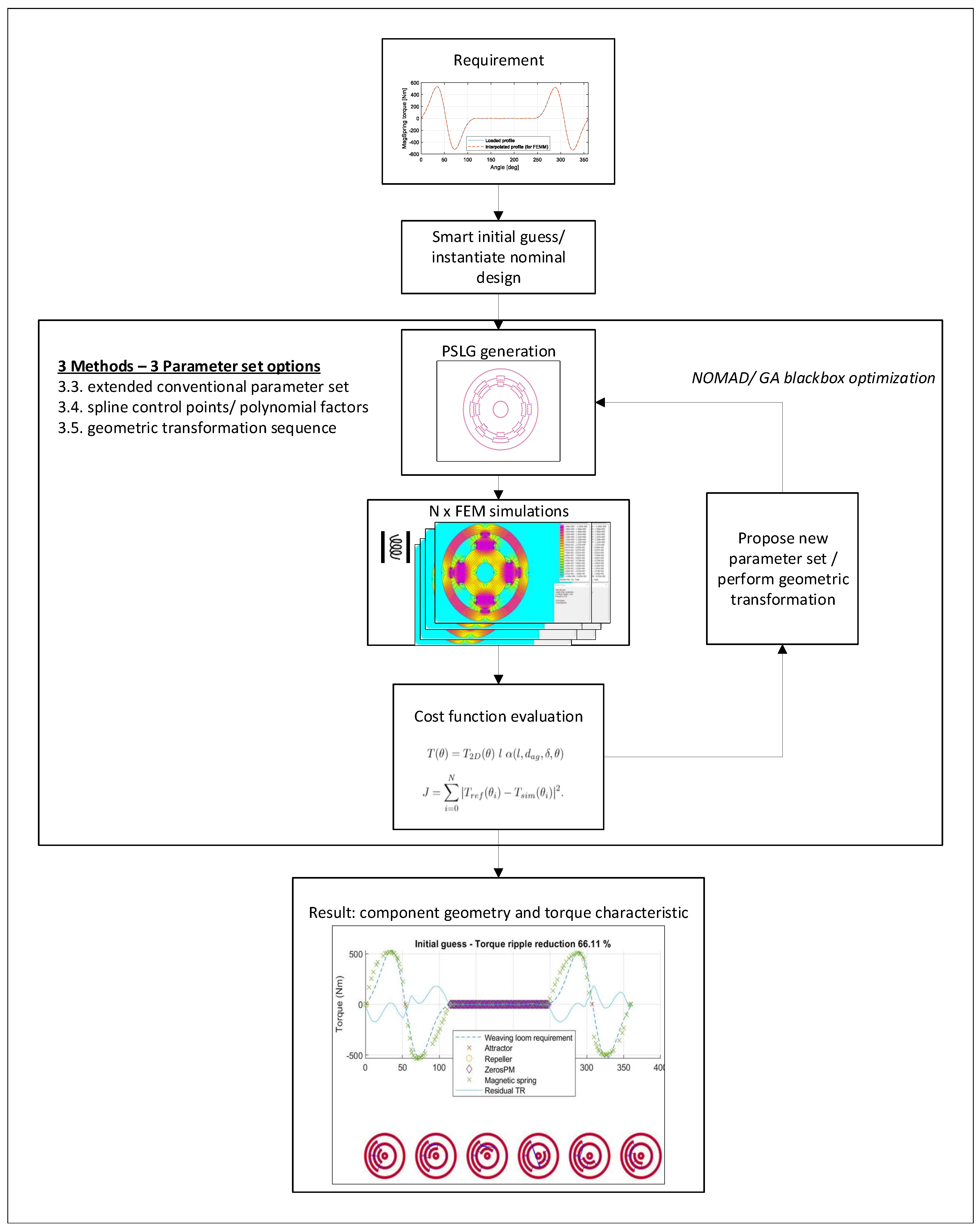
2.2. Shape Optimization
- Parametric optimization with extended set of optimization variable;
- Parametric shape optimization;
- Free shape optimization;
- Topology optimization.
2.2.1. Extended Parametric Optimization
2.2.2. Parametric Shape Optimization
2.2.3. Free Shape Optimization
2.2.4. Regarding Topology Optimization
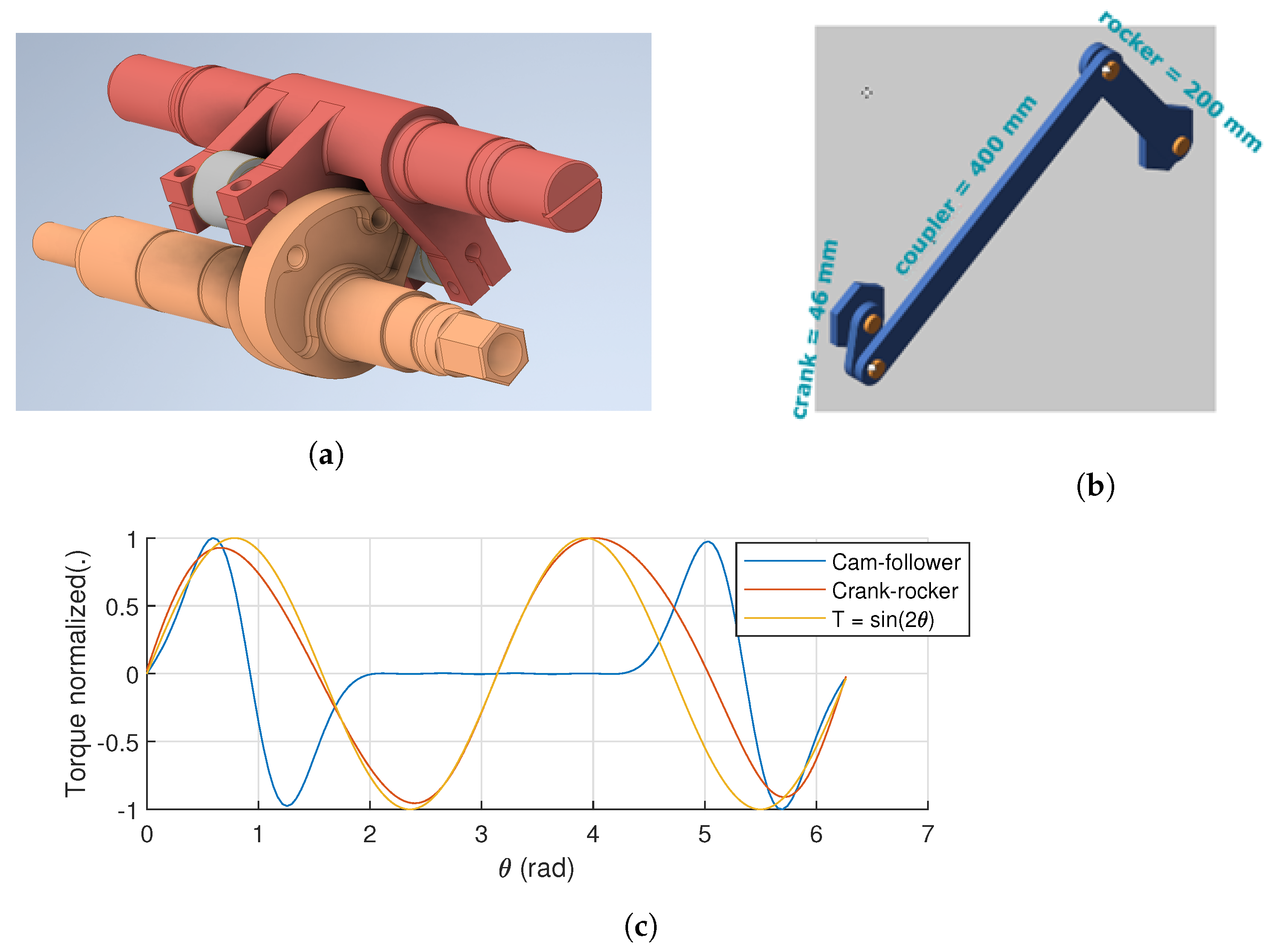
3. Results
- crank-rocker at constant speed;
- conjugate cam-follower at constant speed;
- rectangular torque characteristic (academic use case).
3.1. Extended Parametric Optimization
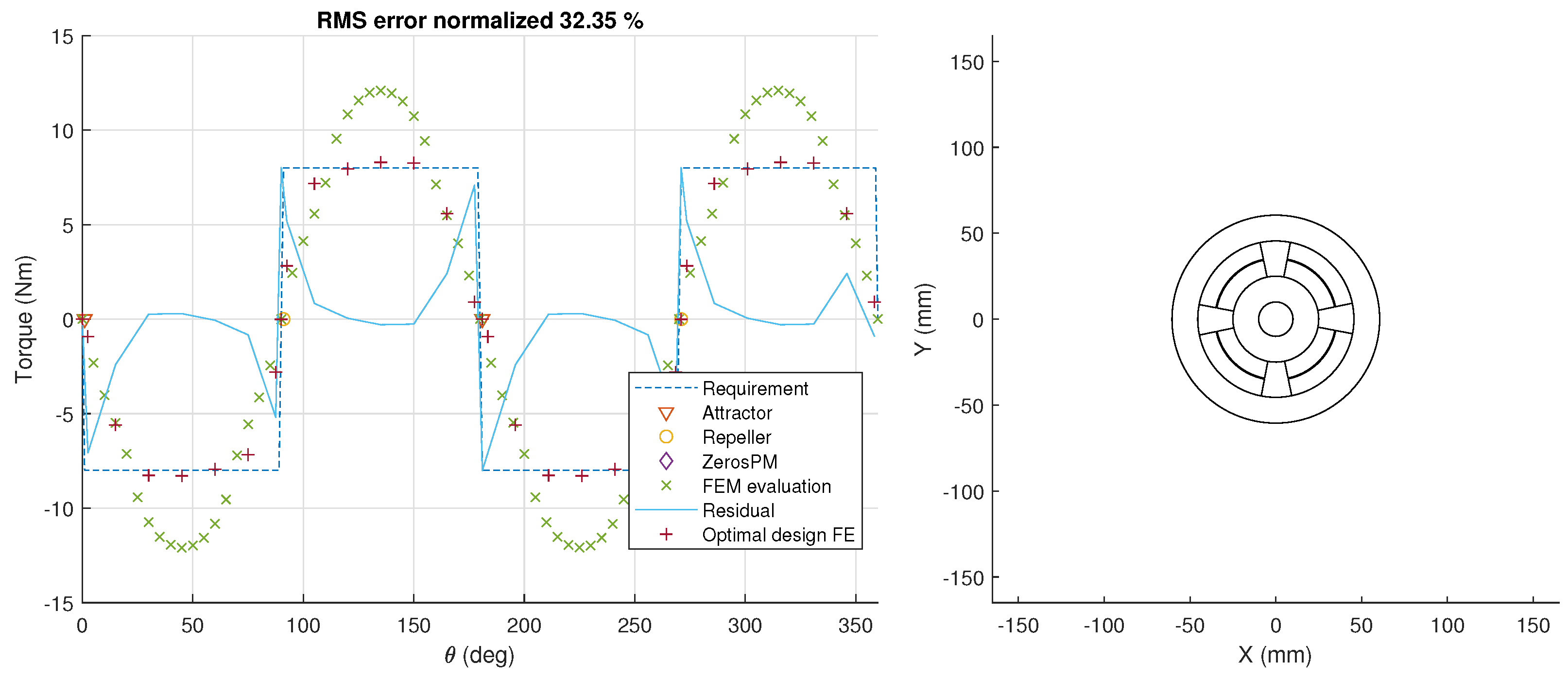
3.2. Parametric Shape Optimization
4. Discussion and Outlook
- extended parametric optimization—breaking design symmetry in conventional designs Section 2.2.1;
- domain boundary shaping using parametric spline approach Section 2.2.2.
- IPM back iron [12] harmonic field shaping for line start sync machines;
- magnet surface shaping in breadloaf magnets;
- generally for additive manufacturing (AM) for electrical motors.
5. Conclusions
- extended parametric optimization—breaking design symmetry in conventional designs Section 2.2.1;
- domain boundary shaping using parametric spline approach Section 2.2.2.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Mrak, B. Magnetic Springs for Improved Performance of Highly Dynamic Drivetrains. Ph.D. Thesis, KU Leuven, Leuven, Belgium, 2020. [Google Scholar]
- Mrak, B.; Lenaerts, B.; Driesen, W.; Desmet, W. Optimal Magnetic Spring for Compliant Actuation—Validated Torque Density Benchmark. Actuators 2019, 8, 18. [Google Scholar] [CrossRef] [Green Version]
- Mrak, B.; Adduci, R.; Weckx, S.; Driesen, W.; Desmet, W. Novel phase-bound magnetic vibration absorber for improved NVH performance of a wind turbine gearbox. In Proceedings of the ISMA 2018—International Conference on Noise and Vibration Engineering and USD 2018—International Conference on Uncertainty in Structural Dynamics, Leuven, Belgium, 17–19 September 2018; pp. 4833–4845. [Google Scholar]
- Meeker, D. FEMM-4.2–Finite Element Method Magnetics. Available online: https://www.femm.info/wiki/HomePage (accessed on 21 April 2019).
- Silber, S.; Koppelstätter, W.; Weidenholzer, G.; Segon, G.; Bramerdorfer, G. Reducing Development Time of Electric Machines with SyMSpace. In Proceedings of the 2018 8th International Electric Drives Production Conference (EDPC), Schweinfurt, Germany, 4–5 December 2018; pp. 1–5. [Google Scholar]
- Joris, G.; Edward, K. Symbolic Equation Extraction from SimScape. In Proceedings of the Benelux Meeting on Systems and Control, Soesterberg, The Netherlands, 27–29 March 2018. [Google Scholar]
- Le Digabel, S. NOMAD: Nonlinear optimization with the MADS algorithm. ACM Trans. Math. Softw. 2011, 37, 44. [Google Scholar] [CrossRef]
- Le, C.; Bruns, T.; Tortorelli, D. A gradient-based, parameter-free approach to shape optimization. Comput. Methods Appl. Mech. Eng. 2011, 200, 985–996. [Google Scholar] [CrossRef]
- Erin, K.; François, H.; Pierre, D.; Christophe, G. Combination of topology optimization and Lie derivative-based shape optimization for electro-mechanical design. Struct. Multidiscip. Optim. 2019, 59, 1723–1731. [Google Scholar] [CrossRef]
- Labbé, T. Topology Optimization Methods for the Design of Electromagnetic Actuators. Ph.D. Thesis, Université catholique de Louvain- Ecole Polytechnique de Louvain, Ottignies-Louvain-la-Neuve, Belgium, 2011. [Google Scholar]
- Gangl, P.; Amstutz, S. Toplogical derivative for nonlinear magnetostatic problem. Electron. Trans. Numer. Anal. 2019, 51, 169–218. [Google Scholar]
- Jan, B.; Ladislav, K.; Gerd, B.; Iveta, L.; Siegfried, S.; Ondrej, V. Topology Optimization of Rotor Bars Geometry and Arrangement for a Line-Start Permanent Magnet Synchronous Machine. IEEE Access 2021, 9, 115192–115204. [Google Scholar] [CrossRef]
- Mrak, B.; Willems, J.; Baake, J.; Ganseman, C. Co-Design of Novel Adaptive Magnetic Springs for Reliable Industrial Variable Stiffness Actuation. ASME/IEEE Trans. Mechatron. 2021; under review. [Google Scholar]
- MIMplus Technologies: How innovation and research is driving developments in NdFeB magnets and sinter-based AM. PIM Int. 2021, 15, 75–82.
- Hartwig, T.; Lopes, L.; Wendhausen, P.; Ünal, N. Metal Injection Molding (MIM) of NdFeB Magnets; EDP Sciences: Les Ulis, Essonne, France, July 2014. [Google Scholar] [CrossRef] [Green Version]
- Klaus, S.; Christian, H.; Iulian, T.; Spomenka, K.; Boris, S.; Daniel, K.; Michael, R.; Christian, L.; Martin, G.; Dieter, S. 3D printing of polymer-bonded anisotropic magnets in an external magnetic field and by a modified production process. Appl. Phys. Lett. 2020, 116, 092403. [Google Scholar]
- Khazdozian, H.A.; Manzano, J.S.; Gandha, K.; Slowing, I.I.; Nlebedim, I.C. Recycled Sm-Co bonded magnet filaments for 3D printing of magnets. AIP Adv. 2018, 8, 056722. [Google Scholar] [CrossRef] [Green Version]

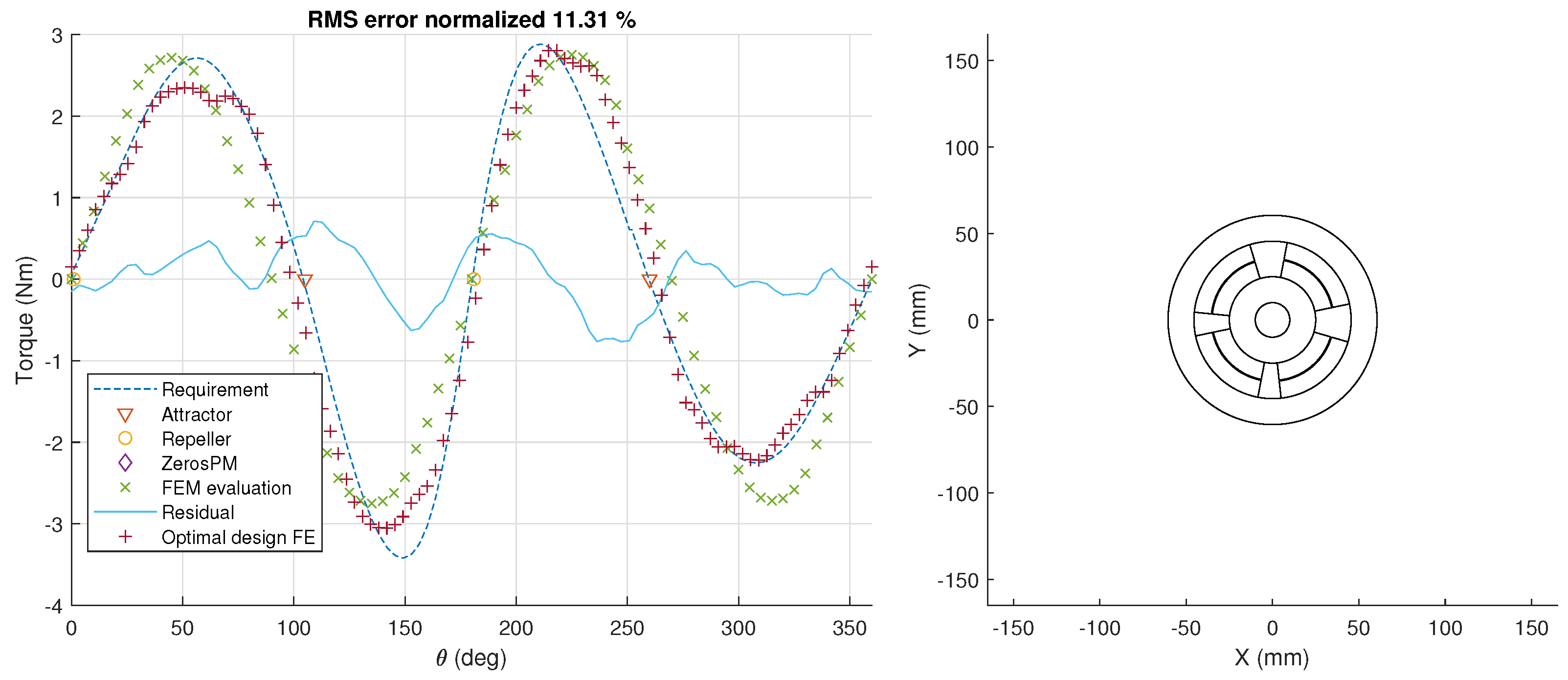
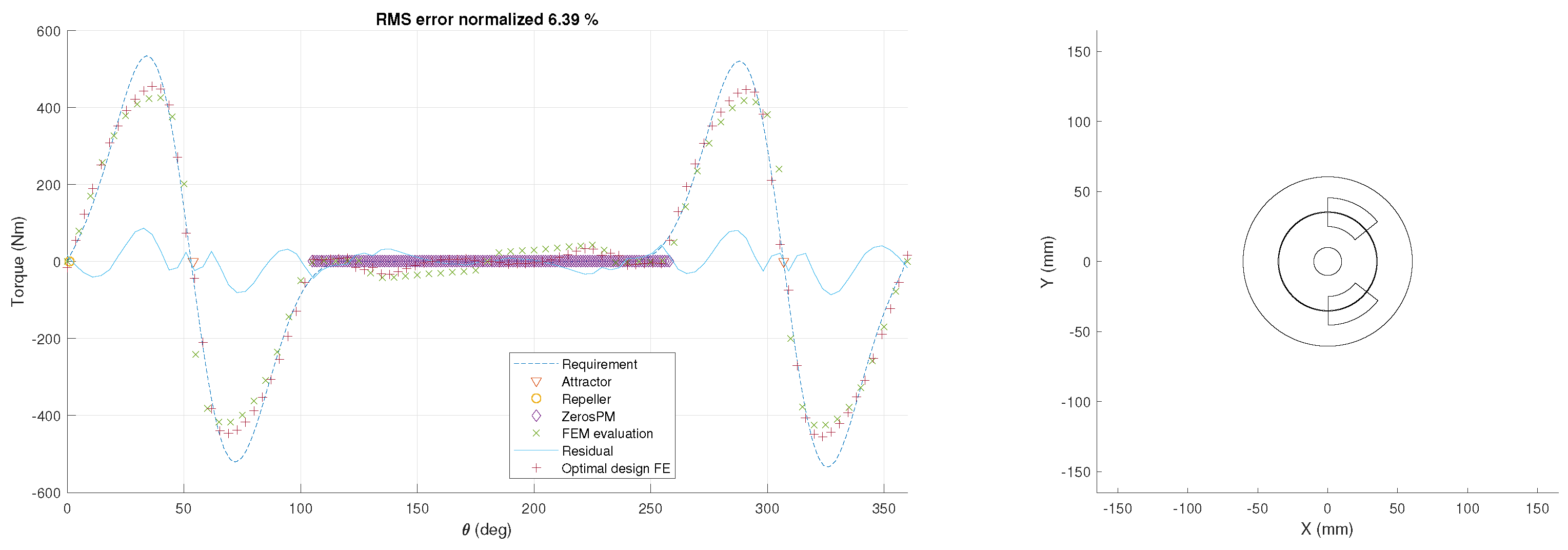
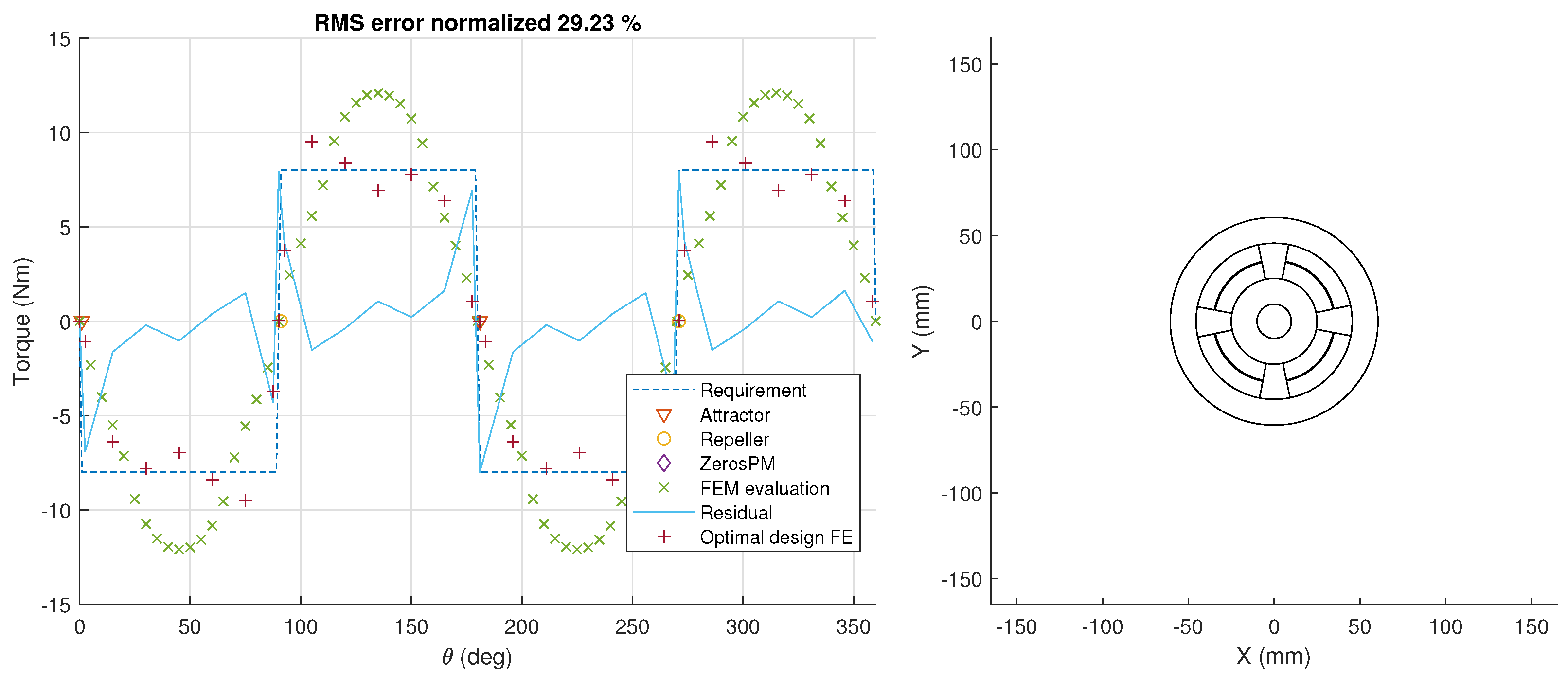



| No. of Magnets—N | No. of Magnets Rotor and Stator—Nr and Ns |
|---|---|
| pole pitch rotor | Nr × pole pitch rotor |
| pole pitch stator | Ns × pole pitch stator |
| magnet thickness rotor | Nr × magnet thickness rotor |
| magnet thickness stator | Ns × magnet thickness rotor |
| Norm. RMS Error | No. Variables | Evaluations Converged/Total | |
|---|---|---|---|
| Section 3.1 | 6.39 | 15 | 260/800 |
| Section 3.2 | 4.00 | 28 | 4000/8900 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mrak, B.; Wex, B.; Mitterhofer, H. Methodology for Shape Optimization of Magnetic Designs: Magnetic Spring Characteristic Tailored to Application Needs. Actuators 2022, 11, 37. https://doi.org/10.3390/act11020037
Mrak B, Wex B, Mitterhofer H. Methodology for Shape Optimization of Magnetic Designs: Magnetic Spring Characteristic Tailored to Application Needs. Actuators. 2022; 11(2):37. https://doi.org/10.3390/act11020037
Chicago/Turabian StyleMrak, Branimir, Bianca Wex, and Hubert Mitterhofer. 2022. "Methodology for Shape Optimization of Magnetic Designs: Magnetic Spring Characteristic Tailored to Application Needs" Actuators 11, no. 2: 37. https://doi.org/10.3390/act11020037
APA StyleMrak, B., Wex, B., & Mitterhofer, H. (2022). Methodology for Shape Optimization of Magnetic Designs: Magnetic Spring Characteristic Tailored to Application Needs. Actuators, 11(2), 37. https://doi.org/10.3390/act11020037






