Abstract
A novel nonlinear dynamic modeling approach is proposed for the T-shaped beam structures widely used in the field of aerospace. All of the geometrical nonlinearities including the terms in the deformation of the beams, the terms at the connections, and the free ends of beams are considered in the dynamic modeling process. The global mode method is employed to determine the natural frequencies and global mode shapes of the linearized system. The validity and accuracy of the derived model are verified by comparing the natural frequencies obtained with those calculated from FEM. Adopting the Galerkin truncation procedure, a set of reduced-order nonlinear ODEs is obtained for the structure. A study on the variation of dynamic responses taking the different numbers of global modes into account is performed to determine the number of modes taken in nonlinear vibration analysis. A comparison between the responses of the system with linear or nonlinear matching and boundary conditions is given to evaluate the importance of neglecting and reserving the nonlinear terms in matching and boundary conditions. It is shown that ignoring the nonlinear terms in both matching and boundary conditions may significantly alter the responses while developing the discretized governing ODEs of the structure.
1. Introduction
A specific structure composed of multiple beams is usually used as a component in large-scale space structures, especially in the design of flexible spacecraft [,,,]. With the advancement of aerospace technology and the diversification of aerospace missions, the use of flexibility and large-scale structures has gradually become an important trend in the development of spacecraft, which leads to geometric nonlinearity as a factor that has to be considered. Hence, establishing accurate nonlinear dynamic models of such systems is an important basis for nonlinear dynamical analysis and active vibration control. This is of important practical significance for predicting and understanding the dynamic behavior of such flexible structures under the effect of applied loadings.
The nonlinear dynamic behavior of a single beam has been widely studied in the literature [,,,,,,]. Compared with a single beam, multi-beam structures have more complex dynamic characteristics due to internal coupling and geometric and inertial nonlinearities. Haddow et al. [] established the equation of motion for the L-shaped beam structure with only quadratic nonlinearities considering the influence of the axial movement of the beam due to bending and obtained the approximate solutions of the obtained equation by using the multiple scales method. Nayfeh et al. [,,,,] conducted a comprehensive experimental and theoretical study on the nonlinear motions of the L-shaped beam structure, and the research showed that small excitation could produce chaotic motion under two-to-one internal resonance. Additionally, the theoretically deduced periodic, quasi-periodic and chaotic responses are in good agreement with experimental results. Hamed Samandari et al. [] obtained the nonlinear differential equations of motion for the L-shaped beam structure by using the Hamilton principle and Euler–Bernoulli beam theory, considering the large deformation of the structure. The differential quadrature method (DQM) is used to discretize the differential equations of motion in the space domain. The harmonic balance method is used to further transform the differential equations of motions into nonlinear algebraic equations for numerical solutions and numerical analysis of nonlinear responses. What is more, Kumar et al. [] conducted an investigation of nonlinear phenomena of dynamic responses of N-link manipulators under 3:1 internal resonance considering geometric nonlinearities.
Dynamic models of the multi-beam structures are continuous with infinite degrees of freedom. To obtain a reduced-order dynamic model, the modal analysis method can be used to discretize the continuous systems. Therefore, the accuracy of the calculation results depends highly on the quality of the mode shapes used in the dynamic modeling. For a multi-beam structure, due to the interaction between the components, the mode function of each beam is different from that of a single beam. Consequently, the dynamical model based on the global mode has the advantages of lower dimensions and high precision, which provides convenience for dynamical analysis. The global mode method (GMM) was proposed to establish the dynamic model of the micro-electric static comb [] and the composite flexible structure of a long-span cable-stayed bridge []. Then, the modal conversion and nonlinear dynamic response analysis were conducted. Wei et al. applied GMM to establish a spacecraft model with a deployable solar panel [], a multi-beam structure with nonlinear hinges [], and a nonlinear motion model of the L-shaped beam mass structure []. Based on these models, a series of inherent characteristic analysis and dynamic response studies were respectively performed. Considering the out-of-plane transverse deformation and torsional deformation of the beams, Cao et al. [] established a linear dynamic model of the T-shaped beams and studied the vibration suppression based on piezoelectric plates.
For multi-beam structures connected by joints, there are many studies on the effect of joints on the dynamic behavior of the system. Vakil et al. [] studied the effect on the inherent properties of the system due to changes in joint stiffness and determined the upper limit of joint stiffness, which can distinguish whether the joint of the manipulator is flexible or rigid. Meng et al. [] investigated the effect of joint stiffness on the vibration response of the system. The research indicated that appropriate joint stiffness can reduce the overall vibration of the structure due to the coupling relationship between flexible rods and flexible joints. Recently, Wei et al. [,,,] derived a reduced-order analytical model for multi-beam structures connected with hinges associated with a nonlinear rotational spring. Based on this model, they investigated the effect of the nonlinear stiffness and damping of the joints on the attitude and position of a spacecraft during maneuvering. Studies had shown that the nonlinear stiffness of the joint has a great influence on the nonlinear response of the system.
Based on previous preliminary research [], in this article, along with the idea of the GMM, a novel nonlinear dynamic modeling approach is proposed for the T-shaped beam. All of the geometrical nonlinearities including the terms in the deformation of the beams, the stress compatibility condition at the connections of beams, and the terms at the free ends are taken into account in the dynamic modeling process. The generalized Hamiltonian principle is employed to establish the nonlinear partial differential equations of motion for the T-shaped beam structure. Using the method proposed in [,,,], the natural frequency of the system and the corresponding global mode functions are worked out. Using the global mode functions and their orthogonality relations, an explicit set of reduced-order nonlinear ordinary differential equations of motion is obtained. Combined with specific examples, the precision and effectiveness of the model are verified by comparing the natural frequency and the global mode shape of the system. Through the dynamic equations given by the numerical examples, the dynamic responses of the system with different numbers of modes are studied. The importance of reserving or neglecting the nonlinear terms in matching and boundary conditions when deriving the discrete control equations is evaluated.
2. Nonlinear Dynamic Model in the Continuous Form
Consider the in-plane motion of a T-shaped beam structure that is fixed on a horizontal moving base, as shown in Figure 1. The T-shaped beam is composed of three lightweight inextensible beams, namely a horizontal beam and two vertical beams. For the sake of description, the horizontal beam is called Beam-1, the lower vertical beam is Beam-2, and the upper vertical beam is Beam-3. Coordinate frame o1x1y1 is a fixed inertial frame with the origin at the left end of the horizontal beam, and coordinate frames o2x2y2 and o3x3y3 are satellite inertial frames with the origins both at . and denote the length, the density, Young’s modulus, the inertia moment, the cross-sectional area, and the axial and lateral displacements of the i-th beam, respectively. Moreover, it is assumed that the shear deformation and warpage of all beams can be ignored and the beams are inextensible [,].
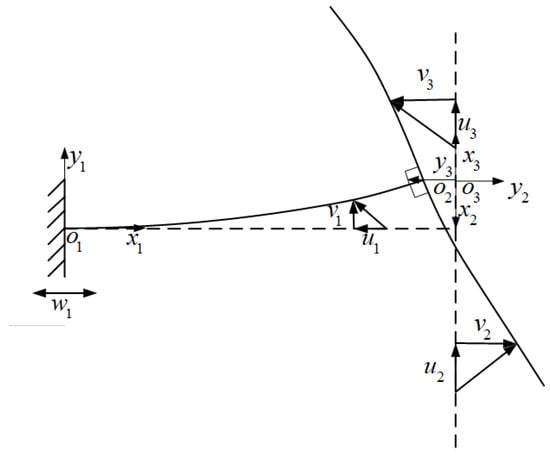
Figure 1.
Schematic of T-shaped beam structure.
The generalized Hamilton’s principle is expressed as
where T, V and W represent the kinetic energy, potential energy and work done by the non-conservative force of the system, respectively.
Take a micro-element on the beam, as shown in Figure 2, where the dashed and solid lines indicate the positions of the micro-element before and after deformation, respectively. The symbol θ (x, t) in Figure 2 represents the angular displacement of the micro element. The in-extensibility condition can be depicted by the schematic .
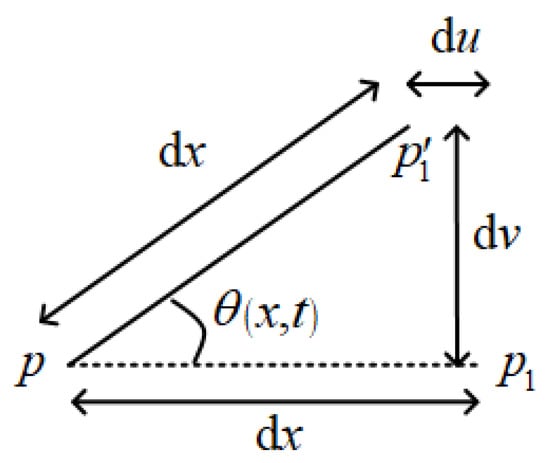
Figure 2.
Schematic of the geometry and the in-extensibility condition.
The relations between these displacements are determined by the geometry. We write []
where the superposed dot represents the partial derivative with respect to time, and (‘) represents the partial derivative with respect to displacement, i = 1, 2, 3. This notation is followed quite extensively from here on. Expanding Equations (2) and (3) yields
Substituting Equation (4) into Equation (5) yields
where is neglected in the subsequent analysis. The axial displacement of any point on the beams can be obtained by integrating Equation (6) from the origin to this point on the beams,
In this way, represents the axial displacement of the right end of the horizontal beam.
The curvature is expressed as
The potential energy of the T-shaped beam structure is given by
Substitute Equation (8) into Equation (9) to obtain
The kinetic energy of the T-shaped beam structure is given by
Substituting Equation (7) into Equation (11) yields
Considering the viscous damping in the structure, the virtual work done by the non-conservative forces is given by
Substituting Equations (10), (12), and (13) into Equation (1) and simplifying, we obtain the nonlinear vibration equations of the structure as
The boundary conditions of the T-shaped beam structure are expressed as
at
at
at
where Equations (18)–(21) have not been simplified in order to preserve the completeness of the nonlinearity of the boundary conditions.
At the connection interfaces of the beams, the displacement and rotation angle of the horizontal beam and the vertical beams must match, and the bending moments and forces must be balanced. The matching conditions at the junction are expressed as
where the Equations (24)–(26) are the matching equations of the bending moment, the vertical force and the horizontal force at the junction, respectively.
3. Natural Characteristics of the System
The natural frequencies and global mode functions of the system can be obtained by linearizing the nonlinear partial differential equations of motion for the structure. Ignoring the nonlinear terms and the damping terms in Equations (14)–(16) yields the linear partial differential equations of the transverse motion of the i-th beam
Similarly, ignoring the nonlinear terms in the boundary and matching conditions (17)–(26), we have
Assume that the displacements are separable in space and time,
where ω is the natural frequency of the system.
Substituting Equation (29) into the linear vibration Equation (27) of the beam yields
The general solution of Equation (30) can be written as
where .
The constants should be determined using the boundary and matching conditions given in Equation (28). Substituting expression (31) into Equation (28), the eigenvalue equation for the linearized system of the T-shaped beam is obtained, and its matrix form is expressed as follows
where , and entries of matrix are given in Appendix A.
The positive roots of the frequency equation , denoted in ascending order by , are the natural frequencies of the T-shaped beam structure. The eigenvector , where , corresponding to the natural frequency , can be obtained from Equation (32). Further, the r-th mode shapes for the T-shaped structure can be determined from Equation (31).
Referring to the derivation process in Reference [], the orthogonality conditions of the global mode with respect to mass and stiffness can be obtained, which are respectively expressed as
And
where and are positive constants and is the Kronecker delta.
4. Nonlinear Dynamic Model in the Discrete Form
The nonlinear dynamic model in the continuous form described by Equations (14)–(16) combined with the nonlinear boundary conditions (17)–(21) and the nonlinear matching conditions (22)–(26) can be simplified to a nonlinear dynamic model in the discrete form by using the global mode functions and their orthogonality relations presented in Section 3. The transverse displacements can be expressed in the following form
where and are the global mode functions for the T-shaped beam structure obtained from Equation (31), respectively, and is the generalized coordinate for the whole system.
Substituting Equation (35) into Equations (14)–(16) yields
Multiply both sides of Equations (36)–(38) by , and , respectively, integrating them along the respective beam lengths. Then, the ordinary differential equations of motion for the T-shaped beam structure can be obtained by adding all resulting equations and simplifying by using matching and boundary conditions (17)–(26) and orthogonality relations (33), (34), namely,
where are constants that can be determined by the global mode functions and damping coefficient, and given in Appendix B are constants that can be determined by the global mode functions. It is worth noting that the underlined terms in the expressions of those constants are from the non-linear terms in matching and boundary conditions. Therefore, the ordinary differential equations of motion under linear matching and boundary conditions, which are called the “incomplete nonlinear dynamic model (INDM)” hereafter, can be obtained by ignoring the underlined terms. Consequently, the ordinary differential Equation (39) with all of the geometrical and inertial nonlinear terms is called the “complete nonlinear dynamic model (CNDM)”.
5. Model Validation for the Linearized System
In this section, a comparison of the natural frequencies and the global mode functions obtained theoretically with the results from commercial software ANSYS is performed by using a typical example to validate the approach proposed in this paper.
Now, a simple example of the T-shaped beam structure is provided. Assume that the material for all beams is steel with density ρ = 7850 kg/m3, Young’s modulus E = 200 GPa, damping ratio c = 0.02, and Poisson’s ratio υ = 0.31. The cross sections of all beams are b = 0.012 m, h = 0.002 m. The lengths of the beams are l1 = 0.3 m, l2 = 0.3 m, l3 = 0.2 m, respectively.
Use the approach proposed to obtain the natural characteristics of the T-shaped beam structure, including natural frequencies and global mode shapes. Table 1 shows the natural frequencies of the T-shaped beam structure, taking the finite element results from ANSYS as a reference. The maximum relative error between the natural frequencies obtained by the current method and those from FEM is 0.1192%. The results show that the approach proposed in this paper is effective and the frequency obtained has higher accuracy because there is no approximation and neglection in the mathematical derivation of this method. Define the relative error as
where and are the natural frequencies calculated by the global modal method and FEM, respectively.

Table 1.
First 8 order frequency of T-shaped beam structure (rad/s).
Figure 3 shows the first 8 modes obtained here and those from FEM, where the mode pictures obtained by FEM are drawn in the ORIGIN software using the data of ANSYS software. It can be seen that the calculation results of the two methods are in good agreement. The reduced-order nonlinear differential equations of motion of the T-shaped beam structure obtained by using the global modes are accurate.
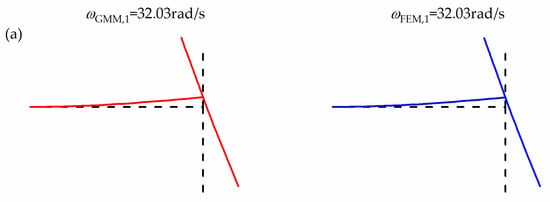
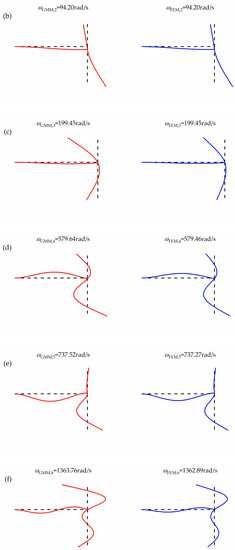

Figure 3.
First 8 order modal shapes: GMM (Left column) and FEM (Right column).
6. Nonlinear Dynamical Responses and Discussions
The T-shaped beam structure is assumed to be fixed on a horizontal moving base, as shown in Figure 1. The displacement of the moving support is assumed as
where is a constant and denotes the amplitude of the displacement, and is the frequency of the moving base in rad/s.
In order to determine the number of modes that should be taken in vibration analysis, the dynamical responses of the linear dynamic model (without all of the nonlinear terms), the INDM, and the CNDM with n modes under the sweeping frequency are given in Figure 4 and Figure 5a,b, respectively, where the excitation amplitude w0 = 0.0008 m, and the amplitudes of the responses are taken from the transverse displacements of the free end of Beam 2. It can be seen from Figure 4 that in the linear dynamic model, the resonance peaks of the system with the first n modes all appear at 32.03 rad/s; that is approximately equal to the first natural frequency, which illustrates that low-order frequencies play a leading role in vibration. There is a sudden increase in response amplitude of the T-shaped beam when the number of modes increases from 3 to 4; this may be because the coupling effect between the 4th mode and the first 3 modes is strong. Moreover, whether the system is a linear dynamic model, INDM or CNDM, the variation trend of the response value of the system with the first n modes is consistent.
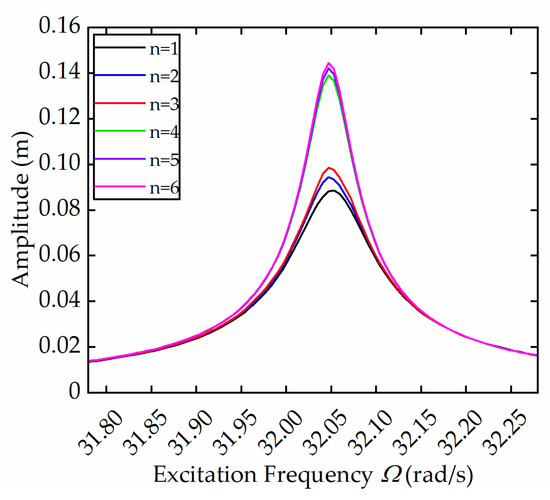
Figure 4.
Frequency responses of the linear dynamic model with the first n modes.

Figure 5.
Frequency response curves of the system with the first n modes under different conditions: (a) INDM; (b) CNDM.
It can be observed from Figure 4 and Figure 5 that 4 or more than 4 modes should be truncated to guarantee the solution accuracy of the system in the primary resonance region. This implies that the first 4 modes should be taken for simulation in the calculations of the nonlinear vibration responses.
The steady-state response time histories of Beam-2 end point under the excitation amplitude w0 = 0.01 m and frequency Ω = 32.34 rad/s are calculated using the proposed method and the FEM, respectively, as shown in Figure 6. The maximum relative error between the displacement amplitudes from the proposed and finite element methods, defined by , is 9.01%. Similarly, the maximum relative error between the velocity amplitudes from the proposed and finite element methods is 9.43%. It shows that the numerical results obtained by our method are matched very well with those from ANSYS software, which further confirms the effectiveness of the proposed method.
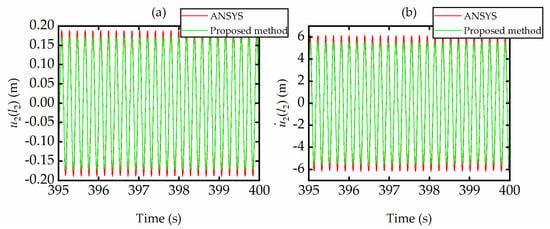
Figure 6.
The steady−state response time histories of Beam−2 end point: (a) displacement; (b) velocity.
The force response curves of the INDM with the first n modes for the excitation frequency Ω = 32.15 rad/s are shown in Figure 7a, and those of the CNDM with the first n modes for the excitation frequency Ω = 32.66 rad/s are shown in Figure 7b. The same jumping phenomenon can be seen in the figure, and as the excitation amplitude increases, the difference in the dynamic response of both systems with the first n modes becomes larger and larger. When the vibration amplitude of the system is relatively large, more modes need to be adopted to meet the accuracy requirements. All of these clearly show that the influence of higher-order modes on the system dynamic response is closely related to the excitation amplitude of the system.
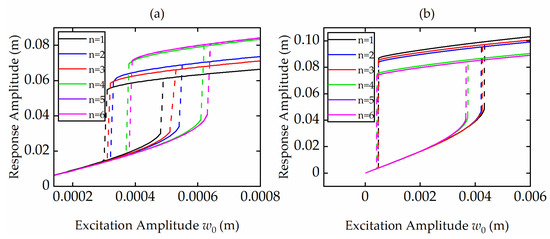
Figure 7.
Amplitude response curves of the INDM (a) and the CNDM (b) with the first n modes.
The influences of excitation amplitudes on the responses of the nonlinear system for the INDM and the CNDM are shown in Figure 8a,b respectively. The curves show that the responses are positively correlated with the excitation amplitude in both systems. It can also be seen that there is a jump phenomenon in the nonlinear response. The greater the excitation, the more obvious the jump, and the higher the corresponding jump frequency.

Figure 8.
Frequency responses of the INDM (a) and the CNDM (b) with different excitation amplitudes.
To evaluate the importance of reserving and ignoring nonlinear matching and boundary conditions when deriving discrete nonlinear governing equations, the dynamic responses of the nonlinear systems INDM and CNDM at the excitation amplitude w0 = 0.0008 m, w0 = 0.0006 m, w0 = 0.0004 m and w0 = 0.0002 m are shown in Figure 9a–d, respectively. The curves show that there is a jumping phenomenon in both systems. As the excitation amplitude increases, the response gap between the two systems gradually increases, the jumping frequency of the system CNDM is much higher, and the hysteresis zone is wider in comparison with that of the system INDM. Therefore, when developing the discretized governing equations of temporal modes under a certain degree of excitation amplitude, we should not only consider the nonlinear terms in the vibration differential equations of beams but also the nonlinear terms in the matching and boundary conditions. Ignoring the nonlinear terms in the matching and boundary conditions may lead to relatively large errors in the dynamic responses of the system.
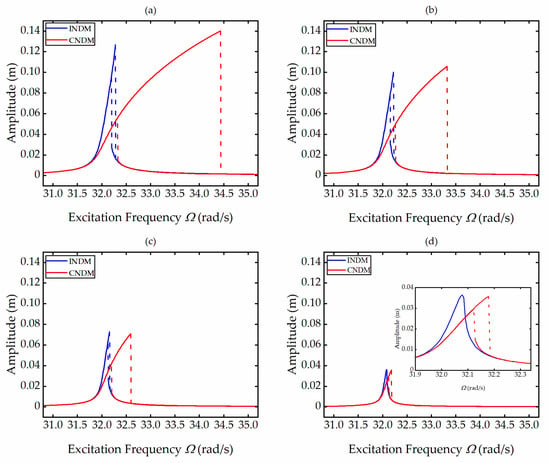
Figure 9.
Frequency responses of the INDM and the CNDM at different excitation amplitudes: (a) w0 = 0.0008 m; (b) w0 = 0.0006 m; (c) w0 = 0.0004 m; (d) w0 = 0.0002 m.
Figure 10, Figure 11 and Figure 12 respectively show the steady-state responses of a system containing second-order and third-order nonlinear terms, second-order nonlinear terms only, and third-order nonlinear terms only, when the excitation frequency is near the first-order natural frequency, where the response is the transverse vibration of the free end of Beam 2, including time history of steady state, phase portrait, Poincare map, and spectrum response. The Poincare map is a point composed of integer multiples of the excitation period in all three pictures, which means that the vibration of the T-shaped beam structure reaches a periodic stable state. In the spectrogram (d) of both Figure 10 and Figure 11, peaks appear at zero, one, two, and three times the fundamental frequency. In the spectrogram (d) of Figure 12, peaks only appear at one and three times the fundamental frequency. It can be inferred that the peaks at three times the fundamental frequency are caused by the second and third nonlinear terms, while the peaks at zero and two times the fundamental frequency are mainly caused by the second-order nonlinear terms in the system.
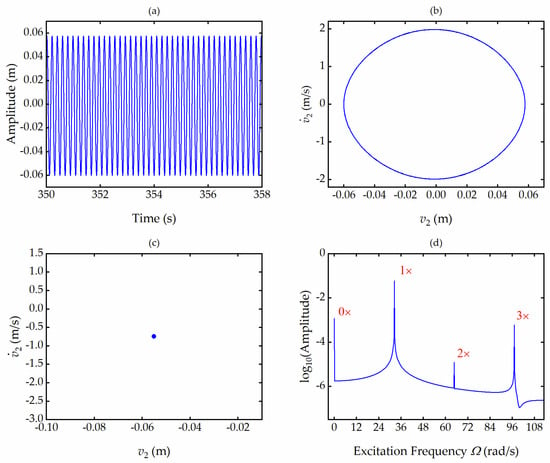
Figure 10.
Response of a system with second− and third−order nonlinear terms for the case of w0 = 0.0008 m, Ω = 32.34 rad/s: (a) Time history of steady state. (b) Phase portrait. (c) Poincare map. (d) Spectrum response.
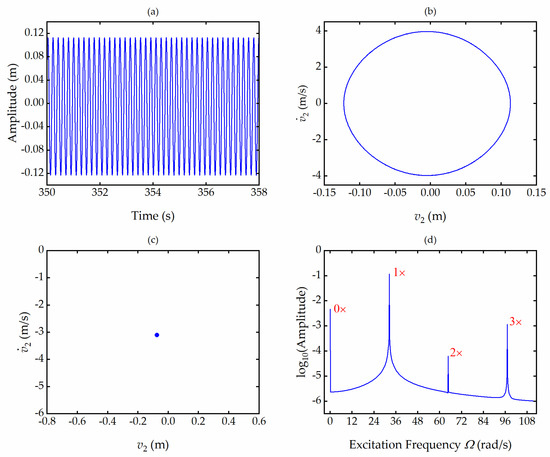
Figure 11.
Response of a system with only second−order nonlinear terms for the case of w0 = 0.0008 m, Ω = 32.34 rad/s: (a) Time history of steady state. (b) Phase portrait. (c) Poincare map. (d) Spectrum response.
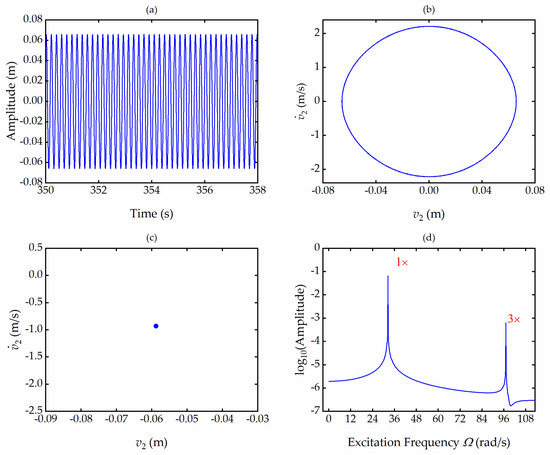
Figure 12.
Response of a system with only third−order nonlinear terms for the case of w0 = 0.0008 m, Ω = 32.34 rad/s: (a) Time history of steady state. (b) Phase portrait. (c) Poincare map. (d) Spectrum response.
7. Conclusions
A novel nonlinear dynamic modeling approach has been presented for the T-shaped beam, in which all of the geometrical nonlinearities including the terms in the deformation of the beams, in the stress compatibility condition at the connections of beams, and the terms at the free ends are taken into account in the dynamic modeling process. The nonlinear ordinary differential equations of motion describing the dynamic behaviors of the T-shaped beam structure have been established, and a series of dynamic response analyses have been performed. Considering the geometric nonlinearity of the beam, and assuming that the beam is not extensible, the partial differential equations of motion of the T-shaped beam structure are obtained by using the generalized Hamiltonian principle, along with their nonlinear matching and boundary conditions. The global mode method was employed to obtain the natural frequency of the system and the corresponding global mode functions. The validity of the dynamic model obtained was verified by comparing the natural frequencies obtained by the proposed approach with the calculation results of FEM. The Galerkin truncation procedure was employed to obtain the nonlinear ordinary differential equations of motion with a lower degree of freedom. To study the effect of the nonlinear terms in the boundary and matching conditions on the dynamical responses, the numerical solutions for the CNDM and the INDM were obtained, respectively, and a comparison of the results was given. Conclusions drawn from the dynamic modeling procedure and discussions on the nonlinear vibration responses are as follows:
- The dynamical responses are dominated by the low-order modes of the system. The numerical example shows that the first 4 modes should be taken for simulation in the calculations of the nonlinear vibration responses. In order to improve the calculation efficiency, fewer modes should be selected for calculation. Moreover, the responses of the nonlinear dynamic systems have a strong dependence on the excitation amplitude.
- Ignoring the nonlinear terms in the matching and boundary conditions may reduce the accuracy of the system. In CNDM, nonlinearity is continuous along the structure; owing to the nonlinear terms of the vibration equations, boundary and matching conditions are all reserved. Contrarily, there are breakpoints of nonlinearity at the junction and boundaries in INDM, where the nonlinear terms of the boundary and matching conditions are neglected, which may lead to unacceptable errors of the dynamic responses. Therefore, when studying the nonlinear dynamic response of a multi-beam structure, the nonlinear terms in the boundary and matching conditions should be reserved and are indeed worthy of our attention.
Author Contributions
Conceptualization, S.C. and Y.L.; methodology, S.C. and D.C.; validation, G.H. and B.F.; data curation, G.H.; writing—original draft preparation, S.C.; writing—review and editing, J.W.; funding acquisition, D.C. and Y.L. All authors have read and agreed to the published version of the manuscript.
Funding
This research was funded by the National Key Technology R&D Program of China, grant number 2020YFB1506702-03, the Civil Space Technology Advance Research Project of the Administration of Science, Technology and Industry, grant number D020201, and the National Natural Science Foundation of China, grant number 11732005.
Data Availability Statement
Not applicable.
Acknowledgments
The authors gratefully acknowledge the support of DQ research group, Institute of Aerospace Vehicle Dynamics and Control, Harbin Institute of Technology.
Conflicts of Interest
The authors declare no conflict of interest.
Appendix A
Entries of the matrix in Equation (32) are
Appendix B
The constants in Equation (39) are
References
- Vakil, M.; Fotouhi, R.; Nikiforuk, P.N. A new method for dynamic modeling of flexible-link flexible-joint manipulators. J. Vib. Acoust. 2012, 134. [Google Scholar] [CrossRef]
- Hablani, H.B. Hinges-free and hinges-locked modes of a deformable multibody space station-a continuum analysis. J. Guid. Control Dyn. 1990, 13, 286–296. [Google Scholar] [CrossRef]
- Wang, J.; Li, D.; Jiang, J. Integrated control of thermally induced vibration and quasi-static deformation of space truss. J. Dyn. Syst. 2016, 138. [Google Scholar] [CrossRef]
- Cao, Y.; Cao, D.; Liu, L.; Huang, W. Dynamical modeling and attitude analysis for the spacecraft with lateral solar arrays. Appl. Math. Model. 2018, 64, 489–509. [Google Scholar] [CrossRef]
- Abou-Rayan, A.M.; Nayfeh, A.H.; Mook, D.T.; Nayfeh, M.A. Nonlinear response of a parametrically excited buckled beam. Nonlinear Dyn. 1993, 4, 499–525. [Google Scholar] [CrossRef]
- Ashworth, R.P.; Barr, A.D.S. The resonances of structures with quadratic inertial non-linearity under direct and parametric harmonic excitation. J. Sound Vib. 1987, 118, 47–68. [Google Scholar] [CrossRef]
- Crespo da Silva, M.R.M.; Glynn, C.C. Nonlinear flexural-flexural-torsional dynamics of inextensional beams. I. Equations of motion. J. Struct. Mech. 1978, 6, 437–448. [Google Scholar] [CrossRef]
- Crespo da Silva, M.R.M.; Glynn, C.C. Nonlinear flexural-flexural-torsional dynamics of inextensional beams. II. Forced motions. J. Struct. Mech. 1978, 6, 449–461. [Google Scholar] [CrossRef]
- Crespo da Silva, M.R.M.; Zaretzky, C.L. Non-linear modal coupling in planar and non-planar responses of inextensional beams. Int. J. Nonlinear Mech. 1990, 25, 227–239. [Google Scholar] [CrossRef]
- Crespo da Silva, M.R.M.; Zaretzky, C.L. Nonlinear flexural-flexural-torsional interactions in beams including the effect of torsional dynamics. I: Primary resonance. Nonlinear Dyn. 1994, 5, 3–23. [Google Scholar] [CrossRef]
- Zaretzky, C.L.; Crespo da Silva, M.R.M. Nonlinear flexural-flexural-torsional interactions in beams including the effect of torsional dynamics. II: Combination resonance. Nonlinear Dyn. 1994, 5, 161–180. [Google Scholar] [CrossRef]
- Haddow, A.G.; Barr, A.D.S.; Mook, D.T. Theoretical and experimental study of modal interaction in a two-degree-of-freedom structure. J. Sound Vib. 1984, 97, 451–473. [Google Scholar] [CrossRef]
- Balachandran, B.; Nayfeh, A.H. Nonlinear motions of beam-mass structure. Nonlinear Dyn. 1990, 1, 39–61. [Google Scholar] [CrossRef]
- Balachandran, B.; Nayfeh, A.H. Observations of modal interactions in resonantly forced beam-mass structures. Nonlinear Dyn. 1991, 2, 77–117. [Google Scholar] [CrossRef]
- Nayfeh, A.H.; Balachandran, B. Experimental investigation of resonantly forced oscillations of a two-degree-of-freedom structure. Int. J. Nonlinear Mech. 1990, 25, 199–209. [Google Scholar] [CrossRef]
- Nayfeh, A.H.; Balachandran, B.; Colbert, M.A.; Nayfeh, M.A. An Experimental Investigation of Complicated Responses of a Two-Degree-of-Freedom Structure. J. Appl. Mech. 1989, 56, 960–967. [Google Scholar] [CrossRef]
- Nayfeh, A.H.; Zavodney, L.D. Experimental Observation of Amplitude- and Phase-Modulated Responses of Two Internally Coupled Oscillators to a Harmonic Excitation. J. Appl. Mech. 1988, 55, 706. [Google Scholar] [CrossRef]
- Cigeroglu, E.; Samandari, H. Nonlinear Vibrations of a Flexible L-shaped Beam Using Differential Quadrature Method. In Name of the Society for Experimental Mechanics Series; Springer: Charm, Switzerland, 2016. [Google Scholar]
- Kumar, P.; Pratiher, B. Modal characterization with nonlinear behaviors of a two-link flexible manipulator. Arch. App. Mech. 2019, 89, 1201–1220. [Google Scholar] [CrossRef]
- Song, M.; Cao, D.; Zhu, W. Dynamic analysis of a micro-resonator driven by electrostatic combs. In Proceedings of the International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Washington, DC, USA, 12 June 2012. [Google Scholar]
- Cao, D.; Song, M.; Zhu, W.; Tucker, R.W.; Wang, C.H.-T. Modeling and analysis of the in-plane vibration of a complex cable-stayed bridge. J. Sound Vib. 2012, 331, 5685–5714. [Google Scholar] [CrossRef]
- Wei, J.; Cao, D.; Wang, L.; Huang, W. Dynamic modeling and simulation for flexible spacecraft with flexible jointed solar panels. Int. J. Mech. Sci. 2017, 130, 558–570. [Google Scholar] [CrossRef]
- Wei, J.; Cao, D.; Huang, H.; Wang, L.; Huang, W. Dynamics of a multi-beam structure connected with nonlinear joints: Modelling and simulation. Arch. App. Mech. 2018, 88, 1059–1074. [Google Scholar] [CrossRef]
- Wei, J.; Cao, D.; Yang, Y.; Huang, W. Nonlinear Dynamical Modeling and Vibration Responses of an L-Shaped Beam-Mass Structure. J. Appl. Nonlinear Dyn. 2017, 1, 91–104. [Google Scholar] [CrossRef]
- Cao, Y.; Cao, D.; Huang, W. Dynamic modeling and vibration control for a T-shaped bending and torsion structure. Int. J. Mech. Sci. 2019, 157, 773–786. [Google Scholar] [CrossRef]
- Vakil, M.; Fotouhi, R.; Nikiforuk, P.N.; Heidari, F. A study of the free vibration of flexible-link flexible-joint manipulators. P. I. Mech. Eng. C-J MEC 2011, 225, 1361–1371. [Google Scholar] [CrossRef]
- Meng, D.; She, Y.; Xu, W.; Lu, W.; Liang, B. Dynamic modeling and vibration characteristics analysis of flexible-link and flexible-joint space manipulator. Multibody Syst. Dyn. 2018, 43, 321–347. [Google Scholar] [CrossRef]
- Wei, J.; Cao, D.; Huang, H. Nonlinear vibration phenomenon of maneuvering spacecraft with flexible jointed appendages. Nonlinear Dyn. 2018, 94, 2863–2877. [Google Scholar] [CrossRef]
- Wei, J.; Yu, T.; Jin, D.; Liu, M.; Tian, Y.; Cao, D. Three-to-one internal resonance in a two-beam structure connected with nonlinear joints. Arch. App. Mech. 2021, 91, 3835–3850. [Google Scholar] [CrossRef]
- Wei, J.; Yu, T.; Jin, D.; Liu, M.; Cao, D.; Wang, J. Nonlinear Dynamic Modeling and Analysis of an L-Shaped Multi-Beam Jointed Structure with Tip Mass. Materials 2021, 14, 7279. [Google Scholar] [CrossRef] [PubMed]
- Chen, S.; Cao, D.; Li, Y.; Chen, Z. Nonlinear Dynamical Modeling and Vibration Responses of A T-Shaped Beam Structure. In Proceedings of the 5th International Conference on Mechanical, System and Control Engineer, Kazan, Russia, 28 July 2021. [Google Scholar]
- Meirovitch, L. Fundamentals of Vibrations; Waveland Press: Long Grove, IL, USA, 2010; pp. 30–34. [Google Scholar]
- Nayfeh, A.H.; Pai, P.F. Linear and Nonlinear Structural Mechanics; John Wiley & Sons: Hoboken, NJ, USA, 2008; pp. 176–180. [Google Scholar]
- Zavodney, L.D.; Nayfeh, A.H. The non-linear response of a slender beam carrying a lumped mass to a principal parametric excitation: Theory and experiment. Int. J. Nonlin. Mech. 1989, 24, 105–125. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).