Refined Modeling Method and Analysis of an Electromagnetic Direct-Drive Hydrostatic Actuation System
Abstract
:1. Introduction
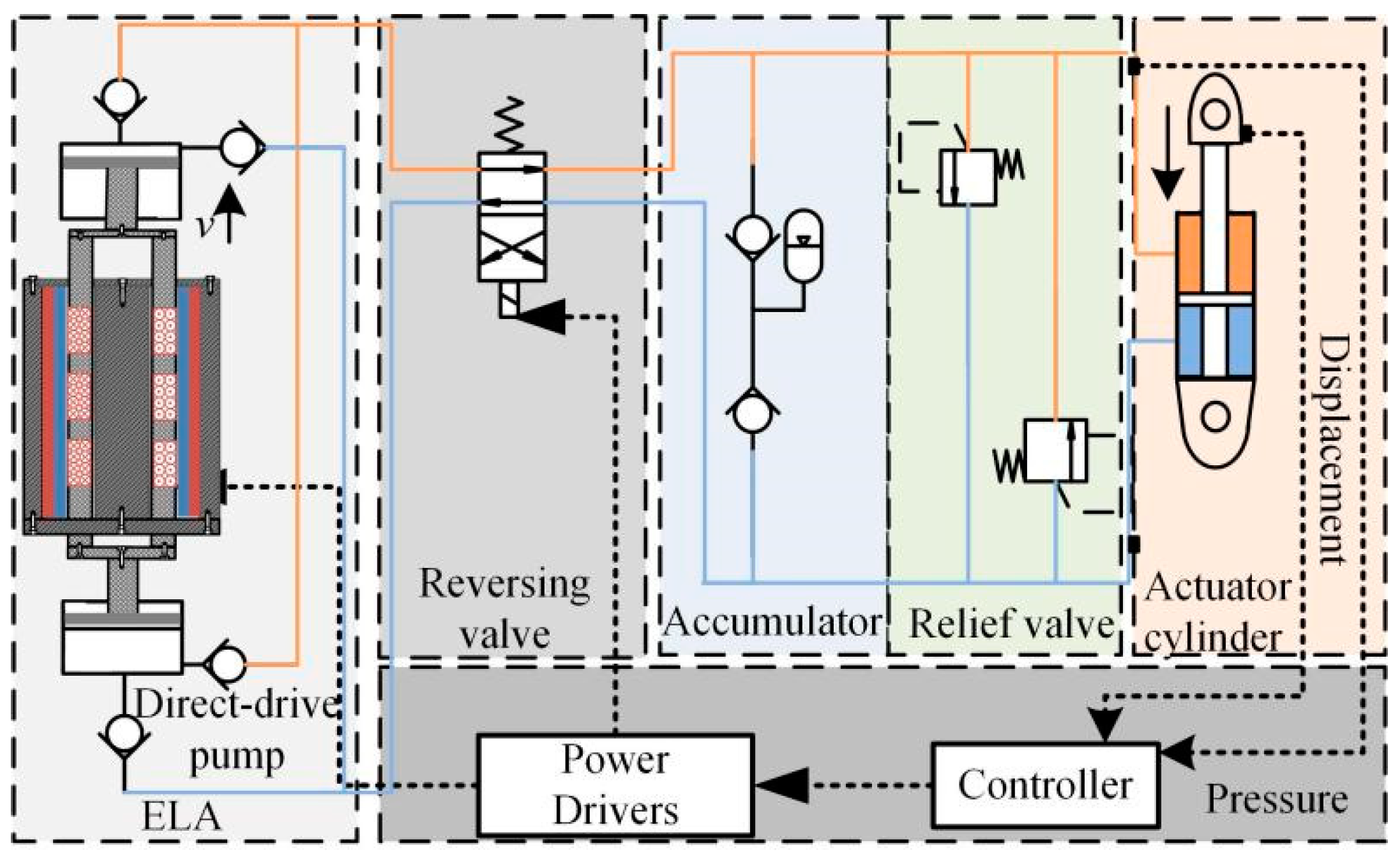
2. Working Principle of EDHAS
3. Multiphysics Coupling Modeling
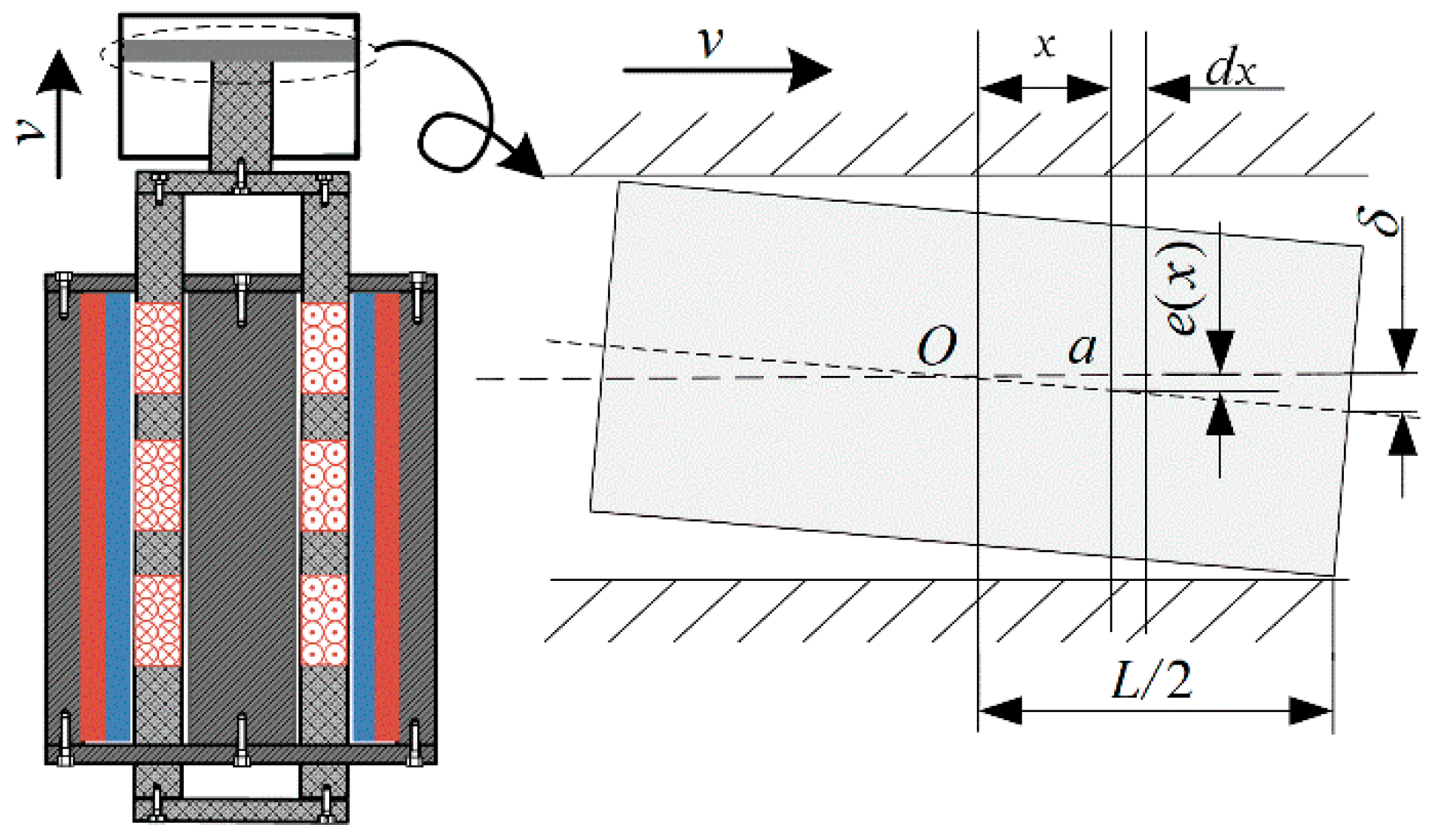
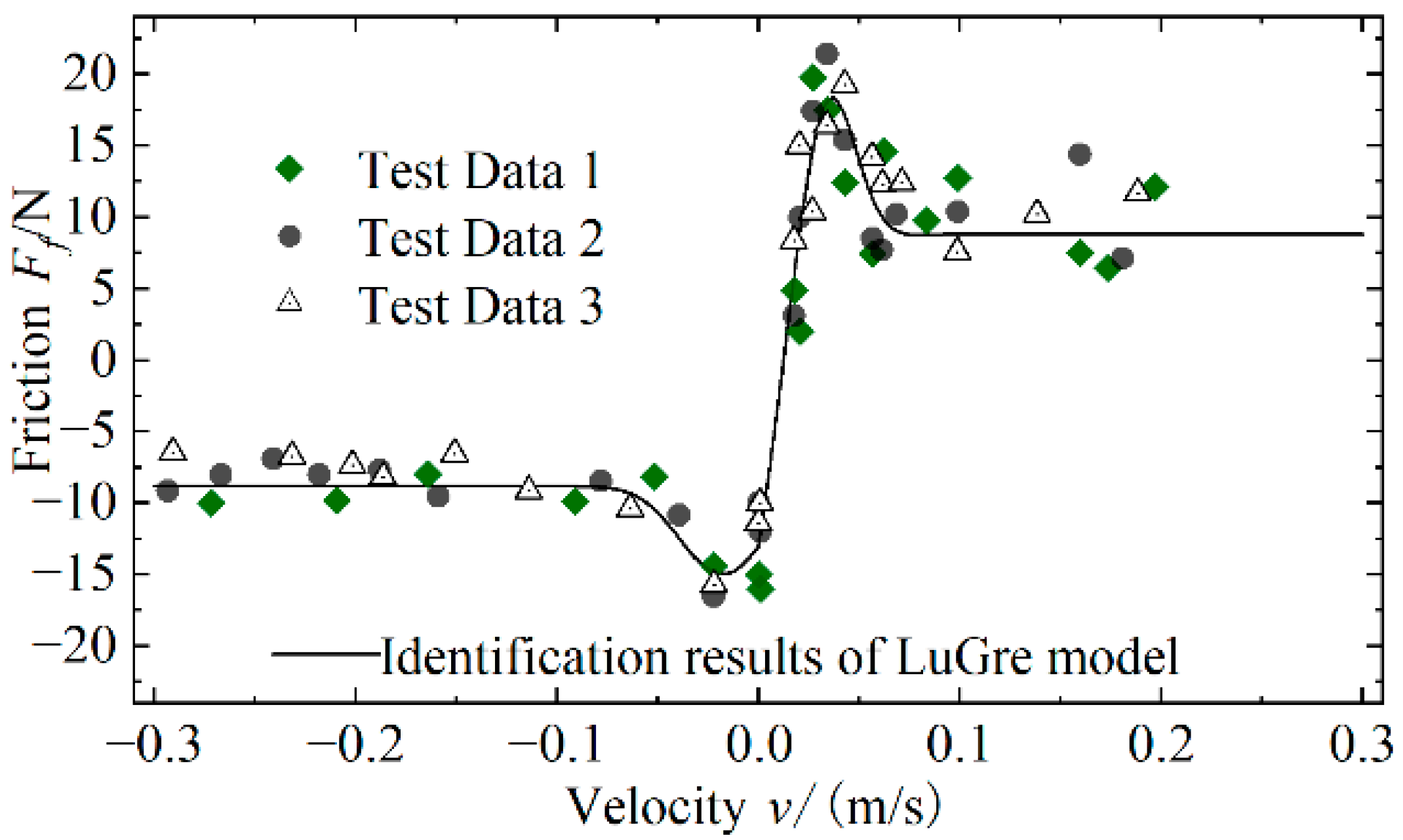
3.1. Electromagnetic Linear Actuator Modeling
3.2. Modeling of Electromagnetic Direct-Drive Pump
3.3. Modeling of Actuator Cylinder
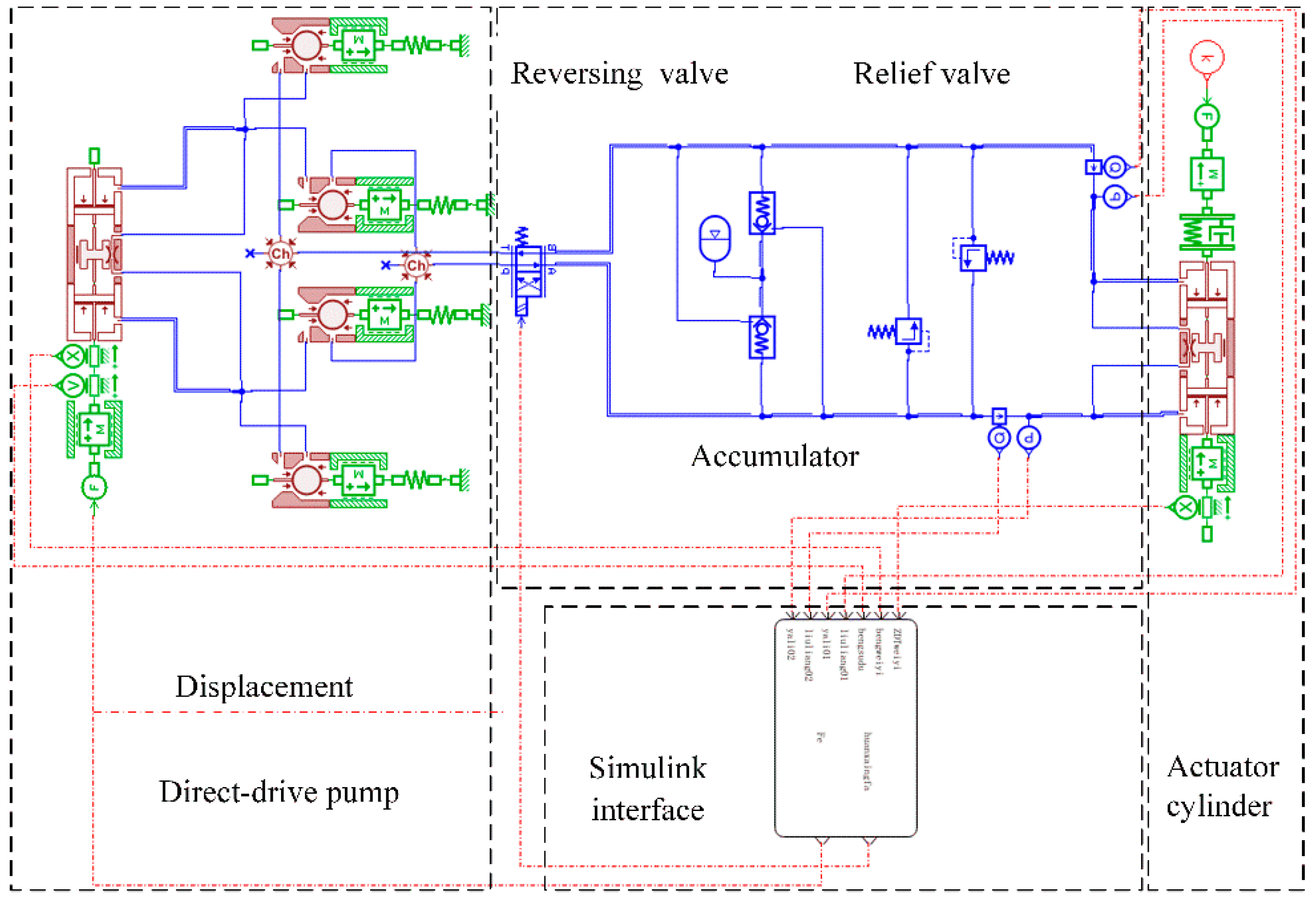
3.4. Establishment of Co-Simulation Model
4. Experimental Verification and Analysis
4.1. Experimental Setup
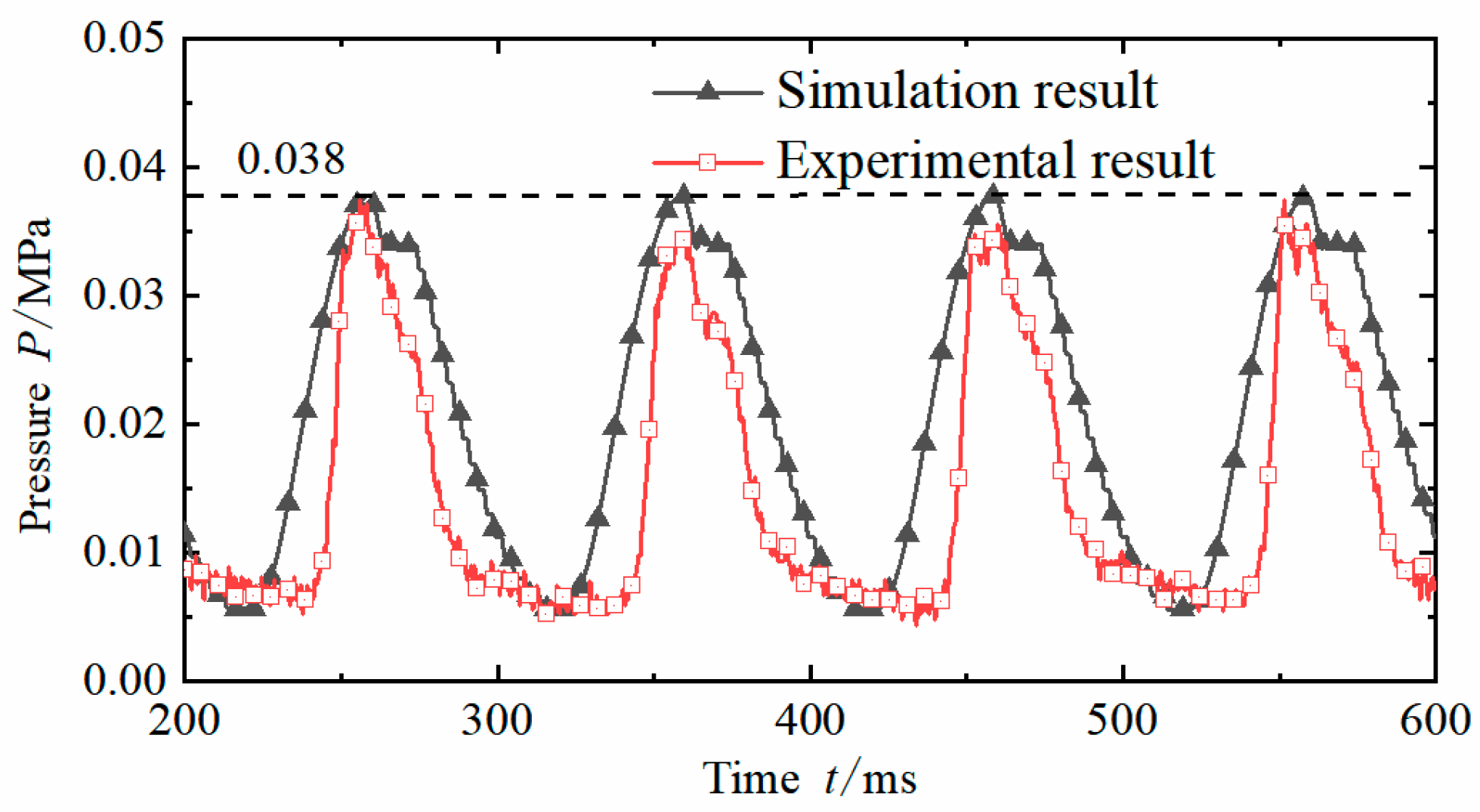
4.2. Pressure Test and Analysis of Actuating System
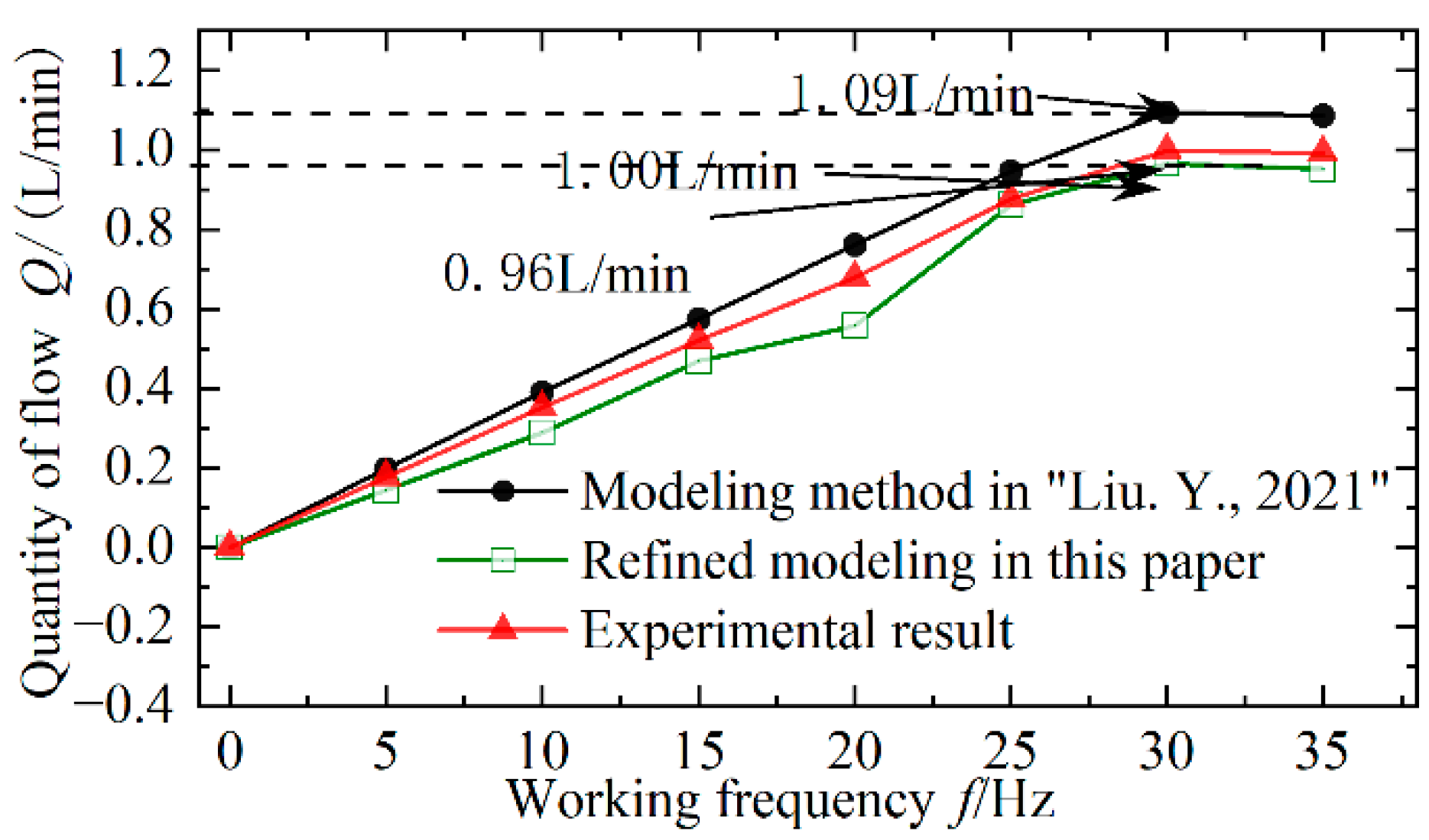
4.3. Flow Test and Analysis of Actuation System
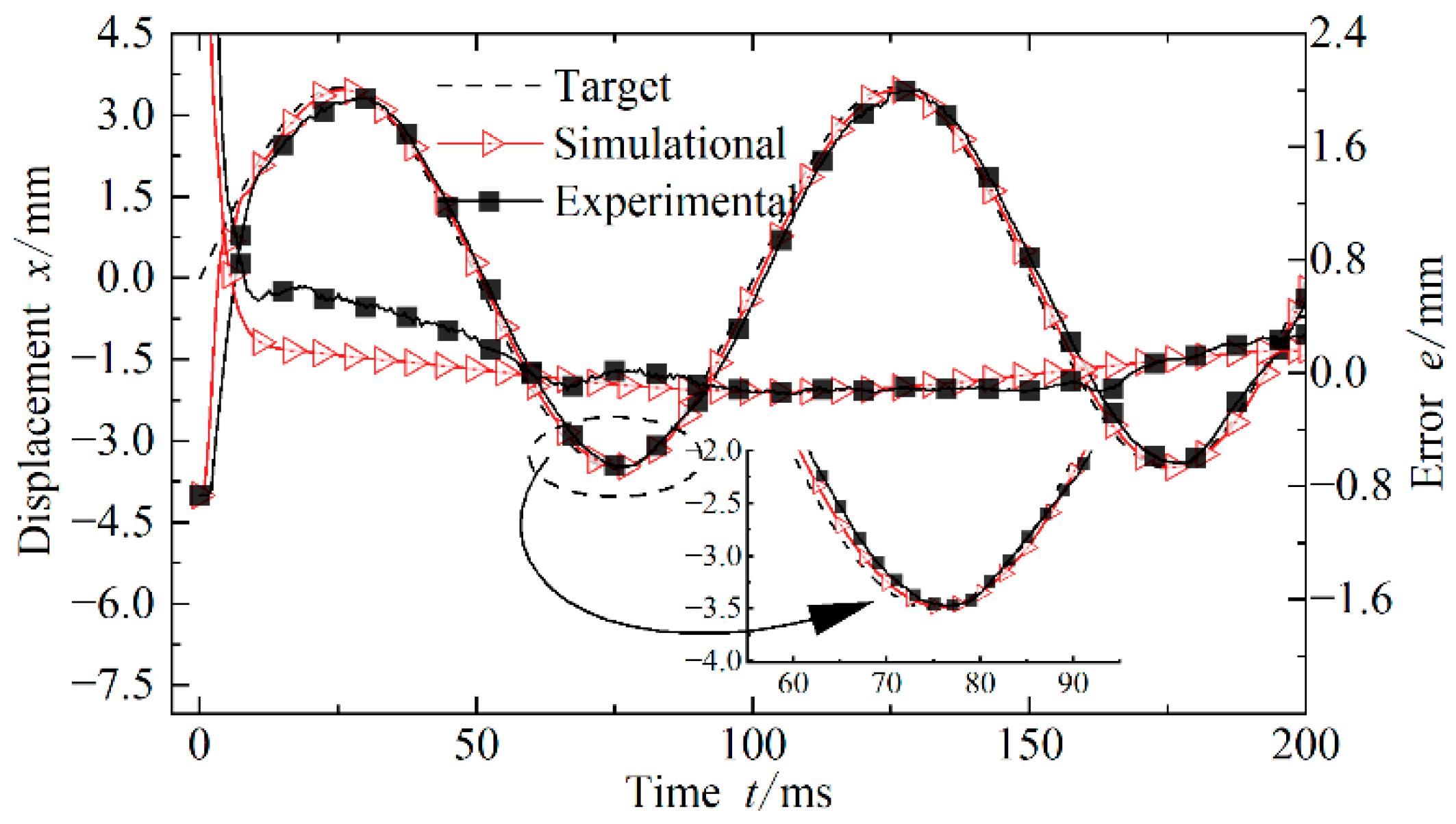
4.4. Displacement Test and Analysis of Actuator Cylinder
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Cheng, C.; Liu, S.; Wu, H. Sliding mode observer-based fractional-order proportional-integral-derivative sliding mode control for electro-hydraulic servo systems. Proc. Inst. Mech. Eng. C J. Mech. Eng. Sci. 2020, 234, 1887–1898. [Google Scholar] [CrossRef]
- Jiao, Z.; Kong, X.; Wang, S.; Xu, B.; He, Y.; Shang, Y. Advancements of basic researches on large aircraft of electro-hydraulic power and actuation system new architecture. China Basic Sci. 2018, 20, 41–47. [Google Scholar]
- Li, B.; Liu, Y.; Tan, C.; Qin, Q.; Lu, Y. Review on electro-hydrostatic actuator: System configurations, design methods and control technologies. Int. J. Mechatron. Manuf. Syst. 2020, 13, 323–346. [Google Scholar] [CrossRef]
- Ouyang, X.; Zhao, T.; Li, F.; Yang, S.; Zhu, Y.; Yang, H. Integral variable pi control on flow load simulator of aircraft hydraulic system. J. Zhejiang Univ. (Eng. Sci.) 2017, 51, 1111–1118. [Google Scholar]
- Yang, G.; Yao, J. High-precision motion servo control of double-rod electro-hydraulic actuators with exact tracking performance. ISA Trans. 2020, 103, 266–279. [Google Scholar] [CrossRef]
- Liu, J.; Jiang, J.; Ou, J.; Zhang, C.; Liu, Q. Theoretical model and dynamic performance of direct drive volume control electro-hydraulic servo system. J. Harbin Inst. Technol. 2011, 43, 61–65. [Google Scholar]
- Zhang, J.; Chao, Q.; Xu, B.; Pan, M.; Chen, Y.; Wang, Q.; Li, Y. Effect of piston-slipper assembly mass difference on the cylinder block tilt in a high-speed electro-hydrostatic actuator pump of aircraft. Int. J. Precis. Eng. Manuf. 2017, 18, 995–1003. [Google Scholar] [CrossRef]
- Wei, X. Research on Dual-Variable Control Algorithm of Electro-Hydrostatic Actuator; Beijing Jiaotong University: Beijing, China, 2020. [Google Scholar]
- Li, Y.; Jiao, Z. Study on electro hydrostatic actuation mechanism of linear drive. J. Mech. Eng. 2016, 52, 37–45. [Google Scholar] [CrossRef]
- Yang, X.; Zhu, Y.; Zhu, Y. Characteristic investigations on magnetic field and fluid field of a giant magnetostrictive material-based electro-hydrostatic actuator. Proc. Inst. Mech. Eng. G J. Aerosp. Eng. 2018, 232, 847–860. [Google Scholar] [CrossRef]
- Ren, G.; Costa, G.K.; Sepehri, N. Position control of an electro-hydrostatic asymmetric actuator operating in all quadrants. Mechatronics 2020, 67, 102344. [Google Scholar] [CrossRef]
- Shang, Y.; Li, X.; Qian, H.; Wu, S.; Pan, Q.; Huang, L.; Jiao, Z. A novel electro hydrostatic actuator system with energy recovery module for more electric aircraft. IEEE. Trans. Ind. Electron. 2019, 67, 2991–2999. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, X.; Zhang, H.; Quan, L. Characteristics of wheel loader lifting device based on closed pump-controlled three-chamber hydraulic cylinder. Trans. Chin. Soc. Agric. Mach. 2019, 50, 410–418. [Google Scholar]
- Yu, Z.; Li, Z.H.; Liu, L. Integrated design of polynomial nonlinear modeling and control of electro-hydraulic servo systems. Contl. Theor. Appl. 2021, 38, 364–372. [Google Scholar]
- Padovani, D.; Ketelsen, S.; Hagen, D.; Schmidt, L. A self-contained electro-hydraulic cylinder with passive load-holding capability. Energies 2019, 12, 292. [Google Scholar] [CrossRef]
- Phan, V.D.; Ahn, K.K. Optimized-Based Fault-Tolerant Control of an Electro-Hydraulic System with Disturbance Rejection. Appl. Sci. 2022, 12, 9197. [Google Scholar] [CrossRef]
- Woongyong, L.; Jun, K.M.; Kyun, C.W. Asymptotically stable disturbance observer-based compliance control of electrohydrostatic actuators. IEEE ASME Trans. Mechatron. 2019, 25, 195–206. [Google Scholar]
- Ge, L.; Quan, L.; Zhang, X.; Dong, Z.; Yang, J. Power matching and energy efficiency improvement of hydraulic excavator driven with speed and displacement variable power source. Chin. J. Mech. Eng. 2019, 32, 100. [Google Scholar] [CrossRef]
- Xu, X.; Li, Z.; Qin, H. Design and integrated simulation of the electro-hydraulic servo system based on AMESim and Matlab. Appl. Mech. Mater. 2011, 44, 1355–1359. [Google Scholar] [CrossRef]
- Chao, Q.; Zhang, J.; Xu, B.; Huang, H.; Pan, M. A review of high-speed electro-hydrostatic actuator pumps in aerospace applications: Challenges and solutions. J. Mech. Des. 2019, 141, 050801. [Google Scholar] [CrossRef]
- Cai, Y.; Ren, G.; Song, J.; Sepehri, N. High precision position control of electro-hydrostatic actuators in the presence of parametric uncertainties and uncertain nonlinearities. Mechatronics 2020, 68, 102363. [Google Scholar] [CrossRef]
- Thien, T.D.; Xuan, B.D.; Kwan, A.K. Adaptive backstepping sliding mode control for equilibrium position tracking of an electrohydraulic elastic manipulator. IEEE Trans. Ind. Electron. 2019, 67, 3860–3869. [Google Scholar]
- Tian, X.; Wang, S.; Wang, X.; Dong, D.; Zhang, Y. Design and Control of a Compliant Electro-Hydrostatic-Powered Ankle Prosthesis. IEEE ASME Trans. Mechatron. 2021; in press. [Google Scholar] [CrossRef]
- Tan, C.; Li, B.; Yu, P.; Lu, J.Y.; Liu, Y.T.; Sun, Z.Y. Multidisciplinary Modeling and Optimization of Direct-Driving Electro-Hydrostatic Actuator. Trans. Beijing Inst. Technol. 2021, 1–9. [Google Scholar] [CrossRef]
- Ge, Y.; Zhu, W.; Liu, J. Refined Modeling and Characteristic Analysis of Electro-hydrostatic Actuator. J. Mech. Eng. 2021, 57, 66–73. [Google Scholar]
- Khurram, B.; Gustavo, K.C.; Nariman, S. Optimization-Driven Controller Design for a High-Performance Electro-Hydrostatic Asymmetric Actuator Operating in All Quadrants. J. Dyn. Syst. Meas. Control 2021, 143, 094503. [Google Scholar]
- Li, B.; Li, D.; Ge, W.; Tan, C.; Lu, J.; Song, A. Precision control of hydraulic pressure in fast-response brake-by-wire system based on direct-drive valve. China J. Highw. Transp. 2021, 34, 121–132. [Google Scholar]
- Lu, J.; Chang, S.; Liu, L.; Fan, X. Point-to-point motions control of an electromagnetic direct-drive gas valve. J. Mech. Sci. Technol. 2018, 32, 363–371. [Google Scholar] [CrossRef]
- Peng, X.; Gong, G.; Liao, X.; Wu, W.; Wang, H.; Lou, H. Modeling and Model Identification of Micro-position-control Hydraulic System. J. Mech. Eng. 2017, 53, 206–211. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, J.; Ding, H.; Zhang, H. Output feedback control of electro-hydraulic asymmetric cylinder system with disturbances rejection. J. Franklin Inst. 2021, 358, 1839–1859. [Google Scholar] [CrossRef]
- Lu, Y.; Lu, J.; Tan, C.; Tian, M.; Dong, G. Adaptive Non-Singular Terminal Sliding Mode Control Method for Electromagnetic Linear Actuator. Micromachines 2022, 13, 1294. [Google Scholar] [CrossRef]
- Lin, C.J.; Yau, H.T.; Tian, Y.C. Identification and compensation of nonlinear friction characteristics and precision control for a linear motor stage. IEEE ASME Trans. Mechatron. 2012, 18, 1385–1396. [Google Scholar] [CrossRef]




| Parameter | Value |
|---|---|
| Diameter of direct-drive pump piston/mm | 12 |
| Working stroke of direct-drive pump/mm | 10 |
| Dead zone volume of direct-drive pump/mm3 | 10 |
| Mover mass of direct-drive pump/Kg | 0.12 |
| Dead zone volume of actuator cylinder /mm3 | 10 |
| Diameter of actuator cylinder output rod/mm | 20 |
| Diameter of actuator cylinder piston/mm | 30 |
| Working stroke of actuator cylinder/mm | 20 |
| Coil resistance of ELA/Ω | 1.41 |
| Coil inductance of ELA/mH | 1.1 |
| Electromagnetic force coefficient of ELA/N/A | 24.61 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, J.; Gu, C.; Zhao, Y.; Tan, C.; Lu, Y.; Fu, C. Refined Modeling Method and Analysis of an Electromagnetic Direct-Drive Hydrostatic Actuation System. Actuators 2022, 11, 281. https://doi.org/10.3390/act11100281
Lu J, Gu C, Zhao Y, Tan C, Lu Y, Fu C. Refined Modeling Method and Analysis of an Electromagnetic Direct-Drive Hydrostatic Actuation System. Actuators. 2022; 11(10):281. https://doi.org/10.3390/act11100281
Chicago/Turabian StyleLu, Jiayu, Chaofan Gu, Yanjun Zhao, Cao Tan, Yingtao Lu, and Changzhong Fu. 2022. "Refined Modeling Method and Analysis of an Electromagnetic Direct-Drive Hydrostatic Actuation System" Actuators 11, no. 10: 281. https://doi.org/10.3390/act11100281
APA StyleLu, J., Gu, C., Zhao, Y., Tan, C., Lu, Y., & Fu, C. (2022). Refined Modeling Method and Analysis of an Electromagnetic Direct-Drive Hydrostatic Actuation System. Actuators, 11(10), 281. https://doi.org/10.3390/act11100281






